Three-Dimensional Imaging of Vortex Electromagnetic Wave Radar with Integer and Fractional Order OAM Modes
Abstract
1. Introduction
2. Echo Model Based on UCA
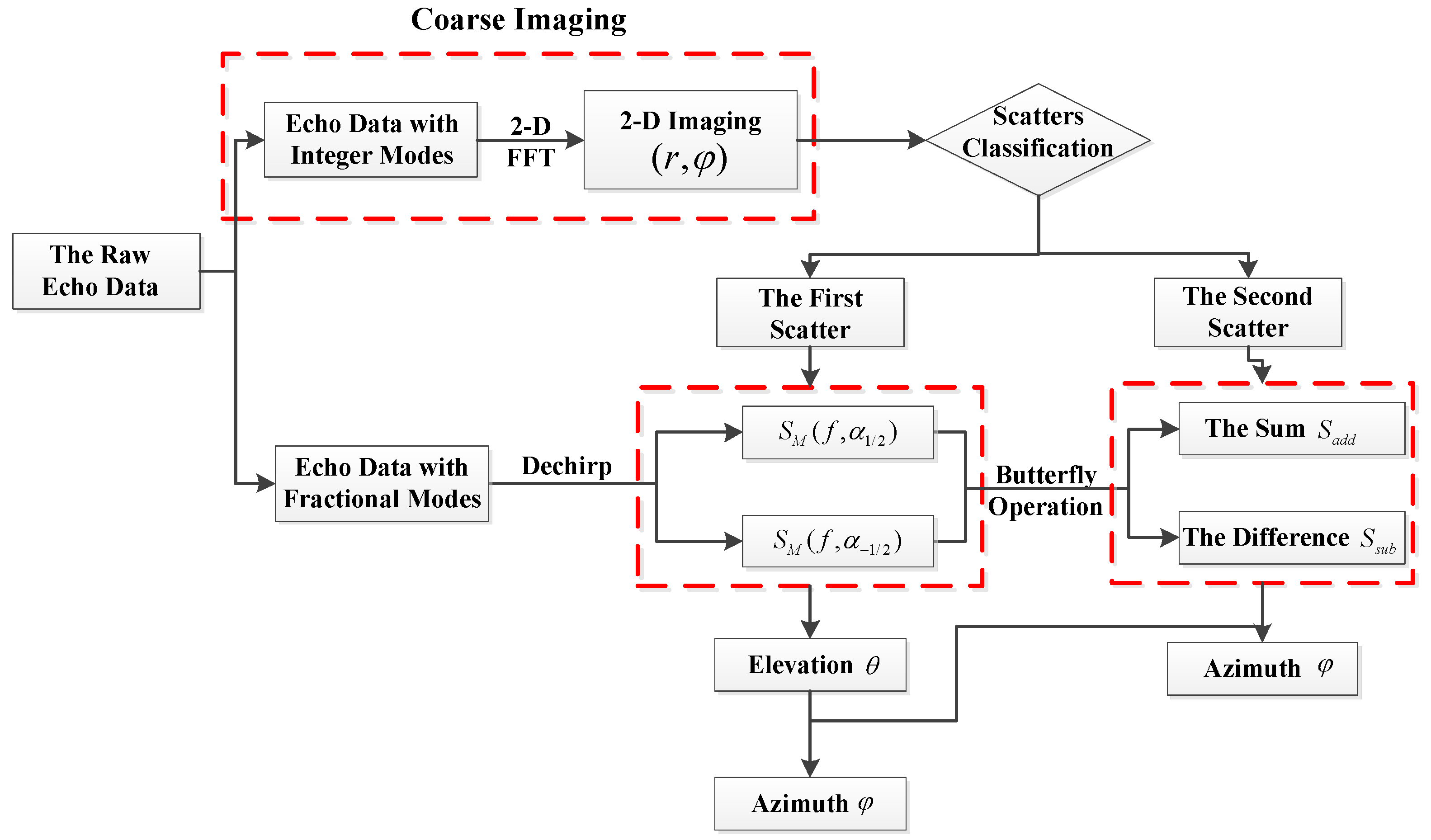
3. Three-Dimensional Imaging Algorithm
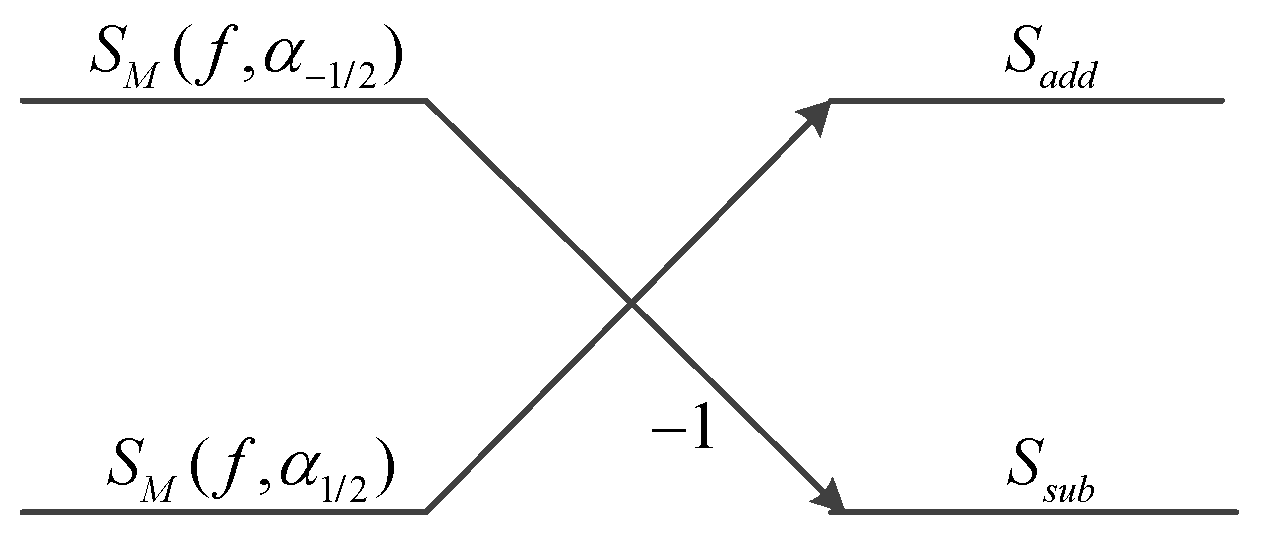
3.1. Coarse Imaging
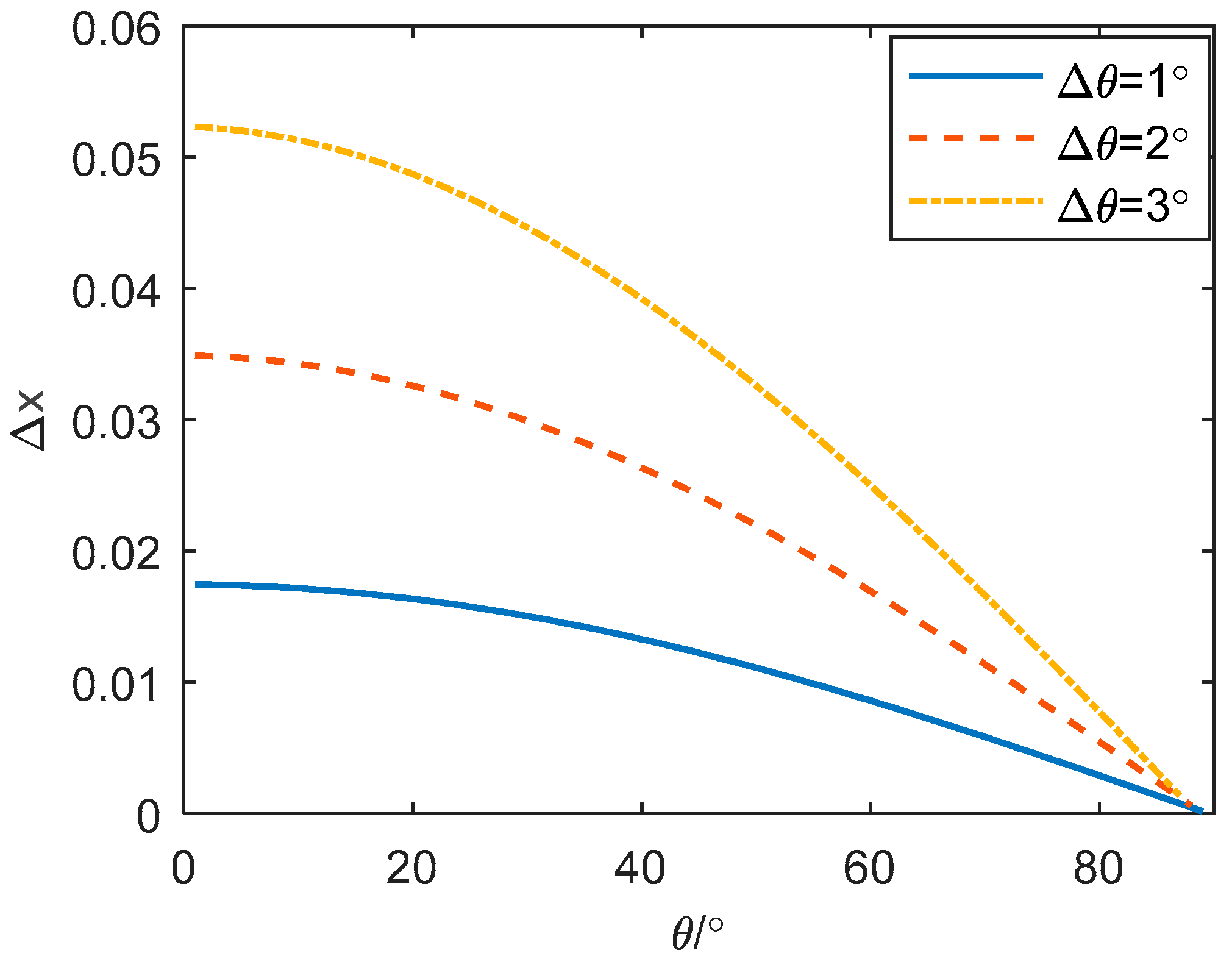
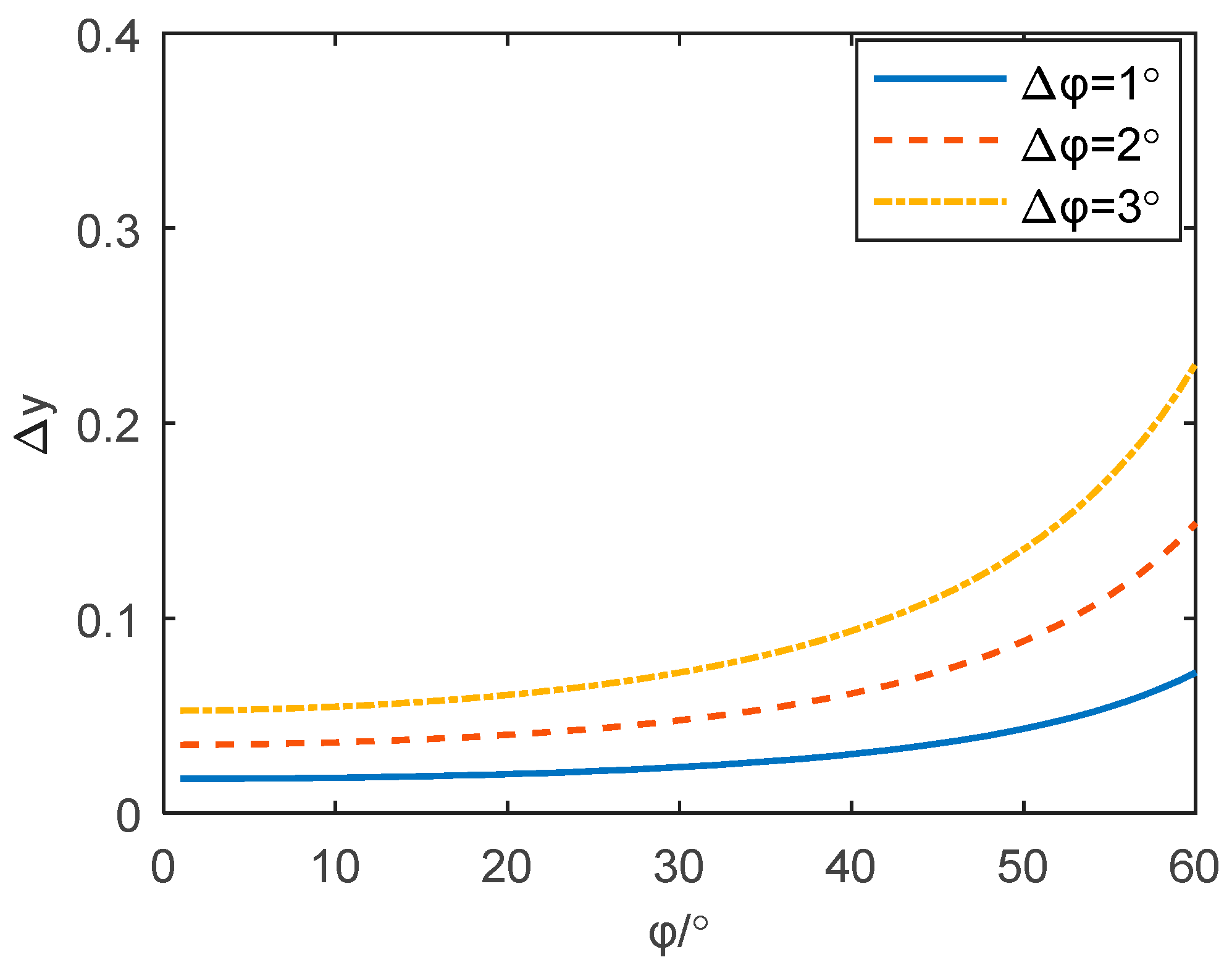
3.2. Three-Dimensional Imaging
4. Simulation
4.1. Processing of Coarse Imaging
4.2. Processing of Signal with Fractional OAM Mode
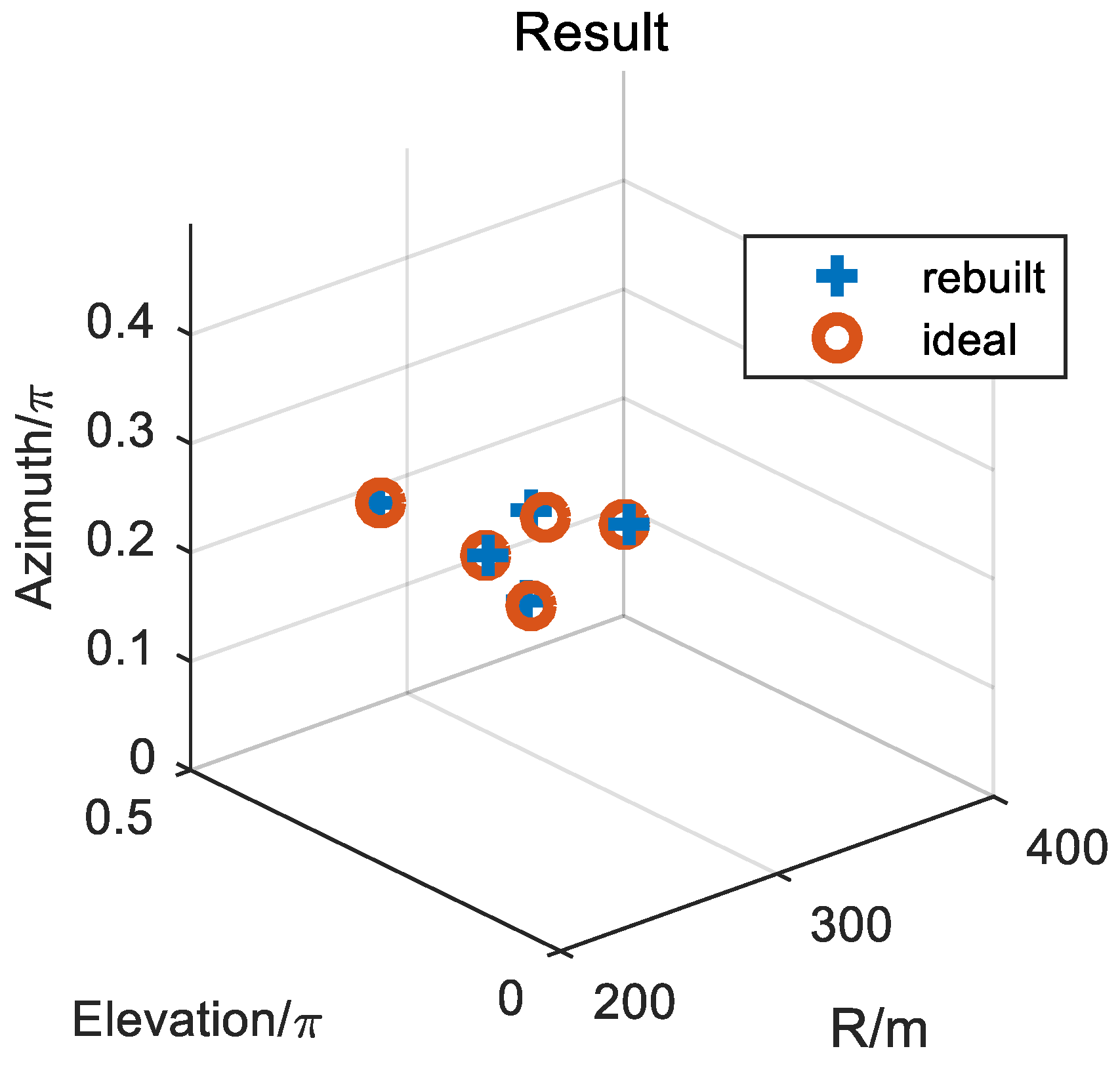
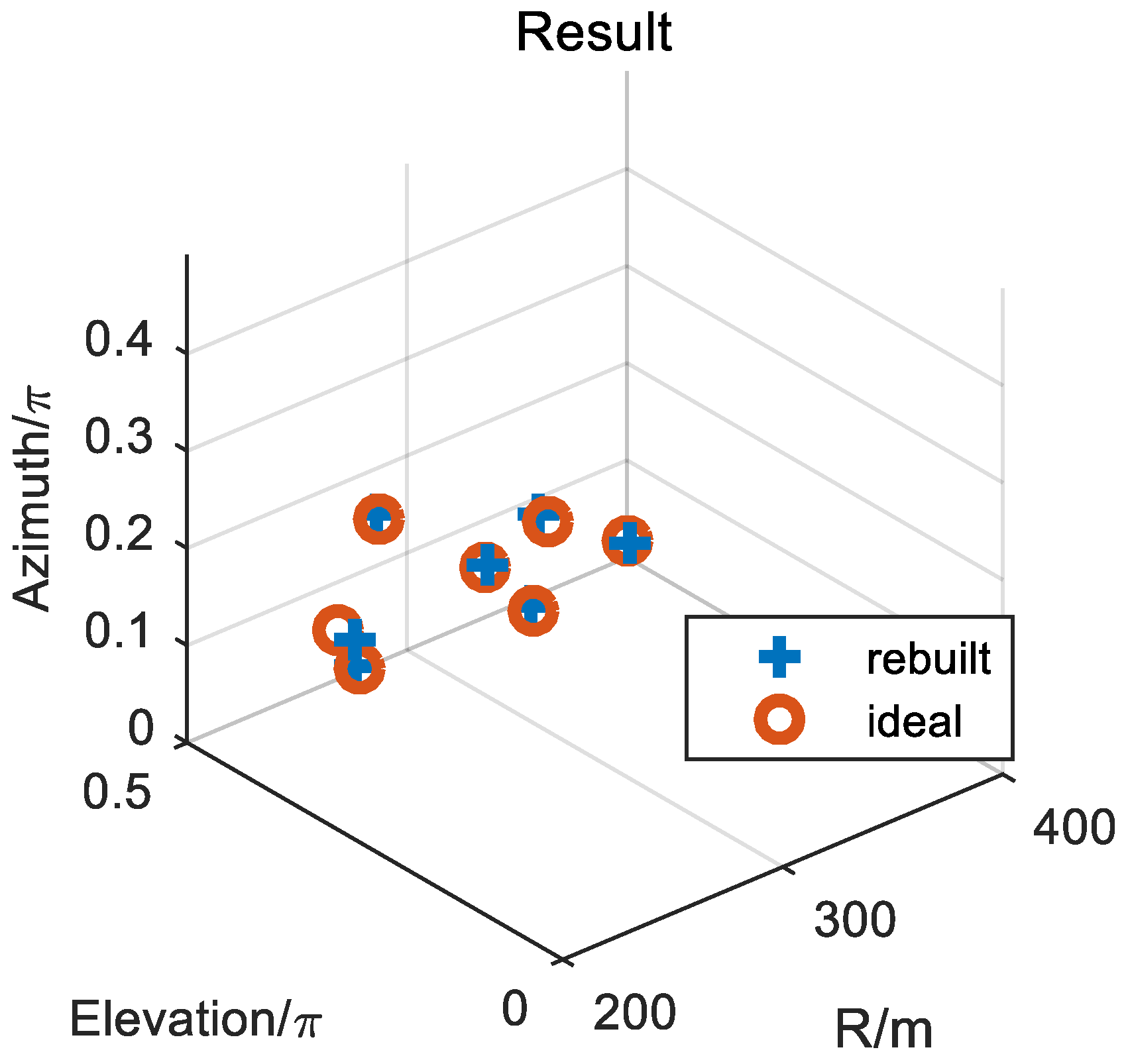
4.3. Reconstruction of the First Kind of Scatterers
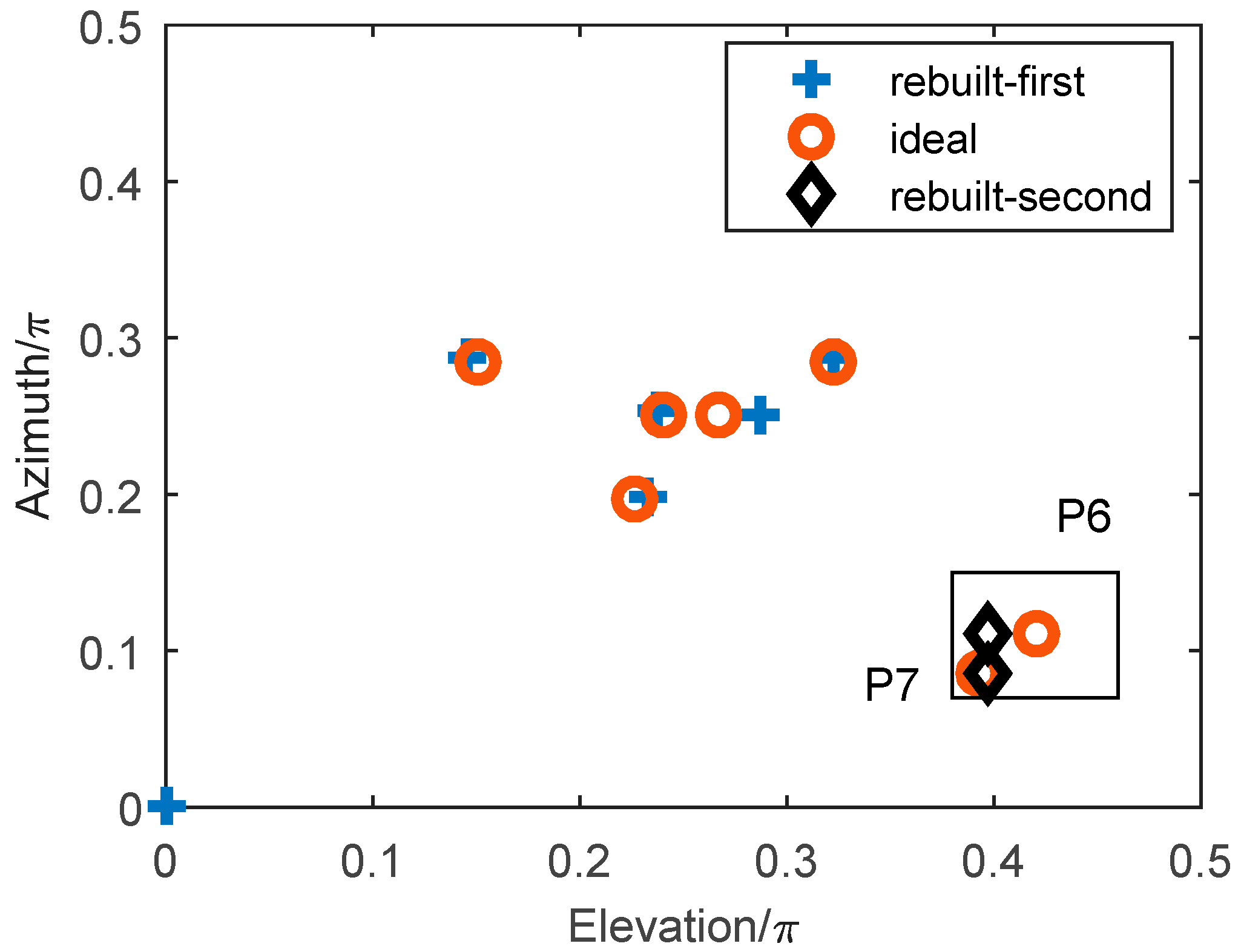
4.4. Reconstruction of the Second Kind of Scatterers
4.5. Three-Dimensional Imaging of the Target
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Allen, L.; Beijersbergen, M.W.; Spreeuw RJ, C.; Woerdman, J.P. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A 1992, 45, 8185–8189. [Google Scholar] [CrossRef] [PubMed]
- Jackson, J.D. Classical Electrodynamics, 3rd ed.; Wiley-VCH: Weinheim, Germany, 1999; p. 832. ISBN 0-471-30932-X. [Google Scholar] [CrossRef]
- Liu, K.; Li, X.S.; Wang, H.Q.; Cheng, Y.Q. The Advances of Vortex Electromagnetic Wave in Radar Applications. Acta Electron. Sin. 2018, 46, 2283–2290. [Google Scholar] [CrossRef]
- Thidé, B.; Then, H.; Sjöholm, J.; Palmer, K.; Bergman, J.; Carozzi, T.D.; Istomin, Y.N.; Ibragimov, N.H.; Khamitova, R. Utilization of Photon Orbital Angular Momentum in the Low-Frequency Radio Domain. Phys. Rev. Lett. 2007, 99, 087701. [Google Scholar] [CrossRef]
- Tamburini, F.; Mari, E.; Sponselli, A.; Thidé, B.; Bianchini, A.; Romanato, F. Encoding many channels on the same frequency through radio vorticity: First experimental test. New J. Phys. 2012, 14, 033001. [Google Scholar] [CrossRef]
- Edfors, O.; Johansson, A.J. Is Orbital Angular Momentum (OAM) Based Radio Communication an Unexploited Area? IEEE Trans. Antennas Propag. 2012, 60, 1126–1131. [Google Scholar] [CrossRef]
- Tamagnone, M.; Craeye, C.; Perruisseau-Carrier, J. Comment on ‘Encoding many channels on the same frequency through radio vorticity: First experimental test’. arXiv 2012, arXiv:1210.5365. [Google Scholar] [CrossRef]
- Sun, X.H.; Li, Q.; Pang, D.X.; Zeng, Z.M. New Research Progress of the Orbital Angular Momentum Technology in Wireless Communication: A Survey. Acta Electron. Sin. 2015, 43, 2305–2314. [Google Scholar]
- Guo, G.; Hu, W.; Du, X. Electromagnetic vortex based radar target imaging. J. Natl. Univ. Def. Technol. 2013, 35, 71–76. [Google Scholar]
- Liu, K.; Cheng, Y.; Li, X.; Wang, H.; Qin, Y.; Jiang, Y. Study on the theory and method of vortex-electromagnetic-wave-based radar imaging. IET Microw. Antennas Propag. 2016, 10, 961–968. [Google Scholar] [CrossRef]
- Liu, K.; Cheng, Y.; Yang, Z.; Wang, H.; Qin, Y.; Li, X. Orbital-Angular-Momentum-Based Electromagnetic Vortex Imaging. IEEE Antennas Wirel. Propag. Lett. 2015, 14, 711–714. [Google Scholar] [CrossRef]
- Liu, K.; Liu, H.; Qin, Y.; Cheng, Y.; Wang, S.; Li, X.; Wang, H. Generation of OAM Beams Using Phased Array in the Microwave Band. IEEE Trans. Antennas Propag. 2016, 64, 3850–3857. [Google Scholar] [CrossRef]
- Yuan, H.; Chen, Y.J.; Luo, Y.; Liang, J.; Wang, Z.H. A resolution-improved imaging algorithm based on uniform circular array. IEEE Antennas Wirel. Propag. Lett. 2022, 21, 461–465. [Google Scholar] [CrossRef]
- Liu, K.; Cheng, Y.; Li, X.; Jiang, Y. Passive OAM-Based Radar Imaging with Single-In-Multiple-Out Mode. IEEE Microw. Wirel. Compon. Lett. 2018, 28, 840–842. [Google Scholar] [CrossRef]
- Liu, K.; Li, X.; Cheng, Y.; Gao, Y.; Fan, B.; Jiang, Y. OAM-Based Multitarget Detection: From Theory to Experiment. IEEE Microw. Wirel. Compon. Lett. 2017, 27, 760–762. [Google Scholar] [CrossRef]
- Yuan, T.; Wang, H.; Cheng, Y.; Qin, Y. Electromagnetic Vortex-Based Radar Imaging Using a Single Receiving Antenna: Theory and Experimental Results. Sensors 2017, 17, 630. [Google Scholar] [CrossRef]
- Liu, K.; Li, X.; Gao, Y.; Cheng, Y.; Wang, H.; Qin, Y. High-Resolution Electromagnetic Vortex Imaging Based on Sparse Bayesian Learning. IEEE Sens. J. 2017, 17, 6918–6927. [Google Scholar] [CrossRef]
- Liu, H.; Liu, K.; Cheng, Y.; Wang, H. Microwave Vortex Imaging Based on Dual Coupled OAM Beams. IEEE Sens. J. 2020, 20, 806–815. [Google Scholar] [CrossRef]
- Liu, H.; Liu, K.; Cheng, Y.; Wang, H.; Wang, Y. Scattering Characteristics of Vortex Electromagnetic Waves by a Metal Plate. In Proceedings of the 2020 9th Asia-Pacific Conference on Antennas and Propagation (APCAP), Xiamen, China, 4–7 August 2020; pp. 1–2. [Google Scholar] [CrossRef]
- Liu, K.; Liu, H.; Sha, W.E.I.; Cheng, Y.; Wang, H. Backward Scattering of Electrically Large Standard Objects Illuminated by OAM Beams. IEEE Antennas Wirel. Propag. Lett. 2020, 19, 1167–1171. [Google Scholar] [CrossRef]
- Yang, L.J.; Sun, S.; Sha, W.E.I. Ultrawideband Reflection-Type Metasurface for Generating Integer and Fractional Orbital Angular Momentum. IEEE Trans. Antennas Propag. 2020, 68, 2166–2175. [Google Scholar] [CrossRef]
- Chen, R.; Zou, M.; Feng, Q.; Li, J. Generation of OAM Radio Waves Using a Single Antenna in Uniform Circular Motion. In Proceedings of the 2019 8th Asia-Pacific Conference on Antennas and Propagation (APCAP), Incheon, Korea, 4–7 August 2019; pp. 593–594. [Google Scholar] [CrossRef]
- Chen, R.; Zou, M.; Wang, X.; Tennant, A. Generation and Beam Steering of Arbitrary-Order OAM with Time-Modulated Circular Arrays. IEEE Syst. J. 2021, 15, 5313–5320. [Google Scholar] [CrossRef]
- Yang, Y.J.; Qiu, C.W. Generation of Optical Vortex Beams, Electromagnetic Vortices: Wave Phenomena and Engineering Applications; IEEE: Piscataway, NJ, USA, 2022; pp. 223–244. [Google Scholar] [CrossRef]
- Jiang, X.; Wang, Y.; Zhang, C. Performance Evaluation Based on Joint Frequency and Orbital Angular Momentum Spectrum. In Proceedings of the 2020 IEEE Globecom Workshops (GC Wkshps), Taipei, Taiwan, 7–11 December 2020; pp. 1–6. [Google Scholar] [CrossRef]
- Liu, H.; Wang, Y.; Wang, J.; Liu, K.; Wang, H. Electromagnetic Vortex Enhanced Imaging Using Fractional OAM Beams. IEEE Antennas Wirel. Propag. Lett. 2021, 20, 948–952. [Google Scholar] [CrossRef]
- Guo, S.; He, Z.; Fan, Z.; Chen, R. CUCA Based Equivalent Fractional Order OAM Mode for Electromagnetic Vortex Imaging. IEEE Access 2020, 8, 91070–91075. [Google Scholar] [CrossRef]
- Chen, Y.; Wang, S.; Luo, Y.; Liu, H. Measurement Matrix Optimization Based on Target Prior Information for Radar Imaging. IEEE Sens. J. 2023, 23, 9808–9819. [Google Scholar] [CrossRef]
- Zhao, S.; Luo, Y.; Zhang, T.; Guo, W.; Zhang, Z. A domain specific knowledge extraction transformer method for multisource satellite-borne SAR images ship detection. ISPRS J. Photogramm. Remote Sens. 2023, 198, 16–29. [Google Scholar] [CrossRef]
- Zhou, X.; Bai, X.; Wang, L.; Zhou, F. Robust ISAR Target Recognition Based on ADRISAR-Net. IEEE Trans. Aerosp. Electron. Syst. 2022, 58, 5494–5505. [Google Scholar] [CrossRef]
- Liu, H.; Du, L.; Wang, P.; Pan, M.; Bao, Z. Radar HRRP Automatic Target Recognition: Algorithms and Applications. In Proceedings of the 2011 IEEE CIE International Conference on Radar, Chengdu, China, 24–27 October 2011; pp. 14–17. [Google Scholar] [CrossRef]
- Wang, J.; Liu, K.; Cheng, Y.; Wang, H. Vortex SAR Imaging Method Based on OAM Beams Design. IEEE Sens. J. 2019, 19, 11873–11879. [Google Scholar] [CrossRef]
- Zeng, Y.; Wang, Y.; Zhou, C.; Cui, J.; Yi, J.; Zhang, J. Super-resolution Electromagnetic Vortex SAR Imaging Based on Compressed Sensing. In Proceedings of the 2020 IEEE/CIC International Conference on Communications in China (ICCC), Chongqing, China, 9–11 August 2020; pp. 629–633. [Google Scholar]
- Bu, X.; Zhang, Z.; Chen, L.; Liang, X.; Tang, H.; Wang, X. Implementation of Vortex Electromagnetic Waves High-Resolution Synthetic Aperture Radar Imaging. IEEE Antennas Wirel. Propag. Lett. 2018, 17, 764–767. [Google Scholar] [CrossRef]
- Fang, Y.; Wang, P.; Chen, J. A Novel Imaging Formation Method for Electromagnetic Vortex SAR Based on Orbital-Angular-Momentum. In Proceedings of the 2018 China International SAR Symposium (CISS), Shanghai, China, 10–12 October 2018; pp. 1–3. [Google Scholar] [CrossRef]
- Bu, X.X.; Zhang, Z.; Chen, L.Y.; Zhu, K.H.; Zhou, S.; Luo, J.P.; Cheng, R.; Liang, X.D. Synthetic Aperture Radar Interferometry Based on Vortex Electromagnetic Waves. IEEE Access 2019, 7, 82693–82700. [Google Scholar] [CrossRef]
- Wang, L.; Tao, L.; Li, Z.; Wu, J.; Yang, J. Three Dimensional Electromagnetic Vortex Radar Imaging Based on the Modified RD Algorithm. In Proceedings of the 2020 IEEE Radar Conference (RadarConf20), Florence, Italy, 21–25 September 2020. [Google Scholar]
- Wang, J.; Liu, K.; Liu, H.; Cao, K.; Cheng, Y.; Wang, H. 3-D Object Imaging Method with Electromagnetic Vortex. IEEE Trans. Geosci. Remote Sens. 2022, 60, 1–12. [Google Scholar] [CrossRef]
- Liu, K.; Cheng, Y.; Wang, H.; Luo, C. OAM-based Imaging with Cylinder-shaped Arrays. In Proceedings of the 2020 International Symposium on Antennas and Propagation (ISAP), Osaka, Japan, 25–28 January 2021; pp. 359–360. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations, North–Holland Mathematics Studies; Elsevier Press: Amsterdam, The Netherlands, 2006; Volume 204. [Google Scholar]



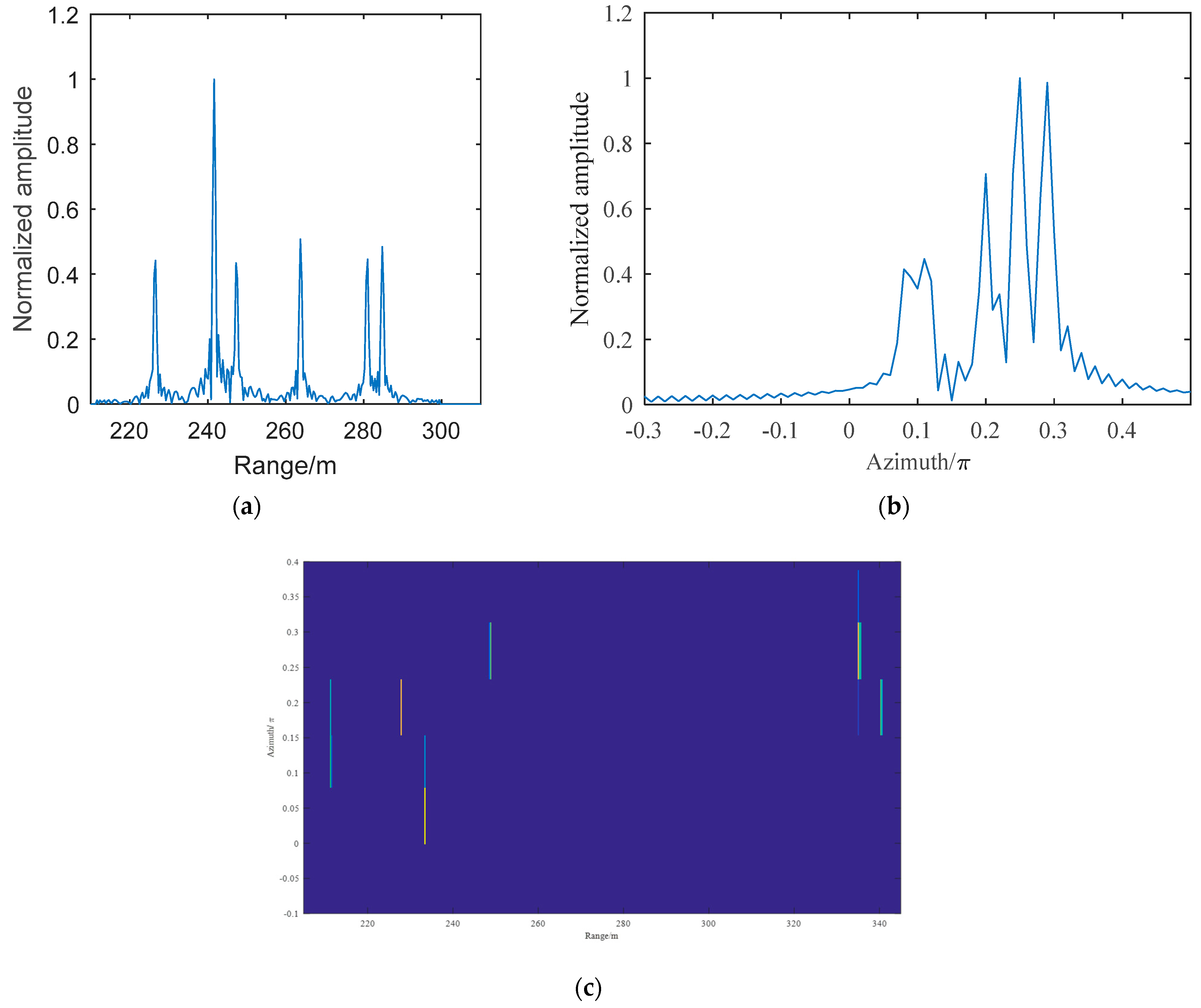
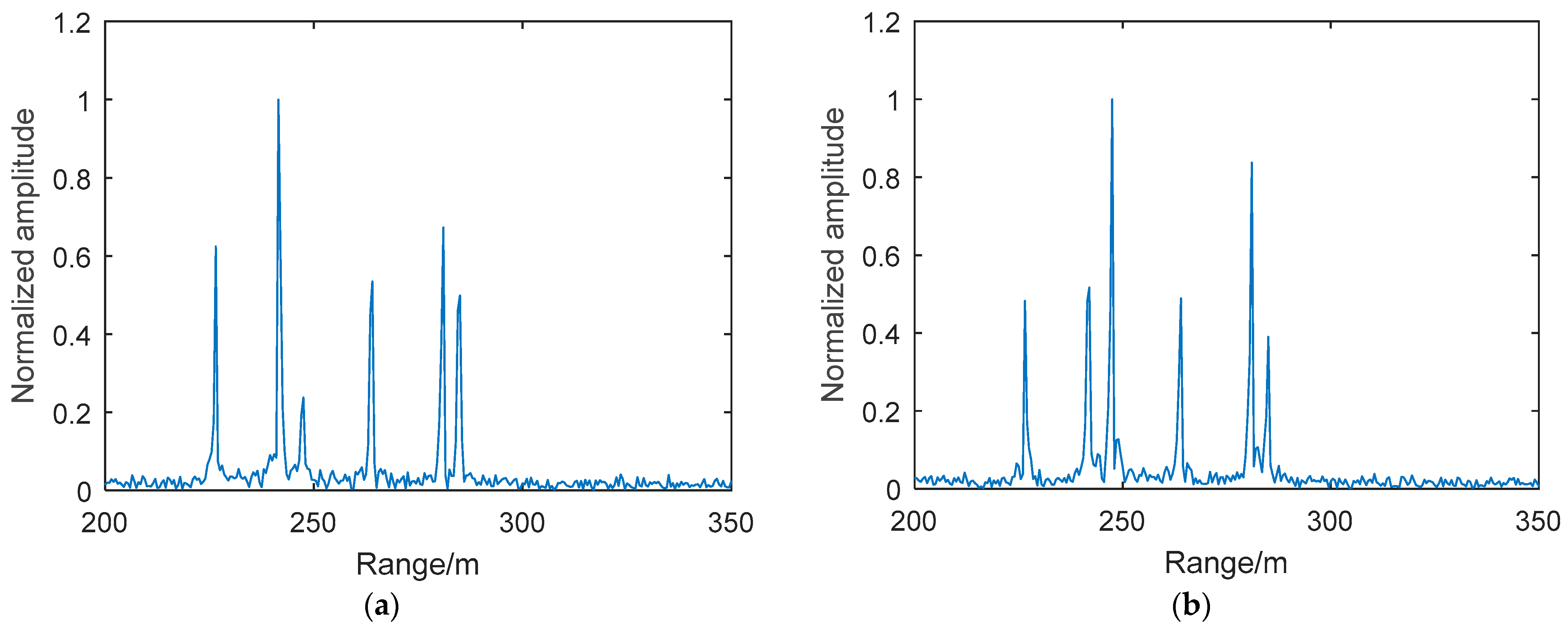
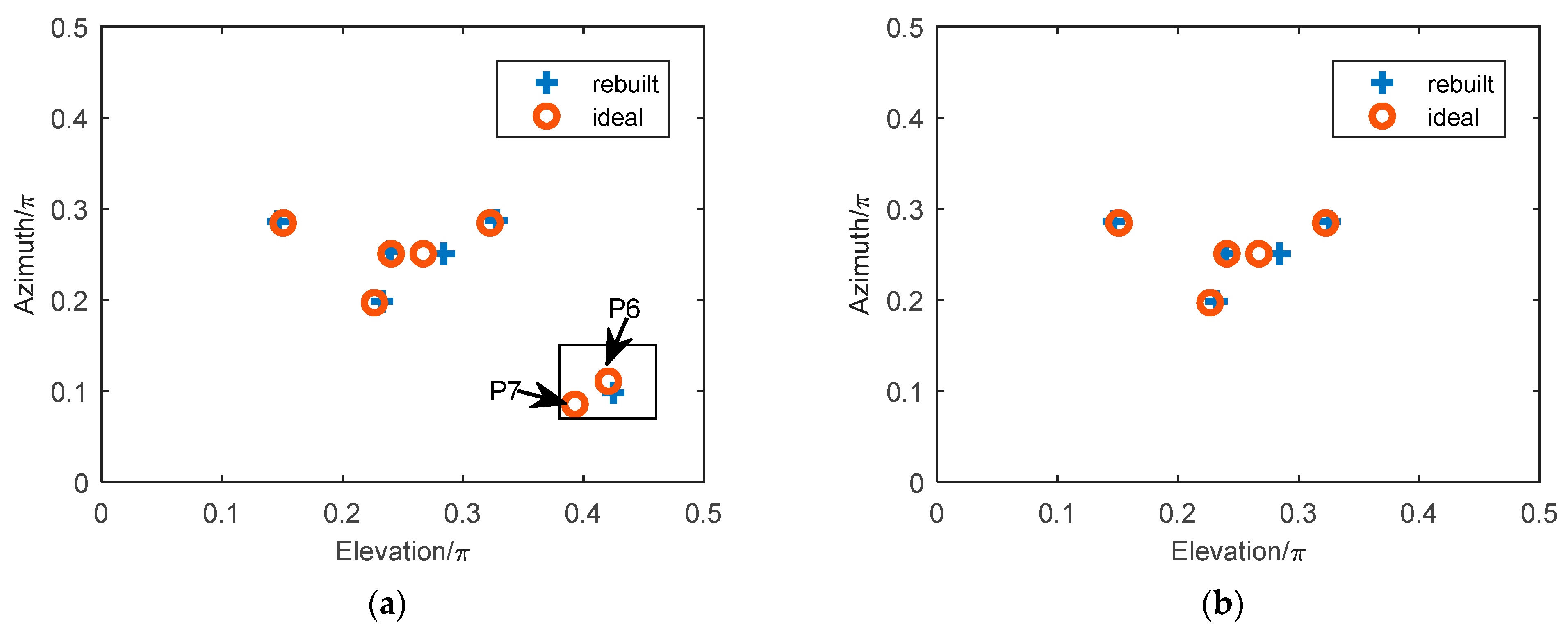
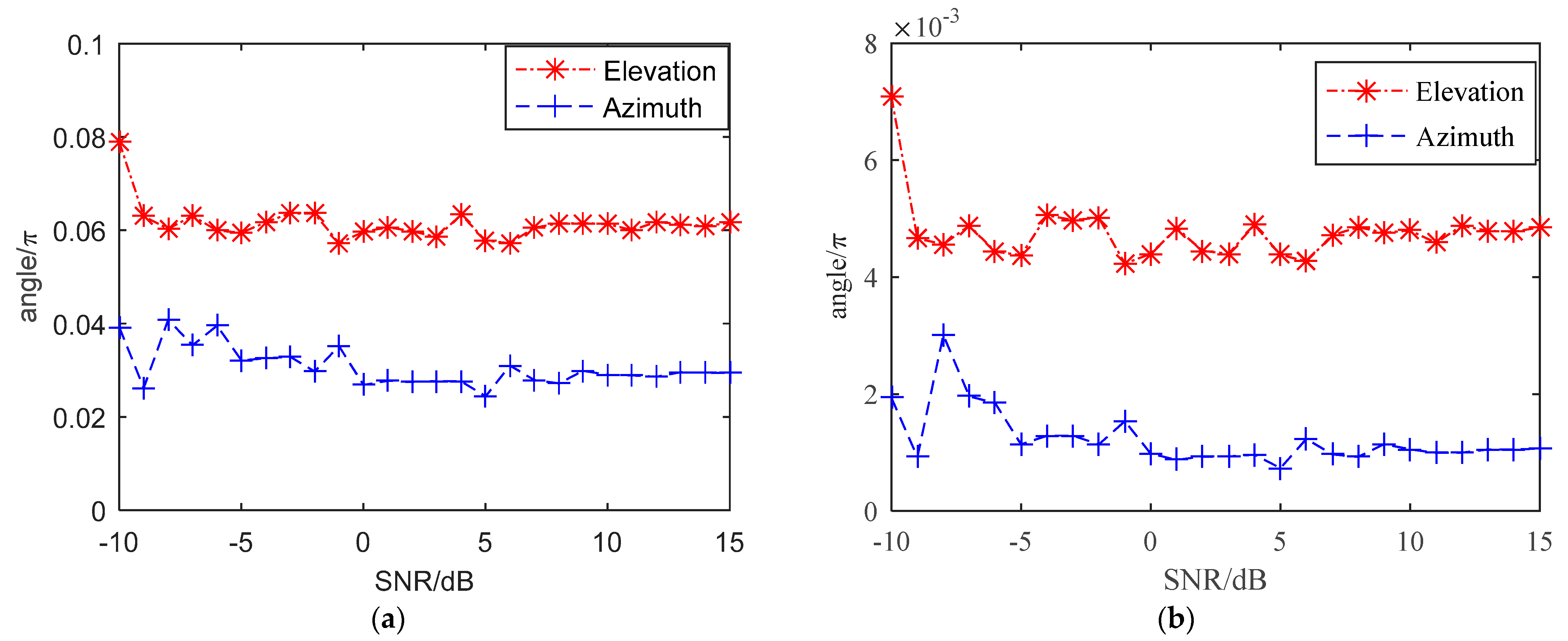

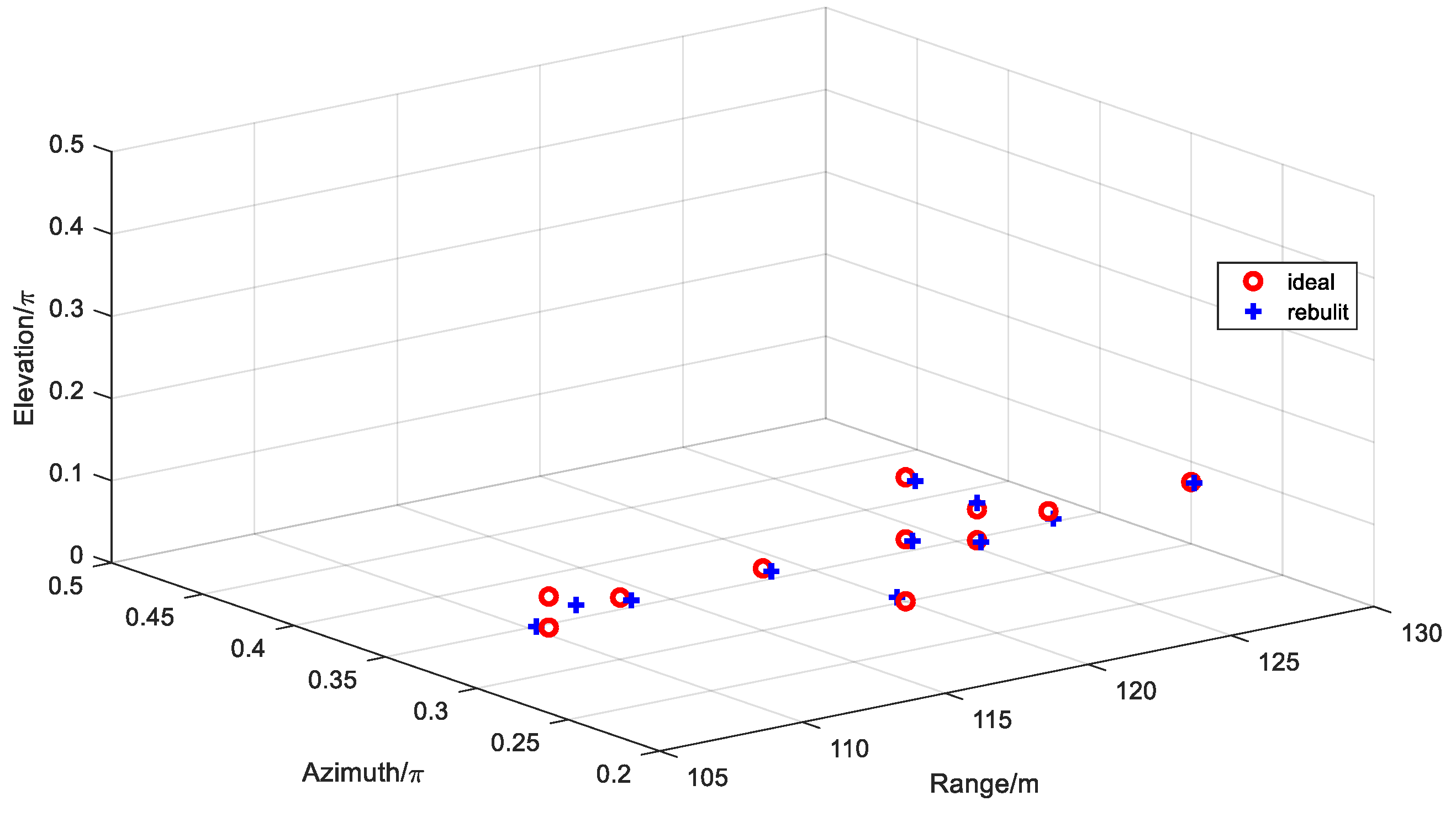
| The Index of Scatterer | X (m) | Y (m) | Z (m) | The Index of Scatterer | X (m) | Y (m) | Z (m) |
|---|---|---|---|---|---|---|---|
| 80 | 100 | 250 | 120 | 150 | 120 | ||
| 140 | 100 | 200 | 220 | 80 | 60 | ||
| 150 | 150 | 190 | 220 | 60 | 80 | ||
| 120 | 120 | 180 |
| The Index of Scatterer | R(m) | (/) | (/) | The Index of Scatterer | R(m) | (/) | (/) |
| 289.89 | 0.29 | 0.17 | 226.49 | 0.29 | 0.32 | ||
| 263.81 | 0.19 | 0.23 | 241.66 | 0.11 | 0.42 | ||
| 284.78 | 0.25 | 0.27 | 241.66 | 0.08 | 0.39 | ||
| 247.38 | 0.25 | 0.24 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, J.; Chen, Y.; Zhang, Q.; Luo, Y.; Li, X. Three-Dimensional Imaging of Vortex Electromagnetic Wave Radar with Integer and Fractional Order OAM Modes. Remote Sens. 2023, 15, 2903. https://doi.org/10.3390/rs15112903
Liang J, Chen Y, Zhang Q, Luo Y, Li X. Three-Dimensional Imaging of Vortex Electromagnetic Wave Radar with Integer and Fractional Order OAM Modes. Remote Sensing. 2023; 15(11):2903. https://doi.org/10.3390/rs15112903
Chicago/Turabian StyleLiang, Jia, Yijun Chen, Qun Zhang, Ying Luo, and Xiaohui Li. 2023. "Three-Dimensional Imaging of Vortex Electromagnetic Wave Radar with Integer and Fractional Order OAM Modes" Remote Sensing 15, no. 11: 2903. https://doi.org/10.3390/rs15112903
APA StyleLiang, J., Chen, Y., Zhang, Q., Luo, Y., & Li, X. (2023). Three-Dimensional Imaging of Vortex Electromagnetic Wave Radar with Integer and Fractional Order OAM Modes. Remote Sensing, 15(11), 2903. https://doi.org/10.3390/rs15112903






