Maritime DGPS System Positioning Accuracy as a Function of the HDOP in the Context of Hydrographic Survey Performance
Abstract
1. Introduction
2. Materials and Methods
- M—measuring position error,
- σ—measurement error of the navigation parameter (pseudorange), and
- DOP—geometric coefficient calculated based on the satellite’s position in relation to the receiver.
- —elements of the matrix G−1, provided G exists.
- A DGPS receiver, similarly to GPS, determines pseudoranges, but these are additionally corrected using a pseudorange correction transmitted by the reference station via radio, which is not the case with the GPS system;
- For the determination of coordinates, only the pseudoranges to which the DGPS reference station has transmitted corrections are used. In contrast to the GPS system, a DGPS receiver only uses these GPS satellites to which the DGPS reference station will transmit a pseudorange correction. The other pseudoranges are not used in positioning.
- ρD—differentially corrected pseudorange,
- ρ—pseudorange measured by the GPS system, and
- PRC(t)—extrapolated pseudorange correction at the moment of observation t.
- PRC(t0)—pseudorange correction determined by the DGPS reference station at the moment t0, and
- RRC(t − t0)—range rate correction over time (t − t0).
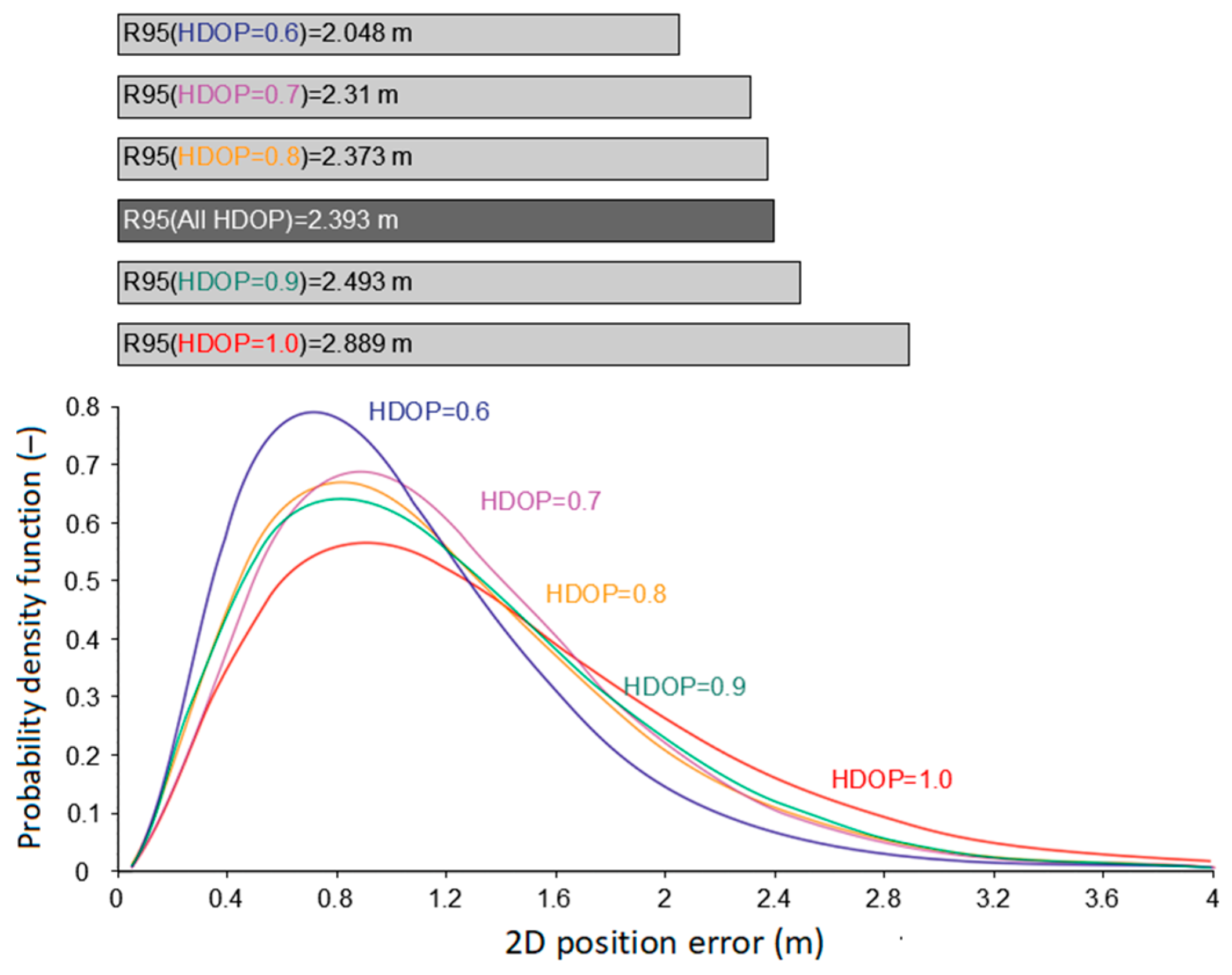
3. Results
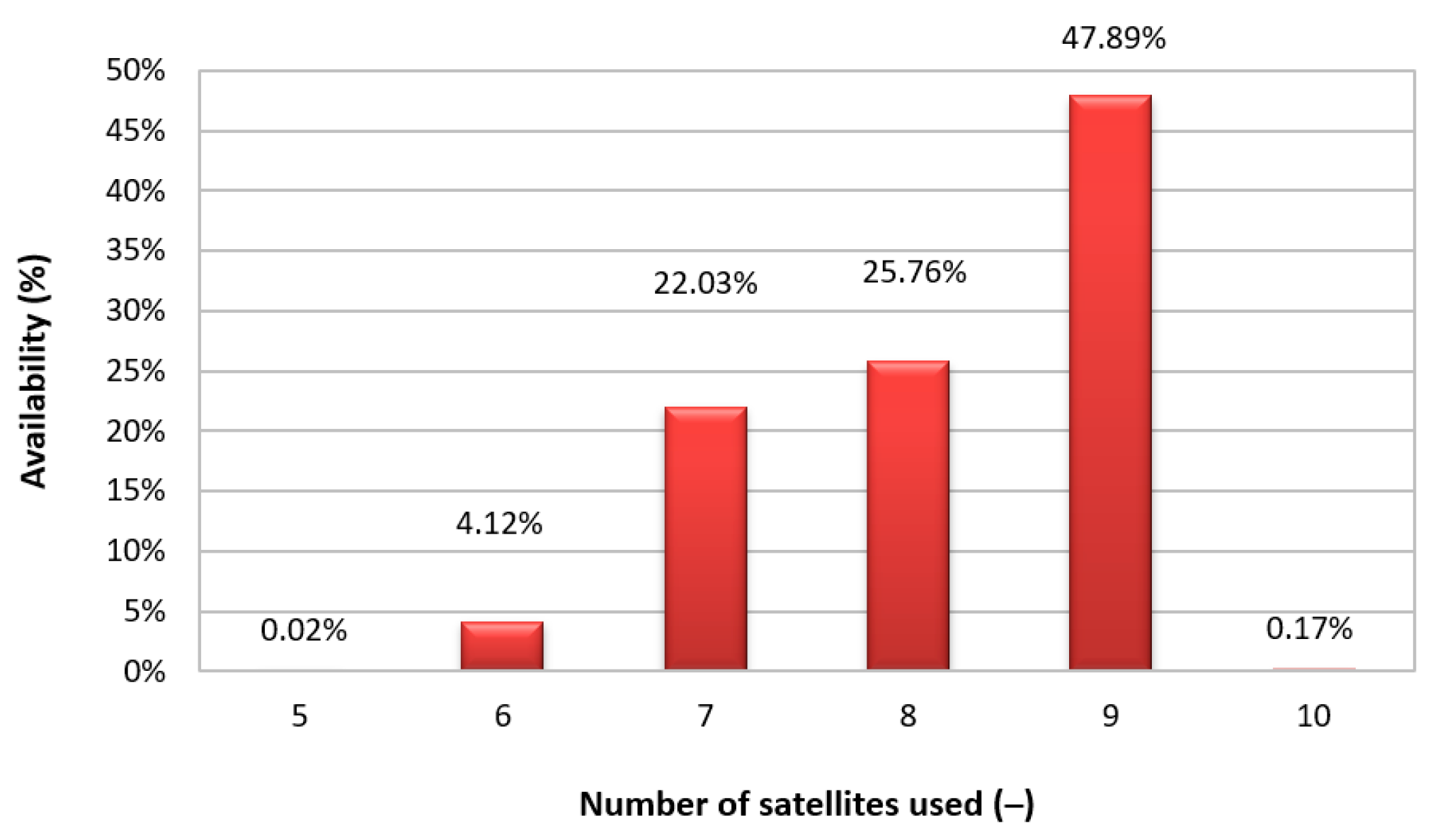
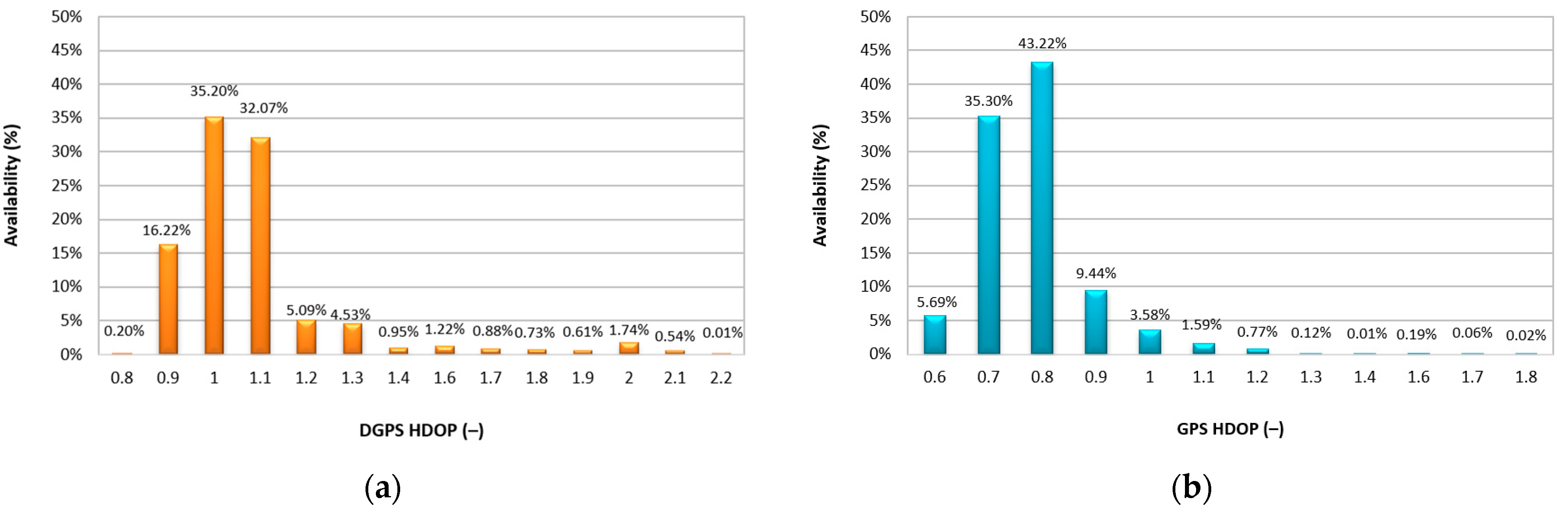
- The typical HDOP values for the maritime DGPS system are ranging from 0.9 to 1.3, which occur a total of 93.11% of the time. The most common values are HDOP = 1.0 and HDOP = 1.1, occurring in 35.2% and 32.07% of the time, respectively;
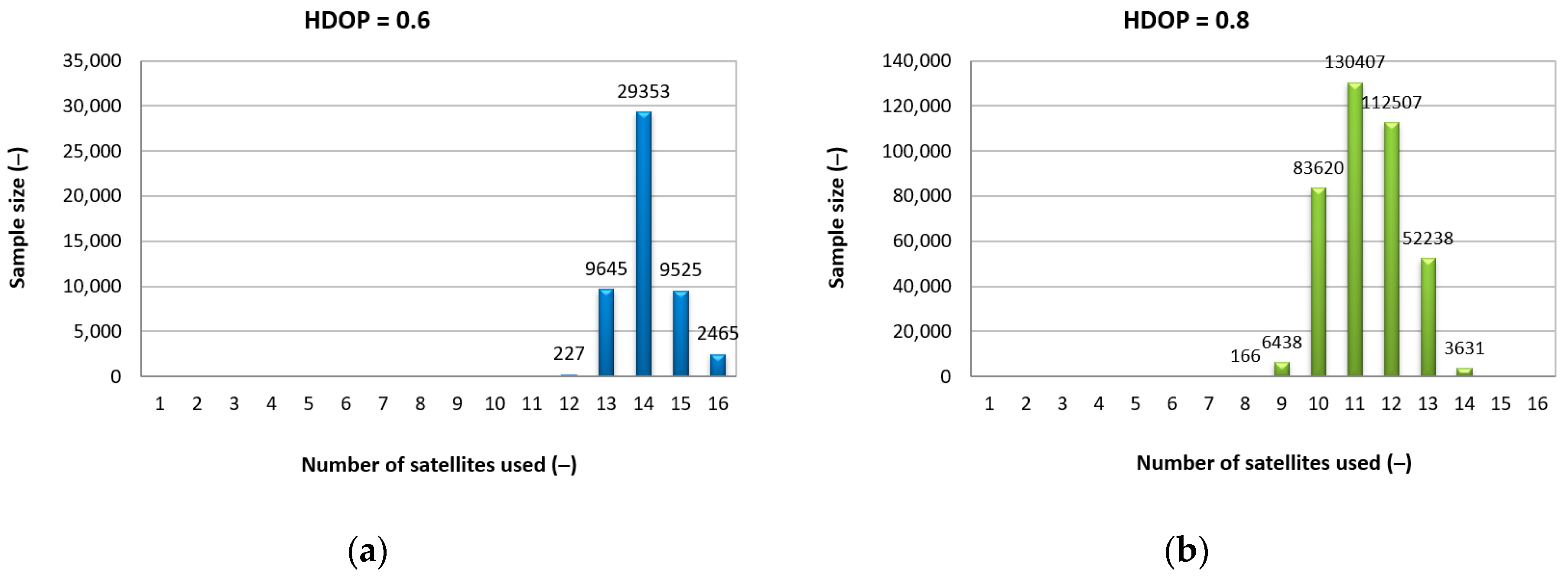
- The DGPS system is characterised by an HDOP value higher than that for the GPS system. A comparison of both systems (GPS and DGPS) shows that the typical HDOP value for the GPS system is lower by 0.2–0.3 in relation to the maritime DGPS system. This results from the differential correction transmission method, which was explained earlier in the publication. However, a study into statistical distributions of the GPS system in 2021 showed that the highest value of HDOP = 0.6 was provided by a GPS constellation consisting of 12, 13, 14, 15, and 16 satellites, of which the value of 14 is predominant. The mean number of satellites was high (14.085), with a relatively small standard deviation of 0.76, which must be emphasised [8].
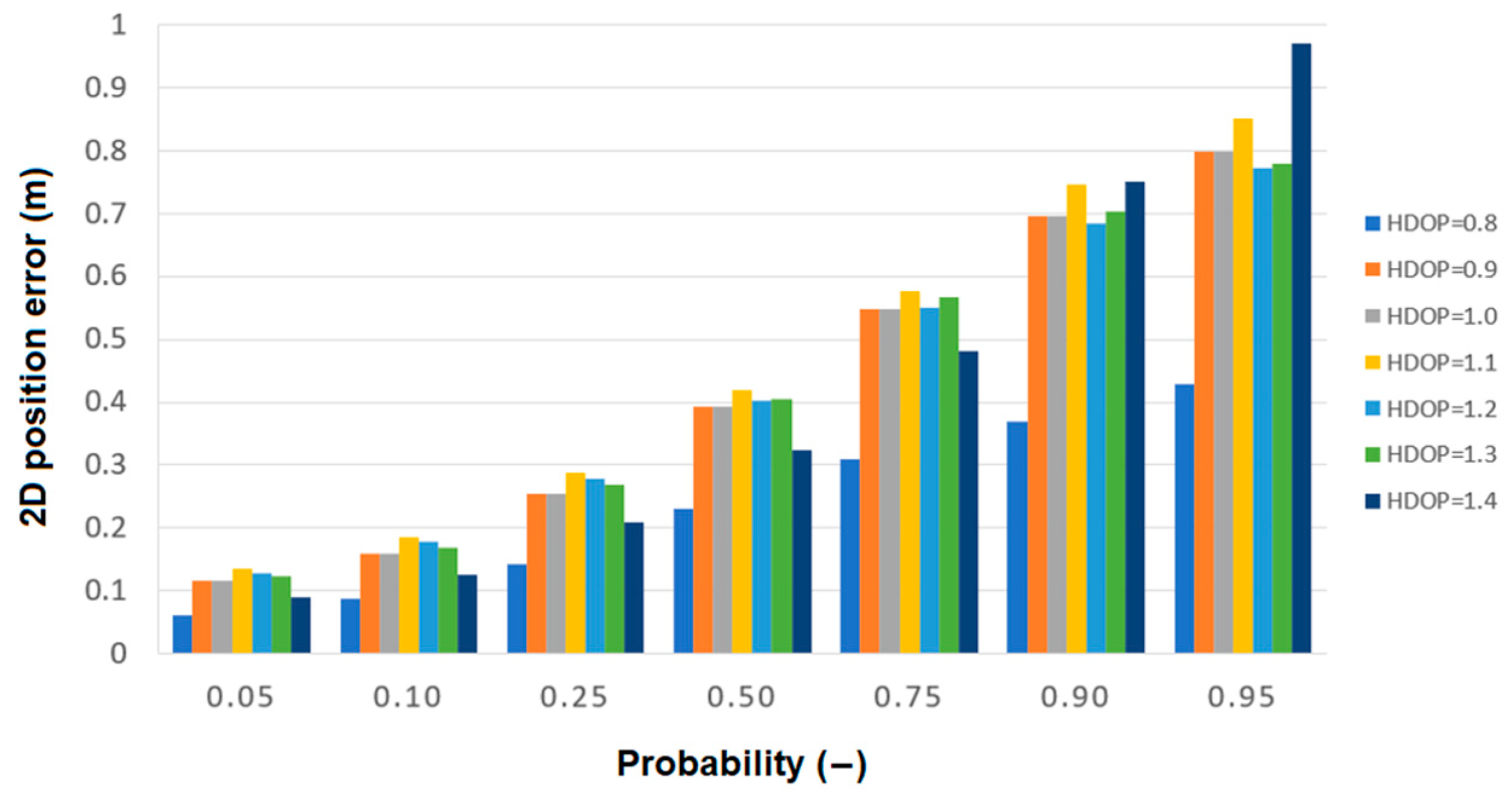
- For the value of HDOP = 0.8, the distribution of DGPS position errors has the smallest error. However, the low number of measurements (0.2% of the population) does not justify making a reliable inference;
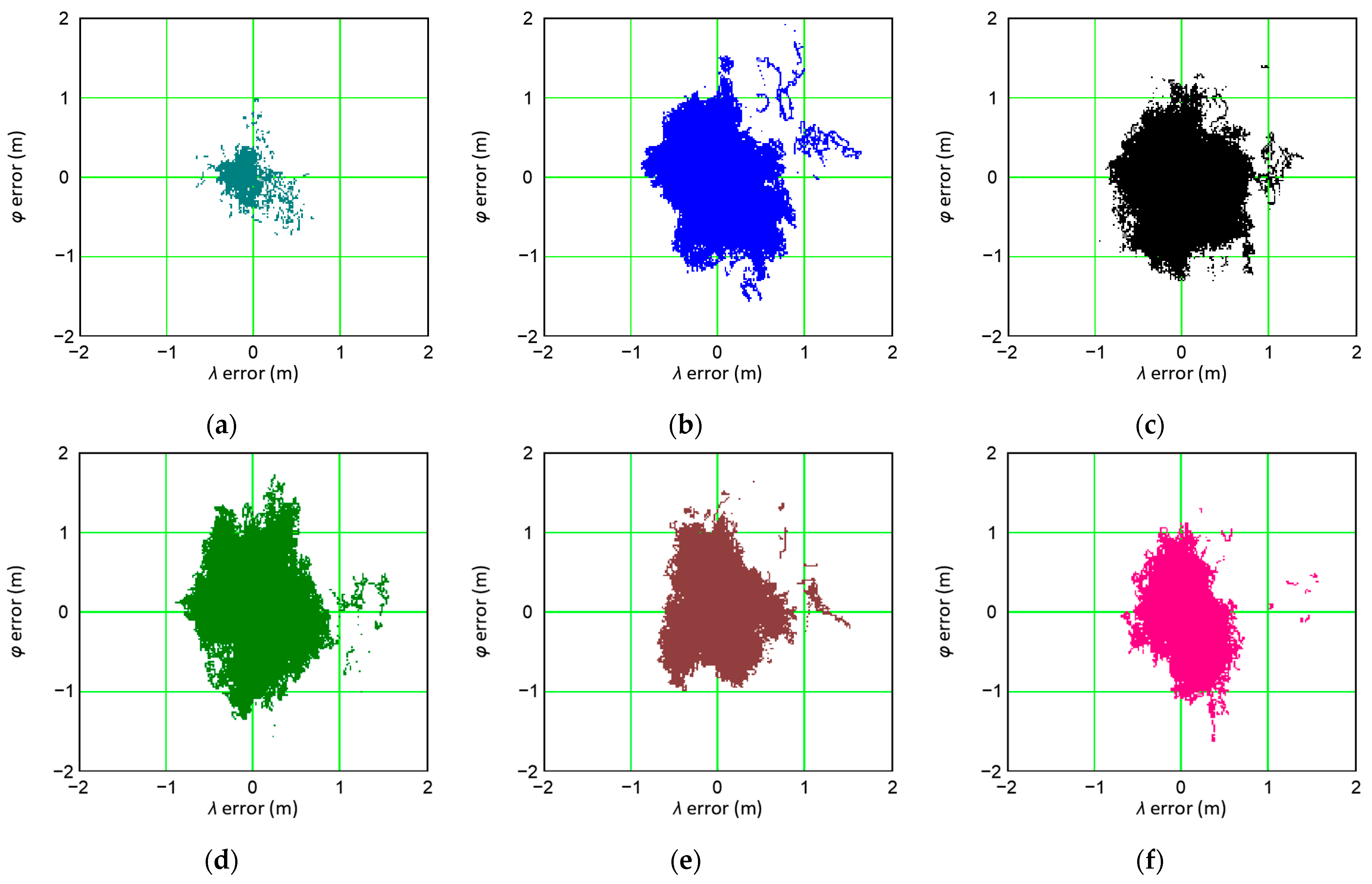
- The distributions of DGPS position errors for the HDOP values ranging from 0.9 to 1.3, from the DGPS position error perspective, have very similar statistical distributions, which may suggest that they have a similar position error value;
- The similar distributions of the DGPS system’s errors may lead to the conclusion that they are virtually independent of the HDOP value for the geometric coefficient range of 0.9–1.3. The very high number of measurements in each set may suggest that this is a particular feature of the DGPS system. It is fundamentally different in relation to the GPS system, where the influence of the HDOP value on the position error is very clear, with changes in the error value of up to 20% [8].
- In the DGPS system, the position error does not change for the HDOP values ranging from 0.9 to 1.3, which means that increasing the HDOP value does not result in a significant increase in the position error, unlike the GPS system in which the position error is strictly determined by the HDOP value;
- For the value of HDOP = 1.4, the DGPS system’s error is noticeably higher than that for other HDOP values from the range of 0.9–1.3. Based on this value, an increase in the position error with the HDOP can be expected, similar to the GPS system.
4. Discussion
5. Conclusions
Funding
Conflicts of Interest
References
- OSTP. Statement by the President Regarding the United States’ Decision to Stop Degrading Global Positioning System Accuracy. Available online: https://clintonwhitehouse4.archives.gov/WH/EOP/OSTP/html/0053_2.html (accessed on 15 December 2022).
- U.S. DoD. Global Positioning System Standard Positioning Service Signal Specification, 1st ed.; U.S. DoD: Arlington, VA, USA, 1993.
- U.S. DoD. Global Positioning System Standard Positioning Service Performance Standard, 3rd ed.; U.S. DoD: Arlington, VA, USA, 2001.
- U.S. DoD. Global Positioning System Standard Positioning Service Performance Standard, 4th ed.; U.S. DoD: Arlington, VA, USA, 2008.
- FAA William J. Hughes Technical Center. Global Positioning System (GPS) Standard Positioning Service (SPS) Performance Analysis Report; Report #86; FAA William J. Hughes Technical Center: Egg Harbor Township, NJ, USA, 2014.
- Renfro, B.A.; Stein, M.; Boeker, N.; Terry, A. An Analysis of Global Positioning System (GPS) Standard Positioning Service (SPS) Performance for 2017; TR-SGL-18-02; SGL: Austin, TX, USA, 2018. [Google Scholar]
- Specht, M. Consistency Analysis of Global Positioning System Position Errors with Typical Statistical Distributions. J. Navig. 2021, 74, 1201–1218. [Google Scholar] [CrossRef]
- Specht, M. Experimental Studies on the Relationship between HDOP and Position Error in the GPS System. Metrol. Meas. Syst. 2022, 29, 17–36. [Google Scholar]
- Ward, N. Future of IALA DGNSS. In Proceedings of the 2006 National Technical Meeting of The Institute of Navigation (ION NTM 2006), Monterey, CA, USA, 18–20 January 2006. [Google Scholar]
- Ferguson, K.; Albright, M.; Remondi, B.W.; Cleveland, A.; Parsons, M. NDGPS Reference Station and Integrity Monitor Architecture Modernization. In Proceedings of the 60th Annual Meeting of The Institute of Navigation, Dayton, OH, USA, 7–9 June 2004. [Google Scholar]
- Hakansson, M.; Jensen, A.B.O.; Horemuz, M.; Hedling, G. Review of Code and Phase Biases in Multi-GNSS Positioning. GPS Solut. 2017, 21, 849–860. [Google Scholar] [CrossRef]
- Krasuski, K.; Ćwiklak, J. Aircraft Positioning Using DGNSS Technique for GPS and GLONASS Data. Sens. Rev. 2020, 40, 559–575. [Google Scholar] [CrossRef]
- Liu, H.; Shu, B.; Xu, L.; Qian, C.; Zhang, R.; Zhang, M. Accounting for Inter-system Bias in DGNSS Positioning with GPS/GLONASS/BDS/Galileo. J. Navig. 2017, 70, 686–698. [Google Scholar] [CrossRef]
- Bakuła, M.; Uradziński, M.; Krasuski, K. Network Code DGNSS Positioning for Faster L1–L5 GPS Ambiguity Initialization. Sensors 2020, 20, 5671. [Google Scholar] [CrossRef] [PubMed]
- Bakuła, M.; Uradziński, M.; Krasuski, K. Performance of DGPS Smartphone Positioning with the Use of P(L1) vs. P(L5) Pseudorange Measurements. Remote Sens. 2022, 14, 929. [Google Scholar] [CrossRef]
- Krasuski, K.; Popielarczyk, D.; Ciećko, A.; Ćwiklak, J. A New Strategy for Improving the Accuracy of Aircraft Positioning Using DGPS Technique in Aerial Navigation. Energies 2021, 14, 4431. [Google Scholar] [CrossRef]
- Alsip, D.H.; Butler, J.M.; Radice, J.T. The Coast Guard’s Differential GPS Program. Navig. J. Inst. Navig. 1992, 39, 345–361. [Google Scholar] [CrossRef]
- U.S. DoT.; U.S. DoD.; U.S. DHS. 2001 Federal Radionavigation Plan; U.S. DoD: Springfield, VA, USA, 2001.
- Johnson, G.; Hartnett, R.; Swaszek, P. DGPS and WAAS Maritime Accuracy and Availability Studies. In Proceedings of the 60th Annual Meeting of The Institute of Navigation, Dayton, OH, USA, 7–9 June 2004. [Google Scholar]
- Monteiro, L.S.; Marreiros, P.R. Analysis of Maritime DGPS Positioning Accuracy at Sea. In Proceedings of the 16th International Technical Meeting of the Satellite Division of The Institute of Navigation (ION GPS/GNSS 2003), Portland, OR, USA, 9–12 September 2003. [Google Scholar]
- Specht, C. Accuracy and Coverage of the Modernized Polish Maritime Differential GPS. Adv. Space Res. 2011, 47, 221–228. [Google Scholar] [CrossRef]
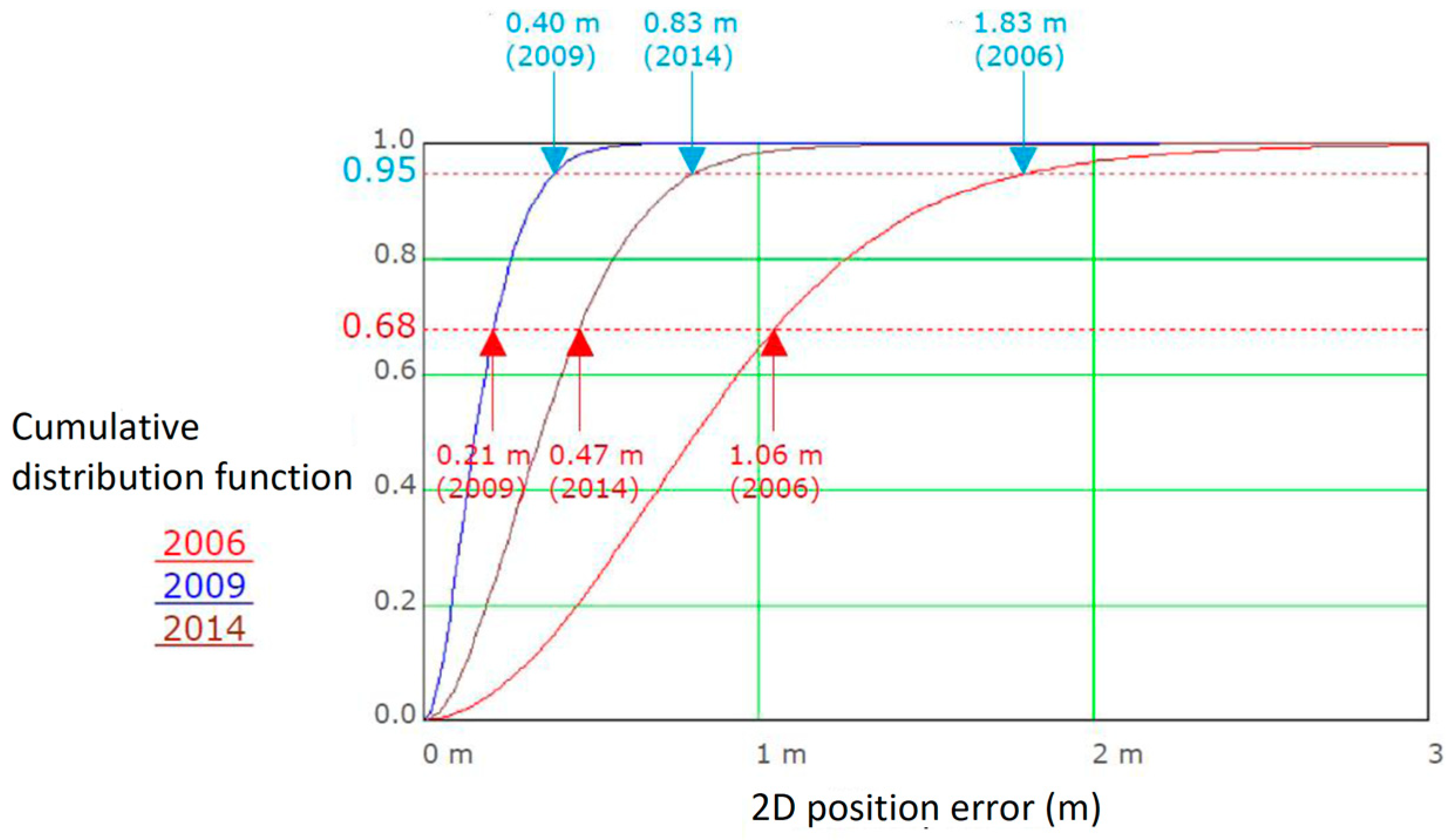
- Sniegocki, H.; Specht, C.; Specht, M. Testing Accuracy of Maritime DGPS System Based on Long-term Measurements Campaigns over the Years 2006–2014. Int. J. Civ. Eng. Technol. 2014, 5, 1–8. [Google Scholar]
- Makar, A. Reliability of the Digital Sea Bottom Model Sourced by Multibeam Echosounder in Shallow Water. In Proceedings of the 5th World Multidisciplinary Earth Sciences Symposium (WMESS 2019), Prague, Czech Republic, 9–13 September 2019. [Google Scholar]
- IHO. IHO Standards for Hydrographic Surveys; Special Publication No. 44, 6th ed.; IHO: Monaco, Monaco, 2020. [Google Scholar]
- Makar, A. Determination of Inland Areas Coastlines. In Proceedings of the 18th International Multidisciplinary Scientific GeoConference (SGEM 2018), Albena, Bulgaria, 2–8 July 2018. [Google Scholar]
- Makar, A. Dynamic Tests of ASG-EUPOS Receiver in Hydrographic Application. In Proceedings of the 18th International Multidisciplinary Scientific GeoConference (SGEM 2018), Albena, Bulgaria, 2–8 July 2018. [Google Scholar]
- Barros, M.S.S.; Rosa, L.C.L.; Walter, F.; Alves, L.H.P.M. Global Positioning System: A Methodology for Modelling the Pseudorange Measurements. Adv. Space Res. 1999, 23, 1529–15232. [Google Scholar] [CrossRef]
- Barros, M.S.S.; Rosa, L.C.L.; Walter, F.; Méndez, D. GPS: Statistical Models to Reproduce the Pseudoranges in Different Scenarios and their Reliability Evaluation. Adv. Space Res. 2001, 28, 227–232. [Google Scholar] [CrossRef]
- Koyama, Y.; Tanaka, T. Improvements in Accurate GPS Positioning Using Time Series Analysis. In Proceedings of the SICE Annual Conference 2010 (SICE 2010), Taipei, Taiwan, 18–21 August 2010. [Google Scholar]
- Belabbas, B.; Hornbostel, A.; Sadeque, M.Z. Error Analysis of Single Frequency GPS Measurements and Impact on Timing and Positioning Accuracy. In Proceedings of the 2nd Workshop on Positioning, Navigation and Communication 2005 (WPNC 2005) and 1st Ultra-wideband Expert Talk 2005 (UET 2005), Hannover, Germany, 17 March 2005. [Google Scholar]
- Belabbas, B.; Petitprez, F.; Hornbostel, A. UERE Analysis for Static Single Frequency Positioning Using Data of IGS Stations. In Proceedings of the 2005 National Technical Meeting of The Institute of Navigation (ION NTM 2005), Long Beach, CA, USA, 13–16 September 2005. [Google Scholar]
- Spilker, J.J., Jr.; Axelrad, P.; Parkinson, B.W.; Enge, P. Global Positioning System: Theory and Applications; AIAA: Reston, VA, USA, 1996; Volume 1. [Google Scholar]
- Hofmann-Wellenhof, B.; Legat, K.; Wieser, M. Navigation—Principles of Positioning and Guidance; Springer: Wien, Austria, 2003. [Google Scholar]
- Langley, R.B. The Mathematics of GPS. GPS World 1991, 2, 45–50. [Google Scholar]
- NGA. Department of Defense World Geodetic System 1984, Its Definition and Relationships with Local Geodetic Systems, 3rd ed.; NGA: Springfield, VA, USA, 2004. [Google Scholar]
- Deakin, R.E.; Hunter, M.N.; Karney, C.F.F. The Gauss-Krüger Projection. In Proceedings of the 23rd Victorian Regional Survey Conference, Warrnambool, Australia, 10–12 September 2010. [Google Scholar]
- Specht, M. Consistency of the Empirical Distributions of Navigation Positioning System Errors with Theoretical Distributions—Comparative Analysis of the DGPS and EGNOS Systems in the Years 2006 and 2014. Sensors 2021, 21, 31. [Google Scholar] [CrossRef] [PubMed]
- Specht, M. Determination of Navigation System Positioning Accuracy Using the Reliability Method Based on Real Measurements. Remote Sens. 2021, 13, 4424. [Google Scholar] [CrossRef]
- Specht, M. Statistical Distribution Analysis of Navigation Positioning System Errors—Issue of the Empirical Sample Size. Sensors 2020, 20, 7144. [Google Scholar] [CrossRef] [PubMed]
- Specht, C.; Smolarek, L.; Pawelski, J.; Specht, M.; Dąbrowski, P. Polish DGPS System: 1995–2017—Study of Positioning Accuracy. Pol. Marit. Res. 2019, 26, 15–21. [Google Scholar] [CrossRef]
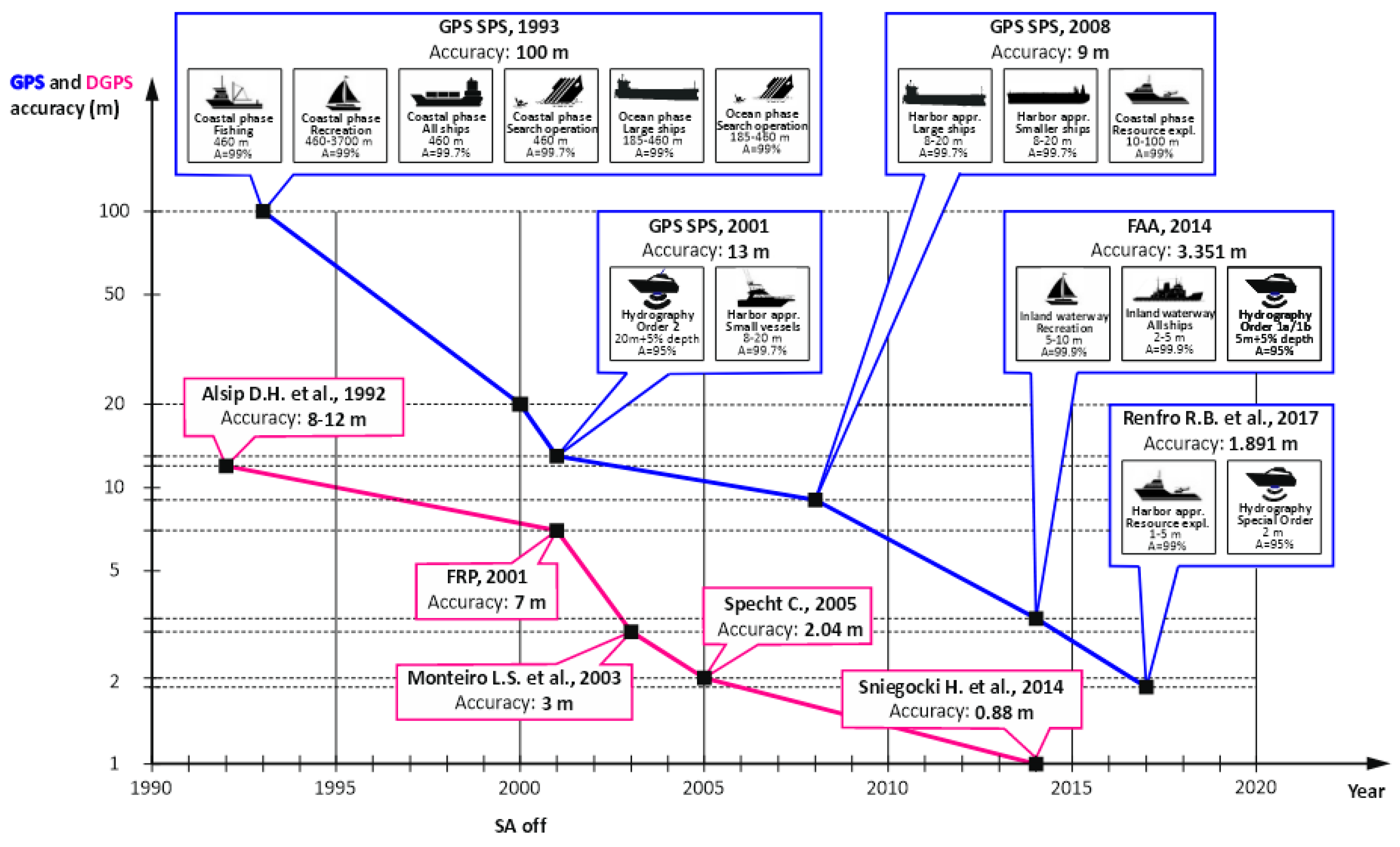
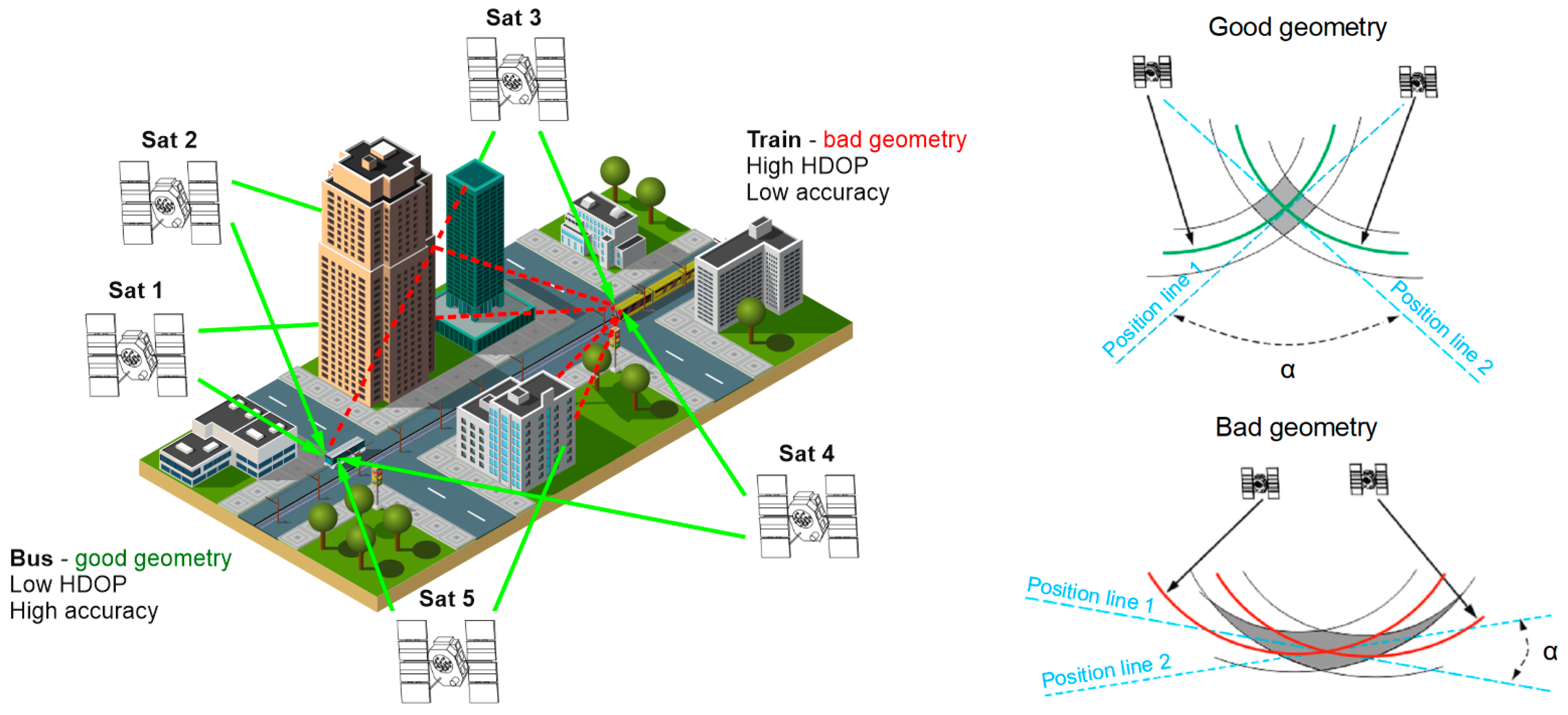
| Criteria | Order 2 | Order 1b | Order 1a | Special Order | Exclusive Order |
|---|---|---|---|---|---|
| Area description | Areas where a general description of the sea floor is considered adequate | Areas where underkeel clearance is not considered to be an issue but surface shipping expected to transit the area | Areas where underkeel clearance is not considered not to be critical but features of concern to surface shipping may exist | Areas where underkeel clearance is critical | Areas where there is strict minimum underkeel clearance and manoeuvrability criteria |
| Depth | 20 m + 10% of depth | 5 m + 5% of depth | 5 m + 5% of depth | 2 m | 1 m |
| Descriptive Statistic | 2D Position Error | ||||||
|---|---|---|---|---|---|---|---|
| HDOP = 0.8 | HDOP = 0.9 | HDOP = 1.0 | HDOP = 1.1 | HDOP = 1.2 | HDOP = 1.3 | HDOP = 1.4 | |
| Range | 0.965 m | 2.063 m | 2.013 m | 10.061 m | 1.684 m | 1.659 m | 2.320 m |
| Mean | 0.236 m | 0.417 m | 0.399 m | 0.448 m | 0.424 m | 0.426 m | 0.387 m |
| Standard deviation | 0.127 m | 0.218 m | 0.208 m | 0.224 m | 0.202 m | 0.208 m | 0.262 m |
| 5th percentile | 0.062 m | 0.116 m | 0.098 m | 0.134 m | 0.127 m | 0.124 m | 0.089 m |
| 10th percentile | 0.087 m | 0.160 m | 0.142 m | 0.186 m | 0.179 m | 0.169 m | 0.125 m |
| 25th percentile (Q1) | 0.142 m | 0.254 m | 0.242 m | 0.289 m | 0.278 m | 0.268 m | 0.209 m |
| 50th percentile (Median) | 0.230 m | 0.392 m | 0.378 m | 0.419 m | 0.403 m | 0.404 m | 0.323 m |
| 75th percentile (Q3) | 0.309 m | 0.549 m | 0.535 m | 0.576 m | 0.551 m | 0.568 m | 0.482 m |
| 90th percentile | 0.369 m | 0.697 m | 0.683 m | 0.746 m | 0.685 m | 0.703 m | 0.751 m |
| 95th percentile | 0.430 m | 0.799 m | 0.767 m | 0.852 m | 0.772 m | 0.781 m | 0.971 m |
| Max percentile | 0.971 m | 2.069 m | 2.019 m | 10.068 m | 1.690 m | 1.666 m | 2.326 m |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Specht, C. Maritime DGPS System Positioning Accuracy as a Function of the HDOP in the Context of Hydrographic Survey Performance. Remote Sens. 2023, 15, 10. https://doi.org/10.3390/rs15010010
Specht C. Maritime DGPS System Positioning Accuracy as a Function of the HDOP in the Context of Hydrographic Survey Performance. Remote Sensing. 2023; 15(1):10. https://doi.org/10.3390/rs15010010
Chicago/Turabian StyleSpecht, Cezary. 2023. "Maritime DGPS System Positioning Accuracy as a Function of the HDOP in the Context of Hydrographic Survey Performance" Remote Sensing 15, no. 1: 10. https://doi.org/10.3390/rs15010010
APA StyleSpecht, C. (2023). Maritime DGPS System Positioning Accuracy as a Function of the HDOP in the Context of Hydrographic Survey Performance. Remote Sensing, 15(1), 10. https://doi.org/10.3390/rs15010010







