Identification of the Potential Critical Slip Surface for Fractured Rock Slope Using the Floyd Algorithm
Abstract
:1. Introduction
2. Materials and Methods
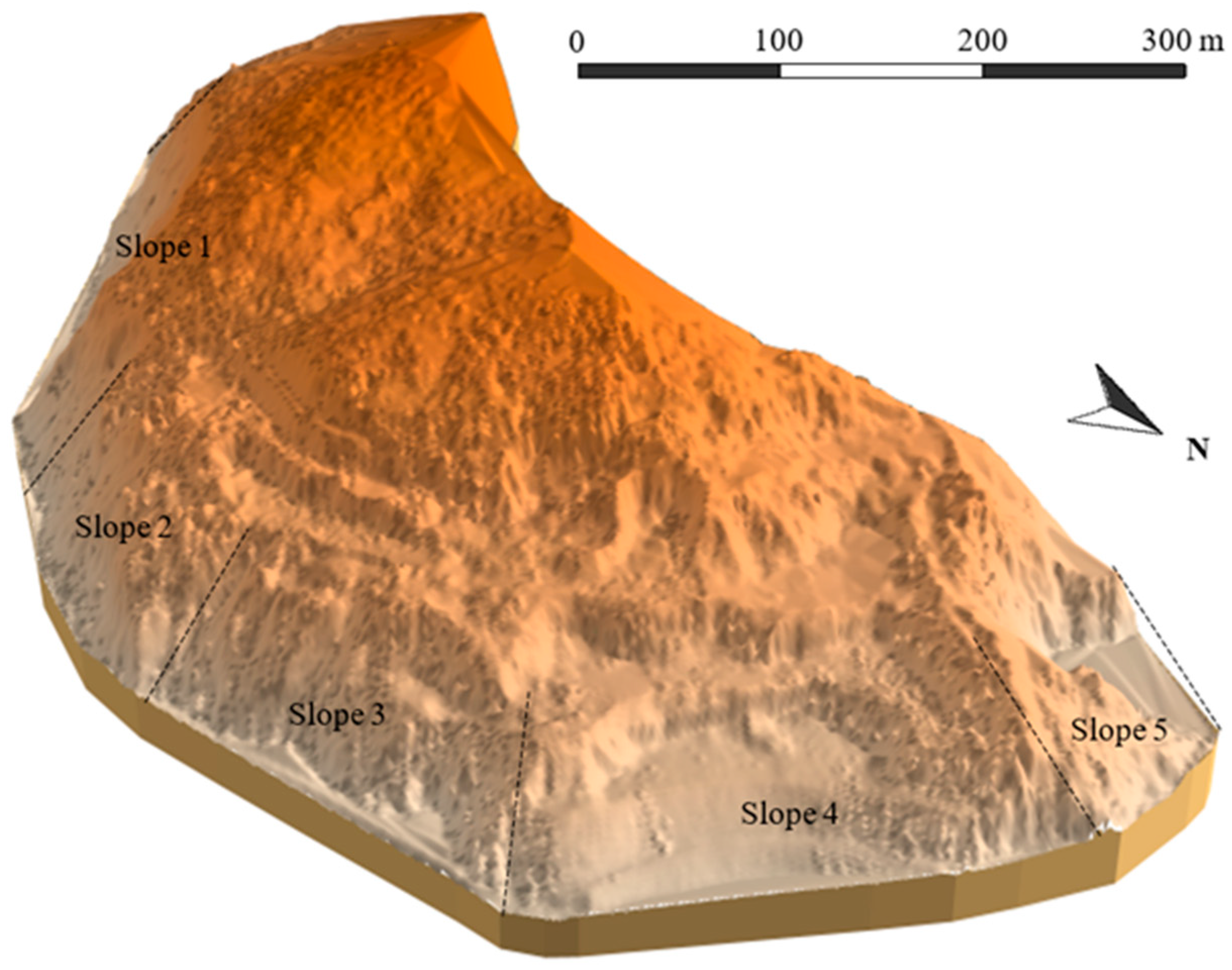
2.1. Study Area
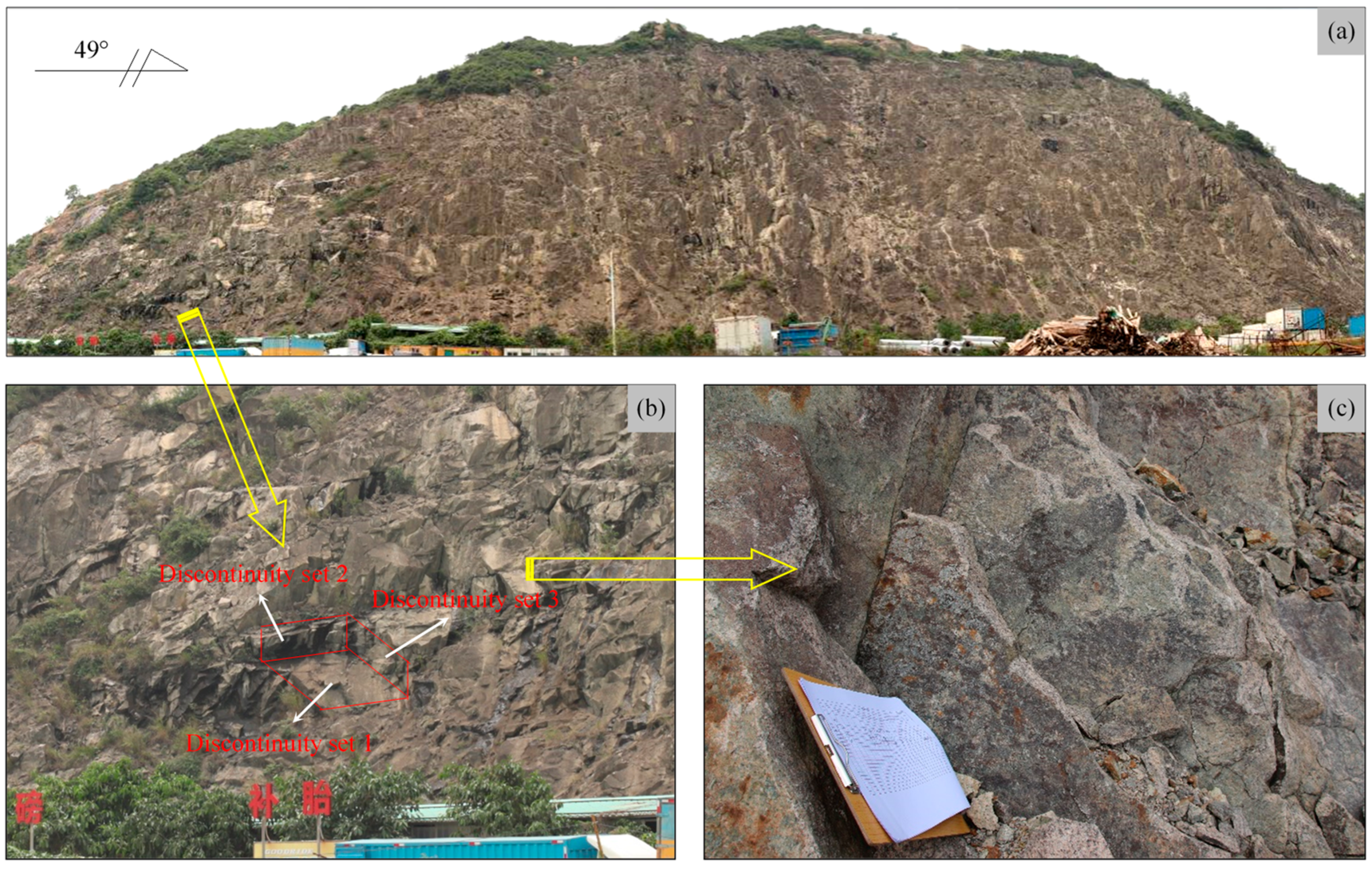
2.2. Collecting Discontinuity by Digital Close-Range Photogrammetry
2.3. Fracture Generation in the Cross-Section of the Slope
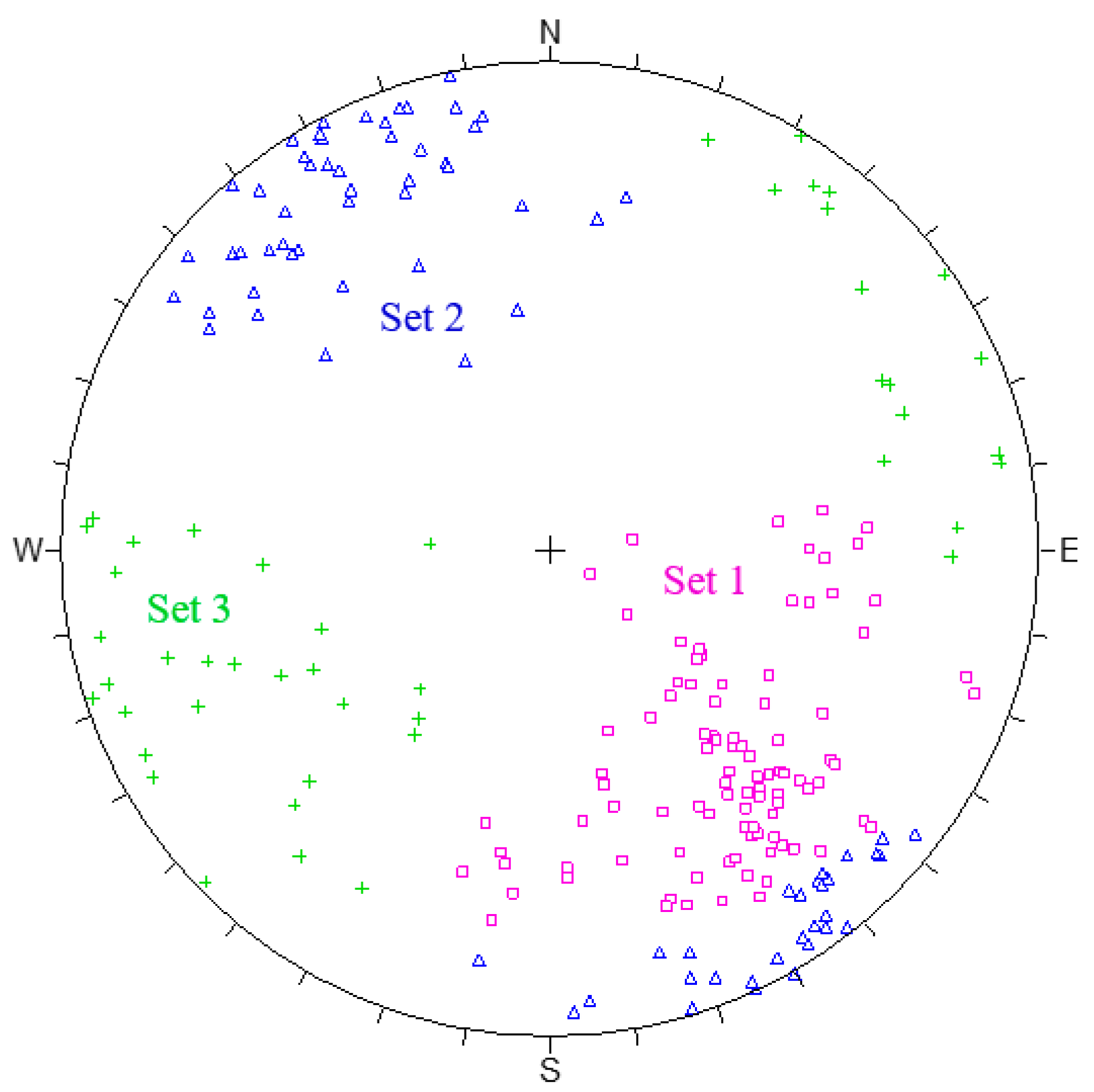
2.3.1. Analysis of Fracture Orientation
2.3.2. Determination of Trace Length, Diameter, and Density
2.3.3. Monte Carlo Simulation and Model Verification
2.3.4. Fracture Extraction in the Cross-Section
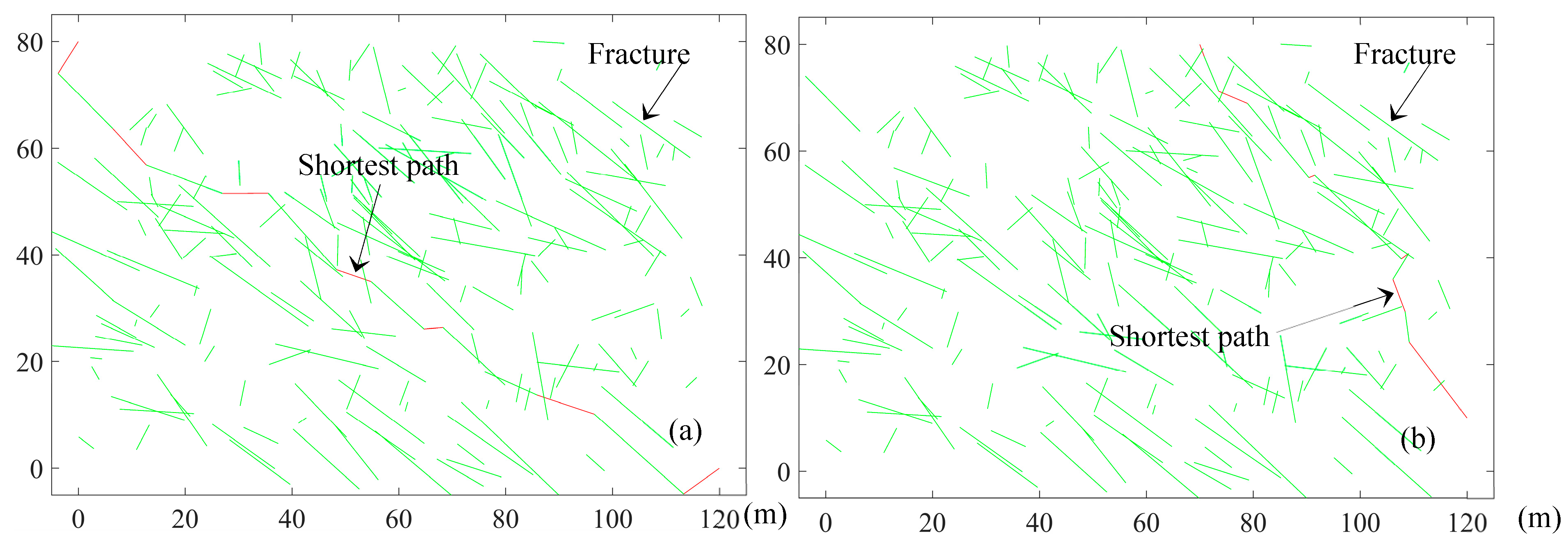
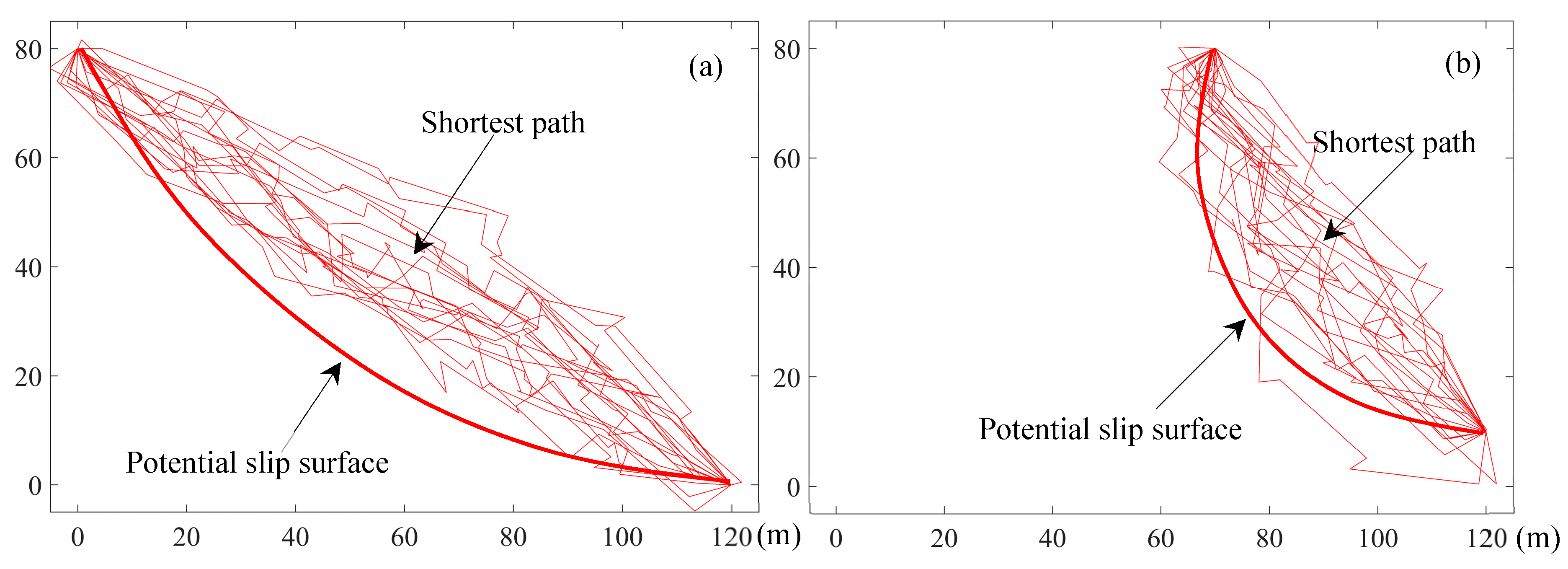
2.4. Floyd Algorithm for Searching the Shortest Path
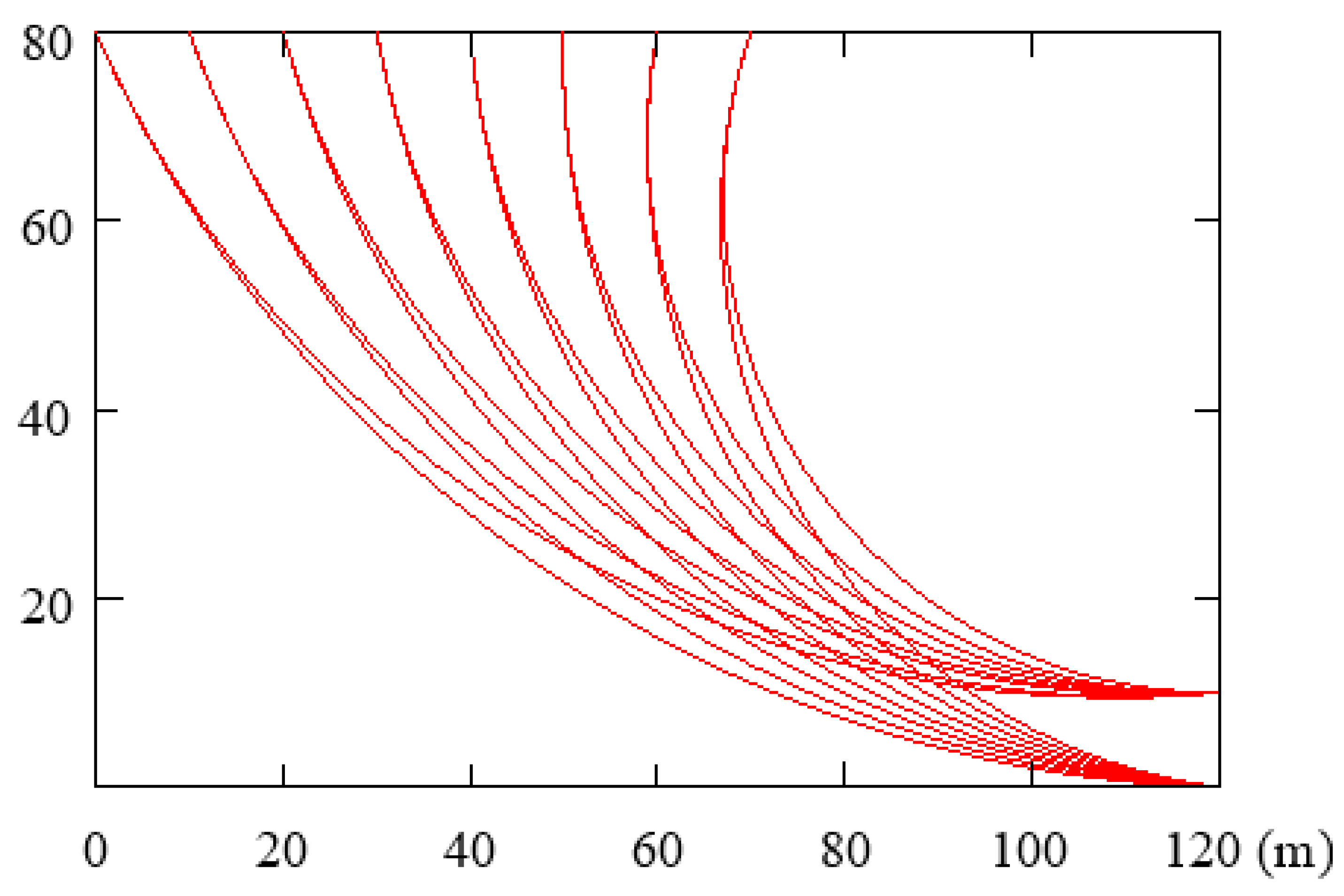
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Singh, R.; Umrao, R.K.; Singh, T.N. Stability evaluation of road-cut slopes in the Lesser Himalaya of Uttarakhand, India: Conventional and numerical approaches. Bull. Eng. Geol. Environ. 2014, 73, 845–857. [Google Scholar] [CrossRef]
- Song, S.Y.; Xu, Q.; Chen, J.P.; Wang, F.Y.; Liu, Y.; Liu, Y.F. Relationship between the representative element volume and discontinuity parameters. Q. J. Eng. Geol. Hydrog. 2020, 54, 1–12. [Google Scholar] [CrossRef]
- Glade, T.; Anderson, M.; Crozier, M.J. (Eds.) Landslide Hazard and Risk; John Wiley & Sons: West Sussex, UK, 2005; pp. 43–74. [Google Scholar]
- Jellali, B.; Frikha, W. Constrained particle swarm optimization algorithm applied to slope stability. Int. J. Geomech. 2017, 17, 06017022. [Google Scholar] [CrossRef]
- Anderson, M.G.; Richards, K.S. (Eds.) Slope Stability; Geotechnical Engineering and Geomorphology; John Wiley & Sons: West Sussex, UK, 1987; pp. 11–75. [Google Scholar]
- Zhang, X.; Zhu, C.; He, M.; Dong, M.; Zhang, G.; Zhang, F. Failure Mechanism and Long Short-Term Memory Neural Network Model for Landslide Risk Prediction. Remote Sens. 2022, 14, 166. [Google Scholar] [CrossRef]
- Bishop, A.W.; Morgenstern, N. Stability coefficients for earth slopes. Géotechnique 1960, 10, 129–153. [Google Scholar] [CrossRef]
- Cheng, Y.M.; Liang, L.; Chi, S.C.; Wei, W.B. Determination of the critical slip surface using artificial fish swarms algorithm. J. Geotech. Geoenviron. Eng. 2008, 134, 244–251. [Google Scholar] [CrossRef]
- Kahatadeniya, K.S.; Nanakorn, P.; Neaupane, K.M. Determination of the critical failure surface for slope stability analysis using ant colony optimization. Eng. Geol. 2009, 108, 133–141. [Google Scholar] [CrossRef]
- Zeng, P.; Jimenez, R.; Jurado-Piña, R. System reliability analysis of layered soil slopes using fully specified slip surfaces and genetic algorithms. Eng. Geol. 2015, 193, 106–117. [Google Scholar] [CrossRef] [Green Version]
- Kashani, A.R.; Gandomi, A.H.; Mousavi, M. Imperialistic competitive algorithm: A metaheuristic algorithm for locating the critical slip surface in 2-Dimensional soil slopes. Geosci. Front. 2016, 26, 83–89. [Google Scholar] [CrossRef] [Green Version]
- Gao, W. Investigating the critical slip surface of soil slope based on an improved black hole algorithm. Soils Found. 2017, 57, 988–1001. [Google Scholar] [CrossRef]
- Tang, S.B.; Huang, R.Q.; Tang, C.A.; Liang, Z.Z.; Heap, M.J. The failure processes analysis of rock slope using numerical modelling techniques. Eng. Fail. Anal. 2017, 79, 999–1016. [Google Scholar] [CrossRef]
- Kıncal, C. Application of two new stereographic projection techniques to slope stability problems. Int. J. Rock Mech. Min. Sci. 2014, 66, 136–150. [Google Scholar] [CrossRef]
- Einstein, H.H.; Veneziano, D.; Baecher, G.B.; O’Reilly, K.J. The effect of discontinuity persistence on rock slope stability. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1983, 20, 227–236. [Google Scholar] [CrossRef]
- Zhu, D.Y.; Qian, Q.H.; Zhou, Z.S.; Zheng, H.T. Technique for computing critical slip field of rock slope and its application to design open pit slope. Chin. J. Rock Mech. Eng. 1999, 18, 497–502. [Google Scholar]
- Zhang, W.; Chen, J.P.; Zhang, W.; Lv, Y.; Ma, Y.F.; Xiong, H. Determination of critical slip surface of fractured rock slopes based on fracture orientation data. Sci. China Technol. Sci. 2013, 56, 1248–1256. [Google Scholar] [CrossRef]
- Zheng, W.B.; Zhuang, X.Y.; Tannant, D.D.; Cai, Y.C.; Nunoo, S. Unified continuum/discontinuum modeling framework for slope stability assessment. Eng. Geol. 2014, 179, 90–101. [Google Scholar] [CrossRef]
- Liu, Y.; Li, H.; Xiao, K.; Li, J.; Xia, X.; Liu, B. Seismic Stability Analysis of a Layered Rock Slope. Comput. Geotech. 2014, 55, 474–481. [Google Scholar] [CrossRef]
- Xu, Q.; Chen, J.; Li, J.; Yue, H. A genetic algorithm for locating the multiscale critical slip surface in jointed rock mass slopes. Math. Probl. Eng. 2014, 2014, 1–10. [Google Scholar] [CrossRef]
- Seedahmed, G.H. Direct Retrieval of Exterior Orientation Parameters Using a 2D Projective Transformation. Photogramm. Rec. 2006, 21, 211–231. [Google Scholar] [CrossRef]
- Chen, J.P.; Xiao, S.F.; Wang, Q. Computer Simulation Theory of 3D Random Discontinuities Network; Northeast Normal University Press: Changchun, China, 1995. [Google Scholar]
- Song, S.Y.; Wang, Q.; Chen, J.P.; Li, Y.Y.; Zhang, W.; Ruan, Y.K. Fuzzy C-means clustering analysis based on quantum particle swarm optimization algorithm for the grouping of rock discontinuity sets. KSCE J. Civ. Eng. 2017, 21, 1115–1122. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W.; Wu, T.H. Sampling bias on orientation of discontinuities. Rock Mech. Rock Eng. 1984, 17, 243–253. [Google Scholar] [CrossRef]
- Priest, S.D. Discontinuity Analysis for Rock Engineering; Chapman & Hall: London, UK, 1993. [Google Scholar]
- Zhang, Q.; Wang, Q.; Chen, J.P.; Li, Y.Y.; Ruan, Y.K. Estimation of mean trace length by setting scanlines in rectangular sampling window. Int. J. Rock Mech. Min. Sci. 2016, 84, 74–79. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W.; Wu, T.H. Relation between Discontinuity Size and Trace Length. In Proceeding of the 27th US Symposium on Rock Mechanics, American Rock Mechanics Association, Tuscaloosa, AL, USA, 23–25 June 1986; pp. 130–133. [Google Scholar]
- Oda, M. Fabric tensor for discontinuous geological materials. Soils Found. 1982, 22, 96–108. [Google Scholar] [CrossRef] [Green Version]
- Meyer, T.; Einstein, H.H. Geologic Stochastic Modeling and Connectivity Assessment of Fracture Systems in the Boston Area. Rock Mech. Rock Eng. 2002, 35, 23–44. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W.; Wathugala, D.N.; Stephansson, O. Joint network modelling with a validation exercise in Stripa mine, Sweden. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1993, 30, 503–526. [Google Scholar] [CrossRef]
- Devoto, S.; Hastewell, L.J.; Prampolini, M.; Furlani, S. Dataset of Gravity-Induced Landforms and Sinkholes of the Northeast Coast of Malta (Central Mediterranean Sea). Data 2021, 6, 81. [Google Scholar] [CrossRef]
- Yong, R.; Ye, J.; Li, B.; Du, S.G. Determining the maximum sampling interval in rock joint roughness measurements using fourier series. Int. J. Rock Mech. Min. Sci. 2018, 101, 78–88. [Google Scholar] [CrossRef]
- Liao, Y.M.; Zhong, J. Floyd Algorithm Based on the Shortest Path in GIS. In Information and Business Intelligence, Proceedings of International Conference on Information and Business Intelligence, Chongqing, China, 23–25 December 2011; Springer: Berlin/Heidelberg, Germany, 2012; pp. 574–579. [Google Scholar]
- Li, Y.; Li, D.; Yu, Y. Multi-Point Shortest Path in the Complex Road Network Based on Floyd Algorithm. In Information Computing and Applications, Proceedings of International Conference on Information Computing and Applications, Singapore, 16–18 August 2013; Springer: Berlin/Heidelberg, Germany, 2013; pp. 364–372. [Google Scholar]
- Lei, T.; Xing, T.; Xin, H.; Xu, H.; Tan, Z.F. Location analysis of electric vehicle charging station based on the Floyd shortest path algorithm. Appl. Mech. Mater. 2013, 389, 1014–1018. [Google Scholar]
- Zhang, W.; Lan, Z.G.; Ma, Z.F.; Tan, C.; Que, J.S.; Wang, F.Y.; Cao, C. Determination of statistical discontinuity persistence for a rock mass characterized by non-persistent fractures. Int. J. Rock Mech. Min. Sci. 2020, 126, 104177. [Google Scholar] [CrossRef]





| Fracture Set | Fracture Number | Trace Length | Fracture Diameter | Density (m−3) | K | ||||
|---|---|---|---|---|---|---|---|---|---|
| Mean (m) | Std. (m) | Distribution Type | Mean (m) | Std. (m) | Distribution Type | ||||
| 1 | 99 | 12.97 | 14.87 | Gamma | 16.98 | 4.73 | Gamma | 0.000544 | 12.97 |
| 2 | 77 | 3.56 | 5.02 | Gamma | 5.52 | 1.33 | Gamma | 0.001035 | 13.78 |
| 3 | 46 | 6.05 | 7.75 | Gamma | 7.58 | 2.94 | Gamma | 0.000422 | 5.51 |
| Fracture Set | Data Type | Fracture Number | Mean Orientation (°) | Mean Trace Length (m) | Trace Type | K | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| Dip Direction | Dip Angle | Observed | Corrected | R0 | R1 | R2 | ||||
| 1 | Surveyed | 99 | 140.8 | 51.2 | 12.97 | 16.05 | 0.00 | 0.07 | 0.93 | 12.97 |
| Simulated | 95 | 141.3 | 48.4 | 12.33 | 12.43 | 0.00 | 0.28 | 0.72 | 15.60 | |
| 2 | Surveyed | 77 | 150.8 | 85.2 | 3.56 | 5.04 | 0.00 | 0.04 | 0.96 | 13.78 |
| Simulated | 75 | 148.0 | 84.8 | 4.54 | 5.45 | 0.00 | 0.15 | 0.85 | 14.70 | |
| 3 | Surveyed | 46 | 246.9 | 81.8 | 6.05 | 8.02 | 0.00 | 0.07 | 0.93 | 5.51 |
| Simulated | 44 | 249.5 | 77.8 | 5.94 | 8.47 | 0.00 | 0.19 | 0.81 | 5.70 | |
| Shear Entrance | Shear Exit | Circle Center | Radius | Fracture Frequency | Shear Entrance | Shear Exit | Circle Center | Radius | Fracture Frequency |
|---|---|---|---|---|---|---|---|---|---|
| (0, 80) | (120, 0) | (123.8, 135.6) | 135.7 | 0.742 | (0, 80) | (120, 10) | (109.1, 129.1) | 119.6 | 0.738 |
| (10, 80) | (125.1, 122.6) | 122.7 | 0.739 | (10, 80) | (110.5, 116.6) | 107.0 | 0.737 | ||
| (20, 80) | (126.1, 110.2) | 110.3 | 0.735 | (20, 80) | (113.8, 107.5) | 97.7 | 0.731 | ||
| (30, 80) | (126.4, 97.9) | 98.1 | 0.734 | (30, 80) | (114.5, 95.8) | 86.0 | 0.735 | ||
| (40, 80) | (127.0, 87.0) | 87.3 | 0.736 | (40, 80) | (115.8, 85.9) | 76.0 | 0.732 | ||
| (50, 80) | (127.3, 77.0) | 77.4 | 0.724 | (50, 80) | (116.8, 76.8) | 66.9 | 0.720 | ||
| (60, 80) | (127.5, 68.1) | 68.5 | 0.715 | (60, 80) | (117.7, 68.8) | 58.8 | 0.714 | ||
| (70, 80) | (127.7, 60.4) | 60.9 | 0.701 | (70, 80) | (119.6, 62.6) | 52.6 | 0.700 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, S.; Zhao, M.; Zhu, C.; Wang, F.; Cao, C.; Li, H.; Ma, M. Identification of the Potential Critical Slip Surface for Fractured Rock Slope Using the Floyd Algorithm. Remote Sens. 2022, 14, 1284. https://doi.org/10.3390/rs14051284
Song S, Zhao M, Zhu C, Wang F, Cao C, Li H, Ma M. Identification of the Potential Critical Slip Surface for Fractured Rock Slope Using the Floyd Algorithm. Remote Sensing. 2022; 14(5):1284. https://doi.org/10.3390/rs14051284
Chicago/Turabian StyleSong, Shengyuan, Mingyu Zhao, Chun Zhu, Fengyan Wang, Chen Cao, Haojie Li, and Muye Ma. 2022. "Identification of the Potential Critical Slip Surface for Fractured Rock Slope Using the Floyd Algorithm" Remote Sensing 14, no. 5: 1284. https://doi.org/10.3390/rs14051284
APA StyleSong, S., Zhao, M., Zhu, C., Wang, F., Cao, C., Li, H., & Ma, M. (2022). Identification of the Potential Critical Slip Surface for Fractured Rock Slope Using the Floyd Algorithm. Remote Sensing, 14(5), 1284. https://doi.org/10.3390/rs14051284









