Epoch-Based Height Reference System for Sea Level Rise Impact Assessment on the Coast of Peninsular Malaysia
Abstract
1. Introduction
2. Materials and Methods
2.1. Epoch-Based Height Reference System
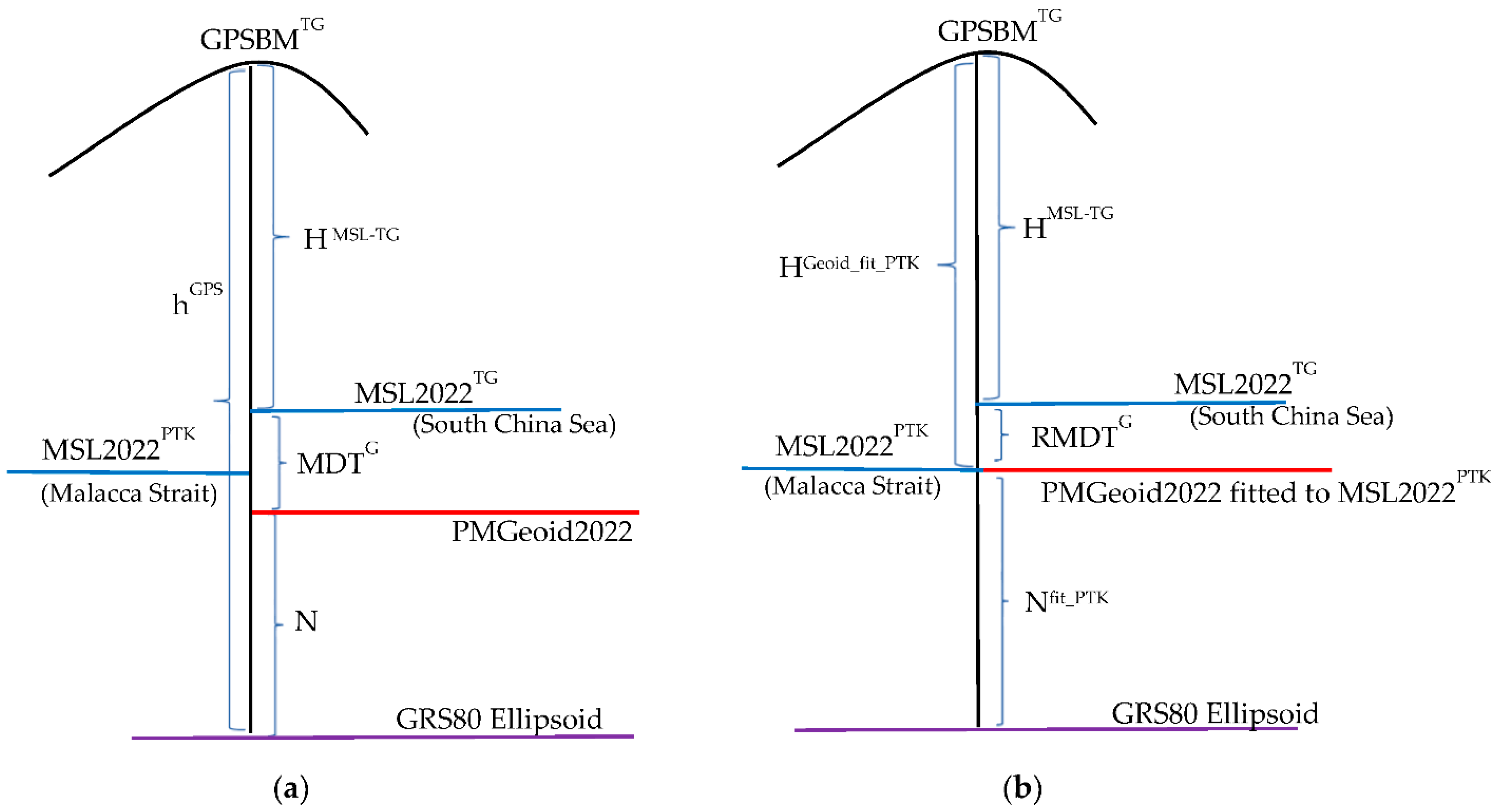
- A physical option of GVD, in which a constant value of the Earth’s gravity potential, W = W0 = constant, is specified, characterising the geoid as a single horizontal surface. The geopotential number at point P (in a geocentric reference frame) is given as CP = W0 − WP (m2s−2), with P0 on the geoid and WP = W(P). Orthometric height, H (m) is the height above the geoid at point P measured along the local vertical or plumb line, and it is always perpendicular to the equipotential surfaces: H = CP/ğ, where ğ (ms−2) is the gravity mean value along the plumb line [24]. Any application in hydrology requires geopotential-based heights since they demonstrate the water’s natural flow. A resolution in defining and realising the International Height Reference System (IHRS) was issued by the International Association of Geodesy (IAG) in July 2015. The IHRS coordinates are defined in this resolution as potential differences with respect to the equipotential surface of the Earth’s gravity field, which is realised by the conventional value of W0 = 62 636 853.4 m2s−2 [25,26].
- A geometrical LVD option in which the selected horizontal surface (the geoid) must resemble the MSL surface in a certain way. In the context of the application where a vertical datum is required to provide heights in a consistent system for a particular nation or region, the geometrical option, i.e., the height above the local MSL datum or gravimetric geoid fitted to the local MSL is normally selected. The orthometric height, H, barely varies from the height measured along the normal to the reference ellipsoid (even for the extreme case of deflection of the vertical, ξ = 1 arcmin and H = 10,000 m, ΔH = H sin ξ tan ξ < 1 mm) [27]. Other error sources are more significant such as random errors in levelling, ellipsoidal height errors, and geoid modelling errors. The geoid undulation or geoid height (N), expressed as H = h − N − N0 is required to convert ellipsoidal heights (h) from coordinates in the geodetic reference system acquired from GNSS levelling to orthometric heights (H) in the vertical datum. N0 is a constant offset between the geoid and the vertical datum [28]. The general description of H is such that H = 0 is the geoid, H > 0 is for terrain heights above the geoid, and H < 0 is below the geoid (depth of the sea floor).
- Geodetic reference frame and reference ellipsoid. It is defined by the semi-kinematic geocentric datum GDM2020/ITRF2014@2020.0 and the GRS80/WGS84 as its ellipsoid of reference [21].
- Geoid surface (N). Is it the gravimetric geoid referenced to the local MSL at TG stations. Because temporal variations in geoid heights should be addressed only when defining geoid models with 1 cm accuracy, the geoid is defined as a static surface (N(t) = N(t0)) [29].
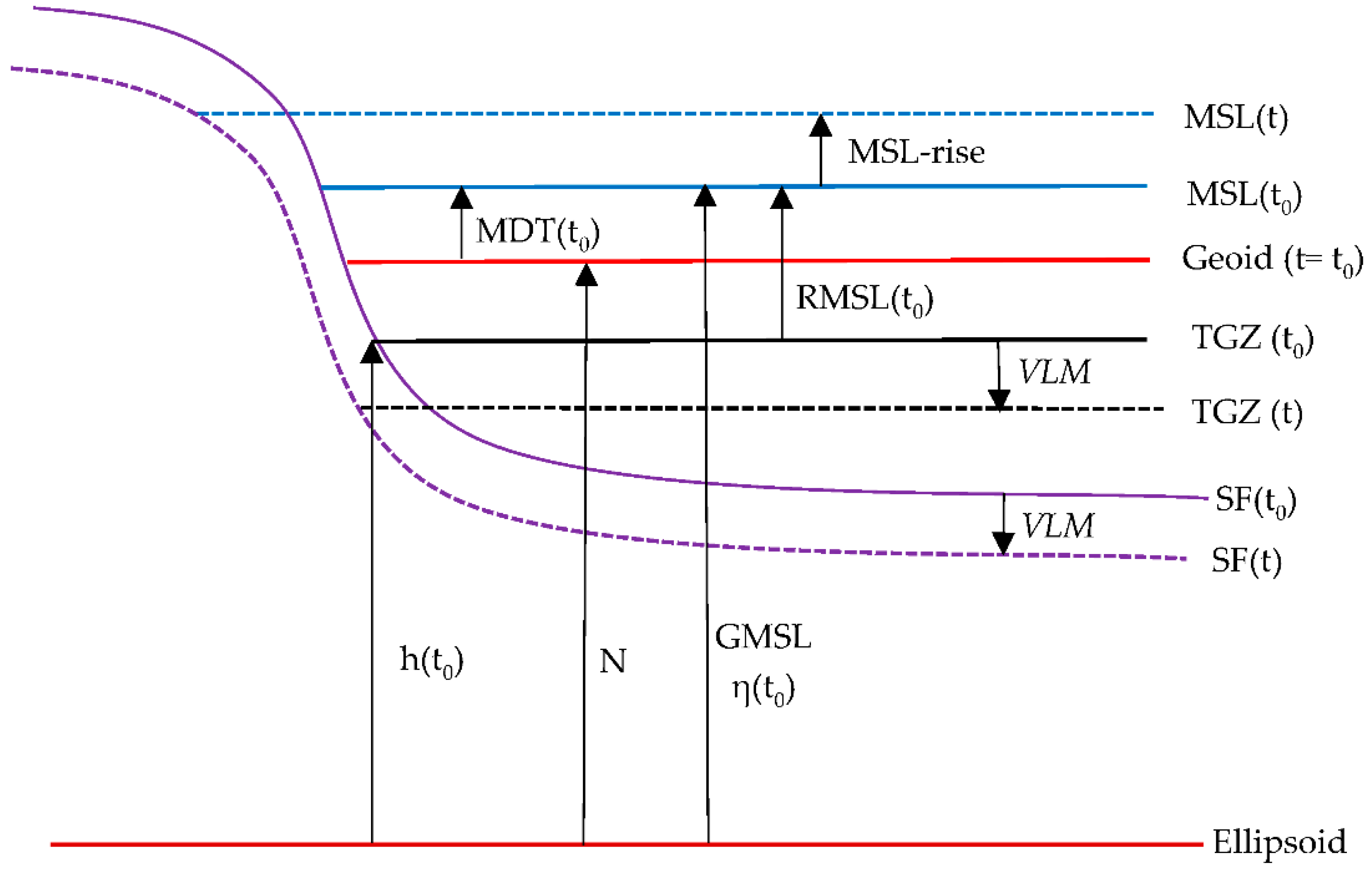
- Mean sea level (η, MSL) is the temporal average of the sea surface. The state of the sea level might be described by a time-mean long enough to exclude tidal influences (approximately 19 years). MSL is defined by its geodetic height (η) above the reference ellipsoid (negative if below) [30]. Epoch-based MSL at TGs (MSL) can be described using a linear expression technique defined as MSLTG(t) = MSLTG(t0) + (t − t0) MSLTG-trend, where the reference epoch is represented by t0 and MSLTG-trend, is the rate of sea level change in mm/year. However, MSL does not depict an equipotential surface because different height datums may refer to distinct equipotential surfaces, resulting in constant offsets between them.
- Mean dynamic ocean topography (MDT). MDT is defined as the difference between the averaged sea surface over time and the geoid, which is expressed as MDT(t) = η(t) − N(t) (see Figure 2). Every geoid slopes are ‘horizontal’. The strength of surface ‘geostrophic’ currents is measured by a tilt of the sea surface relative to the horizontal. The ‘steady-state’ circulation is observed by MDT from the long-term-averaged strength of ocean currents (https://gracejpl.nasa.gov, accessed on 3 August 2021).
- Vertical land motion (VLM). The level of the sea floor (SF) fluctuates over time owing to solid-Earth tides, accumulation of sediment, and VLM. VLM refers to either subsidence or uplift, and its sources include isostasy, elastic flexure of the lithosphere and plate tectonics in plate boundary zones. Some anthropogenic effects near coastal zones, such as extraction of groundwater and hydrocarbon, may cause subsidence and thus alter the coastline [30].
2.2. TGs Vertical Stability Analysis
2.2.1. ITRF2014 Geodetic Coordinates
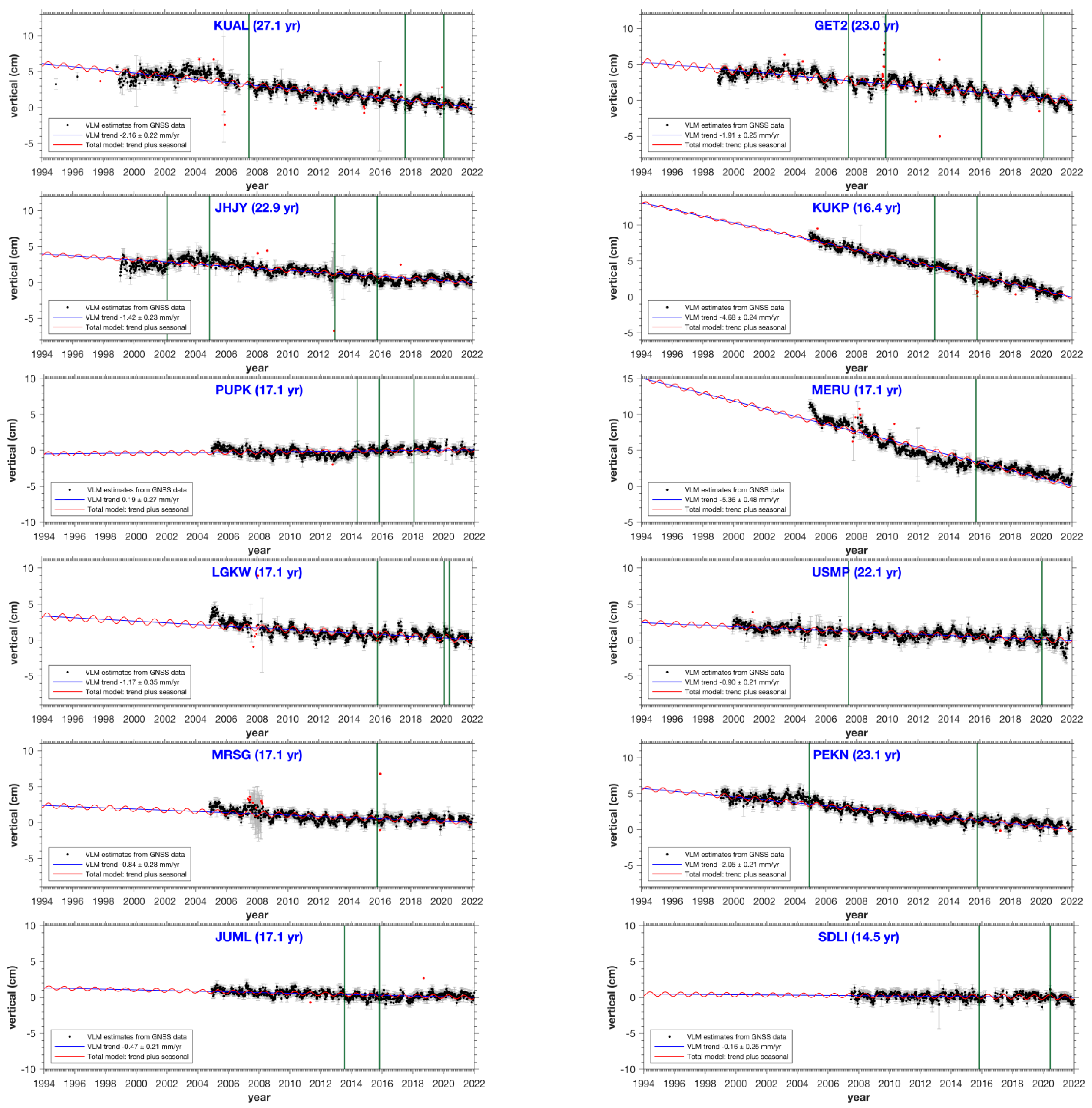
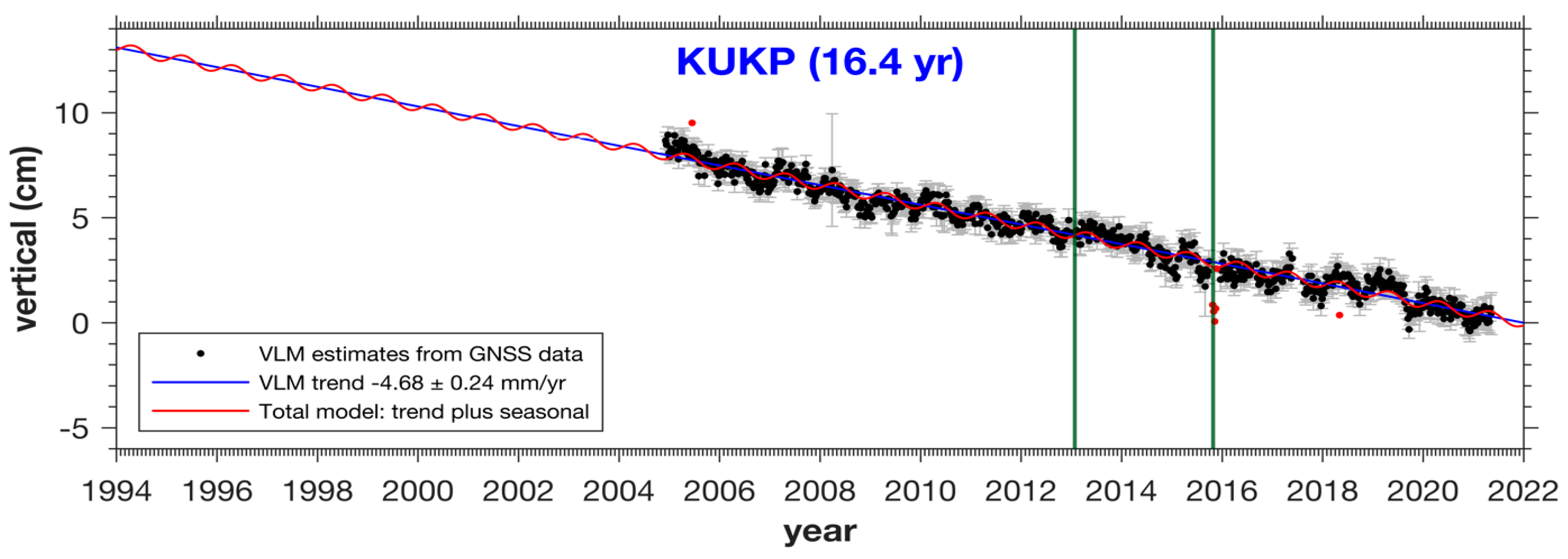
2.2.2. Vertical Land Motion (VLM)
2.3. MSL Determination and Trends Analysis
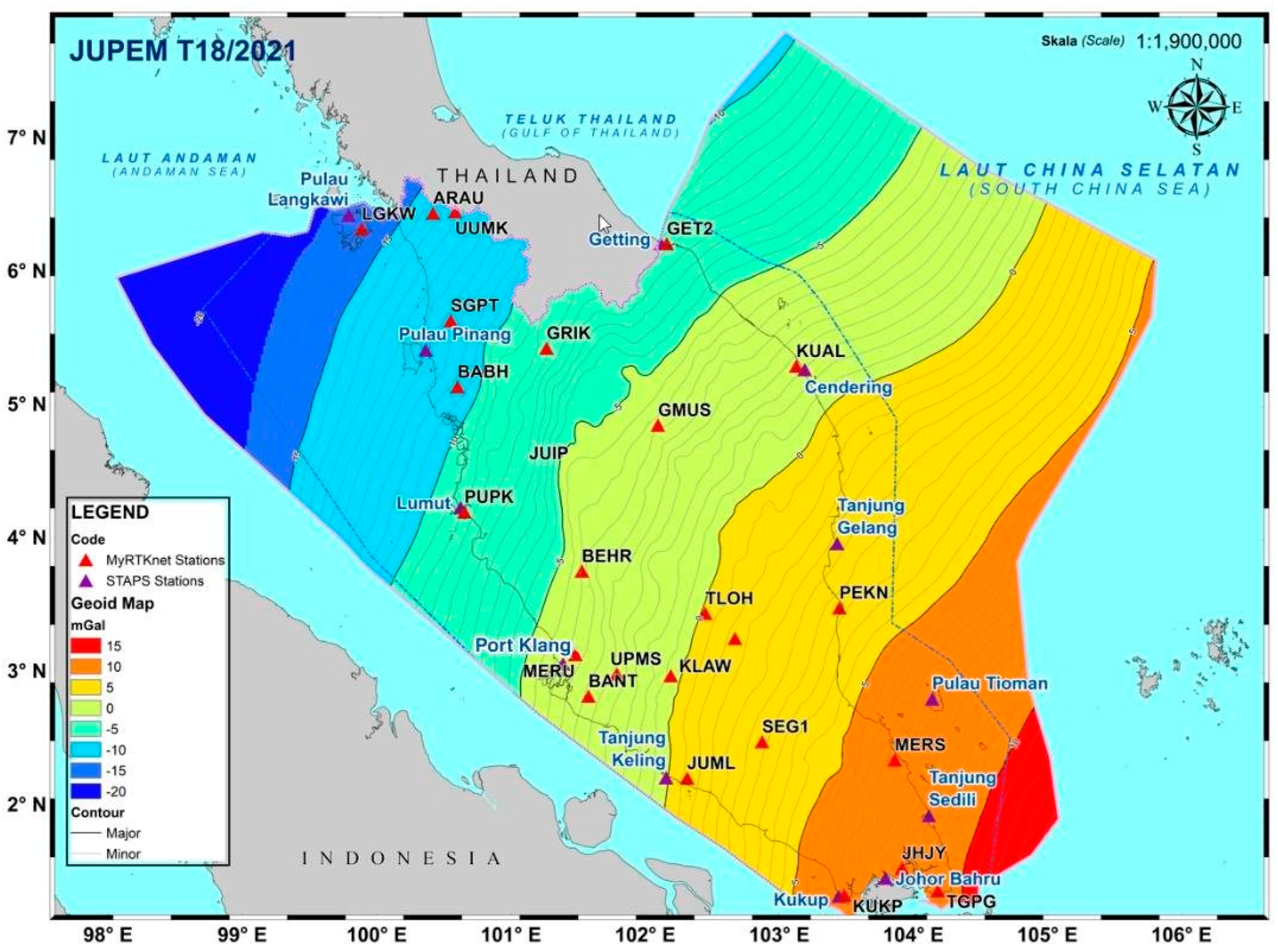
2.4. Geoid Mapping
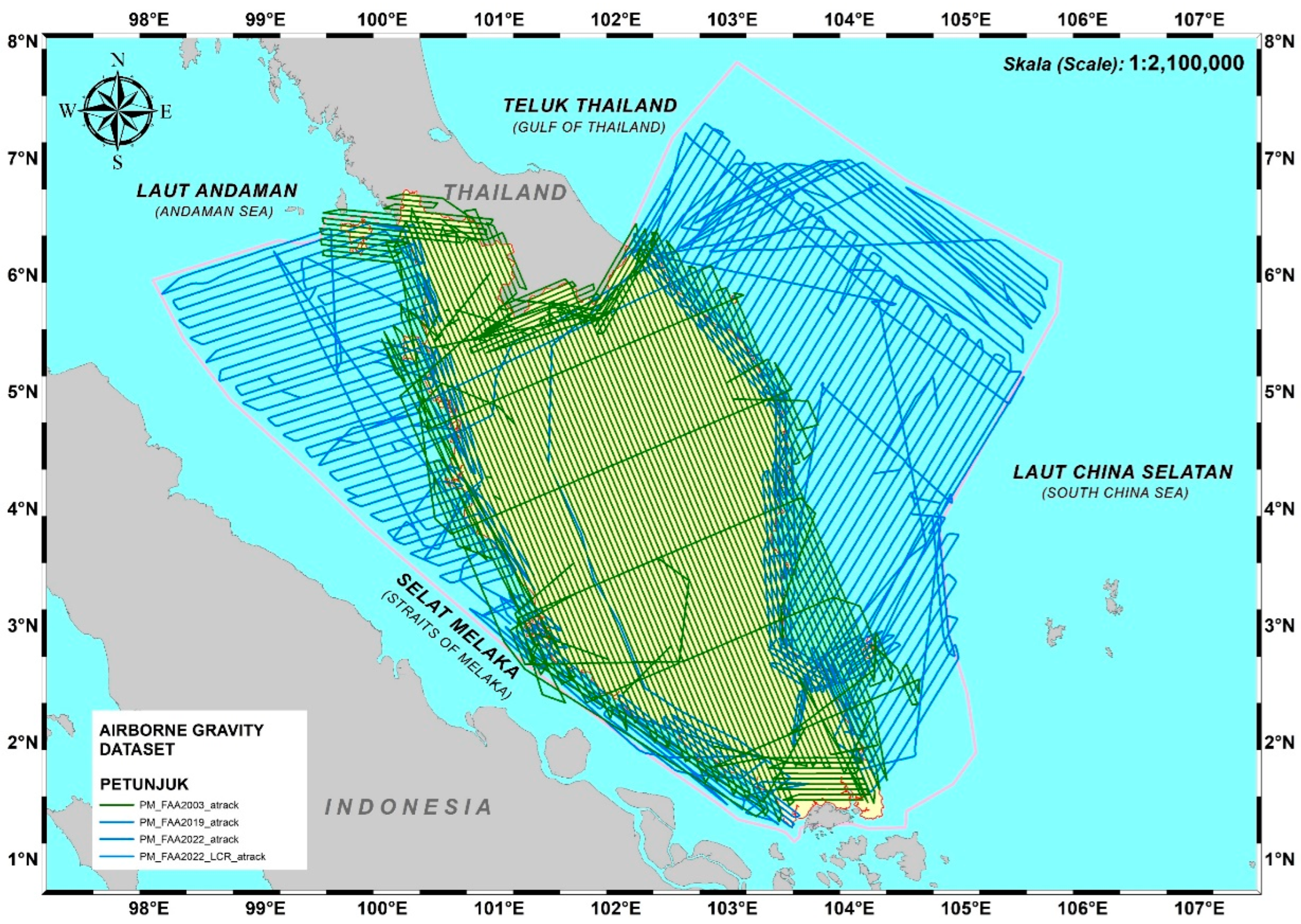
2.4.1. Data Sources
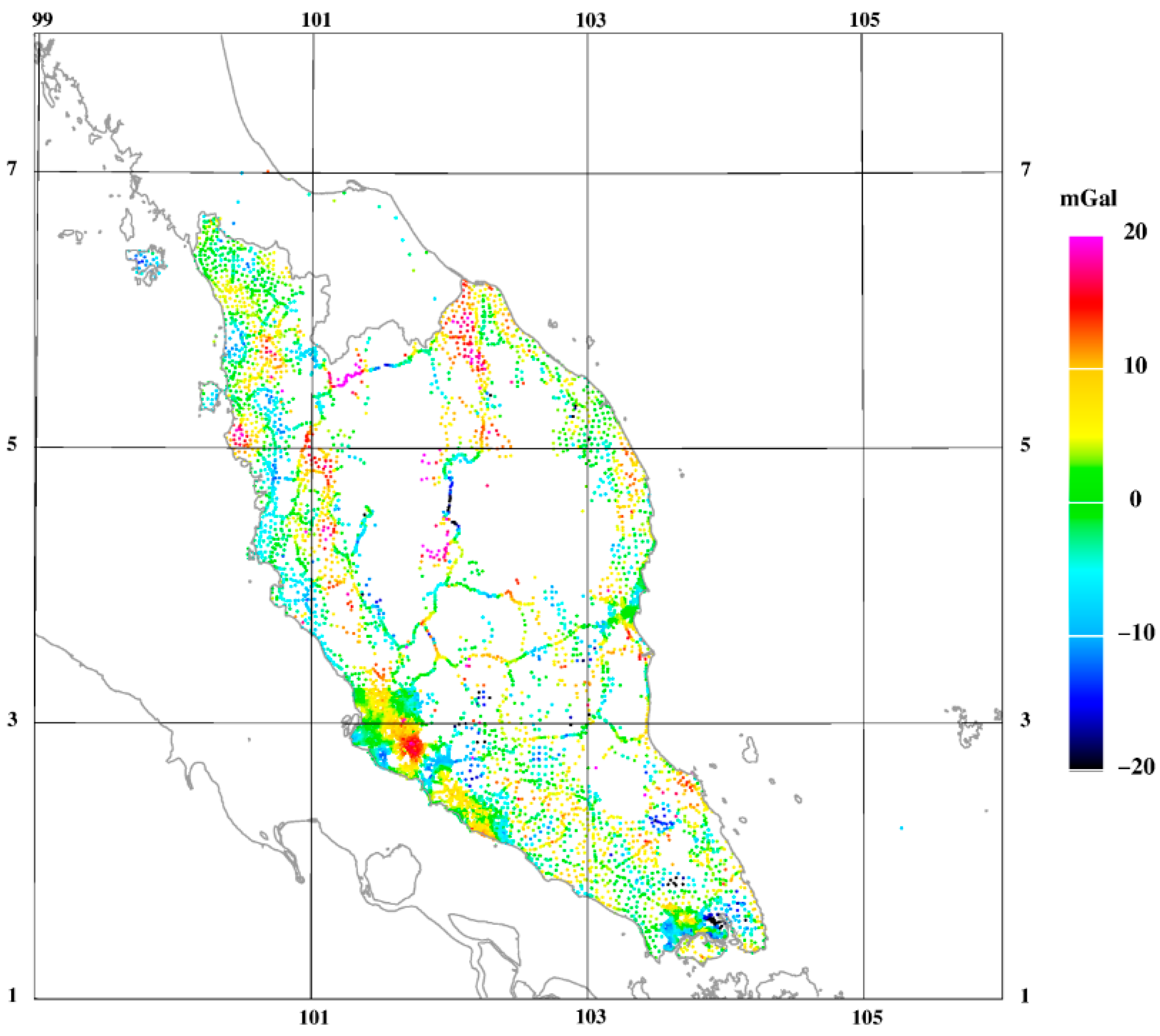
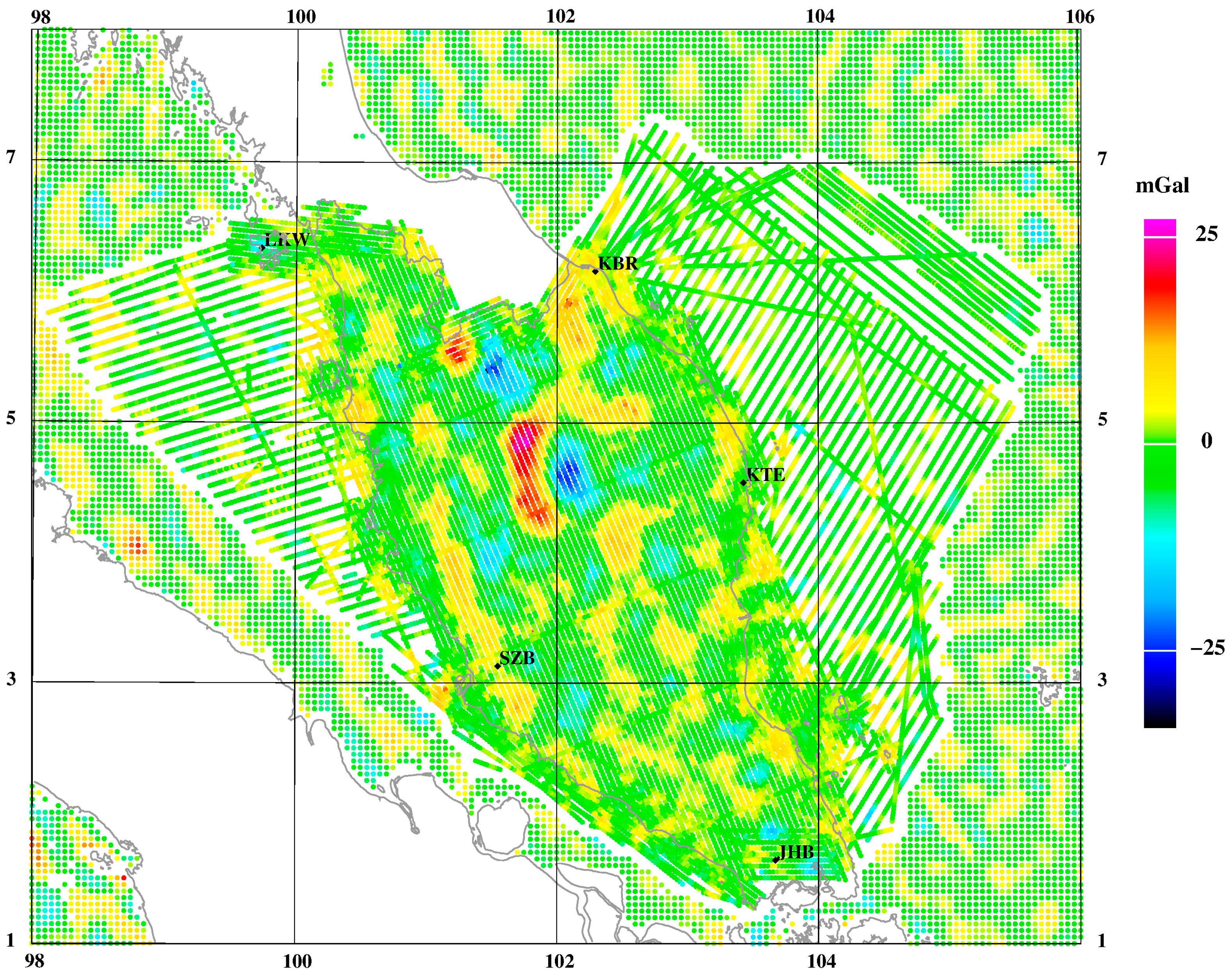
- 2019 and 2022 airborne gravity survey results (free-air anomalies at altitude).
- 2003 airborne gravity survey (5 km line spacing).
- Edited DSMM surface gravimetry data (as used in the 2019 geoid model).
- DSMM Height Modernization System (HMS) gravimetry data in the vicinity of Kuala Lumpur, Melaka, and Johor Bharu.
- Satellite altimetry gravity offshore was selected away from other data (DTU21).
2.4.2. Geoid Modelling Method
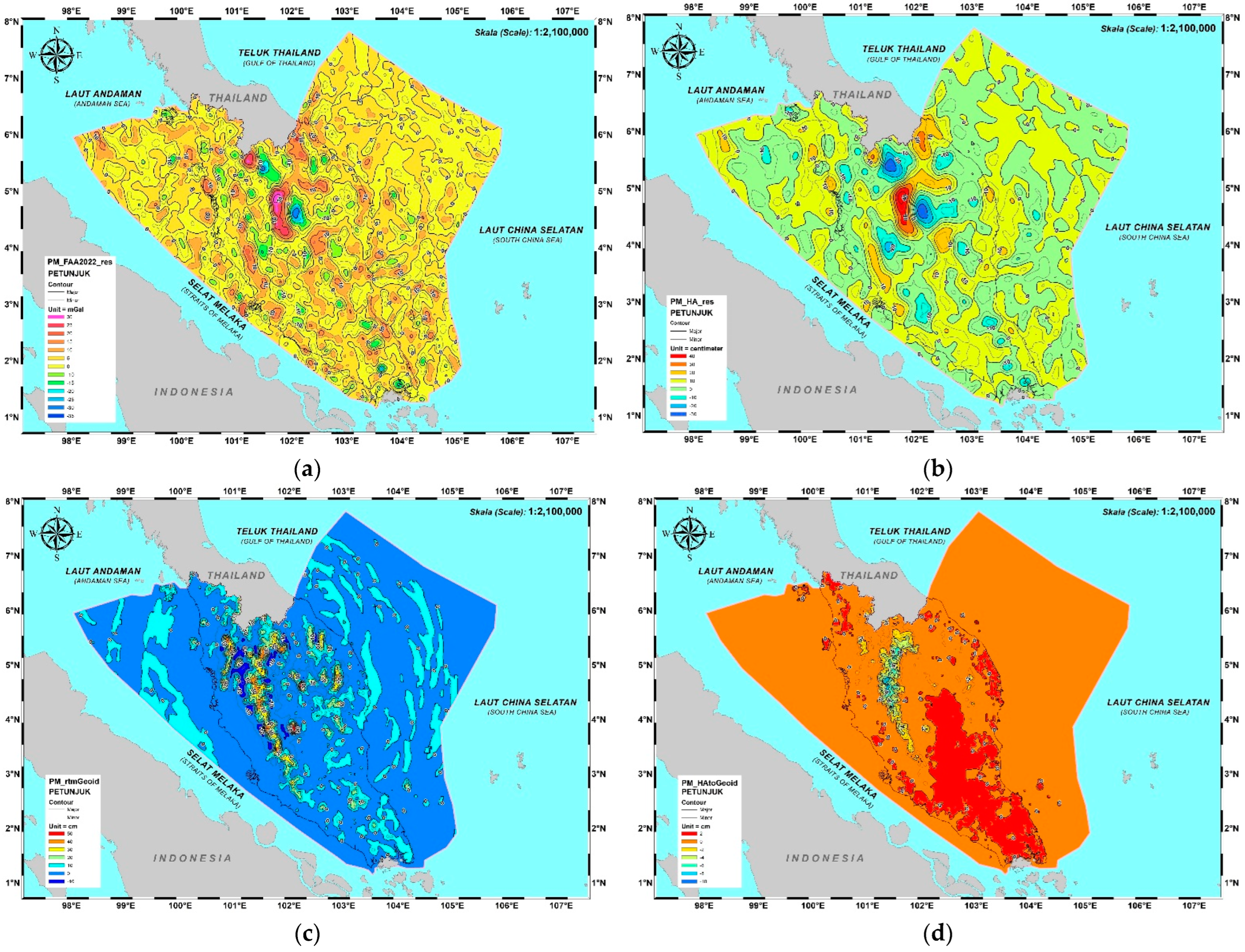
- Remove Step: The residual gravity anomaly field is calculated by subtracting the XGM2019e and RTM components from the total anomalies, resulting in Δgres = Δg − ΔgEGM − ΔgRTM. The free-air anomaly residual, Δgres, calculated here, remains in the gravity data after subtracting the residual terrain effect contributions ΔgRTM, and the global field ΔgEGM (Figure 12a).
- Compute Step: Spherical Fast Fourier Transform (FFT) with optimised kernels is utilised to determine the gravimetric geoid, a rather new variant to the classical geoid integral (Stokes’ integral), where a fitted weighting of the XGM2019e long wavelengths and the local gravity data shorter wavelengths is presented. The residual height anomaly (ζres) is computed mathematically by analysing convolution equations of the following form: ζres = Sref (Δφ, Δλ) × [Δgres (φ, λ)sinφ] = F−1 [F(Sref) F (Δgres sinφ)], where Sref, denotes a modified “Stokes” kernel and F denotes the operator of 2-dimensional Fourier Transform. The block-wise LSC using the planar logarithmic covariance model in 1° × 1° degree blocks with overlap has been employed to generate gridded data, taking into account the varied elevations of the airborne and surface gravimetry data. Forsberg et al. [50] or Forsberg et al. [51] and references therein provide more information on the geoid determination approach. Figure 12b illustrates the FFT method output, i.e., the gridded height anomalies.
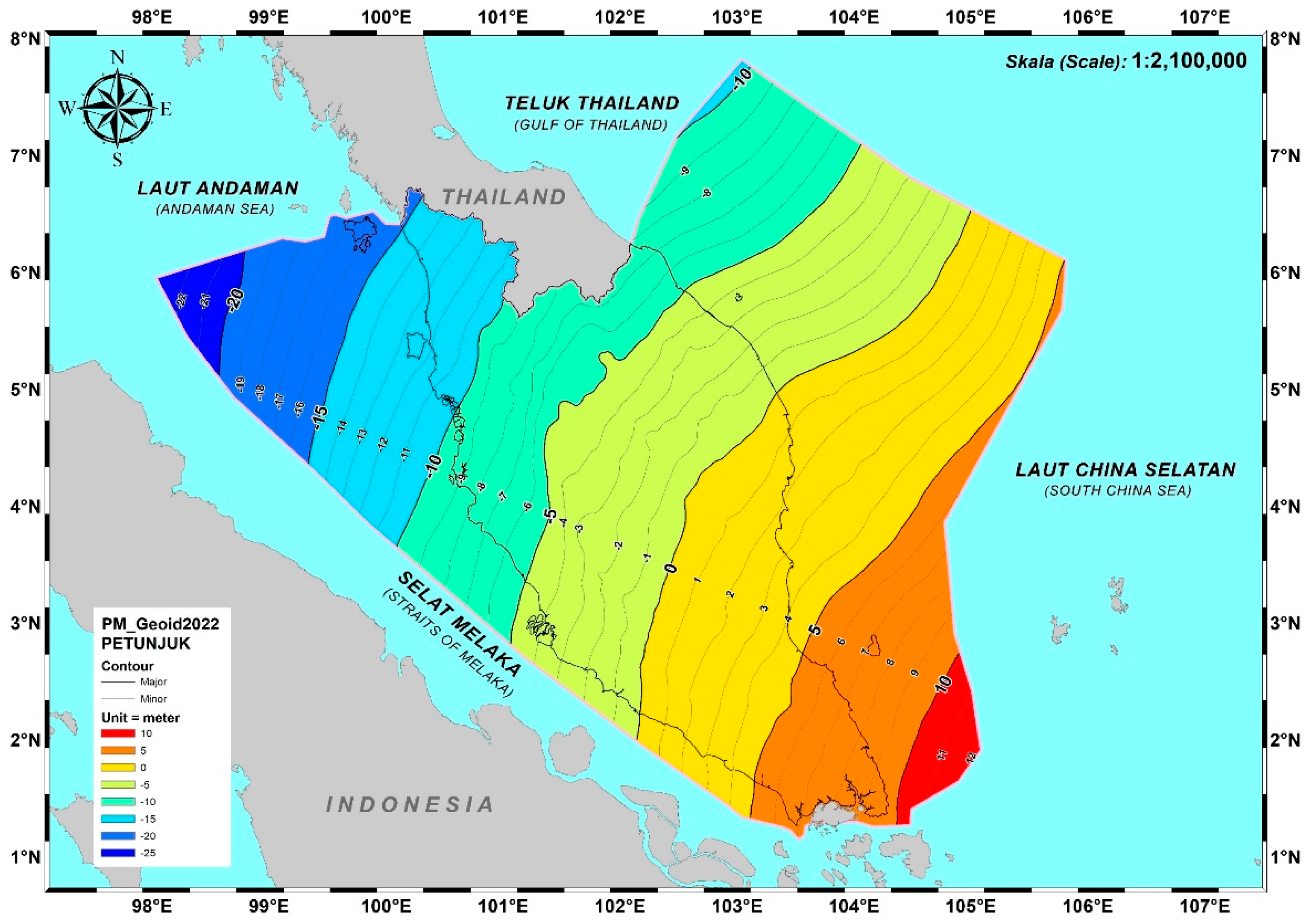
- Restore Step: The contributions from XGM2019e and RTM (Figure 12c) are added back following the computation of the residual height anomaly (ζres) from Δgres to obtain total height anomalies (quasi geoid) resulting in ζ = ζEGM + ζRTM + ζres. The relationship between geoid (N) and quasi-geoid (ζ) is expressed as N − ζ = (ΔgB/γ)H [52], where ΔgB is the Bouguer anomaly and H is the orthometric height. This is readily implemented as a minor correction (typically <10 cm) to a final gravimetric geoid estimated from surface data. H = 0 is often found in the marine environment, and it also indicates that the quasi-geoid coincides with the geoid (N = ζ). In Peninsular Malaysia, the term, N − ζ is relatively minor, reaching an extreme value of only–11 cm in the highest mountains (see Figure 12d). Figure 13 displays the final computed gravimetric geoid (PMGeoid2022).
3. Results
3.1. PMGeoid2022 Fitting to Local MSL
3.2. Fitting to a Single TG at Port Klang
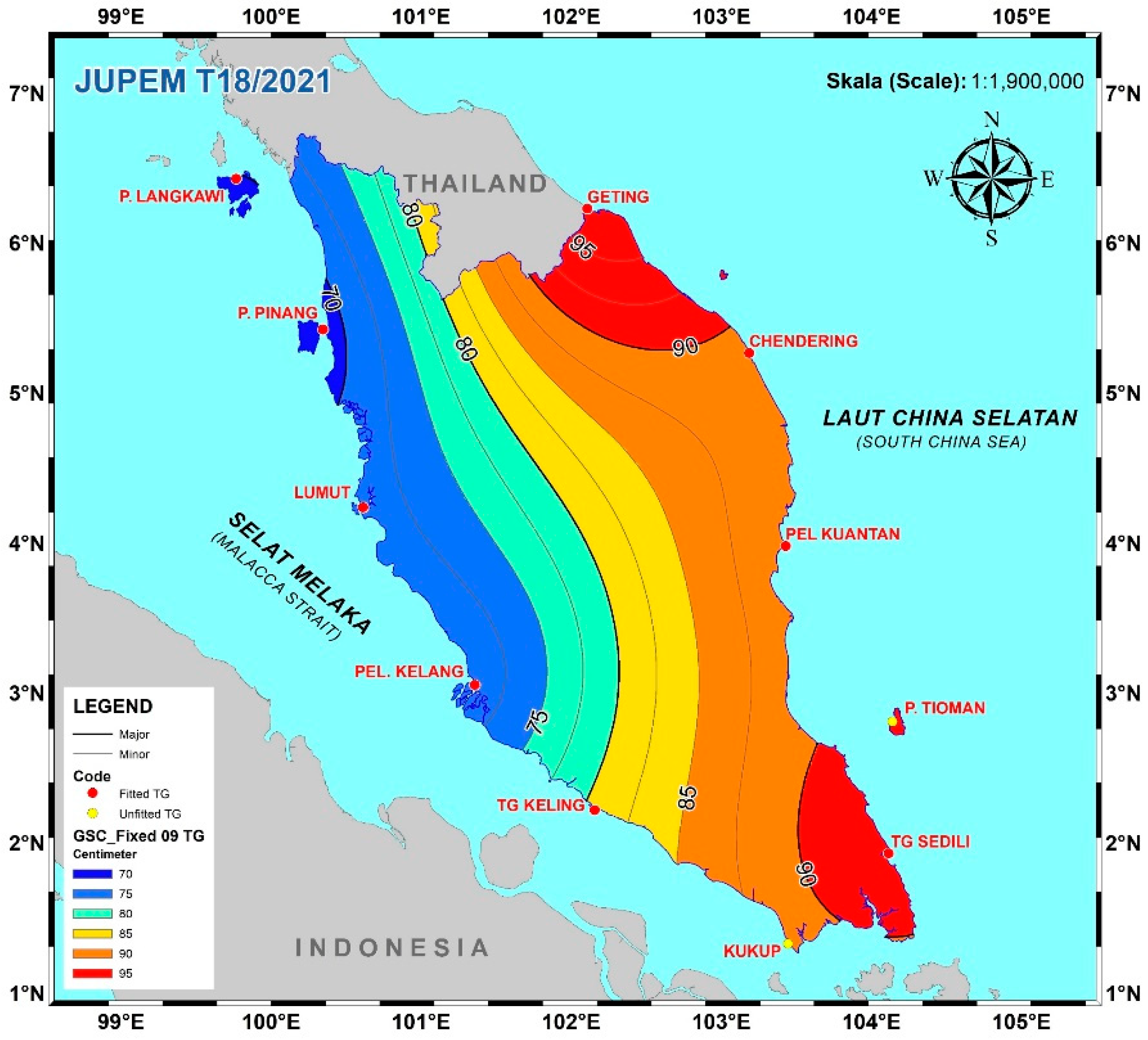
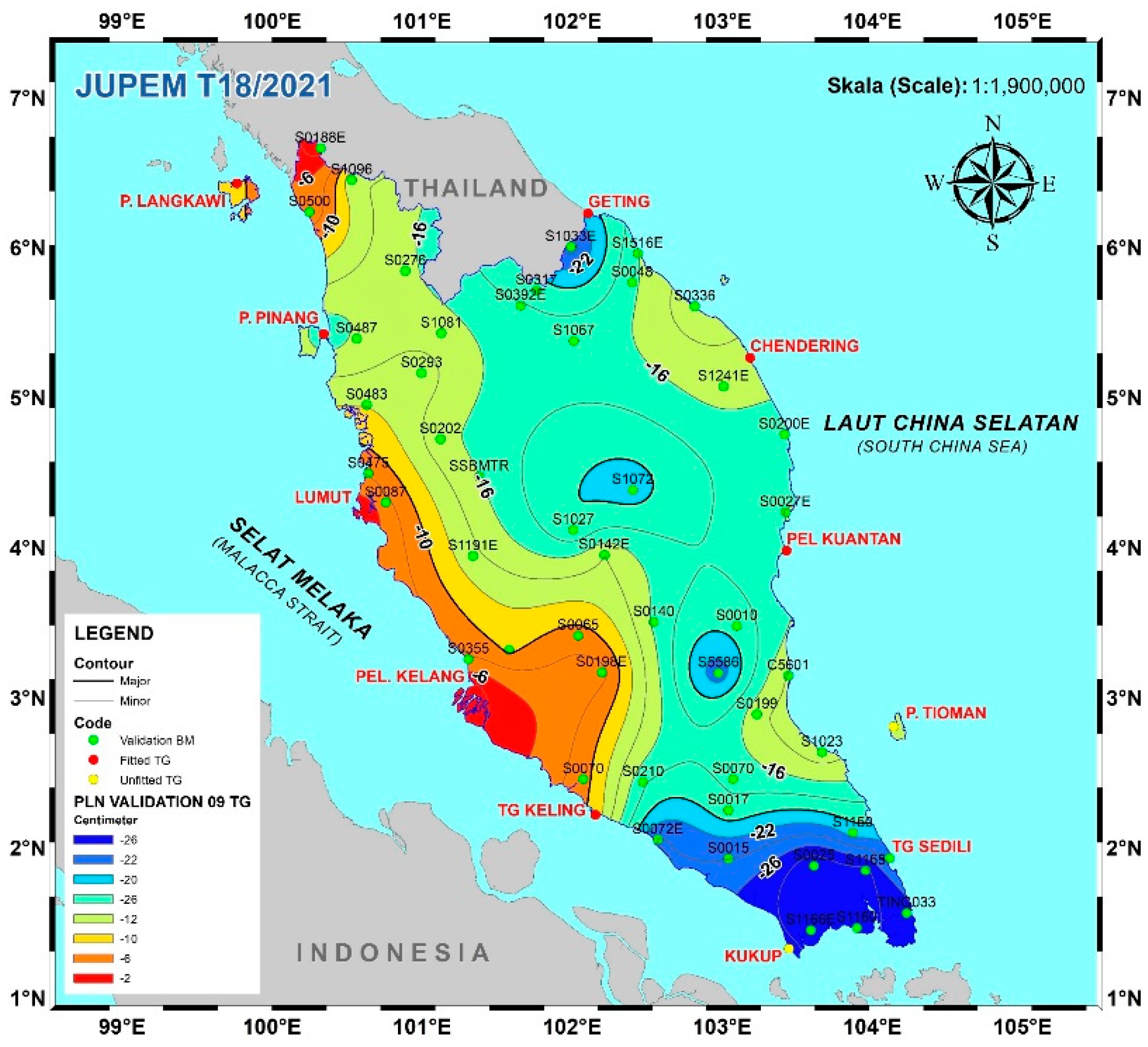
3.3. Fitting to Eleven TGs
4. Discussions
4.1. Peninsular Malaysia Geodetic Vertical Datum 2022: PMGVD2022
- Semi-kinematic RMSL Model: RMSL(t) = RMSL(t0) + (t − t0) RMSLtrend.
- Unified within the Land Mass and Definitive: A single recognised vertical datum is provided by PMGVD2022 so that benchmarks may be issued with a single definitive height.
- Good Coverage: Access to the PMGVD2022 datum is available everywhere in the nation, even in new development zones. The GNSS levelling technique will allow users to easily generate heights for points no matter where they are.
- Consistent with Gravimetric Geoid Model: PMGVD2022 is consistent with the fitted PMGeoid2022 model, with an additional local datum bias correction, NRMDT: H = hGNSS − Nfit_PTK − NRMDT.
- Zero Height Close to Sea Level: PMGVD2022, which is based on MSL at eleven (11) TG stations, provides epoch-based sea level that accounts for sea level rise and tilts along Peninsular Malaysia’s coast.
- Applicable to Continental Shelf and Consistent with International Standards: PMGVD2022, together with the 3D geocentric datum of GDM2020/ITRF2014 [21], support the development of the Marine Geodetic Network (MGN) and other marine-related positioning in the Continental Shelf of Peninsular Malaysia.
4.2. Transformation from PMGVD2000 to PMGVD2022
5. Conclusions
- TGs MSL@2022.0—which consists of a network of eleven (11) TG Benchmarks (TGBM) with their height measured above RMSL derived from more than 30 years tidal observation. Time-dependent RMSL at epoch t can be expressed as RMSL(t) = RMSL(t0) + (t − t0) RMSLtrend, where t0 is the reference epoch, RMSLtrend is the relative sea level change in mm/year. VLM has also been calculated at the TG locations to convert relative RMSLtrend to geocentric GMSLtrend.
- Geodetic Relative Mean Dynamic Ocean Topography (RMDTG)—which consists of the RMDTG values at ten (10) TG stations along the Peninsular Malaysia coast relative to the TG at PTK (RMDTG = HPMGeoid2022_fit_PTK – HMSL2022B).
- Fitted Gravimetric Geoid Surface—which consists of a new MSL-geoid PMGeoid2022-fit-PTK and is referred to as a seamless height reference surface for the land and marine area of Peninsular Malaysia. The new reference height surface has been fitted with respect to the local MSL at PTK.
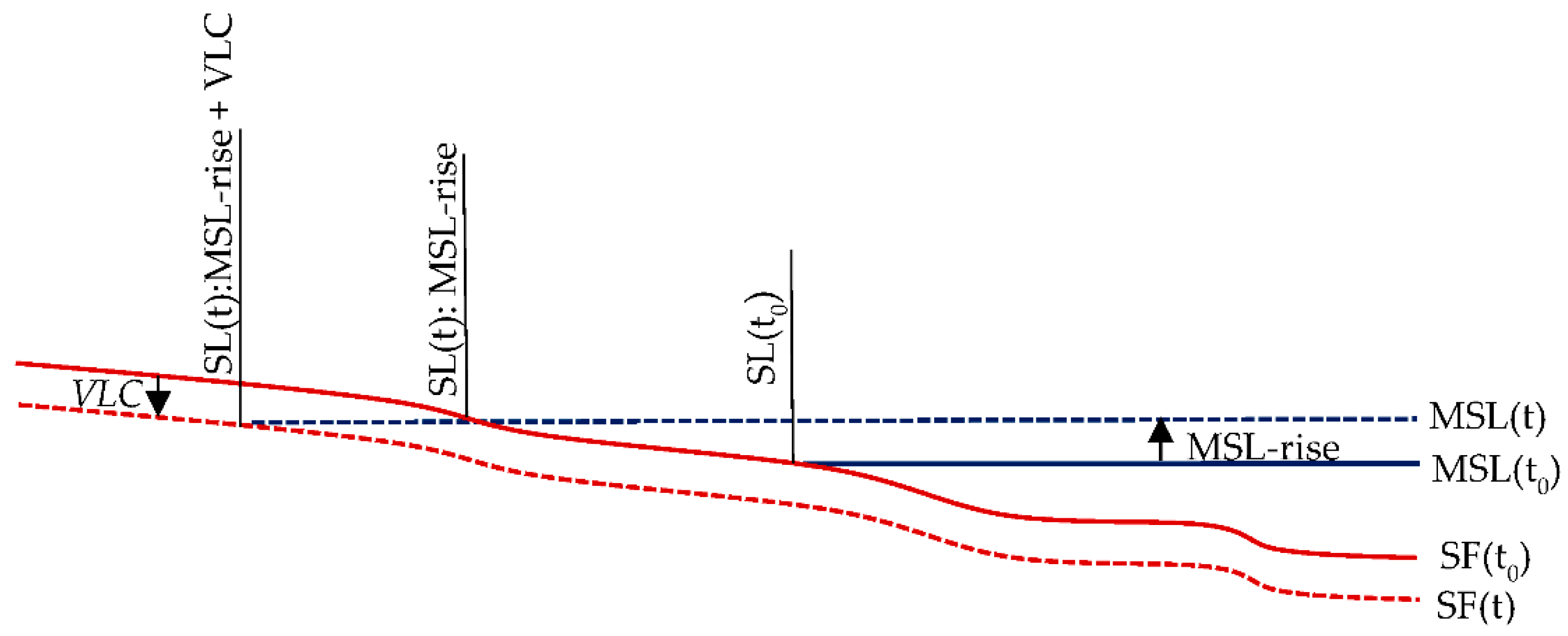
- Precise Levelling Network—which consists of a network SBMs and BMs from existing PLN of Peninsular Malaysia and then transformed to PMGVD2022. It is worth noting that any re-levelling and maintenance of the existing PLN-BM will be expensive and require more human labour. Orthometric heights in the PMGVD2022 system can be expressed as a semi-kinematic model of H(t) = H(t0) − (t − t0) MSLtrend + δVLC(t), which takes into account the sea level rise and vertical land changes (subsidence or uplift) at epoch t.
- TG stations in Peninsular Malaysia should be appropriately maintained for continuous and up-to-date sea level data, which is critical for the analysis of the stability of the vertical datum and the impact of sea level rise. TG data is a valuable source of information for a wide variety of activities over a wide variety of time scales, including scientific research as well as for a variety of industrial applications [31]. The TGBM is also incredibly essential since it serves as the reference point for sea level data. It may be required to redefine the TGBM and TG-GPSBM to account for variations in orthometric and ellipsoidal heights caused by VLMs.
- Sea level rise influenced by global warming is reinforced by (negative) vertical land motion, and their impacts on coastal zone should be seriously considered (see Figure 18). Regarding its impact on shoreline changes, land subsidence has a shorter time span and more measurable magnitude compared to the influences of the tectonic setting [63]. Therefore, VLM detection and monitoring using GNSS observations co-located at all TGs must be performed religiously. GNSS reflection methods should be thoroughly researched as an alternative method for simultaneously monitoring sea level and VLM [64]. The integration of GNSS and InSAR techniques should also be considered to map VLM along the coasts.
- The existing PLN may be replaced by Malaysia Real-Time Kinematic GNSS Network (MyRTKnet) for heighting purposes. MyRTKnet stations should be used as reference stations for local height updates or densification either by traditional methods or by GPS levelling. PMGVD2022 geoid model and GPS levelling have been successfully used in detecting systematic errors in PMGVD2000 due to sea slopes, MSL rise, land subsidence and levelling error propagation. The future of levelling will be a mixture of traditional levelling and GNSS. Local precise survey work will continue to be performed using traditional methods, but less accurate survey work will be handled by GPS/GNSS levelling.
- Future GPS/GNSS campaigns on TGBMs will have a minimum duration of three days (24-h sessions). To ensure that the same satellite visibility and multipath environment are experienced during the re-occupations, the same antenna setup (type and height) must be maintained. Minimal obstruction for the satellite visibility by the antenna shall be maintained.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| DSMM | Department of Survey and Mapping Malaysia |
| DTU | Technical University of Denmark |
| FOLN67 | First Order Levelling Network 1967 |
| GDM | Geocentric Datum for Malaysia |
| GMSL | Geocentric Mean Sea Level |
| GNSS | Global Navigation Satellite System |
| GPS | Global Positioning System |
| GPSBM | GPS benchmark |
| GRS80 | Geodetic Reference System 1980 |
| GVD | Global Vertical Datum |
| Hz | Hertz |
| IGS | International GNSS Service |
| IHRS | International Height Reference System |
| IMU | Inertial Measurement Unit |
| ITRF2014 | International Terrestrial Reference Frame 2014 |
| KMS | Kort & Matrikelstyrelsen, a Danish geodata agency. |
| LC&R | LaCoste and Romberg |
| LE | Levelling Errors |
| LSD12 | Land Survey Datum 1912 |
| LVD | Local vertical datum |
| MASS | Malaysian Active GPS System |
| MDT | Mean Dynamic Ocean Topography |
| MDTG | Geodetic MDT |
| mGal | milligal |
| MGN | Marine Geodetic Network |
| MGRF2020 | Malaysian Geodetic Reference Frame 2020 |
| MSL | Mean Sea Level |
| Mw | Magnitude |
| MyGeoid | Malaysian Gravimetric Geoid |
| MyMarineGI | Marine Geodetic Infrastructures in Malaysian Waters |
| MyRTKNet | Malaysia Real-Time Kinematic GNSS Network |
| NM | Nautical Miles |
| PLN | Precise Levelling Network |
| PMGVD | Peninsular Malaysia Geodetic Vertical Datum |
| PSMSL | Permanent Service for Mean Sea Level |
| PTK | Port Klang |
| RCR | Remove-Compute-Restore technique |
| RMDT | Relative Mean Dynamic Ocean Topography |
| RMDTG | Geodetic Relative Mean Dynamic Ocean Topography |
| RMS | Root Mean Square |
| RMSL | Relative Mean Sea Level |
| RMSLrise | Relative Mean Sea Level Rise |
| RTM | Residual Terrain Modelling |
| SALT | Satellite Altimeter |
| SBM | Standard Benchmarks |
| SF | Seafloor |
| SL | Shoreline |
| TG | Tide Gauge |
| TGBM | Tide Gauge Benchmark |
| TG-GPSBM | GPSBM at tide gauge locations |
| TGZ | Tide Gauge Zero |
| TON | Tidal Observation Network |
| TUD | Technical University Darmstadt, Germany |
| VLM | Vertical Land Motion |
| WGS84 | World Geodetic System 1984 |
| WRMS | Weighted Root Mean Square |
References
- Janssen, V.; McElroy, S. The Australian Height Datum Turns 50: Past, Present & Future. In APAS Webinar Series 2021 (AWS2021); APAS: Bathurst, Australia, 2021; pp. 3–27. [Google Scholar]
- Jamil, H. GNSS Heighting and Its Potential Use in Malaysia. In GNSS Processing and Analysis; GNSS: Marrakech, Morocco, 2011; pp. 1–19. [Google Scholar]
- Mohamed, A. An Investigation of the Vertical Control Network of Peninsular Malaysia Using a Combination of Levelling, Gravity, GPS and Tidal Data. Ph.D. Thesis, Universiti Teknologi Malaysia, Johor, Malaysia, 2003. [Google Scholar]
- Kadir, M.; Ses, S.; Hisam, A.; Abu, S. Current and Future Geodetic Activities in Malaysia; GNSS: Hong Kong, China, 2022; 19p. [Google Scholar]
- Matsuo, K.; Kuroishi, Y. Refinement of a Gravimetric Geoid Model for Japan Using GOCE and an Updated Regional Gravity Field Model. Earth Planets Space 2020, 72, 33. [Google Scholar] [CrossRef]
- Hwang, C.; Hsu, H.-J.; Featherstone, W.E.; Cheng, C.-C.; Yang, M.; Huang, W.; Wang, C.-Y.; Huang, J.-F.; Chen, K.-H.; Huang, C.-H.; et al. New Gravimetric-Only and Hybrid Geoid Models of Taiwan for Height Modernisation, Cross-Island Datum Connection and Airborne LiDAR Mapping. J. Geod. 2020, 94, 83. [Google Scholar] [CrossRef]
- Véronneau, M.; Duval, R.; Huang, J. A Gravimetric Geoid Model as a Vertical Datum in Canada. Geomatica 2006, 60, 165–172. [Google Scholar]
- Véronneau, M. The Canadian Geodetic Vertical Datum of 2013 (CGVD2013). Geomatica 2014, 60, 9–19. [Google Scholar] [CrossRef]
- Amos, M. Improving New Zealand’s Geoid Based Datum with Airborne Gravimetry. In Proceedings of the FIG Working Week 2016, Christchurch, New Zealand, 2–6 May 2016. [Google Scholar]
- Smith, D. The GRAV-D Project: Gravity for the Redefinition of the American Vertical Datum; National Oceanic and Atmosphoric Administration (NOAA)/National Geodetic Survey: Silver Spring, MD, USA, 2007; 40p. [Google Scholar]
- DSMM. Airborne Gravity Survey and Geoid Determination Project for Peninsular Malaysia, Sabah and Sarawak; Internal Report; Department of Survey and Mapping Malaysia, Geodetic Division: Kuala Lumpur, Malaysia, 2003. [Google Scholar]
- Brown, N.J.; McCubbine, J.C.; Featherstone, W.E.; Gowans, N.; Woods, A.; Baran, I. AUSGeoid2020 Combined Gravimetric–Geometric Model: Location-Specific Uncertainties and Baseline-Length-Dependent Error Decorrelation. J. Geod. 2018, 92, 1457–1465. [Google Scholar] [CrossRef]
- Ses, S.; Mohamed, A. The Second Precise Levelling Network of Peninsular Malaysia. Surv. Rev. 2009, 41, 384–394. [Google Scholar] [CrossRef]
- Hamid, A.I.A.; Din, A.H.M.; Hwang, C.; Khalid, N.; Tugi, A.; Omar, K. Contemporary Sea Level Rise Rates around Malaysia: Altimeter Data Optimization for Assessing Coastal Impact. J. Asian Earth Sci. 2018, 166, 247–259. [Google Scholar] [CrossRef]
- Sweet, W.V.; Hamlington, B.D.; Kopp, R.E.; Weaver, C.P.; Barnard, P.L.; Bekaert, D.; Brooks, W.; Craghan, M.; Dusek, G.; Frederikse, T.; et al. Global and Regional Sea Level Rise Scenarios for the United States: Updated Mean Projections and Extreme Water Level Probabilities Along U.S. Coastlines; NOAA Technical Report NOS 01; National Ocean Service: National Oceanic and Atmospheric Administration: Silver Spring, MD, USA, 2022; 111p. [Google Scholar]
- Woodworth, P.L.; Melet, A.; Marcos, M.; Ray, R.D.; Wöppelmann, G.; Sasaki, Y.N.; Cirano, M.; Hibbert, A.; Huthnance, J.M.; Monserrat, S.; et al. Forcing Factors Affecting Sea Level Changes at the Coast. Surv. Geophys. 2019, 40, 1351–1397. [Google Scholar] [CrossRef]
- Ehsan, S.; Ara Begum, R.; Ghani Md Nor, N.; Nizam Abdul Maulud, K. Current and Potential Impacts of Sea Level Rise in the Coastal Areas of Malaysia. IOP Conf. Ser. Earth Environ. Sci. 2019, 228, 012023. [Google Scholar] [CrossRef]
- NAHRIM. Impact of Climate Change to Sea Level Rise in Malaysia; NAHRIM: Seri Kembangan, Malaysia, 2019. [Google Scholar]
- Gesch, D.B. Best Practices for Elevation-Based Assessments of Sea-Level Rise and Coastal Flooding Exposure. Front. Earth Sci. 2018, 6, 230. [Google Scholar] [CrossRef]
- Durand, G.; van den Broeke, M.R.; Le Cozannet, G.; Edwards, T.L.; Holland, P.R.; Jourdain, N.C.; Marzeion, B.; Mottram, R.; Nicholls, R.J.; Pattyn, F.; et al. Sea-Level Rise: From Global Perspectives to Local Services. Front. Mar. Sci. 2022, 8, 709595. [Google Scholar] [CrossRef]
- Azhari, M.; Altamimi, Z.; Azman, G.; Kadir, M.; Simons, W.J.F.; Sohaime, R.; Yunus, M.Y.; Irwan, M.J.; Asyran, C.A.; Soeb, N.; et al. Semi-Kinematic Geodetic Reference Frame Based on the ITRF2014 for Malaysia. J. Geod. Sci. 2020, 10, 91–109. [Google Scholar] [CrossRef]
- Vaníček, P. Vertical Datum and the NAVD 88. Surv. Land Inf. Syst. 1990, 51, 83–86. [Google Scholar]
- Sjöberg, L. On the Definition and Realization of a Global Vertical Datum. J. Geod. Sci. 2011, 1, 154–157. [Google Scholar] [CrossRef]
- Hofmann-Wellenhof, B.; Moritz, H. Physical Geodesy, 2nd ed.; corrected ed.; Springer: Wien, Austria; New York, NY, USA, 2006. [Google Scholar]
- Barzaghi, R.; De Gaetani, C.I.; Betti, B. The Worldwide Physical Height Datum Project. Rend. Fis. Acc. Lincei 2020, 31 (Suppl. S1), 27–34. [Google Scholar] [CrossRef]
- Sánchez, L.; Sideris, M.G. Vertical Datum Unification for the International Height Reference System (IHRS). Geophys. J. Int. 2017, 209, 570–586. [Google Scholar] [CrossRef]
- Jekeli, C. Heights, the Geopotential, and Vertical Datums; Ohio Sea Grant Development Program, NOAA; Technical Report 459; Ohio State University, Department of Civil and Environmental Engineering and Geodetic Science: Columbus, OH, USA, 2000; pp. 1–34. [Google Scholar]
- Jekeli, C.; Montenbruck, O. Time and Reference Systems. In Springer Handbook of Global Navigation Satellite Systems; Springer: Cham, Switzerland, 2017; pp. 25–28. [Google Scholar]
- Godah, W.; Szelachowska, M.; Krynski, J. On the Analysis of Temporal Geoid Height Variations Obtained from GRACE-Based GGMs over the Area of Poland. Acta Geophys. 2017, 65, 713–725. [Google Scholar] [CrossRef]
- Gregory, J.M.; Griffies, S.M.; Hughes, C.W.; Lowe, J.A.; Church, J.A.; Fukimori, I.; Gomez, N.; Kopp, R.E.; Landerer, F.; Cozannet, G.L.; et al. Concepts and Terminology for Sea Level: Mean, Variability and Change, Both Local and Global. Surv. Geophys. 2019, 40, 1251–1289. [Google Scholar] [CrossRef]
- Wöppelmann, G.; Zerbini, S.; Marcos, M. TGs and Geodesy: A Secular Synergy Illustrated by Three Present-Day Case Studies. Comptes Rendus Geosci. 2006, 338, 980–991. [Google Scholar] [CrossRef]
- Marcos, M.; Wöppelmann, G.; Matthews, A.; Ponte, R.M.; Birol, F.; Ardhuin, F.; Coco, G.; Santamaría-Gómez, A.; Ballu, V.; Testut, L.; et al. Coastal Sea Level and Related Fields from Existing Observing Systems. Surv. Geophys. 2019, 40, 1293–1317. [Google Scholar] [CrossRef]
- Vignudelli, S.; Birol, F.; Benveniste, J.; Fu, L.-L.; Picot, N.; Raynal, M.; Roinard, H. Satellite Altimetry Measurements of Sea Level in the Coastal Zone. Surv. Geophys. 2019, 40, 1319–1349. [Google Scholar] [CrossRef]
- Jet Propulsion Laboratory. GNSS-Inferred Positioning System and Orbit Analysis Simulation Software (GIPSY-OASIS). Available online: https://gipsy-oasis.jpl.nasa.gov (accessed on 1 November 2019).
- Rebischung, P.; Schmid, R. IGS14/Igs14.Atx: A New Framework for the IGS Products. In Proceedings of the AGU Fall Meeting 2016, San Francisco, CA, USA, 12–16 December 2016. [Google Scholar]
- Altamimi, Z.; Rebischung, P.; Métivier, L.; Collilieux, X. ITRF2014: A New Release of the International Terrestrial Reference Frame Modeling Nonlinear Station Motions: ITRF2014. J. Geophys. Res. Solid Earth 2016, 121, 6109–6131. [Google Scholar] [CrossRef]
- Zumberge, J.F.; Heflin, M.B.; Jefferson, D.C.; Watkins, M.M.; Webb, F.H. Precise Point Positioning for the Efficient and Robust Analysis of GPS Data from Large Networks. J. Geophys. Res. 1997, 102, 5005–5017. [Google Scholar] [CrossRef]
- Simons, W.J.F.; Naeije, M.C.; Brown, B.E.; Niemnil, S.; Pradit, S.; Thongtham, N.; Mustafar, M.A.; Towatana, P.; Darnsawasdi, R.; Yucharoen, M.; et al. Vertical Motion of Phuket Island (1994–2018) Due to the Sumatra-Andaman Mega-Thrust Earthquake Cycle: Impact on Sea-Level and Consequences for Coral Reefs. Mar. Geol. 2019, 414, 92–102. [Google Scholar] [CrossRef]
- Janssen, V.; McElroy, S. A Practical Guide to AUSPOS. In Proceedings of the 25th Association of Public Authority Surveyors Conference (APAS2022), Leura, Australia, 21–23 March 2022. [Google Scholar]
- Simons, W.J.F.; Ambrosius, B.A.C.; Noomen, R.; Angermann, D.; Wilson, P.; Becker, M.; Reinhart, E.; Walpersdorf, A.; Vigny, C. Observing Plate Motions in S.E. Asia: Geodetic Results of the GEODYSSEA Project. Geophys. Res. Lett. 1999, 26, 2081–2084. [Google Scholar] [CrossRef]
- Blewitt, G.; Lavallée, D. Effect of Annual Signals on Geodetic Velocity. J. Geophys. Res. 2002, 107, ETG 9-1–ETG 9-11. [Google Scholar] [CrossRef]
- Simons, W.J.F.; Socquet, A.; Vigny, C.; Ambrosius, B.A.C.; Haji Abu, S.; Promthong, C.; Subarya, C.; Sarsito, D.A.; Matheussen, S.; Morgan, P.; et al. A Decade of GPS in Southeast Asia: Resolving Sundaland Motion and Boundaries. J. Geophys. Res. 2007, 112, B06420. [Google Scholar] [CrossRef]
- Jamil, H.; Kadir, M.; Forsberg, R.; Olesen, A.; Isa, M.N.; Rasidi, S.; Mohamed, A.; Chihat, Z.; Nielsen, E.; Majid, F.; et al. Airborne Geoid Mapping of Land and Sea Areas of East Malaysia. J. Geod. Sci. 2017, 7, 84–93. [Google Scholar] [CrossRef]
- Jensen, T.E.; Olesen, A.V.; Forsberg, R.; Olsson, P.-A.; Josefsson, Ö. New Results from Strapdown Airborne Gravimetry Using Temperature Stabilisation. Remote Sens. 2019, 11, 2682. [Google Scholar] [CrossRef]
- Johann, F.; Becker, D.; Becker, M.; Ince, E.S. Multi-Scenario Evaluation of the Direct Method in Strapdown Airborne and Shipborne Gravimetry. In International Association of Geodesy Symposia; Springer: Berlin/Heidelberg, Germany, 2020. [Google Scholar] [CrossRef]
- Forsberg, R. A New Covariance Model for Inertial Gravimetry and Gradiometry. J. Geophys. Res. Solid Earth 1987, 92, 1305–1310. [Google Scholar] [CrossRef]
- Forsberg, R. Local Covariance Functions and Density Distributions; Scientific Report No. 6; The Ohio State University: Columbus, OH, USA, 1984. [Google Scholar]
- Zingerle, P.; Pail, R.; Gruber, T.; Oikonomidou, X. The Combined Global Gravity Field Model XGM2019e. J. Geod. 2020, 94, 66. [Google Scholar] [CrossRef]
- Forsberg, R.; Ses, S.; Alshamsi, A.; Din, A.H. Coastal Geoid Improvement Using Airborne Gravimetric Data in the United Arab Emirates. Int. J. Phys. Sci. 2012, 7, 6012–6023. [Google Scholar] [CrossRef]
- Forsberg, R.; Olesen, A.; Bastos, L.; Gidskehaug, A.; Meyer, U.; Timmen, L. Airborne Geoid Determination. Earth Planet Space 2000, 52, 863–866. [Google Scholar] [CrossRef]
- Forsberg, R.; Olesen, A.V. Airborne Gravity Field Determination. In Sciences of Geodesy—I; Xu, G., Ed.; Springer: Berlin/Heidelberg, Germany, 2010; pp. 83–104. [Google Scholar] [CrossRef]
- Tziavos, I.N.; Sideris, M.G. Topographic Reductions in Gravity and Geoid Modeling. In Geoid Determination; Sansò, F., Sideris, M.G., Eds.; Lecture Notes in Earth System Sciences; Springer: Berlin/Heidelberg, Germany, 2013; Volume 110, pp. 337–400. [Google Scholar]
- Forsberg, R.; Tscherning, C.C. An Overview Manual for the GRAVSOFT Geodetic Gravity Field Modelling Programs, 2nd ed.; DTU Space: Kongens Lyngby, Denmark, 2008. [Google Scholar]
- Forsberg, R.; Tscherning, C.C. An Overview Manual for the GRAVSOFT Geodetic Gravity Field Modelling Programs, 3rd ed.; DTU Space: Kongens Lyngby, Denmark, 2014. [Google Scholar]
- Luu, Q.H.; Tkalich, P.; Tay, T.W. Sea Level Trend and Variability around Peninsular Malaysia. Ocean Sci. 2015, 11, 617–628. [Google Scholar] [CrossRef]
- Strassburg, M.W.; Hamlington, B.D.; Leben, R.R.; Manurung, P.; Lumban Gaol, J.; Nababan, B.; Vignudelli, S.; Kim, K.-Y. Sea Level Trends in Southeast Asian Seas. Clim. Past 2015, 11, 743–750. [Google Scholar] [CrossRef]
- Rizal, S.; Damm, P.; Wahid, M.A.; Sundermann, J.; Ilhamsyah, Y.; Iskandar, T.; Muhammad. General Circulation in the Malacca Strait and Andaman Sea: A Numerical Model Study. Am. J. Environ. Sci. 2012, 8, 479–488. [Google Scholar] [CrossRef]
- Haditiar, Y.; Putri, M.R.; Ismail, N.; Muchlisin, Z.A.; Ikhwan, M.; Rizal, S. Numerical Study of Tides in the Malacca Strait with a 3-D Model. Heliyon 2020, 6, e04828. [Google Scholar] [CrossRef]
- Mansor, K.N.A.A.K.; Pa’suya, M.F.; Abbas, M.A.; Ali, T.A.T.; Aziz, M.A.C.; Din, A.H.M. Ocean Surface Circulation in Strait of Malacca Using Satellite Altimeter and Low-Cost GPS-Tracked Drifting Buoys. In Proceedings of the 2016 7th IEEE Control and System Graduate Research Colloquium (ICSGRC), Shah Alam, Malaysia, 8 August 2016; pp. 175–180. [Google Scholar] [CrossRef]
- Grant, D.B.; Blick, G.H. A National Vertical Datum Independent of Local Mean Sea Level? In Vistas for Geodesy in the New Millennium: IAG 2001 Scientific Assembly; Józef, Á., Schwarz, K.P., Eds.; International Association of Geodesy Symposia; Springer: Budapest, Hungary, 2001; Volume 1. [Google Scholar]
- Mills, J.; Dodd, D. Ellipsoidally Referenced Surveying for Hydrography, 1st ed.; The International Federation of Surveyors (FIG): Frederiksberg, Denmark, 2014. [Google Scholar]
- Wu, F.; Zeng, A.; Ming, F. Analyzing the Long-Term Changes in China’s National Height Datum. Adv. Space Res. 2020, 66, 1342–1350. [Google Scholar] [CrossRef]
- Triana, K.; Wahyudi, A.J. Sea Level Rise in Indonesia: The Drivers and the Combined Impacts from Land Subsidence. ASEAN J. Sci. Technol. Dev. 2020, 37, 115–121. [Google Scholar] [CrossRef]
- Dewi, R.S.; Putra, A.A.; Widyantoro, B.T.; Meilano, I.; Safi’i, A.N. Sea-Level Variations from Co-Located TG and GNSS Stations Using GNSS-Reflectometry in Indonesia. IOP Conf. Ser. Earth Environ. Sci. 2021, 824, 012066. [Google Scholar] [CrossRef]
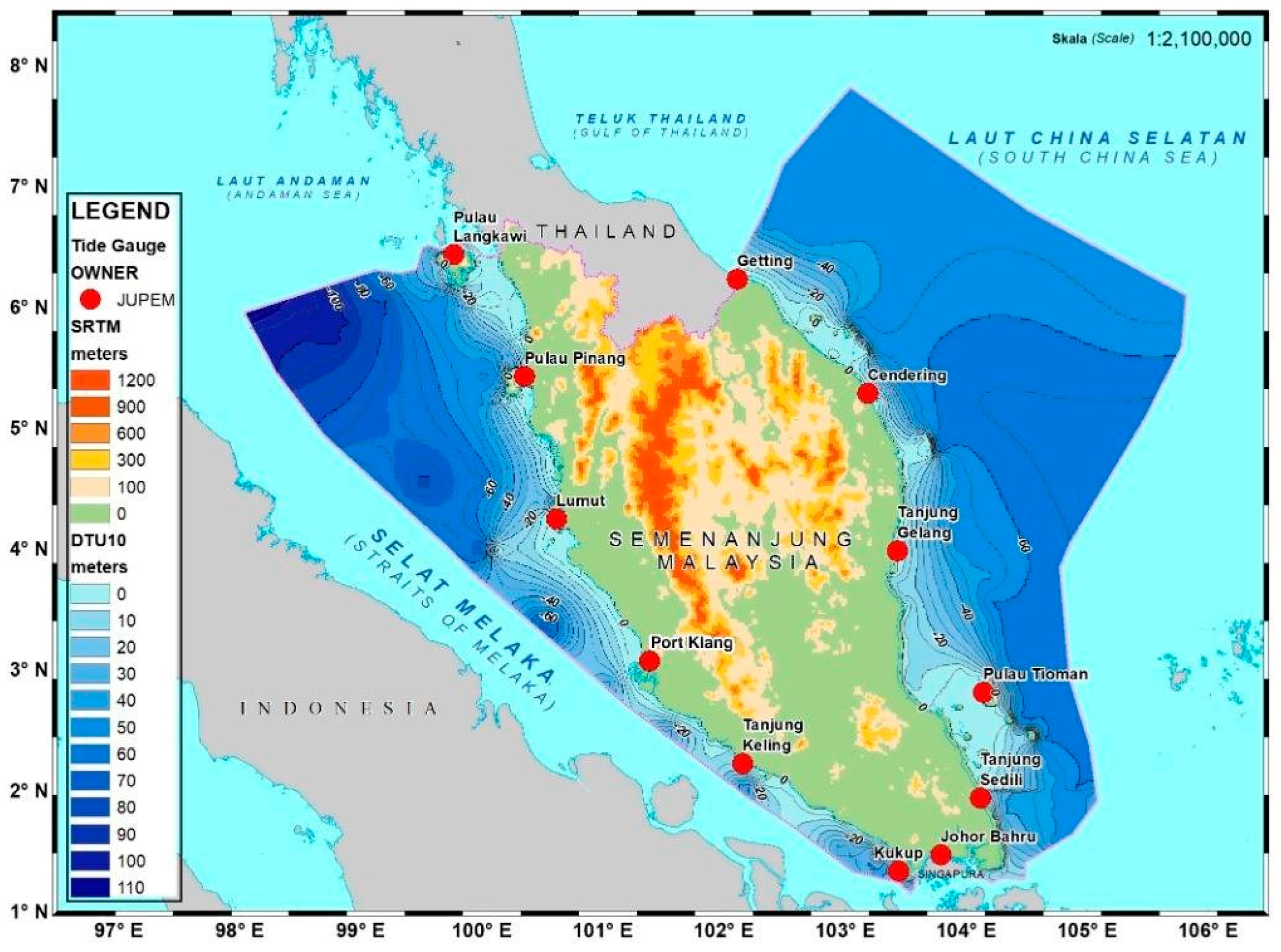
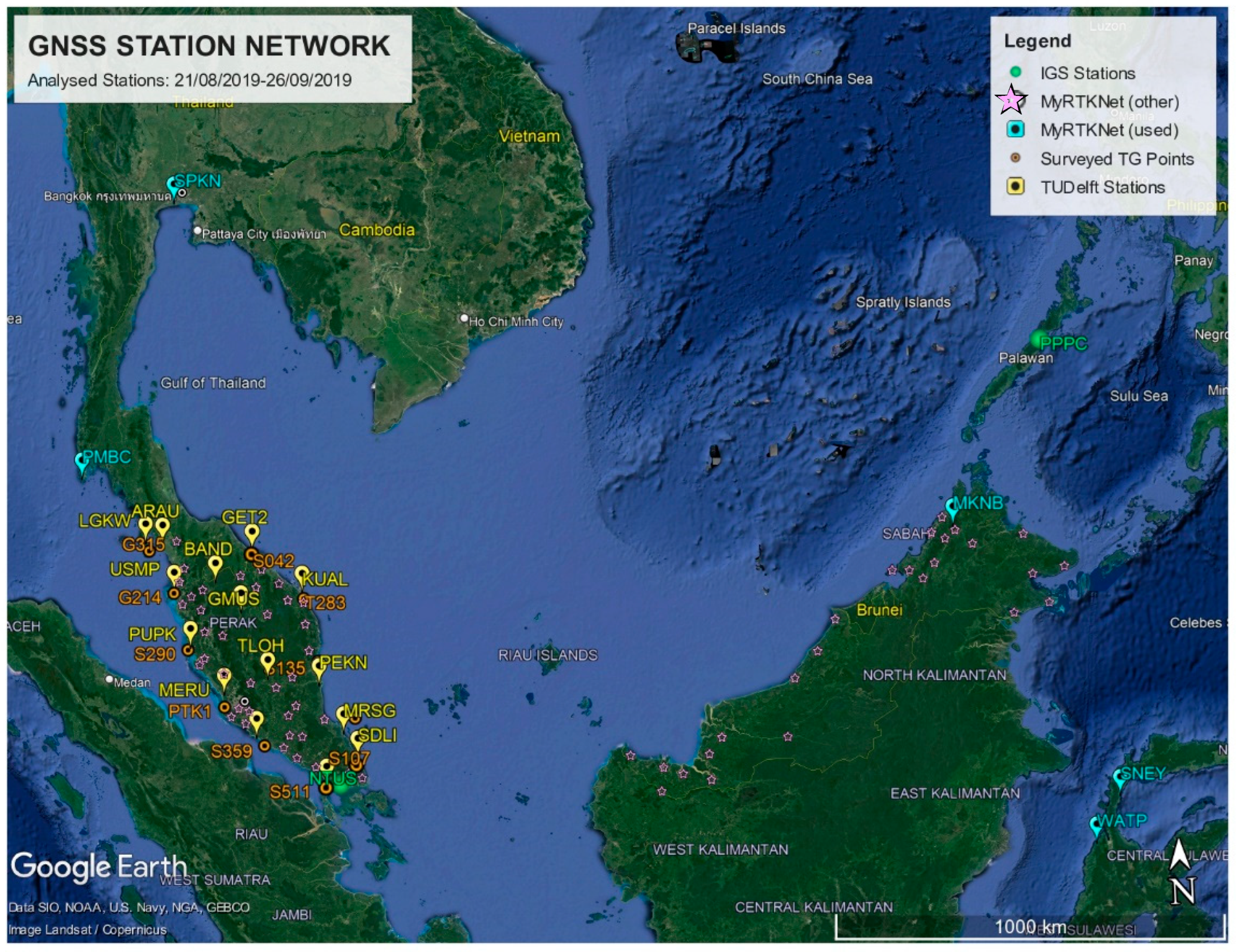


| Tide Gauge Locations | Tide Gauge Station Abbreviations | Local Vertical Datum | TGBM | LVD above TG-Zero (m) | TGBM above TG-Zero (m) | TGBM above LVD (m) |
|---|---|---|---|---|---|---|
| P. Langkawi | LAN | LSD12 | K0172 | 2.128 | 5.545 | 3.417 |
| P. Pinang | PEN | LSD12 | PP0379 | 2.535 | 4.962 | 2.427 |
| Lumut | LUM | PMGVD2000 | A0401 | 2.168 | 5.685 | 3.517 |
| Port Klang | PTK | PMGVD2000 MSL (1984–1993) | B0169 | 3.624 | 7.494 | 3.870 |
| Tg. Keling | TGK | PMGVD2000 | M0331 | 2.759 | 6.427 | 3.668 |
| Kukup | KUK | PMGVD2000 | J5328 | 3.873 | 6.880 | 3.007 |
| Tg. Sedili | SED | PMGVD2000 | J0801 | 2.202 | 4.459 | 2.257 |
| P. Tioman | TIO | TIOMAN MSL (1986–2018) | C0501 | 2.762 | 6.586 | 3.824 |
| Port Kuantan | NKP | PMGVD2000 | C0331 | 2.661 | 6.496 | 3.835 |
| Cendering | CHD | PMGVD2000 | T0283 | 2.084 | 4.688 | 2.604 |
| Geting | GET | PMGVD2000 | D0354 | 2.112 | 5.964 | 3.852 |
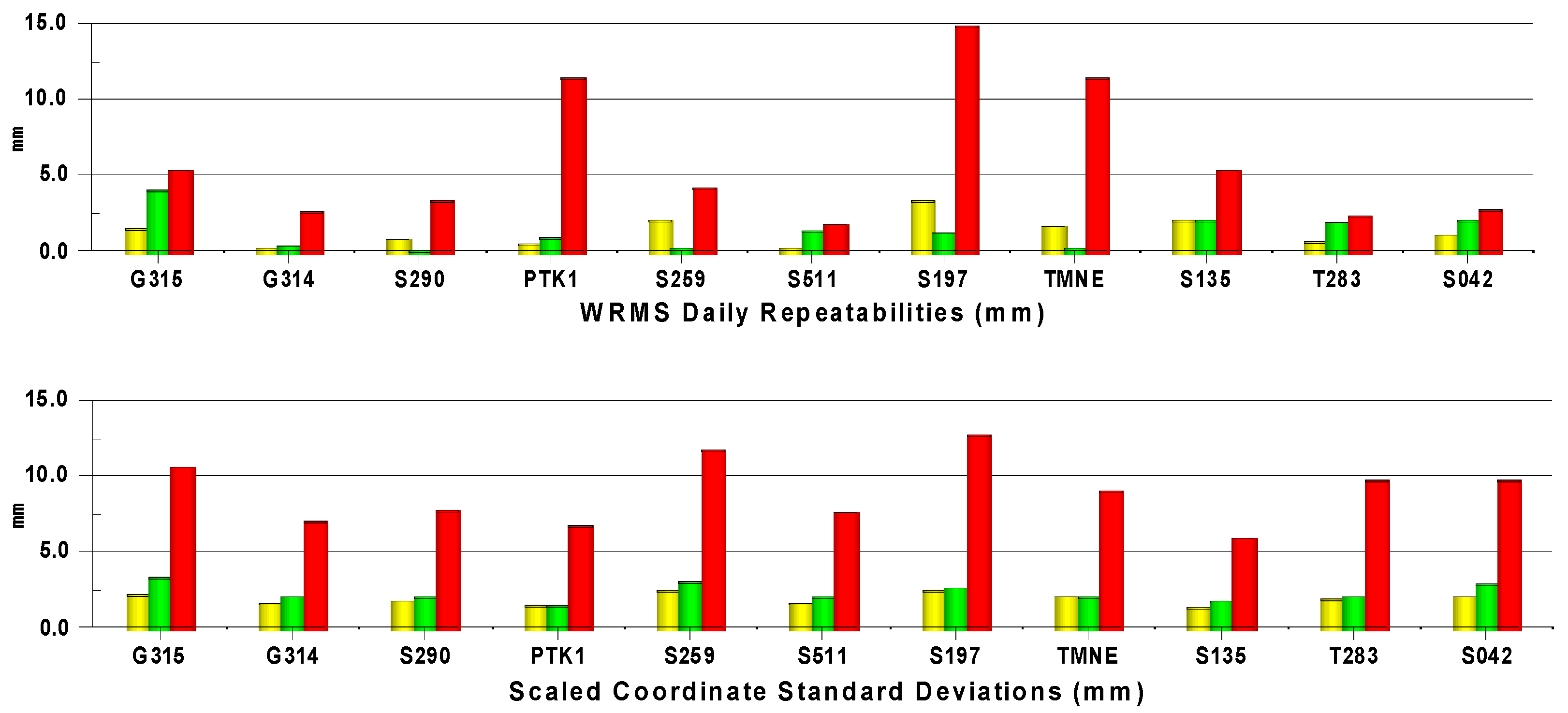
| TG | GPSBM | GPS Data Span | Bernese 5.2 Minus GIPSY-OASIS II Coordinates (cm) | |||
|---|---|---|---|---|---|---|
| Δφ | Δλ | Δh | 3D | |||
| LAN | GPS315 | 48 h | 0.1 | 3.5 | −1.2 | 3.7 |
| PEN | GPS314 | 48 h | −0.1 | 0.7 | 0.1 | 0.7 |
| LUM | S0290 | 48 h | 0.0 | 1.1 | 0.2 | 1.1 |
| PTK | PTK1 | 96 h | 0.0 | 0.2 | 0.5 | 0.5 |
| TGK | S0259 | 48 h | 0.0 | 0.0 | −0.6 | 0.6 |
| KUK | S5110 | 48 h | 0.0 | 0.8 | 0.9 | 1.2 |
| SED | S0197 | 48 h | 0.2 | 0.7 | −3.1 | 3.2 |
| TIO | C0501 | 48 h | 0.0 | 0.6 | 0.5 | 0.8 |
| NKP | S0135 | 96 h | 0.4 | 0.3 | 0.3 | 0.6 |
| CHD | T0283 | 48 h | −0.2 | 0.9 | −0.7 | 1.2 |
| GET | S0042 | 48 h | −0.3 | −0.1 | −0.1 | 0.3 |
| RMS | 0.1 | 1.2 | 1.1 | 1.7 | ||
| TG Stations | LAN | PEN | LUM | PTK | TGK | KUK | JHB | SED | TIO | NKP | CHD | GET |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| RMSL (2022A) (m) | 2.237 | 2.708 | 2.212 | 3.657 | 2.866 | 4.032 | 2.869 | 2.424 | 2.853 | 2.822 | 2.231 | 2.318 |
| RMSL (2022B) (m) | 2.303 | 2.777 | 2.273 | 3.708 | 2.918 | 4.117 | 2.944 | 2.467 | 2.910 | 2.891 | 2.296 | 2.384 |
| RMSL diff. (cm) | −6.6 | −6.9 | −6.1 | −5.1 | −5.2 | −8.5 | −7.5 | −4.3 | −5.7 | −6.9 | −6.5 | −6.6 |
| RMSLtrend (A) (mm/year) | 3.1 | 3.3 | 2.6 | 2.3 | 2.2 | 4.3 | 3.3 | 2.4 | 3.1 | 3.4 | 3.4 | 3.7 |
| RMSLtrend (B) (mm/year) | 3.2 | 3.4 | 2.8 | 2.4 | 2.3 | 4.3 | 3.5 | 2.4 | 3.1 | 3.4 | 3.5 | 3.7 |
| RMSLtrend diff. (mm/year) | −0.1 | −0.1 | −0.2 | −0.1 | −0.1 | 0 | −0.2 | 0 | 0 | 0 | −0.1 | 0 |
| Location | Stn Abbr. | Latitude | Longitude | Data Span | RMSL @2000.0 | RMSL @2022.0 | RMSLtrend @2022.0 | RMSLrise (1993–2022) |
|---|---|---|---|---|---|---|---|---|
| (N) | (E) | (cm) | (cm) | (mm/year) | (cm) | |||
| P. Langkawi | LAN | 6° 25.9′ | 99° 45.9′ | 1986–2019 | 223.29 | 230.27 | 3.1692 ± 0.34 | 9.2 |
| P. Pinang | PEN | 5° 25.3′ | 100° 20.8′ | 1985–2019 | 270.24 | 277.71 | 3.3943 ± 0.32 | 9.8 |
| Lumut | LUM | 4° 14.4′ | 100° 36.8′ | 1985–2019 | 221.19 | 227.26 | 2.7571 ± 0.31 | 8.0 |
| Port Klang | PTK | 3° 03.0′ | 101° 21.5′ | 1984–2019 | 365.47 | 370.75 | 2.4015 ± 0.34 | 8.4 |
| Tg. Keling | TGK | 2° 12.9′ | 102° 09.2′ | 1985–2019 | 286.75 | 291.84 | 2.3109 ± 0.26 | 6.7 |
| Kukup | KUK | 1° 19.5′ | 103° 26.6′ | 1986–2019 | 402.38 | 411.75 | 4.2614 ± 0.24 | 12.4 |
| Johor Bahru | JHB | 1° 27.7′ | 103° 47.5′ | 1984–2014 | 286.84 | 294.45 | 3.4591 ± 0.23 | 10.0 |
| Tg Sedili | SED | 1° 55.9′ | 104° 06.9′ | 1986–2019 | 241.32 | 246.68 | 2.4332 ± 0.21 | 7.1 |
| P. Tioman | TIO | 2° 48.4′ | 104° 08.4′ | 1986–2019 | 284.25 | 290.99 | 3.0647 ± 0.20 | 8.9 |
| Port Kuantan | NKP | 3° 58.5′ | 103° 25.8′ | 1984–2019 | 281.53 | 289.09 | 3.4363 ± 0.18 | 10.0 |
| Cendering | CHD | 5° 15.9′ | 103° 11.2′ | 1985–2019 | 222.01 | 229.64 | 3.4650 ± 0.23 | 10.1 |
| Geting | GET | 6° 13.6′ | 102° 06.4′ | 1986–2017 | 230.25 | 238.42 | 3.7107 ± 0.30 | 10.8 |
| Epoch | 1993.0 | 2022.0 |
|---|---|---|
| RMSL at epoch | 3.624 m | 3.708 m |
|
Observed RMSLrise = RMSL (2022.0) − RMSL (1993.0) | +8.4 cm | |
|
Predicted RMSLrise = (2022.0 − 1993.0) × 2.4015 mm/year | +7.0 cm | |
| Observed minus predicted | +1.4 cm |
| TG Station | TGBM | GPSBM | GPSBM above TG-Zero | MSL 2022B above TG-Zero | GPSBM above MSL2022B | GPSBM above PMGVD2000 | MSL2022B Minus PMGVD2000 |
|---|---|---|---|---|---|---|---|
| (m) | (m) | (m) | (m) | (m) | |||
| P. Langkawi | K0172 | GPS315 | 5.465 | 2.3027 | 3.162 | 3.316 | −0.154 |
| P. Pinang | PP0379 | GPS314 | 6.442 | 2.7771 | 3.665 | 3.886 | −0.221 |
| Lumut | A0401 | S0290 | 5.610 | 2.2726 | 3.337 | 3.450 | −0.113 |
| Port Klang | B0169 | PTK1 | 7.449 | 3.7075 | 3.742 | 3.869 | −0.128 |
| Tg. Keling | M0331 | S0259 | 6.424 | 2.9184 | 3.506 | 3.665 | −0.159 |
| Kukup | J5328 | S5110 | 5.992 | 4.1175 | 1.875 | 2.120 | −0.245 |
| Tg. Sedeli | J0801 | S0197 | 4.476 | 2.4668 | 2.009 | 2.274 | −0.265 |
| P. Tioman | C0501 | C0501 | 6.640 | 2.9099 | 3.730 | 3.811 | −0.081 |
| Port Kuantan | C0331 | S0135 | 6.959 | 2.8909 | 4.068 | 4.32 | −0.252 |
| Cendering | T0283 | T0283 | 4.672 | 2.2964 | 2.376 | 2.604 | −0.228 |
| Geting | D0354 | S0024 | 5.815 | 2.3842 | 3.431 | 3.704 | −0.273 |
| TG | GPSBM | TG-GPSBM Geodetic Coordinates (ITRF2014/WGS84) | TG-GPSBM Orthometric Height | |||
|---|---|---|---|---|---|---|
| Latitude (DD) | Longitude (DD) | Ellip- Soidal Height | above PMGVD 2000 | above MSL 2022B | ||
| (N) | (E) | (m) | (m) | (m) | ||
| LAN | GPS315 | 6.426020643 | 99.765413951 | −12.706 | 3.316 | 3.162 |
| PEN | GPS314 | 5.421515981 | 100.344378832 | −7.798 | 3.886 | 3.665 |
| LUM | S0290 | 4.234707265 | 100.611870447 | −5.013 | 3.450 | 3.337 |
| PTK | PTK1 | 3.050868774 | 101.356374164 | 0.581 | 3.869 | 3.742 |
| TGK | S0259 | 2.217544267 | 102.153995869 | 4.294 | 3.665 | 3.506 |
| KUK | S5110 | 1.326204310 | 103.444161820 | 9.209 | 2.120 | 1.715 |
| SED | S0197 | 1.928887297 | 104.113042835 | 11.434 | 2.274 | 2.009 |
| TIO | C0501 | 2.806658225 | 104.139219984 | 11.884 | 3.811 | 3.730 |
| NKP | S0135 | 3.976607024 | 103.427745967 | 7.263 | 4.320 | 4.068 |
| CHD | T0283 | 5.264132659 | 103.184385249 | 1.944 | 2.604 | 2.376 |
| GET | S0042 | 6.225672793 | 102.104655988 | −3.375 | 3.704 | 3.431 |
| Project/Year | Airborne Gravity Survey Coverage | Aircraft Type | Airborne Gravity/IMU Equipment | Airborne Survey (km-Line) | Data Line Spacing (km) |
|---|---|---|---|---|---|
| MyGeoid (2002–2003) | Land Area | Antonov-38 (Layang-Layang Air) | LC&R air-sea/ Honeywell H764G IMU | 38,200 | 5 km |
| MyMarineGI (2019–2022) | Marine Area | BE200 (Sabah Air Aviation) | LC&R air-sea/ iMAR-IMU | 30,000 | 5 km < 12 NM 10 km > 12 NM |
| Gravity Anomaly Data | Original Data | Minus XGM2019 | Full Reduction | |||
|---|---|---|---|---|---|---|
| Mean | Standard Deviation | Mean | Standard Deviation | Mean | Standard Deviation | |
| Airborne 2022 (DTU) | 14.71 | 12.89 | −0.86 | 4.65 | −0.08 | 4.14 |
| Airborne 2022 (TUD) | 14.71 | 12.69 | −0.86 | 4.13 | −0.08 | 3.73 |
| Airborne 2019 | 16.03 | 11.98 | 0.73 | 3.72 | 0.85 | 3.63 |
| Airborne 2003 | 24.39 | 13.27 | −0.03 | 8.09 | −0.12 | 5.22 |
| DSMM gravimetry, edited | 14.83 | 14.55 | −5.41 | 12.97 | 1.73 | 7.09 |
| HMS project data. edited | 19.84 | 8.14 | −2.15 | 7.90 | −0.07 | 6.80 |
| DTU21 altimetry, no-data area | 14.53 | 13.34 | −0.24 | 3.93 | −0.20 | 3.91 |
| Geoid Models | DSMM Pre-2019 GPS/Levelling Data | HMS 2008 GPS/Levelling Data (Kuala Lumpur Area) | ||
|---|---|---|---|---|
| Mean | Std. Dev. | Mean | Std. Dev. | |
| XGM2019 | 0.686 | 0.120 | 0.774 | 0.055 |
| Geoid 1: Airborne-only | 0.698 | 0.092 | 0.745 | 0.016 |
| Geoid 2: Airborne + terrestrial | 0.695 | 0.092 | 0.734 | 0.015 |
| TG | GPSBM | hGPS | Nfit_PTK | HGeoid = hGPS − Nfit_PTK | HPMGVD2000 | ∆H = HGeoid − HPMGVD2000 |
|---|---|---|---|---|---|---|
| (m) | (m) | (m) | (m) | (cm) | ||
| LAN | GPS315 | −12.706 | −15.950 | 3.244 | 3.316 | −7.2 |
| PEN | GPS314 | −7.798 | −11.557 | 3.759 | 3.886 | −12.7 |
| LUM | S0290 | −5.013 | −8.450 | 3.437 | 3.45 | −1.3 |
| PTK | PTK1 | 0.581 | −3.288 | 3.869 | 3.869 | 0.0 |
| TGK | S0259 | 4.294 | 0.596 | 3.698 | 3.665 | 3.3 |
| KUK | S5110 | 9.209 | 7.257 | 1.952 | 2.120 | −16.8 |
| SED | S0197 | 11.434 | 9.139 | 2.295 | 2.274 | 2.1 |
| TIO | C0501 | 11.884 | 7.864 | 4.02 | 3.811 | 20.9 |
| NKP | S0135 | 7.263 | 2.923 | 4.34 | 4.32 | 2.0 |
| CHD | T0283 | 1.944 | −0.720 | 2.664 | 2.604 | 6.0 |
| GET | S0042 | −3.375 | −7.140 | 3.765 | 3.704 | 6.1 |
| TG | GPSBM | hGPS | Nfit_PTK | HGeoid = hGPS − Nfit_PTK | HMSL2022B | ∆H = HGeoid – HMSL2022B |
|---|---|---|---|---|---|---|
| (m) | (m) | (m) | (m) | (cm) | ||
| LAN | GPS315 | −12.706 | −15.823 | 3.117 | 3.162 | −4.5 |
| PEN | GPS314 | −7.798 | −11.430 | 3.632 | 3.665 | −3.3 |
| LUM | S0290 | −5.013 | −8.323 | 3.310 | 3.337 | −2.7 |
| PTK | PTK1 | 0.581 | −3.161 | 3.742 | 3.742 | 0.0 |
| TGK | S0259 | 4.294 | 0.723 | 3.571 | 3.506 | 6.5 |
| KUK | S5110 | 9.209 | 7.384 | 1.825 | 1.715 | 11.0 |
| SED | S0197 | 11.434 | 9.266 | 2.168 | 2.009 | 15.9 |
| TIO | C0501 | 11.884 | 7.991 | 3.893 | 3.73 | 16.3 |
| NKP | S0135 | 7.263 | 3.050 | 4.213 | 4.068 | 14.5 |
| CHD | T0283 | 1.944 | −0.593 | 2.537 | 2.376 | 16.1 |
| GET | S0042 | −3.375 | −7.013 | 3.638 | 3.431 | 20.7 |
| National Geodetic Reference Frame | MGRF2020 |
|---|---|
| Reference Ellipsoid | GRS80 |
| RMSL at TG Stations | Refer to Table 4 |
| RMSL Reference Epoch | t0 = 2022.0 |
| RMSL Trends | Refer to Table 4 |
| Datum Offset (relative MDT) | Refer to Table 12 |
| MSL-Geoid | PMGeoid2022_fit_PTK |
| Precise Levelling Network | PLN2022 |
| PMGVD2022 Extent | Land and marine areas of Peninsular Malaysia |
| TG | HPMGVD2000 | HMSL2022B | ΔH = HMSL2022 – HPMGVD2000 | ΔH = ΔHRMSLrise + ΔHRMDT + ΔHLVD offset + LE | ΔH2LVD_offset + LE | Remark | ||
|---|---|---|---|---|---|---|---|---|
| ΔHRMSLrise | ΔHRMDT | ΔH1 LVD_offset + LE | ||||||
| (m) | (m) | (cm) | (cm) | (cm) | (cm) | (cm) | ||
| LAN | 3.316 | 3.162 | −15.4 | −9.2 | 4.5 | −10.7 | −7.2 | LSD12-offset |
| PEN | 3.886 | 3.665 | −22.1 | −9.8 | 3.3 | −15.6 | −12.7 | LSD12-offset |
| LUM | 3.450 | 3.337 | −11.3 | −8.0 | 2.7 | −6.0 | −1.3 | LE |
| PTK | 3.869 | 3.742 | −12.7 | −8.4 | 0.0 | −4.3 | 0.0 | Datum Point |
| TGK | 3.665 | 3.506 | −15.9 | −6.7 | −6.5 | −2.7 | 3.3 | LE |
| KUK | 2.120 | 1.715 | −40.5 | −12.4 | −11.0 | −17.1 | −16.8 | LE + Subsidence |
| SED | 2.274 | 2.009 | −26.5 | −7.1 | −15.9 | −3.5 | 2.1 | LE |
| TIO | 3.811 | 3.73 | −8.1 | −8.9 | −16.3 | 17.1 | 20.9 | Tioman-offset |
| NKP | 4.320 | 4.068 | −25.2 | −10.0 | −14.5 | −0.7 | 2.0 | LE |
| CHD | 2.604 | 2.376 | −22.8 | −10.1 | −16.1 | 3.4 | 6.0 | LE |
| GET | 3.704 | 3.431 | −27.3 | −10.8 | −20.7 | 4.2 | 6.1 | LE |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cob, S.; Kadir, M.; Forsberg, R.; Simons, W.; Naeije, M.; Din, A.H.; Yacob, H.; Amat, A.; Mahdzur, D.; Ibrahim, Z.; et al. Epoch-Based Height Reference System for Sea Level Rise Impact Assessment on the Coast of Peninsular Malaysia. Remote Sens. 2022, 14, 6179. https://doi.org/10.3390/rs14236179
Cob S, Kadir M, Forsberg R, Simons W, Naeije M, Din AH, Yacob H, Amat A, Mahdzur D, Ibrahim Z, et al. Epoch-Based Height Reference System for Sea Level Rise Impact Assessment on the Coast of Peninsular Malaysia. Remote Sensing. 2022; 14(23):6179. https://doi.org/10.3390/rs14236179
Chicago/Turabian StyleCob, Sanusi, Majid Kadir, Rene Forsberg, Wim Simons, Marc Naeije, Ami Hassan Din, Husaini Yacob, Asyran Amat, Daud Mahdzur, Zuhairy Ibrahim, and et al. 2022. "Epoch-Based Height Reference System for Sea Level Rise Impact Assessment on the Coast of Peninsular Malaysia" Remote Sensing 14, no. 23: 6179. https://doi.org/10.3390/rs14236179
APA StyleCob, S., Kadir, M., Forsberg, R., Simons, W., Naeije, M., Din, A. H., Yacob, H., Amat, A., Mahdzur, D., Ibrahim, Z., Aziz, K., Yaacob, N., Johann, F., Jensen, T., Teitsson, H., Ses, S., Yahaya, A., Nordin, S., & Majid, F. (2022). Epoch-Based Height Reference System for Sea Level Rise Impact Assessment on the Coast of Peninsular Malaysia. Remote Sensing, 14(23), 6179. https://doi.org/10.3390/rs14236179






