DTM-Based Comparative Geomorphometric Analysis of Four Scoria Cone Areas—Suggestions for Additional Approaches
Abstract
1. Introduction
2. Theoretical Background
2.1. Geomorphometry of Scoria Cones—Previous Research
2.1.1. Based on Classical (Field) Surveys
2.1.2. DTM-Based Research
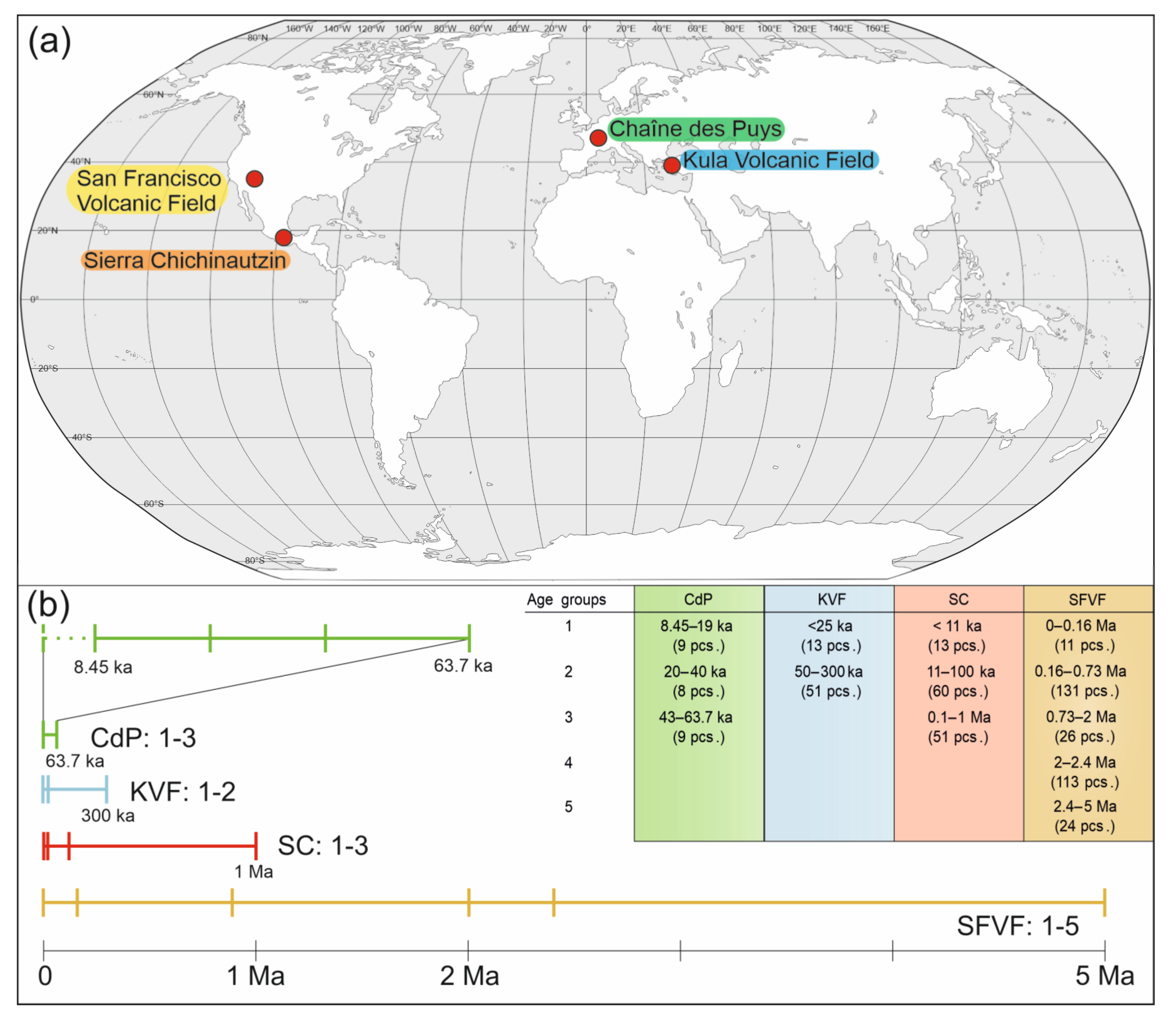
2.2. Study Areas and Age Groups
2.2.1. Chaîne des Puys (CdP)
2.2.2. San Francisco Volcanic Field (SFVF)
2.2.3. Sierra Chichinautzin (SC)
2.2.4. Kula Volcanic Field (KVF)
3. Materials and Methods
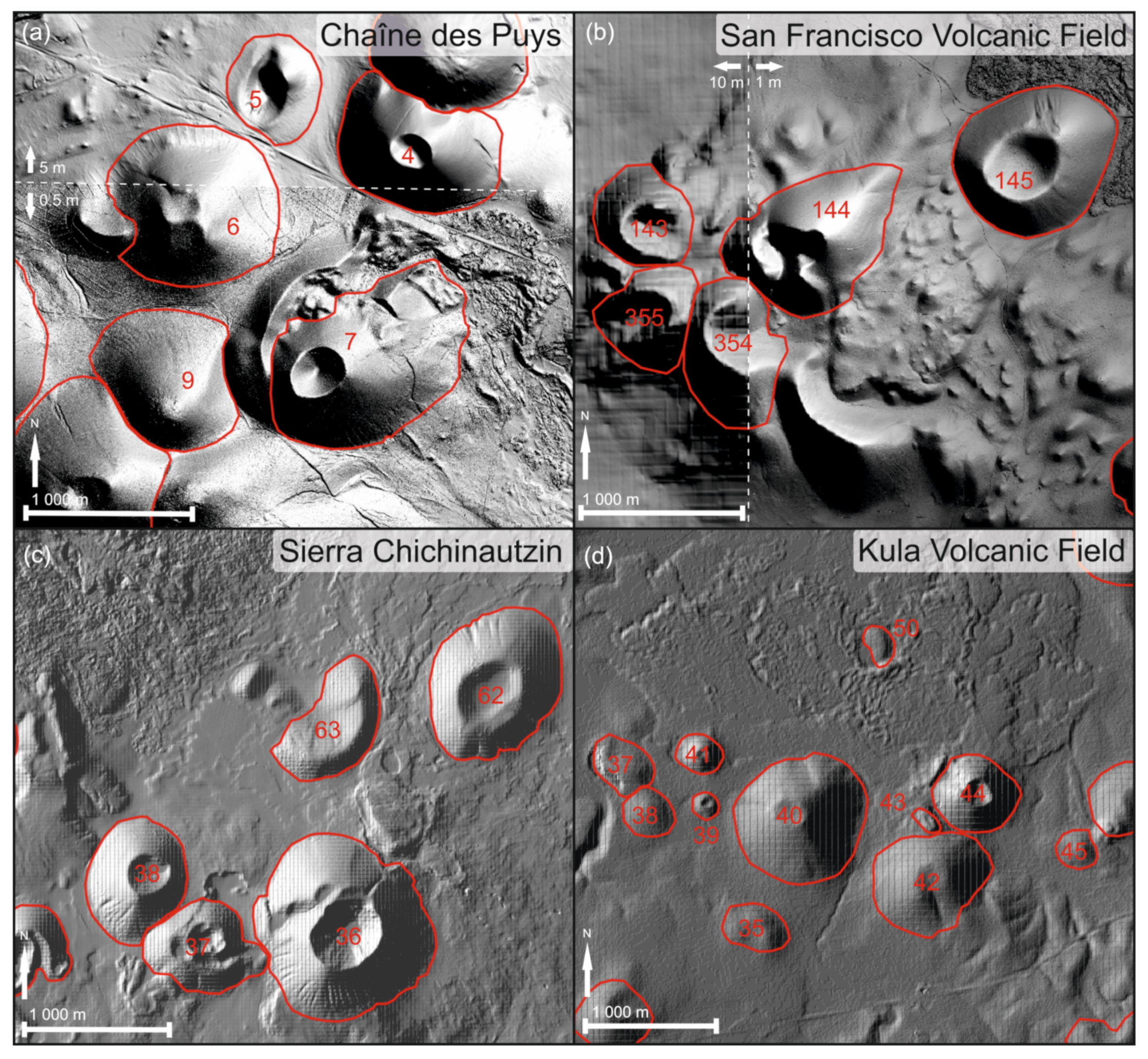
3.1. Use of DTMs
3.1.1. Chaîne des Puys
3.1.2. San Francisco Volcanic Field
3.1.3. Sierra Chichinautzin
3.1.4. Kula Volcanic Field
3.2. Classical Approach in Scoria Cone Morphometry
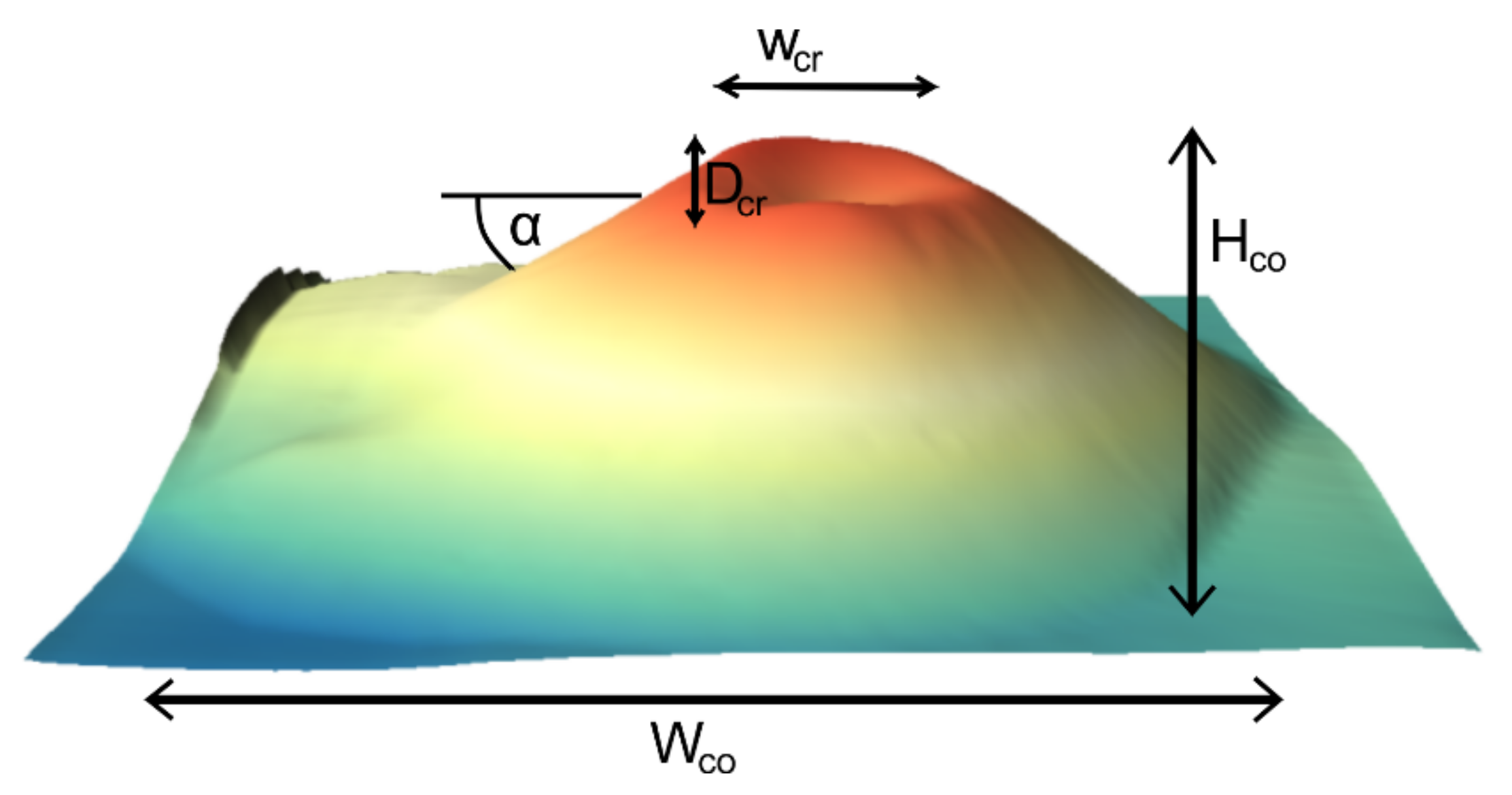
3.2.1. Parameter Calculations
3.2.2. Mann–Whitney Statistical Test
3.3. New Symmetry Approaches in Scoria Cone Morphometry
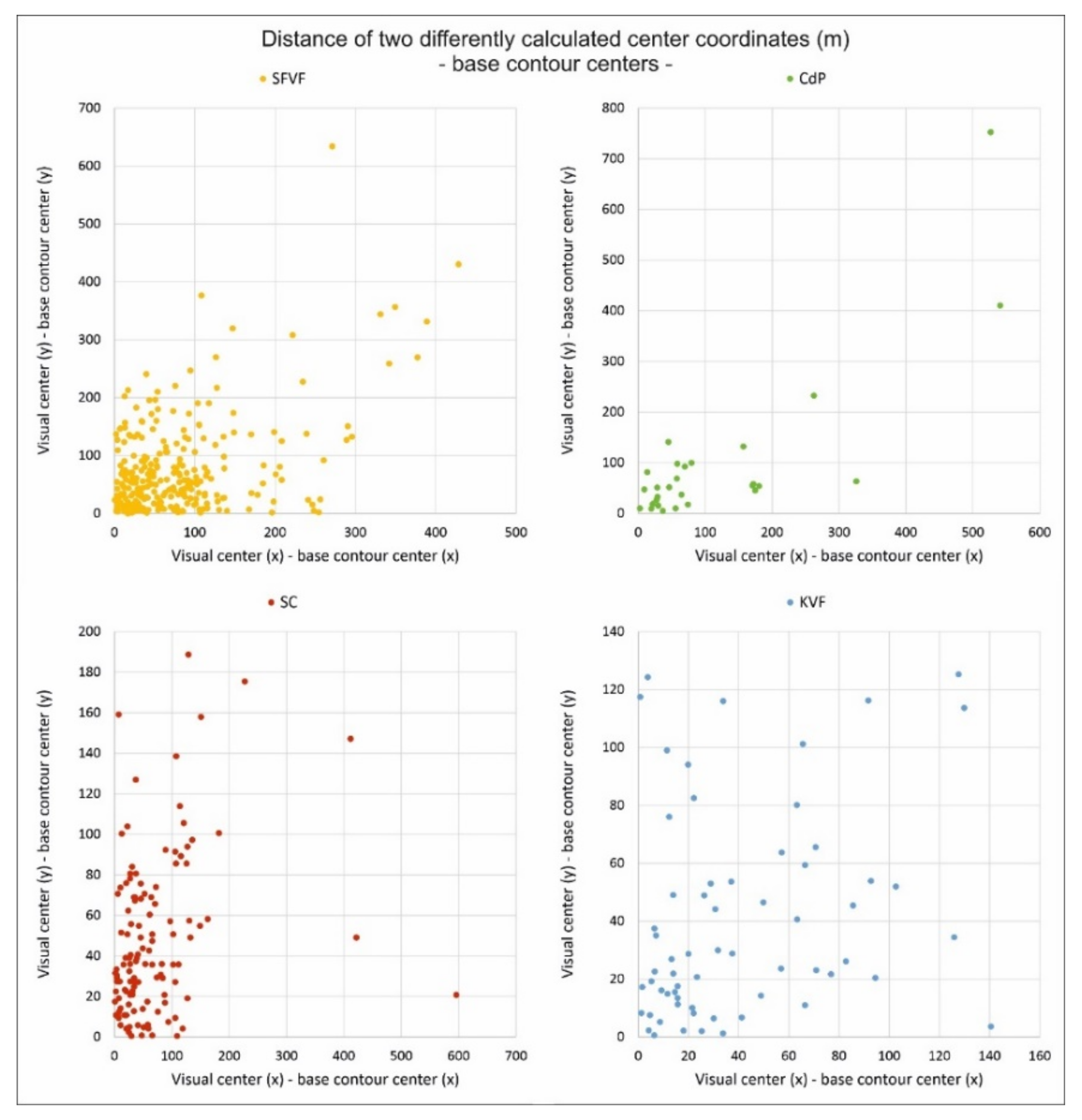
3.3.1. Detecting the Base Contour and the Centre Coordinates
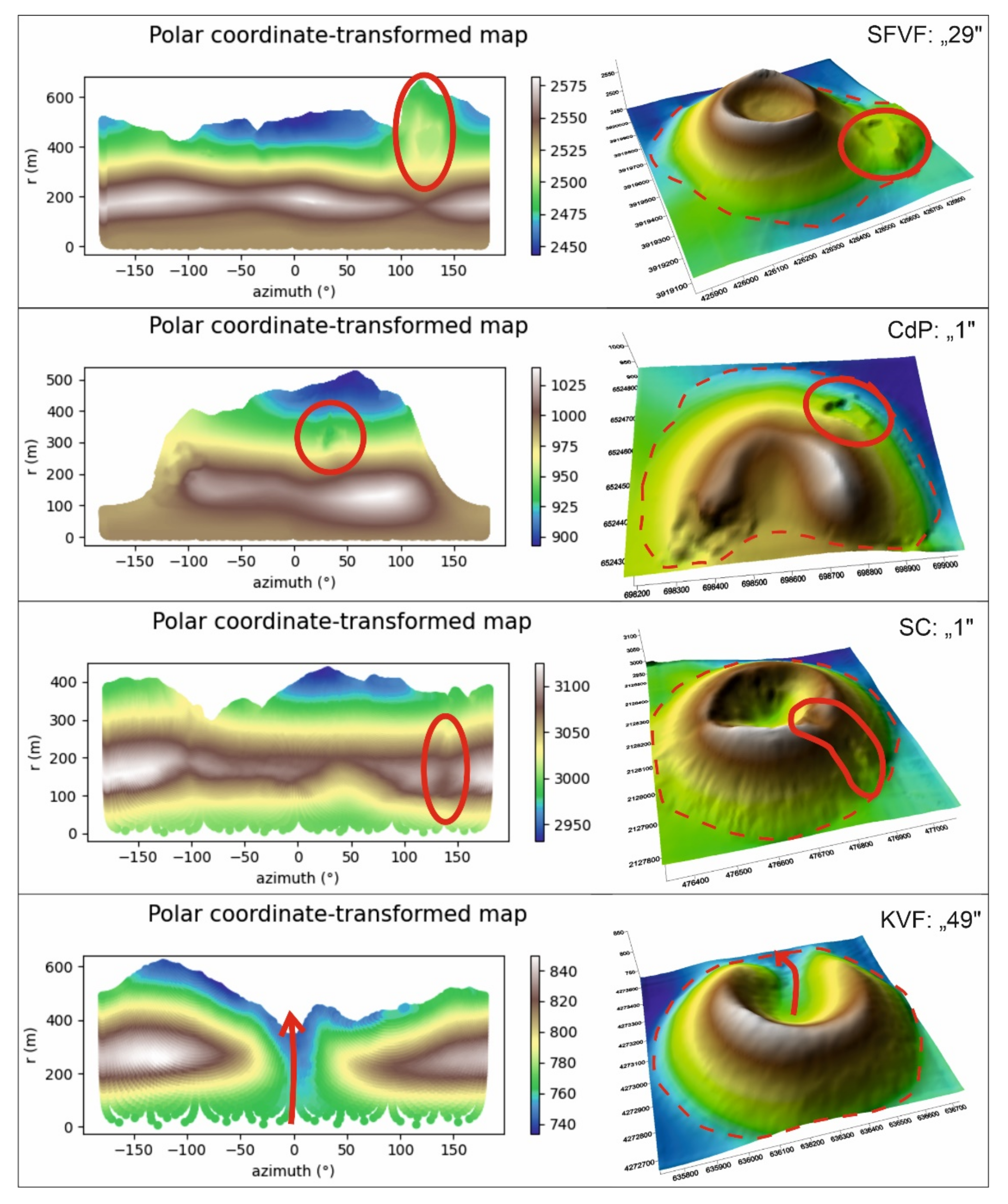
3.3.2. Polar Coordinate-Transformed Maps
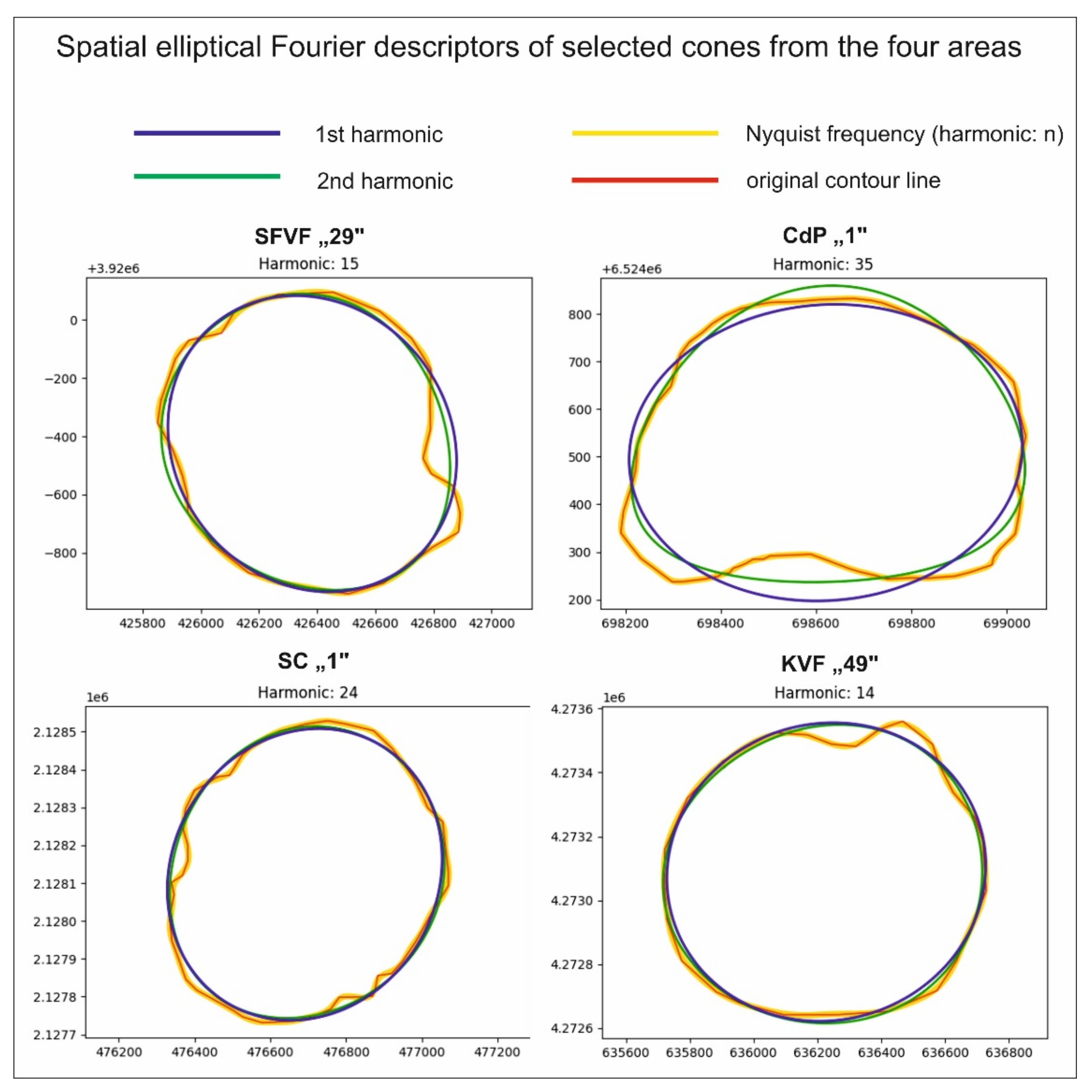
3.3.3. Spatial Elliptical Fourier Descriptors
4. Results
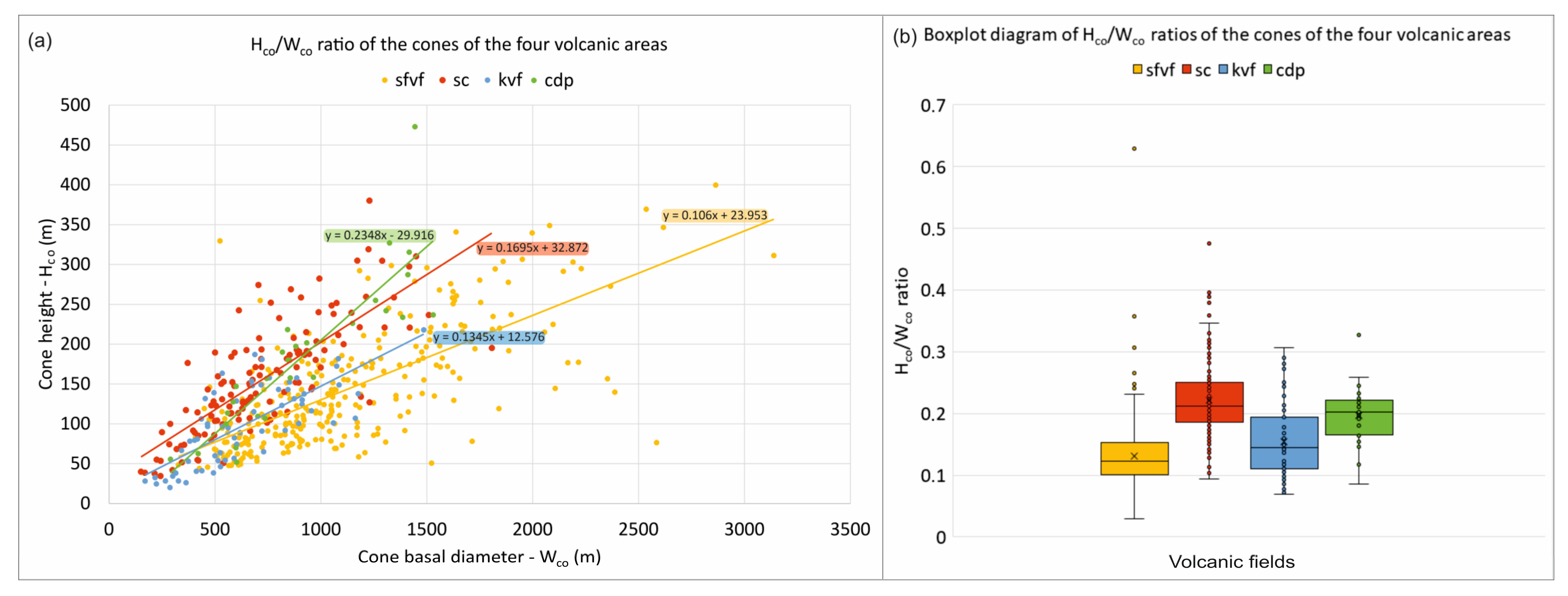
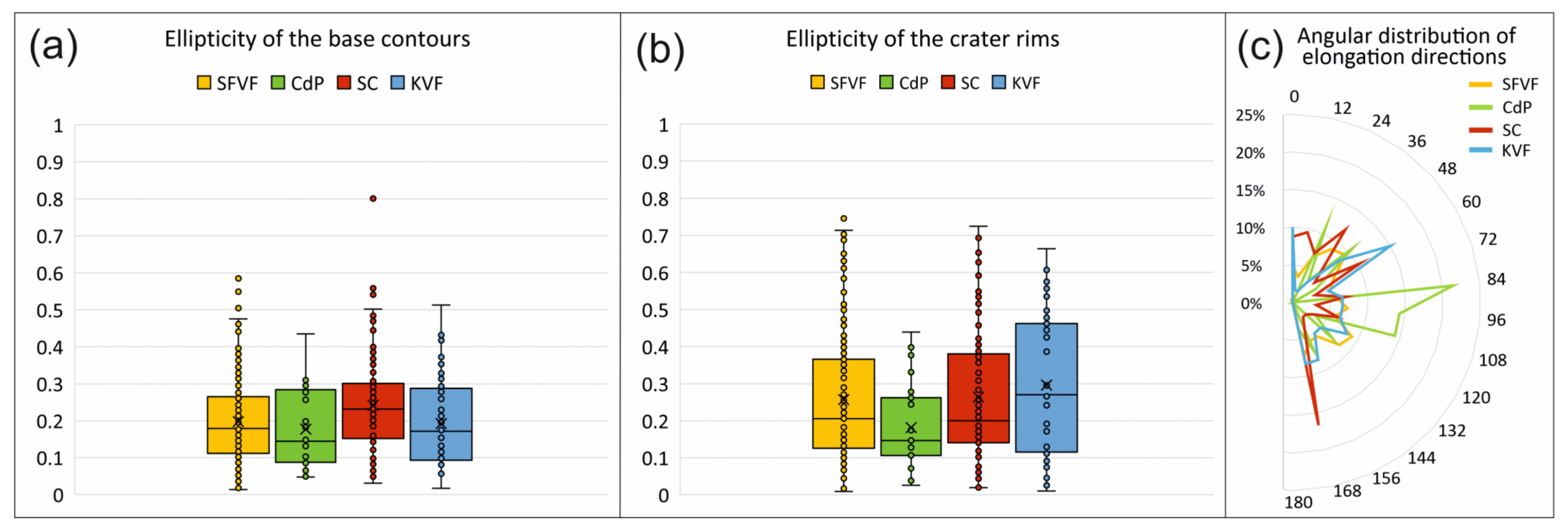
4.1. Results Based on the Classic Parameters
4.2. Results of the New Approaches
4.2.1. Polar Coordinate-Transformed Maps
4.2.2. Spatial Elliptical Fourier Descriptors
5. Discussion
6. Conclusions
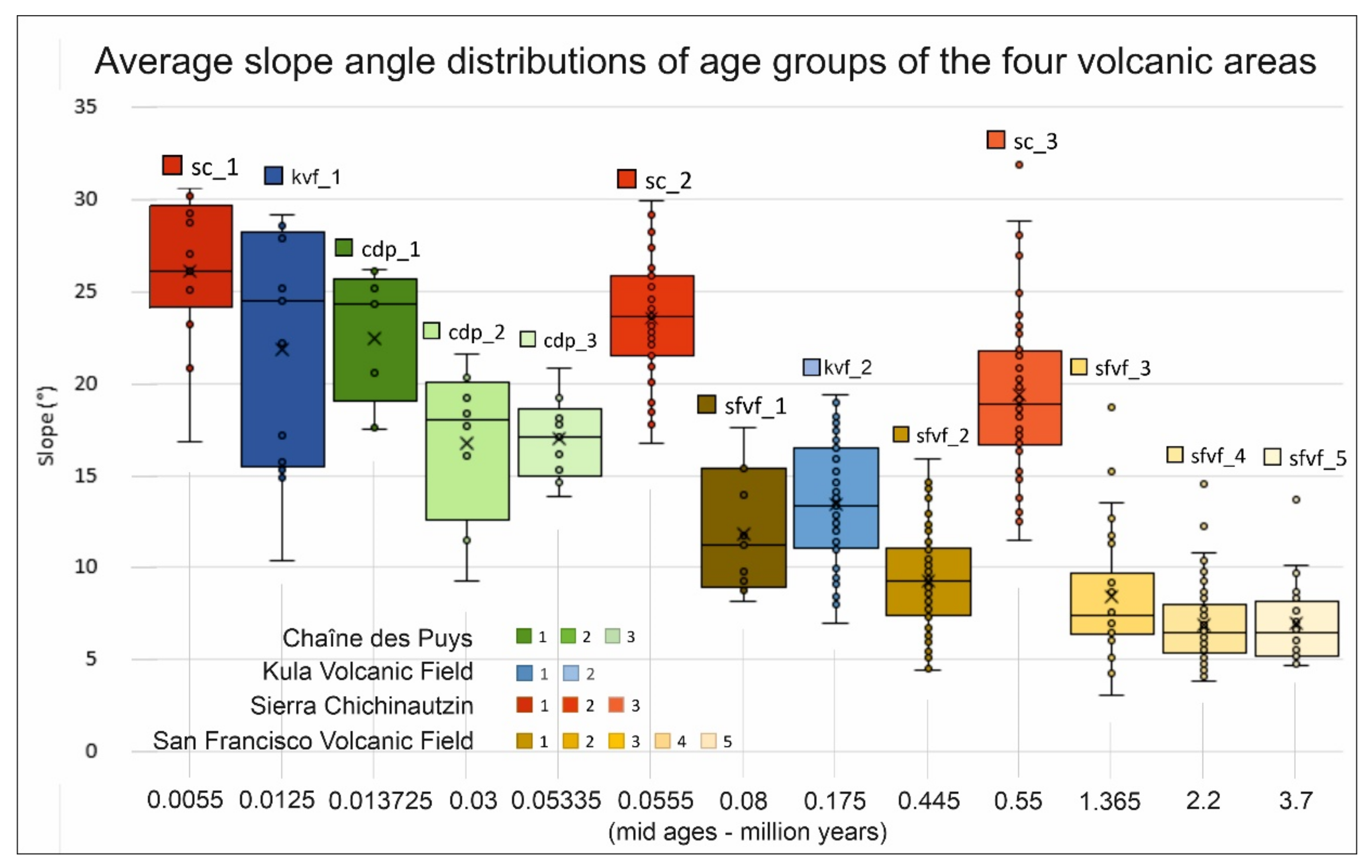
- Whereas the individual behaviour of the slope patterns of scoria cones may vary and old and young cones may show similar morphometric values, the distributions of the average slope angles of the cone age groups follow a trend. According to the statistical tests, the distribution of the slope angles of the older groups is significantly different from the younger ones, with slope angles tending to decrease towards the older groups.
- Applying our innovative idea, to merge the age groups of all four volcanic areas into a combined dataset and treating the age groups independently from the specific area, the distributions ordered by the increasing mid-period age show a similar trend with decreasing median values, except for Sierra Chichinautzin. The latter area shows heteroscedastic but also trend-like behaviour.
- From the study of the interquartile ranges (IQR) of the average slope distributions, we can conclude that not only the median values of the slope distributions decrease with increasing mid-period age, but they also become more uniform with age, with the interquartile range of slopes decreasing in a trend-like manner.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wood, C.A. Morphometric evolution of cinder cones. J. Volcanol. Geotherm. Res. 1980, 7, 387–413. [Google Scholar] [CrossRef]
- Wood, C.A. Morphometric analysis of cinder cone degradation. J. Volcanol. Geotherm. Res. 1980, 8, 137–160. [Google Scholar] [CrossRef]
- Székely, B.; Király, E.; Karátson, D.; Bata, T. A parameterisation attempt of scoria cones of the San Francisco Volcanic Field (Arizona, USA) by conical fitting. In Proceedings of the Proceedings of Geomorphometry, Zurich, Switzerland, 31 August–2 September 2009; Purves, R., Gruber, S., Hengl, T., Straumann, R., Eds.; Department of Geography, University of Zurich: Zurich, Switzerland, 2009; pp. 178–182. [Google Scholar]
- Vörös, F.; Koma, Z.; Székely, B. The application of polar coordinate transformation on the cinder cones of the San Francisco Volcanic Field. In Proceedings of the Student V4 Geoscience Conference and Scientific Meeting GISÁČEK: Conference Proceedings, Ostrava, Czech Republic, 22 March 2017; Tesla, J., Ed.; Institute of Geoinformatics, Technical University of Ostrava: Ostrava, Czech Republic, 2017; pp. 102–110. [Google Scholar]
- Székely, B.; Vörös, F. Studying the distributions of DTM derivatives of cinder cones: A statistical approach in volcanic morphometry. EGU Gen. Assem. 2020, 2020, 10465. [Google Scholar] [CrossRef]
- Porter, S.C. Distribution, morphology, and size frequency of cinder cones on Mauna Kea volcano, Hawaii. Bull. Geol. Soc. Am. 1972, 83, 3607–3612. [Google Scholar] [CrossRef]
- Colton, S.H. The basaltic cinder cones and lava flows of the San Francisco Mountain volcanic field. Museum North. Arizona Bull. 1967, 10, 1–49. [Google Scholar]
- Kear, D. Erosional stages of volcanic cones as indicators of age. N. Z. J. Sci. Tech. 1957, 38, 671–682. [Google Scholar]
- Segerstrom, K. Erosion Studies at Paricutin, State of Michoacán, Mexico. U. S. Geol. Surv. Bull. 1950, 965, 1–163. [Google Scholar] [CrossRef]
- Segerstrom, K. Erosion and related phenomena at Parıcutin in 1957. U. S. Geol. Surv. Bull. 1960, 1104, 1–18. [Google Scholar]
- Segerstrom, K. Parıcutin, 1965-Aftermath of eruption. U. S. Geol. Surv. Prof. Pap. 1966, 550, 93–101. [Google Scholar]
- Bloomfield, K. A late-Quaternary monogenetic volcano field in central Mexico. Geol. Rundsch. 1975, 64, 476–497. [Google Scholar] [CrossRef]
- Scott, D.H.; Trask, N.J. Geology of the Lunar Crater Volcanic Field, Nye County, Nevada. U. S. Geol. Surv. Prof. Pap. 1971, 599, 22. [Google Scholar]
- Settle, M. The structure and emplacement of cinder cone fields. Am. J. Sci. 1979, 279, 1089–1107. [Google Scholar] [CrossRef]
- Moore, R.B.; Wolfe, E. Geologic Map of the Eastern San Francisco Volcanic Field, Arizona, Scale 1:50 000; U. S. Geological Survey: Reston, VA, USA, 1976. [Google Scholar]
- Hooper, D.M.; Sheridan, M.F. Computer-simulation models of scoria cone degradation. J. Volcanol. Geotherm. Res. 1998, 83, 241–267. [Google Scholar] [CrossRef]
- Favalli, M.; Karátson, D.; Mazzarini, F.; Pareschi, M.T.; Boschi, E. Morphometry of scoria cones located on a volcano flank: A case study from Mt. Etna (Italy), based on high-resolution LiDAR data. J. Volcanol. Geotherm. Res. 2009, 186, 320–330. [Google Scholar] [CrossRef]
- Fornaciai, A.; Favalli, M.; Karátson, D.; Tarquini, S.; Boschi, E. Morphometry of scoria cones, and their relation to geodynamic setting: A DEM-based analysis. J. Volcanol. Geotherm. Res. 2012, 217–218, 56–72. [Google Scholar] [CrossRef]
- Vörös, F.; van Wyk de Vries, B.; Székely, B. Geomorphometric descriptive parameters of scoria cones from different DTMs: A resolution invariance study. In Proceedings of the 7th International Conference on Cartography and GIS, Sozopol, Bulgaria, 18–23 June 2018; Bandrova, T., Konečný, M., Eds.; pp. 603–612. [Google Scholar]
- Priest, S.; Duffield, W.; Malis-Clark, K.; Hendley, J., II; Stauffer, P. The San Francisco Volcanic Field, Arizona; U. S. Geological Survey Fact Sheet 017-01; USGS: Reston, VA, USA, 2001. [Google Scholar] [CrossRef]
- Bata, T. Morfometriai Paraméterek Meghatározása Vulkáni Kúpokon a San Francisco Vulkáni Terület (USA, Arizona) Példáján. Master’s Thesis, Eötvös Loránd Tudományegyetem, Budapest, Hungary, 2007. [Google Scholar]
- Wood, C.A.; Baldridge, S. Volcano tectonics of the Western United States. In Volcanoes of North America; Wood, C.A., Kienle, J., Eds.; Cambridge Univ Press: Cambridge, UK, 1990; pp. 147–154. [Google Scholar]
- Karátson, D.; Telbisz, T.; Singer, B.S. Late-stage volcano geomorphic evolution of the Pleistocene San Francisco Mountain, Arizona (USA), based on high-resolution DEM analysis and 40Ar/39Ar chronology. Bull. Volcanol. 2010, 72, 833–846. [Google Scholar] [CrossRef]
- Wolfe, E.W.; Ulrich, G.E.; Holm, R.F.; Moore, R.B.; Newhall, C.G. Geologic map of the central part of the San Francisco Volcanic Field, north-central Arizona. Misc. F. Stud. Map 1987. [Google Scholar] [CrossRef]
- Tanaka, K.L.; Shoemaker, E.M.; Ulrich, G.E.; Wolfe, E.W. Migration of Volcanism in the San-Francisco Volcanic Field, Arizona. Geol. Soc. Am. Bull. 1986, 97, 129–141. [Google Scholar] [CrossRef]
- Del Pozzo, A.L.M. Monogenetic vulcanism in sierra Chichinautzin, Mexico. Bull. Volcanol. 1982, 45, 9–24. [Google Scholar] [CrossRef]
- Siebe, C.; Rodríguez-Lara, V.; Schaaf, P.; Abrams, M. Radiocarbon ages of Holocene Pelado, Guespalapa and Chichinautzin scoria cones, south of Mexico city: Implications for archaeology and future hazards. Bull. Volcanol. 2004, 66, 203–225. [Google Scholar] [CrossRef]
- Agustín-Flores, J.; Siebe, C.; Guilbaud, M.N. Geology and geochemistry of Pelagatos, Cerro del Agua, and Dos Cerros monogenetic volcanoes in the Sierra Chichinautzin Volcanic Field, south of México City. J. Volcanol. Geotherm. Res. 2011, 201, 143–162. [Google Scholar] [CrossRef]
- Lorenzo-Merino, A.; Guilbaud, M.N.; Roberge, J. The violent Strombolian eruption of 10 ka Pelado shield volcano, Sierra Chichinautzin, Central Mexico. Bull. Volcanol. 2018, 80, 27. [Google Scholar] [CrossRef]
- Guilbaud, M.N.; Arana-Salinas, L.; Siebe, C.; Barba-Pingarrón, L.A.; Ortiz, A. Volcanic stratigraphy of a high-altitude Mammuthus columbi (Tlacotenco, Sierra Chichinautzin), Central México. Bull. Volcanol. 2015, 77, 17. [Google Scholar] [CrossRef]
- Siebe, C.; Arana-Salinas, L.; Abrams, M. Geology and radiocarbon ages of Tláloc, Tlacotenco, Cuauhtzin, Hijo del Cuauhtzin, Teuhtli, and Ocusacayo monogenetic volcanoes in the central part of the Sierra Chichinautzin, México. J. Volcanol. Geotherm. Res. 2005, 141, 225–243. [Google Scholar] [CrossRef]
- Wallace, P.J.; Carmichael, I.S.E. Quaternary volcanism near the Valley of Mexico: Implications for subduction zone magmatism and the effects of crustal thickness variations on primitive magma compositions. Contrib. Mineral. Petrol. 1999, 135, 291–314. [Google Scholar] [CrossRef]
- Schaaf, P.; Stimac, J.; Siebe, C.; Macías, J.L. Geochemical evidence for mantle origin and crustal processes in volcanic rocks from Popocatépetl and surrounding monogenetic volcanoes, central Mexico. J. Petrol. 2005, 46, 1243–1282. [Google Scholar] [CrossRef]
- Siebe, C. Age and archaeological implications of Xitle volcano, southwestern Basin of Mexico-City. J. Volcanol. Geotherm. Res. 2000, 104, 45–64. [Google Scholar] [CrossRef]
- Arce, J.L.; Layer, P.W.; Lassiter, J.C.; Benowitz, J.A.; Macías, J.L.; Ramírez-Espinosa, J. 40Ar/39Ar dating, geochemistry, and isotopic analyses of the quaternary Chichinautzin volcanic field, south of Mexico City: Implications for timing, eruption rate, and distribution of volcanism. Bull. Volcanol. 2013, 75, 774. [Google Scholar] [CrossRef]
- Vivó Vázquez, G. Variabilidad Geomorfológica de los Conos de Escoria de la Porción Centro-Oriental de la Sierra Chichinautzin a Partir de Modelos Digitales de Elevación; Universidad Nacional Autónoma de México: Mexico City, Mexico, 2017. [Google Scholar]
- Arce, J.L.; Muñoz-Salinas, E.; Castillo, M.; Salinas, I. The ~2000 yr BP Jumento volcano, one of the youngest edifices of the Chichinautzin Volcanic Field, Central Mexico. J. Volcanol. Geotherm. Res. 2015, 308, 30–38. [Google Scholar] [CrossRef]
- Jolivet, L.; Faccenna, C.; Huet, B.; Labrousse, L.; Le Pourhiet, L.; Lacombe, O.; Lecomte, E.; Burov, E.; Denèle, Y.; Brun, J.P.; et al. Aegean tectonics: Strain localisation, slab tearing and trench retreat. Tectonophysics 2013, 597–598, 1–33. [Google Scholar] [CrossRef]
- Özpolat, E.; Yıldırım, C.; Görüm, T.; Gosse, J.C.; Şahiner, E.; Sarıkaya, M.A.; Owen, L.A. Three-dimensional control of alluvial fans by rock uplift in an extensional regime: Aydın Range, Aegean extensional province. Sci. Rep. 2022, 12, 15306. [Google Scholar] [CrossRef]
- Hamilton, W.J.; Strickland, H.E. On the Geology of Western Part of Asia Minor. Trans. Geol. Soc. London 1841, I, 81. [Google Scholar] [CrossRef]
- Washington, H.S. The Volcanoes of the Kula Basin in Lydia; University of Leipzig: Leipzig, Germany, 1893. [Google Scholar]
- Erinç, S. Kula ve Adala arasinda gene, volkan reliyefi. Istanbul Üniversitesi Cograf. Enstitüsü Derg. 1970, 9, 7–32. [Google Scholar]
- Bunbury, J.M.; Hall, L.; Anderson, G.J.; Stannard, A. The determination of fault movement history from the interaction of local drainage with volcanic episodes. Geol. Mag. 2001, 138, 185–192. [Google Scholar] [CrossRef]
- Westaway, R.; Guillou, H.; Yurtmen, S.; Beck, A.; Bridgland, D.; Demir, T.; Scaillet, S.; Rowbotham, G. Late Cenozoic uplift of western Turkey: Improved dating of the Kula Quaternary volcanic field and numerical modelling of the Gediz River terrace staircase. Glob. Planet. Chang. 2006, 51, 131–171. [Google Scholar] [CrossRef]
- Westaway, R.; Pringle, M.; Yurtmen, S.; Demir, T.; Bridgland, D.; Rowbotham, G.; Maddy, D. Pliocene and Quaternary regional uplift in western Turkey: The Gediz River terrace staircase and the volcanism at Kula. Tectonophysics 2004, 391, 121–169. [Google Scholar] [CrossRef]
- Ulusoy, İ.; Sarıkaya, M.A.; Schmitt, A.K.; Şen, E.; Danišík, M.; Gümüş, E. Volcanic eruption eye-witnessed and recorded by prehistoric humans. Quat. Sci. Rev. 2019, 212, 187–198. [Google Scholar] [CrossRef]
- Richardson-Bunbury, J.M. The Kula Volcanic Field, western Turkey: The development of a Holocene alkali basalt province and the adjacent normal-faulting graben. Geol. Mag. 1996, 133, 275–283. [Google Scholar] [CrossRef]
- Şen, E.; Erturaç, M.K.; Gümüş, E. Quaternary Monogenetic Volcanoes Scattered on a Horst: The Bountiful Landscape of Kula. World Geomorphol. Landscapes 2019, 577–588. [Google Scholar] [CrossRef]
- Şen, E.; Aydar, E.; Bayhan, H.; Gourgaud, A. Volcanological characteristics of alkaline basalt and pyroclastic deposits, Kula volcanoes, western Anatolia. Yerbilimleri 2014, 35, 219–252. [Google Scholar]
- Accueil|Craig. Available online: https://www.craig.fr/ (accessed on 29 April 2021).
- 2011_Site_Puy_De_Dome_Lidarverne-Fichiers-Drive Opendata du CRAIG. Available online: https://drive.opendata.craig.fr/s/opendata?path=%2Flidar%2Fautres_zones%2F2011_site_puy_de_dome_lidarverne (accessed on 27 April 2021).
- TNM Download v2. Available online: https://apps.nationalmap.gov/downloader/#/ (accessed on 20 August 2022).
- 1 Meter Digital Elevation Models (DEMs)-USGS National Map 3DEP Downloadable Data Collection|USGS Science Data Catalog. Available online: https://data.usgs.gov/datacatalog/data/USGS:77ae0551-c61e-4979-aedd-d797abdcde0e (accessed on 20 August 2022).
- 1/3rd Arc-Second Digital Elevation Models (DEMs)-USGS National Map 3DEP Downloadable Data Collection|USGS Science Data Catalog. Available online: https://data.usgs.gov/datacatalog/data/USGS:3a81321b-c153-416f-98b7-cc8e5f0e17c3 (accessed on 20 August 2022).
- Instituto Nacional de Estadística y Geografía (INEGI). Available online: https://www.inegi.org.mx/ (accessed on 29 September 2022).
- Hasenaka, T.; Carmichael, I.S.E. The cinder cones of Michoacán-Guanajuato, central Mexico: Their age, volume and distribution, and magma discharge rate. J. Volcanol. Geotherm. Res. 1985, 25, 105–124. [Google Scholar] [CrossRef]
- Karátson, D.; Németh, K.; Székely, B.; Ruszkiczay-Rüdiger, Z.; Pécskay, Z. Incision of a river curvature due to exhumed Miocene volcanic landforms: Danube Bend, Hungary. Int. J. Earth Sci. 2006, 95, 929–944. [Google Scholar] [CrossRef]
- Székely, B.; Karátson, D. DEM-based morphometry as a tool for reconstructing primary volcanic landforms: Examples from the Börzsöny Mountains, Hungary. Geomorphology 2004, 63, 25–37. [Google Scholar] [CrossRef]
- Székely, B.; Hampton, S.J. DEM-aided volcanic reconstruction and collapse recognition of degraded Miocene volcanic edifices: A case history of Lyttelton Volcano, New Zealand. Geophys. Res. Abstr. 2007, 9, 10295. [Google Scholar]
- Kuhl, F.P.; Giardina, C.R. Elliptic Fourier features of a closed contour. Comput. Graph. Image Process. 1982, 18, 236–258. [Google Scholar] [CrossRef]
- Zarazúa-Carbajal, M.C.; De la Cruz-Reyna, S. Morpho-chronology of monogenetic scoria cones from their level contour curves. Applications to the Chichinautzin monogenetic field, Central Mexico. J. Volcanol. Geotherm. Res. 2020, 407, 107093. [Google Scholar] [CrossRef]
- Grieve, S.W.D. Spatial-efd: A spatial-aware implementation of elliptical Fourier analysis. J. Open Source Softw. 2017, 2, 189. [Google Scholar] [CrossRef]
- Vörös, F.; de van Vries, B.W.; Karátson, D.; Székely, B. DTM-based morphometric analysis of scoria cones of the Chaîne des Puys (France)—the classic and a new approach. Remote Sens. 2021, 13, 1983. [Google Scholar] [CrossRef]
- Vörös, F.; Székely, B. High-resolution DTM-based estimation of geomorphometric parameters of selected putative martian scoria cones. Icarus 2022, 377, 114923. [Google Scholar] [CrossRef]
- Bemis, K.; Walker, J.; Borgia, A.; Turrin, B.; Neri, M.; Swisher, C. The growth and erosion of cinder cones in Guatemala and El Salvador: Models and statistics. J. Volcanol. Geotherm. Res. 2011, 201, 39–52. [Google Scholar] [CrossRef]
- Tibaldi, A. Morphology of pyroclastic cones and tectonics. J. Geophys. Res. Solid Earth 1995, 100, 24521–24535. [Google Scholar] [CrossRef]
- Becerra-Ramírez, R.; Dóniz-Páez, J.; González, E. Morphometric Analysis of Scoria Cones to Define the “Volcano-Type” of the Campo de Calatrava Volcanic Region (Central Spain). Land 2022, 11, 917. [Google Scholar] [CrossRef]
- Lowry, R. VassarStats: Website for Statistical Computation. Available online: http://vassarstats.net/ (accessed on 28 November 2022).




| Volcanic Field | Abbreviation | DTM Resolution | Number of Cones Studied | Number of Age Groups | Source of Age Grouping |
|---|---|---|---|---|---|
| San Francisco Volcanic Field | SFVF | 10 m/1 m | 283 | 5 | [7,15] |
| Chaîne des Puys | CdP | 5 m/0.5 m | 28 | 3 | This study |
| Sierra Chichinautzin | SC | 5 m | 126 | 3 | [27,29,30,31,34,35,37] |
| Kula Volcanic Field | KVF | 12.5 m | 64 | 2 | [40,41,42,43,44,45,46] |
| Age Group Pairs | SFVF | CdP | SC | KVF |
|---|---|---|---|---|
| 1-2 | 0.0183 | 0.0238 | 0.0214 | 0.0001 |
| 1-3 | 0.0036 | 0.008 | <0.0001 | |
| 1-4 | <0.0001 | |||
| 1-5 | 0.0001 | |||
| 2-3 | 0.0488 | 0.7339 | <0.0001 | |
| 2-4 | <0.0001 | |||
| 2-5 | <0.0001 | |||
| 3-4 | 0.0366 | |||
| 3-5 | 0.1052 | |||
| 4-5 | 0.9203 |
| Age Group Pairs | sc_1 | cdp_1 | kvf_1 | cdp_2 | cdp_3 | sc_2 | kvf_2 | sfvf_1 | sfvf_2 | sc_3 | sfvf_3 | sfvf_4 | sfvf_5 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| sc_1 | - | 0.0455 | 0.05118 | 0.00096 | 0.00052 | 0.0214 | <0.00001 | <0.00001 | <0.00001 | <0.0001 | <0.00001 | <0.00001 | <0.00001 |
| cdp_1 | 0.0455 | - | 1 | 0.0238 | 0.008 | 0.44726 | <0.00001 | 0.00036 | <0.00001 | 0.03752 | <0.00001 | <0.00001 | <0.00001 |
| kvf_1 | 0.05118 | 1 | - | 0.11876 | 0.09492 | 0.65272 | 0.0001 | 0.00096 | <0.00001 | 0.16152 | <0.00001 | <0.00001 | <0.00001 |
| cdp_2 | 0.00096 | 0.0238 | 0.11876 | - | 0.7339 | 0.00014 | 0.02444 | 0.01174 | 0.00008 | 0.2113 | 0.00028 | <0.00001 | 0.00006 |
| cdp_3 | 0.00052 | 0.008 | 0.09492 | 0.7339 | - | <0.00001 | 0.0048 | 0.00496 | <0.00001 | 0.09296 | <0.00001 | <0.00001 | <0.00001 |
| sc_2 | 0.0214 | 0.44726 | 0.65272 | 0.00014 | <0.00001 | - | <0.00001 | <0.00001 | <0.00001 | <0.00001 | <0.00001 | <0.00001 | <0.00001 |
| kvf_2 | <0.00001 | <0.00001 | 0.0001 | 0.02444 | 0.0048 | <0.00001 | - | 0.1443 | <0.00001 | <0.00001 | <0.00001 | <0.00001 | <0.00001 |
| sfvf_1 | <0.00001 | 0.00036 | 0.00096 | 0.01174 | 0.00496 | <0.00001 | 0.1443 | - | 0.0183 | <0.00001 | 0.0036 | <0.0001 | 0.0001 |
| sfvf_2 | <0.00001 | <0.00001 | <0.00001 | 0.00008 | <0.00001 | <0.00001 | <0.00001 | 0.0183 | - | <0.00001 | 0.0488 | <0.0001 | <0.0001 |
| sc_3 | <0.0001 | 0.03752 | 0.16152 | 0.2113 | 0.09296 | <0.00001 | <0.00001 | <0.00001 | <0.00001 | - | <0.00001 | <0.00001 | <0.00001 |
| sfvf_3 | <0.00001 | <0.00001 | <0.00001 | 0.00028 | <0.00001 | <0.00001 | <0.00001 | 0.0036 | 0.0488 | <0.00001 | - | 0.0366 | 0.1052 |
| sfvf_4 | <0.00001 | <0.00001 | <0.00001 | <0.00001 | <0.00001 | <0.00001 | <0.00001 | <0.0001 | <0.0001 | <0.00001 | 0.0366 | - | 0.9203 |
| sfvf_5 | <0.00001 | <0.00001 | <0.00001 | 0.00006 | <0.00001 | <0.00001 | <0.00001 | 0.0001 | <0.0001 | <0.00001 | 0.1052 | 0.9203 | - |
| without SC | with SC | |
|---|---|---|
| p value | 0.0107 | 0.0091 |
| U value | 1 | 4 |
| sample size (A/B) | 5/5 | 7/6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vörös, F.; van Wyk de Vries, B.; Guilbaud, M.-N.; Görüm, T.; Karátson, D.; Székely, B. DTM-Based Comparative Geomorphometric Analysis of Four Scoria Cone Areas—Suggestions for Additional Approaches. Remote Sens. 2022, 14, 6152. https://doi.org/10.3390/rs14236152
Vörös F, van Wyk de Vries B, Guilbaud M-N, Görüm T, Karátson D, Székely B. DTM-Based Comparative Geomorphometric Analysis of Four Scoria Cone Areas—Suggestions for Additional Approaches. Remote Sensing. 2022; 14(23):6152. https://doi.org/10.3390/rs14236152
Chicago/Turabian StyleVörös, Fanni, Benjamin van Wyk de Vries, Marie-Noëlle Guilbaud, Tolga Görüm, Dávid Karátson, and Balázs Székely. 2022. "DTM-Based Comparative Geomorphometric Analysis of Four Scoria Cone Areas—Suggestions for Additional Approaches" Remote Sensing 14, no. 23: 6152. https://doi.org/10.3390/rs14236152
APA StyleVörös, F., van Wyk de Vries, B., Guilbaud, M.-N., Görüm, T., Karátson, D., & Székely, B. (2022). DTM-Based Comparative Geomorphometric Analysis of Four Scoria Cone Areas—Suggestions for Additional Approaches. Remote Sensing, 14(23), 6152. https://doi.org/10.3390/rs14236152







