A Calibrated Lunar Microwave Radiative Transfer Model Based on Satellite Observations
Abstract
1. Introduction
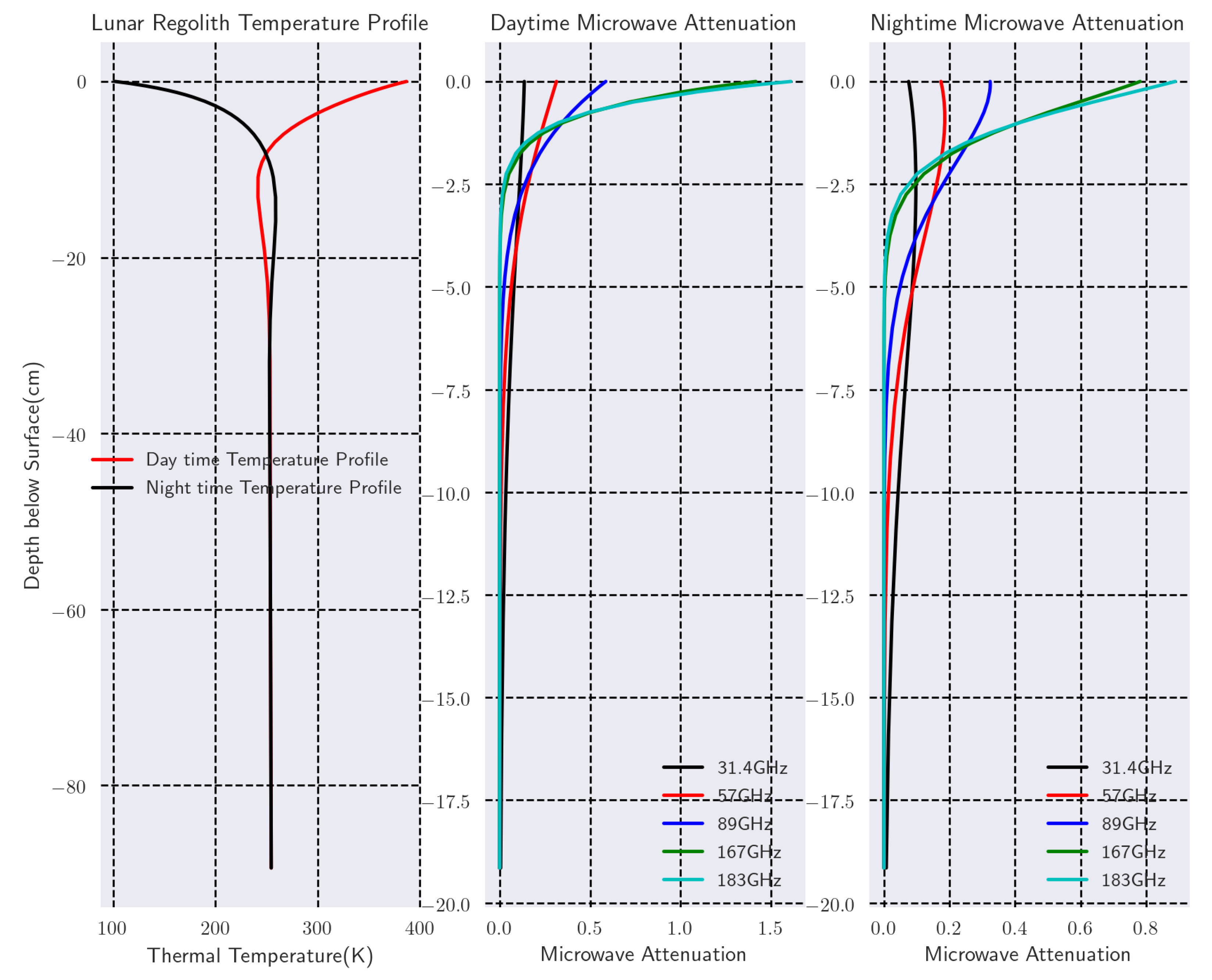
2. Lunar Microwave Radiative Transfer Model
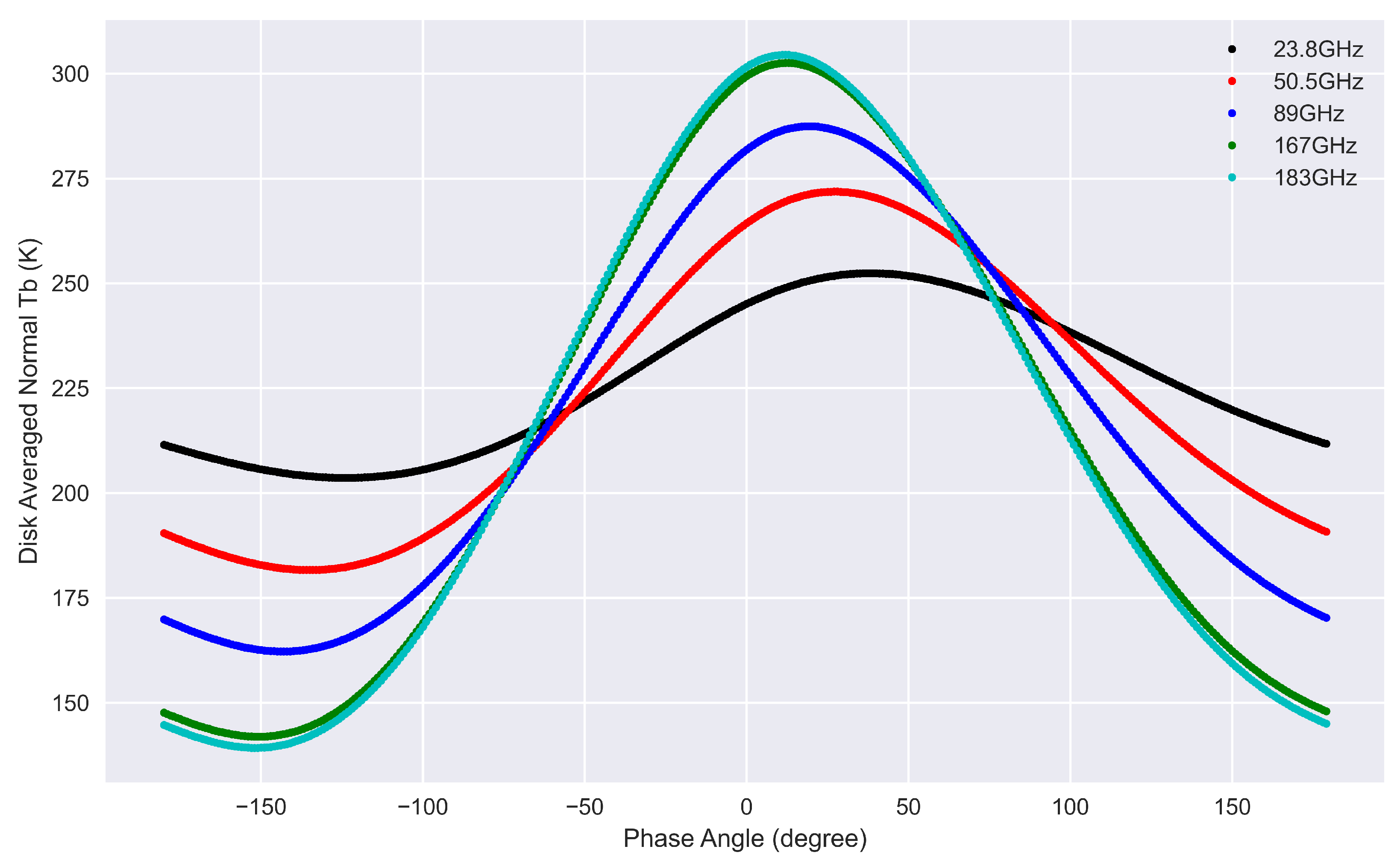
3. Lunar Disk Microwave Brightness Temperature Simulations
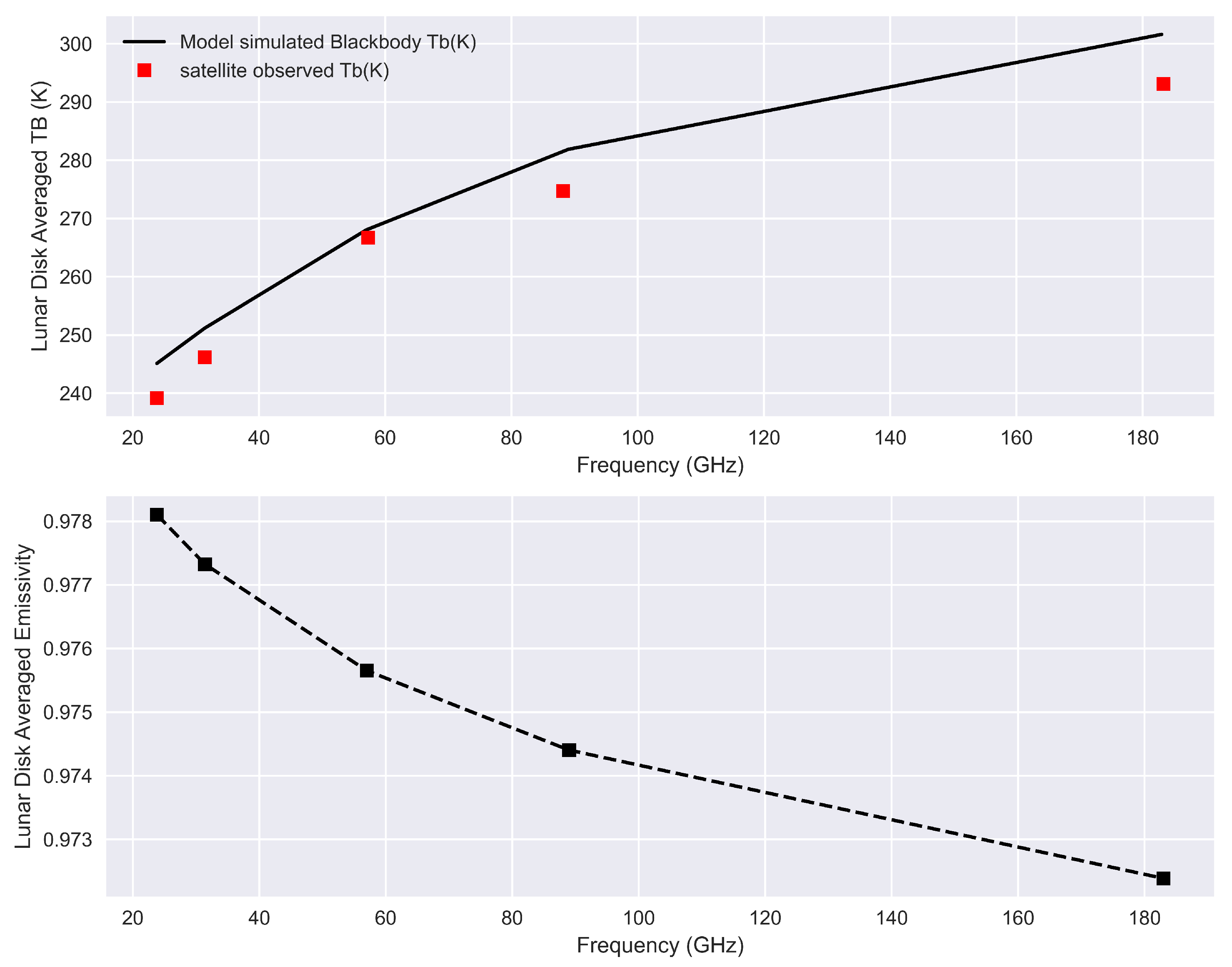
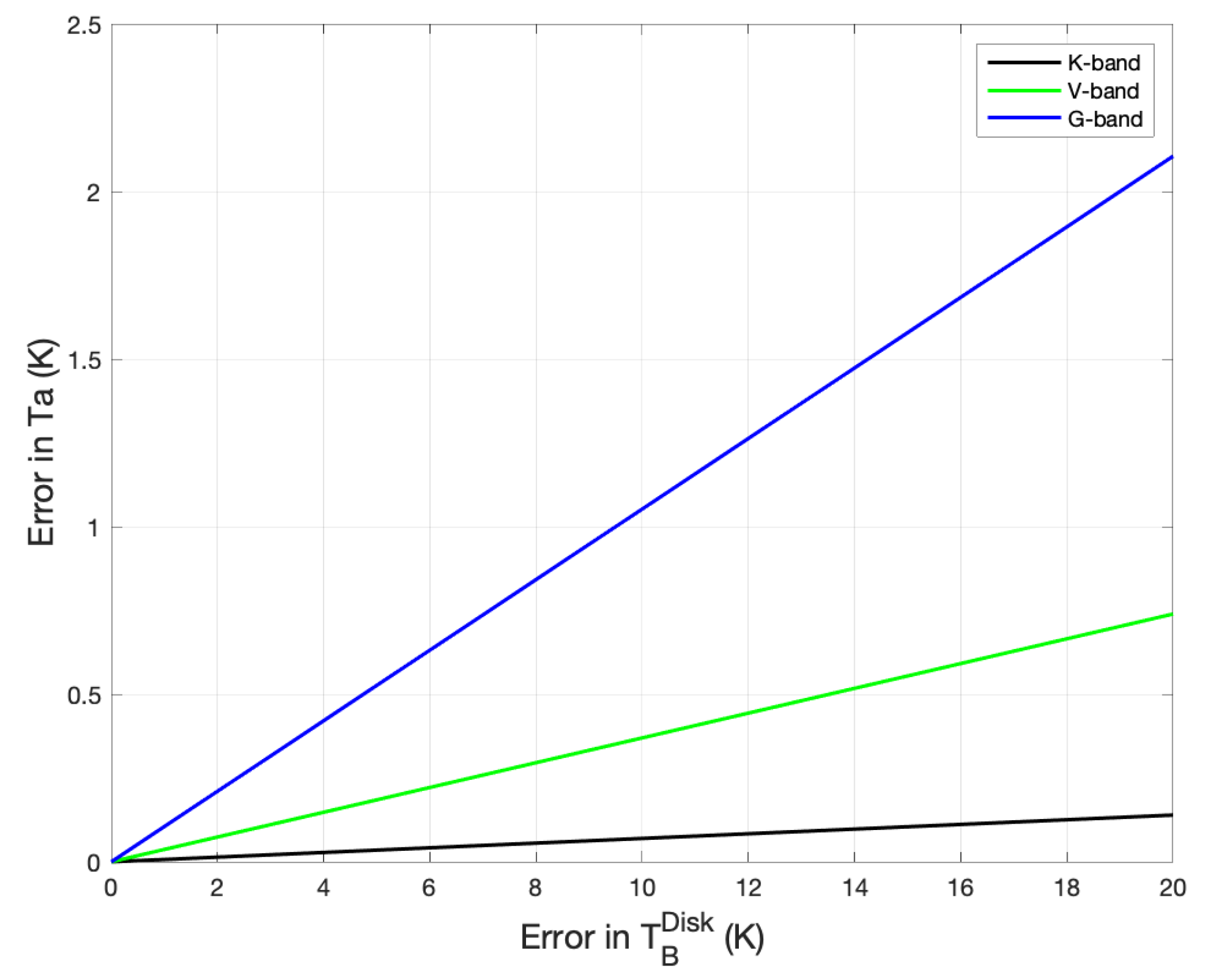
4. Model Calibration with Satellite Observations
5. Model Performance Evaluation with the Satellite Observations
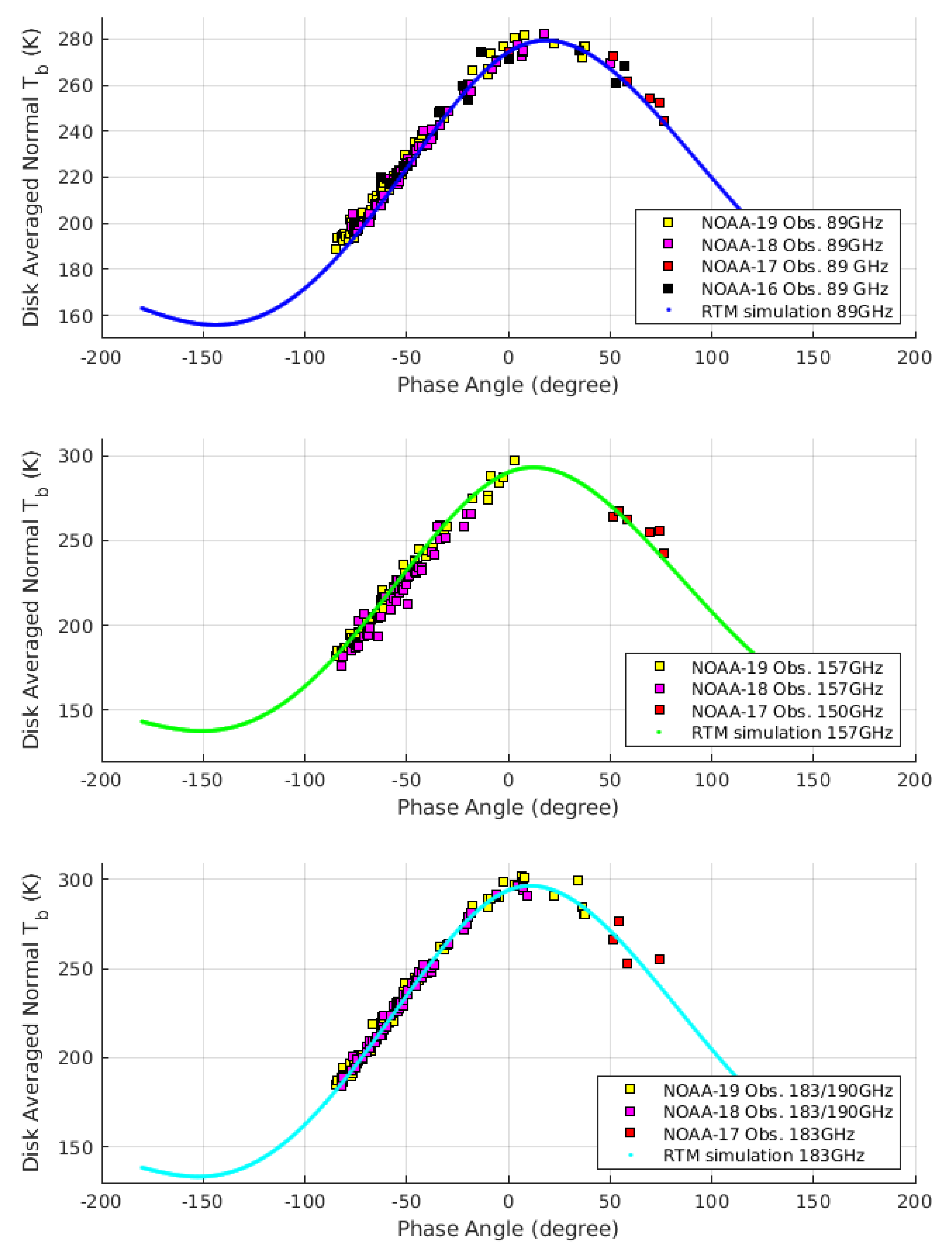
5.1. AMSU-A
5.2. AMSU-B and MHS
6. Conclusions and Discussions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yang, H.; Zhou, J.; Weng, F.; Sun, N.; Anderson, K.; Liu, Q.; Kim, E.J. Developing vicarious calibration for microwave sounding instruments by using lunar radiation. IEEE Geosci. Remote Sens. 2018, 56, 6723–6733. [Google Scholar] [CrossRef]
- Burgdorf, M.; Buehler, S.A.; Lang, T.; Michel, S.; Hans, I. The Moon as a photometric calibration standard for microwave sensors. Atmos. Meas. Tech. 2016, 9, 3467–3475. [Google Scholar] [CrossRef]
- Hu, G.; Zheng, Y.; Xu, A.; Tang, Z. Microwave Brightness Temperature of the Moon: The Possibility of Setting a Calibration Source of the Lunar Surface. IEEE Geosci. Remote Sens. Lett. 2016, 13, 182–186. [Google Scholar] [CrossRef]
- Williams, J.-P.; Paige, D.A.; Greenhagen, B.T.; Sefton-Nash, E. The global surface temperatures of the Moon as measured by the Diviner Lunar Radiometer Experiment. ICARUS 2016, 283, 300–325. [Google Scholar] [CrossRef]
- Hayne, P.O.; Bandfield, J.L.; Siegler, M.A.; Vasavada, A.R.; Ghent, R.R.; Williams, J.-P.; Paige, D.A. Global regolith thermophysical properties of the Moon from the Diviner Lunar Radiometer Experiment. J. Geophys. Res. Planets 2017, 122, 2371–2400. [Google Scholar] [CrossRef]
- Krotikov, V.D.; Troitskii, V.S. Radio Emission And Nature of The Moon. Soviet Phys. Uspekhi 1964, 6, 841–871. [Google Scholar] [CrossRef]
- Keihm, S.J.; Cutts, J.A. Vertical-Structure Effects on Planetary Microwave Brightness Temperature Measurements: Applications to the Lunar Regolith. ICARUS 1981, 48, 201–229. [Google Scholar] [CrossRef]
- Keihm, S.J. Effects of Subsurface Volume Scattering on the Lunar Microwave Brightness Temperature Spectrum. ICARUS 1982, 52, 570–584. [Google Scholar] [CrossRef]
- Keihm, S.J. Interpretation of the Lunar Microwave Brightness Temperature Spectrum: Feasibility of Orbital Heat Flow Mapping. ICARUS 1984, 60, 568–589. [Google Scholar] [CrossRef]
- Liu, N.; Jin, Y. Average Brightness Temperature of Lunar Surface for Calibration of Multichannel Millimeter-Wave Radiometer From 89 to 183 GHz and Data Validation. IEEE Trans. Geosci. Remote. Sens. 2021, 59, 1345–1354. [Google Scholar] [CrossRef]
- Hu, G.-P.; Chan, K.L.; Zheng, Y.-C.; Tsang, K.T.; Xu, A.-A. Comparison and evaluation of the Chang’E microwave radiometer data based on theoretical computation of brightness temperatures at the Apollo 15 and 17 sites. ICARUS 2017, 294, 72–80. [Google Scholar] [CrossRef]
- Yang, H.; Zhou, J.; Sun, N.; Liu, Q.; Leslie, R.; Anderson, K.; Kim, E.; Lyu, C.-H.; Smith, C.; McCormick, L. 2-D Lunar Microwave Radiance Observations From the NOAA-20 ATMS. IEEE Geosci. Remote Sens. Lett. 2021, 18, 2021–2024. [Google Scholar] [CrossRef]
- Piddington, H.; Minnett, H.C. Microwave thermal radiation from the Moon. Aust. J. Sci. Res. Ser. A 1949, 2, 63–77. [Google Scholar] [CrossRef]
- Jaeger, J.C. The Surface Temperature of the Moon; Provided by the NASA Astrophysics Data System; CSIRO: Canberra, Australia, 1952. [Google Scholar]
- Yang, H.; Burgdorf, M. A Study of Lunar Microwave Radiation Based on Satellite Observations. Remote Sens. 2020, 12, 1129. [Google Scholar] [CrossRef]
- WMO OSCAR. Space-Based Capabilities-Instruments. 2016. Available online: http://www.wmo-sat.info/oscar/instruments (accessed on 29 August 2022).


| Sat. | Instr. | Frequency (GHz) | Mean Error (K) | Mean Error (K) | Std. (K) |
|---|---|---|---|---|---|
| N18 | AMSU-A | 23.8 | 1.87 | 20.44 | 4.12 |
| N18 | AMSU-A | 31.4 | −6.32 | 12.16 | 6.32 |
| N18 | AMSU-A | 57 | −8.74 | 9.67 | 8.03 |
| N18 | AMSU-A | 89 | −9.07 | 9.38 | 4.35 |
| Sat. | Instr. | Frequency (GHz) | Mean Error (K) | Mean Error (K) | Std. (K) |
|---|---|---|---|---|---|
| N15 | AMSU-B | 89 | −0.7 | 18.5 | 9.2 |
| N16 | AMSU-B | 89 | −2.2 | 16.4 | 6.7 |
| N17 | AMSU-B | 89 | 11 | 30.3 | 6.4 |
| N18 | MHS | 89 | 4.9 | 22.5 | 3.6 |
| N19 | MHS | 89 | 6.4 | 24.1 | 4.3 |
| M-A | MHS | 89 | 6.4 | 25.8 | 5.1 |
| MB | MHS | 89 | 2.8 | 22.2 | 3.4 |
| MC | MHS | 89 | 11.3 | 30.9 | 7.9 |
| N15 | AMSU-B | 150 | 17 | 36.8 | 22.2 |
| N16 | AMSU-B | 150 | 23.2 | 42 | 10 |
| N17 | AMSU-B | 150 | 9.6 | 28.8 | 22.4 |
| N18 | MHS | 157 | −6.6 | 11.2 | 7.2 |
| N19 | MHS | 157 | 1.6 | 19.4 | 5.2 |
| MA | MHS | 157 | −2.7 | 16.8 | 9.1 |
| MB | MHS | 157 | −6.1 | 13.2 | 4.2 |
| MC | MHS | 157 | −2 | 17.6 | 8.7 |
| N15 | AMSU-B | 183 | 10.2 | 30.2 | 16 |
| N16 | AMSU-B | 183 | 10.4 | 28.6 | 10.4 |
| N17 | AMSU-B | 183 | −2.2 | 16.8 | 17.3 |
| N18 | MHS | 183/190 | 4.4 | 22.1 | 4.1 |
| N19 | MHS | 183/190 | 2.3 | 20.1 | 6.6 |
| MA | MHS | 183/190 | 4.7 | 24.1 | 6.1 |
| MB | MHS | 183/190 | 0.7 | 19.9 | 3.5 |
| MC | MHS | 183/190 | 13.3 | 32.8 | 13.7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, H.; Burgdorf, M. A Calibrated Lunar Microwave Radiative Transfer Model Based on Satellite Observations. Remote Sens. 2022, 14, 5501. https://doi.org/10.3390/rs14215501
Yang H, Burgdorf M. A Calibrated Lunar Microwave Radiative Transfer Model Based on Satellite Observations. Remote Sensing. 2022; 14(21):5501. https://doi.org/10.3390/rs14215501
Chicago/Turabian StyleYang, Hu, and Martin Burgdorf. 2022. "A Calibrated Lunar Microwave Radiative Transfer Model Based on Satellite Observations" Remote Sensing 14, no. 21: 5501. https://doi.org/10.3390/rs14215501
APA StyleYang, H., & Burgdorf, M. (2022). A Calibrated Lunar Microwave Radiative Transfer Model Based on Satellite Observations. Remote Sensing, 14(21), 5501. https://doi.org/10.3390/rs14215501







