New Advances of the Multiscale Approach for the Analyses of InSAR Ground Measurements: The Yellowstone Caldera Case-Study
Abstract
1. Introduction
2. Materials and Methods
2.1. Harmonic Deformation Field
2.2. Homogeneous Deformation Field
2.3. Multiridge Method
2.4. ScalFun Method
2.5. Multiridge and ScalFun Methods for General Deformation Sources
2.6. Total Horizontal Derivative Technique
2.7. Simulated Ground Deformation Fields
2.8. SAR Data at Yellowstone Caldera
3. Results
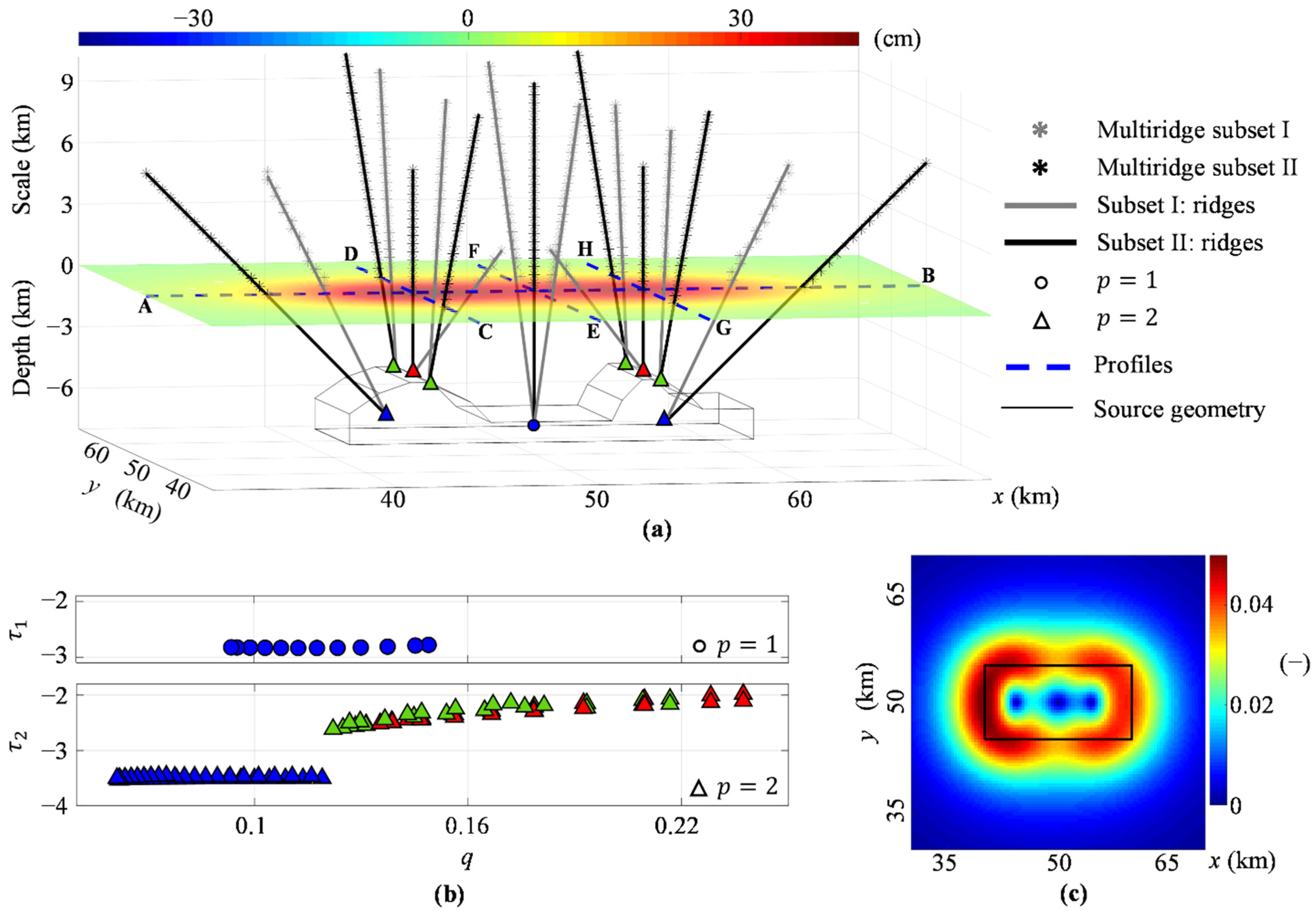
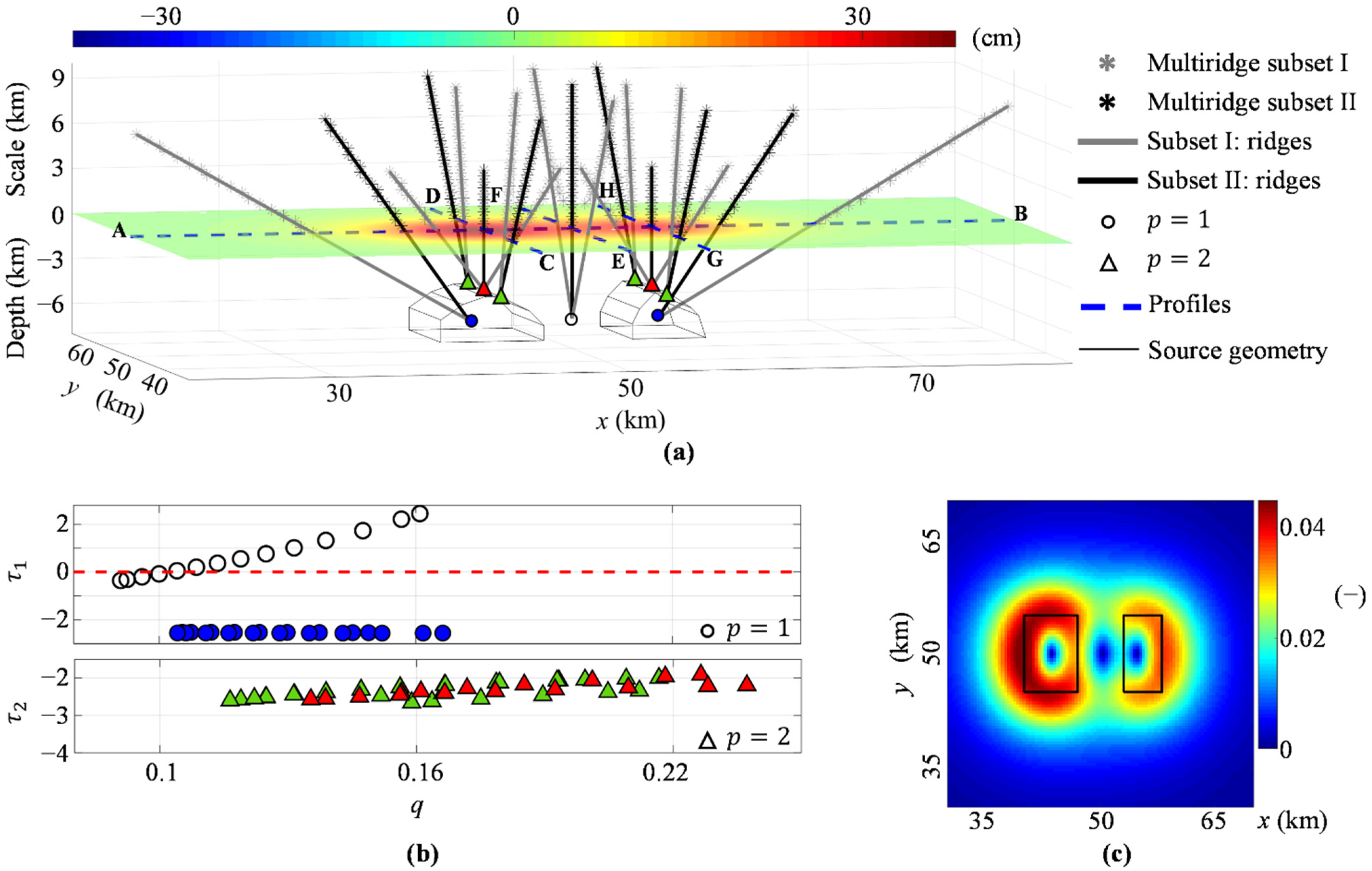
3.1. Application to Simulated Deformation Fields
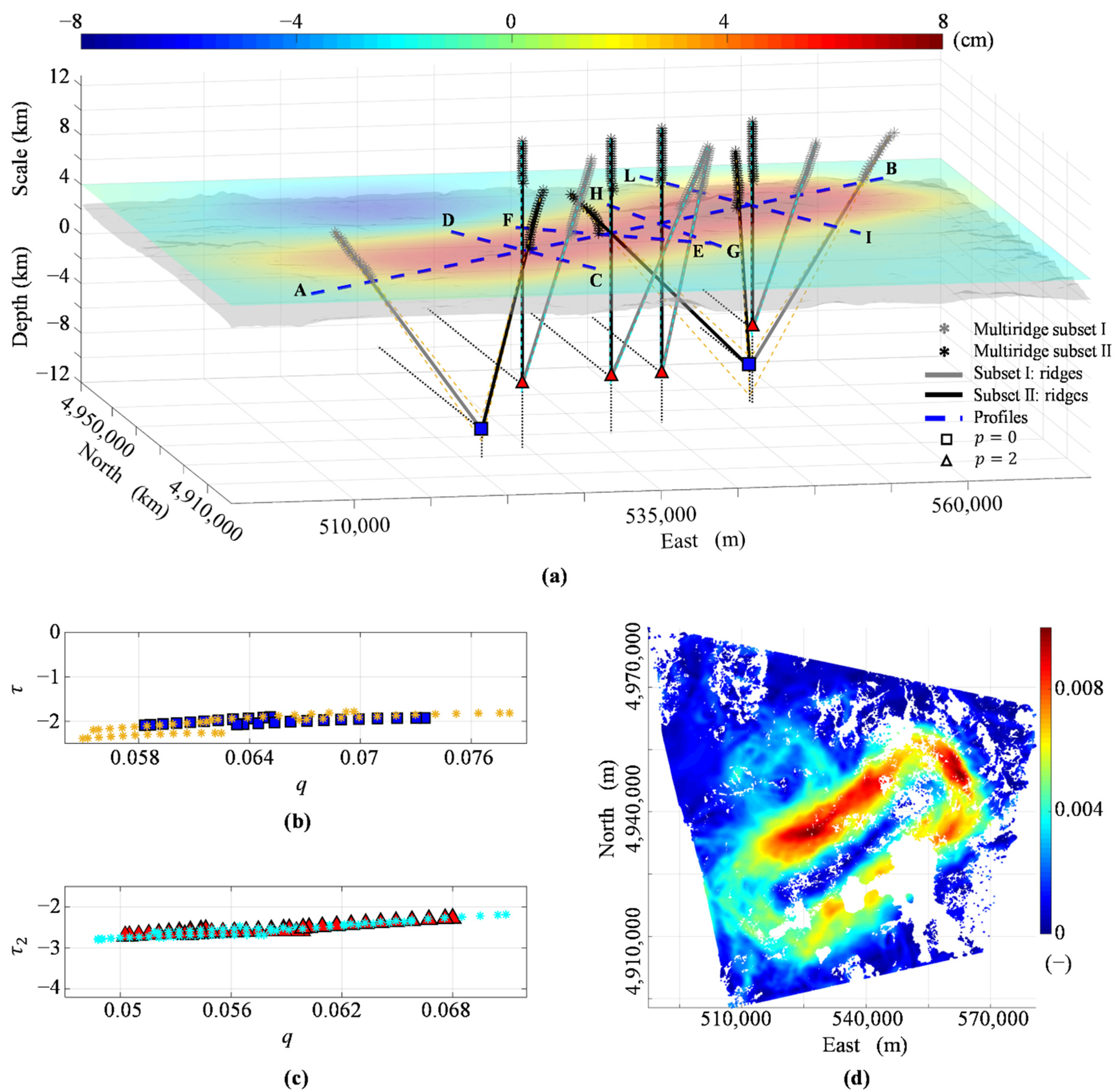
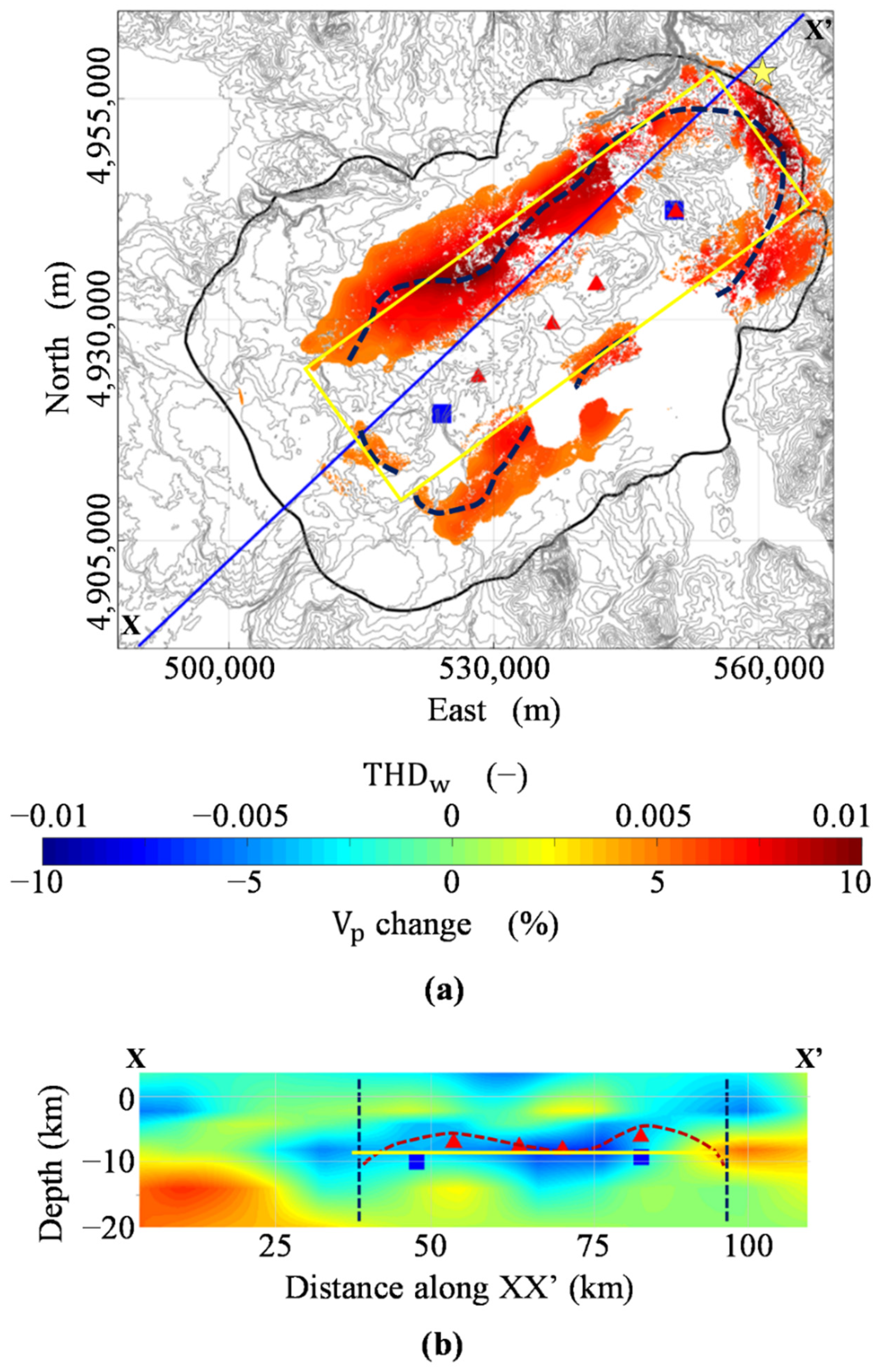
3.2. Application to Real Case: 2005–2007 Uplift Episode at Yellowstone Caldera
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Farrell, J.; Smith, R.B.; Husen, S.; Diehl, T. Tomography from 26 years of seismicity revealing that the spatial extent of the Yellowstone crustal magma reservoir extends well beyond the Yellowstone caldera. Geophys. Res. Lett. 2014, 41, 3068–3073. [Google Scholar] [CrossRef]
- Fedi, M.; Cella, F.; D’Antonio, M.; Florio, G.; Paoletti, V.; Morra, V. Gravity modeling finds a large magma body in the deep crust below the Gulf of Naples, Italy. Sci. Rep. 2018, 8, 8229. [Google Scholar] [CrossRef] [PubMed]
- Vitale, A.; Fedi, M. Self-constrained inversion of potential fields through a 3D depth weighting. Geophysics 2020, 85, G143–G156. [Google Scholar] [CrossRef]
- Castaldo, R.; Tizzani, P.; Solaro, G. Inflating Source Imaging and Stress/Strain Field Analysis at Campi Flegrei Caldera: The 2009–2013 Unrest Episode. Remote Sens. 2021, 13, 2298. [Google Scholar] [CrossRef]
- Rodríguez-Molina, S.; González, P.J.; Charco, M.; Negredo, A.M.; Schmidt, D.A. Time-Scales of Inter-Eruptive Volcano Uplift Signals: Three Sisters Volcanic Center, Oregon (United States). Front. Earth Sci. 2021, 8, 577588. [Google Scholar] [CrossRef]
- Tizzani, P.; Battaglia, M.; Castaldo, R.; Pepe, A.; Zeni, G.; Lanari, R. Magma and fluid migration at Yellowstone Caldera in the last three decades inferred from InSAR, leveling, and gravity measurements. J. Geophys. Res. Solid Earth 2015, 120, 2627–2647. [Google Scholar] [CrossRef]
- Pepe, S.; De Siena, L.; Barone, A.; Castaldo, R.; D’Auria, L.; Manzo, M.; Casu, F.; Fedi, M.; Lanari, R.; Bianco, F.; et al. Volcanic structures investigation through SAR and seismic interferometric methods: The 2011–2013 Campi Flegrei unrest episode. Remote Sens. Environ. 2019, 234, 111440. [Google Scholar] [CrossRef]
- Gola, G.; Barone, A.; Castaldo, R.; Chiodini, G.; D’Auria, L.; García-Hernández, R.; Pepe, S.; Solaro, G.; Tizzani, P. A Novel Multidisciplinary Approach for the Thermo-Rheological Study of Volcanic Areas: The Case Study of Long Valley Caldera. J. Geophys. Res. Solid Earth 2021, 126, e2020JB020331. [Google Scholar] [CrossRef]
- Gabriel, A.K.; Goldstein, R.M.; Zebker, H.A. Mapping small elevation changes over large areas: Differential radar interferometry. J. Geophys. Res. Earth Surf. 1989, 94, 9183–9191. [Google Scholar] [CrossRef]
- Massonnet, D.; Rossi, M.; Carmona, C.; Adragna, F.; Peltzer, G.; Feigl, K.; Rabaute, T. The displacement field of the Landers earthquake mapped by radar interferometry. Nature 1993, 364, 138–142. [Google Scholar] [CrossRef]
- Battaglia, M.; Cervelli, P.F.; Murray, J.R. dMODELS: A MATLAB software package for modeling crustal deformation near active faults and volcanic centers. J. Volcanol. Geotherm. Res. 2013, 254, 1–4. [Google Scholar] [CrossRef]
- Mogi, K. Relation between eruptions of various volcanoes and the deformations of ground surfaces around them. Bull. Earthq. Res. Institute Univ. Tokio 1958, 36, 99–134. [Google Scholar]
- Sun, R.J. Theoretical size of hydraulically induced horizontal fractures and corresponding surface uplift in an idealized medium. J. Geophys. Res. Earth Surf. 1969, 74, 5995–6011. [Google Scholar] [CrossRef]
- Geerstma, J.; van Opstal, K. A numerical technique for predicting subsidence above compacting reservoirs, based on the nucleus of strain concept. Verhandelingen Kon. Ned. Geol. Mijnbouwk. Gen. 1973, 28, 63–78. [Google Scholar]
- Okada, Y. Surface deformation due to shear and tensile faults in a half-space. Bull. Seismol. Soc. Am. 1985, 75, 1135–1154. [Google Scholar] [CrossRef]
- McTigue, D.F. Elastic stress and deformation near a finite spherical magma body: Resolution of the point source paradox. J. Geophys. Res. Earth Surf. 1987, 92, 12931–12940. [Google Scholar] [CrossRef]
- Yang, X.-M.; Davis, P.M.; Dieterich, J.H. Deformation from inflation of a dipping finite prolate spheroid in an elastic half-space as a model for volcanic stressing. J. Geophys. Res. Earth Surf. 1988, 93, 4249–4257. [Google Scholar] [CrossRef]
- Bonaccorso, A.; Davis, P.M. Models of ground deformation from vertical volcanic conduits with application to eruptions of Mount St. Helens and Mount Etna. J. Geophys. Res. Earth Surf. 1999, 104, 10531–10542. [Google Scholar] [CrossRef]
- Fialko, Y.; Khazan, Y.; Simons, M. Deformation due to a pressurized horizontal circular crack in an elastic half-space, with applications to volcano geodesy. Geophys. J. Int. 2001, 146, 181–190. [Google Scholar] [CrossRef]
- D’Auria, L.; Pepe, S.; Castaldo, R.; Giudicepietro, F.; Macedonio, G.; Ricciolino, P.; Tizzani, P.; Casu, F.; Lanari, R.; Manzo, M.; et al. Magma injection beneath the urban area of Naples: A new mechanism for the 2012–2013 volcanic unrest at Campi Flegrei caldera. Sci. Rep. 2015, 5, 13100. [Google Scholar] [CrossRef]
- Delgado, F.; Grandin, R. Dynamics of Episodic Magma Injection and Migration at Yellowstone Caldera: Revisiting the 2004–2009 Episode of Caldera Uplift with InSAR and GPS Data. J. Geophys. Res. Solid Earth 2021, 126, e2021JB022341. [Google Scholar] [CrossRef]
- Camacho, A.G.; Fernández, J.; Samsonov, S.V.; Tiampo, K.F.; Palano, M. 3D multi-source model of elastic volcanic ground deformation. Earth Planet. Sci. Lett. 2020, 547, 116445. [Google Scholar] [CrossRef]
- Castaldo, R.; Barone, A.; Fedi, M.; Tizzani, P. Multiridge Method for Studying Ground-Deformation Sources: Application to Volcanic Environments. Sci. Rep. 2018, 8, 13420. [Google Scholar] [CrossRef]
- Barone, A.; Fedi, M.; Tizzani, P.; Castaldo, R. Multiscale Analysis of DInSAR Measurements for Multi-Source Investigation at Uturuncu Volcano (Bolivia). Remote Sens. 2019, 11, 703. [Google Scholar] [CrossRef]
- Barone, A.; Fedi, M.; Pepe, S.; Solaro, G.; Tizzani, P.; Castaldo, R. Modeling the Deformation Sources in Volcanic Environments Through Multi-Scale Analysis of DInSAR Measurements. Front. Earth Sci. 2022, 10, 859479. [Google Scholar] [CrossRef]
- Blakely, J.R. The potential, Consequences of the Potential, Transformations. In Potential Theory in Gravity and Magnetic Applications; Cambridge University Press: Cambridge, UK, 1996. [Google Scholar]
- Olmsted, J.M.A. Advanced Calculus, 1st ed.; Prentice-Hall: Hoboken, NJ, USA, 1961. [Google Scholar]
- Fedi, M.; Florio, G.; Quarta, T.A. Multiridge analysis of potential fields: Geometric method and reduced Euler deconvolution. Geophysics 2009, 74, L53–L65. [Google Scholar] [CrossRef]
- Fedi, M. DEXP: A fast method to determine the depth and the structural index of potential fields sources. Geophysics 2007, 72, I1–I11. [Google Scholar] [CrossRef]
- Fedi, M.; Florio, G.; Paoletti, V. MHODE: A local-homogeneity theory for improved source-parameter estimation of potential fields. Geophys. J. Int. 2015, 202, 887–900. [Google Scholar] [CrossRef]
- Love, A.E.H. A Treatise on the Mathematical Theory of Elasticity, 2nd ed.; Cambridge University Press: Cambridge, UK, 1906. [Google Scholar]
- Gerovska, D.; Stavrev, P. Finite-difference Euler Deconvolution Algorithm Applied to the Interpretation of Magnetic Data from Northern Bulgaria. Pure Appl. Geophys. 2005, 162, 591–608. [Google Scholar] [CrossRef]
- Chang, W.-L.; Smith, R.B.; Wicks, C.; Farrell, J.M.; Puskas, C.M. Accelerated Uplift and Magmatic Intrusion of the Yellowstone Caldera, 2004 to 2006. Science 2007, 318, 952–956. [Google Scholar] [CrossRef]
- Chang, W.-L.; Smith, R.B.; Farrell, J.; Puskas, C.M. An extraordinary episode of Yellowstone caldera uplift, 2004-2010, from GPS and InSAR observations. Geophys. Res. Lett. 2010, 37, L23302. [Google Scholar] [CrossRef]
- Aly, M.H.; Cochran, E.S. Spatio-temporal evolution of Yellowstone deformation between 1992 and 2009 from InSAR and GPS observations. Bull. Volcanol. 2011, 73, 1407–1419. [Google Scholar] [CrossRef]
- Sadd, M.H. Elasticity Theory, Applications, and Numerics; Elsevier: Berkeley, CA, USA, 2005. [Google Scholar]
- Milano, M.; Fedi, M.; Fairhead, J.D. The deep crust beneath the Trans-European Suture Zone from a multiscale magnetic model. J. Geophys. Res. Solid Earth 2016, 121, 6276–6292. [Google Scholar] [CrossRef]
- Paoletti, V.; Milano, M.; Baniamerian, J.; Fedi, M. Magnetic Field Imaging of Salt Structures at Nordkapp Basin, Barents Sea. Geophys. Res. Lett. 2020, 47, e2020GL089026. [Google Scholar] [CrossRef]
- Ridsdill-Smith, T.A.; Dentith, M. The wavelet transform in aeromagnetic processing. Geophysics 1999, 64, 1003–1013. [Google Scholar] [CrossRef]
- Cella, F.; Fedi, M. High-Resolution Geophysical 3D Imaging for Archaeology by Magnetic and EM data: The Case of the Iron Age Settlement of Torre Galli, Southern Italy. Surv. Geophys. 2015, 36, 831–850. [Google Scholar] [CrossRef]
- Paoletti, V.; Fedi, M.; Florio, G. Magnetic Structure of the Ischia volcanic island, Southern Italy. Ann. Geophys. 2017, 60, 674. [Google Scholar] [CrossRef]
- Christiansen, R.L. The Quaternary and Pliocene Yellowstone Plateau Volcanic Field of Wyoming, Idaho, and Montana; USGS: Reston, VA, USA, 2001. [Google Scholar]
- Fournier, R.O. Geochemistry and Dynamics of the Yellowstone National Park Hydrothermal System. Annu. Rev. Earth Planet. Sci. 1989, 17, 13–53. [Google Scholar] [CrossRef]
- Berardino, P.; Fornaro, G.; Lanari, R.; Sansosti, E. A new algorithm for surface deformation monitoring based on small baseline differential SAR interferograms. IEEE Trans. Geosci. Remote Sens. 2002, 40, 2375–2383. [Google Scholar] [CrossRef]
- Rosen, P.A.; Hensley, S.; Gurrola, E.; Rogez, F.; Chan, S.; Martin, J.; Rodriguez, E. SRTM C-band topographic data: Quality assessments and calibration activities. In Proceedings of the IEEE 2001 International Geoscience and Remote Sensing Symposium (Cat. No.01CH37217), Sydney, NSW, Australia, 9–13 July 2001. [Google Scholar] [CrossRef]
- Rosen, P.A.; Hensley, S.; Joughin, I.R.; Li, F.K.; Madsen, S.N.; Rodriguez, E.; Goldstein, R.M. Synthetic aperture radar interferometry. Proc. IEEE 2000, 88, 333–382. [Google Scholar] [CrossRef]
- Yang, Y.; Pepe, A.; Manzo, M.; Bonano, M.; Liang, D.N.; Lanari, R. A simple solution to mitigate noise effects in time-redundant sequences of small baseline multi-look DInSAR interferograms. Remote Sens. Lett. 2013, 4, 609–618. [Google Scholar] [CrossRef]
- Pepe, A. Theory and Statistical Description of the Enhanced Multi-Temporal InSAR (E-MTInSAR) Noise-Filtering Algorithm. Remote Sens. 2019, 11, 363. [Google Scholar] [CrossRef]
- Pepe, A.; Yang, Y.; Manzo, M.; Lanari, R. Improved EMCF-SBAS Processing Chain Based on Advanced Techniques for the Noise-Filtering and Selection of Small Baseline Multi-Look DInSAR Interferograms. IEEE Trans. Geosci. Remote Sens. 2015, 53, 4394–4417. [Google Scholar] [CrossRef]
- Festa, D.; Bonano, M.; Casagli, N.; Confuorto, P.; De Luca, C.; Del Soldato, M.; Lanari, R.; Lu, P.; Manunta, M.; Manzo, M.; et al. Nation-wide mapping and classification of ground deformation phenomena through the spatial clustering of P-SBAS InSAR measurements: Italy case study. ISPRS J. Photogramm. Remote Sens. 2022, 189, 1–22. [Google Scholar] [CrossRef]
- Monterroso, F.; Antonioli, A.; Atzori, S.; de Luca, C.; Lanari, R.; Manunta, M.; Valerio, E.; Casu, F. New advances of the P-SBAS based automatic and unsupervised tool for the co-seismic Sentinel-1 DInSAR products generation. In Proceedings of the EGU General Assembly 2022, Vienna, Austria, 23–27 May 2022. [Google Scholar] [CrossRef]
- Manunta, M.; De Luca, C.; Zinno, I.; Casu, F.; Manzo, M.; Bonano, M.; Fusco, A.; Pepe, A.; Onorato, G.; Berardino, P.; et al. The Parallel SBAS Approach for Sentinel-1 Interferometric Wide Swath Deformation Time-Series Generation: Algorithm Description and Products Quality Assessment. IEEE Trans. Geosci. Remote Sens. 2019, 57, 6259–6281. [Google Scholar] [CrossRef]
- Pepe, A.; Lanari, R. On the Extension of the Minimum Cost Flow Algorithm for Phase Unwrapping of Multitemporal Differential SAR Interferograms. IEEE Trans. Geosci. Remote Sens. 2006, 44, 2374–2383. [Google Scholar] [CrossRef]
- Pepe, A.; Solaro, G.; Calo, F.; Dema, C. A Minimum Acceleration Approach for the Retrieval of Multiplatform InSAR Deformation Time Series. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2016, 9, 3883–3898. [Google Scholar] [CrossRef]
- Pepe, A.; Calò, F. A Review of Interferometric Synthetic Aperture RADAR (InSAR) Multi-Track Approaches for the Retrieval of Earth’s Surface Displacements. Appl. Sci. 2017, 7, 1264. [Google Scholar] [CrossRef]
- Jorgensen, M.; Zhdanov, M. Imaging Yellowstone magmatic system by the joint Gramian inversion of gravity and magnetotelluric data. Phys. Earth Planet. Inter. 2019, 292, 12–20. [Google Scholar] [CrossRef]
- Tu, X.; Zhdanov, M.S. Joint Gramian inversion of geophysical data with different resolution capabilities: Case study in Yellowstone. Geophys. J. Int. 2021, 226, 1058–1085. [Google Scholar] [CrossRef]


| Domain Extent | Elastic Parameters | ||
| -direction: | 100 km | Young’s modulus: | 1 GPa |
| -direction: | 100 km | Poisson’s coefficient: | 0.25 (-) |
| -direction: | 50 km | : | 0.5 MPa |
| Mesh Parameters | Boundary Conditions | ||
| Source (tetrahedral): | 0.3–0.8 km | Half-space bottom: | Fixed Constraint |
| Domain (tetrahedral): | 0.3–6 km | Half-space sides: | Roller |
| Free surface (mapped): | 0.4 km (step) | Half-space top: | Free |
| Profile | East (km) | North (km) | Depth (km b.s.l.) | N | |
|---|---|---|---|---|---|
| AB | A (507,400 E, 4,904,700 N) B (564,800 E, 4,955,000 N) | 524.2 ± 0.5 550.6 ± 1 | 4919.4 ± 0.5 4942.5 ± 1 | −9.8 ± 1 −9.1 ± 1.6 | 3 3 |
| CD | C (524,431 E, 4,933,700 N) D (532,800 E, 4,911,300 N) | 528.25 ± 0.2 | 4923.5 ± 0.2 | −6.9 ± 0.6 | 1.6 |
| EF | E (529,900 E, 4,934,800 N) F (543,900 E, 4,923,700 N) | 536.6 ± 0.2 | 4929.5 ± 0.2 | −7.5 ± 0.5 | 1.6 |
| GH | G (539,300 E, 4,944,900 N) H (544,300 E, 4,921,500 N) | 541.6 ± 0.2 | 4933.95 ± 0.2 | −8 ± 0.4 | 1.5 |
| IL | I (544,600 E, 4,958,400 N) L (556,400 E, 4,926,600 N) | 550.6 ± 0.2 | 4942.2 ± 0.2 | −6 ± 0.6 | 1.4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barone, A.; Pepe, A.; Tizzani, P.; Fedi, M.; Castaldo, R. New Advances of the Multiscale Approach for the Analyses of InSAR Ground Measurements: The Yellowstone Caldera Case-Study. Remote Sens. 2022, 14, 5328. https://doi.org/10.3390/rs14215328
Barone A, Pepe A, Tizzani P, Fedi M, Castaldo R. New Advances of the Multiscale Approach for the Analyses of InSAR Ground Measurements: The Yellowstone Caldera Case-Study. Remote Sensing. 2022; 14(21):5328. https://doi.org/10.3390/rs14215328
Chicago/Turabian StyleBarone, Andrea, Antonio Pepe, Pietro Tizzani, Maurizio Fedi, and Raffaele Castaldo. 2022. "New Advances of the Multiscale Approach for the Analyses of InSAR Ground Measurements: The Yellowstone Caldera Case-Study" Remote Sensing 14, no. 21: 5328. https://doi.org/10.3390/rs14215328
APA StyleBarone, A., Pepe, A., Tizzani, P., Fedi, M., & Castaldo, R. (2022). New Advances of the Multiscale Approach for the Analyses of InSAR Ground Measurements: The Yellowstone Caldera Case-Study. Remote Sensing, 14(21), 5328. https://doi.org/10.3390/rs14215328








