Revisiting Ice Flux and Mass Balance of the Lambert Glacier–Amery Ice Shelf System Using Multi-Remote-Sensing Datasets, East Antarctica
Abstract
1. Introduction

2. Materials and Methods
2.1. Data Sources
2.1.1. Drainage Basin Delineation and Grounding Line
2.1.2. Ice Velocity
2.1.3. Ice Thickness
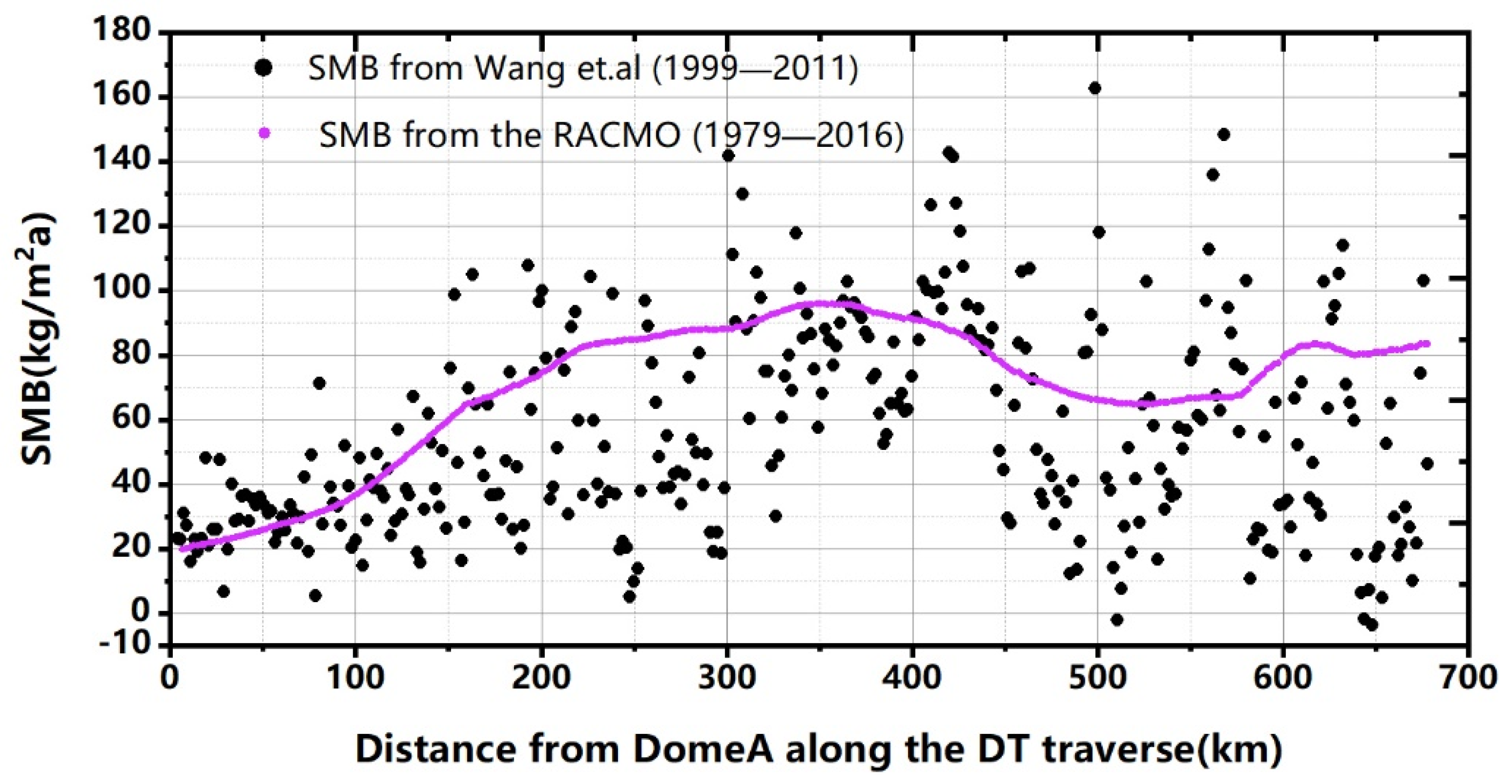
2.1.4. Surface Mass Balance
2.2. Method
2.2.1. Ice Flux Calculation
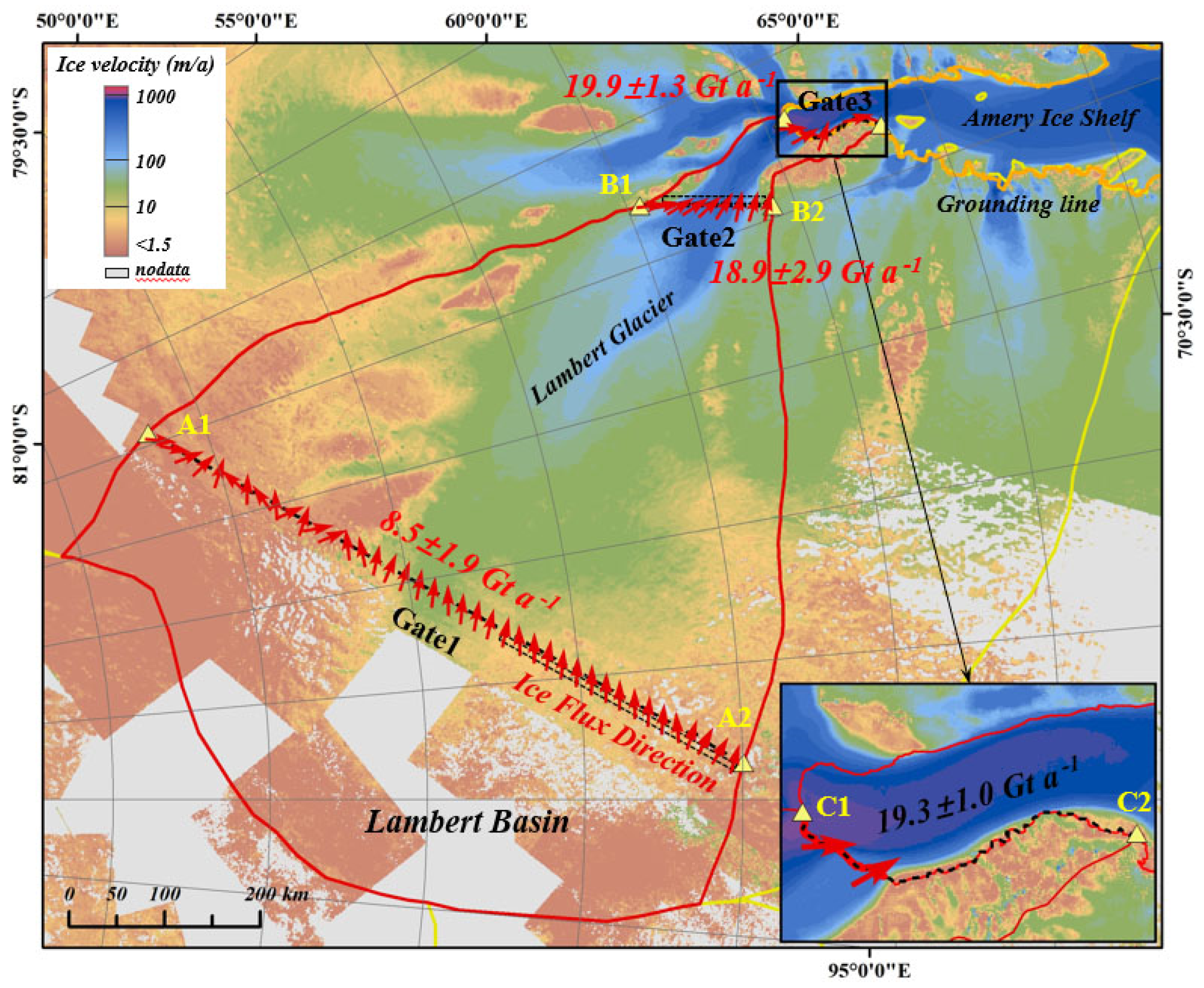
2.2.2. Ice Gate Location
2.2.3. Surface Mass Balance Processing
3. Results and Discussion
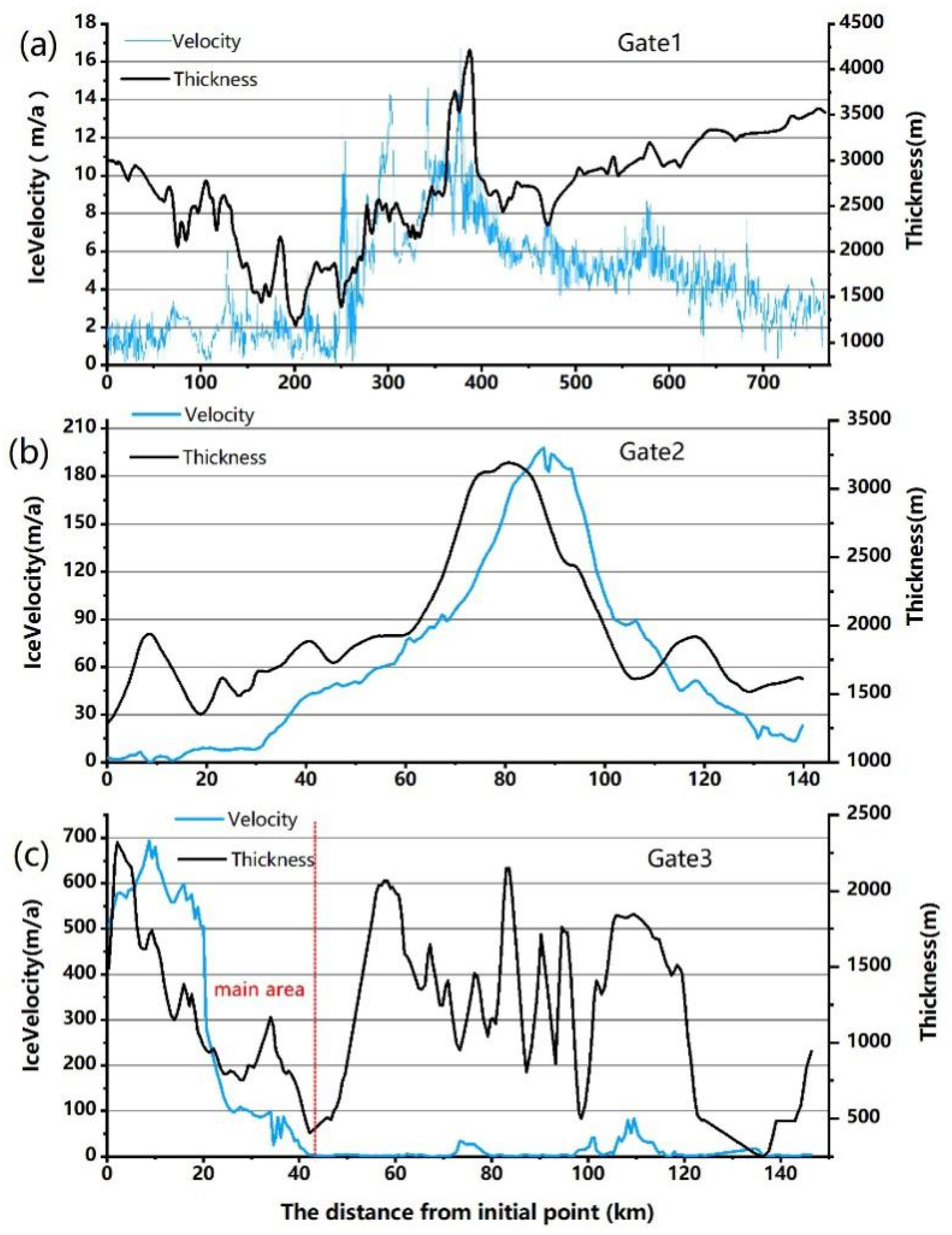
3.1. Depth-Averaged Velocity
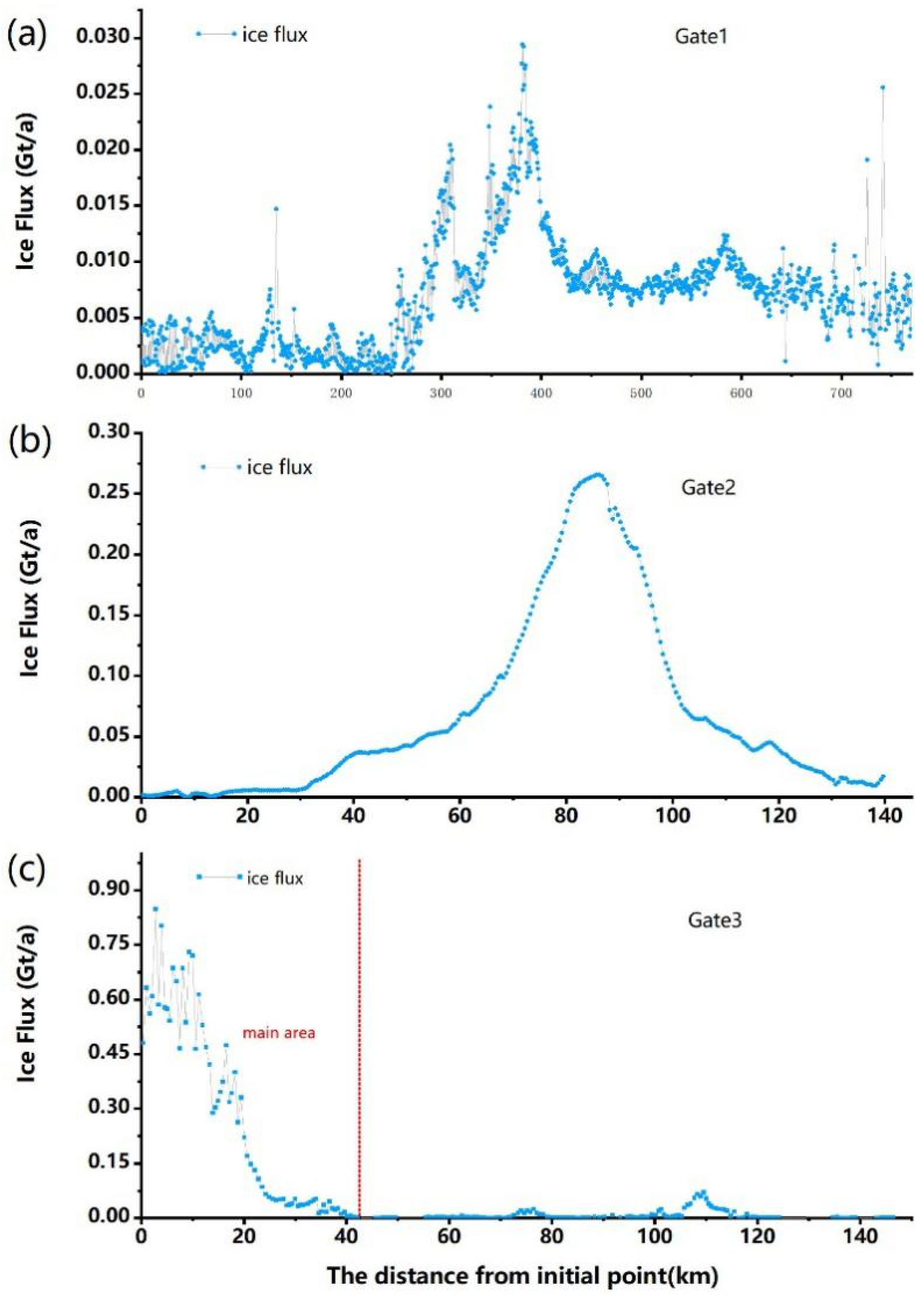
3.2. Ice Flux and Mass Balance
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rignot, E.; Mouginot, J.; Scheuchl, B. Four decades of Antarctic Ice Sheet mass balance from 1979–2017. Proc. Natl. Acad. Sci. USA 2019, 116, 1095–1103. [Google Scholar] [CrossRef]
- Wen, J.H.; Jezek, K.C.; Csatho, B.M. Mass budgets of the Lambert, Mellor and Fisher Glaciers and basal fluxes beneath their flowbands on Amery Ice Shelf. SCES 2007, 50, 1693–1706. [Google Scholar] [CrossRef][Green Version]
- Kingslake, J.; Ely, J.C.; Das, I.; Bell, R.E. Widespread movement of meltwater onto and across Antarctic ice shelves. Nature 2017, 544, 349–352. [Google Scholar] [CrossRef]
- Rignot, E.; Velicogna, I.; Broeke, M.R.V.D.; Monaghan, A.; Lenaerts, J.T.M. Acceleration of the contribution of the Greenland and Antarctic ice sheets to sea level rise. Geophys. Res. Lett. 2011, 38, 5. [Google Scholar] [CrossRef]
- Wouters, B.; Martin-Espanol, A.; Helm, V.; Flament, T.; van Wessem, J.M.; Ligtenberg, S.R.M.; Van Den Broeke, M.R.; Bamber, J.L. Dynamic thinning of glaciers on the Southern Antarctic Peninsula. Science 2015, 348, 899–903. [Google Scholar] [CrossRef] [PubMed]
- Cui, X.; Du, W.; Xie, H.; Sun, B. The ice flux to the Lambert Glacier and Amery Ice Shelf along the Chinese inland traverse and implications for mass balance of the drainage basins, East Antarctica. Polar Res. 2020, 39. [Google Scholar] [CrossRef]
- Golledge, N.R.; Levy, R.H.; Mckay, R.M.; McKay, R.M.; Naish, T.R. East Antarctic ice sheet most vulnerable to Weddell Sea warming. Geophys. Res. Lett. 2017, 44, 2343–2351. [Google Scholar] [CrossRef]
- Gong, Y.; Cornford, S.L.; Payne, A.J. Modelling the response of the Lambert Glacier–Amery Ice Shelf system, East Antarc-tica, to uncertain climate forcing over the 21st and 22nd centuries. Cryosphere 2014, 8, 1057–1068. [Google Scholar] [CrossRef]
- Pittard, M.L.; Galton-Fenzi, B.K.; Watson, C.S.; Roberts, J.L. Future sea level change from Antarctica’s Lambert-Amery glacial system. Geophys. Res. Lett. 2017, 44, 7347–7355. [Google Scholar] [CrossRef]
- Wen, J.; Huang, L.; Wang, W.; Jacka, T.; Damm, V.; Liu, Y. Ice thickness over the southern limit of the Amery Ice Shelf, East Antarctica, and reas-sessment of the mass balance of the central portion of the Lambert Glacier-Amery Ice Shelf system. Ann. Glaciol. 2014, 55, 81–86. [Google Scholar] [CrossRef]
- Budd, W.F.; Corry, M.J.; Jacka, T.H. Results From The Amery Ice Shelf Project. Ann. Glaciol. 1982, 3, 36–41. [Google Scholar] [CrossRef]
- Allison, I. The Mass Budget of the Lambert Glacier Drainage Basin, Antarctica. J. Glaciol. 1979, 24, 511. [Google Scholar] [CrossRef]
- Budd, W. The dynamics of the Amery Ice Shelf. J. Glaciol. 1966, 6, 335–358. [Google Scholar] [CrossRef][Green Version]
- Higham, M.; Craven, M.; Ruddell, A.; Allison, I. Snow-accumulation distribution in the interior of the Lambert Glacier basin, Antarctica. Ann. Glaciol. 1997, 25, 412–417. [Google Scholar] [CrossRef][Green Version]
- Yu, J.; Liu, H.; Jezek, K.C.; Warner, R.C.; Wen, J. Analysis of velocity field, mass balance, and basal melt of the Lambert Glacier–Amery Ice Shelf system by incorporating Radarsat SAR interferometry and ICESat laser altimetry measurements. J. Geophys. Res. Solid Earth 2010, 115, B11. [Google Scholar] [CrossRef]
- Fricker, H.A.; Warner, R.C.; Allison, I. Mass balance of the Lambert Glacier—Amery Ice Shelf system, East Antarctica: A comparison of computed balance fluxes and measured fluxes. J. Glaciol. 2000, 46, 561–570. [Google Scholar] [CrossRef]
- Jezek, K.C. RADARSAT-1 Antarctic Mapping Project: Change-detection and surface velocity campaign. Ann. Glaciol. 2001, 34, 263–268. [Google Scholar] [CrossRef]
- King, M.; Nguyen, L.N.; Coleman, R.; Morgan, P. Strategies for High Precision Processing of GPS Measurements with Application to the Amery Ice Shelf, East Antarctica. GPS Solut. 2000, 4, 2–12. [Google Scholar] [CrossRef]
- Lythe, M.B.; Vaughan, D.G. BEDMAP: A new ice thickness and subglacial topographic model of Antarctica. J. Geophys. Res. Solid Earth 2001, 106, 11335–11351. [Google Scholar] [CrossRef]
- Fretwell, P.; Pritchard, H.D.; Vaughan, D.G.; Bamber, J.L.; Barrand, N.E.; Bell, R.; Bianchi, C.; Bingham, R.G.; Blankenship, D.D.; Casassa, G.; et al. Bedmap2: Improved ice bed, surface and thickness datasets for Antarcti-ca. Cryosphere 2013, 7, 375–393. [Google Scholar] [CrossRef]
- The IMBIE Team. Mass balance of the Antarctic ice sheet from 1992 to 2017. Nature 2018, 558, 219–222. [Google Scholar] [CrossRef] [PubMed]
- IPCC. Climate Change 2021: The Physical Science Basis. Contribution of Working Group I to the Sixth Assessment Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK, 2021; Chapter 9; Available online: https://www.ipcc.ch/assessment-report/ar6/ (accessed on 1 September 2021).
- Haran, T.; Klinger, M.; Bohlander, J.; Fahnestock, M.; Painter, T.; Scambos, T. MEaSUREs MODIS Mosaic of Antarctica 2013–2014 (MOA2014) Image Map, Version 1; NSIDC: National Snow and Ice Data Center: Boulder, CO, USA, 2018. [Google Scholar] [CrossRef]
- Scambos, T.A.; Haran, T.M.; Fahnestock, M. MODIS-based Mosaic of Antarctica (MOA) data sets: Continent-wide surface morphology and snow grain size. Remote Sens. Environ. 2007, 111, 242–257. [Google Scholar] [CrossRef]
- Mouginot, J.; Scheuchl, B.; Rignot, E. MEaSUREs Antarctic Boundaries for IPY 2007–2009 from Satellite Radar, Version 2; NSIDC: National Snow and Ice Data Center: Boulder, CO, USA, 2017. [Google Scholar] [CrossRef]
- Rignot, E.; Jacobs, S.; Mouginot, J.; Scheuchl, B. Ice-shelf melting around Antarctica. Science 2013, 341, 266–270. [Google Scholar] [CrossRef] [PubMed]
- Shen, Q.; Wang, H.; Shum, C.K.; Jiang, L.; Hsu, H.; Gao, F.; Zhao, Y. Antarctic-wide annual ice flow maps from Landsat 8 imagery between 2013 and 2019. Int. J. Digit. Earth 2020, 14, 597–618. [Google Scholar] [CrossRef]
- Morlighem, M. MEaSUREs BedMachine Antarctica, Version 2; NSIDC: National Snow and Ice Data Center: Boulder, CO, USA, 2020. [Google Scholar] [CrossRef]
- Morlighem, M.; Rignot, E.; Binder, T.; Blankenship, D.; Drews, R.; Eagles, G.; Eisen, O.; Ferraccioli, F.; Forsberg, R.; Fretwell, P.; et al. Deep glacial troughs and stabilizing ridges unveiled beneath the margins of the Antarctic ice sheet. Nat. Geosci. 2020, 13, 132–137. [Google Scholar] [CrossRef]
- Van Wessem, J.M.; van de Berg, W.J.; Noël, B.P.Y.; van Meijgaard, E.; Amory, C.; Birnbaum, G.; Jakobs, C.L.; Krüger, K.; Lenaerts, J.T.M.; Lhermitte, S.; et al. Modelling the climate and surface mass balance of polar ice sheetsusing RACMO2, part 2: Antarctica (1979–2016). Cryosphere 2018, 12, 1–35. [Google Scholar] [CrossRef]
- Taylor, J. Introduction to Error Analysis, the Study of Uncertainties in Physical Measurements, 2nd ed.; University Science Books: South Orange, NJ, USA, 1997; pp. 13–79. [Google Scholar]
- Rignot, E.; Mouginot, J.; Scheuchl, B. Antarctic grounding line mapping from differential satellite radar interferometry. Geophys. Res. Lett. 2011, 38, 10. [Google Scholar] [CrossRef]
- Mouginot, J.; Scheuchl, B.; Rignot, E. Mapping of Ice Motion in Antarctica Using Synthetic-Aperture Radar Data. Remote Sens. 2012, 4, 2753–2767. [Google Scholar] [CrossRef]
- Rignot, E.; Mouginot, J.; Scheuchl, B. Ice Flow of the Antarctic Ice Sheet. Science 2011, 333, 1427–1430. [Google Scholar] [CrossRef]
- Shen, Q.; Wang, H.; Shum, C.K.; Jiang, L.; Hsu, H.T.; Dong, J.; Mao, S.; Gao, F. Present-day high-resolution ice velocity map of the Antarctic ice sheet. Earth Syst. Sci. 2018, 1–25. [Google Scholar] [CrossRef]
- Cui, X.; Jeofry, H.; Greenbaum, J.S.; Guo, J.; Li, L.; Lindzey, L.E.; Habbal, F.A.; Wei, W.; Young, D.A.; Ross, N.; et al. Bed topography of Princess Elizabeth Land in East Antarctica. Earth Syst. Sci. 2020, 12, 2765–2774. [Google Scholar] [CrossRef]
- Van Wessem, J.M.; Reijmer, C.H.; Morlighem, M.; Mouginot, J.; Rignot, E.; Medley, B.; Joughin, I.; Wouters, B.; Depoorter, M.A.; Bamber, J.L.; et al. Improved representation of East Antarctic surface mass balance in a re-gional atmospheric climate model. J. Glaciol. 2014, 60, 761–770. [Google Scholar] [CrossRef]
- Wang, Y.; Ding, M.; Reijmer, C.H.; Smeets, P.C.J.P.; Hou, S.; Xiao, C. The AntSMB dataset: A comprehensive compilation of surface mass balance field observations over the Antarctic Ice Sheet. Earth Syst. Sci. Data 2021, 13, 3057–3074. [Google Scholar] [CrossRef]
- Cuffey, K.M.; Paterson, W.S.B. The Physics of Glaciers, 4th ed.; Elsevier: Burlington, NJ, USA; Oxford, UK, 2010; pp. 285–398. [Google Scholar]
- Ren, J.A. Traverse Expedition to the Lambert Glacier Basin, Eastern Antarctica. J. Glaciol. Geocryol. 1995. Available online: http://en.cnki.com.cn/Article_en/CJFDTOTAL-BCDT504.004.htm (accessed on 1 June 2021).
- Guo, J.; Yang, W.; Dou, Y.; Tang, X.; Greenbaum, J.S.; Dou, R.; Pan, Y.; Zhang, Y.; Ding, M.; Jiang, S.; et al. Historical surface mass balance from a frequency-modulated continuous-wave (FMCW) radar survey from Zhongshan station to Dome A. J. Glaciol. 2020, 66, 965–977. [Google Scholar] [CrossRef]
- Gardner, A.S.; Moholdt, G.; Scambos, T.; Fahnstock, M.; Ligtenberg, S.; Broeke, M.V.D.; Nilsson, J. Increased West Antarctic and unchanged East Antarctic ice discharge over the last 7 years. Cryosphere 2018, 12, 521–547. [Google Scholar] [CrossRef]
- Thakur, P.K.; Swain, A.K.; Dhote, P.R.; Kumar, P.; Kaushik, S.; Gajbhiye, D.; Mahagaonkar, A.; Sharma, V.; Dharwadkar, A.; Aggarwal, S.; et al. Satellite and ground based estimates for ice surface velocities in the part of central Dronning Maud Land, East Antarctica: Implications for ice flux calculations. Polar Sci. 2021, 30, 100737. [Google Scholar] [CrossRef]
| Products | Version | Location and Accessed Date | Usage |
|---|---|---|---|
| MEaSUREs Antarctic Boundaries for IPY 2007–2009 from Satellite Radar | Basins_Antarctica_v02 | https://doi.org/10.5067/AXE4121732AD (1 July 2021) | Drainage Basin Delineation |
| MEaSUREs MODIS Mosaic of Antarctica 2013–2014 (MOA2014) Image Map | Moa2014_grounding_line_v01 | https://doi.org/10.5067/RNF17BP824UM (1 July 2021) | Grounding line |
| Present-day high-resolution ice velocity and footprints maps of the Antarctic ice sheet | Shen Qiang, Wang HanSheng, et al. (2020) | https://doi.pangaea.de/10.1594/PANGAEA.908845 (15 March 2021) | Ice Surface velocity |
| MEaSUREs BedMachine Antarctica | Bedmachine v2: Ice surface elevation & Bed topography map | https://doi.org/10.5067/E1QL9HFQ7A8M (1 July 2021) | Slope |
| MEaSUREs BedMachine Antarctica | Bedmachine v2: Ice thickness | https://doi.org/10.5067/E1QL9HFQ7A8M (1 July 2021) | Thickness |
| RACMO2.3p2 | ANT27_1979-2016 (yearly) | https://www.projects.science.uu.nl/iceclimate/publictions/data/2018/index (1 August 2021) | Surface mass balance (SMB) |
| FluxGate Name | Initial Point | End Point | Distance along Gate (km) |
|---|---|---|---|
| Gate 1 (G1) | A1 (80.0333°S, 69.3335°E) | A2 (75.0013°S, 88.6496°E) | 791.7 |
| Gate 2 (G2) | B1 (74.8872°S, 67.9234°E) | B2 (73.7075°S, 69.6156°E) | 139.8 |
| Gate 3 (G3) | C1 (73.3088° S, 67.0301° E) | C2 (72.4824° S, 68.4121° E) | 146.4 |
| Location | Input Flux | Accumulation from RACMO2.3 | Output Flux | Net Budget | |
|---|---|---|---|---|---|
| The south of G1 | / | 40.84 | |||
| G1–G2 | 67.74 | ||||
| G2–G3 | 48.14 | ||||
| Total Lambert | / | 53.98 |
| Studies | Area | Accumulation | Ice Flux | Mass Budget |
|---|---|---|---|---|
| Wen (2007) | 424,930 | |||
| Yu (2010) | ||||
| Wen (2014) | ||||
| This Study |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, D.; Tang, X.; Yang, S.; Zhang, Y.; Wang, L.; Li, L.; Sun, B. Revisiting Ice Flux and Mass Balance of the Lambert Glacier–Amery Ice Shelf System Using Multi-Remote-Sensing Datasets, East Antarctica. Remote Sens. 2022, 14, 391. https://doi.org/10.3390/rs14020391
Xu D, Tang X, Yang S, Zhang Y, Wang L, Li L, Sun B. Revisiting Ice Flux and Mass Balance of the Lambert Glacier–Amery Ice Shelf System Using Multi-Remote-Sensing Datasets, East Antarctica. Remote Sensing. 2022; 14(2):391. https://doi.org/10.3390/rs14020391
Chicago/Turabian StyleXu, Derui, Xueyuan Tang, Shuhu Yang, Yun Zhang, Lijuan Wang, Lin Li, and Bo Sun. 2022. "Revisiting Ice Flux and Mass Balance of the Lambert Glacier–Amery Ice Shelf System Using Multi-Remote-Sensing Datasets, East Antarctica" Remote Sensing 14, no. 2: 391. https://doi.org/10.3390/rs14020391
APA StyleXu, D., Tang, X., Yang, S., Zhang, Y., Wang, L., Li, L., & Sun, B. (2022). Revisiting Ice Flux and Mass Balance of the Lambert Glacier–Amery Ice Shelf System Using Multi-Remote-Sensing Datasets, East Antarctica. Remote Sensing, 14(2), 391. https://doi.org/10.3390/rs14020391







