Geodetic Mass Balance of Haxilegen Glacier No. 51, Eastern Tien Shan, from 1964 to 2018
Abstract
:1. Introduction
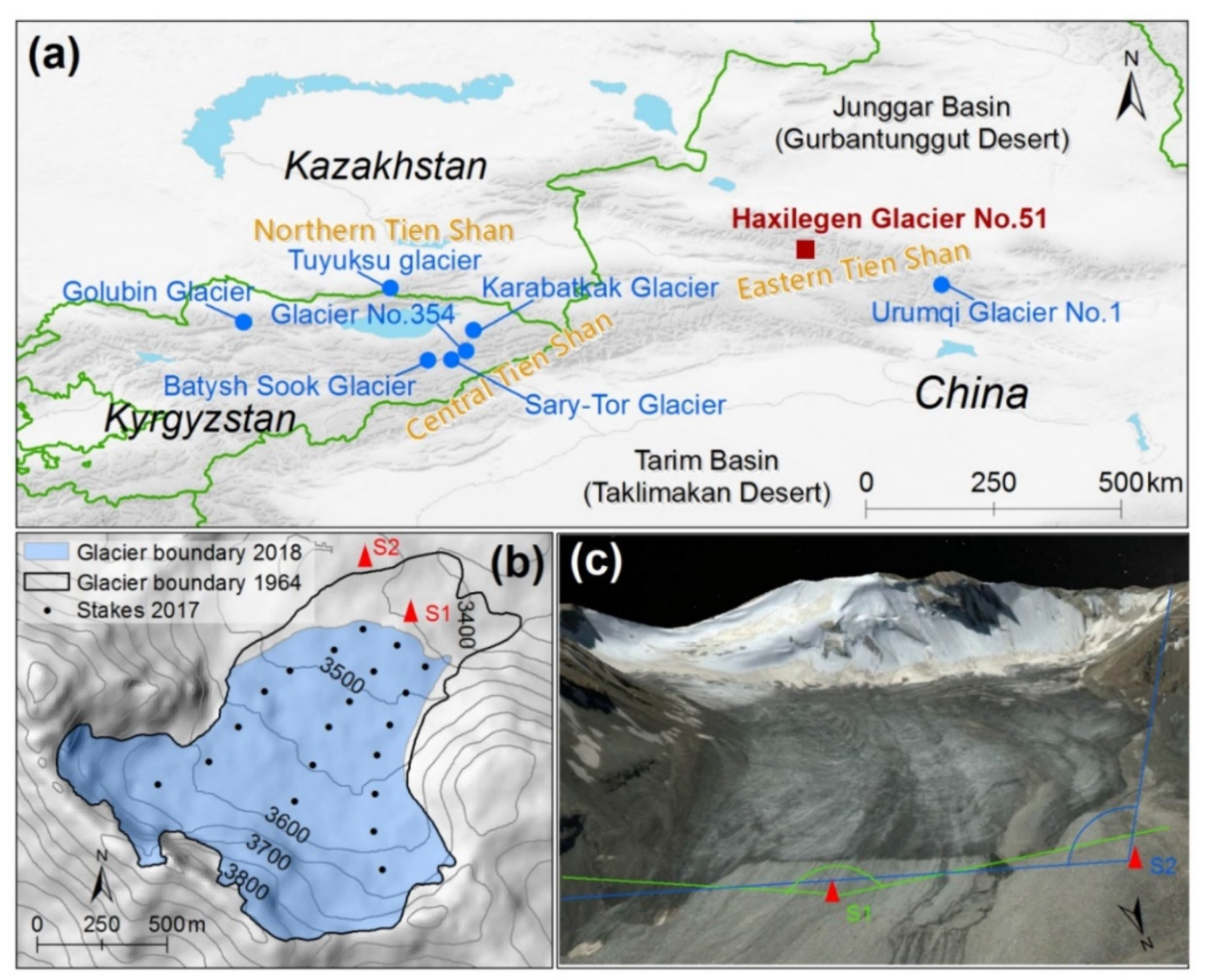
2. Study Site
3. Data and Methods
3.1. DEM Generated from Riegl VZ®-6000 TLS
3.2. DEM Generated from the Topographic Map and Co-Registration
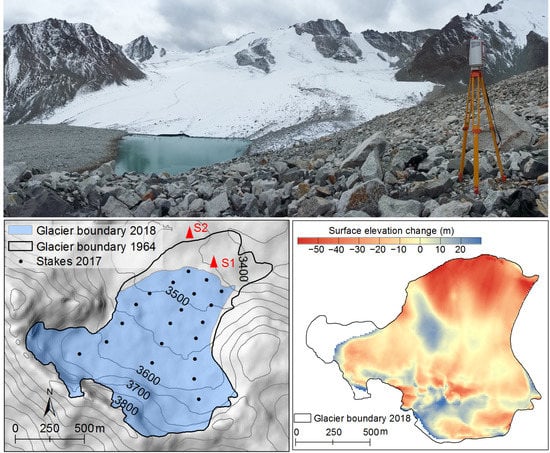
3.3. Glacier Boundary Delineation
3.4. Geodetic Mass Balance Calculations
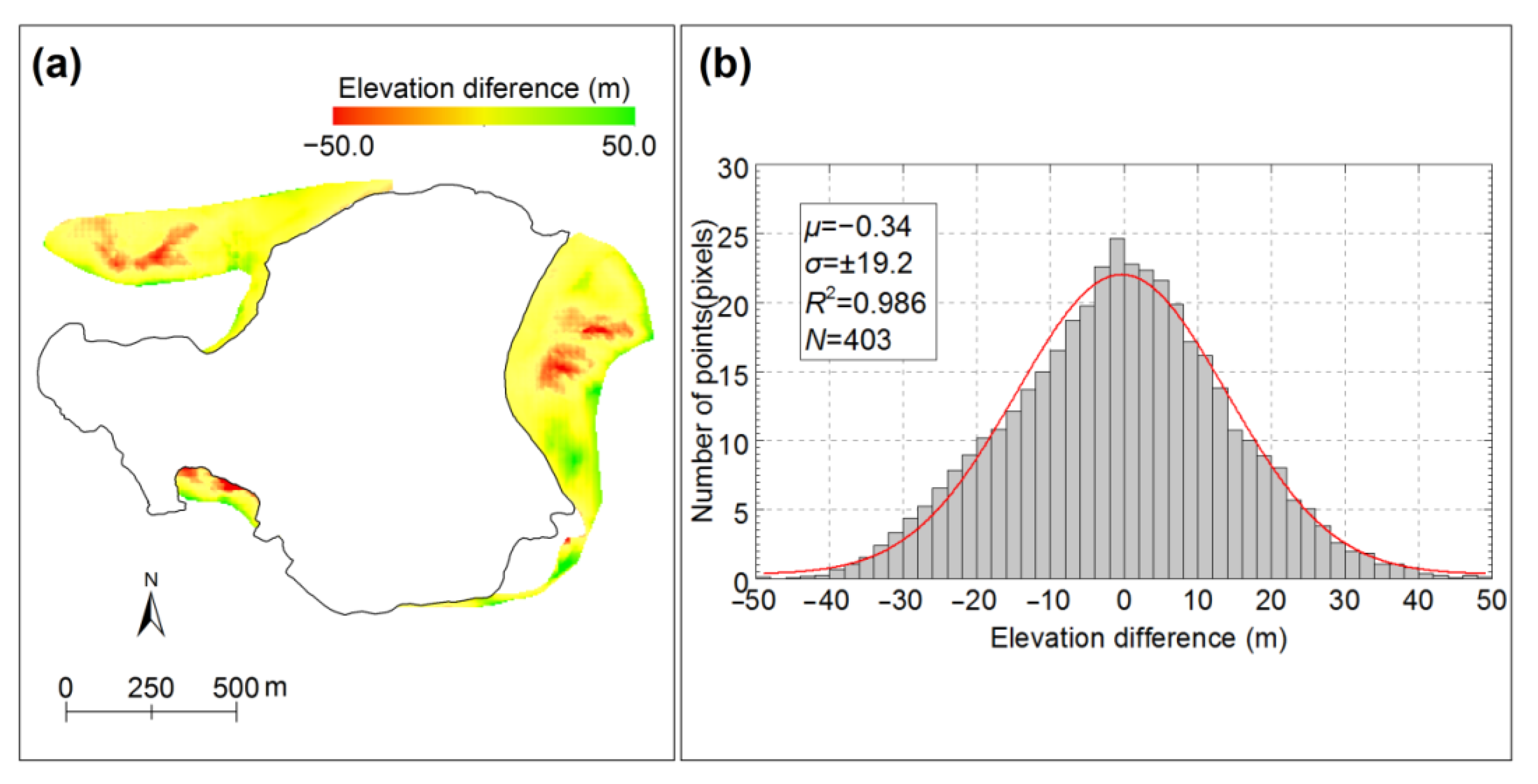
3.5. Uncertainty Estimates
3.5.1. Uncertainties in Glacier Area
3.5.2. Uncertainties in Geodetic Mass Balance
4. Results
4.1. Changes in Glacier Area
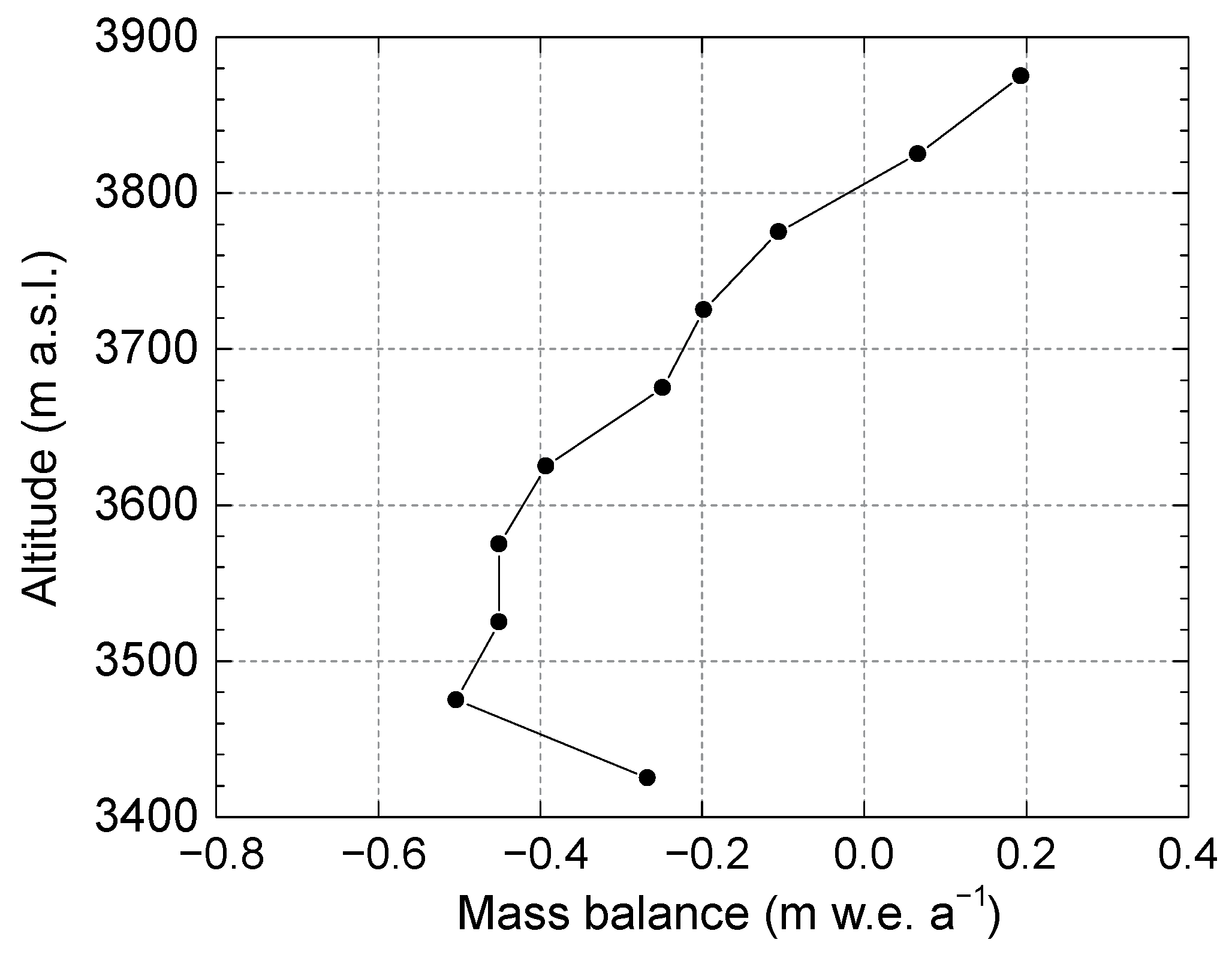
4.2. Changes in Glacier Surface Elevation and Mass Balance
5. Discussion
5.1. Comparison with Previous Studies
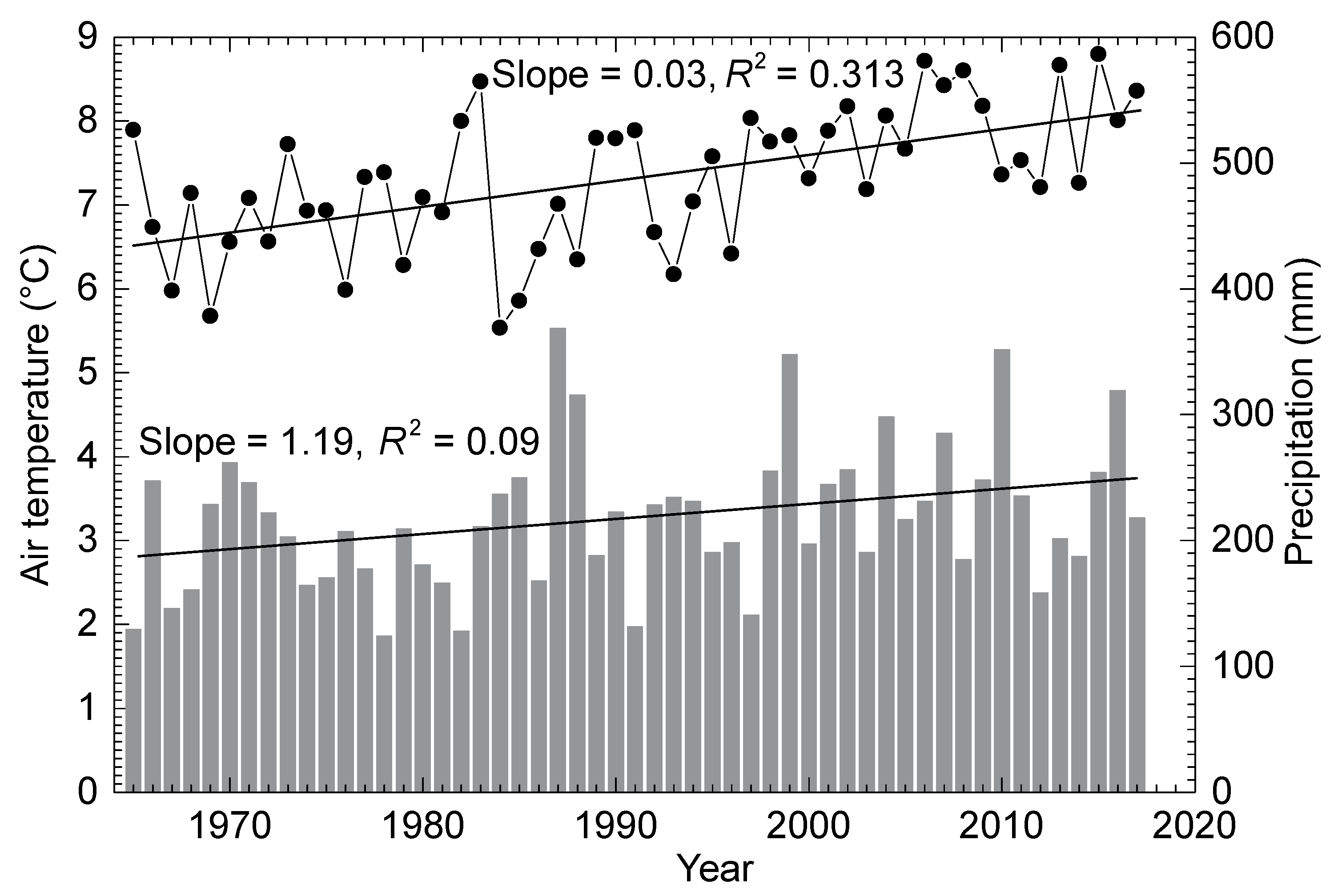
5.2. Possible Drivers for Glacier Mass Changes
5.3. Implications and Recommendations
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Pritchard, H.D. Asia’s shrinking glaciers protect large populations from drought stress. Nature 2019, 569, 649–654. [Google Scholar] [CrossRef] [PubMed]
- Pfeffer, W.T.; Arendt, A.A.; Bliss, A.; Bolch, T.; Cogley, J.G.; Gardner, A.S.; Hagen, J.O.; Hock, R.; Kaser, G.; Kienholz, C.; et al. The randolph glacier inventory: A globally complete inventory of glaciers. J. Glaciol. 2014, 60, 537–552. [Google Scholar] [CrossRef] [Green Version]
- Farinotti, D.; Longuevergne, L.; Moholdt, G.; Duethmann, D.; Mölg, T.; Bolch, T.; Vorogushyn, S.; Güntner, A. Substantial glacier mass loss in the Tien Shan over the past 50 years. Nat. Geosci. 2015, 8, 716–722. [Google Scholar] [CrossRef]
- Sorg, A.; Bolch, T.; Stoffel, M.; Solomina, O.; Beniston, M. Climate change impacts on glaciers and runoff in Tien Shan (Central Asia). Nat. Clim. Chang. 2012, 2, 725–731. [Google Scholar] [CrossRef]
- Huss, M.; Hock, R. Global-scale hydrological response to future glacier mass loss. Nat. Clim. Chang. 2018, 8, 135–140. [Google Scholar] [CrossRef] [Green Version]
- Zemp, M.; Huss, M.; Thibert, E.; Eckert, N.; McNabb, R.; Huber, J.; Barandun, M.; Machguth, H.; Nussbaumer, S.U.; Gärtner-Roer, I.; et al. Global glacier mass changes and their contributions to sea-level rise from 1961 to 2016. Nature 2019, 568, 382–386. [Google Scholar] [CrossRef]
- Hoelzle, M.; Azisov, E.; Barandun, M.; Huss, M.; Farinotti, D.; Gafurov, A.; Hagg, W.; Kenzhebaev, R.; Kronenberg, M.; Machguth, H.; et al. Re-establishing glacier monitoring in Kyrgyzstan and Uzbekistan, Central Asia. Geosci. Instrum. Methods Data Syst. 2017, 6, 397–418. [Google Scholar] [CrossRef] [Green Version]
- Barandun, M.; Pohl, E.; Naegeli, K.; McNabb, R.; Huss, M.; Berthier, E.; Saks, T.; Hoelzle, M. Hot spots of glacier mass balance variability in Central Asia. Geophys. Res. Lett. 2021, 48, e2020GL092084. [Google Scholar] [CrossRef] [PubMed]
- Barandun, M.; Huss, M.; Sold, L.; Farinotti, D.; Azisov, E.; Salzmann, N.; Usubaliev, R.; Merkushkin, A.; Hoelzle, M. Re-analysis of seasonal mass balance at Abramov glacier 1968–2014. J. Glaciol. 2015, 61, 1103–1117. [Google Scholar] [CrossRef] [Green Version]
- Kronenberg, M.; Barandun, M.; Hoelzle, M.; Huss, M.; Farinotti, D.; Azisov, E.; Usubaliev, R.; Gafurov, A.; Petrakov, D.; Kääb, A. Mass balance reconstruction for Glacier No. 354, Tien Shan, from 2003 to 2014. Ann. Glaciol. 2016, 57, 92–102. [Google Scholar] [CrossRef] [Green Version]
- Thomson, L.; Brun, F.; Braun, M.; Zemp, M. Editorial: Observational Assessments of Glacier Mass Changes at Regional and Global Level. Front. Earth Sci. 2021, 8, 641710. [Google Scholar] [CrossRef]
- Zemp, M.; Thibert, E.; Huss, M.; Stumm, D.; Rolstad Denby, C.; Nuth, C.; Nussbaumer, S.U.; Moholdt, G.; Mercer, A.; Mayer, C.; et al. Reanalysing glacier mass balance measurement series. Cryosphere 2013, 7, 1227–1245. [Google Scholar] [CrossRef] [Green Version]
- Hagg, W.J.; Braun, L.N.; Uvarov, V.N.; Makarevich, K.G. A comparison of three methods of mass balance determination in the Tuyuksu glacier region, Tien Shan, Central Asia. J. Glaciol. 2004, 50, 505–510. [Google Scholar] [CrossRef] [Green Version]
- Kapitsa, V.; Shahgedanova, M.; Severskiy, I.; Kasatkin, N.; White, K.; Usmanova, Z. Assessment of Changes in Mass Balance of the Tuyuksu Group of Glaciers, Northern Tien Shan, Between 1958 and 2016 Using Ground-Based Observations and Pléiades Satellite Imagery. Front. Earth Sci. 2020, 8, 259. [Google Scholar] [CrossRef]
- Bolch, T. Glacier area and mass changes since 1964 in the Ala Archa Valley, Kyrgyz Ala-Too, northern Tien Shan. Ice Snow 2015, 129, 28–39. [Google Scholar] [CrossRef]
- Barandun, M.; Huss, M.; Usubaliev, R.; Azisov, E.; Berthier, E.; Kääb, A.; Bolch, T.; Hoelzle, M. Multi-decadal mass balance series of three Kyrgyz glaciers inferred from modelling constrained with repeated snow line observations. Cryosphere 2018, 12, 1899–1919. [Google Scholar] [CrossRef] [Green Version]
- Pieczonka, T.; Bolch, T. Region-wide glacier mass budgets and area changes for the Central Tien Shan between ~1975 and 1999 using Hexagon KH-9 imagery. Glob. Planet. Chang. 2015, 128, 1–13. [Google Scholar] [CrossRef]
- Kenzhebaev, R.; Barandun, M.; Kronenberg, M.; Chen, Y.; Usubaliev, R.; Hoelzle, M. Mass balance observations and reconstruction for Batysh Sook Glacier, Tien Shan, from 2004 to 2016. Cold Reg. Sci. Technol. 2017, 135, 76–89. [Google Scholar] [CrossRef] [Green Version]
- Wang, P.; Li, Z.; Li, H.; Wang, W.; Yao, H. Comparison of glaciological and geodetic mass balance at Urumqi Glacier No. 1, Tian Shan, central Asia. Glob. Planet. Chang. 2014, 114, 14–22. [Google Scholar] [CrossRef]
- Xu, C.; Li, Z.; Wang, P.; Anjum, M.N.; Li, H.; Wang, F. Detailed comparison of glaciological and geodetic mass balances for Urumqi Glacier No. 1, eastern Tien Shan, China, from 1981 to 2015. Cold Reg. Sci. Technol. 2018, 155, 137–148. [Google Scholar] [CrossRef]
- Fischer, M.; Huss, M.; Hoelzle, M. Surface elevation and mass changes of all Swiss glaciers 1980–2010. Cryosphere 2015, 9, 525–540. [Google Scholar] [CrossRef] [Green Version]
- Chu, T.; Lindenschmidt, K.-E. Comparison and validation of digital elevation models derived from InSAR for a flat inland delta in the high latitudes of Northern Canada. Can. J. Remote Sens. 2017, 43, 109–123. [Google Scholar] [CrossRef]
- Schumann, G.J.; Bates, P.D. The need for a high-accuracy, open-access global DEM. Front. Earth Sci. 2018, 6, 225. [Google Scholar] [CrossRef]
- Podgórski, J.; Kinnard, C.; Pętlicki, M.; Urrutia, R. Performance assessment of TanDEM-X DEM for mountain glacier elevation change detection. Remote Sens. 2019, 11, 187. [Google Scholar] [CrossRef] [Green Version]
- Caglar, B.; Becek, K.; Mekik, C.; Ozendi, M. On the vertical accuracy of the ALOS world 3D-30m digital elevation model. Remote Sens. Lett. 2018, 9, 607–615. [Google Scholar] [CrossRef]
- Fugazza, D.; Scaioni, M.; Corti, M.; D’Agata, C.; Azzoni, R.S.; Cernuschi, M.; Claudio, S.; Diolaiuti, G.A. Combination of UAV and terrestrial photogrammetry to assess rapid glacier evolution and map glacier hazards. Nat. Hazards Earth Syst. Sci. 2018, 18, 1055–1071. [Google Scholar] [CrossRef] [Green Version]
- Réveillet, M.; Vincent, C.; Six, D.; Rabatel, A.; Sanchez, O.; Piard, L.; Laarman, O. Spatio-temporal variability of surface mass balance in the accumulation zone of the Mer de Glace, French Alps, from multitemporal terrestrial LiDAR measurements. J. Glaciol. 2021, 67, 137–146. [Google Scholar] [CrossRef]
- Immerzeel, W.W.; Kraaijenbrink, P.D.A.; Shea, J.M.; Shrestha, A.B.; Pellicciotti, F.; Bierkens, M.F.P.; Jong, S.M.D. High-resolution monitoring of Himalayan glacier dynamics using unmanned aerial vehicles. Remote Sens. Environ. 2014, 150, 93–103. [Google Scholar] [CrossRef]
- Wigmore, O.; Mark, B. Monitoring tropical debris-covered glacier dynamics from high-resolution unmanned aerial vehicle photogrammetry, Cordillera Blanca, Peru. Cryosphere 2017, 11, 2463–2480. [Google Scholar] [CrossRef] [Green Version]
- Kukko, A.; Kaartinen, H.; Hyyppä, J.; Chen, Y. Multiplatform mobile Laser scanning: Usability and performance. Sensors 2012, 12, 11712–11733. [Google Scholar] [CrossRef] [Green Version]
- Gabbud, C.; Micheletti, N.; Lane, S.N. Lidar measurement of surface melt for a temperate Alpine glacier at the seasonal and hourly scales. J. Glaciol. 2015, 61, 963–974. [Google Scholar] [CrossRef] [Green Version]
- Xu, C.; Li, Z.; Wang, F.; Li, H.; Wang, W.; Wang, L. Using an ultra-long-range terrestrial laser scanner to monitor the net mass balance of Urumqi Glacier No. 1, eastern Tien Shan, China, at the monthly scale. J. Glaciol. 2017, 63, 792–802. [Google Scholar] [CrossRef]
- Zhang, H.; Li, Z.; Zhou, P.; Zhu, X.; Wang, L. Mass balance observations and reconstruction for Haxilegen Glacier No. 51, eastern Tien Shan, from 1999 to 2015. J. Glaciol. 2018, 64, 689–699. [Google Scholar] [CrossRef] [Green Version]
- Jing, Z.; Ye, B.; Jiao, K.; Yang, H. Surface velocity on the Glacier No. 51 at Haxilegen of the Kuytun River, Tianshan Mountains. J. Glaciol. Geocryol. 2002, 24, 564–566. [Google Scholar]
- Jiao, K.; Jing, Z.; Cheng, P. Monitoring results on the Glacier No. 51 at Haxilegen in the Kuytun River Basin, Tianshan Mountains. Arid Land Geogr. 2009, 32, 733–738. [Google Scholar]
- Wang, P.; Li, Z.; Li, H.; Wang, W.; Wu, L.; Zhang, H.; Huai, B.; Wang, L. Recent evolution in extent, thickness, and velocity of Haxilegen glacier no. 51, Kuytun River Basin, Eastern Tianshan Mountains. Arct. Antarct. Alp. Res. 2016, 48, 241–252. [Google Scholar] [CrossRef] [Green Version]
- Zhang, H.; Li, Z.; Wang, P.; Huai, B. Variation of Haxilegen No. 51 Glacier at the Headwater of Kuytun River in Tianshan Mountains and Its Response to Climate Change. Arid Zone Res. 2015, 32, 88–93. [Google Scholar]
- Zhang, H.; Li, Z.; Mu, J. Impact of the glacier change on water resources in the Kuytun River Basin, Tianshan Mountains during recent 50 years. Sci. Geogr. Sin. 2017, 37, 1771–1777. [Google Scholar]
- RGI Consortium. Randolph Glacier Inventory—A Dataset of Global Glacier Outlines: Version 6.0: Technical Report, Global Land Ice Measurements from Space; Digital Media: Denver, CO, USA, 2017. [Google Scholar] [CrossRef]
- RIEGL Laser Measurement Systems. 3D Terrestrial Laser Scanner Riegl VZ®-4000/Riegl VZ®-6000 General Description and Data Interfaces; RIEGL Laser Measurement Systems: Horn, Austria, 2014; pp. 1–466. [Google Scholar]
- Xu, C.; Li, Z.; Li, H.; Wang, F.; Zhou, P. Long-range terrestrial laser scanning measurements of annual and intra-annual mass balances for Urumqi Glacier No. 1, eastern Tien Shan, China. Cryosphere 2019, 13, 2361–2383. [Google Scholar] [CrossRef] [Green Version]
- Nuth, C.; Kääb, A. Co-registration and bias corrections of satellite elevation data sets for quantifying glacier thickness change. Cryosphere 2011, 5, 271–290. [Google Scholar] [CrossRef] [Green Version]
- GB/T 12343.1-2008; Compilation Specifications for National Fundamental Scale Maps—Part 1: Compilation Specifications for 1:25,000/1:50,000/1:100,000 Topographic Maps. General Administration of Quality Supervision, Inspection and Quarantine of the People’s Republic of China: Beijing, China; Standardization Administration of the People’s Republic of China: Beijing, China, 2008; pp. 1–40.
- Liu, S.; Yao, X.; Guo, W.; Xu, J.; Shangguan, D.; Wei, J.; Bao, W.; Wu, L. The contemporary glaciers in China based on the Second Chinese Glacier Inventory. Acta Geogr. Sin. 2015, 70, 3–16. [Google Scholar]
- Huss, M. Density assumptions for converting geodetic glacier volume change to mass change. Cryosphere 2013, 7, 877–887. [Google Scholar] [CrossRef] [Green Version]
- Bolch, T.; Menounos, B.; Wheate, R. Landsat-based inventory of glaciers in western Canada, 1985–2005. Remote Sens. Environ. 2010, 114, 127–137. [Google Scholar] [CrossRef]
- Galos, S.P.; Klug, C.; Maussion, F.; Covi, F.; Nicholson, L.; Rieg, L.; Gurgiser, W.; Mölg, T.; Kaser, G. Reanalysis of a 10-year record (2004–2013) of seasonal mass balances at Langen-ferner/Vedretta Lunga, Ortler Alps, Italy. Cryosphere 2017, 11, 1417–1439. [Google Scholar] [CrossRef] [Green Version]
- Fischer, M.; Huss, M.; Kummert, M.; Hoelzle, M. Application and validation of long-range terrestrial laser scanning to monitor the mass balance of very small glaciers in the Swiss Alps. Cryosphere 2016, 10, 1279–1295. [Google Scholar] [CrossRef] [Green Version]
- Bolch, T.; Pieczonka, T.; Mukherjee, K.; Shea, J. Glaciers in the Hunza catchment (Karakoram) have been nearly in balance since the 1970s. Cryosphere 2017, 11, 531–539. [Google Scholar] [CrossRef] [Green Version]
- Gardelle, J.; Berthier, E.; Arnaud, Y.; Kääb, A. Region-wide glacier mass balances over the Pamir–Karakoram–Himalaya during 1999–2011. Cryosphere 2013, 7, 1263–1286. [Google Scholar] [CrossRef] [Green Version]
- WGMS (World Glacier Monitoring Service). Global Glacier Change Bulletin No. 3 (2016–2017); ISC(WDS)/IUGG(IACS)/UNEP/UNESCO/WMO; Zemp, M., Gärtner-Roer, I., Nussbaumer, S.U., Bannwart, J., Rastner, P., Paul, F., Hoelzle, M., Eds.; World Glacier Monitoring Service: Zurich, Switzerland, 2020; pp. 1–274. [Google Scholar] [CrossRef]
- Mu, J.; Li, Z.; Zhang, H.; Liang, P. The global glacierized area: Current situation and recent change, based on the Randolph Glacier Inventory (RGI 6.0) published in 2017. J. Glaciol. Geocryol. 2018, 40, 238–248. [Google Scholar]
- Xing, W.; Li, Z.; Zhang, H.; Zhang, M.; Liang, P.; Mu, J. Spatial-temporal variation of glacier resources in Chinese Tianshan Mountains since 1959. Acta Geogr. Sin. 2017, 72, 1594–1605. [Google Scholar]
- Li, H.; Wang, P.; Li, Z.; Jin, S.; Xu, C.; Liu, S.; Zhang, Z.; Xu, L. An application of three different field methods to monitor changes in Urumqi Glacier No. 1, Chinese Tien Shan, during 2012–2018. J. Glaciol. 2021, 1–13. [Google Scholar] [CrossRef]
- Brun, F.; Wagnon, P.; Berthier, E.; Jomelli, V.; Maharjan, S.B.; Shrestha, F.; Kraaijenbrink, P.D.A. Heterogeneous influence of glacier morphology on the mass balance variability in High Mountain Asia. J. Geophys. Res. Earth Surf. 2019, 124, 1331–1345. [Google Scholar] [CrossRef]
- Azisov, E.; Barandun, M.; Saks, T.; Vorogushyn, S.; Usubaliev, R.; Hoelzle, M. Reconstructed centennial mass balance change for Golubin Glacier, Northern Tien Shan. In Proceedings of the EGU General Assembly Conference, Vienna, Austria, 7–12 April 2019. [Google Scholar]
- Brun, F.; Berthier, E.; Wagnon, P.; Kääb, A.; Treichler, D.A. Spatially resolved estimate of High Mountain Asia glacier mass balances from 2000 to 2016. Nat. Geosci. 2017, 10, 668–673. [Google Scholar] [CrossRef] [PubMed]
- Bhattacharya, A.; Bolch, T.; Mukherjee, K.; King, O.; Menounos, B.; Kapitsa, V.; Neckel, N.; Yang, W.; Yao, T. High Mountain Asian glacier response to climate revealed by multi-temporal satellite observations since the 1960s. Nat. Commun. 2021, 12, 4133. [Google Scholar] [CrossRef]
- Kaser, G.; Cogley, J.G.; Dyurgerov, M.B.; Meier, M.F.; Ohmura, A. Mass balance of glaciers and ice caps: Consensus estimates for 1961–2004. Geophys. Res. Lett. 2006, 33, L19501. [Google Scholar] [CrossRef] [Green Version]
- Yao, T.; Yu, W.; Wu, G.; Xu, B.; Yang, W.; Zhao, H.; Wang, W.; Li, S.; Wang, N.; Li, Z.; et al. Glacier anomalies and relevant disaster risks on the Tibetan Plateau and surroundings. Chin. Sci. Bull. 2019, 64, 2770–2782. [Google Scholar] [CrossRef] [Green Version]
- Li, Z.; Li, H.; Chen, Y. Mechanisms and simulation of accelerated shrinkage of continental glaciers: A case study of Urumqi Glacier No. 1 in Eastern Tianshan, central Asia. J. Earth Sci. 2011, 22, 423–430. [Google Scholar] [CrossRef]
- Zhang, Y.; Gao, T.; Kang, S.; Shangguan, D.; Luo, X. Albedo reduction as an important driver for glacier melting in Tibetan Plateau and its surrounding areas. Earth-Sci. Rev. 2021, 220, 103735. [Google Scholar] [CrossRef]
- Dehecq, A.; Gourmelen, N.; Gardner, A.S.; Brun, F.; Goldberg, D.; Nienow, P.W.; Berthier, E.; Vincent, C.; Wagnon, P.; Trouvé, E. Twenty-first century glacier slowdown driven by mass loss in High Mountain Asia. Nat. Geosci. 2019, 12, 22–27. [Google Scholar] [CrossRef]



| Period | Area in 2018 (km2) | Area Reduction (%) | Δh (m a−1) | σΔh (m a−1) | σgeod.a (m w.e. a−1) | Bgeod.a (m w.e. a−1) |
|---|---|---|---|---|---|---|
| 1964–2018 | 1.14 ± 0.09 | 22.9 | −0.43 | 0.12 | 0.11 | −0.36 |
| Glacier | Period | Mass Balance | Method | Region | Source |
|---|---|---|---|---|---|
| Haxilegen Glacier No. 51 | 1964–2018 | −0.36 ± 0.11 | Geodetic | Eastern TS | This study |
| 1999–2015 | −0.37 ± 0.22 | Glaciological/modeled | Eastern TS | [33] | |
| Urumqi Glacier No. 1 | 1964–2018 | −0.37 ± 0.20 1 | Glaciological | Eastern TS | [51] |
| Glacier No. 354 | 2003–2014 | −0.43 ± 0.09 | Glaciological/modeled | Central TS | [10] |
| Batysh Sook Glacier | 2004–2016 | −0.39 ± 0.26 | Glaciological/modeled | Central TS | [18] |
| Sary-Tor Glacier | ~1975–1999 | −0.51 ± 0.25 | Geodetic | Central TS | [17] |
| Karabatkak Glacier | 1957–1998 | −0.44 ± 0.20 1 | Glaciological | Central TS | [4] |
| ~1975–1999 | −0.54 ± 0.25 | Geodetic | [17] | ||
| Tuyuksu glacier | 1964–2018 | −0.43 ± 0.20 1 | Glaciological | Northern TS | [51] |
| Golubin Glacier | 1950–2018 | −0.21 ± 0.42 | Glaciological/modeled | Northern TS | [56] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, C.; Li, Z.; Wang, F.; Mu, J.; Zhang, X. Geodetic Mass Balance of Haxilegen Glacier No. 51, Eastern Tien Shan, from 1964 to 2018. Remote Sens. 2022, 14, 272. https://doi.org/10.3390/rs14020272
Xu C, Li Z, Wang F, Mu J, Zhang X. Geodetic Mass Balance of Haxilegen Glacier No. 51, Eastern Tien Shan, from 1964 to 2018. Remote Sensing. 2022; 14(2):272. https://doi.org/10.3390/rs14020272
Chicago/Turabian StyleXu, Chunhai, Zhongqin Li, Feiteng Wang, Jianxin Mu, and Xin Zhang. 2022. "Geodetic Mass Balance of Haxilegen Glacier No. 51, Eastern Tien Shan, from 1964 to 2018" Remote Sensing 14, no. 2: 272. https://doi.org/10.3390/rs14020272
APA StyleXu, C., Li, Z., Wang, F., Mu, J., & Zhang, X. (2022). Geodetic Mass Balance of Haxilegen Glacier No. 51, Eastern Tien Shan, from 1964 to 2018. Remote Sensing, 14(2), 272. https://doi.org/10.3390/rs14020272