Tropospheric Second-Order Horizontal Gradient Modeling for GNSS PPP
Abstract
1. Introduction
2. Asymmetric Delay Modeling Methods
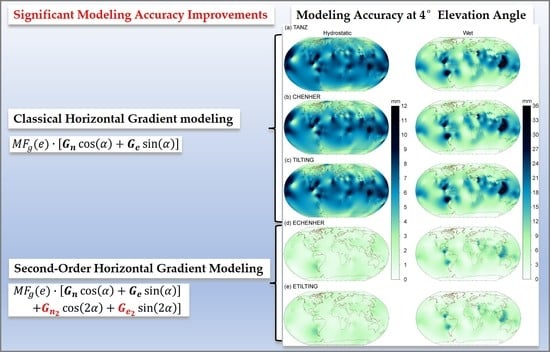
2.1. Classical Methods
2.2. Extended Methods
3. Modeling Evaluations
3.1. Modeling Strategies
3.2. Modeling Residual Evaluations
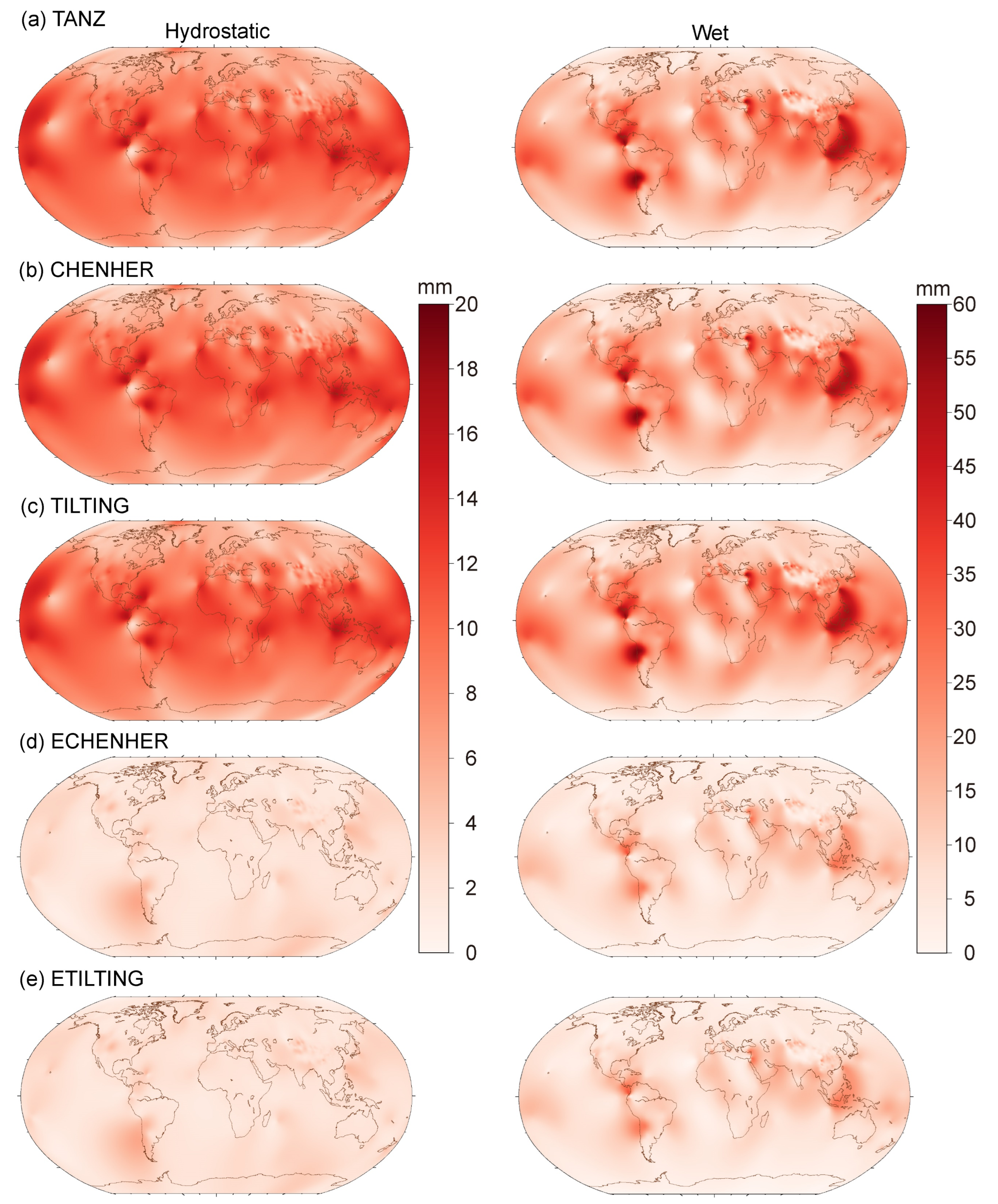
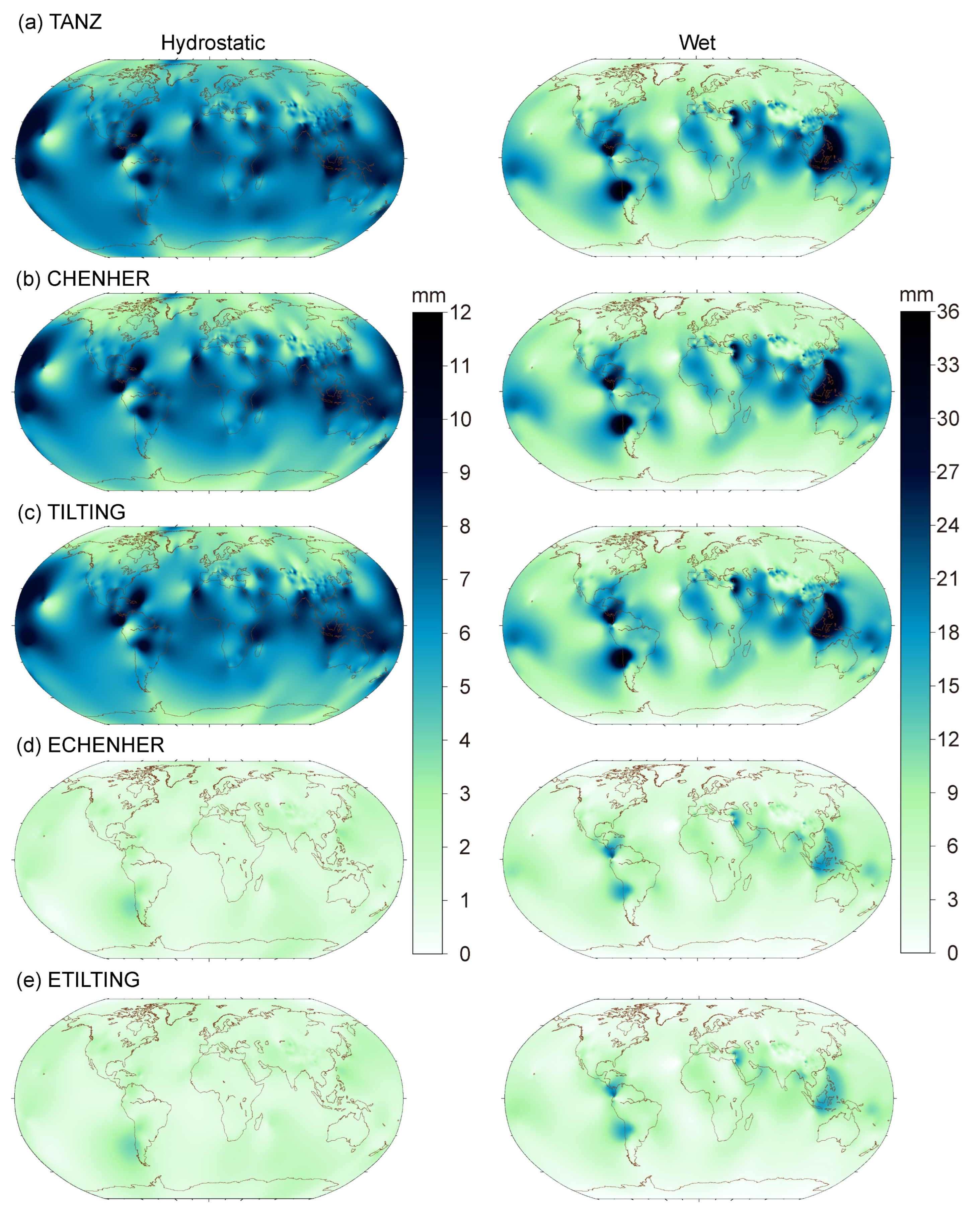
3.3. Modeling Accuracy Evaluations
4. GNSS PPP Validations
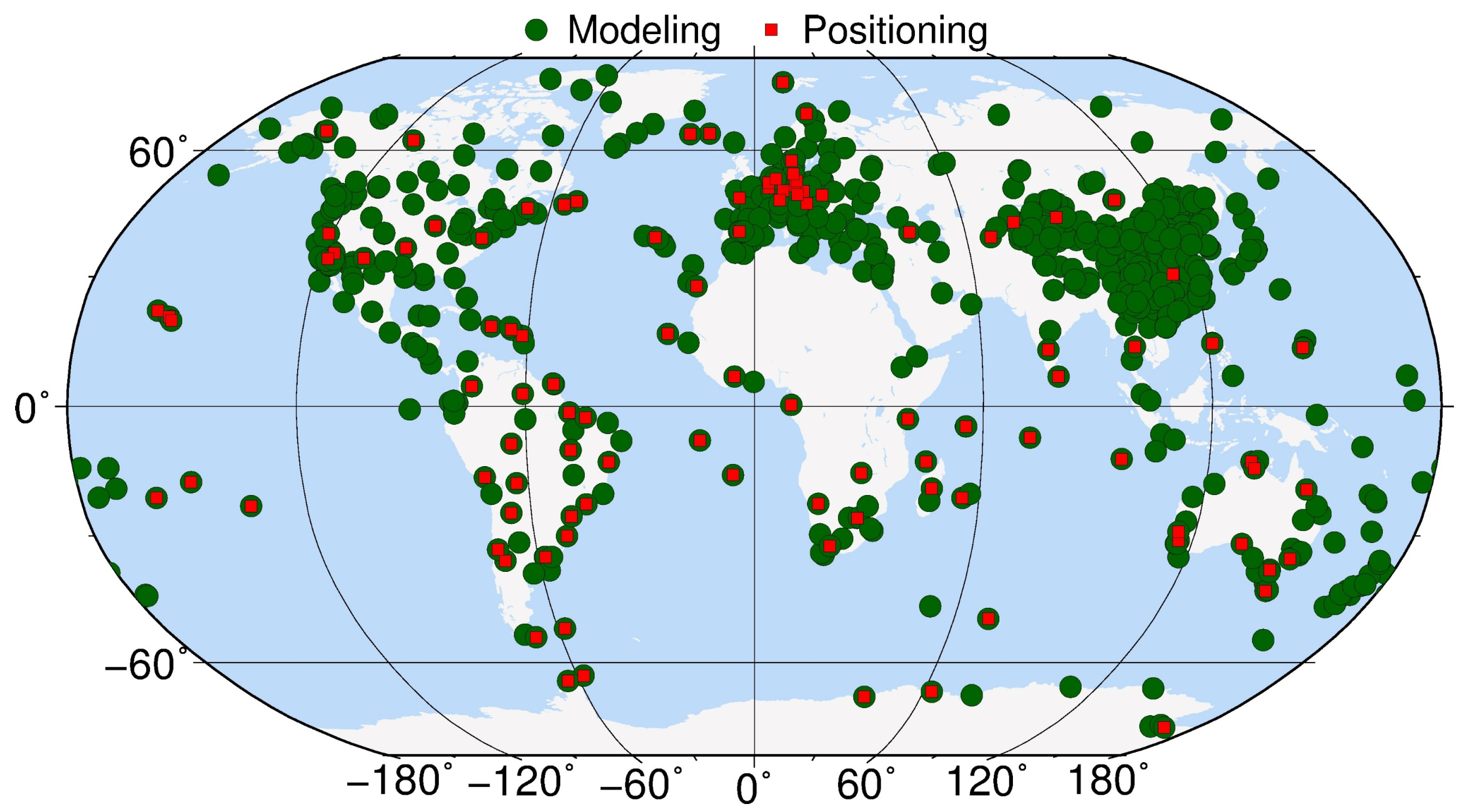
4.1. Data Processing Strategies
4.2. Coordinate Repeatability
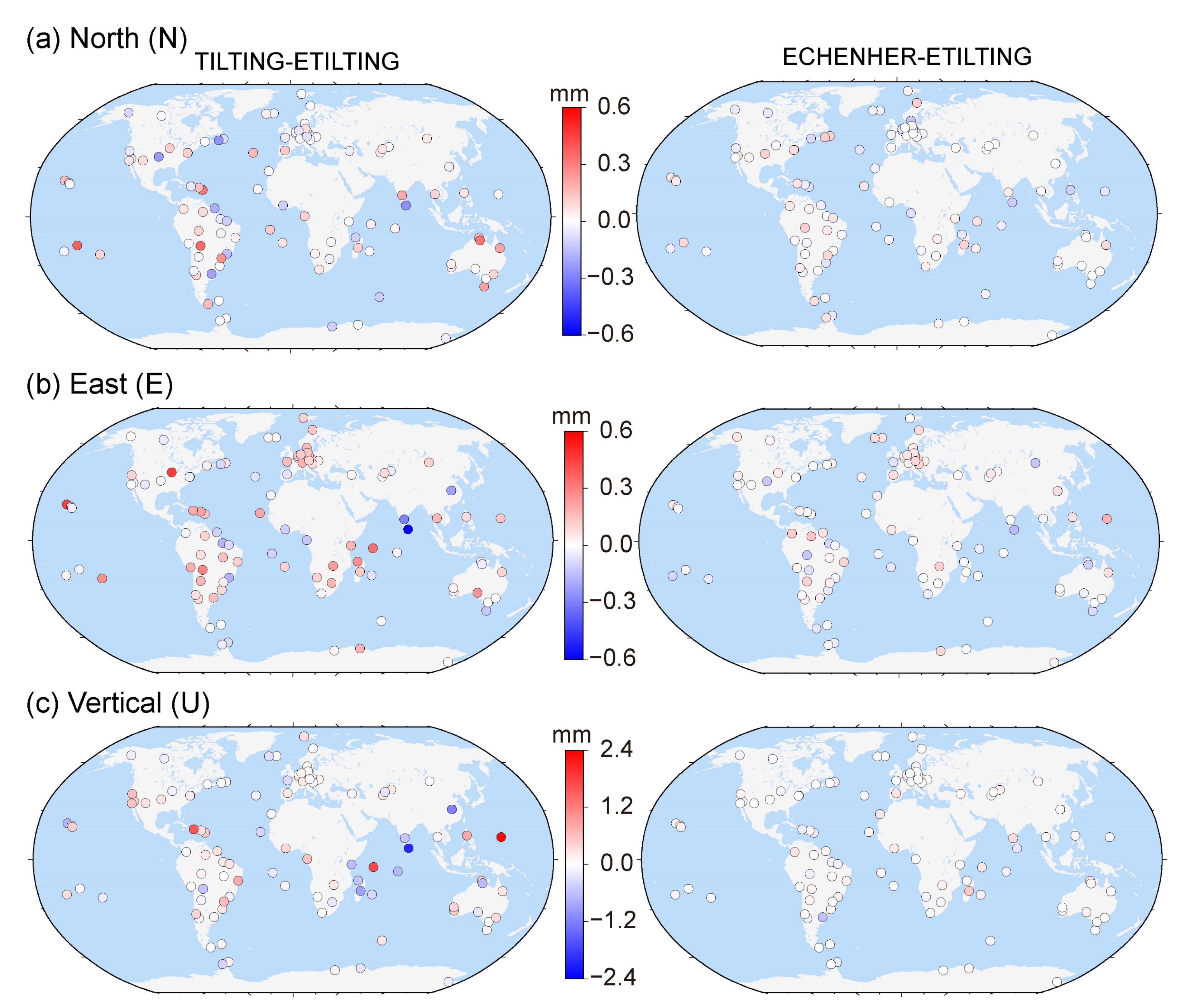
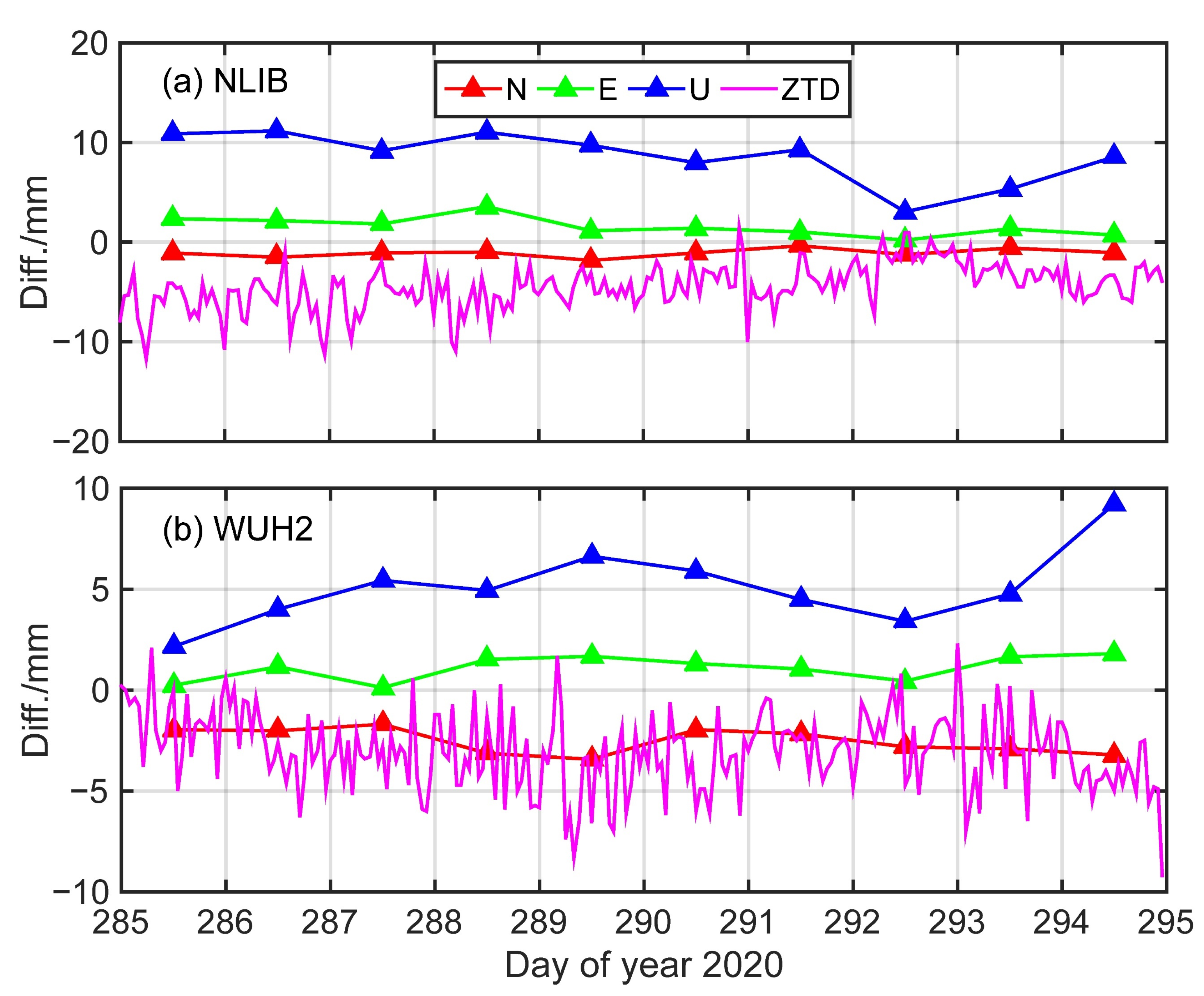
4.3. Coordinate and ZTD Differences
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Landskron, D. Modeling Tropospheric Delays for Space Geodetic Techniques. Doctoral Dissertation, Department of Geodesy and Geoinformation, TU Wien, Vienna, Austria, 2017. Supervisor: Böhm J. Available online: http://repositum.tuwien.ac.at/obvutwhs/content/titleinfo/2099559 (accessed on 7 September 2022).
- Landskron, D.; Böhm, J. Refined discrete and empirical horizontal gradients in VLBI analysis. J. Geod. 2018, 92, 1387–1399. [Google Scholar] [CrossRef]
- Liu, J.; Gao, K.; Guo, W.; Cui, J.; Guo, C. Role, path, and vision of “5G + BDS/GNSS”. Satell. Navig. 2020, 1, 23. [Google Scholar] [CrossRef]
- Huang, L.; Zhu, G.; Liu, L.; Chen, H.; Jiang, W. A global grid model for the correction of the vertical zenith total delay based on a sliding window algorithm. GPS Solut. 2021, 25, 98. [Google Scholar] [CrossRef]
- Huang, L.; Chen, H.; Liu, L.; Jiang, W. A new high-precision global model for calculating zenith tropospheric delay. Chin. J. Geophys.-CH 2021, 64, 782–795. [Google Scholar] [CrossRef]
- Zhu, G.; Huang, L.; Liu, L.; Li, C.; Li, J.; Huang, L.; Zhou, L.; He, H. A new approach for the development of grid models calculating tropospheric key parameters over China. Remote Sens. 2021, 13, 3546. [Google Scholar] [CrossRef]
- Huang, L.; Zhu, G.; Peng, H.; Chen, H.; Liu, L.; Jiang, W. A global grid model for the vertical correction of zenith wet delay based on the sliding window algorithm. Acta Geod. Cartogr. Sin. 2021, 50, 685–694. [Google Scholar] [CrossRef]
- Böhm, J.; Werl, B.; Schuh, H. Troposphere mapping functions for GPS and very long baseline interferometry from European Centre for Medium-Range Weather Forecasts operational analysis data. J. Geophys. Res. Solid Earth 2006, 111, 1–9. [Google Scholar] [CrossRef]
- Landskron, D.; Böhm, J. VMF3/GPT3: Refined discrete and empirical troposphere mapping functions. J. Geod. 2018, 92, 349–360. [Google Scholar] [CrossRef]
- Zhou, Y.; Lou, Y.; Zhang, W.; Bai, J.; Zhang, Z. An improved tropospheric mapping function modeling method for space geodetic techniques. J. Geod. 2021, 95, 98. [Google Scholar] [CrossRef]
- MacMillan, D. Atmospheric gradients from very long baseline interferometry observations. Geophys. Res. Lett. 1995, 22, 1041–1044. [Google Scholar] [CrossRef]
- Chen, G.; Herring, T. Effects of atmospheric azimuthal asymmetry on the analysis of space geodetic data. J. Geophys. Res. Solid Earth 1997, 102, 20489–20502. [Google Scholar] [CrossRef]
- Meindl, M.; Schaer, S.; Hugentobler, U.; Beutler, G. Tropospheric gradient estimation at CODE: Results from global solutions. J. Meteorol. Soc. JPN 2004, 82, 331–338. [Google Scholar] [CrossRef]
- Gegout, P.; Biancale, R.; Soudarin, L. Adaptive mapping functions to the azimuthal anisotropy of the neutral atmosphere. J. Geod. 2011, 85, 661–677. [Google Scholar] [CrossRef]
- Masoumi, S.; McClusky, S.; Koulali, A.; Tregoning, P. A directional model of tropospheric horizontal gradients in Global Positioning System and its application for particular weather scenarios. J. Geophys. Res. Atmos. 2017, 122, 4401–4425. [Google Scholar] [CrossRef]
- Hofmeister, A. Determination of Path Delays in the Atmosphere for Geodetic VLBI by Means of Ray-Tracing. Doctoral Dissertation, Department of Geodesy and Geoinformation, TU Wien, Vienna, Austria, 2016. Supervisor: Böhm J. Available online: http://resolver.obvsg.at/urn:nbn:at:at-ubtuw:1-3444 (accessed on 7 September 2022).
- Hofmeister, A.; Böhm, J. Application of ray-traced tropospheric slant delays to geodetic VLBI analysis. J. Geod. 2017, 91, 945–964. [Google Scholar] [CrossRef] [PubMed]
- Hersbach, H.; Bell, B.; Berrisford, P. The ERA5 global reanalysis. Q. J. Roy. Meteor. Soc. 2020, 146, 1999–2049. [Google Scholar] [CrossRef]
- Huang, L.; Wang, X.; Xiong, S.; Li, J.; Liu, L.; Mo, Z.; Fu, B.; He, H. High-precision GNSS PWV retrieval using dense GNSS sites and in-situ meteorological observations for the evaluation of MERRA-2 and ERA5 reanalysis products over China. Atmos. Res. 2022, 106247. [Google Scholar] [CrossRef]
- Zhou, Y.; Lou, Y.; Zhang, W.; Kuang, C.; Liu, W.; Bai, J. Improved performance of ERA5 in global tropospheric delay retrieval. J. Geod. 2020, 94, 103. [Google Scholar] [CrossRef]
- Urquhart, L.; Nievinski, F.G.; Santos, M.C. Assessment of troposphere mapping functions using three-dimensional ray-tracing. GPS Solut. 2014, 18, 345–354. [Google Scholar] [CrossRef]
- Nikolaidou, T.; Balidakis, K.; Nievinski, F.G.; Santos, M.C.; Schuh, H. Impact of different NWM-derived mapping functions on VLBI and GPS analysis. Earth Planets Space 2018, 70, 95. [Google Scholar] [CrossRef]
- Shi, C.; Zhao, Q.; Geng, J.; Lou, Y.; Ge, M.; Liu, J. Recent development of PANDA software in GNSS data processing. In International Conference on Earth Observation Data Processing and Analysis (ICEODPA); International Society for Optics and Photonics: Bellingham, WA, USA, 2008; Volume 7285, p. 72851S. [Google Scholar] [CrossRef]
- Montenbruck, O.; Steigenberger, P.; Prange, L.; Deng, Z.; Zhao, Q.; Perosanz, F.; Romero, I.; Noll, C.; Stürze, A.; Weber, G. The Multi-GNSS Experiment (MGEX) of the International GNSS Service (IGS)–achievements, prospects and challenges. Adv. Space Res. 2017, 59, 1671–1697. [Google Scholar] [CrossRef]
- Fritsche, M.; Dietrich, R.; Knöfel, C.; Rülke, A.; Vey, S.; Rothacher, M.; Steigenberger, P. Impact of higher-order ionospheric terms on GPS estimates. Geophys. Res. Lett. 2005, 32, 1–5. [Google Scholar] [CrossRef]
- Tregoning, P.; Dam, T.V. Atmospheric pressure loading corrections applied to GPS data at the observation level. Geophys. Res. Lett. 2005, 32, 1–4. [Google Scholar] [CrossRef]
- Wijaya, D.D.; Böhm, J.; Karbon, M.; Kràsnà, H.; Schuh, H. Atmospheric pressure loading. In Atmospheric Effects in Space Geodesy; Springer: Berlin/Heidelberg, Germany, 2013; pp. 137–157. Available online: https://link.springer.com/book/10.1007/978-3-642-36932-2 (accessed on 7 September 2022).
- Tregoning, P.; Herring, T.A. Impact of a priori zenith hydrostatic delay errors on GPS estimates of station heights and zenith total delays. Geophys. Res. Lett. 2006, 33, 1–5. [Google Scholar] [CrossRef]

| Types | Methods | Gradient Mapping Function | Higher-Order Variation | Parameters | Number of Parameters |
|---|---|---|---|---|---|
| Classical | TANZ | 2 | |||
| CHENHER | 2 | ||||
| TILTING | 2 | ||||
| Extended | ECHENHER | √ | , , | 4 | |
| ETILTING | √ | , , | 4 |
| Methods | Components | e = 3° | e = 5° | e = 7° | e = 10° | e = 15° | e = 30° | e = 70° |
|---|---|---|---|---|---|---|---|---|
| TANZ | Hydrostatic | 8.78 | 5.01 | 3.64 | 2.23 | 1.14 | 0.31 | 0.04 |
| CHENHER | 8.49 | 3.63 | 2.11 | 1.21 | 0.63 | 0.18 | 0.02 | |
| TILTING | 8.53 | 3.75 | 2.25 | 1.30 | 0.67 | 0.19 | 0.02 | |
| ECHENHER | 2.39 | 1.72 | 1.66 | 1.22 | 0.71 | 0.22 | 0.03 | |
| ETILTING | 2.45 | 1.92 | 1.80 | 1.29 | 0.74 | 0.22 | 0.03 | |
| TANZ | Wet | 17.79 | 8.57 | 5.62 | 3.29 | 1.64 | 0.44 | 0.05 |
| CHENHER | 17.73 | 8.04 | 5.18 | 3.04 | 1.53 | 0.41 | 0.05 | |
| TILTING | 17.67 | 7.55 | 4.66 | 2.70 | 1.36 | 0.37 | 0.04 | |
| ECHENHER | 8.04 | 5.50 | 4.54 | 2.96 | 1.57 | 0.44 | 0.05 | |
| ETILTING | 7.98 | 4.83 | 4.03 | 2.68 | 1.45 | 0.41 | 0.05 |
| Methods | Components | e = 4° | e = 6° | e = 8° | e = 12° | e = 20° | e = 50° | e = 80° |
|---|---|---|---|---|---|---|---|---|
| TANZ | Hydrostatic | 5.81 | 4.30 | 3.08 | 1.67 | 0.68 | 0.11 | 0.03 |
| CHENHER | 5.27 | 2.69 | 1.71 | 0.91 | 0.39 | 0.07 | 0.02 | |
| TILTING | 5.31 | 2.84 | 1.84 | 0.97 | 0.41 | 0.07 | 0.02 | |
| ECHENHER | 1.51 | 1.76 | 1.52 | 0.98 | 0.45 | 0.08 | 0.02 | |
| ETILTING | 1.63 | 1.93 | 1.62 | 1.02 | 0.47 | 0.08 | 0.03 | |
| TANZ | Wet | 11.14 | 6.89 | 4.64 | 2.43 | 0.97 | 0.15 | 0.03 |
| CHENHER | 10.84 | 6.37 | 4.28 | 2.25 | 0.90 | 0.14 | 0.03 | |
| TILTING | 10.64 | 5.81 | 3.82 | 2.00 | 0.81 | 0.13 | 0.03 | |
| ECHENHER | 5.31 | 5.13 | 3.95 | 2.26 | 0.95 | 0.15 | 0.03 | |
| ETILTING | 4.91 | 4.51 | 3.53 | 2.07 | 0.88 | 0.14 | 0.03 |
| Observation | Sampling interval | 300 s |
| Frequency combination | Ionosphere-free combination | |
| Elevation cutoff angle | 3° | |
| Satellite system weighting factors | GPS: 1; GALILEO: 1; GLONASS: 1.5; BDS MEO and IGSO: 1.5; BDS GEO: 2.5 | |
| Elevation weighting strategy | ||
| Error correction | Phase center variations | igs14.atx |
| Higher-order ionospheric delay | GIM and IGRF13 [25] | |
| Ocean tide loading | FES2014b | |
| A priori tropospheric delay | MFlsmcom+ZPD [10] | |
| Parameter estimation | Satellite orbits | Fixed from GBM 5 min products |
| Satellite clock corrections | Fixed from estimated 5 min products | |
| Mapping function | Wet MFlsmcom [10] | |
| ZWD stochastic model | Piece-wise constant (1 h), random walk between segments () | |
| Gradient mapping function | Scheme 1 NONE: NONE Scheme 2 TANZ: Scheme 3 Scheme 4 Scheme 5 Scheme 6 | |
| Gradient stochastic model | Piece-wise constant (2 h), random walk between segments () | |
| Horizontal gradient | Scheme 1 NONE: NONE Scheme 2 Scheme 3 Scheme 4 Scheme 5 Scheme 6 | |
| Station coordinates | Daily constant | |
| Receiver clock corrections | White noise | |
| Ambiguities | Fixed |
| Schemes | Without APL Correction | With APL Correction | ||||
|---|---|---|---|---|---|---|
| N | E | U | N | E | U | |
| NONE | 1.82 | 1.48 | 4.10 | 1.84 | 1.52 | 3.83 |
| TANZ | 0.92 | 0.88 | 3.39 | 0.95 | 0.94 | 3.10 |
| CHENHER | 0.91 | 0.88 | 3.41 | 0.94 | 0.94 | 3.13 |
| TILTING | 0.91 | 0.87 | 3.40 | 0.94 | 0.94 | 3.11 |
| ECHENHER | 0.89 | 0.85 | 3.37 | 0.93 | 0.90 | 3.09 |
| ETILTING | 0.88 | 0.84 | 3.35 | 0.92 | 0.89 | 3.06 |
| Components | TILTING-ETILTING | ECHENHER-ETILTING | ||
|---|---|---|---|---|
| Bias | RMS | Bias | RMS | |
| N | −0.04 | 0.36 | 0.01 | 0.16 |
| E | −0.07 | 0.44 | 0.02 | 0.18 |
| U | 0.49 | 1.45 | −0.01 | 0.39 |
| ZTD | −0.19 | 0.99 | −0.01 | 0.30 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Y.; Lou, Y.; Zhang, W.; Wu, P.; Bai, J.; Zhang, Z. Tropospheric Second-Order Horizontal Gradient Modeling for GNSS PPP. Remote Sens. 2022, 14, 4807. https://doi.org/10.3390/rs14194807
Zhou Y, Lou Y, Zhang W, Wu P, Bai J, Zhang Z. Tropospheric Second-Order Horizontal Gradient Modeling for GNSS PPP. Remote Sensing. 2022; 14(19):4807. https://doi.org/10.3390/rs14194807
Chicago/Turabian StyleZhou, Yaozong, Yidong Lou, Weixing Zhang, Peida Wu, Jingna Bai, and Zhenyi Zhang. 2022. "Tropospheric Second-Order Horizontal Gradient Modeling for GNSS PPP" Remote Sensing 14, no. 19: 4807. https://doi.org/10.3390/rs14194807
APA StyleZhou, Y., Lou, Y., Zhang, W., Wu, P., Bai, J., & Zhang, Z. (2022). Tropospheric Second-Order Horizontal Gradient Modeling for GNSS PPP. Remote Sensing, 14(19), 4807. https://doi.org/10.3390/rs14194807