Using Multi-Source Real Landform Data to Predict and Analyze Intercity Remote Interference of 5G Communication with Ducting and Troposcatter Effects
Abstract
:1. Introduction
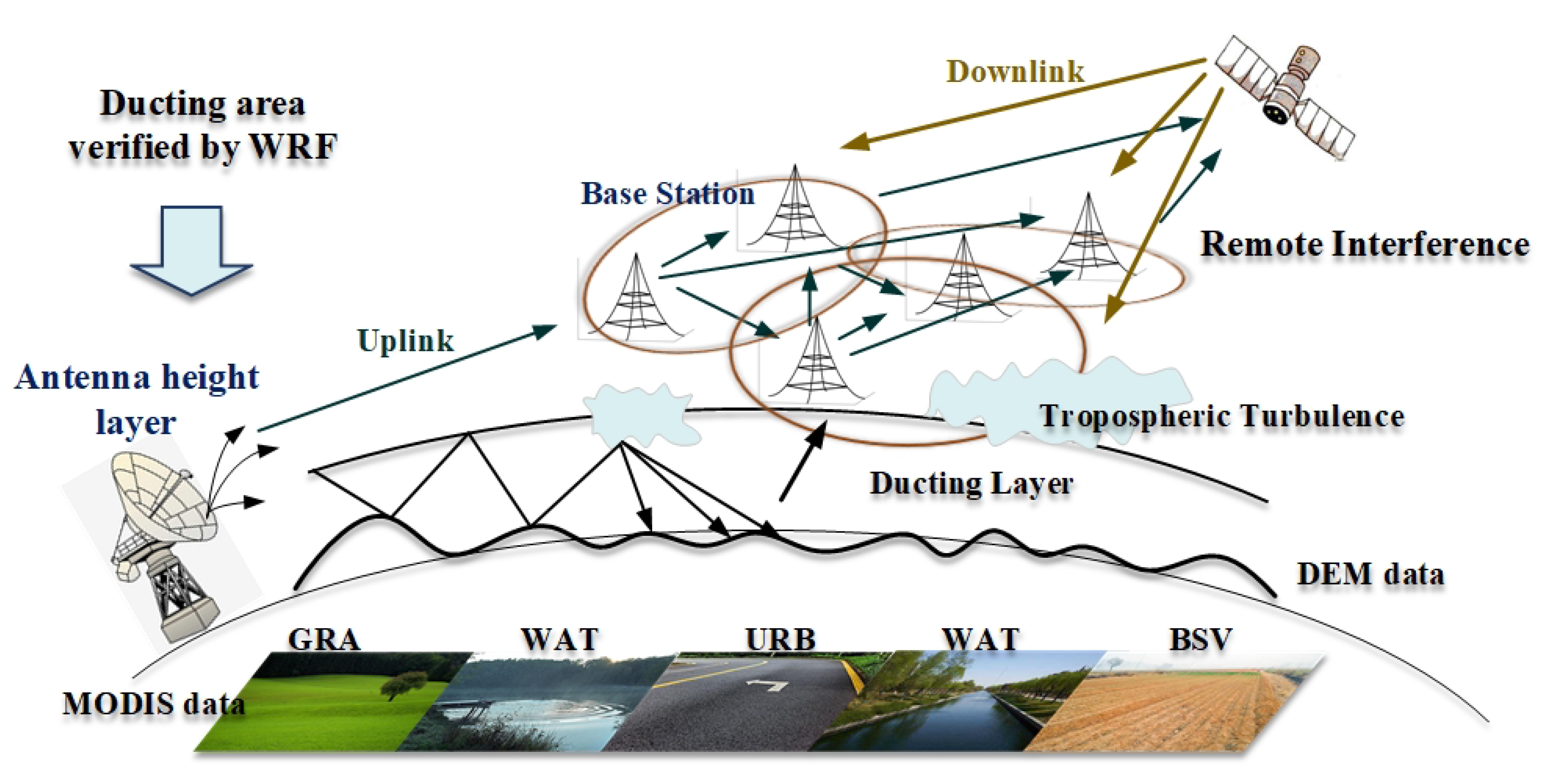
2. A Multi-Source Real Data Model for Intercity Link Communication Propagation Computing
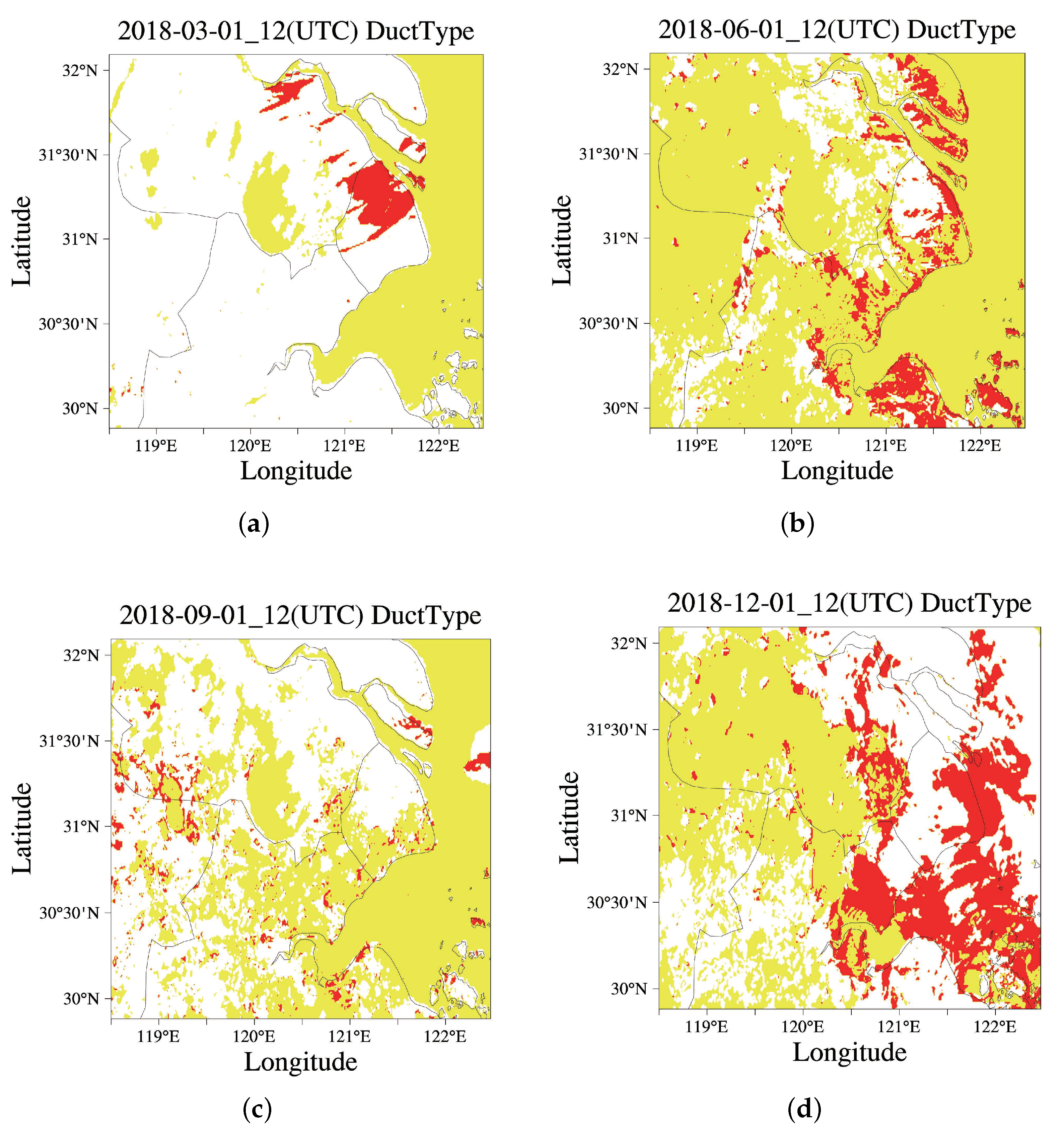
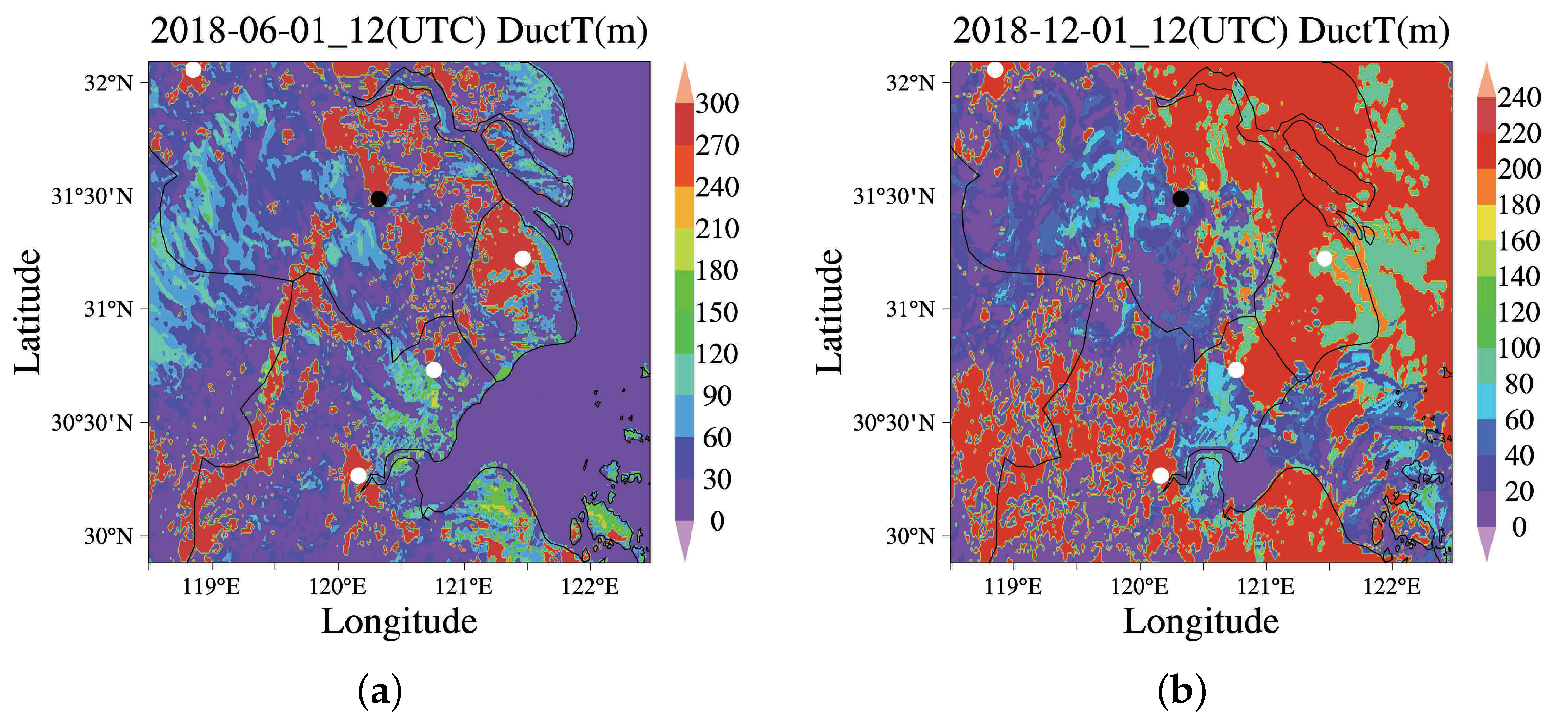
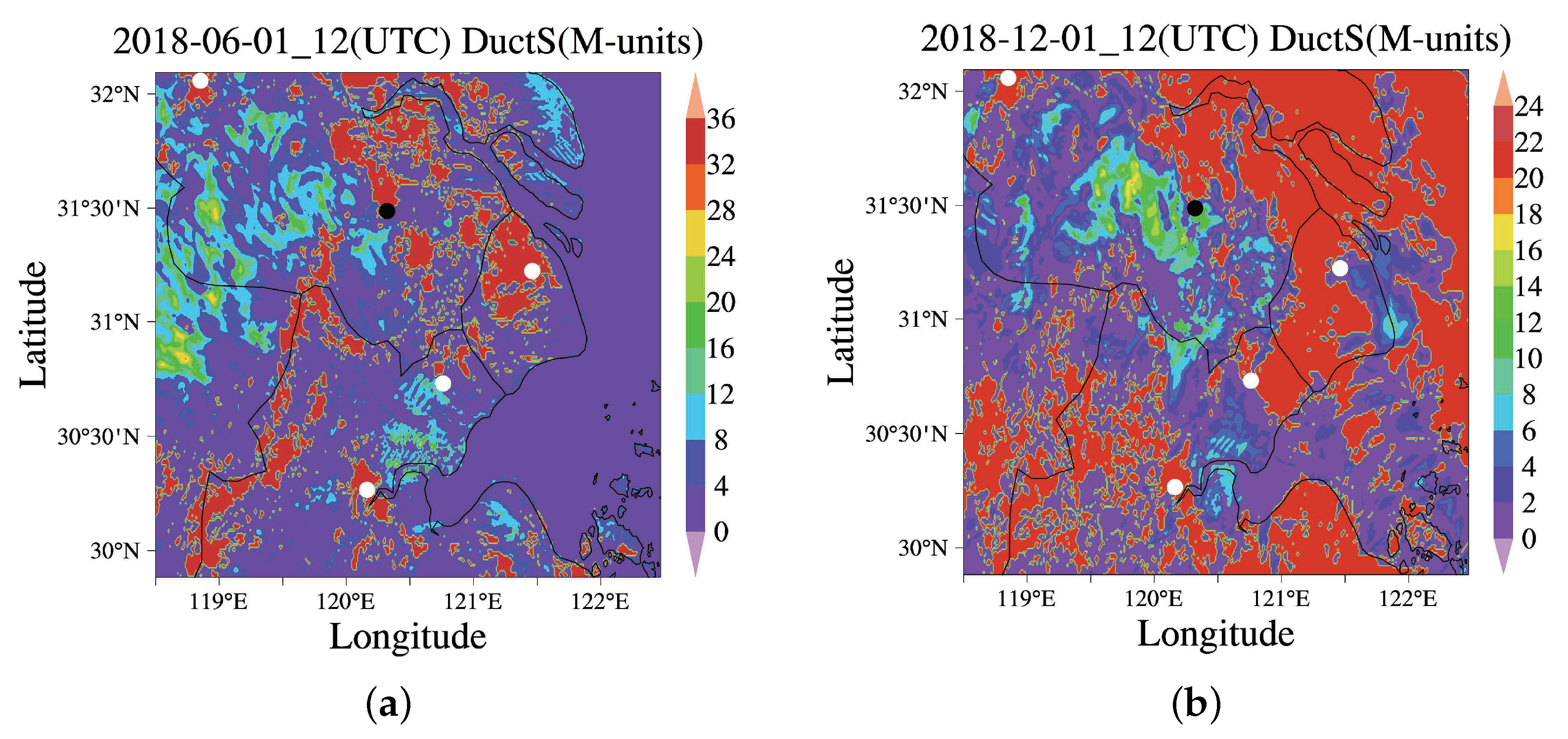
2.1. WRF Models Regional Duct Distribution
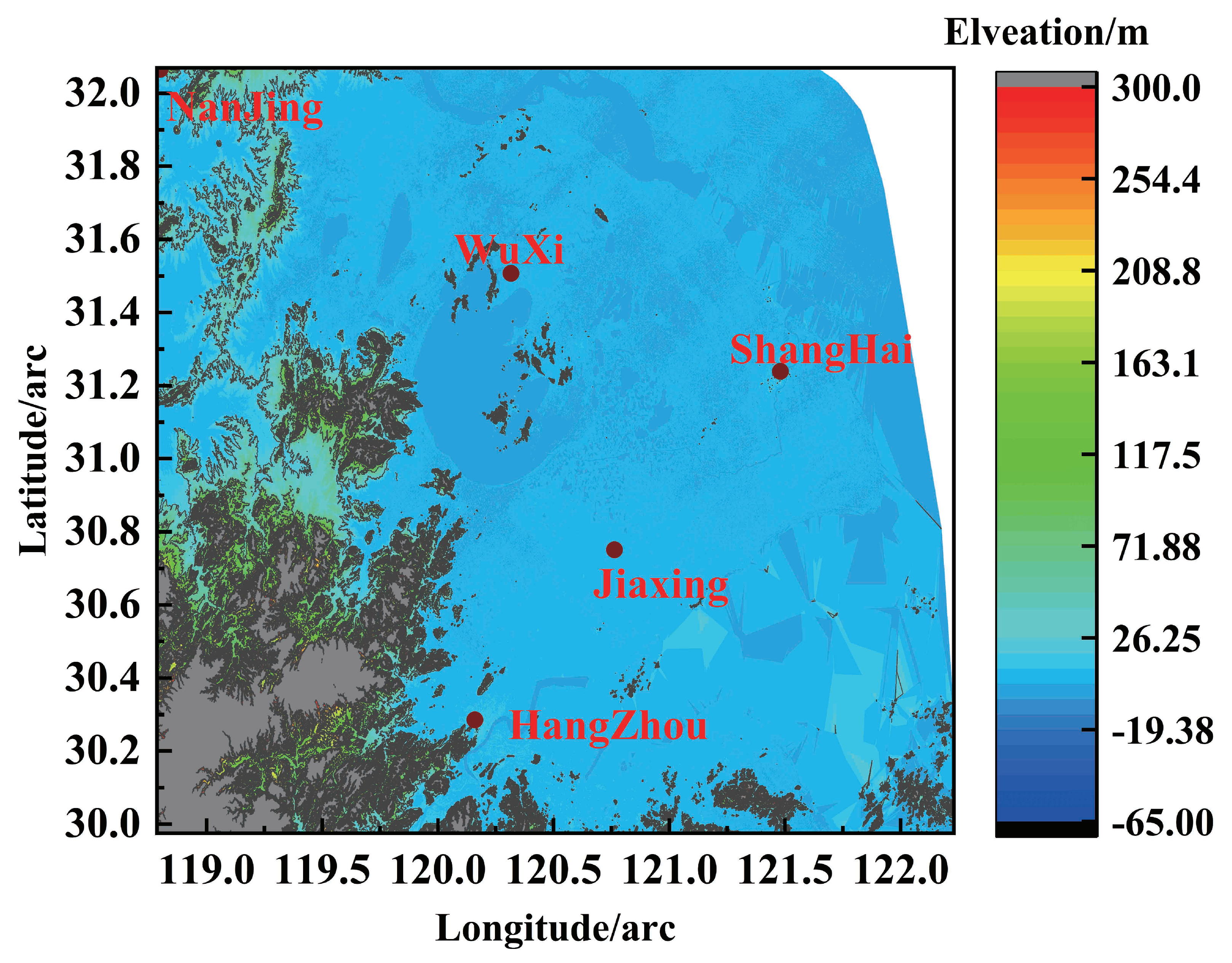
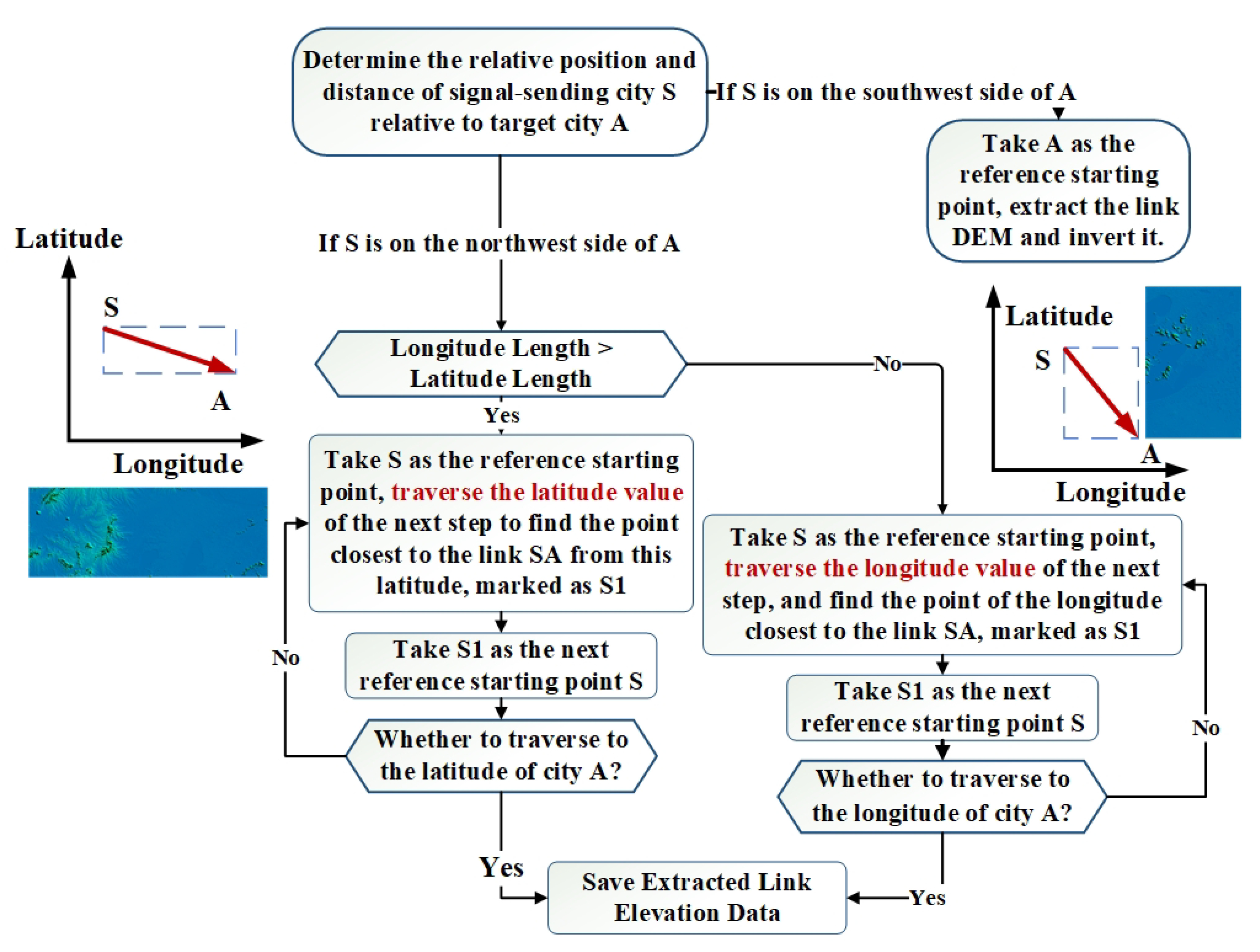
2.2. Extraction of Digital Elevation Data on Intercity Link
2.3. Data Extraction of Land Cover Distribution Types of Intercity Links from MODIS
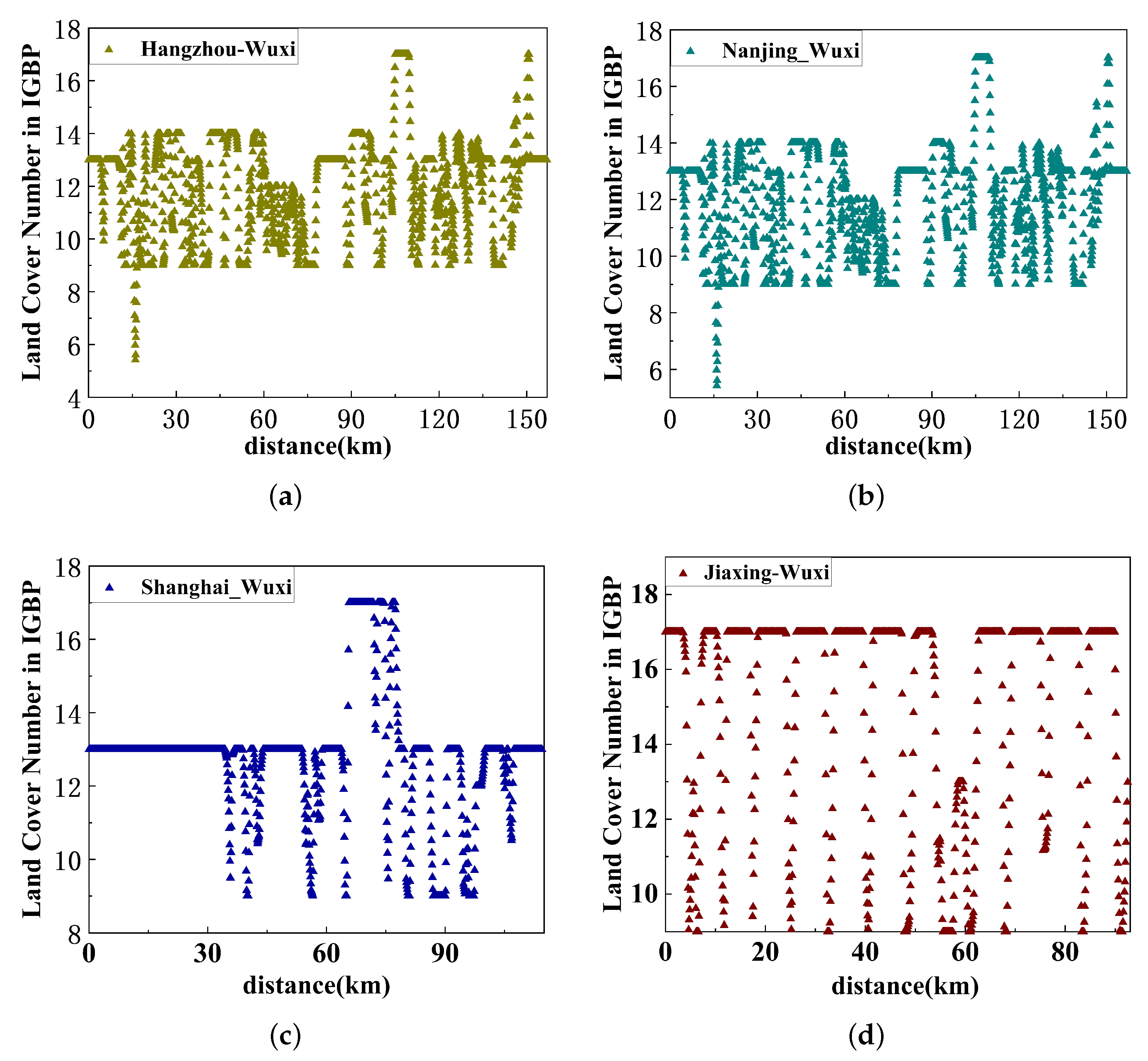
2.3.1. Land Cover Type Distribution on Intercity Links of East China
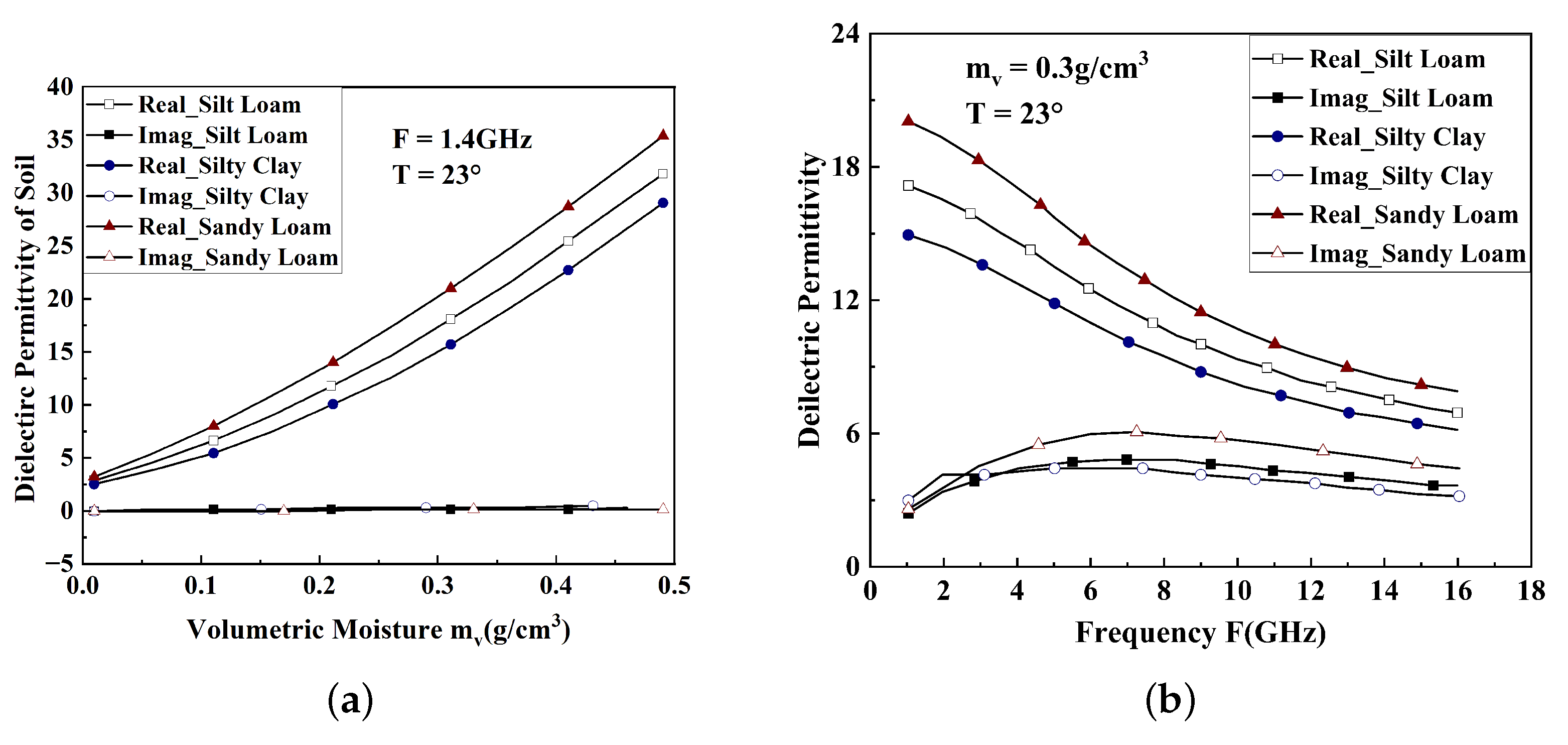
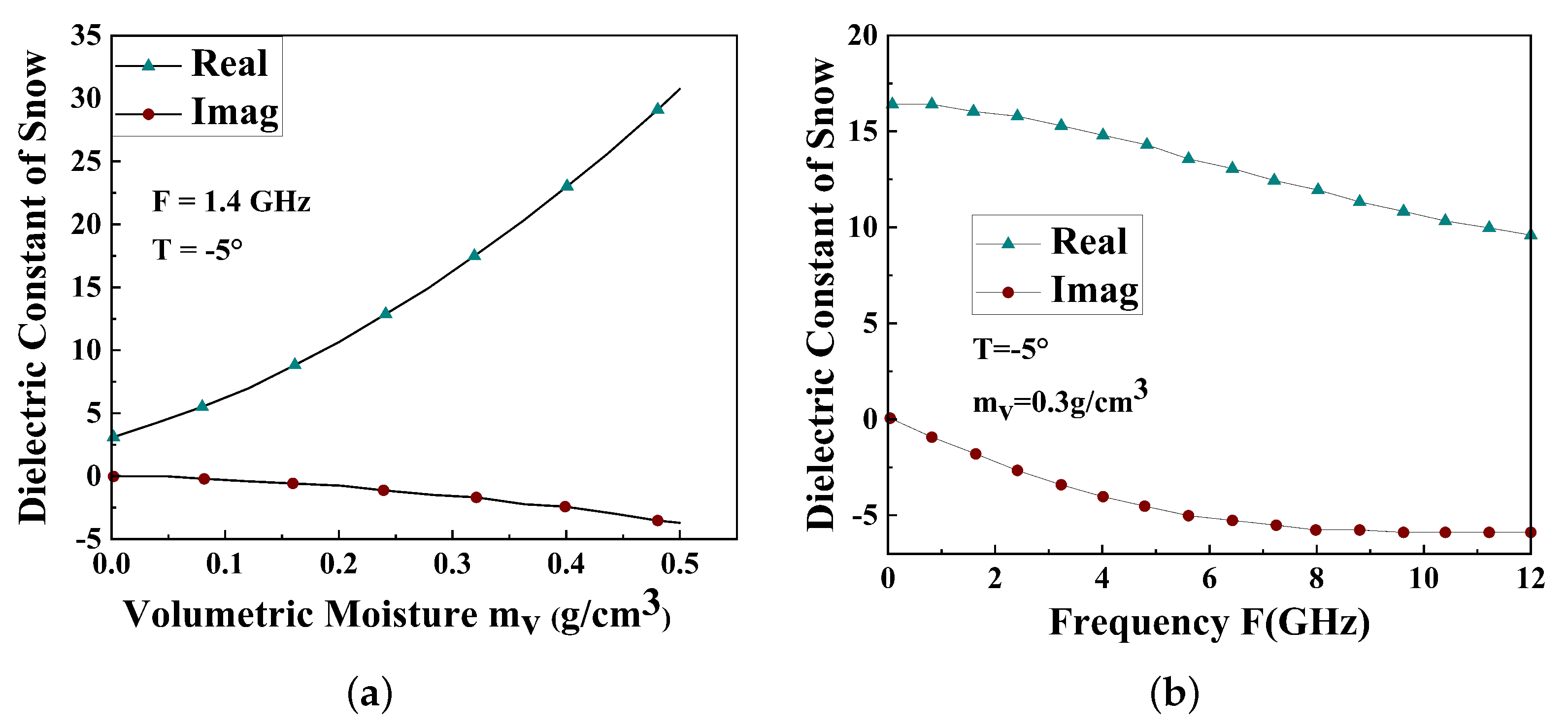
2.3.2. Determination of Corresponding Dielectric Parameters in the Area Covered by the Intercity Link
3. Scattering Parabolic Equation Algorithm for Irregular Terrain and Inhomogeneous Turbulent Atmosphere
4. Propagation Loss Calculation Using the Improved TWPE Model
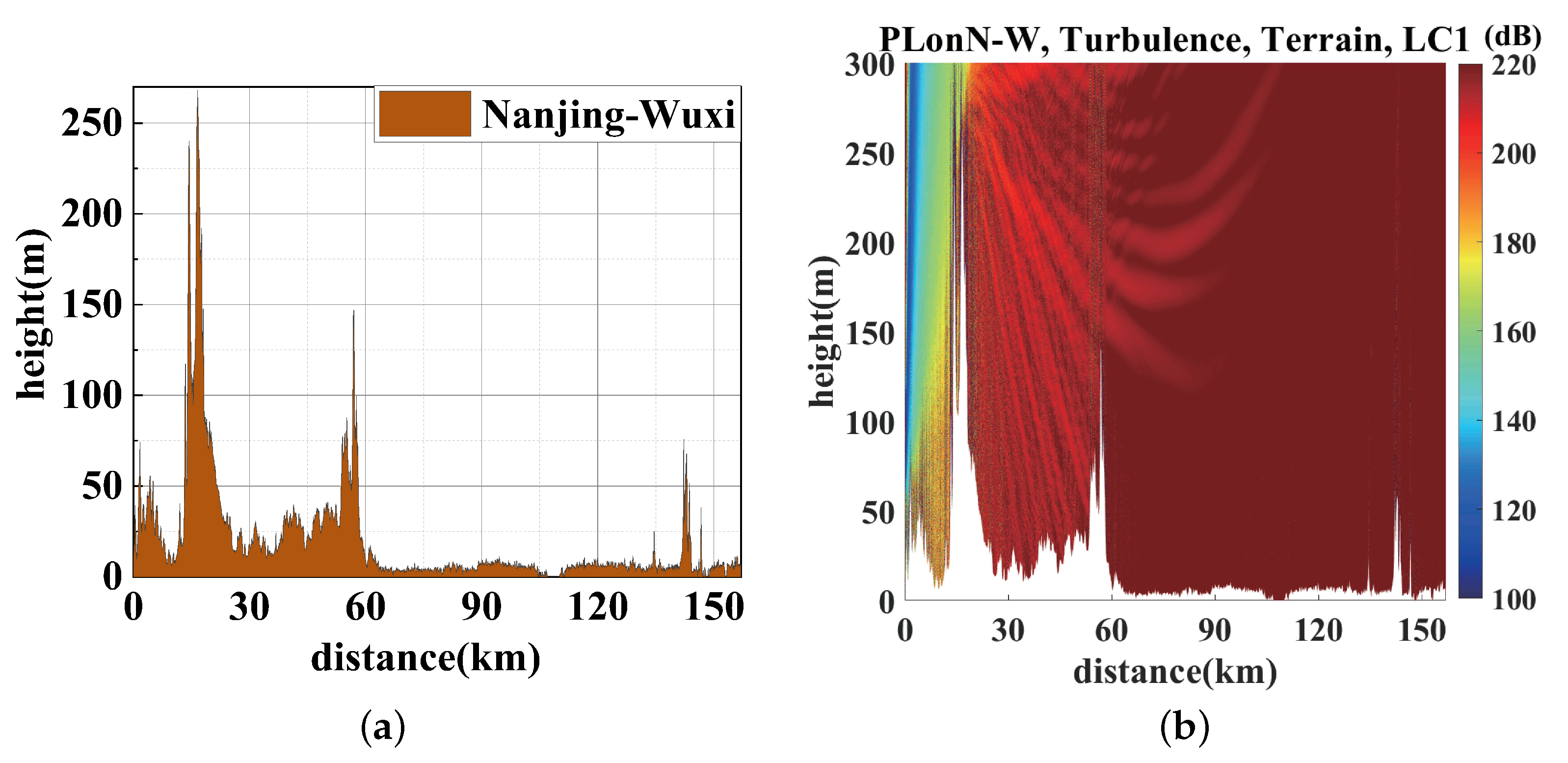
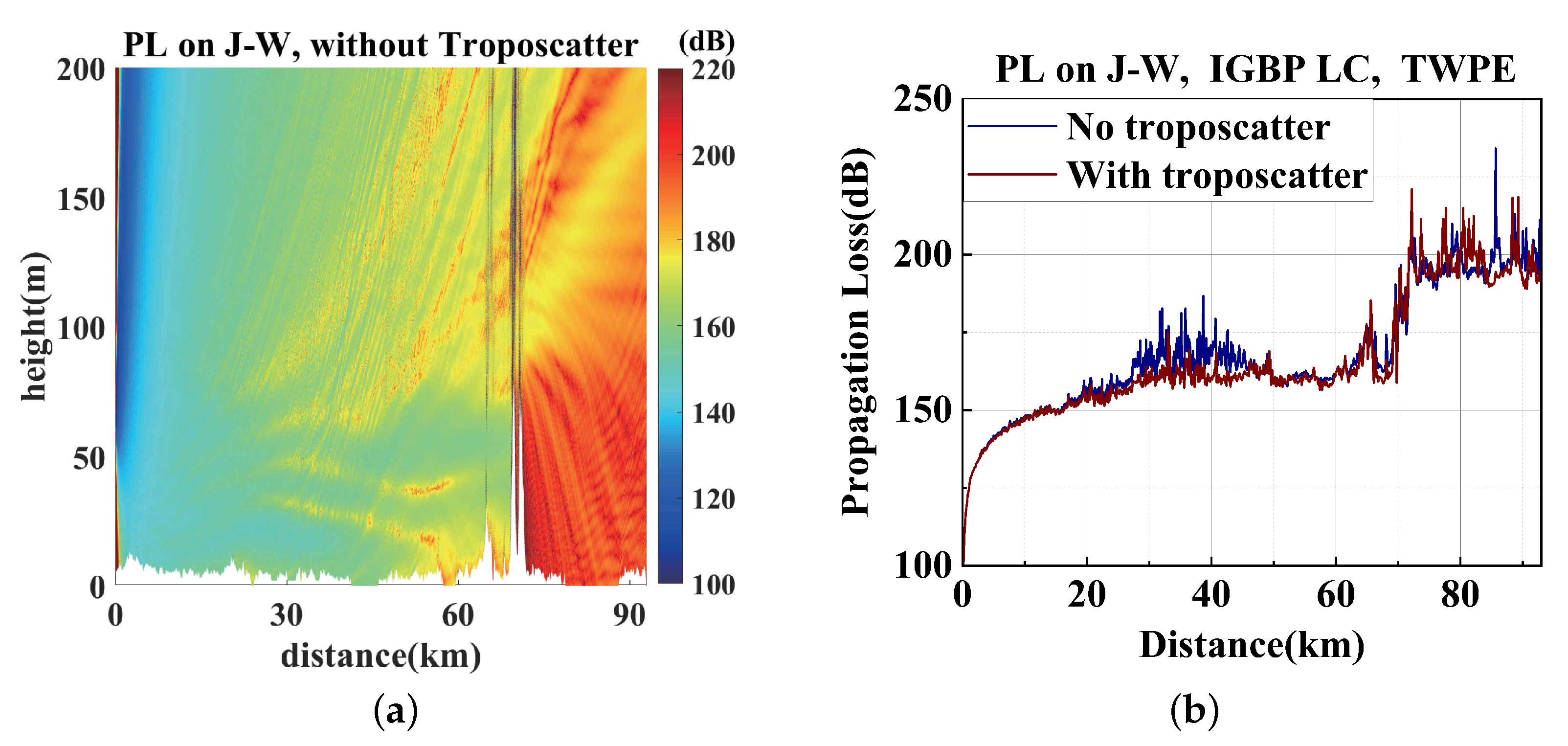
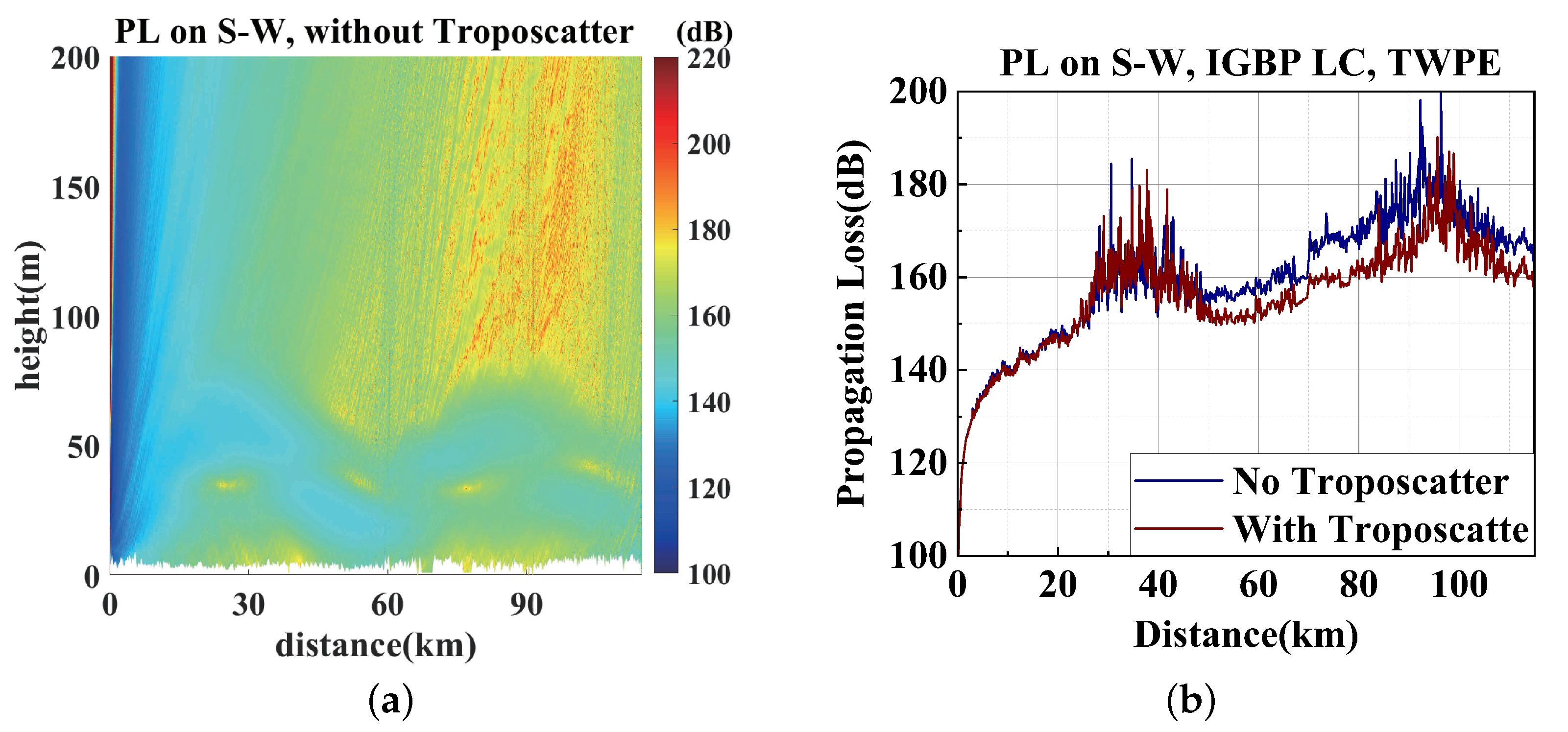
4.1. Joint Effects of Tropospheric Turbulence and Duct
4.2. Verification of TWPE
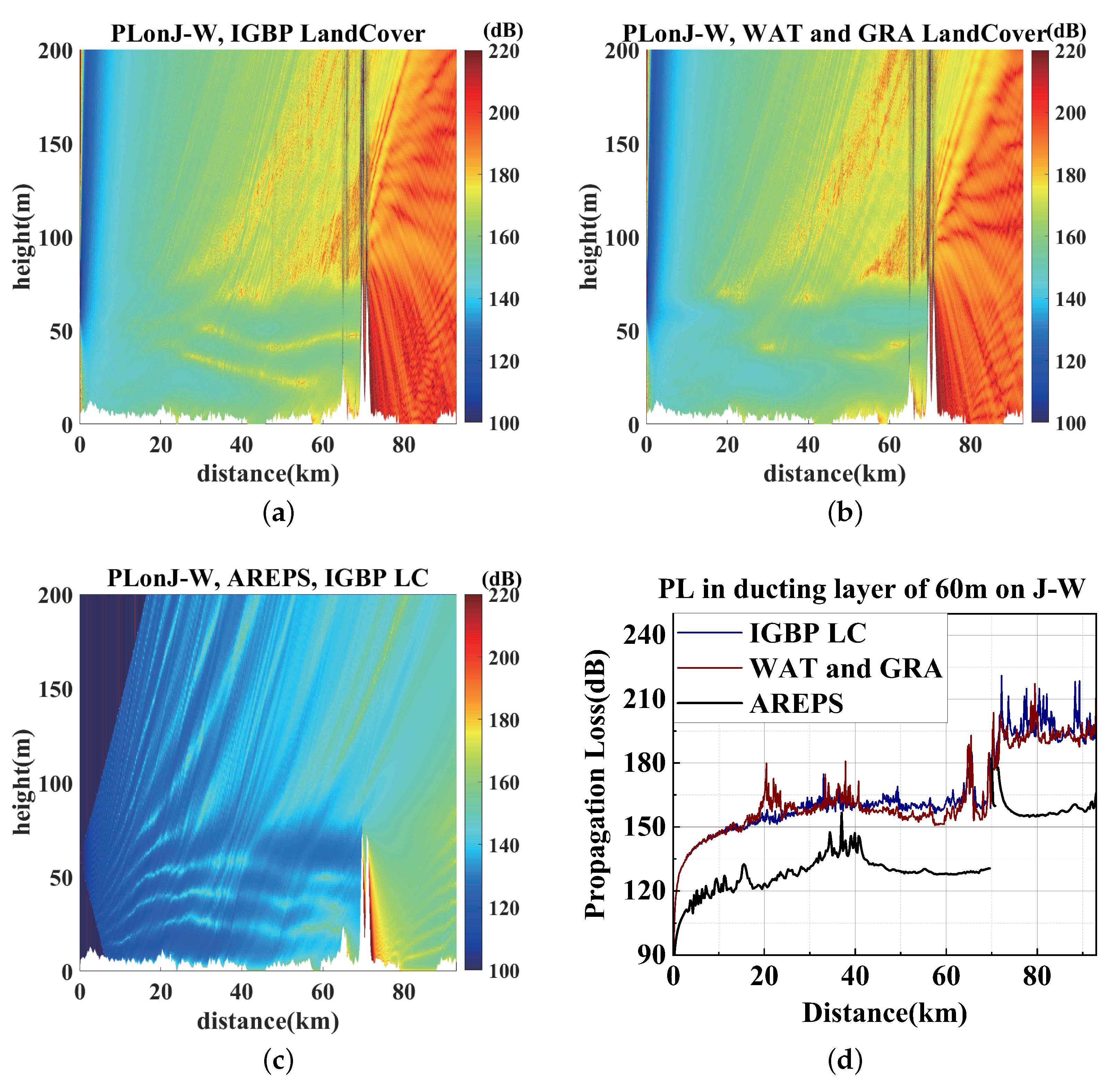
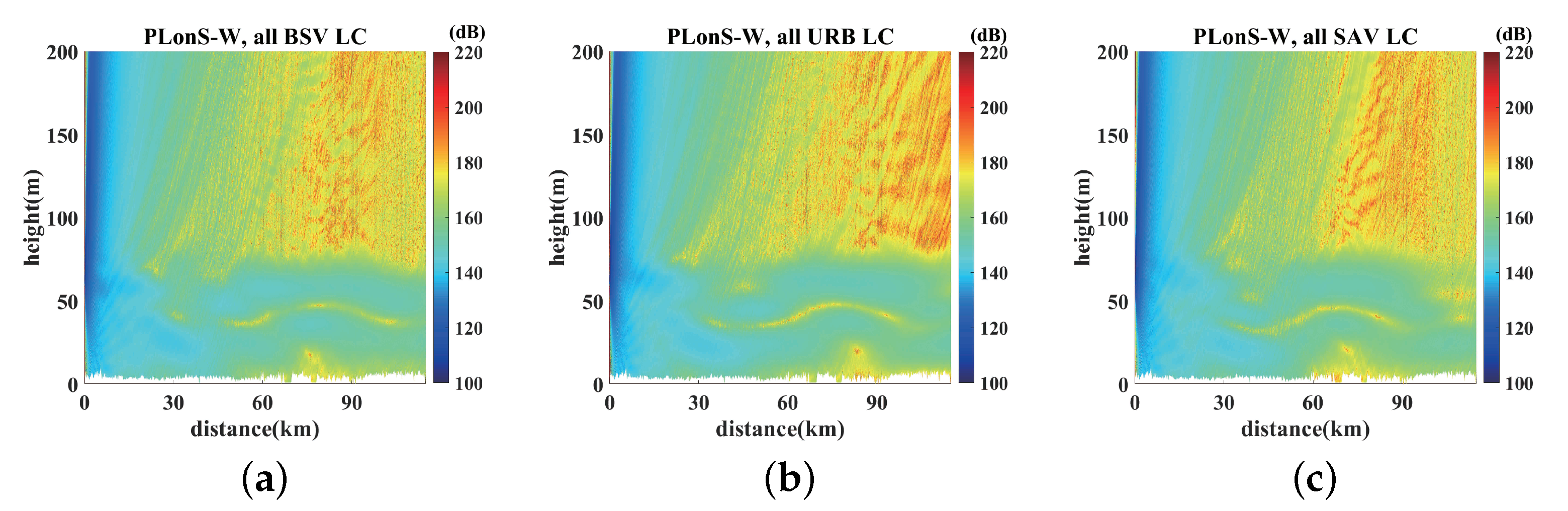
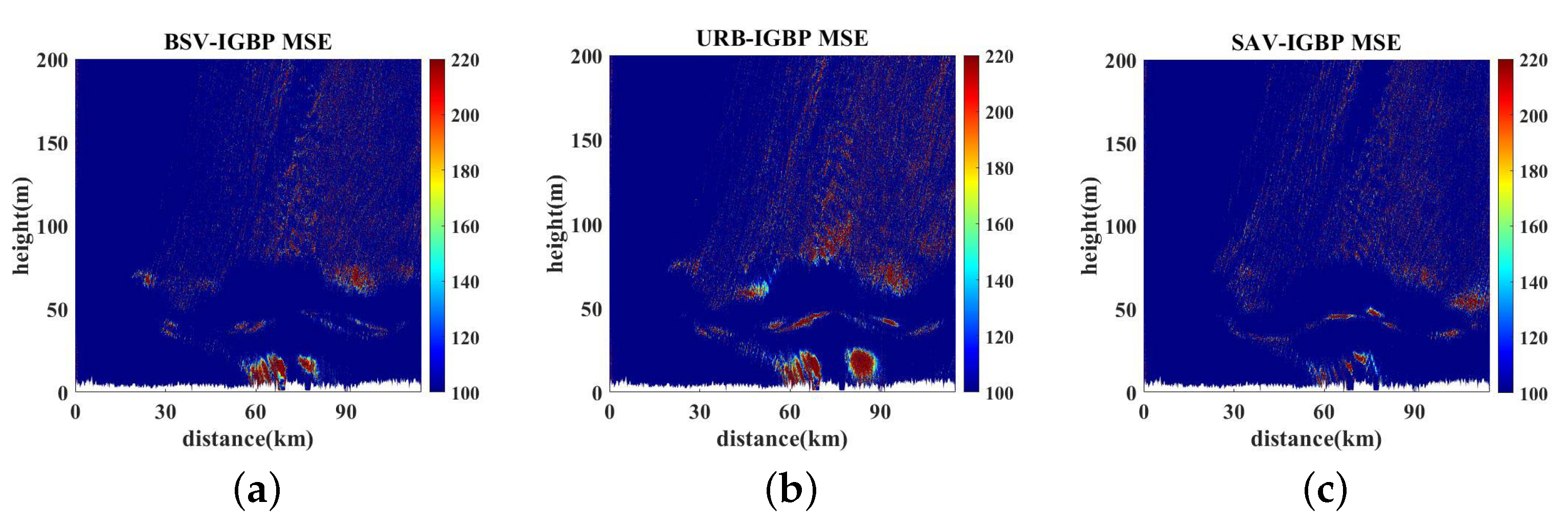
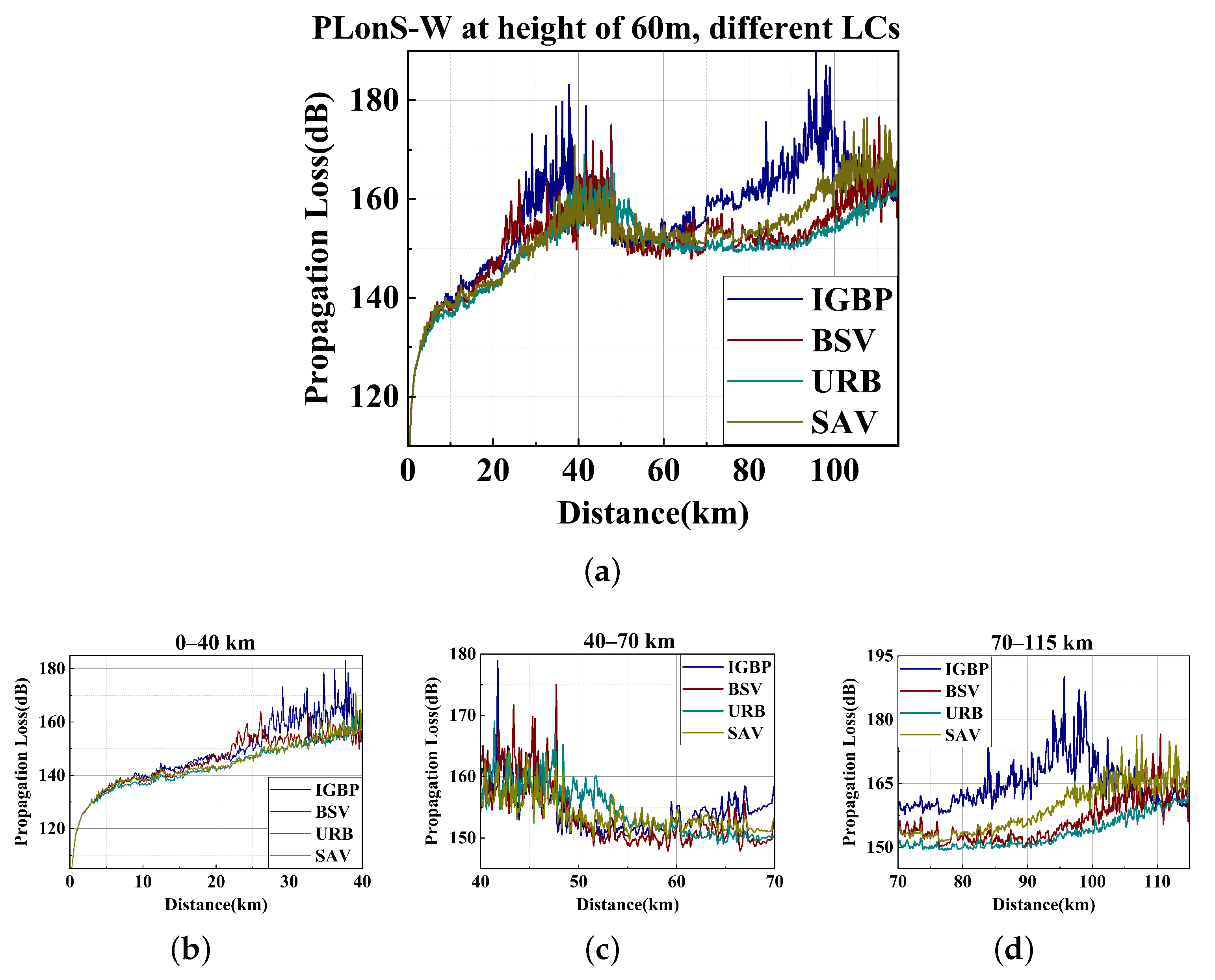
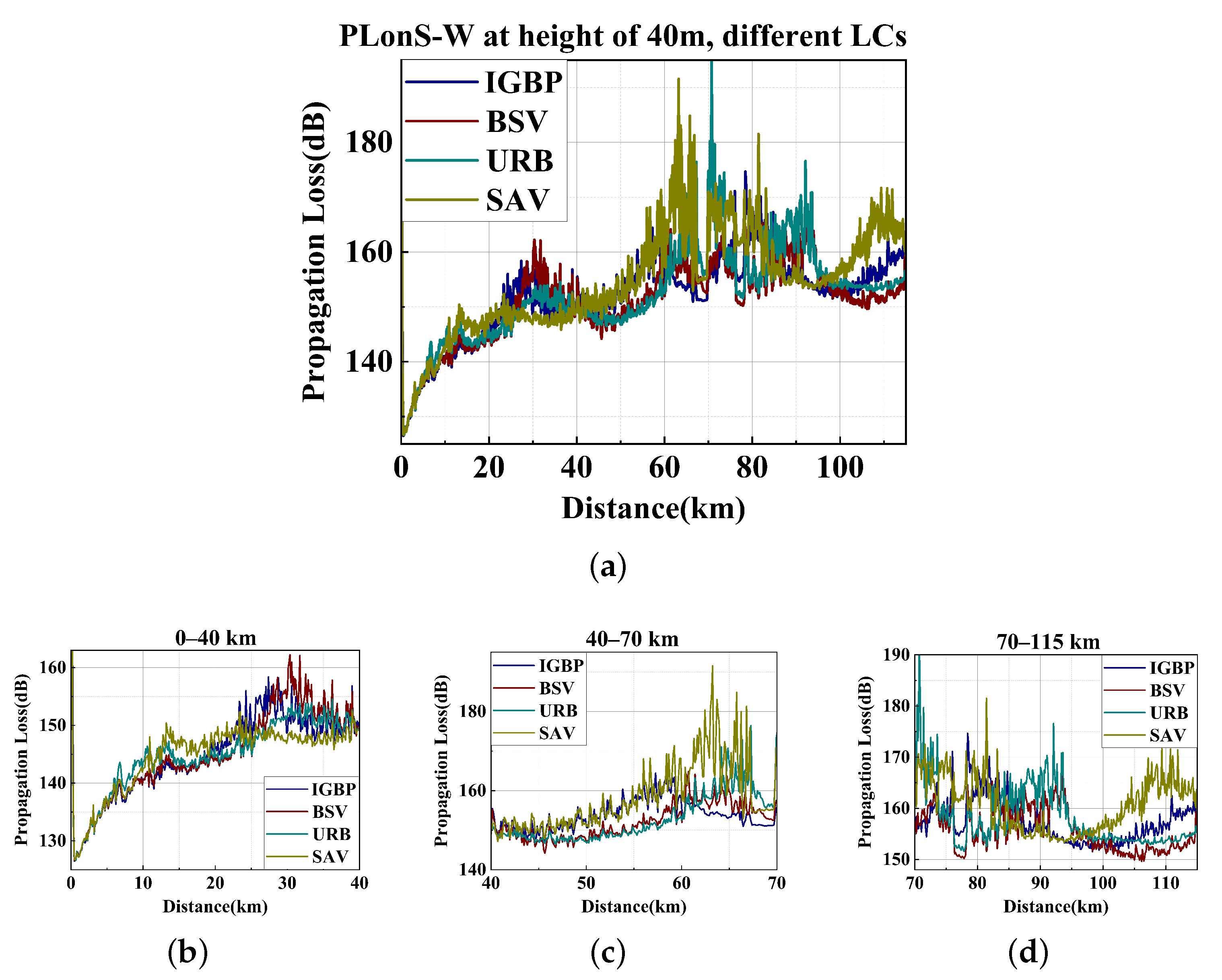
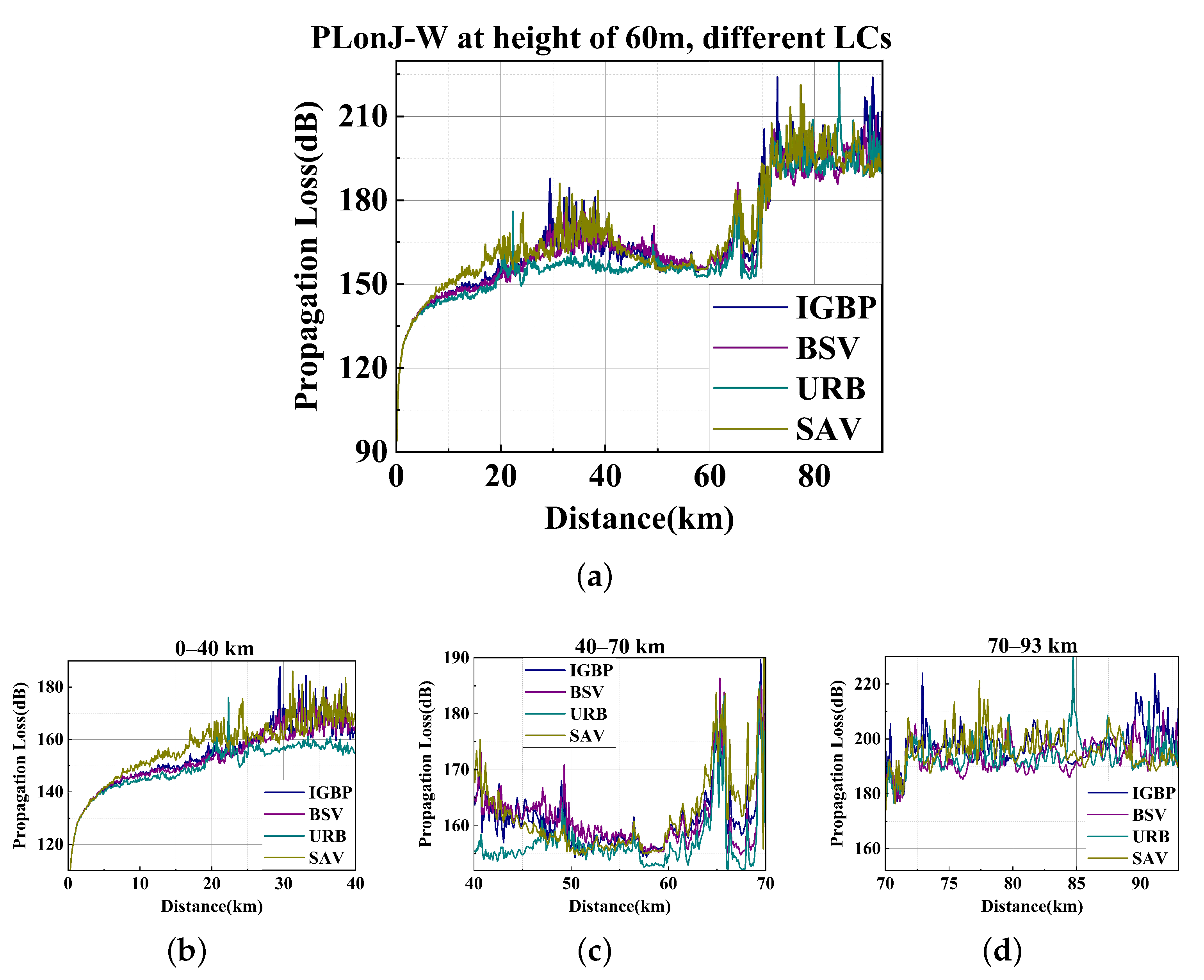
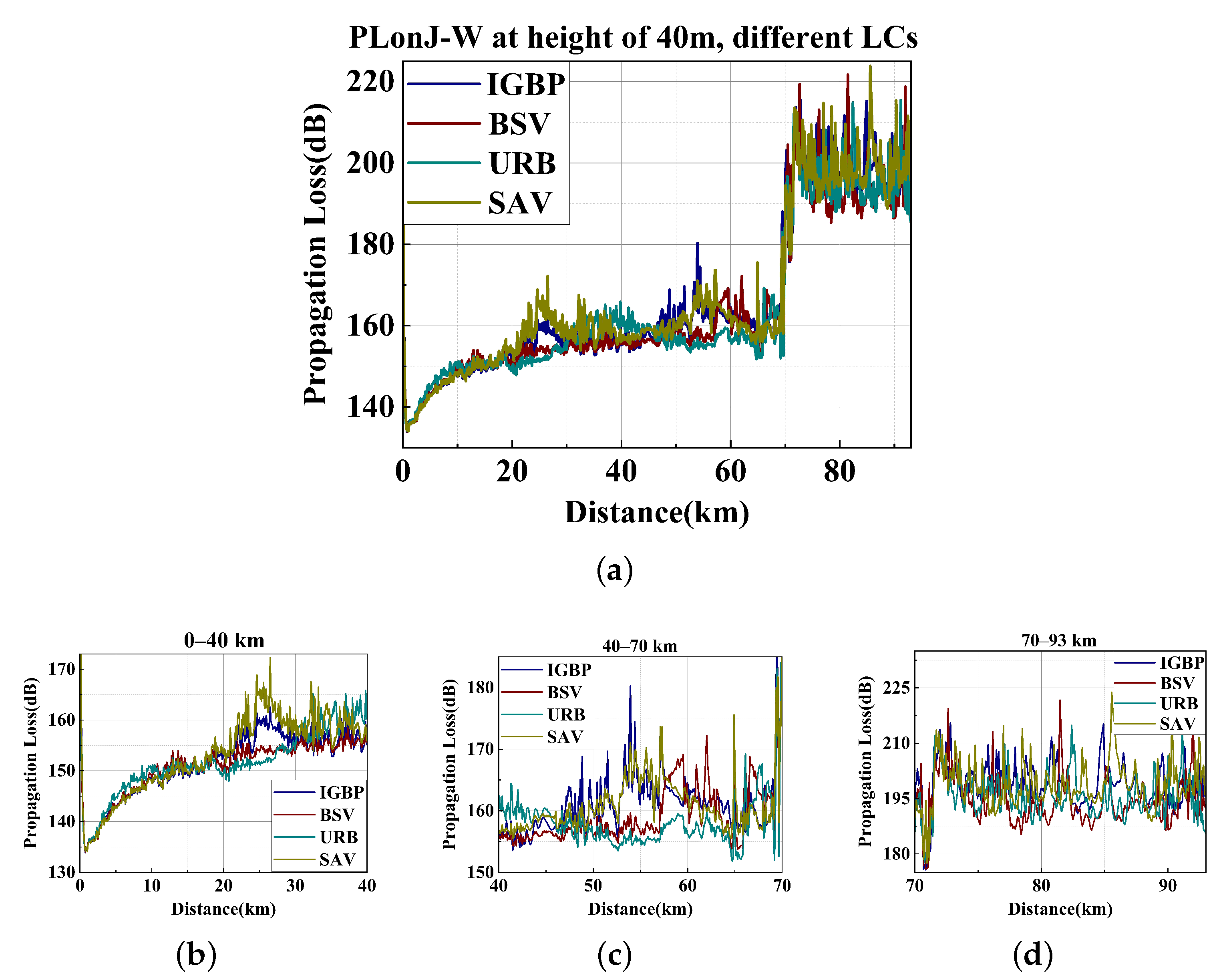
4.3. Effects of Different Land Covers
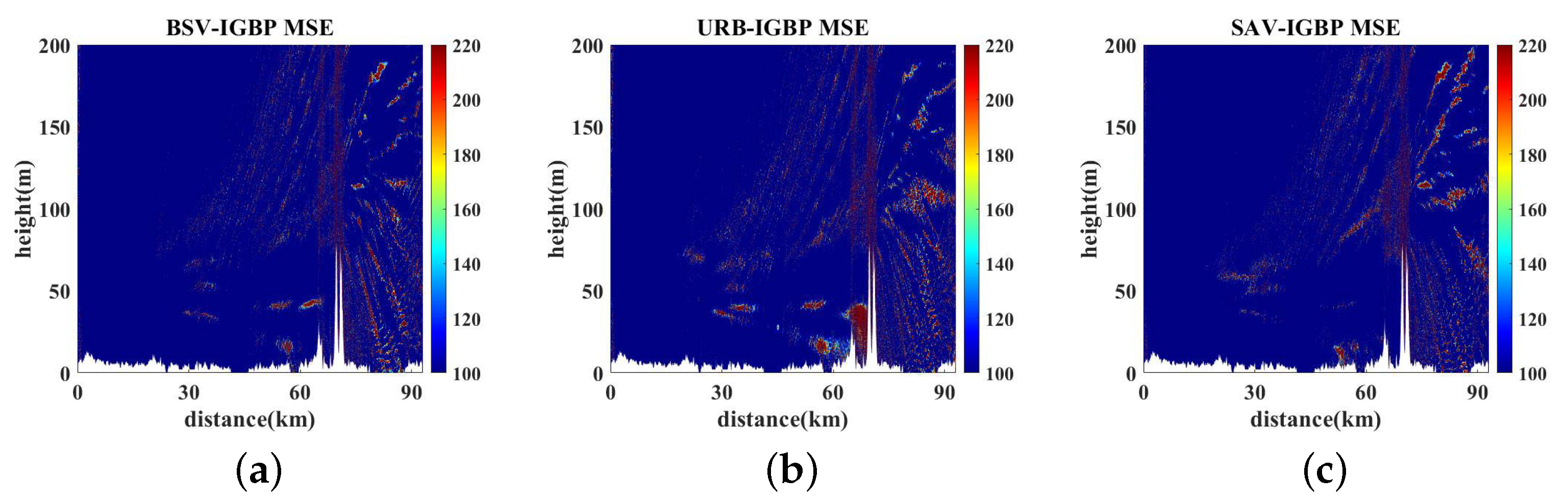
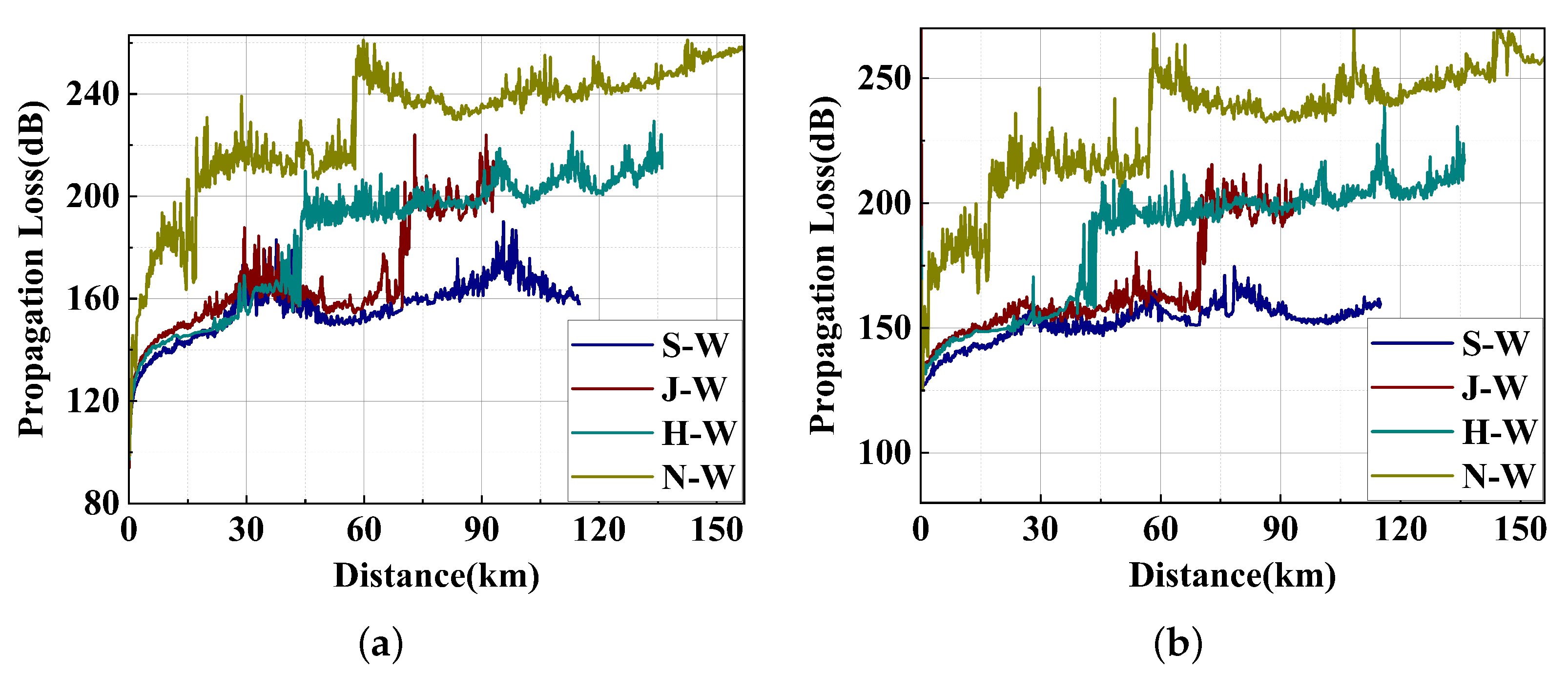
4.4. Analysis of Remote Interference between Different Intercity Links
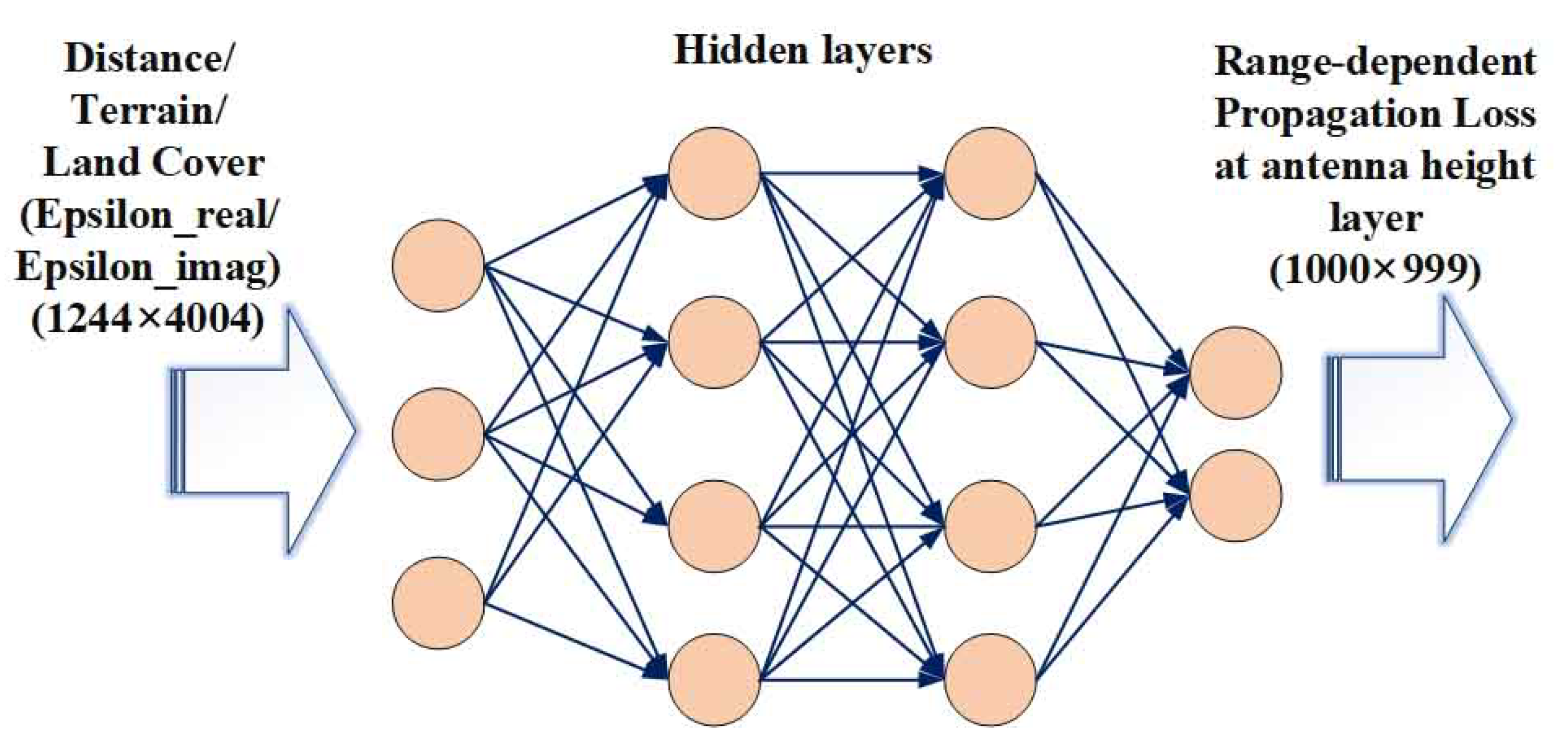
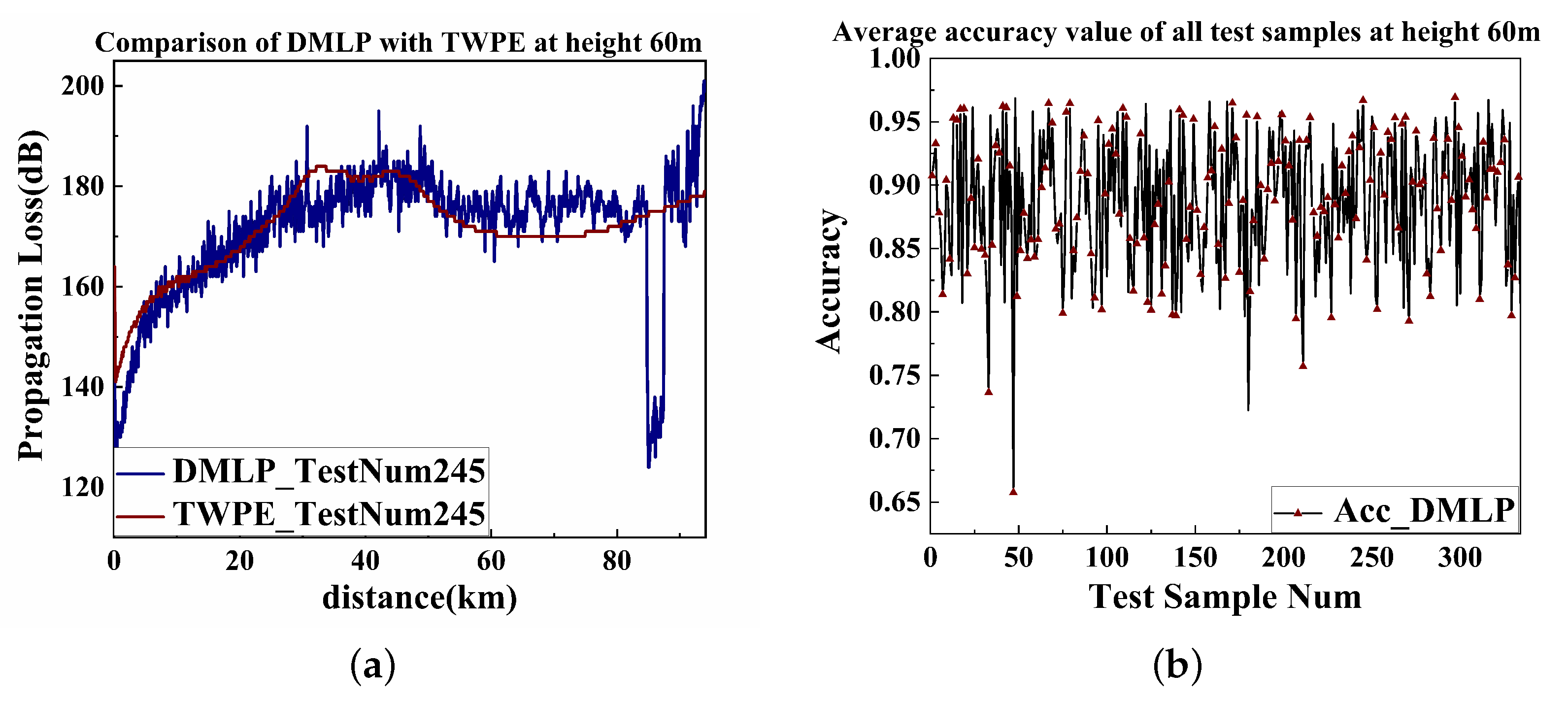
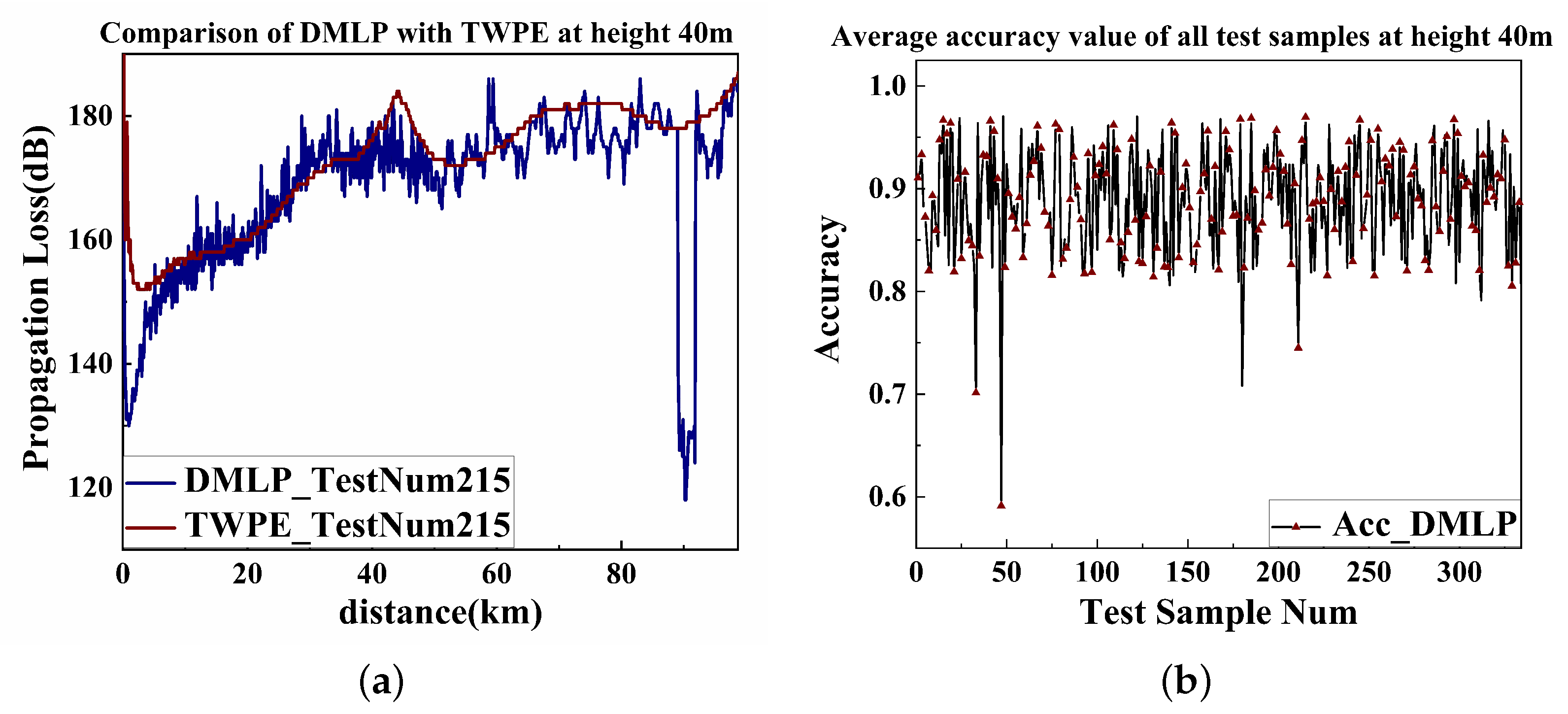
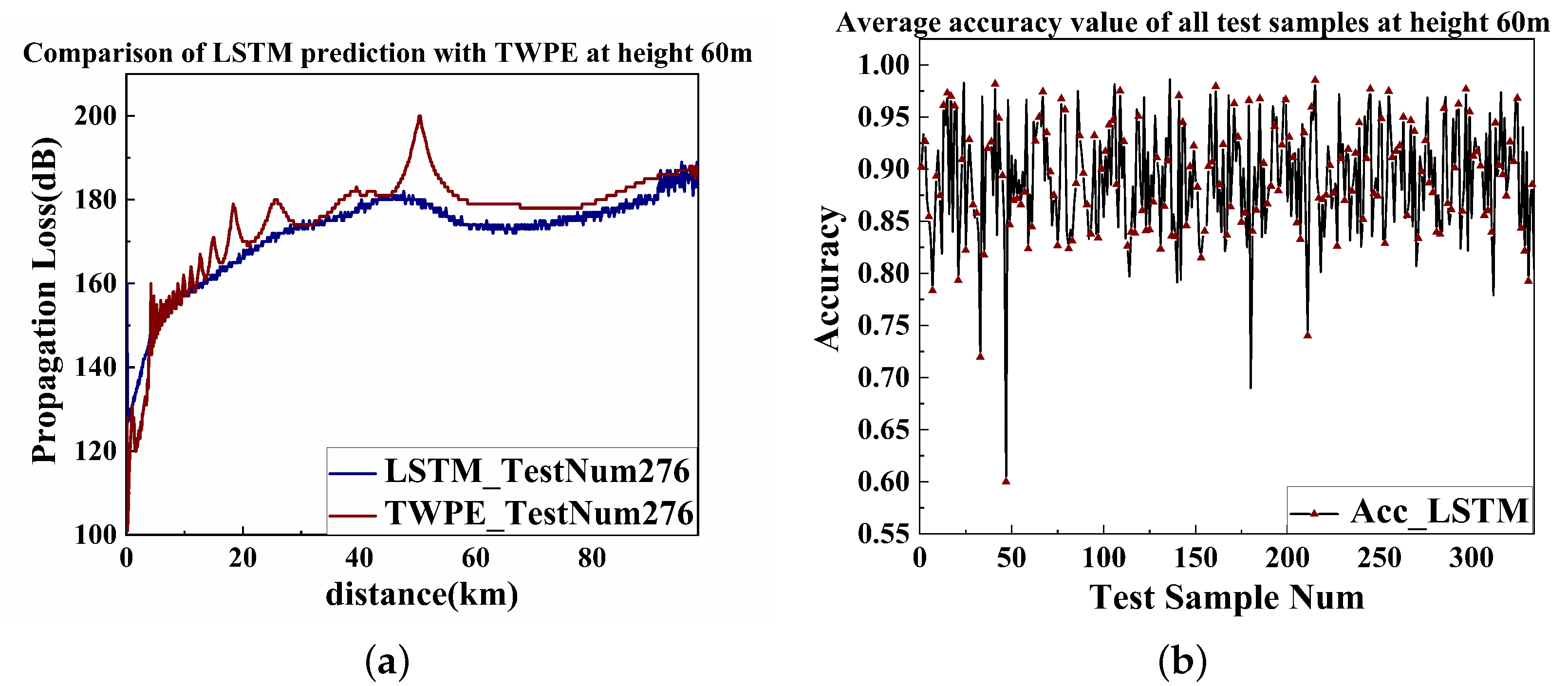
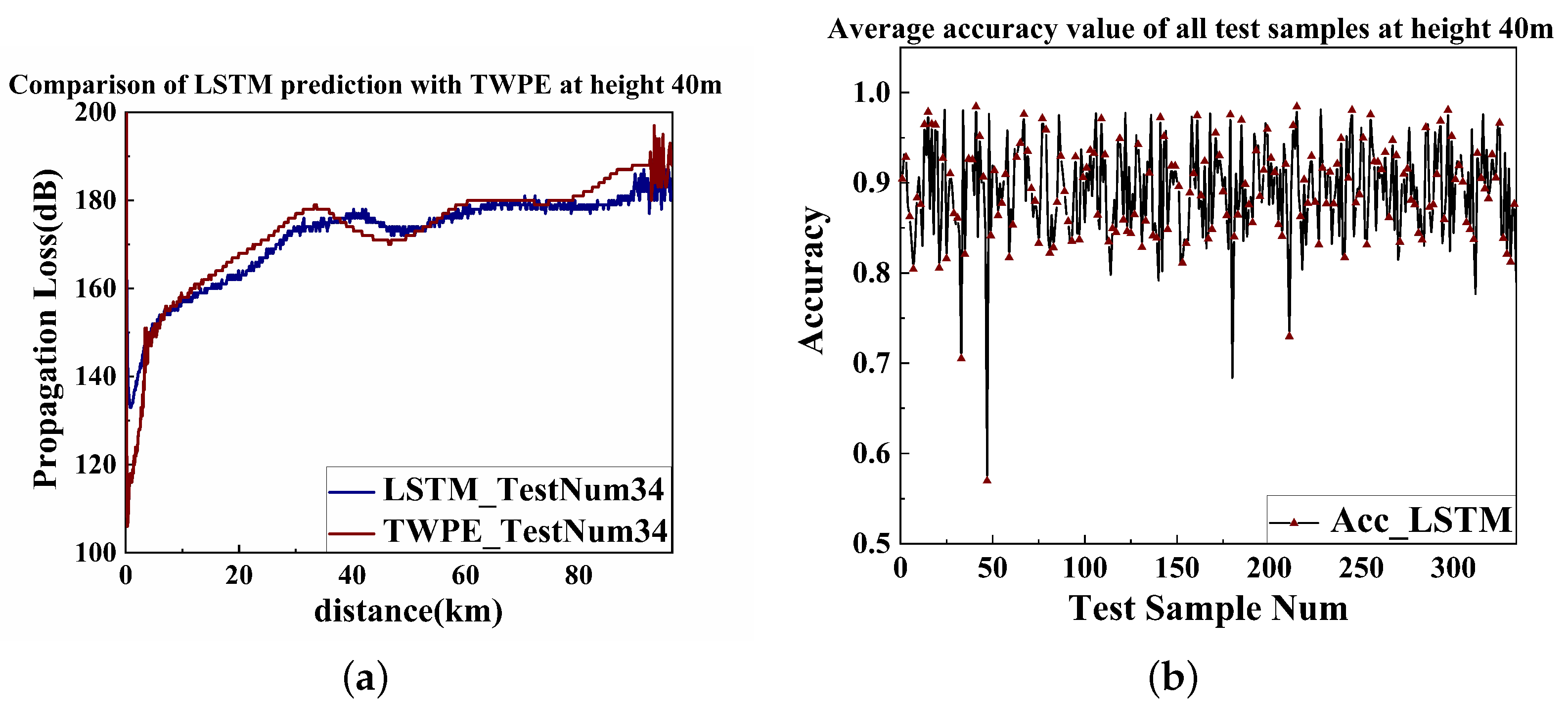
4.5. Deep Learning Model Predicts Land-Based Ducting Propagation Using Geomorphology Data
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| 5G | The fifth generation |
| PE | parabolic equation |
| DEM | digital elevation model |
| MODIS | moderate-resolution imaging spectroradiometer |
| IGBP | International Geosphere-Biosphere Program |
| WAPE | wide-angle parabolic equation |
| TWPE | terrain wide-angle parabolic equation |
| AREPS | advanced refractive effects prediction system |
| CCI | co-channel interference |
| PL | propagation loss |
| LC | land cover |
| MAP | maximum a prior |
| ML | maximum likelihood |
| DMLP | deep multilayer perceptron |
| LSTM | long and short-term memory |
References
- Huang, X.; Dong, J.; Wang, W.; Xu, H. A case study of 5G network planning based on Massive MIMO. Telecom Eng. Tech. Stand. Abbr. 2017, 30, 50–54. [Google Scholar]
- Turton, J.D.; Bennetts, D.A.; Farmer, S.F.G. An introduction to radio ducting. Meteorol. Mag. 1988, 117, 245–254. [Google Scholar]
- Leontovich, M.; Fock, V.A. Solution of the problem of propagation of electromagnetic waves along the earth’s surface by the method of parabolic equation. J. Phys. USSR 1946, 10, 13–23. [Google Scholar]
- Hardin, R.H.; Tappert, F.D. Applications of the Split-Step Fourier Method to the Numerical Solution of Nonlinear and Variable Coefficient Wave Equations. Siam Rev. 1973, 15, 423. [Google Scholar]
- Feit, M.D.; Fleck, J.A. Light propagation in graded-index optical fibers. Appl. Opt. 1978, 17, 3990–3998. [Google Scholar] [CrossRef]
- Thomson, D.J.; Chapman, N. A wide-angle split-step algorithm for the parabolic equation. J. Acoust. Soc. Am. 1983, 74, 1848–1854. [Google Scholar] [CrossRef]
- Kuttler, J.R. Differences between the narrow-angle and wide-angle propagators in the split-step Fourier solution of the parabolic wave equation. IEEE Trans. Antennas Propag. 1999, 47, 1131–1140. [Google Scholar] [CrossRef]
- Levy, M. Parabolic Equation Methods for Electromagnetic Wave Propagation, 1st ed.; The Institution of Engineering and Technology: London, UK, 2000; pp. 268–272. [Google Scholar]
- Janaswamy, R. Path loss predictions in the presence of buildings on flat terrain: A 3-D vector parabolic equation approach. IEEE Trans. Antennas Propag. 2003, 51, 1716–1728. [Google Scholar] [CrossRef]
- Hu, H.; Chen, Z.; Chai, S.; Mao, J. Research on mobile communication radio propagation characteristic based on 3DPE. In Proceedings of the 2005 Asia-Pacific Microwave Conference, Suzhou, China, 4–7 December 2005. [Google Scholar]
- Rasool, H.F.; Pan, X.M.; Sheng, X.Q. A fourier split-step based wide-angle three-dimensional vector parabolic wave equation algorithm predicting the field strength over flat and irregular forest environments. Appl. Comput. Electromagn. Soc. J. 2019, 34, 874–881. [Google Scholar]
- Dockery, G.D.; Konstanzer, G.C. Recent advances in prediction of tropospheric propagation using the parabolic equation. Johns Hopkins APL Tech. Dig. 1987, 8, 404–412. [Google Scholar]
- Dockery, D.; Kuttler, J.R. An improved impedance-boundary algorithm for Fourier split-step solutions of the parabolic wave equation. IEEE Trans. Antennas Propag. 1996, 44, 1592–1599. [Google Scholar] [CrossRef]
- Kuttler, J.R.; Dockery, G.D. Theoretical description of the parabolic approximation/Fourier split-step method of representing electromagnetic propagation in the troposphere. Radio Sci. 1991, 26, 381–393. [Google Scholar] [CrossRef]
- Donohue, D.J.; Kuttler, J.R. Propagation modeling over terrain using the parabolic wave equation. IEEE Trans. Antennas Propag. 2000, 48, 260–277. [Google Scholar] [CrossRef]
- Hitney, H.V. A practical tropospheric scatter model using the parabolic equation. IEEE Trans. Antennas Propag. 1993, 41, 905–909. [Google Scholar] [CrossRef]
- Ivanov, V.; Shalyapin, V.; Levadny, Y.V. Microwave scattering by tropospheric fluctuations in an evaporation duct. Radiophys. Quantum Electron. 2009, 52, 277–286. [Google Scholar] [CrossRef]
- Wang, H.G.; Zhang, L.J.; Guo, X.M.; Wu, Z.S. Study of Fading Characteristics of Evaporation Duct Channel for Microwave Over-the-Horizon Propagtaion. J. Microw. 2013, 29, 1–5. [Google Scholar]
- Song, S.T. Analysis on Influence of Tropospheric Atmosphere State on the Transmission Loss of Troposcatter Communication Link. Radio Commun. Technol. 2015, 41, 46–48. [Google Scholar]
- Wanger, M.; Gerstoft, P.; Rogers, T. Estimating Refractivity from Propagation Loss in Turbulent Media. Radio Sci. 2016, 51, 1876–1894. [Google Scholar]
- Liu, X.; Wu, Z.; Wang, H. Inversion method of regional range-dependent surface ducts with a base layer by Doppler weather radar echoes based on WRF model. Atmosphere 2020, 11, 754. [Google Scholar] [CrossRef]
- Liu, X.; Wu, Z.; Wang, H. Inversion for Inhomogeneous Surface Duct without a Base Layer Based on Ocean-Scattered Low-Elevation BDS Signals. Remote Sens. 2021, 13, 3914. [Google Scholar] [CrossRef]
- Reuter, H.I.; Nelson, A.; Jarvis, A. An evaluation of void-filling interpolation methods for SRTM data. Int. J. Geogr. Inf. Sci. 2007, 21, 983–1008. [Google Scholar] [CrossRef]
- Dobson, M.C.; Ulaby, F.T.; Hallikainen, M.T.; El-rayes, M.A. Microwave Dielectric Behavior of Wet Soil-Part II: Dielectric Mixing Models. IEEE Trans. Geosci. Remote Sens. 1985, GE-23, 35–46. [Google Scholar]
- Tang, Y.H.; Huang, Y. Spatial Distribution Characteristics of the Percentage of Soil Fertility Contribution and Its Associated Basic Crop Yield in Mainland China. J. Agro-Environ. Sci. 2009, 28, 1070–1078. [Google Scholar]
- Xiao, J.K. Microwave Dielectric Property of Solid Bitumen. Geochimica 1983, 1, 24–31. [Google Scholar]
- Zhang, J.R. The dielectric constant in microwave remote sensing. Remote Sens. Technol. Appl. 1994, 9, 30–43. [Google Scholar]
- Hitney, H.V. Hybrid ray optics and parabolic equation methods for radar propagation modeling. In Proceedings of the 92 International Conference on Radar, International Conference, Brighton, UK, 12–13 October 1992; pp. 58–61. [Google Scholar]
- Yang, K.; Wu, Z.; Guo, X.; Wu, J.; Cao, Y.; Qu, T.; Xue, J. Estimation of co-channel interference between cities caused by ducting and turbulence. Chin. Phys. B 2021, 31, 024102. [Google Scholar] [CrossRef]
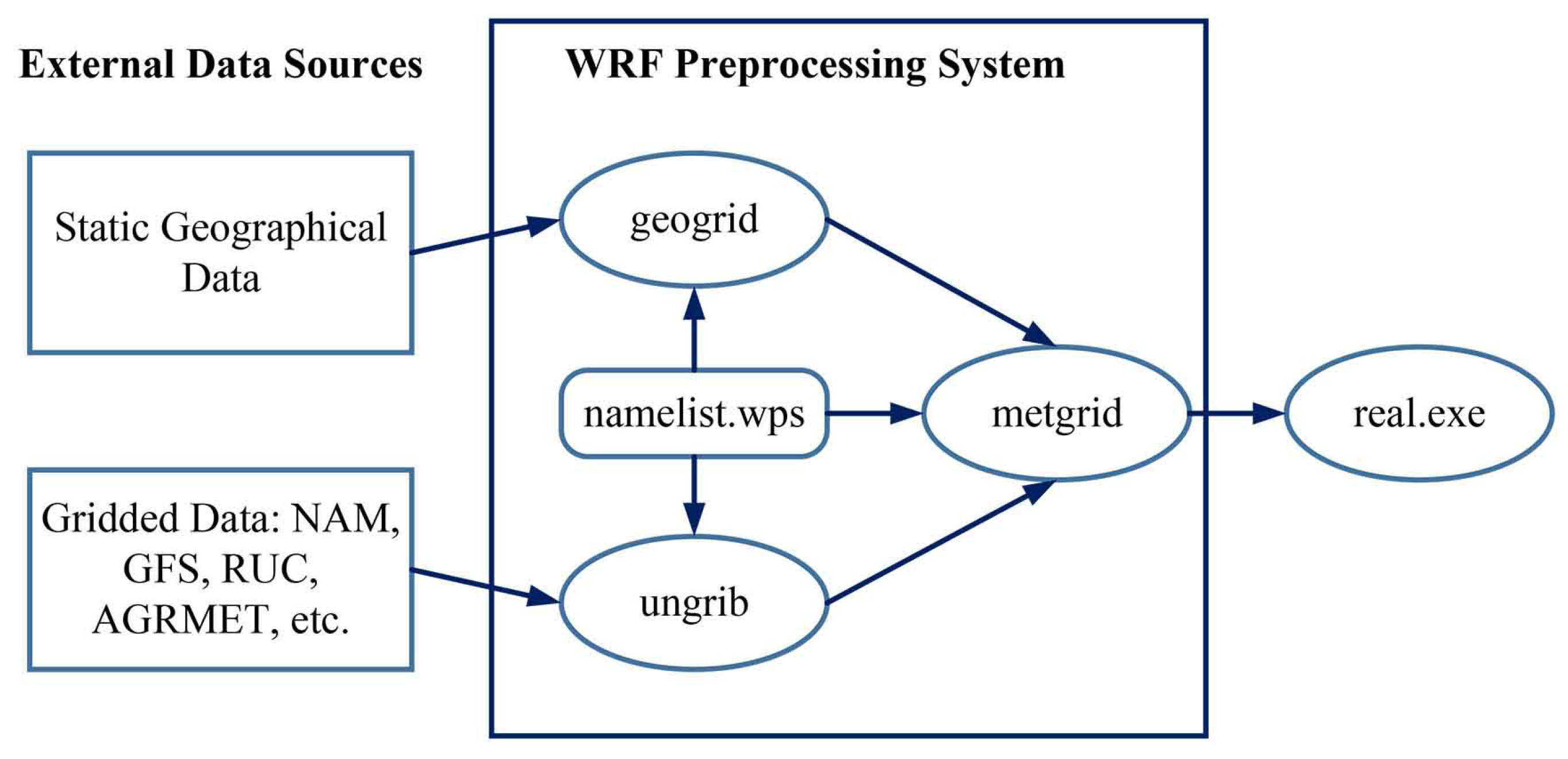
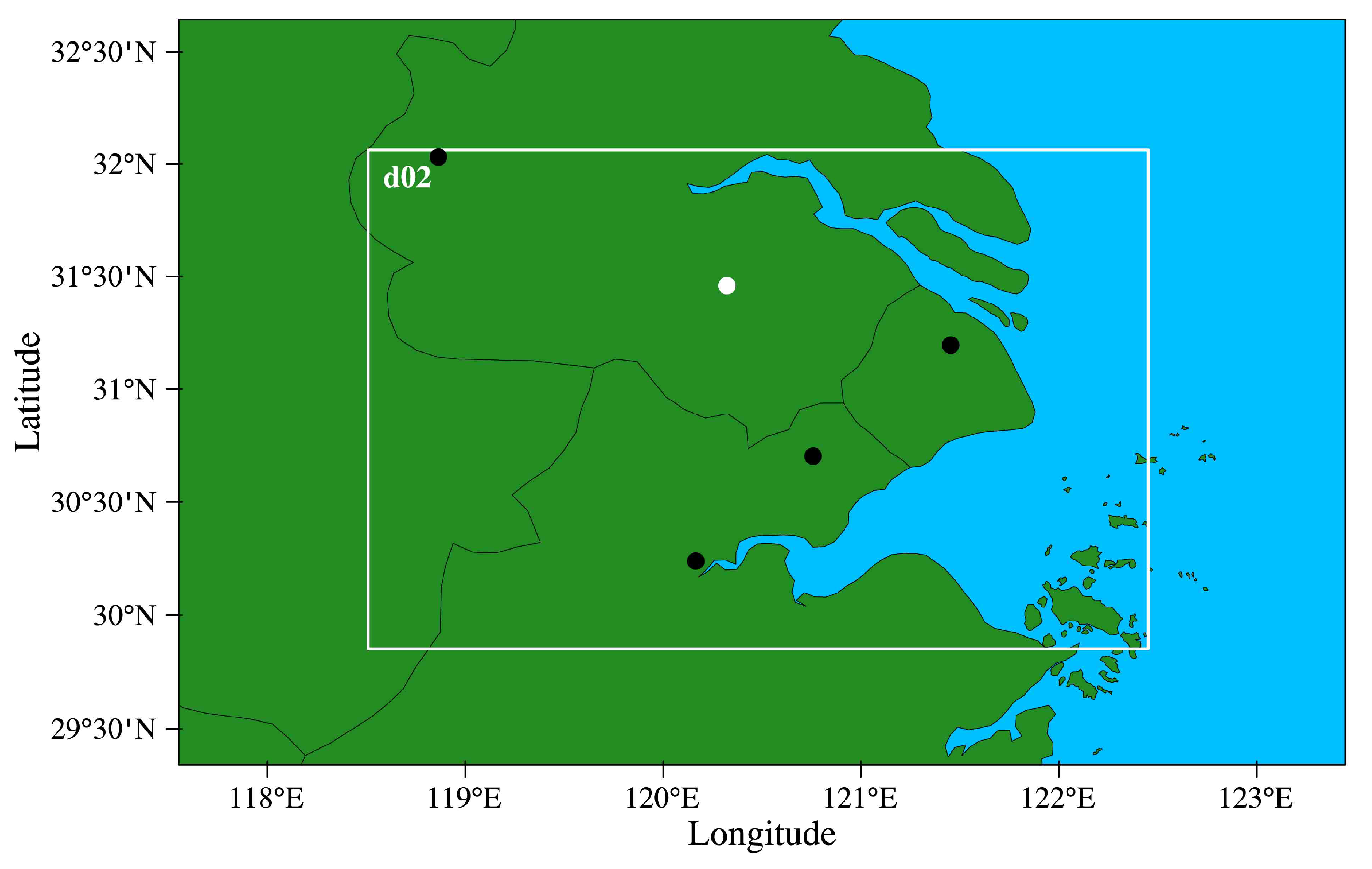




| Options | Settings | |
|---|---|---|
| Nested Levels | Double Nesting | |
| Coarse grid settings | Longitude and latitude coordinate of grid center | (120.5°E, 31.035°N) |
| Number of grids | ||
| Horizontal resolution | 3 km × 3 km | |
| Fine grid settings | Position in the coarse grid | (32, 20) |
| Number of grids | ||
| Horizontal resolution | 1 km × 1 km | |
| Number | IGBP Land Cover Type |
|---|---|
| 1 | Evergreen needleleaf forest (ENF) |
| 2 | Evergreen broadleaf forest (EBF) |
| 3 | Deciduous needleleaf forest (DNF) |
| 4 | Deciduous broadleaf forest (DBF) |
| 5 | Mixed forest (MF) |
| 6 | Closed shrublands (CSH) |
| 7 | Open shrublands (OSH) |
| 8 | Woody savannas (WSA) |
| 9 | Savannas (SAV) |
| 10 | Grasslands (GRA) |
| 11 | Permanent wetlands (WET) |
| 12 | Croplands (CRO) |
| 13 | Urban and built-up (URB) |
| 14 | Cropland/natural vegetation mosaic (CVM) |
| 15 | Snow and ice (SNO) |
| 16 | Barren or sparsely vegetated (BSV) |
| 17 | Water bodies (WAT) |
| IGBP Land Cover Type | Dielectric Constant |
|---|---|
| Savannas (SAV) | 2.85 + i0.33 |
| Grasslands (GRA) | 40 + i0.085 |
| Permanent wetlands (WET) | 26.5 + i1.2 |
| Croplands (CRO) | 4.0 + i0.93 |
| Urban and built-up (URB) | 5 + i0.070 |
| Snow and ice (SNO) | 15.8 − i1.8 |
| Barren or sparsely vegetated (BSV) | 18.2 + i4.5 |
| Water Bodies (WAT) | 81 + i0.22 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, K.; Guo, X.; Wu, Z.; Wu, J.; Wu, T.; Zhao, K.; Qu, T.; Linghu, L. Using Multi-Source Real Landform Data to Predict and Analyze Intercity Remote Interference of 5G Communication with Ducting and Troposcatter Effects. Remote Sens. 2022, 14, 4515. https://doi.org/10.3390/rs14184515
Yang K, Guo X, Wu Z, Wu J, Wu T, Zhao K, Qu T, Linghu L. Using Multi-Source Real Landform Data to Predict and Analyze Intercity Remote Interference of 5G Communication with Ducting and Troposcatter Effects. Remote Sensing. 2022; 14(18):4515. https://doi.org/10.3390/rs14184515
Chicago/Turabian StyleYang, Kai, Xing Guo, Zhensen Wu, Jiaji Wu, Tao Wu, Kun Zhao, Tan Qu, and Longxiang Linghu. 2022. "Using Multi-Source Real Landform Data to Predict and Analyze Intercity Remote Interference of 5G Communication with Ducting and Troposcatter Effects" Remote Sensing 14, no. 18: 4515. https://doi.org/10.3390/rs14184515
APA StyleYang, K., Guo, X., Wu, Z., Wu, J., Wu, T., Zhao, K., Qu, T., & Linghu, L. (2022). Using Multi-Source Real Landform Data to Predict and Analyze Intercity Remote Interference of 5G Communication with Ducting and Troposcatter Effects. Remote Sensing, 14(18), 4515. https://doi.org/10.3390/rs14184515







