The Dynamic Sea Clutter Simulation of Shore-Based Radar Based on Stokes Waves
Abstract
:1. Introduction
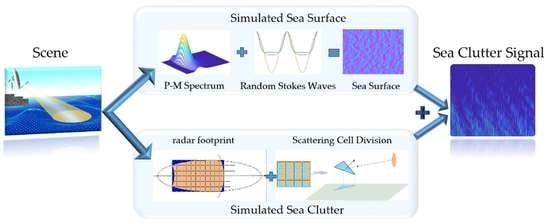
2. Sea Clutter Signal Model
2.1. Simulated Sea Surface Composed of Stokes Wave
2.1.1. Stokes Wave
2.1.2. Sea Surface Based on Spectrum
2.1.3. Calculation of Nonlinear Parameter
2.2. Simulated Sea Clutter
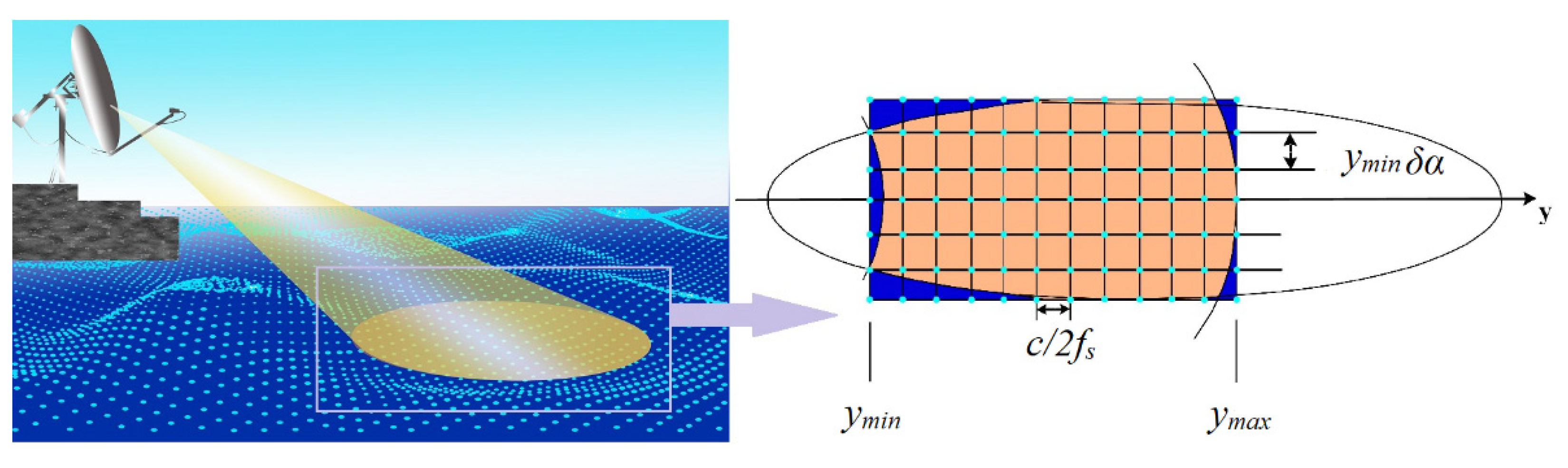
2.2.1. Radar Signal Transmission
2.2.2. Shadow Modulation Model
2.2.3. Scattering Cell Division
2.2.4. The Backscattering Coefficient Model
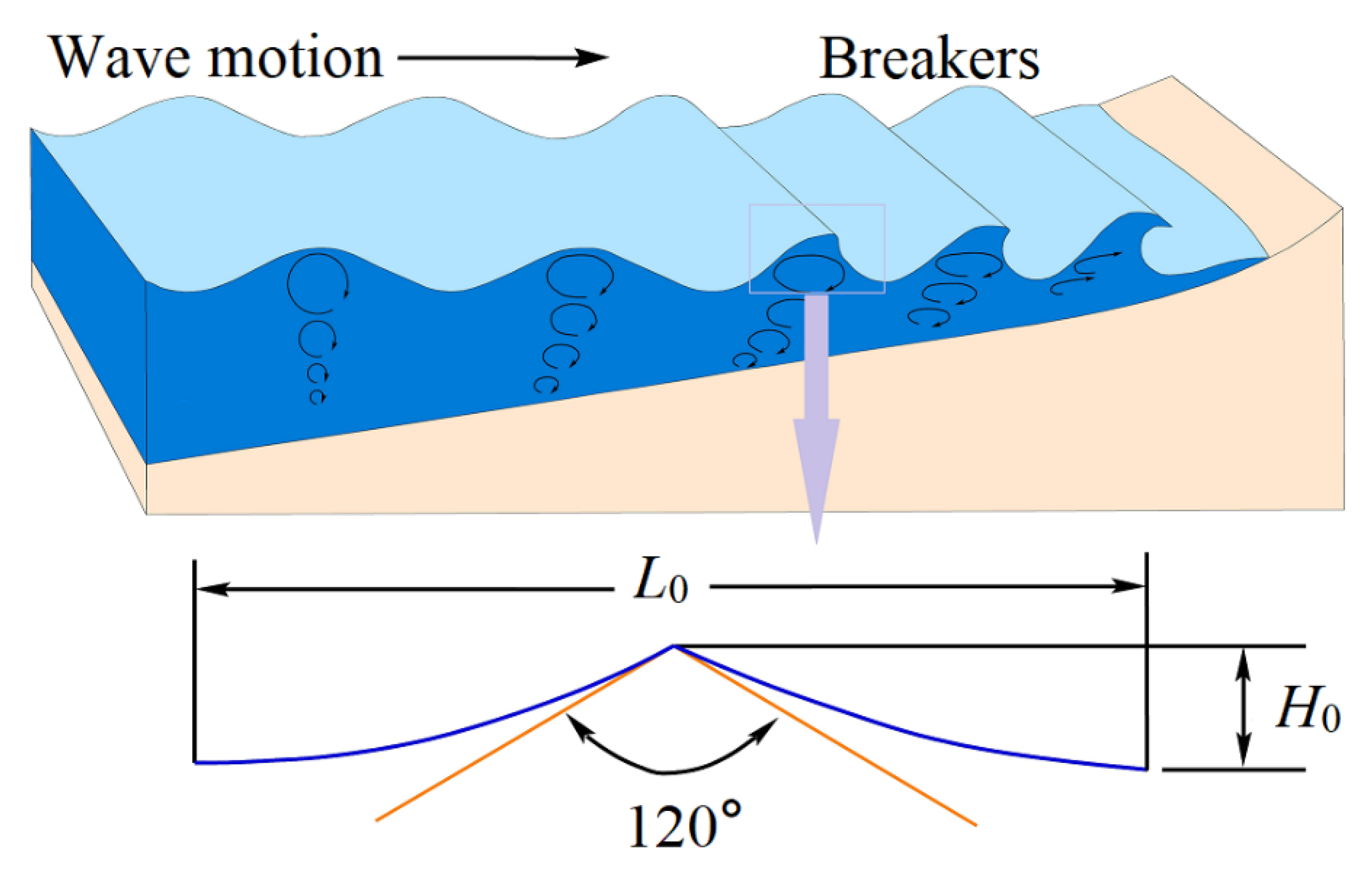
2.2.5. Breaking Wave Model
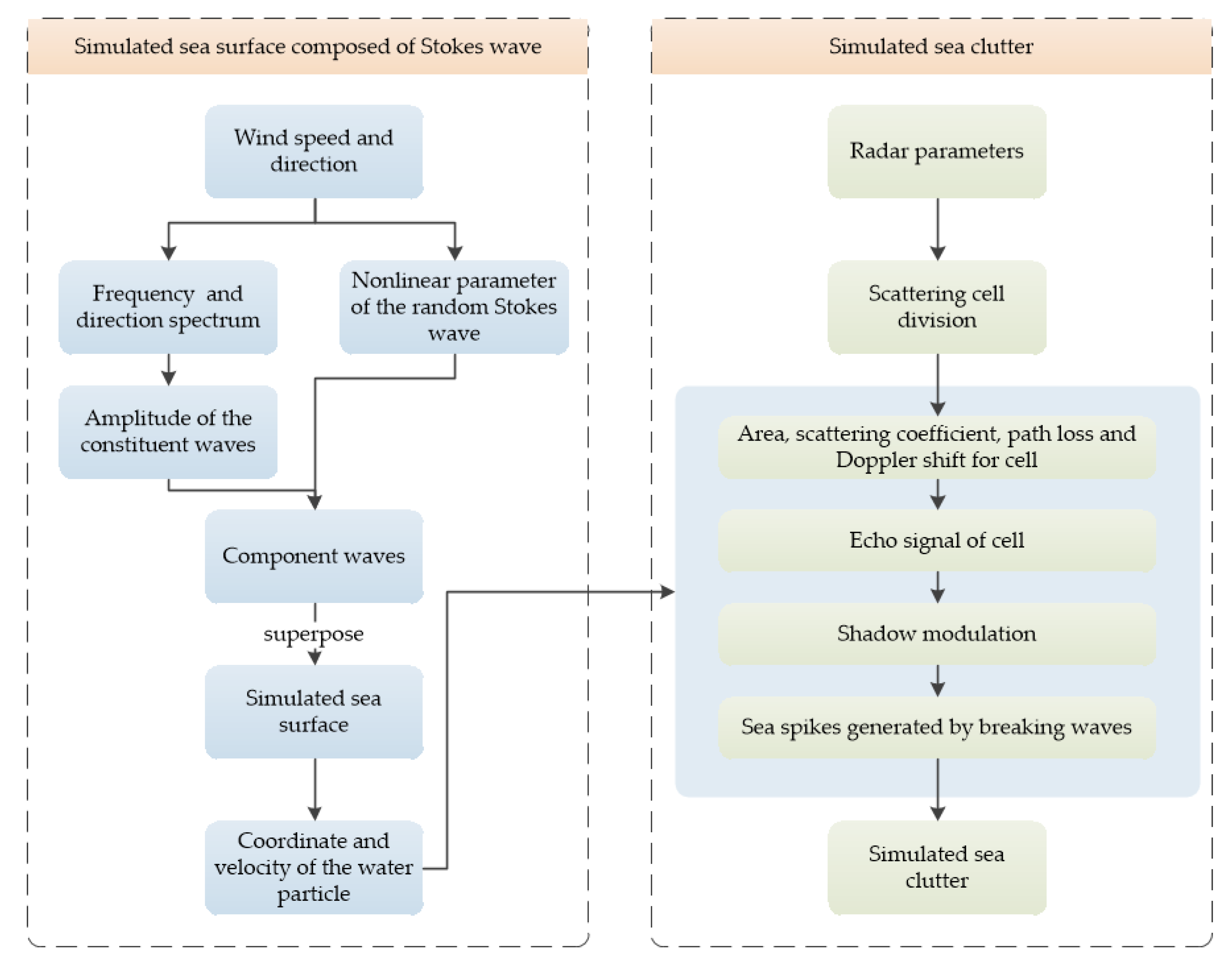
2.3. Workflow of Sea Clutter Signal Model
3. Results
3.1. Simulations Results
3.2. Comparison with Simulations and Actual Measurements
3.2.1. Comparisons of Clutter Intensity
3.2.2. Comparisons of Amplitude Statistics
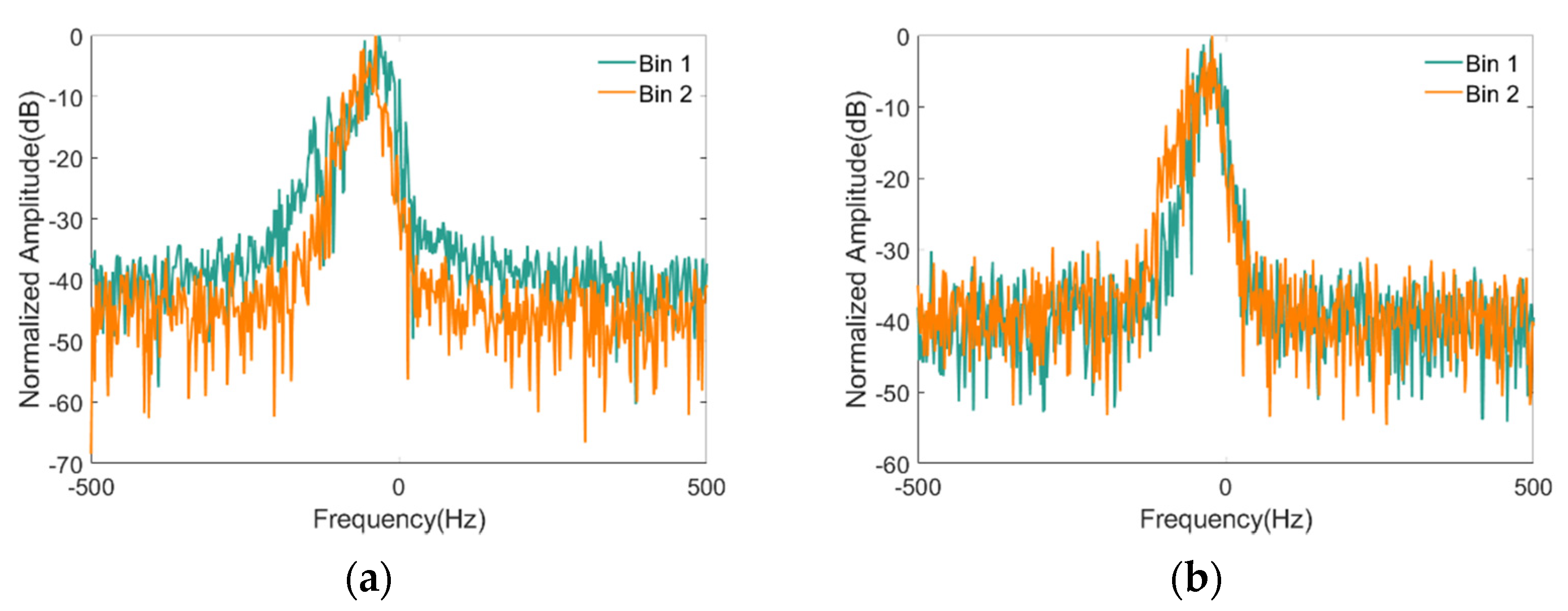
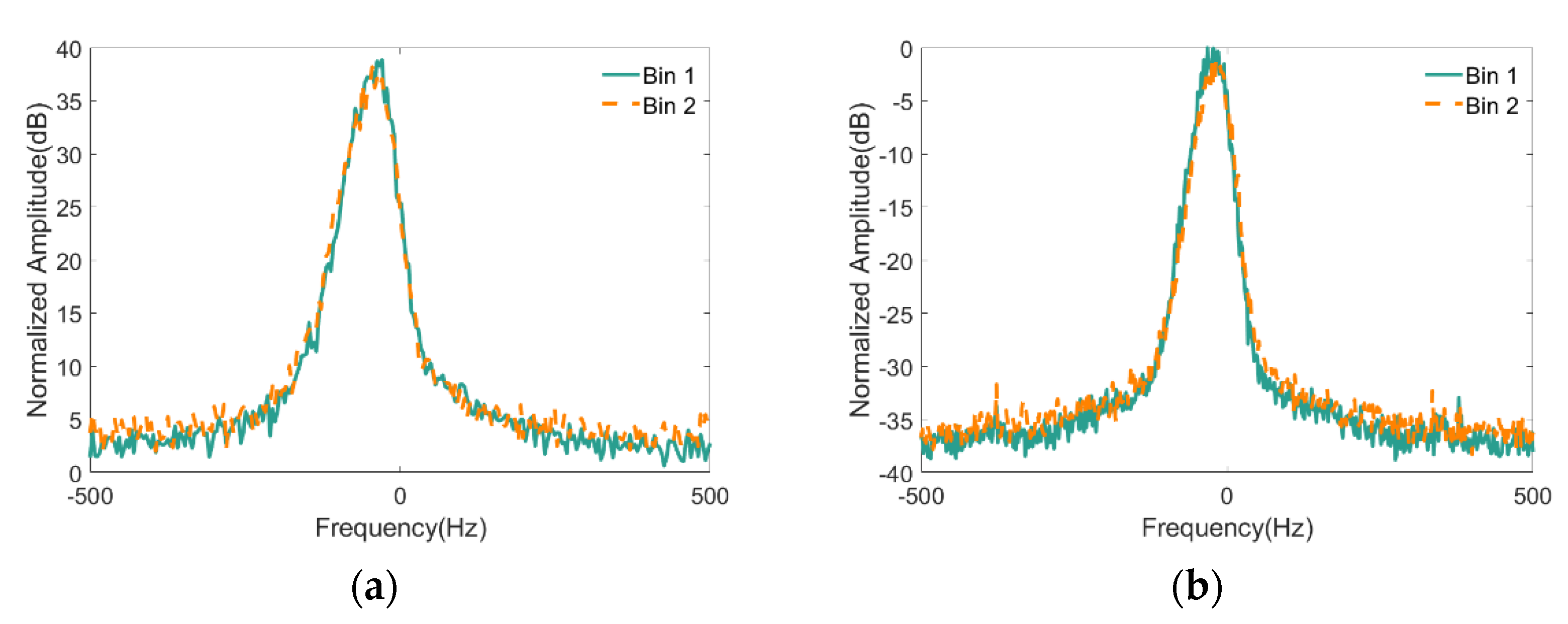
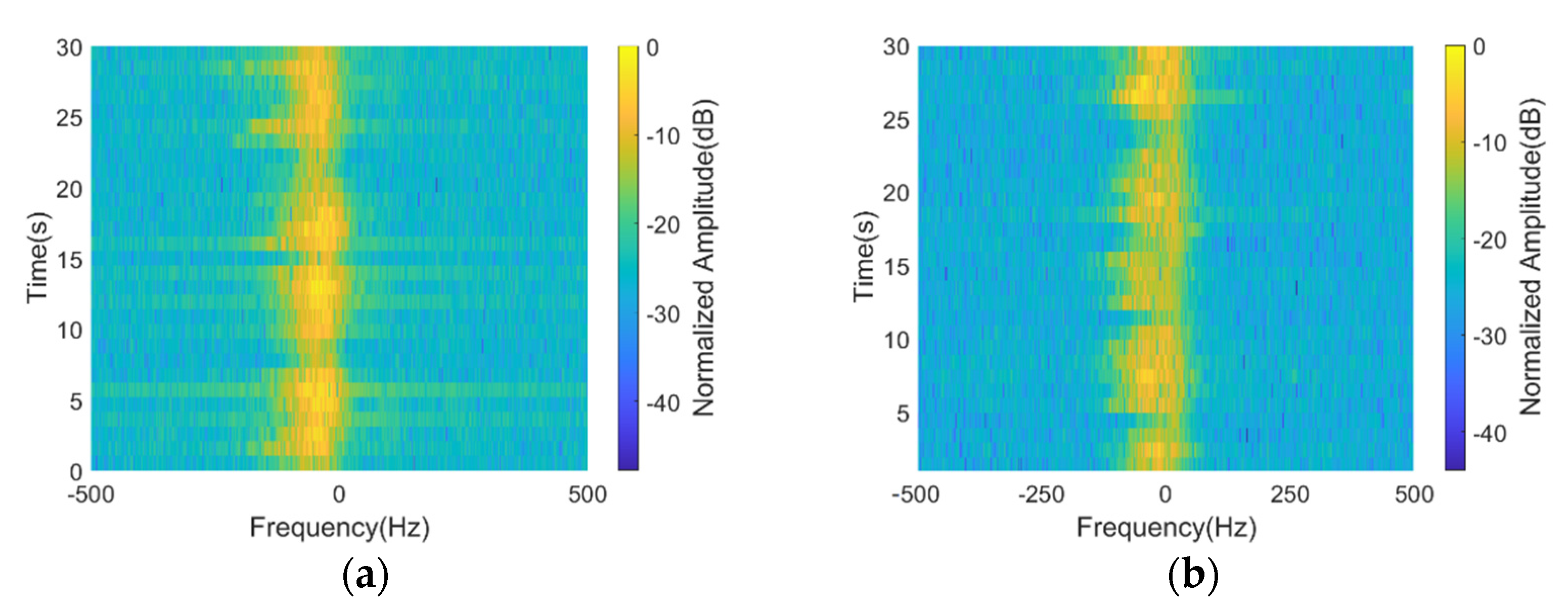
3.2.3. Comparisons of Doppler Spectrum Features
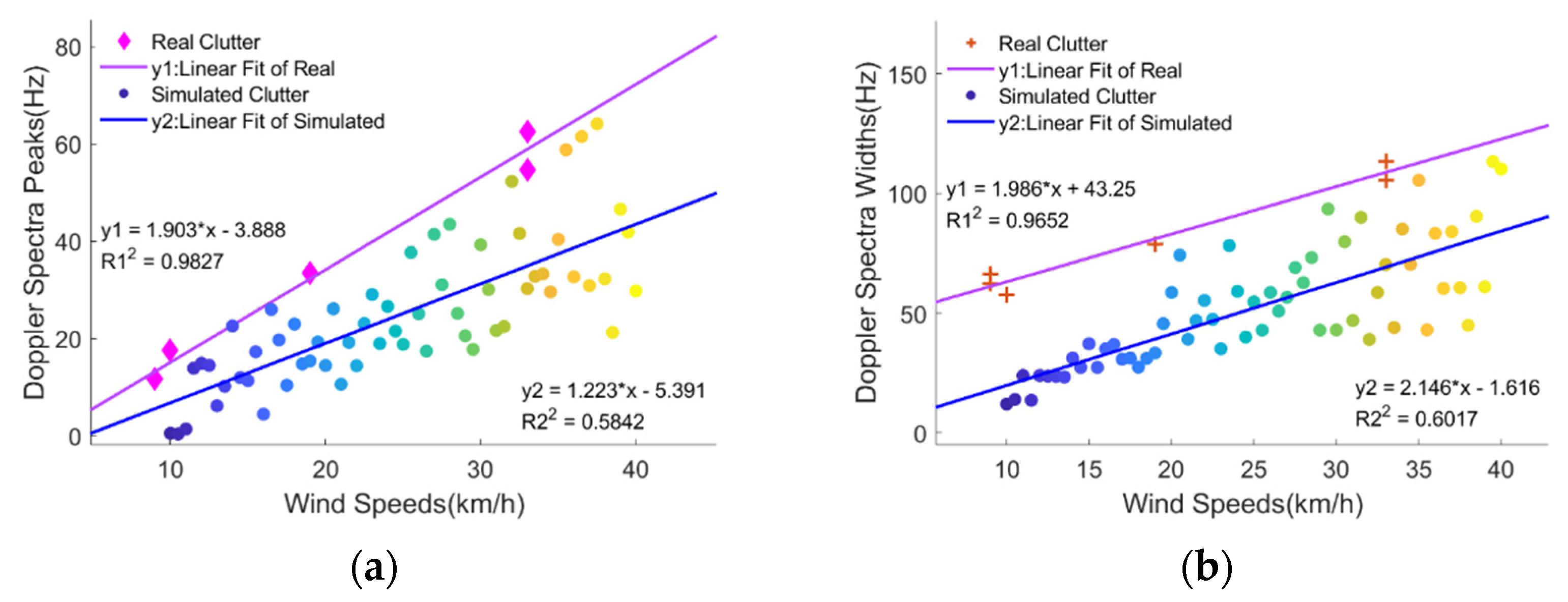
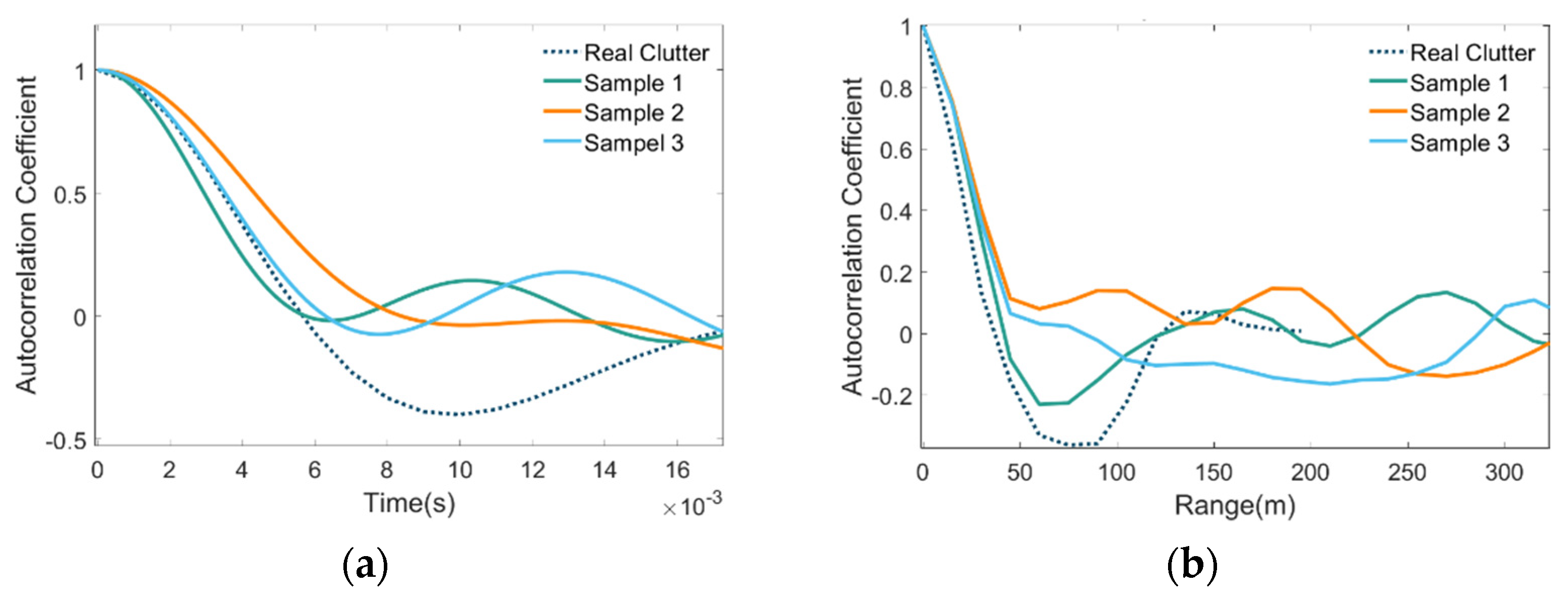
3.2.4. Comparisons of Related Features
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wright, J. A new model for sea clutter. IEEE Trans. Antennas Propag. 1968, 10, 217–223. [Google Scholar] [CrossRef]
- Plant, W.J.; Keller, W.C. Evidence of Bragg scattering in microwave Doppler spectra of sea return. J. Geophys. Res. 1990, 95, 16299–16310. [Google Scholar] [CrossRef]
- Elfouhaily, T.; Chapron, B.; Katsaros, K.; Vandemark, D. A unified directional spectrum for long and short wind-driven waves. J. Geophys. Res. 1997, 102, 15781–15796. [Google Scholar] [CrossRef]
- Karimian, A.; Yardim, C.; Gerstoft, P.; Hodgkiss, W.S.; Barrios, A.E. Multiple Grazing Angle Sea Clutter Modeling. IEEE T. Antenn. Propag. 2012, 60, 4408–4417. [Google Scholar] [CrossRef]
- Horst, M.M.; Dyer, F.B.; Tuley, M.T. Radar sea clutter model. In Proceedings of the IEEE International Conference of Antennas and Propagation, London, UK, 28–30 November 1978. [Google Scholar]
- Ward, K.D.; Watts, S. Use of sea clutter models in radar design and development. IET Radar Sonar Nav. 2010, 4, 146–157. [Google Scholar] [CrossRef]
- Alpers, W.R.; Ross, D.B.; Rufenach, C.L. On the detectability of ocean surface waves by real and synthetic aperture radar. J. Geophys. Res. 1981, 86, 6481–6498. [Google Scholar] [CrossRef]
- Romeiser, R.; Alpers, W.; Wismann, V. An improved composite surface model for the radar backscattering cross section of the ocean surface: 1. Theory of the model and optimization/validation by scatterometer data. J. Geophys. Res. 1997, 102, 25237–25250. [Google Scholar] [CrossRef]
- Jakeman, E.; Pusey, P. A model for non-Rayleigh sea echo. IEEE T. Antenn. Propag. 1976, 24, 806–814. [Google Scholar] [CrossRef]
- Ward, K.D.; Watts, S.; Tough, R.J. Sea Clutter: Scattering, the K Distribution and Radar Performance, 2nd ed.; IET: London, UK, 2013. [Google Scholar]
- Rosenberg, L.; Crisp, D.J.; Stacy, N.J. Analysis of the KK-distribution with medium grazing angle sea-clutter. IET Radar Sonar Nav. 2010, 4, 209–222. [Google Scholar] [CrossRef]
- Farshchian, M.; Posner, F.L. The Pareto distribution for low grazing angle and high resolution X-band sea clutter. In Proceedings of the IEEE Radar Conference, Arlington, VA, USA, 10–14 May 2010. [Google Scholar]
- Al-Ashwal, W.A.; Woodbridge, K.; Griffiths, H.D. Analysis of bistatic sea clutter—Part II: Amplitude statistics. IEEE T. Aero. Elec. Sys. 2014, 50, 1293–1303. [Google Scholar] [CrossRef]
- Ward, K.D. Compound representation of high resolution sea clutter. Electron. Lett. 1981, 17, 561–563. [Google Scholar] [CrossRef]
- Rangaswamy, M.; Weiner, D.; Ozturk, A. Computer generation of correlated non-Gaussian radar clutter. IEEE T. Aero. Elec. Sys. 1995, 31, 106–116. [Google Scholar] [CrossRef]
- Marier, L.J. Correlated K-distributed clutter generation for radar detection and track. IEEE T. Aero. Elec. Sys. 1995, 31, 568–580. [Google Scholar] [CrossRef]
- Walker, D. Experimentally Motivated Model for Low Grazing Angle Radar Doppler Spectra of the Sea Surface. IET Radar Sonar Nav. 2000, 147, 114–120. [Google Scholar] [CrossRef]
- Davidson, G. Simulation of coherent sea clutter. IET Radar Sonar Nav. 2010, 4, 168–177. [Google Scholar] [CrossRef]
- Watts, S. Modeling and Simulation of Coherent Sea Clutter. IEEE T. Aero. Elec. Sys. 2012, 48, 3303–3317. [Google Scholar] [CrossRef]
- Watts, S.; Rosenberg, L.; Bocquet, S.; Ritchie, M. Doppler spectra of medium grazing angle sea clutter; Part 1: Characterisation. IET Radar Sonar Nav. 2016, 10, 24–31. [Google Scholar] [CrossRef]
- McDonald, M.; Cerutti-Maori, D. Multi-phase centre coherent radar sea clutter modelling and simulation. IET Radar Sonar Nav. 2017, 11, 1359–1366. [Google Scholar] [CrossRef]
- Bocquet, S. Compound G2 model of coherent radar sea clutter. IET Radar Sonar Nav. 2019, 13, 1508–1518. [Google Scholar] [CrossRef]
- Watts, S.; Rosenberg, L. Challenges in radar sea clutter modelling. IET Radar Sonar Nav. 2022, 16, 1403–1414. [Google Scholar] [CrossRef]
- Xin, Z.; Liao, G.; Yang, Z.; Zhang, Y.; Dang, H. A Deterministic Sea-Clutter Space–Time Model Based on Physical Sea Surface. IEEE T. Geosci. Remote. 2016, 54, 6659–6673. [Google Scholar] [CrossRef]
- Huang, P.; Zou, Z.; Xia, X.; Liu, X.; Liao, G.; Xin, Z. Multichannel Sea Clutter Modeling for Spaceborne Early Warning Radar and Clutter Suppression Performance Analysis. IEEE T. Geosci. Remote Sens. 2021, 59, 8349–8366. [Google Scholar] [CrossRef]
- Bai, W.; Eatock Taylor, R. Fully nonlinear simulation of wave interaction with fixed and floating flared structures. Ocean Eng. 2009, 36, 223–236. [Google Scholar] [CrossRef]
- Nouguier, F.; Guérin, C.; Chapron, B. “Choppy wave” model for nonlinear gravity waves. J. Geophys. Res. 2009, 114, 1–16. [Google Scholar] [CrossRef]
- Zakharov, V.E.; Dyachenko, A.I.; Prokofiev, A.O. Freak waves as nonlinear stage of Stokes wave modulation instability. Eur. J. Mech. B Fluids 2006, 25, 677–692. [Google Scholar] [CrossRef]
- Nieto-Borge, J.C.; Rodriguez, G.; Hessner, K.; Gonzalez, P.I. Inversion of marine radar images forsurface wave analysis. J. Atmos. Ocean. Techn. 2004, 21, 1291–1300. [Google Scholar] [CrossRef]
- Lee, P.H.Y.; Barter, J.D.; Beach, K.L.; Caponi, E.; Hindman, L.; Lake, B.M.; Rungaldier, H.; Shelton, J.C. Power spectral lineshapes of microwave radiation backscattered from sea surfaces at small grazing angles. IET Radar Sonar Nav. 1995, 142, 252–258. [Google Scholar] [CrossRef]
- Hou, Y.; Guo, P.; Song, G.; Song, J.; Yin, B.; Zhao, X. Statistical distribution of nonlinear random wave height. Sci. China Ser. D 2006, 49, 443–448. [Google Scholar] [CrossRef]
- Pierson, W.J.; Moskowitz, L. A proposed spectral form for fully developed wind seas based on the similarity theory of S. A. Kitaigorodskii. J. Geophys. Res. 1964, 69, 5181–5190. [Google Scholar] [CrossRef]
- Yuxiu, Y. Random Wave and Its Applications to Engineering, 3rd ed.; Dalian University of Technology Press: Dalian, China, 2003. [Google Scholar]
- Longuet-Higgins, M.S.; Stewart, R.W. Radiation stresses in water waves; a physical discussion, with applications. Deep. Sea Res. 1964, 11, 529–562. [Google Scholar] [CrossRef]
- Fung, A.; Lee, K. A semi-empirical sea-spectrum model for scattering coefficient estimation. IEEE J. Ocean. Eng. 1982, 7, 166–176. [Google Scholar] [CrossRef]
- Dankert, H. Ocean surface determination from X-band radar-image sequences. J. Geophys. Res. 2004, 109, C04016. [Google Scholar] [CrossRef]
- Valenzuela, G.R. Theories for the interaction of electromagnetic and oceanic waves—A review. Bound-Lay. Meteorol. 1978, 13, 61–85. [Google Scholar] [CrossRef]
- Stokes, G.G. On the Theory of Oscillatory Waves; Mathematical and press: Cambridge, UK, 1880. [Google Scholar]
- Michell, J.H. XLIV. The highest waves in water. Lond. Edinb. Dublin Philos. Mag. J. Sci. 2009, 36, 430–437. [Google Scholar] [CrossRef]
- Miche, M. Mouvements ondulatoires de la mer en profondeur constante ou decroissante. Ann. Ponts Chaussees 1944, 114, 270–292. [Google Scholar]
- Stansell, P.; Wolfram, J.; Linfoot, B. Improved joint probability distribution for ocean wave heights and periods. J. Fluid Mech. 1999, 503, 273–297. [Google Scholar] [CrossRef]
- Monahan, E.C.; Fairall, C.W.; Davidson, K.L.; Boyle, P.J. Observed inter-relations between 10 m winds, ocean whitecaps and marine aerosols. Q. J. Roy. Meteor. Soc. 1983, 109, 379–392. [Google Scholar] [CrossRef]

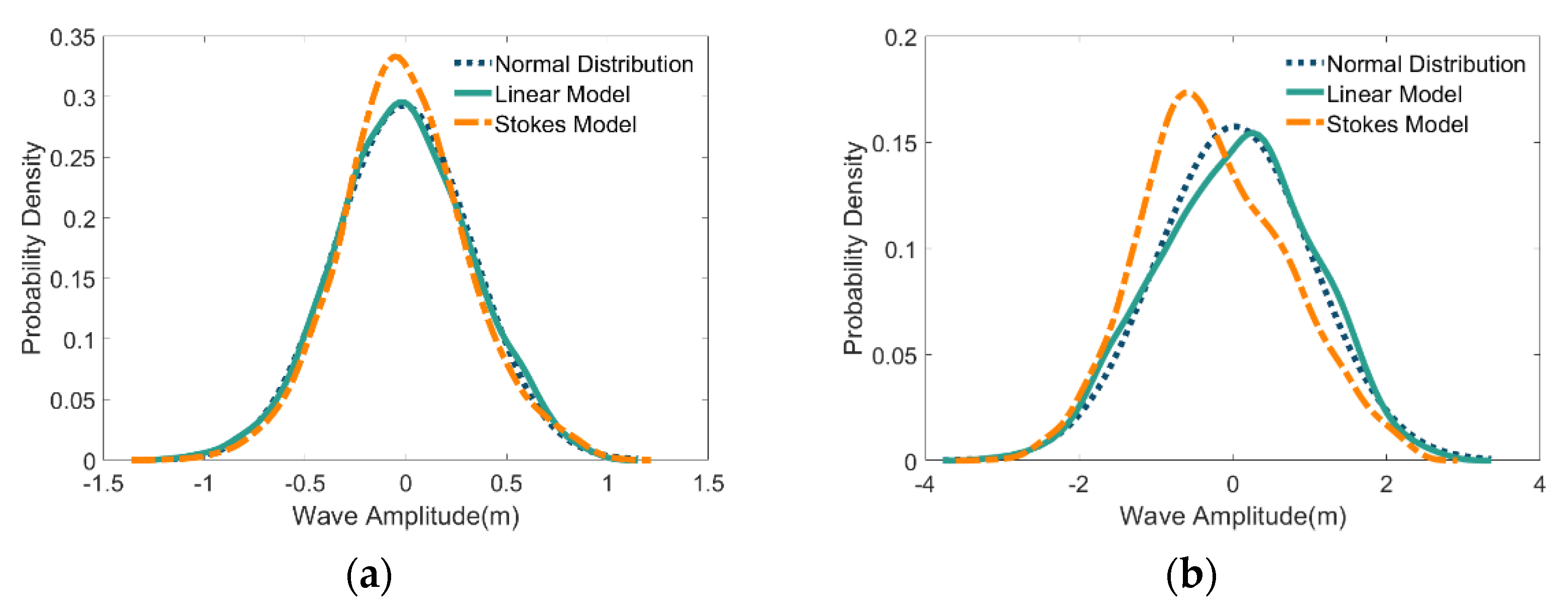
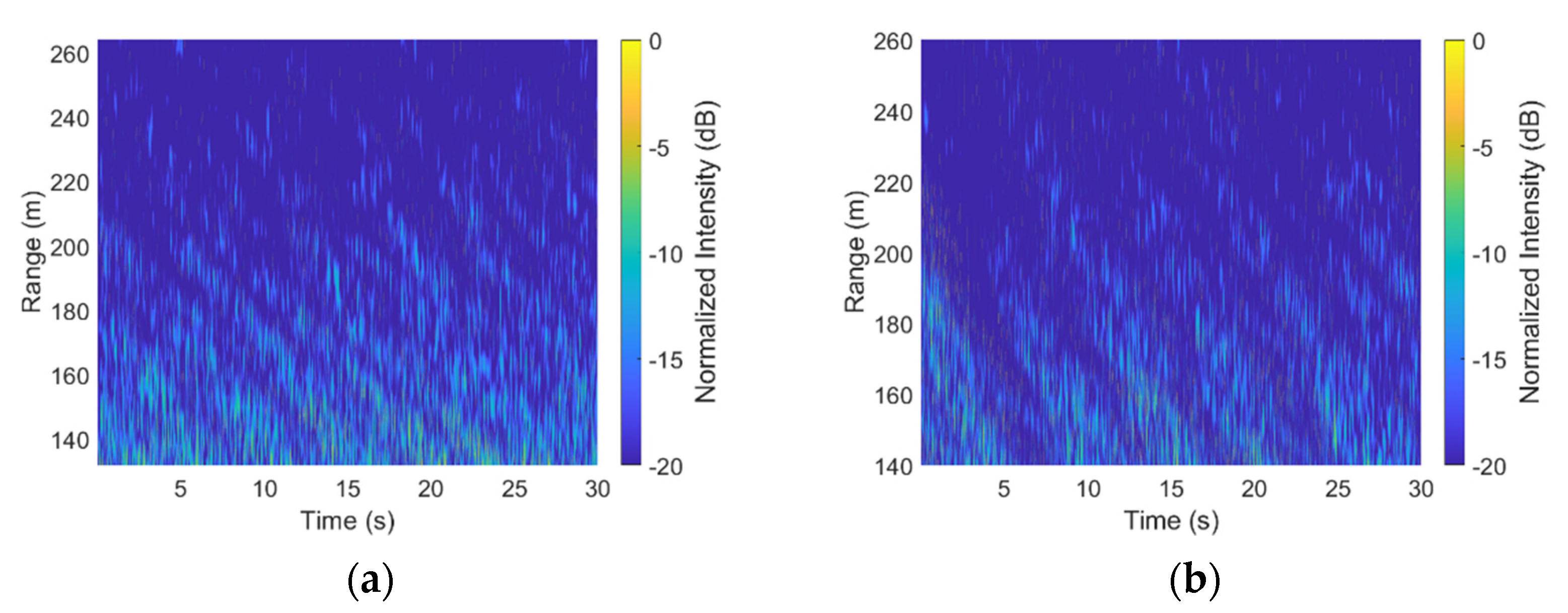


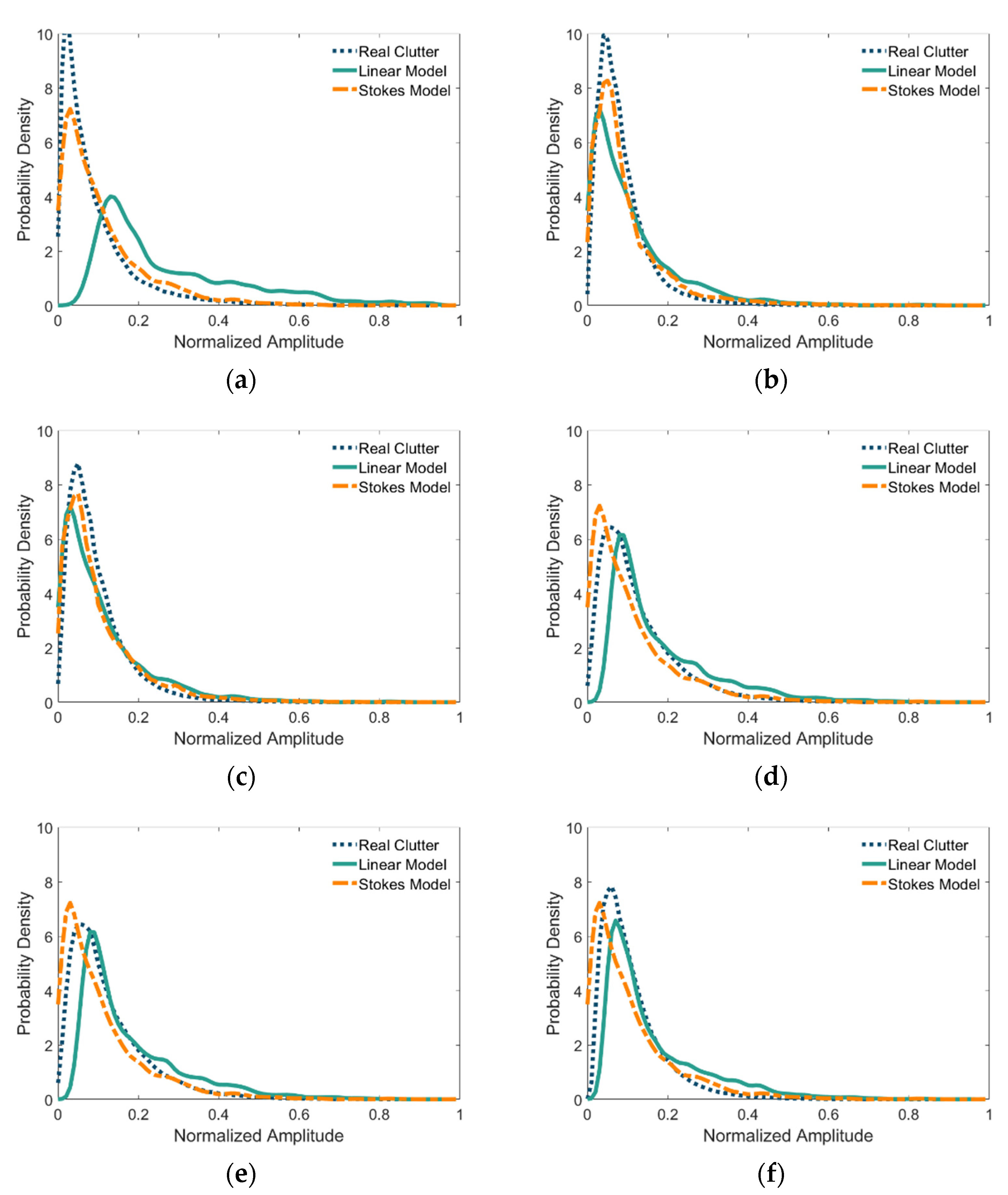
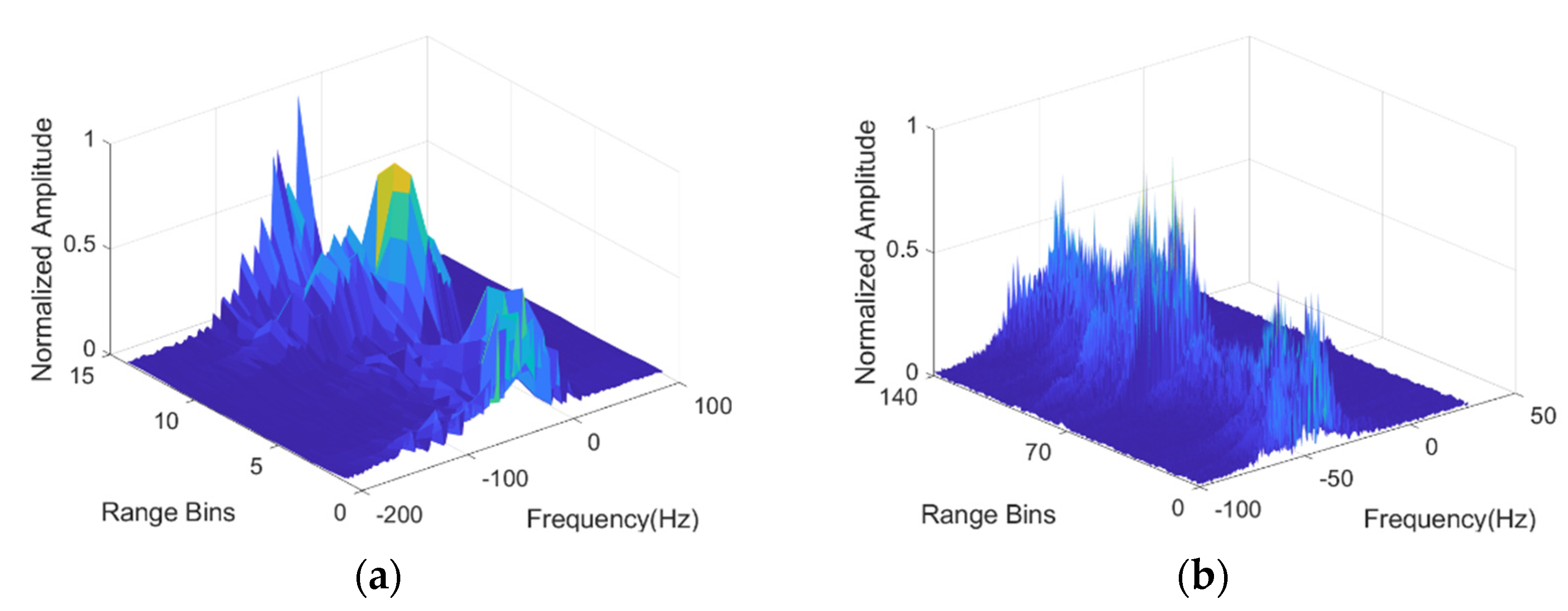


| Parameter | Value |
|---|---|
| Simulation Range | 2 km × 2 km |
| First Point Distance | 1 km |
| Distance Resolution | 15 m |
| Radar Beam Width | 0.9° |
| Radar Antenna Height | 30 m |
| Signal Carrier Frequency | 9.39 GHz |
| Pulse Repetition Frequency | 1 kHz |
| Sampling Frequency | 50 MHz |
| Transmit Bandwidth | 25 MHz |
| System Noise Figure | 1.2 dB |
| Polarization Mode | VV |
| NO. | Target Bin | U (km/h) | Wd (Degree) |
|---|---|---|---|
| #17 | 9 | 9 | 300 |
| #18 | 9 | 9 | 300 |
| #19 | 8 | 10 | 300 |
| #54 | 8 | 19 | 300 |
| #310 | 7 | 33 | 310 |
| #320 | 7 | 33 | 310 |
| Rayleigh | Lognormal | Weibull | K | |
|---|---|---|---|---|
| Chi-square | 4.33 | 3.85 | 5.42 | 2.58 |
| K-S | 9.63 | 6.39 | 11.25 | 4.02 |
| MSD (×10−4) | 1.97 | 6.03 | 4.2 | 1.79 |
| NO. | Peak (Hz) | Width (Hz) |
|---|---|---|
| #17 | 11.7 | 66.3 |
| #18 | 11.7 | 62.5 |
| #19 | 17.6 | 57.6 |
| #54 | 33.5 | 78.8 |
| #310 | 62.5 | 113.3 |
| #320 | 54.7 | 105.4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luo, F.; Feng, Y.; Liao, G.; Zhang, L. The Dynamic Sea Clutter Simulation of Shore-Based Radar Based on Stokes Waves. Remote Sens. 2022, 14, 3915. https://doi.org/10.3390/rs14163915
Luo F, Feng Y, Liao G, Zhang L. The Dynamic Sea Clutter Simulation of Shore-Based Radar Based on Stokes Waves. Remote Sensing. 2022; 14(16):3915. https://doi.org/10.3390/rs14163915
Chicago/Turabian StyleLuo, Feng, Yao Feng, Guisheng Liao, and Linrang Zhang. 2022. "The Dynamic Sea Clutter Simulation of Shore-Based Radar Based on Stokes Waves" Remote Sensing 14, no. 16: 3915. https://doi.org/10.3390/rs14163915
APA StyleLuo, F., Feng, Y., Liao, G., & Zhang, L. (2022). The Dynamic Sea Clutter Simulation of Shore-Based Radar Based on Stokes Waves. Remote Sensing, 14(16), 3915. https://doi.org/10.3390/rs14163915