Analysis of Orbital Atmospheric Density from QQ-Satellite Precision Orbits Based on GNSS Observations
Abstract
:1. Introduction
2. Materials and Methods
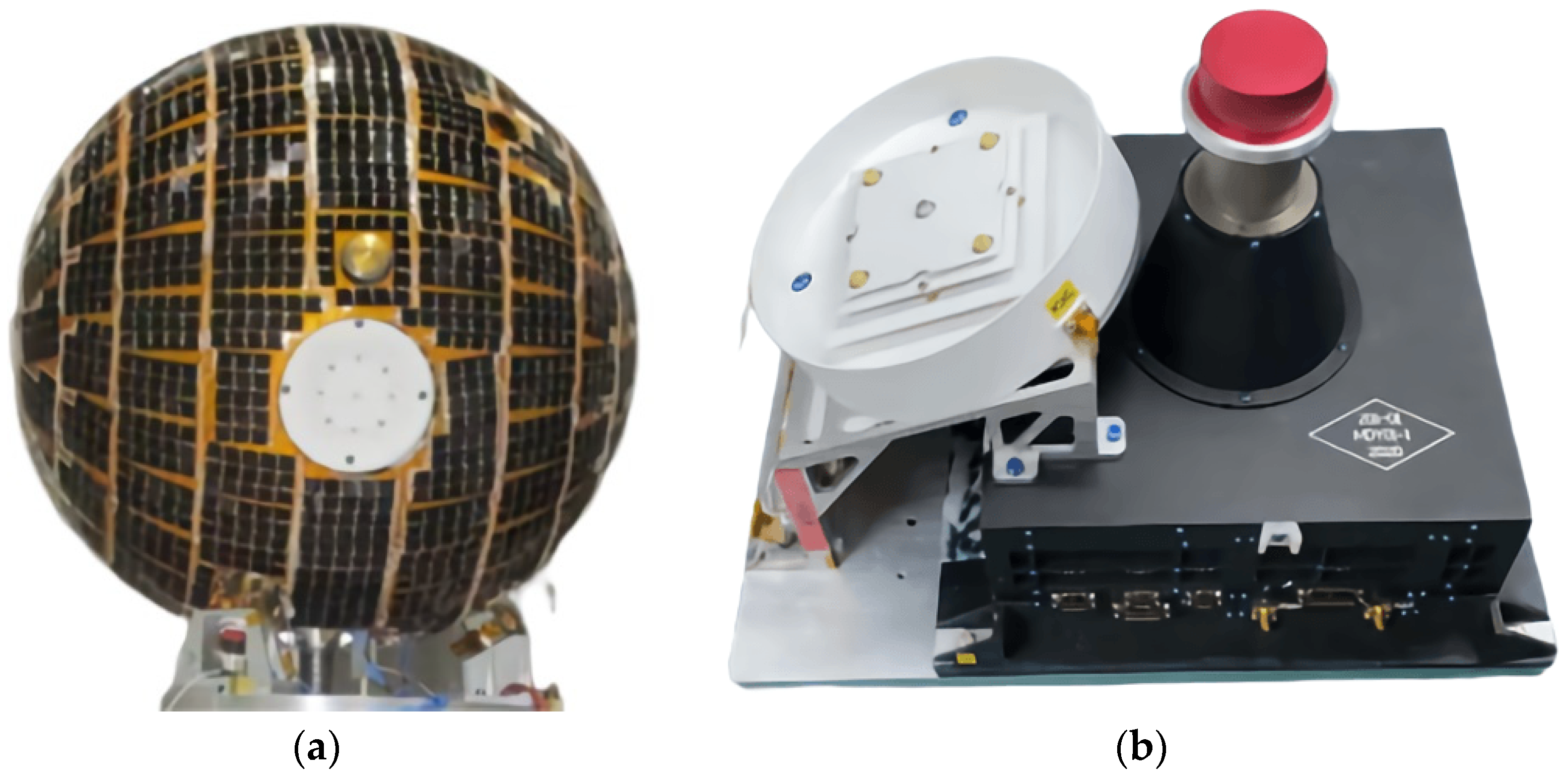
2.1. Data Source
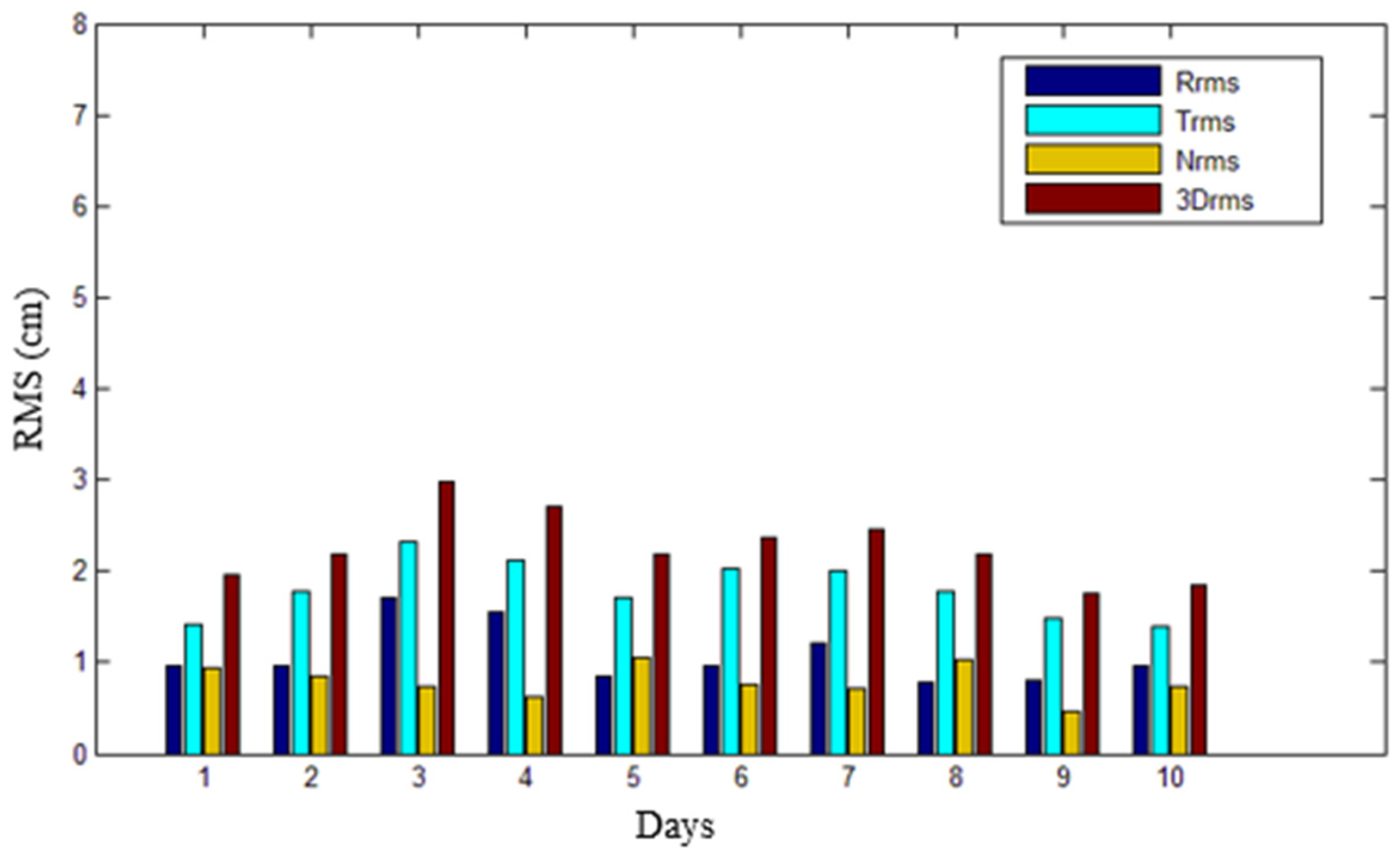
2.2. Processing Strategy
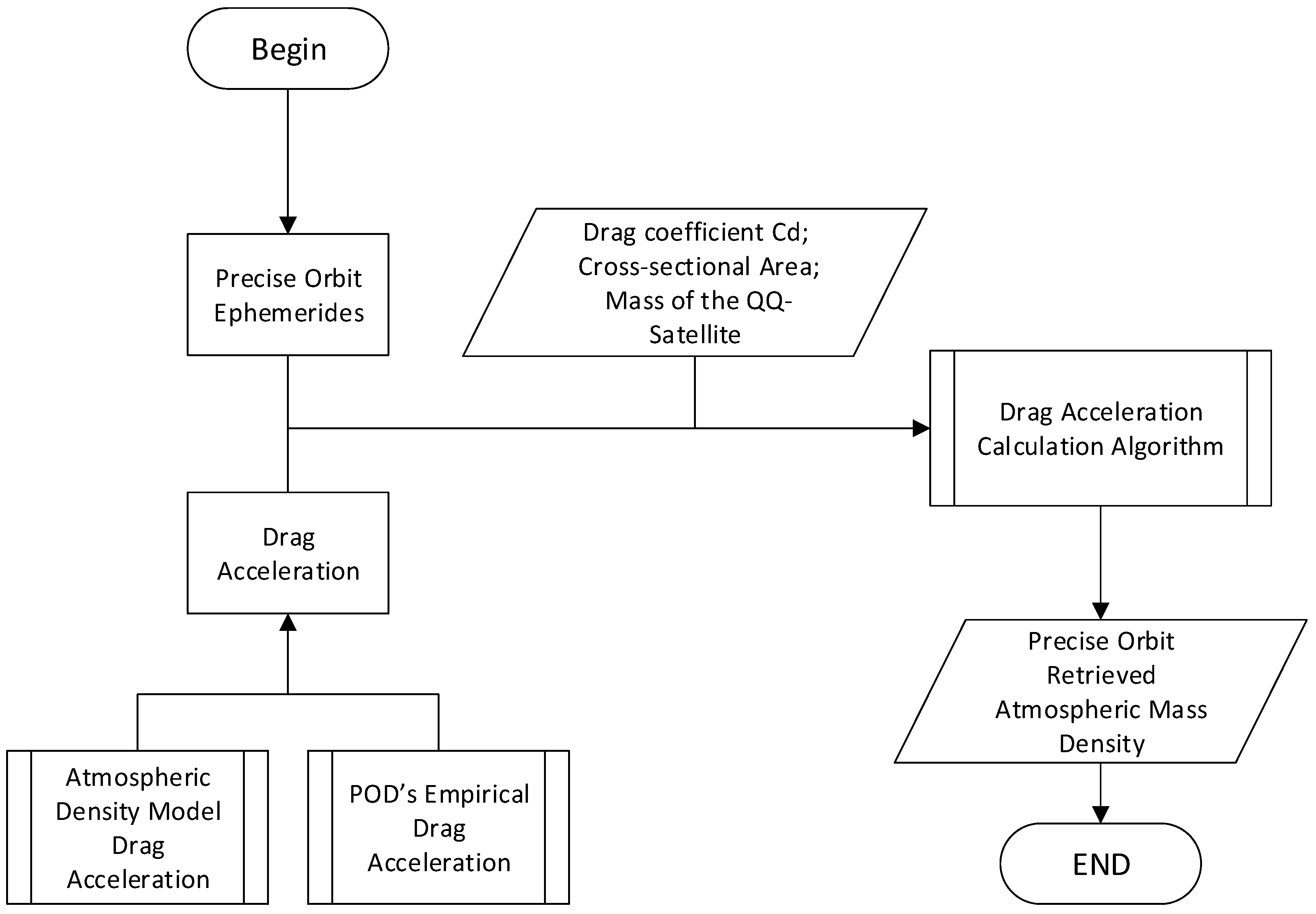
2.3. Orbit Propagation
- Geopotential using the EGM-96 [30] to the degree and order of 120 × 120;
- CSR 3.0 [31] for the ocean tidal model truncated to 30 × 30;
- Solid earth and pole tides using the IERS2010 conventions [32];
- DE 430 planetary ephemeris [33] for third-body perturbations by the sun and moon;
- Radiation pressures, mainly including the solar and Earth radiation pressures; and
- Atmospheric drag, considering a corotated atmosphere for calculating the horizontal wind velocity. The spherical QQ-Satellite cross-sectional area is 2.011 m2.
3. Results
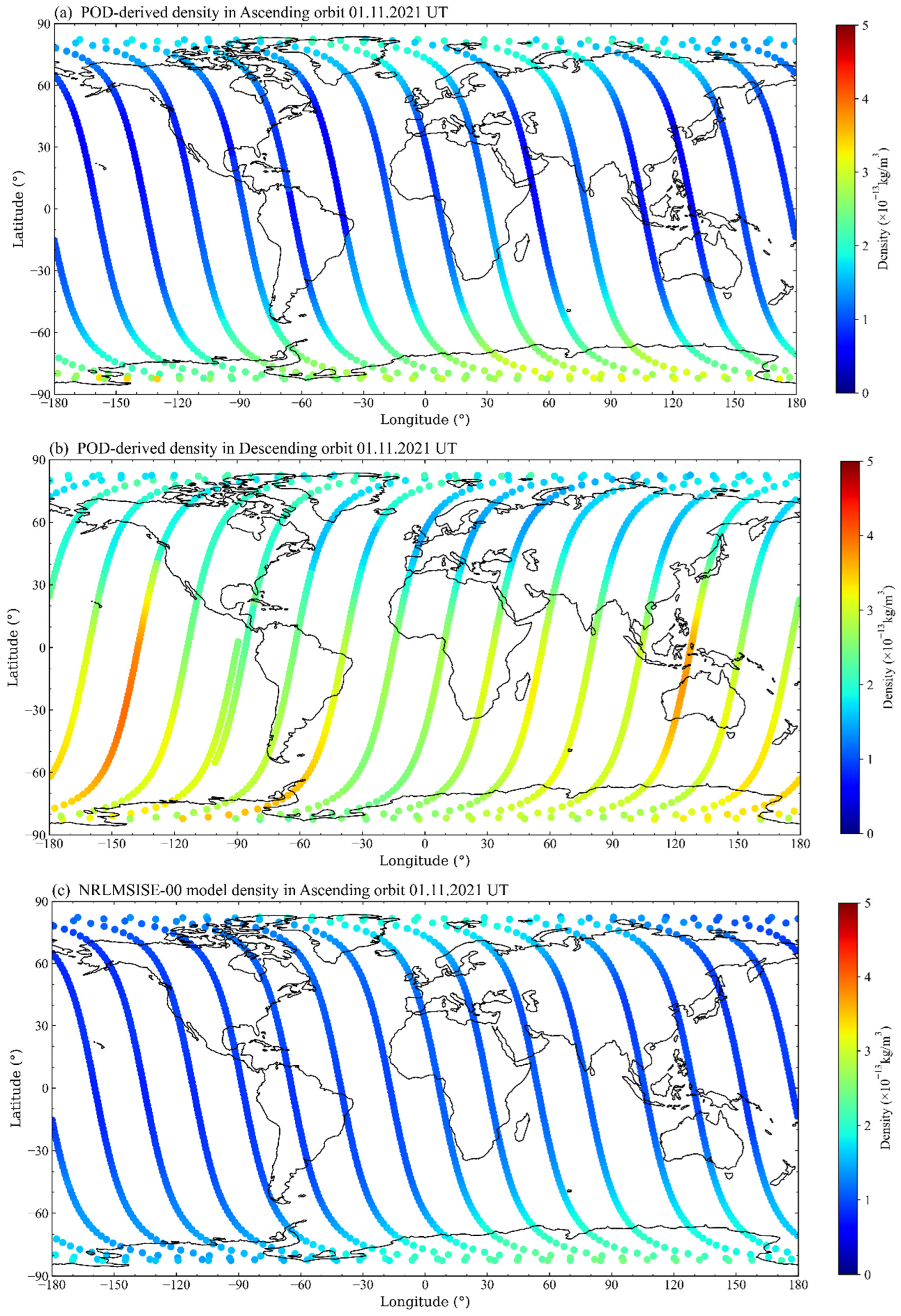
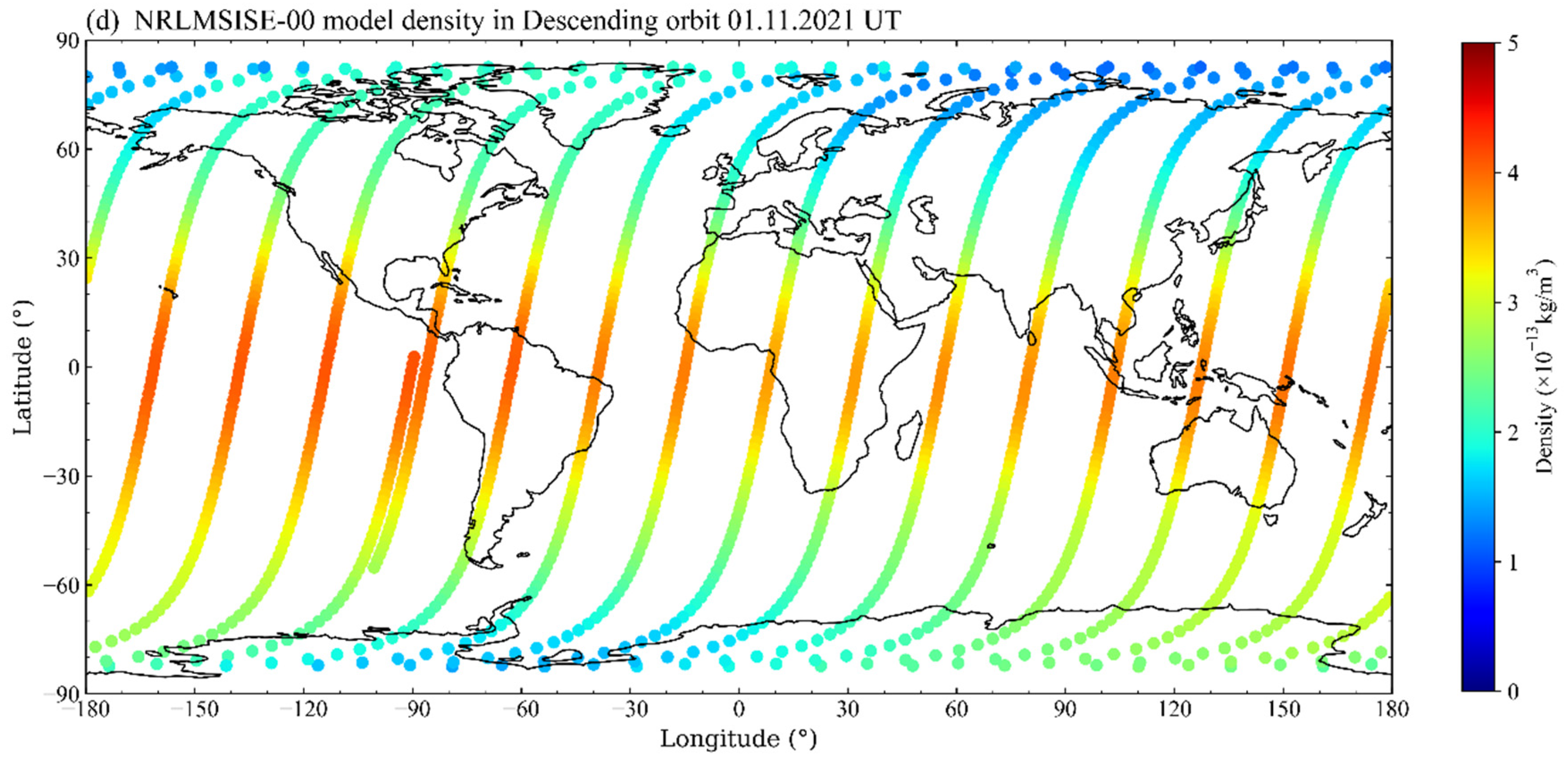
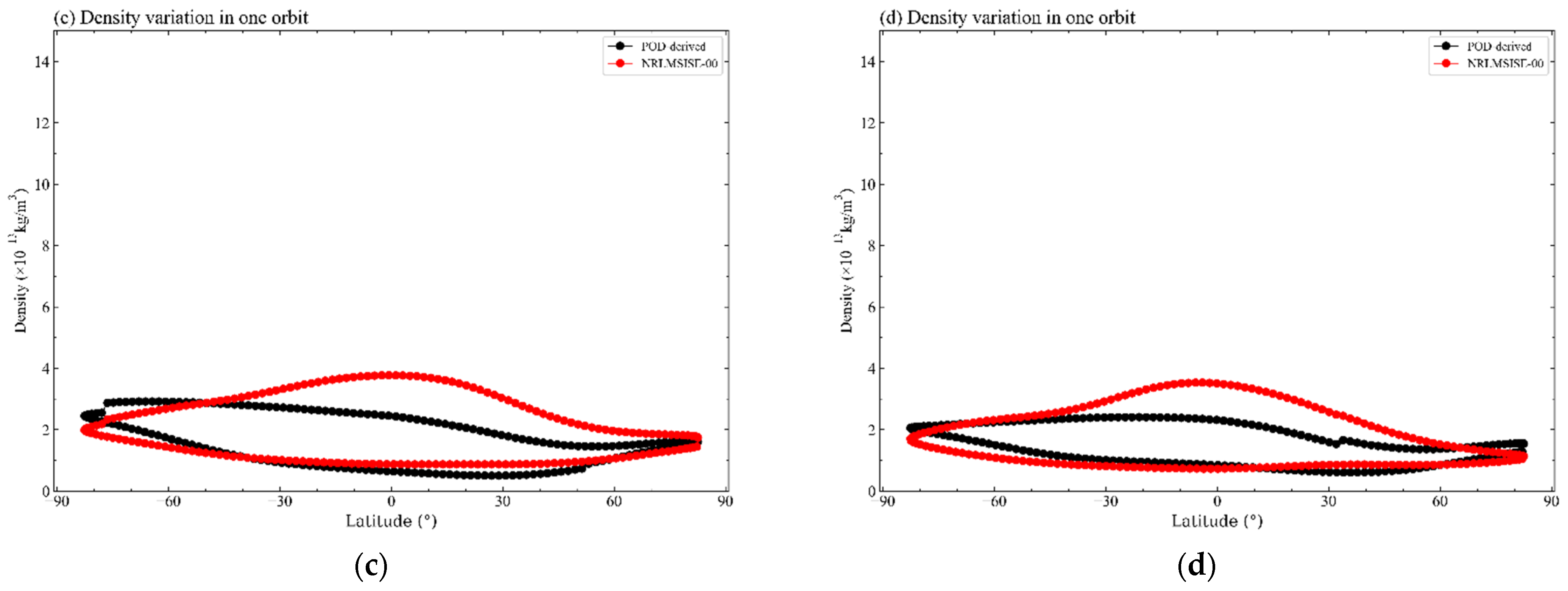
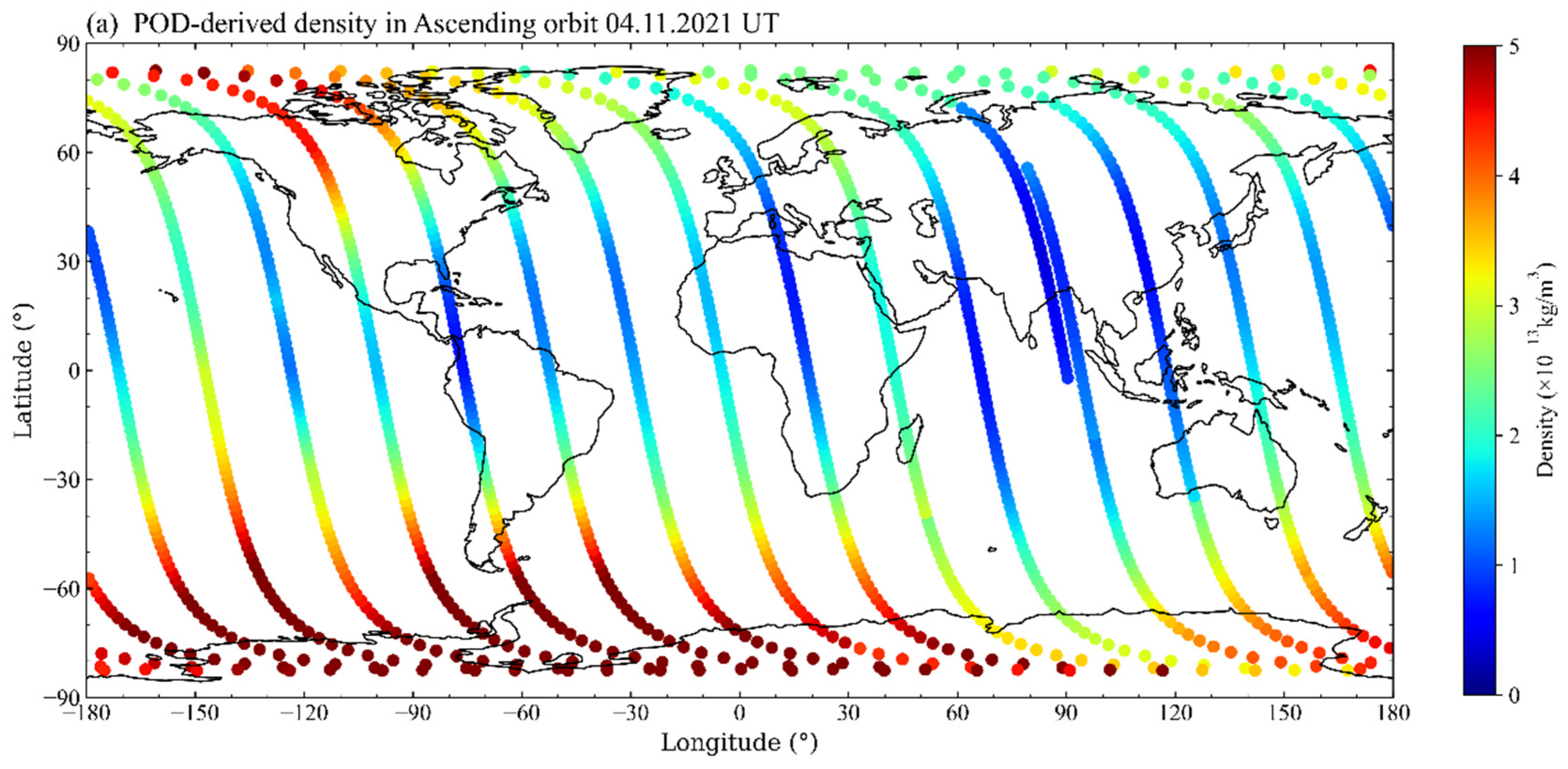
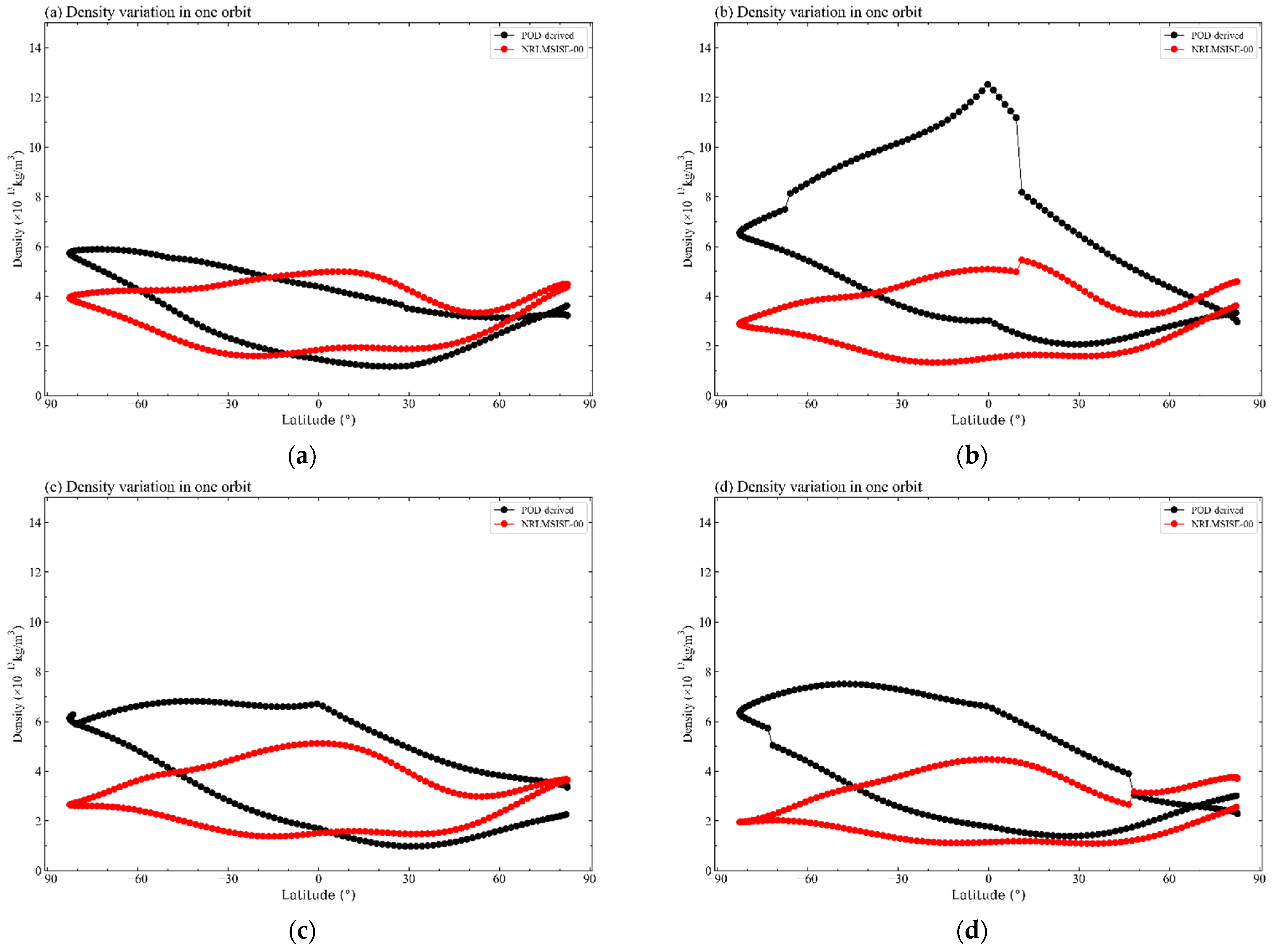
3.1. Atmospheric Mass Density Derived from the QQ-Satellite POD Observation
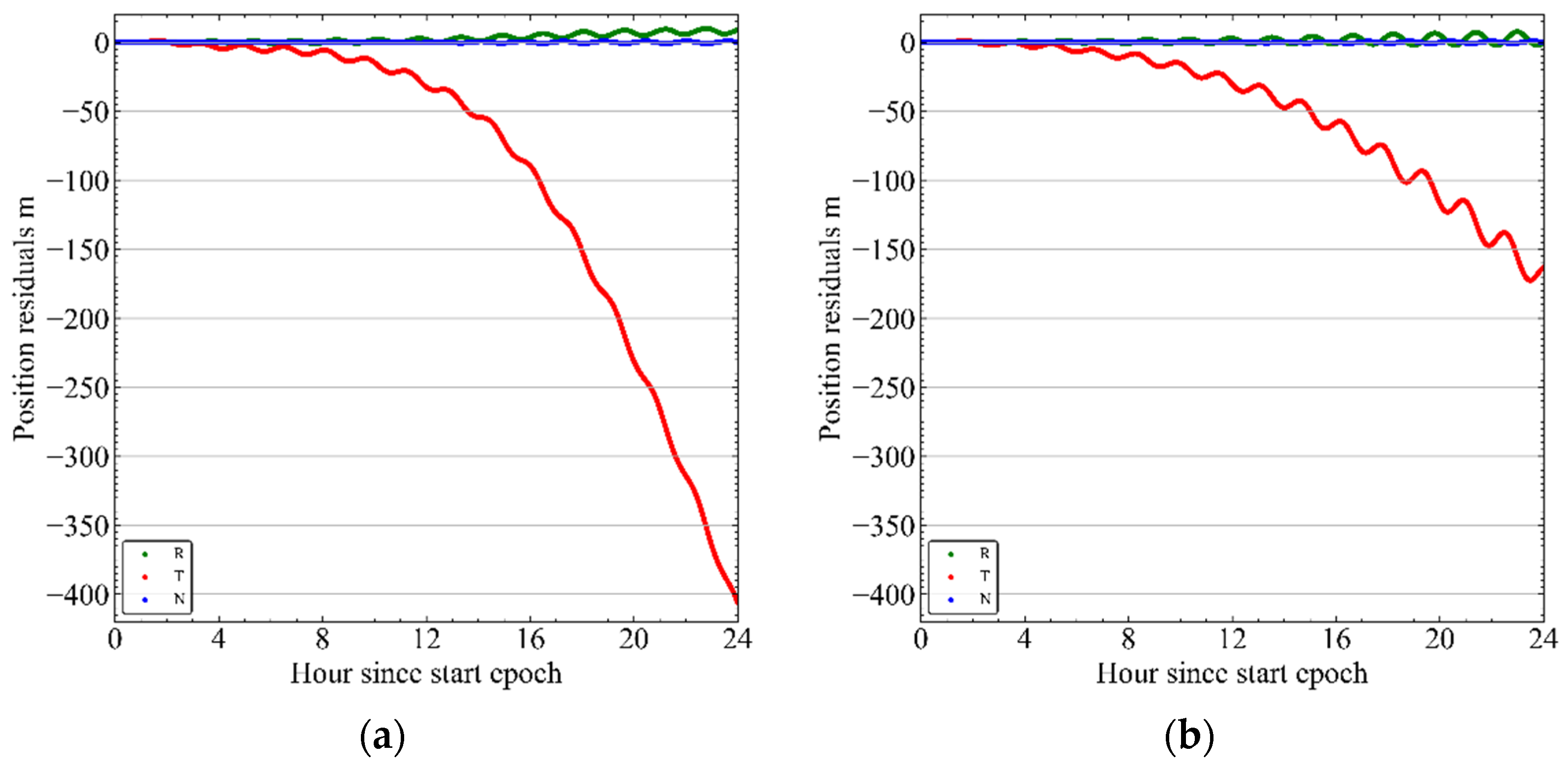
3.2. Application of the QQ-Satellite Derived Mass Density for Improved Orbit Prediction
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Oliveira, D.M.; Zesta, E.; Mehta, P.M.; Licata, R.J.; Pilinski, M.D.; Tobiska, W.K.; Hayakawa, H. The Current State and Future Directions of Modeling Thermosphere Density Enhancements During Extreme Magnetic Storms. Front. Astron. Space Sci. 2021, 8, 764144. [Google Scholar] [CrossRef]
- Zesta, E.; Huang, C.Y. Satellite Orbital Drag. In Space Weather Fundamentals; Khazanov, G.V., Ed.; CRC Press: Boca Raton, FL, USA, 2016; pp. 329–351. ISBN 978-1-315-36847-4. [Google Scholar]
- Prölss, G.W. Density Perturbations in the Upper Atmosphere Caused by the Dissipation of Solar Wind Energy. Surv. Geophys. 2011, 32, 101–195. [Google Scholar] [CrossRef]
- Emmert, J.T. Thermospheric Mass Density: A Review. Adv. Space Res. 2015, 56, 773–824. [Google Scholar] [CrossRef]
- Sang, J.; Smith, C.; Zhang, K. Towards Accurate Atmospheric Mass Density Determination Using Precise Positional Information of Space Objects. Adv. Space Res. 2012, 49, 1088–1096. [Google Scholar] [CrossRef]
- Bowman, B.; Tobiska, W.K.; Marcos, F.; Huang, C.; Lin, C.; Burke, W. A New Empirical Thermospheric Density Model JB2008 Using New Solar and Geomagnetic Indices. In Proceedings of the AIAA/AAS Astrodynamics Specialist Conference and Exhibit, American Institute of Aeronautics and Astronautics, Honolulu, HI, USA, 18–21 August 2008. [Google Scholar]
- Bruinsma, S.; Thuillier, G.; Barlier, F. The DTM-2000 Empirical Thermosphere Model with New Data Assimilation and Constraints at Lower Boundary: Accuracy and Properties. J. Atmos. Sol. Terr. Phys. 2003, 65, 1053–1070. [Google Scholar] [CrossRef]
- Picone, J.M.; Hedin, A.E.; Drob, D.P.; Aikin, A.C. NRLMSISE-00 Empirical Model of the Atmosphere: Statistical Comparisons and Scientific Issues: TECHNIQUES. J. Geophys. Res. 2002, 107, SIA 15-1–SIA 15-16. [Google Scholar] [CrossRef]
- Bruinsma, S.; Tamagnan, D.; Biancale, R. Atmospheric Densities Derived from CHAMP/STAR Accelerometer Observations. Planet. Space Sci. 2004, 52, 297–312. [Google Scholar] [CrossRef]
- Gondelach, D.J.; Linares, R. Real-Time Thermospheric Density Estimation via Radar and GPS Tracking Data Assimilation. Space Weather 2021, 19, e2020SW002620. [Google Scholar] [CrossRef]
- Montenbruck, O.; Gill, E. Satellite Orbits: Models, Methods, and Applications: With... 47 Tables, 1st ed.; Corr. 3. Printing; Springer: Berlin/Heidelberg, Germany, 2005; ISBN 978-3-642-58351-3. [Google Scholar]
- Storz, M.F.; Bowman, B.R.; Branson, M.J.I.; Casali, S.J.; Tobiska, W.K. High Accuracy Satellite Drag Model (HASDM). Adv. Space Res. 2005, 36, 2497–2505. [Google Scholar] [CrossRef]
- Doornbos, E.; Klinkrad, H.; Visser, P. Atmospheric Density Calibration Using Satellite Drag Observations. Adv. Space Res. 2005, 36, 515–521. [Google Scholar] [CrossRef]
- Reigber, C.; Lühr, H.; Schwintzer, P. CHAMP Mission Status. Adv. Space Res. 2002, 30, 129–134. [Google Scholar] [CrossRef]
- Tapley, B.D.; Bettadpur, S.; Watkins, M.; Reigber, C. The Gravity Recovery and Climate Experiment: Mission Overview and Early Results: Grace mission overview and early results. Geophys. Res. Lett. 2004, 31, 9. [Google Scholar] [CrossRef] [Green Version]
- Picone, J.M. Thermospheric Densities Derived from Spacecraft Orbits: Accurate Processing of Two-Line Element Sets. J. Geophys. Res. 2005, 110, A03301. [Google Scholar] [CrossRef]
- Chen, J.; Sang, J. Thermospheric Mass Density Measurement from Precise Orbit Ephemeris. Geod. Geodyn. 2016, 7, 210–215. [Google Scholar] [CrossRef] [Green Version]
- Kuang, D.; Desai, S.; Sibthorpe, A.; Pi, X. Measuring Atmospheric Density Using GPS–LEO Tracking Data. Adv. Space Res. 2014, 53, 243–256. [Google Scholar] [CrossRef]
- Van den IJssel, J.; Doornbos, E.; Iorfida, E.; March, G.; Siemes, C.; Montenbruck, O. Thermosphere Densities Derived from Swarm GPS Observations. Adv. Space Res. 2020, 65, 1758–1771. [Google Scholar] [CrossRef]
- Montenbruck, O.; van Helleputte, T.; Kroes, R.; Gill, E. Reduced Dynamic Orbit Determination Using GPS Code and Carrier Measurements. Aerosp. Sci. Technol. 2005, 9, 261–271. [Google Scholar] [CrossRef]
- Bowman, B.R.; Moe, K. Drag Coefficient Variability at 175–500 km from the Orbit Decay Analyses of Spheres (AAS 05-257). Adv. Astronaut. Sci. 2006, 123, 117. [Google Scholar]
- Lean, J.L.; Picone, J.M.; Emmert, J.T.; Moore, G. Thermospheric Densities Derived from Spacecraft Orbits: Application to the Starshine Satellites. J. Geophys. Res. 2006, 111, A04301. [Google Scholar] [CrossRef] [Green Version]
- Bai, S.; Wen, G.; Wang, Z. Atmospheric Density Model Optimization and Spacecraft Orbit Prediction Improvements Based on Q-Sat Orbit Data. arXiv 2021, arXiv:2112.03113. [Google Scholar]
- Jäggi, A.; Hugentobler, U.; Bock, H.; Beutler, G. Precise Orbit Determination for GRACE Using Undifferenced or Doubly Differenced GPS Data. Adv. Space Res. 2007, 39, 1612–1619. [Google Scholar] [CrossRef]
- McLaughlin, C.A.; Lechtenberg, T.; Fattig, E.; Krishna, D.M. Estimating Density Using Precision Satellite Orbits from Multiple Satellites. J. Astronaut. Sci. 2012, 59, 84–100. [Google Scholar] [CrossRef]
- McLaughlin, C.A.; Hiatt, A.; Lechtenberg, T. Precision Orbit Derived Total Density. J. Spacecr. Rocket. 2011, 48, 166–174. [Google Scholar] [CrossRef]
- Vallado, D.A. Fundamentals of Astrodynamics and Applications, 2nd ed.; Space Technology Library; Springer: Dordrecht, The Netherlands, 2001. [Google Scholar]
- Doornbos, E. Air Density Models Derived from Multi-Satellite Drag Observations. In Proceedings of the ESAs Second Swarm International Science Meeting, Potsdam, Germany, 24 June 2009; p. 317. [Google Scholar]
- Cook, G.E. Satellite Drag Coefficients; Planetary and Space Science: McLean, VA, USA, 1966; p. 39. [Google Scholar]
- Lemoine, F.G.; Kenyon, S.C.; Factor, J.K.; Trimmer, R.G.; Pavlis, N.K.; Chinn, D.S.; Cox, C.M.; Klosko, S.M.; Luthcke, S.B.; Torrence, M.H.; et al. The Development of the Joint NASA GSFC and the National Imagery and Mapping Agency (NIMA) Geopotential Model EGM96; NASA Goddard Space Flight Center: Greenbelt, Maryland, USA, 1998; p. 63. [Google Scholar]
- Eanes, R.J.; Bettadpur, S. The CSR 3.0 Global Ocean Tide Model: Diurnal and Semidiurnal Tides from TOPEX/POSEIDON Altimetry; Technical Memorandum; Center for Space Research, the University of Texas at Austin: Austin, TX, USA, 1996. [Google Scholar]
- Petit, G.; Luzum, B. IERS Technical Note; IERS Standards: Paris, French, 1989; No. 36; p. 179. [Google Scholar]
- Folkner, W.M.; Williams, J.G.; Boggs, D.H.; Park, R.S.; Kuchynka, P. The Planetary and Lunar Ephemerides DE430 and DE431. Interplanet. Netw. Prog. Rep. 2014, 196, 42–196. [Google Scholar]
- Annex, A.; Carcich, B.; Murakami, S.; Kulumani, S.; de Val-Borro, M.; Stefko, M.; Diaz del Rio, J.; Seignovert, B. SpiceyPy: Python Wrapper for the NAIF C SPICE Toolkit; Astrophysics Source Code Library: Pasadena, CA, USA, 2019. [Google Scholar]
- He, C.; Yang, Y.; Carter, B.; Kerr, E.; Wu, S.; Deleflie, F.; Cai, H.; Zhang, K.; Sagnières, L.; Norman, R. Review and Comparison of Empirical Thermospheric Mass Density Models. Prog. Aerosp. Sci. 2018, 103, 31–51. [Google Scholar] [CrossRef]
- Nettleton, D. Chapter 6—Selection of Variables and Factor Derivation. In Commercial Data Mining; Nettleton, D., Ed.; Morgan Kaufmann: Boston, MA, USA, 2014; pp. 79–104. ISBN 978-0-12-416602-8. [Google Scholar]
- Sang, J.; Zhang, K. A New Concept of Real Time Improvement of Atmospheric Mass Density Models and Its Validation Using CHAMP GPS-Derived Precision Orbit Data. J. Glob. Position. Syst. 2010, 9, 104–111. [Google Scholar]
- Peng, Y.; Scales, W.A.; Lin, D. GNSS-Based Hardware-in-the-Loop Simulations of Spacecraft Formation Flying with the Global Ionospheric Model TIEGCM. GPS Solut. 2021, 25, 65. [Google Scholar] [CrossRef]
- Codrescu, M.V.; Negrea, C.; Fedrizzi, M.; Fuller-Rowell, T.J.; Dobin, A.; Jakowsky, N.; Khalsa, H.; Matsuo, T.; Maruyama, N. A Real-Time Run of the Coupled Thermosphere Ionosphere Plasmasphere Electrodynamics (CTIPe) Model. Space Weather 2012, 10, S02001. [Google Scholar] [CrossRef]
- Ridley, A.J.; Deng, Y.; Tóth, G. The Global Ionosphere–Thermosphere Model. J. Atmos. Sol.-Terr. Phys. 2006, 68, 839–864. [Google Scholar] [CrossRef]
- Tapping, K.F. The 10.7 Cm Solar Radio Flux (F10.7). Space Weather 2013, 11, 394–406. [Google Scholar] [CrossRef]
- Vallado, D.A.; Finkleman, D. A Critical Assessment of Satellite Drag and Atmospheric Density Modeling. Acta Astronaut. 2014, 95, 141–165. [Google Scholar] [CrossRef]
- Menvielle, M.; Berthelier, A. The K-Derived Planetary Indices: Description and Availability. Rev. Geophys. 1991, 29, 415–432. [Google Scholar] [CrossRef]



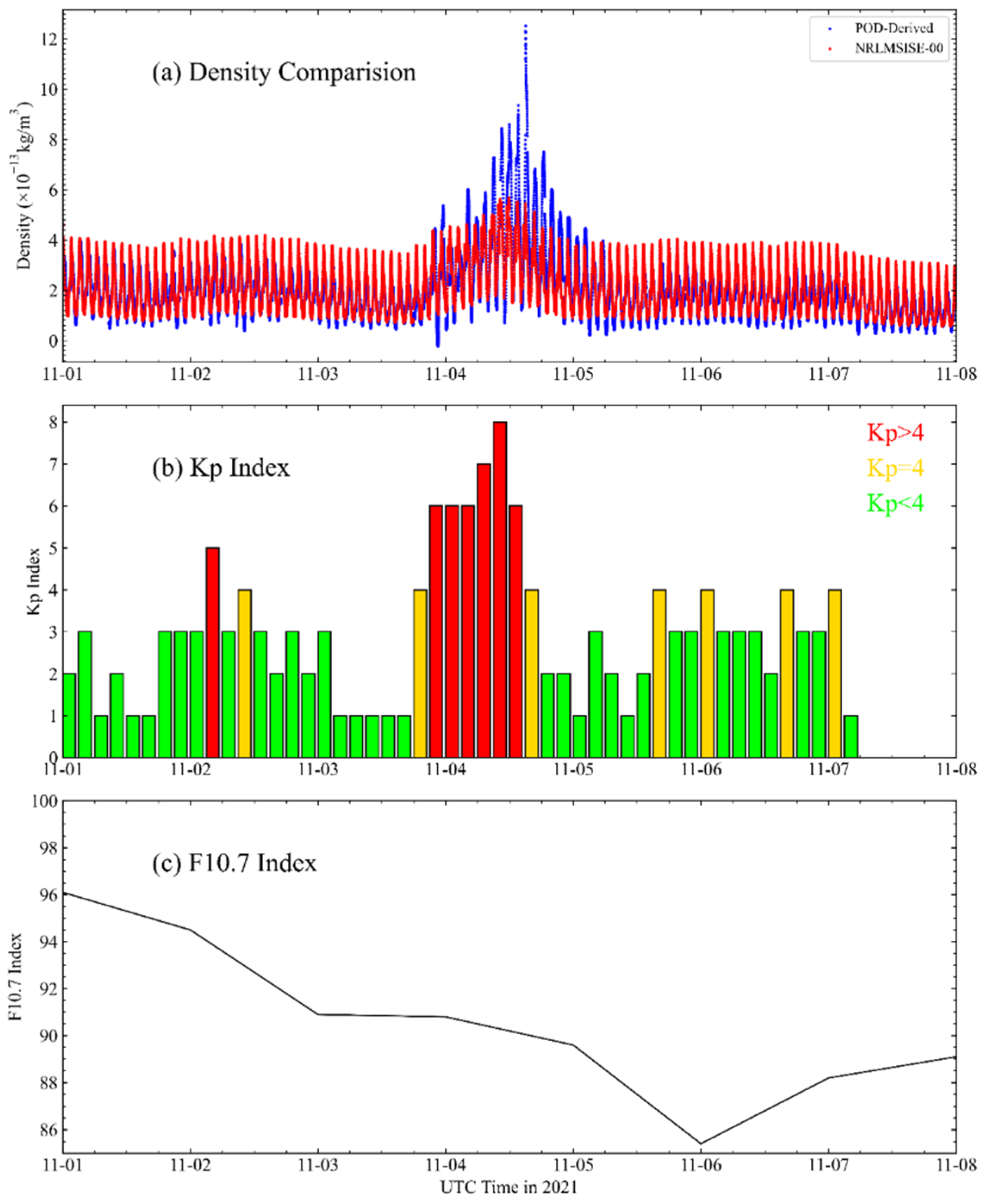
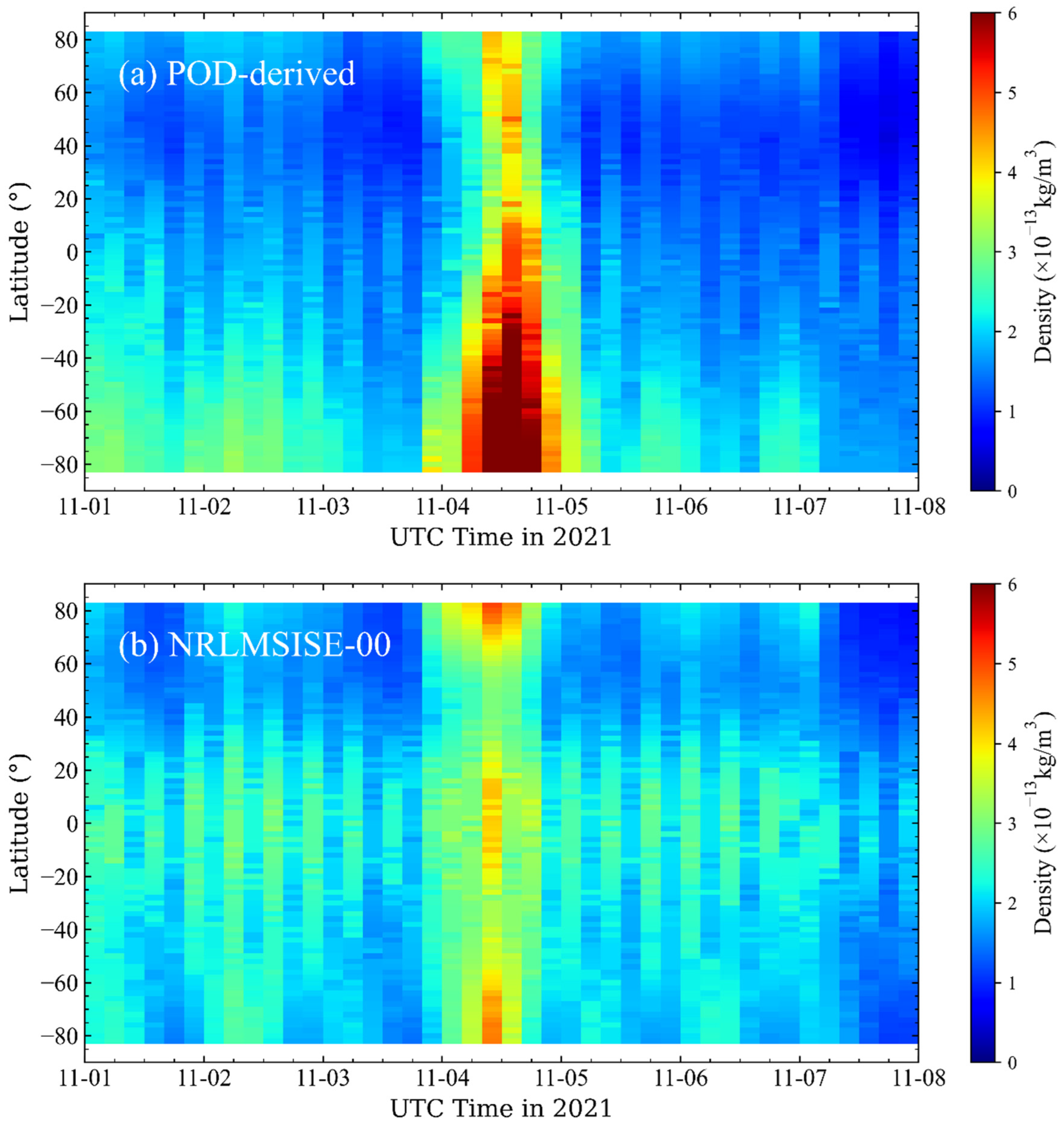
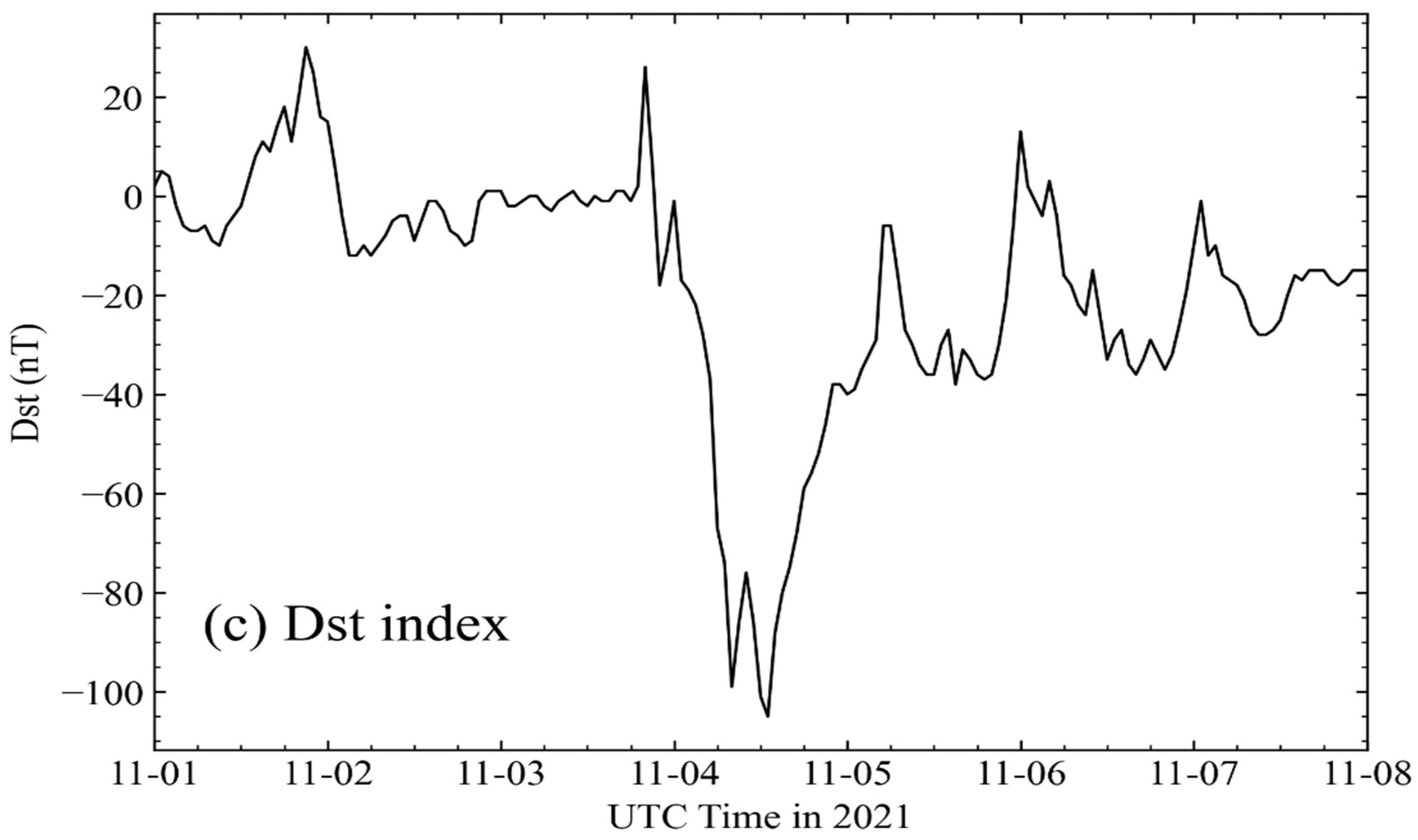
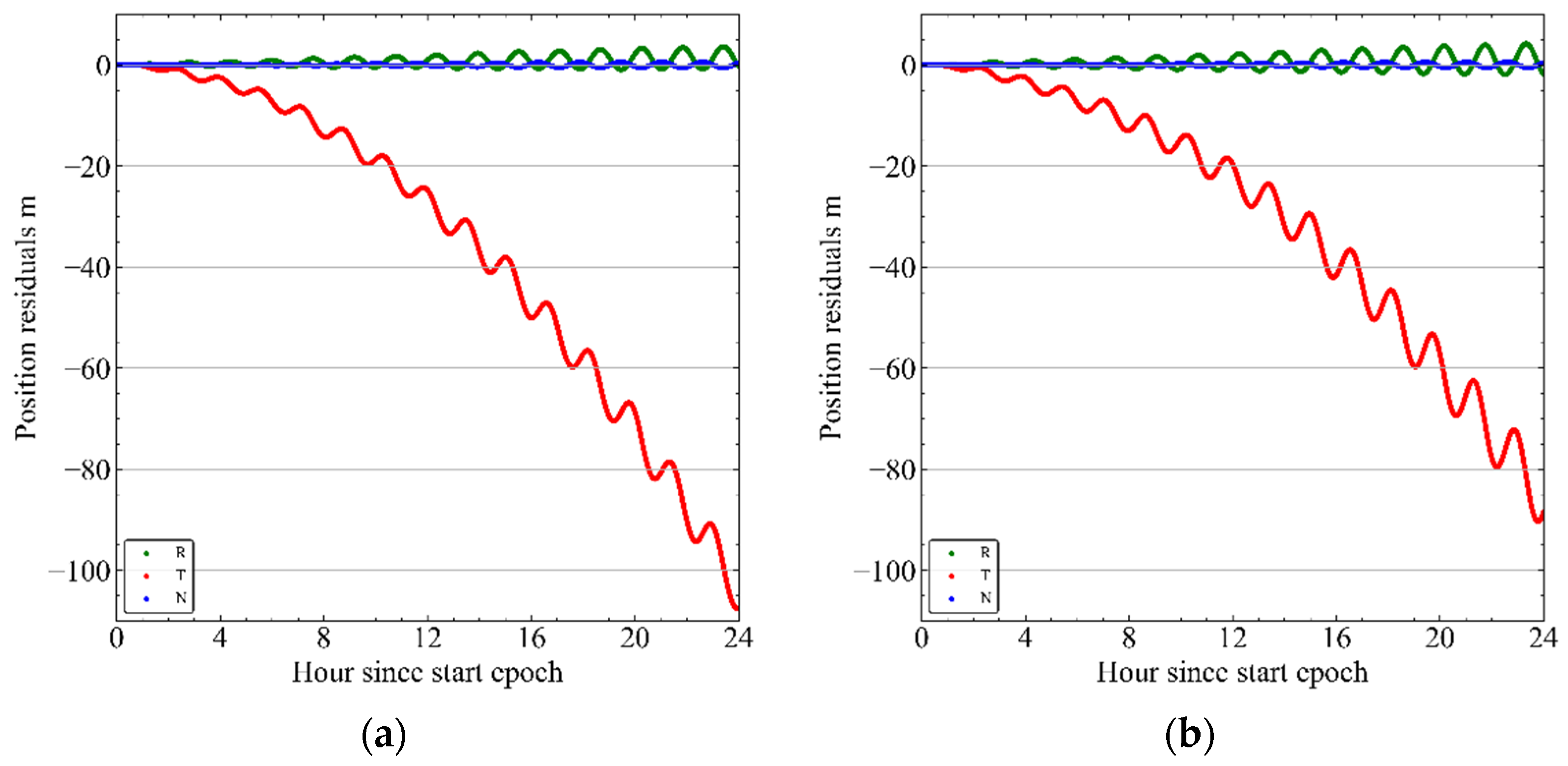
| UTC Time in 2021 | Correlation Coefficient | Mean Difference |
|---|---|---|
| 1 November | 0.823 | −3.41% |
| 2 November | 0.762 | −4.21% |
| 3 November | 0.725 | −8.74% |
| 4 November | 0.686 | −27.1% |
| 5 November | 0.749 | −6.22% |
| 6 November | 0.758 | −8.14% |
| 7 November | 0.833 | −10.8% |
| UTC Time | 3D RMS Model | 3D RMS Calibrated | Improved Percentage |
|---|---|---|---|
| 2021.11.1 | 25.46 m | 22.53 m | 11.51% |
| 2021.11.4 | 85.51 m | 40.89 m | 52.18% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, Y.; Wang, B.; Meng, X.; Tang, X.; Yan, F.; Zhang, X.; Bai, W.; Du, Q.; Wang, X.; Cai, Y.; et al. Analysis of Orbital Atmospheric Density from QQ-Satellite Precision Orbits Based on GNSS Observations. Remote Sens. 2022, 14, 3873. https://doi.org/10.3390/rs14163873
Sun Y, Wang B, Meng X, Tang X, Yan F, Zhang X, Bai W, Du Q, Wang X, Cai Y, et al. Analysis of Orbital Atmospheric Density from QQ-Satellite Precision Orbits Based on GNSS Observations. Remote Sensing. 2022; 14(16):3873. https://doi.org/10.3390/rs14163873
Chicago/Turabian StyleSun, Yueqiang, Bowen Wang, Xiangguang Meng, Xinchun Tang, Feng Yan, Xianguo Zhang, Weihua Bai, Qifei Du, Xianyi Wang, Yuerong Cai, and et al. 2022. "Analysis of Orbital Atmospheric Density from QQ-Satellite Precision Orbits Based on GNSS Observations" Remote Sensing 14, no. 16: 3873. https://doi.org/10.3390/rs14163873
APA StyleSun, Y., Wang, B., Meng, X., Tang, X., Yan, F., Zhang, X., Bai, W., Du, Q., Wang, X., Cai, Y., Guo, B., Wei, S., Qiao, H., Hu, P., Li, Y., & Wang, X. (2022). Analysis of Orbital Atmospheric Density from QQ-Satellite Precision Orbits Based on GNSS Observations. Remote Sensing, 14(16), 3873. https://doi.org/10.3390/rs14163873






