Dual-Sideband Constant-Envelope Frequency-Hopping Binary Offset Carrier Multiplexing Modulation for Satellite Navigation
Abstract
1. Introduction
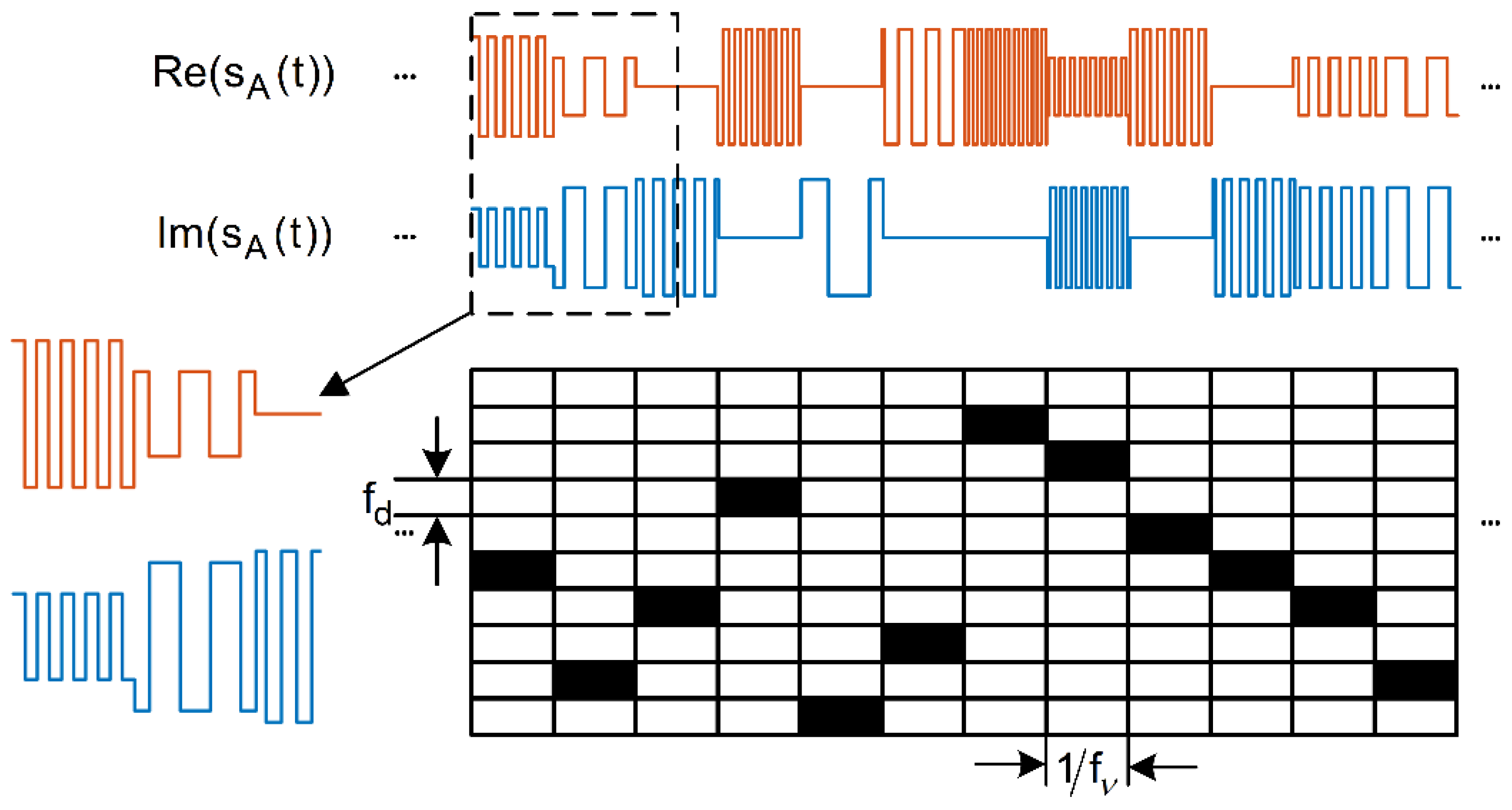
2. ACE-FHBOC Multiplexing
3. Typical Applications of ACE-FHBOC Multiplexing
3.1. Three Typical Applications
3.2. Decomposed Form of ACE-FHBOC
4. Generation Scheme
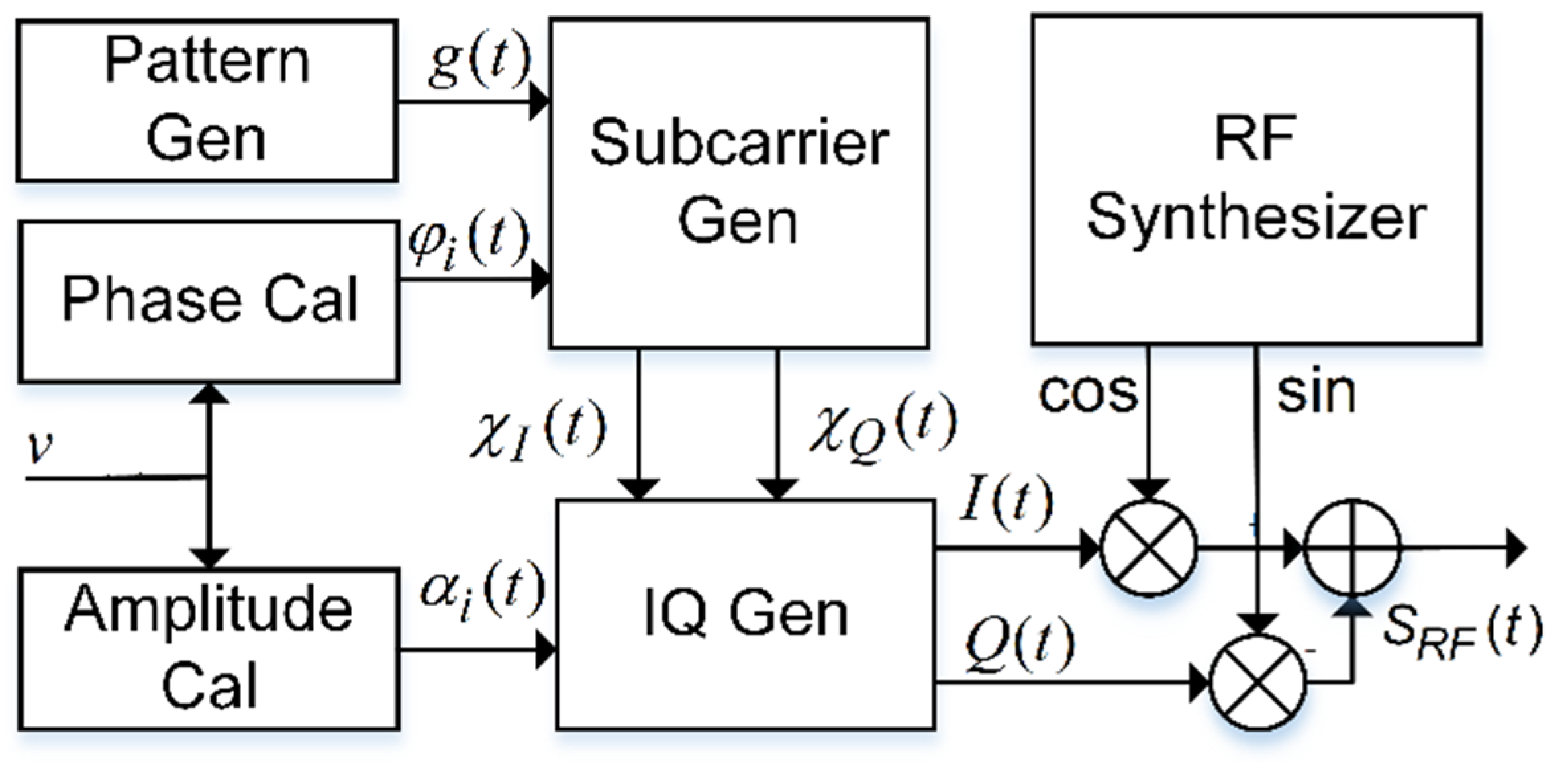
4.1. Direct Generation
4.1.1. Direct Generation with Composed Subcarriers
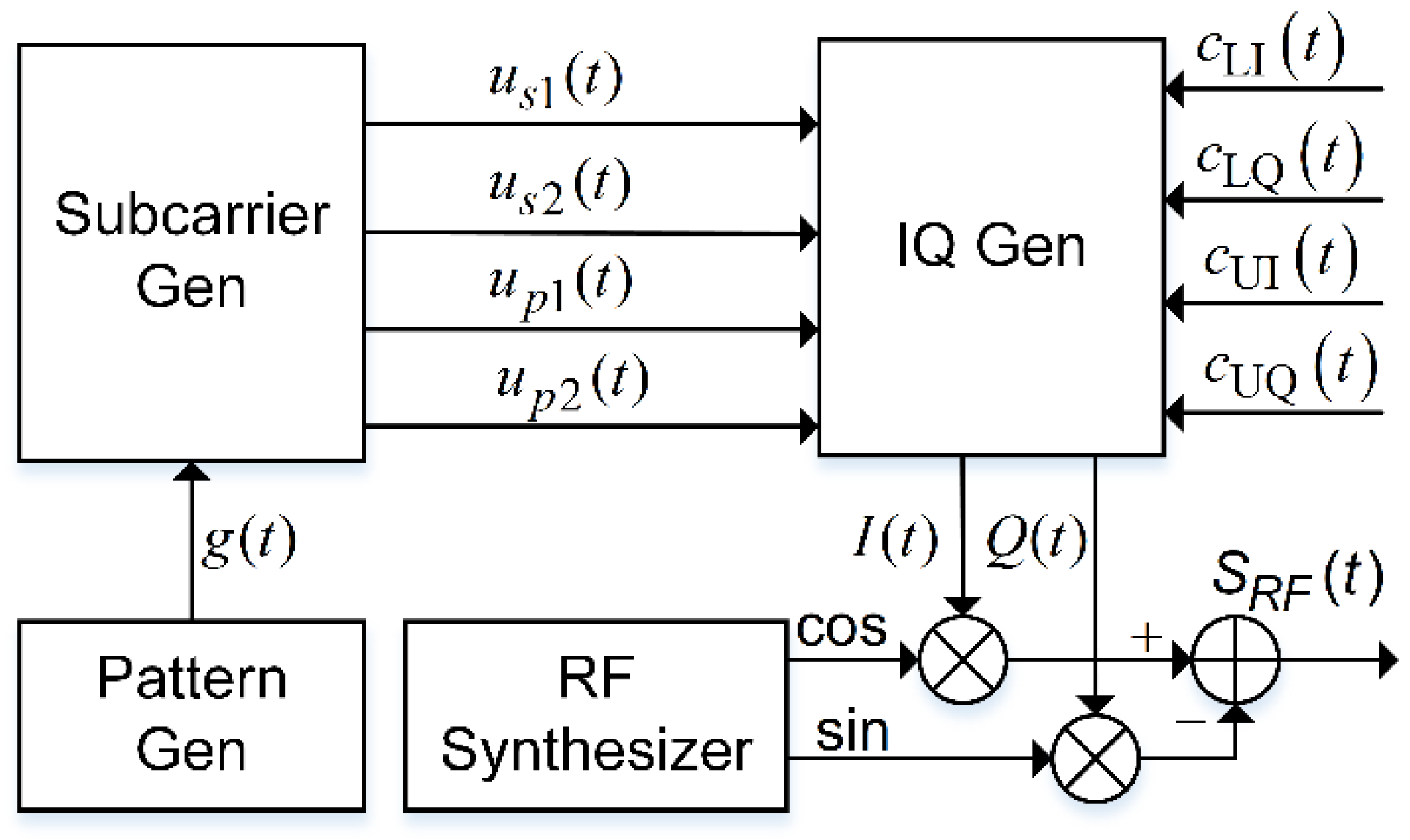
4.1.2. Direct Generation with Decomposed Subcarriers
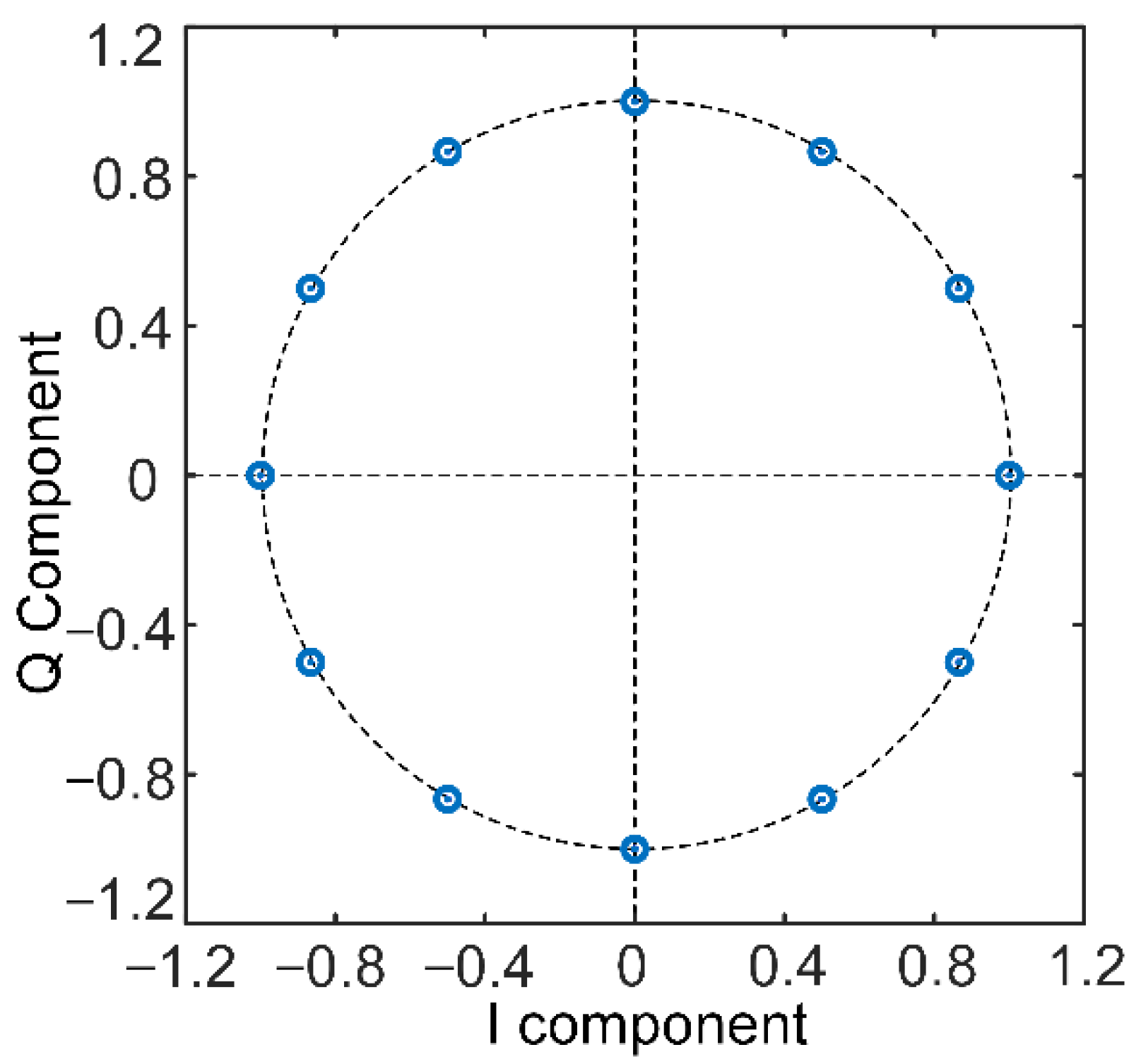
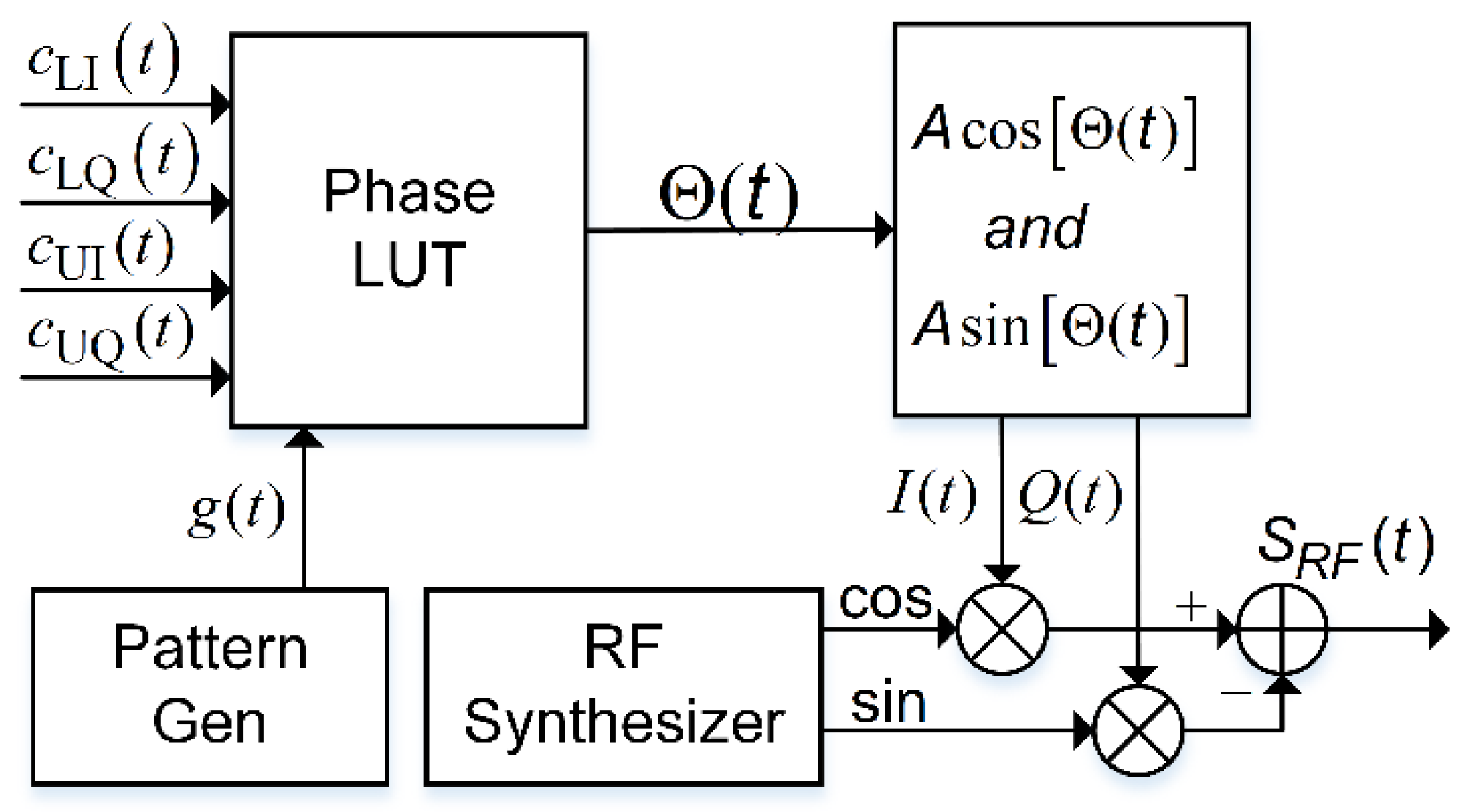
4.2. Phase Rotation Generation
4.3. Generation Complexity
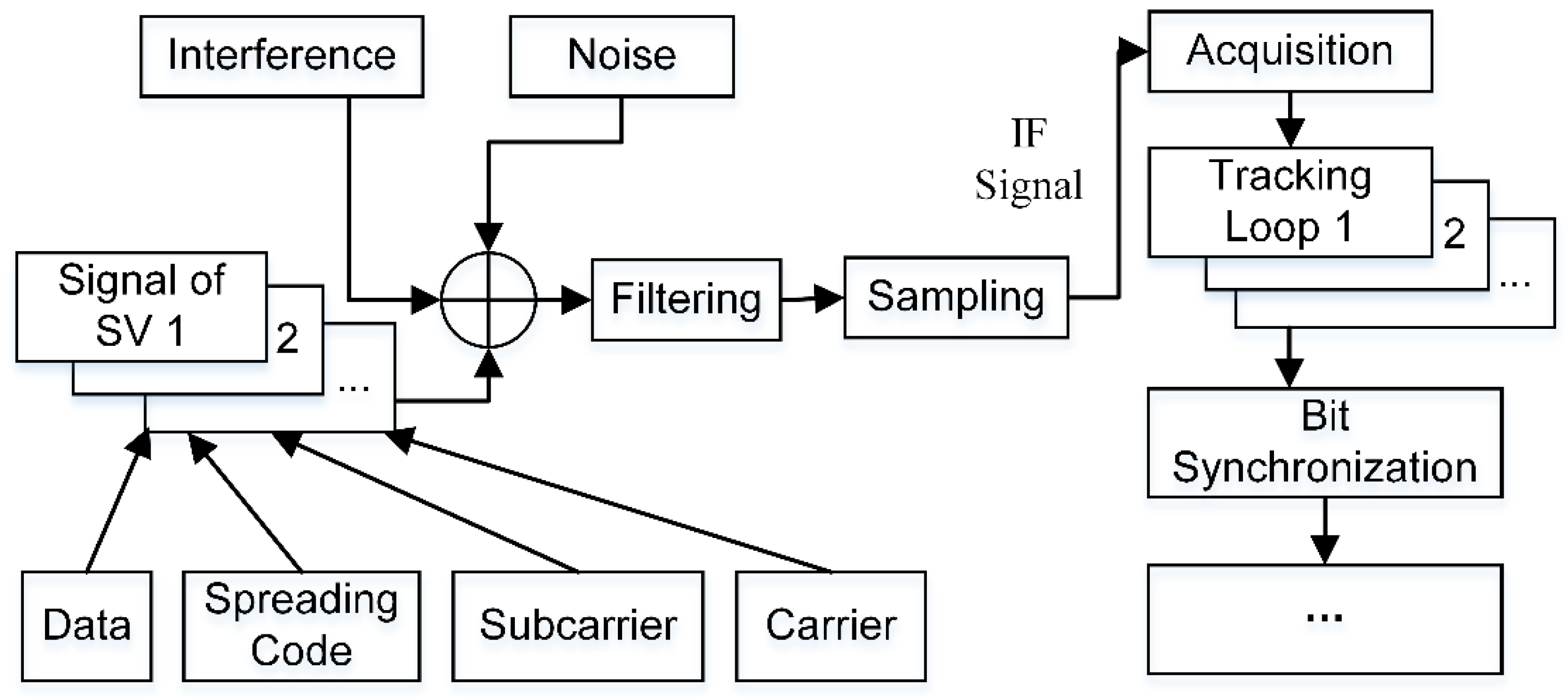
5. Performance Analysis
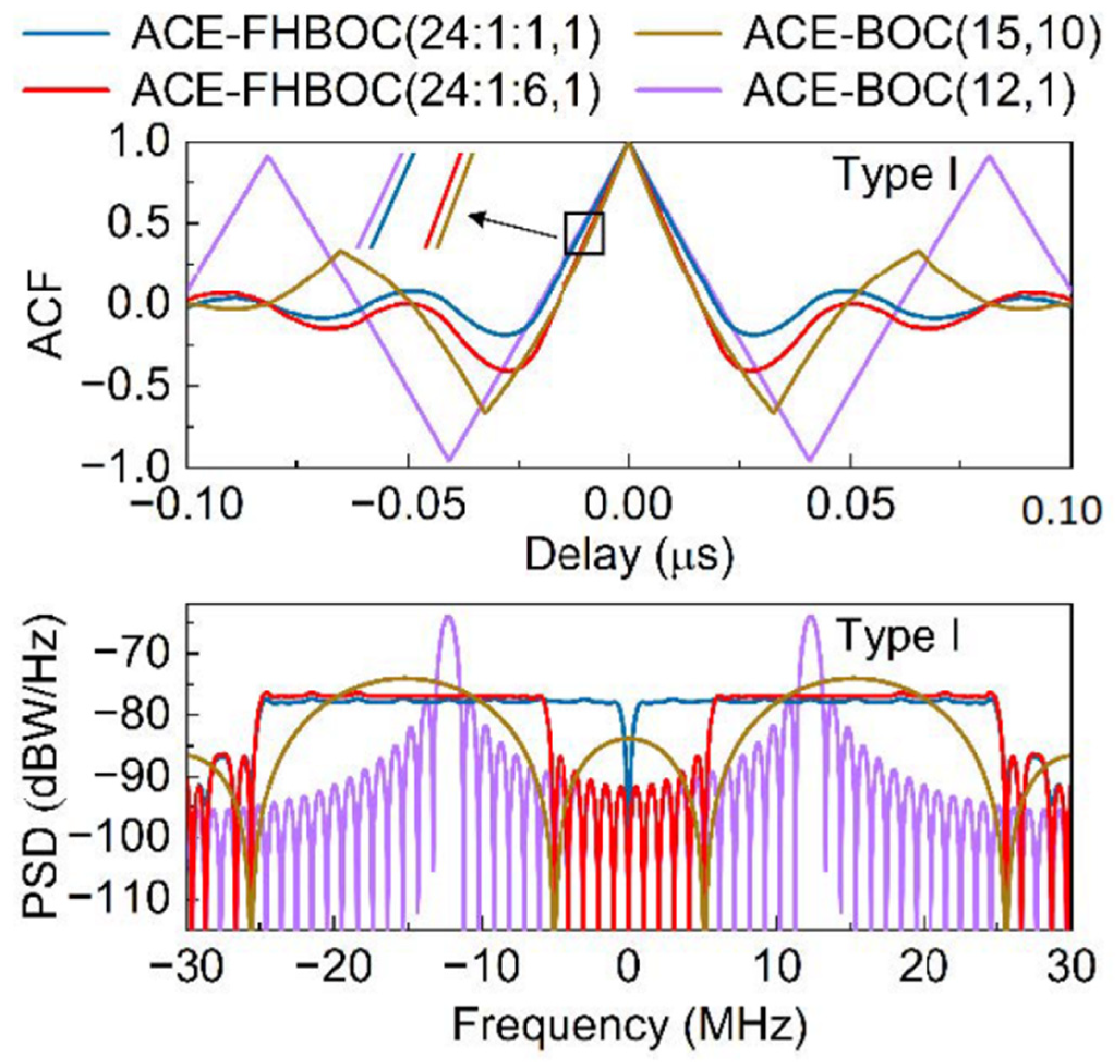
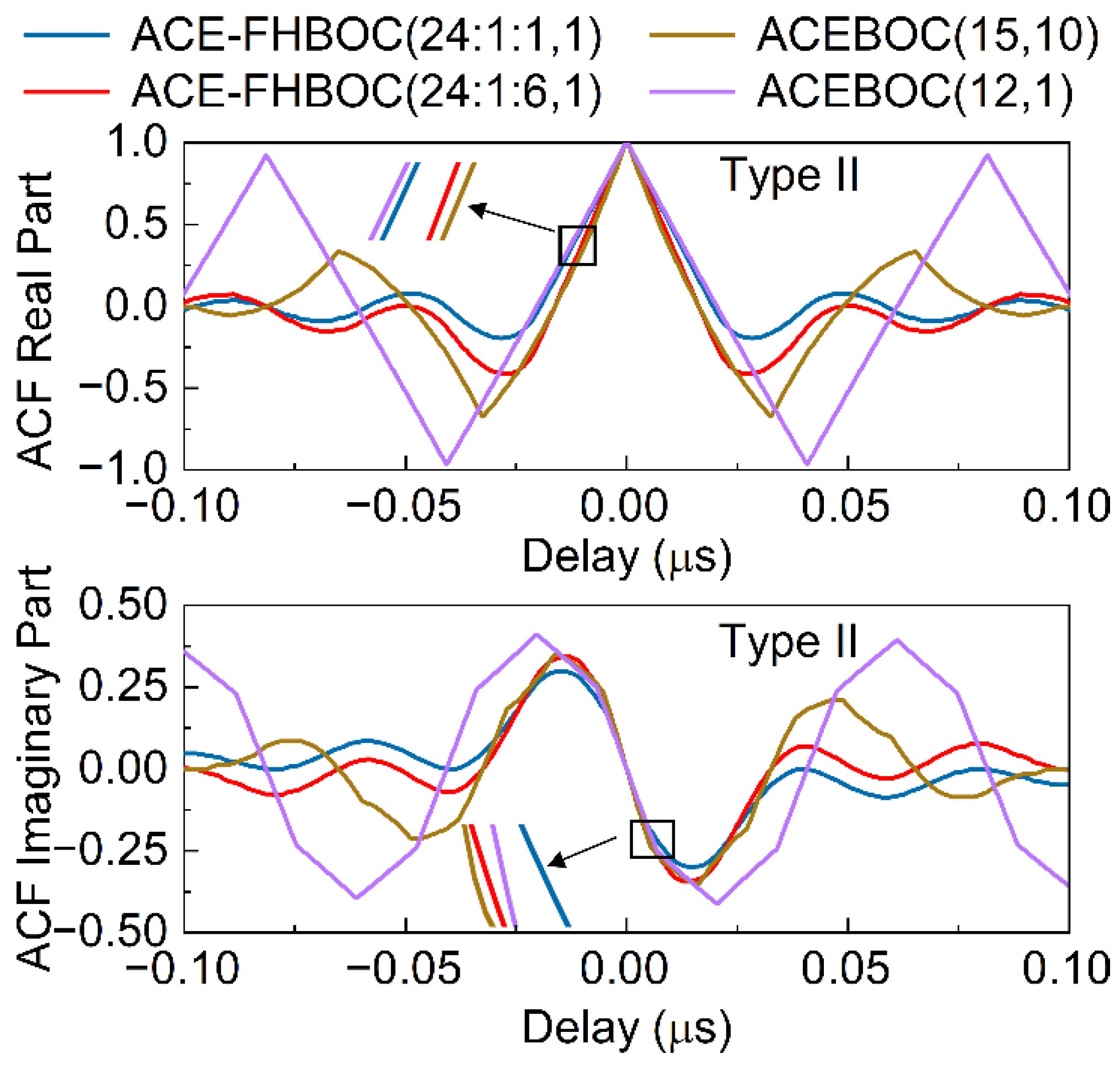
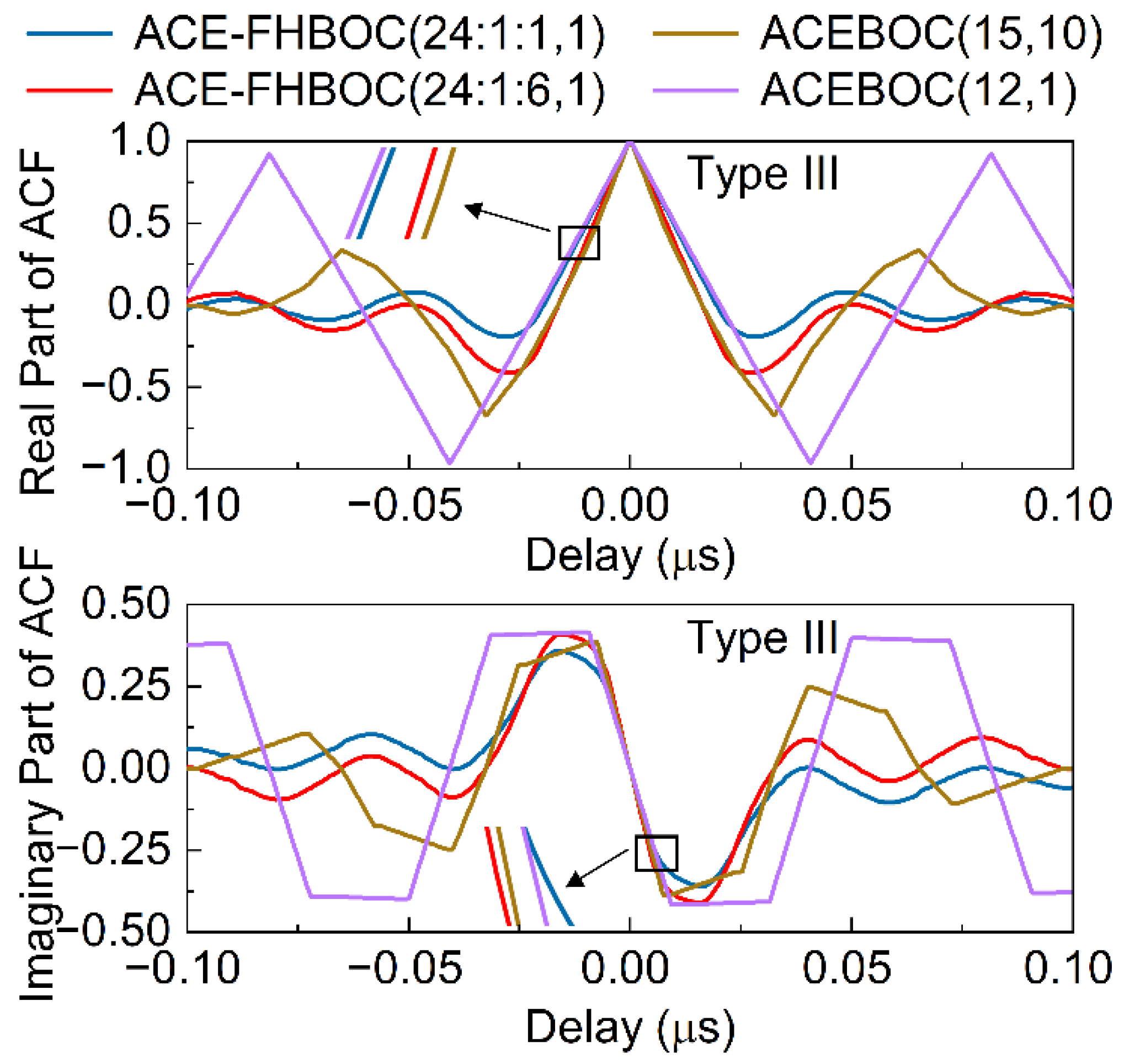
5.1. ACF and PSD
5.2. Numerical Simulation
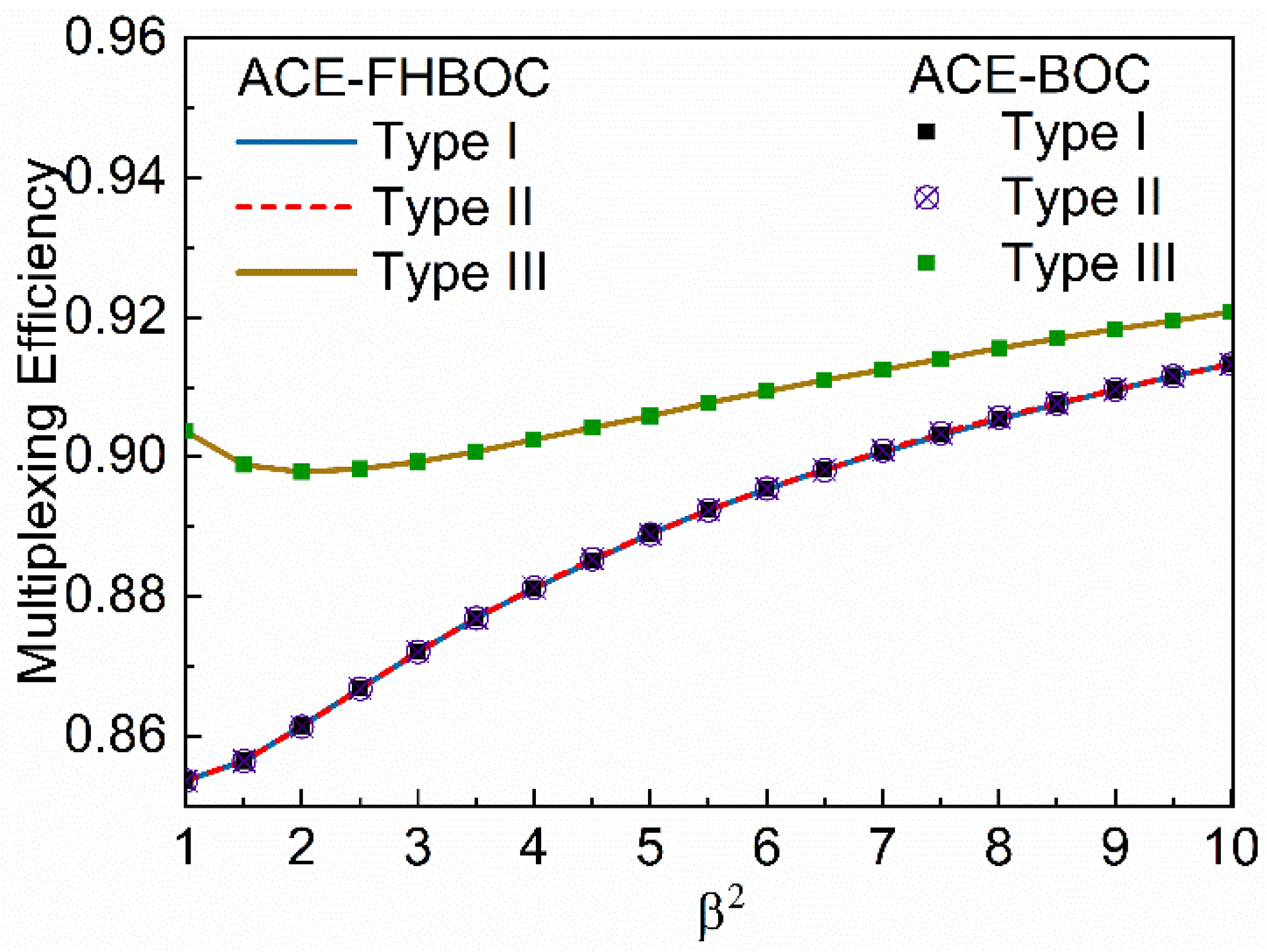
5.2.1. Multiplexing Efficiency
5.2.2. Probability of False Locking on ACF Side Peaks
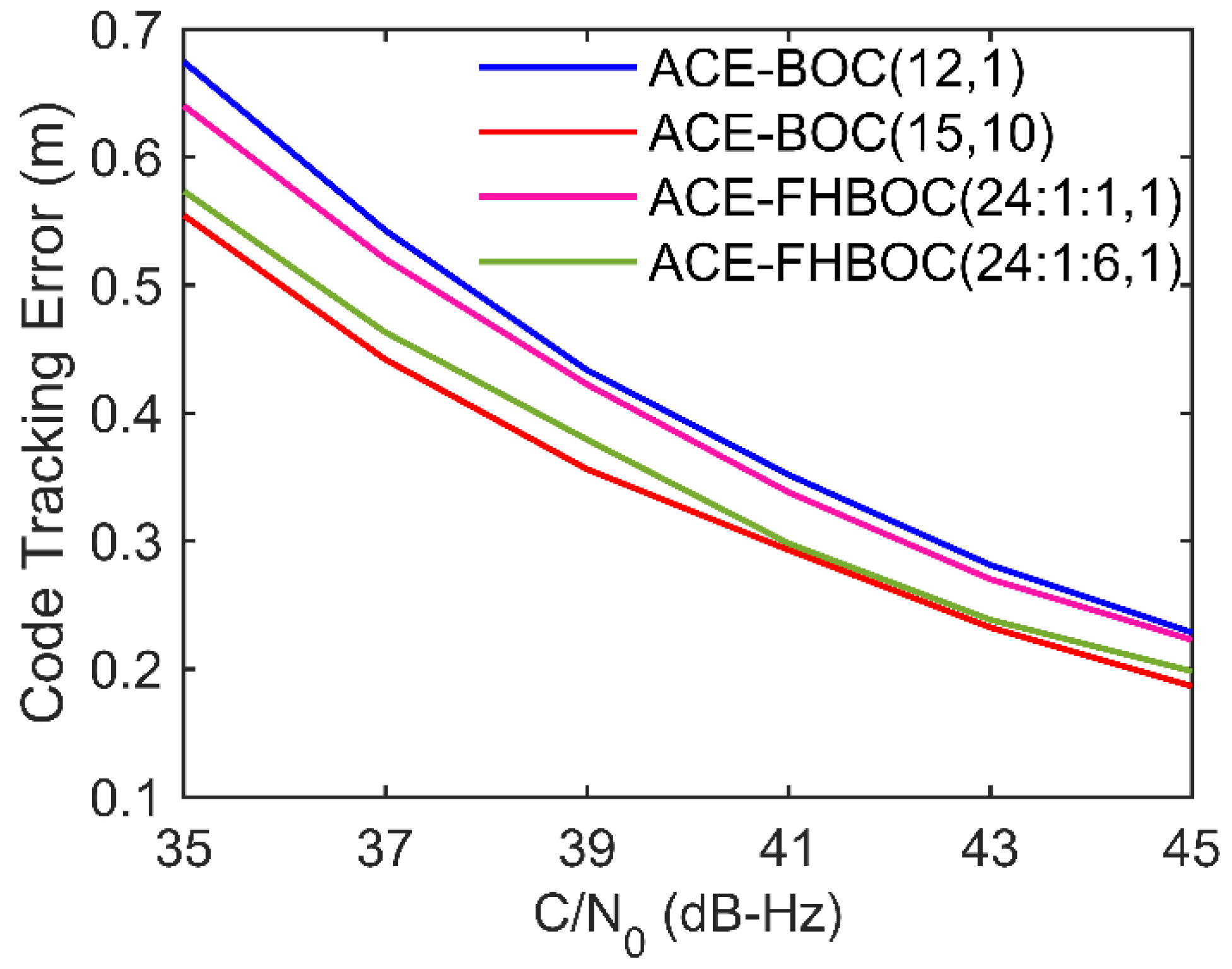
5.2.3. Tracking Performance
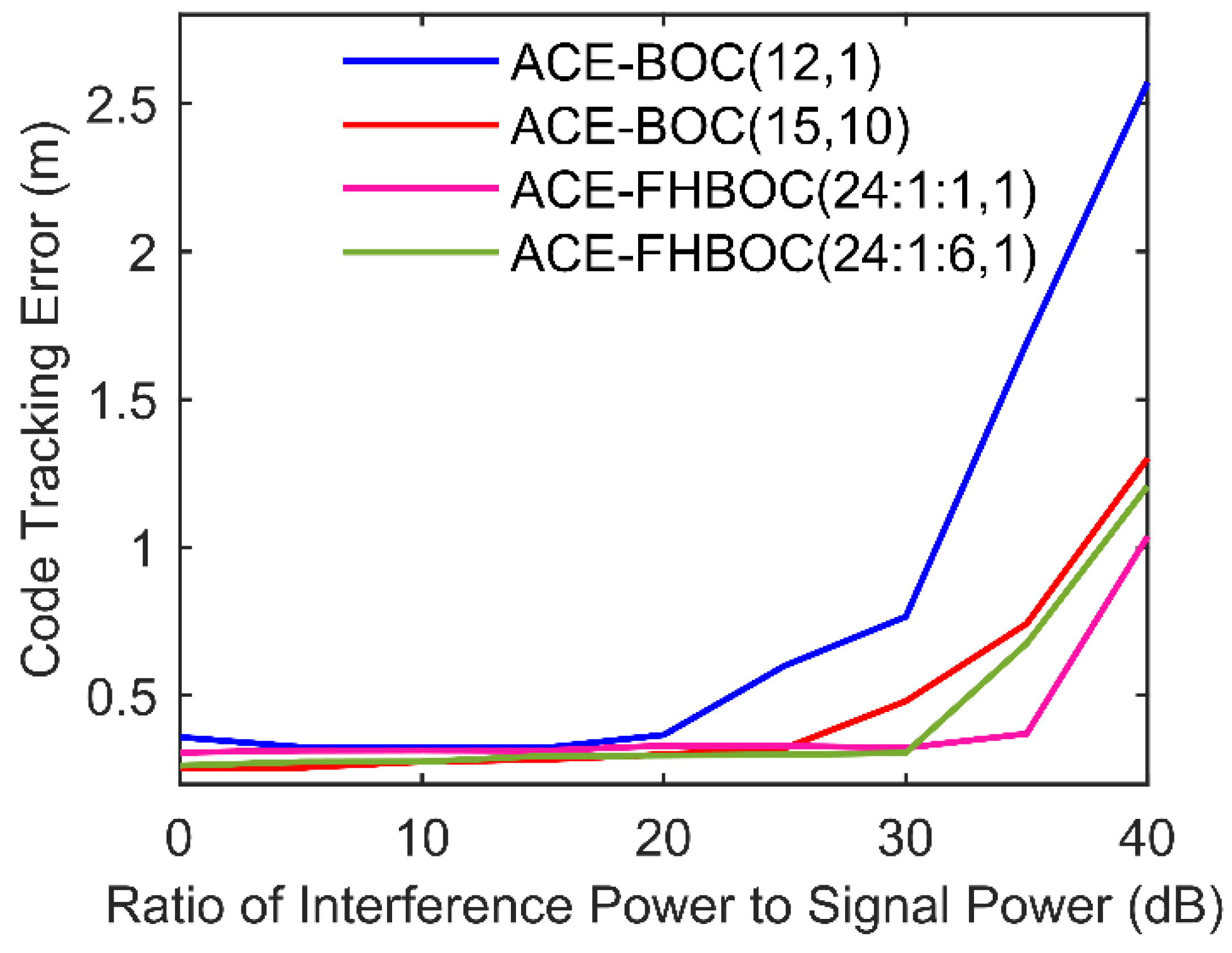
5.2.4. Anti-Narrowband Interference Performance
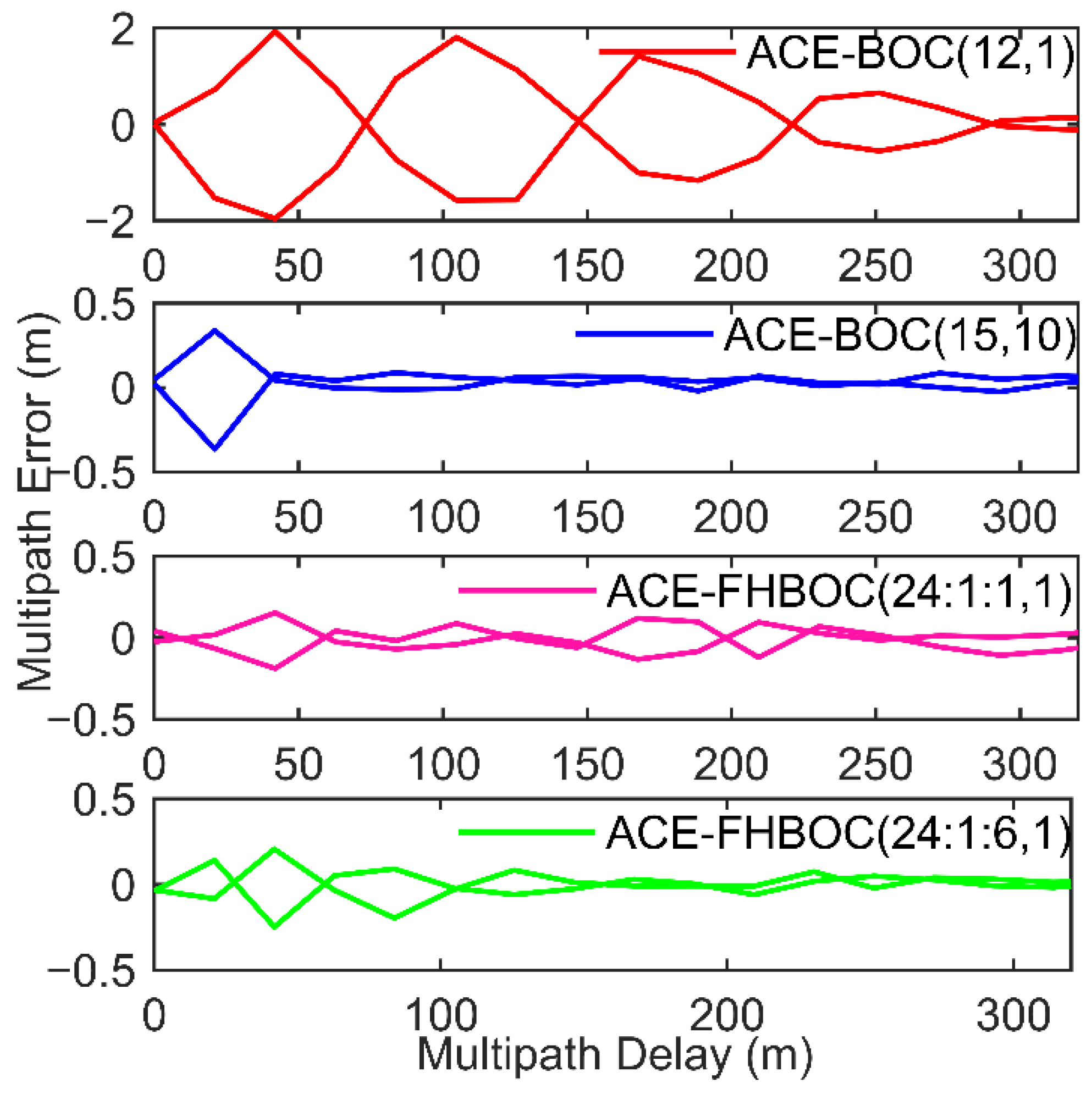
5.2.5. Multipath Performance
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
References
- Zhu, N.; Marais, J.; Bétaille, D.; Berbineau, M. GNSS position integrity in urban environments: A review of literature. IEEE Trans. Intell. Transport. Syst. 2018, 19, 2762–2778. [Google Scholar] [CrossRef]
- Causa, F.; Fasano, G. Improving navigation in GNSS-challenging environments: Multi-UAS cooperation and generalized dilution of precision. IEEE Trans. Aerosp. Electron. Syst. 2020, 57, 1462–1479. [Google Scholar] [CrossRef]
- Chiang, K.-W.; Le, D.T.; Duong, T.T.; Sun, R. The performance analysis of INS/GNSS/V-SLAM integration scheme using smartphone sensors for land vehicle navigation applications in GNSS-challenging environments. Remote Sens. 2020, 12, 1732. [Google Scholar] [CrossRef]
- Ye, L.; Yang, Y.; Jing, X.; Ma, J.; Deng, L.; Li, H. Single-Satellite Integrated Navigation Algorithm Based on Broadband LEOConstellation Communication Links. Remote Sens. 2021, 13, 703. [Google Scholar] [CrossRef]
- Ma, J.G.; Yang, Y.K.; Li, H.N.; Li, J.S. FH-BOC: Generalized low-ambiguity anti-interference spread spectrum modulation based on frequency-hopping binary offset carrier. GPS Solut. 2020, 24, 70. [Google Scholar] [CrossRef]
- Yao, Z.; Lu, M.Q. Signal multiplexing techniques for GNSS: The principle, progress, and challenges within a uniform framework. IEEE Signal Process Mag. 2017, 34, 16–26. [Google Scholar] [CrossRef]
- Xue, L.; Li, X.; Wu, W.; Dong, J. Multifunctional Signal Design for Measurement, Navigation and Communication Based on BOC and BPSK Modulation. Remote Sens. 2022, 14, 1653. [Google Scholar] [CrossRef]
- Yao, Z.; Lu, M.Q. Next-Generation GNSS Signal Design: Theories, Principles, and Technologies; Springer Nature: Berlin/Heidelberg, Germany, 2021. [Google Scholar]
- Butman, S.; Timor, U. Interplex—An efficient multichannel PSK/PM telemetry system. IEEE Trans. Commun. 1972, 20, 415–419. [Google Scholar] [CrossRef]
- Spilker, J.J.; Orr, R.S. Code multiplexing via majority logic for GPS modernization. In Proceedings of the ION GPS 1998, Institute of Navigation, Nashville, TN, USA, 15–18 September 1998; pp. 265–273. [Google Scholar]
- Dafesh, P.; Nguyen, T.; Lazar, S. Coherent adaptive subcarrier modulation (CASM) for GPS modernization. In Proceedings of the ION NTM 1999, San Diego, CA, USA, 25–27 January 1999; pp. 649–660. [Google Scholar]
- Dafesh, P.; Cahn, C. Phase-optimized constant-envelope transmission (POCET) modulation method for GNSS signals. In Proceeding of the ION GNSS 2009, Savannah, GA, USA, 22–25 September 2009; pp. 2860–2866. [Google Scholar]
- Yan, T.; Tang, Z.P.; Wei, J.L.; Qu, B.; Zhou, Z.H. A Quasi-constant Envelope Multiplexing Technique for GNSS Signals. J. Navig. 2015, 68, 791–808. [Google Scholar] [CrossRef][Green Version]
- Lestarquit, L.; Artaud, G.; Issler, J.L. AltBOC for dummies or everything you always wanted to know about AltBOC. In Proceedings of the ION GNSS 2008, Savannah, GA, USA, 16–19 September 2008; pp. 961–970. [Google Scholar]
- Tang, Z.P.; Zhou, H.; Wei, J.L.; Yan, T.; Liu, Y.Q.; Ran, Y.J.; Zhou, Y.L. TD-AltBOC: A new COMPASS B2 modulation. In Proceedings of the China Satellite Navigation Conference 2010, Shanghai, China, 18–22 October 2010. [Google Scholar]
- Zhu, L.; Yao, Z.; Lu, M.Q.; Feng, Z. Non-symmetrical AltBOC multiplexing for Compass B1 signal design. J. Tsinghua Univ. 2012, 52, 869–873. [Google Scholar]
- Zhang, K. Generalised constant-envelope DualQPSK and AltBOC modulations for modern GNSS signals. Electron. Lett. 2013, 49, 1335–1337. [Google Scholar] [CrossRef]
- Zhang, K.; Zhou, H.; Wang, F. Unbalanced AltBOC: A Compass B1 candidate with generalized MPOCET technique. GPS Solut. 2013, 17, 153–164. [Google Scholar] [CrossRef]
- Yao, Z.; Lu, M.Q. Design, Implementation, and Performance Analysis of ACE-BOC Modulation. In Proceedings of the ION GNSS+ 2013, Nashville, TN, USA, 16–20 September 2013; pp. 361–368. [Google Scholar]
- Huang, X.; Zhu, X.; Tang, X.; Gong, H.; Ou, G. GCE-BOC modulation: A generalized multiplexing technology for modern GNSS dual-frequency signals. In Proceedings of the China satellite navigation conference (CSNC) 2015, Xi’an, China, 13–15 May 2015; pp. 47–55. [Google Scholar]
- Yan, T.; Wei, J.L.; Tang, Z.P.; Zhou, Z. General AltBOC Modulation with Adjustable Power Allocation Ratio for GNSS. J. Navig. 2016, 69, 531–560. [Google Scholar] [CrossRef]
- Yao, Z.; Zhang, J.; Lu, M.Q. ACE-BOC dual-frequency constant envelope multiplexing for satellite navigation. IEEE Trans. Aerosp. Electron. Syst. 2016, 52, 466–485. [Google Scholar] [CrossRef]
- Guo, F.; Yao, Z.; Lu, M.Q. BS-ACEBOC: A generalized low-complexity dual-frequency constant-envelope multiplexing modulation for GNSS. GPS Solut. 2017, 21, 561–575. [Google Scholar] [CrossRef]
- Dafesh, P.A.; Cahn, C.R. Application of POCET Method to Combine GNSS Signals at Different Carrier Frequencies. In Proceedings of the ION ITM 2011, San Diego, CA, USA, 24–26 January 2011; pp. 1201–1206. [Google Scholar]
- Yao, Z.; Guo, F.; Ma, J.; Lu, M.Q. Orthogonality-based generalized multicarrier constant envelope multiplexing for DSSS signals. IEEE Trans. Aerosp. Electron. Syst. 2017, 53, 1685–1698. [Google Scholar] [CrossRef]
- Ma, J.; Yao, Z.; Lu, M.Q. Multicarrier Constant-Envelope Multiplexing Technique by Subcarrier Vectorization for New Generation GNSSs. IEEE Commun. Lett. 2019, 23, 991–994. [Google Scholar] [CrossRef]
- Zhang, X.; Zhang, X.; Yao, Z.; Lu, M.Q. Implementations of constant envelope multiplexing based on extended Interplex and inter-modulation construction method. In Proceedings of the ION GNSS 2012, Nashville, TN, USA, 17–21 September 2012; pp. 1201–1206. [Google Scholar]
- Xie, G. Principle of GNSS: GPA, GLONASS, and Galileo; Publishing House of Electronics Industry: Beijing, China, 2013; p. 54. [Google Scholar]
- Sousa, F.M.; Nunes, F.D. New expressions for the autocorrelation function of BOC GNSS signals. NAVIGATION J. Inst. Navig. 2013, 60, 1–9. [Google Scholar] [CrossRef]
- Borre, K.; Akos, D.M.; Bertelsen, N.; Rinder, P.; Jensen, S.H. A Software-Defined GPS and Galileo Receiver: A Single-Frequency Approach; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Betz, J.W.; Kolodziejski, K.R. Generalized theory of code tracking with an early-late discriminator part II: Noncoherent processing and numerical results. IEEE Trans. Aerosp. Electron. Syst. 2009, 45, 1557–1564. [Google Scholar] [CrossRef]
- Zhang, J.; Cui, X.; Xu, H.; Lu, M. A Two-Stage Interference Suppression Scheme Based on Antenna Array for GNSS Jamming and Spoofing. Sensors 2019, 19, 3870. [Google Scholar] [CrossRef]
- Qin, W.; Gamba, M.T.; Falletti, E.; Dovis, F. An assessment of impact of adaptive notch filters for interference removal on the signal processing stages of a GNSS receiver. IEEE Trans. Aerosp. Electron. Syst. 2020, 56, 4067–4082. [Google Scholar] [CrossRef]
- Huang, L.; Lu, Z.; Xiao, Z.; Ren, C.; Song, J.; Li, B. Suppression of Jammer Multipath in GNSS Antenna Array Receiver. Remote Sens. 2022, 14, 350. [Google Scholar] [CrossRef]
- Hein, G.W.; Avila-Rodriguez, J.-A.; Wallner, S.; Pratt, A.R.; Owen, J.; Issler, J.; Betz, J.W.; Hegarty, C.J.; Lenahan, S.; Rushanan, J.J.; et al. MBOC: The new optimized spreading modulation recommended for GALILEO L1 O.S. and GPS L1C. In Proceedings of the IEEE/ION PLANS 2006, San Diego, CA, USA, 25–27 April 2006; pp. 883–892. [Google Scholar]



| 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | −1 | −1 | −1 | −1 | −1 | −1 | −1 | −1 | |
| 1 | 1 | 1 | 1 | −1 | −1 | −1 | −1 | 1 | 1 | 1 | 1 | −1 | −1 | −1 | −1 | |
| 1 | 1 | −1 | −1 | 1 | 1 | −1 | −1 | 1 | 1 | −1 | −1 | 1 | 1 | −1 | −1 | |
| 1 | −1 | 1 | −1 | 1 | −1 | 1 | −1 | 1 | −1 | 1 | −1 | 1 | −1 | 1 | −1 | |
| 2 | 12 | 12 | 10 | 3 | 5 | 1 | 9 | 3 | 7 | 11 | 9 | 4 | 6 | 6 | 8 | |
| 2 | 6 | 12 | 10 | 3 | 5 | 1 | 9 | 3 | 7 | 11 | 9 | 4 | 6 | 12 | 8 | |
| 2 | 6 | 12 | 10 | 3 | 5 | 1 | 3 | 9 | 7 | 11 | 9 | 4 | 6 | 12 | 8 | |
| 8 | 6 | 12 | 4 | 3 | 5 | 1 | 3 | 9 | 7 | 11 | 9 | 10 | 6 | 12 | 2 | |
| 8 | 6 | 12 | 4 | 3 | 5 | 1 | 3 | 9 | 7 | 11 | 9 | 10 | 6 | 12 | 2 | |
| 8 | 6 | 6 | 4 | 9 | 5 | 1 | 3 | 9 | 7 | 11 | 3 | 10 | 12 | 12 | 2 | |
| 8 | 6 | 6 | 4 | 9 | 11 | 7 | 3 | 9 | 1 | 5 | 3 | 10 | 12 | 12 | 2 | |
| 8 | 12 | 6 | 4 | 9 | 11 | 7 | 3 | 9 | 1 | 5 | 3 | 10 | 12 | 6 | 2 | |
| 8 | 12 | 6 | 4 | 9 | 11 | 7 | 9 | 3 | 1 | 5 | 3 | 10 | 12 | 6 | 2 | |
| 8 | 12 | 6 | 4 | 9 | 11 | 7 | 9 | 3 | 1 | 5 | 3 | 10 | 12 | 6 | 2 | |
| 2 | 12 | 6 | 10 | 3 | 11 | 7 | 9 | 3 | 1 | 5 | 9 | 4 | 12 | 6 | 8 | |
| 2 | 12 | 12 | 10 | 3 | 11 | 7 | 9 | 3 | 1 | 5 | 9 | 4 | 6 | 6 | 8 | |
| Resource Consumption | ACE-FHBOC | ACE-BOC |
|---|---|---|
| Minimum Subchip Rate | ||
| Minimum number of Bits of Direct Subcarriers Generator | 3 | 3 |
| LUT of Phase Rotation Generation Method | LUT | LUT |
| (dB/Hz) | (12,1) | (15,10) | (24:1:1,1) | (24:1:6,1) |
|---|---|---|---|---|
| BPSK(1) | −92.2 | −83.9 | −82.4 | −92.0 |
| BPSK(10) | −86.5 | −84.2 | −78.3 | −84.7 |
| MBOC(6,1,1/11) | −92.2 | −84.1 | −78.5 | −86.1 |
| BOC(10,5) | −76.1 | −79.4 | −78.4 | −78.1 |
| Self | −66.7 | −76.6 | −78.6 | −78.0 |
| Parameters | Value |
|---|---|
| Signal Power Ratio | [1,1,3,3] |
| Data Rate | 50 bit/s |
| Length of Spreading Code | 10230 |
| Length of Frequency-hopping Pattern | 10230 |
| Sampling Frequency | 286.44 MHz |
| Intermediate Frequency | 71.61 MHz |
| Filter Bandwidth | 50 MHz |
| Simulated Signal Duration | 100 ms |
| Data Type of Sampled Signal | Eight bit signed char values |
| Parameters | (24:1:1,1) | (24:1:6,1) | (15,10) | (12,1) |
|---|---|---|---|---|
| (dB) | 59.9 | 58.9 | 56.1 | 46.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, J.; Yang, Y.; Ye, L.; Deng, L.; Li, H. Dual-Sideband Constant-Envelope Frequency-Hopping Binary Offset Carrier Multiplexing Modulation for Satellite Navigation. Remote Sens. 2022, 14, 3871. https://doi.org/10.3390/rs14163871
Ma J, Yang Y, Ye L, Deng L, Li H. Dual-Sideband Constant-Envelope Frequency-Hopping Binary Offset Carrier Multiplexing Modulation for Satellite Navigation. Remote Sensing. 2022; 14(16):3871. https://doi.org/10.3390/rs14163871
Chicago/Turabian StyleMa, Jiangang, Yikang Yang, Lvyang Ye, Lingyu Deng, and Hengnian Li. 2022. "Dual-Sideband Constant-Envelope Frequency-Hopping Binary Offset Carrier Multiplexing Modulation for Satellite Navigation" Remote Sensing 14, no. 16: 3871. https://doi.org/10.3390/rs14163871
APA StyleMa, J., Yang, Y., Ye, L., Deng, L., & Li, H. (2022). Dual-Sideband Constant-Envelope Frequency-Hopping Binary Offset Carrier Multiplexing Modulation for Satellite Navigation. Remote Sensing, 14(16), 3871. https://doi.org/10.3390/rs14163871






