Investigating the Magnetotelluric Responses in Electrical Anisotropic Media
Abstract
1. Introduction
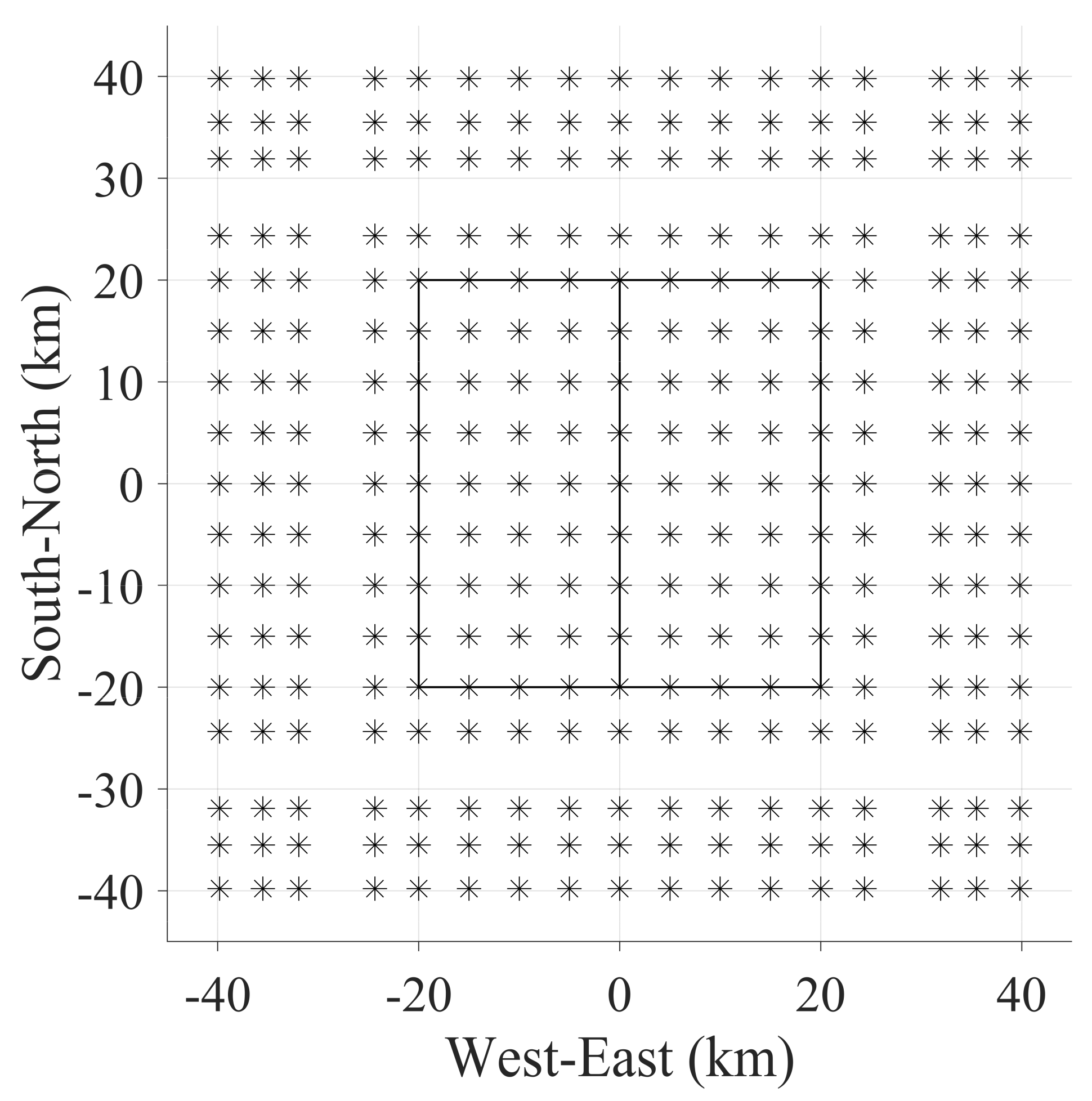
2. Methods
3. Synthetic Examples
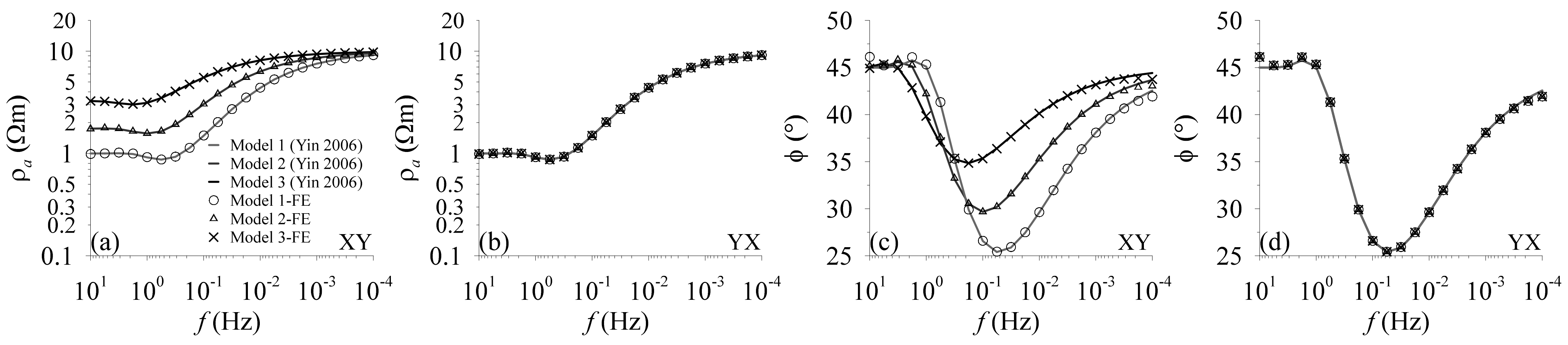
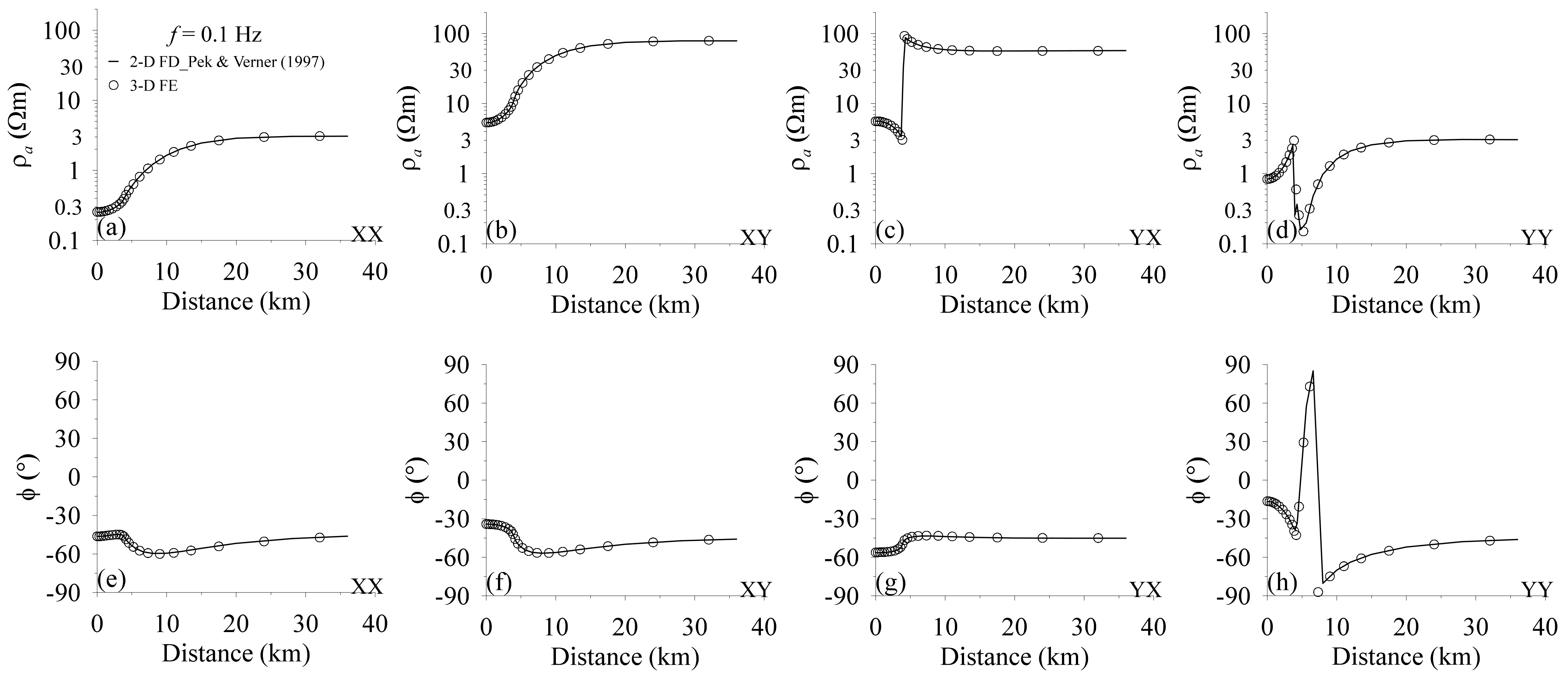
3.1. Verification Models
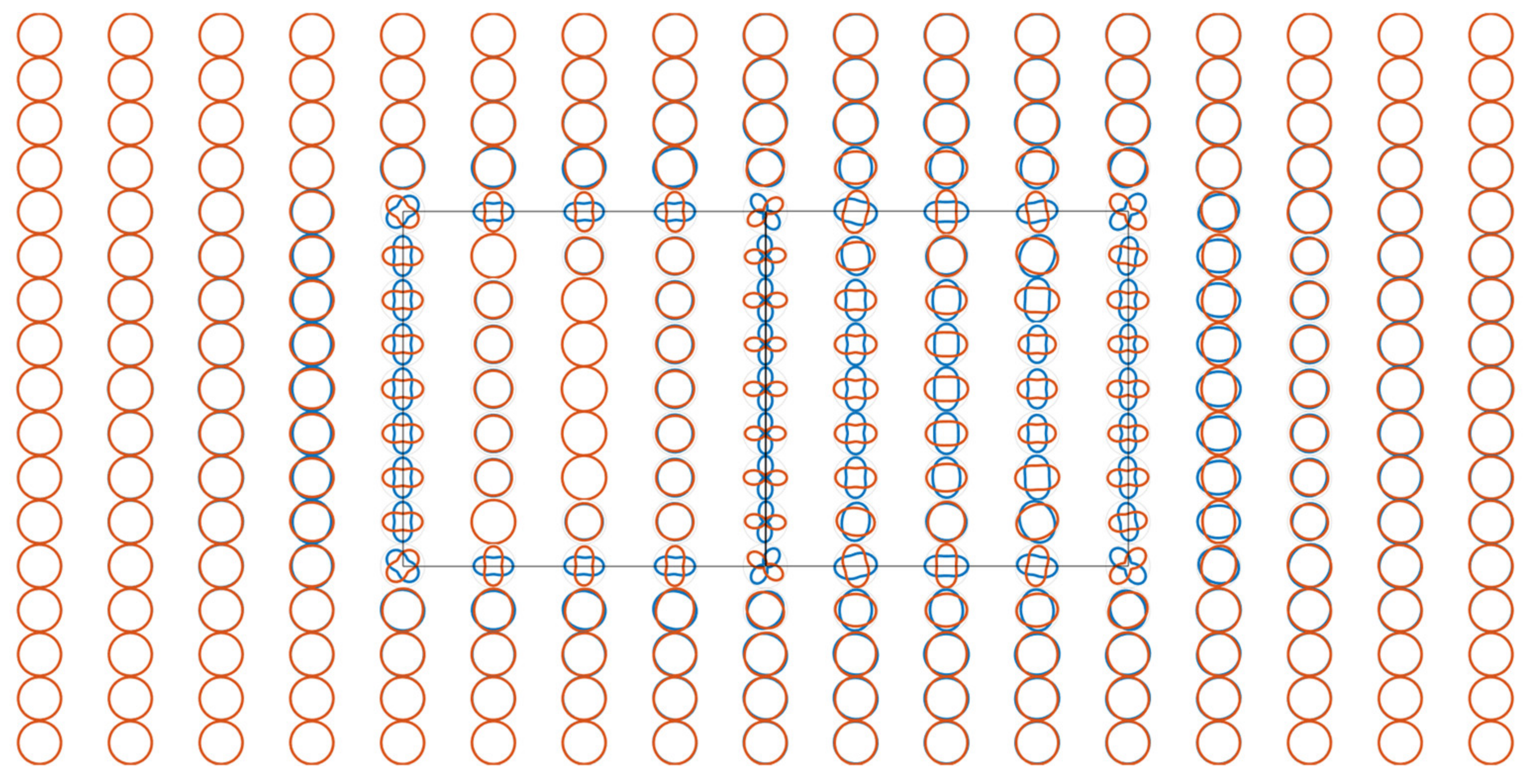
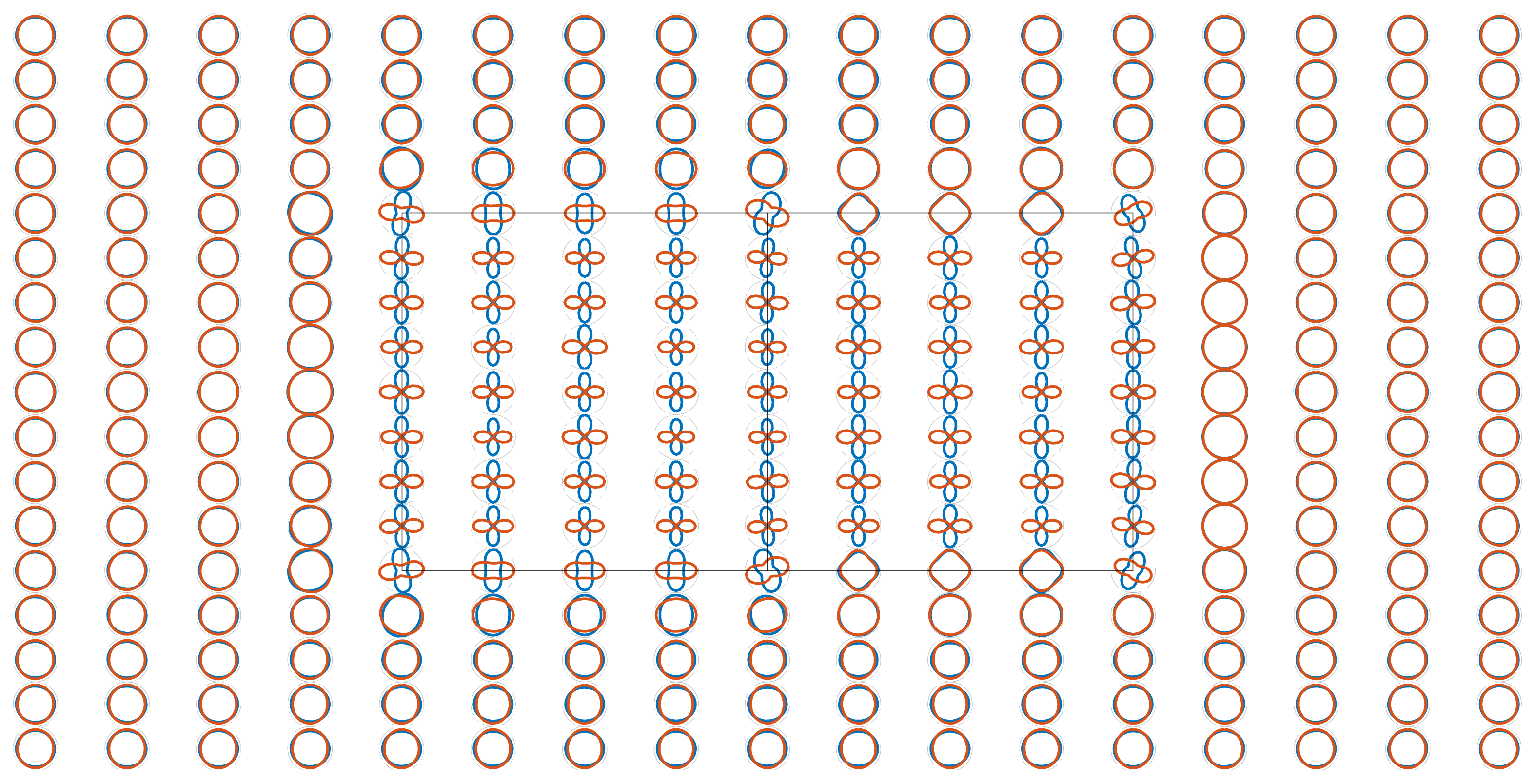
3.2. Identification of Electrical Anisotropy
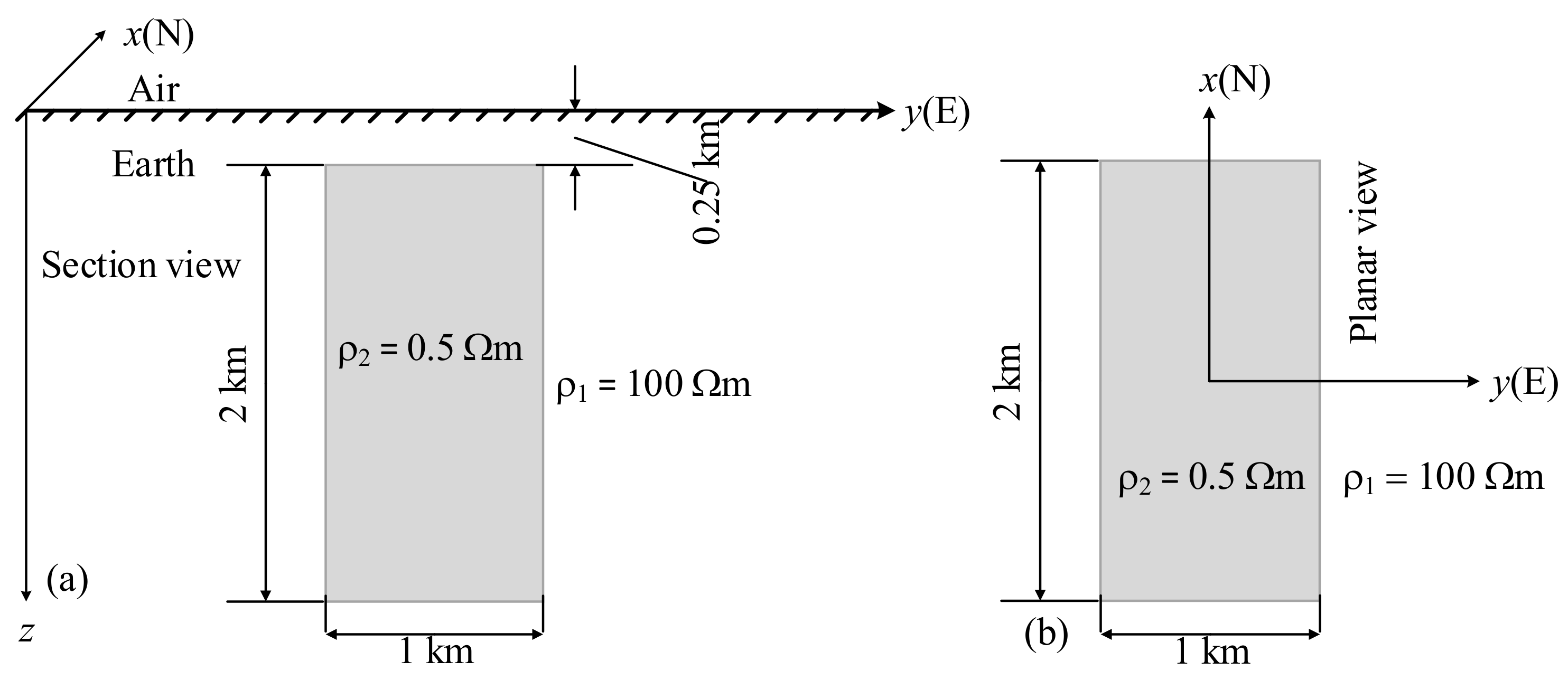
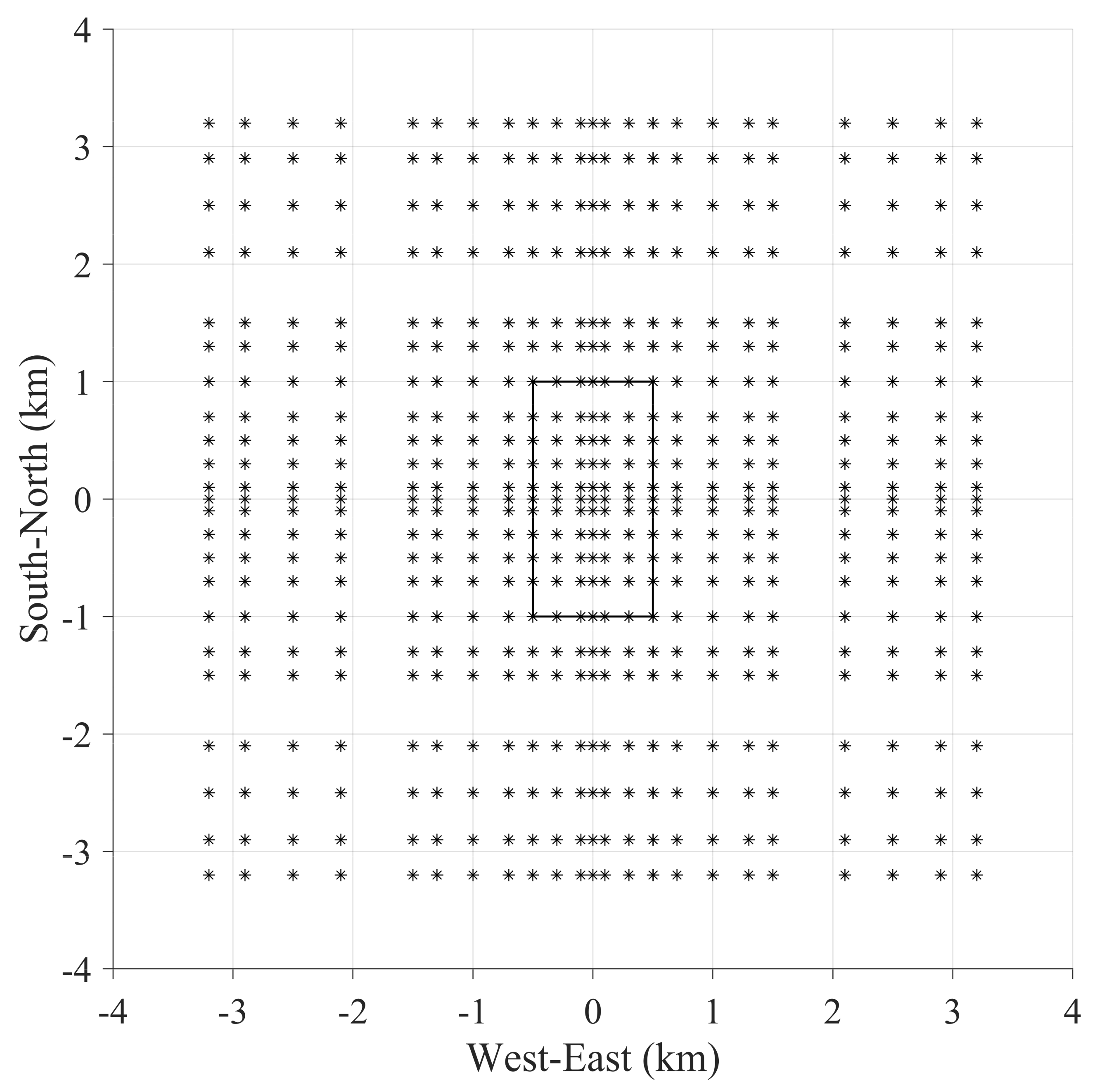
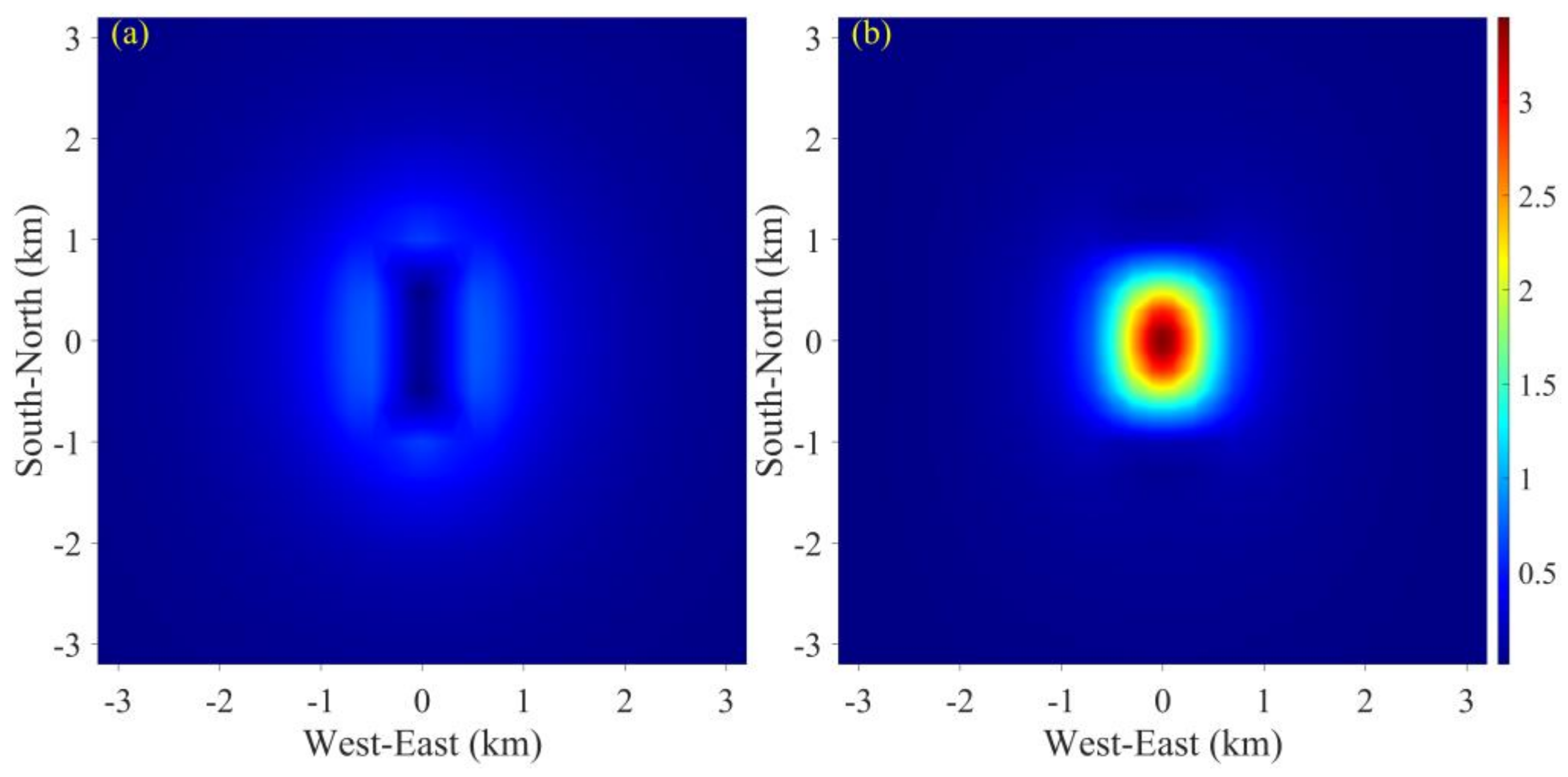
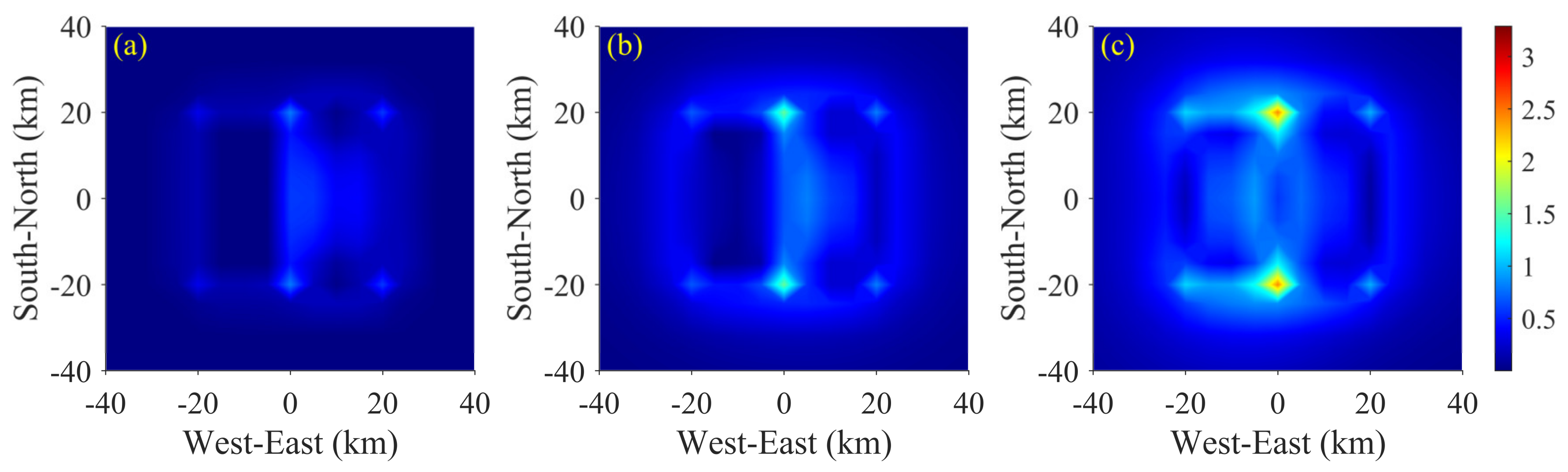
3.2.1. COMMEMI 3D-1A Model
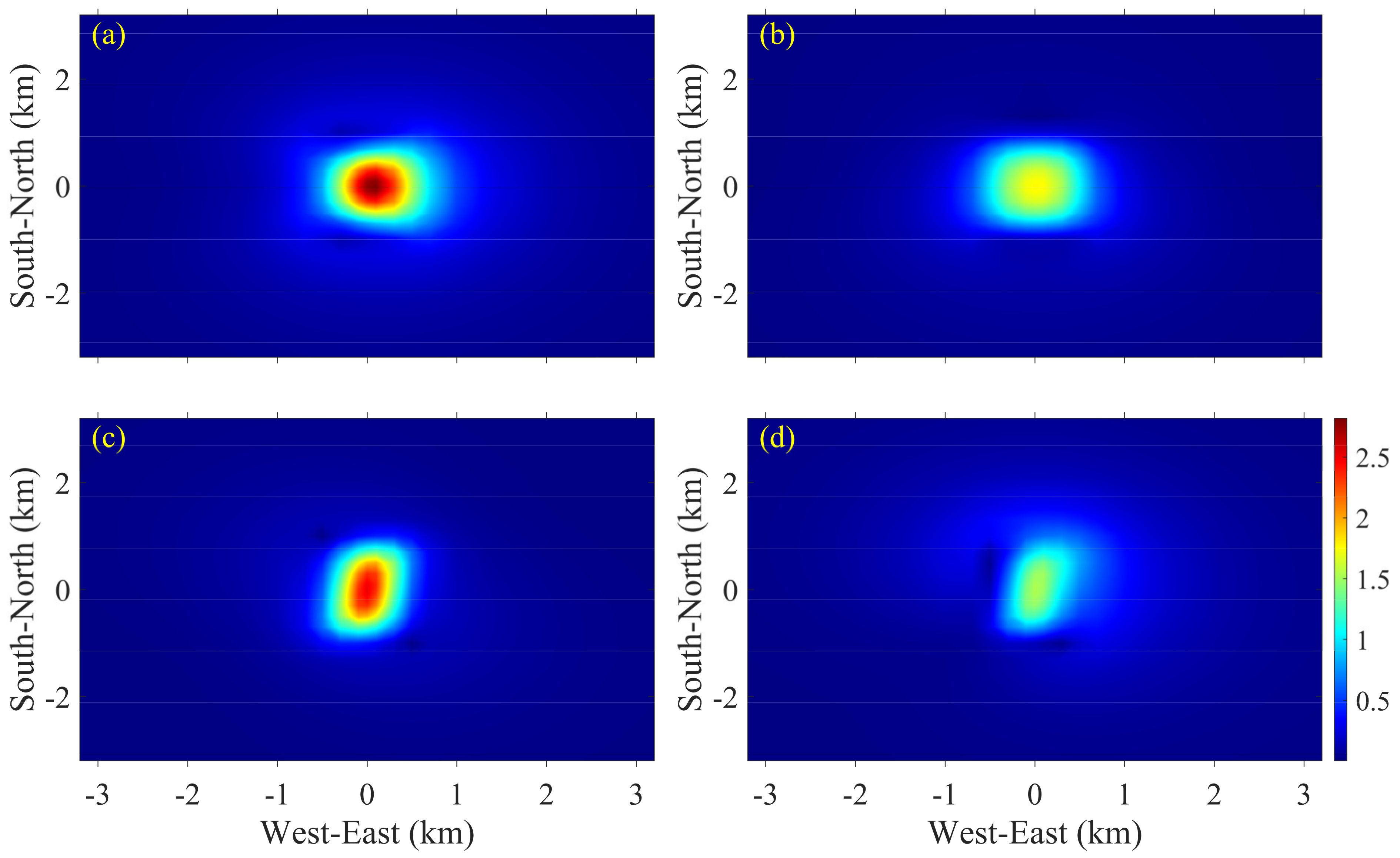
3.2.2. COMMEMI 3D-2A Model
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Martí, A.; Queralt, P.; Ledo, J.; Farquharson, C. Dimensionality imprint of electrical anisotropy in magnetotelluric responses. Phys. Earth Planet. Inter. 2010, 182, 139–151. [Google Scholar] [CrossRef]
- Wannamaker, P.E. Anisotropy Versus Heterogeneity in Continental Solid Earth Electromagnetic Studies: Fundamental Response Characteristics and Implications for Physicochemical State. Surv. Geophys. 2005, 26, 733–765. [Google Scholar] [CrossRef]
- Martí, A. The Role of Electrical Anisotropy in Magnetotelluric Responses: From Modelling and Dimensionality Analysis to Inversion and Interpretation. Surv. Geophys. 2014, 35, 179–218. [Google Scholar] [CrossRef]
- Eaton, D.W.; Jones, A. Tectonic fabric of the subcontinental lithosphere: Evidence from seismic, magnetotelluric and mechanical anisotropy. Phys. Earth Planet. Inter. 2006, 158, 85–91. [Google Scholar] [CrossRef]
- Adetunji, A.Q.; Ferguson, I.J.; Jones, A.G. Reexamination of magnetotelluric responses and electrical anisotropy of the lithospheric mantle in the Grenville Province, Canada. J. Geophys. Res. Solid Earth 2015, 120, 1890–1908. [Google Scholar] [CrossRef]
- Jones, A.G. Electromagnetic interrogation of the anisotropic Earth: Looking into the Earth with polarized spectacles. Phys. Earth Planet. Inter. 2006, 158, 281–291. [Google Scholar] [CrossRef]
- Jones, A.G. Distortion decomposition of the magnetotelluric impedance tensors from a one-dimensional anisotropic Earth. Geophys. J. Int. 2012, 189, 268–284. [Google Scholar] [CrossRef]
- Heise, W.; Pous, J. Anomalous phases exceeding 90° in magnetotellurics: Anisotropic model studies and a field example. Geophys. J. Int. 2003, 155, 308–318. [Google Scholar] [CrossRef]
- Yin, C.C. MMT forward modeling for a layered earth with arbitrary anisotropy. Geophysics 2006, 71, G115–G128. [Google Scholar] [CrossRef]
- Pek, J.; Santos, F.A.M. Magnetotelluric impedances and parametric sensitivities for 1-D anisotropic layered media. Comput. Geosci. 2002, 28, 939–950. [Google Scholar] [CrossRef]
- Dekker, D.L.; Hastie, L.M. Magneto-telluric impedances of an anisotropic layered Earth model. Geophys. J. Int. 1980, 61, 11–20. [Google Scholar] [CrossRef][Green Version]
- Okazaki, T.; Oshiman, N.; Yoshimura, R. Analytical investigations of the magnetotelluric directionality estimation in 1-D anisotropic layered media. Phys. Earth Planet. Inter. 2016, 260, 25–31. [Google Scholar] [CrossRef]
- Osella, A.M.; Martinelli, P. Magnetotelluric response of anisotropic 2-D structures. Geophys. J. Int. 1993, 115, 819–828. [Google Scholar] [CrossRef]
- Martinelli, P.; Osella, A. MT Forward Modeling of 3-D Anisotropic Electrical Conductivity Structures Using the Rayleigh-Fourier Method. J. Geomagn. Geoelectr. 1997, 49, 1499–1518. [Google Scholar] [CrossRef]
- Pek, J.; Verner, T. Finite-difference modelling of magnetotelluric fields in two-dimensional anisotropic media. Geophys. J. Int. 1997, 128, 505–521. [Google Scholar] [CrossRef]
- Hu, X.Y.; Huo, G.P.; Gao, R.; Wang, H.Y.; Huang, Y.F.; Zhang, Y.X.; Zuo, B.X.; Cai, J.C. The magnetotelluric anisotropic two-dimensional simulation and case analysis. Chin. J. Geophys.-Chin. Ed. 2013, 56, 4268–4277. [Google Scholar] [CrossRef]
- Han, B.; Li, Y.; Li, G. 3D forward modeling of magnetotelluric fields in general anisotropic media and its numerical implementation in Julia. Geophysics 2018, 83, F29–F40. [Google Scholar] [CrossRef]
- Castillo-Reyes, O.; de la Puente, J.; García-Castillo, L.E.; Cela, J.M. Parallel 3-D marine controlled-source electromagnetic modelling using high-order tetrahedral Nédélec elements. Geophys. J. Int. 2019, 219, 39–65. [Google Scholar] [CrossRef]
- Castillo-Reyes, O.; de la Puente, J.; Cela, J.M. PETGEM: A parallel code for 3D CSEM forward modeling using edge finite elements. Comput. Geosci. 2018, 119, 123–136. [Google Scholar] [CrossRef]
- Reddy, I.K.; Rankin, D. Magnetotelluric response of laterally inhomogeneous and anisotropic media. Geophysics 1975, 40, 1035–1045. [Google Scholar] [CrossRef]
- Xiao, T.; Liu, Y.; Wang, Y.; Fu, L.-Y. Three-dimensional magnetotelluric modeling in anisotropic media using edge-based finite element method. J. Appl. Geophys. 2018, 149, 1–9. [Google Scholar] [CrossRef]
- Cai, H.; Xiong, B.; Han, M.; Zhdanov, M. 3D controlled-source electromagnetic modeling in anisotropic medium using edge-based finite element method. Comput. Geosci. 2014, 73, 164–176. [Google Scholar] [CrossRef]
- Li, Y.; Dai, S. Finite element modelling of marine controlled-source electromagnetic responses in two-dimensional dipping anisotropic conductivity structures. Geophys. J. Int. 2011, 185, 622–636. [Google Scholar] [CrossRef][Green Version]
- Li, Y. A finite-element algorithm for electromagnetic induction in two-dimensional anisotropic conductivity structures. Geophys. J. Int. 2002, 148, 389–401. [Google Scholar] [CrossRef]
- Key, K. MARE2DEM: A 2-D inversion code for controlled-source electromagnetic and magnetotelluric data. Geophys. J. Int. 2016, 207, 571–588. [Google Scholar] [CrossRef]
- Li, Y.; Pek, J. Adaptive finite element modelling of two-dimensional magnetotelluric fields in general anisotropic media. Geophys. J. Int. 2008, 175, 942–954. [Google Scholar] [CrossRef]
- Qin, L.; Yang, C. Analytic magnetotelluric responses to a two-segment model with axially anisotropic conductivity structures overlying a perfect conductor. Geophys. J. Int. 2016, 205, 1729–1739. [Google Scholar] [CrossRef]
- Weaver, J.T.; Agarwal, A.K.; Lilley, F.E.M. Characterization of the magnetotelluric tensor in terms of its invariants. Geophys. J. Int. 2000, 141, 321–336. [Google Scholar] [CrossRef]
- Martí, A.; Queralt, P.; Ledo, J. WALDIM: A code for the dimensionality analysis of magnetotelluric data using the rotational invariants of the magnetotelluric tensor. Comput. Geosci. 2009, 35, 2295–2303. [Google Scholar] [CrossRef]
- Lilley, F.E.M. Magnetotelluric analysis using Mohr circles. Geophysics 1993, 58, 1498–1506. [Google Scholar] [CrossRef][Green Version]
- Cao, X.Y.; Yin, C.C.; Zhang, B.; Huang, X.; Liu, Y.H.; Cai, J. A goal-oriented adaptive finite-element method for 3D MT anisotropic modeling with topography. Chin. J. Geophys.-Chin. Ed. 2018, 61, 2618–2628. [Google Scholar] [CrossRef]
- Taylor, R.W.; Fleming, A.H. Characterizing Jointed Systems by Azimuthal Resistivity Surveys. Groundwater 1988, 26, 464–474. [Google Scholar] [CrossRef]
- Ritzi, R.W.; Andolsek, R.H. Relation Between Anisotropic Transmissivity and Azimuthal Resistivity Surveys in Shallow, Fractured, Carbonate Flow Systems. Groundwater 1992, 30, 774–780. [Google Scholar] [CrossRef]
- Busby, J.P. The effectiveness of azimuthal apparent-resistivity measurements as a method for determining fracture strike orientations. Geophys. Prospect. 2000, 48, 677–695. [Google Scholar] [CrossRef]
- Wishart, D.N.; Slater, L.D.; Gates, A.E. Fracture anisotropy characterization in crystalline bedrock using field-scale azimuthal self potential gradient. J. Hydrol. 2008, 358, 35–45. [Google Scholar] [CrossRef]
- Yang, C.-f.; Qin, L.-j. Graphical Representation and Explanation of the Conductivity Tensor of Anisotropic Media. Surv. Geophys. 2020, 41, 249–281. [Google Scholar] [CrossRef]
- Farquharson, C.G.; Miensopust, M.P. Three-dimensional finite-element modelling of magnetotelluric data with a divergence correction. J. Appl. Geophys. 2011, 75, 699–710. [Google Scholar] [CrossRef]
- Rivera-Rios, A.M.; Zhou, B.; Heinson, G.; Krieger, L. Multi-order vector finite element modeling of 3D magnetotelluric data including complex geometry and anisotropy. Earth Planets Space 2019, 71, 92. [Google Scholar] [CrossRef]
- Yin, C.C. Geoelectrical inversion for a one-dimensional anisotropic model and inherent non-uniqueness. Geophys. J. Int. 2000, 140, 11–23. [Google Scholar] [CrossRef]
- Xu, S.Z. Finite Element Method in Geophysics, 1st ed.; Science Press: Beijing, China, 1994. [Google Scholar]
- Jin, J.-M. The Finite Element Method in Electromagnetics, 2nd ed.; John Wiley & Sons: New York, NY, USA, 2002. [Google Scholar]
- Saad, Y. Iterative Methods for Sparse Linear Systems, Second Edition, 2nd ed.; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 2003; p. 528. [Google Scholar]
- Zhdanov, M.S.; Varentsov, I.M.; Weaver, J.T.; Golubev, N.G.; Krylov, V.A. Methods for modelling electromagnetic fields Results from COMMEMI—The international project on the comparison of modelling methods for electromagnetic induction. J. Appl. Geophys. 1997, 37, 133–271. [Google Scholar] [CrossRef]
- Luo, T.; Hu, X.; Chen, L.; Xu, G. Investigating the Magnetotelluric Responses in Electrical Anisotropic Media. 2022. Available online: https://figshare.com/articles/dataset/Investigating_the_magnetotelluric_responses_in_electrical_aniso-tropic_media/19682199 (accessed on 29 April 2022).



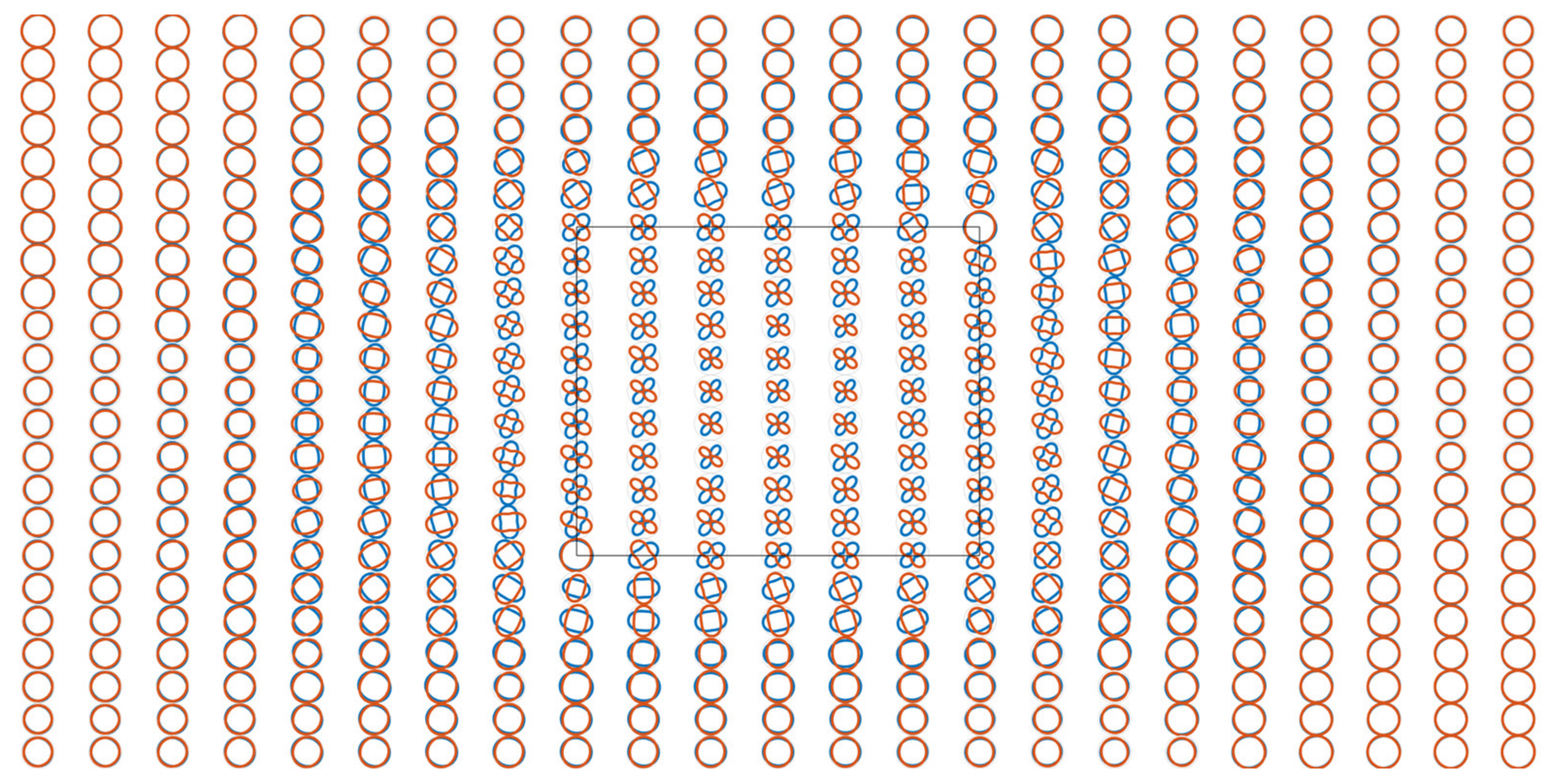
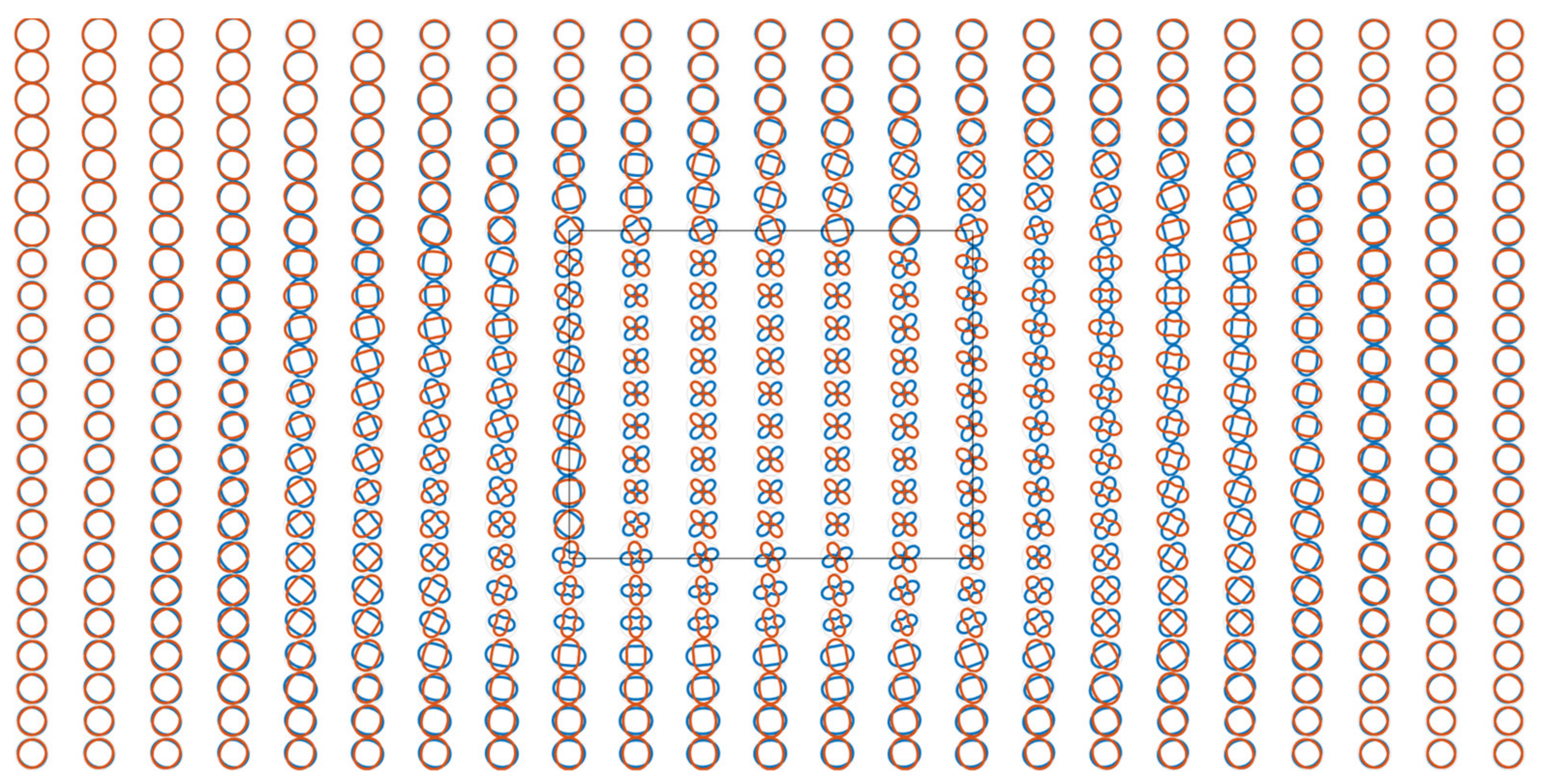


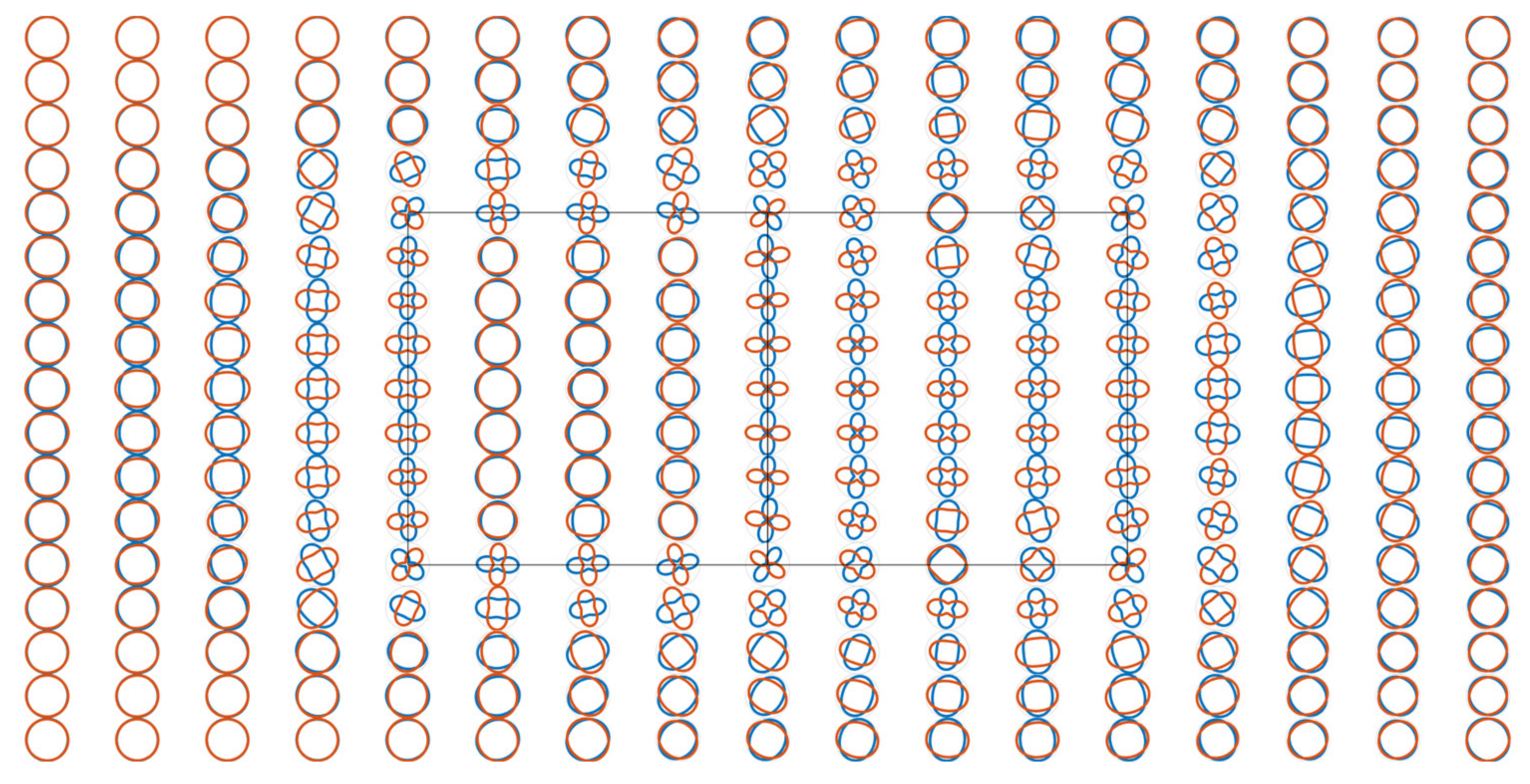
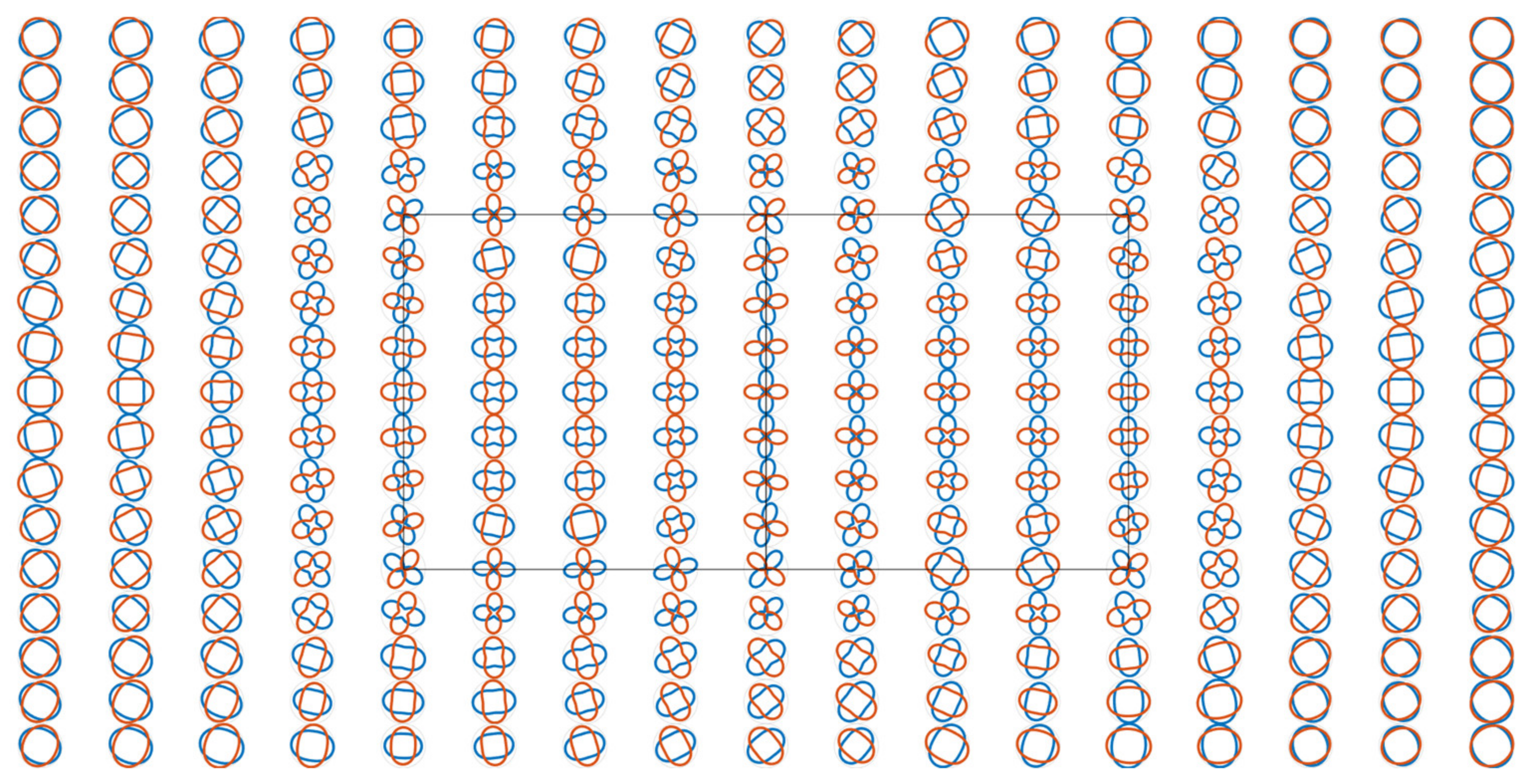

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luo, T.; Hu, X.; Chen, L.; Xu, G. Investigating the Magnetotelluric Responses in Electrical Anisotropic Media. Remote Sens. 2022, 14, 2328. https://doi.org/10.3390/rs14102328
Luo T, Hu X, Chen L, Xu G. Investigating the Magnetotelluric Responses in Electrical Anisotropic Media. Remote Sensing. 2022; 14(10):2328. https://doi.org/10.3390/rs14102328
Chicago/Turabian StyleLuo, Tianya, Xiangyun Hu, Longwei Chen, and Guilin Xu. 2022. "Investigating the Magnetotelluric Responses in Electrical Anisotropic Media" Remote Sensing 14, no. 10: 2328. https://doi.org/10.3390/rs14102328
APA StyleLuo, T., Hu, X., Chen, L., & Xu, G. (2022). Investigating the Magnetotelluric Responses in Electrical Anisotropic Media. Remote Sensing, 14(10), 2328. https://doi.org/10.3390/rs14102328





