From 1/4° to 1/8°: Influence of Spatial Resolution on Eddy Detection Using Altimeter Data
Abstract
1. Introduction
2. Materials and Methods
2.1. SLA Data
2.2. Methods
2.2.1. Eddy Identification
- They contain no more than one “seed” (local minimum/maximum);
- 9 pixels ≤ eddy size ≤ 2000 pixels (endure mesoscale);
- Eddy shape test within an error threshold of 55%;
- Eddy amplitude (A) ≥ 0.25 cm can be described by Equation (1).
2.2.2. Eddy Tracking
2.2.3. Eddy Matching
- Situation A: There is a 1/8° eddy in a 1/4° eddy, and we call these eddies “correct eddies”;
- Situation B: There is no 1/8° eddy in a 1/4° eddy, we call the situation a “redundant” one;
- Situation C: There are multiple (usually two) 1/8° eddies in one 1/4° eddy. These are called “confused eddies”;
- Situation D: There is no 1/4° eddy matched with a 1/8° eddy. This is referred to as “missed”.
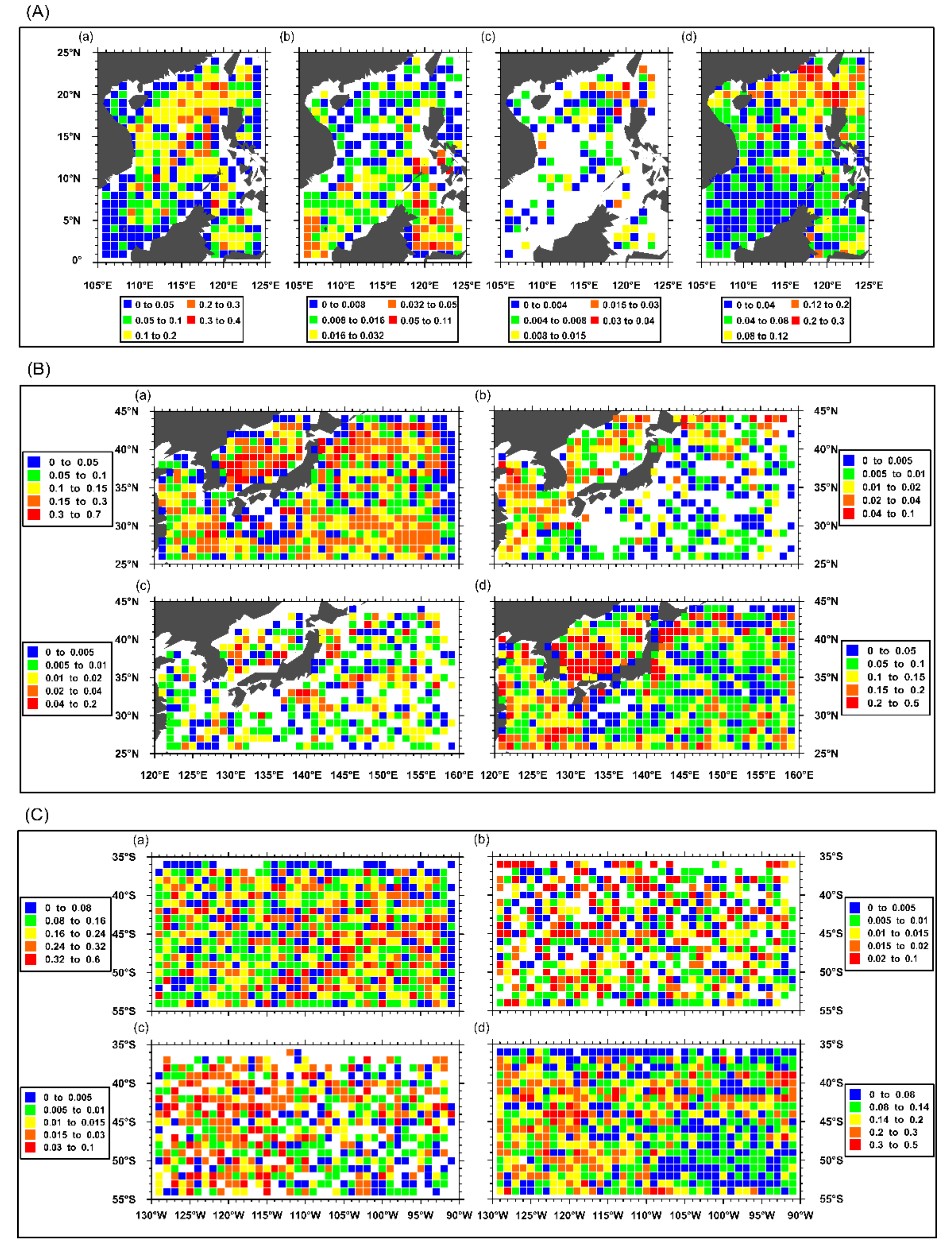
3. Results
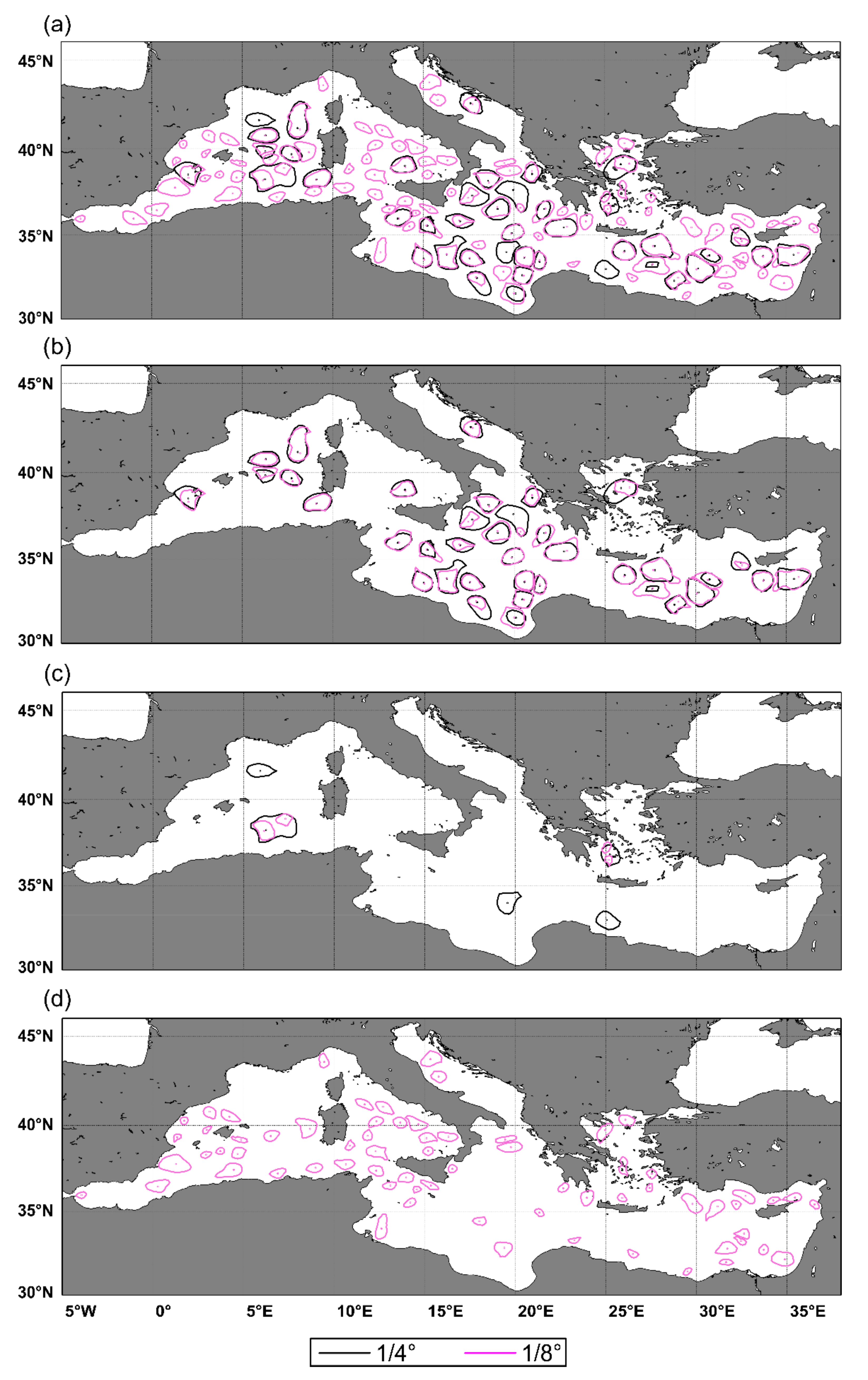
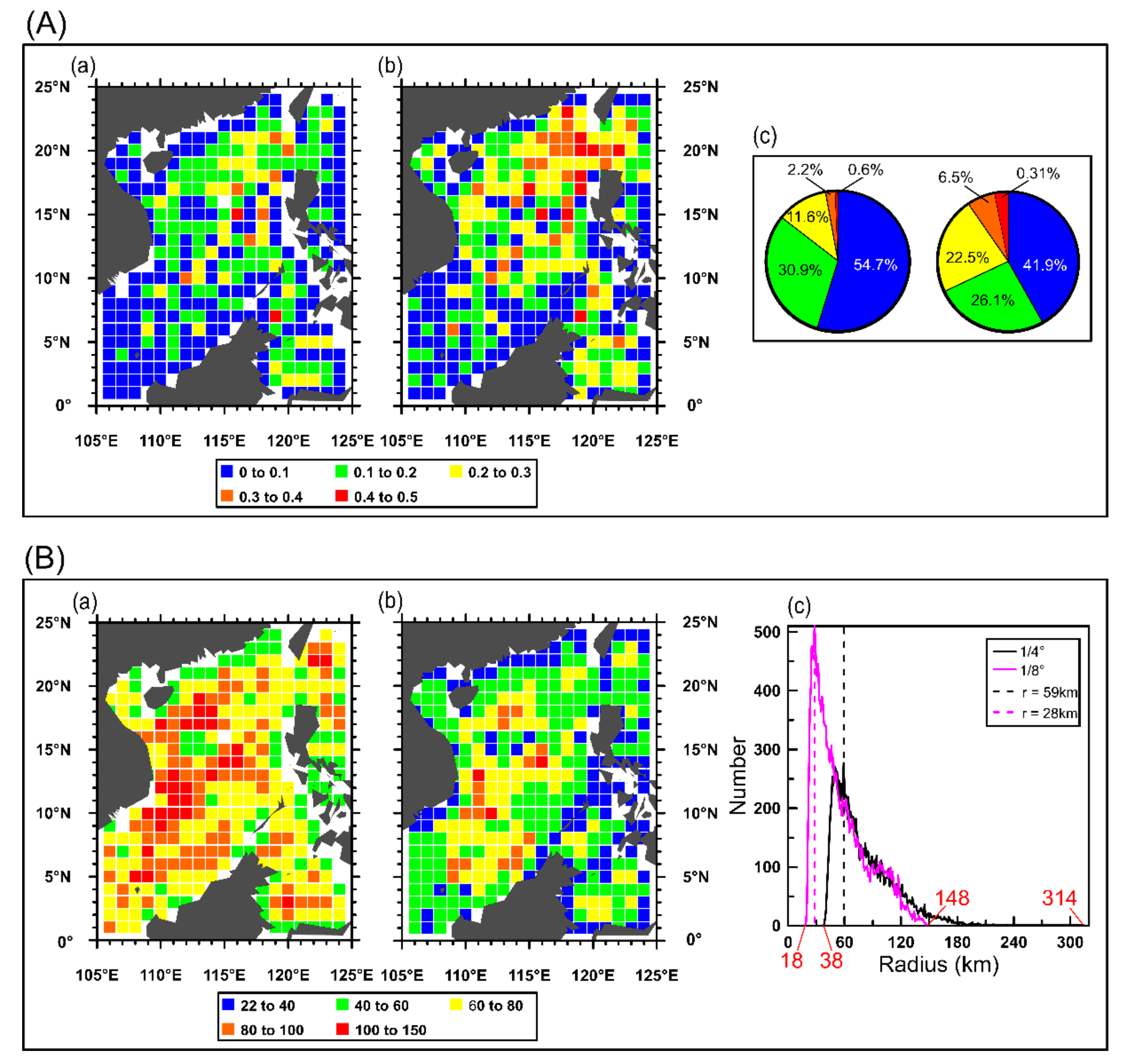
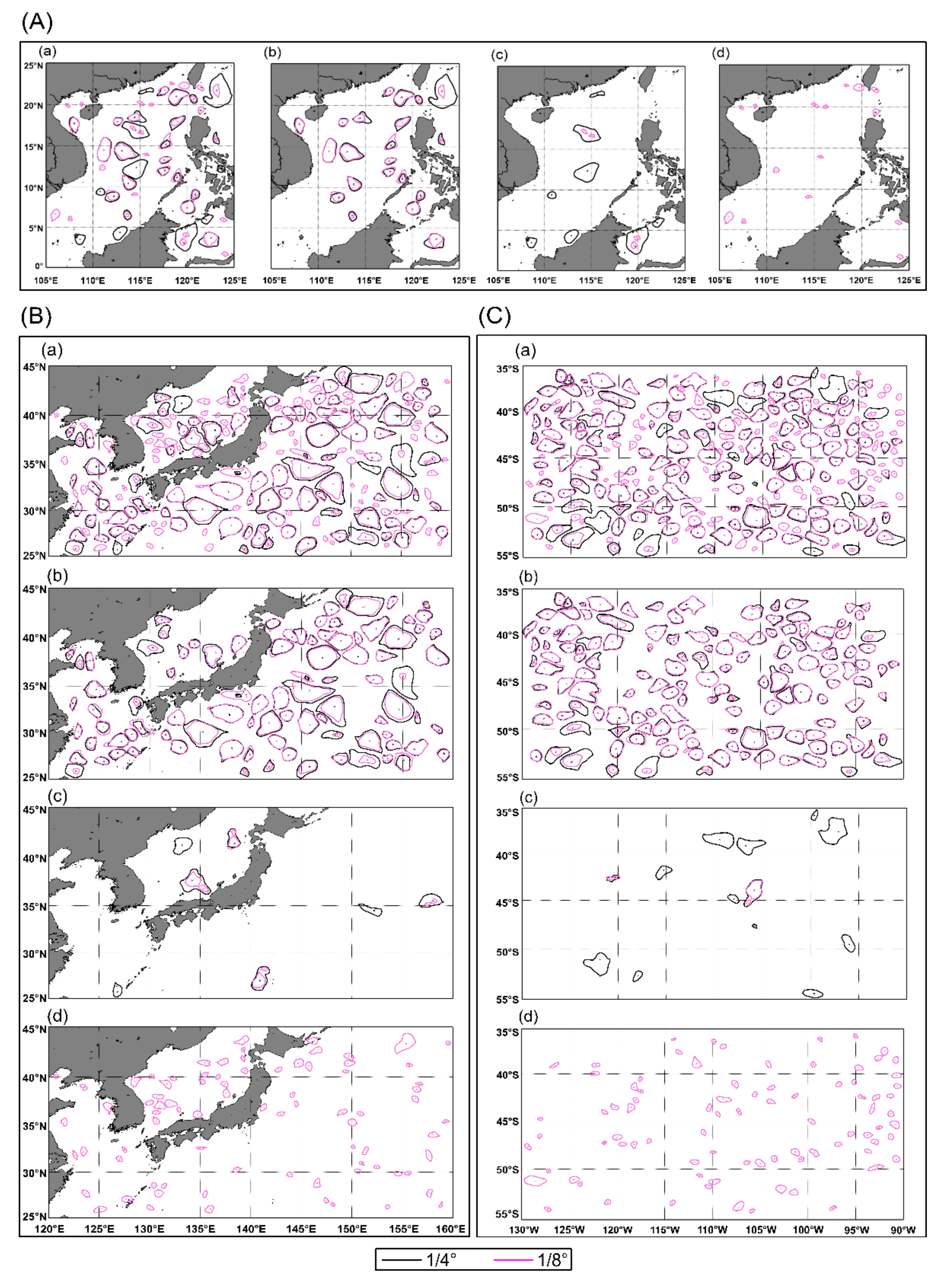
3.1. Eddy Identification in the Mediterranean Sea
3.2. Eddy Tracking in the Mediterranean Sea
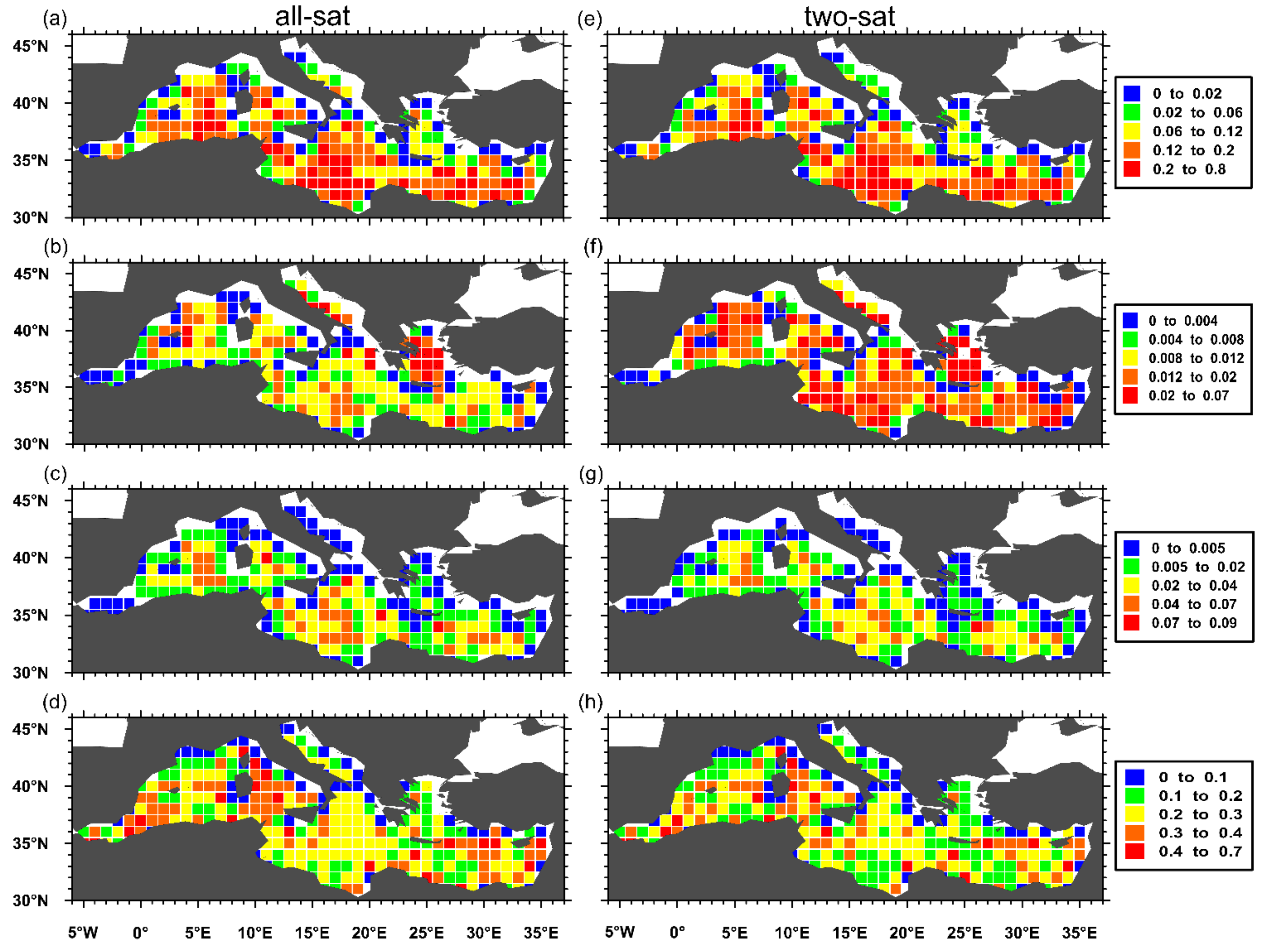
3.3. Eddy Matching in the Mediterranean Sea
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chelton, D.B.; Schlax, M.G.; Samelson, R.M. Global Observations of Nonlinear Mesoscale Eddies. Prog. Oceanogr. 2011, 91, 167–216. [Google Scholar] [CrossRef]
- Fu, L.L. Pattern and Velocity of Propagation of the Global Ocean Eddy Variability. J. Geophys. Res.-Ocean. 2009, 114, C11017. [Google Scholar] [CrossRef]
- Cheng, Y.H.; Ho, C.R.; Zheng, Q.; Kuo, N.J. Statistical Characteristics of Mesoscale Eddies in the North Pacific Derived from Satellite Altimetry. Remote Sens. 2014, 6, 5164–5183. [Google Scholar] [CrossRef]
- Raffaele, F.; Wunsch, C. The Distribution of Eddy Kinetic and Potential Energies in the Global Ocean. Tellus A 2010, 62, 92–108. [Google Scholar] [CrossRef]
- Bennett, A.F.; White, W.B. Eddy Heat Flux in the Subtropical North Pacific. J. Phys. Oceanogr. 1986, 16, 728–740. [Google Scholar] [CrossRef]
- Roemmich, D.; Gilson, J. Eddy Transport of Heat and Thermocline Waters in the North Pacific: A Key to Interannual/Decadal Climate Variability. J. Phys. Oceanogr. 2001, 31, 675–687. [Google Scholar] [CrossRef]
- Johnson, K.S.; Riser, S.C.; Karl, D.M. Nitrate Supply from Deep to Near-Surface Waters of the North Pacific Subtropical Gyre. Nature 2010, 465, 1062–1065. [Google Scholar] [CrossRef] [PubMed]
- Dong, C.; McWilliams, J.C.; Liu, Y.; Chen, D. Global Heat and Salt Transports by Eddy Movement. Nat. Commun. 2014, 5, 3294. [Google Scholar] [CrossRef]
- Thompson, A.F.; Heywood, K.J.; Schmidtko, S.; Stewart, A.L. Eddy Transport as a Key Component of the Antarctic Overturning Circulation. Nat. Geosci. 2014, 7, 879–884. [Google Scholar] [CrossRef]
- McGillicuddy, D.J.; Dennis, J. Mechanisms of Physical-Biological-Biogeochemical Interaction at the Oceanic Mesoscale. Annu. Rev. Mar. Sci. 2016, 8, 125–159. [Google Scholar] [CrossRef] [PubMed]
- Melnichenko, O.; Amores, A.; Maximenko, N.; Hacker, P.; Potemra, J. Signature of Mesoscale Eddies in Satellite Sea Surface Salinity Data. J. Geophys. Res.-Ocean. 2017, 122, 1416–1424. [Google Scholar] [CrossRef]
- Chelton, D.B.; Gaube, P.; Schlax, M.G.; Early, J.J.; Samelson, R.M. The Influence of Nonlinear Mesoscale Eddies on Near-Surface Oceanic Chlorophyll. Science 2011, 334, 328–332. [Google Scholar] [CrossRef]
- Gaube, P.; Chelton, D.B.; Samelson, R.M.; Schlax, M.G.; O’Neill, L.W. Satellite Observations of Mesoscale Eddy-Induced Ekman Pumping. J. Phys. Oceanogr. 2015, 45, 104–132. [Google Scholar] [CrossRef]
- Villas Bôas, A.B.; Sato, O.T.; Chaigneau, A.; Castelão, G.P. The Signature of Mesoscale Eddies on the Air-Sea Turbulent Heat Fluxes in the South Atlantic Ocean. Geophys. Res. Lett. 2015, 42, 1856–1862. [Google Scholar] [CrossRef]
- Ma, X.; Chang, P.; Liu, X.; Montuoro, R.; Small, R.J.; Bryan, F.O.; Greatbatch, R.J.; Brandt, P.; Wu, D. Western Boundary Currents Regulated by Interaction between Ocean Eddies and the Atmosphere. Nature 2016, 535, 533–537. [Google Scholar] [CrossRef] [PubMed]
- Fu, L.L.; Chelton, D.B.; Traon, P.Y.L.; Morrow, R. Eddy Dynamics from Satellite Altimetry. Oceanography 2010, 23, 14–25. [Google Scholar] [CrossRef]
- Stumpf, H.G.; Legeckis, R.V. Satellite Observations of Mesoscale Eddy Dynamics in the Eastern Tropical Pacific Ocean. J. Phys. Oceanogr. 1977, 7, 648–658. [Google Scholar] [CrossRef][Green Version]
- Chelton, D.B.; Schlax, M.G.; Samelson, R.M.; de Szoeke, R.A. Global Observations of Large Oceanic Eddies. Geophys. Res. Lett. 2007, 34, L15606. [Google Scholar] [CrossRef]
- D’Alimonte, D. Detection of Mesoscale Eddy-Related Structures through Iso-SST Patterns. IEEE Geosci. Remote Sens. Lett. 2009, 6, 189–193. [Google Scholar] [CrossRef]
- Dong, C.; Nencioli, F.; Liu, Y.; McWilliams, J.C. An Automated Approach to Detect Oceanic Eddies from Satellite Remotely Sensed Sea Surface Temperature Data. IEEE Geosci. Remote Sens. Lett. 2011, 8, 1055–1059. [Google Scholar] [CrossRef]
- Gonzalez-Silvera, A.; Santamaria-del-Angel, E.; Millán-Nuñez, R.; Manzo-Monroy, H. Satellite Observations of Mesoscale Eddies in the Gulfs of Tehuantepec and Papagayo (Eastern Tropical Pacific). Deep-Sea Res. Part II 2004, 51, 587–600. [Google Scholar] [CrossRef]
- Park, J.E.; Park, K.A.; Ullman, D.S.; Cornillon, P.C.; Park, Y.J. Observation of Diurnal Variations in Mesoscale Eddy Sea-Surface Currents Using GOCI Data. Remote Sens. Lett. 2016, 7, 1131–1140. [Google Scholar] [CrossRef]
- Du, Y.; Song, W.; He, Q.; Huang, D.; Liotta, A.; Su, C. Deep Learning with Multi-Scale Feature Fusion in Remote Sensing for Automatic Oceanic Eddy Detection. Inf. Fusion 2019, 49, 89–99. [Google Scholar] [CrossRef]
- Carsey, F.D.; Garwood, R.W. Identification of Modeled Ocean Plumes in Greenland Gyre ERS-1 SAR Data. Geophys. Res. Lett. 1993, 20, 2207–2210. [Google Scholar] [CrossRef]
- Rouault, M.J.; Mouche, A.; Collard, F.; Johannessen, J.A.; Chapron, B. Mapping the Agulhas Current from Space: An Assessment of ASAR Surface Current Velocities. J. Geophys. Res.-Ocean. 2010, 115, C10026. [Google Scholar] [CrossRef]
- Chen, G.; Chen, X.; Huang, B. Independent Eddy Identification with Profiling Argo as Calibrated by Altimetry. J. Geophys. Res.-Ocean. 2021, 126, e2020JC016729. [Google Scholar] [CrossRef]
- Robinson, A.R. Overview and Summary of Eddy Science. In Eddies in Marine Science; Robinson, A.R., Ed.; Springer: Berlin/Heidelberg, Germany, 1983; Volume 609, pp. 3–15. [Google Scholar] [CrossRef]
- Faghmous, J.H.; Frenger, I.; Yao, Y.; Warmka, R.; Lindell, A.; Kumar, V. A Daily Global Mesoscale Ocean Eddy Dataset from Satellite Altimetry. Sci. Data 2015, 2, 150028. [Google Scholar] [CrossRef]
- Frenger, I.; Gruber, N.; Knutti, R.; Münnich, M. Imprint of Southern Ocean Eddies on Winds, Clouds and Rainfall. Nat. Geosci. 2013, 6, 608–612. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, W.; Qiu, B. Oceanic Mass Transport by Mesoscale Eddies. Science 2014, 345, 322–324. [Google Scholar] [CrossRef]
- Amores, A.; Jordà, G.; Arsouze, T.; Le Sommer, J. Up to What Extent Can We Characterize Ocean Eddies Using Present-Day Gridded Altimetric Products? J. Geophys. Res.-Ocean. 2018, 123, 7220–7236. [Google Scholar] [CrossRef]
- Xu, Y.; Fu, L.L. The Effects of Altimeter Instrument Noise on the Estimation of the Wavenumber Spectrum of Sea Surface Height. J. Phys. Oceanogr. 2012, 42, 2229–2233. [Google Scholar] [CrossRef]
- Dufau, C.; Orsztynowicz, M.; Dibarboure, G.; Morrow, R.; Le Traon, P.-Y. Mesoscale Resolution Capability of Altimetry: Present and Future. J. Geophys. Res.-Ocean. 2016, 121, 4910–4927. [Google Scholar] [CrossRef]
- Treguier, A.M.; Deshayes, J.; Lique, C.; Dussin, R.; Molines, J.M. Eddy Contributions to The Meridional Transport of Salt in the North Atlantic. J. Geophys. Res.-Ocean. 2012, 177, C05010. [Google Scholar] [CrossRef]
- Mkhinini, N.; Coimbra, A.L.S.; Stegner, A.; Arsouze, T.; Taupier-Letage, I.; Béranger, K. Long-Lived Mesoscale Eddies in the Eastern Mediterranean Sea: Analysis of 20 Years of AVISO Geostrophic Velocities. J. Geophys. Res.-Ocean. 2014, 119, 8603–8626. [Google Scholar] [CrossRef]
- Escudier, R.; Renault, L.; Ananda, P.; Pierre, B.; Chelton, D.; Jonathan, B. Eddy Properties in the Western Mediterranean Sea from Satellite Altimetry and a Numerical Simulation. J. Geophys. Res.-Ocean. 2016, 121, 3990–4006. [Google Scholar] [CrossRef]
- Le, V.B.; Stegner, A.; Arsouze, T. Angular Momentum Eddy Detection and Tracking Algorithm (AMEDA) and Its Application to Coastal Eddy Formation. J. Atmos. Ocean. Technol. 2018, 35, 739–762. [Google Scholar] [CrossRef]
- Morrow, R.; Fu, L.L.; Ardhuin, F.; Benkiran, M.; Chapron, B.; Cosme, E.; D’Ovidio, F.; Farrar, J.T.; Gille, S.T.; Lapeyre, G.; et al. Global Observations of Fine-Scale Ocean Surface Topography With the Surface Water and Ocean Topography (SWOT) Mission. Front. Mar. Sci. 2019, 6, 232. [Google Scholar] [CrossRef]
- Pujol, M.I.; Faugère, Y.; Taburet, G.; Dupuy, S.; Pelloquin, C.; Ablain, M.; Picot, N. DUACS DT2014: The New Multi-Mission Altimeter Data Set Reprocessed over 20 Years. Ocean. Sci. 2016, 12, 1067–1090. [Google Scholar] [CrossRef]
- Doglioli, A.M.; Blanke, B.; Speich, S.; Lapeyre, G. Tracking Coherent Structures in a Regional Ocean Model with Wavelet Analysis: Application to Cape Basin Eddies. J. Geophys. Res.-Ocean. 2007, 112, C05043. [Google Scholar] [CrossRef]
- Chaigneau, A.; Gizolme, A.; Grados, C. Mesoscale Eddies off Peru in Altimeter Records: Identification Algorithms and Eddy Spatio-Temporal Patterns. Prog. Oceanogr. 2008, 79, 106–119. [Google Scholar] [CrossRef]
- Williams, S.; Hecht, M.; Petersen, M.; Strelitz, R.; Maltrud, M.; Ahrens, J.P.; Hlawitschka, M.; Hamann, B. Visualization and Analysis of Eddies in a Global Ocean Simulation. Comput. Graph. Forum 2011, 30, 991–1000. [Google Scholar] [CrossRef]
- Haller, G.; Hadjighasem, A.; Farazmand, M.; Huhn, F. Defining Coherent Vortices Objectively from the Vorticity. J. Fluid. Mech. 2015, 795, 136–173. [Google Scholar] [CrossRef]
- Isern-Fontanet, J.; Garcia-Ladona, E.; Font, J. Identification of Marine Eddies from Altimetric Maps. J. Atmos. Ocean. Tech. 2003, 20, 772–778. [Google Scholar] [CrossRef]
- Penven, P.; Echevin, V.; Pasapera, J.; Colas, F.; Tam, J. Average Circulation, Seasonal Cycle, and Mesoscale Dynamics of the Peru Current System: A Modeling Approach. J. Geophys. Res.-Ocean. 2005, 110, C10021. [Google Scholar] [CrossRef]
- Henson, S.A.; Thomas, A.C. A Census of Oceanic Anticyclonic Eddies in the Gulf of Alaska. Deep.-Sea Res. Part I 2008, 55, 163–176. [Google Scholar] [CrossRef]
- Liu, Y.; Chen, G.; Sun, M.; Liu, S.; Tian, F. A Parallel SLA-Based Algorithm for Global Mesoscale Eddy Identification. J. Atmos. Ocean. Tech. 2016, 33, 2743–2754. [Google Scholar] [CrossRef]
- Tian, F.; Wu, D.; Yuan, L.; Chen, G. Impacts of the Efficiencies of Identification and Tracking Algorithms on the Statistical Properties of Global Mesoscale Eddies Using Merged Altimeter Data. Int. J. Remote Sens. 2020, 41, 2835–2860. [Google Scholar] [CrossRef]
- Arbic, B.K.; Scott, R.B.; Chelton, D.B.; Richman, J.G.; Shriver, J.F. Effects of Stencil Width on Surface Ocean Geostrophic Velocity and Vorticity Estimation from Gridded Satellite Altimeter Data. J. Geophys. Res.-Atmos. 2012, 117, 3029. [Google Scholar] [CrossRef]
- Durand, M.; Fu, L.L.; Lettenmaier, D.P.; Alsdorf, D.E.; Rodriguez, E.; Esteban-Fernandez, D. The Surface Water and Ocean Topography Mission: Observing Terrestrial Surface Water and Oceanic Submesoscale Eddies. Proc. IEEE 2010, 98, 766–779. [Google Scholar] [CrossRef]
- Pujol, M.I.; Dibarboure, G.; Le Traon, P.Y.; Klein, P. Using High-Resolution Altimetry to Observe Mesoscale Signals. J. Atmos. Ocean. Tech. 2013, 29, 1409–1416. [Google Scholar] [CrossRef]
- Chen, G.; Han, G.; Yang, X. On the Intrinsic Shape of Oceanic Eddies Derived from Satellite Altimetry. Remote Sens. Environ. 2019, 228, 75–89. [Google Scholar] [CrossRef]
- Chelton, D.B.; Deszoeke, R.A.; Schlax, M.G.; Naggar, K.E.; Siwertz, N. Geographical Variability of the First Baroclinic Rossby Radius of Deformation. J. Phys. Oceanogr. 1998, 28, 433–460. [Google Scholar] [CrossRef]
- Chen, G.; Han, G. Contrasting Short-Lived with Long-Lived Mesoscale Eddies in the Global Ocean. J. Geophys. Res.-Ocean. 2019, 124, 3149–3167. [Google Scholar] [CrossRef]
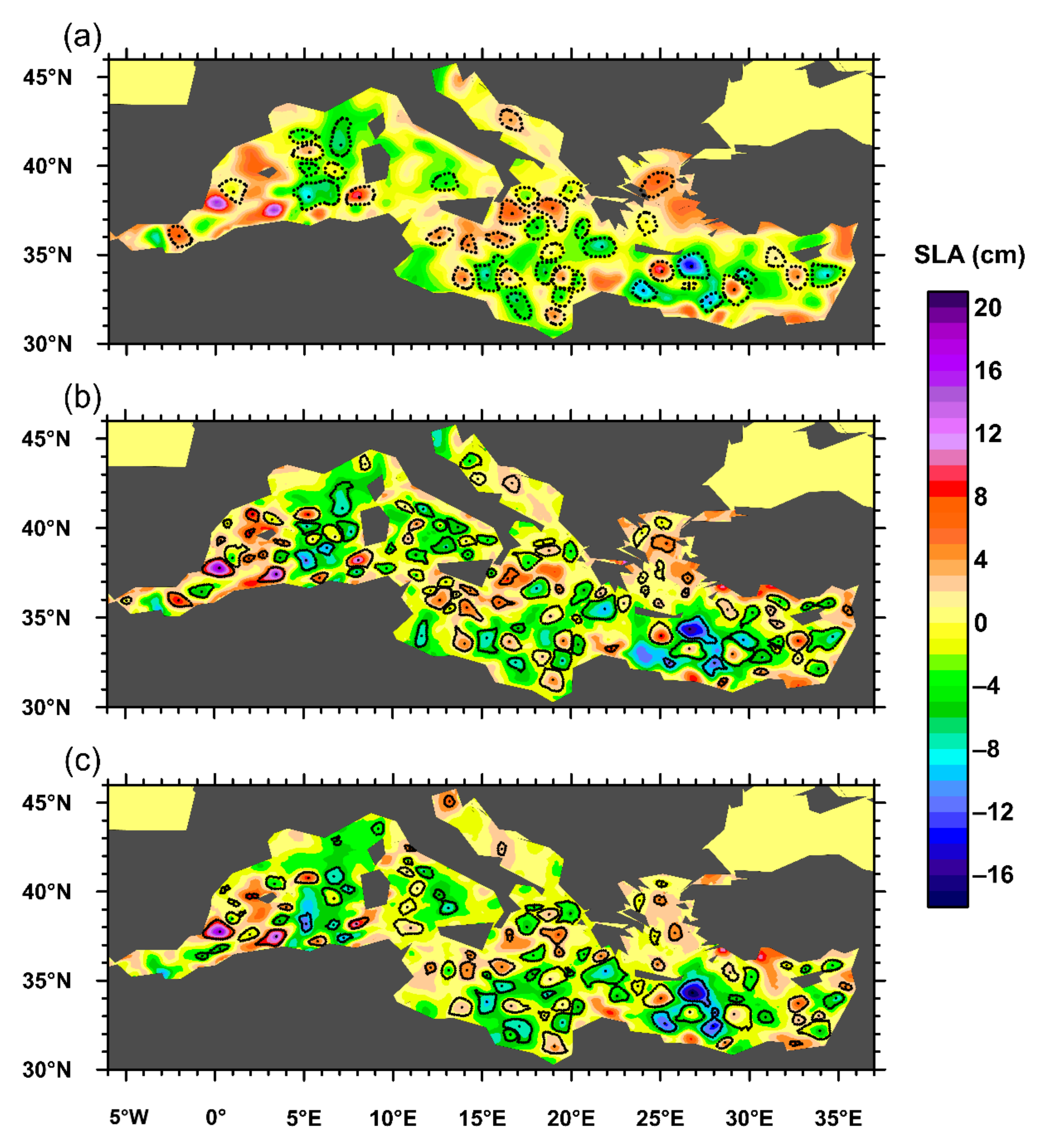
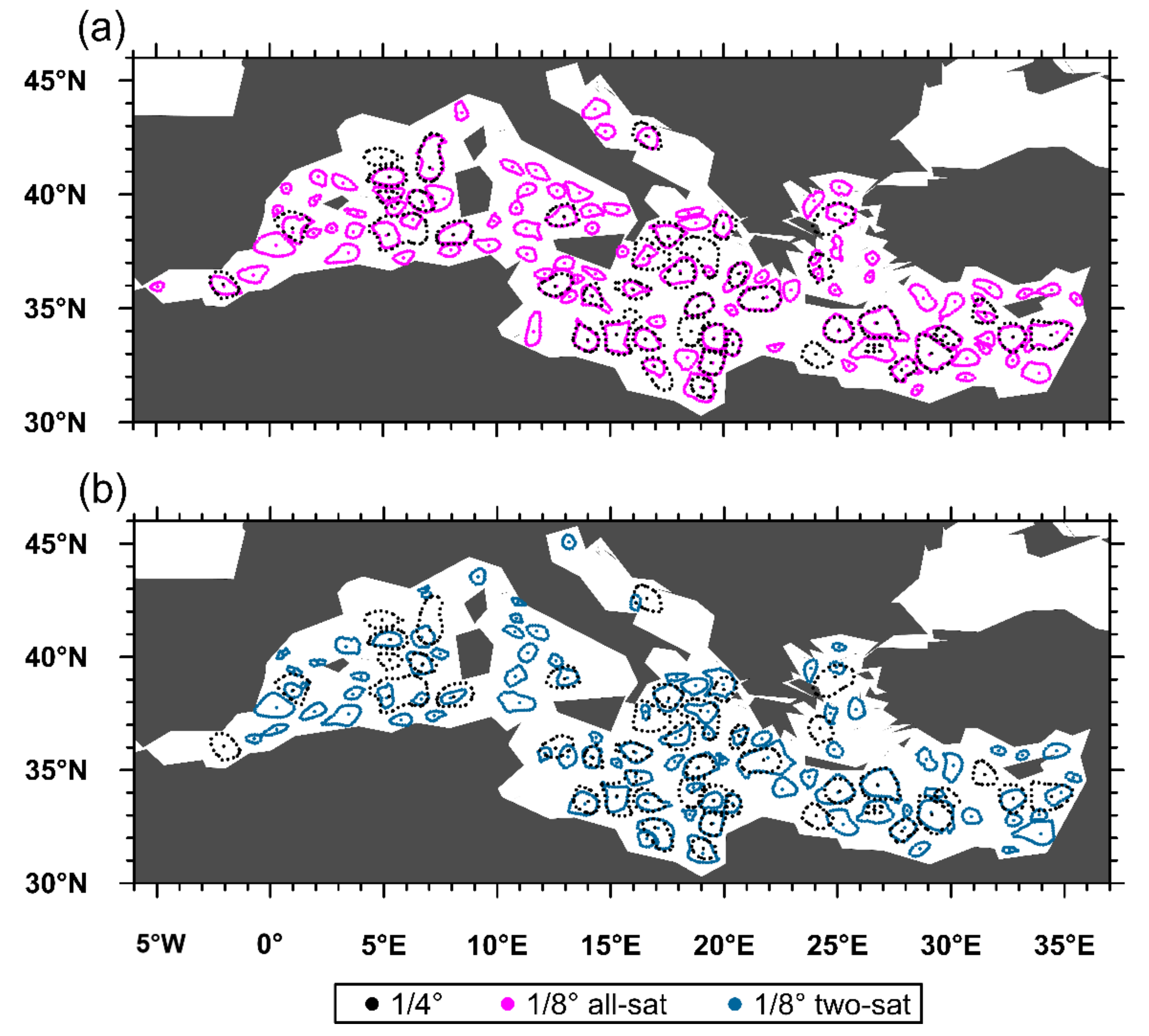
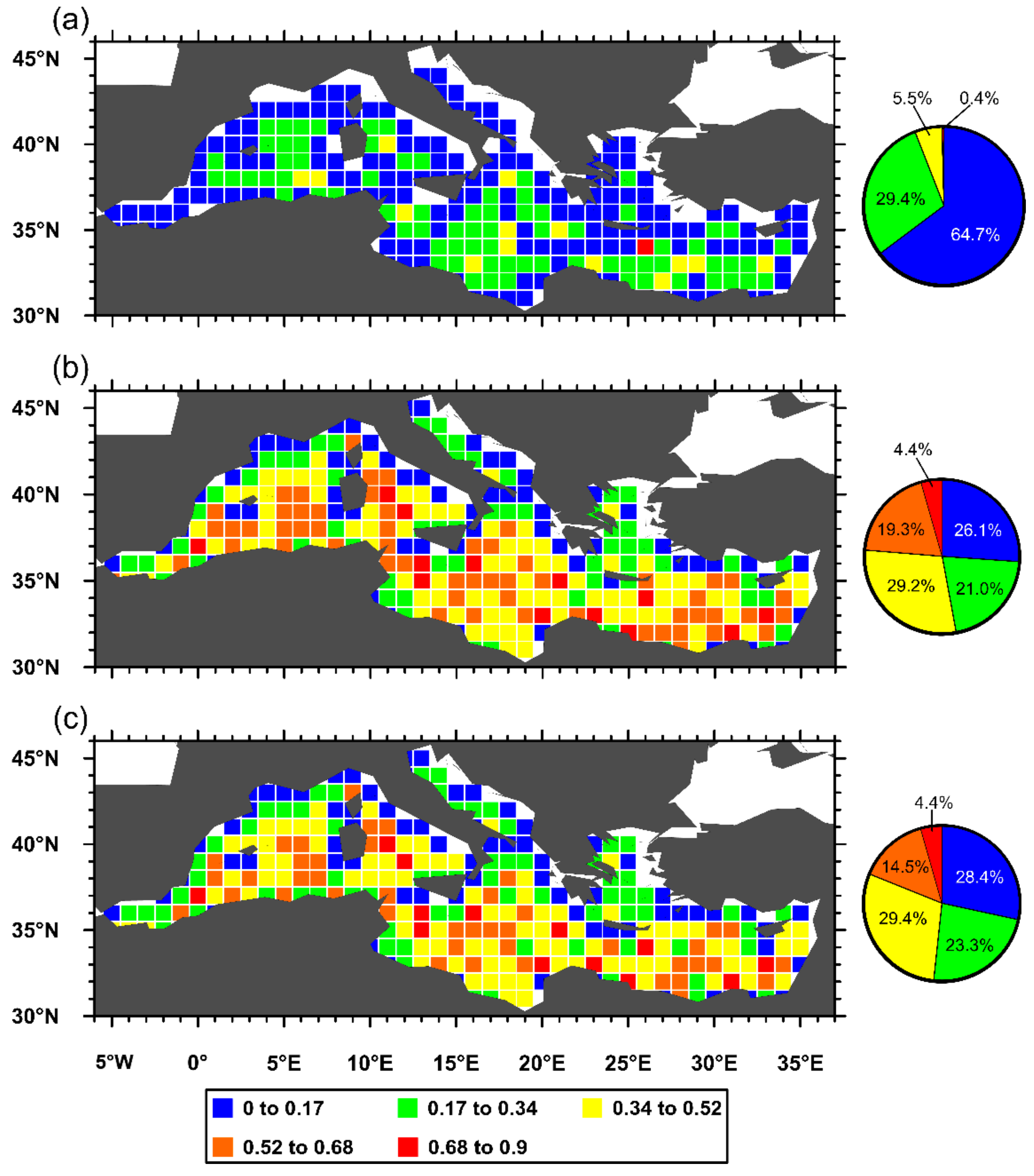

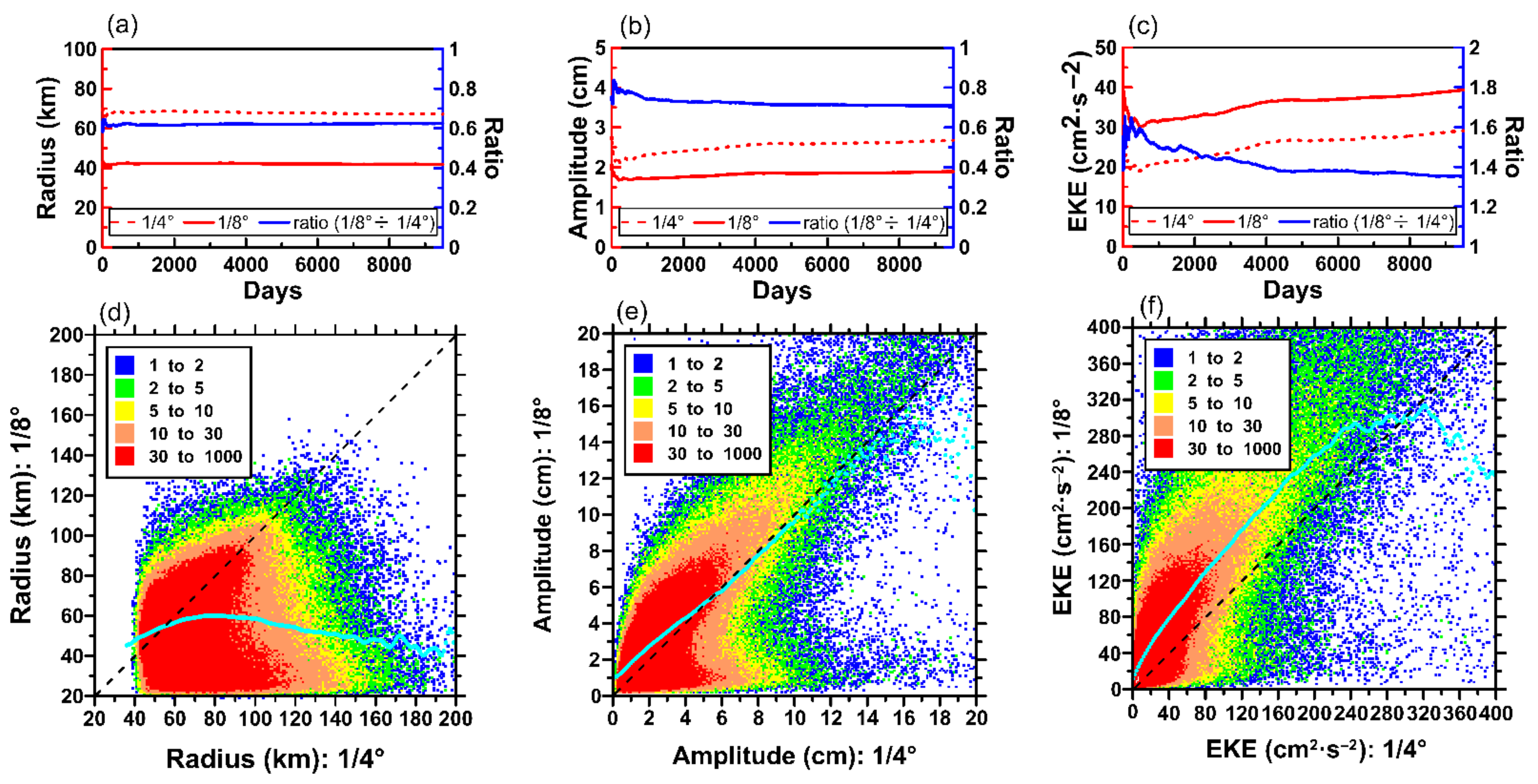

| Resolution | Coverage | Range | Time Series | Satellites | Type |
|---|---|---|---|---|---|
| 1/4° × 1/4° Daily | Global Ocean | 90°S–90°N 180°W–180°E | 19930101–20181231 | All-Sat➀ | Gridded |
| 1/8° × 1/8° Daily | Mediterranean Sea | 30°N–46°N 6°W–37°E | All-Sat➁ | ||
| Two-Sat➂ | |||||
| South-China Sea | 0°–25°N 105°E–125°E | 20180101–20181231 | All-Sat | ||
| North-West Pacific | 25°N–45°N 120°E–160°E | ||||
| South-East Pacific | 55°S–35°S 130°W–90°W |
| Situation | A | B | C | D |
|---|---|---|---|---|
| 1/4° eddy number | 1 | 1 | 1 | 0 |
| 1/8° eddy number | 1 | 0 | >1 | 1 |
| Sea Area | Mediterranean Sea | ||
|---|---|---|---|
| resolution | 1/4° | 1/8° all-sat | 1/8° two-sat |
| Eddy number | 39 | 102 | 96 |
| Eddy density (num·deg−2·day−1) | 0.15 | 0.38 | 0.35 |
| Eddy radius (km) | 67 | 42 | 43 |
| Eddy amplitude (cm) | 2.68 | 1.90 | 1.86 |
| EKE (cm2·s−2) | 29 | 39 | 36 |
| Eddy eccentricity | 0.77 | 0.78 | 0.78 |
| Situations | Datasets Foundations | Mediterranean Sea | |
|---|---|---|---|
| All-Sat | Two-Sat | ||
| A | 1/4° | 0.72 | 0.70 |
| B | 0.17 | 0.20 | |
| C | 0.11 | 0.10 | |
| D | 1/8° | 0.63 | 0.63 |
| Sea Area | South-China Sea | North-West Pacific | South-East Pacific | |||
|---|---|---|---|---|---|---|
| Resolution | 1/4° | 1/8° | 1/4° | 1/8° | 1/4° | 1/8° |
| Eddy number | 35 | 54 | 94 | 165 | 148 | 254 |
| Eddy density (num·deg−2·day−1) | 0.09 | 0.14 | 0.14 | 0.24 | 0.19 | 0.32 |
| Eddy radius (km) | 72 | 48 | 77 | 47 | 79 | 49 |
| Eddy amplitude (cm) | 2.55 | 1.43 | 6.18 | 3.02 | 3.43 | 1.88 |
| EKE (cm2·s−2) | 162 | 111 | 146 | 94 | 31 | 23 |
| Eddy eccentricity | 0.79 | 0.78 | 0.79 | 0.78 | 0.78 | 0.77 |
| Situations | Datasets Foundations | South-China Sea | North-West Pacific | South-East Pacific |
|---|---|---|---|---|
| A | 1/4° | 0.84 | 0.88 | 0.89 |
| B | 0.13 | 0.06 | 0.06 | |
| C | 0.03 | 0.06 | 0.05 | |
| D | 1/8° | 0.43 | 0.44 | 0.42 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Chen, X.; Han, G.; Jin, P.; Yang, J. From 1/4° to 1/8°: Influence of Spatial Resolution on Eddy Detection Using Altimeter Data. Remote Sens. 2022, 14, 149. https://doi.org/10.3390/rs14010149
Wang Y, Chen X, Han G, Jin P, Yang J. From 1/4° to 1/8°: Influence of Spatial Resolution on Eddy Detection Using Altimeter Data. Remote Sensing. 2022; 14(1):149. https://doi.org/10.3390/rs14010149
Chicago/Turabian StyleWang, Yinuo, Xiaoyan Chen, Guiyan Han, Pingping Jin, and Jie Yang. 2022. "From 1/4° to 1/8°: Influence of Spatial Resolution on Eddy Detection Using Altimeter Data" Remote Sensing 14, no. 1: 149. https://doi.org/10.3390/rs14010149
APA StyleWang, Y., Chen, X., Han, G., Jin, P., & Yang, J. (2022). From 1/4° to 1/8°: Influence of Spatial Resolution on Eddy Detection Using Altimeter Data. Remote Sensing, 14(1), 149. https://doi.org/10.3390/rs14010149






