Targeted Rock Slope Assessment Using Voxels and Object-Oriented Classification
Abstract
1. Introduction
2. Current State and Related Works
- Single-sized point neighbourhoods;
- Multi-sized point neighbourhoods; and,
- Adjusted-sized point neighbourhoods.
3. Materials and Methods
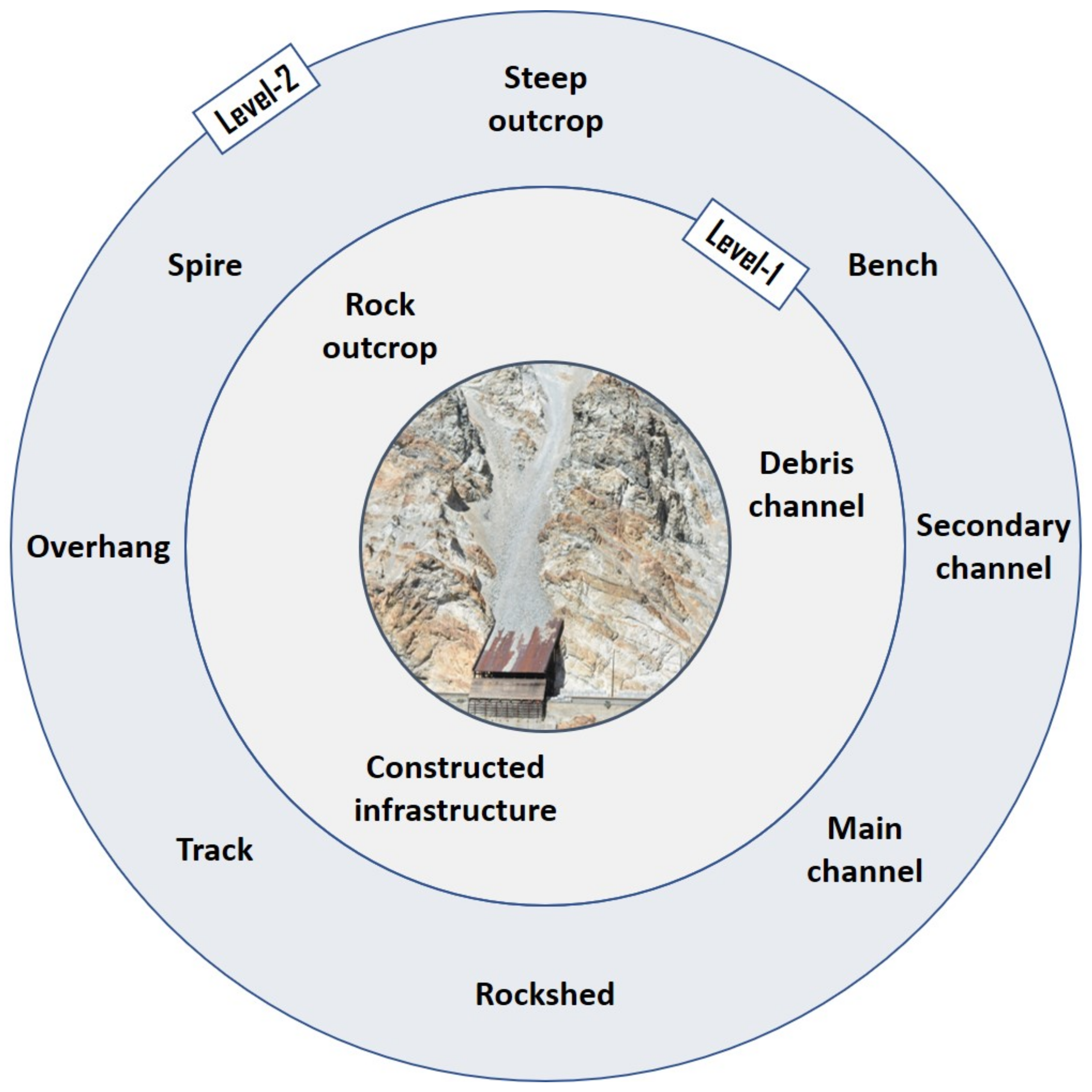
3.1. Study Dataset
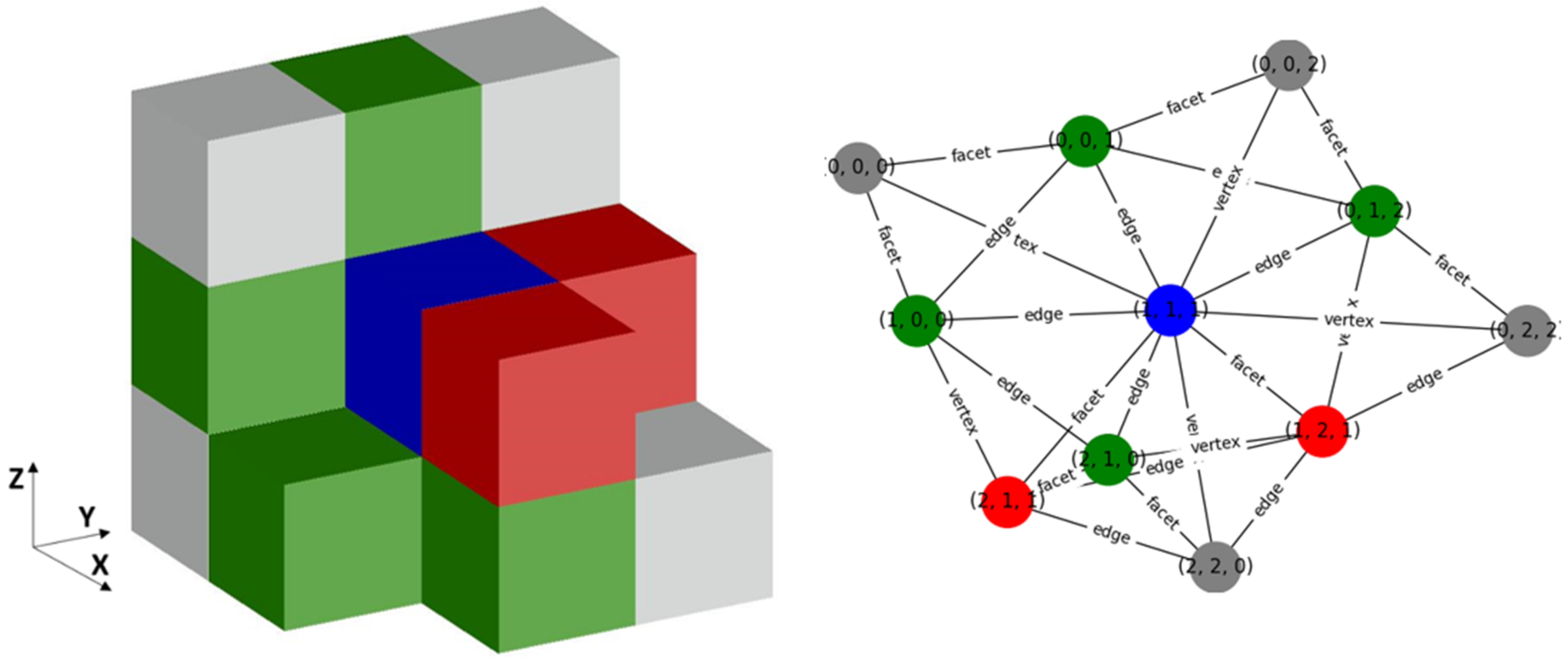
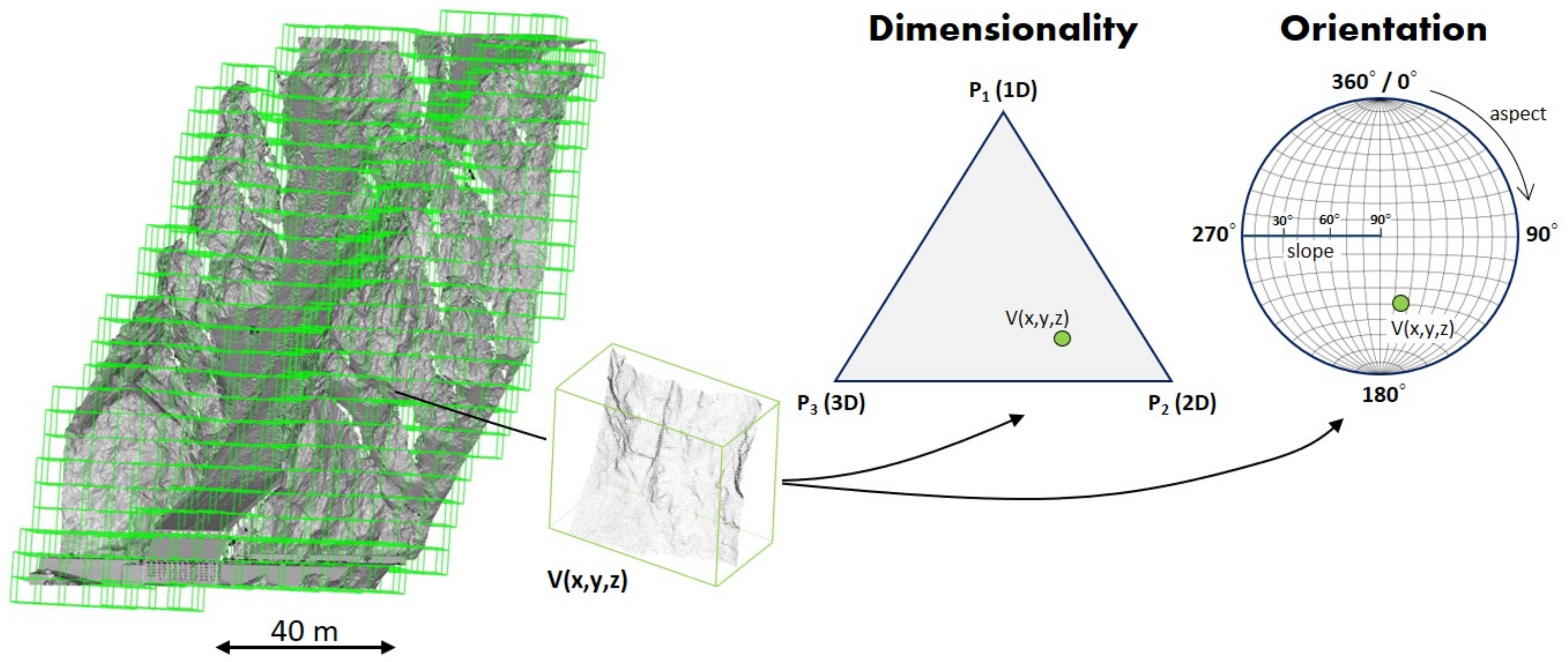
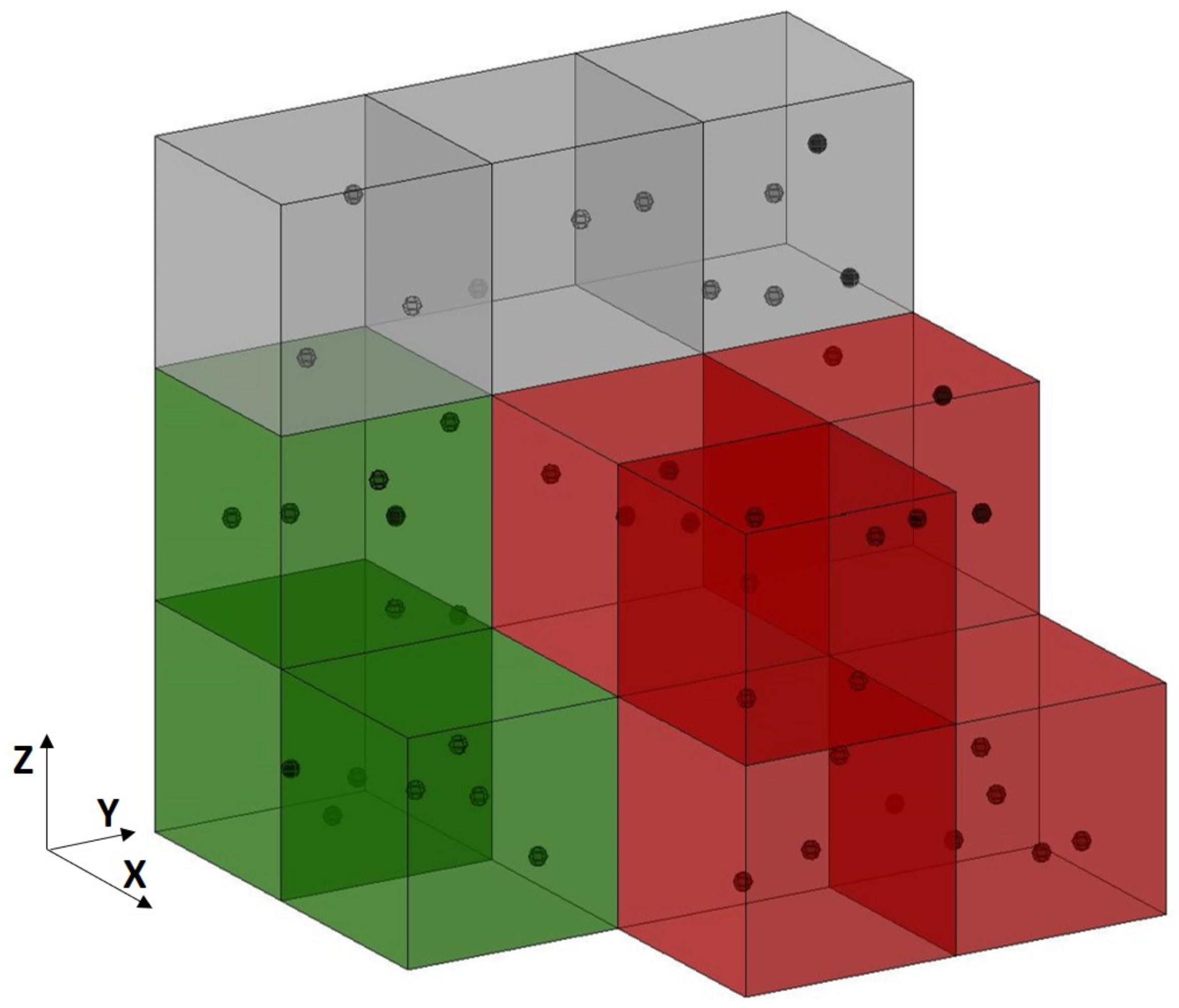
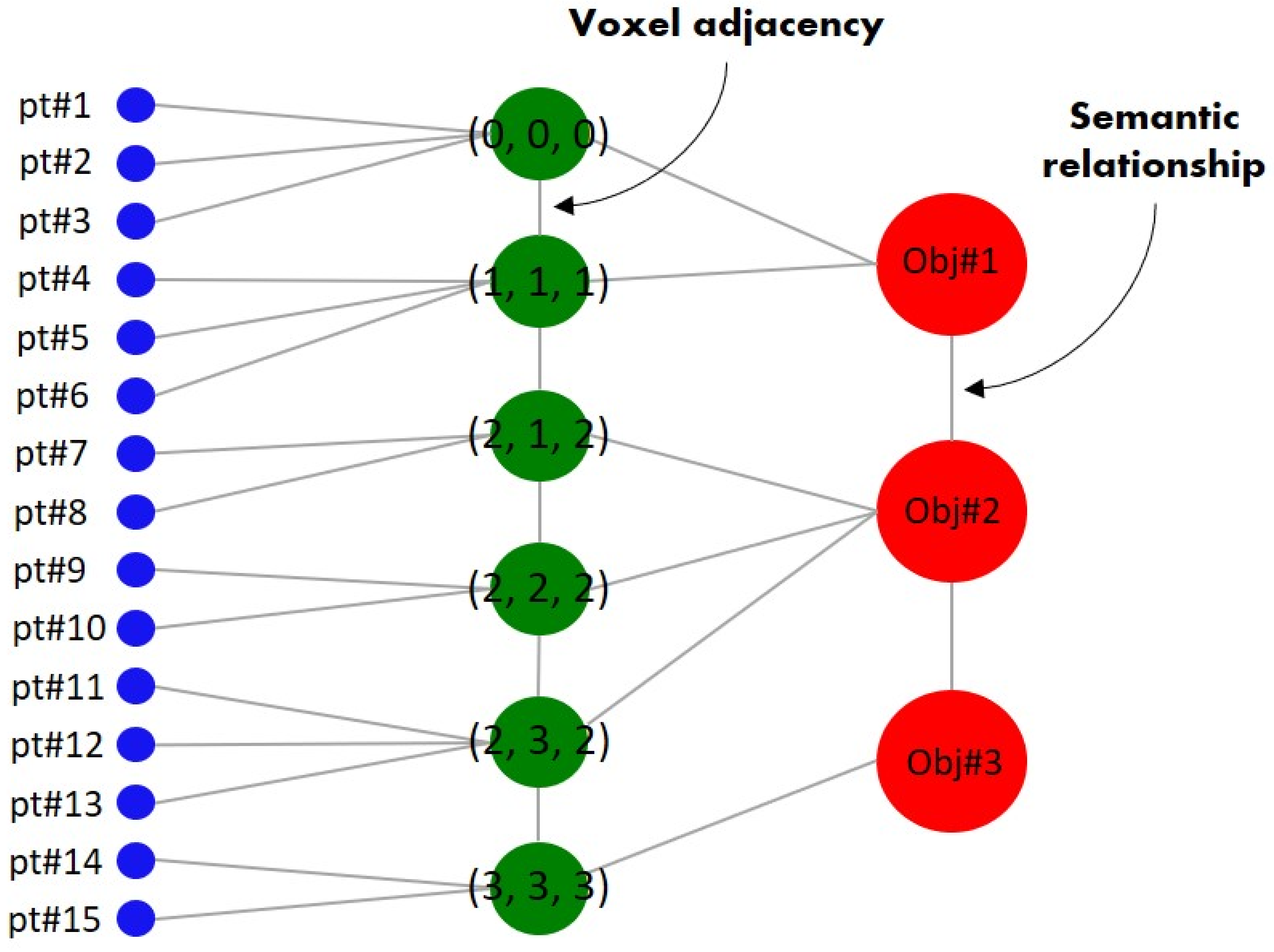
3.2. Voxelization
3.3. Characterization
3.4. Scene Homogenization
3.5. Classification
4. Analysis and Results
4.1. Metrics
- TP: Instances correctly predicted to be positive.
- TN: Instances correctly predicted to be negative.
- FP: Instances erroneously predicted to be positive.
- FN: Instances erroneously predicted to be negative.
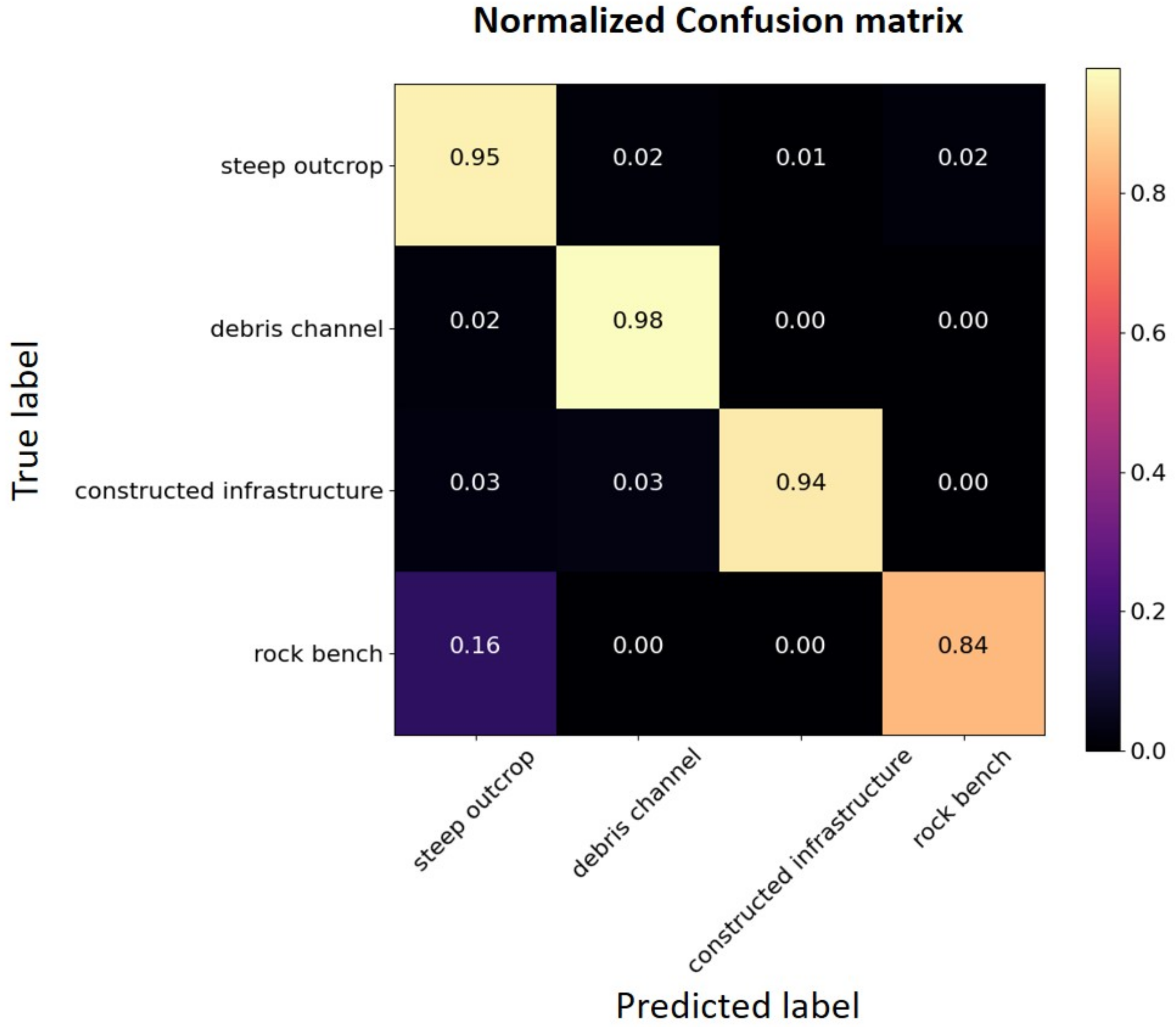
4.2. Evaluation
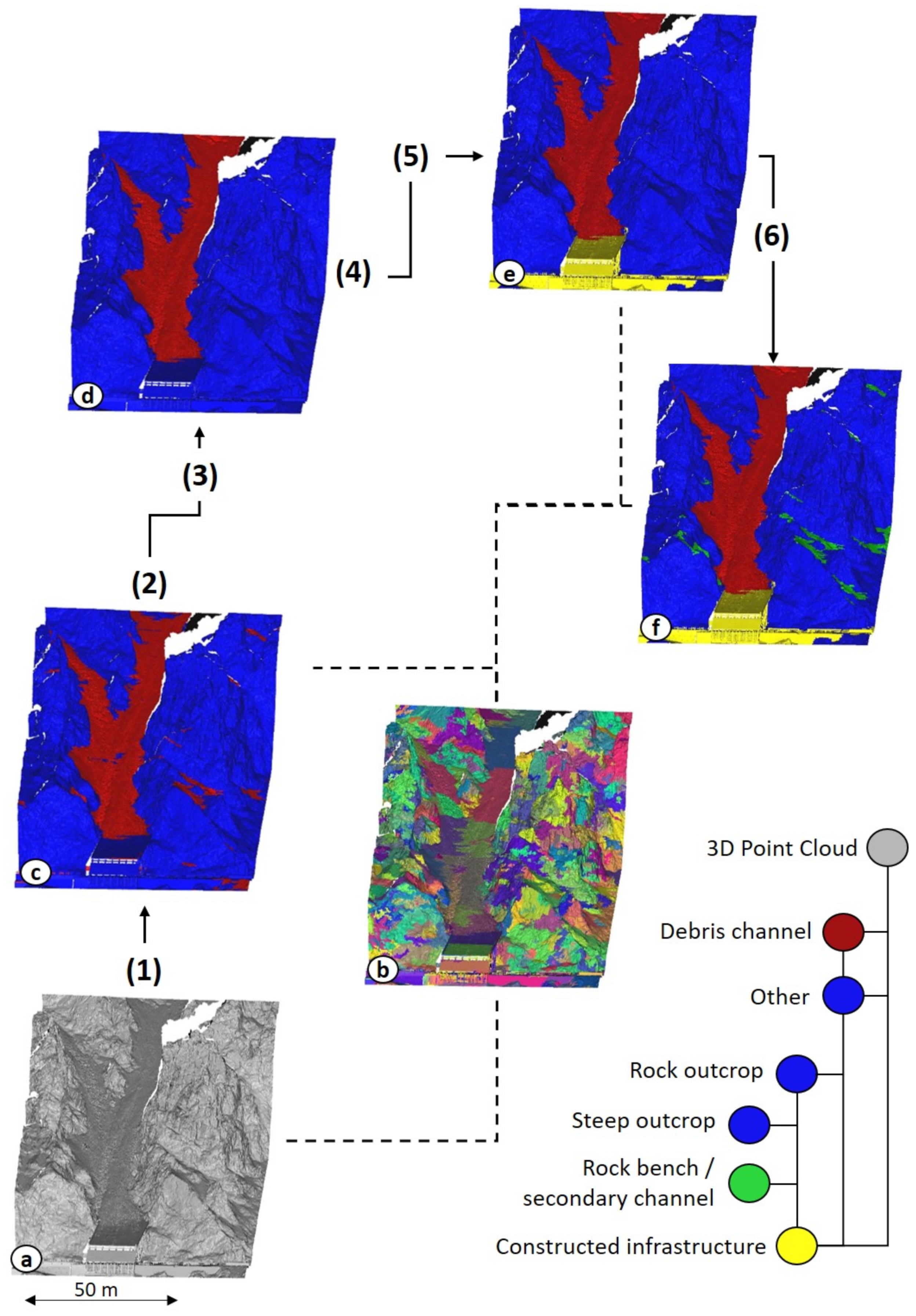
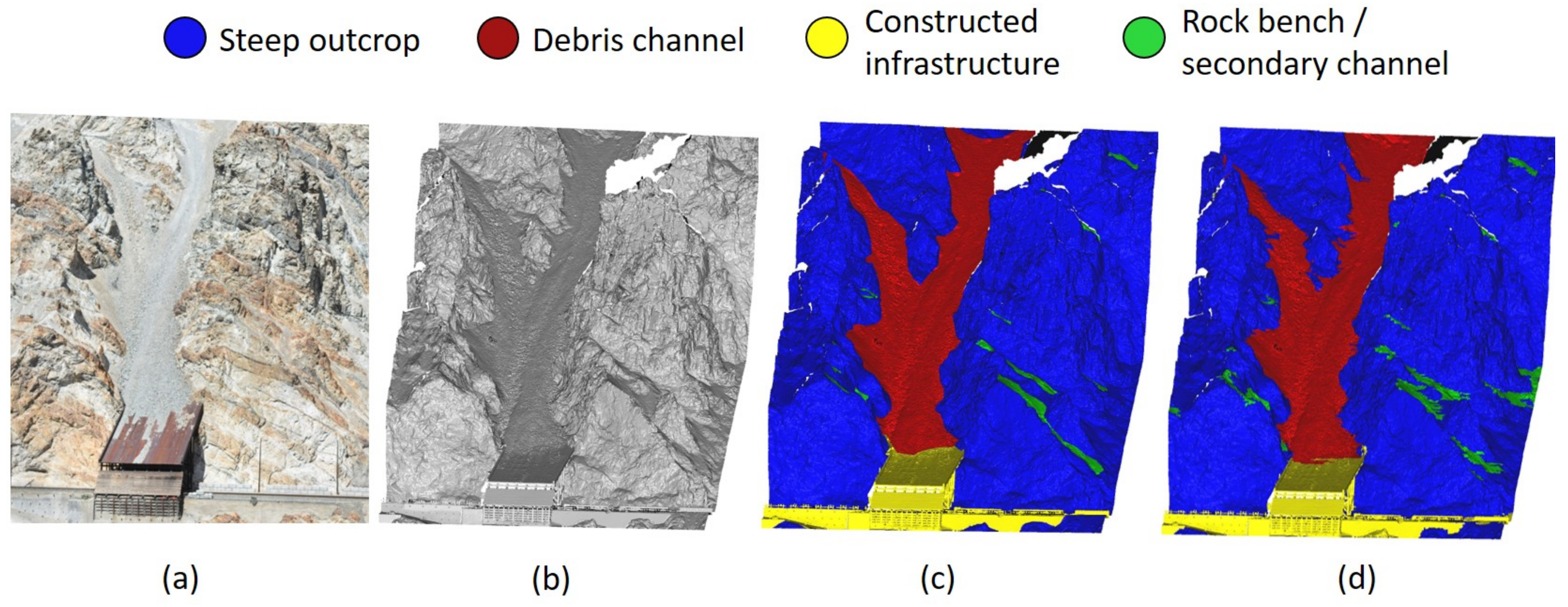
4.2.1. Predictions
- 4.
- “Debris channel” candidate segments are labeled based on their slope. Debris channels represent features generated by erosion and usually dip at lower angles relative to the rest of the slope.
- 5.
- Non-debris channel candidate segments surrounded by “debris channel” candidates are aggregated and refined. This includes potential large rock boulders within the main channel.
- 6.
- A refined object is then tagged “debris channel” if its Z-axis range at the point level is higher than a length threshold.
- 7.
- A linear or compact object located lower than the “debris channel” is tagged “constructed infrastructure”. Rail lines and barrier walls along the track are aggregated as linear elements while rockshed components are defined as compact.
- 8.
- Remaining objects surrounded by “constructed infrastructure” are incorporated and refined. The remaining objects are tagged “rock outcrop”. This rule is applied to prevent potential misclassifications of parts of the infrastructure as natural features.
- 9.
- At a lower-granularity level, lower-slope “rock outcrop” objects dipping at an aspect (sub)-perpendicular to the average rock slope aspect are tagged “rock bench/secondary channel”.
4.2.2. Comparison to Point-Based Machine Learning
4.3. Application
5. Discussion
6. Summary and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| 2, 2.3, 3D | 2-, 2.5, 3-dimensional; |
| CS | computer science; |
| CV | computer vision; |
| DEM | digital elevation model; |
| GIS | geographic information system; |
| ML | machine learning; |
| PCA | principal component analysis; |
| SVM | support vector machine; |
| RF | random forests; |
| TLS | terrestrial laser scanner; |
| SCV | singular value decomposition; |
| LV | local variance; |
| ICP | iterative closest point; |
| SLIDO | statewide landslide information database for Oregon; |
| TP | true positive; |
| TN | true negative; |
| FP | false positive; |
| FN | false negative; |
| RGB | red-green-blue; |
| M3C2 | Multi-scale model to model cloud comparison; |
| DL | deep learning. |
References
- Rutzinger, M.; Höfle, B.; Lindenbergh, R.; Oude Elberink, S.; Pirotti, F.; Sailer, R.; Scaioni, M.; Stötter, J.; Wujanz, D. Close-Range Sensing Techniques in Alpine Terrain. ISPRS Ann. Photogramm. Remote Sens. Spat. Inf. Sci. 2016, III–6, 15–22. [Google Scholar] [CrossRef]
- Scaioni, M.; Longoni, L.; Melillo, V.; Papini, M. Remote sensing for landslide investigations: An overview of recent achievements and perspectives. Remote Sens. 2014, 6, 9600–9652. [Google Scholar] [CrossRef]
- Hutchinson, D.J.; Lato, M.; Gauthier, D.; Kromer, R.; Ondercin, M.; Van Veen, M.; Harrap, R. Applications of remote sensing techniques to managing rock slope instability risk. In Proceedings of the 68th Canadian Geotechnical Conference-GEOQuébec 2015, Quebec City, QC, Canada, 21–23 September 2015; p. 10. [Google Scholar]
- Abellán, A.; Jaboyedoff, M.; Oppikofer, T.; Vilaplana, J.M. Detection of millimetric deformation using a terrestrial laser scanner: Experiment and application to a rockfall event. Nat. Hazards Earth Syst. Sci. 2009, 9, 365–372. [Google Scholar] [CrossRef]
- Kromer, R.A.; Hutchinson, D.J.; Lato, M.J.; Gauthier, D.; Edwards, T. Identifying rock slope failure precursors using LiDAR for transportation corridor hazard management. Eng. Geol. 2015, 195, 93–103. [Google Scholar] [CrossRef]
- Lato, M.; Diederichs, M.S.; Hutchinson, D.J.; Harrap, R. Optimization of LiDAR scanning and processing for automated structural evaluation of discontinuities in rockmasses. Int. J. Rock Mech. Min. Sci. 2009, 46, 194–199. [Google Scholar] [CrossRef]
- Bonneau, D.A.; Jean Hutchinson, D.; DiFrancesco, P.M.; Coombs, M.; Sala, Z. Three-dimensional rockfall shape back analysis: Methods and implications. Nat. Hazards Earth Syst. Sci. 2019, 19, 2745–2765. [Google Scholar] [CrossRef]
- Bonneau, D.; DiFrancesco, P.M.; Jean Hutchinson, D. Surface reconstruction for three-dimensional rockfall volumetric analysis. ISPRS Int. J. Geo-Inf. 2019, 8, 548. [Google Scholar] [CrossRef]
- Rowe, E.; Hutchinson, D.J.; Kromer, R.A. An analysis of failure mechanism constraints on pre-failure rock block deformation using TLS and roto-translation methods. Landslides 2018, 15, 409–421. [Google Scholar] [CrossRef]
- Oppikofer, T.; Jaboyedoff, M.; Blikra, L.; Derron, M.H.; Metzger, R. Characterization and monitoring of the Åknes rockslide using terrestrial laser scanning. Nat. Hazards Earth Syst. Sci. 2009, 9, 1003–1019. [Google Scholar] [CrossRef]
- Williams, J.G.; Rosser, N.J.; Hardy, R.J.; Brain, M.J.; Afana, A.A. Optimising 4-D surface change detection: An approach for capturing rockfall magnitude-frequency. Earth Surf. Dyn. 2018, 6, 101–119. [Google Scholar] [CrossRef]
- Martha, T.R.; Kerle, N.; Jetten, V.; van Westen, C.J.; Kumar, K.V. Characterising spectral, spatial and morphometric properties of landslides for semi-automatic detection using object-oriented methods. Geomorphology 2010, 116, 24–36. [Google Scholar] [CrossRef]
- Feizizadeh, B.; Blaschke, T. A semi-automated object based image analysis approach for landslide delineation. In Proceedings of the 2013 European Space Agency Living Planet Symposium, Edinburgh, UK, 9–13 September 2013; pp. 9–13. [Google Scholar] [CrossRef]
- Hölbling, D.; Eisank, C.; Albrecht, F.; Vecchiotti, F.; Friedl, B.; Weinke, E.; Kociu, A. Comparing manual and semi-automated landslide mapping based on optical satellite images from different sensors. Geosciences 2017, 7, 37. [Google Scholar] [CrossRef]
- Karantanellis, E.; Marinos, V.; Vassilakis, E.; Christaras, B. Object-based analysis using unmanned aerial vehicles (UAVs) for site-specific landslide assessment. Remote Sens. 2020, 12, 1711. [Google Scholar] [CrossRef]
- Farmakis, I.; Bonneau, D.; Hutchinson, D.J.; Vlachopoulos, N. Supervoxel-Based Multi-Scale Point Cloud Segmentation Using FNEA for Object-Oriented Rock Slope Classification Using TLS. Int. Arch. Photogramm. Remote Sens. Spat. Inf. Sci. 2020, 43, 1049–1056. [Google Scholar] [CrossRef]
- Pauly, M.; Keiser, R.; Gross, M. Multi-scale Feature Extraction on Point-Sampled Surfaces. Comput. Graph. Forum 2003, 22, 281–289. [Google Scholar] [CrossRef]
- Weinmann, M.; Jutzi, B.; Hinz, S.; Mallet, C. Semantic point cloud interpretation based on optimal neighborhoods, relevant features and efficient classifiers. ISPRS J. Photogramm. Remote Sens. 2015, 105, 286–304. [Google Scholar] [CrossRef]
- Brodu, N.; Lague, D. 3D terrestrial lidar data classification of complex natural scenes using a multi-scale dimensionality criterion: Applications in geomorphology. ISPRS J. Photogramm. Remote Sens. 2012, 68, 121–134. [Google Scholar] [CrossRef]
- Bosser, B.; Guyon, I.; Vapnik, V. A Training Algorithm for Optimal Margin Classifiers. In Proceedings of the Fifth Annual Workshop on Computational Learning Theory, New York, NY, USA, 27–29 July 1992; pp. 144–152. [Google Scholar]
- Bonneau, D.A.; Hutchinson, D.J. The use of terrestrial laser scanning for the characterization of a cliff-talus system in the Thompson River Valley, British Columbia, Canada. Geomorphology 2019, 327, 598–609. [Google Scholar] [CrossRef]
- Weidner, L.; Walton, G.; Kromer, R. Classification methods for point clouds in rock slope monitoring: A novel machine learning approach and comparative analysis. Eng. Geol. 2019, 263, 105326. [Google Scholar] [CrossRef]
- Breiman, L. Random Forests. Mach. Learn. 2001, 45, 5–32. [Google Scholar] [CrossRef]
- Feng, C.C.; Guo, Z. Automating parameter learning for classifying terrestrial LiDAR point cloud using 2D land cover maps. Remote Sens. 2018, 10, 1192. [Google Scholar] [CrossRef]
- Blomley, R.; Weinmann, M.; Leitloff, J.; Jutzi, B. Shape distribution features for point cloud analysis—A geometric histogram approach on multiple scales. ISPRS Ann. Photogramm. Remote Sens. Spat. Inf. Sci. 2014, II–3, 9–16. [Google Scholar] [CrossRef]
- Thomas, H.; Goulette, F.; Deschaud, J.E.; Marcotegui, B.; Gall, Y. Le Semantic classification of 3d point clouds with multiscale spherical neighborhoods. In Proceedings of the 2018 International conference on 3D vision (3DV), Verona, Italy, 5–8 September 2018; pp. 390–398. [Google Scholar] [CrossRef]
- Ioannou, Y.; Taati, B.; Harrap, R.; Greenspan, M. Difference of normals as a multi-scale operator in unorganized point clouds. In Proceedings of the 2012 Second International Conference on 3D Imaging, Modeling, Processing, Visualization & Transmission, Zurich, Switzerland, 13–15 October 2012; pp. 501–508. [Google Scholar] [CrossRef]
- Shannon, C.E. A Mathematical Theory of Communication. Bell Syst. Tech. J. 1948, 27, 623–656. [Google Scholar] [CrossRef]
- Mayr, A.; Rutzinger, M.; Bremer, M.; Oude Elberink, S.; Stumpf, F.; Geitner, C. Object-based classification of terrestrial laser scanning point clouds for landslide monitoring. Photogramm. Rec. 2017, 32, 377–397. [Google Scholar] [CrossRef]
- Dunham, L.; Wartman, J.; Olsen, M.J.; O’Banion, M.; Cunningham, K. Rockfall Activity Index (RAI): A lidar-derived, morphology-based method for hazard assessment. Eng. Geol. 2017, 221, 184–192. [Google Scholar] [CrossRef]
- Hutchinson, D.J.; Lato, M.; Gauthier, D.; Kromer, R.; Ondercin, M.; MacGowan, T.; Edwards, T. Rock Slope Monitoring and Risk Management for Railway Infrastructure in the White Canyon, British Columbia, Canada. In Engineering Geology for Society and Territory; Springer International Publishing: Cham, Switzerland, 2015; Volume 2, pp. 435–439. [Google Scholar]
- Besl, P.J.; McKay, N.D. A method for registration of 3-D shapes. IEEE Trans. Pattern Anal. Mach. Intell. 1992, 14, 239–256. [Google Scholar] [CrossRef]
- Wu, L.; Wang, Y.; Long, J.; Liu, Z. Image and Graphics; Zhang, Y.-J., Ed.; Lecture Notes in Computer Science; Springer International Publishing: Cham, Switzerland, 2015; Volume 9217, ISBN 9783319219776. [Google Scholar]
- Baatz, M.; Schape, A. Object-Oriented and Multi-Scale Image Analysis in Semantic Networks. In Proceedings of the 2nd International Symposium on Operationalization of Remote Sensing, ITC, Enschede, The Netherlands, 16–20 August 1999. [Google Scholar]
- Drǎguţ, L.; Csillik, O.; Eisank, C.; Tiede, D. Automated parameterisation for multi-scale image segmentation on multiple layers. ISPRS J. Photogramm. Remote Sens. 2014, 88, 119–127. [Google Scholar] [CrossRef]
- Lague, D.; Brodu, N.; Leroux, J. Accurate 3D comparison of complex topography with terrestrial laser scanner: Application to the Rangitikei canyon (N-Z). ISPRS J. Photogramm. Remote Sens. 2013, 82, 10–26. [Google Scholar] [CrossRef]
- Charles, R.Q.; Su, H.; Kaichun, M.; Guibas, L.J. PointNet: Deep Learning on Point Sets for 3D Classification and Segmentation. In Proceedings of the 2017 IEEE Conference on Computer Vision and Pattern Recognition (CVPR), Honolulu, HI, USA, 21–26 July 2017; IEEE: Piscataway Township, NJ, USA, 2017; pp. 77–85. [Google Scholar]
- Qi, C.R.; Yi, L.; Su, H.; Guibas, L.J. PointNet++: Deep Hierarchical Feature Learning on point sets in a metric space. arXiv 2017, arXiv:1706.02413, 5105–5114. [Google Scholar]
- Maturana, D.; Scherer, S. VoxNet: A 3D Convolutional Neural Network for Real-Time Object Recognition. In Proceedings of the 2015 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Hamburg, Germany, 28 September–2 October 2015; IEEE: Piscataway Township, NJ, USA, 2015; pp. 922–928. [Google Scholar]
- Ben-Shabat, Y.; Lindenbaum, M.; Fischer, A. 3DmFV: Three-Dimensional Point Cloud Classification in Real-Time Using Convolutional Neural Networks. IEEE Robot. Autom. Lett. 2018, 3, 3145–3152. [Google Scholar] [CrossRef]
- Hackel, T.; Savinov, N.; Ladicky, L.; Wegner, J.D.; Schindler, K.; Pollefeys, M. Semantic3D.Net: A New Large-Scale Point Cloud Classification Benchmark. In Proceedings of the ISPRS Annals of the Photogrammetry, Remote Sensing and Spatial Information Sciences, Melbourne, Australia, 26–27 October 2017; Volume 4, pp. 91–98. [Google Scholar]
- Hu, S.M.; Cai, J.X.; Lai, Y.K. Semantic labeling and instance segmentation of 3d point clouds using patch context analysis and multiscale processing. IEEE Trans. Vis. Comput. Graph. 2018, 14, 2485–2498. [Google Scholar] [CrossRef]
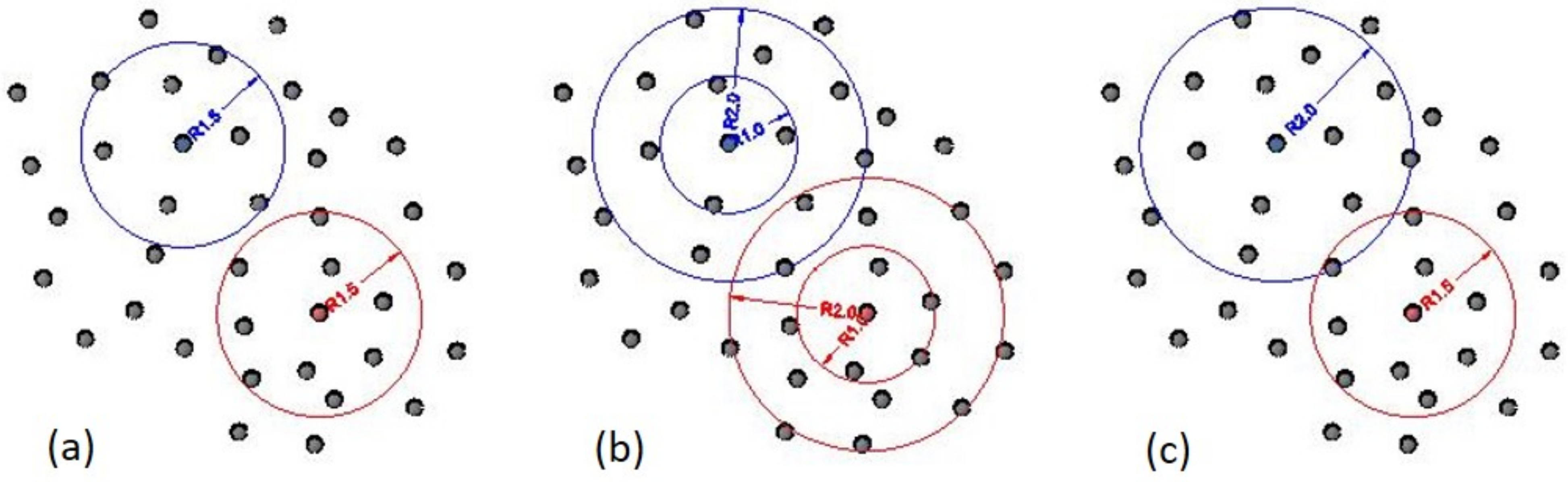
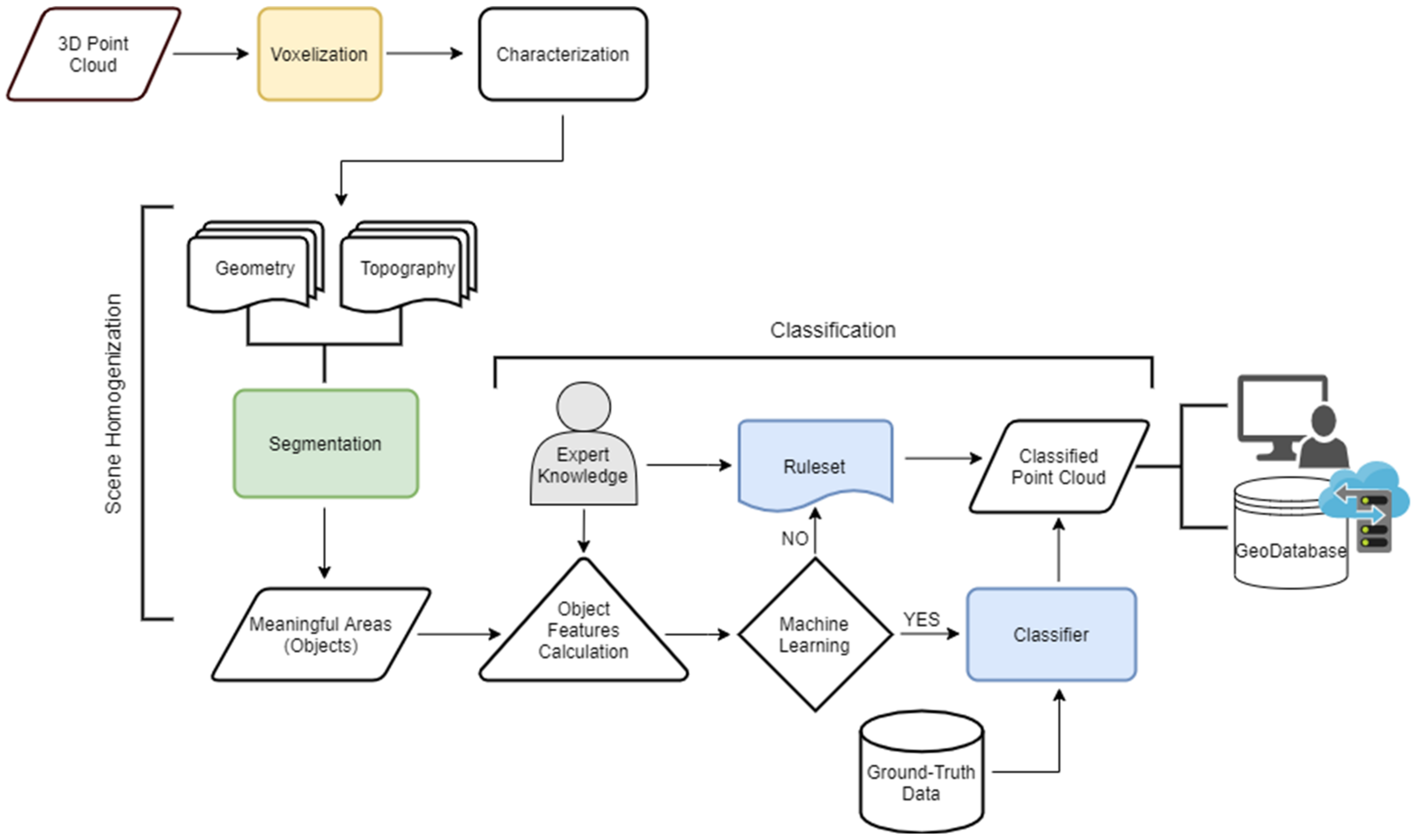
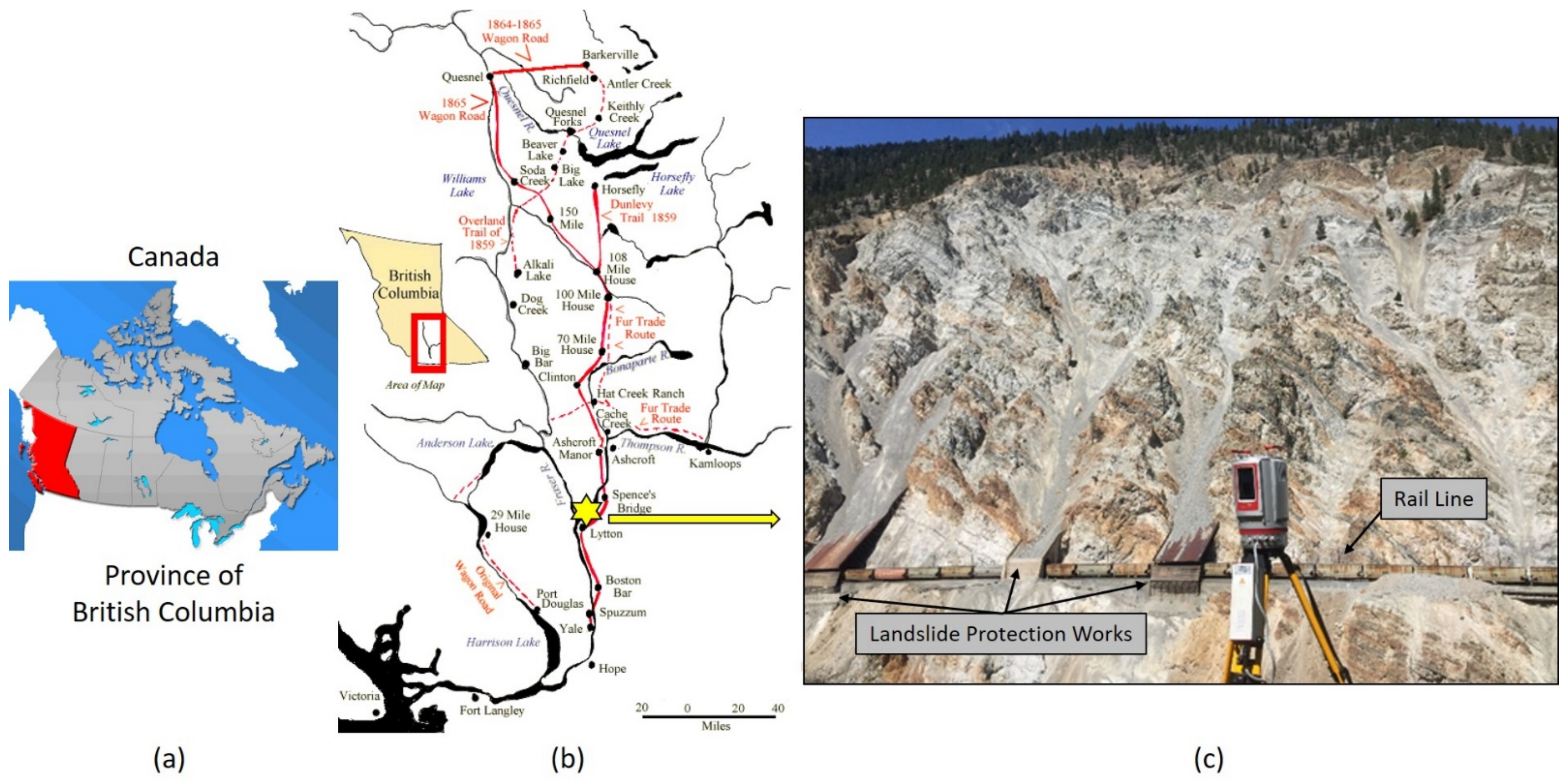
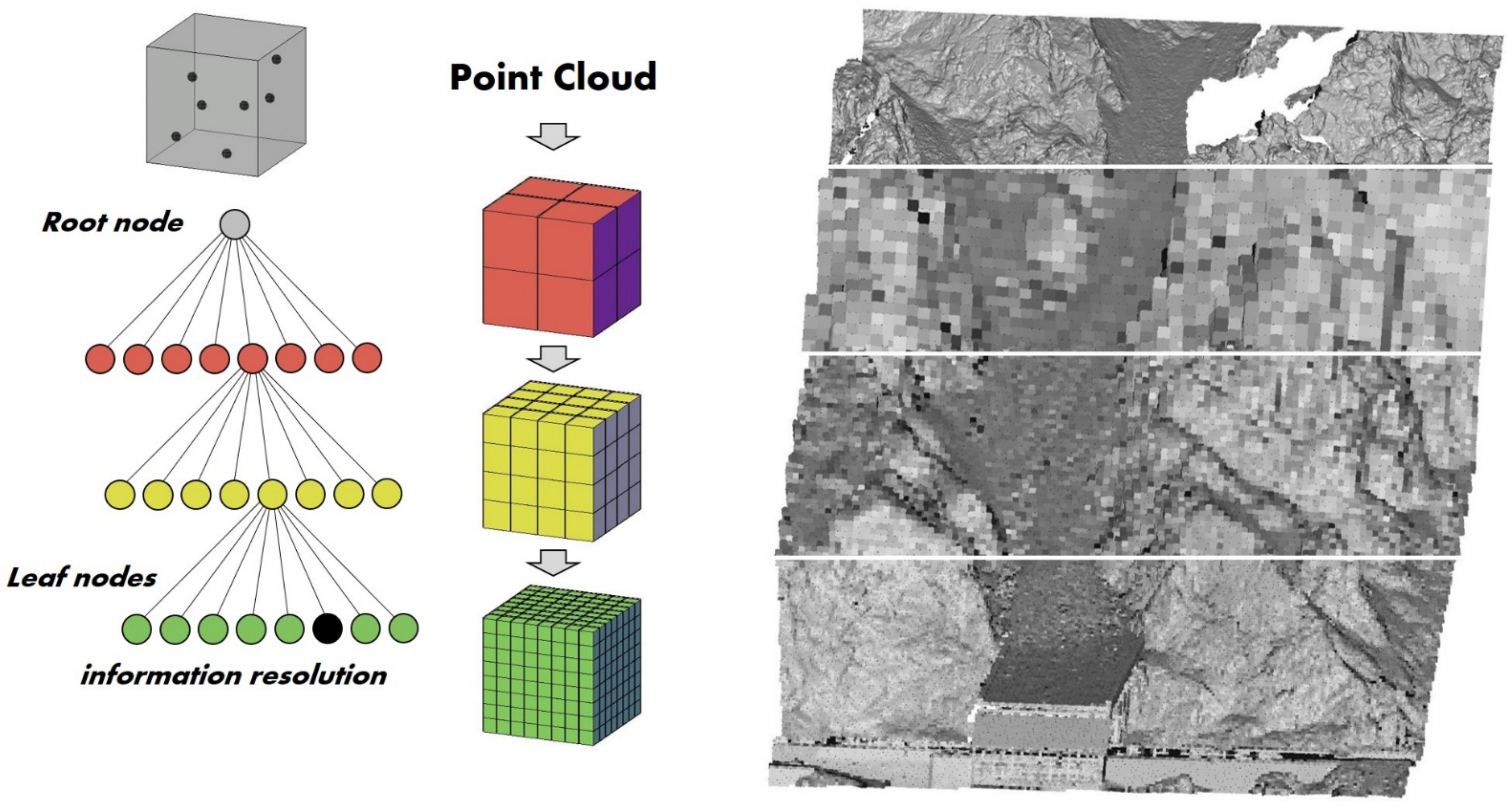


| Descriptor Name | Description | Purpose/Expert Reasoning |
|---|---|---|
| Slope | Dip angle between 0–90° to the horizontal plane. Is used to describe slope variations associated with the objects of interest. | Eroded areas such as debris channels usually retain lower slope angles than the surrounding rock mass. Constructed infrastructure is usually vertical or horizontal. |
| Aspect | Angle between 0–360° (North). Is used to describe the alignment of the various objects based on the azimuth. | Features such as rock benches formed from the bedrock structure are usually oriented perpendicular to the main slope dip direction. |
| Linearity | The difference of the two major axes of a 3D shape divided by the longest. | Transportation corridor structures appear to be more elongated than geological structures. |
| 2D compactness | Expresses how close to a square is a 3D shape projected to its best-fitting plane. | Constructed infrastructure appears to be more squared-shaped and platy than geological structures. |
| Relative adjacent class | Expresses the object based to its adjacent classes. | Topological rules used for refinement. |
| Relative elevation to class | Object’s position along Z-axis relative to other class objects. | Topological rules used for refinement. |
| Precision | Recall | F1-Score | |
|---|---|---|---|
| Steep outcrop | 0.99 | 0.95 | 0.97 |
| Debris channel | 0.91 | 0.98 | 0.95 |
| Constructed infrastructure | 0.95 | 0.94 | 0.94 |
| Rock bench/secondary channel | 0.51 | 0.84 | 0.64 |
| Model | Steep OutCrop | Debris Channel | Constructed Infrastructure | Rock Bench/ Secondary Channel |
|---|---|---|---|---|
| Multi-sized RF | 0.94 | 0.89 | 0.81 | 0.23 |
| Adjusted-sized RF | 0.83 | 0.52 | 0.58 | 0.03 |
| Knowledge-based | 0.97 | 0.95 | 0.94 | 0.64 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Farmakis, I.; Bonneau, D.; Hutchinson, D.J.; Vlachopoulos, N. Targeted Rock Slope Assessment Using Voxels and Object-Oriented Classification. Remote Sens. 2021, 13, 1354. https://doi.org/10.3390/rs13071354
Farmakis I, Bonneau D, Hutchinson DJ, Vlachopoulos N. Targeted Rock Slope Assessment Using Voxels and Object-Oriented Classification. Remote Sensing. 2021; 13(7):1354. https://doi.org/10.3390/rs13071354
Chicago/Turabian StyleFarmakis, Ioannis, David Bonneau, D. Jean Hutchinson, and Nicholas Vlachopoulos. 2021. "Targeted Rock Slope Assessment Using Voxels and Object-Oriented Classification" Remote Sensing 13, no. 7: 1354. https://doi.org/10.3390/rs13071354
APA StyleFarmakis, I., Bonneau, D., Hutchinson, D. J., & Vlachopoulos, N. (2021). Targeted Rock Slope Assessment Using Voxels and Object-Oriented Classification. Remote Sensing, 13(7), 1354. https://doi.org/10.3390/rs13071354






