Self-Secondaries Formed by Cold Spot Craters on the Moon
Abstract
1. Introduction
2. Materials and Methods
3. Results
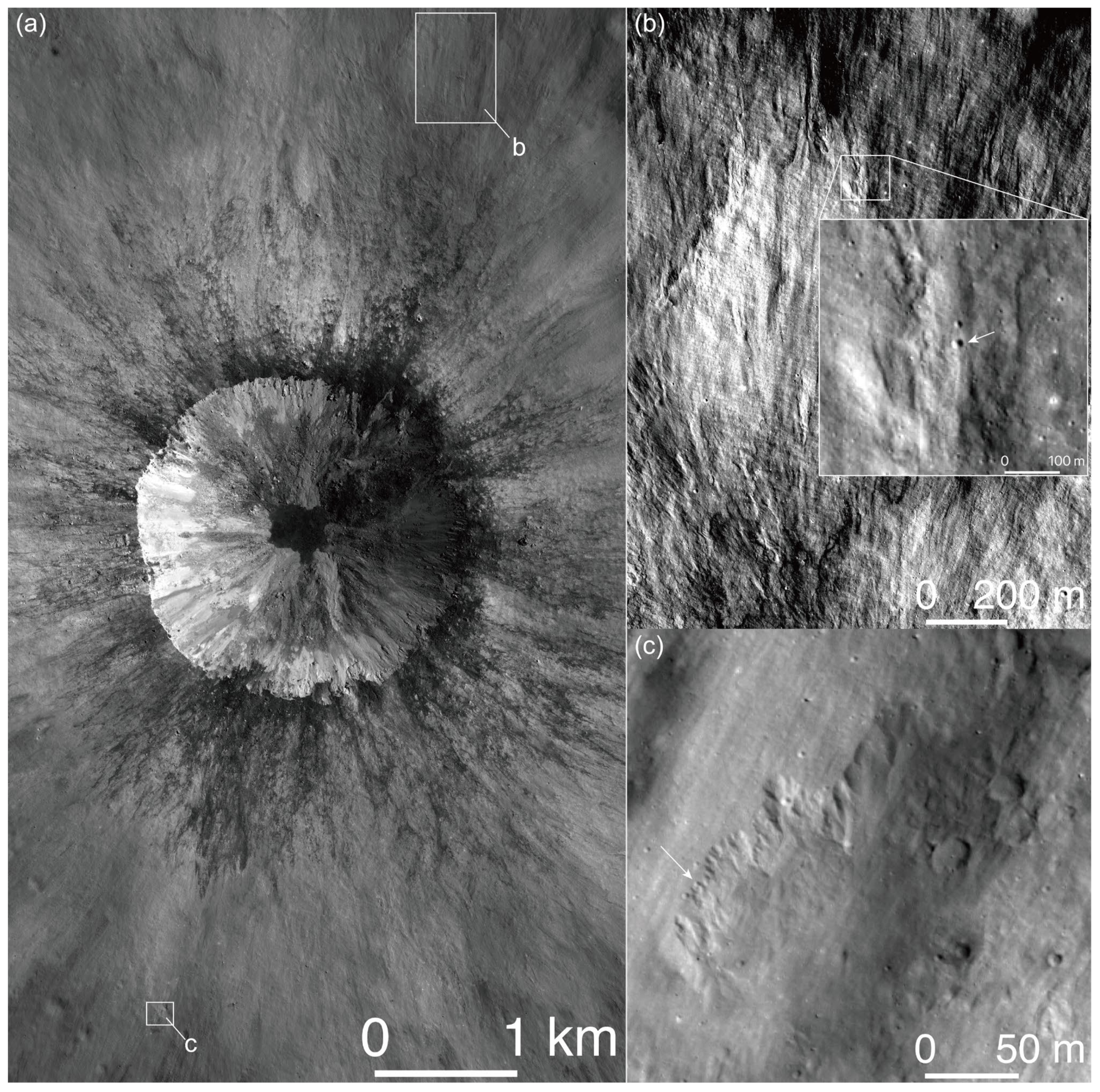
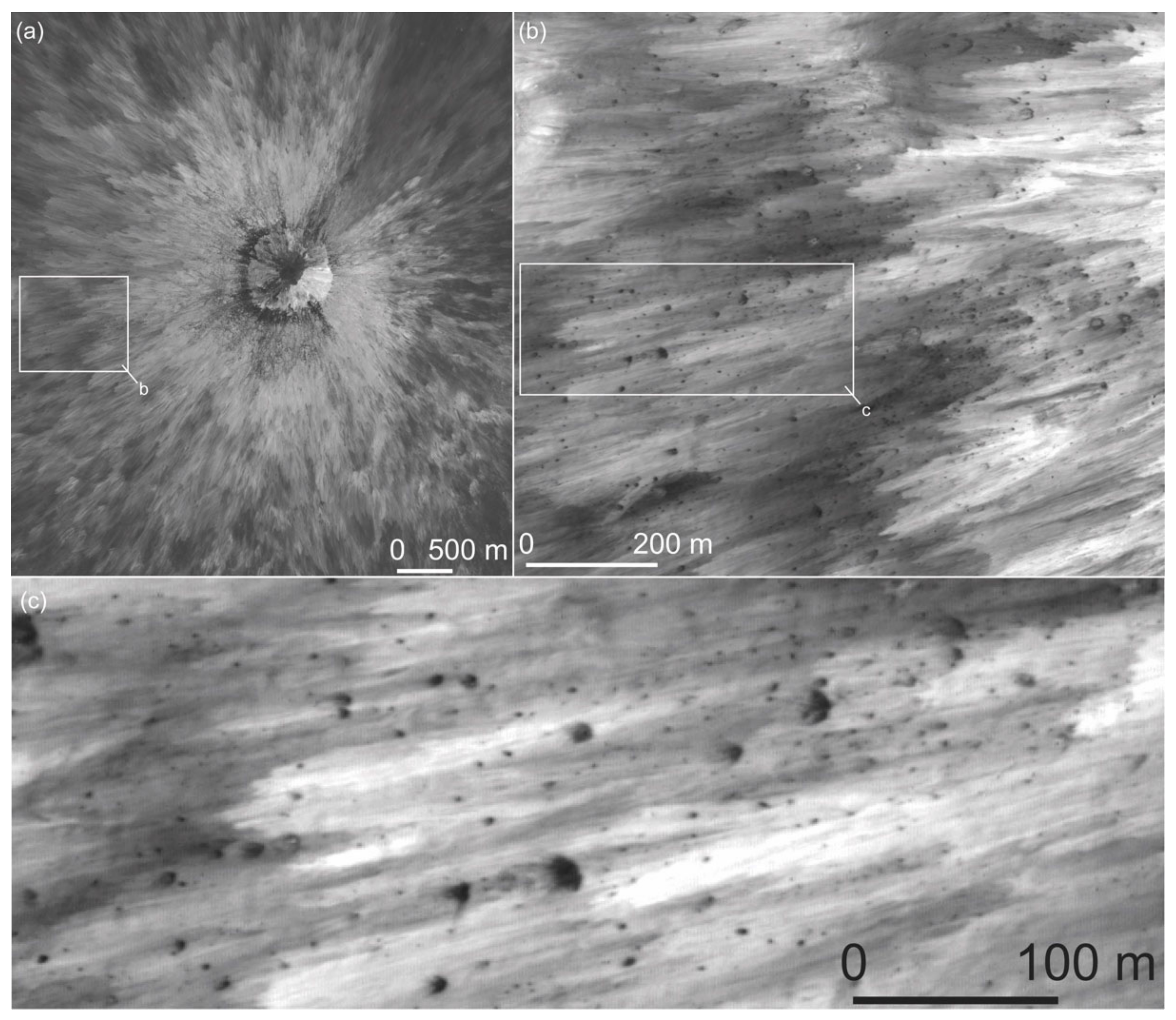
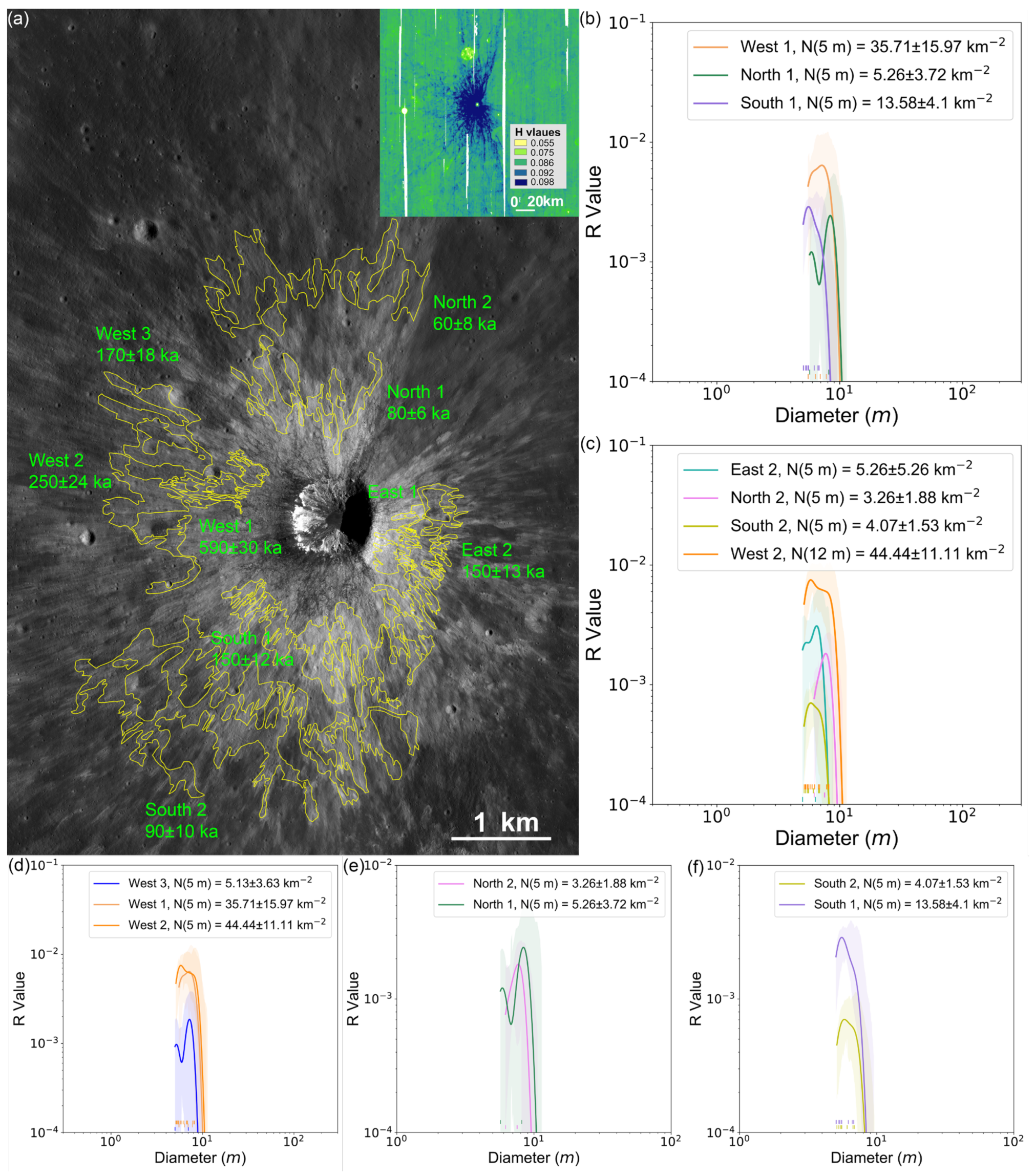
3.1. Morphological Evidence of Self-Secondaries around Cold Spot Craters
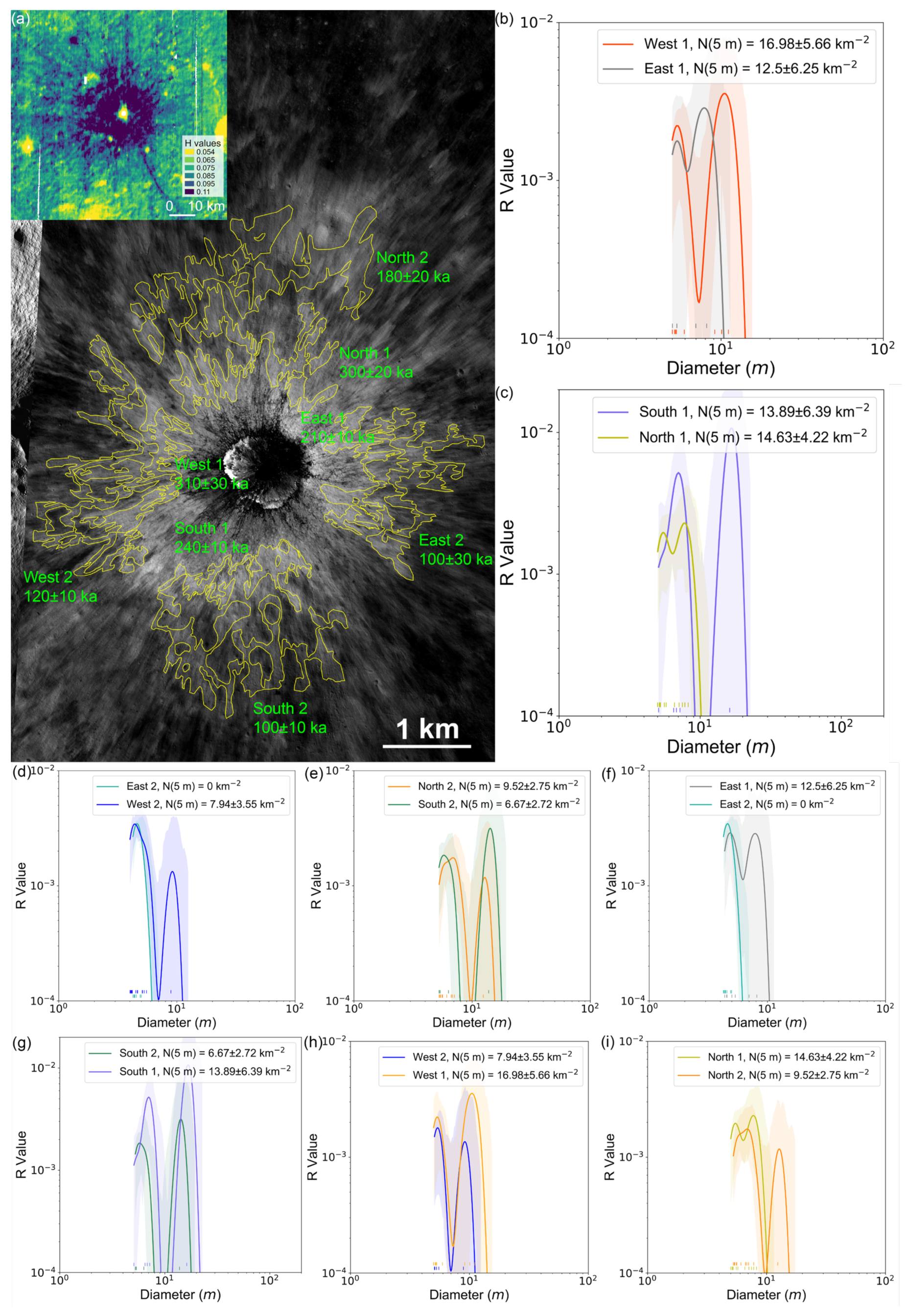
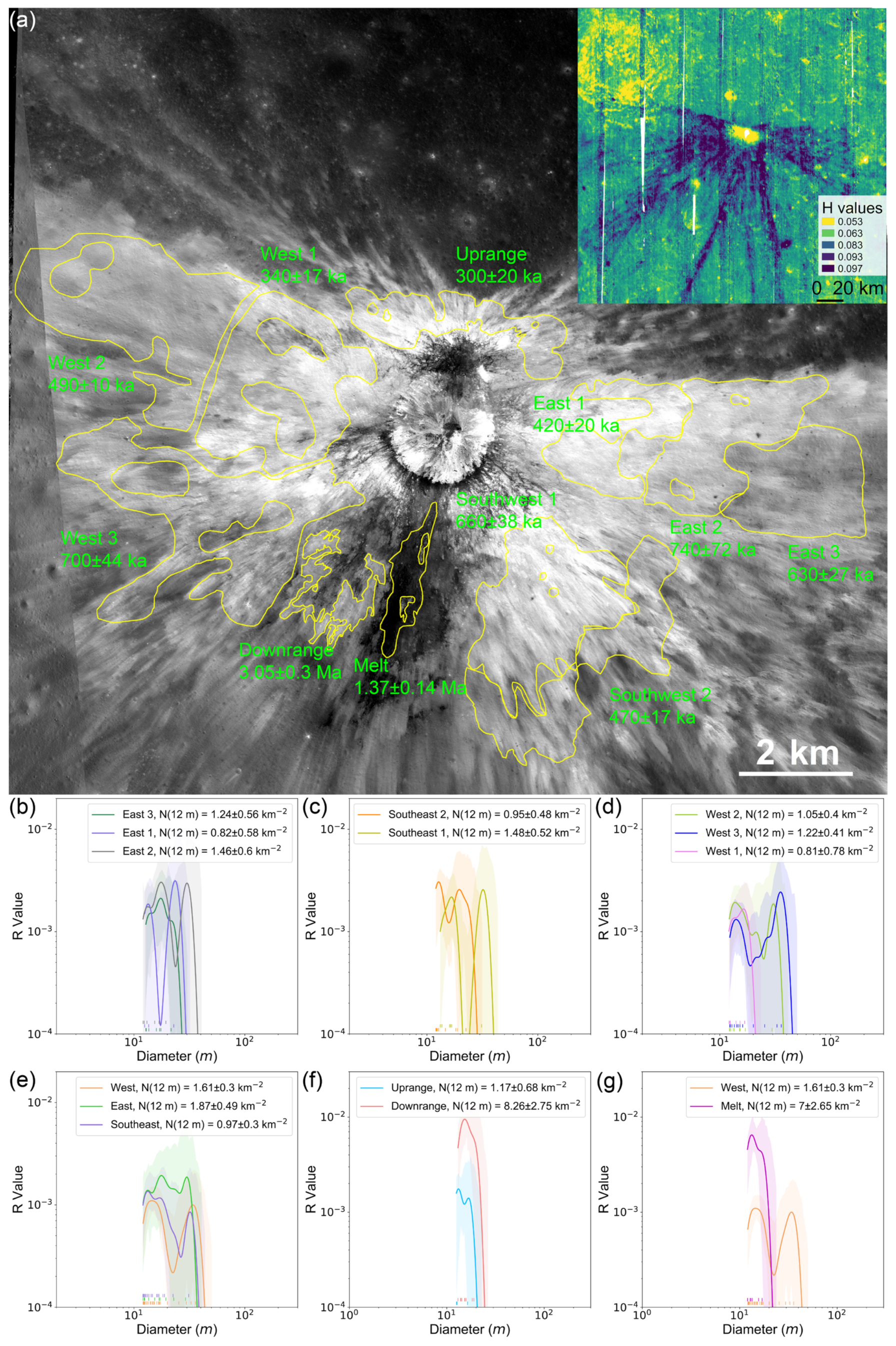
3.2. Heterogeneous Distribution of Self-Secondaries
3.3. Size-Frequency Distribution (SFD) of Self-Secondaries
4. Discussion
4.1. Formation Mechanism of Self-Secondaries
4.2. Production SFD of Self-Secondaries and Its Effect on Lunar Crater Chronology
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Melosh, H.J. Impact Cratering: A Geologic Process; Oxford University: New York, NY, USA, 1989; pp. 1–255. [Google Scholar]
- Werner, S.C.; Ivanov, B.A. Exogenic dynamics, cratering, and surface ages. In Treatise on Geophysics, 2nd ed.; Elsevier: Amsterdam, The Netherlands, 2015; Volume 10, pp. 327–365. [Google Scholar] [CrossRef]
- Fassett, C.I. Analysis of impact crater populations and the geochronology of planetary surfaces in the inner solar system. J. Geophys. Res. Planets 2016, 121, 1900–1926. [Google Scholar] [CrossRef]
- Hartmann, W.K. Terrestrial and lunar flux of meteorites in the last two billion years. Icarus 1965, 4, 157–165. [Google Scholar] [CrossRef]
- Neukum, G. Meteoritenbombardement and Datierung Planetarer Oberflächen. In Habilitation Dissertation for Faculty Membership; University of Munich: Munich, Germany, 1983. [Google Scholar]
- Stöffler, D.; Ryder, G. Stratigraphy and isotope ages of lunar geologic units: Chronological standard for the inner solar system. Space Sci. Rev. 2001, 96, 9–54. [Google Scholar] [CrossRef]
- Strom, R.G.; Malhotra, R.; Takashi, I.; Yoshida, F.; Kring, D.A. The origin of planetary impactors in the inner solar system. Science 2005, 309, 1847–1850. [Google Scholar] [CrossRef] [PubMed]
- Neukum, G.; Ivanov, B.A.; Hartmann, W.K. Cratering records in the inner solar system in relation to the lunar reference system. Space Sci. Rev. 2001, 96, 55–86. [Google Scholar] [CrossRef]
- Robbins, S.J. A new crater calibrations for the lunar crater-age chronology. Earth Planet. Sci. Lett. 2014, 403, 188–198. [Google Scholar] [CrossRef]
- Qian, Y.; Xiao, L.; Head, J.W.; van der Bogert, C.; Hiesinger, H.; Wilson, L. Young lunar mare basalts in the Chang’e-5 sample return region, northern Oceanus Procellarum. Earth Planet. Sci. Lett. 2021, 555, 116702. [Google Scholar] [CrossRef]
- McEwen, A.S.; Bierhaus, E.B. The importance of secondary cratering to age constraints on planetary surfaces. Annu. Rev. Earth Planet. Sci. 2005, 34, 535–567. [Google Scholar] [CrossRef]
- Bierhaus, E.B.; McEwen, A.S.; Robbins, S.J.; Singer, K.N.; Dones, L.; Kirchoff, M.R.; Williams, J.P. Secondary craters and ejecta across the solar system: Populations and effects on impact-crater based chronologies. Meteorit. Planet. Sci. 2018, 53, 638–671. [Google Scholar] [CrossRef]
- Shoemaker, E.M. Preliminary analysis of the fine structure of the lunar surface in Mare Cognitum. In The Nature of the Lunar Surface; Johns Hopkins Press: Baltimore, MD, USA, 1966; pp. 75–134. [Google Scholar]
- Xiao, Z. On the importance of self-secondaries. Geosci. Lett. 2018, 5, 17. [Google Scholar] [CrossRef]
- Oberbeck, V.R. The role of ballistic erosion and sedimentation in lunar stratigraphy. Rev. Geophys. Space Phys. 1975, 13, 337–362. [Google Scholar] [CrossRef]
- Schultz, P.H.; Gault, D.E. Clustered impacts: Experiments and implications. J. Geophys Res. 1985, 90, 3701–3732. [Google Scholar] [CrossRef]
- Speyerer, E.J.; Povilaitis, R.Z.; Robinson, M.S.; Thomas, P.C.; Wagner, R.V. Quantifying crater production and regolith overturn on the Moon with temporal imaging. Nature 2016, 538, 215–218. [Google Scholar] [CrossRef]
- Xiao, Z. Size-frequency distribution of different secondary crater populations: 1. Equilibrium caused by secondary impacts. J. Geophys. Res. Planets 2016, 121, 2404–2425. [Google Scholar] [CrossRef]
- Shoemaker, E.M.; Batson, R.M.; Holt, H.E.; Morris, E.C.; Rennilson, J.J.; Whitaker, E.A. Observations of the lunar regolith and the Earth from the television camera on Surveyor 7. J. Geophys. Res. 1969, 74, 6081–6119. [Google Scholar] [CrossRef]
- Plescia, J.B.; Robinson, M.S. New constraints on the absolute lunar crater chronology. In Proceedings of the 42nd Lunar & Planetary Science Conference, The Woodlands, TX, USA, 7–11 March 2011. [Google Scholar]
- Xiao, Z.; Werner, S.C. Size-frequency distribution of crater populations in equilibrium on the Moon. J. Geophys. Res. Planets 2015, 120, 2277–2292. [Google Scholar] [CrossRef]
- van der Bogert, C.H.; Hiesinger, H.; Dundas, C.M.; Kruger, T.; McEwen, A.S.; Zanetti, M.; Robinson, M.S. Origin of discrepancies between crater size-frequency distributions of coeval lunar geologic units via target property contrasts. Icarus 2017, 298, 49–63. [Google Scholar] [CrossRef]
- Zanetti, M.; Stadermann, A.; Joliff, B.; Hiesinger, H.; van der Bogert, C.H.; Plescia, J.B. Evidence for self-secondary cratering of Copernican-age continuous ejecta deposits on the Moon. Icarus 2017, 298, 64–77. [Google Scholar] [CrossRef]
- Williams, J.; van der Bogert, C.H.; Pathare, A.V.; Michael, G.G.; Kirchoff, M.R.; Hiesinger, H. Dating very young planetary surfaces from crater statistics: A review of issues and challenges. Meteorit. Planet Sci. 2017, 53, 554–582. [Google Scholar] [CrossRef]
- Xiao, Z.; Prieur, N.C.; Werner, S.C. The self-secondary crater population of the Hokusai crater on Mercury. Geophys. Res. Lett. 2016, 43, 7424–7432. [Google Scholar] [CrossRef]
- Boyce, J.M.; Mouginismark, P.J. Anomalous areas of high crater density on the rim of the Martian crater Tooting. In Proceedings of the Workshop on Issues in Crater Studies and the Dating of Planetary Surfaces, Laurel, MD, USA, 19–22 May 2015; pp. 19–22. [Google Scholar]
- Neesemann, A.; Gasselt, S.V.; Schmedemann, N.; Marchi, S.; Walter, S.; Preusker, F.; Michael, G.G.; Kneissl, T.; Hiesinger, H.; Jaumann, R.; et al. The various ages of Occator crater, Ceres: Results of a comprehensive synthesis approach. Icarus 2019, 320, 60–82. [Google Scholar] [CrossRef]
- Schenk, P.; Kirchoff, M.; Hoogenboom, T.; Rivera-Valentin, E. The anatomy of fresh complex craters on the mid-sized icy moons of Saturn and self-secondary cratering at the rayed crater Inktomi (Rhea). Meteorit. Planet. Sci. 2020, 55, 2440–2460. [Google Scholar] [CrossRef]
- Plescia, J.B.; Robinson, M.S. Lunar self-secondary cratering: Implications for cratering and chronology. Proc. Lunar Planet. Sci. 2015, 46, 16–20. [Google Scholar]
- Plescia, J.B.; Robbins, M.S. Giordano Bruno: Small crater populations––Implications for self-secondary cratering. Icarus 2019, 321, 974–993. [Google Scholar] [CrossRef]
- Mendell, W.W.; Low, F.J. Preliminary results of the Apollo 17 infrared scanning radiometer. Moon 1974, 9, 97–103. [Google Scholar] [CrossRef]
- Bandfield, J.L.; Ghent, R.R.; Vasavada, A.R.; Paige, D.A.; Lawrence, S.J.; Robinson, M.S. Lunar surface rock abundance and regolith fines temperatures derived from LRO Diviner radiometer data. J. Geophys. Res. 2011, 116, E00H02. [Google Scholar] [CrossRef]
- Paige, D.A.; Foote, M.C.; Greenhagen, B.T.; Schofield, J.T.; Calcutt, S.; Vasavada, D.J.; Preston, A.R.; Taylor, F.W.; Allen, C.C.; Snook, K.J.; et al. The Lunar Reconnaissance Orbiter Diviner Lunar Radiometer Experiment. Space Sci. Rev. 2010, 150, 125–160. [Google Scholar] [CrossRef]
- Hayne, P.O.; Bandfield, J.L.; Siegler, M.A.; Vasavada, A.R.; Ghent, R.R.; Williams, J.P.; Greenhagen, B.T.; Aharonson, O.; Elder, C.M.; Lucey, P.G.; et al. Global regolith thermophysical properties of the Moon from the Diviner Lunar Radiometer Experiment. J. Geophys. Res. 2017, 122, 2371–2400. [Google Scholar] [CrossRef]
- Bandfield, J.L.; Song, E.; Hayne, P.O.; Brand, B.D.; Ghent, R.R.; Vasavada, A.R.; Paige, D.A. Lunar cold spots: Granular flow features and extensive insulating materials surrounding young craters. Icarus 2014, 231, 221–231. [Google Scholar] [CrossRef]
- Powell, T.M.; Greenhagen, B.T.; Taylor, S.; Williams, J.P.; Hayne, P.O.; Paige, D.A. Lunar cold spot properties and degradation. In Proceedings of the Lunar and Planetary Science Conference, The Woodlands, TX, USA, 19–23 March 2018; p. 49. [Google Scholar]
- Williams, J.P.; Bandfield, J.L.; Paige, D.A.; Powell, T.; Greenhagen, B.T.; Taylor, S.; Hayne, P.O.; Speyerer, E.J.; Ghent, R.R.; Costello, E.S. Lunar cold spots and crater production on the Moon. J. Geophys. Res. Planets 2018, 123, 2380–2392. [Google Scholar] [CrossRef]
- Robinson, M.S.; Brylow, S.M.; Tschimmel, M.; Humm, D.; Lawrence, S.J.; Thomas, P.C.; Denevi, B.W.; Bowman-Cisneros, E.; Zerr, J.; Ravine, M.A.; et al. Lunar Reconnaissance Orbiter Camera (LROC) instrument overview. Space Sci. Rev. 2010, 150, 81–124. [Google Scholar] [CrossRef]
- Becker, K.J.; Anderson, J.A.; Weller, L.A.; Becker, T.L. ISIS Support for NASA Mission Instrument Ground Data Processing Systems. In Proceedings of the 44th Lunar and Planetary Science Conference, The Woodlands, TX, USA, 18–22 March 2013. [Google Scholar]
- Plescia, J.B.; Robinson, M.S.; Paige, D.A. Giordano Bruno: The young and the restless. In Proceedings of the 41st Lunar and Planetary Science Conference, The Woodlands, TX, USA, 1–5 March 2010; p. 41. [Google Scholar]
- Antonenko, I.; Robbins, S.J.; Gay, P.L.; Lehan, C.; Moore, J. Effects of incidence angle on crater detection and the lunar isochron system: Preliminary results from the cosmoquest moonmappers citizen science project. In Proceedings of the Lunar and Planetary Science Conference, The Woodlands, TX, USA, 18–22 March 2013; Volume 44, p. 2705. [Google Scholar]
- Ostrach, L.R.; Robinson, M.S.; Denevi, B.W.; Thomas, P.C. Effects of incidence angle on crater counting observations. In Proceedings of the 42nd Lunar and Planetary Science Conference, The Woodlands, TX, USA, 7–11 March 2011; p. 42. [Google Scholar]
- Kneissl, T.; van Gasselt, S.; Neukum, G. Map-projection-independent crater size–frequency determination in GIS environments—New software tool for ArcGIS. Planet. Space Sci. 2010, 59, 1243–1254. [Google Scholar] [CrossRef]
- Wang, Y.C.; Xie, M.G.; Xiao, Z.Y.; Cui, J. The minimum confidence limit for diameters in crater counts. Icarus 2020, 341, 113645. [Google Scholar] [CrossRef]
- Vasavada, A.R.; Bandfield, J.L.; Greenhagen, B.T.; Hayne, P.O.; Siegler, M.A.; Williams, J.P.; Paige, D.A. Lunar equatorial surface temperatures and regolith properties from the Diviner Lunar Radiometer Experiment. J. Geophys. Res. 2012, 117. [Google Scholar] [CrossRef]
- Robbins, S.J.; Riggs, J.D.; Weaver, B.P.; Bierhaus, E.B.; Chapman, C.R.; Kirchoff, M.R.; Singer, K.N.; Gaddis, L.R. Revised recommended methods for analyzing crater size-frequency distributions. Meteorit. Planet. Sci. 2018, 53, 891–931. [Google Scholar] [CrossRef]
- Crater Analysis Techniques Working Group; Arvidson, R.; Boyce, J.; Chapman, C.; Cintala, M.; Fulchignoni, M.; Moore, H.; Neukum, G.; Schultz, P.; Soderblom, L.; et al. Standard techniques for presentation and analysis of crater size–frequency data. Icarus 1979, 37, 467–474. [Google Scholar] [CrossRef]
- Williams, J.P.; Pathare, A.V.; Aharonson, O. The production of small primary craters on Mars and the Moon. Icarus 2014, 235, 23–36. [Google Scholar] [CrossRef]
- Denevi, B.W.; Koeber, S.D.; Robinson, M.S.; Garry, W.B.; Hawke, B.R.; Tran, T.N.; Lawrence, S.J.; Keszthelyi, L.P.; Barnouin, O.S.; Ernst, C.M.; et al. Physical constraints on impact melt properties from Lunar Reconnaissance Orbiter Camera images. Icarus 2012, 219, 665–675. [Google Scholar] [CrossRef]
- Ding, C.; Li, C.; Xiao, Z.; Su, Y.; Xing, S.; Wang, Y.; Feng, J.; Xiao, S.D.Y.; Yao, M. Layering structures in the porous material beneath the Chang’e-3 landing site. Earth Space Sci. 2020, 7, e2019EA000862. [Google Scholar] [CrossRef]
- Xiao, Z.; Ding, C.; Xie, M.; Cai, Y.; Cui, J.; Zhang, K.; Wang, J. Ejecta from the orientale basin at the Chang’E-4 landing site. Geophys. Res. Lett. 2021, 48, e2020GL090935. [Google Scholar] [CrossRef]
- Osinski, G.R.; Tornabene, L.L.; Grieve, R.A.F. Impact ejecta emplacement on terrestrial planets. Earth Planet. Sci. Lett. 2011, 310, 167–181. [Google Scholar] [CrossRef]
- Xiao, Z.; Zeng, Z.; Li, Z.; Blair, D.M.; Xiao, L. Cooling fractures in impact melt deposits on the Moon and Mercury: Implications for cooling solely by thermal radiation. J. Geophys. Res. Planets 2014, 119, 1496–1515. [Google Scholar] [CrossRef]
- Bray, V.J.; Atwood-Stone, C.; Neish, C.D.; Artemieva, N.A.; McEwen, A.S.; Mcelwaine, J.N. Lobate impact melt flows within the extended ejecta blanket of Pierazzo crater. Icarus 2018, 301, 26–36. [Google Scholar] [CrossRef]
- Pike, R.J. Depth/diameter relations of fresh lunar craters: Revision from spacecraft data. Geophys. Res. Lett. 1974, 1, 291–294. [Google Scholar] [CrossRef]
- Gault, D.E.; Wedekind, J.A. Experimental studies of oblique impact. Lunar Planet. Sci. 1978, 9, 3843–3875. [Google Scholar]
- Herrick, R.R.; Forsberg-Taylor, N.K. The shape and appearance of craters formed by oblique impact on the Moon and Venus. Meteorit. Planet. Sci. 2003, 38, 1551–1578. [Google Scholar] [CrossRef]
- Maxwell, D.E. Simple Z model of cratering, ejection, and the overturned flap. In Impact and Explosion Cratering; Pergamon Press: New York, NY, USA, 1977; pp. 1003–1008. [Google Scholar]
- Polanskey, C.A.; Ahrens, T.J. Impact spallation experiments: Fracture patterns and spall velocities. Icarus 1990, 87, 140–155. [Google Scholar] [CrossRef]
- Kurosawa, K.; Okamoto, T.; Genda, H. Hydrocode modeling of the spallation process during hypervelocity impacts: Implications for the ejection of Martian meteorites. Icarus 2018, 301, 219–234. [Google Scholar] [CrossRef]
- Xiao, Z.; Strom, R.G. Problems determining relative and absolute ages using the small crater population. Icarus 2012, 220, 254–267. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chang, Y.; Xiao, Z.; Liu, Y.; Cui, J. Self-Secondaries Formed by Cold Spot Craters on the Moon. Remote Sens. 2021, 13, 1087. https://doi.org/10.3390/rs13061087
Chang Y, Xiao Z, Liu Y, Cui J. Self-Secondaries Formed by Cold Spot Craters on the Moon. Remote Sensing. 2021; 13(6):1087. https://doi.org/10.3390/rs13061087
Chicago/Turabian StyleChang, Yiren, Zhiyong Xiao, Yang Liu, and Jun Cui. 2021. "Self-Secondaries Formed by Cold Spot Craters on the Moon" Remote Sensing 13, no. 6: 1087. https://doi.org/10.3390/rs13061087
APA StyleChang, Y., Xiao, Z., Liu, Y., & Cui, J. (2021). Self-Secondaries Formed by Cold Spot Craters on the Moon. Remote Sensing, 13(6), 1087. https://doi.org/10.3390/rs13061087







