An Advanced Phase Synchronization Scheme Based on Coherent Integration and Waveform Diversity for Bistatic SAR
Abstract
:1. Introduction
2. Materials and Methods
2.1. Oscillator Phase Error Model
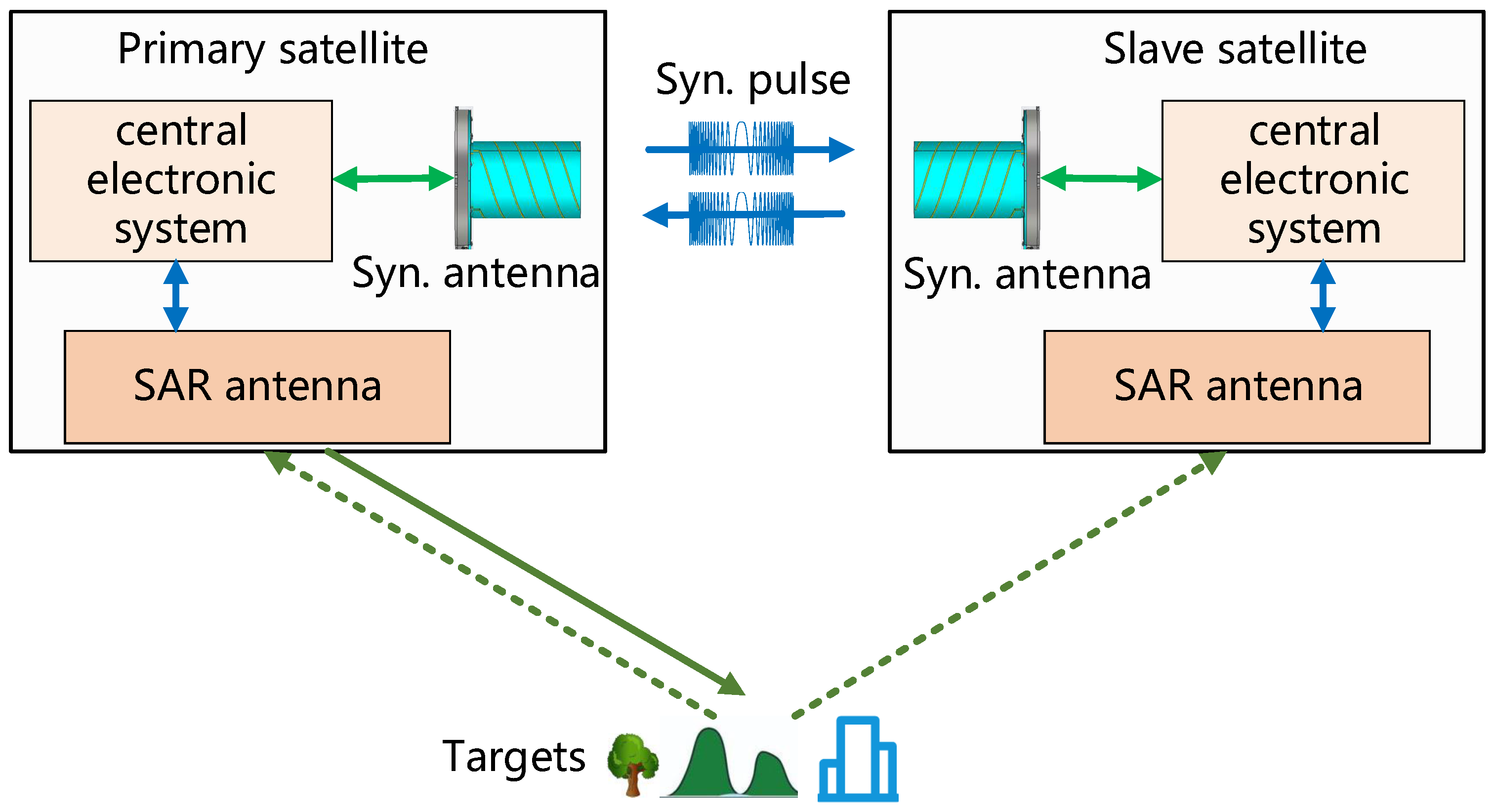
2.2. Principle of Phase Synchronization
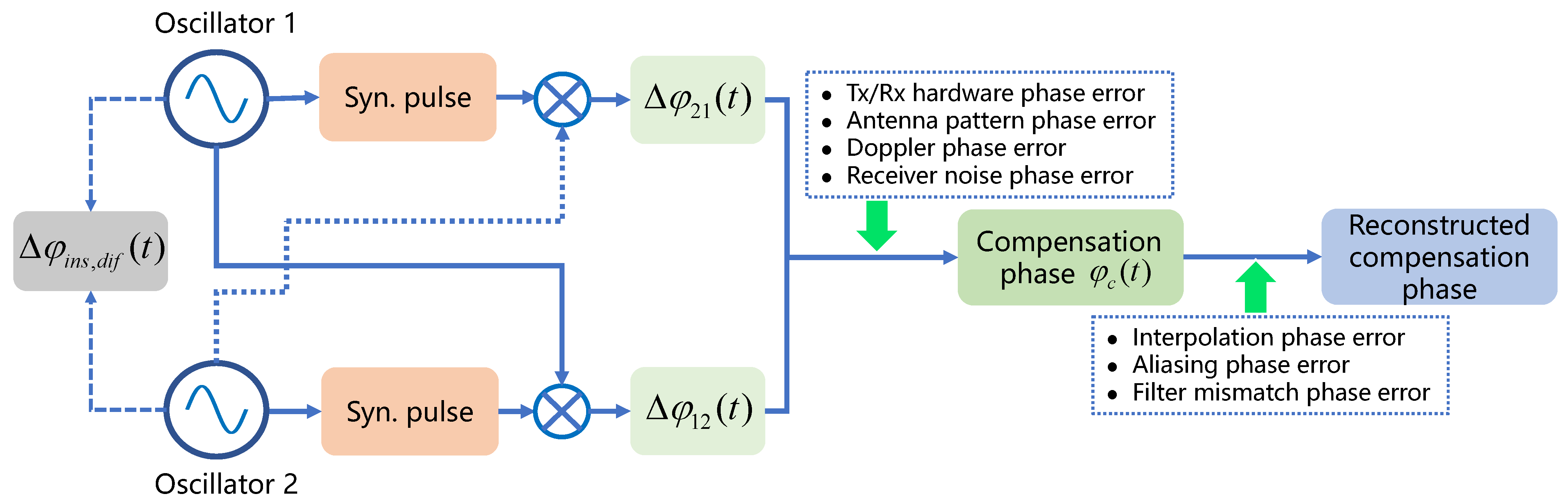
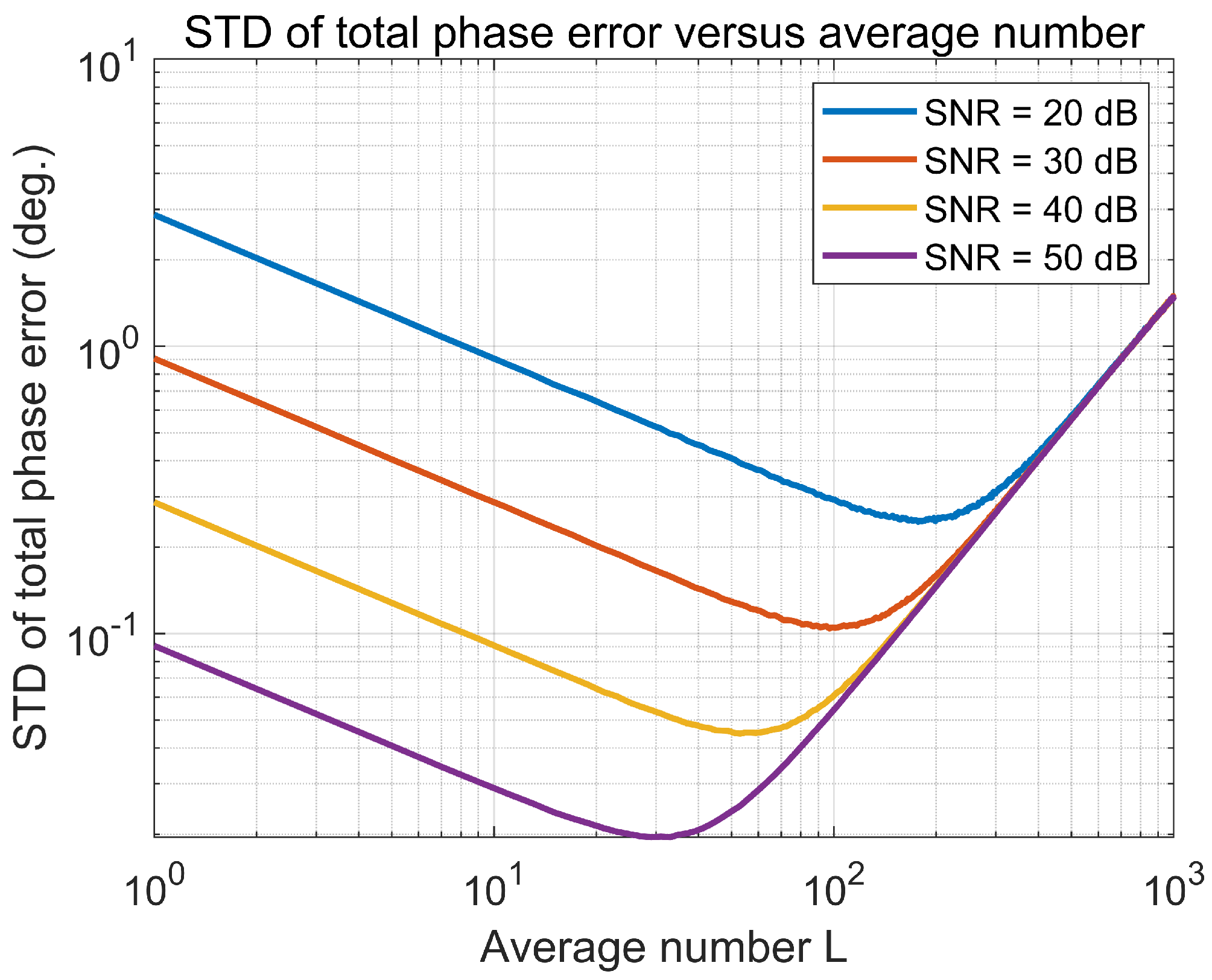
2.3. Error Analysis of Phase Synchronization
2.4. Advanced Synchronization Scheme
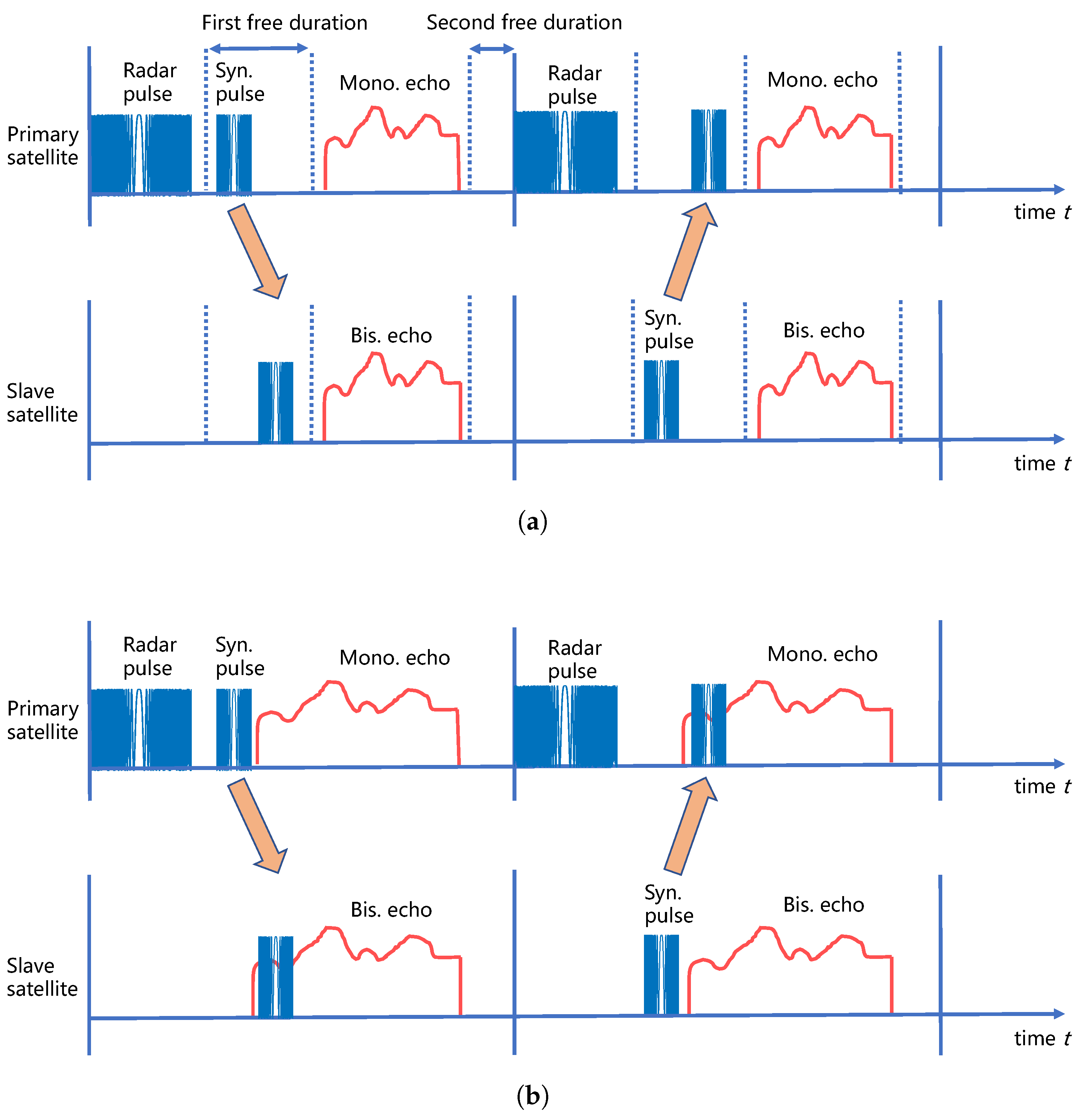
2.4.1. Timing Diagram
2.4.2. Waveform Diversity
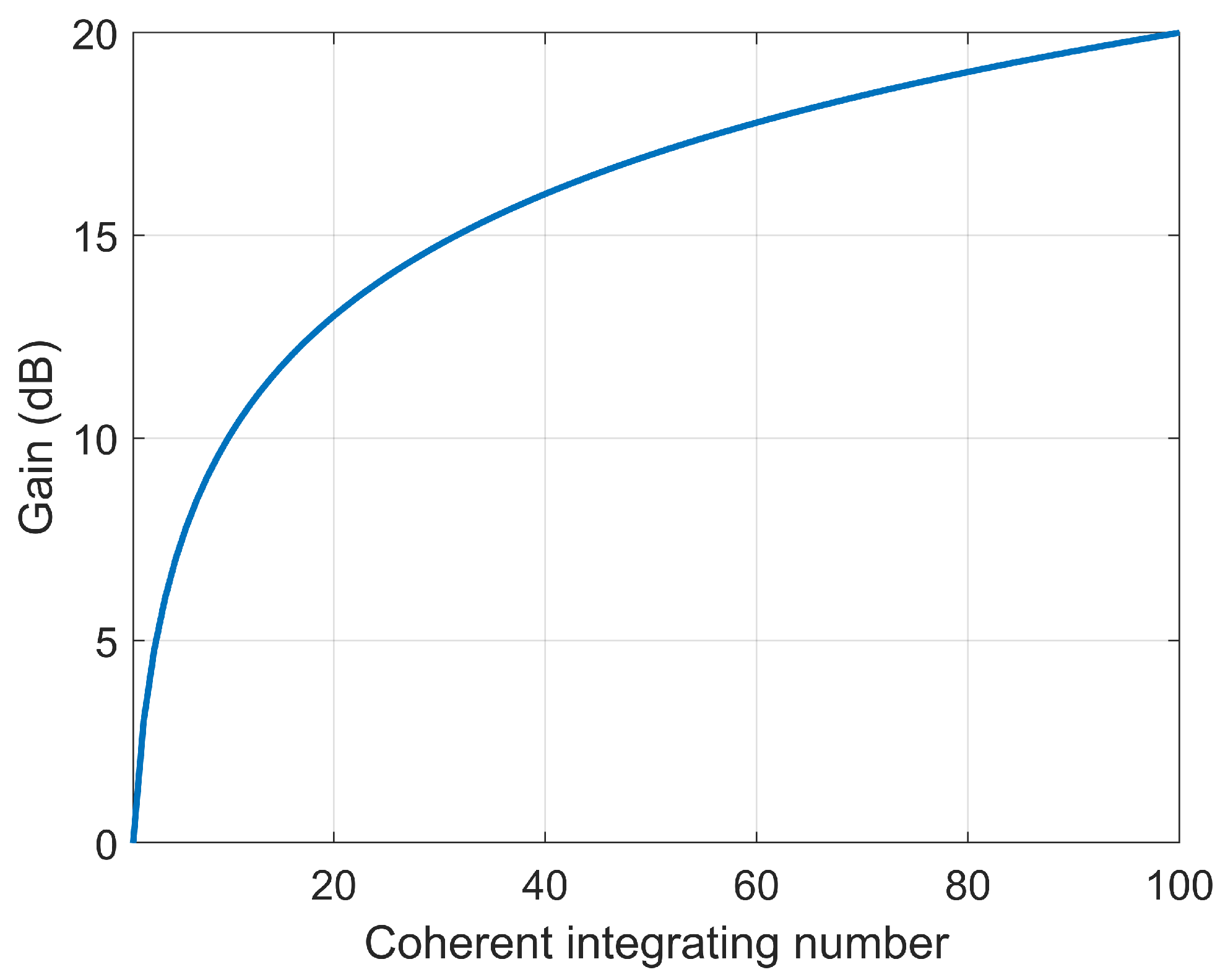
2.4.3. Coherent Integration
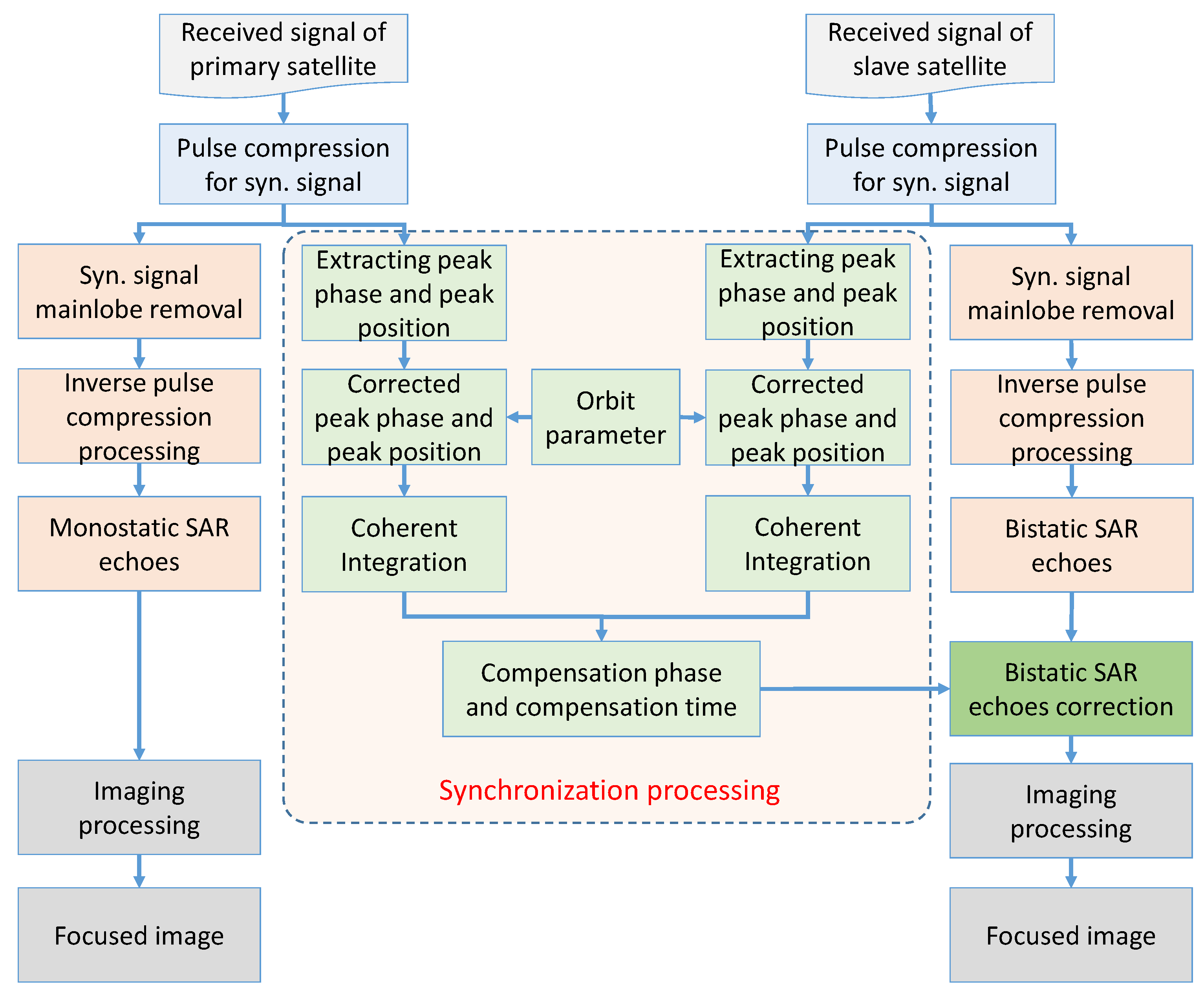
2.5. Processing Flowchart
2.5.1. Processing for Extracting the Synchronization Signal
- step1
- Pulse compression for synchronization signal. The received signal is compressed using a filter “matched” to the synchronization signal.
- step2
- Extract peak phase and peak position.
- step3
- Correction by orbit parameters. The Doppler effect and relativistic effect should be corrected according to the orbit parameters.
- step4
- Coherent Integration. The coherent integration technique is used to improve the SNR of synchronization signal.
- step5
- Obtain compensation phase and compensation time. The compensation time can be obtained by compensation phase and corrected peak position.
- step6
- Compensation for bistatic SAR echoes.
2.5.2. Processing for Extracting the Echoes
- step1
- Pulse compression for synchronization signal. The received signal is compressed using a filter “matched” to the synchronization signal.
- step2
- Remove the synchronization signal. The synchronization signal can be removed by blanking the pixels where there is the mainlobe of compressed synchronization signal.
- step3
- Inverse pulse compression processing. The data is processed by the inverse filter “matched” to the synchronization signal. After that, the data is transformed back into raw data, which can be regarded as the SAR echoes.
- step4
- Synchronization compensation. The compensation phase and compensation time is compensated for the BiSAR echoes.
- step5
- Imaging processing. The final image can be obtained after imaging processing.
3. Results
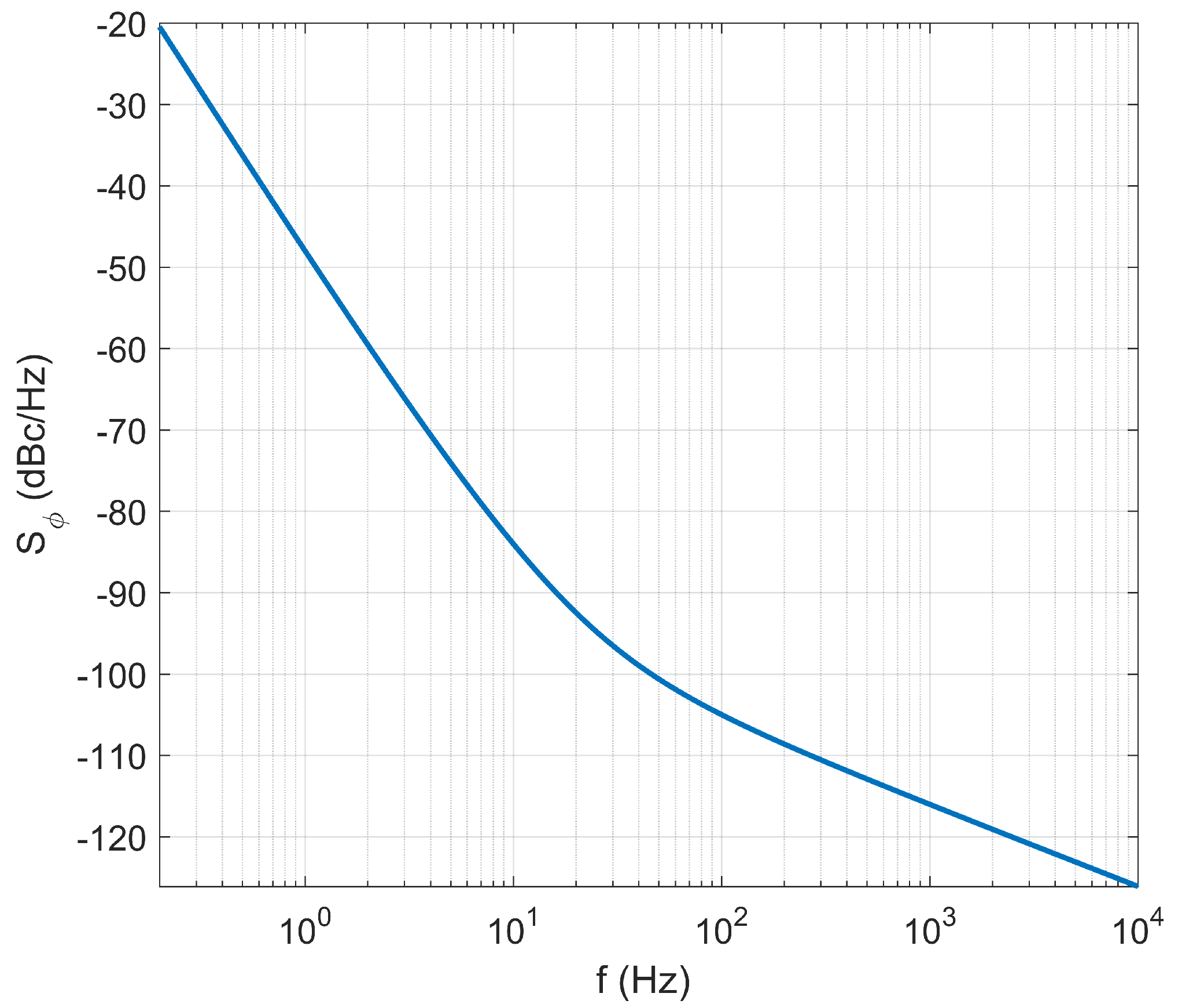
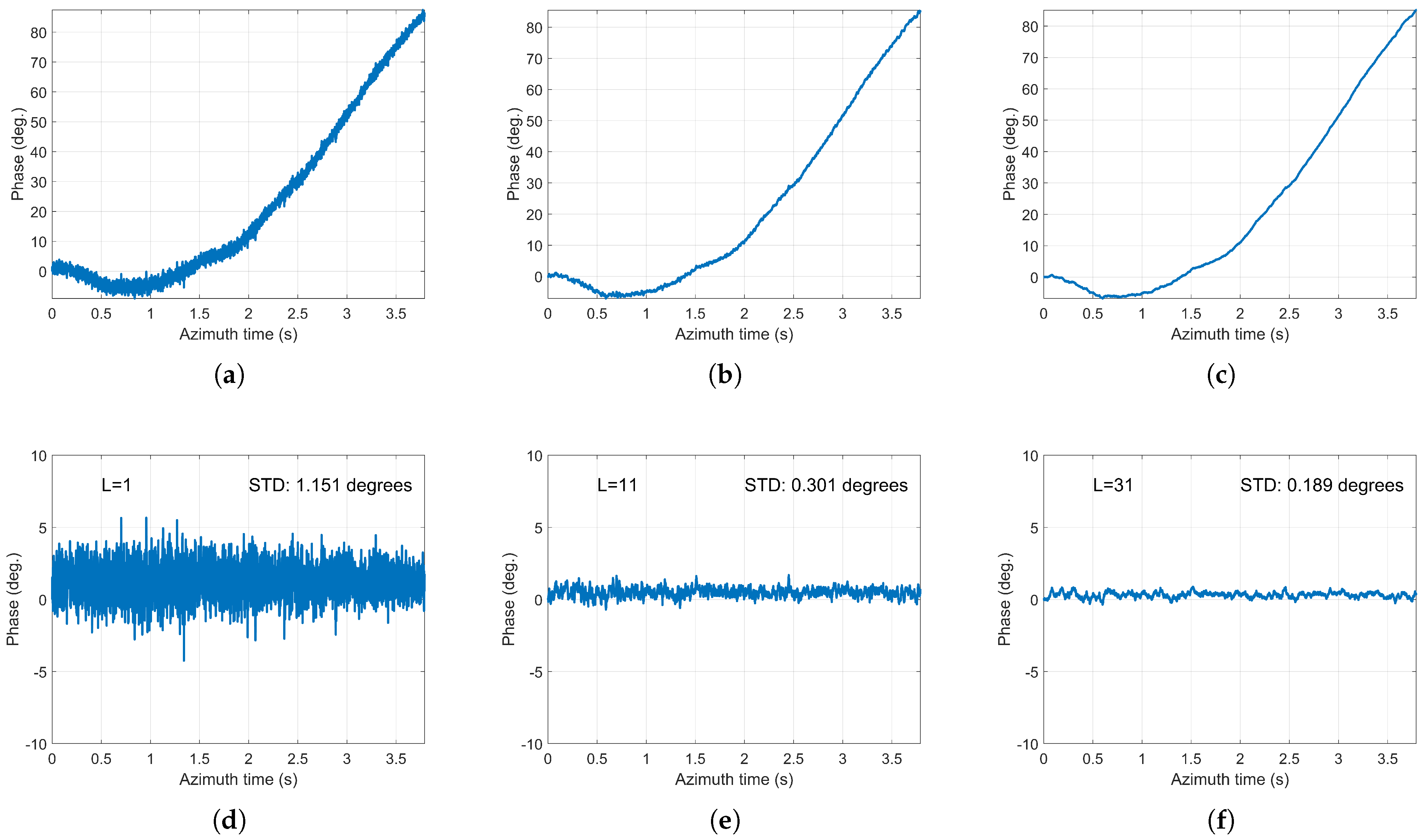
3.1. Oscillator Phase Error Simulation
3.2. Distributed Targets Simulation
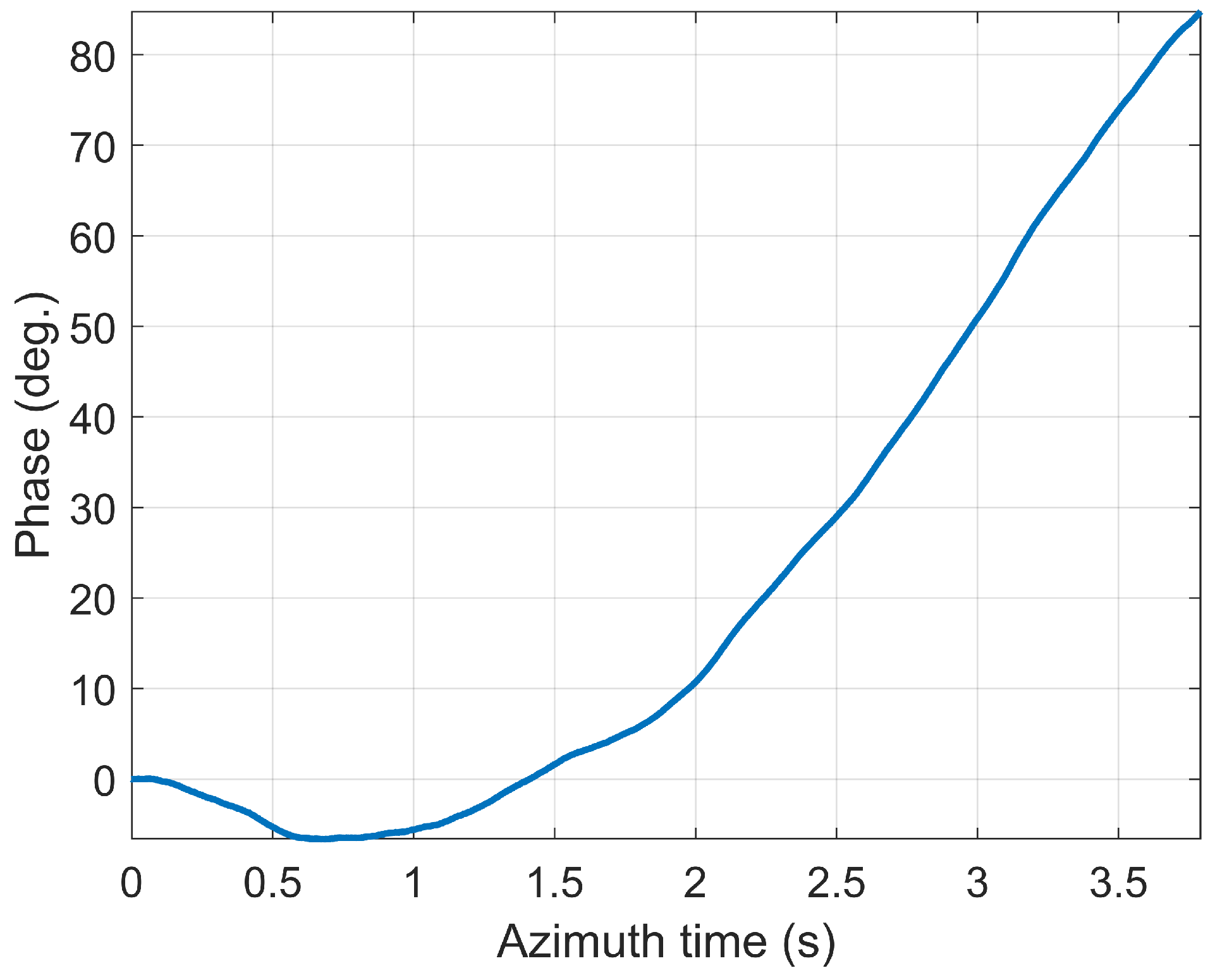
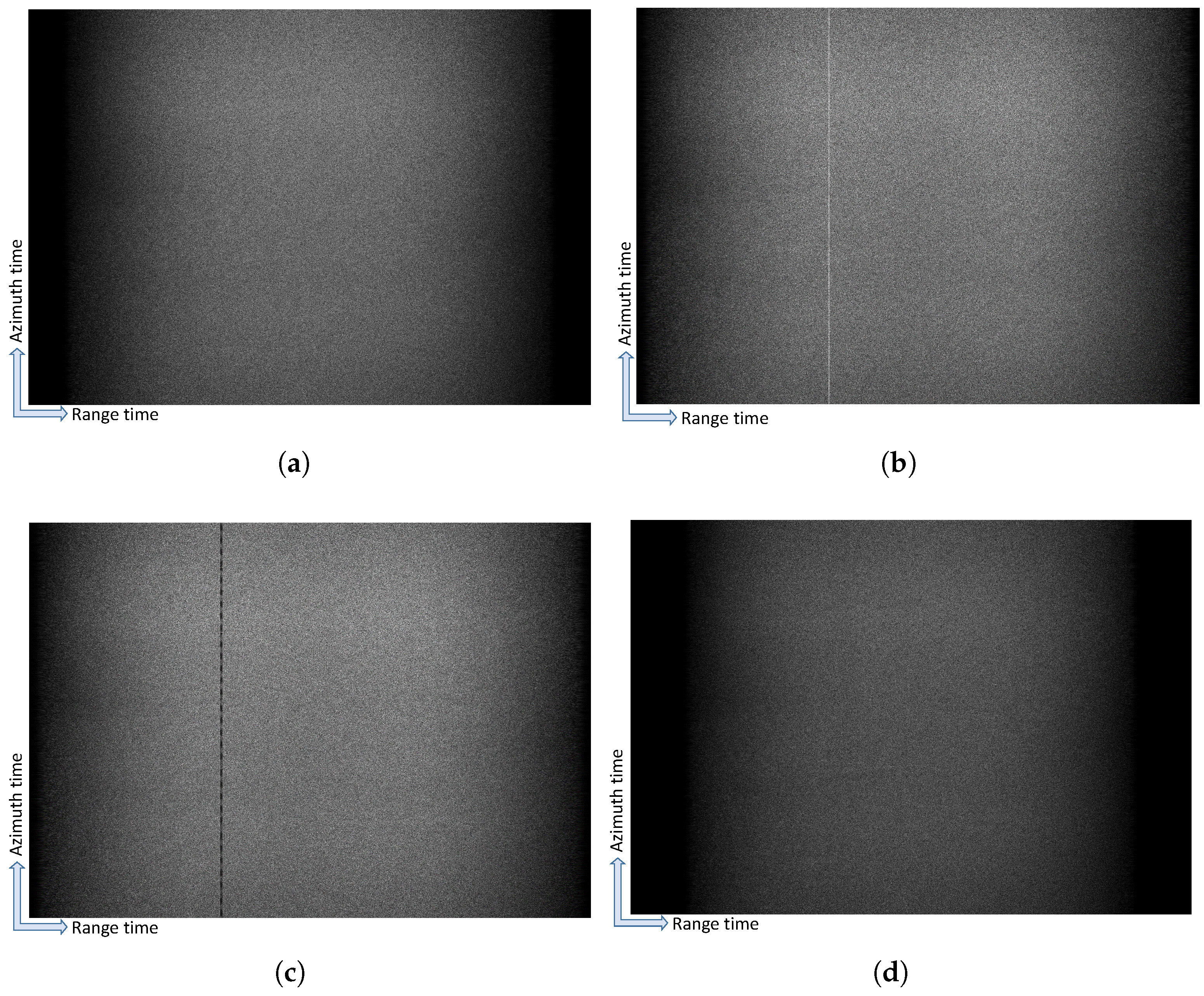
3.2.1. Extracting Synchronization Phase
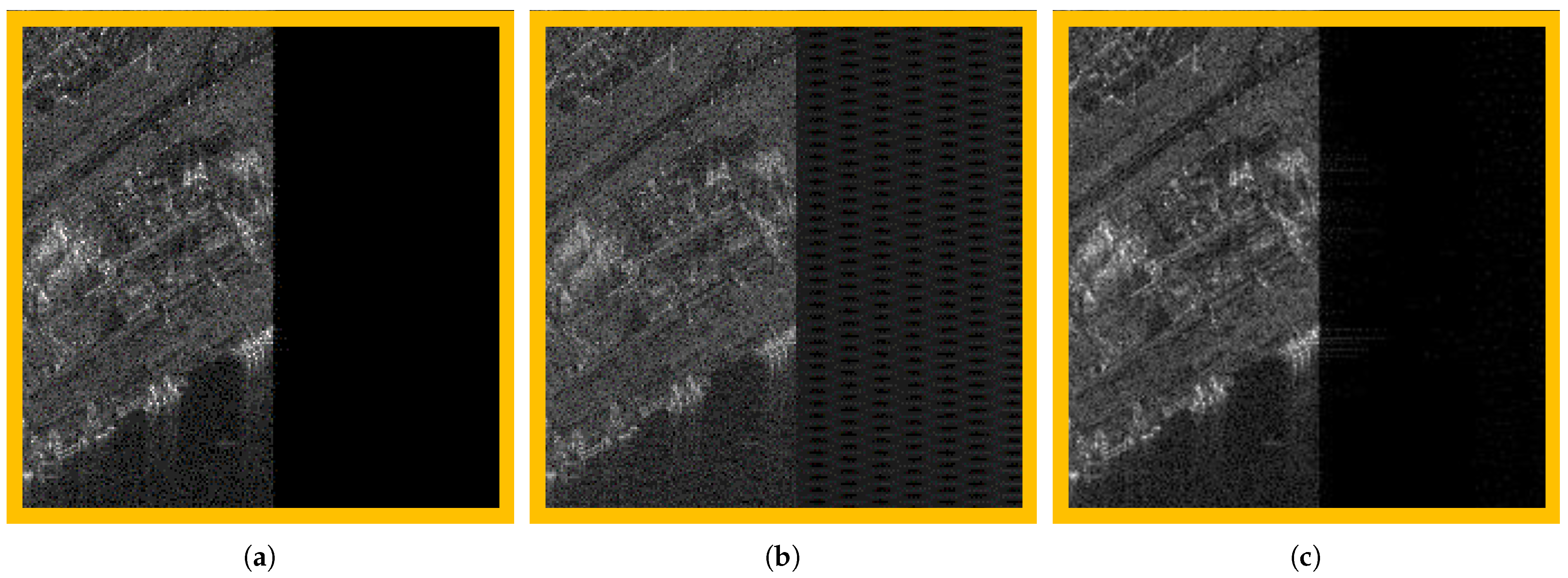
3.2.2. Extracting Echo Signal
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Moreira, A.; Prats-Iraola, P.; Younis, M.; Krieger, G.; Hajnsek, I.; Papathanassiou, K.P. A Tutorial On Synthetic Aperture Radar. IEEE Geosci. Remote Sens. Mag. 2013, 1, 6–43. [Google Scholar] [CrossRef] [Green Version]
- Duque, S.; López-Dekker, P.; Mallorqui, J.J. Single-pass bistatic SAR interferometry using fixed-receiver configurations: Theory and experimental validation. IEEE Trans. Geosci. Remote Sens. 2010, 48, 2740–2749. [Google Scholar] [CrossRef]
- Krieger, G.; Moreira, A.; Fiedler, H.; Hajnsek, I.; Werner, M.; Younis, M.; Zink, M. TanDEM-X: A Satellite Formation for High-Resolution SAR Interferometry. IEEE Trans. Geosci. Remote Sens. 2007, 45, 3317–3341. [Google Scholar] [CrossRef] [Green Version]
- Krieger, G.; Moreira, A. Spaceborne bi- and multistatic SAR: Potential and challenges. IEEE Proc. Radar Sonar Navig. 2006, 153, 184–198. [Google Scholar] [CrossRef] [Green Version]
- Eineder, M. Ocillator clock drift compensation in bistatic interferometric SAR. In Proceedings of the IGARSS 2013—2003 IEEE International Geoscience and Remote Sensing Symposium, Toulouse, France, 21–25 July 2003; Volume 3, pp. 1449–1451. [Google Scholar]
- Younis, M.; Metzig, R.; Krieger, G. Performance prediction of a phase synchronization link for bistatic SAR. IEEE Geosci. Remote Sens. Lett. 2006, 3, 429–433. [Google Scholar] [CrossRef] [Green Version]
- Krieger, G.; Younis, M. Impact of oscillator noise in bistatic and multistatic SAR. IEEE Geosci. Remote Sens. Lett. 2006, 3, 424–428. [Google Scholar] [CrossRef] [Green Version]
- Krieger, G. Advanced bistatic and multistatic SAR concepts and applications. In Proceedings of the EUSAR 2006—6th European Synthetic Aperture Radar Conference Tutorial, Dresden, Germany, 16–18 May 2006; pp. 1–100. [Google Scholar]
- Ludlow, A.D.; Zelevinsky, T.; Campbell, G.; Blatt, S.; Boyd, M.; de Miranda, M.H.; Martin, M.; Thomsen, J.; Foreman, S.M.; Ye, J.; et al. Sr lattice clock at 1 × 10−16 fractional uncertainty by remote optical evaluation with a Ca clock. Science 2008, 319, 1805–1808. [Google Scholar] [CrossRef] [PubMed]
- Nicholson, T.; Campbell, S.; Hutson, R.; Marti, G.; Bloom, B.; McNally, R.; Zhang, W.; Barrett, M.; Safronova, M.; Strouse, G.; et al. Systematic evaluation of an atomic clock at 2 × 10−18 total uncertainty. Nat. Commun. 2015, 6, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Nemitz, N.; Ohkubo, T.; Takamoto, M.; Ushijima, I.; Das, M.; Ohmae, N.; Katori, H. Frequency ratio of Yb and Sr clocks with 5 × 10−17 uncertainty at 150 seconds averaging time. Nat. Photonics 2016, 10, 258. [Google Scholar] [CrossRef]
- Lezius, M.; Wilken, T.; Deutsch, C.; Giunta, M.; Mandel, O.; Thaller, A.; Schkolnik, V.; Schiemangk, M.; Dinkelaker, A.; Kohfeldt, A.; et al. Space-borne frequency comb metrology. Optica 2016, 3, 1381–1387. [Google Scholar] [CrossRef]
- Oelker, E.; Hutson, R.; Kennedy, C.; Sonderhouse, L.; Bothwell, T.; Goban, A.; Kedar, D.; Sanner, C.; Robinson, J.; Marti, G.; et al. Demonstration of 4.8 × 10−17 stability at 1 s for two independent optical clocks. Nat. Photonics 2019, 13, 714–719. [Google Scholar] [CrossRef] [Green Version]
- Rodriguez-Cassola, M.; Prats-Iraola, P.; Lopez-Dekker, P.; Reigber, A.; Krieger, G.; Moreira, A. Autonomous time and phase calibration of spaceborne bistatic SAR systems. In Proceedings of the EUSAR 2014—10th European Conference on Synthetic Aperture Radar, Berlin, Germany, 3–5 June 2014; pp. 264–267. [Google Scholar]
- Lopez-Dekker, P.; Mallorqui, J.J.; Serra-Morales, P.; Sanz-Marcos, J. Phase Synchronization and Doppler Centroid Estimation in Fixed Receiver Bistatic SAR Systems. IEEE Trans. Geosci. Remote Sens. 2008, 46, 3459–3471. [Google Scholar] [CrossRef]
- Weib, M. Synchronisation of bistatic radar systems. In Proceedings of the IGARSS 2004—2004 IEEE International Geoscience and Remote Sensing Symposium, Anchorage, AK, USA, 20–24 September 2004; Volume 3, pp. 1750–1753. [Google Scholar]
- Breit, H.; Younis, M.; Balss, U.; Niedermeier, A.; Grigorov, C.; Hueso-Gonzalez, J.; Krieger, G.; Eineder, M.; Fritz, T. Bistatic synchronization and processing of TanDEM-X data. In Proceedings of the IGARSS 2011—2011 IEEE International Geoscience and Remote Sensing Symposium, Vancouver, BC, Canada, 24–29 July 2011; pp. 2424–2427. [Google Scholar]
- Weigt, M.; Grigorov, C.; Steinbrecher, U.; Schulze, D. TanDEM-X Mission: Long Term in Orbit Synchronisation Link Performance Analysis. In Proceedings of the EUSAR 2014—10th European Conference on Synthetic Aperture Radar, Berlin, Germany, 3–5 June 2014; pp. 1–4. [Google Scholar]
- Lipiński, M.; Włostowski, T.; Serrano, J.; Alvarez, P. White rabbit: A PTP application for robust sub-nanosecond synchronization. In Proceedings of the 2011 IEEE International Symposium on Precision Clock Synchronization for Measurement, Control and Communication, Munich, Germany, 12–16 September 2011; IEEE: Piscataway, NJ, USA, 2011; pp. 25–30. [Google Scholar]
- Two Way Time Transfer. Available online: https://tf.nist.gov/time/twoway.htm (accessed on 12 December 2020).
- Pinheiro, M.; Rodriguez-Cassola, M. Reconstruction Methods of Missing SAR Data: Analysis in the Frame of TanDEM-X Synchronization Link. In Proceedings of the EUSAR 2012—9th European Conference on Synthetic Aperture Radar, Nuremberg, Germany, 23–26 April 2012; pp. 742–745. [Google Scholar]
- Pinheiro, M.; Rodriguez-Cassola, M.; Prats-Iraola, P.; Reigber, A.; Krieger, G.; Moreira, A. Reconstruction of Coherent Pairs of Synthetic Aperture Radar Data Acquired in Interrupted Mode. IEEE Trans. Geosci. Remote Sens. 2015, 53, 1876–1893. [Google Scholar] [CrossRef]
- Liang, D.; Liu, K.; Yue, H.; Chen, Y.; Deng, Y.; Zhang, H.; Li, C.; Jin, G.; Wang, R. An advanced non-interrupted synchronization scheme for bistatic synthetic aperture radar. In Proceedings of the IGARSS 2019—2019 IEEE International Geoscience and Remote Sensing Symposium, Yokohama, Japan, 28 July–2 August 2019; pp. 1116–1119. [Google Scholar]
- Liang, D.; Liu, K.; Zhang, H.; Deng, Y.; Liu, D.; Chen, Y.; Li, C.; Yue, H.; Wang, R. A High-Accuracy Synchronization Phase-Compensation Method Based on Kalman Filter for Bistatic Synthetic Aperture Radar. IEEE Geosci. Remote Sens. Lett. 2020, 17, 1722–1726. [Google Scholar] [CrossRef]
- Jin, G.; Liu, K.; Liu, D.; Liang, D.; Zhang, H.; Ou, N.; Zhang, Y.; Deng, Y.; Li, C.; Wang, R. An Advanced Phase Synchronization Scheme for LT-1. IEEE Trans. Geosci. Remote Sens. 2020, 58, 1735–1746. [Google Scholar] [CrossRef]
- Liang, D.; Liu, K.; Zhang, H.; Chen, Y.; Yue, H.; Liu, D.; Deng, Y.; Lin, H.; Fang, T.; Li, C.; et al. The Processing Framework and Experimental Verification for the Noninterrupted Synchronization Scheme of LuTan-1. IEEE Trans. Geosci. Remote Sens. 2020. [Google Scholar] [CrossRef]
- Calderbank, R.; Howard, S.D.; Moran, B. Waveform diversity in radar signal processing. IEEE Signal Process. Mag. 2009, 26, 32–41. [Google Scholar] [CrossRef]
- Gini, F.; De Maio, A.; Patton, L. Waveform Design and Diversity for Advanced Radar Systems; Institution of Engineering and Technology: London, UK, 2012. [Google Scholar]
- Zhao, P.; Deng, Y.; Wang, W.; Liu, D.; Wang, R. Azimuth Ambiguity Suppression for Hybrid Polarimetric Synthetic Aperture Radar via Waveform Diversity. Remote Sens. 2020, 12, 1226. [Google Scholar] [CrossRef] [Green Version]
- Mittermayer, J.; Martinez, J.M. Analysis of range ambiguity suppression in SAR by up and down chirp modulation for point and distributed targets. In Proceedings of the IGARSS 2003–2003 IEEE International Geoscience and Remote Sensing Symposium. Proceedings (IEEE Cat. No.03CH37477), Toulouse, France, 21–25 July 2003; Volume 6, pp. 4077–4079. [Google Scholar]
- Krieger, G. MIMO-SAR: Opportunities and Pitfalls. IEEE Trans. Geosci. Remote Sens. 2014, 52, 2628–2645. [Google Scholar] [CrossRef] [Green Version]
- Villano, M.; Krieger, G.; Moreira, A. Nadir Echo Removal in Synthetic Aperture Radar via Waveform Diversity and Dual-Focus Postprocessing. IEEE Geosci. Remote Sens. Lett. 2018, 15, 719–723. [Google Scholar] [CrossRef]
- D’Errico, M. Distributed Space Missions for Earth System Monitoring; Springer Science & Business Media: New York, NY, USA, 2012; Volume 31. [Google Scholar]
- Younis, M.; Metzig, R.; Krieger, G.; Bachmann, M.; Klein, R. Performance prediction and verification for the synchronization link of TanDEM-X. In Proceedings of the IGARSS 2007—IEEE International Geoscience and Remote Sensing Symposium, Barcelona, Spain, 23–27 July 2007; pp. 5206–5209. [Google Scholar]
- Breit, H.; Lachaise, M.; Balss, U.; Rossi, C.; Fritz, T.; Niedermeier, A. Bistatic and Interferometric Processing of TanDEM-X Data. In Proceedings of the EUSAR 2012; 9th European Conference on Synthetic Aperture Radar, Nuremberg, Germany, 23–26 April 2012; pp. 93–96. [Google Scholar]
- Balanis, C.A. Antenna Theory: Analysis and Design, 3rd ed.; Wiley-Interscience: New York, NY, USA, 2005. [Google Scholar]
- Younis, M.; Huber, S.; Patyuchenko, A.; Bordoni, F.; Krieger, G. Performance comparison of reflector-and planar-antenna based digital beam-forming SAR. Int. J. Antennas Propag. 2009, 2009. [Google Scholar] [CrossRef]
- Mahafza, B.R.; Elsherbeni, A. MATLAB Simulations for Radar Systems Design; Chapman and Hall/CRC: Boca Raton, FL, USA, 2003. [Google Scholar]
- Krieger, G.; De Zan, F. Relativistic Effects in Bistatic Synthetic Aperture Radar. IEEE Trans. Geosci. Remote Sens. 2014, 52, 1480–1488. [Google Scholar] [CrossRef]
- Kasdin, N.J. Discrete simulation of colored noise and stochastic processes and 1/fα power law noise generation. Proc. IEEE 1995, 83, 802–827. [Google Scholar] [CrossRef]
- Liang, D.; Zhang, H.; Fang, T.; Lin, H.; Liu, D.; Jia, X. A Modified Cartesian Factorized Backprojection Algorithm Integrating with Non-Start-Stop Model for High Resolution SAR Imaging. Remote Sens. 2020, 12, 3807. [Google Scholar] [CrossRef]
- Krieger, G.; Zonno, M.; Mittermayer, J.; Moreira, A.; Huber, S.; Rodriguezcassola, M. MirrorSAR: A Fractionated Space Transponder Concept for the Implementation of Low-Cost Multistatic SAR Missions. In Proceedings of the EUSAR 2018—12th European Conference on Synthetic Aperture Radar, Aachen, Germany, 4–7 June 2018; pp. 1359–1364. [Google Scholar]
- Cumming, I.G.; Wong, F.H. Digital Processing of Synthetic Aperture Radar Data: Algorithms and Implementation; Artech House: Norwood, MA, USA, 2005. [Google Scholar]







| Parameter | Symbol | Value |
|---|---|---|
| Syn. antenna transmitting gain | 0 dB | |
| Syn. antenna receiving gain | 0 dB | |
| Boltzmann’s constant | 1.38 J/K | |
| Receiver temperature | 300 K | |
| Distance | R | 0.1∼10 km |
| Synchronization signal pulse width | 0.5∼20 s |
| Frequency | 1 Hz | 10 Hz | Hz | Hz | Hz |
|---|---|---|---|---|---|
| Phase noise level | −48 | −84 | −105 | −116 | −124 |
| Parameter | Value |
|---|---|
| Carrier frequency | 1.26 GHz |
| Orbit height | 600 km |
| Incidence angle | 30 |
| Radar signal bandwidth | 80 MHz |
| Radar signal pulse width | 60 s |
| Radar signal FM rate | Hz/s |
| Synchronization signal bandwidth | 80 MHz |
| Synchronization signal pulse width | 20 s |
| Synchronization signal FM rate | Hz/s |
| Sampling frequency | 90 MHz |
| PRF | 1898 Hz |
| Doppler bandwidth | 1400 Hz |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, D.; Zhang, H.; Cai, Y.; Liu, K.; Zhang, K. An Advanced Phase Synchronization Scheme Based on Coherent Integration and Waveform Diversity for Bistatic SAR. Remote Sens. 2021, 13, 981. https://doi.org/10.3390/rs13050981
Liang D, Zhang H, Cai Y, Liu K, Zhang K. An Advanced Phase Synchronization Scheme Based on Coherent Integration and Waveform Diversity for Bistatic SAR. Remote Sensing. 2021; 13(5):981. https://doi.org/10.3390/rs13050981
Chicago/Turabian StyleLiang, Da, Heng Zhang, Yonghua Cai, Kaiyu Liu, and Ke Zhang. 2021. "An Advanced Phase Synchronization Scheme Based on Coherent Integration and Waveform Diversity for Bistatic SAR" Remote Sensing 13, no. 5: 981. https://doi.org/10.3390/rs13050981
APA StyleLiang, D., Zhang, H., Cai, Y., Liu, K., & Zhang, K. (2021). An Advanced Phase Synchronization Scheme Based on Coherent Integration and Waveform Diversity for Bistatic SAR. Remote Sensing, 13(5), 981. https://doi.org/10.3390/rs13050981






