Modeling and Analysis of BDS-2 and BDS-3 Combined Precise Time and Frequency Transfer Considering Stochastic Models of Inter-System Bias
Abstract
1. Introduction
2. Methods
2.1. BDS-2 and BDS-3 Combined PPP Model
2.2. The Stochastic Models for ISB Parameters
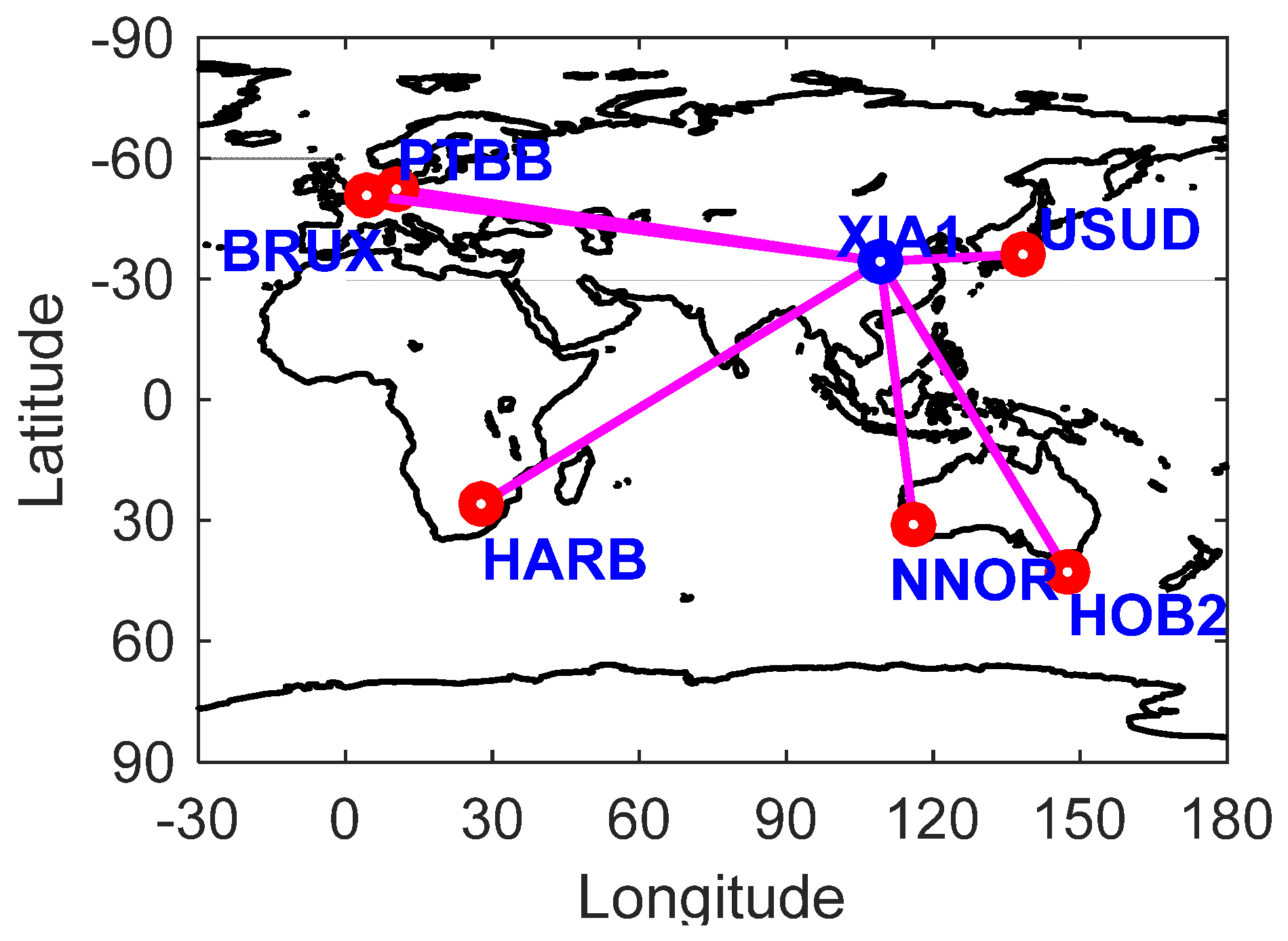
3. Data and Processing Strategies
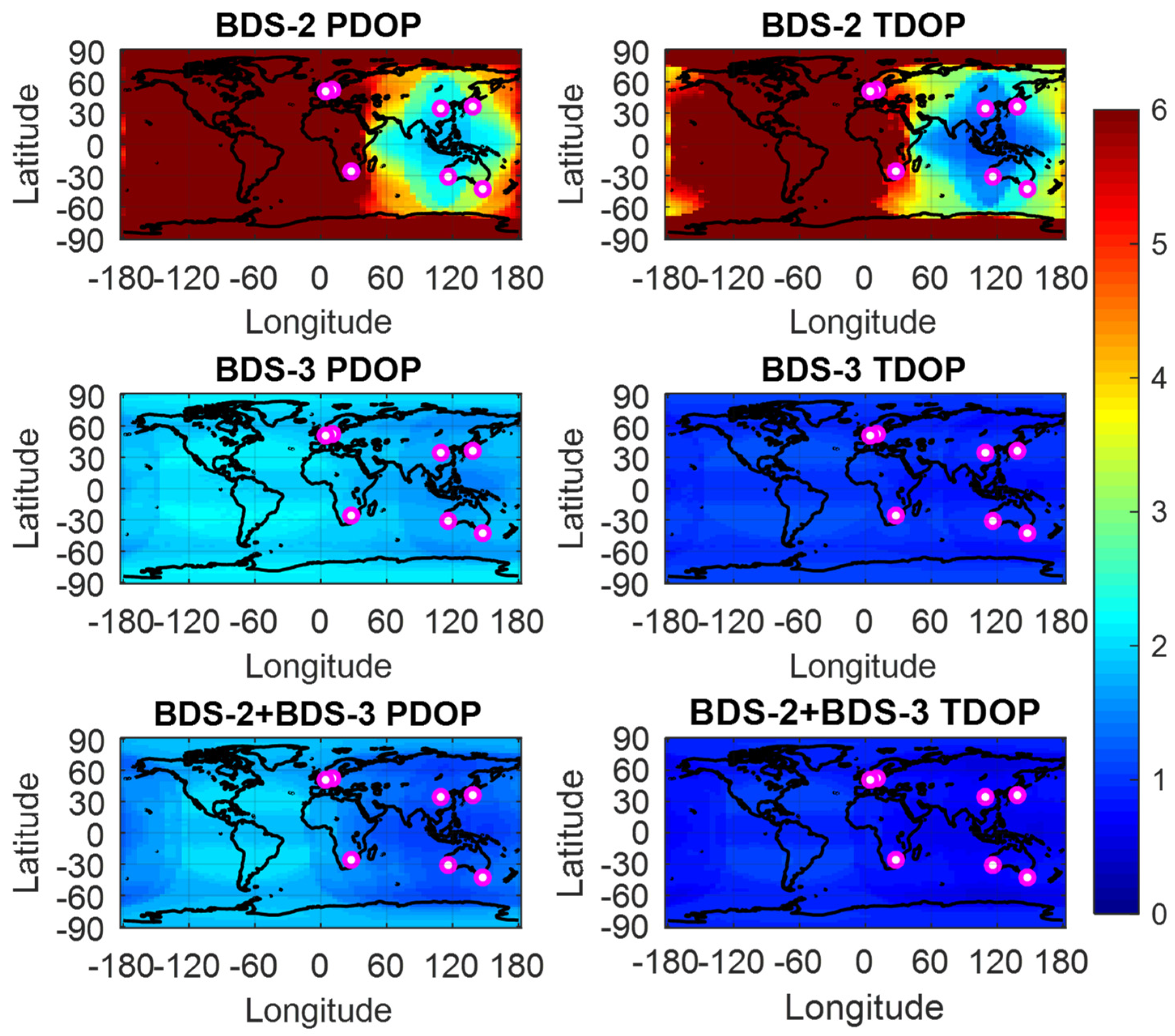
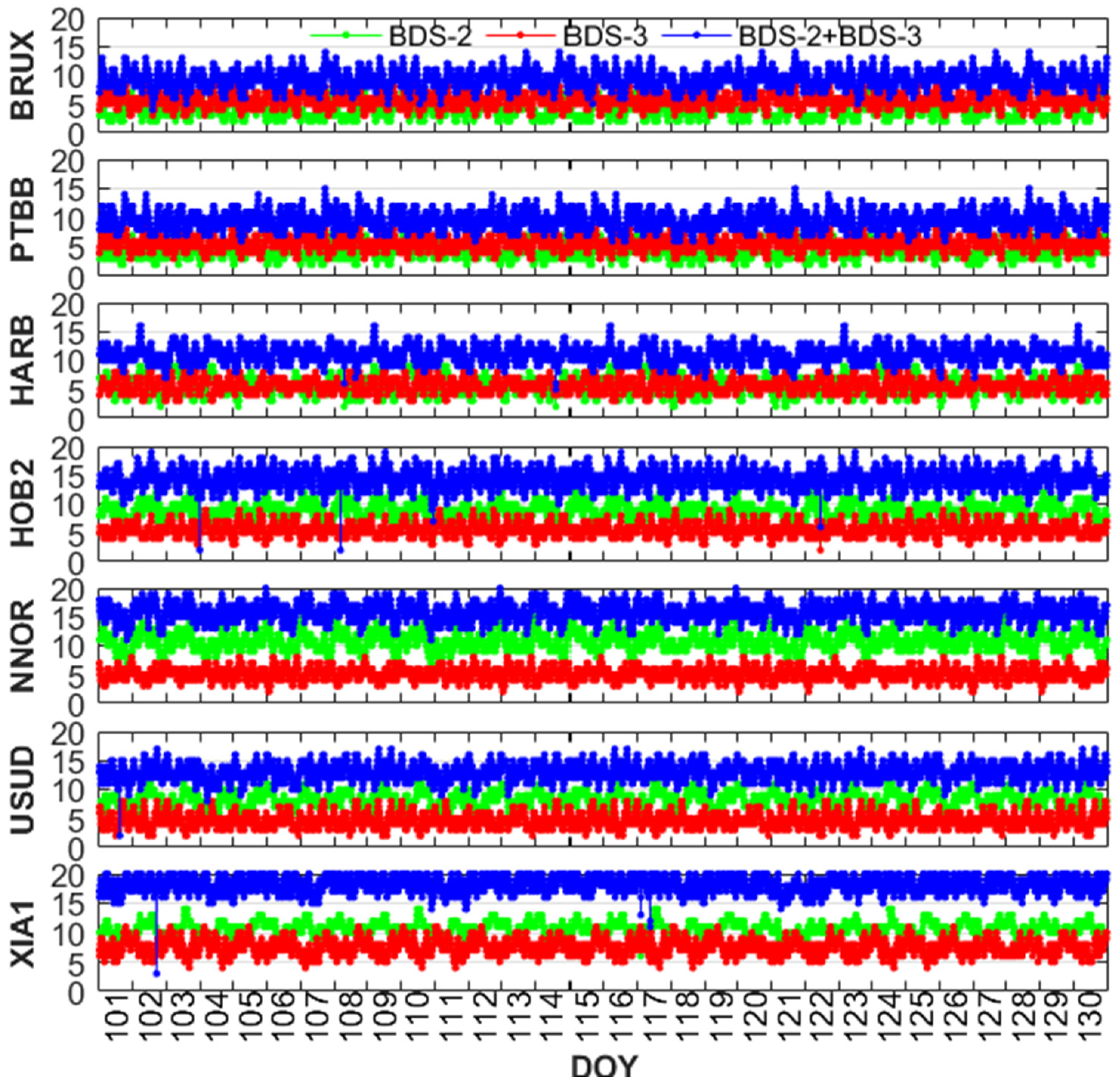
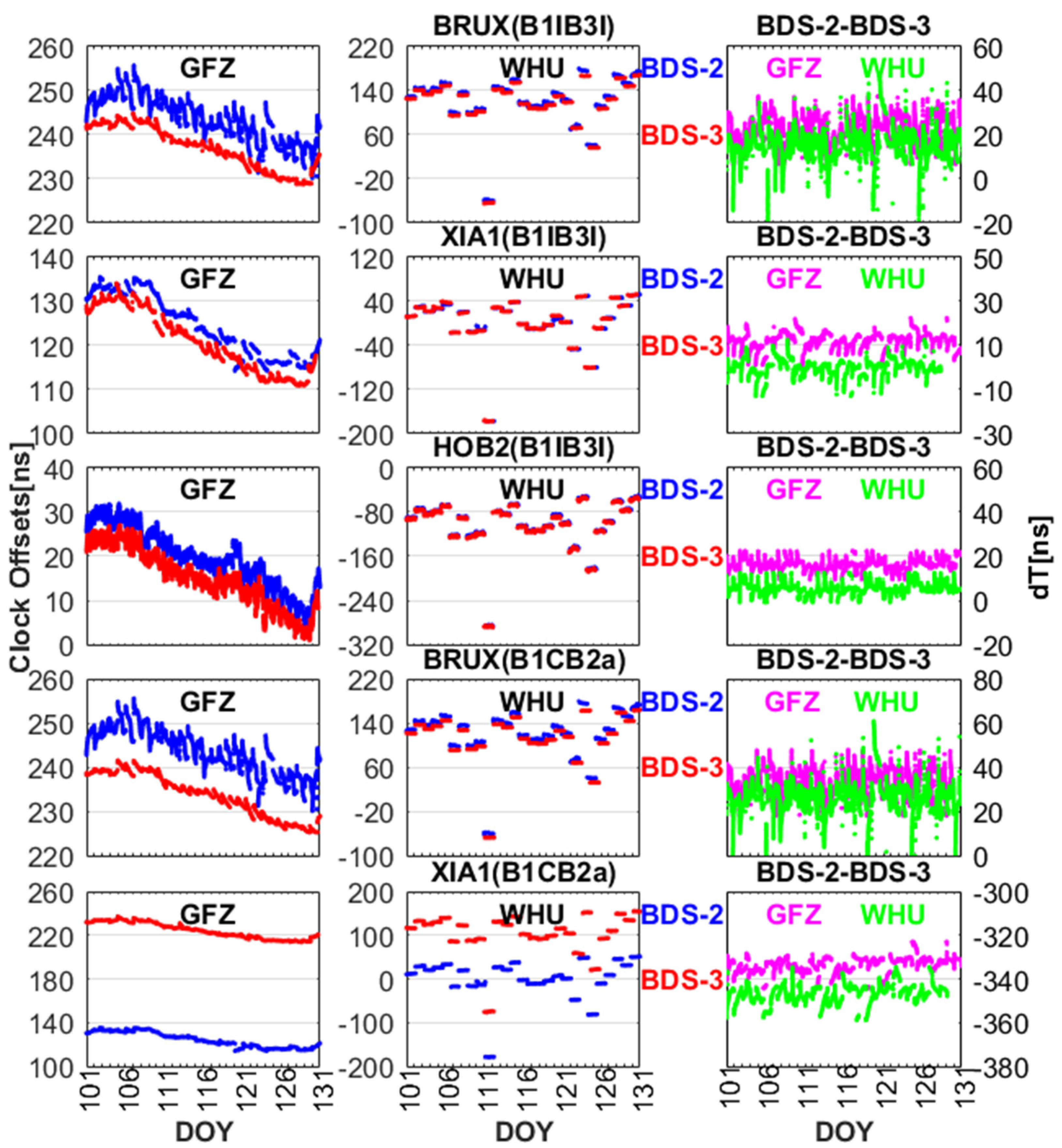
4. Results and Analysis
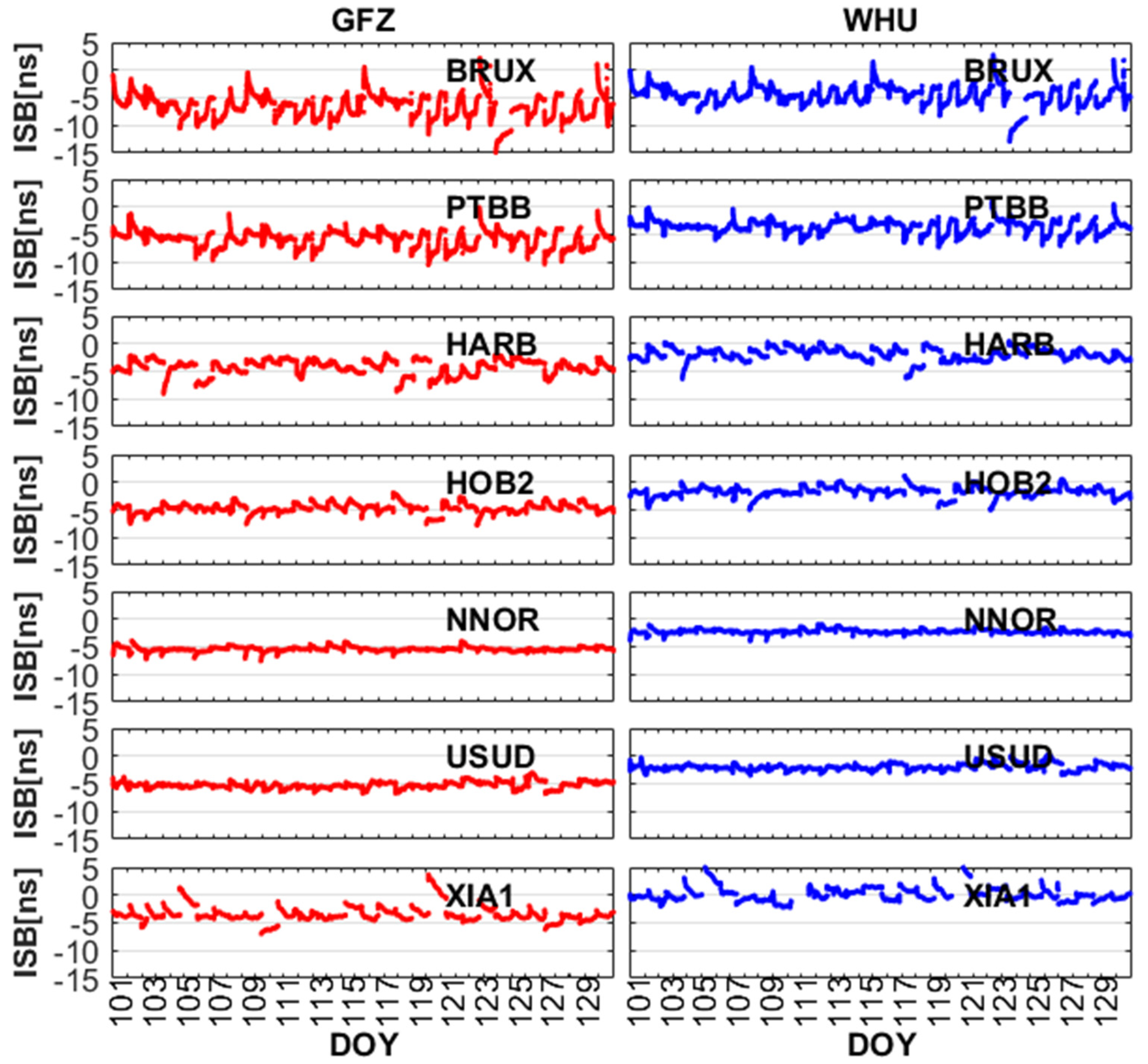
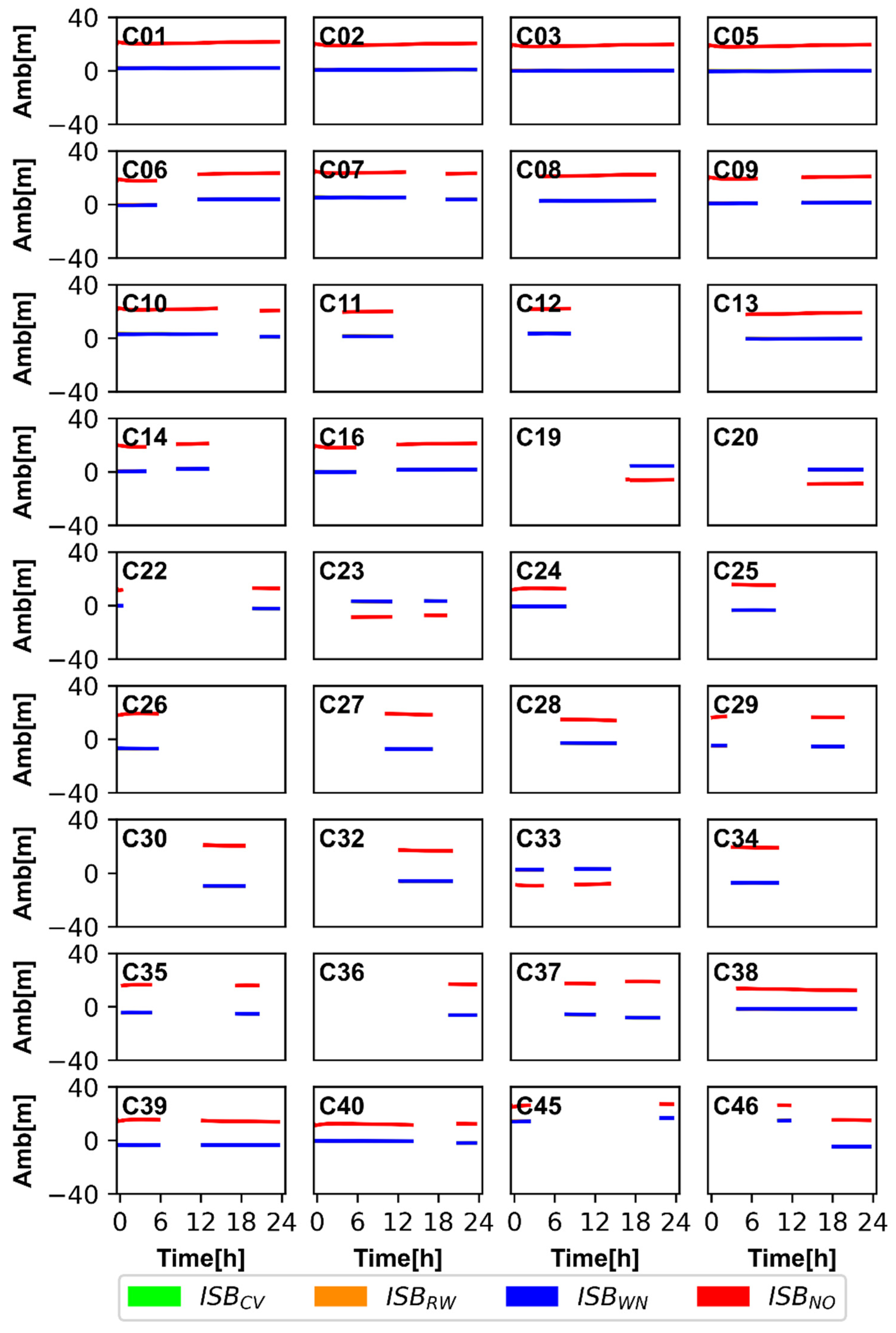
4.1. The Characteristic of ISB between BDS-2 and BDS-3
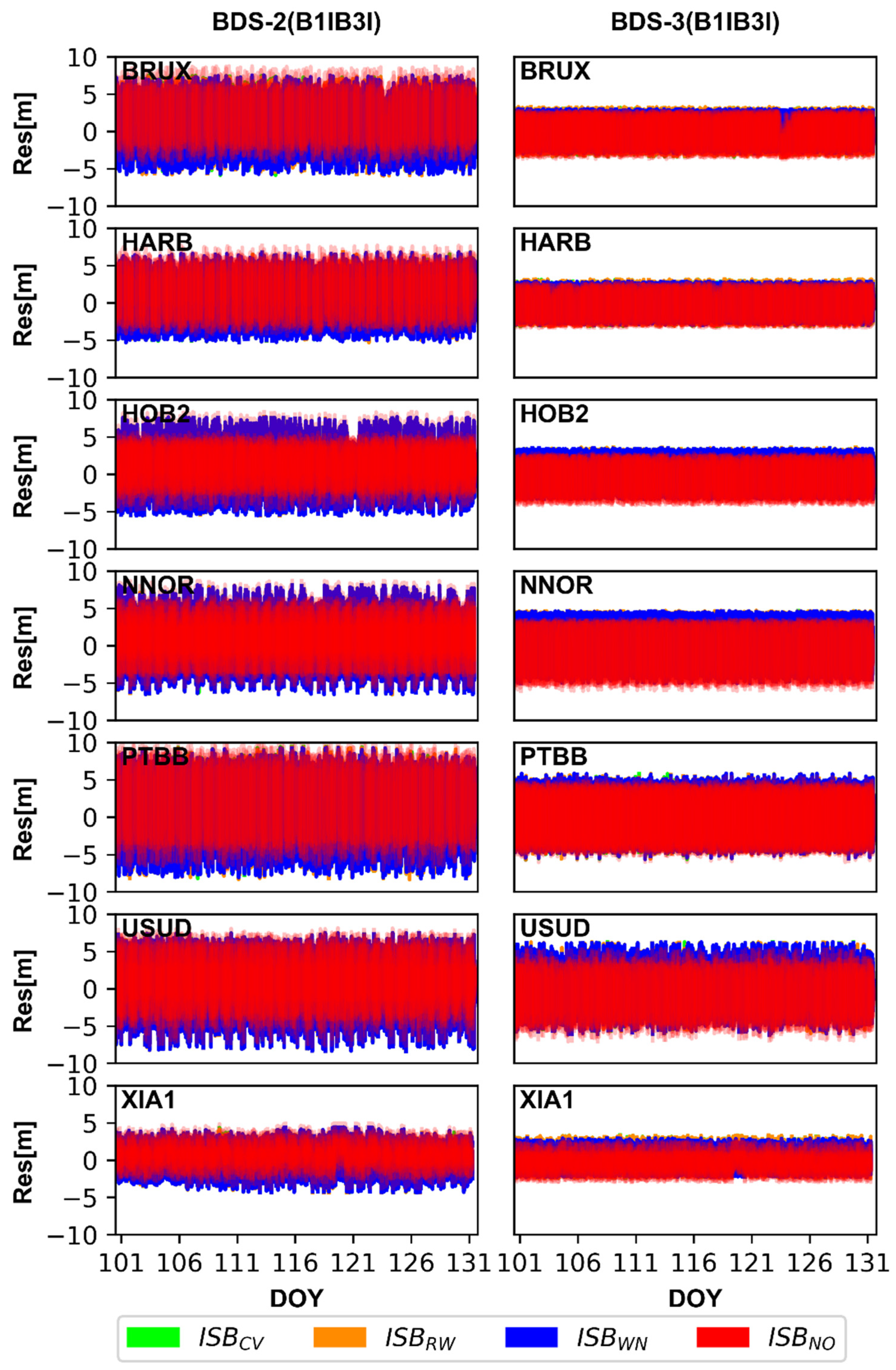
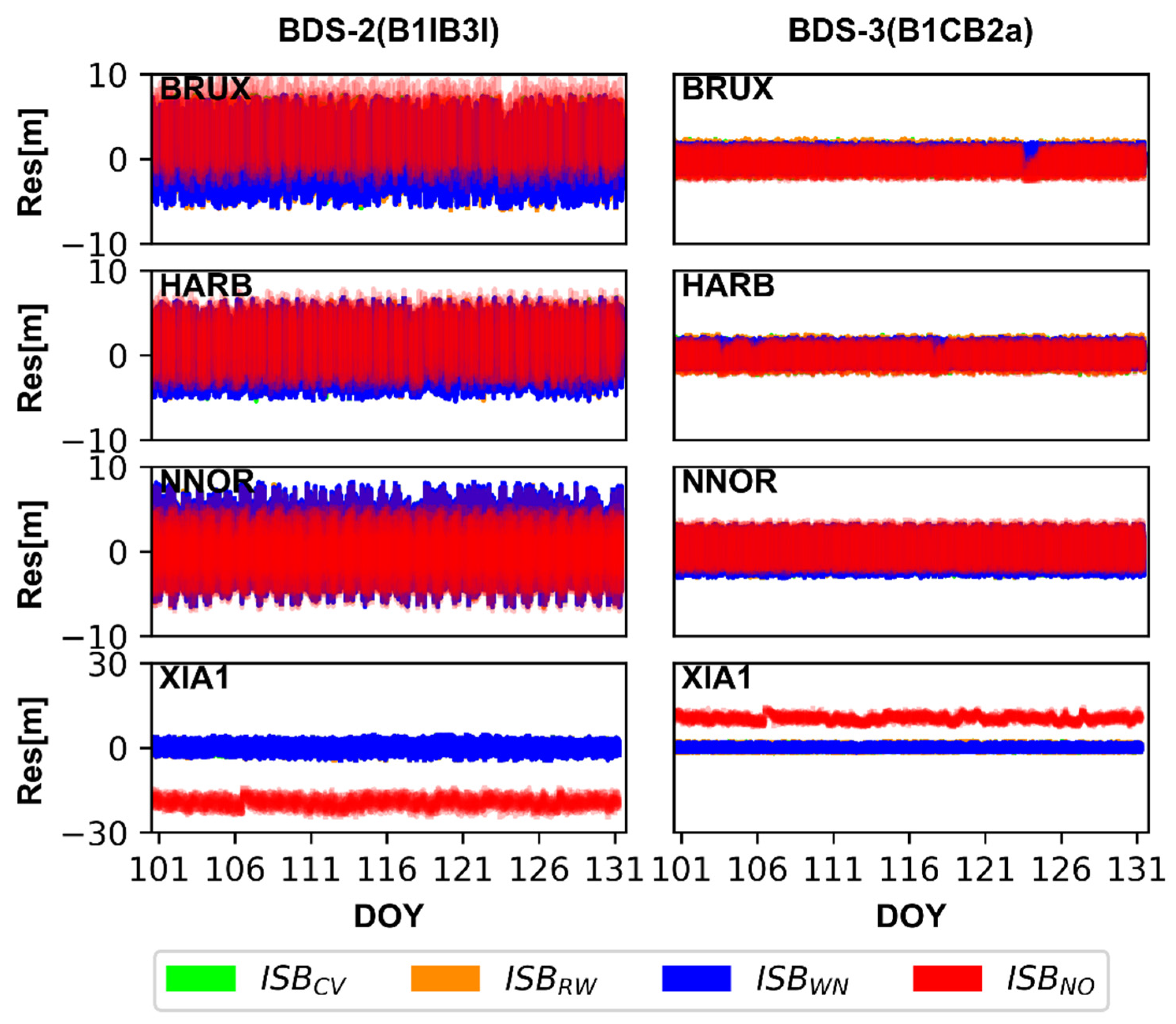
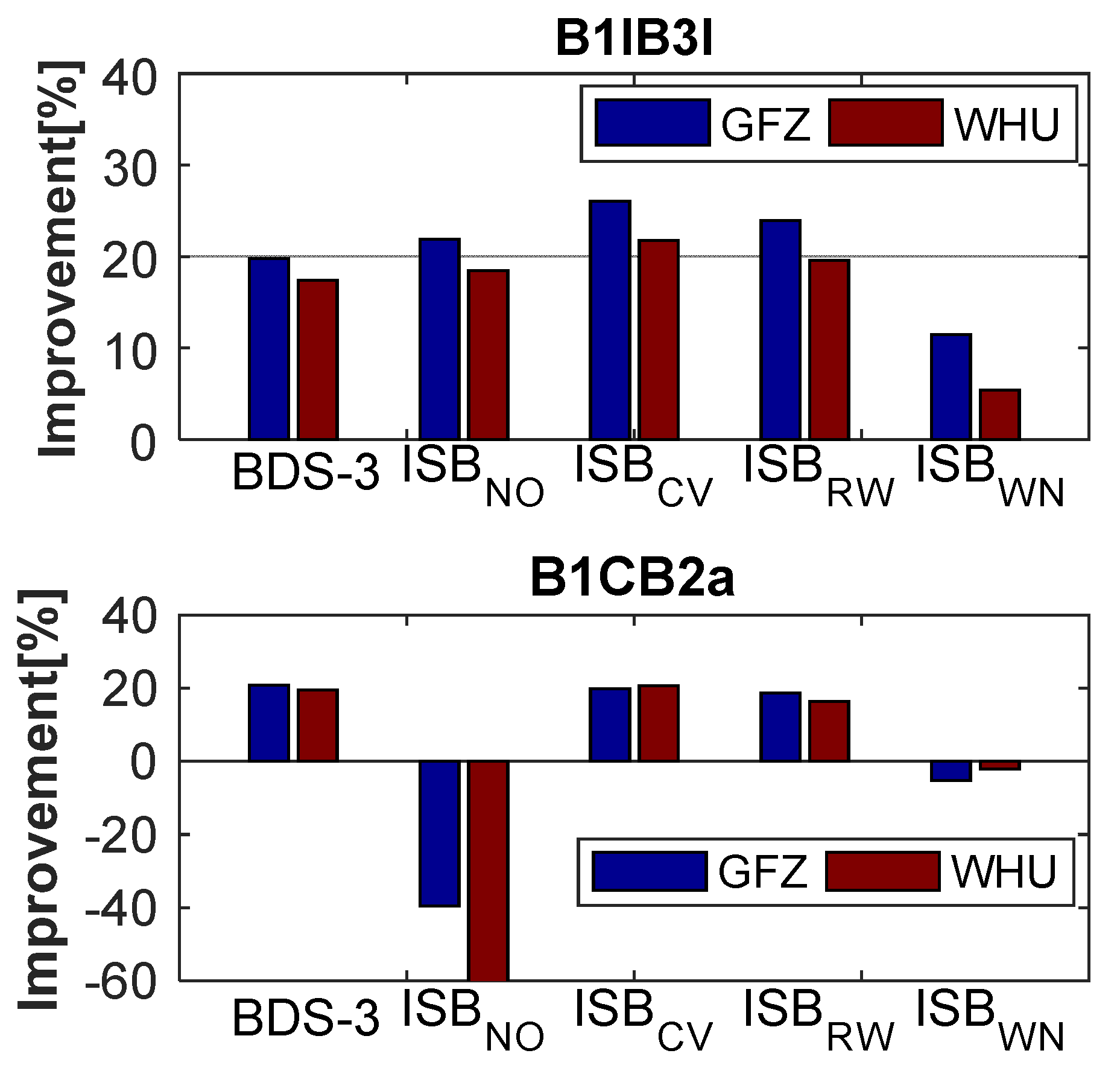
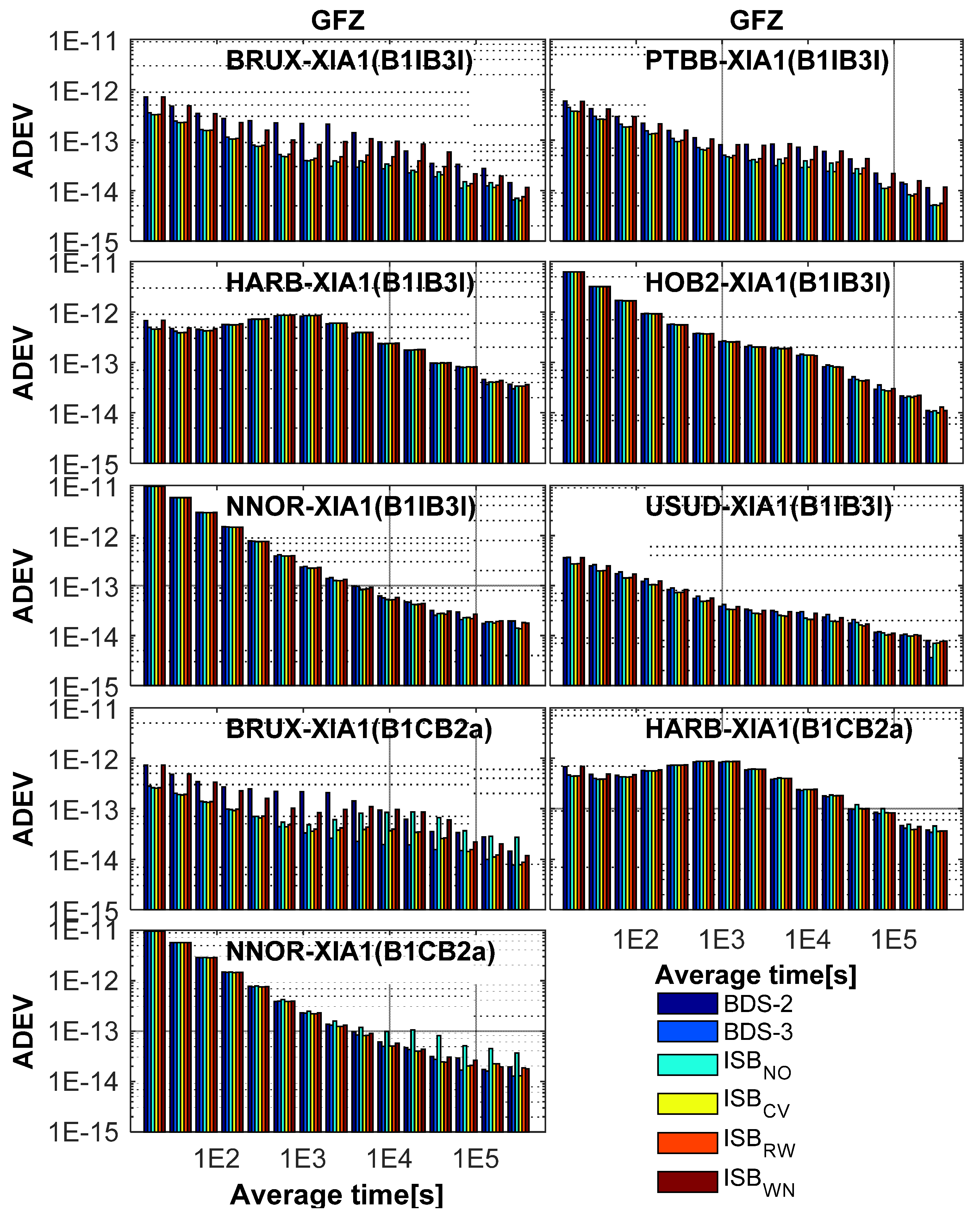
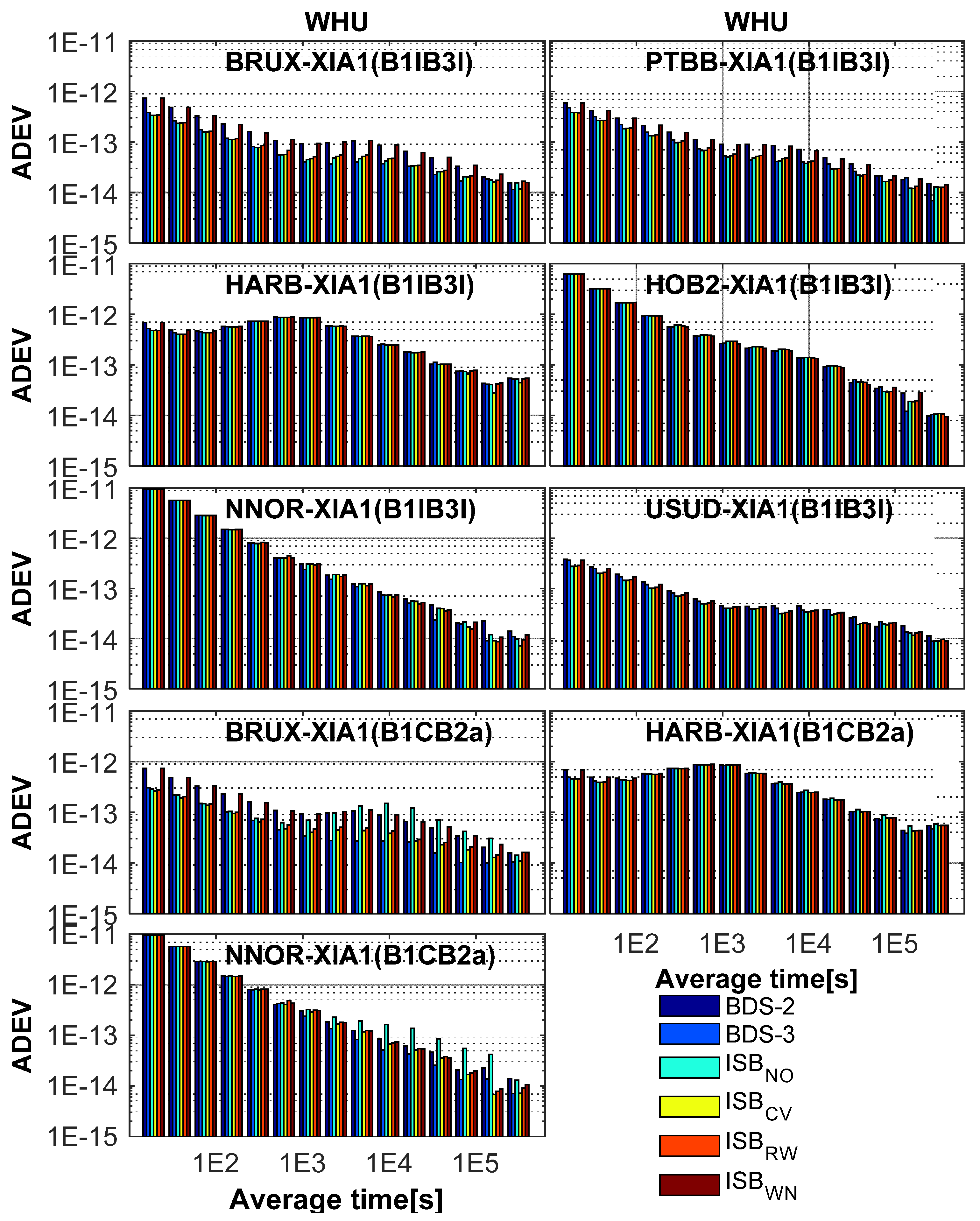
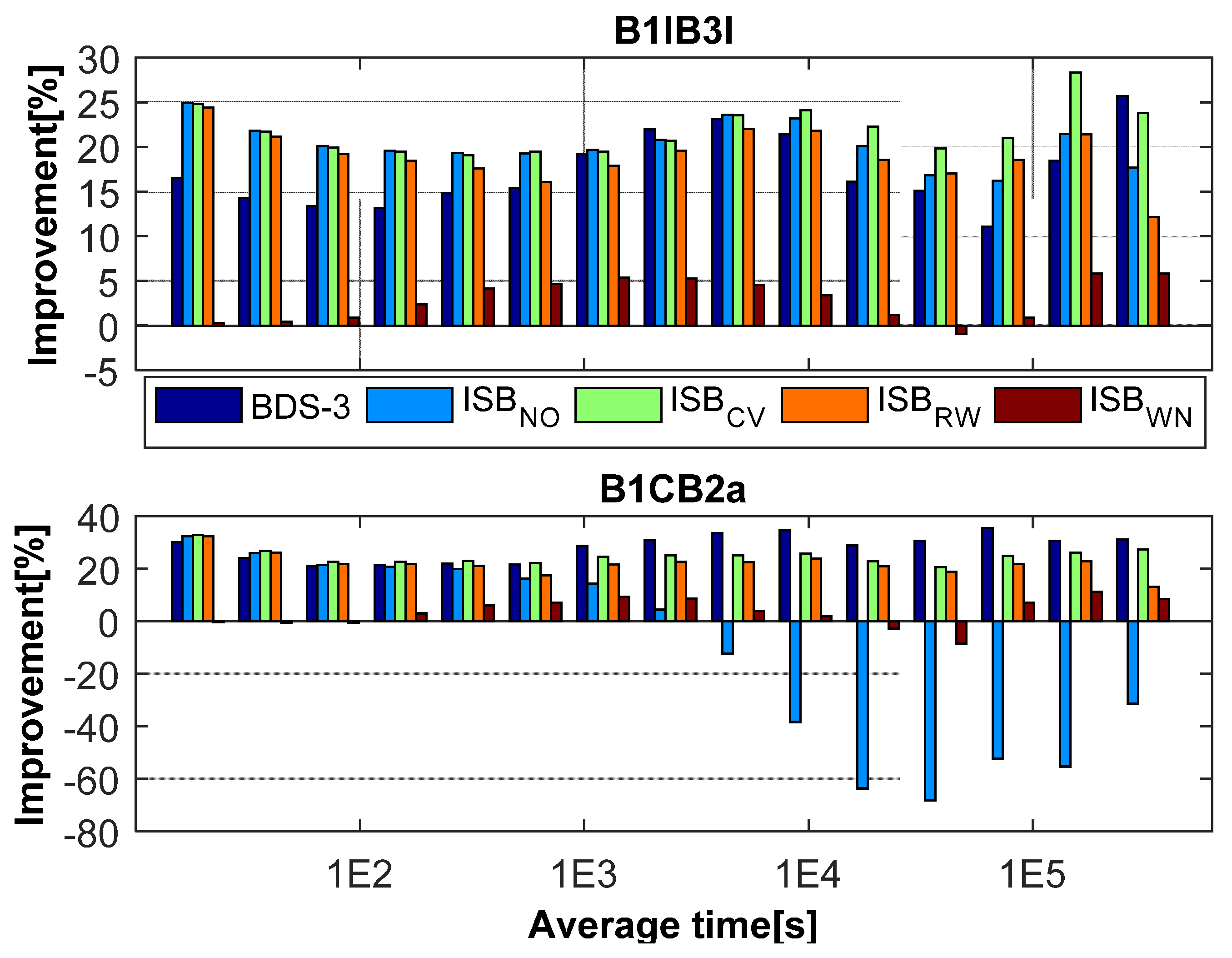
4.2. Precise Time and Frequency Transfer Using Different ISB Stochastic Models
5. Conclusions and Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yang, Y.; Gao, W.; Guo, S.; Mao, Y.; Yang, Y. Introduction to BeiDou-3 navigation satellite system. Navigation 2019, 66, 7–18. [Google Scholar] [CrossRef]
- Yang, Y.; Li, J.; Xu, J.; Tang, J.; Guo, H.; He, H. Contribution of the Compass satellite navigation system to global PNT users. Chin. Sci. Bull. 2011, 56, 2813–2819. [Google Scholar] [CrossRef]
- Yang, Y.; Tang, J.; Montenbruck, O. Chinese Navigation Satellite Systems. In Springer Handbook of Global Navigation Satellite Systems; Teunissen, P.J.G., Montenbruck, O., Eds.; Springer International Publishing: Cham, Switzerland, 2017; pp. 273–304. [Google Scholar] [CrossRef]
- Montenbruck, O.; Hauschild, A.; Steigenberger, P.; Hugentobler, U.; Teunissen, P.; Nakamura, S. Initial assessment of the COMPASS/BeiDou-2 regional navigation satellite system. GPS Solut. 2012, 17, 211–222. [Google Scholar] [CrossRef]
- Lee, S.W.; Schutz, B.E.; Lee, C.-B.; Yang, S.H. A study on the Common-View and All-in-View GPS time transfer using carrier-phase measurements. Metrologia 2008, 45, 156–167. [Google Scholar] [CrossRef]
- Petit, G.; Jiang, Z. GPS All in View time transfer for TAI computation. Metrologia 2008, 45, 35–45. [Google Scholar] [CrossRef]
- Weiss, M.A.; Petit, G.; Jiang, Z. A comparison of GPS common-view time transfer to all-in-view. In Proceedings of the 2005 IEEE International Frequency Control. Symposium and Exposition, Vancouver, BC, Canada, 29–31 August 2005; pp. 324–328. [Google Scholar] [CrossRef]
- Larson, K.M.; Levine, J. Carrier-phase time transfer. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 1999, 46, 1001–1012. [Google Scholar] [CrossRef]
- Defraigne, P.; Aerts, W.; Pottiaux, E. Monitoring of UTC(k)’s using PPP and IGS real-time products. GPS Solut. 2014, 19, 165–172. [Google Scholar] [CrossRef]
- Defraigne, P.; Baire, Q. Combining GPS and GLONASS for time and frequency transfer. Adv. Space Res. 2011, 47, 265–275. [Google Scholar] [CrossRef]
- Ge, Y.; Dai, P.; Qin, W.; Yang, X.; Zhou, F.; Wang, S.; Zhao, X. Performance of Multi-GNSS Precise Point Positioning Time and Frequency Transfer with Clock Modeling. Remote Sens. 2019, 11, 347. [Google Scholar] [CrossRef]
- Zhang, P.; Tu, R.; Zhang, R.; Gao, Y.; Cai, H. Combining GPS, BeiDou, and Galileo Satellite Systems for Time and Frequency Transfer Based on Carrier Phase Observations. Remote Sens. 2018, 10, 324. [Google Scholar] [CrossRef]
- Tu, R.; Zhang, P.; Zhang, R.; Liu, J.; Lu, X. Modeling and performance analysis of precise time transfer based on BDS triple-frequency un-combined observations. J. Geod. 2018, 93, 837–847. [Google Scholar] [CrossRef]
- Su, K.; Jin, S. Triple-frequency carrier phase precise time and frequency transfer models for BDS-3. GPS Solut. 2019, 23, 1–12. [Google Scholar] [CrossRef]
- Jiang, N.; Xu, Y.; Xu, T.; Xu, G.; Sun, Z.; Schuh, H. GPS/BDS short-term ISB modelling and prediction. GPS Solut. 2016, 21, 163–175. [Google Scholar] [CrossRef]
- Tu, R.; Hong, J.; Zhang, P.; Zhang, R.; Fan, L.; Liu, J.; Lu, X. Multiple GNSS inter-system biases in precise time transfer. Meas. Sci. Technol. 2019, 30. [Google Scholar] [CrossRef]
- CSNO. BeiDou Navigation Satellite System Signal in Space Interface Control Document Open Service Signal (Version 2.1); China Satellite Navigation Office (China): Beijing, China, 2016. [Google Scholar]
- CSNO. BeiDou Navigation Satellite System Signal in Space Interface Control Document Open Service Signal B3I (Version 1.0); China Satellite Navigation Office (China): Beijing, China, 2018. [Google Scholar]
- CSNO. BeiDou Navigation Satellite System Signal in Space Interface Control Document Open Service Signal B1I (Version 3.0); China Satellite Navigation Office (China): Beijing, China, 2019. [Google Scholar]
- Lu, M.; Li, W.; Yao, Z.; Cui, X. Overview of BDS III new signals. Navigation 2019, 66, 19–35. [Google Scholar] [CrossRef]
- Jiao, G.; Song, S.; Jiao, W. Improving BDS-2 and BDS-3 joint precise point positioning with time delay bias estimation. Meas. Sci. Technol. 2020, 31. [Google Scholar] [CrossRef]
- Zhang, Y.; Kubo, N.; Chen, J.; Wang, J.; Wang, H. Initial Positioning Assessment of BDS New Satellites and New Signals. Remote Sens. 2019, 11, 1320. [Google Scholar] [CrossRef]
- Qin, W.; Ge, Y.; Zhang, Z.; Su, H.; Wei, P.; Yang, X. Accounting BDS3–BDS2 inter-system biases for precise time transfer. Measurement 2020, 156. [Google Scholar] [CrossRef]
- Montenbruck, O.; Steigenberger, P.; Hauschild, A. Multi-GNSS signal-in-space range error assessment–Methodology and results. Adv. Space Res. 2018, 61, 3020–3038. [Google Scholar] [CrossRef]
- Deng, Z.; Ge, M.; Uhlemann, M.; Zhao, Q. Precise orbit determination of BeiDou satellites at GFZ. In Proceedings of the IGS Workshop, Pasadena, CA, USA, 23–27 June 2014; pp. 23–27. [Google Scholar]
- Deng, Z.; Schuh, H. Improvement of multi-GNSS orbit and clock prediction at GFZ. In Proceedings of the EGU General Assembly Conference Abstracts, Vienna, Austria, 4–13 April 2018; p. 2017. [Google Scholar]
- Ge, M.; Chen, J.; Douša, J.; Gendt, G.; Wickert, J. A computationally efficient approach for estimating high-rate satellite clock corrections in realtime. GPS Solut. 2011, 16, 9–17. [Google Scholar] [CrossRef]
- Guo, J.; Xu, X.; Zhao, Q.; Liu, J. Precise orbit determination for quad-constellation satellites at Wuhan University: Strategy, result validation, and comparison. J. Geod. 2015, 90, 143–159. [Google Scholar] [CrossRef]
- Wang, C.; Zhao, Q.; Guo, J.; Liu, J.; Chen, G. The contribution of intersatellite links to BDS-3 orbit determination: Model refinement and comparisons. Navigation 2019, 66, 71–82. [Google Scholar] [CrossRef]
- Bock, H.; Dach, R.; Jäggi, A.; Beutler, G. High-rate GPS clock corrections from CODE: Support of 1 Hz applications. J. Geod. 2009, 83, 1083–1094. [Google Scholar] [CrossRef]
- Hadas, T.; Krypiak-Gregorczyk, A.; Hernández-Pajares, M.; Kaplon, J.; Paziewski, J.; Wielgosz, P.; Garcia-Rigo, A.; Kazmierski, K.; Sosnica, K.; Kwasniak, D.; et al. Impact and Implementation of Higher-Order Ionospheric Effects on Precise GNSS Applications. J. Geophys. Res. Solid Earth 2017, 122, 9420–9436. [Google Scholar] [CrossRef]
- Odijk, D.; Teunissen, P.J.G. Characterization of between-receiver GPS-Galileo inter-system biases and their effect on mixed ambiguity resolution. GPS Solut. 2012, 17, 521–533. [Google Scholar] [CrossRef]
- Rebischung, P.; Schmid, R. IGS14/igs14.atx: A new framework for the IGS products. In Proceedings of the AGU Fall Meeting 2016, San Francisco, CA, USA, 12–16 December 2016; Volume 121, pp. 6109–6131. [Google Scholar]
- Wang, N.; Yuan, Y.; Li, Z.; Montenbruck, O.; Tan, B. Determination of differential code biases with multi-GNSS observations. J. Geod. 2015, 90, 209–228. [Google Scholar] [CrossRef]
- Montenbruck, O.; Steigenberger, P.; Prange, L.; Deng, Z.; Zhao, Q.; Perosanz, F.; Romero, I.; Noll, C.; Stürze, A.; Weber, G.; et al. The Multi-GNSS Experiment (MGEX) of the International GNSS Service (IGS)–Achievements, prospects and challenges. Adv. Space Res. 2017, 59, 1671–1697. [Google Scholar] [CrossRef]
- Landskron, D.; Bohm, J. VMF3/GPT3: Refined discrete and empirical troposphere mapping functions. J. Geod. 2018, 92, 349–360. [Google Scholar] [CrossRef] [PubMed]
- Wanninger, L.; Beer, S. BeiDou satellite-induced code pseudorange variations: Diagnosis and therapy. GPS Solut. 2014, 19, 639–648. [Google Scholar] [CrossRef]
- Yang, Y.; Xu, Y.; Li, J.; Yang, C. Progress and performance evaluation of BeiDou global navigation satellite system: Data analysis based on BDS-3 demonstration system. Sci. China Earth Sci. 2018, 61, 614–624. [Google Scholar] [CrossRef]
- Su, K.; Jin, S.; Hoque, M. Evaluation of Ionospheric Delay Effects on Multi-GNSS Positioning Performance. Remote Sens. 2019, 11, 171. [Google Scholar] [CrossRef]
- Kazmierski, K.; Sośnica, K.; Hadas, T. Quality assessment of multi-GNSS orbits and clocks for real-time precise point positioning. GPS Solut. 2017, 22, 1–12. [Google Scholar] [CrossRef]
- Wu, J.-T.; Wu, S.C.; Hajj, G.A.; Bertiger, W.I.; Lichten, S.M. Effects of antenna orientation on GPS carrier phase. In Proceedings of the Astrodynamics 1991, San Diego, CA, USA, 19–22 August 1991; pp. 1647–1660. [Google Scholar]
- Kouba, J. A Guide to Using International GNSS Service (IGS) Products; Jet Propulsion Laboratory: Pasadena, CA, USA, 2009; p. 34. [Google Scholar]
- Petit, G.; Luzum, B. IERS Conventions (2010); IERS Technical Note No. 36; Verlag des Bundesamts für Kartographie und Geodäsie: Frankfurt am Main, Germany, 2010. [Google Scholar]




| System | Signal | Signal Component | Carrier Frequency (MHz) | Modulation | Chip Rate (Mcps) |
|---|---|---|---|---|---|
| BDS-2 | B1I | - | 1561.098 | Quadrature phase-shift keying (QPSK) | 2.046 |
| B2I | - | 1207.140 | QPSK | 2.046 | |
| B3I | - | 1268.520 | Binary phase-shift keying (BPSK) | 10.23 | |
| BDS-3 | B1I | - | 1561.098 | BPSK | 2.046 |
| B1C | B1C_data | 1575.420 | Binary offset carrier (BOC) | 1.023 | |
| B1C_pilot | Quadrature Multiplexed Binary Offset Carrier (QMBOC) | ||||
| B2a | - | 1176.450 | BPSK | 10.23 | |
| B2b | - | 1207.140 | BPSK | 10.23 | |
| B3I | - | 1268.520 | BPSK | 10.23 |
| Station | Receiver | Antenna | Signal | Clock |
|---|---|---|---|---|
| XIA1 | GNSS-GGR | RINT-8CH | B1I/B3I/B1C/B2a | H-MASTER |
| BRUX | SEPT POLARX5TR | JAVRINGANT_DM | B1I/B3I/B1C/B2a | H-MASTER |
| NNOR | SEPT POLARX5TR | SEPCHOKE_B3E6 | B1I/B3I/B1C/B2a | SLAVED CRYSTAL |
| HARB | SEPT POLARX5TR | TRM59800.00 | B1I/B3I/B1C/B2a | CESIUM |
| PTBB | SEPT POLARX5TR | LEIAR25.R4 | B1I/B3I | H-MASTER |
| HOB2 | SEPT POLARX5 | AOAD/M_T | B1I/B3I | H-MASTER |
| USUD | SEPT POLARX5 | AOAD/M_T | B1I/B3I | H-MASTER |
| Items | Strategies |
|---|---|
| Observations | Pseudo-range and carrier phase observations |
| Frequency point | BDS-2: B1I/B3I BDS-3: B1I/B3I and B1C/B2a |
| Elevation cutoff | 7.5° |
| Observation weighting | Elevation weight [sin(elevation)] |
| Satellite orbit | Fixed to GFZ or WHU precise orbit products |
| Satellite clock offsets | Fixed to GFZ or WHU precise clock products |
| Tropospheric delay | Modified Hopfield for dry part and estimated for wet part (10−9 m2/s) |
| Ionospheric delay | IF-PPP: eliminated first order by IF observations |
| Satellite antenna | IGS MGEX values |
| Receiver antenna | IGS MGEX values |
| Phase windup effect | Corrected [41] |
| Relativistic effect | Corrected [42] |
| Earth rotation | Corrected [43] |
| Tide effect | Solid Earth, Pole and Ocean tide [43] |
| Satellite multipath effect | BDS-2: Corrected BDS-3: Non-existent |
| Receiver coordinate | Estimated as constants |
| Receiver clock offsets | Estimated as white noise process (105 m2/s) |
| ISB | ISBNO; ISBCV; ISBRW (10−6 m2/s); ISBWN (105 m2/s) |
| Satellite DCB | Corrected using CAS products |
| Ambiguity | Estimated as constants |
| ISB | Station | GFZ | WHU | ||||
|---|---|---|---|---|---|---|---|
| Mean | STD | RMS | Mean | STD | RMS | ||
| BDS-2(B1I/B3I)/ BDS-3(B1I/B3I) | BRUX | −6.53 ns | 1.36 ns | 6.77 ns | −4.40 ns | 1.06 ns | 4.60 ns |
| PTBB | −5.77 ns | 0.93 ns | 5.90 ns | −3.53 ns | 0.70 ns | 3.63 ns | |
| HARB | −4.16 ns | 0.56 ns | 4.20 ns | −1.83 ns | 0.53 ns | 1.96 ns | |
| HOB2 | −4.77 ns | 0.46 ns | 4.77 ns | −1.76 ns | 0.46 ns | 1.80 ns | |
| NNOR | −5.43 ns | 0.23 ns | 5.43 ns | −2.23 ns | 0.23 ns | 2.23 ns | |
| USUD | −5.00 ns | 0.26 ns | 5.07 ns | −1.70 ns | 0.30 ns | 2.00 ns | |
| XIA1 | −3.56 ns | 0.40 ns | 3.73 ns | 0.13 ns | 0.43 ns | 1.10 ns | |
| BDS-2(B1I/B3I)/ BDS-3(B1C/B2a) | BRUX | −9.70 ns | 1.43 ns | 9.87 ns | −7.60 ns | 1.06 ns | 7.70 ns |
| HARB | −4.40 ns | 0.53 ns | 4.46 ns | −2.10 ns | 0.40 ns | 2.13 ns | |
| NNOR | 3.23 ns | 0.23 ns | 3.26 ns | 6.43 ns | 0.16 ns | 6.47 ns | |
| XIA1 | 99.96 ns | 0.36 ns | 99.96 ns | 103.97 ns | 0.56 ns | 103.97 ns |
| Items | Station | BDS-2 (B1I/B3I) | BDS-3 (B1I/B3I) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| ISBNO | ISBCV | ISBRW | ISBWN | ISBNO | ISBCV | ISBRW | ISBWN | ||
| Mean | BRUX | 2.11 m | 0.86 m | 0.77 m | 0.61 m | −0.33 m | −0.03 m | 0.02 m | 0.03 m |
| PTBB | 2.05 m | 0.88 m | 0.83 m | 0.73 m | −0.26 m | 0.09 m | 0.12 m | 0.11 m | |
| HARB | 1.53 m | 0.60 m | 0.60 m | 0.58 m | −0.34 m | −0.06 m | −0.05 m | −0.06 m | |
| HOB2 | 1.16 m | 0.50 m | 0.50 m | 0.50 m | −0.74 m | 0.01 m | 0.01 m | 0.01 m | |
| NNOR | 0.93 m | 0.33 m | 0.32 m | 0.33 m | −1.04 m | 0.01 m | 0.02 m | 0.01 m | |
| USUD | 0.91 m | 0.25 m | 0.24 m | 0.24 m | −0.87 m | 0.03 m | 0.05 m | 0.04 m | |
| XIA1 | 0.50 m | −0.07 m | −0.07 m | −0.07 m | −0.48 m | 0.06 m | 0.07 m | 0.04 m | |
| STD | BRUX | 1.62 m | 1.62 m | 1.62 m | 1.65 m | 0.71 m | 0.71 m | 0.71 m | 0.66 m |
| PTBB | 2.04 m | 2.04 m | 2.04 m | 2.05 m | 1.25 m | 1.25 m | 1.25 m | 1.25 m | |
| HARB | 1.27 m | 1.27 m | 1.27 m | 1.29 m | 0.72 m | 0.72 m | 0.72 m | 0.70 m | |
| HOB2 | 1.07 m | 1.07 m | 1.07 m | 1.07 m | 0.83 m | 0.83 m | 0.83 m | 0.82 m | |
| NNOR | 1.08 m | 1.08 m | 1.08 m | 1.08 m | 0.99 m | 0.99 m | 0.99 m | 0.99 m | |
| USUD | 1.26 m | 1.26 m | 1.26 m | 1.26 m | 1.04 m | 1.04 m | 1.04 m | 1.01 m | |
| XIA1 | 1.04 m | 1.04 m | 1.03 m | 1.03 m | 0.80 m | 0.80 m | 0.79 m | 0.78 m | |
| Items | Station | BDS-2 (B1I/B3I) | BDS-3 (B1C/B2a) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| ISBNO | ISBCV | ISBRW | ISBWN | ISBNO | ISBCV | ISBRW | ISBWN | ||
| Mean | BRUX | 3.10 m | 0.86 m | 0.76 m | 0.61 m | −0.31 m | −0.02 m | 0.01 m | 0.01 m |
| HARB | 1.64 m | 0.64 m | 0.62 m | 0.57 m | −0.12 m | 0.05 m | 0.08 m | 0.09 m | |
| NNOR | −0.46 m | 0.34 m | 0.33 m | 0.34 m | 0.47 m | −0.01 m | 0.02 m | 0.01 m | |
| XIA1 | −19.72 m | −0.08 m | −0.08 m | −0.07 m | 10.30 m | 0.06 m | 0.05 m | 0.03 m | |
| STD | BRUX | 1.62 m | 1.62 m | 1.62 m | 1.66 m | 0.48 m | 0.47 m | 0.47 m | 0.43 m |
| HARB | 1.29 m | 1.28 m | 1.28 m | 1.29 m | 0.49 m | 0.49 m | 0.50 m | 0.43 m | |
| NNOR | 1.08 m | 1.08 m | 1.08 m | 1.08 m | 0.66 m | 0.65 m | 0.65 m | 0.65 m | |
| XIA1 | 1.21 m | 1.03 m | 1.03 m | 1.03 m | 0.82 m | 0.52 m | 0.52 m | 0.45 m | |
| Agency /Frequency | Strategy | BRUX-XIA1 | PTBB-XIA1 | HARB-XIA1 | HOB2-XIA1 | NNOR-XIA1 | USUD-XIA1 | Mean |
|---|---|---|---|---|---|---|---|---|
| GFZ (B1IB3I) | BDS-2–IGSGPS | 1.58 ns | 1.29 ns | 0.85 ns | 0.57 ns | 0.77 ns | 0.72 ns | 0.96 ns |
| BDS-3 –IGSGPS | 0.78 ns | 0.85 ns | 0.80 ns | 0.71 ns | 0.79 ns | 0.69 ns | 0.77 ns | |
| ISBNO–IGSGPS | 0.79 ns | 0.89 ns | 0.78 ns | 0.61 ns | 0.72 ns | 0.72 ns | 0.75 ns | |
| ISBCV–IGSGPS | 0.76 ns | 0.86 ns | 0.78 ns | 0.56 ns | 0.67 ns | 0.68 ns | 0.71 ns | |
| ISBRW–IGSGPS | 0.82 ns | 0.87 ns | 0.79 ns | 0.57 ns | 0.68 ns | 0.69 ns | 0.73 ns | |
| ISBWN–IGSGPS | 1.10 ns | 1.24 ns | 0.84 ns | 0.58 ns | 0.70 ns | 0.68 ns | 0.85 ns | |
| GFZ (B1CB2a) | BDS-3–IGSGPS | 0.74 ns | - | 0.84 ns | - | 0.72 ns | - | 0.76 ns |
| ISBNO–IGSGPS | 1.33 ns | - | 1.40 ns | - | 1.29 ns | - | 1.34 ns | |
| ISBCV–IGSGPS | 0.84 ns | - | 0.80 ns | - | 0.68 ns | - | 0.77 ns | |
| ISBRW–IGSGPS | 0.85 ns | - | 0.82 ns | - | 0.68 ns | - | 0.78 ns | |
| ISBWN–IGSGPS | 1.51 ns | - | 0.88 ns | - | 0.66 ns | - | 1.01 ns | |
| WHU (B1IB3I) | BDS-2-IGSGPS | 1.39 ns | 1.14 ns | 0.87 ns | 0.68 ns | 0.75 ns | 0.74 ns | 0.92 ns |
| BDS-3-IGSGPS | 0.82 ns | 0.89 ns | 0.79 ns | 0.73 ns | 0.69 ns | 0.68 ns | 0.76 ns | |
| ISBNO–IGSGPS | 0.87 ns | 0.88 ns | 0.78 ns | 0.64 ns | 0.68 ns | 0.68 ns | 0.75 ns | |
| ISBCV–IGSGPS | 0.80 ns | 0.84 ns | 0.77 ns | 0.62 ns | 0.66 ns | 0.66 ns | 0.72 ns | |
| ISBR–IGSGPS | 0.82 ns | 0.85 ns | 0.80 ns | 0.64 ns | 0.68 ns | 0.67 ns | 0.74 ns | |
| ISBWN–IGSGPS | 1.31 ns | 1.10 ns | 0.83 ns | 0.65 ns | 0.70 ns | 0.68 ns | 0.87 ns | |
| WHU (B1CB2a) | BDS-3-IGSGPS | 0.74 ns | - | 0.82 ns | - | 0.68 ns | - | 0.74 ns |
| ISBNO–IGSGPS | 1.51 ns | - | 1.53 ns | - | 1.43 ns | - | 1.49 ns | |
| ISBCV–IGSGPS | 0.77 ns | - | 0.75 ns | - | 0.67 ns | - | 0.73 ns | |
| ISBRW–IGSGPS | 0.82 ns | - | 0.82 ns | - | 0.68 ns | - | 0.77 ns | |
| ISBWN–IGSGPS | 1.31 ns | - | 0.85 ns | - | 0.68 ns | - | 0.94 ns |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiao, G.; Song, S.; Chen, Q.; Huang, C.; Su, K.; Wang, Z.; Cheng, N. Modeling and Analysis of BDS-2 and BDS-3 Combined Precise Time and Frequency Transfer Considering Stochastic Models of Inter-System Bias. Remote Sens. 2021, 13, 793. https://doi.org/10.3390/rs13040793
Jiao G, Song S, Chen Q, Huang C, Su K, Wang Z, Cheng N. Modeling and Analysis of BDS-2 and BDS-3 Combined Precise Time and Frequency Transfer Considering Stochastic Models of Inter-System Bias. Remote Sensing. 2021; 13(4):793. https://doi.org/10.3390/rs13040793
Chicago/Turabian StyleJiao, Guoqiang, Shuli Song, Qinming Chen, Chao Huang, Ke Su, Zhitao Wang, and Na Cheng. 2021. "Modeling and Analysis of BDS-2 and BDS-3 Combined Precise Time and Frequency Transfer Considering Stochastic Models of Inter-System Bias" Remote Sensing 13, no. 4: 793. https://doi.org/10.3390/rs13040793
APA StyleJiao, G., Song, S., Chen, Q., Huang, C., Su, K., Wang, Z., & Cheng, N. (2021). Modeling and Analysis of BDS-2 and BDS-3 Combined Precise Time and Frequency Transfer Considering Stochastic Models of Inter-System Bias. Remote Sensing, 13(4), 793. https://doi.org/10.3390/rs13040793







