Altitude on Cartographic Materials and Its Correction According to New Measurement Techniques
Abstract
1. Introduction
2. Background on Altitude Measurements in the Mountains
2.1. Historical Methods
2.2. GNSS and Its Accuracy
2.3. LiDAR Measurements
2.4. Other Methods for Height Determination
2.5. Reference System and the Geoid Model Issue
3. Materials and Methods
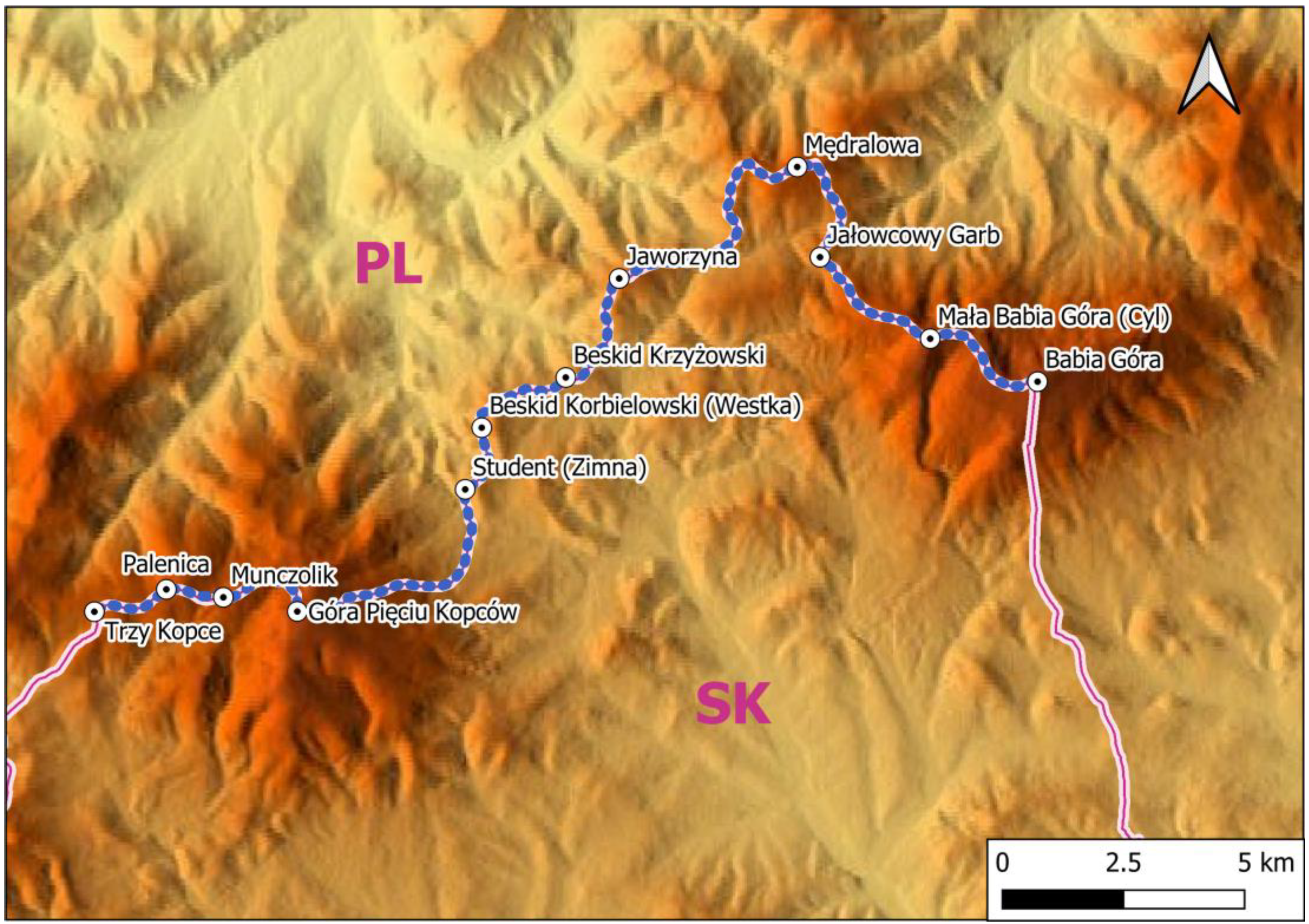
3.1. Study Area
3.2. Methods
- Quasi-geoid model error, which, according to the pl-geoid-2011 model [109], was adopted as the maximum—10 cm—due to measuring in mountaineering areas. This was the same for all peaks.
- Errors of determining the highest point, levelling, and centric error—2 cm. This was the same for all peaks.
- GNSS positioning error (Table A1, column 27: GNSS accuracy), which was based on the RTK/static post-processing reports, and altitude error in the range from 1.3 cm to 4.6 cm.
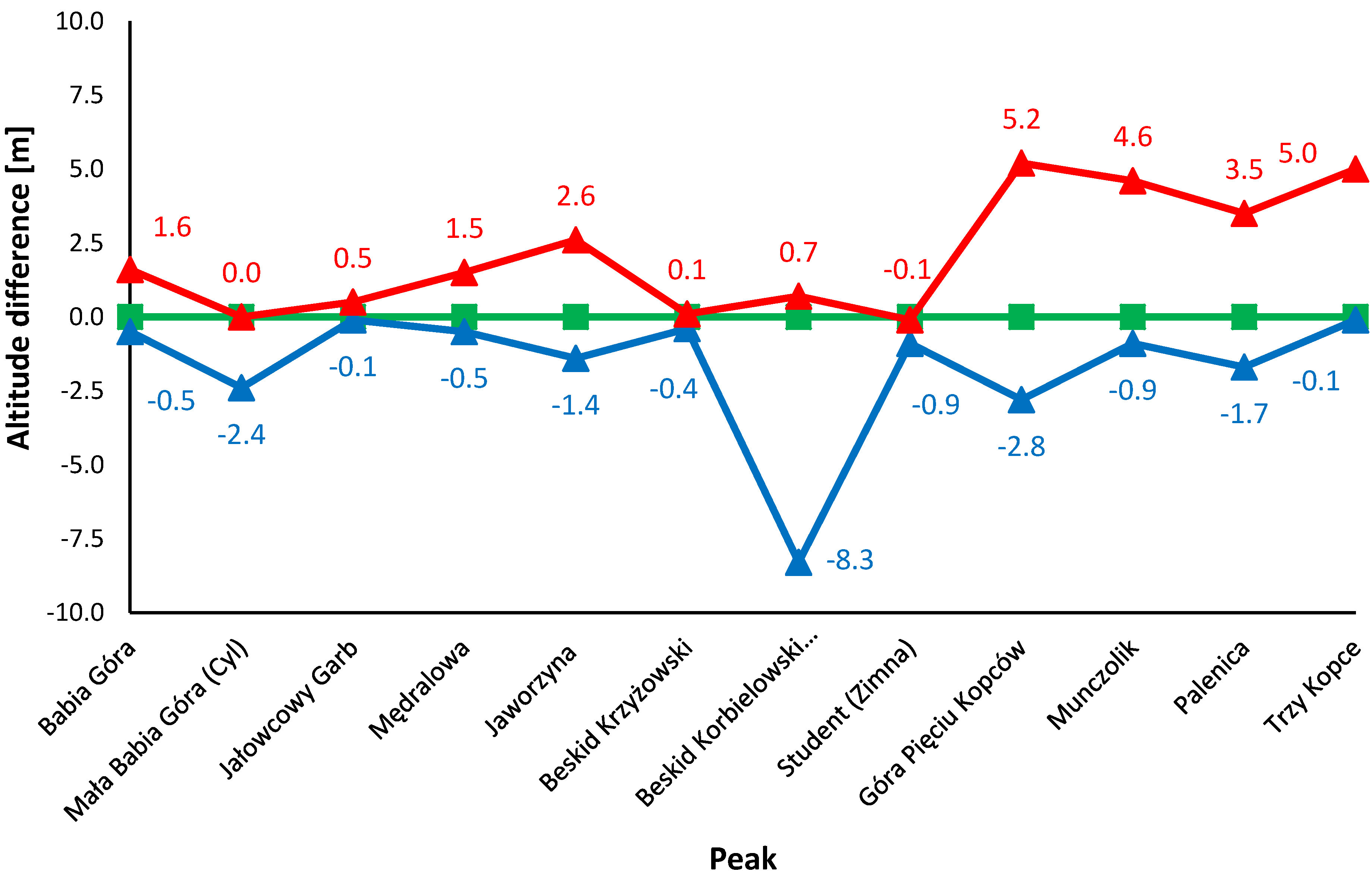
4. Results and Discussion
- -
- The uncertain position of the “peak” and simultaneous difficulty in measuring an orthometric/normal height not directly, but, e.g., by stereoscopic photogrammetry, which has been a popular method in Poland since the 1950s;
- -
- The presence of vegetation and therefore photogrammetric identification errors (height differences between the DSM and DTM), especially for small-scale maps and photos;
- -
- Different survey techniques and equipment used;
- -
- Different height systems and vertical data used from 1875;
- -
- Systematic errors.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | 25 | 26 | 27 | 28 | 29 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| No | Source (Table 2) | I | II | III | IV | V | VI | VII | VIII | IX | X | XI | XII | XIII | XIV | XV | XVI | XVII | XVIII | XIV | XX | Max | No of Sources | Method * | GNSS | GNSS Accuracy ** | Altitude Accuracy *** | LiDAR | |
| Summit | |||||||||||||||||||||||||||||
| 1 | Babia Góra | 1725 | 1723 | 1724 | 1725 | 1725 | 1724 | 1724 | 1725 | 1724.8 | 1725 | 1725 | 1722.9 | 1722.9 | 1725 | 1724.6 | 1724.6 | 1725 | 1725 | 2.1 | 18 | R | 1723.4 | 0.030 | 0.11 | 1723.0 | |||
| 2 | Mała Babia Góra (Cyl) | 1517 | 1515 | 1517 | 1515 | 1517 | 1517 | 1517 | 1517 | 1514.6 | 1517 | 1517 | 1514.8 | 1514.8 | 1515 | 1515 | 1515 | 1517 | 1517 | 2.4 | 18 | S | 1517.0 | 0.018 | 0.10 | 1516.9 | |||
| 3 | Jałowcowy Garb | 1017 | 1017 | 1017 | 1017 | 1017 | 1017 | 0.0 | 6 | S | 1016.5 | 0.046 | 0.11 | 1016.4 | |||||||||||||||
| 4 | Mędralowa | 1169 | 1169 | 1169 | 1168 | 1169 | 1169 | 1169 | 1170 | 1168.1 | 1170 | 1170 | 1168.5 | 1168.5 | 1169 | 1169 | 1169 | 1169 | 1169 | 2.0 | 18 | S | 1168.5 | 0.041 | 0.11 | 1168.4 | |||
| 5 | Jaworzyna | 1047 | 1047 | 1050 | 1047 | 1047 | 1050 | 1050 | 1050 | 1046.5 | 1050 | 1050 | 1046 | 1046 | 1047.3 | 1047.3 | 1047 | 1047 | 1047 | 1047 | 1047 | 4.0 | 20 | S | 1047.4 | 0.029 | 0.11 | 1047.3 | |
| 6 | Beskid Krzyżowski | 923 | 923 | 923 | 923 | 923 | 923.4 | 923 | 923 | 923.4 | 923.4 | 923 | 923 | 923 | 923 | 923 | 0.4 | 15 | R | 923.4 | 0.027 | 0.11 | 923.5 | ||||||
| 7 | Beskid Korbielowski (Westka) | 955 | 954 | 954 | 954 | 946 | 946 | 948 | 954.5 | 954 | 954 | 954 | 955 | 955 | 954 | 955 | 9.0 | 15 | R | 954.3 | 0.015 | 0.10 | 954.1 | ||||||
| 8 | Student (Zimna) | 935 | 935 | 935 | 935 | 935 | 935 | 935 | 935 | 935 | 935 | 935 | 935 | 0.0 | 12 | R | 935.9 | 0.020 | 0.10 | 935.8 | |||||||||
| 9 | Góra Pięciu Kopców | 1534 | 1534 | 1542 | 1542 | 8.0 | 4 | S | 1536.8 | 0.013 | 0.10 | 1536.9 | |||||||||||||||||
| 10 | Munczolik | 1356 | 1356 | 1356 | 1356 | 1350.5 | 1356 | 1356 | 1350.8 | 1356 | 1356 | 1356 | 1356 | 5.5 | 12 | R | 1351.4 | 0.022 | 0.10 | 1351.5 | |||||||||
| 11 | Palenica | 1343 | 1338 | 1343 | 1339 | 1343 | 1343 | 1338.8 | 1343 | 1343 | 1337.8 | 1339.1 | 1343 | 1343 | 1343 | 1338 | 1343 | 5.2 | 16 | R | 1339.5 | 0.019 | 0.10 | 1339.4 | |||||
| 12 | Trzy Kopce | 1216 | 1216 | 1216 | 1216 | 1216 | 1211.4 | 1216 | 1216 | 1213.4 | 1211.7 | 1216 | 1216 | 1216 | 1216 | 4.6 | 14 | S | 1211.0 | 0.032 | 0.11 | 1210.9 | |||||||
| No of summits | 12 | 12 | 7 | 6 | 7 | 9 | 9 | 7 | 9 | 4 | 4 | 7 | 7 | 7 | 8 | 7 | 11 | 12 | 11 | 12 | |||||||||
References
- Podobnikar, T. Detecting Mountain Peaks and Delineating Their Shapes Using Digital Elevation Models, Remote Sensing and Geographic Information Systems Using Autometric Methodological Procedures. Remote Sens. 2012, 4, 784–809. [Google Scholar] [CrossRef]
- Buscaini, G.; Labande, F.; Brandt, M. The 4000ers of the Alps—Official UIAA List. UIAA Bull. 1994, 9–16. Available online: http://www.hikr.org/files/40196.pdf (accessed on 14 January 2021).
- Munro, H. Tables Giving All the Scottish Mountains Exceeding 3000 Feet in Height. Scott. Mt. Club J. 1891, 1, 279–281. [Google Scholar]
- Apollo, M.; Mostowska, J.; Maciuk, K.; Wengel, Y.; Jones, T.E.; Cheer, J.M. Peak-Bagging and Cartographic Misrepresentations: A Call to Correction. Curr. Issues Tour. 2020, 1–6. [Google Scholar] [CrossRef]
- Bilham, R. Height Measurements Mt Everest. Available online: http://dos.gov.np/everest/downloads/Roger_Bilham.pdf (accessed on 15 November 2020).
- Cajori, F. History of Determinations of the Heights of Mountains. Isis 1929, 12, 482–514. [Google Scholar] [CrossRef]
- Kudrys, J.; Buśko, M.; Kozioł, K.; Maciuk, K. Determination of the Normal Height of Chornohora Summits by a Precise Modern Measurement Techniques. Maejo Int. J. Sci. Technol. 2020, 14, 156–165. [Google Scholar]
- Langley, R.B. Innovation: GLONASS—Past, Present and Future. GPS World. Available online: https://www.gpsworld.com/innovation-glonass-past-present-and-future/ (accessed on 21 November 2020).
- Benedicto, J. Directions 2020: Galileo Moves Ahead. GPS World. Available online: https://www.gpsworld.com/directions-2020-galileo-moves-ahead/ (accessed on 1 December 2020).
- Barnes, D. GPS Status and Modernization Plans. In Proceedings of the Munich Satellite Navigation Summit 2019, Munich, Germany, 25–27 March 2019. [Google Scholar]
- Hein, G.W. Status, Perspectives and Trends of Satellite Navigation. Satell. Navig. 2020, 1, 22. [Google Scholar] [CrossRef]
- Zrinjski, M.; Matika, K.; Barković, Đ. Razvoj i Modernizacija GNSS-a. Geod. List 2019, 73, 45–65. [Google Scholar]
- Ward, M. The Height of Mount Everest. Alp. J. 1995, 100, 30–33. [Google Scholar] [CrossRef]
- Junyong, C.; Yanping, Z.; Janli, Y.; Chunxi, G.; Peng, Z. Height Determination of Qomolangma Feng (MT. Everest) in 2005. Surv. Rev. 2010, 42, 122–131. [Google Scholar] [CrossRef]
- De Graaff-Hunter, J. De Various Determinations over a Century of the Height of Mount Everest. Geogr. J. 1955, 121, 21. [Google Scholar] [CrossRef]
- Poretti, G. Is Mt. Everest Higher Than 100 Years Ago. In The Seventy Great Mysteries of the Natural World; Thames & Hudson: London, UK, 2008; pp. 68–70. [Google Scholar]
- De Beer, G. The History of the Altimetry of Mont Blanc. Ann. Sci. 1956, 12, 3–29. [Google Scholar] [CrossRef]
- Poretti, G.G. America’s highest peak now measures 6962 metres! Report. Mag. Leica Geosystems. 1999, 47, 28–29. [Google Scholar]
- Saburi, J.; Angelakis, N.; Jaeger, R.; Illner, M.; Jackson, P.; Pugh, K.T. Height Measurement of Kilimanjaro. Surv. Rev. 2000, 35, 552–562. [Google Scholar] [CrossRef]
- Pérez, O.J.; Hoyer, M.; Hernández, J.; Rodríguez, C.; Márques, V.; Sué, N.; Velandia, J.; Fernandes, J.; Deiros, D. GPS Height Measurement of Peak Bolivar, Venezuela. Surv. Rev. 2006, 38, 697–702. [Google Scholar] [CrossRef]
- Kozioł, K.; Maciuk, K. New Heights of the Highest Peaks of Polish Mountain ranges. Remote Sens. 2020, 12, 1446. [Google Scholar] [CrossRef]
- Gulatee, B.L. Heights of Himalayan Snow Peaks. J. Meteorol. Geophys. 1952, 17, 165–172. [Google Scholar]
- Pugh, K.T. Height Determination of Kilimanjaro. Emp. Surv. Rev. 1954, 91, 194–206. [Google Scholar] [CrossRef]
- Poretti, G.; Mandler, R.; Lipizer, M. The Height of Mountains. Boll. Geofis. Teor. Appl. 2006, 47, 557–575. [Google Scholar]
- Poretti, G. Quanto è Alto il Monte Everest? Tessere, G., Ed.; CUEN Napoli: Napoli, Italy, 1995. [Google Scholar]
- Poretti, G. Geophysical, Geological and Geographycal features of the Himalayas. In Ecovision World Monograph Series; Schweizerbart Science Publishers: Sttutgart, Germany, 1998; pp. 19–34. [Google Scholar]
- Poretti, G.; Lohmar, F.J.; Puruckherr, R.; Marchesini, C.; Beinat, A.; Eckardt, M.; Marchesini, A. Geodetic Measurements in the Himalayas and New Determination of the Height of Mount K2. Boll. Geofis. Teor. Appl. 2000, 41, 219–231. [Google Scholar]
- Rondeau, M.C. Scanning the Top of Europe 2020. Available online: https://leica-geosystems.com/case-studies/surveying-and-engineering/scanning-the-top-of-europe (accessed on 15 November 2020).
- Yamagishi, H.; Bhandary, N.P. (Eds.) GIS Landslide; Springer: Tokyo, Japan, 2017; ISBN 978-4-431-54390-9. [Google Scholar]
- Liu, X. Accuracy Assessment of Lidar Elevation Data Using Survey Marks. Surv. Rev. 2011, 43, 80–93. [Google Scholar] [CrossRef]
- Featherstone, W.E.; Kirby, J.F.; Kearsley, A.H.W.; Gilliland, J.R.; Johnston, G.M.; Steed, J.; Forsberg, R.; Sideris, M.G. The AUSGeoid98 Geoid Model of Australia: Data Treatment, Computations and Comparisons with GPS-Levelling Data. J. Geod. 2001, 75, 313–330. [Google Scholar] [CrossRef]
- Kenyeres, A. GPS/Leveling; Springer: Cham, Switzeraland, 1994. [Google Scholar] [CrossRef]
- Fotopoulos, G. UCGE Reports an Analysis on the Optimal Combination of Geoid, Orthometric and Ellipsoidal Height Data. 2003. Available online: https://www.ucalgary.ca/engo_webdocs/MGS/03.20185.GFotopoulos.pdf (accessed on 15 November 2020).
- Meyer, T.H.; Roman, D.R.; Zilkoski, D.B. What Does Height Really Mean? Part IV: GPS Orthometric Heighting; American Association for Geodetic Surveying: Gaithersburg, MD, USA, 2006. [Google Scholar]
- Hirt, C.; Wübbena, G. Mutual Validation of GNSS Height Measurements and High-Precision Geometric-Astronomical Levelling. GPS Solut. 2011, 15, 149–159. [Google Scholar] [CrossRef]
- Erenoglu, R.C.; Yucel, M.A.; Pirti, A.; Sanli, D.U. On the Performance of GNSS Levelling over Steep Slopes. Bol. Ciências Geodésicas 2012, 18, 645–660. [Google Scholar] [CrossRef]
- Erol, B.; Erol, S. GNSS in Practical Determination of Regional Heights. Glob. Navig. Satell. Syst. Signal Theory Appl. 2012. [Google Scholar] [CrossRef]
- Featherstone, W.E.; Stewart, M.P. Possible Evidence for Distortions in the Australian Height Datum in Western Australia. Aust. J. Geod. Photogramm. Surv. 1998, 68, 1–11. [Google Scholar]
- Zumberge, J.F.; Heflin, M.B.; Jefferson, D.C.; Watkins, M.M.; Webb, F.H. Precise Point Positioning for the Efficient and Robust Analysis of GPS Data from Large Networks. J. Geophys. Res. Solid Earth 1997, 102, 5005–5017. [Google Scholar] [CrossRef]
- Kouba, J.A.N.; Heroux, P. Precise Point Positioning Using IGS Orbit and Clock Products. GPS Solut. 2001, 5, 12–28. [Google Scholar] [CrossRef]
- Gao, Y.; Chen, K. Performance Analysis of Precise Point Positioning Using Rea-Time Orbit and Clock Products. J. Glob. Position. Syst. 2004, 3, 95–100. [Google Scholar] [CrossRef]
- Leandro, R.F.; Santos, M.; Langley, R.B. Analyzing GNSS Data in Precise Point Positioning Software Analyzing GNSS Data in Precise Point Positioning Software. GPS Solut. 2011. [Google Scholar] [CrossRef]
- Li, X.; Ge, M.; Dai, X.; Ren, X.; Fritsche, M.; Wickert, J.; Schuh, H. Accuracy and Reliability of Multi-GNSS Real-Time Precise Positioning: GPS, GLONASS, BeiDou, and Galileo. J. Geod. 2015, 89, 607–635. [Google Scholar] [CrossRef]
- Sterle, O.; Stopar, B.; Pavlovčič Prešeren, P. Single-Frequency Precise Point Positioning: An Analytical Approach. J. Geod. 2015, 89. [Google Scholar] [CrossRef]
- Higgins, M.B. Heighting with GPS: Possibilities and Limitations. Comm. 5 Int. Fed. Surv. 1999, 1–10. Available online: https://www.semanticscholar.org/paper/HEIGHTING-WITH-GPS-%3A-POSSIBILITIES-AND-LIMITATIONS-Higgins/8b6f8a55603742d5a17e2735f4322be6311ef341 (accessed on 14 January 2021).
- Wu, J.; Yiu, F.-G. Local Height Determination Using GPS-Monitored Atmospheric Path Delays. J. Surv. Eng. 2001, 127, 1–11. [Google Scholar] [CrossRef]
- Wu, J.; Lin, S.-G. Leveling by GPS Relative Positioning with Carrier Phases. J. Surv. Eng. 1996, 122, 145–157. [Google Scholar] [CrossRef]
- Featherstone, W.E.E.; Dentith, M.C.C.; Kirby, J.F.F. Strategies for the Accurate Determination of Orthometric Heights from GPS. Surv. Rev. 1998, 34, 278–296. [Google Scholar] [CrossRef]
- Jamal, S.; Arif, T.; Hassan, A.; Anom, W.; Aris, W.; Shen, W.; Faiz, M. Geodesy and Geodynamics Influencing Factors on the Accuracy of Local Geoid Model. Geod. Geodyn. 2019, 10. [Google Scholar] [CrossRef]
- Gruber, T. Evaluation of the EGM2008 Gravity Field by Means of GPS-Levelling and Sea Surface Topography Solutions. Newtons Bull 2009, 4, 3–17. [Google Scholar]
- Mouratidis, A.; Ampatzidis, D. European Digital Elevation Model Validation against Extensive Global Navigation Satellite Systems Data and Comparison with SRTM DEM and ASTER GDEM in Central Macedonia (Greece). ISPRS Int. J. Geo Inf. 2019, 8, 108. [Google Scholar] [CrossRef]
- Featherstone, W.E. Improvement to Long-Wavelength Australian Gravity Anomalies Expected from the CHAMP, GRACE and GOCE Dedicated Satellite Gravimetry Missions. Explor. Geophys. 2003, 34, 69–76. [Google Scholar] [CrossRef]
- Bilker, M. Evaluation of the New Global Gravity Field Models from CHAMP and GRACE with GPS-Levelling Data in Fennoscandia. In Proceedings of the XXII Geofysiikan Päivät, Helsinki, Finland, 19–20 May 2005; pp. 21–26. [Google Scholar]
- Kotsakis, C.; Katsambalos, K. Quality Analysis of Global Geopotential Models at 1542 GPS/Levelling Benchmarks over the Hellenic Mainland. Surv. Rev. 2010, 42, 327–344. [Google Scholar] [CrossRef]
- Gad, M.A.; Odalović, O.R. Evaluation the Performance of Recently Global Geopotential Models GGMs Over Egypt. Int. J. Sci. Eng. Res. 2017, 8, 936. [Google Scholar] [CrossRef]
- Erol, B.; Celik, R.N. Precise Local Geoid Determination to Make GPS Technique More Effective in Practical Applications of Geodesy. In Proceedings of the FIG Working Week 2004, Athens, Greece, 22–27 May 2004; pp. 1–13. [Google Scholar]
- Banasik, P.; Bujakowski, K. The Use of Quasigeoid in Leveling Through Terrain Obstacles. Rep. Geod. Geoinformatics 2018, 104, 57–64. [Google Scholar] [CrossRef]
- Heiskanen, W.A.; Moritz, H. Physical Geodesy; Springer: Wien, Austria, 1967. [Google Scholar]
- Yilmaz, S.A. Improvement of Elipsoidal Heights with Athmospherical Data Calculated from GNSS Data Improvement of Elipsoidal Heights with Athmospherical Data Calculated from GNSS Data. 2011. Available online: https://www.fig.net/resources/proceedings/fig_proceedings/fig2011/papers/ts08i/ts08i_yilmaz_5203.pdf (accessed on 22 November 2020).
- Schu, T. Impact of Systematic Errors on Precise Long-Baseline Kinematic GPS Positioning. GPS Solut. 2006, 108–125. [Google Scholar] [CrossRef]
- Mousa, A.E.-K.; Aboualy, N.; Sharaf, M.; Zahra, H.; Darrag, M. Tropospheric Wet Delay Estimation Using GNSS: Case Study of a Permanent Network in Egypt. NRIAG J. Astron. Geophys. 2016, 5, 76–86. [Google Scholar] [CrossRef]
- Dach, R.; Lutz, S.; Walser, P.; Fridez, P. Bernese GNSS Software Version 5.2; Astronomical Institute, University of Bern: Bern, Switzerland, 2015; Volume 47, ISBN 1879621142. [Google Scholar]
- Ekaso, D.; Nex, F.; Kerle, N.; Nex, F.; Kerle, N. Geo-Spatial Information Science Accuracy Assessment of Real-Time Kinematics (RTK) Measurements on Unmanned Aerial Vehicles (UAV) for Direct Geo-Referencing. Geo Spat. Inf. Sci. 2020, 23, 165–181. [Google Scholar] [CrossRef]
- Oštir, K. Daljinsko Zaznavanje; Inštitut za Antropološke in Prostorske Študije ZRC SAZU: Ljubljana, Slovenia, 2006. [Google Scholar]
- Dong, P.; Chen, Q. LiDAR Remote Sensing and Applications; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Fujii, T.; Fukushi, T. Lidar: Introduction. In Laser Remote Sensing; CRC Press: Boca Raton, FL, USA, 2005; ISBN 9780824742560. [Google Scholar]
- Kovačič, B.; Supej, B. Najsodobnejša Tehnologija Lidar za Zajem Terestičnih Podatkov. Gradb. Rev. Gradnjo Sanacije Gradb. Mater. 2007, 10, 41–43. [Google Scholar]
- Gao, J. Towards Accurate Determination of Surface Height Using Modern Geoinformatic Methods: Possibilities and Limitations. Prog. Phys. Geogr. 2007, 31, 591–605. [Google Scholar] [CrossRef]
- Wehr, A.; Lohr, U. Airborne Laser Scanning—An Introduction and Overview. ISPRS J. Photogramm. Remote Sens. 1999, 54, 68–82. [Google Scholar] [CrossRef]
- Belcher, T. Leica ALS40 and ADS40 Systems Dominate LIDAR and Digital Airborne Data Acquisition. Report. Mag. Leica Geosystems 2002, 47, 14–15. [Google Scholar]
- Webster, T.L.; Forbes, D.L.; Dickie, S.; Shreenan, R. Using Topographic Lidar to Map Flood Risk from Storm-Surge Events for Charlottetown, Prince Edward Island, Canada. Can. J. Remote Sens. 2004, 30, 64–76. [Google Scholar] [CrossRef]
- Gomes Pereira, L.M.; Wicherson, R.J. Suitability of Laser Data for Deriving Geographical Information. A Case Study in the Context of Management of Fluvial Zones. ISPRS J. Photogramm. Remote Sens. 1999, 54, 105–114. [Google Scholar] [CrossRef]
- Jones, J.L. Mapping a Flood...Before It Happens. Available online: https://pubs.usgs.gov/fs/2004/3060/ (accessed on 15 November 2020).
- Means, J.E.; Acker, S.A.; Fitt, B.J.; Renslow, M.; Emerson, L.; Hendrix, C.J. Predicting Forest Stand Characteristics with Airborne Scanning Lidar. Photogramm. Eng. Remote Sens. 2000, 66, 1367–1371. [Google Scholar]
- Andersen, H.E.; McGaughey, R.J.; Reutebuch, S.E. Estimating Forest Canopy Fuel Parameters Using LIDAR Data. Remote Sens. Environ. 2005, 94, 441–449. [Google Scholar] [CrossRef]
- Hollaus, M.; Wagner, W.; Eberhöfer, C.; Karel, W. Accuracy of Large-Scale Canopy Heights Derived from LiDAR Data under Operational Constraints in a Complex Alpine Environment. ISPRS J. Photogramm. Remote Sens. 2006, 60, 323–338. [Google Scholar] [CrossRef]
- Viñas, O.; Ruiz, A.; Xandri, R.; Palà, V.; Arbiol, R. Combined Use of LiDAR and QuickBird Data for the Generation of Land Use Maps. Int. Arch. Photogramm. Remote Sens. Spat. Inf. Sci. 2006, 36. [Google Scholar]
- Ho, H.Y.; Hsiao, K.H.; Yang, M.S.; Liu, J.K. Landuse Classification Using Aerial Photographs and LiDAR Data. In Proceedings of the ACRS 2008 29th Asian Conference on Remote Sensing, Colombo, Sri Lanka, 10–14 November 2008; Volume 3, pp. 1741–1746. [Google Scholar]
- Engelkemeir, R.M.; Khan, S.D. Lidar Mapping of Faults in Houston, Texas, USA. Geosphere 2008, 4, 170–182. [Google Scholar] [CrossRef]
- Ridgway, J.R.; Minster, J.B.; Williams, N.; Bufton, J.L.; Krabill, W.B. Airborne Laser Altimeter Survey of Long Valley, California. Geophys. J. Int. 1997, 131, 267–280. [Google Scholar] [CrossRef]
- Kroh, P. Identification of Landing Sites for Rescue Helicopters in Mountains with Use of Geographic Information Systems. J. Mt. Sci. 2020, 17, 261–270. [Google Scholar] [CrossRef]
- Saari, T.; Poutanen, M.; Saaranen, V.; Kaartinen, H.; Kukko, A.; Lahtinen, S. Height Determination Techniques for the Next National Height System of Finland—A Case Study. Geod. Cartogr. 2015, 41, 145–155. [Google Scholar] [CrossRef]
- Becker, J.-M. History and Evolution of Height Determination Techniques Especially in Sweden. In Proceedings of the Geodesy and Surveying in the Future—The Importance of Heights, Gavle, Sweden, 15–17 March 1999; pp. 43–57. [Google Scholar]
- Karila, K.; Karjalainen, M.; Hyyppä, J.; Koskinen, J.; Saaranen, V.; Rouhiainen, P. A Comparison of Precise Leveling and Persistent Scatterer SAR Interferometry for Building Subsidence Rate Measurement. ISPRS Int. J. Geo Inf. 2013, 2, 797–816. [Google Scholar] [CrossRef]
- Burgmann, R.; Rosen, P.A.; Fielding, E.J. Synthetic Aperture Radar Interferometry to Measure Earth’s Surface Topography and Its Deformation. Annu. Rev. Earth Planet. Sci. 2000, 28, 169–209. [Google Scholar] [CrossRef]
- Hanssen, R.F. Radar Interferometry, Data Interpretation and Error Analysis; Springer: Dordrecht, The Netherlands, 2002. [Google Scholar]
- Yongjun, Z.; Zemin, W. Analyses and Solutions of Errors on GPS/GLONASS Positioning. Geo Spat. Inf. Sci. 2002, 5, 6–12. [Google Scholar] [CrossRef]
- Bamler, R.; Hartl, P. Synthetic Aperture Radar Interferometry. Inverse Probl. 1998, 14. [Google Scholar] [CrossRef]
- SkyGeo InSAR Technical Background 2020. Available online: https://skygeo.com/insar-technical-background/ (accessed on 15 November 2020).
- Alganci, U. Accuracy Assessment of Different Digital Surface Models. ISPRS Int. J. Geo Inf. 2018, 7, 114. [Google Scholar] [CrossRef]
- Bates, P.D.; Lane, S.N.; Ferguson, R.I. Computational Fluid Dynamics: Applications in Environmental Hydraulics; John Wiley & Sons: Chichester, UK, 2005; ISBN 0470843594. [Google Scholar]
- Piras, M.; Taddia, G.; Forno, M.G.; Gattiglio, M.; Aicardi, I.; Dabove, P.; Russo, S.L.; Lingua, A. Detailed Geological Mapping in Mountain Areas Using an Unmanned Aerial Vehicle: Application to the Rodoretto Valley, NW Italian Alps. Geomat. Nat. Hazards Risk 2017, 8, 137–149. [Google Scholar] [CrossRef]
- Forsberg, R.; Tscherning, C. An Overview Manual for the GRAVSOFT Geodetic Gravity Field Modelling Programs; DTU Space: Kongens Lyngby, Denmark, 2008; pp. 1–59. [Google Scholar]
- Sjöberg, L.E. A Solution to the Downward Continuation Effect on the Geoid Determined by Stokes’ Formula. J. Geod. 2003, 77, 94–100. [Google Scholar] [CrossRef]
- Tenzer, R.; Jának, J. Stokes-Helmert’s Scheme for Precise Geoid Determination. Rev. Cartogr. 2002, 74–75, 135. [Google Scholar]
- Ssengendo, R. A Height Datum for Uganda Based on a Gravimetric Quasigeoid Model and GNSS/Levelling. Ph.D. Thesis, KTH, School of Architecture and the Built Environment (ABE), Urban Planning and Environment, Geodesy and Satellite Positioning, Stockholm, Sweden, 2015. [Google Scholar]
- Borowski, L.; Banasik, P. The Conversion of Heights of the Benchmarks of the Detailed Vertical Reference Network into the PL-EVRF2007-NH frame. Rep. Geod. Geoinform. 2020, 109, 1–7. [Google Scholar] [CrossRef]
- Borowski, Ł.; Lal, A.; Nepelski, K. Analiza Osiadania Wybranych Typów Znaków Osnowy Geodezyjnej. Bud. Archit. 2017, 16, 135–142. [Google Scholar] [CrossRef]
- Balon, J.; Jodłowski, M. Regionalizacja Fizycznogeograficzna Karpat Zachodnich—Studium Metodologiczne. In Struktura Środowiska Przyrodniczego a Fizjonomia Krajobrazu; Instytut Geografii i Gospodarki Przestrzennej UJ: Krakow, Poland, 2014; pp. 85–106. ISBN 978-83-64089-02-2. [Google Scholar]
- Solon, J.; Borzyszkowski, J.; Bidłasik, M.; Richling, A.; Badora, K.; Balon, J.; Brzezińska-Wójcik, T.; Chabudziński, Ł.; Dobrowolski, R.; Grzegorczyk, I.; et al. Physico-Geographical Mesoregions of Poland: Verification and Adjustment of Boundaries on the Basis of Contemporary Spatial Data. Geogr. Pol. 2018, 91, 143–170. [Google Scholar] [CrossRef]
- Mazúr, E.; Činčura, J.; Kvitkovič, J. Geomorfológia. Atlas SSR, SAV, SÚGK; Bratislava, Slovakia, 1980. [Google Scholar]
- Štúra, 8. Štátny Geologický Ústav Dionýza Geologická Mapa Slovenska M 1:50 000. Available online: http://apl.geology.sk/gm50js (accessed on 15 November 2020).
- Izmaiłow, B.; Kaszowski, L.; Krzemień, K.; Święchowicz, J. Rzeźba. In Karpaty Polskie; Warszyńska, J., Ed.; Uniwersytet Jagielloński: Kraków, Poland, 1995; pp. 23–30. [Google Scholar]
- Rączkowska, Z.; Łajczak, A.; Margielewski, W.; Święchowicz, J. Recent Landform Evolution in the Polish Carpathians. In Recent Landform Evolution; Springer: Dordrecht, The Netherlands, 2012; pp. 47–101. [Google Scholar]
- Midriak, R. Morfogenéza Povrchu Vysokých Pohorí; VEDA: Bratislava, Slovakia, 1983. [Google Scholar]
- Marciniec, P.; Granoszewski, W.; Zimnal, Z. Osuwisko na Stokach Magury Witowskiej (Podhale). Przegląd Geol. 2019, 67, 405–413. [Google Scholar] [CrossRef]
- Państwowy Instytut Geologiczny—Państwowy Instytut Badawczy. Wyszukiwanie i Przeglądanie Informacji o Zagrożeniach Ruchami Masowymi. Available online: http://geoportal.pgi.gov.pl/portal/page/portal/SOPO/Wyszukaj3 (accessed on 1 December 2020).
- Obrębska-Starklowa, B. Differentiation of Topoclimatic Conditions in a Carpathian Foreland Valley Based on Multiannual Observations. Zesz. Nauk. Uniw. Jagiellońskiego. Pr. Geogr. 1995, 101, 1–120. [Google Scholar]
- Kadaj, R. Algorytm Opracowania Modelu PL-geoid-2011. In Proceedings of the Realizacja Osnów Geodezyjnych a Problemy Geodynamiki, Grybów, Poland, 25–27 September 2012; p. 26. [Google Scholar]
- Wężyk, P. Podręcznik dla Uczestników Szkoleń z Wykorzystania Produktów LiDAR. 2014. Available online: http://www.gugik.gov.pl/__data/assets/pdf_file/0019/23752/PODRECZNIK_ISOK_wyd.2.pdf (accessed on 15 November 2020).
- GUGiK Numeryczny Model Terenu. Available online: http://www.gugik.gov.pl/pzgik/zamow-dane/numeryczny-model-terenu (accessed on 14 October 2020).
- Raicich, F. A Study of Early Trieste Sea Level Data (1875–1914). J. Coast. Res. 2007, 23, 1067–1073. [Google Scholar] [CrossRef]
- Zbiorowa, P. Niwelacja Precyzyjna, 2nd ed.; Baran, W., Ed.; Polskie Przedsiębiorstwo Wydawnictw Kartograficznych: Warszawa/Wrocław, Poland, 1993; ISBN 83-7000-082-7. [Google Scholar]



| Laser Point Density | Transversal Scan Angle | Laser Point Elevation Accuracy after Alignment | Laser Point Situation Accuracy after the Alignment | Registration of Multiple Reflections | Data of Scanner Raids |
|---|---|---|---|---|---|
| 4 points/m2 | ≤ ±25° | ≤0.15 m | ≤0.50 m | Minimum 4 echoes | From mid-October to late April |
| No. | Year | Source |
|---|---|---|
| I | internet | https://pl.wikipedia.org/wiki/Wikipedia:Strona_g%C5%82%C3%B3wna |
| II | internet | https://mapa-turystyczna.pl/ |
| III | internet | http://igrek.amzp.pl/mapindex.php?cat=WIG25 |
| IV | internet | https://zbgis.skgeodesy.sk/mkzbgis/en/kataster/?bm=zbgis&z=12&c=19.504251,49.539836# |
| V | internet | https://mapy.hiking.sk/?ref=navigator |
| VI | 1884 | III. MS: The Third Military Survey (1875–1884) |
| VII | 1934 | III. MS 1920–1934_reamb.: The Third Military Survey—updated in 1920–1934 |
| VIII | 1951 | Pagaczewski, Staniwsław (1951). Babia Góra, Gorce, Beskid Wyspowy. Bydgoszcz: Spółdzielczy Instytut Wydawniczy KRAJ. |
| IX | 1957 | TM25 1952–1957: Military topographic maps 1:25,000 /1952–1957) |
| X | 1978 | Wojterski, Teofil (1978). Babia Góra. Warszawa: Wiedza Powszechna. |
| XI | 1986 | Miodowicz, Władysław (1986). Babia Góra. Przewodnik Turystyczny. Kraków: Wydawnictwo PTTK. |
| XII | 1987 | Mapa Beskid Śląski i Żywiecki (1987). 1:75,000. Warszawa-Kraków: Państwowe Przedsiębiorstwo Wydań Kartograficznych im. E. Romera. |
| XIII | 1993 | Mapa Beskid Śląski i Żywiecki (1993). 1:75,000. WYD. 13. Warszawa-Kraków: Państwowe Przedsiębiorstwo Wydań Kartograficznych im. E. Romera. |
| XIV | 90’s | RETM_50: raster equivalent of the topographic map 1:50,000 (the 90’s of the 20th century) |
| XV | 90’s | RETM_25: raster equivalent map of the topographic map 1:25,000 (the 90´s of the 20th century) |
| XVI | 1999 | Warzecha-Tober, Agnieszka; Stańczyk, Wojciech; Figiel, Stanisław (1999). Beskidy 2: Mału, Makowski i Babia Góra. Bielsko-Biała: Wydawnictwo Pascal |
| XVII | 2003 | Mapa Beskid Żywiecki (2003). 1:50,000. Wyd. 3. Kraków: Compas. |
| XVIII | 2011 | Mapa Beskid Żywiecki (2011). 1:50,000. Wyd. 11. Kraków: Compas. |
| XIX | 2013 | Beskid Śląski i Żywiecki–mapa turystyczna (skala 1:50,000), Express map 2013, Warszawa |
| XX | 2015 | Beskid Żywiecki, Wydanie: 15, Skala: 1:50,000, Compass 2015, Kraków |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maciuk, K.; Apollo, M.; Mostowska, J.; Lepeška, T.; Poklar, M.; Noszczyk, T.; Kroh, P.; Krawczyk, A.; Borowski, Ł.; Pavlovčič-Prešeren, P. Altitude on Cartographic Materials and Its Correction According to New Measurement Techniques. Remote Sens. 2021, 13, 444. https://doi.org/10.3390/rs13030444
Maciuk K, Apollo M, Mostowska J, Lepeška T, Poklar M, Noszczyk T, Kroh P, Krawczyk A, Borowski Ł, Pavlovčič-Prešeren P. Altitude on Cartographic Materials and Its Correction According to New Measurement Techniques. Remote Sensing. 2021; 13(3):444. https://doi.org/10.3390/rs13030444
Chicago/Turabian StyleMaciuk, Kamil, Michał Apollo, Joanna Mostowska, Tomáš Lepeška, Mojca Poklar, Tomasz Noszczyk, Pawel Kroh, Artur Krawczyk, Łukasz Borowski, and Polona Pavlovčič-Prešeren. 2021. "Altitude on Cartographic Materials and Its Correction According to New Measurement Techniques" Remote Sensing 13, no. 3: 444. https://doi.org/10.3390/rs13030444
APA StyleMaciuk, K., Apollo, M., Mostowska, J., Lepeška, T., Poklar, M., Noszczyk, T., Kroh, P., Krawczyk, A., Borowski, Ł., & Pavlovčič-Prešeren, P. (2021). Altitude on Cartographic Materials and Its Correction According to New Measurement Techniques. Remote Sensing, 13(3), 444. https://doi.org/10.3390/rs13030444











