1. Introduction
Our modern society increasingly relies on continuous and reliable GNSS positioning, navigation, and timing, from surveying to safety-critical applications such as autonomous navigation [
1,
2,
3]. However, irregularities in the ionospheric electron density distribution can induce disturbances in the GNSS signals which propagate. These disturbances take the form of temporal fluctuations in the intensity and phase of received GNSS radio signals and cause higher-order errors that cannot be eliminated through the dual-frequency combination of observables [
4] or through standard global/regional models. These higher-order residual errors on dual-frequency combinations introduced by ionospheric irregularities along GNSS ray paths lead to an increase in the magnitude and variability of the positioning error [
4].
The size of ionospheric irregularities varies between larger and smaller spatial scales due to the action of plasma instability mechanisms in the equatorial, mid-latitude, and high-latitude ionosphere [
5]. In the case of GNSS signals, propagation through large-scale ionospheric irregularities induces phase fluctuations over longer temporal intervals, whereas the propagation through small-scale irregularities leads to scintillation (i.e., fluctuations over shorter temporal intervals) in the intensity and phase of the received GNSS signals as a consequence of a scattering process. Phase fluctuations originating from the propagation through larger-scale ionospheric irregularities are typically quantifiable through the rate of change of the slant Total Electron Content (TEC) [
6] (i.e., temporal fluctuations in Slant TEC). On the other hand, intensity and phase scintillation arising from propagation through small-scale ionospheric irregularities is quantified by means of the scintillation indices
and
, respectively [
7,
8,
9,
10].
In the equatorial ionosphere, GNSS signals experience both TEC fluctuations and scintillation as they propagate through large-to-small scale field-aligned irregularities that form in conjunction with plasma bubbles and plumes of ionisation. The occurrence of large-to-small scale field-aligned irregularities in the equatorial ionosphere maximises the post-sunset sector and increases with solar activity [
7,
11,
12,
13,
14].
In the auroral and polar ionospheres, phase fluctuations originate from large-scale field-aligned irregularities forming during particle precipitation, whilst in the polar ionosphere phase fluctuations also arise from polar patches drifting across GNSS ray paths [
9,
10,
15,
16,
17,
18,
19,
20]. The occurrence of large-scale field-aligned irregularities in the auroral and polar ionospheres increases during disturbed magnetic conditions, for example, in the presence of magnetic storms and substorms [
10,
21,
22,
23,
24]. In the presence of enhancements in temporal TEC fluctuations and scintillation, higher-order error terms in the observables induce an increase in positioning errors and in the occurrence of outages [
4,
8,
23].
In the case of the positioning required for high-accuracy applications, errors in the observables need to be modelled to higher orders [
1,
4,
25]: the positioning algorithms (e.g., precise point positioning) are equipped with various models for the correction of these errors, which introduces high levels of complexity. In general, the complexity of the positioning algorithms increases as the models attempt to correct for higher-order error terms in order to achieve higher accuracy in the positioning solution [
1,
4]. In the case of errors induced by ionospheric irregularities through enhancements in TEC fluctuations and scintillation, several approaches are possible for the improvement of the positioning solution through the modelling of higher-order error terms. However, these models typically require external information (e.g., knowledge of scintillation indices, network PPP corrections), operate in post-processing (e.g., refinement of the precise point positioning), and assume a linear behaviour in the receiver (e.g., by modelling stand-alone variances) [
2,
22,
23,
24,
26,
27]. The net result is that the positioning algorithms depend upon a large number of inputs, their complexity increases significantly, and their effects are limited by the validity of the assumptions utilised.
Here, the origin of the increase in positioning errors and in the occurrence of outages in the presence of field-aligned irregularities in the auroral and polar ionosphere was investigated by taking advantage of an EISCAT UHF (European Incoherent SCATter Ultra High Frequency radar) and ESR (EISCAT Svalbard Radar) experiment that sampled the volume where GPS ray paths propagated. The experiment was conducted during quiet-to-moderate magnetic conditions in March 2018 and two different positioning solutions were considered: (a) a single-point single-epoch positioning with dual-frequency L1 and L2 carrier phases and pseudoranges, and with broadcast ephemeris (labelled as Broadcast Kinematic, BK) and (b) a single-point single-epoch with dual-frequency L1 and L2 carrier phases and pseudoranges, and with precise ephemeris (labelled as Precise Static, PS). The former is typical of real-time applications, whilst the latter is equivalent to post-processing Precise Point Positioning. Only the GPS constellation was considered in the positioning solutions. In order to understand the type of errors induced by irregularities in the auroral and polar ionospheres, EISCAT UHF/ESR electron density profiles were compared with the 3-D positioning error estimated both at 30 s and 1 s rates. This study addressed the following questions: (1) what are the irregularities in the auroral and polar ionospheres that determine higher variability and gaps in the positioning solution? (2) whether a shorter sampling interval can mitigate against higher variability and outages in the positioning solutions.
2. Materials and Methods
An experiment with EISCAT UHF/ESR incoherent scatter radars was conducted during March 2018 and it was aimed at characterising ionospheric irregularities occurring in the auroral and polar ionospheres. EISCAT UHF/ESR beams sampled the ionospheric volume where GPS ray paths propagated: the radars’ beams were directed along lines typically transverse to GPS ray paths. The EISCAT UHF/ESR beams aimed at different shell heights (i.e., 120, 250, and 350 km) alternately, by taking into account GPS ray paths propagating to a given GPS ground station. In the case of EISCAT, GPS ray paths to the International GNSS Service (IGS) station in Kiruna were considered; in the case of ESR, GPS ray paths to the IGS station in Ny-Ålesund were considered (more details are illustrated in a companion paper, indicated here as [
20]). The alternance of the beam directions over time together with a 60 s integration time [
15,
16] necessary to calculate electron density profiles imply that EISCAT UHF/ESR beams were sampling the ionospheric volume traversed by GPS ray paths sparsely in space and time. The sparsity of the electron density profiles implies a limitation in the resolution of the spatial and temporal variability of the irregularities detected: this limitation is expected to be overcome with EISCAT_3D.
The increase in positioning errors and in the occurrence of outages were interpreted in view of the electron density profiles measured through EISCAT UHF/ESR as well as of the phase fluctuations occurring on GPS signals.
The phase fluctuations occurring in GPS signals propagating through the volume sampled by EISCAT UHF/ESR beams were quantified by means of temporal fluctuations in the uncalibrated Slant TEC [
6]. The uncalibrated slant TEC was estimated for each Pseudorandom Noise (PRN) of relevance by utilising RINEX files containing observables at 30 s and at 1 s sampling intervals. RINEX Navigation files containing standard satellite information and RINEX observations files containing 1 s (after downloading, 15-min individual files were concatenated to ensure continuity in the estimates) and 30 s (single 24-h file) observables were obtained by using the software RTKLIB (RTKGET,
http://www.rtklib.com/) which downloaded the files from the Crustal Dynamics Data Information System (CDDIS,
https://cddis.nasa.gov/) repository.
From RINEX observation files, the uncalibrated slant TEC was estimated from 1 s as well as 30 s observables as:
where
is time,
and
are carrier phases in cycles corresponding to frequencies
and
in
(
,
, and fundamental frequency
),
and
are the wavelengths in m. TEC is given in
(
) [
28,
29,
30]. Equation (1) states that the geometry-free combination for carrier phases is proportional to the uncalibrated TEC due to the presence of additional biases and errors which are typically assumed to be constant or to vary slowly with time. For the purposes of this study, the temporal variation in the uncalibrated TEC was utilised to quantify fluctuations on the carrier phases in the presence of irregularities given its proportionality to the geometry-free combination which has relevance for the positioning solutions. Therefore, temporal TEC fluctuations (or rate of change) (
) for each PRN and at each epoch were estimated as:
where
is the uncalibrated slant TEC at epoch
k,
is TEC at epoch
, and
is the change in time [
15,
31,
32]. Here,
can be 1 s or 30 s. TEC fluctuations
were calculated for different ionospheric conditions in conjunction with EISCAT UHF/ESR measurements. In view of Equations (1) and (2), an enhancement in temporal TEC fluctuations indicates the presence of residual errors in the carrier phases that are not fully removed through dual-frequency combinations, thus typically leading to an increase in positioning errors [
4].
The BK and PS positioning solutions were calculated at 1 s and at 30 s rates by means of the software gLAB, available on-line at
https://gage.upc.es/gLAB/ [
33,
34]. In each of the days during EISCAT UHF/ESR measurements BK and PS solutions were calculated for the IGS ground stations in Kiruna (KIRU, in relation to EISCAT electron density profiles) and Ny-Ålesund (NYA1, in relation to ESR electron density profiles). The software gLAB utilised GPS carrier phases (L1 and L2) and pseudoranges (P1 and P2) for the calculation of the BK and PS positioning estimates [
34].
The Antenna Exchange Format (ANTEX) files, which provide information on the antenna phase centre of the GNSS satellites and of the ground stations, were obtained from standard repositories through gLAB (
tp://ftp.igs.org/pub/station/general/pcv_archive/).
In the case of the BK solution, the orbit and clock data were obtained from the RINEX Navigation files, whereas in the case of the PS solution the orbit and clock data were obtained from Standard Product (SP3) files and Clock (CLK) files (
ftp://cddis.gsfc.nasa.gov/pub/gps/products) [
35].
The BK solution was considered because of its relevance to real-time applications (e.g., autonomous navigation, civil aviation, precision agriculture), whereas the PS solution was considered because of its relevance to post-processing applications (e.g., geodesy, surveying) [
36,
37,
38].
The performance of the positioning solutions during the EISCAT UHF/ESR experiment was investigated on the basis of the instantaneous 3-D positioning error
. The instantaneous 3-D positioning error
was calculated as [
39,
40,
41]:
where
,
, and
are the ground stations coordinates in the Earth-centred Earth-fixed (ECEF) reference frame at each epoch
and
,
, and
are the receiver a-priori ECEF coordinates contained in the RINEX observation files [
39,
40,
41,
42].
, , and and, consequently, the 3-D positioning error were calculated at 1 s and at 30 s sampling intervals for both the BK and the PS solutions. was then compared with TEC fluctuations at 1 s and 30 s intervals respectively, in correspondence to the electron density structures detected through EISCAT UHF/ESR.
The temporal fluctuations in TEC,
, were considered for all PRNs with elevation angle above 5° for consistency with the positioning solutions which were based on the same elevation mask angle for minimisation of Dilution of Precision (DOP) [
34].
The software gLAB utilised both the geometry-free combination and the Melbourne-Wübbena combination [
43,
44,
45] to detect cycle slips and data gaps [
33,
34,
46,
47]. If enhancements in temporal TEC fluctuations corresponded to an increase in cycle slips and data gaps, then higher-order residual errors were to become dominant in the carrier phases and, hence, on their combination.
3. Results
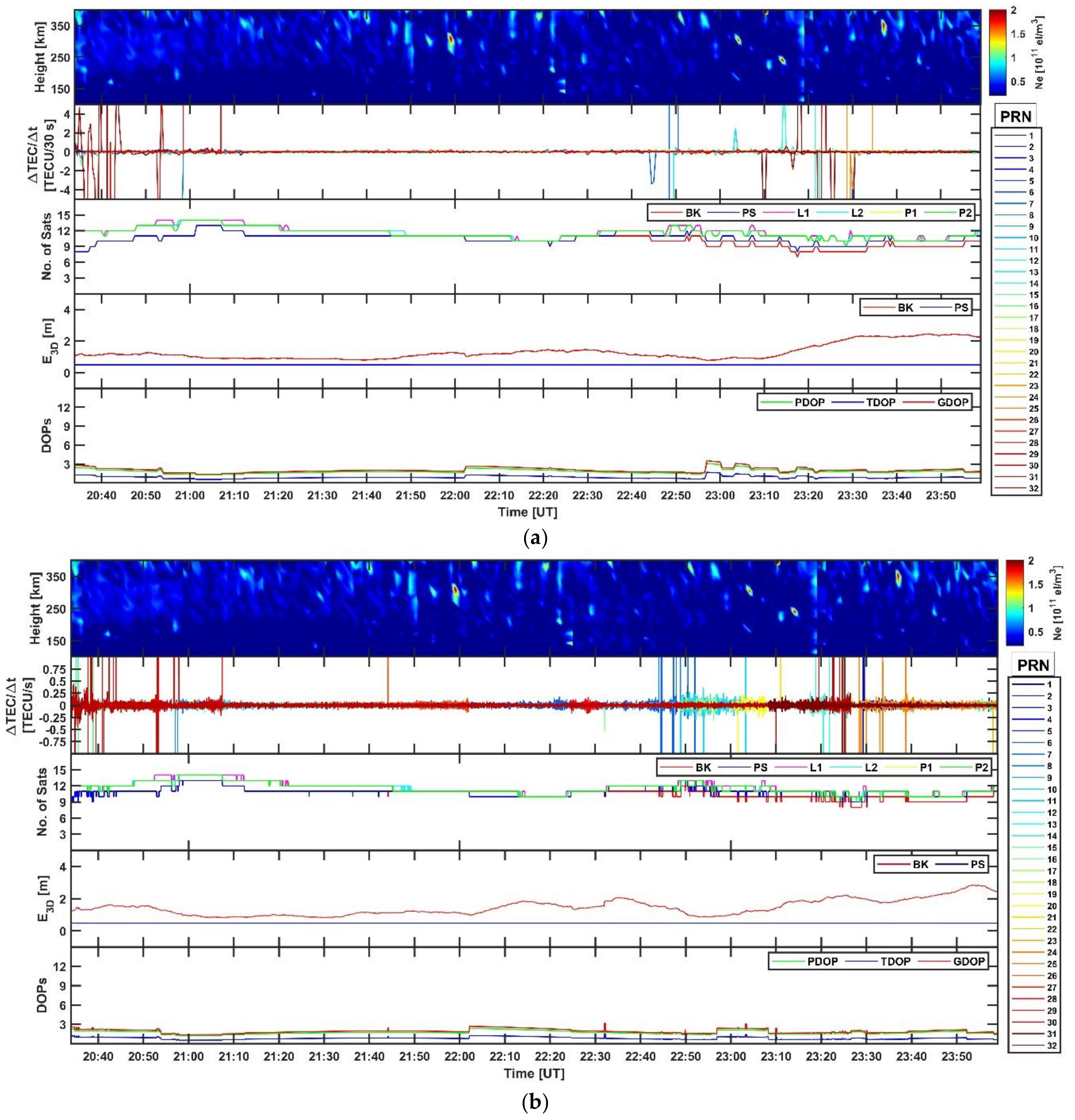
Figure 1 shows the positioning results in conjunction with the EISCAT measurements carried out on 12 March 2018.
Figure 1a shows the results in the case of 30 s observables (i.e.,
calculated every 30 s), whilst
Figure 1b shows the results in the case of 1 s observables (i.e.,
calculated every 1 s).
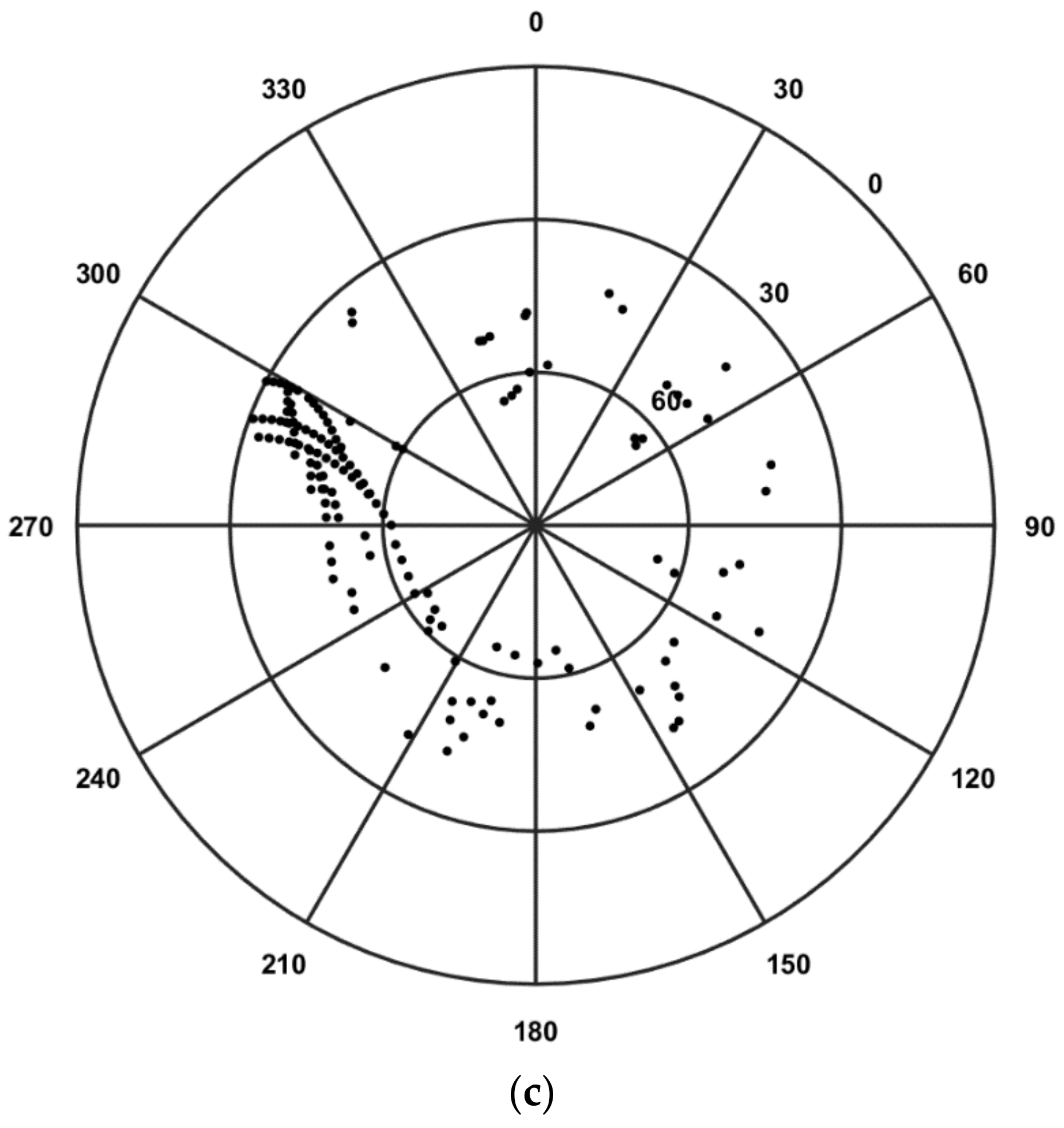
Figure 1c shows the directions of EISCAT beams in terms of azimuth and elevation angles from the EISCAT UHF antenna’s position in Tromsø.
Figure 1a,b show (from top to bottom) electron density profiles, TEC fluctuations, number of available observables from all PRNs in view together with the number of PRNs considered within the positioning solutions, the 3-D positioning error and the dilutions of precision. In
Figure 1a,b the electron density profiles are repeated in the top panels to facilitate the comparison.
The magnetic conditions on 12 March 2018 were very quiet with
(
https://www.swpc.noaa.gov/products/planetary-k-index) and with no significant structures detected by EISCAT in the auroral ionosphere. Almost all the available PRNs were considered in the positioning solutions with only a very few of them showing larger residual errors, leading to low
values and low
variability in both positioning solutions. In this case, enhancements in TEC fluctuations occurred in a few isolated instances and did not produce any impact on the positioning solutions:
did not show any significant increase and there were no gaps in the solutions. Furthermore, no significant difference between the 30 s and the 1 s positioning solutions could be noticed. The measurements on 12 March 2018,
(
Figure 1) can be considered as a quiet reference case study which more active case studies (15 and 16 March 2018,
) can be compared with.
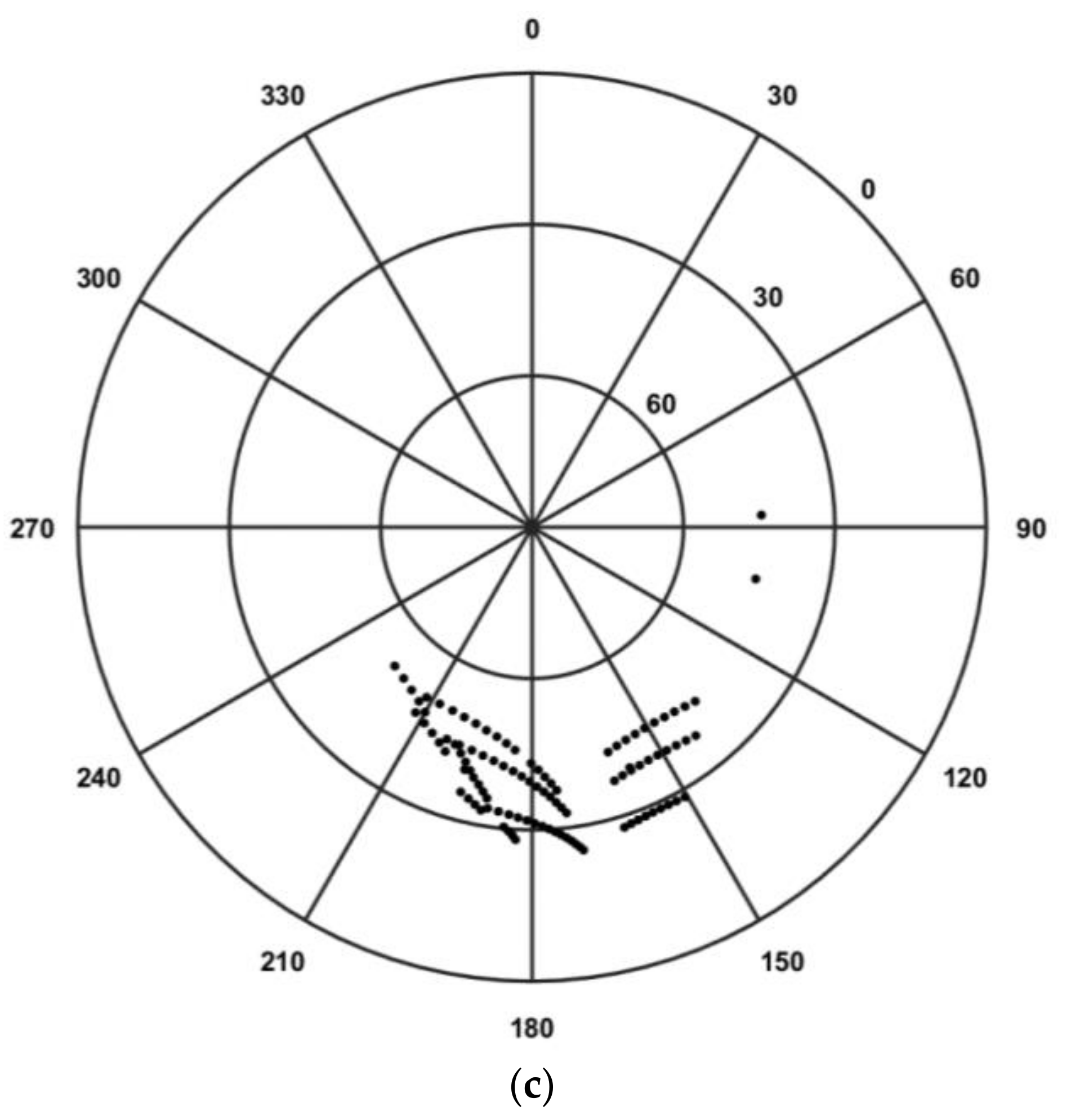
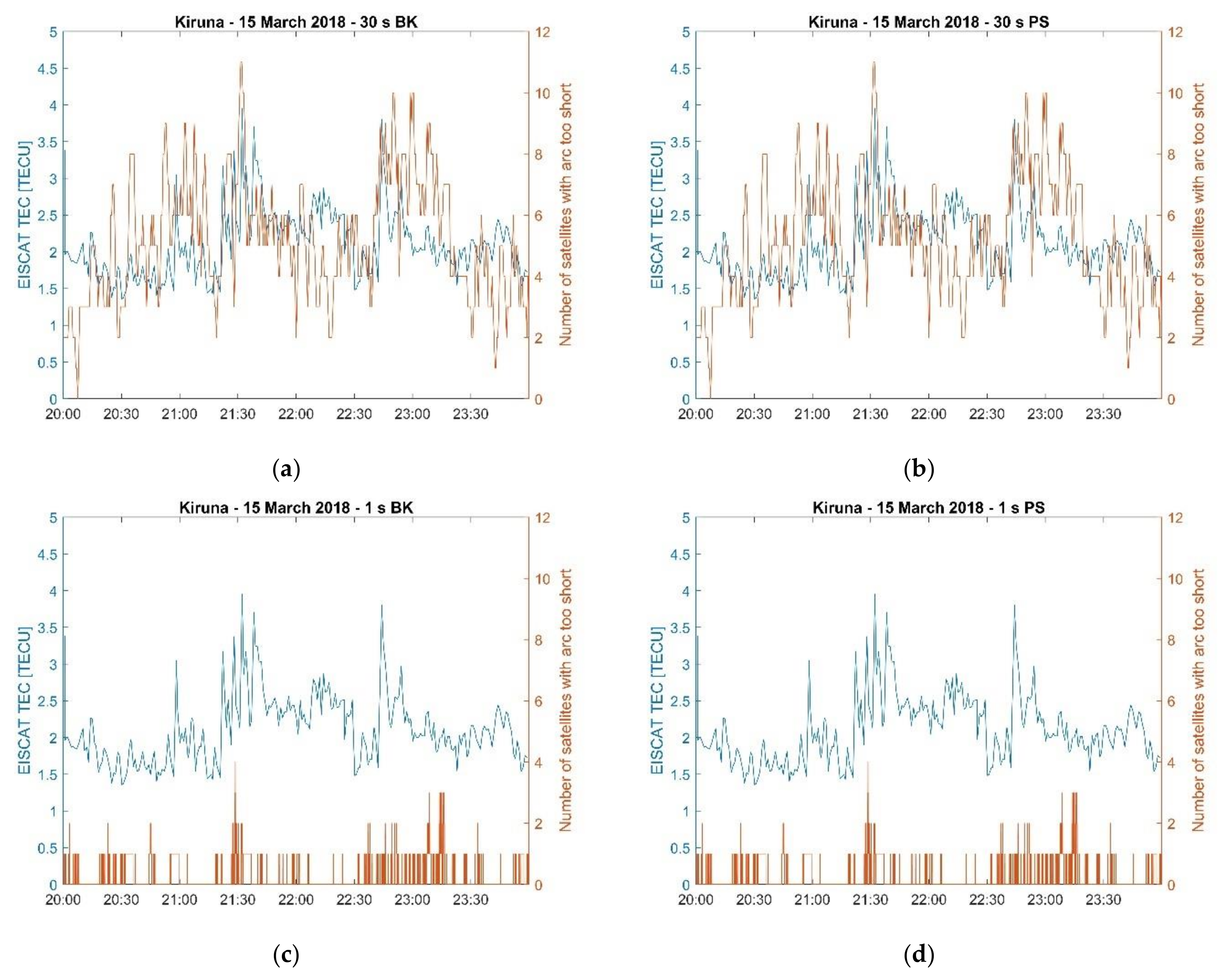
Figure 2 shows same quantities as in
Figure 1 but for EISCAT measurements collected during 15 March 2018. In this case, more active magnetic conditions (
) favoured inhomogeneous and intermittent particle precipitation in the auroral oval which originated ionisation irregularities and, consequently, enhancements in temporal TEC fluctuations visible over 30 s and 1 s intervals (more details are provided in [
20]). In the case of the 30 s positioning solutions, fewer PRNs were utilised for the positioning solutions in correspondence of enhancements in TEC fluctuations. This fact resulted in increases of
and of its variability accompanied by gaps in the 30 s BK solution. In the case of the 30 s PS solution,
maintained lower values with low variability but showed an increase in the presence of gaps similarly to the 30 s BK solution. Gaps in both the 30 s PS and BK solutions occurred when the number of PRNs usable in the positioning solutions became less than 4 (
https://gage.upc.edu/sites/default/files/gLAB/gLAB_SUM.pdf). On the contrary,
showed low variability in the case of the 1 s BK solution and no gaps occurred on both BK and PS solutions at 1 s despite enhancements in 1 s TEC fluctuations occurred in correspondence of irregularities detected through EISCAT.
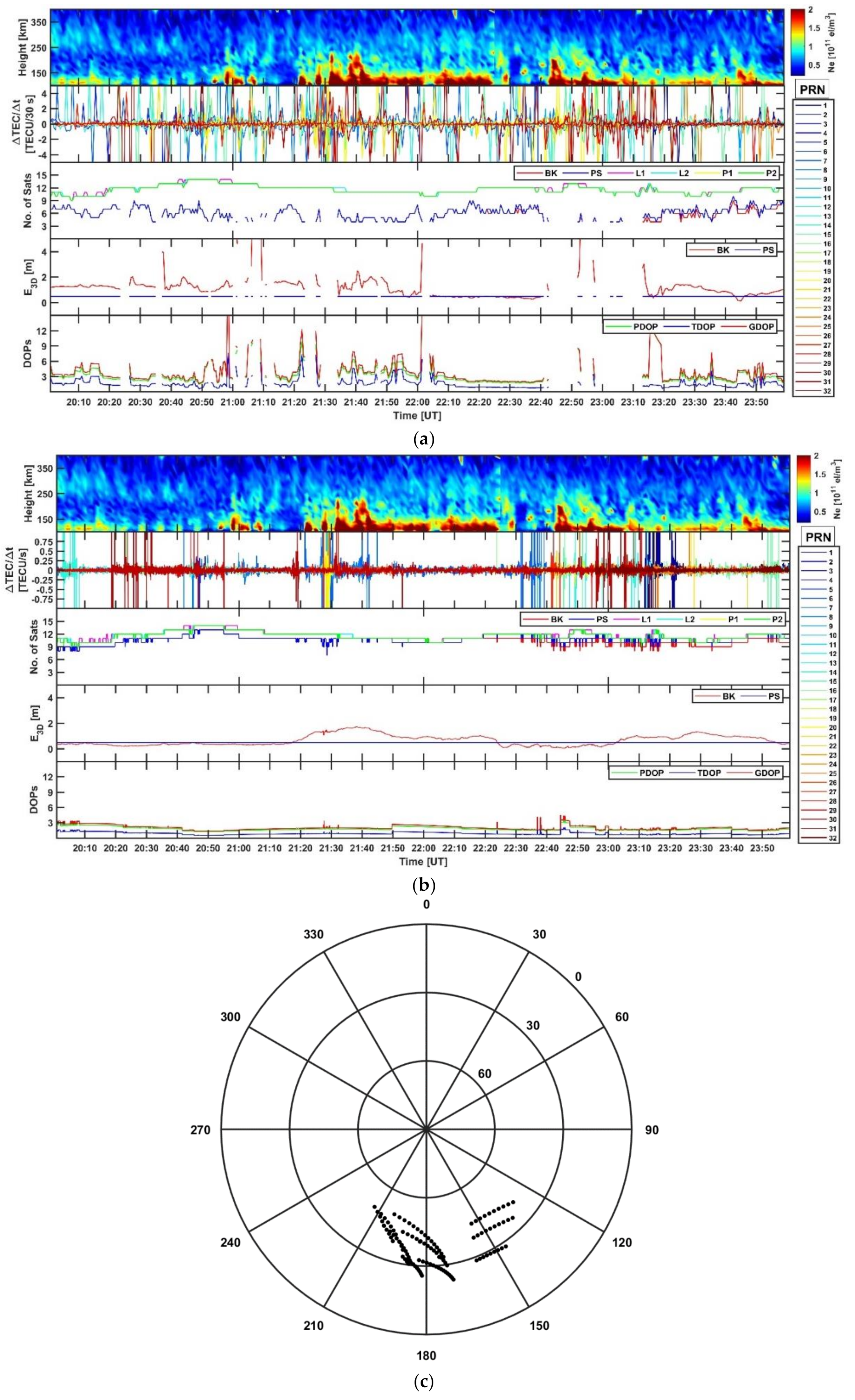
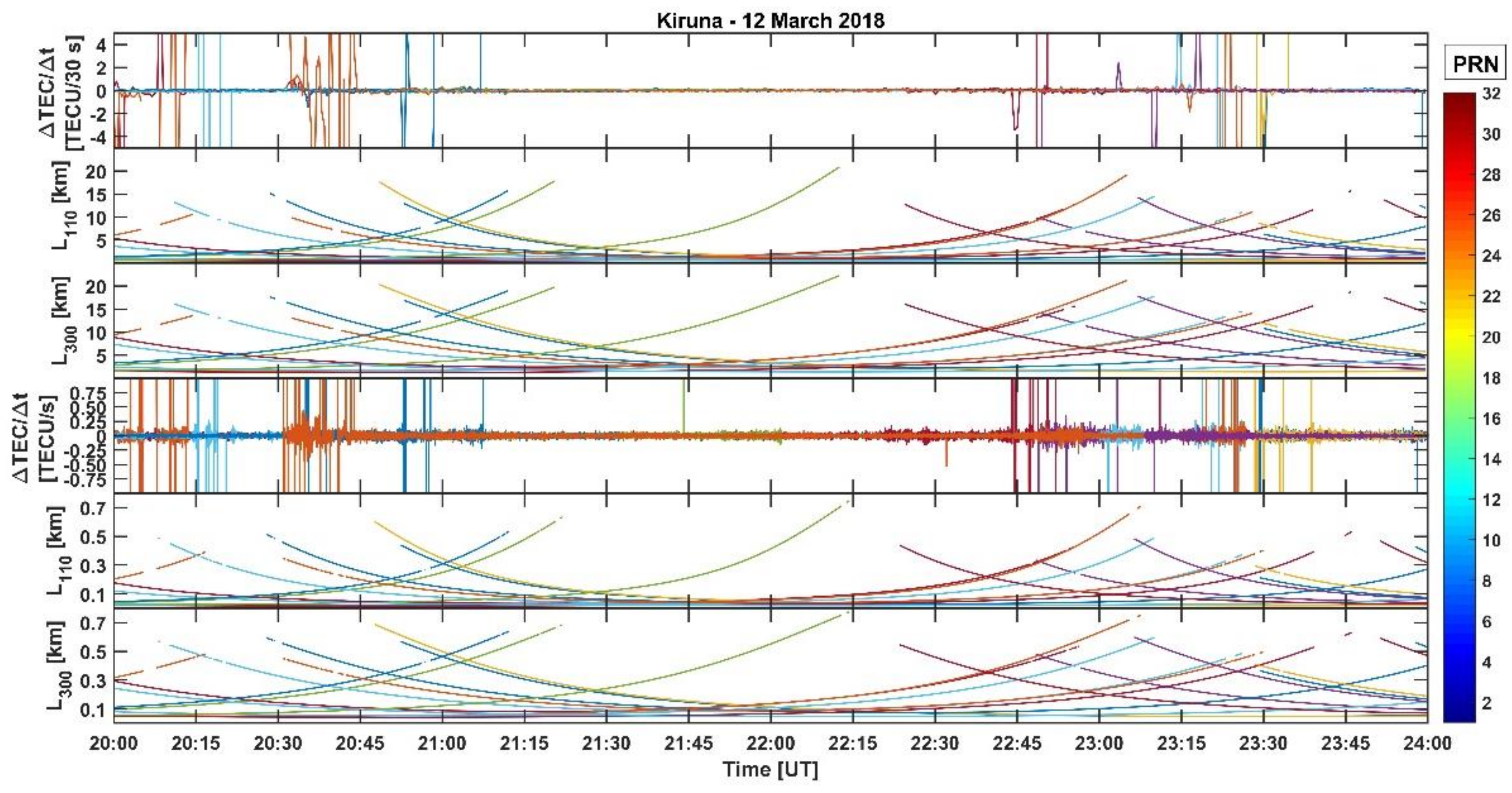
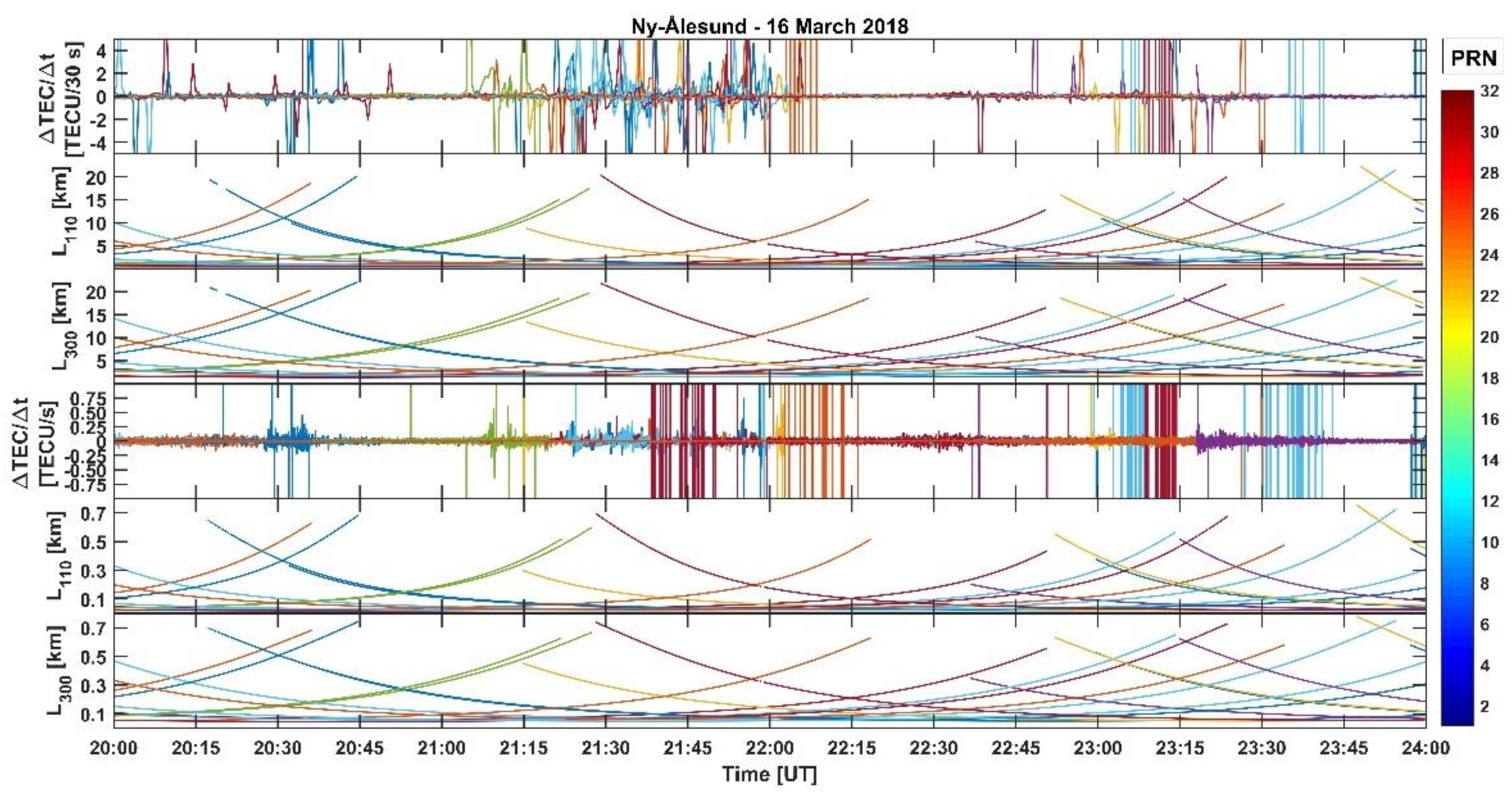
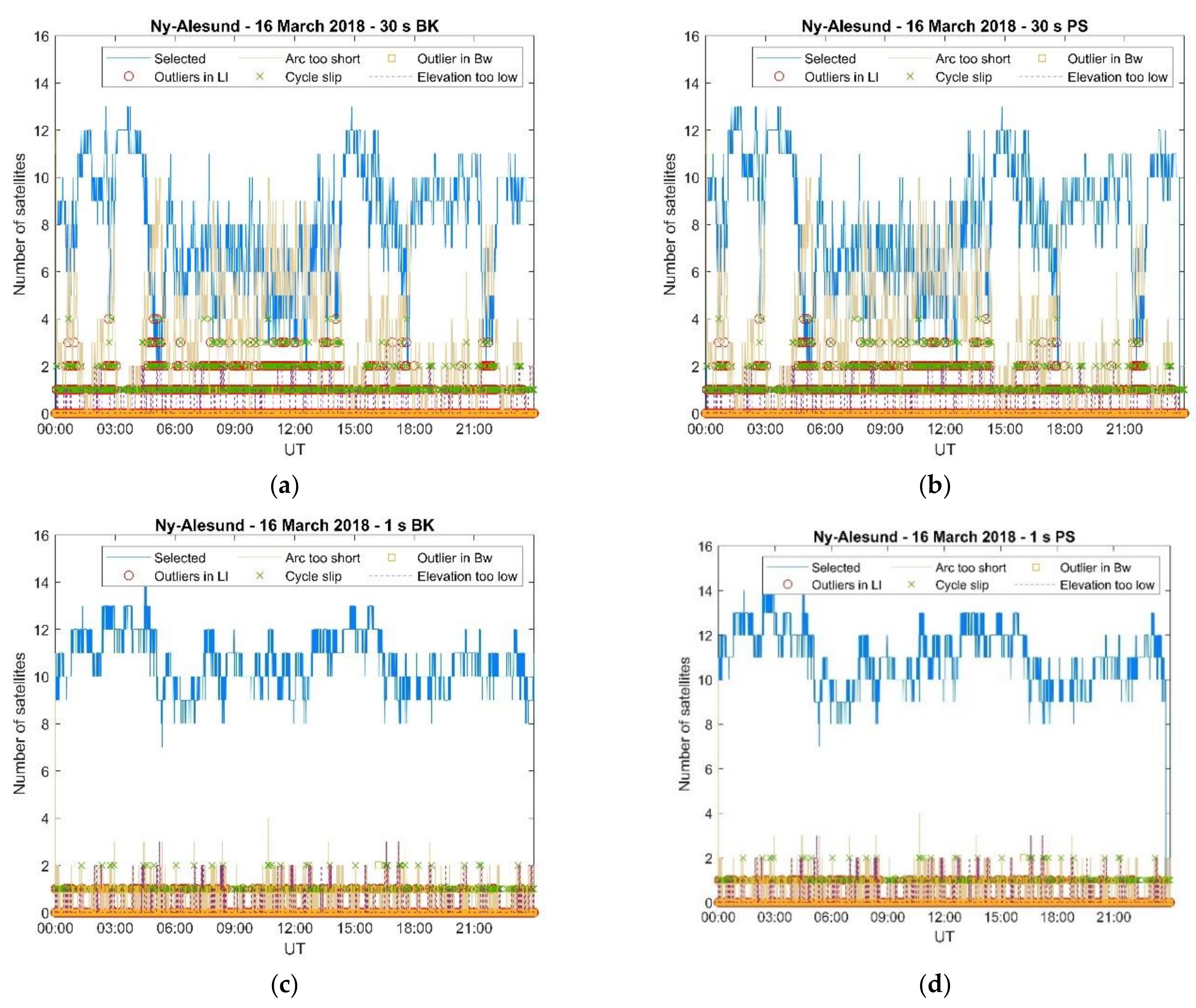
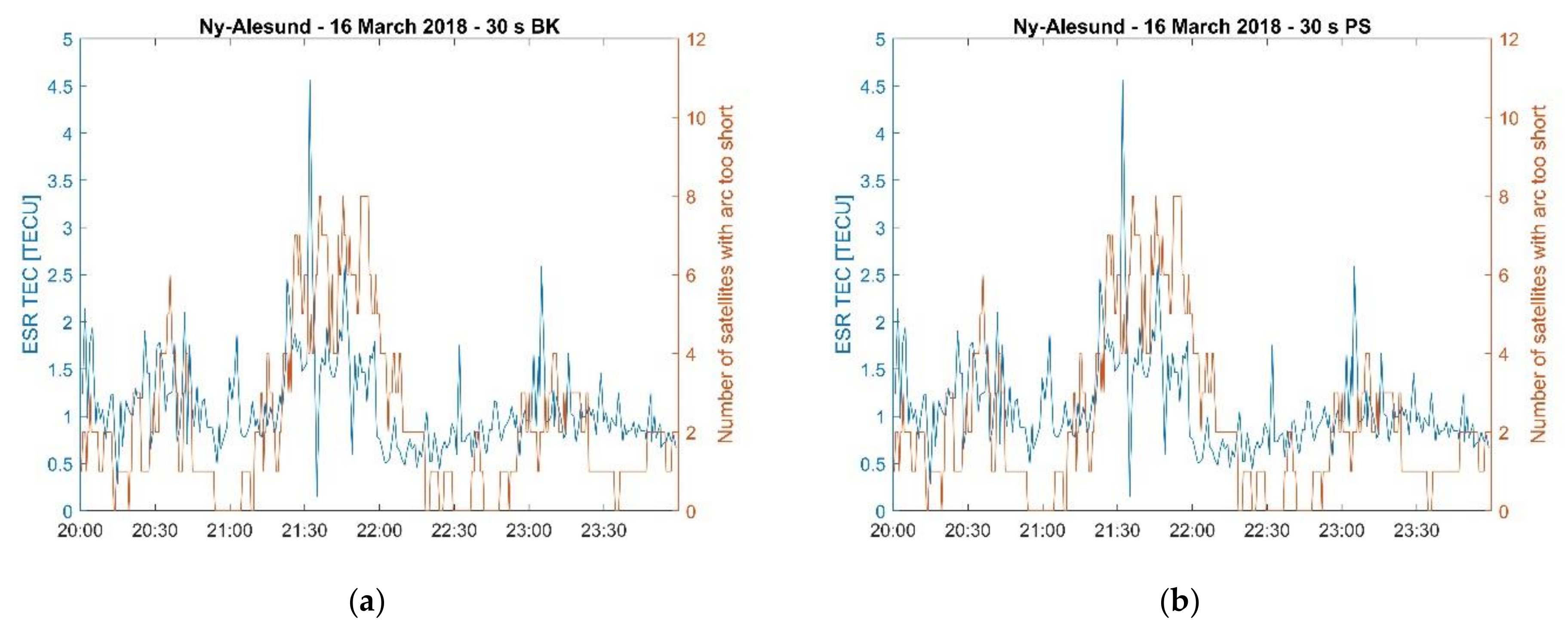
Figure 3 shows same quantities as in
Figure 1 and
Figure 2 but for ESR measurements collected on 16 March 2018 (
). ESR beams executed some north-south scans between 20:30–21:00 UT and 23:00–24:00 UT because of mechanical constraints in the radar’s antenna which led to the points in the azimuth/elevation plot being scattered over larger areas [
20]. Enhancements in TEC fluctuations occurred between 21:20 and 21:40 UT in correspondence of a fast-moving plasma patch and between 21:00–21:10, 21:40–22:00 UT, and 23:00–23:20 UT in correspondence of particle precipitation [
20], during which the 30 s BK
showed larger values with larger variability together with gaps whereas only gaps were noticeable in the 30 s PS
.
The 1 s BK showed lower variability than the 30 s BK and no gaps were noticeable in both BK and PS positioning solutions calculated at 1 s intervals.
4. Discussion
In the presence of enhancements in temporal TEC fluctuations originating from irregularities forming between the E and F regions in the auroral and polar ionospheres, the positioning solutions calculated at 30 s and at 1 s intervals showed different behaviours. In general, the 1 s positioning solutions tended to be characterised by a lower 3-D positioning error with lower variability and by the absence of gaps. On the other hand, the 30 s BK positioning solution typically showed higher variability in and gaps, whereas the 30 s PS solution showed lower values and lower variability for although with the presence of gaps.
Therefore, the two questions that arise here are: (1) what type of irregularities determines higher variability and gaps in the positioning solutions; (2) whether a higher sampling rate for the observables can produce any mitigation against higher variability and outages in the positioning solutions.
In order to investigate the first question, the link between electron density irregularities detected through EISCAT UHF/ESR, temporal TEC fluctuations, and positioning solutions was explored in detail. In this analysis, gLAB utilised by default both carrier phases (L1 and L2) and pseudoranges (P1 and P2) to compute BK and PS positioning solutions. For both PS and BK solutions, gLAB used both Melbourne-Wübbena (geometry-free and ionosphere-free) and geometry-free (dependent upon the ionosphere) combinations to detect cycle slips (
https://gage.upc.edu/sites/default/files/gLAB/gLAB_SUM.pdf).
From the results, it emerges that whenever 30 s TEC fluctuations enhanced simultaneously on various PRNs, the dilutions of precision (DOPs) and the 3-D positioning error increased their values and variabilities in the case of the 30 s BK solution; gaps occurred on both the 30 s BK and PS solutions due to the decrease in the number of available satellites (dropping below 4) with carrier phases and pseudoranges suitable for the positioning algorithm. On the contrary, enhancements in 1 s TEC fluctuations occurred on fewer PRNs and with lower magnitude over shorter time intervals in comparison with 30 s observables; the values and variabilities of DOPs and of were lower, and no gaps occurred on the 1 s BK and PS solutions. Therefore, the identification of those irregularities in the auroral and polar ionosphere introducing these effects on positioning can be researched through the evaluation of enhancements in TEC fluctuations in correspondence of structures detected through EISCAT UHF/ESR.
GPS ray paths were propagating through irregularities forming as a consequence of particle precipitation in the auroral ionosphere between 21:20–22:30 UT and 22:40–24:00 UT on 15 March 2018 (
Figure 2) and in the polar ionosphere between 21:00–21:10, 21:40–22:00 UT, and 23:00–23:20 UT on 16 March 2018 (
Figure 3); irregularities also formed in conjunction with a fast-moving patch in the polar ionosphere between 21:20–21:40 UT on 16 March 2018 (
Figure 3) [
20]. In general, enhancements in 1 s TEC fluctuations showed lower values over shorter time intervals in comparison with 30 s TEC fluctuations. EISCAT UHF/ESR electron density profiles indicate that enhancements in TEC fluctuations occurred in correspondence of irregularities forming between the E and F regions following particle precipitation in the auroral and polar ionospheres (
Figure 2 and
Figure 3) as well as a fast-moving patch in the polar ionosphere (
Figure 3) [
20].
It is plausible to assume that the irregularities traversed by GPS ray paths must have had a larger scale size such that the changes in TEC over 1 s intervals were smaller as compared to larger changes over 30 s intervals. This is supported by the fact that the resolution in range of EISCAT UHF/ESR changes between few kilometres in the E region and tens of kilometres in the F region [
15,
16,
20].
The spatial scales over which the TEC changes took place are illustrated in
Figure 4,
Figure 5 and
Figure 6: distances between consecutive pierce points every 30 s (based on 30 s navigation files) and every 1 s (based on interpolated 1 s navigation files) for GPS ray paths above 5° elevation angle were calculated at 110 km (E region) and 300 km (F region) shell heights. TEC fluctuations from
Figure 1,
Figure 2 and
Figure 3 are repeated in
Figure 4,
Figure 5 and
Figure 6 to facilitate the comparison.
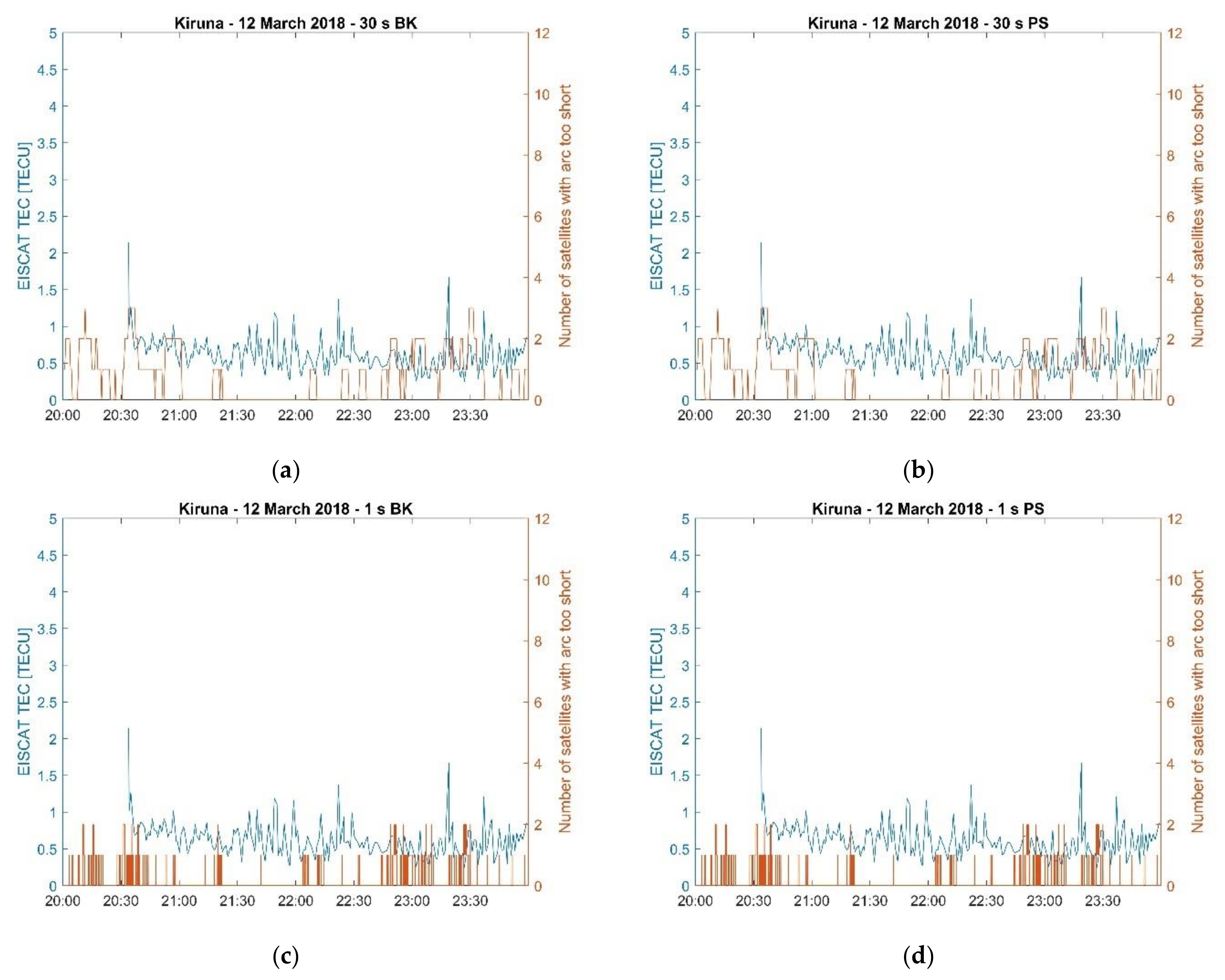
In the case of a quiet (
) and benign ionosphere (12 March 2018) only few PRNs experienced isolated enhancements in TEC fluctuations at lower elevation angles (
Figure 4). During more active (
) auroral conditions (15 March 2018,
Figure 5), more PRNs experienced enhancements in TEC fluctuations over 30 s than over 1 s at all elevation angles. Similarly, in the polar ionosphere enhancements in TEC fluctuations were experienced by more PRNs over 30 s than over 1 s at various elevation angles due to particle precipitation as well as to the transit of a polar patch (16 March 2018,
Figure 6). On average, 30 s TEC changes took place over distances approximately between 1–20 km and between the E and F regions, respectively (
Figure 5 and
Figure 6). The TEC changes over 1 s intervals occurred over distances smaller than approximately 1 km between the E and F regions. Therefore, the observations at 1 s rate originated from areas with smaller differences in TEC; on the other hand, observations at 30 s originated from areas with larger differences in TEC. That is, if the irregularities had scale sizes larger than 1 km it is likely to expect smaller changes in TEC over 1 s intervals and larger changes over 30 s intervals. As indicated by the EISCAT UHF/ESR electron density profiles (
Figure 2 and
Figure 3), the sharpest changes in TEC over 30 s intervals corresponding to gaps in the positioning solutions were induced by irregularities forming between the E and F regions in both the auroral and polar ionospheres.
Hence, it can be deduced that irregularities introducing outages in the 30 s positioning solutions: (a) had an individual scale size ranging from approximately few kilometres in the E region to few tens of kilometres in the F region, (b) were forming between the E and the F regions, (c) were occurring over spatial distances between 400 km in the E region and 800 km in the F region (these facts are demonstrated in [
20]), (d) their intersections with GPS ray paths had separation distances of few kilometres in the E region and few tens of kilometres in the F region.
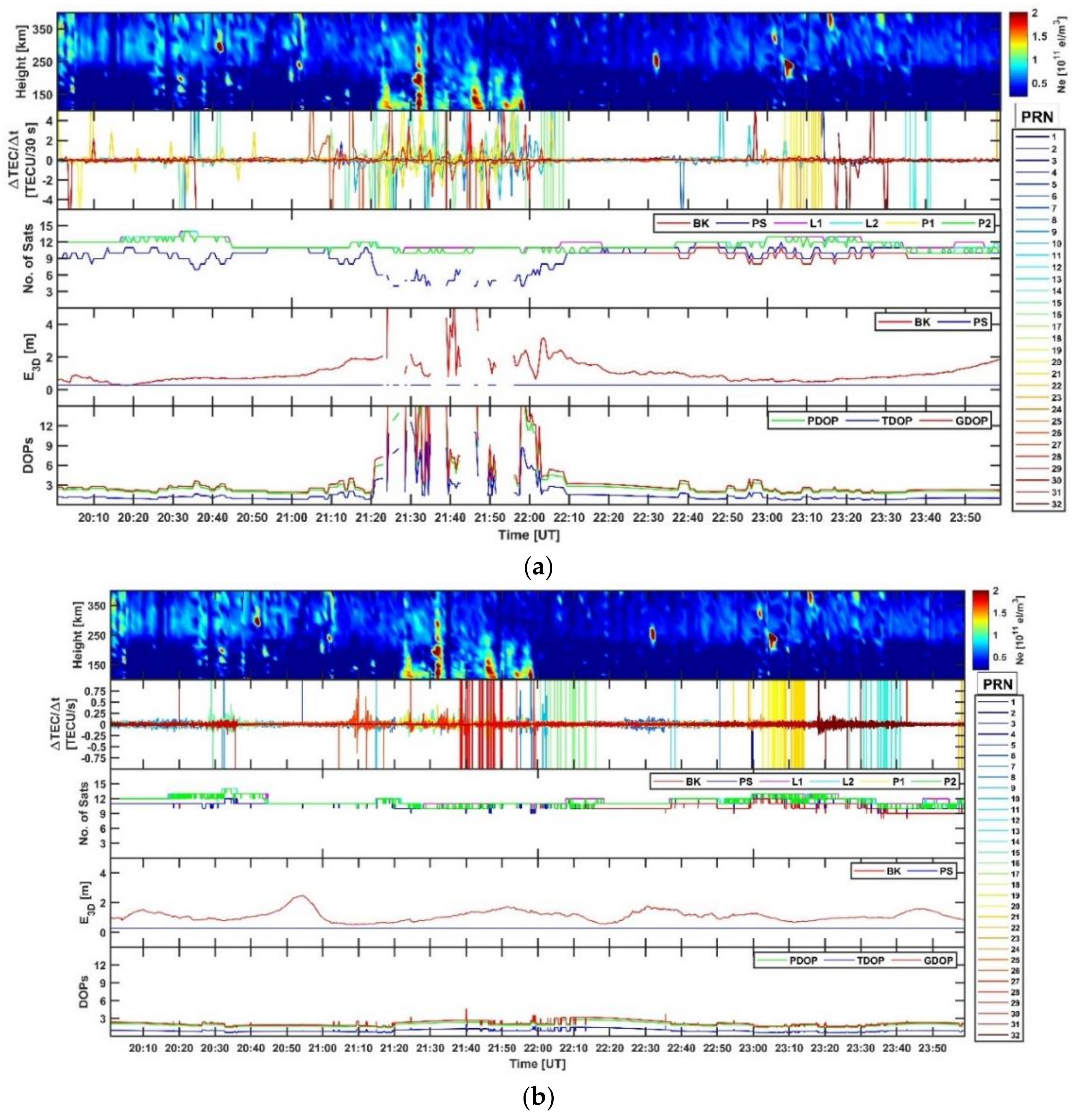
In order to investigate the second question about any advantage offered by a higher sampling rate, the origin of gaps in the positioning solutions was investigated through
Figure 7,
Figure 8,
Figure 9,
Figure 10,
Figure 11,
Figure 12 and
Figure 13.
Figure 7,
Figure 8,
Figure 9 and
Figure 10 show the number of satellites in view together with the number of satellites excluded from a given positioning solution (both at 30 s and at 1 s intervals) due to specific reasons (different line colours and styles in
Figure 7,
Figure 8 and
Figure 9). The specific reasons for the exclusion of a PRN from the positioning solution were obtained from the PRINT SATSEL message output from gLAB (
https://gage.upc.edu/sites/default/files/gLAB/gLAB_SUM.pdf). Possible specific reasons for the exclusion of a PRN include: the arc being too short, the occurrence of a cycle slip, an outlier in the geometry-free (
) combination, an outlier in the Melbourne-Wübbena (
) combination, elevation angles lower than 5°. These specific reasons appeared to be the most recurrent in the case studies analysed here although other reasons are in principle recognised by gLAB (
https://gage.upc.edu/sites/default/files/gLAB/gLAB_SUM.pdf). In
Figure 7,
Figure 8 and
Figure 9,
Figure 11,
Figure 12 and
Figure 13 the number of satellites excluded is essentially the same in the BK and PS solutions because the two solutions utilised the same cycle-slip detectors (
https://gage.upc.edu/sites/default/files/gLAB/gLAB_SUM.pdf) [
34].
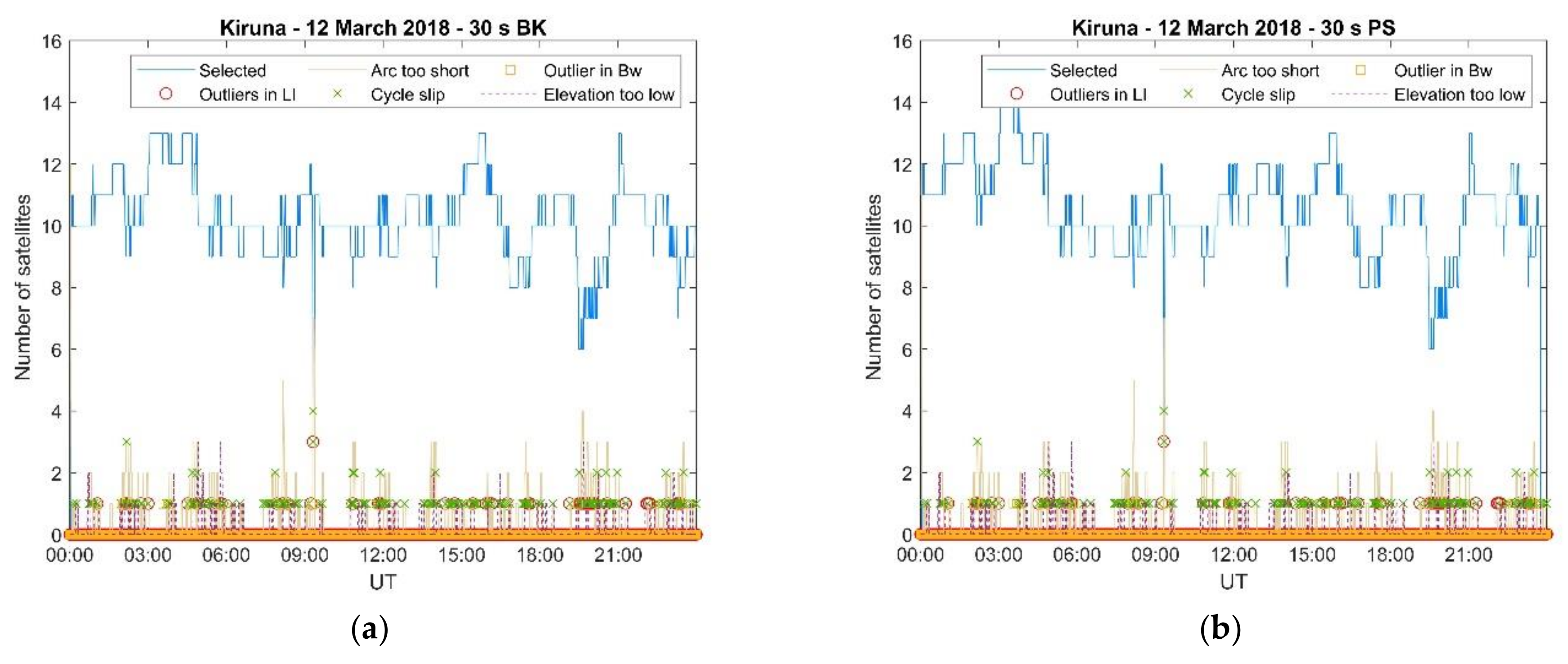
Figure 7,
Figure 8 and
Figure 9 illustrates how the number of satellites utilised in the positioning solutions varied between 00:00 and 24:00 UT on 12 March 2018 (Kiruna), 15 March 2018 (Kiruna) and 16 March 2018 (Ny-Ålesund), respectively. The number of satellites utilised in the 30 s positioning solution decreased in the more active days in the auroral and polar ionospheres because of a combination of aspects, i.e., arc too short, occurrence of a cycle slip, outliers in the geometry-free (
) combination, outliers in the Melbourne–Wübbena (
) combination. Occasionally, PRNs were excluded because of an elevation angle lower than 5° although this aspect seemed to be independent of geomagnetic conditions and related to the orbits. On the contrary, the number of satellites utilised in the 1 s positioning solutions remained stable throughout the days considered here.
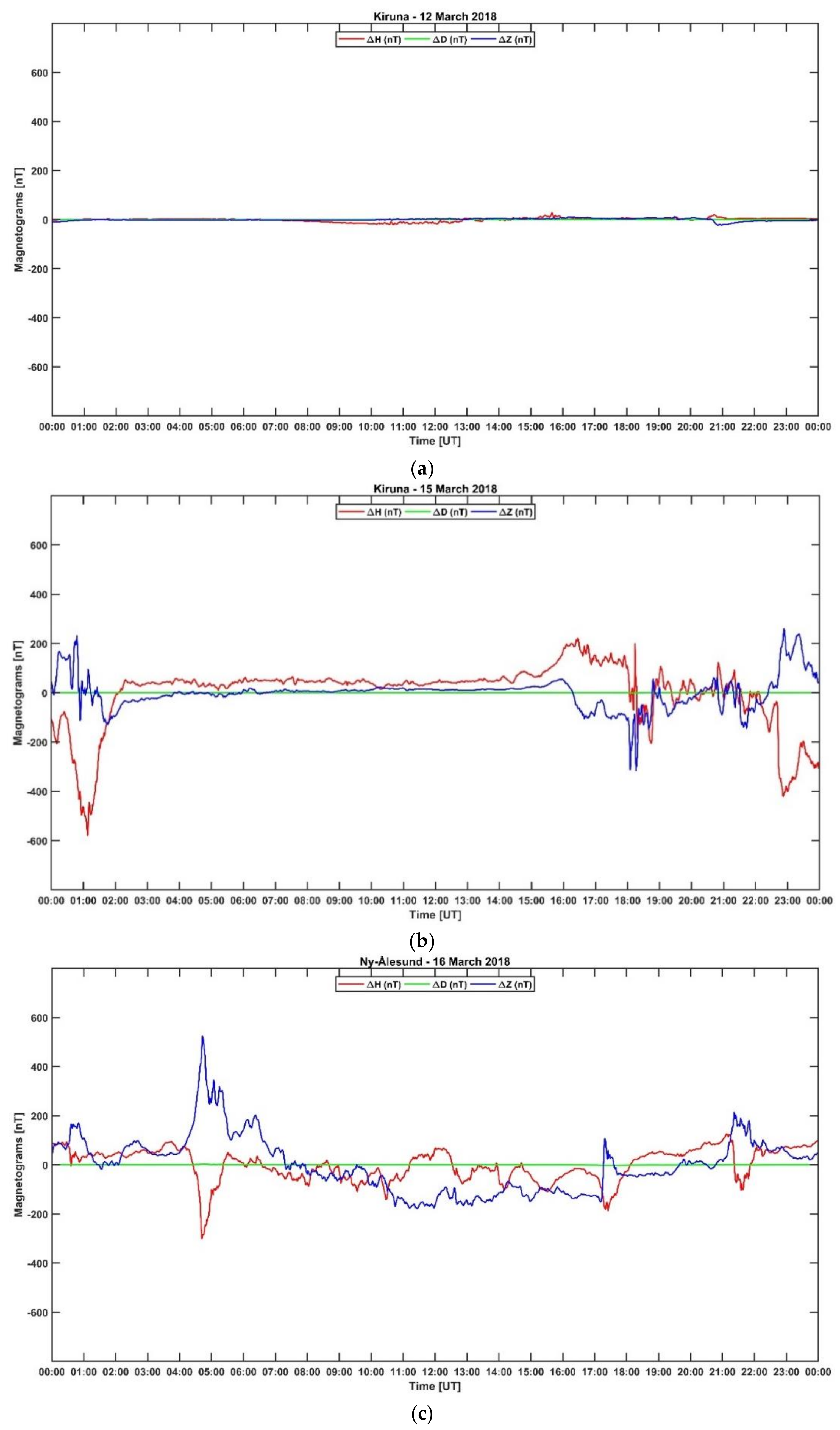
The inspection of the magnetograms recorded in Kiruna on 12 March 2018 and 15 March 2018, and in Ny-Ålesund on 16 March 2018 (
Figure 10) reveals how sensitive the 30 s positioning solutions were to magnetic conditions: the decrease in the number of satellites utilised closely matched the perturbations in the geomagnetic field.
During the EISCAT UHF/ESR measurements (20:00–24:00 UT) such a decrease in the number of satellites utilised in the positioning solutions occurred in correspondence of irregularities forming due to particle precipitation and a polar plasma patch which were detected on the electron density profiles. This aspect is further illustrated through
Figure 11,
Figure 12 and
Figure 13 which show the variation of the radars’ Vertical Total Electron Content (VTEC) estimated by integrating the EISCAT UHF/ESR electron density profiles with height between 100 km and 400 km of altitude [
20].
Figure 11,
Figure 12 and
Figure 13 show that the number of satellites excluded from the 30 s positioning solutions increased when the radar’s VTEC showed higher values and higher variability in correspondence of irregularities forming between the E and the F regions. Here, the number of satellites with too short an arc represents the cumulative number of satellites excluded for individual specific reasons (as shown in
Figure 7,
Figure 8 and
Figure 9). On the contrary, the 1 s positioning solutions were not affected as the number of satellites utilised remained almost constant in the presence of those irregularities.
The geometry-free combination
is given by the combination of L1 and L2 carrier phases and it can be written as [
48]:
where
and
are the carrier phases in cycles corresponding to the carrier frequencies
and
in
;
and
are the wavelengths in m; the slant TEC is given in TECU. The terms
and
contain biases and errors, and are given by:
where
,
describes receiver and satellite instrumental biases,
describes the ambiguity in the carrier phases,
describes the phase wind-up,
describes errors due to multipath, and
describes errors due to receiver noise [
44].
In the Melbourne-Wübbena combination (
) [
43,
44,
45] carrier phases and codes measurements are combined through the wide-lane (
) and narrow-lane (
) combinations [
49,
50]. It can be shown that the Melbourne-Wübbena combination is given by:
where the wide-lane combination for the carrier phases is given by:
and the narrow-lane combination for the code pseudoranges is given by:
with
and
the pseudoranges (in units of m) estimated from the code at the carrier frequencies
and
.
In Equation (6), it can be shown that:
where
describes receiver and satellite instrumental biases,
describes errors due to multipath, and
describes errors due to the receiver noise [
49].
Residual errors in the presence of ionospheric irregularities during active geomagnetic conditions (
) must arise from the error terms described through Equations (4)–(9). A close inspection of Equations (5)–(9) indicates that the receiver and satellite instrumental biases together are not expected to vary with geomagnetic conditions and can be considered constant over the short-term measurement epochs of relevance. Errors due to ground multipath could, in principle, have an effect since all PRNs with elevation angles higher than 5° were considered: however, ground multipath cannot justify the results presented here as it depends upon the orbits and does not vary with geomagnetic conditions. Necessarily, the error terms that can be affected by a degradation in the geomagnetic conditions are the terms referring to the ambiguities, to the phase wind-up, and to the receiver noise. The presence of irregularities (as indicated by enhancements in GPS TEC fluctuations as well as by higher values and higher variability in radars’ VTEC) introduces higher phase errors with higher variability in the receiver carrier and code tracking [
4,
49]. These higher errors with higher variability generated at the signal tracking stage then propagate onto the observables to produce higher residual errors with higher variability: these residual errors increase the uncertainty on the phase ambiguities, the phase wind-up and the receiver noise over shorter temporal intervals.
In Equations (4)–(9) these error terms are typically indicated as constants (or as very slow functions of time). However, the results presented through
Figure 1,
Figure 2,
Figure 3 and
Figure 7,
Figure 8,
Figure 9,
Figure 10,
Figure 11,
Figure 12,
Figure 13 indicate that they become faster functions of time over shorter temporal intervals (i.e., temporal transients) in the presence of ionospheric irregularities: that is, they increase with the variability in the ionisation (summarised through the radars’ VTEC in
Figure 11,
Figure 12 and
Figure 13). These higher-order temporal transients in the error terms in Equations (5) and (9) are not removed through the dual-frequency combination of the observables and therefore introduce residual errors in the positioning solution. Due to their higher variability these transients introduce outliers in the cycle-slip detectors based upon the geometry-free and the Melbourne–Wübbena combinations, hence leading to gaps in the positioning solutions. These higher-order temporal transients remain low and vary slowly with time over 1 s intervals whereas they vary more significantly over 30 s intervals given that GPS TEC fluctuations attain lower variability over 1 s intervals as opposed to 30 s intervals in the presence of irregularities with a scale size ranging from approximately few kilometres in the E region to few tens of kilometres in the F region (compare
Figure 1,
Figure 2,
Figure 3 and
Figure 11,
Figure 12,
Figure 13).
Although the results presented here depend upon the specific implementation of the cycle-slip detectors within a given positioning algorithm, it is evident how irregularities in the active auroral and polar ionosphere introduce a degradation due to the increase of errors in the phase and code measurements, with the net result of reducing the number of satellites available for the positioning solutions.
These results indicate that the thresholds and the internal parameters of the models utilised in the cycle-slip detectors within positioning algorithms such as gLAB can be improved for the 30 s positioning solutions [
51] to reflect the variability in TEC, as illustrated here in the case of active auroral and polar ionospheres. However, the use of a higher sampling rate immediately increased the performance of the positioning solutions both in real-time and in post-processing. This aspect is in line with the results shown in Vani et al. [
42] where a high sampling rate was found necessary to reduce errors and gaps in the positioning solution during the presence of intensity scintillation originating from small-scale irregularities in the post-sunset equatorial ionosphere.
A higher sampling rate for the observables measurements and a higher rate at which positioning is performed seemed to provide an immediate mitigation against residual errors introduced during active geomagnetic conditions. A higher sampling rate has the advantage of reducing the complexity of both the receiver and the positioning algorithms: it is indeed not necessary to optimise the tracking stages of the receiver or the specific settings of the positioning algorithms (e.g., by introducing additional models that depend upon external information in order to specifically correct for higher-order error terms due to enhanced TEC fluctuations and scintillation) as they become optimal over shorter sampling intervals in their standard configuration. The trade-off between a larger memory storage requirement (to accommodate for higher sampling rates) and the reduction in the complexity of both the receiver logics and the positioning algorithm seems to provide a suitable solution for precision applications in both real-time and post-processing.
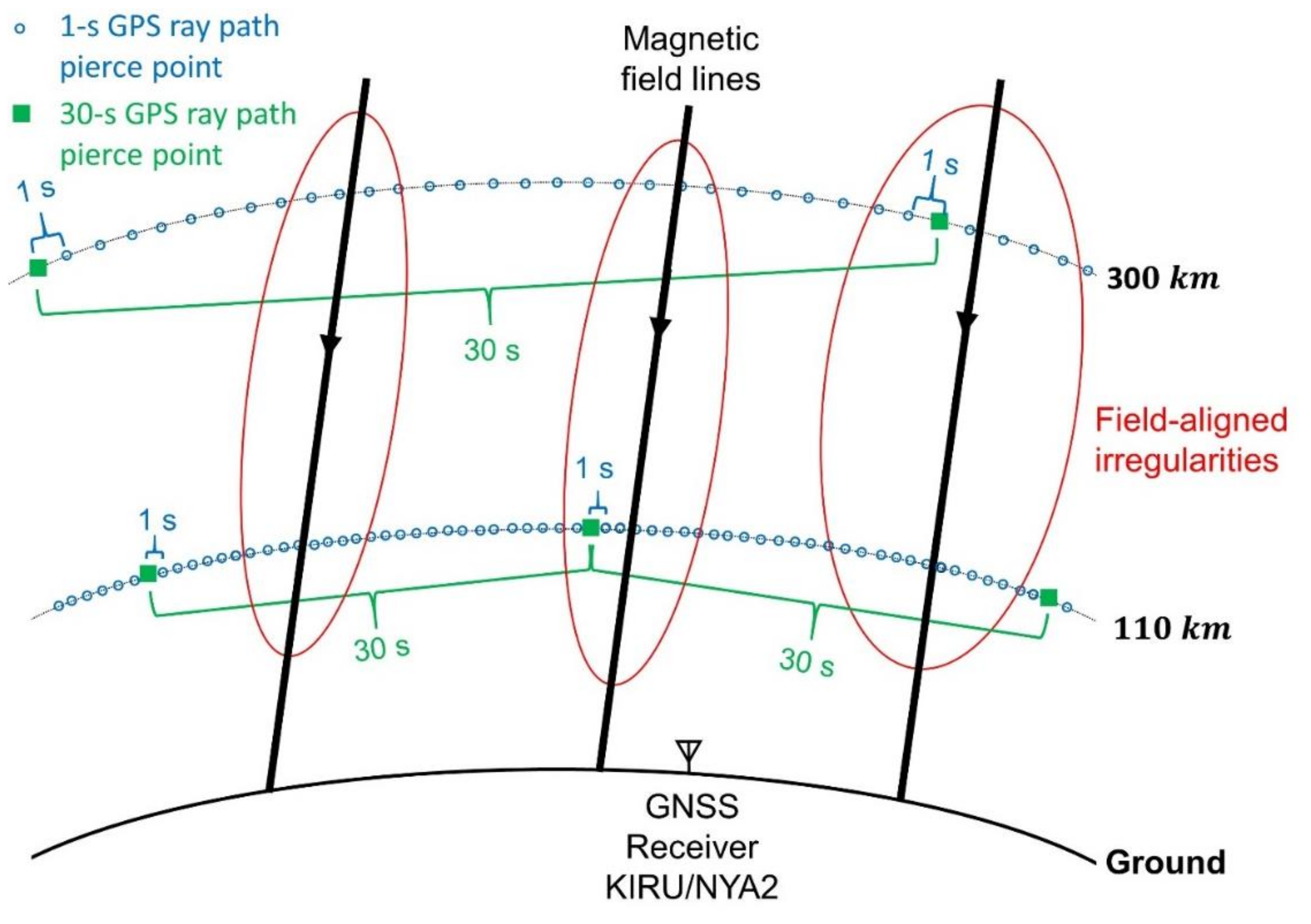
Therefore, in relation to question (1), the irregularities responsible for higher variability and gaps in the positioning solutions were those forming between the E and the F regions with scale sizes ranging approximately from few kilometres in the E region to few tens of kilometres in the F region. This scale size is comparable to the separation distance between their intersections with GPS ray paths. These irregularities originated enhancements in GPS TEC fluctuations over 30 s intervals and over 1 s intervals. However, TEC changes over 1 s intervals were smaller than those occurring over 30 s: this aspect can be visualised in the schematic diagram in
Figure 14 where GPS ray paths spent more time within the irregularities when the sampling interval is 1 s. At 30 s sampling interval these irregularities were responsible for higher phase errors and gaps in the positioning solutions.
In relation to question (2), higher-order temporal transients introduced residual errors on the observables as well as outliers in the cycle-slip detectors: these transients were responsible for the increase in the 3-D positioning error and in the occurrence of gaps. Whilst these transients have lower values and lower variability over 1 s intervals, they become significant over 30 s intervals in the presence of irregularities forming between the E and the F regions in the active auroral and polar ionospheres.