Assessment of Multi-Frequency PPP Ambiguity Resolution Using Galileo and BeiDou-3 Signals
Abstract
:1. Introduction
2. Methods
2.1. Multi-Frequency PPP Model
2.2. Triple-Frequency PPP Ambiguity Resolution
2.3. Galileo/BeiDou-3 Combination Observables for Rapid PPP-AR
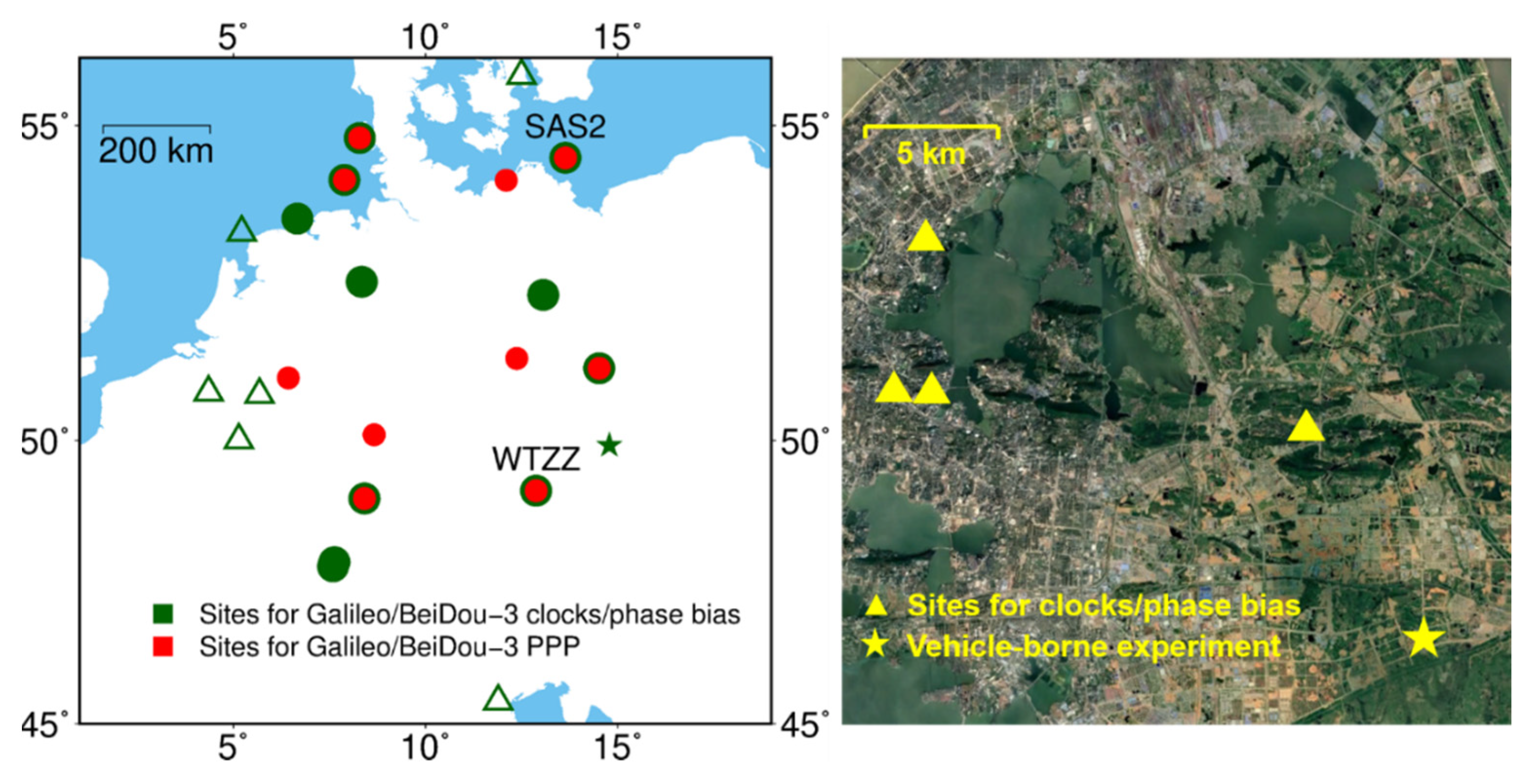
3. Data Processing
4. Results
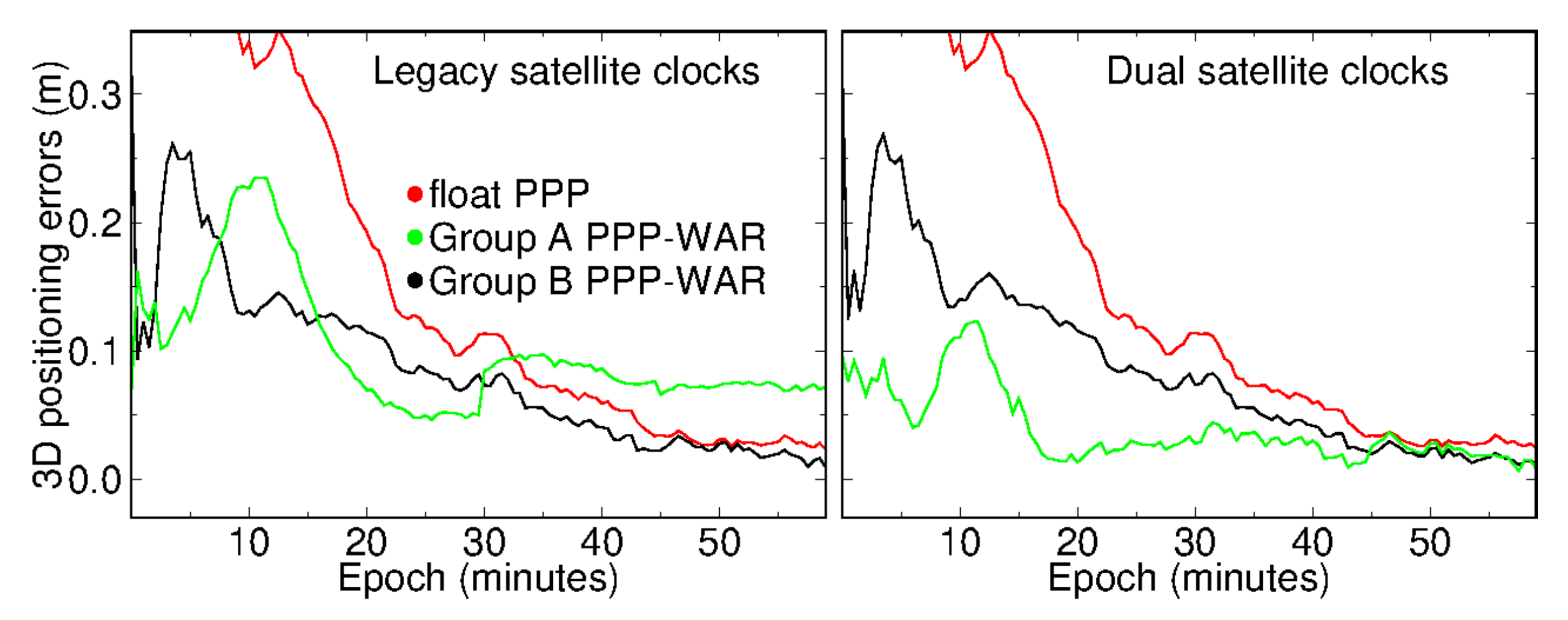
4.1. PPP-WAR
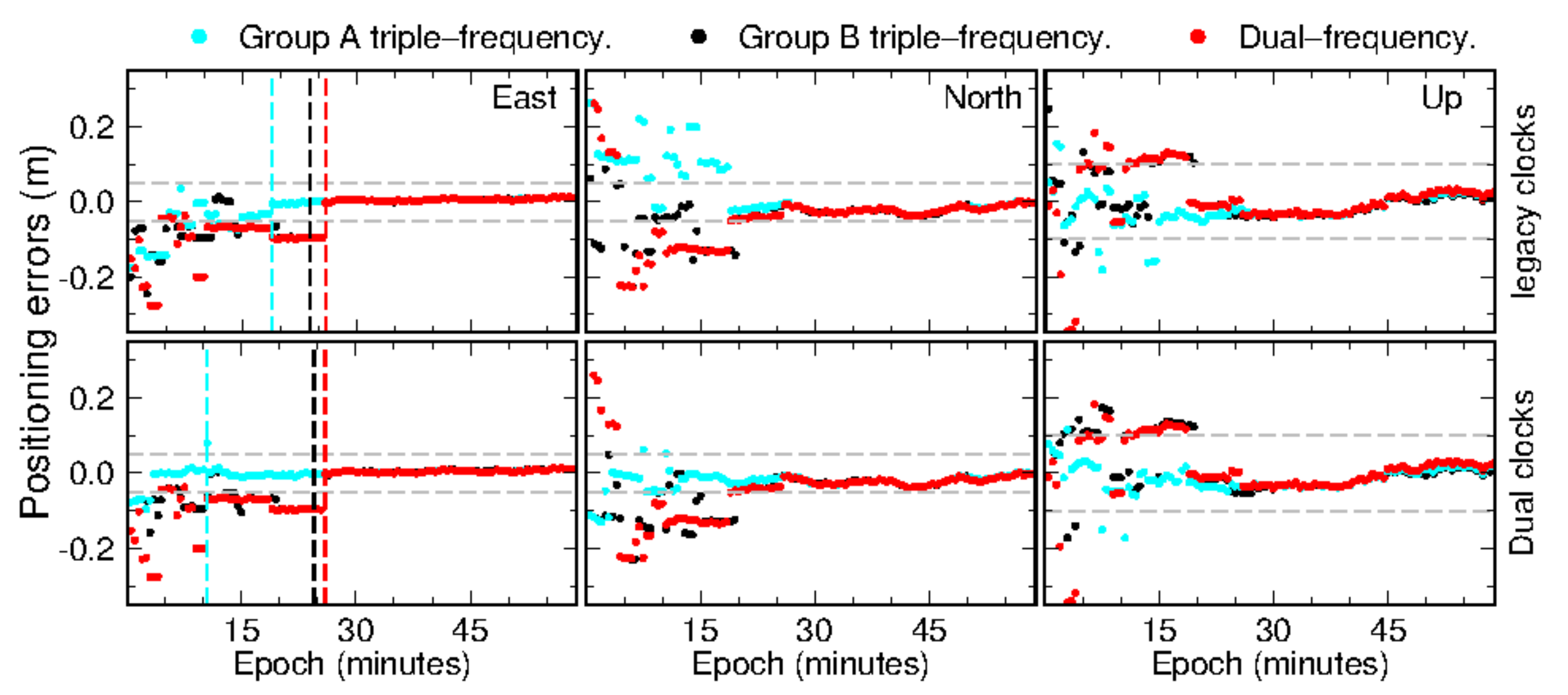
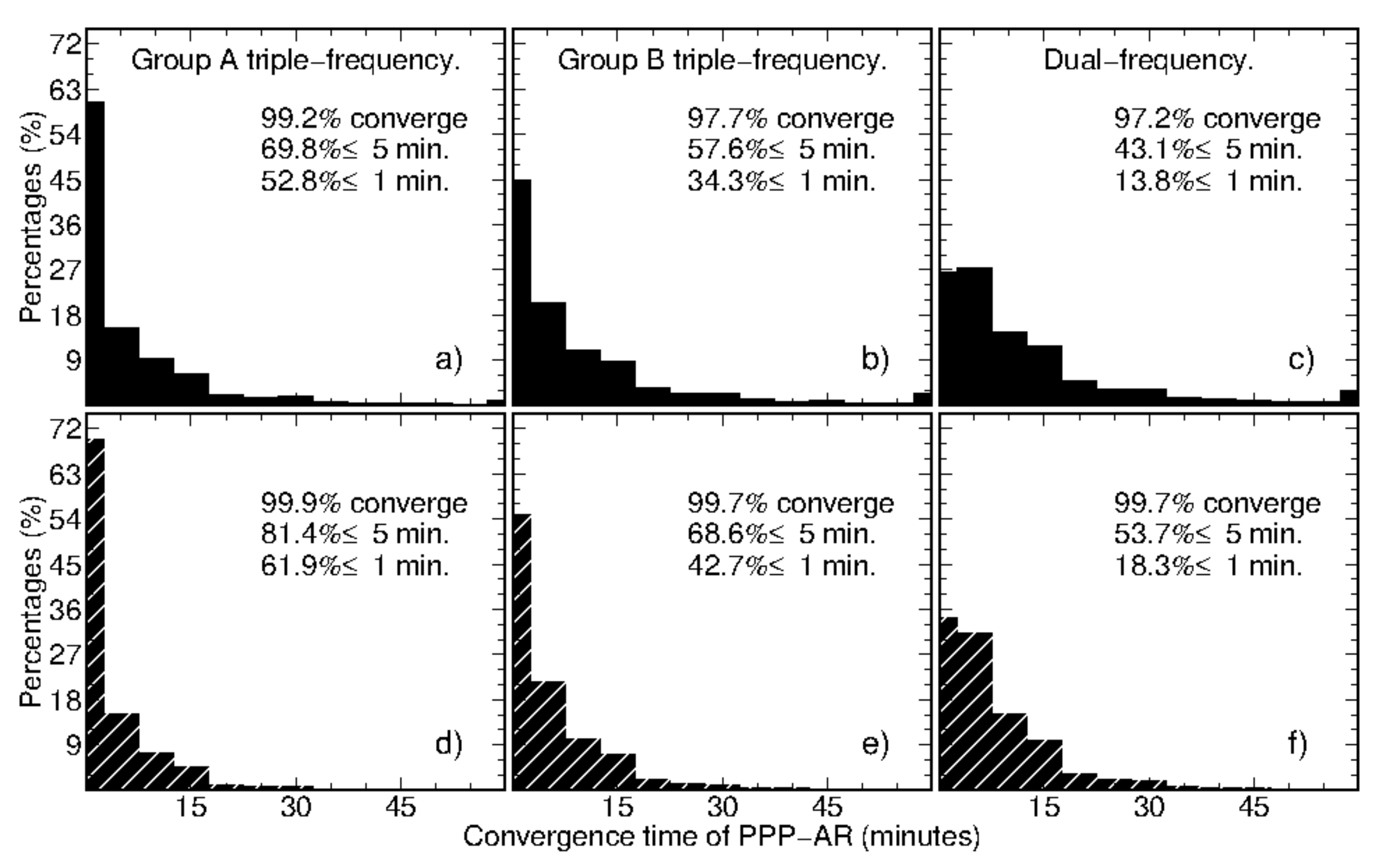
4.2. PPP-AR
4.3. Vehicle-Borne Experiment
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Malys, S.; Jensen, P.A. Geodetic point positioning with GPS carrier beat phase data from the CASA UNO Experiment. Geophys. Res. Lett. 1990, 17, 651–654. [Google Scholar] [CrossRef]
- Zumberge, J.F.; Heflin, M.B.; Jefferson, D.C.; Watkins, M.M.; Webb, F.H. Precise point positioning for the efficient and robust analysis of GPS data from large networks. J. Geophys. Res. Space Phys. 1997, 102, 5005–5017. [Google Scholar] [CrossRef] [Green Version]
- An, X.; Meng, X.; Jiang, W. Multi-constellation GNSS precise point positioning with multi-frequency raw observations and dual-frequency observations of ionospheric-free linear combination. Satell. Navig. 2020, 1, 1–13. [Google Scholar] [CrossRef]
- Bisnath, S.; Gao, Y. Current state of precise point positioning and future prospects and limitations. In Observing Our Changing Earth; Sideris, M.G., Ed.; Springer: Berlin/Heidelberg, Germany, 2009; pp. 615–623. [Google Scholar]
- Teunissen, P.J.G.; Odolinski, R.; Odijk, D. Instantaneous BeiDou+GPS RTK positioning with high cut-off elevation angles. J. Geod. 2014, 88, 335–350. [Google Scholar] [CrossRef]
- Wübbena, G.; Schmitz, M.; Bagge, A. PPP-RTK: Precise point positioning using state-space representation in RTK networks. In Proceedings of the ION GNSS 2005, Institute of Navigation, Inc., Fairfax, VA, USA, 13–16 September 2005; pp. 2584–2594. [Google Scholar]
- Geng, J.; Meng, X.; Dodson, A.H.; Ge, M.; Teferle, F.N. Rapid re-convergences to ambiguity-fixed solutions in precise point positioning. J. Geod. 2010, 84, 705–714. [Google Scholar] [CrossRef] [Green Version]
- Zhang, B.; Chen, Y.; Yuan, Y. PPP-RTK based on undifferenced and uncombined observations: Theoretical and practical aspects. J. Geod. 2019, 93, 1011–1024. [Google Scholar] [CrossRef]
- Hein, G.W. Status, perspectives and trends of satellite navigation. Satell. Navig. 2020, 1, 22. [Google Scholar] [CrossRef]
- Geng, J.; Shi, C. Rapid initialization of real-time PPP by resolving undifferenced GPS and GLONASS ambiguities simultaneously. J. Geod. 2017, 91, 1–14. [Google Scholar] [CrossRef]
- Liu, Y.; Lou, Y.; Ye, S.; Zhang, R.; Song, W.; Li, Q. Assessment of PPP integer ambiguity resolution using GPS, GLONASS and BeiDou (IGSO, MEO) constellations. GPS Solut. 2017, 21, 1647–1659. [Google Scholar] [CrossRef]
- Hatch, R.; Jung, J.; Enge, P.; Pervan, B. Civilian GPS: The Benefits of Three Frequencies. GPS Solut. 2000, 3, 1–9. [Google Scholar] [CrossRef]
- Geng, J.; Bock, Y. Triple-frequency GPS precise point positioning with rapid ambiguity resolution. J. Geod. 2013, 87, 449–460. [Google Scholar] [CrossRef]
- Melbourne, W.G. The case for ranging in GPS-based geodetic systems. In Proceedings of the 1st International Symposium on Precise Positioning with the Global Positioning System, Rockville, MD, USA, 15–19 April 1985; pp. 373–386. [Google Scholar]
- Wübbena, G. Software developments for geodetic positioning with GPS using TI-4100 code and carrier measurements. In Proceedings of the 1st International Symposium on Precise Positioning with the Global Positioning System, Rockville, MD, USA, 15–19 April 1985; pp. 403–412. [Google Scholar]
- Geng, J.; Guo, J.; Meng, X.; Gao, K. Speeding up PPP ambiguity resolution using triple-frequency GPS/BeiDou/Galileo/QZSS data. J. Geod. 2020, 94, 1–15. [Google Scholar] [CrossRef] [Green Version]
- Xiao, G.; Li, P.; Gao, Y.; Heck, B. A Unified Model for Multi-Frequency PPP Ambiguity Resolution and Test Results with Galileo and BeiDou Triple-Frequency Observations. Remote Sens. 2019, 11, 116. [Google Scholar] [CrossRef] [Green Version]
- Liu, G.; Zhang, X.; Li, P. Improving the performance of Galileo uncombined precise point positioning ambiguity resolution using triple-frequency observations. Remote Sens. 2019, 11, 341. [Google Scholar] [CrossRef] [Green Version]
- Duong, V.; Harima, K.; Choy, S.; Laurichesse, D.; Rizos, C. Assessing the performance of multi-frequency GPS, Galileo and BeiDou PPP ambiguity resolution. J. Spat. Sci. 2019, 65, 61–78. [Google Scholar] [CrossRef]
- Guo, J.; Xin, S. Toward single-epoch 10-centimeter precise point positioning using Galileo E1/E5a and E6 signals. In Proceedings of the ION GNSS 2019, Institute of Navigation, Miami, FL, USA, 16–20 September 2019; pp. 2870–2887. [Google Scholar]
- Psychas, D.; Teunissen, P.; Verhagen, S. A Multi-Frequency Galileo PPP-RTK Convergence Analysis with an Emphasis on the Role of Frequency Spacing. Remote Sens. 2021, 13, 3077. [Google Scholar] [CrossRef]
- Geng, J.; Guo, J. Beyond three frequencies: An extendable model for single-epoch decimeter-level point positioning by exploiting Galileo and BeiDou-3 signals. J. Geod. 2020, 94, 14. [Google Scholar] [CrossRef]
- Odijk, D.; Zhang, B.; Khodabandeh, A.; Odolinski, R.; Teunissen, P. On the estimability of parameters in undifferenced, uncombined GNSS network and PPP-RTK user models by means of S-system theory. J. Geod. 2016, 90, 15–44. [Google Scholar] [CrossRef]
- Guo, J.; Geng, J. GPS satellite clock determination in case of inter-frequency clock biases for triple-frequency precise point positioning. J. Geod. 2017, 92, 1133–1142. [Google Scholar] [CrossRef]
- Ge, M.; Gendt, G.; Rothacher, M.; Shi, C.; Liu, J. Resolution of GPS carrier-phase ambiguities in Precise Point Positioning (PPP) with daily observations. J. Geod. 2008, 82, 389–399. [Google Scholar] [CrossRef]
- Laurichesse, D.; Mercier, F.; Berthias, J.-P.; Broca, P.; Cerri, L. Integer ambiguity resolution on undifferenced GPS phase measurements and its application to PPP and satellite precise orbit determination. Navig. J. Inst. Navig. 2009, 56, 135–149. [Google Scholar] [CrossRef]
- Geng, J.; Meng, X.; Dodson, A.H.; Teferle, F.N. Integer ambiguity resolution in precise point positioning: Method comparison. J. Geod. 2010, 84, 569–581. [Google Scholar] [CrossRef] [Green Version]
- Teunissen, P.J.G. The least-squares ambiguity decorrelation adjustment: A method for fast GPS integer ambiguity estimation. J. Geod. 1995, 70, 65–82. [Google Scholar] [CrossRef]
- Guo, J.; Xu, X.; Zhao, Q.; Liu, J. Precise orbit determination for quad-constellation satellites at Wuhan University: Strategy, result validation, and comparison. J. Geod. 2016, 90, 143–159. [Google Scholar] [CrossRef]
- IGS AC Coordinator. Conventions and Modeling for Repro3. 2019. Available online: http://acc.igs.org/repro3/repro3.html (accessed on 6 June 2020).
- Saastamoinen, J. Contribution to the theory of atmospheric refraction: Refraction corrections in satellite geodesy. Bull. Geod. 1973, 107, 13–34. [Google Scholar] [CrossRef]
- Boehm, J.; Niell, A.; Tregoning, P.; Schuh, H. Global Mapping Function (GMF): A new empirical mapping function based on numerical weather model data. Geophys. Res. Lett. 2006, 33, L07304. [Google Scholar] [CrossRef] [Green Version]
- Teunissen, P.J.G. The geometry-free GPS ambiguity search space with a weighted ionosphere. J. Geod. 1997, 71, 370–383. [Google Scholar] [CrossRef] [Green Version]
- Geng, J.; Guo, J.; Wang, C.; Zhang, Q. Satellite antenna phase center errors: Magnified threat to multi-frequency PPP ambiguity resolution. J. Geod. 2021, 95, 72. [Google Scholar] [CrossRef]
- Xie, X.; Fang, R.; Geng, T.; Wang, G.; Zhao, Q.; Liu, J. Characterization of GNSS signals tracked by the iGMAS network considering recent BDS-3 satellites. Remote Sens. 2018, 10, 1736. [Google Scholar] [CrossRef] [Green Version]
- Steigenberger, P.; Fritsche, M.; Dach, R.; Schmid, R.; Montenbruck, O.; Uhlemann, M.; Prange, L. Estimation of satellite antenna phase center offsets for Galileo. J. Geod. 2016, 90, 773–785. [Google Scholar] [CrossRef]
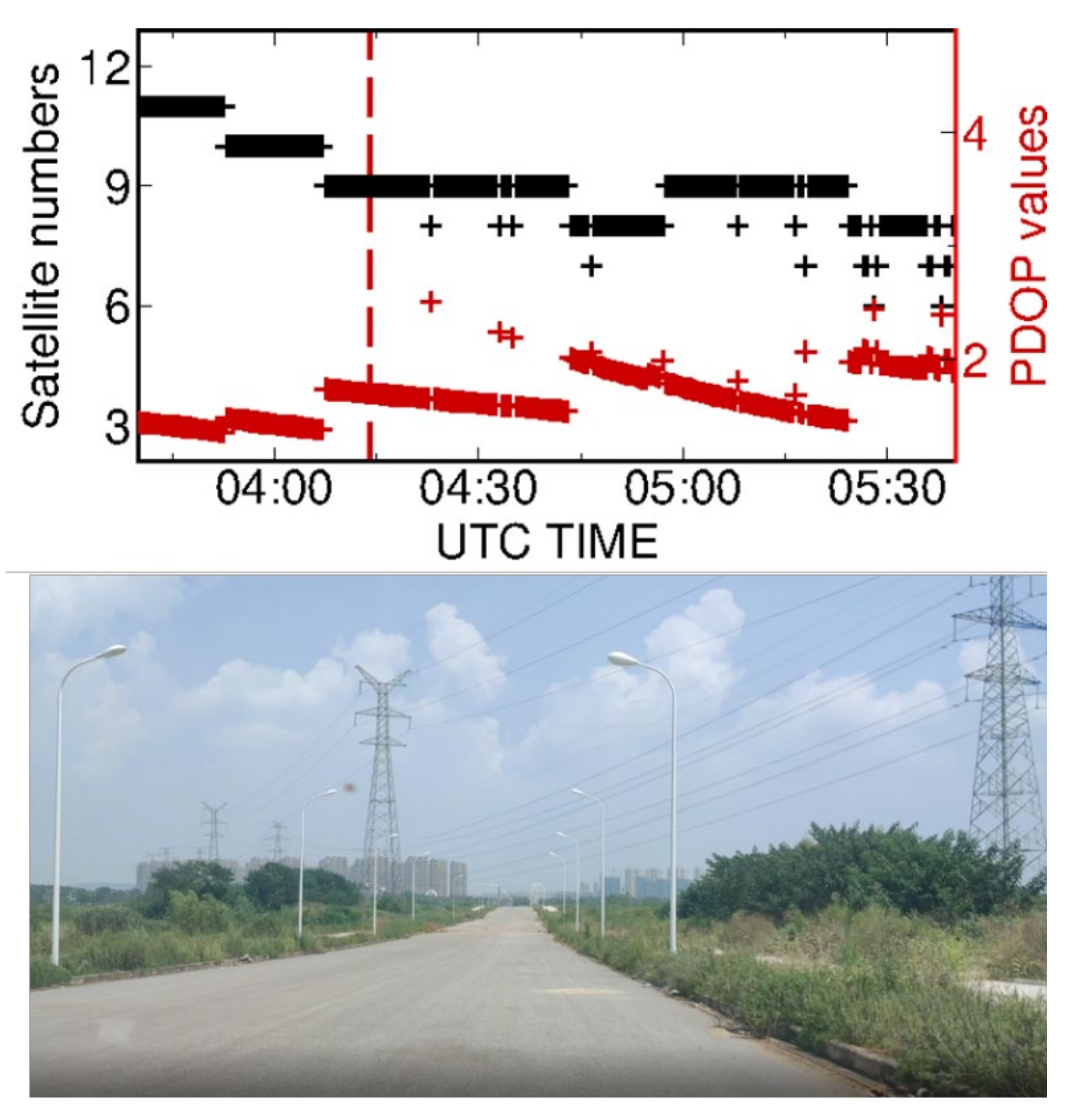
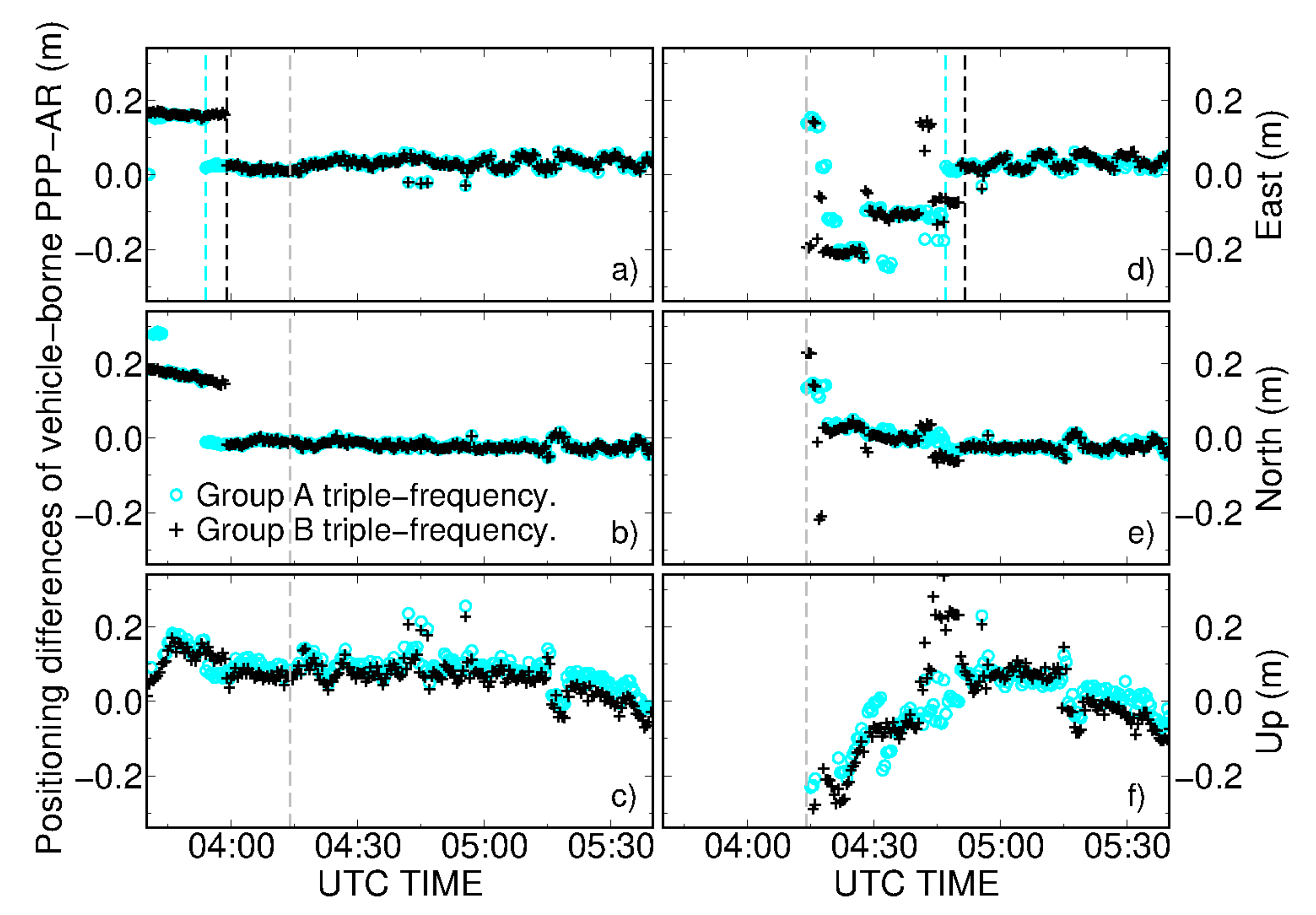
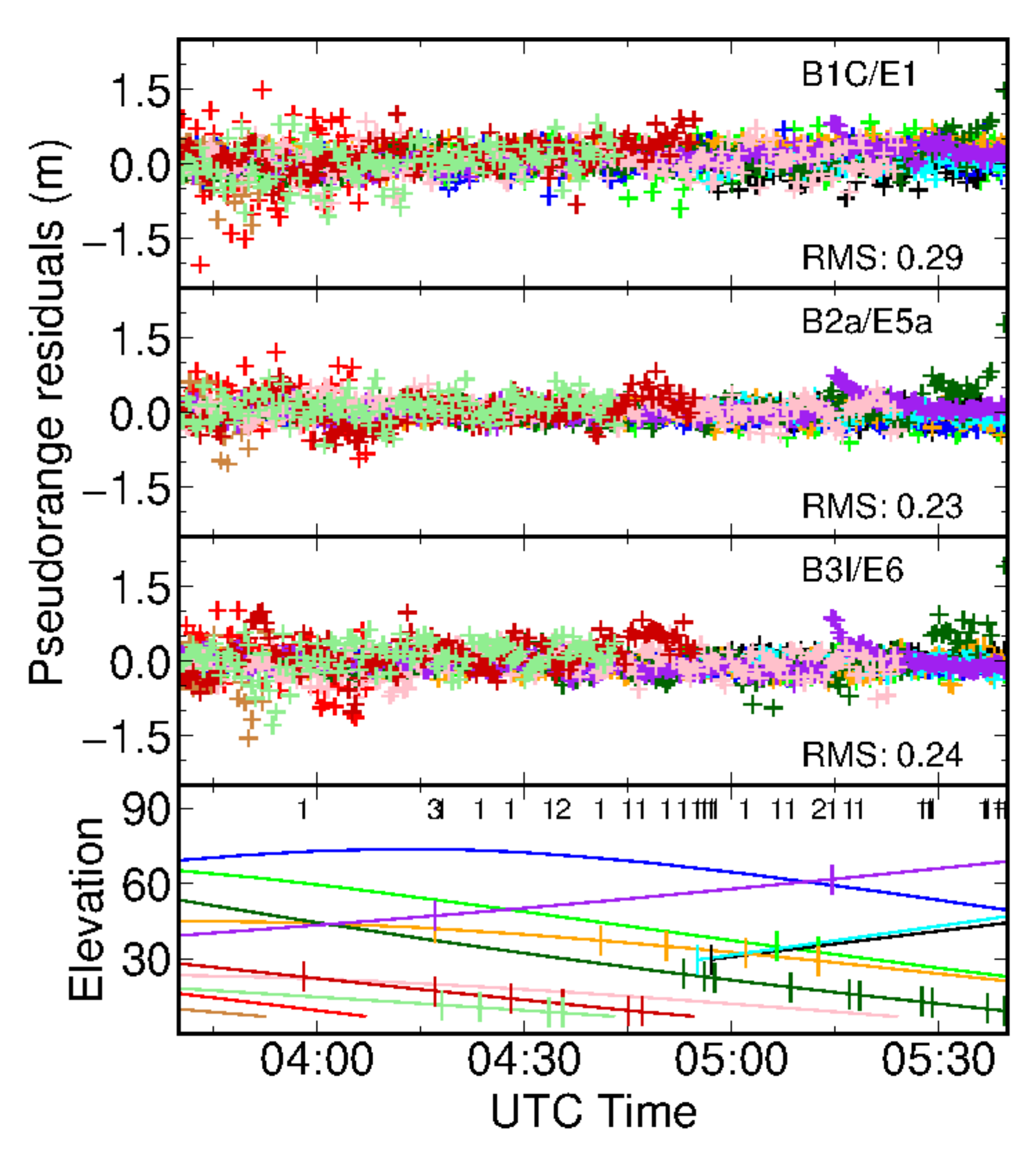

| Constellation | Signals | Noise Factor | |||
|---|---|---|---|---|---|
| GPS/QZSS | L1/L2/L5 | 109.98 | 5.86 | 0.86 | 0.11 |
| Galileo | E1/E5a/E6 | 67.03 | 2.93 | 0.75 | 0.11 |
| E1/E5a/E5b | 172.29 | 9.77 | 0.75 | 0.11 | |
| E1/E5a/E5 | 331.04 | 19.54 | 0.75 | 0.11 | |
| BeiDou-2 | B1I/B2I/B3I | 114.37 | 4.88 | 0.85 | 0.11 |
| BeiDou-3 | B1C/B2a/B3I | 71.23 | 3.26 | 0.75 | 0.11 |
| B1C/B2a/B2b | 172.29 | 9.77 | 0.75 | 0.11 | |
| B1C/B1I/B2a | 620.04 | 20.93 | 0.78 | 0.10 |
| Receiver Type | Stations | Signal |
|---|---|---|
| Javad TRE_3 DELTA | 15 | Galileo: E1/E5a/E5b/E6 BeiDou-3: B1I/B1C/B2a/B2b/B3I |
| Septentrio PolaRX5 | 6 | Galileo: E1/E5a/E5b/E6 BeiDou-3: B1I/B1C/B2a/B3I |
| Trimble Alloy | 1 | Galileo: E1/E5a/E5b/E6 BeiDou-3: B1I/B2a/B2b/B3I |
| Item | Strategy |
|---|---|
| Observation used | Galileo: E1/E5a/E5b/E6 BeiDou-3: B1I/B1C/B2a/B2b/B3I |
| Filter | Square root information filter |
| Elevation cut-off angle | 7° |
| Weighting strategy | Elevation-dependent; a priori noise of 3 mm and 0.3 m for carrier-phase and pseudorange, respectively |
| Troposphere delay | Saastamoinen model [31]; estimated hourly based on global mapping function [32] with a process noise of |
| Ionosphere delay | Estimated as random-walk-like parameters with process noise of |
| Receiver clock | Estimated as white-noise-like parameter |
| Satellite clock | Estimated as white-noise like parameter |
| Inter-system bias | Estimated as a constant |
| Ambiguity resolution | Searched by LAMBDA with a ratio test threshold of 3; |
| Partial ambiguity resolution [33] | Maximum exclude: 4 Minimum reserve: 4 |
| Data processing length | Clock estimation: 1 day PPP-WAR/PPP-AR: 1 h |
| Strategy | Legacy Satellite Clocks | Dual Satellite Clocks | |||
|---|---|---|---|---|---|
| Convergence Time (min) | Positioning RMS (m) | Convergence Time (min) | Positioning RMS (m) | ||
| Group A | Float PPP | 31.9/28.5/- | 0.15/0.14/0.23 | 31.8/28.5/- | 0.15/0.14/0.23 |
| PPP-WAR | 26.3/20.0/- | 0.06/0.08/0.14 | 18.2/13.5/44.0 | 0.05/0.07/0.13 | |
| Group B | Float PPP | 32.0/28.5/- | 0.15/0.14/0.23 | 31.9/28.5/- | 0.15/0.14/0.23 |
| PPP-WAR | 25.1/19.0/- | 0.07/0.09/0.17 | 25.0/19.5/- | 0.08/0.09/0.17 | |
| Strategy | Convergence Time (Minutes) | Rates (%) | |
|---|---|---|---|
| Legacy Satellite Clocks | Dual Satellite Clocks | ||
| All Solutions | |||
| Dual. PPP-AR | 11.6/7.0/29.0 | -- | -- |
| Group A triple. PPP-AR | 8.1/2.0/21.5 | 5.6/1.0/16.0 | 31% |
| Group B triple. PPP-AR | 9.0/3.5/26.5 | 8.8/3.5/25.0 | 2% |
| More than 10 satellites | |||
| Group A triple. PPP-AR | 5.1/1.0/15.0 | 3.1/0.5/10.0 | 39% |
| Group B triple. PPP-AR | 5.3/2.0/15.0 | 5.2/2.0/14.5 | 2% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, J.; Zhang, Q.; Li, G.; Zhang, K. Assessment of Multi-Frequency PPP Ambiguity Resolution Using Galileo and BeiDou-3 Signals. Remote Sens. 2021, 13, 4746. https://doi.org/10.3390/rs13234746
Guo J, Zhang Q, Li G, Zhang K. Assessment of Multi-Frequency PPP Ambiguity Resolution Using Galileo and BeiDou-3 Signals. Remote Sensing. 2021; 13(23):4746. https://doi.org/10.3390/rs13234746
Chicago/Turabian StyleGuo, Jiang, Qiyuan Zhang, Guangcai Li, and Kunlun Zhang. 2021. "Assessment of Multi-Frequency PPP Ambiguity Resolution Using Galileo and BeiDou-3 Signals" Remote Sensing 13, no. 23: 4746. https://doi.org/10.3390/rs13234746
APA StyleGuo, J., Zhang, Q., Li, G., & Zhang, K. (2021). Assessment of Multi-Frequency PPP Ambiguity Resolution Using Galileo and BeiDou-3 Signals. Remote Sensing, 13(23), 4746. https://doi.org/10.3390/rs13234746






