Environmental Sensitivity of Large Stealth Longwave Antenna Systems
Abstract
:1. Introduction
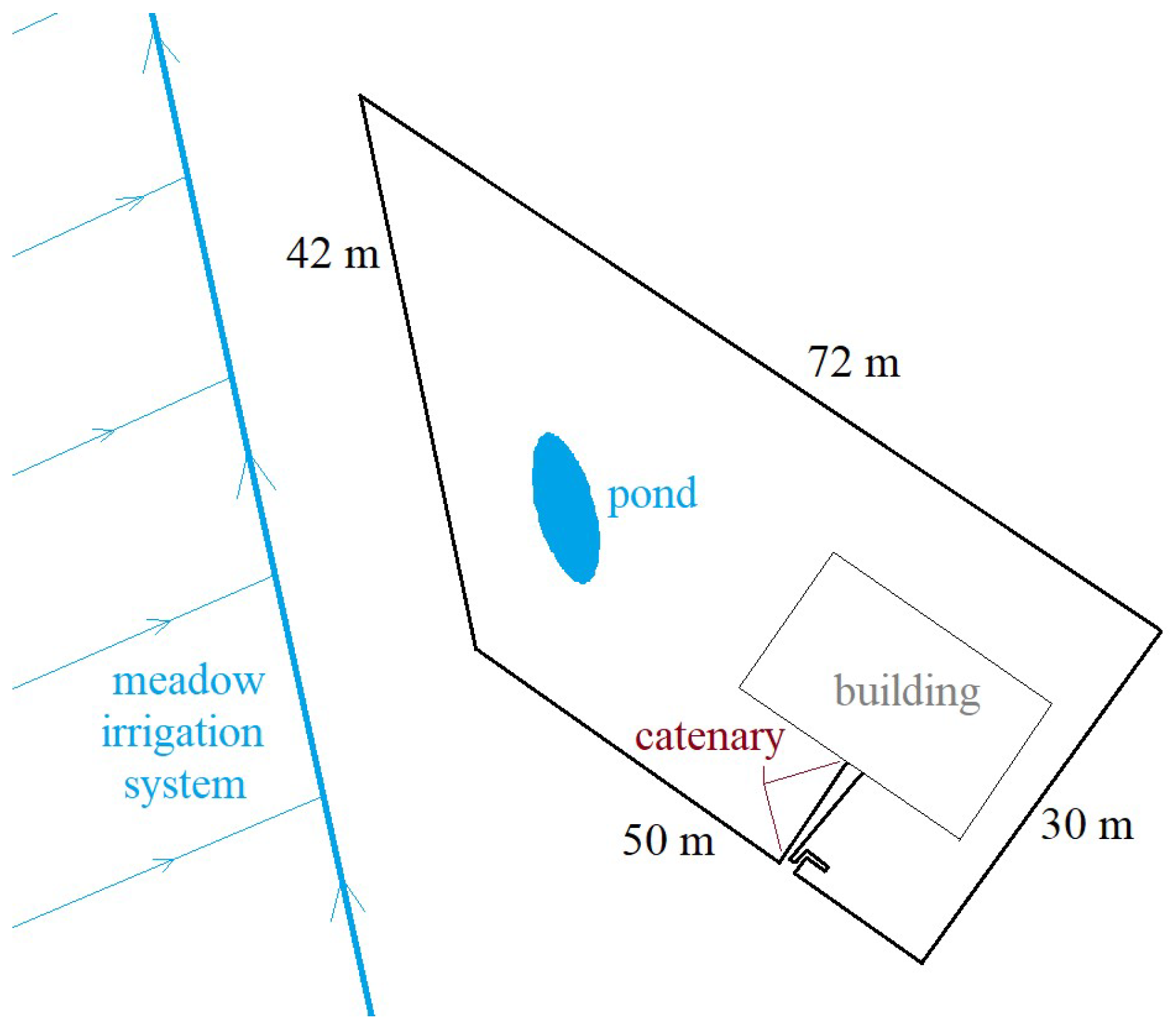
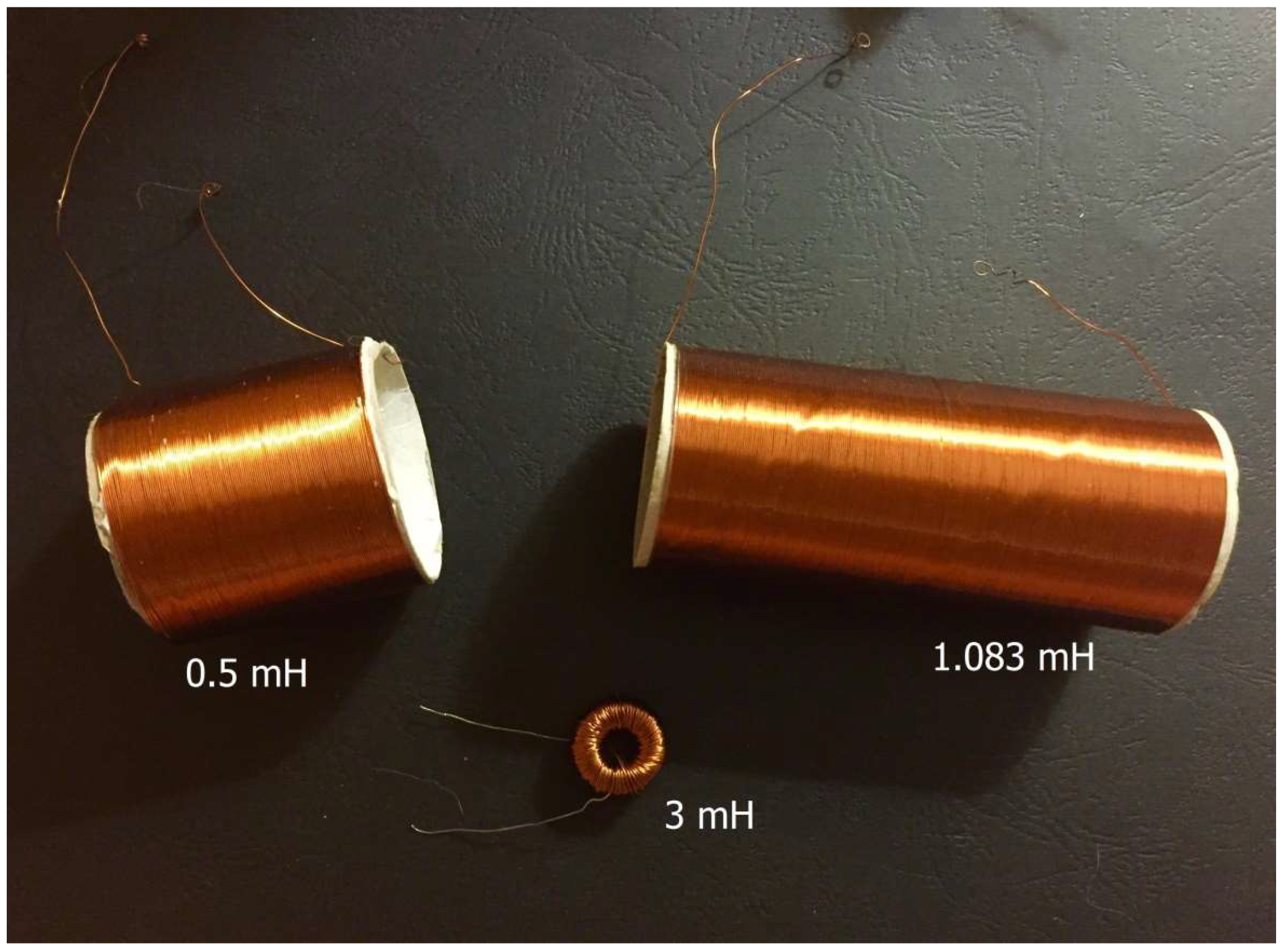
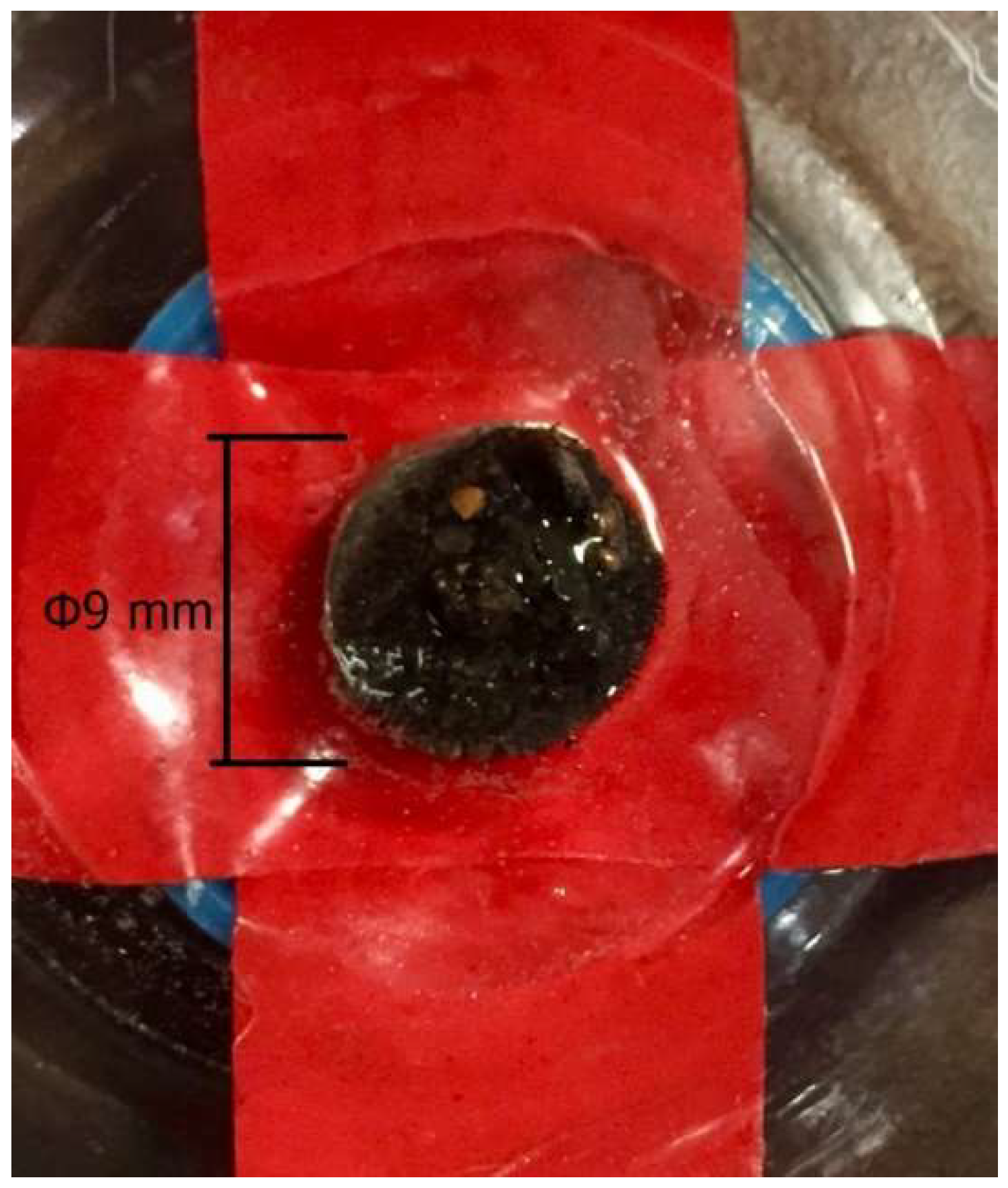
2. Experiment Setup
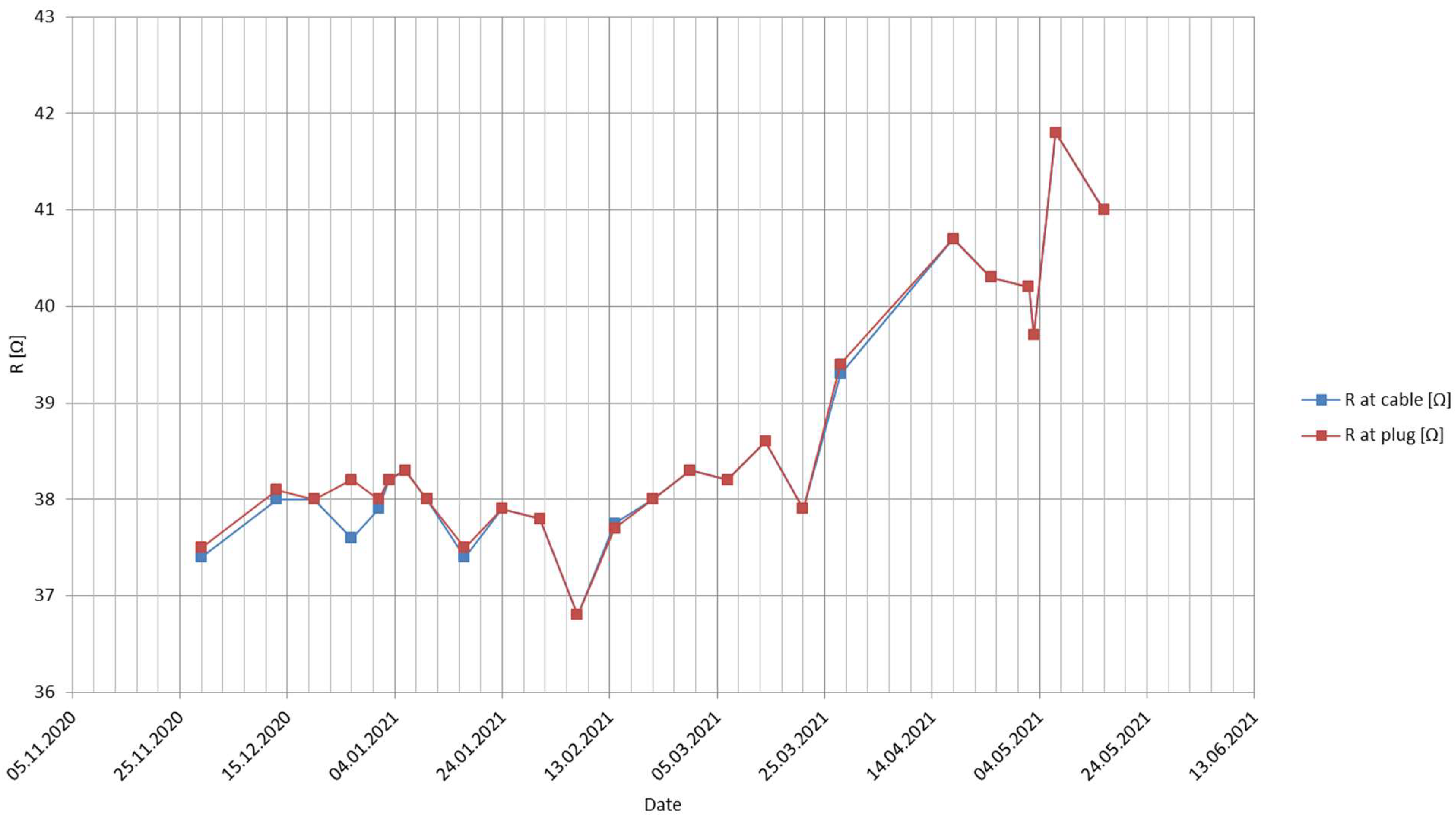
3. Longtime Measurements
4. System Parameters’ Simulations
- •
- Main relation to the measured primary parameter of the loop (the inductance);
- •
- Dependence on the temperature and humidity;
- •
- Detectability by the employed measuring device.
- •
- The measured inductance values are directly related to the inductance of the components of the surrounding environment, which may change with temperature and humidity (liquid water causing ferromagnetic particle movements over a large area etc.)—Section 4.1;
- •
- The changing state of the environment (liquid water, snow, ice) may change the linear parameters of the antenna considered as a transmission line, which—given the probing frequency of the measuring device—may show the changes on the measured parameters—Section 4.2;
- •
- The functioning of the measuring device (the differentiating circuit of the digital meter) may indirectly employ a physical parameter, which is environmentally dependent and, therefore, may influence the measured parameters—Section 4.3.
4.1. Approach for The Environment’s Inductance
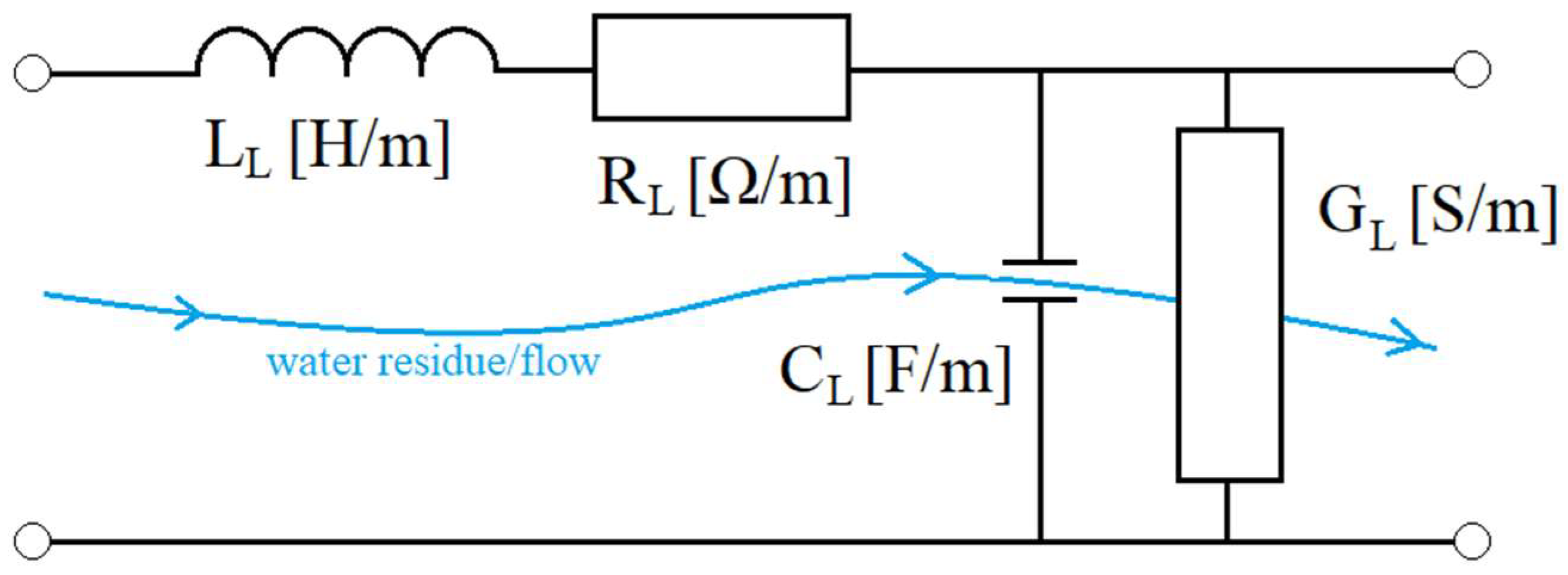
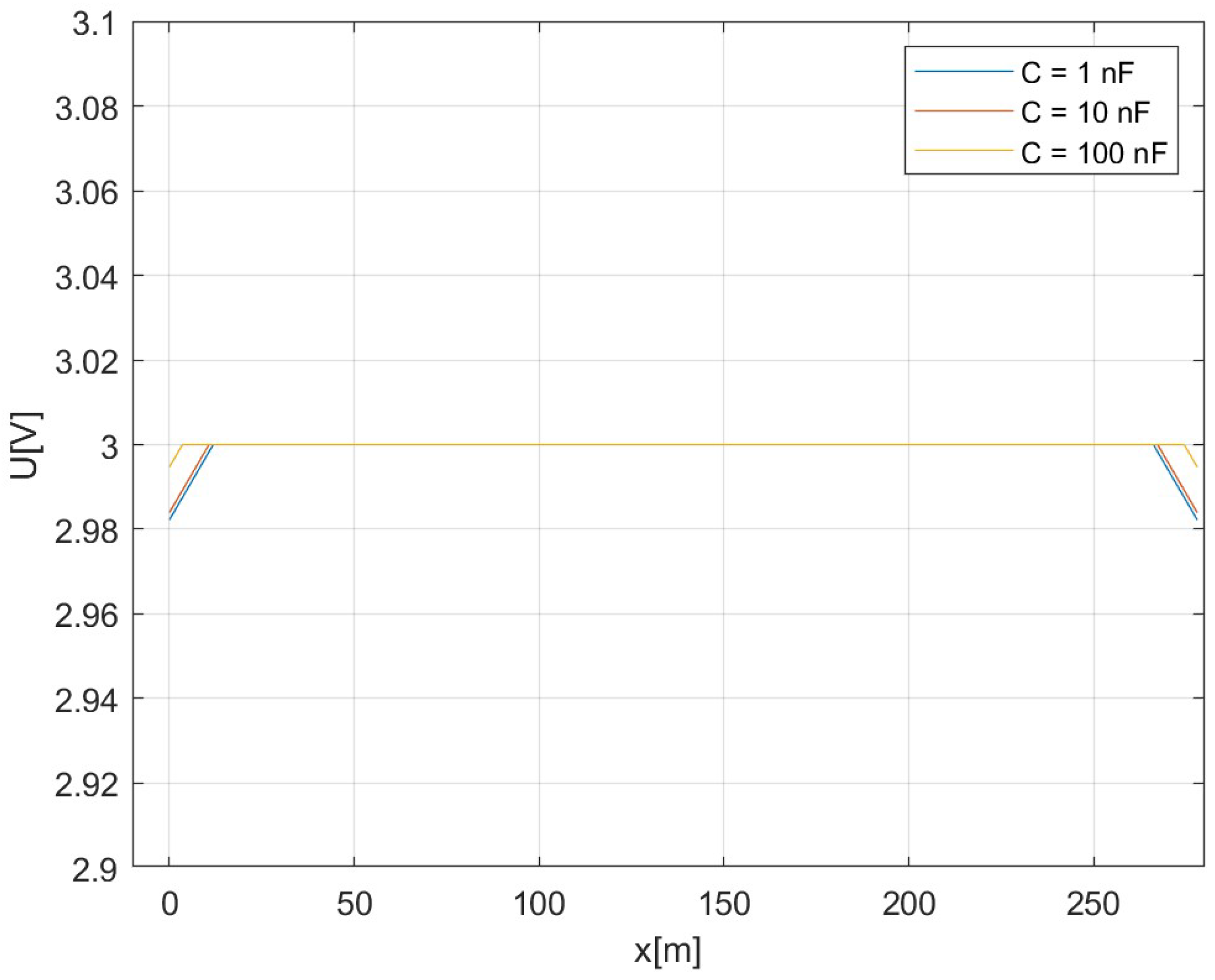
4.2. Transmission Line Model
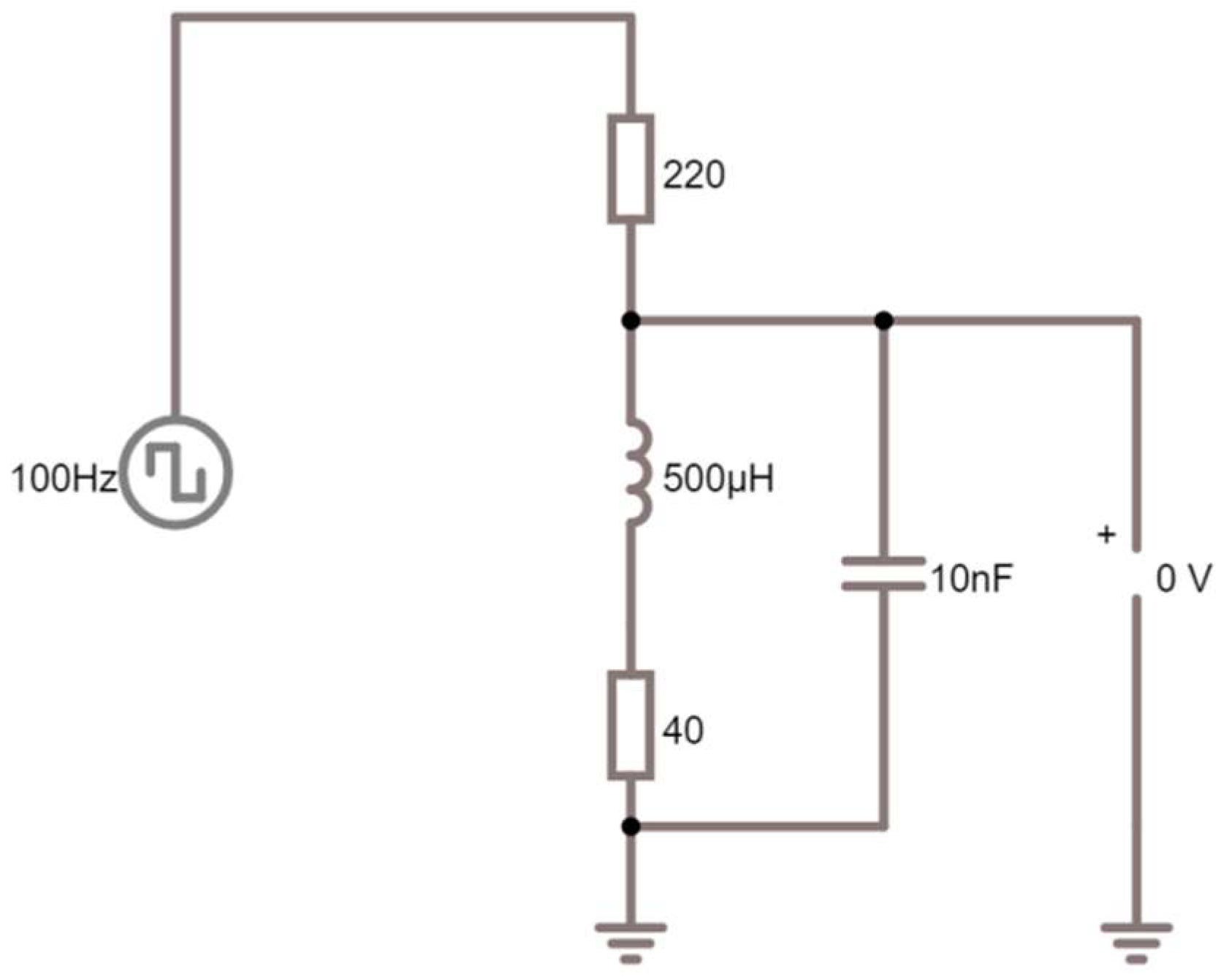
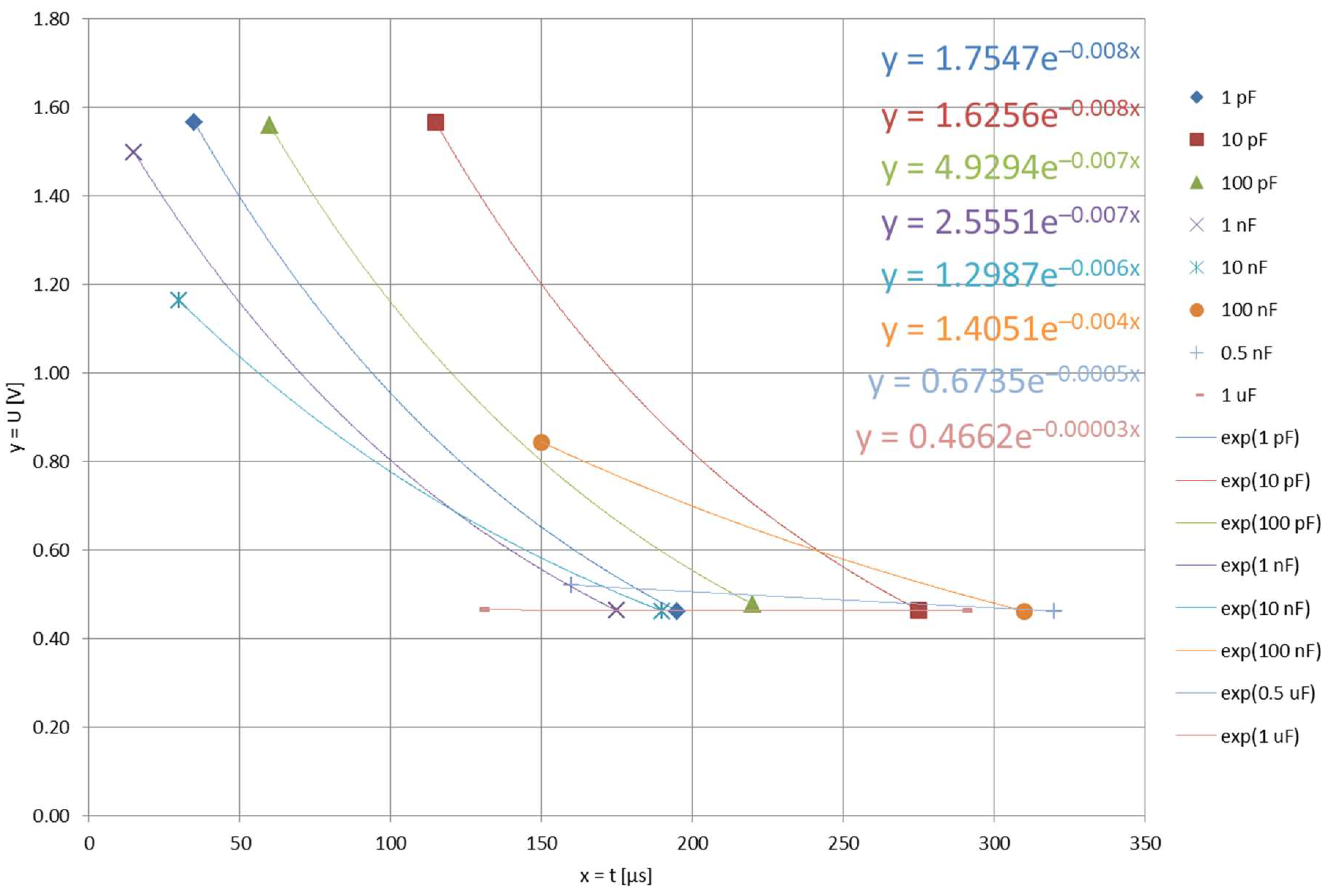
4.3. Differentiating Circuit
4.4. Approach for The Electrical Permittivity
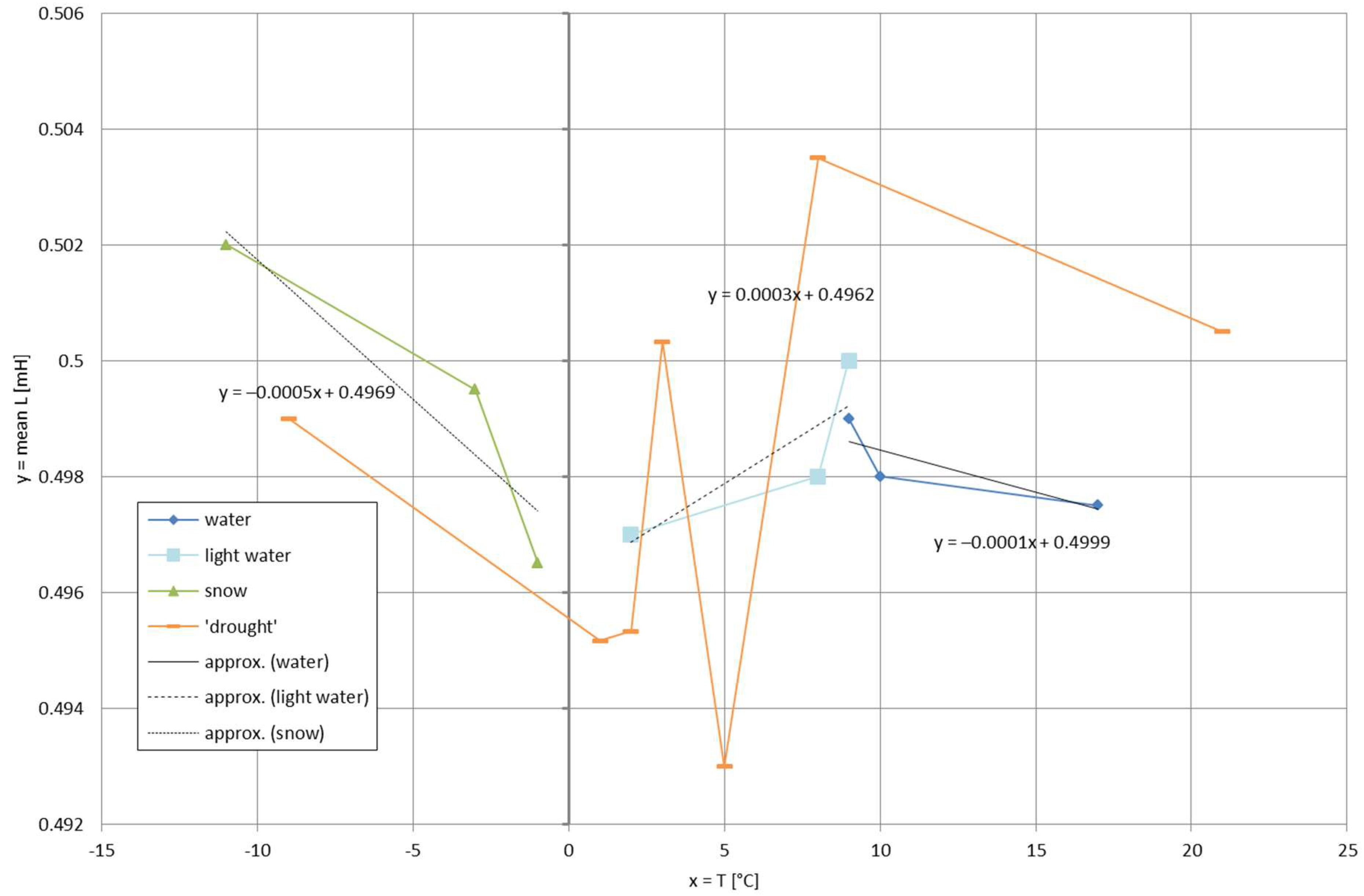
5. Results
- •
- A snow region, with a recorded intense presence of snow and temperatures lower than 1 °C;
- •
- A water region, with recorded intense rain precipitation for temperatures above 1 °C;
- •
- A light water region, with recorded low rain precipitation for temperatures above 1 °C.
- •
- The snow region: −5 × 10−4 T + 0.4969;
- •
- The light water region: 3 × 10−4 T + 0.4962;
- •
- The water region: −10−4 T + 0.4999.
- •
- The snow region: −2 × 10−4 T + 0.0144;
- •
- The light water region: 4 × 10−4x + 0.0315;
- •
- The water region: 10−5x + 0.0286.
6. Discussion
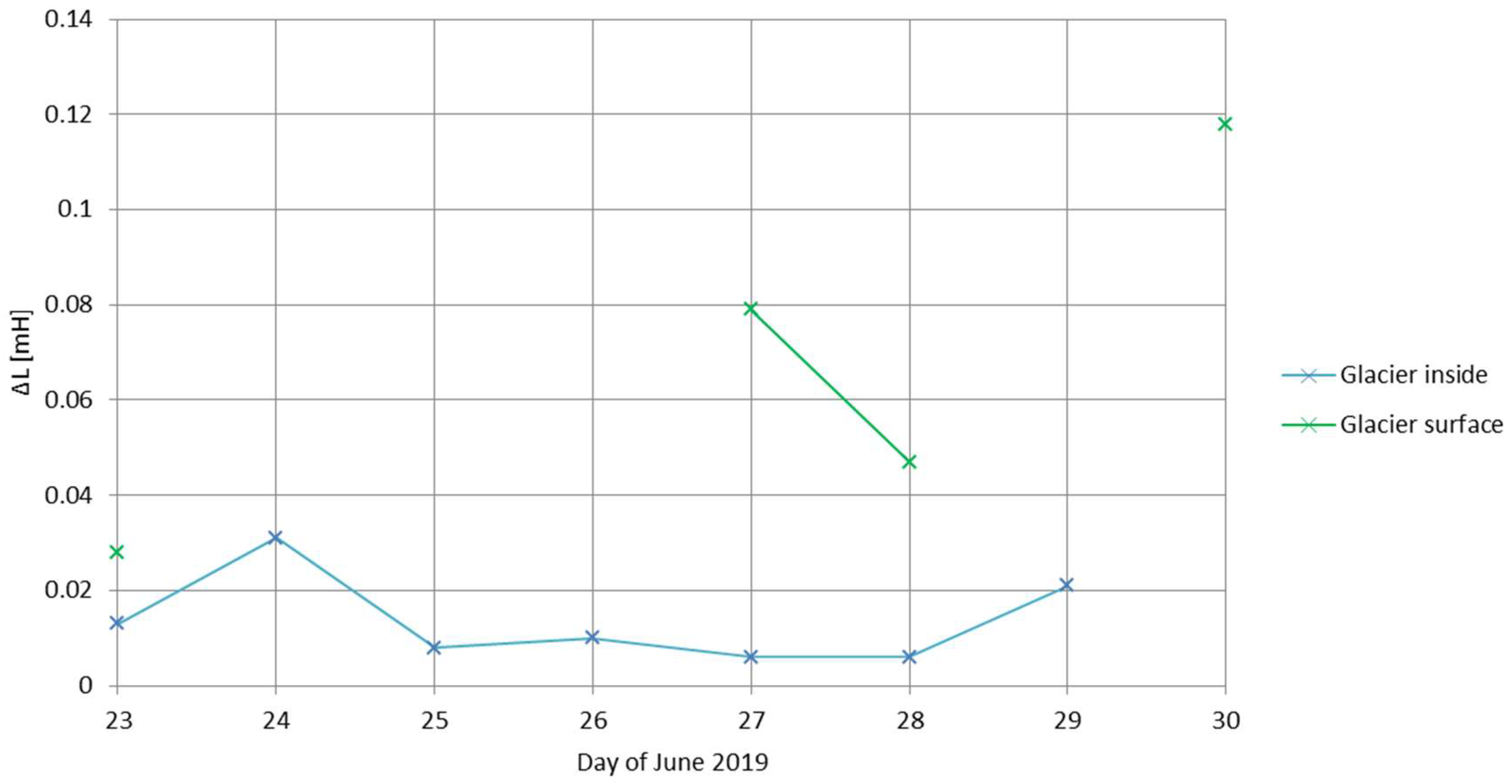
6.1. Comparison to IGLUNA 2019 Glacier Antennas
6.2. Comparison to Homer Tunnel Antenna Experiments (1993)
7. Conclusions
- •
- For the given antenna dimensions and probing frequency, the method of measurement by the digital meter indicated that the variability of the measured inductance can be linked to the changes in the antenna’s parasitic capacitance, and therefore to the electrical permittivity of the surrounding medium;
- •
- An increase in the environment’s humidity caused the convergence of inductance measurements to a certain value in long-time measurements;
- •
- An increase in the environment’s humidity caused an increase in the range of the measured inductance;
- •
- The parameters of the ground, when investigated separately, can be directly linked to the capacitance changes they caused and, therefore, to the changes in the measured inductance;
- •
- The defined functions of changes of measured antenna parameters for the encountered environmental factors can be considered as boundary conditions, between which other (for example, less humid or vaporised) cases are to be allocated;
- •
- Developing a precise definition of the influence of electrical permittivity on the measured antenna parameters requires precise knowledge on the parameters of the measuring circuitry;
- •
- The dependencies described in this paper are consistent with previous experiments in different locations and setups.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bosch, F.; Gurk, M. Comparison of RF-EM, RMT and SP measurements on a karstic terrain in the Jura mountains (Switzerland). In Proceedings of the Seminar ‘Electromagnetische Tiefenforschung’, Altenberg, Germany, 20–24 March 2000; Deutsche Geophysikalische Gesselschaft: Hamburg, Germany, 2000. [Google Scholar]
- Groundwater Geophysics: A Tool for Hydrogeology, 2th ed.; Kirsch, R. (Ed.) Springer-Verlag: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Bem, D.J. Auxiliary Materials for Propagation Calculations; Wrocław University of Technology: Wrocław, Poland, 1974. [Google Scholar]
- Bem, D.J. Antenna Array in the Radio Broadcasting Centre Solec Kujawski (Polish Radio, JSC). Przegląd Telekomun. Wiadomości Telekomun. 2000, 8–9, 577–585. [Google Scholar]
- Fenwick, R.C.; Weeks, W.L. Submerged antenna characteristics. IEEE Trans. Antennas Propag. 1963, 11, 296–305. [Google Scholar] [CrossRef]
- Miś, T.A.; Modelski, J. Stratospheric VLF Vertical Electric Mono- and Dipole Antenna Tests in 2014–2015. In Proceedings of the 2018 Baltic URSI Symposium, Poznań, Poland, 15–17 May 2018; Volume I. [Google Scholar] [CrossRef]
- Miś, T.A. The concept of an airborne VLF transmitter with vertical electric dipole antenna. In Proceedings of the IEEE International Symposium on Antennas and Propagation and USNC-URSI Radio Science Meeting, Boston, MA, USA, 8–13 July 2018. [Google Scholar] [CrossRef]
- Wilson, D. Channel Characterization and System Design for Sub-Surface Communications. Ph.D. Dissertation, The University of Leeds, School of Electronic and Electrical Engineering, Leeds, UK, February 2003. [Google Scholar]
- Burrows, M.L. ELF Communications Antennas; Peter Peregrinus Ltd.: London, UK, 1978. [Google Scholar]
- Morgan, M.G. An island as a natural very-low-frequency transmitting antenna. IRE Trans. Antennas Propag. 1960, 8, 528–530. [Google Scholar] [CrossRef]
- Pickworth, G. VLF Earth loop Antennas. Part I. Electron. Today Int. 1991, 1, 30–32. [Google Scholar]
- Uzunoglu, N.K.; Kouridakis, S.J. Radiation of Very Low and Extremely Low Frequencies (VLF & ELF) by a natural antenna based on an island or a peninsula structure. Radio Sci. Bull. 2004, 2004, 7–12. [Google Scholar]
- Turczyński, Z.; Łakomy, W. Personal safety indicator for the detection of pre-fainting stage of the miners and others. Wiadomości Górnicze 1987, 1, 15–21. (In Polish) [Google Scholar]
- Barr, R.; Ireland, W. Low-frequency input impedance of a very large loop antenna with a mountain core. IEE Proc. 1993, 140, 84–90. [Google Scholar] [CrossRef]
- Miś, T.A.; Modelski, J. The Analysis of Experimental Deployment of IGLUNA 2019 Trans-Ice Longwave System. Remote Sens. 2020, 12, 4045. [Google Scholar] [CrossRef]
- Republic of Poland's Office of Electronic Communications. Document DC.WRT.5104.6.2020.6; Republic of Poland's Office of Electronic Communications: Warszawa, Poland, 21 July 2021. [Google Scholar]
- Miś, T.A. The design, development and demonstration of longwave communication system for lunar surface operations. In Proceedings of the SECESA Conference on Space System Engineering, Delft, The Netherlands, 30 September–2 October 2020; European Space Agency: Noordwijk, The Netherlands, 2020. [Google Scholar]
- Miś, T.A. The results of IGLUNA 2019 trans-ice longwave communication system tests. In Proceedings of the MIKON Conference, Warszawa, Poland, 5–8 October 2020. [Google Scholar]
- Miś, T.A. The performance analysis and optimization of IGLUNA 2019 lunar-analogue longwave transmitting system. In Proceedings of the Baltic URSI Conference, Warszawa, Poland, 5–8 October 2020. [Google Scholar]
- Digital Multimeter AXIOMET AX-588B. In User Manual; Transfer Multisort Electronic: Atlanta, GA, USA, 2018.
- Titow, W. PVC Technology; Elsevier Applied Science Publishers: London, UK, 1984. [Google Scholar]
- Kaczyński, N. Soil; PIWR: Warszawa, Poland, 1950. (In Polish) [Google Scholar]
- Wieczysty, A. Engineering Hydrogeology; PWN: Warszawa-Kraków, Poland, 1970. (In Polish) [Google Scholar]
- Falkowski, M.; Nowak, M.; Prończuk, J.; Ralski, E. Meadowing. Vol II: Meadow Management; PWRiL: Warszawa, Poland, 1970. (In Polish) [Google Scholar]
- Badru, R.A.; Adeniran, S.A.; Atijosan, A.O. Effect of magnetic field on charged water vapour in motion. Eur. J. Eng. Technol. 2015, 3. [Google Scholar]
- Rusiniak, L. Electric properties of water. New experimental data in the 5 Hz—13 MHz frequency range. Acta Geophys. Pol. 2004, 52, 63–79. [Google Scholar]
- Janeczek, A. Simple induction meter. Electron. All 2003, 50–51. (In Polish) [Google Scholar]
- Poisel, R.A. Antenna Systems and Electronic Warfare Applications; Artech House: Norwood, MA, USA, 2012. [Google Scholar]
- Bogdanov, A.F.; Vasin, V.V.; Dulin, W.N.; Ilin, V.A.; Krivitskiy, V.H.; Kuznietsov, V.A.; Labutin, V.K.; Molochkov Yu, B.; Piertsov, S.V.; Stiepanov, B.M.; et al. Radioelectronics—A Handbook. Volume 1; Kulikovski, A.A., Ed.; WKiŁ: Warsaw, Poland, 1971. (In Polish) [Google Scholar]
- Jeffery, J.E. Impedance control of conductors acting as transmission lines in printed boards for high-frequency digital applications. Circuit World 1997, 23. [Google Scholar] [CrossRef]
- Investigation of a Transmission Line; Częstochowa University of Technology, Division of Electrotechnology, WZET Laboratory: Częstochowa, Poland, 2019. (In Polish)
- Electron Tube Handbook. Vol. II; Brown, Boveri & Co., Ltd.: Baden, Switzerland, 1971.
- Usowicz, B. Statistical-physical models of mass and energy flow in porous environments. Acta Agroph. 2000, 29, 3–113. (In Polish) [Google Scholar]
- Recommendation ITU-R P.527–4. In Electrical Characteristics of the Surface of the Earth; ITU: Geneva, Switzerland, 2017.
- Electrical Permittivity Characterization of Aqueous Solutions. Available online: https://chem.libretexts.org/Bookshelves/Analytical_Chemistry (accessed on 1 February 2021).
- Fraser-Smith, A.C.; Bannister, P.R. Reception of ELF signals at antipodal distances. Interaction of electromagnetic fields of ELF controlled sources with the ionosphere and Earth's crust. Proc. All-Russ. Res. Pract. Workshop 2014, 1, 144–149. [Google Scholar]
- Gierlotka, S. Geomagnetic properties of rocks. Propuls. Steer. 2019, 1, 40–43. (In Polish) [Google Scholar]
- Finkyelshteyn, M.I.; Mendyelson, W.L.; Kutyev, W.A. Radiolocation of Layered Ground Covers; Sovetskoye Radio: Moskva, Russia, 1977. (In Russian) [Google Scholar]
- Guide on the measurements of conductivity. In Theory and Practice Of Measurement Execution; Mettler-Toledo Sp.z.o.o: Warszawa, Poland, 2013. (In Polish)




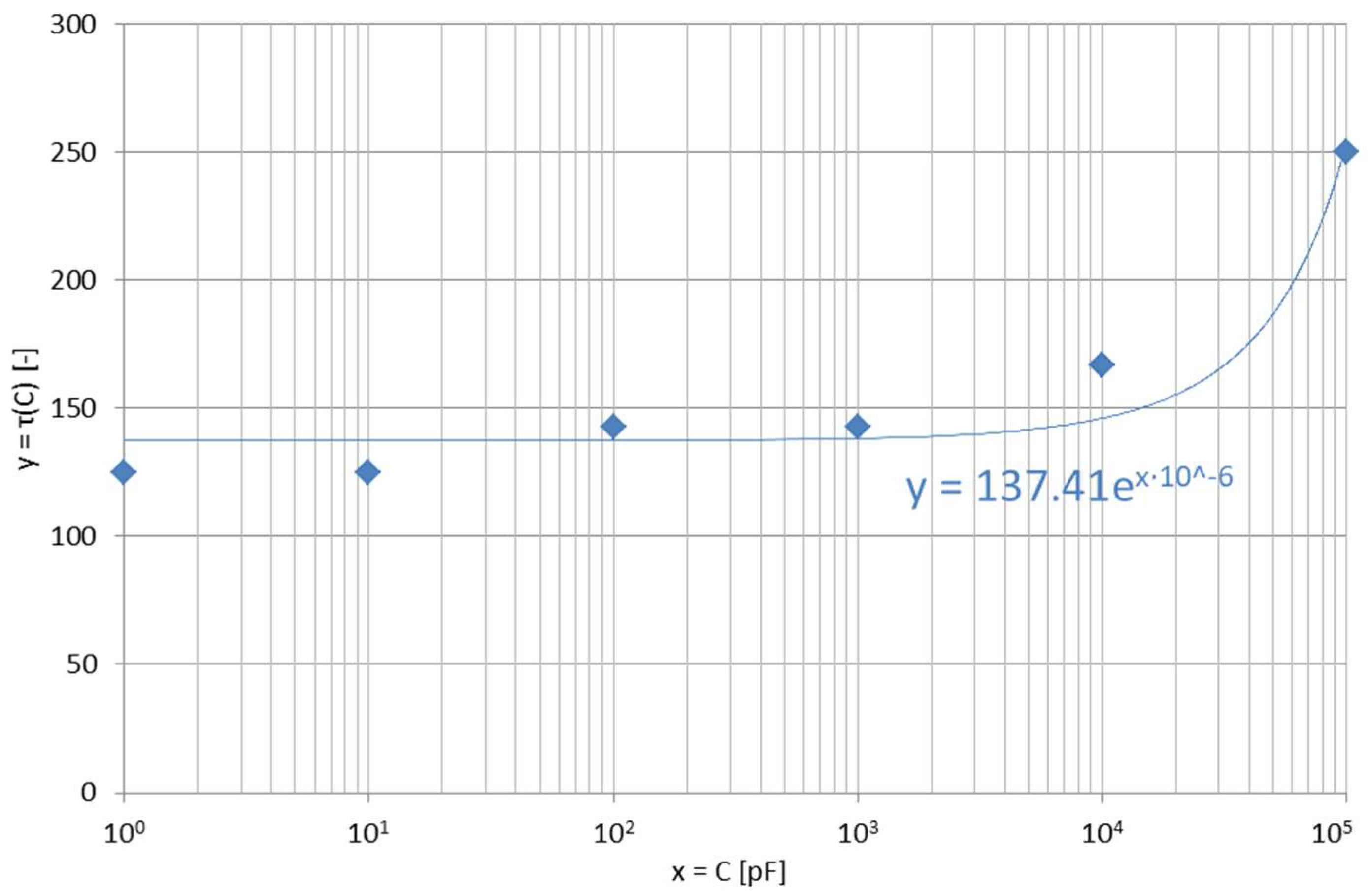
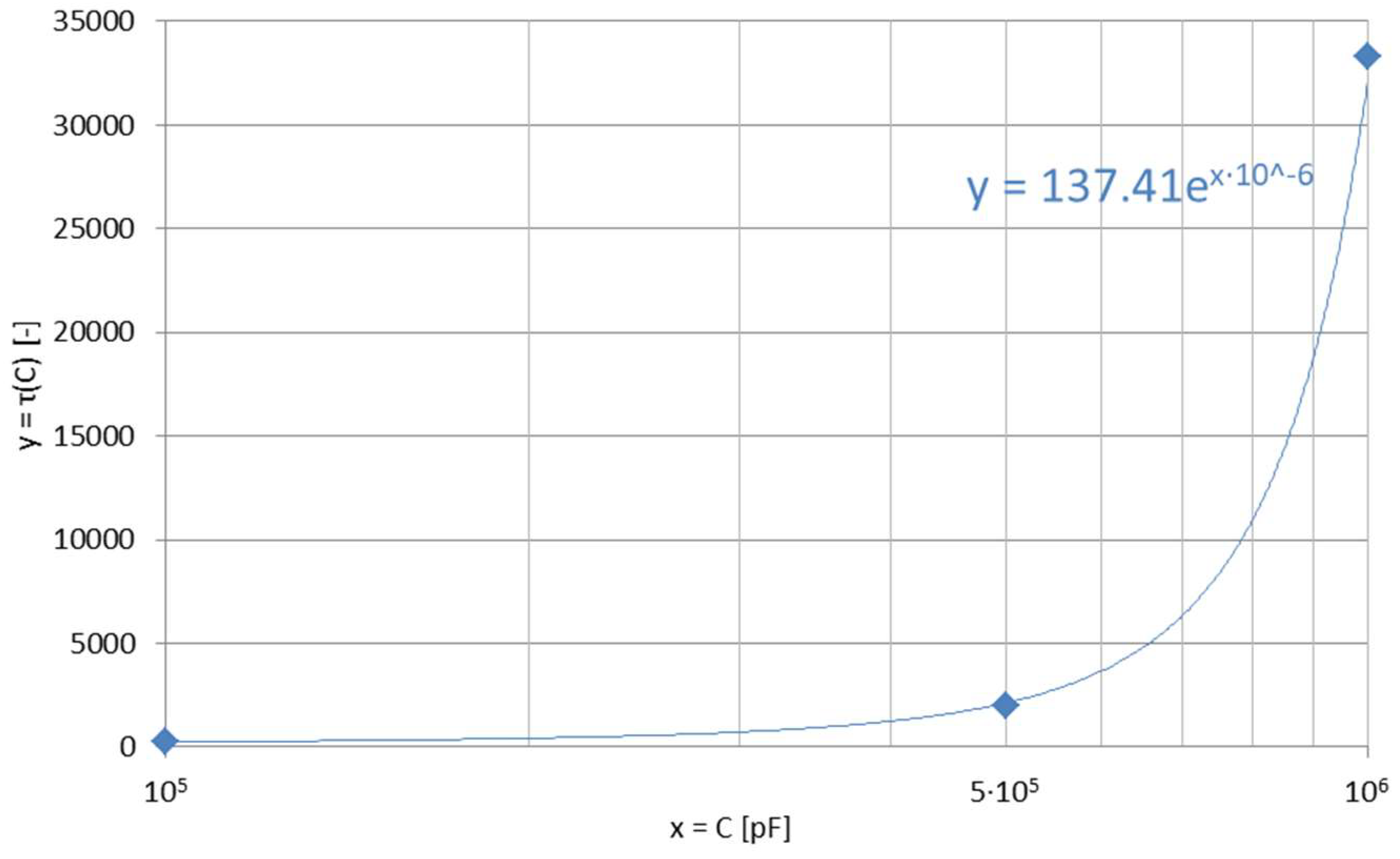
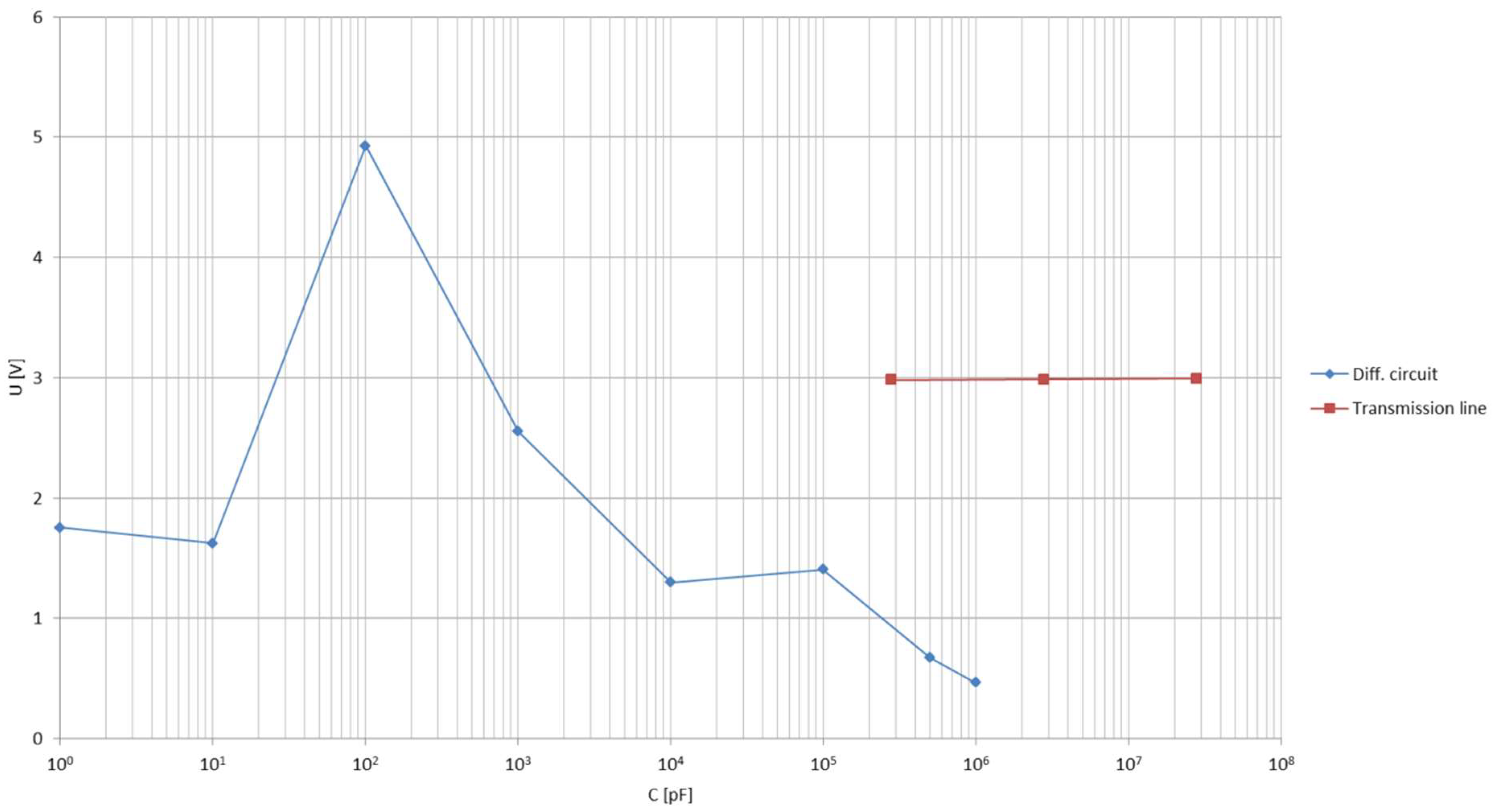
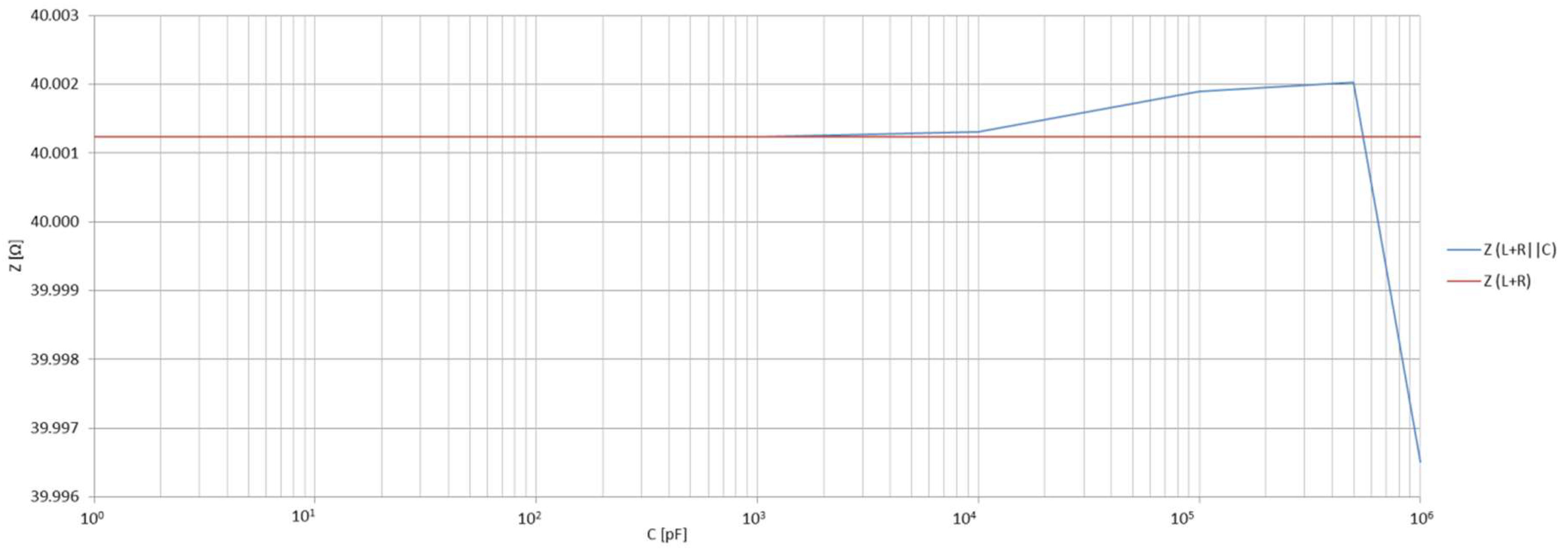
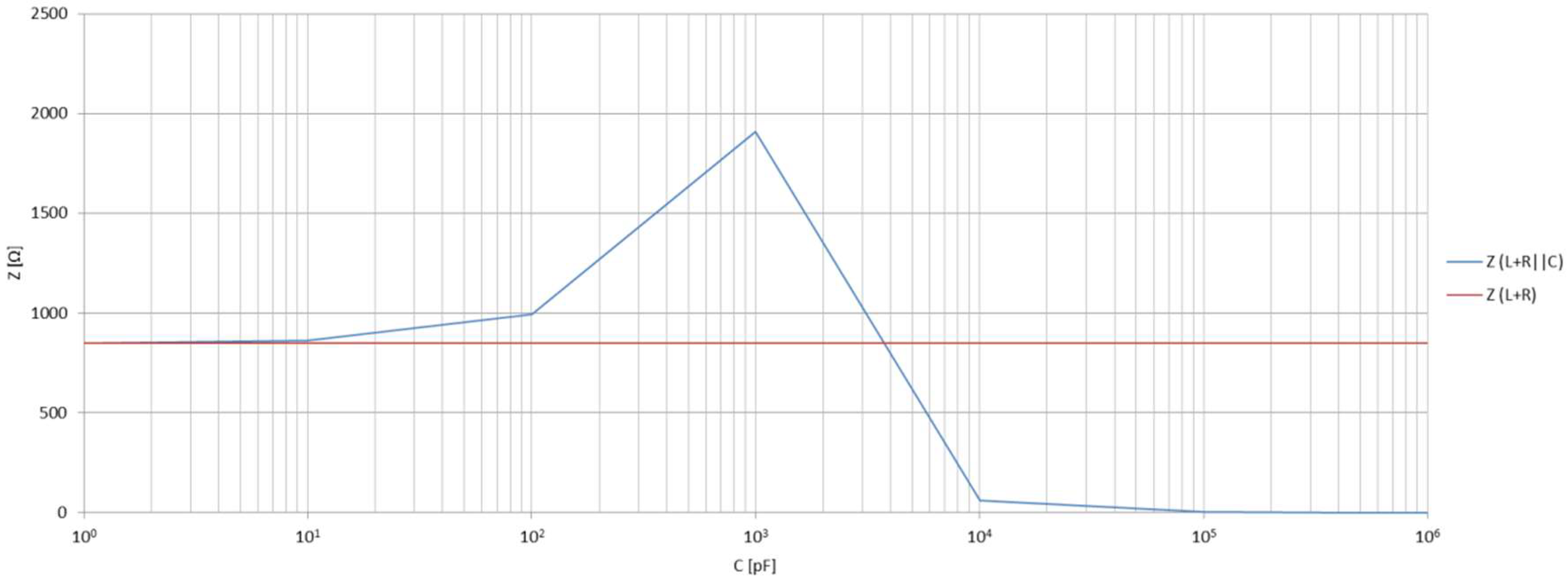
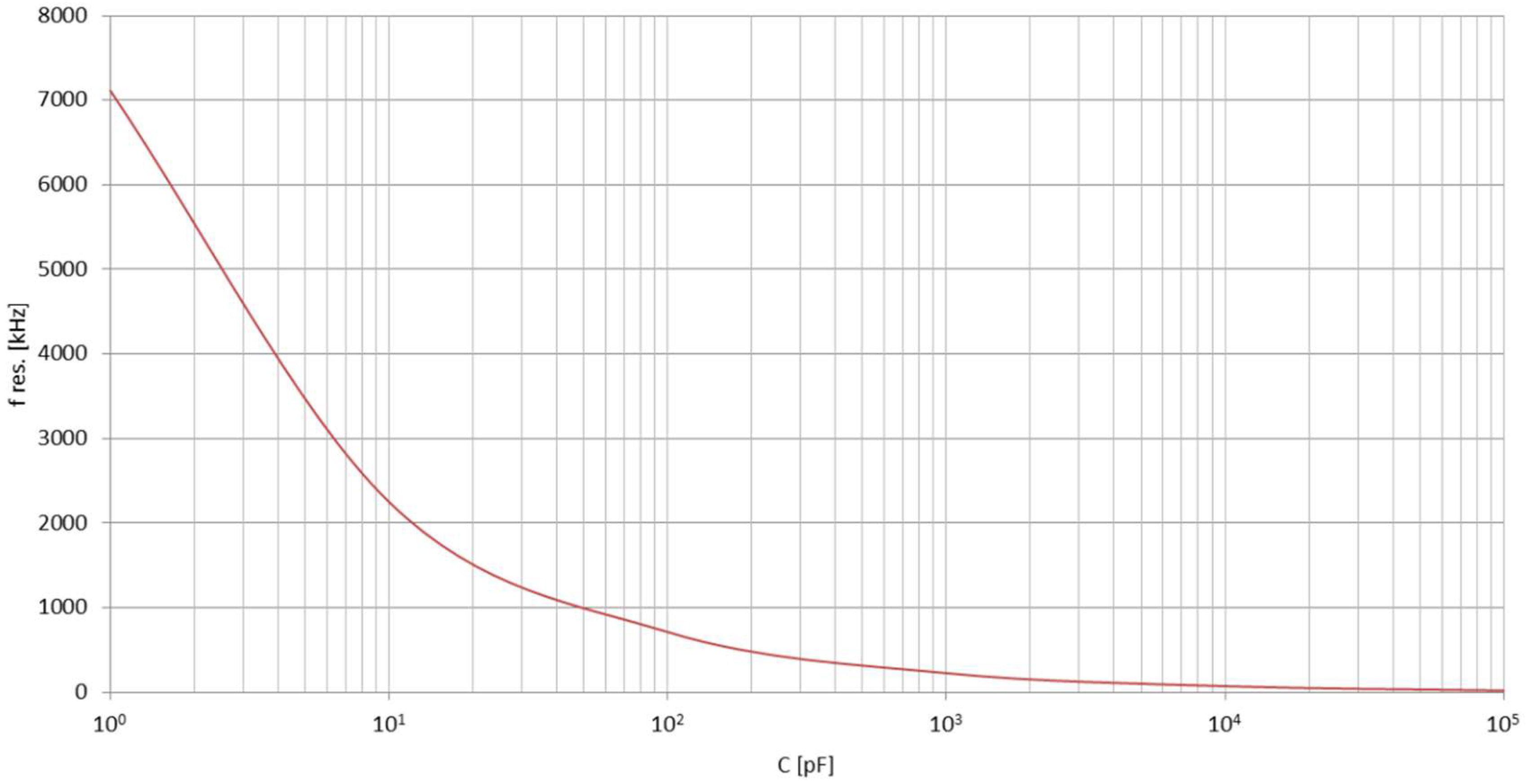
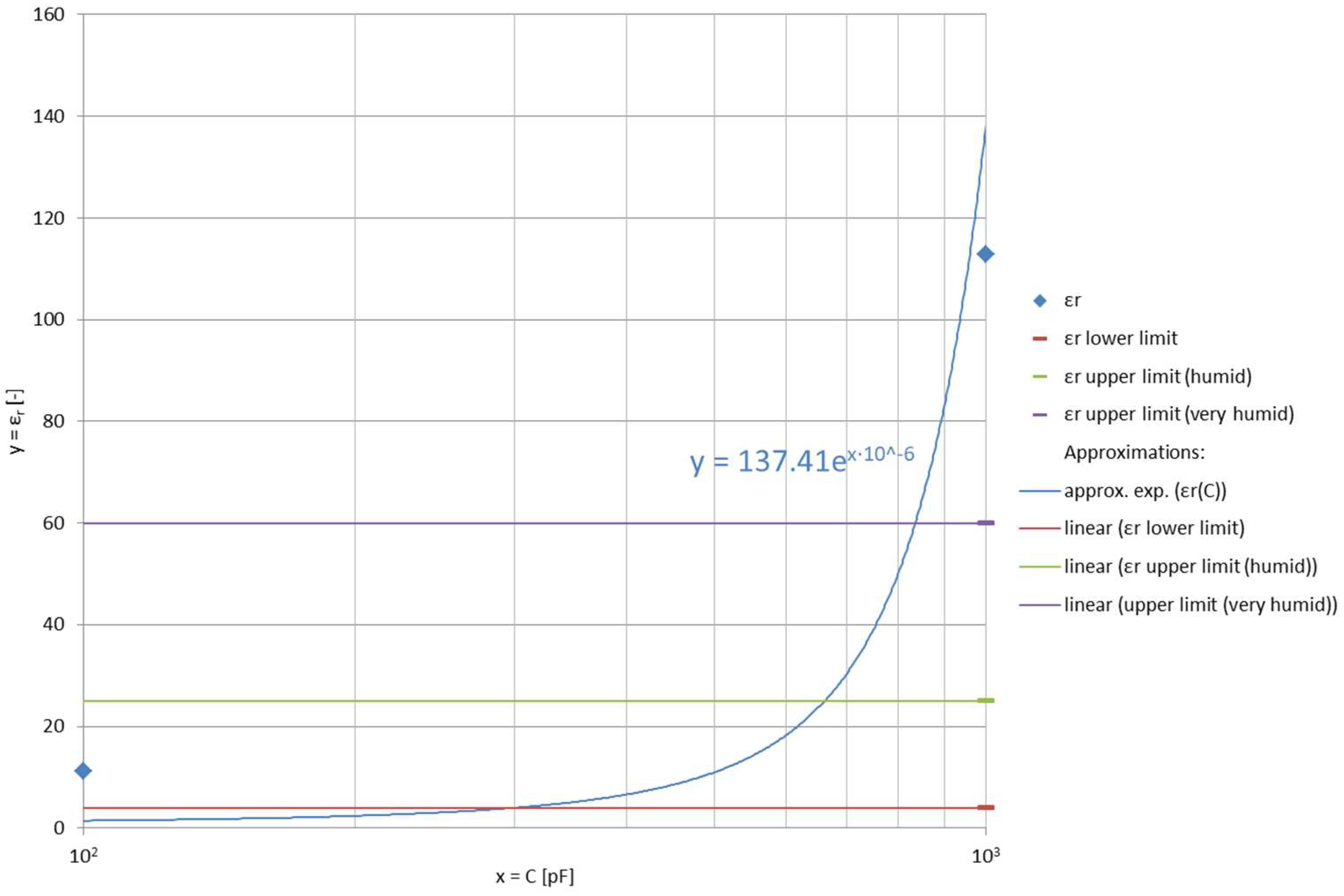
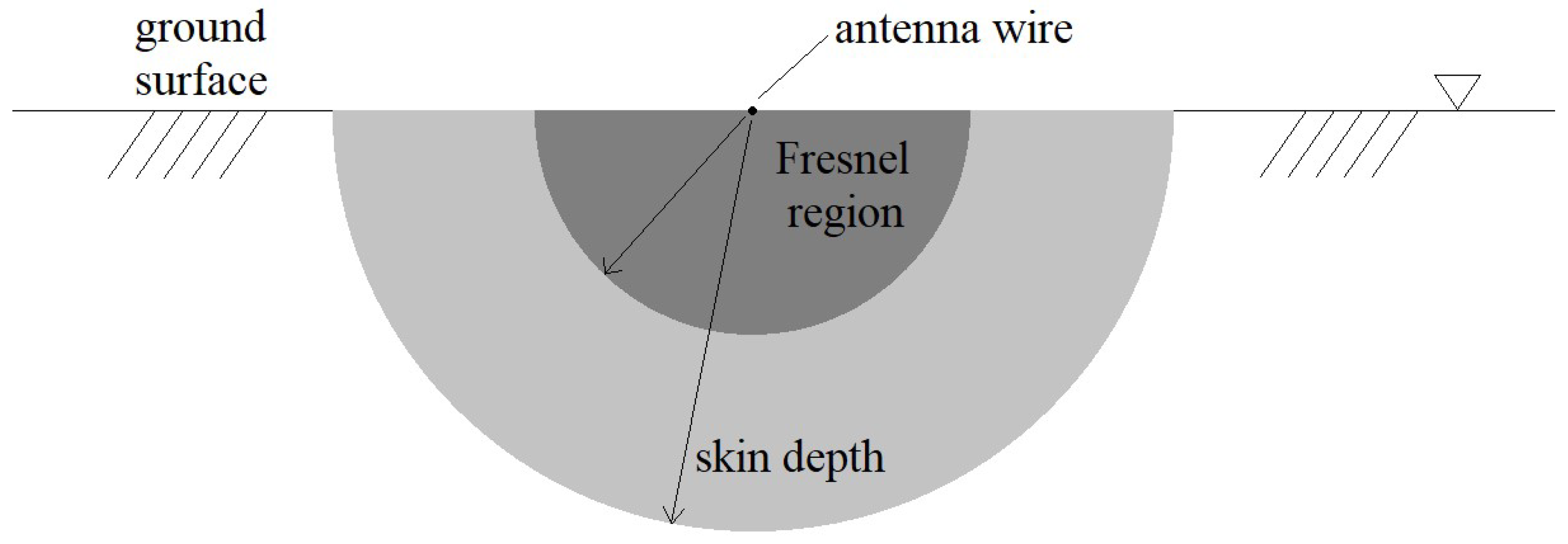


| Condition | f [kHz] | X [Ω] | L [H] |
|---|---|---|---|
| Heavy rain during measurements | 19.42 | 1580 | 0.01294875 |
| Rain day before measurements | 21.36 | 2252 | 0.01677982 |
| No rain for 2 weeks before measurements | 21.36 | 2910 | 0.02168263 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Miś, T.A.; Modelski, J. Environmental Sensitivity of Large Stealth Longwave Antenna Systems. Remote Sens. 2021, 13, 3946. https://doi.org/10.3390/rs13193946
Miś TA, Modelski J. Environmental Sensitivity of Large Stealth Longwave Antenna Systems. Remote Sensing. 2021; 13(19):3946. https://doi.org/10.3390/rs13193946
Chicago/Turabian StyleMiś, Tomasz Aleksander, and Józef Modelski. 2021. "Environmental Sensitivity of Large Stealth Longwave Antenna Systems" Remote Sensing 13, no. 19: 3946. https://doi.org/10.3390/rs13193946
APA StyleMiś, T. A., & Modelski, J. (2021). Environmental Sensitivity of Large Stealth Longwave Antenna Systems. Remote Sensing, 13(19), 3946. https://doi.org/10.3390/rs13193946






