Investigation on Global Distribution of the Atmospheric Trapping Layer by Using Radio Occultation Dataset
Abstract
:1. Introduction
2. Method and Data
2.1. Method Description
- Since the minimum effective height of each atmospheric profile is different, in order to ensure that the selected profile contains the effective information of the boundary layer and a sufficient number of samples, the lowest effective record height of the lower bound of the selected data is within 1 km from the ground.
- Calculate the double-weighted average and standard deviation [26] of all refractivity profiles, and eliminate the profile that is greater than (less than) the average plus (minus) 5 times the standard deviation.
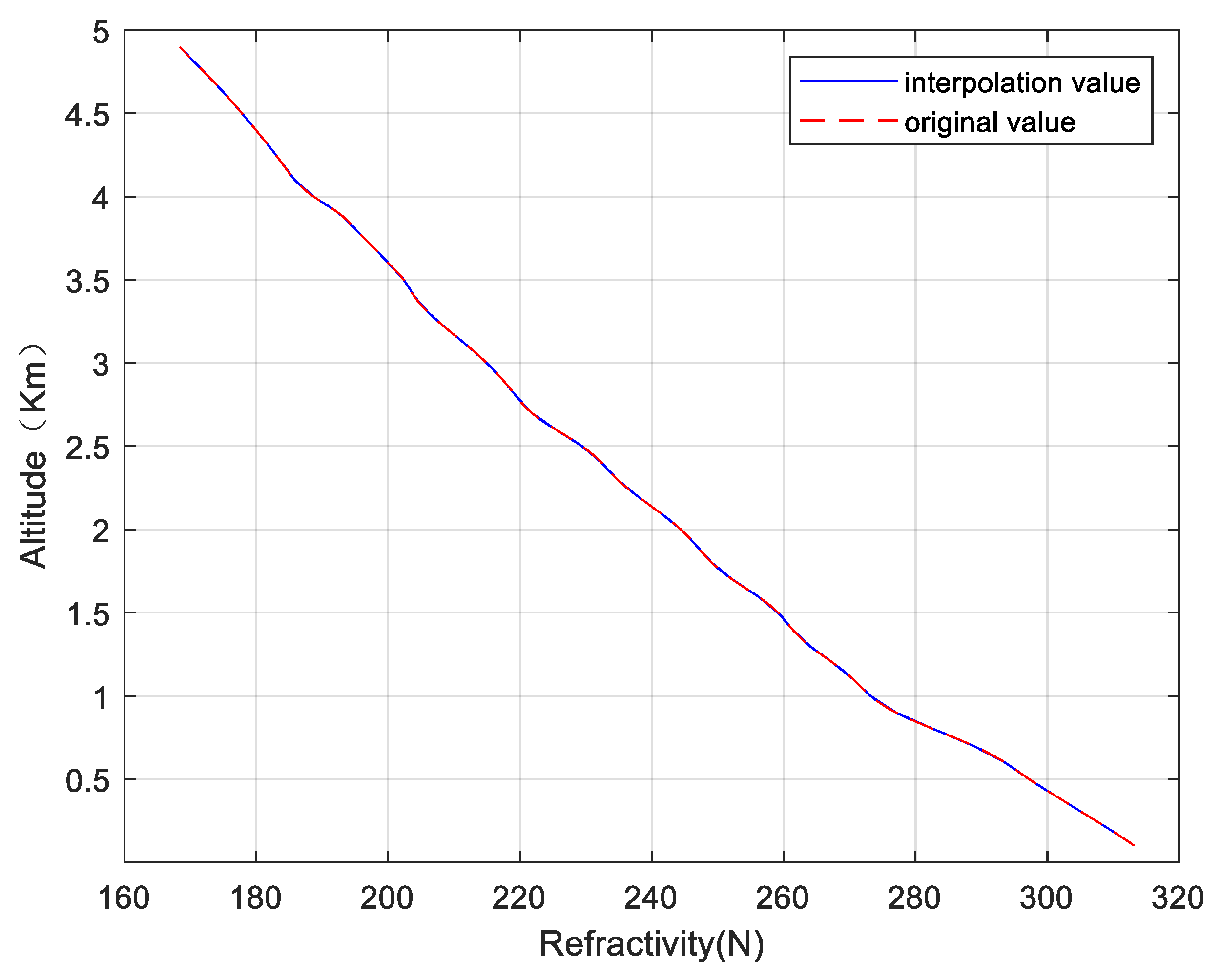
- Using cubic spline interpolation to interpolate the profile to 5 km at the vertical height at intervals of 10 m. The formula of a cubic spline interpolation iswhere Ci = ai + bix + cix2 + dix3 (di ≠ 0), i = 1, …, n [8]. The error analysis of cubic spline interpolation has been presented in Figure 2. The comparative result indicates that the height distribution of refractivity is not affected by cubic spline interpolation. The interpolation results are shown in Figure 2. The red dotted line represents the original data value, and the solid blue line represents the interpolated data values. The results indicate that cubic spline interpolation has no effect on the data. The higher height resolution of refractivity data can observe some of the finer structures of trapping layer, which is helpful for investigating the generation mechanism of trapping layer.
- Using the central difference method to calculate the vertical gradient of the refractivity at each height:where the number of profile layers.
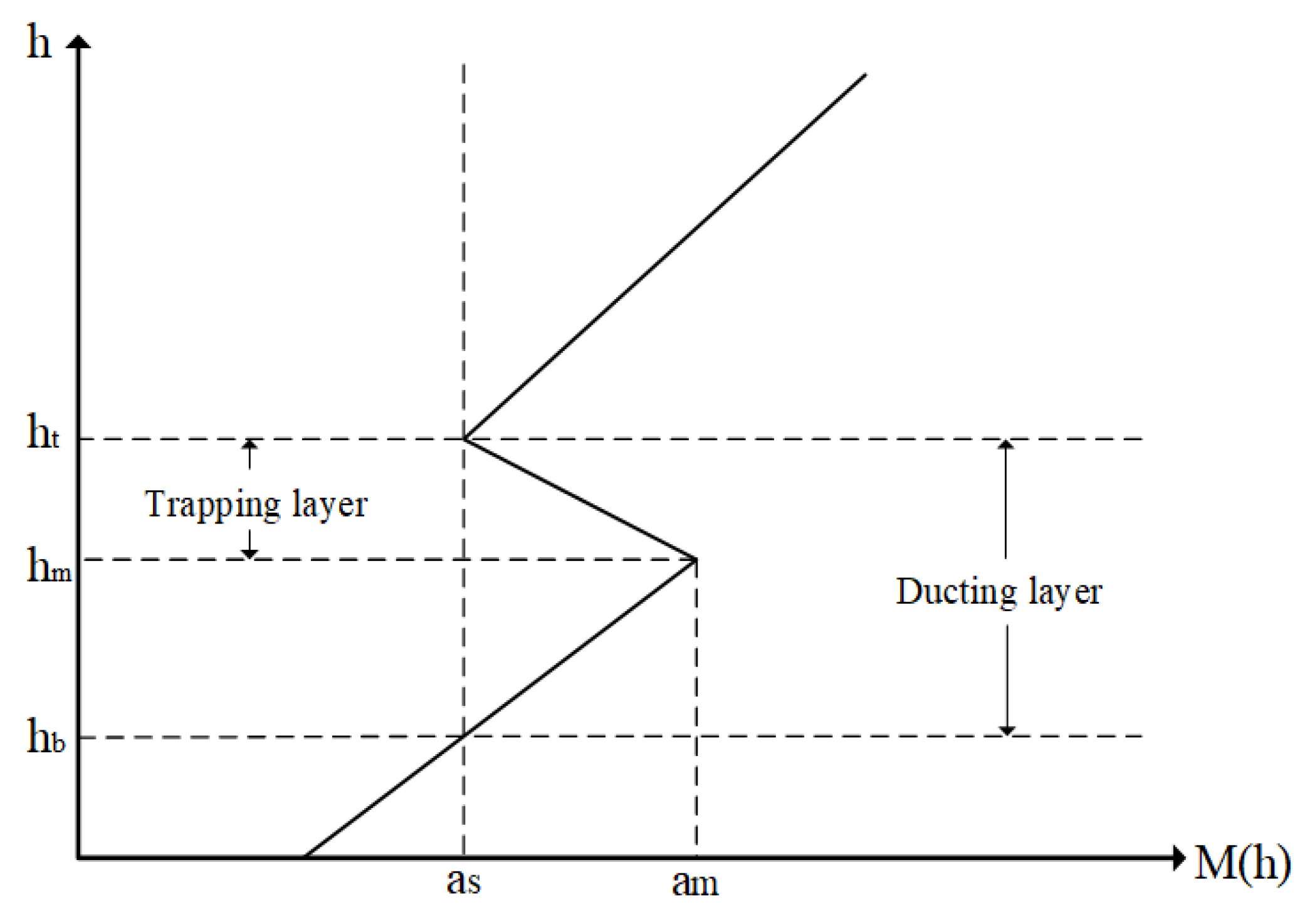
- Checking the value of from the bottom up. When , we find the bottom of the trapping layer . When , we find the top of the trapping layer . Here, only select the first trapping layer retrieved. In Figure 1, the height of the trapping layer is, the thickness of the trapping layer is, and the intensity of the trapping layer is.
2.2. Data Description
3. Statistical Results and Discussion
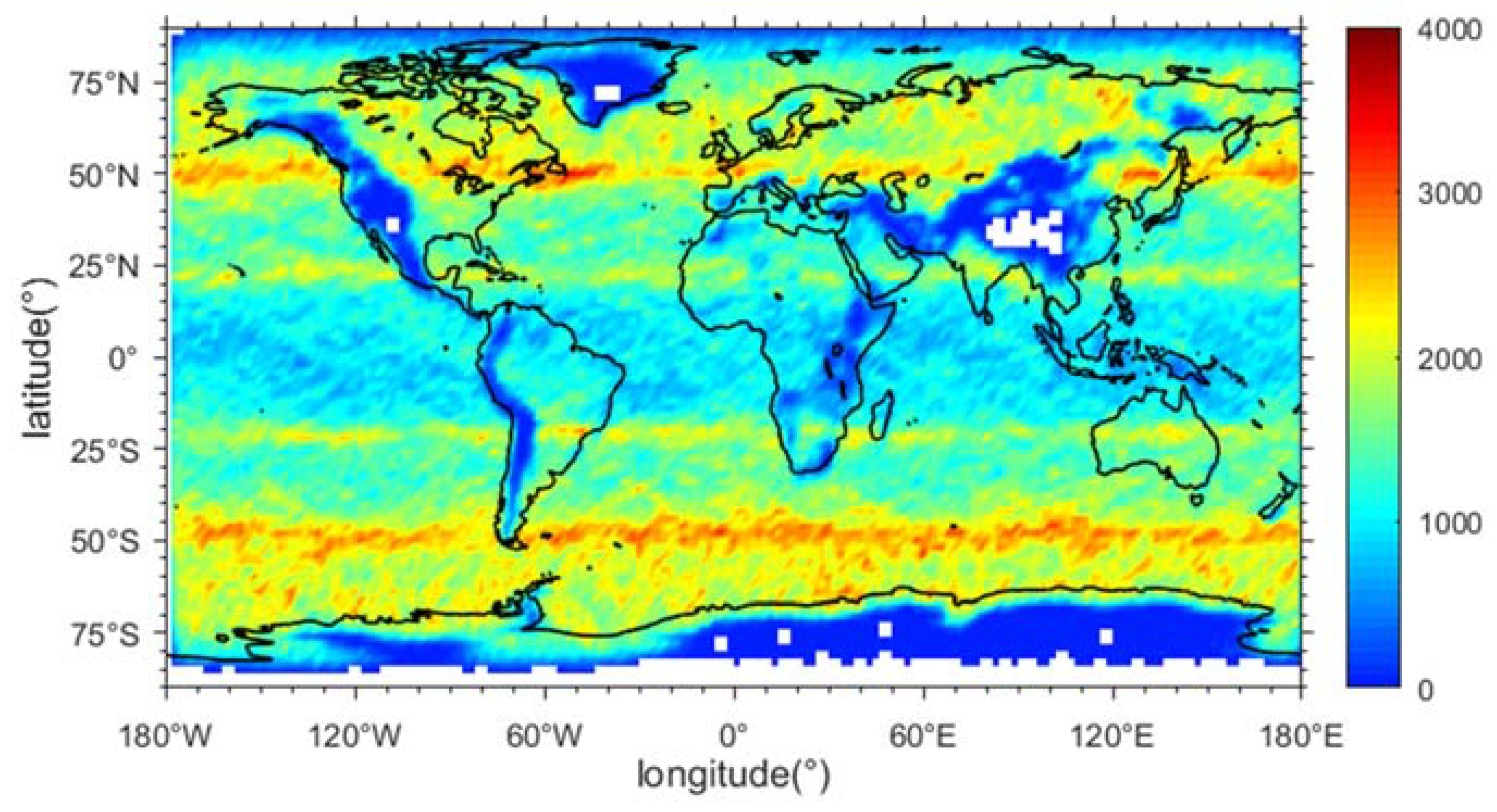
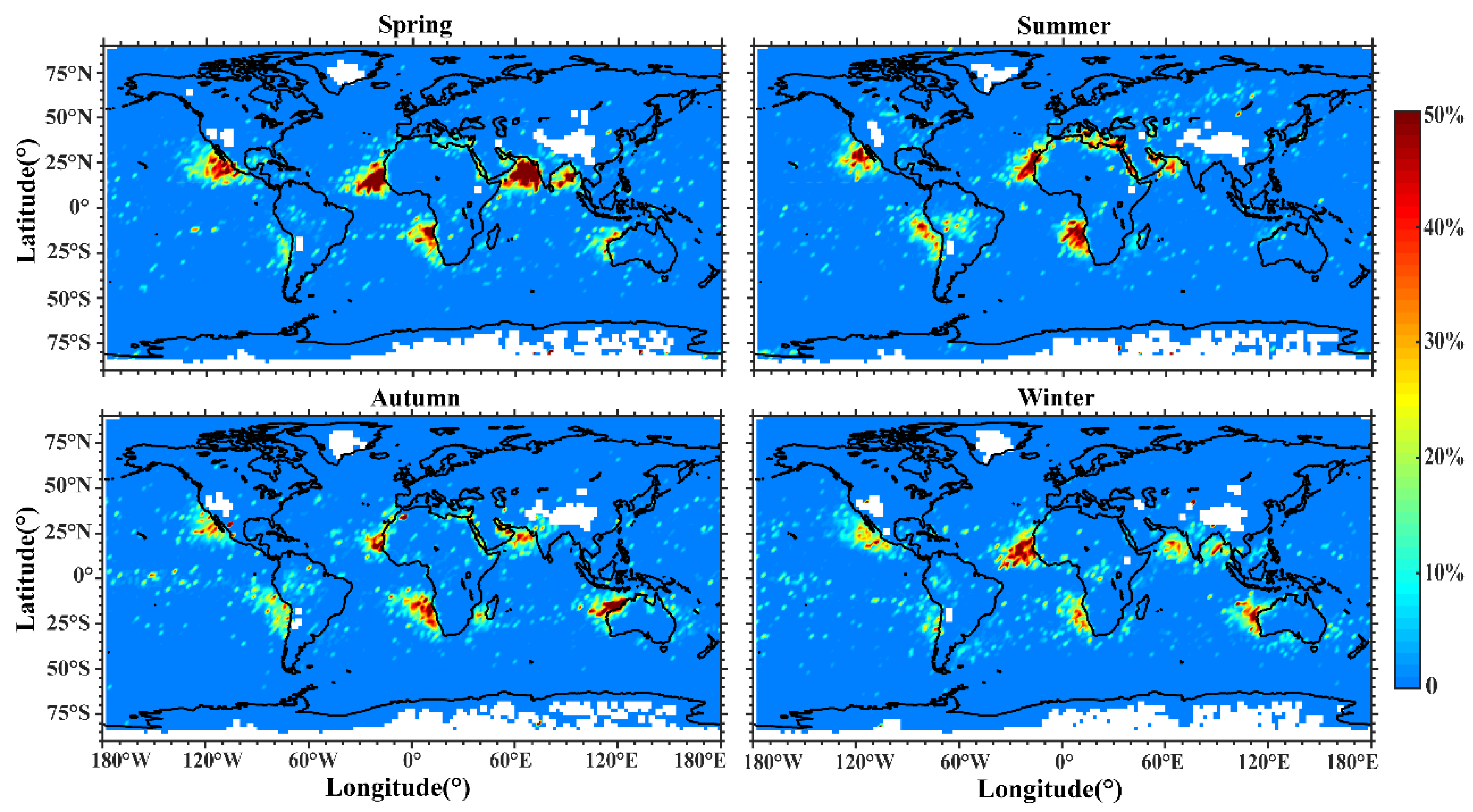
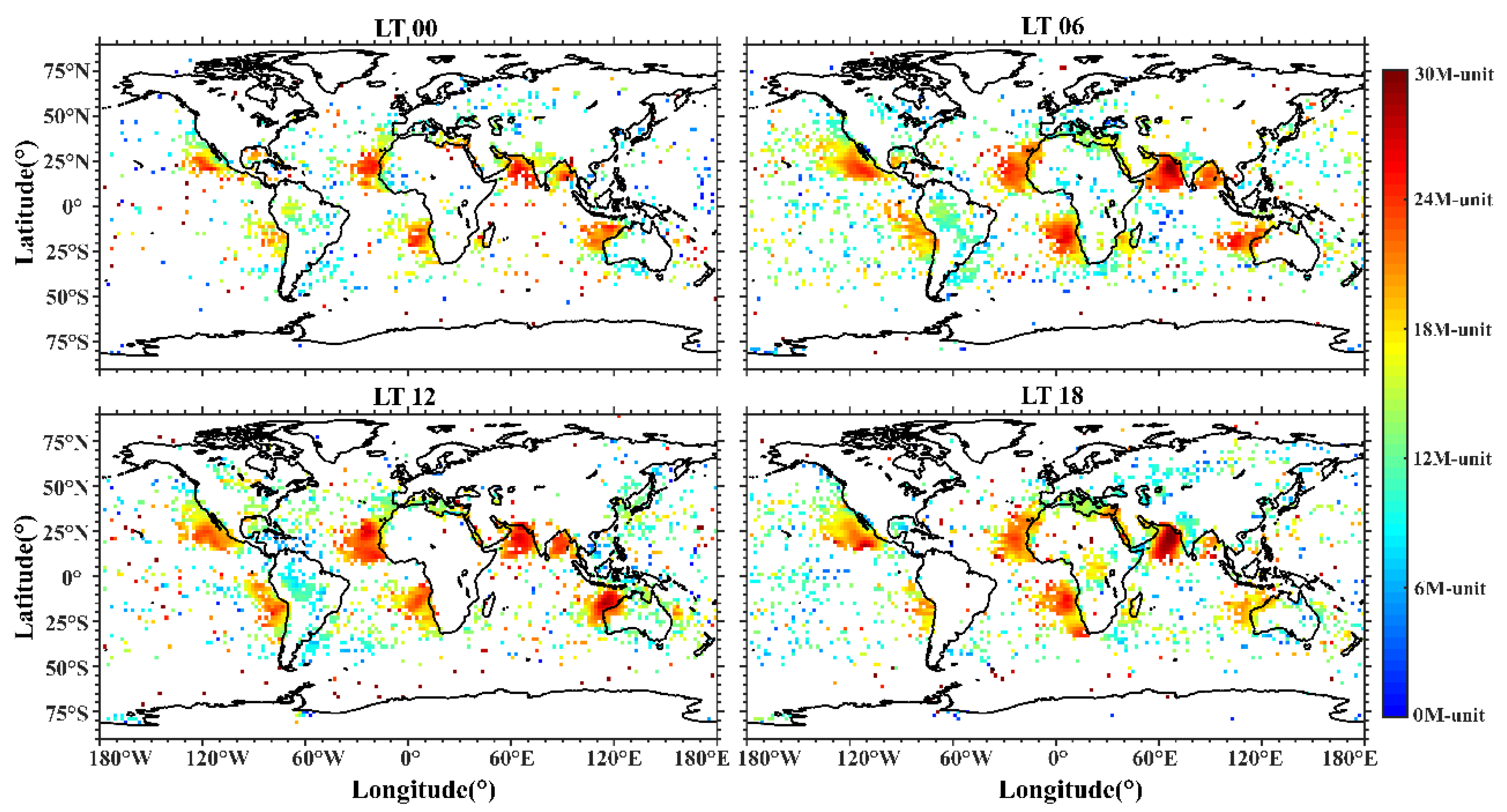
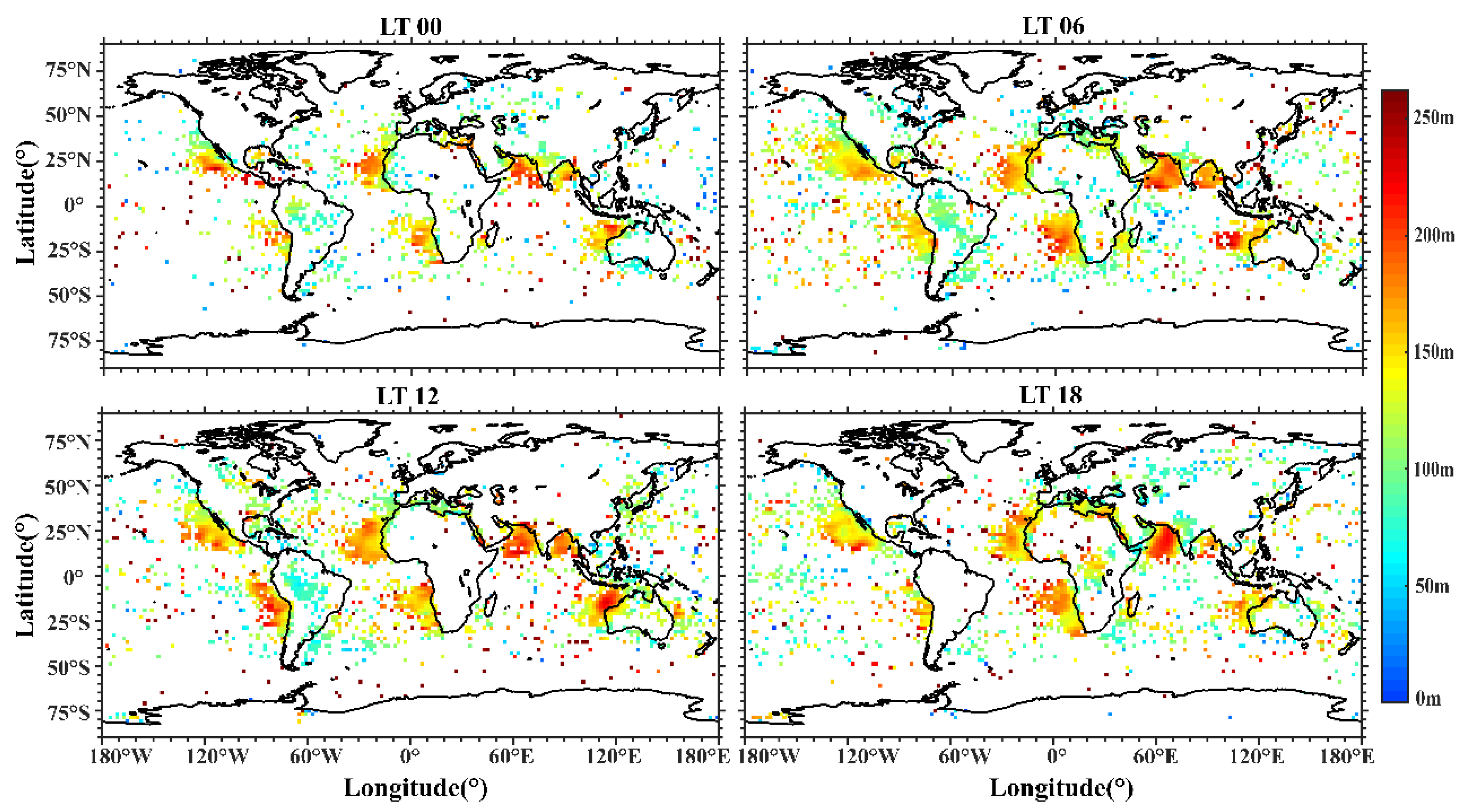
3.1. Probability of Trapping Occurrence
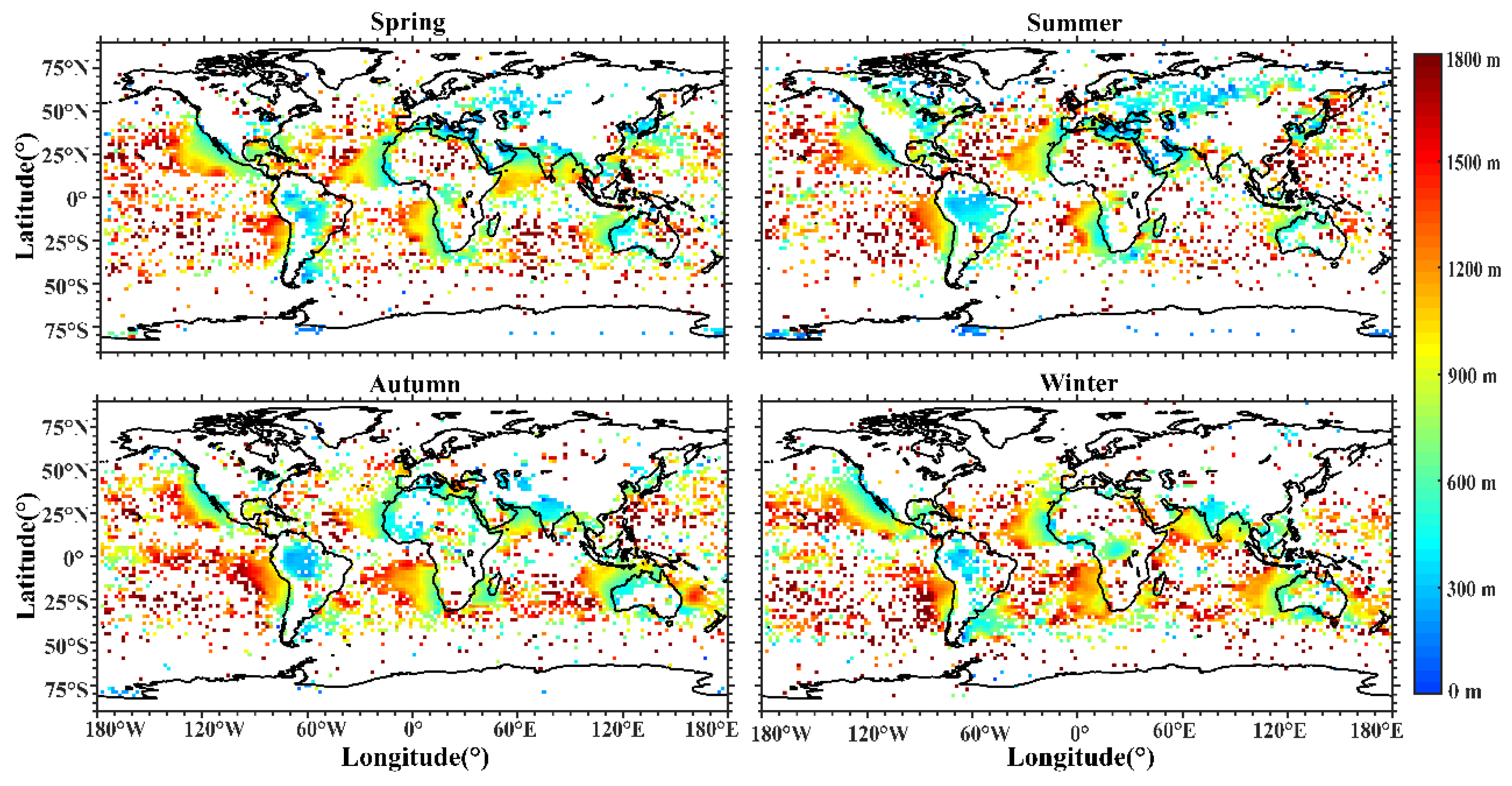
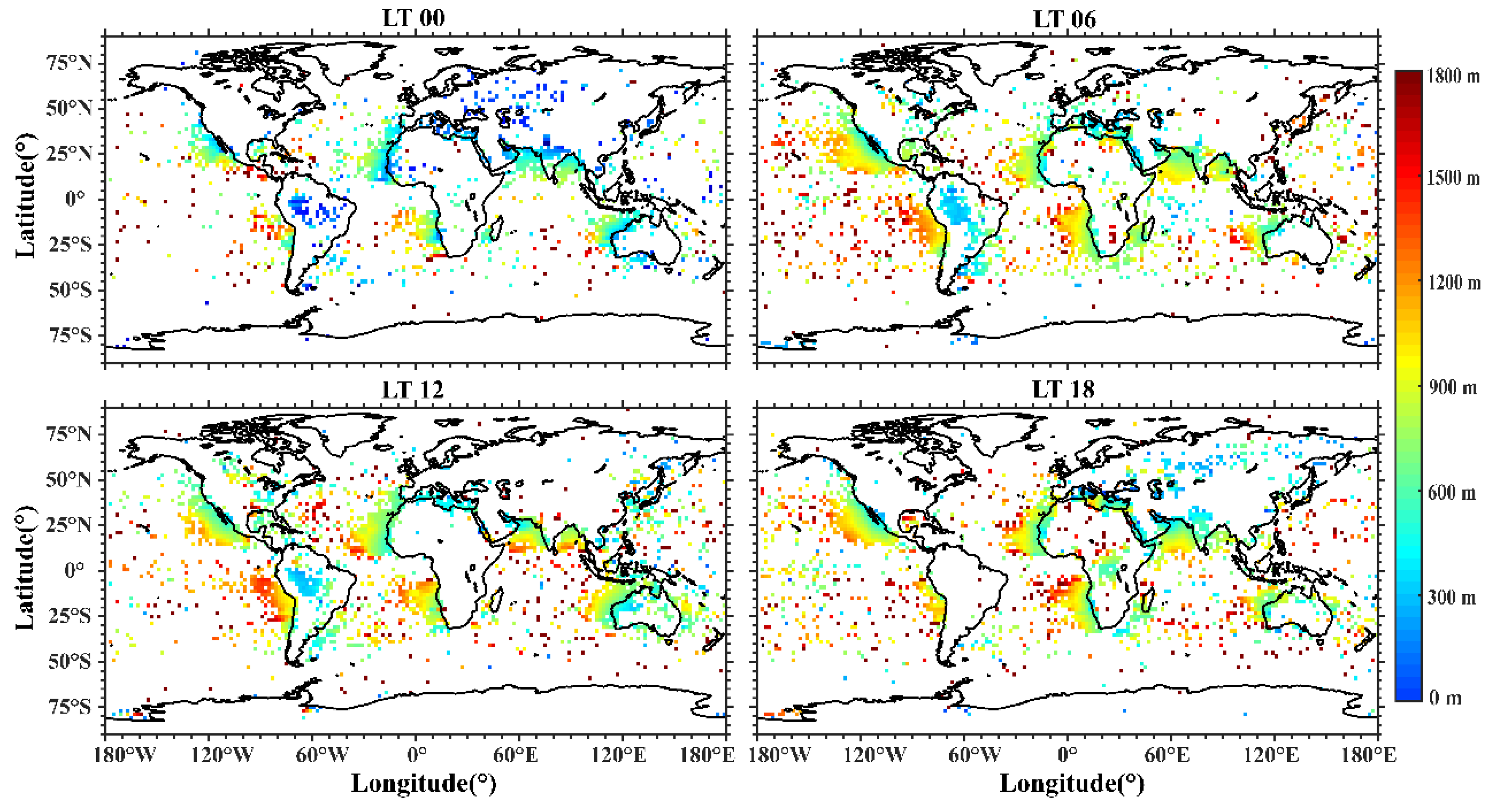
3.2. Altitude of Trapping
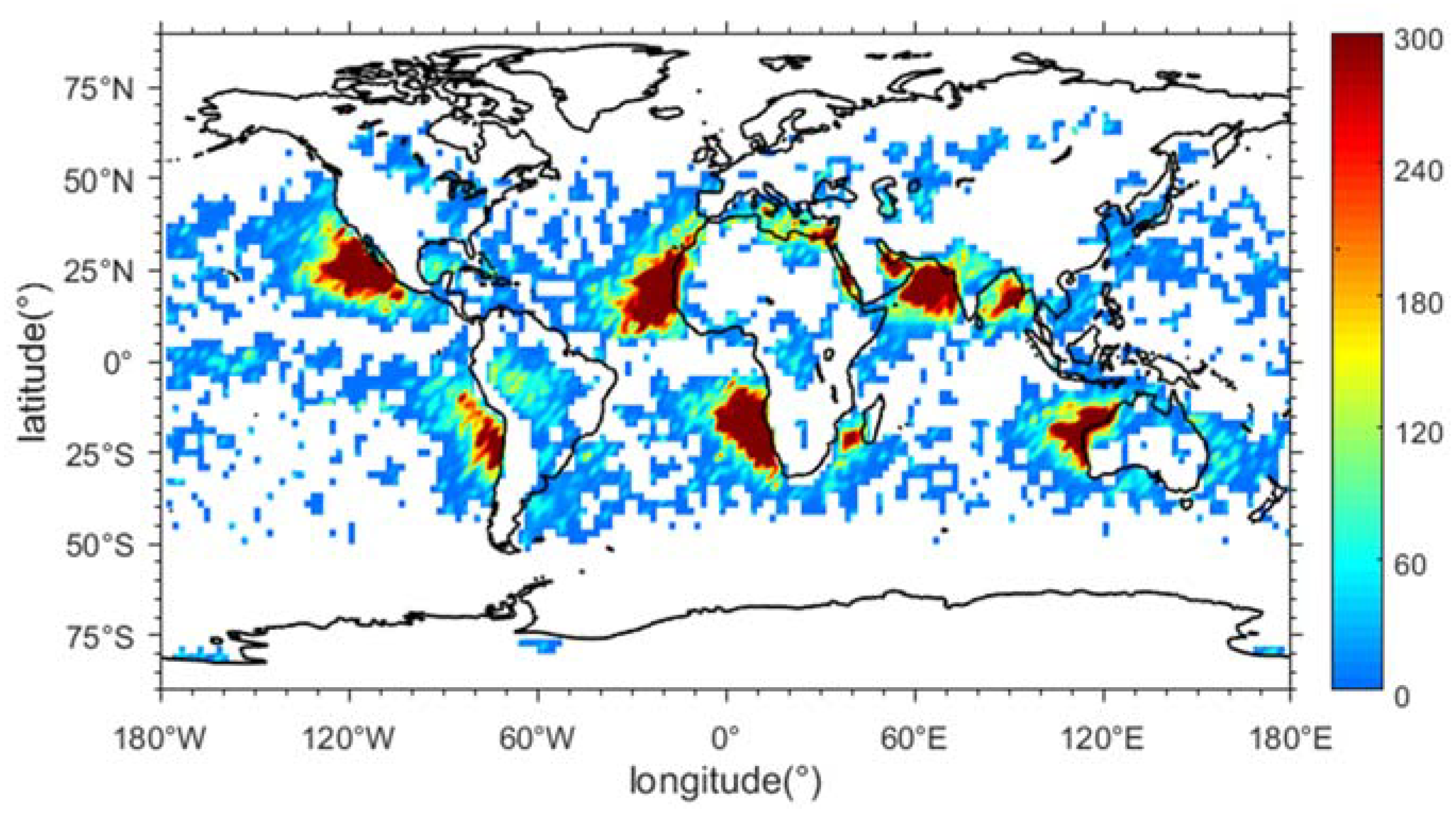
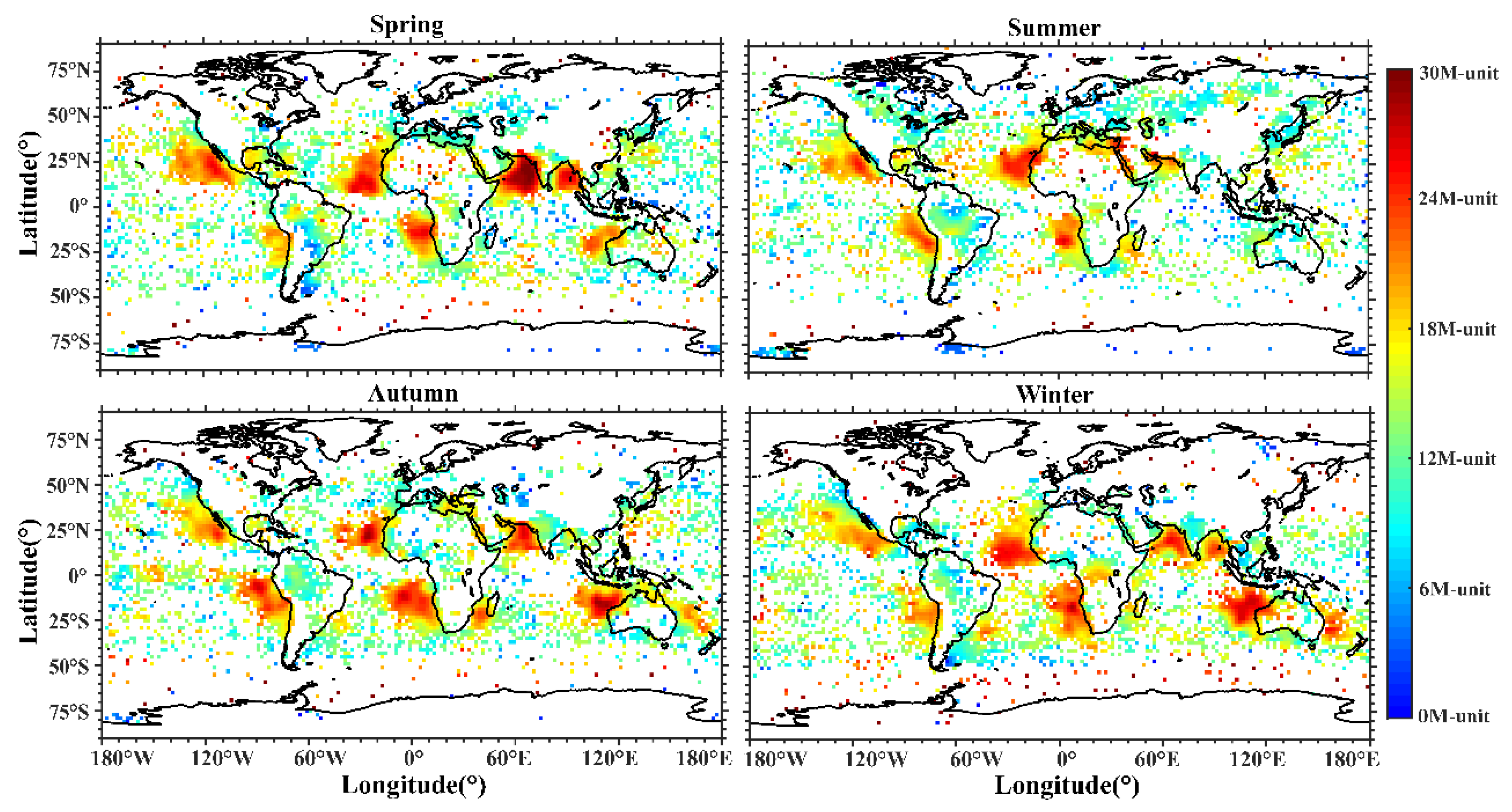
3.3. Intensity of Trapping
3.4. Thickness of Trapping
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bean, B.R.; Dutton, E.J. Radio meteorology. In Technical Report Archive and Image Library; U.S. Government Publishing Office: Washington, DC, USA, 1966. [Google Scholar]
- Kerr, D.E. Propagation of short radio waves (Revised edition). IEE Electromagn. Waves Ser. 1987, 24, 754. [Google Scholar]
- Ao, C.O. Effect of ducting on radio occultation measurements: An assessment based on high-resolution radiosonde soundings. Radio Sci. 2007, 42, 1–15. [Google Scholar] [CrossRef] [Green Version]
- Almond, T.; Clarke, J. Consideration of the usefulness of microwave propagation prediction methods on air-to-ground paths. In IEE Proceedings F (Communications, Radar and Signal Processing); IET Digital Library: London, UK, 1983; Volume 130, pp. 649–656. [Google Scholar]
- Crane, R.K. Refraction. In Propagation Handbook for Wireless Communication System Design; CRC Press LLC: Boca Raton, FL, USA, 2003. [Google Scholar]
- Turton, J.D.; Bennets, D.A.; Farmer, S.F.G. An introduction to radio ducting. Meteorol. Mag. 1988, 117, 245–254. [Google Scholar]
- Engeln, A.V.; Nedoluha, G.; Teixeira, J. An analysis of the frequency and distribution of ducting events in simulated radio occultation measurements based on ECMWF fields. J. Geophys. Res. Atmos. 2003, 108. [Google Scholar] [CrossRef] [Green Version]
- Engeln, V. A ducting climatology derived from the European Centre for Medium-Range Weather Forecasts global analysis fields. J. Geophys. Res. 2004, 109, 159–172. [Google Scholar] [CrossRef]
- Basha, G.; Ratnam, M.V.; Manjula, G. Anomalous propagation conditions observed over a tropical station using high-resolution GPS radiosonde observations. Radio Sci. 2013, 48, 42–49. [Google Scholar] [CrossRef]
- Saleem, M.U. Atmospheric Ducts Their Applications in Radio Frequency Propagation Using Satellite Remote Sensing Techniques Saarbrücken, 1st ed.; LAMBERT Academic Publishing: Sunnyvale, Germany, 2015; pp. 1–57. [Google Scholar]
- Klein, S.A.; Hartmann, D.L. The seasonal cycle of low stratiform clouds. J. Clim. 1993, 6, 1587–1606. [Google Scholar] [CrossRef] [Green Version]
- Duynkerke, P. Intercomparison of three- and one-dimensionalmodel simulations and aircraft observations of stratocumulus. Bound.-Layer Meteorol. 1999, 92, 453–487. [Google Scholar] [CrossRef]
- Teixeira, J. Simulation of fog with the ECMWF prognostic cloud Scheme. Q. J. R. Meteorol. Soc. 1999, 125, 529–552. [Google Scholar] [CrossRef]
- Tomczak, M.; Godfrey, J. Regional Oceanography: An Introduction; Daya Publishing House: Delhi, India, 2005; Volume 2. [Google Scholar] [CrossRef]
- Kursinski, E.R.; Hajj, G.A.; Schofield, J.T. Observing Earth’s atmosphere with radio occultation measurements using the Global Positioning System. J. Geophys. Res. Atmos. 1997, 102, 23429–23465. [Google Scholar] [CrossRef]
- Ware, R.; Rocken, C.; Solheim, F. GPS Sounding of the Atmosphere from Low Earth Orbit: Preliminary Results. Bull. Am. Meteorol. Soc. 1996, 77, 19–40. [Google Scholar] [CrossRef] [Green Version]
- Wickert, J.; Reigber, C.; Beyerle, G. Atmosphere sounding by GPS radio occultation: First results from CHAMP. Geophys. Res. Lett. 2001, 28, 3263–3266. [Google Scholar] [CrossRef] [Green Version]
- Hajj, G.A. CHAMP and SAC-C atmospheric occultation results and intercomparisons. J. Geophys. Res. 2004, 109. [Google Scholar] [CrossRef]
- Anthes, R.; Ector, C.; Hunt, D.C. The COSMIC/FORMOSAT-3 mission early results. Bull. Am. Meteorol. Soc. 2008, 3, 313–334. [Google Scholar] [CrossRef]
- Rocken, C.; Kuo, Y.H.; Scheriner, W.S. COSMIC System Description. Terr. Atmos. Ocean. Sci. 2000, 11, 21–52. [Google Scholar] [CrossRef] [Green Version]
- Anthes, R.A.; Rocken, C.; Kuo, Y.H. Applications of COSMIC to Meteorology and Climate. Terr. Atmos. Ocean. Sci. 2000, 11, 115–156. [Google Scholar] [CrossRef] [Green Version]
- Xie, F.; Wu, D.; Ao, C.O. Super-refraction effects on GPS radio occultation refractivity in marine boundary layers. Geophys. Res. Lett. 2010, 37, 174–187. [Google Scholar] [CrossRef]
- Xu, X.; Luo, J.; Chuang, S. Comparison of COSMIC Radio Occultation Refractivity Profiles with Radiosonde Measurements. Adv. Atmos. Sci. 2009, 26, 1137–1145. [Google Scholar] [CrossRef]
- ITU-R Rec. P.453-12. The Radio Refractive Index: Its Formula and Refractivity Data; International Telecommunication Union: Geneva, Switzerland, 2016. [Google Scholar]
- Hitney, H.V.; Richter, J.H.; Pappert, R.A. Tropospheric radio propagation assessment. Proc. IEEE 1985, 3, 265–283. [Google Scholar] [CrossRef]
- Zou, X.; Zeng, Z. A quality control procedure for GPS radio occultation data. Geophys. Res. 2006, 111. [Google Scholar] [CrossRef] [Green Version]
- Sokolovskiy, S.V. Tracking tropospheric radio occultation signals from low Earth orbit. Radio Sci. 2001, 36, 483–498. [Google Scholar] [CrossRef] [Green Version]
- Ao, C.O. Lower troposphere refractivity bias in GPS occultation retrievals. J. Geophys. Res. Atmos. 2003, 108, 4577. [Google Scholar] [CrossRef] [Green Version]
- Beyerle, G.; Gorbunov, M.E.; Ao, C.O. Simulation studies of GPS radio occultation measurements. Radio Sci. 2003, 38, 1084. [Google Scholar] [CrossRef] [Green Version]
- Xie, F.; Syndergaard, S.; Kursinski, E.R. An Approach for Retrieving Marine Boundary Layer Refractivity from GPS Occultation Data in the Presence of Super-refraction. J. Atmos. Ocean. Technol. 2006, 23, 1629. [Google Scholar] [CrossRef]
- Rocken, C.; Anthes, R.; Exner, M. Analysis and validation of GPS/MET data in the neutral atmosphere. J. Geophys. Res. Atmos. 1997, 1022, 29849–29866. [Google Scholar] [CrossRef]
- Marquardt, C.K.; Schöllhammer, K.; Beyerle, G. Validation and data quality of CHAMP radio occultation data. In First Champ Mission Results for Gravity Magnetic and Atmospheric Studies; Springer: Berlin/Heidelberg, Germany, 2003; pp. 384–396. [Google Scholar] [CrossRef]
- Sokolovskiy, S. Effect of super-refraction on inversions of radio occultation signals in the lower troposphere. Radio Sci. 2003, 38, 1058. [Google Scholar] [CrossRef] [Green Version]
- Ao, C.O.; Hajj, G.; Meehan, T.K. Rising and Setting GPS Occultations by Use of Open-Loop Tracking. J. Geophys. Res. Atmos. 2009, 114. [Google Scholar] [CrossRef] [Green Version]
- Gossard, E.E.; Strauch, R.G. Radar Observation of Clear Air and Cloud. J. R. Meteorol. Soc. 1983, 110, 283–284. [Google Scholar]
- Lopez, P. A 5-yr 40-km-Resolution Global Climatology of Super-refraction for Ground-Based Weather Radars. J. Appl. Meteorol. Climatol. 2008, 999, 89–110. [Google Scholar] [CrossRef]
- Steele, J.; Thorpe, S.; Turekian, K. Ocean Currents; Academic Press: Cambridge, MA, USA, 2010. [Google Scholar]
- Zhu, M.; Atkinson, B.W. Simulated Climatology of Atmospheric Ducts over the Persian Gulf. Bound.-Layer Meteorol. 2005, 115, 433–452. [Google Scholar] [CrossRef]
- Teixeira, J.; Hogan, T.F. Boundary Layer Clouds in a Global Atmospheric Model: Simple Cloud Cover Parameterizations. J. Clim. 2001, 15, 1261–1276. [Google Scholar] [CrossRef]
- Cheng, Y.H. Observed characteristics of atmospheric ducts over the South China Sea in autumn. Chin. J. Oceanol. Limnol. 2015, 34, 619–628. [Google Scholar] [CrossRef]
- Siebesma, A.P.; Bretherton, C.S.; Brown, A. A large eddy simulation intercomparison study of shallow cumulus convection. J. Atmos. Sci. 2003, 60, 1201–1219. [Google Scholar] [CrossRef]


| Refraction Types | N Gradient (N-Units/km) | M Gradient (M-Units/km) |
|---|---|---|
| Trapping layer | ||
| Super-refractive | ||
| Standard | ||
| Sub-refractive |
| Data Type | Data Date |
|---|---|
| METOPA2016 METOPB2016 METOPA KOMPSAT5 COSMIC METOPB METOPC PAZ GRACE SACC TANDEM-X TERRASAR-X | 2007.024~2015.305 2013.032~2015.365 2016.001~2020.091 2015.022~2020.091 2006.112~2020.116 2016.001~2020.091 2019.195~2020.091 2018.130~2020.091 2007.059~2017.334 2006.068~2011.215 2016.001~2020.121 2005.041~2020.121 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Y.; Liu, Y.; Qiao, J.; Lv, M.; Du, Z.; Fan, Z.; Zhao, J.; Yu, Z.; Li, J.; Zhao, Z.; et al. Investigation on Global Distribution of the Atmospheric Trapping Layer by Using Radio Occultation Dataset. Remote Sens. 2021, 13, 3839. https://doi.org/10.3390/rs13193839
Zhou Y, Liu Y, Qiao J, Lv M, Du Z, Fan Z, Zhao J, Yu Z, Li J, Zhao Z, et al. Investigation on Global Distribution of the Atmospheric Trapping Layer by Using Radio Occultation Dataset. Remote Sensing. 2021; 13(19):3839. https://doi.org/10.3390/rs13193839
Chicago/Turabian StyleZhou, Yong, Yi Liu, Jiandong Qiao, Mingjie Lv, Zhitao Du, Zhiqiang Fan, Jiaqi Zhao, Zhibin Yu, Jiang Li, Zhengyu Zhao, and et al. 2021. "Investigation on Global Distribution of the Atmospheric Trapping Layer by Using Radio Occultation Dataset" Remote Sensing 13, no. 19: 3839. https://doi.org/10.3390/rs13193839
APA StyleZhou, Y., Liu, Y., Qiao, J., Lv, M., Du, Z., Fan, Z., Zhao, J., Yu, Z., Li, J., Zhao, Z., He, F., & Zhou, C. (2021). Investigation on Global Distribution of the Atmospheric Trapping Layer by Using Radio Occultation Dataset. Remote Sensing, 13(19), 3839. https://doi.org/10.3390/rs13193839









