Pass-by-Pass Ambiguity Resolution in Single GPS Receiver PPP Using Observations for Two Sequential Days: An Exploratory Study
Abstract
:1. Introduction
2. Methods
2.1. Observation Models
2.2. Ambiguity Resolution
2.3. Least-Squares Estimation with Fixed Ambiguities
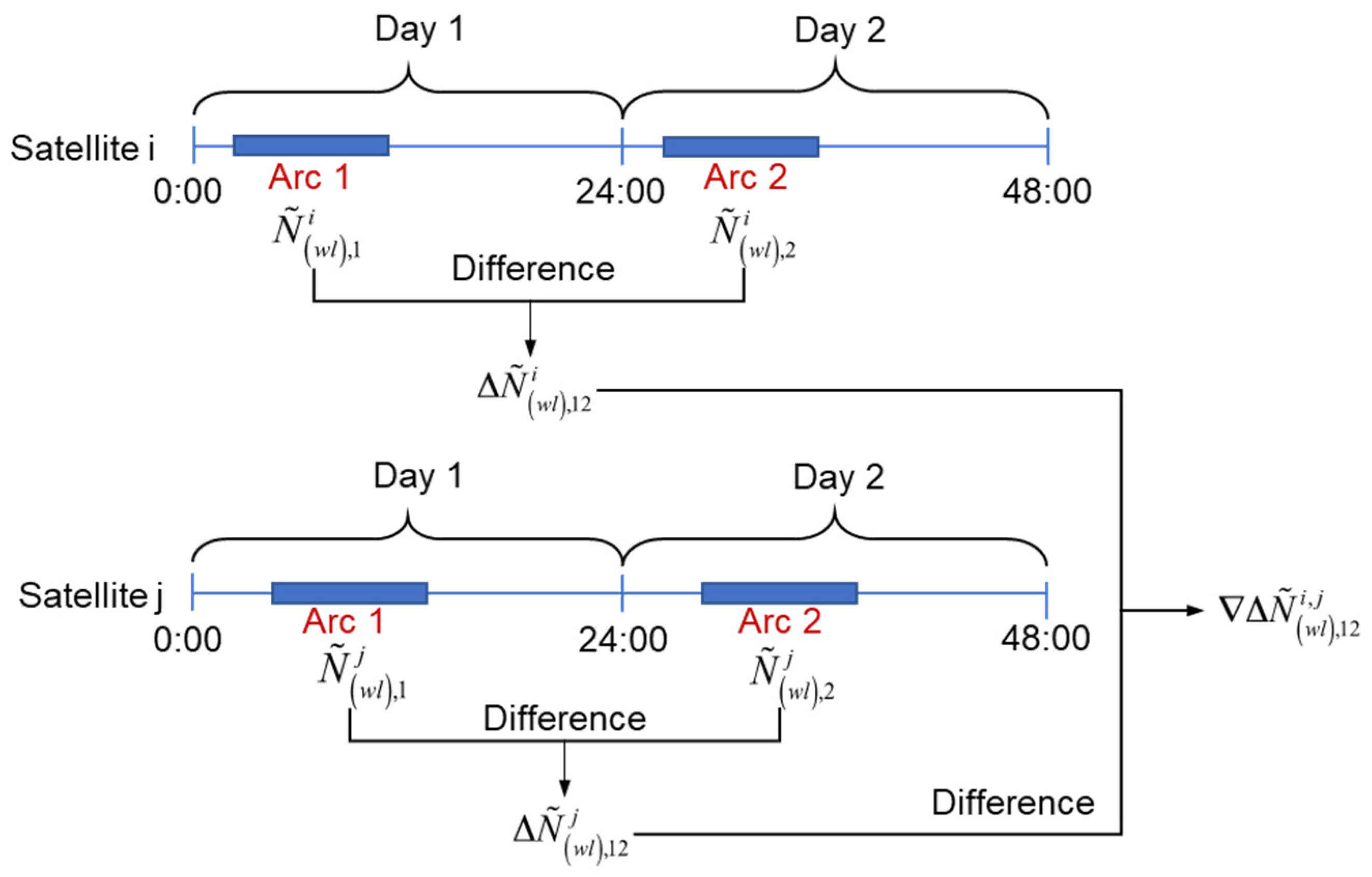
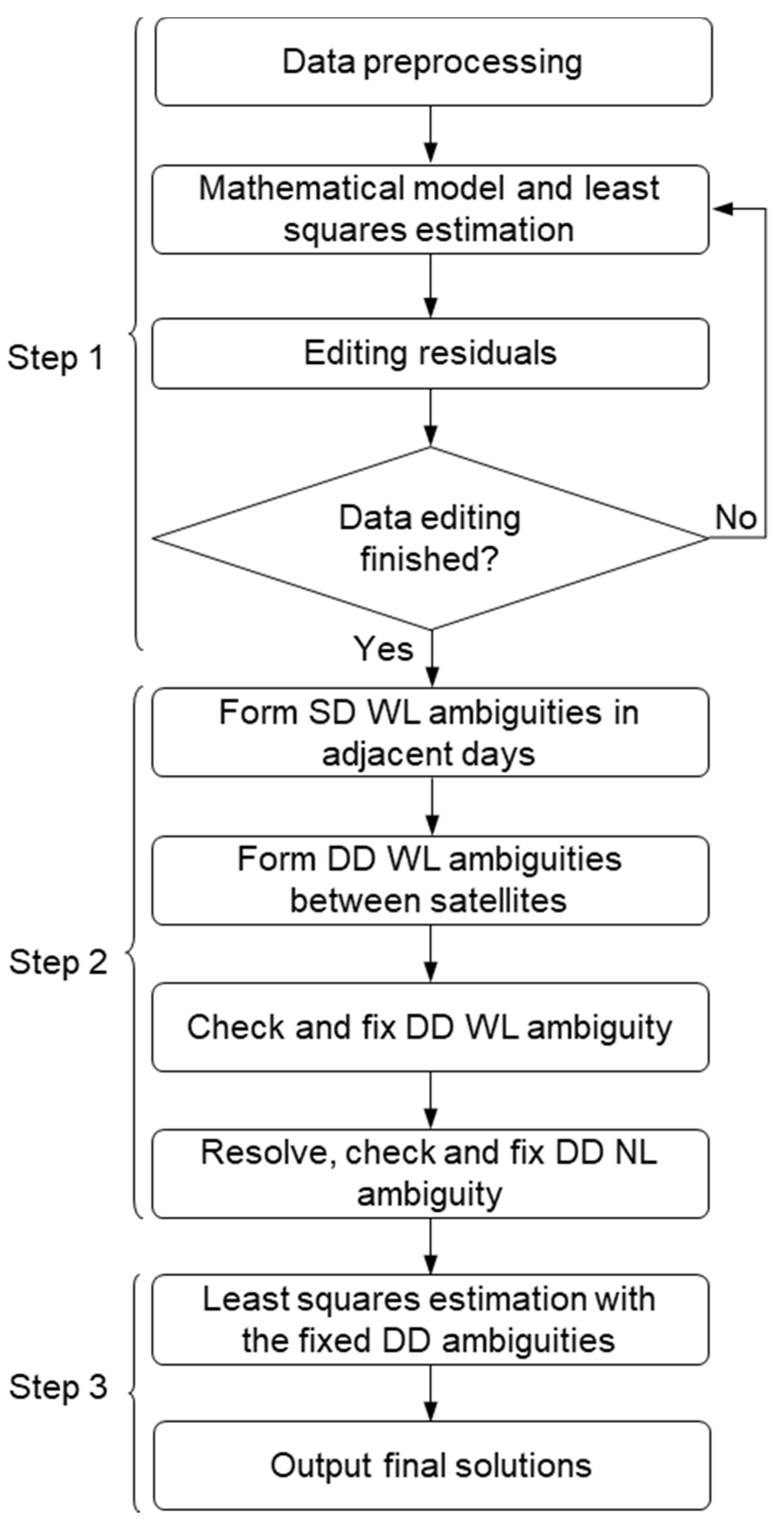
2.4. Data Processing Scheme
- The first step includes data preprocessing mathematical modelling, parameter estimation and residual editing. In data preprocessing, the data gaps and cycle-slips are detected based on the Turboedit algorithm [27]. Then, the code and carrier-phase observations are modelled with the IF combinations, and the parameters are estimated with the sequential least squares method to obtain the real-valued solution. It should be noted that we do not eliminate the ambiguity parameter as [7] did, because the number of parameters is not large for a single station.
- The second step is on the ambiguity resolution. Firstly, the un-differenced estimates of wide-lane ambiguities are derived from HMW combinations. Then, for each satellite, search the corresponding WL ambiguity for the second sidereal day through the start and end epochs plus 2864, and form the SD WL ambiguity. Note that, even though the specific GPS satellite has slightly different repeat periods [28,29], observations with 30s sampling rate would not have to consider the specific repeat period. However, for the higher sampling rates, such as less than 10s, the exact repeat period needs to be considered for the specific satellite. Next, the DD ambiguity is formed between satellites and inserted to Equation (14) to check whether the ambiguity can be successfully resolved. If it is fixable, the un-differenced IF ambiguities are mapped as the WL ambiguities did to obtain DD IF ambiguity, and DD NL ambiguity is derived by the fixed WL ambiguity and the DD IF ambiguity as in Equation (16). Then, Equation (14) will be applied to fix the DD NL ambiguity.
- All the ambiguities are searched and fixed in step 2, however, only a group of independent DD ambiguities are added and constrained to the NEQ. Then, the normal equation will be resolved again based on the least squares estimation to obtain the ambiguity-fixed solution.
3. Results
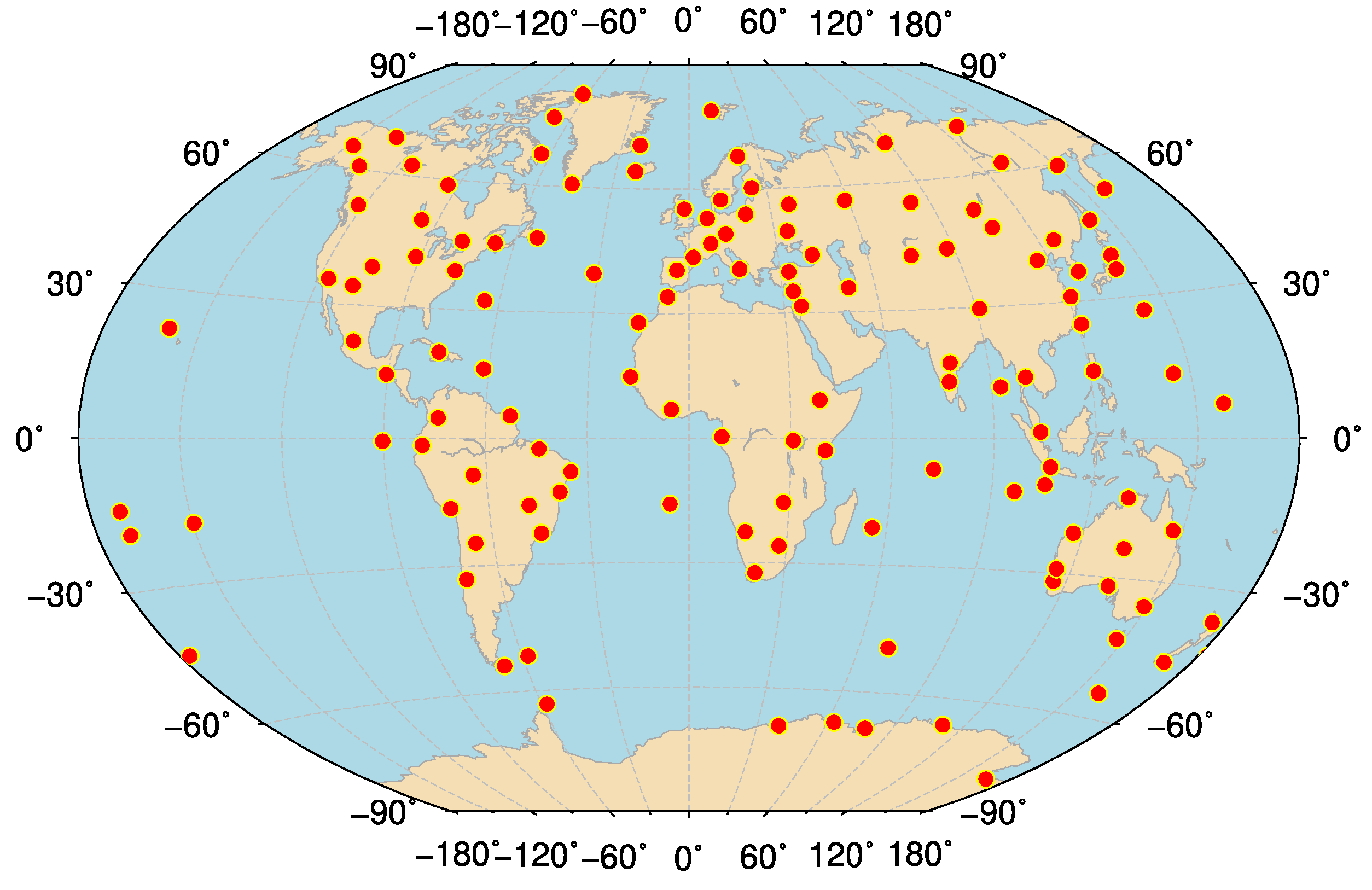
3.1. Data Collection and Processing Strategies
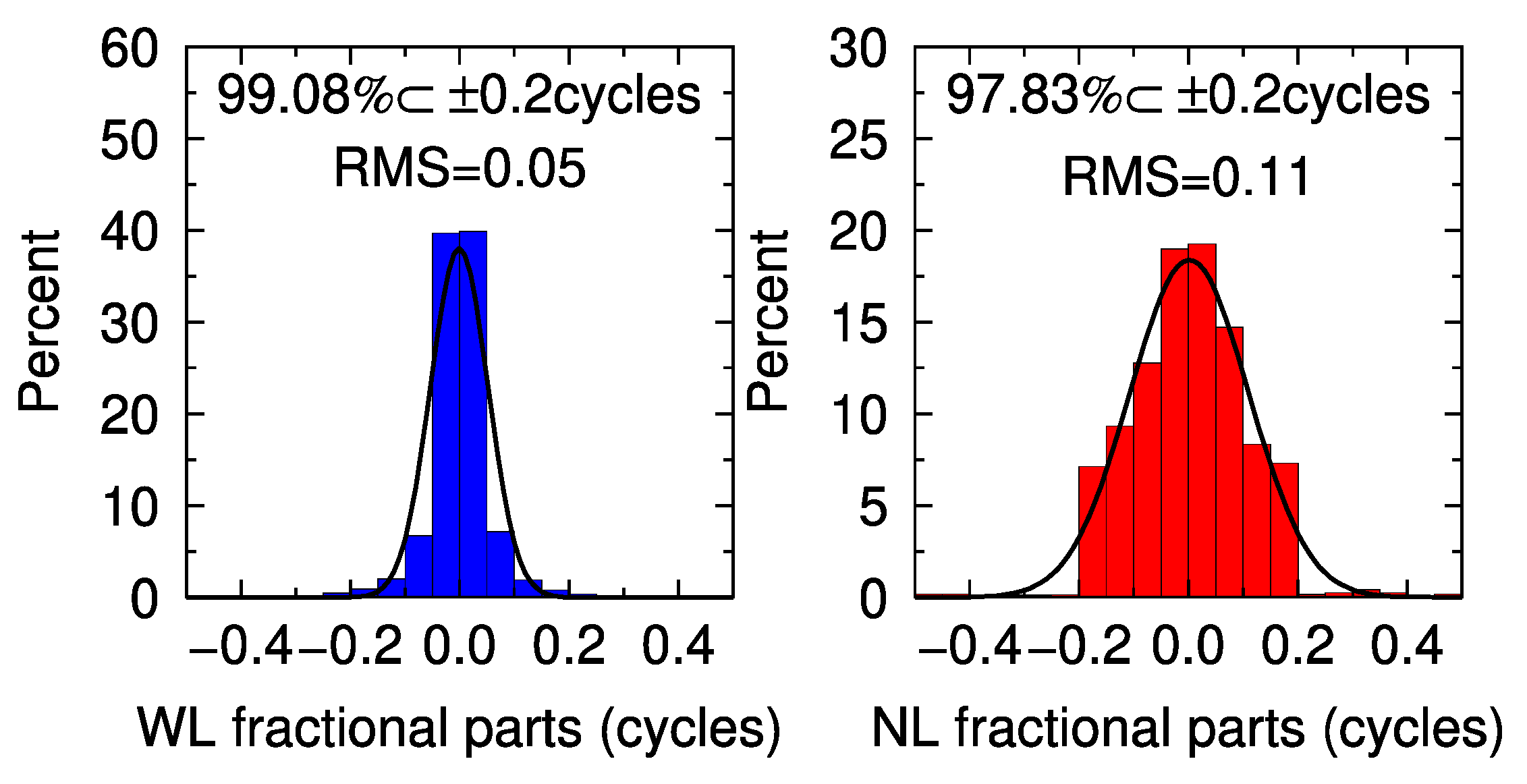
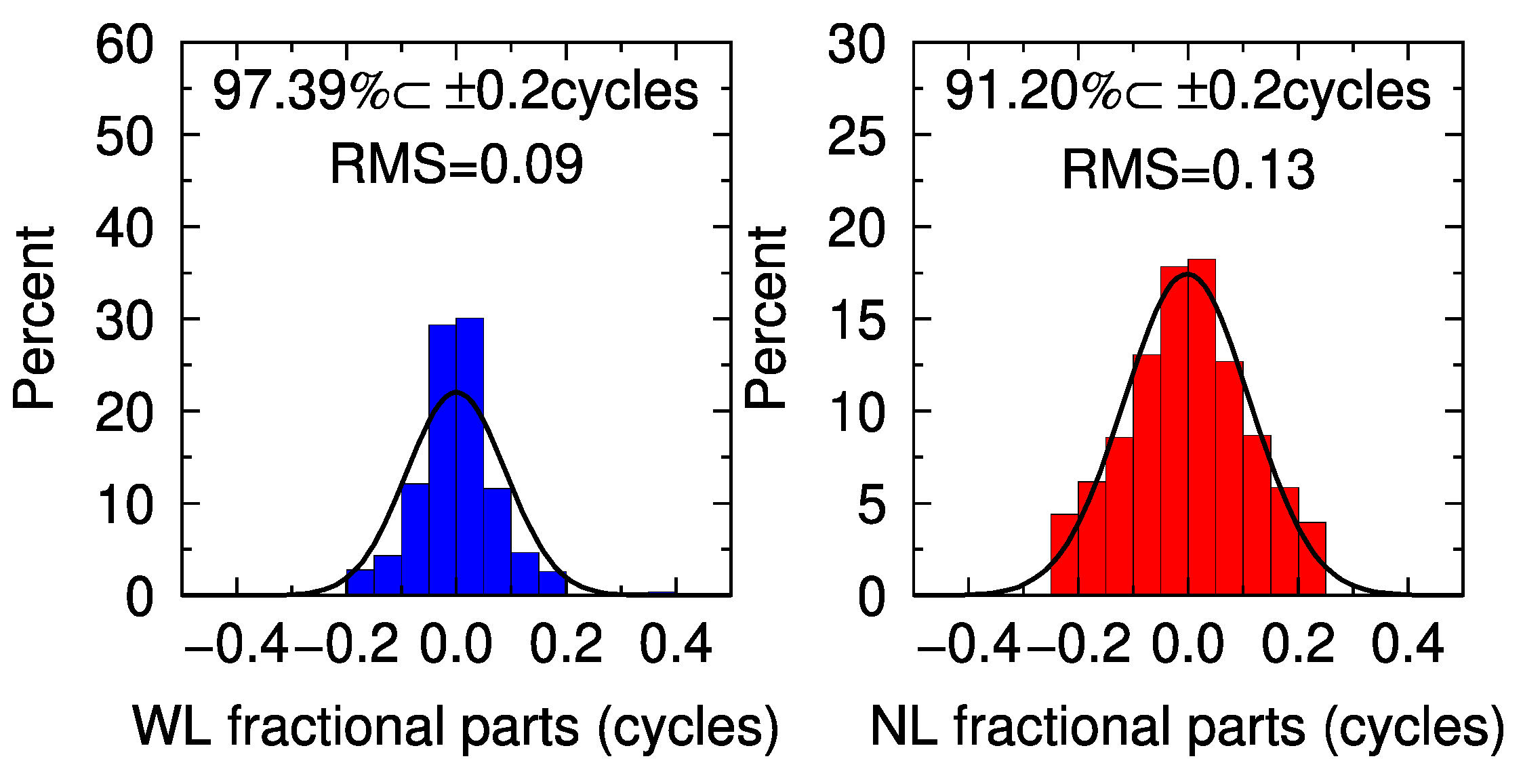
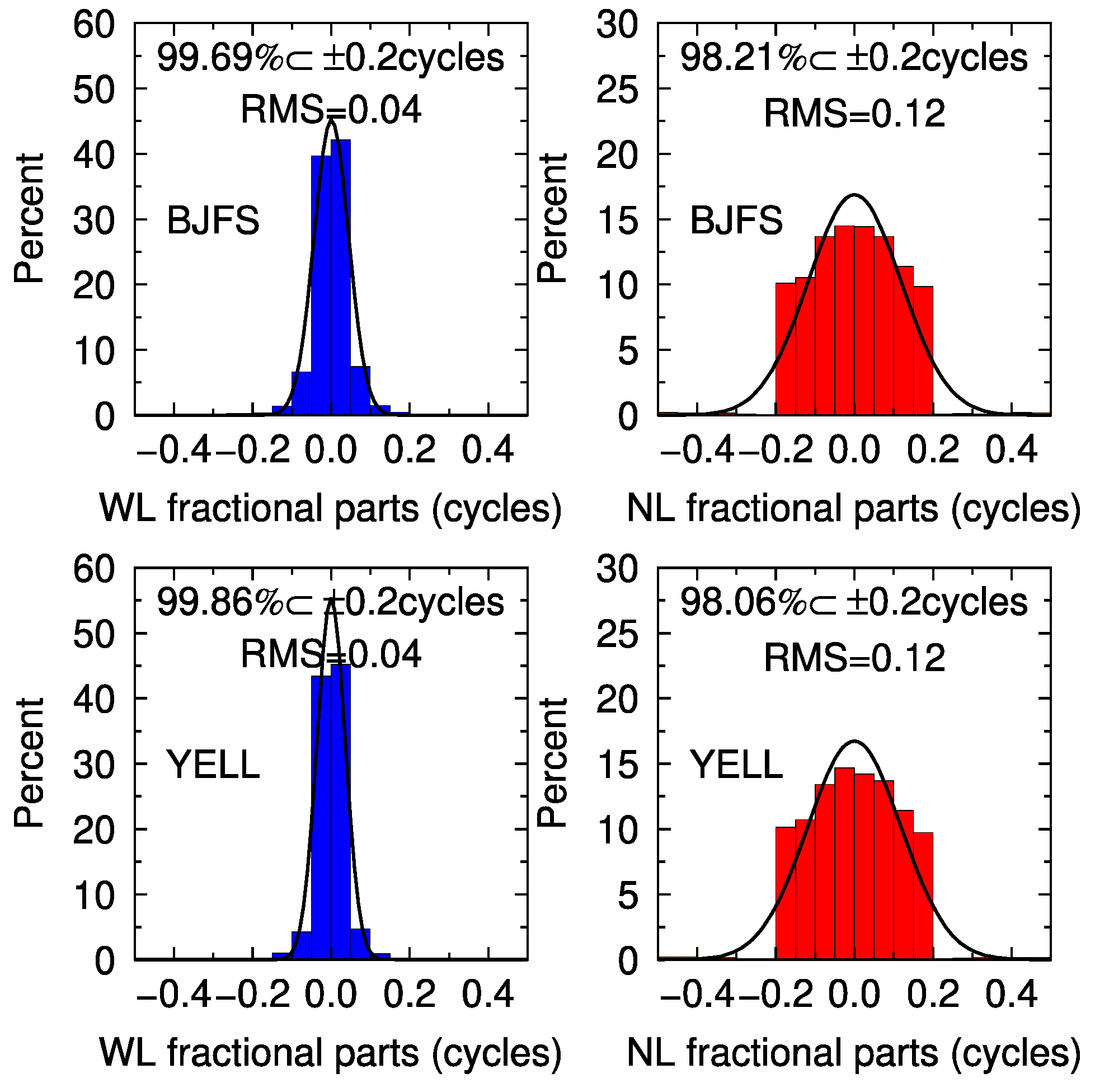
3.2. Fractional Parts of DD WL and NL Ambiguities
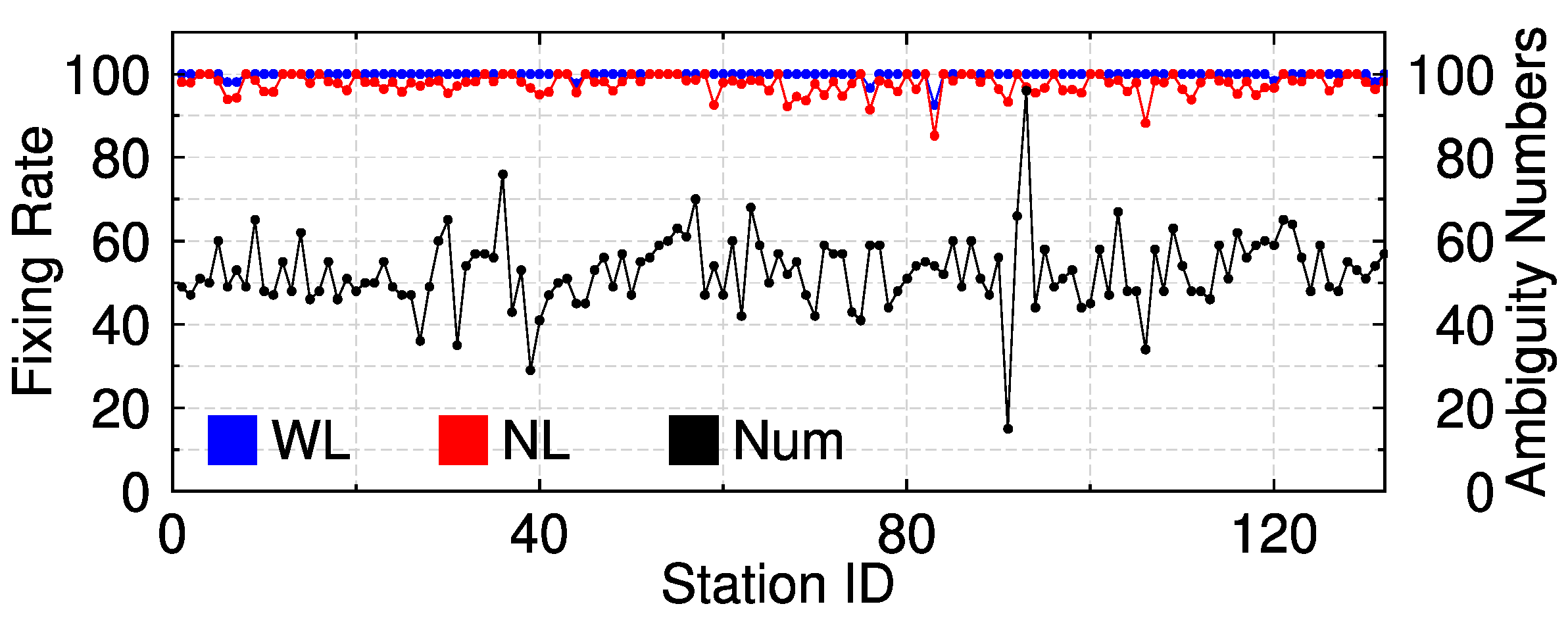
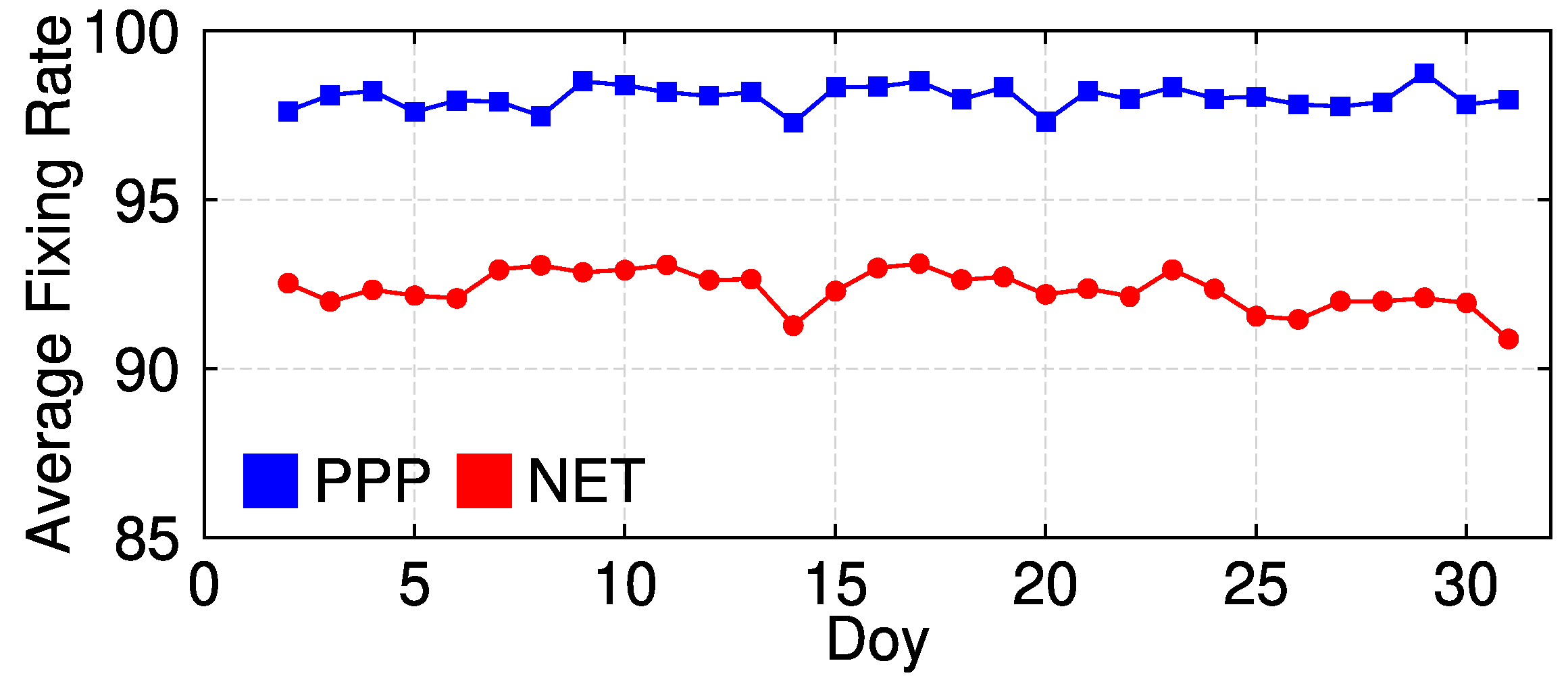
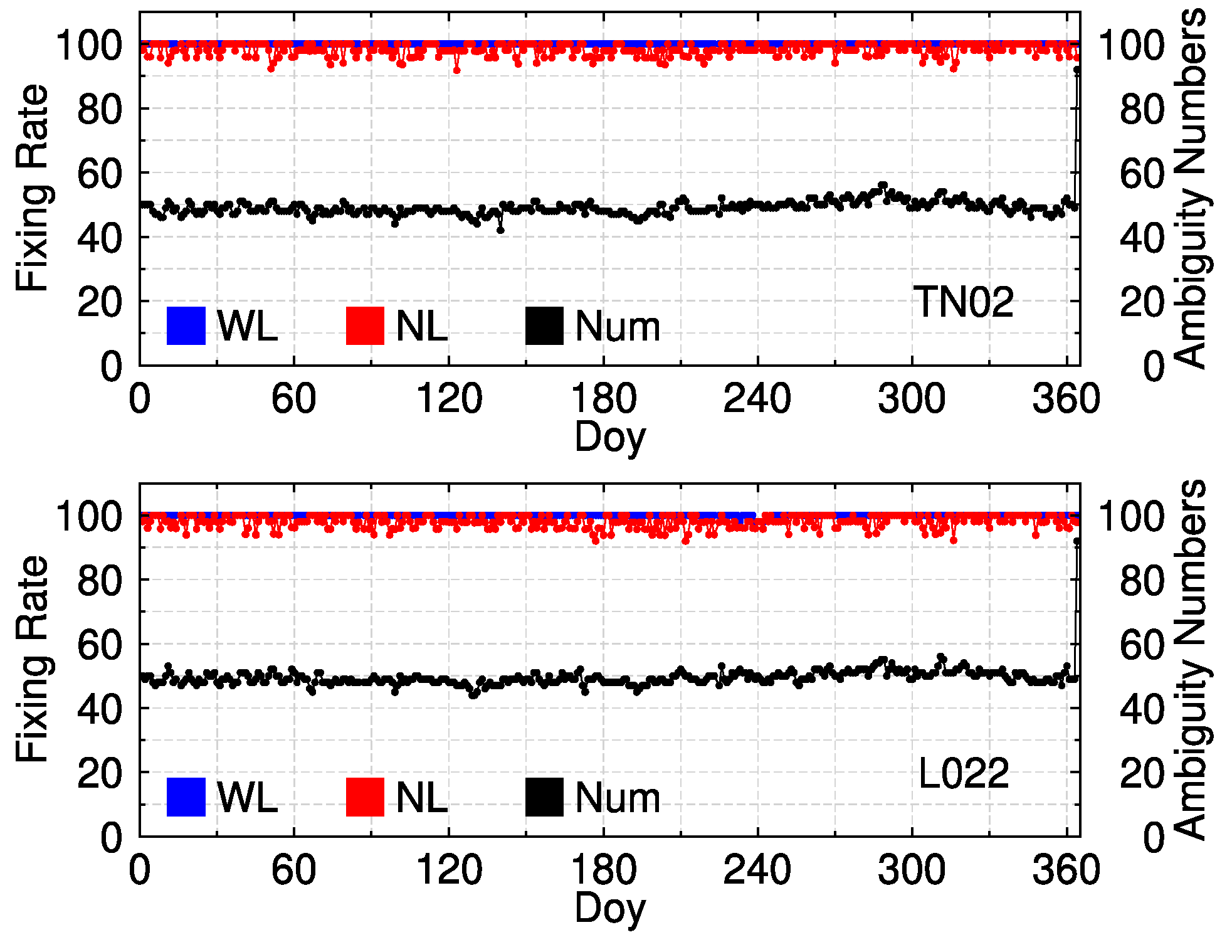
3.3. Ambiguity Fixing Rate
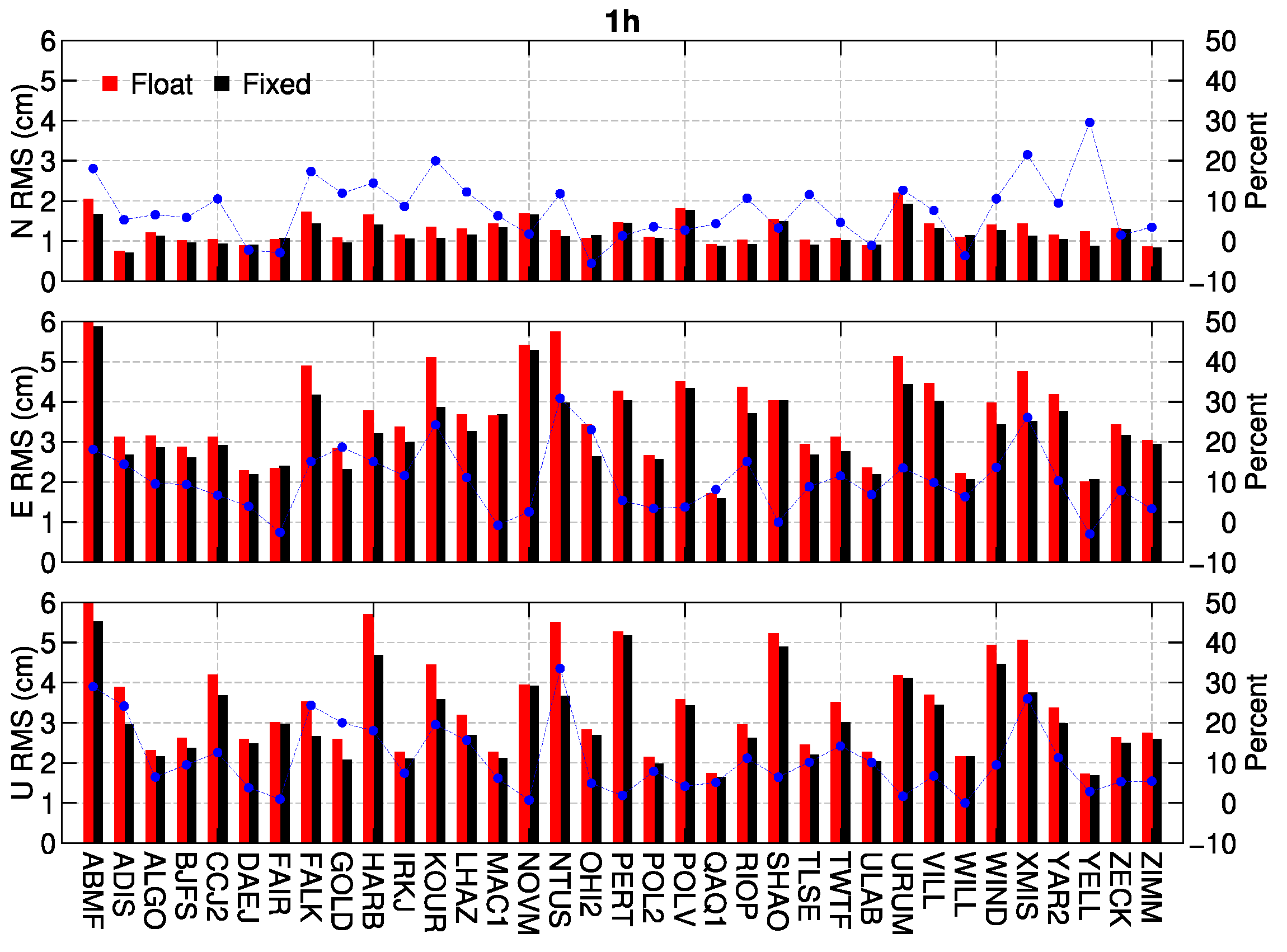
3.4. Positioning Accuracy Assessment
3.5. Deformation Monitoring Application
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Psimoulis, P.A.; Houlie, N.; Habboub, M.; Michel, C.; Rothacher, M. Detection of ground motions using high-rate GPS time-series. Geophys. J. Int. 2018, 214, 1237–1251. [Google Scholar] [CrossRef] [Green Version]
- Newman, A.V.; Stiros, S.; Feng, L.; Psimoulis, P.; Vamvakaris, D. Recent geodetic unrest at Santorini Caldera, Greece. Geophys. Res. Lett. 2012, 39, L06309. [Google Scholar] [CrossRef]
- Hudnut, K.W.; Behr, J.A. Continuous GPS Monitoring of Structural Deformation at Pacoima Dam, California. Seismol. Res. Lett. 1998, 69, 299–308. [Google Scholar] [CrossRef]
- Meng, X.; Nguyen, D.T.; Xie, Y.; Owen, J.S.; Psimoulis, P.; Ince, S.; Chen, Q.; Ye, J.; Bhatia, P. Design and Implementation of a New System for Large Bridge Monitoring—GeoSHM. Sensors 2018, 18, 775. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Psimoulis, P.; Ghilardi, M.; Fouache, E.; Stiros, S. Subsidence and evolution of the Thessaloniki plain, Greece, based on historical leveling and GPS data. Eng. Geol. 2007, 90, 55–70. [Google Scholar] [CrossRef]
- Ge, M.; Gendt, G.; Dick, G.; Zhang, F.P. Improving carrier-phase ambiguity resolution in global GPS network solutions. J. Geod. 2005, 79, 103–110. [Google Scholar] [CrossRef]
- Ge, M.; Gendt, G.; Dick, G.; Zhang, F.P.; Rothacher, M. A new data processing strategy for huge GNSS global networks. J. Geod. 2006, 80, 199–203. [Google Scholar] [CrossRef]
- Ge, M.; Gendt, G.; Rothacher, M.A.; Shi, C.; Liu, J. Resolution of GPS carrier-phase ambiguities in precise point positioning (PPP) with daily observations. J. Geod. 2008, 82, 389–399. [Google Scholar] [CrossRef]
- Khodabandeh, A.; Teunissen, P. Integer estimability in GNSS networks. J. Geod. 2019, 93, 1805–1819. [Google Scholar] [CrossRef] [Green Version]
- Teunissen, P.; Khodabandeh, A. Review and principles of PPP-RTK methods. J. Geod. 2015, 89, 217–240. [Google Scholar] [CrossRef]
- King, M.; Colemar, R.; Nguyen, L.N. Spurious periodic horizontal signals in sub-daily GPS position estimates. J. Geod. 2003, 77, 15–21. [Google Scholar] [CrossRef]
- Tregoning, P.; Watson, C. Atmospheric effects and spurious signals in GPS analyses. J. Geophys. Res. Solid Earth 2009, 114, B09403. [Google Scholar] [CrossRef] [Green Version]
- Khodabandeh, A. Single-station PPP-RTK: Correction latency and ambiguity resolution performance. J. Geod. 2021, 95, 42–66. [Google Scholar] [CrossRef]
- Laurichesse, D.; Mercier, F.; Berthias, J.P.; Broca, P.; Cerri, L. Integer Ambiguity Resolution on Undifferenced GPS Phase Measurements and Its Application to PPP and Satellite Precise Orbit Determination. Navigation 2009, 56, 135–149. [Google Scholar] [CrossRef]
- Collins, P. Isolating and estimating undifferenced GPS integer ambiguities. In Proceedings of the ION National Technical Meeting, San Diego, CA, USA, 28–30 January 2008; pp. 720–732. [Google Scholar]
- Geng, J.; Meng, X.; Dodson, A.H.; Teferle, F.N. Integer ambiguity resolution in precise point positioning: Method comparison. J. Geod. 2010, 84, 569–581. [Google Scholar] [CrossRef] [Green Version]
- Shi, J.; Gao, Y. A comparison of three PPP integer ambiguity resolution methods. GPS Solut. 2014, 18, 519–528. [Google Scholar] [CrossRef]
- Montenbruck, O.; Hackel, S.; Ijssel, J.; Arnold, D. Reduced dynamic and kinematic precise orbit determination for the Swarm mission from 4 years of GPS tracking. GPS Solut. 2018, 22, 79. [Google Scholar] [CrossRef]
- Zhou, X.; Chen, H.; Fan, W.; Zhou, X.; Chen, Q.; Jiang, W. Assessment of single-difference and track-to-track ambiguity resolution in LEO precise orbit determination. GPS Solut. 2021, 25, 1–15. [Google Scholar] [CrossRef]
- Montenbruck, O.; Hackel, S.; Jaggi, A. Precise orbit determination of the Sentinel-3A altimetry satellite using ambiguity-fixed GPS carrier phase observations. J. Geod. 2018, 92, 711–726. [Google Scholar] [CrossRef] [Green Version]
- An, X.; Meng, X.; Chen, H.; Jiang, W.; Xi, R.; Chen, Q. Combined precise orbit determination of GPS and GLONASS with ambiguity resolution. J. Geod. 2019, 93, 2585–2603. [Google Scholar] [CrossRef]
- Saastamoinen, J. Atmospheric correction for the troposphere and stratosphere in radio ranging satellites. Use Artif. Satell. Geod. 1972, 15, 247–251. [Google Scholar]
- Hatch, R. The synergism of GPS code and carrier measurements. In Proceedings of the Third International Symposium on Satellite Doppler Positioning, Las Cruces, NM, USA, 8–12 February 1982; Volume 2, pp. 1213–1231. [Google Scholar]
- Melbourne, W.G. The case for ranging in GPS-based geodetic systems. In Proceedings of the First International Symposium on Precise Positioning with the Global Positioning System, Rockville, MD, USA, 15–19 April 1985. [Google Scholar]
- Wübbena, G. Software developments for geodetic positioning with GPS using TI-4100 code and carrier measurements. In Proceedings of the First International Symposium on Precise Positioning with the Global Positioning System, Rockville, MD, USA, 15–19 April 1985. [Google Scholar]
- Dong, D.; Bock, Y. Global Positioning System network analysis with phase ambiguity resolution applied to crustal deformation studies in California. J. Geophys. Res. Solid Earth 1989, 94, 3949–3966. [Google Scholar] [CrossRef]
- Blewitt, G. An Automatic Editing Algorithm for GPS data. Geophys. Res. Lett. 1990, 17, 199–202. [Google Scholar] [CrossRef] [Green Version]
- Agnew, D.C.; Larson, K.M. Finding the repeat times of the GPS constellation. GPS Solut. 2007, 11, 71–76. [Google Scholar] [CrossRef]
- Larson, K.M.; Bilich, A.; Axelrad, P. Improving the precision of high-rate GPS. J. Geophys. Res. 2007, 112, B05422. [Google Scholar] [CrossRef]
- Liu, J.; Ge, M. PANDA software and its preliminary result of positioning and orbit determination. Wuhan Univ. J. Nat. Sci. 2003, 8, 603–609. [Google Scholar]
- Shi, C.; Zhao, Q.; Geng, J.; Lou, Y.; Liu, J. Recent development of PANDA software in GNSS data processing. In Proceedings of the SPIE 7285, International Conference on Earth Observation Data Processing and Analysis (ICEODPA), Wuhan, China, 28–30 December 2008. [Google Scholar]
- Boehm, J.; Niell, A.; Tregoning, P.; Schuh, H. Global mapping function (GMF): A new empirical mapping function based on numerical weather model data. Geophys. Res. Lett. 2006, 33, L07304. [Google Scholar] [CrossRef] [Green Version]
- Chen, G.; Herring, T.A. Effects of atmospheric azimuthal asymmetry on the analysis of space geodetic data. J. Geophys. Res. 1997, 102, 20489–20502. [Google Scholar] [CrossRef]
- Lyard, F.; Lefevre, F.; Letellier, T.; Francis, O. Modelling the global ocean tides: Modern insights from FES2004. Ocean Dyn. 2006, 56, 394–415. [Google Scholar] [CrossRef]
- Chen, H.; Jiang, W.; Ge, M.; Wickert, J.; Schuh, H. An enhanced strategy for GNSS data processing of massive networks. J. Geod. 2014, 88, 857–867. [Google Scholar] [CrossRef]



| Items | Strategies |
|---|---|
| Observations | Undifferenced IF combination of code and phase observations |
| Parameter estimation | Least squares |
| Reference Frame | ITRF2014 |
| Cut-off elevation | 7º |
| Sampling rate | 30s |
| Session length | 48h |
| Weight method | Elevation dependent weighting method |
| Phase Center Offset | PCO/PCV corrections, igs14_2045.atx |
| Phase wind-up | Corrected |
| Tropospheric delays | Mapping function: GMF [32]; Zenith delay parameters for station with a 1 h interval; 48 h gradients for north and east horizontal delays [33] |
| Receiver clocks | Solved at each epoch (white noise process) |
| Tidal Corrections | FES2004 [34] |
| Session Length | Float Solutions (cm) | Fixed Solutions (cm) | Improvement | ||||||
|---|---|---|---|---|---|---|---|---|---|
| N | E | U | N | E | U | N | E | U | |
| 1 h | 1.3 | 3.7 | 3.5 | 1.2 | 3.2 | 3.1 | 8.4% | 11.4% | 12.4% |
| 2 h | 0.8 | 2.0 | 2.1 | 0.7 | 1.7 | 1.8 | 10.8% | 15.8% | 11.8% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xi, R.; Chen, Q.; Meng, X.; Psimoulis, P.; Jiang, W.; Xu, C. Pass-by-Pass Ambiguity Resolution in Single GPS Receiver PPP Using Observations for Two Sequential Days: An Exploratory Study. Remote Sens. 2021, 13, 3728. https://doi.org/10.3390/rs13183728
Xi R, Chen Q, Meng X, Psimoulis P, Jiang W, Xu C. Pass-by-Pass Ambiguity Resolution in Single GPS Receiver PPP Using Observations for Two Sequential Days: An Exploratory Study. Remote Sensing. 2021; 13(18):3728. https://doi.org/10.3390/rs13183728
Chicago/Turabian StyleXi, Ruijie, Qusen Chen, Xiaolin Meng, Panos Psimoulis, Weiping Jiang, and Caijun Xu. 2021. "Pass-by-Pass Ambiguity Resolution in Single GPS Receiver PPP Using Observations for Two Sequential Days: An Exploratory Study" Remote Sensing 13, no. 18: 3728. https://doi.org/10.3390/rs13183728
APA StyleXi, R., Chen, Q., Meng, X., Psimoulis, P., Jiang, W., & Xu, C. (2021). Pass-by-Pass Ambiguity Resolution in Single GPS Receiver PPP Using Observations for Two Sequential Days: An Exploratory Study. Remote Sensing, 13(18), 3728. https://doi.org/10.3390/rs13183728









