Observed Near-Inertial Waves in the Northern South China Sea
Abstract
:1. Introduction
2. Tropical Cyclone, Data and Methods
2.1. Tropical Cyclone and Data
2.2. Methods
3. Results
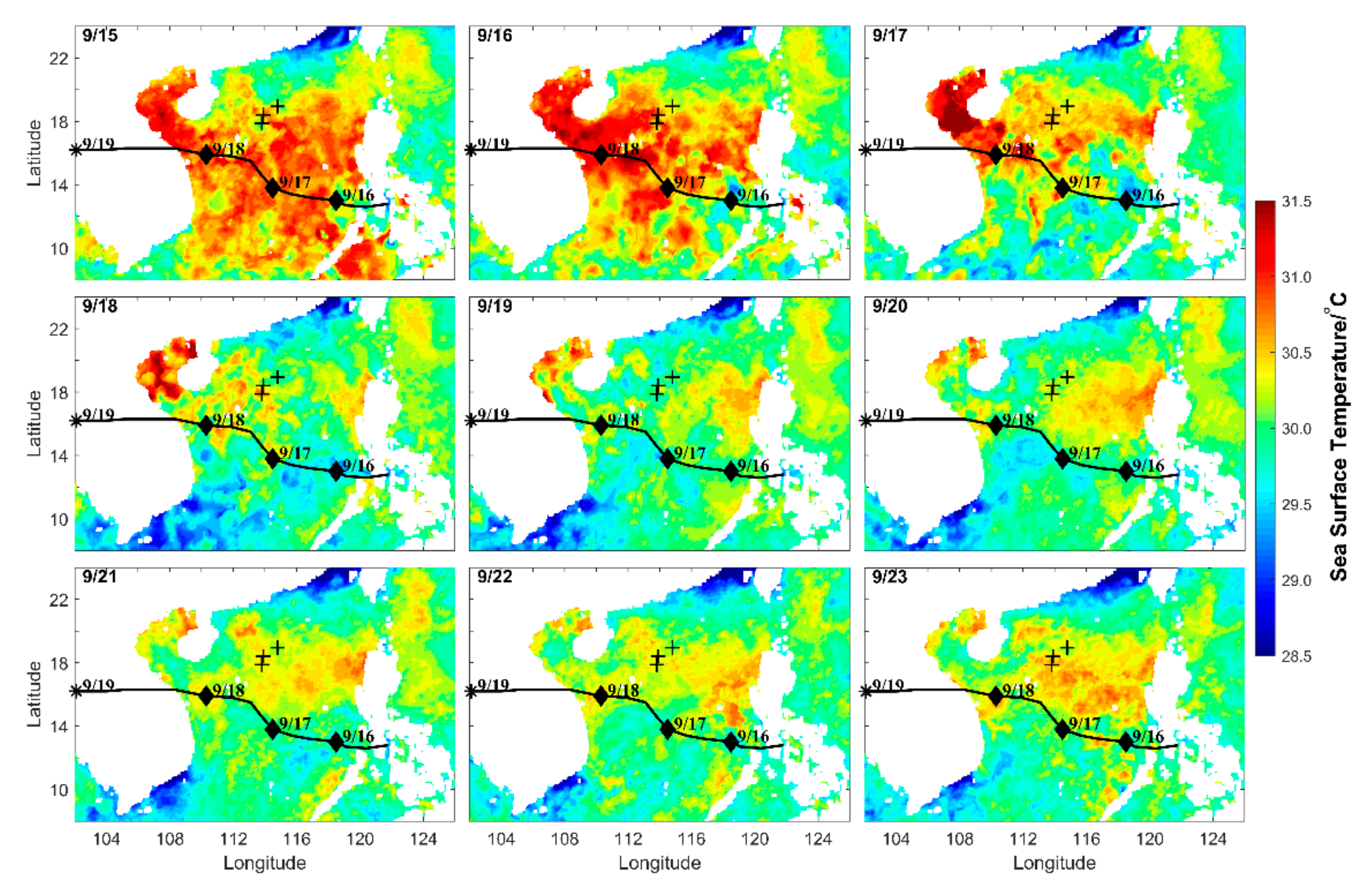
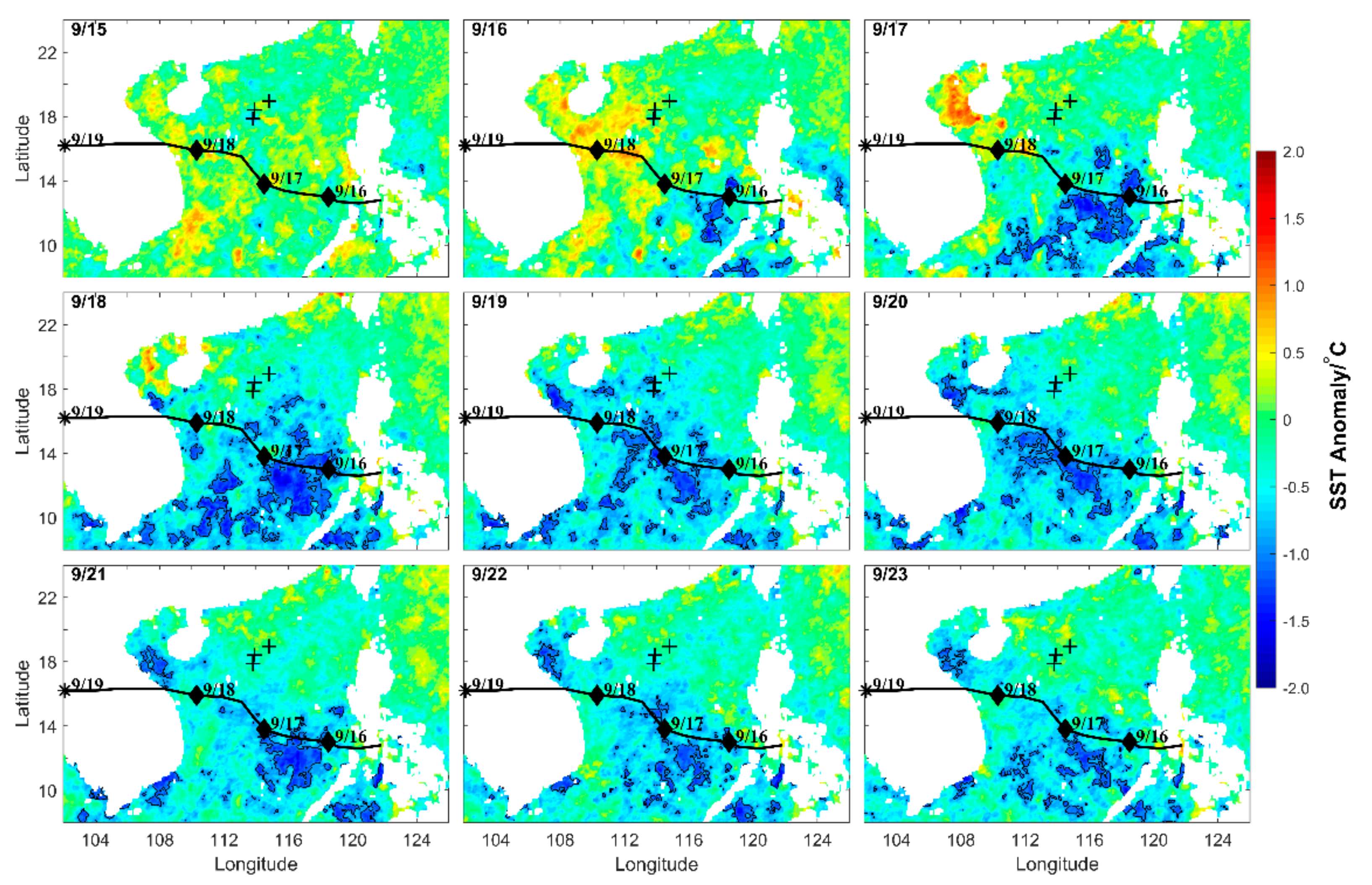
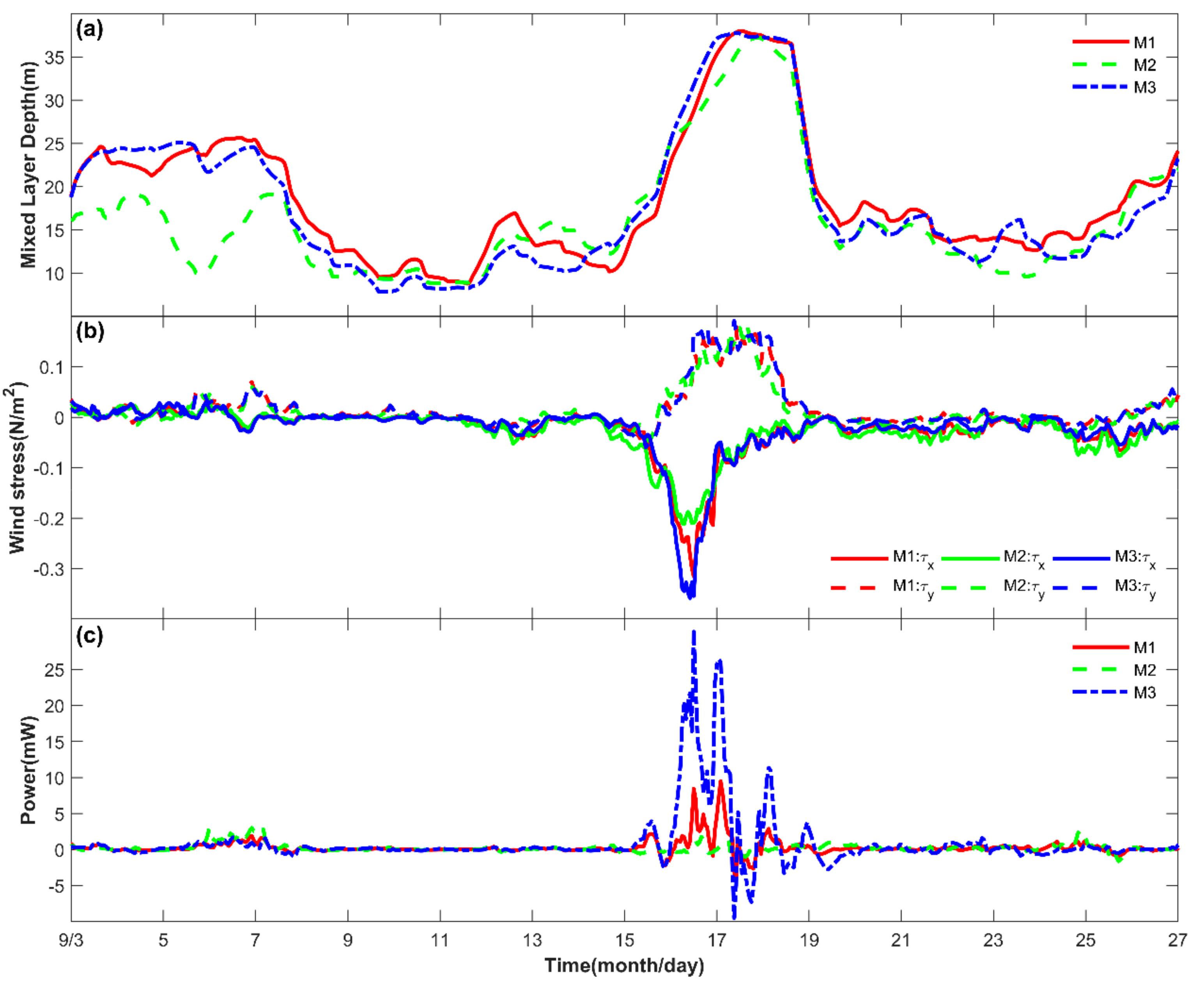
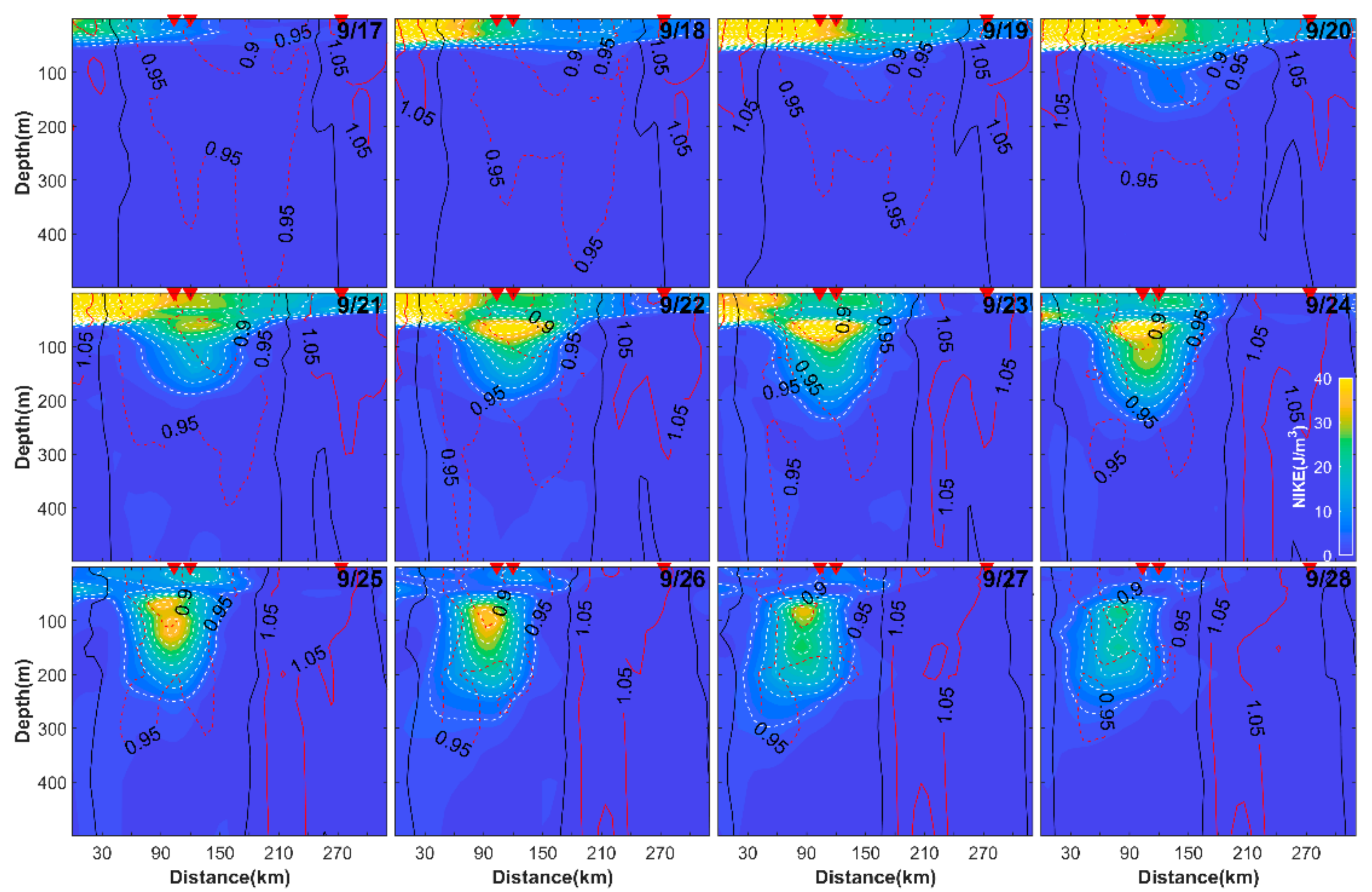
3.1. Thermal Response
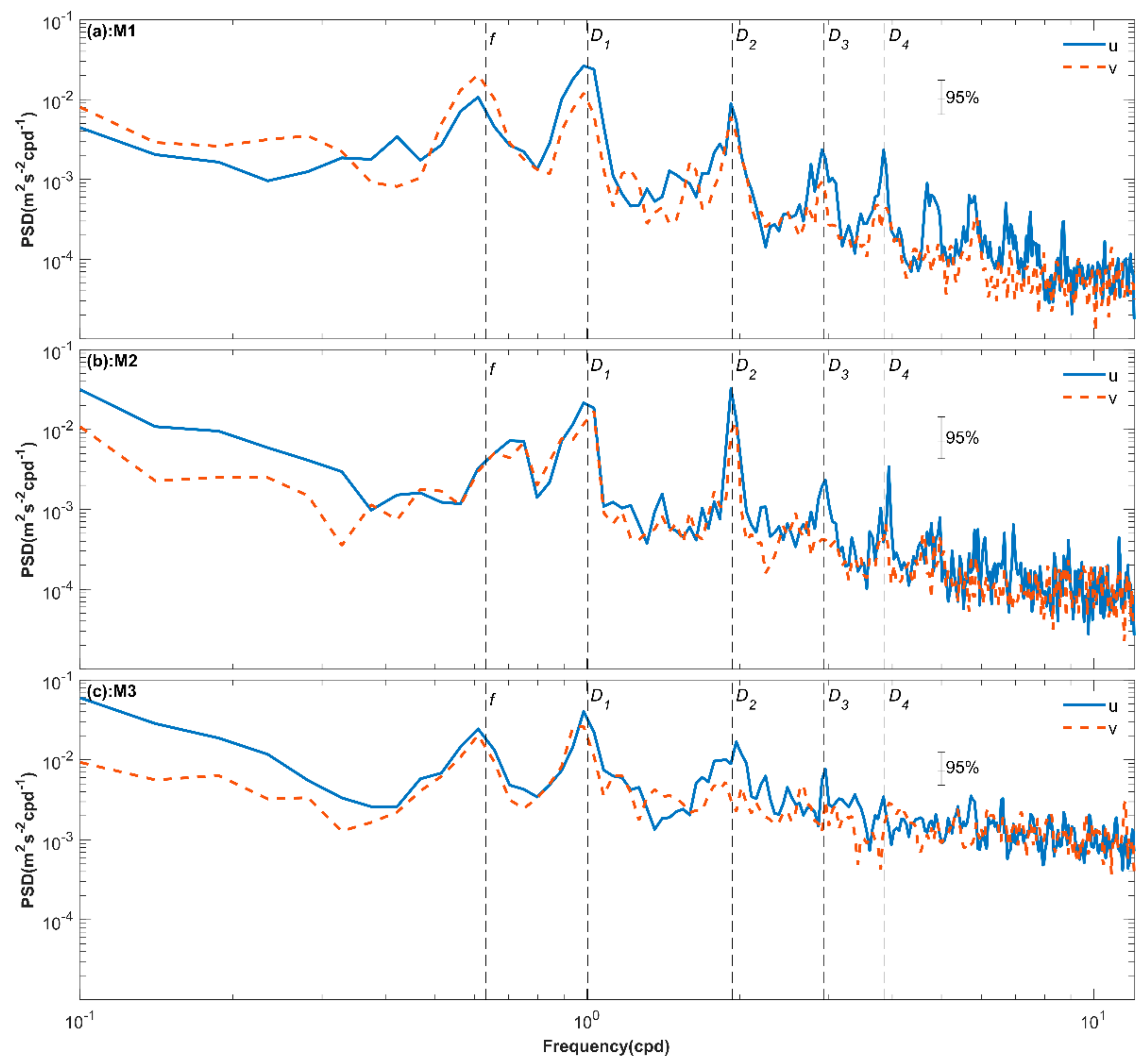
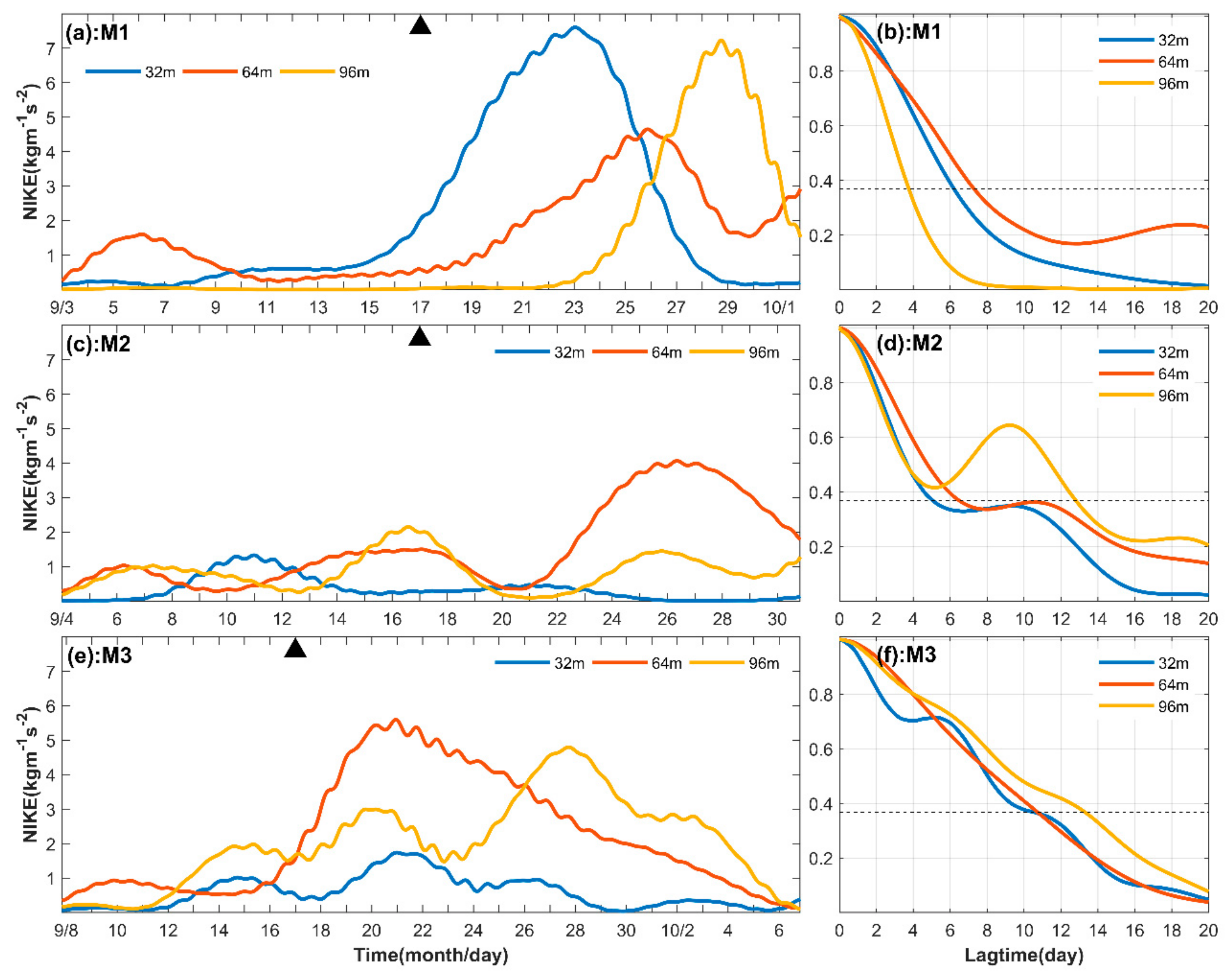
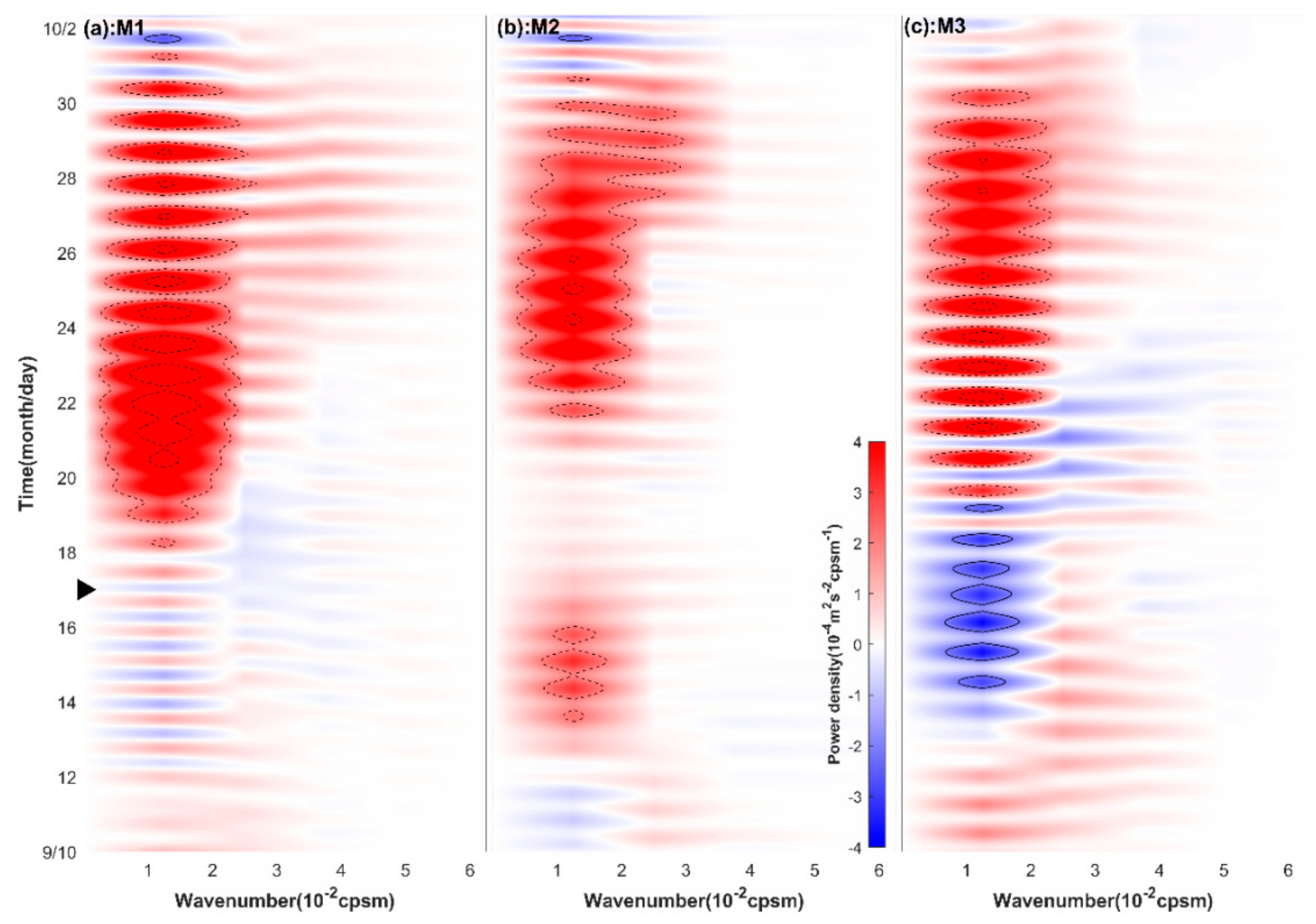
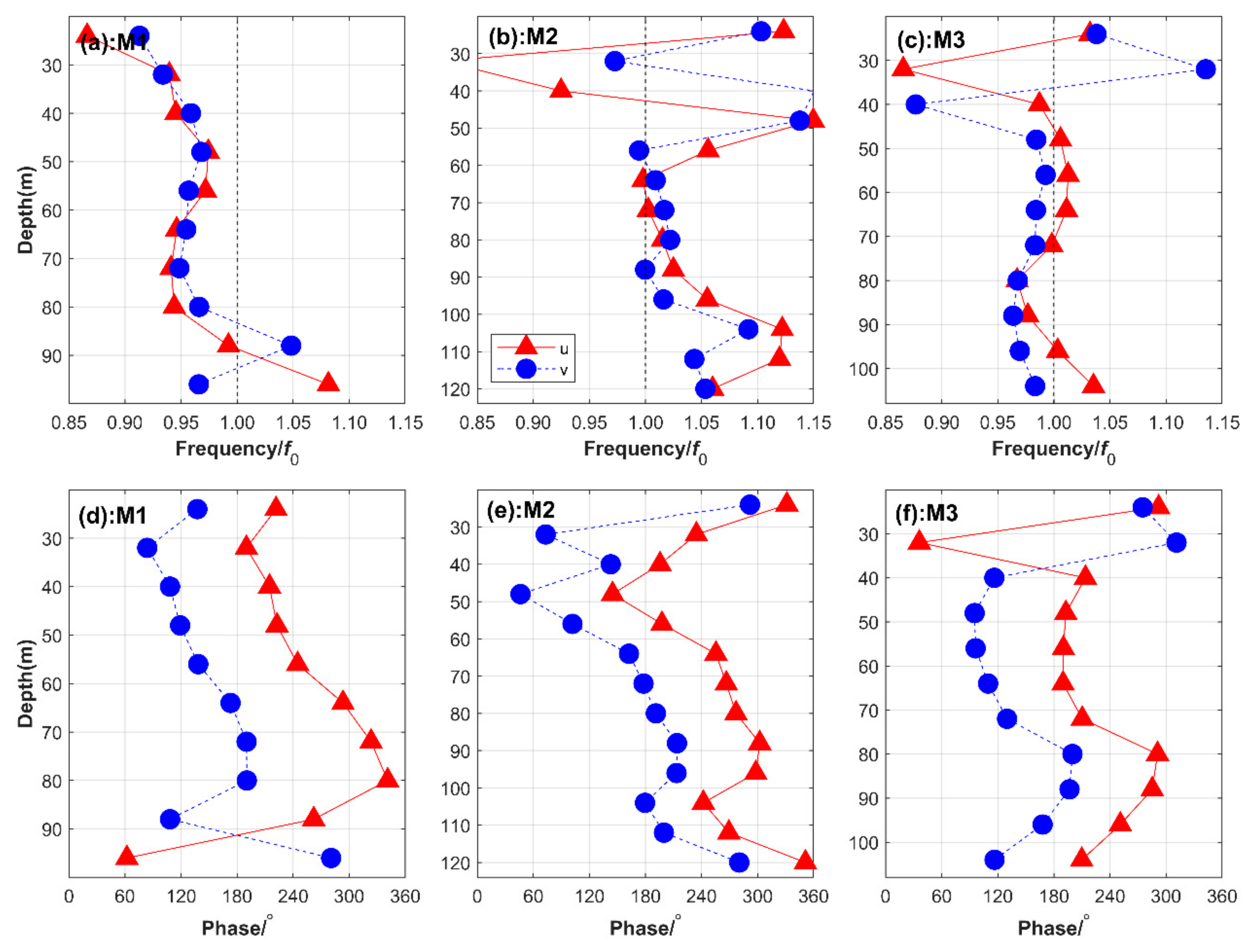
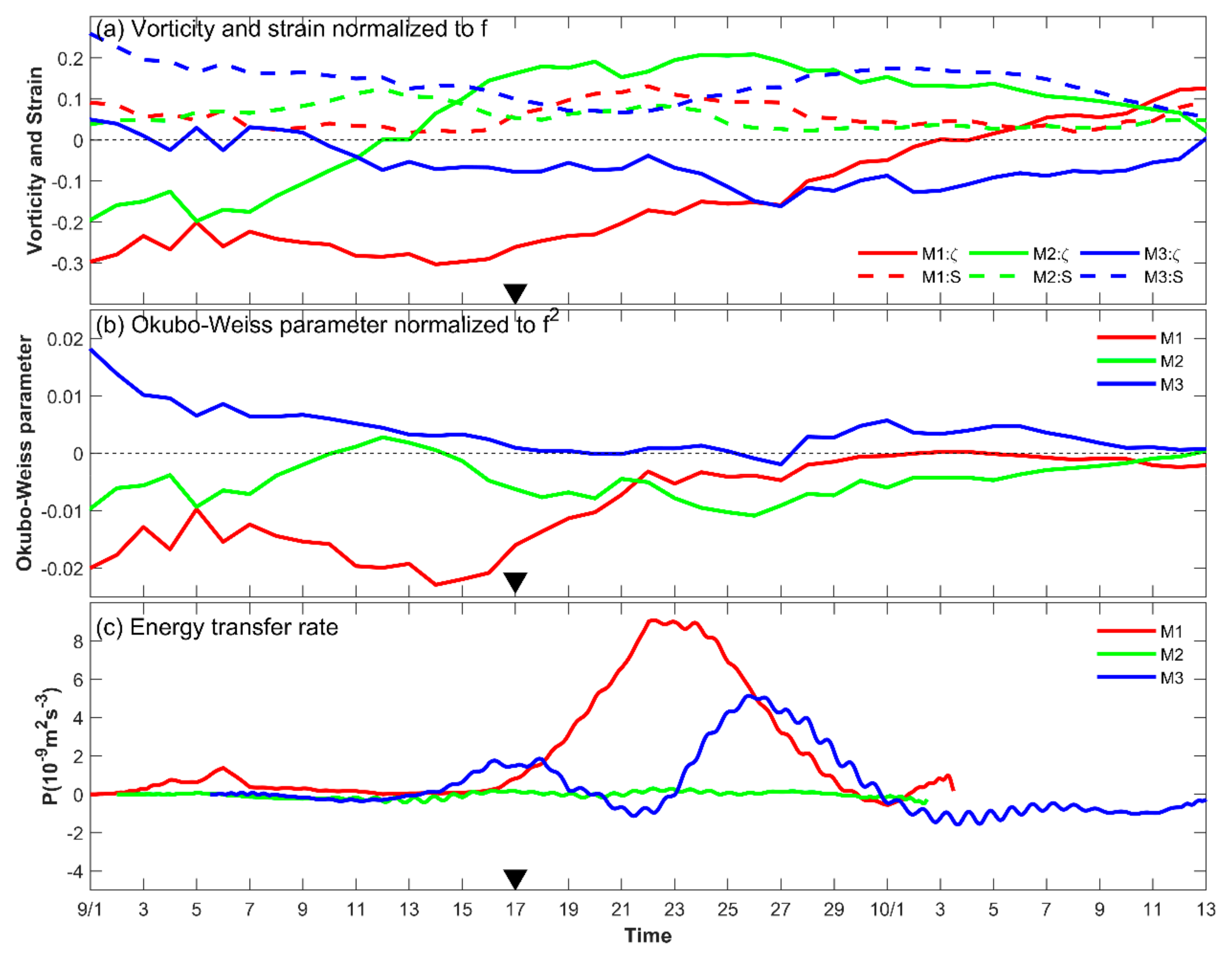
3.2. Dynamical Response
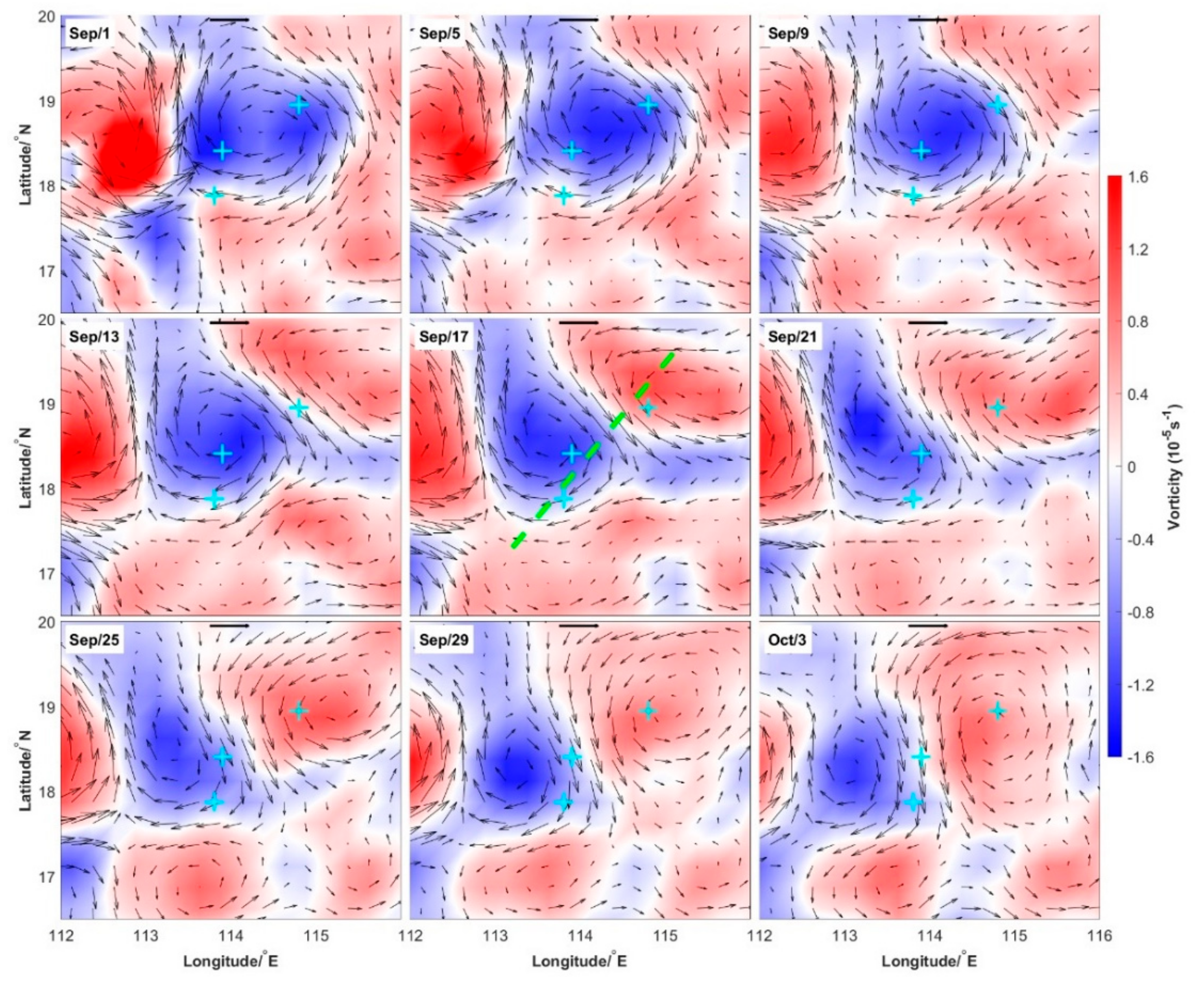
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Alford, M.H.; MacKinnon, J.A.; Simmons, H.L.; Nash, J.D. Near-inertial internal gravity waves in the ocean. Annu. Rev. Mar. Sci. 2016, 8, 95–123. [Google Scholar] [CrossRef] [Green Version]
- MaKinnon, J.A.; Winters, K.B. Subtropical catastrophe: Significant loss of low-mode tidal energy at 28.9°. Geophys. Res. Lett. 2005, 32, L15605. [Google Scholar] [CrossRef] [Green Version]
- Nikurashin, M.; Ferrari, R. Radiation and dissipation of internal waves generated by geostrophic motions impinging on small-scale topography: Theory. J. Phys. Oceanogr. 2010, 40, 1055–1074. [Google Scholar] [CrossRef]
- Rossby, C.G. On the mutual adjustment of pressure and velocity distributions in certain simple current systems, II. J. Mar. Res. 1937, 1, 239–263. [Google Scholar] [CrossRef]
- Gill, A.E. On the behavior of internal waves in the wakes of storms. J. Phys. Oceanogr. 1984, 14, 1129–1151. [Google Scholar] [CrossRef] [Green Version]
- D’Asaro, E.A. A collection of papers on the ocean storms experiment. J. Phys. Oceanogr. 1995, 25, 2817–2818. [Google Scholar] [CrossRef]
- Moehlis, J.; Llewellyn-Smith, S.G. Radiation of mixed layer near-inertial oscillations into the ocean interior. J. Phys. Oceanogr. 2001, 31, 1550–1560. [Google Scholar] [CrossRef]
- Alford, M.H. Improved global maps and 54-year history of wind-work on ocean inertial motions. Geophys. Res. Lett. 2003, 30, 1424. [Google Scholar] [CrossRef] [Green Version]
- Jiang, J.; Lu, Y.; Perrie, W. Estimating the energy flux form the wind to ocean inertial motions: The sensitivity to surface wind fields. Geophys. Res. Lett. 2005, 32, L15610. [Google Scholar] [CrossRef]
- Furuichi, N.; Hibiya, T.; Niwa, Y. Model-predicted distribution of wind-induced internal wave energy in the world’s oceans. J. Geophys. Res. 2008, 113, C09034. [Google Scholar] [CrossRef]
- Simmons, H.L.; Alford, M.H. Simulating the long-range swell of internal waves generated by ocean storms. Oceanography 2012, 25, 30–41. [Google Scholar] [CrossRef]
- Rimac, A.; von Storch, J.S.; Eden, C.; Haak, H. The influence of high-resolution wind stress field on the power input to near-inertial motions in the ocean. Geophys. Res. Lett. 2013, 40, 4882–4886. [Google Scholar] [CrossRef]
- Egbert, G.D.; Erofeeva, S.Y. Efficient inverse modeling of barotropic ocean tides. J. Atmos. Ocean. Technol. 2002, 19, 183–204. [Google Scholar] [CrossRef] [Green Version]
- Wunsch, C. The work done by the wind on the oceanic general circulation. J. Phys. Oceanogr. 1998, 28, 2332–2340. [Google Scholar] [CrossRef]
- Price, J.F. Internal wave wake of a moving storm. part I. scales, energy budget and observations. J. Phys. Oceanogr. 1983, 13, 949–965. [Google Scholar] [CrossRef] [Green Version]
- Cuypers, Y.; Vaillant, X.L.; Bouruet-Aubertot, P.; Vialard, J.; McPhaden, M.J. Tropical storm induced near-inertial internal waves during the Cirene experiment: Energy fluxes and impact on vertical mixing. J. Geophys. Res. Ocean. 2013, 118, 358–380. [Google Scholar] [CrossRef] [Green Version]
- Garrett, C. What is the “near-inertial” band and why is it different from the rest of the internal wave spectrum? J. Phys. Oceanogr. 2001, 31, 962–971. [Google Scholar] [CrossRef]
- Kunze, E. Near-inertial wave propagation in geostrophic shear. J. Phys. Oceanogr. 1985, 15, 544–565. [Google Scholar] [CrossRef]
- Yang, B.; Hou, Y.J. Near-inertial waves in the wake of 2011 Typhoon Nesat in the northern South China Sea. Acta Oceanol. Sin. 2014, 33, 102–111. [Google Scholar] [CrossRef]
- Yang, B.; Hou, Y.J.; Hu, P.; Liu, Z.; Liu, Y.H. Shallow ocean response to tropical cyclones observed on the continental shelf of the northwestern South China Sea. J. Geophys. Res. Ocean. 2015, 120, 3817–3836. [Google Scholar] [CrossRef]
- Wang, G.H.; Su, J.L.; Ding, Y.H.; Chen, D. Tropical cyclone genesis over the South China Sea. J. Mar. Syst. 2007, 68, 318–326. [Google Scholar] [CrossRef]
- Sun, L.; Zheng, Q.A.; Tang, T.Y.; Chuang, W.S.; Li, L.; Hu, J.Y.; Wang, D.X. Upper ocean near-inertial response to 1998 Typhoon Faith in the South China Sea. Acta Oceanol. Sin. 2012, 31, 25–32. [Google Scholar] [CrossRef]
- Chen, G.X.; Xue, H.J.; Wang, D.X.; Xie, Q. Observed near-inertial kinetic energy in the northwestern South China Sea. J. Geophys. Res. Ocean. 2013, 118, 4965–4977. [Google Scholar] [CrossRef] [Green Version]
- Yang, B.; Hou, Y.J.; Hu, P. Observed near-inertial waves in the wake of Typhoon Hagupit in the northern South China Sea. Chin. J. Oceanol. Limnol. 2015, 33, 1265–1278. [Google Scholar] [CrossRef]
- Cao, A.; Guo, Z.; Song, J.; Lv, X.; He, H.; Fan, W. Near-inertial waves and their underlying mechanisms based on the South China Sea internal wave experiment (2010–2011). J. Geophys. Res. Ocean. 2018, 123, 5026–5040. [Google Scholar] [CrossRef]
- Ma, Y.; Zhang, S.; Qi, Y.; Jing, Z. Upper ocean near-inertial response to the passage of two sequential typhoons in the northwestern South China Sea. Sci. China Earth Sci. 2019, 62, 863–871. [Google Scholar] [CrossRef]
- Wang, G.; Li, D.; Wei, Z.; Li, S.; Wang, Y.; Xu, T. Observed near-inertial waves in the wake of typhoon Linfa (2015) in the northern South China Sea. J. Ocean Univ. China 2019, 18, 1013–1021. [Google Scholar] [CrossRef]
- Li, R.X.; Chen, C.S.; Dong, W.J.; Beardsley, R.C.; Wu, Z.X.; Gong, W.P.; Liu, Y.Q.; Liu, T.M.; Xu, D.Y. Slope-intensified storm-induced near-inertial oscillations in the South China Sea. J. Geophys. Res. Oceans 2021, 126, e2020JC016713. [Google Scholar] [CrossRef]
- Ding, W.; Liang, C.; Liao, G.; Li, J.; Lin, F.; Jin, W.; Zhu, L. Propagation characteristics of near-inertial waves along the continental shelf in the wake of the 2008 typhoon Hagupit in the northers South China Sea. Bull. Mar. Sci. 2018, 94, 1293–1311. [Google Scholar] [CrossRef]
- Wu, R.; Zhang, H.; Chen, D.; Li, C.; Lin, J. Impact of typhoon Kalmaegi (2014) on the South China Sea: Simulations using a fully coupled atmosphere-ocean-wave model. Ocean Model. 2018, 131, 132–151. [Google Scholar] [CrossRef]
- Xu, J.; Huang, Y.; Chen, Z.; Liu, J.; Liu, T.; Li, J.; Cai, S.; Ning, D. Horizontal variations of typhoon-forced near-inertial oscillations in the South China Sea simulated by a numerical model. Cont. Shelf Res. 2019, 180, 24–34. [Google Scholar] [CrossRef]
- Cao, A.; Guo, Z.; Pan, Y.H.; Song, J.B.; He, H.L.; Li, P.L. Near-inertial waves induced by Typhoon Megi (2010) in the South China Sea. J. Mar. Sci. Eng. 2021, 9, 440. [Google Scholar] [CrossRef]
- Sun, L.; Zheng, Q.A.; Wang, D.X.; Hu, J.Y.; Tai, C.K.; Sun, Z.Y. A case study of near-inertial oscillation in the South China Sea using mooring observations and satellite altimeter data. J. Oceanogr. 2011, 67, 677–687. [Google Scholar] [CrossRef]
- Sun, Z.Y.; Hu, J.Y.; Zheng, Q.A.; Li, C.Y. Strong near-inertial oscillations in geostrophic shear in the northern South China Sea. J. Oceanogr. 2011, 67, 377–384. [Google Scholar] [CrossRef]
- Guan, S.D.; Zhao, W.; Huthnance, J.; Tian, J.W.; Wang, J.H. Observed upper ocean response to typhoon Megi (2010) in the Northern South China Sea. J. Geophys. Res. Ocean. 2014, 119, 3134–3157. [Google Scholar] [CrossRef] [Green Version]
- Shen, J.Q.; Fang, W.D.; Zhang, S.W.; Qiu, Y.; Zhang, J.P.; Xie, X.H. Observed internal tides and near-inertial waves in the northern South China Sea: Intensified f-band energy induced by Parametric Subharmonic Instability. J. Geophys. Res. Ocean. 2020, 125, e2020JC016324. [Google Scholar] [CrossRef]
- Liu, J.; He, Y.; Li, J.; Cai, S.; Wang, D.; Huang, Y. Case study of nonlinear interaction between near-inertial waves induced by typhoon and diurnal tides near Xisha Islands. J. Geophys. Res. Ocean. 2018, 123, 2768–2784. [Google Scholar] [CrossRef]
- Boyer, A.L.; Alford, M.H.; Pinkel, R.; Hennon, T.D.; Yang, Y.J.; Ko, D.; Nash, J. Frequency shift of near-inertial waves in the South China Sea. J. Phys. Oceanogr. 2020, 50, 1121–1135. [Google Scholar] [CrossRef] [Green Version]
- Gonella, J. A rotary-component method for analyzing meteorological and oceanographic vector time series. Deep Sea Res. Oceanogr. Abstr. 1972, 19, 833–846. [Google Scholar] [CrossRef]
- Byun, S.S.; Park, J.J.; Chang, K.I.; Schmitt, R.W. Observation of near-inertial wave reflections within the thermostad layer of an anticyclonic mesoscale eddy. Geophys. Res. Lett. 2010, 37, L01606. [Google Scholar] [CrossRef] [Green Version]
- Pollard, R.T.; Millard, R.C. Comparison between observed and simulated wind-generated inertial oscillations. Deep Res. Oceanogr. Abstr. 1970, 17, 813–821. [Google Scholar] [CrossRef]
- D’Asaro, E.A. The energy flux from the wind to near-inertial motions in the surface mixed layer. J. Phys. Oceanogr. 1985, 15, 1043–1059. [Google Scholar] [CrossRef] [Green Version]
- Alford, M.H. Internal swell generation: The spatial distribution of energy flux from the wind to mixed layer near-inertial motions. J. Phys. Oceanogr. 2001, 31, 2359–2368. [Google Scholar] [CrossRef]
- Oey, L.Y.; Ezer, T.; Wang, D.; Fan, S.; Yin, X. Loop Current warming by Hurricane Wilma. Geophys. Res. Lett. 2006, 33, L08613. [Google Scholar] [CrossRef] [Green Version]
- Leaman, K.D.; Sanford, T.B. Vertical energy propagation of inertial waves: A vector spectral analysis of velocity profiles. J. Geophys. Res. 1975, 80, 1975–1978. [Google Scholar] [CrossRef]
- Liu, J.L.; Cai, S.Q.; Wang, S.G. Observations of strong near-bottom current after the passage of Typhoon Pabuk in the South China Sea. J. Mar. Res. 2011, 87, 102–108. [Google Scholar] [CrossRef]
- Silverthorne, K.E.; Toole, J.M. Seasonal kinetic energy variability of near-inertial motions. J. Phys. Oceanogr. 2009, 39, 1035–1049. [Google Scholar] [CrossRef]
- Alford, M.H.; Gregg, M.C. Near-inertial mixing: Modulation of shear, strain and microstructure at low latitude. J. Geophys. Res.-Ocean. 2001, 106, 16947–16968. [Google Scholar] [CrossRef]
- Okubo, A. Horizontal dispersion of floatable particles in the vicinity of velocity singularities such as convergences. Deep Res. Oceanogr. Abstr. 1970, 17, 445–454. [Google Scholar] [CrossRef]
- Jing, Z.; Chang, P.; Dimarco, S.F.; Wu, L. Observed energy exchange between low-frequency flows and internal waves in the Gulf of Mexico. J. Phys. Oceanogr. 2018, 48, 995–1008. [Google Scholar] [CrossRef]
- Polzin, K.L. Mesoscale eddy-internal wave coupling. Part II: Energetics and results from PolyMode. J. Phys. Oceanogr. 2010, 40, 789–801. [Google Scholar] [CrossRef]
- Price, J.F.; Sanford, T.B.; Forristall, G.Z. Forced stage response to a moving hurricane. J. Phys. Oceanogr. 1994, 24, 233–260. [Google Scholar] [CrossRef]
- Zhang, H.; Chen, D.; Zhou, L.; Liu, X.; Ding, T.; Zhou, B. Upper ocean response to typhoon Kalmaegi (2014). J. Geophys. Res. Ocean. 2016, 121, 6520–6535. [Google Scholar] [CrossRef]
- Whitt, D.B.; Thomas, L.N. Resonant generation and energetics of wind-forced near-inertial motions in a geostrophic flow. J. Phys. Oceanogr. 2015, 45, 181–208. [Google Scholar] [CrossRef]
- Jing, Z.; Wu, L.; Ma, X. Energy exchange between the mesoscale oceanic eddies and wind-forced near-inertial oscillations. J. Phys. Oceanogr. 2017, 47, 721–733. [Google Scholar] [CrossRef]
- Noh, S.; Nam, S. Observations of enhanced internal waves in an area of strong mesoscale variability in the southwestern East Sea (Japan Sea). Sci. Rep. 2020, 10, 9068. [Google Scholar] [CrossRef] [PubMed]
- Jeon, C.; Park, J.H.; Park, Y.G. Temporal and spatial variability of near-inertial waves in the East/Japan Sea from a high-resolution wind-forced ocean model. Geophys. Res. Ocean. 2019, 124, 6015–6029. [Google Scholar] [CrossRef]
- Kawaguchi, Y.; Wagawa, T.; Igeta, Y. Near-inertial internal waves and multiple-inertial oscillations trapped by negative vorticity anomaly in the central Sea of Japan. Progr. Oceanogr. 2020, 181, 102240. [Google Scholar] [CrossRef]



| Mooring | M1 | M2 | M3 |
|---|---|---|---|
| Position | 113.90°E, 18.41°N | 114.80°E, 18.95°N | 113.80°E, 17.88°N |
| Water depth (m) | 2205 | 2450 | 2485 |
| Inertial frequency (cpd) | 0.6316 | 0.6495 | 0.6139 |
| Range of ADCP | 24–96 m | 24–120 m | 24–104 m |
| Range of thermometers | 100–1500 m | 100–1600 m | 100–1500 m |
| Observation period | 9/1–10/3 | 9/2–10/1 | 9/6–10/14 |
| Mooring | M1 | M2 | M3 | |
|---|---|---|---|---|
| Frequency (f) | 0.95 | 1.03 | 0.98 | |
| e-folding time (days) | 4~7 | 5~6 | 11~13 | |
| Phase speed (m/h) | upward | NA 1 | 3.18 | 4.58 |
| downward | 3.53 | 1.08 | 2.69 | |
| Wavelength (m) | upward | NA1 | 118 | 179 |
| downward | 134 | 40 | 105 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, B.; Hu, P.; Hou, Y. Observed Near-Inertial Waves in the Northern South China Sea. Remote Sens. 2021, 13, 3223. https://doi.org/10.3390/rs13163223
Yang B, Hu P, Hou Y. Observed Near-Inertial Waves in the Northern South China Sea. Remote Sensing. 2021; 13(16):3223. https://doi.org/10.3390/rs13163223
Chicago/Turabian StyleYang, Bing, Po Hu, and Yijun Hou. 2021. "Observed Near-Inertial Waves in the Northern South China Sea" Remote Sensing 13, no. 16: 3223. https://doi.org/10.3390/rs13163223
APA StyleYang, B., Hu, P., & Hou, Y. (2021). Observed Near-Inertial Waves in the Northern South China Sea. Remote Sensing, 13(16), 3223. https://doi.org/10.3390/rs13163223







