Geomagnetic Activity at Lampedusa Island: Characterization and Comparison with the Other Italian Observatories, Also in Response to Space Weather Events
Abstract
:1. Introduction
2. Materials
3. Methods and Results
3.1. Secular Variation
3.2. Diurnal Variation and Its Seasonal Dependence
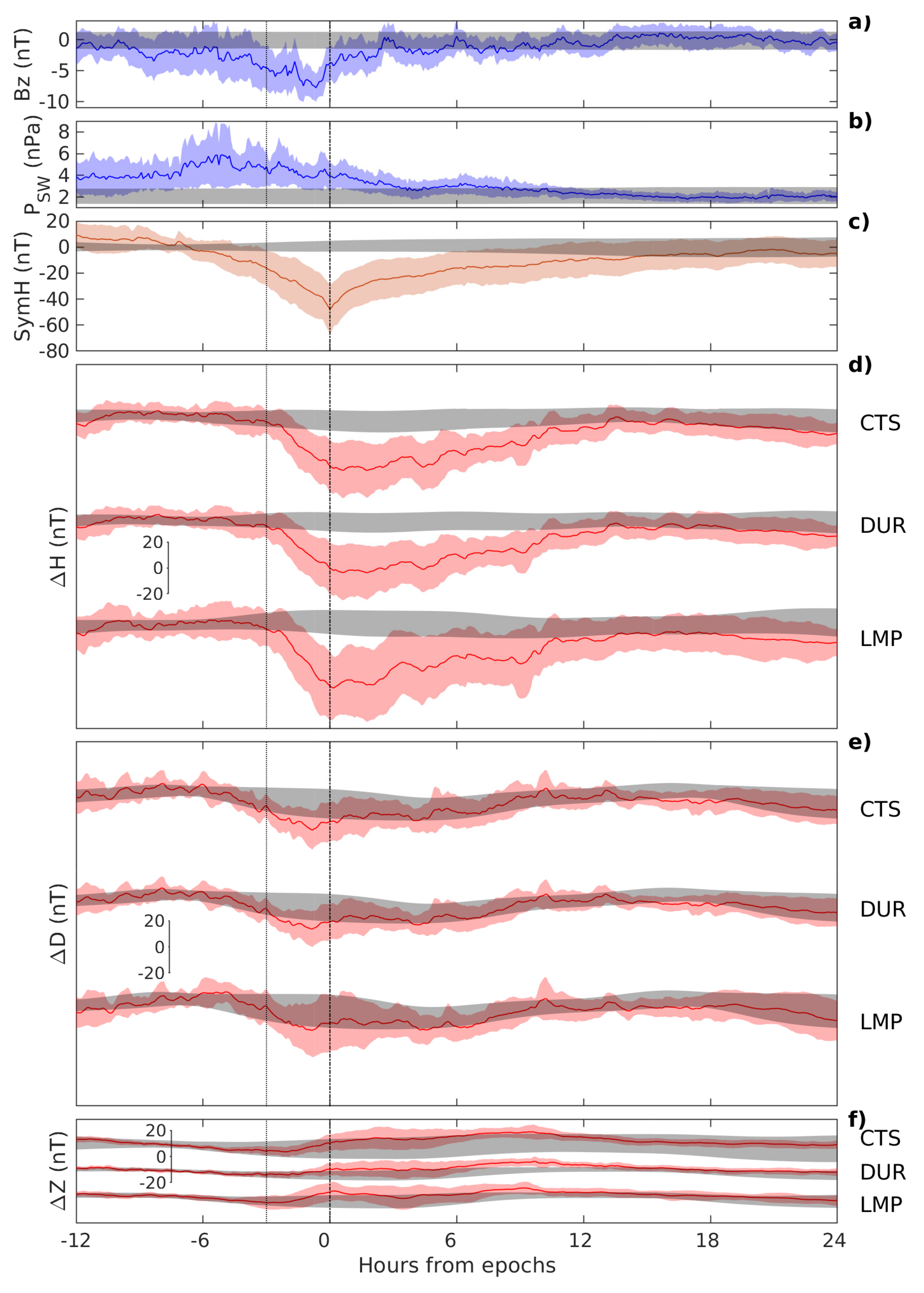
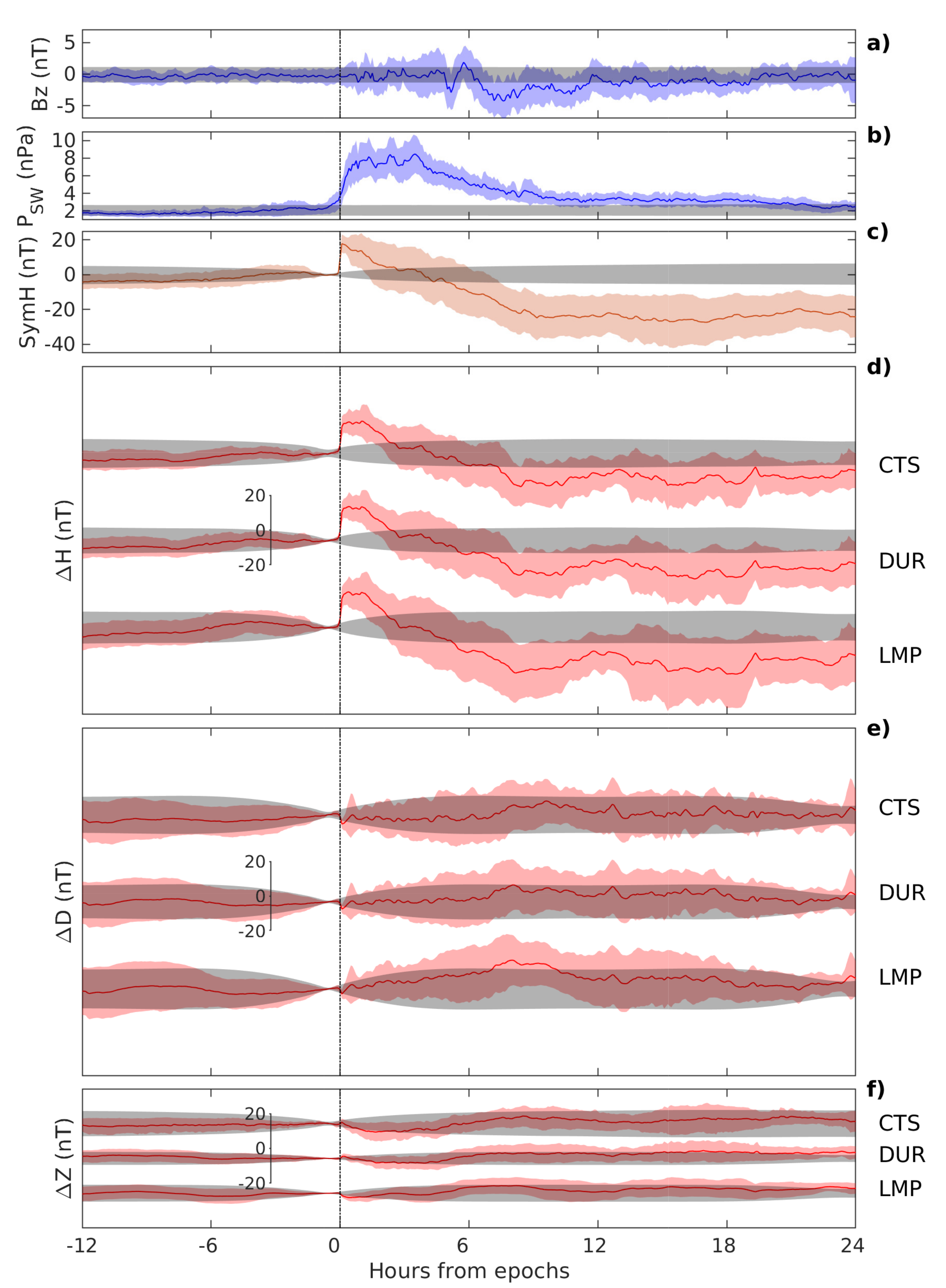
3.3. Geomagnetic Field Variations at Italian Observatories during Intense Space Weather Events
- For consistency with OMNI data, the geomagnetic field variations are resampled at 5 min time resolution;
- The linear trends of geomagnetic field variations are computed over the years 2017–2019 considering only the time intervals 1–4 LT, which represent the nominal minimum of geomagnetic activity, and subtracted the entire time series of magnetic field measurements, obtaining a detrended time series;
- The diurnal variation, as well as any shorter period variation, is removed by subtracting the moving average computed over 3 days from the time series.
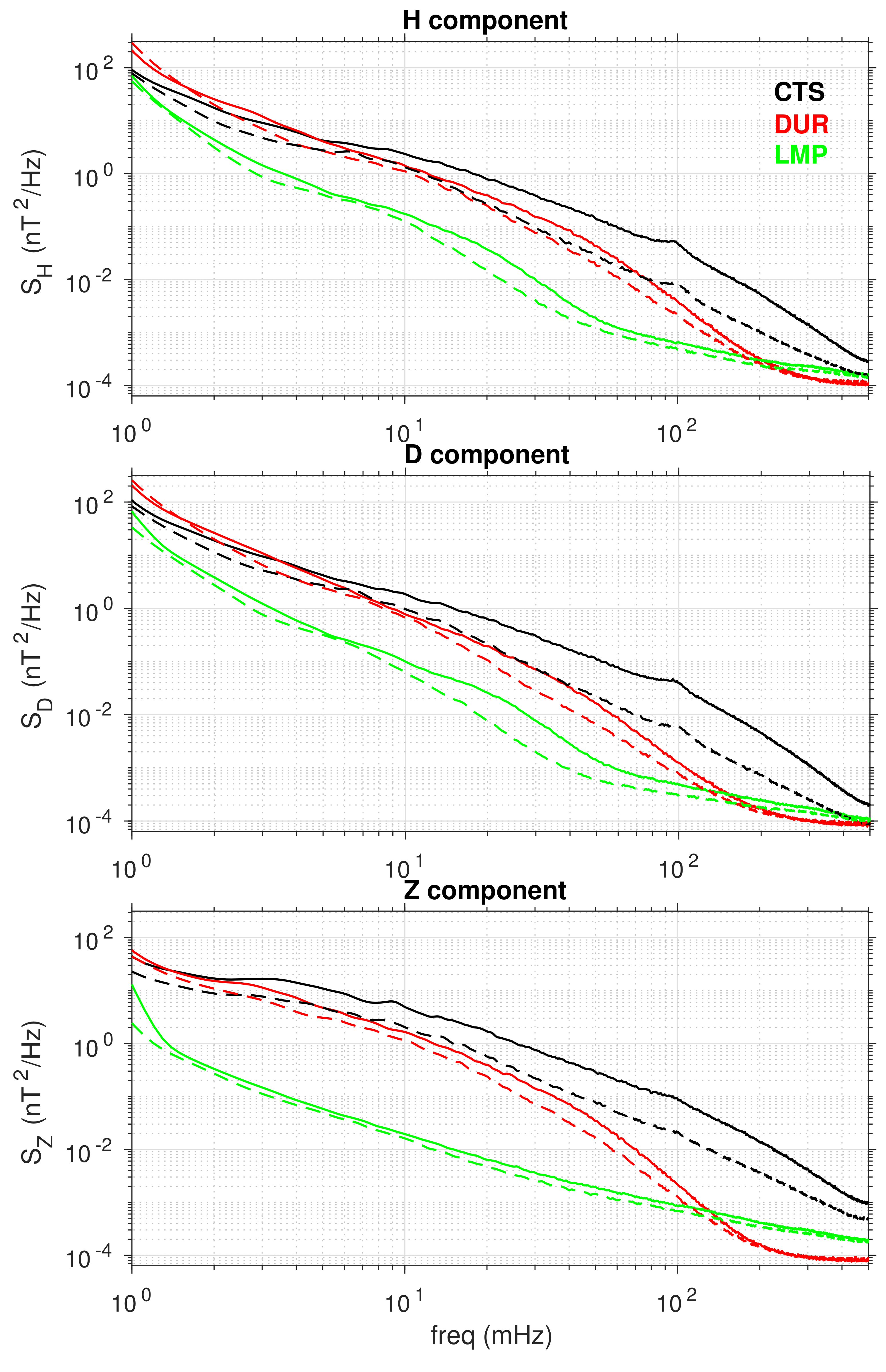
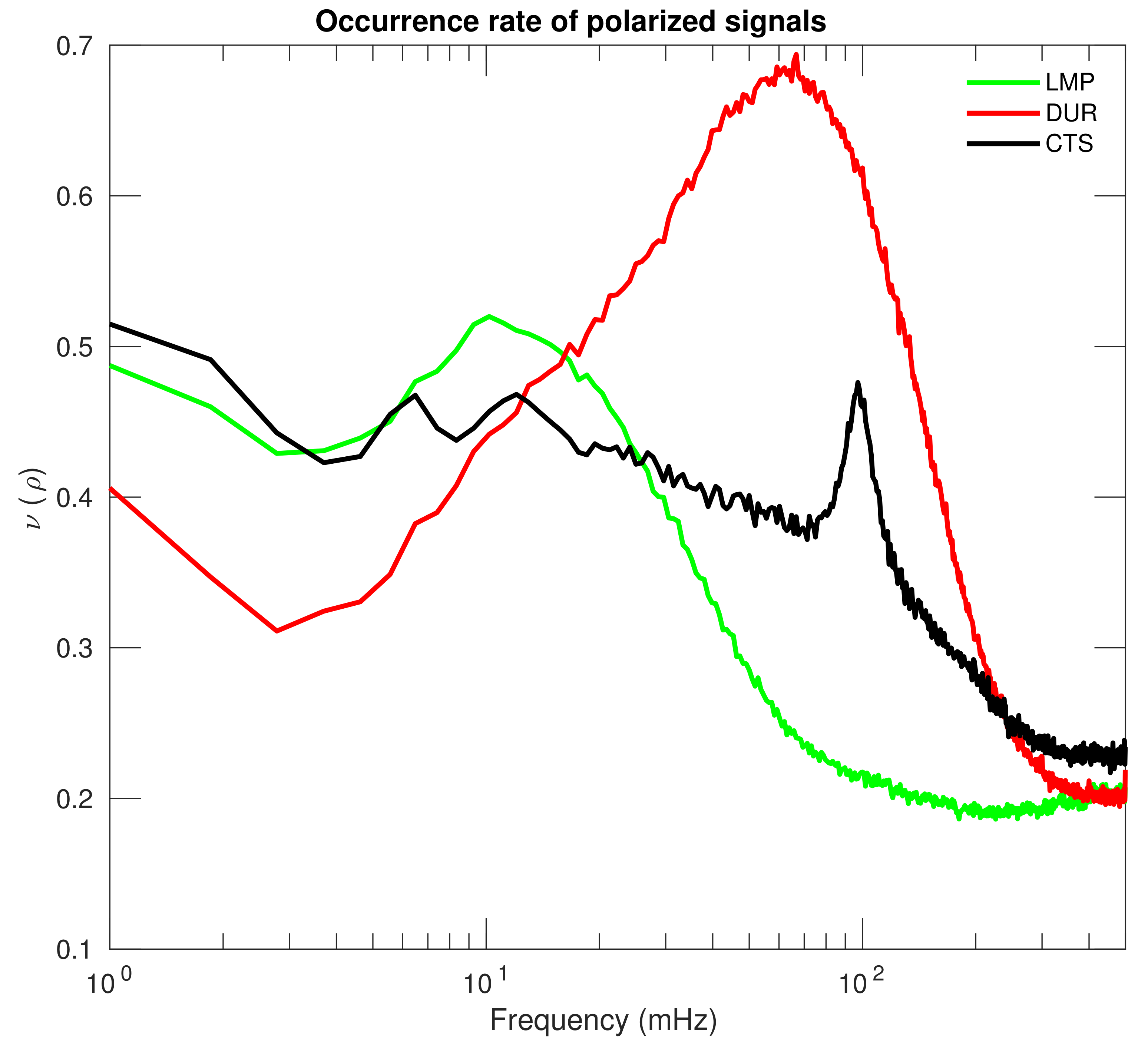
3.4. Spectral Characteristics
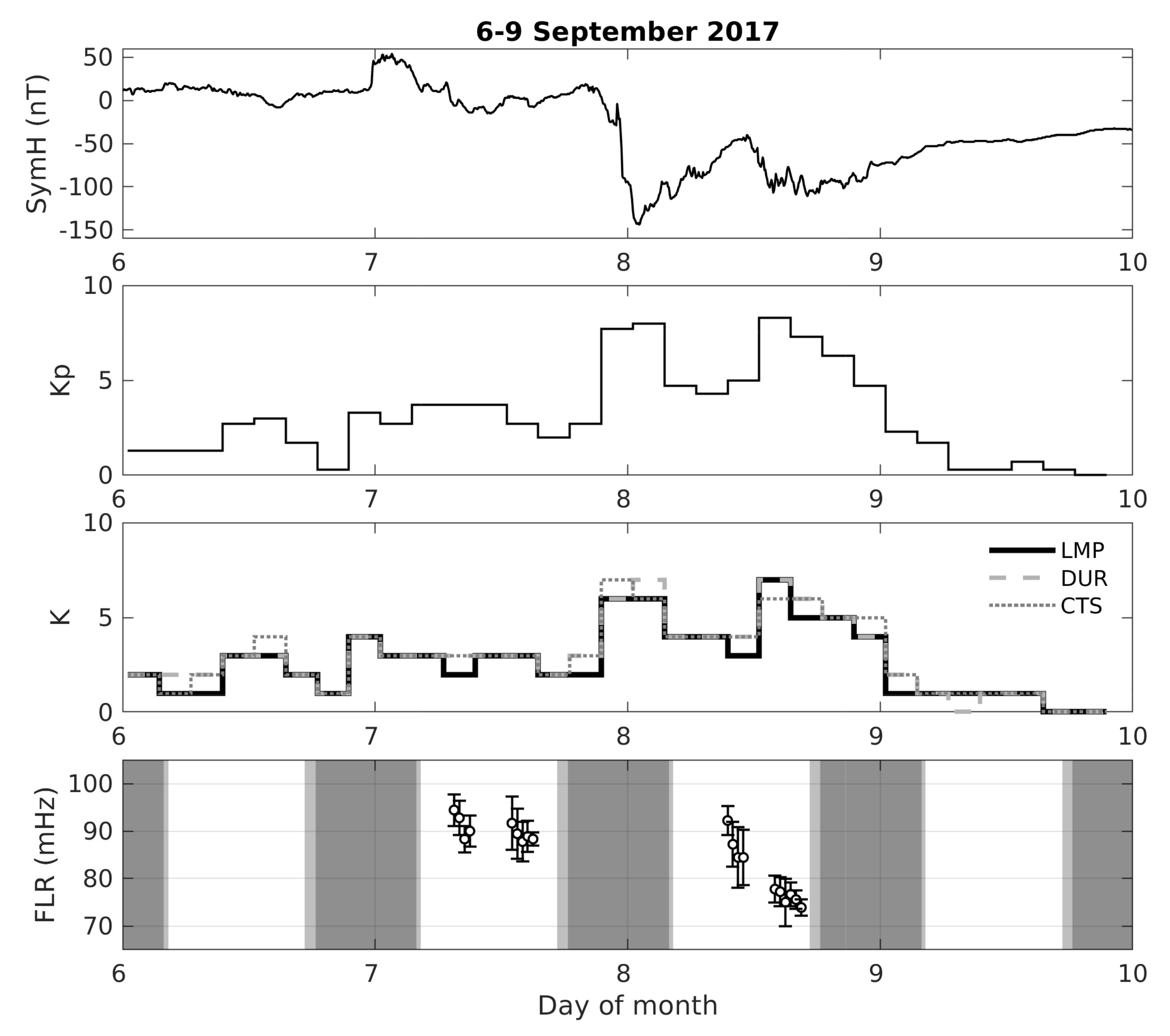
3.5. Observation of Field Line Resonances
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AACGM | Altitude-Adjusted Corrected Geomagnetic (coordinates) |
| AQU | L’Aquila observatory |
| CME | Coronal Mass Ejection |
| CTS | Castello Tesino observatory |
| DUR | Duronia observatory |
| EMIC | Electromagnetic Ion Cyclotron (waves) |
| EMMA | European quasi-Meridional Magnetometer Array |
| FAC | Field Aligned Current |
| FLR | Field Line Resonance |
| GNSS | Global Navigation Satellite System |
| IGRF | International Geomagnetic Reference Field model |
| IMF | Interplanetary Magnetic Field |
| LMP | Lampedusa observatory |
| MHD | Magnetohydrodynamic |
| MLT | Magnetic Local Time |
| Pc | Continuous Pulsation |
| Pi | Irregular Pulsation |
| SEA | Superposed Epoch Analysis |
| SEM | Standard Error of the Mean |
| SNR | Signal-to-Noise Ratio |
| SSC | Sudden Storm Commencement |
| SI | Sudden Impulse |
| SW | Solar Wind |
| TAD | Traveling Atmospheric Disturbance |
| TID | Traveling Ionospheric Disturbance |
| ULF | Ultra Low Frequency |
References
- Bullard, E.C.; Freedman, C.; Gellman, H.; Nixon, J. The westward drift of the Earth’s magnetic field. Philos. Trans. R. Soc. Lond. Ser. Math. Phys. Sci. 1950, 243, 67–92. [Google Scholar] [CrossRef]
- Newitt, L.R.; Chulliat, A.; Orgeval, J.J. Location of the North Magnetic Pole in April 2007. Earth Planets Space 2009, 61, 703–710. [Google Scholar] [CrossRef] [Green Version]
- Regi, M.; Di Mauro, D.; Lepidi, S. The Location of the Earth’s Magnetic Poles From Circum-Terrestrial Observations. J. Geophys. Res. Space Phys. 2021, 126, e2020JA028513:1–e2020JA028513:19. [Google Scholar] [CrossRef]
- Gubbins, D. Convection in the Earth’s Core and Mantle—Harold Jeffreys Lecture 1990. Q. J. R. Astron. Soc. 1991, 32, 69. [Google Scholar]
- Lanza, R.; Meloni, A. The Earth’s Magnetism: An Introduction for Geologists; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar] [CrossRef]
- Akasofu, S.I. A Review of Studies of Geomagnetic Storms and Auroral/Magnetospheric Substorms Based on the Electric Current Approach. Front. Astron. Space Sci. 2021, 7, 604750:1–604750:20. [Google Scholar] [CrossRef]
- Zakharenkova, I.; Astafyeva, E.; Cherniak, I. GPS and in situ Swarm observations of the equatorial plasma density irregularities in the topside ionosphere. Earth Planets Space 2016, 68, 120:1–120:11. [Google Scholar] [CrossRef] [Green Version]
- National Research Council. Severe Space Weather Events: Understanding Societal and Economic Impacts: A Workshop Report; The National Academies Press: Washington, DC, USA, 2008. [CrossRef]
- MacAlester, M.H.; Murtagh, W. Extreme Space Weather Impact: An Emergency Management Perspective. Space Weather 2014, 12, 530–537. [Google Scholar] [CrossRef]
- Jacobs, J.A. Geomagnetic Micropulsations; Physics and Chemistry in Space; Springer: Berlin/Heidelberg, Germany, 1970. [Google Scholar] [CrossRef]
- Menk, F.W. Magnetospheric ULF Waves: A Review. In The Dynamic Magnetosphere; Liu, W., Fujimoto, M., Eds.; Springer: Dordrecht, The Netherlands, 2011; pp. 223–256. [Google Scholar] [CrossRef]
- Kivelson, M.G.; Zu-Yin, P. The Kelvin-Helmholtz instability on the magnetopause. Planet. Space Sci. 1984, 32, 1335–1341. [Google Scholar] [CrossRef]
- Takahashi, K.; Ukhorskiy, A.Y. Timing analysis of the relationship between solar wind parameters and geosynchronous Pc5 amplitude. J. Geophys. Res. Space Phys. 2008, 113, A12204:1–A12204:13. [Google Scholar] [CrossRef] [Green Version]
- De Lauretis, M.; Regi, M.; Francia, P.; Marcucci, M.F.; Amata, E.; Pallocchia, G. Solar wind-driven Pc5 waves observed at a polar cap station and in the near cusp ionosphere. J. Geophys. Res. Space Phys. 2016, 121, 11145–11156. [Google Scholar] [CrossRef]
- Regi, M.; De Lauretis, M.; Francia, P. The occurrence of upstream waves in relation with the solar wind parameters: A statistical approach to estimate the size of the foreshock region. Planet. Space Sci. 2014, 90, 100–105. [Google Scholar] [CrossRef]
- Altman, C.; Fijalkow, E. Mechanism of Transmission of Hydromagnetic Waves through the Earth’s Lower Ionosphere. Nature 1968, 220, 53–55. [Google Scholar] [CrossRef]
- Kim, H.; Lessard, M.R.; Engebretson, M.J.; Young, M.A. Statistical study of Pc1–2 wave propagation characteristics in the high-latitude ionospheric waveguide. J. Geophys. Res. Space Phys. 2011, 116, A07227:1–A07227:20. [Google Scholar] [CrossRef] [Green Version]
- Regi, M.; Marzocchetti, M.; Francia, P.; De Lauretis, M. A statistical analysis of Pc1–2 waves at a near-cusp station in Antarctica. Earth Planets Space 2017, 69, 152:1–152:16. [Google Scholar] [CrossRef] [Green Version]
- Seppälä, A.; Matthes, K.; Randall, C.E.; Mironova, I.A. What is the solar influence on climate? Overview of activities during CAWSES-II. Prog. Earth Planet. Sci. 2014, 1, 24:1–24:12. [Google Scholar] [CrossRef] [Green Version]
- Tinsley, B.A. The global atmospheric electric circuit and its effects on cloud microphysics. Rep. Prog. Phys. 2008, 71, 066801:1–066801:31. [Google Scholar] [CrossRef]
- Lam, M.M.; Tinsley, B.A. Solar wind-atmospheric electricity-cloud microphysics connections to weather and climate. J. Atmos. Sol. Terr. Phys. 2016, 149, 277–290. [Google Scholar] [CrossRef] [Green Version]
- Alfonsi, L.; Cesaroni, C.; Spogli, L.; Regi, M.; Paul, A.; Ray, S.; Lepidi, S.; Di Mauro, D.; Haralambous, H.; Oikonomou, C.; et al. Ionospheric disturbances over the Indian sector during 8 September 2017 geomagnetic storm: Plasma structuring and propagation. Space Weather 2021, 19, e2020SW002607:1–e2020SW002607:16. [Google Scholar] [CrossRef]
- Spogli, L.; Sabbagh, D.; Regi, M.; Cesaroni, C.; Perrone, L.; Alfonsi, L.; Di Mauro, D.; Lepidi, S.; Campuzano, S.A.; Marchetti, D.; et al. Ionospheric Response Over Brazil to the August 2018 Geomagnetic Storm as Probed by CSES-01 and Swarm Satellites and by Local Ground-Based Observations. J. Geophys. Res. Space Phys. 2021, 126, e2020JA028368:1–e2020JA028368:16. [Google Scholar] [CrossRef]
- Pawlowicz, R. M_Map: A mapping Package for MATLAB, Computer Software. 2020. Available online: http://www.eoas.ubc.ca/~rich/map.html (accessed on 4 August 2021).
- US National Geophysical Data Center (NGDC); National Environmental Satellite, Data, and Information Service (NESDIS); National Oceanic and Atmospheric Administration (NOAA); U.S. Department of Commerce. TerrainBase, Global 5 Arc-minute Ocean Depth and Land Elevation from the US National Geophysical Data Center (NGDC); National Center for Atmospheric Research: Boulder, CO, USA, 1995.
- McIlwain, C.E. Coordinates for mapping the distribution of magnetically trapped particles. J. Geophys. Res. 1961, 66, 3681–3691. [Google Scholar] [CrossRef]
- Shepherd, S.G. Altitude-adjusted corrected geomagnetic coordinates: Definition and functional approximations. J. Geophys. Res. (Space Phys.) 2014, 119, 7501–7521. [Google Scholar] [CrossRef]
- Civile, D.; Lodolo, E.; Tortorici, L.; Lanzafame, G.; Brancolini, G. Relationships between magmatism and tectonics in a continental rift: The Pantelleria Island region (Sicily Channel, Italy). Mar. Geol. 2008, 251, 32–46. [Google Scholar] [CrossRef]
- Civile, D.; Lodolo, E.; Alp, H.; Ben-Avraham, Z.; Cova, A.; Baradello, L.; Accettella, D.; Burca, M.; Centonze, J. Seismic stratigraphy and structural setting of the Adventure Plateau (Sicily Channel). Mar. Geophys. Res. 2014, 35, 37–53. [Google Scholar] [CrossRef]
- Iyemori, T.; Takeda, M.; Nose, M.; Odagi, Y.; Toh, H. Mid-latitude Geomagnetic Indices ASY and SYM for 2009 (Provisional): Internal Report of Data Analysis Center for Geomagnetism and Space Magnetism; Kyoto University: Kyoto, Japan, 2010. [Google Scholar]
- Bartels, J. The geomagnetic measures for the time-variations of solar corpuscular radiation, described for use in correlation studies in other geophysical fields. Ann. Intern. Geophys. 2010, 4, 227–236. [Google Scholar]
- Nowozyński, K.; Ernst, T.; Jankowski, J. Adaptive smoothing method for computer derivation of K-indices. Geophys. J. Int. 1991, 104, 85–93. [Google Scholar] [CrossRef] [Green Version]
- Regi, M.; Bagiacchi, P.; Di Mauro, D.; Lepidi, S.; Cafarella, L. On the validation of K-index values at Italian geomagnetic observatories. Geosci. Instrum. Methods Data Syst. 2020, 9, 105–115. [Google Scholar] [CrossRef] [Green Version]
- Laken, B.A.; Čalogović, J. Composite analysis with Monte Carlo methods: An example with cosmic rays and clouds. J. Space Weather Space Clim. 2013, 3, A29:1–A29:13. [Google Scholar] [CrossRef]
- Regi, M.; Del Corpo, A.; De Lauretis, M. The use of the empirical mode decomposition for the identification of mean field aligned reference frames. Ann. Geophys. 2016, 59, G0651:1–G0651:16. [Google Scholar] [CrossRef]
- Regi, M.; Redaelli, G.; Francia, P.; De Lauretis, M. ULF geomagnetic activity effects on tropospheric temperature, specific humidity, and cloud cover in Antarctica, during 2003–2010. J. Geophys. Res. Atmos. 2017, 122, 6488–6501. [Google Scholar] [CrossRef]
- Regi, M.; De Lauretis, M.; Francia, P.; Lepidi, L.; Piancatelli, A.; Urbini, S. The geomagnetic coast effect at two 80°S stations in Antarctica, observed in the ULF range. Ann. Geophys. 2018, 36, 193–203. [Google Scholar] [CrossRef] [Green Version]
- Chree, C. Some Phenomena of Sunspots and of Terrestrial Magnetism at Kew Observatory. Philos. Trans. R. Soc. Lond. Ser. 1913, 212, 75–116. [Google Scholar] [CrossRef]
- Campbell, W.H. Introduction to Geomagnetic Fields, 2nd ed.; Cambridge University Press: Cambridge, UK, 2003. [Google Scholar]
- Kivelson, M.G.; Russell, C.T. Introduction to Space Physics; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Lockwood, M.; Sandholt, P.E.; Cowley, S.W.H.; Oguti, T. Interplanetary magnetic field control of dayside auroral activity and the transfer of momentum across the dayside magnetopause. Planet. Space Sci. 1989, 37, 1347–1365. [Google Scholar] [CrossRef]
- Shue, J.H.; Song, P.; Russell, C.T.; Steinberg, J.T.; Chao, J.K.; Zastenker, G.; Vaisberg, O.L.; Kokubun, S.; Singer, H.J.; Detman, T.R.; et al. Magnetopause location under extreme solar wind conditions. J. Geophys. Res. Space Phys. 1998, 103, 17691–17700. [Google Scholar] [CrossRef]
- Peredo, M.; Slavin, J.A.; Mazur, E.; Curtis, S.A. Three-dimensional position and shape of the bow shock and their variation with Alfvénic, sonic and magnetosonic Mach numbers and interplanetary magnetic field orientation. J. Geophys. Res. Space Phys. 1995, 100, 7907–7916. [Google Scholar] [CrossRef]
- Li, H.; Wang, C.; Kan, J.R. Contribution of the partial ring current to the SYMH index during magnetic storms. J. Geophys. Res. Space Phys. 2011, 116, A11222:1–A11222:12. [Google Scholar] [CrossRef] [Green Version]
- Sugiura, M.; Kamei, T. Equatorial Dst Index 1957–1986; Number 40 in IAGA Bulletin; ISGI Publications Office: Paris, Italy, 1991. [Google Scholar]
- Francia, P.; Regi, M.; De Lauretis, M.; Villante, U.; Pilipenko, V.A. A case study of upstream wave transmission to the ground at polar and low latitudes. J. Geophys. Res. Space Phys. 2012, 117, A01210:1–A01210:14. [Google Scholar] [CrossRef]
- Regi, M.; Francia, P.; De Lauretis, M.; Glassmeier, K.H.; Villante, U. Coherent transmission of upstream waves to polar latitudes through magnetotail lobes. J. Geophys. Res. Space Phys. 2013, 118, 6955–6963. [Google Scholar] [CrossRef]
- Southwood, D.J. The hydromagnetic stability of the magnetospheric boundary. Planet. Space Sci. 1968, 16, 587–605. [Google Scholar] [CrossRef]
- Regi, M.; De Lauretis, M.; Francia, P. Pc5 geomagnetic fluctuations in response to solar wind excitation and their relationship with relativistic electron fluxes in the outer radiation belt. Earth Planets Space 2015, 67, 9:1–9:9. [Google Scholar] [CrossRef] [Green Version]
- Kepko, L. Observations of discrete, global magnetospheric oscillations directly driven by solar wind density variations. J. Geophys. Res. Space Phys. 2003, 108, 1257:1–1257:13. [Google Scholar] [CrossRef] [Green Version]
- Villante, U.; Francia, P.; Lepidi, S.; De Lauretis, M.; Pietropaolo, E.; Cafarella, L.; Meloni, A.; Lazarus, A.J.; Lepping, R.P.; Mariani, F. Geomagnetic field variations at low and high latitude during the 10–11 January 1997 magnetic cloud. Geophys. Res. Lett. 1998, 25, 2593–2596. [Google Scholar] [CrossRef] [Green Version]
- Ponomarenko, P.V.; Fraser, B.J.; Menk, F.W.; Ables, S.T.; Morris, R.J. Cusp-latitude Pc3 spectra: Band-limited and power-law components. Ann. Geophys. 2002, 20, 1539–1551. [Google Scholar] [CrossRef] [Green Version]
- De Lauretis, M.; Francia, P.; Regi, M.; Villante, U.; Piancatelli, A. Pc3 pulsations in the polar cap and at low latitude. J. Geophys. Res. Space Phys. 2010, 115, A11223:1–A11223:10. [Google Scholar] [CrossRef] [Green Version]
- Greenstadt, E.W.; McPherron, R.L.; Takahashi, K. Solar Wind Control of Daytime, Midperiod Geomagnetic Pulsations. J. Geomagn. Geoelectr. 1980, 32, SII89–SII110. [Google Scholar] [CrossRef] [Green Version]
- Russell, C.T.; Luhmann, J.G.; Odera, T.J.; Stuart, W.F. The rate of occurrence of dayside Pc 3,4 pulsations: The L-value dependence of the IMF cone angle effect. Geophys. Res. Lett. 1983, 10, 663–666. [Google Scholar] [CrossRef]
- Fowler, R.A.; Kotick, B.J.; Elliott, R.D. Polarization analysis of natural and artificially induced geomagnetic micropulsations. J. Geophys. Res. 1967, 72, 2871–2883. [Google Scholar] [CrossRef]
- Southwood, D. Some features of field line resonances in the magnetosphere. Planet. Space Sci. 1974, 22, 483–491. [Google Scholar] [CrossRef]
- Allan, W.; Knox, F. A dipole field model for axisymmetric alfvén waves with finite ionosphere conductivities. Planet. Space Sci. 1979, 27, 79–85. [Google Scholar] [CrossRef]
- Takahashi, K.; Vellante, M.; Del Corpo, A.; Claudepierre, S.G.; Kletzing, C.; Wygant, J.; Koga, K. Multiharmonic Toroidal Standing Alfvén Waves in the Midnight Sector Observed During a Geomagnetically Quiet Period. J. Geophys. Res. Space Phys. 2020, 125, e2019JA027370:1–e2019JA027370:20. [Google Scholar] [CrossRef]
- Chi, P.J.; Engebretson, M.J.; Moldwin, M.B.; Russell, C.T.; Mann, I.R.; Samson, J.C.; López Cruz-Abeyro, J.A.; Yumoto, K.; Lee, D.H. Mid-continent Magnetoseismic Chain (McMAC): A Meridional Magnetometer Chain for Magnetospheric Sounding. In Proceedings of the Enviroment Modeling Workshop, Monterey, CA, USA, 1–3 June 2005; pp. 17–22. [Google Scholar]
- Mann, I.; Milling, D.; Rae, I.J.; Ozeke, L.; Kale, A.; Kale, Z.; Murphy, K.; Parent, A.; Usanova, M.; Pahud, D.; et al. The upgraded CARISMA magnetometer array in the THEMIS era. Space Sci. Rev. 2008, 141, 413–451. [Google Scholar] [CrossRef]
- Lichtenberger, J.; Clilverd, M.A.; Heilig, B.; Vellante, M.; Manninen, J.; Rodger, C.J.; Collier, A.B.; Jørgensen, A.M.; Reda, J.; Holzworth, R.H.; et al. The plasmasphere during a space weather event: First results from the PLASMON project. J. Space Weather Space Clim. 2013, 3, A23:1–A23:13. [Google Scholar] [CrossRef] [Green Version]
- Singer, H.; Southwood, D.; Walker, R.; Kivelson, M. Alfvén wave resonances in a realistic magnetospheric magnetic field geometry. J. Geophys. Res. Space Phys. 1981, 86, 4589–4596. [Google Scholar] [CrossRef] [Green Version]
- Baransky, L.; Borovkov, J.; Gokhberg, M.; Krylov, S.; Troitskaya, V. High resolution method of direct measurement of the magnetic field lines’ eigen frequencies. Planet. Space Sci. 1985, 33, 1369–1374. [Google Scholar] [CrossRef]
- Waters, C.L.; Menk, F.W.; Fraser, B.J. The resonance structure of low latitude Pc3 geomagnetic pulsations. Geophys. Res. Lett. 1991, 18, 2293–2296. [Google Scholar] [CrossRef] [Green Version]
- Kale, Z.C.; Mann, I.R.; Waters, C.L.; Goldstein, J.; Menk, F.W.; Ozeke, L.G. Ground magnetometer observation of a cross-phase reversal at a steep plasmapause. J. Geophys. Res. Space Phys. 2007, 112, A10222:1–A10222:9. [Google Scholar] [CrossRef] [Green Version]
- Menk, F.W.; Waters, C.L.; Fraser, B.J. Field line resonances and waveguide modes at low latitudes: 1. Observations. J. Geophys. Res. Space Phys. 2000, 105, 7747–7761. [Google Scholar] [CrossRef]
- Kawano, H.; Yumoto, K.; Pilipenko, V.A.; Tanaka, Y.M.; Takasaki, S.; Iizima, M.; Seto, M. Using two ground stations to identify magnetospheric field line eigenfrequency as a continuous function of ground latitude. J. Geophys. Res. Space Phys. 2002, 107, SMP25-1–SMP25-12. [Google Scholar] [CrossRef]
- Chi, P.J.; Engebretson, M.J.; Moldwin, M.B.; Russell, C.T.; Mann, I.R.; Hairston, M.R.; Reno, M.; Goldstein, J.; Winkler, L.I.; Cruz-Abeyro, J.L.; et al. Sounding of the plasmasphere by Mid-continent MAgnetoseismic Chain (McMAC) magnetometers. J. Geophys. Res. Space Phys. 2013, 118, 3077–3086. [Google Scholar] [CrossRef]
- Del Corpo, A.; Vellante, M.; Heilig, B.; Pietropaolo, E.; Reda, J.; Lichtenberger, J. Observing the cold plasma in the Earth’s magnetosphere with the EMMA network. Ann. Geophys. 2019, 62, GM447:1–GM447:19. [Google Scholar] [CrossRef]
- Chi, P.J.; Russell, C.T. An interpretation of the cross-phase spectrum of geomagnetic pulsations by the field line resonance theory. Geophys. Res. Lett. 1998, 25, 4445–4448. [Google Scholar] [CrossRef]
- Del Corpo, A.; Vellante, M.; Heilig, B.; Pietropaolo, E.; Reda, J.; Lichtenberger, J. An Empirical Model for the Dayside Magnetospheric Plasma Mass Density Derived From EMMA Magnetometer Network Observations. J. Geophys. Res. Space Phys. 2020, 125, e2019JA027381:1–e2019JA027381:19. [Google Scholar] [CrossRef]
- Yamazaki, Y.; Maute, A. Sq and EEJ—A review on the daily variation of the geomagnetic field caused by ionospheric dynamo currents. Space Sci. Rev. 2017, 206, 299–405. [Google Scholar] [CrossRef] [Green Version]
- Parkinson, W.D.; Jones, F.W. The geomagnetic coast effect. Rev. Geophys. 1979, 17, 1999–2015. [Google Scholar] [CrossRef]
- Hitchman, A.P.; Milligan, P.R.; Lilley, F.T.; White, A.; Heinson, G.S. The total-field geomagnetic coast effect: The CICADA97 line from deep Tasman Sea to inland New South Wales. Explor. Geophys. 2000, 31, 52–57. [Google Scholar] [CrossRef] [Green Version]
- Borovsky, J.E.; Denton, M.H. Differences between CME-driven storms and CIR-driven storms. J. Geophys. Res. 2006, 111, A07S08:1–A07S08:17. [Google Scholar] [CrossRef] [Green Version]
- Pulkkinen, A.; Lindahl, S.; Viljanen, A.; Pirjola, R. Geomagnetic storm of 29–31 October 2003: Geomagnetically induced currents and their relation to problems in the Swedish high-voltage power transmission system. Space Weather 2005, 3, S08C03:1–S08C03:19. [Google Scholar] [CrossRef]
- Tontini, F.C.; Stefanelli, P.; Giori, I.; Faggioni, O.; Carmisciano, C. The revised aeromagnetic anomaly map of Italy. Ann. Geophys. 2009, 47. [Google Scholar] [CrossRef]
- Green, A.W.; Worthington, E.W.; Baransky, L.N.; Fedorov, E.N.; Kurneva, N.A.; Pilipenko, V.A.; Shvetzov, D.N.; Bektemirov, A.A.; Philipov, G.V. Alfven field line resonances at low latitudes (L = 1.5). J. Geophys. Res. Space Phys. 1993, 98, 15693–15699. [Google Scholar] [CrossRef]
- Vellante, M.; Förster, M.; Villante, U.; Zhang, T.L.; Magnes, W. Solar activity dependence of geomagnetic field line resonance frequencies at low latitudes. J. Geophys. Res. Space Phys. 2007, 112, A02205:1–A02205:14. [Google Scholar] [CrossRef] [Green Version]
- Takahashi, K.; Denton, R.E.; Anderson, R.R.; Hughes, W.J. Frequencies of standing Alfvén wave harmonics and their implication for plasma mass distribution along geomagnetic field lines: Statistical analysis of CRRES data. J. Geophys. Res. Space Phys. 2004, 109, A08202:1–A08202:15. [Google Scholar] [CrossRef] [Green Version]
- Vellante, M.; Förster, M. Inference of the magnetospheric plasma mass density from field line resonances: A test using a plasmasphere model. J. Geophys. Res. Space Phys. 2006, 111, A11204:1–A11204:13. [Google Scholar] [CrossRef] [Green Version]
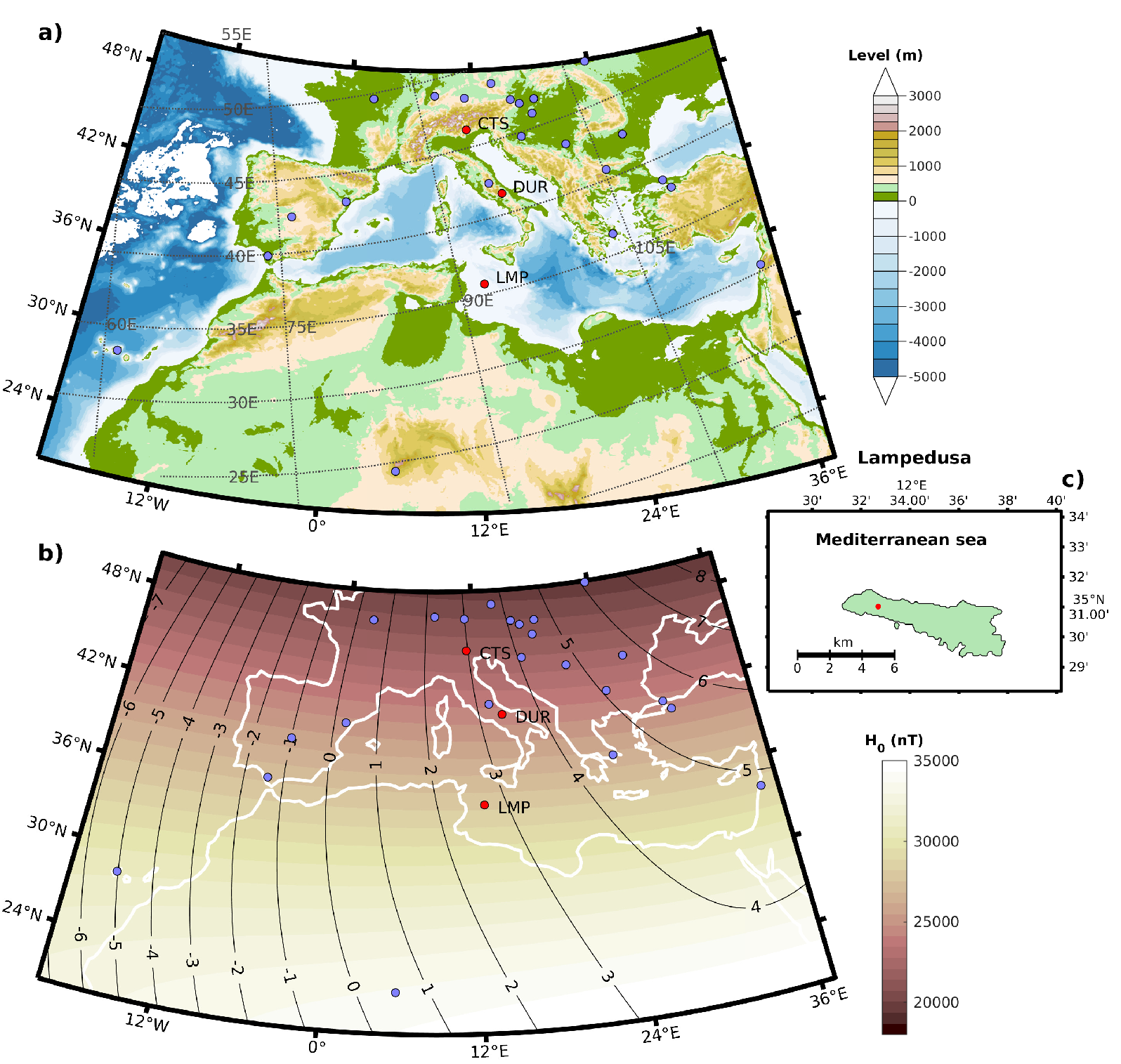
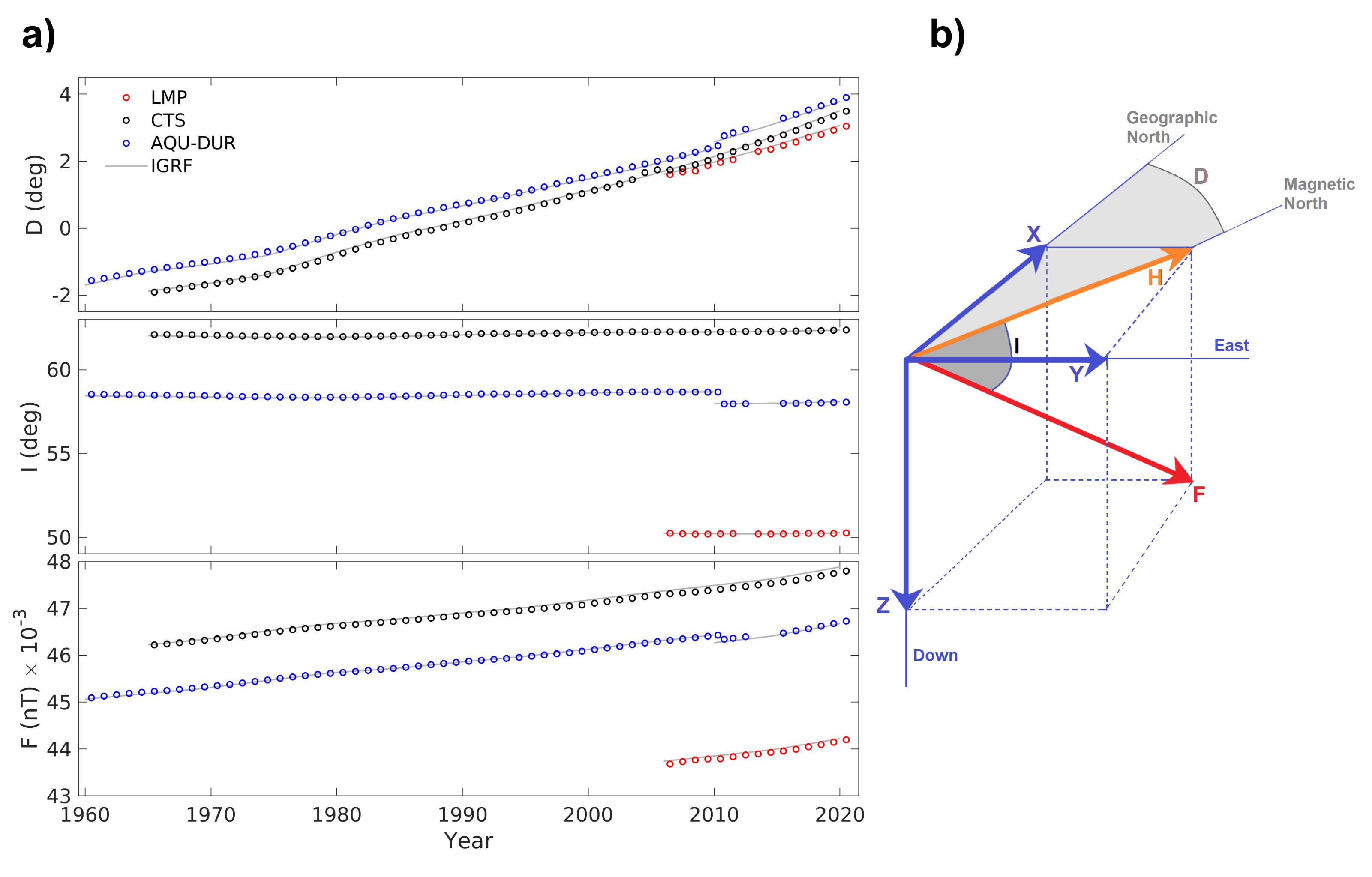




| IAGA CODE | Geographic Latitude (deg N) | Geographic Longitude (deg E) | AACGM Latitude (deg N) | AACGM Longitude (deg E) | L (Re) | MLT at 00 UT (hh:mm) |
|---|---|---|---|---|---|---|
| LMP | 35.52 | 12.55 | 28.10 | 86.00 | 1.28 | 00:29 |
| DUR | 41.65 | 14.47 | 36.09 | 88.45 | 1.53 | 00:40 |
| CTS | 46.05 | 11.39 | 41.31 | 86.44 | 1.78 | 00:31 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Di Mauro, D.; Regi, M.; Lepidi, S.; Del Corpo, A.; Dominici, G.; Bagiacchi, P.; Benedetti, G.; Cafarella, L. Geomagnetic Activity at Lampedusa Island: Characterization and Comparison with the Other Italian Observatories, Also in Response to Space Weather Events. Remote Sens. 2021, 13, 3111. https://doi.org/10.3390/rs13163111
Di Mauro D, Regi M, Lepidi S, Del Corpo A, Dominici G, Bagiacchi P, Benedetti G, Cafarella L. Geomagnetic Activity at Lampedusa Island: Characterization and Comparison with the Other Italian Observatories, Also in Response to Space Weather Events. Remote Sensing. 2021; 13(16):3111. https://doi.org/10.3390/rs13163111
Chicago/Turabian StyleDi Mauro, Domenico, Mauro Regi, Stefania Lepidi, Alfredo Del Corpo, Guido Dominici, Paolo Bagiacchi, Giovanni Benedetti, and Lili Cafarella. 2021. "Geomagnetic Activity at Lampedusa Island: Characterization and Comparison with the Other Italian Observatories, Also in Response to Space Weather Events" Remote Sensing 13, no. 16: 3111. https://doi.org/10.3390/rs13163111
APA StyleDi Mauro, D., Regi, M., Lepidi, S., Del Corpo, A., Dominici, G., Bagiacchi, P., Benedetti, G., & Cafarella, L. (2021). Geomagnetic Activity at Lampedusa Island: Characterization and Comparison with the Other Italian Observatories, Also in Response to Space Weather Events. Remote Sensing, 13(16), 3111. https://doi.org/10.3390/rs13163111






