Uncertainty Assessments of Load Deformation from Different GPS Time Series Products, GRACE Estimates and Model Predictions: A Case Study over Europe

Abstract

A good understanding of the accuracy of the Global Positioning System (GPS) surface displacements provided by different processing centers plays an important role in load deformation analysis. We estimate the noise level in both vertical and horizontal directions for four representative GPS time series products, and compare GPS results with load deformation derived from the Gravity Recovery and Climate Experiment (GRACE) gravity measurements and climate models in Europe. For the extracted linear trend signals, the differences among different GPS series are small in all the three (east, north, and up) directions, while for the annual signals the differences are large. The mean standard deviations of annual amplitudes retrieved from the four GPS series are 3.54 mm in the vertical component (69% of the signal itself) and ~ 0.3 mm in the horizontal component (30% of the signal itself). The Scripps Orbit and Permanent Array Center (SOPAC) and MEaSUREs series have the lowest noise level in vertical and horizontal directions, respectively. Through consistency/discrepancy analysis among GPS, GRACE, and model vertical series, we find that the Jet Propulsion Laboratory (JPL) and Nevada Geodetic Laboratory (NGL) series show good consistency, the SOPAC series show good agreements in annual signal with the GRACE and model, and the MEaSUREs series show substantially large annual amplitude. We discuss the possible reasons for the notable differences among GPS time series products.

1. Introduction

Surface load deformations are caused by mass redistributions in the geophysical fluids system, including the atmosphere, ocean, terrestrial water, ice sheets and glaciers, etc. With gradually increasing precision of the Global Positioning System (GPS), one of the most representative Global Navigation Satellite System (GNSS), surface deformation can be fairly accurately observed. In early studies, GPS measurements were combined with data from other geodetic techniques (e.g., satellite gravimetry, altimetry) to study large scale surface loads, such as global water cycling, and polar ice melting [1,2]. With dense and continuous observation sites, GPS can be used as an effective constraint or even an independent means to quantify the magnitude and spatial distribution of surface load [3,4]. A recent study by Knappe et al. [5] showed that GPS observations can be downscaled to reflect local load changes at scales of several tens of kilometers.

For load deformation studies, most researchers prefer directly using the surface displacement time series provided by GPS data centers rather than processing GPS observation data (e.g., pseudo-ranges or -phases). In previous studies, Fu and Freymueller [6] used observations from GPS stations installed by Caltech and other organizations to analyze load deformation in Nepal Himalaya, and Wahr et al. [3] used GPS three-dimensional daily time series provided by the Plate Boundary Observatory Analysis Centers (PBOACs) to study horizontal deformation in California. Besides these products from regional GPS station networks, some global network GPS post-processing time series products using different sorts of software are also used in load deformation studies, such as the Jet Propulsion Laboratory’s (JPL’s) GNSS-Inferred Positioning System (GIPSY) solutions, the Scripps Orbit and Permanent Array Center’s (SOPAC’s) GAMIT solutions, the MEaSUREs combination solutions using the JPL analyze_tserist_filter software package [7,8,9,10], etc. Recently, some new GPS time series products are also available, such as the Nevada Geodetic Laboratory (NGL) GPS series used in Han [11], the Crustal Movement Observation Network of China (CMONOC) used in Wang et al. [12], and the EUREF Permanent Network (EPN) used in Springer et al. [13], etc.

The magnitude of surface load deformation is at the millimeter level for both seasonal and long-term signals in the vertical direction, and even smaller in the horizontal direction, and the ratio of the vertical to the horizontal is ~ 2.0 to 3.0 [3,14]. The discrepancies among the currently available GPS time series products reach as large as several millimeters at some stations, which are at the same level as the load deformation signal, and are expected to affect load deformation analyses [15,16]. Gu et al. [17] quantified the vertical load deformations from multi-institution GPS solutions at some selected global sites, and found that the post-processing error of GPS data is the main reason for the differences among different time series products. However, for horizontal and regional GPS surface displacements, which also provide important mass load constraint especially for regional or local load study, the differences and their corresponding impact on load analysis need further study.

Besides, Kenyeres et al. [18] integrated the GNSS positions and velocities of the EPN stations and analyzed the quality of their homogenization. They focused on the evaluation of data processing approaches, such as mathematical testing and assessment of the alignment with the International Terrestrial Reference Frame (ITRF) position, rather than analysis of the contained geophysical signals in the time series. Martens et al. [19] applied a total root mean square (RMS) scatter to the five GPS series and discussed their overall quality in the contiguous USA and Alaska, and further explored the impacts of the different atmospheric models.

In this study, we chose four representative public GPS time series products, and carried out a comprehensive evaluation of the consistency/discrepancy among these solutions for both the vertical and horizontal components of surface displacements in the Europe region. The GPS results were compared with load deformations derived from the Gravity Recovery and Climate Experiment (GRACE) satellite gravity measurements and surface mass loads predicted by advanced atmospheric, oceanic, and hydrological models. Relative accuracies of the six load deformation results (four GPS series, GRACE, and models) were assessed using the Three-Cornered Hat (TCH) method. In-depth assessment of GPS measurement accuracy is important for understanding and interpreting load deformation and reconciling different GPS processing strategies.

2. Materials and Methods

2.1. GPS Time Series Products

We use the four three-dimensional daily GPS time series from JPL, SOPAC, MEaSUREs combination [20], and NGL [21]. As suggested by the International Global Navigation Satellite Systems Service (IGS), the nontidal load signals (from the atmosphere, ocean, and surface hydrology) are fully retained in these products, while the tidal effects are removed. To be comparable with GRACE time series, we select the GPS time series over the period January 2007 to June 2017, which cover the GRACE mission period and has a time span of 10 years. This data length is adequate to retrieve the annual signals as well as the linear trend in recent years.

We select 31 sites in the Europe region based on the data quality (with data gaps no longer than 1 month, and without obvious “jumps” due to receiver changing or tectonic motions). Compared with the sites used in Kenyeres et al. [18], as mentioned above, the number of the selected sites in this study is far less than theirs (several hundred) due to the limited number of sites to guarantee continuous data availability of the four selected GPS post-processing time series products. Despite the relatively smaller number of selected sites, the variety of GPS data processing software (described in the websites of products) and the generally uniform spatial distribution of our site selection well satisfy the uncertainty study of load deformation. Figure 1 shows the locations of the 31 GPS sites in Europe. The daily GPS height time series at four selected sites (marked with blue dots in Figure 1) are shown in Figure 2, displaying the surface height changes from the four products, with artificial offsets added (to each series) for clarity. Substantially large discrepancies among the four GPS series clearly exist, especially at seasonal time scales. The comparison for the other sites is provided in Figures S1 and S2 for conciseness.

In order to be comparable with the GRACE and model data (described later), the GPS daily series are averaged and re-adjusted to align at monthly temporal resolution through the following steps. We first fill the gaps using the method based on Singular Spectrum Analysis (SSA) introduced by Kondrashov et al. [22], and then apply a 31-day moving average filter and interpolate into monthly intervals afterwards.

2.2. GRACE Load Deformation

We use the GRACE Release 6 (RL06) mascon solutions from January 2007 to June 2017, provided by the Center for Space Research (CSR) at the University of Texas at Austin (http://www2.csr.utexas.edu/grace/RL06_mascons.html, accessed on 18 June 2020) [23]. In the CSR RL06 mascon solutions (named as “CSR GRACE/GRACE-FO RL06 v02 Mascon Grids w/ Corrections Applied”), the C20 and C30 coefficients are replaced by Satellite Laser Ranging estimates [24], and degree-1 coefficients (C11, S11, C10) are included using the geocenter series provided in the GRACE supplementary datasets (in GRACE Technical Note 13) [25,26]. The Glacial Isostatic Adjustment (GIA) effects have been removed using the ICE-6G_D (VM5a) model [27], and the GRACE GAD fields have been restored (so over the ocean, the mascon mass change represents ocean bottom pressure change). We remove the GAD contributions and add back the GAC contributions to make the GRACE results comparable with the GPS solutions (both including atmospheric, oceanic, and hydrological load effects).

To estimate the vertical displacements using GRACE mascon products, we first convert the ${0.5}^{°}\times {0.5}^{°}$ gridded mass distribution $dh\left(\theta ,\varphi \right)$ to fully normalized spherical harmonic (SH) Stokes coefficients up to degree and order 360, and then calculate the vertical load displacements $dr\left(\theta ,\varphi \right)$ at a given point (with the colatitude $\theta$ and longitude $\varphi$) using the following Equations (1)–(3) [28,29].

$$\{\begin{array}{c}{\widehat{C}}_{lm}=\frac{1}{\mathrm{R}}\times \frac{1}{4\mathsf{\pi }}{\iint }_{\sigma }dh\left(\theta ,\varphi \right){\tilde{P}}_{l,m}\left(\cos \theta \right)\cos \left(m\varphi \right)d\sigma \\ {\widehat{S}}_{lm}=\frac{1}{\mathrm{R}}\times \frac{1}{4\mathsf{\pi }}{\iint }_{\sigma }dh\left(\theta ,\varphi \right){\tilde{P}}_{l,m}\left(\cos \theta \right)\sin \left(m\varphi \right)d\sigma \end{array},$$

(1)

$$\left\{\begin{array}{c}{C}_{lm}\\ S_{lm}\end{array}\right\}=\frac{3{\rho }_w}{{\rho }_{ave}} \frac{1+k_l^{\text{'}}}{2l+1}\left\{\begin{array}{c}{\widehat{C}}_{lm}\\ {\widehat{S}}_{lm}\end{array}\right\},$$

(2)

$$dr\left(\theta ,\varphi \right)=\mathrm{R}{\displaystyle \sum }_{l=0}^{360}{\displaystyle \sum }_{m=0}^l{\tilde{P}}_{l,m}\left(\cos \theta \right)\left(C_{lm}\cos \left(m\varphi \right)+S_{lm}\sin \left(m\varphi \right)\right)\frac{h_l^{\text{'}}}{1+k_l^{\text{'}}},$$

(3)

where ${\widehat{C}}_{lm}$ and ${\widehat{S}}_{lm}$ are the dimensionless SH coefficients defined in Equation (9) of Wahr et al. [29], $\mathrm{R}$ is the mean radius of the Earth, ${\tilde{P}}_{l,m}$ are the normalized Legendre functions of degree $l$ and order $m$, $d\sigma$ is the area element (namely, $\sin \left(\theta \right)d\theta d\varphi$), ${\rho }_w$ is the density of water, ${\rho }_{ave}$ is the average density of the Earth, and $h_l^{\text{'}}$ and $k_l^{\text{'}}$ are the elastic load Love numbers of degree $l$ for the Preliminary reference Earth model (PREM) [30] given by Han and Wahr [31]. The GRACE series are also re-aligned into monthly intervals using the same method as applied to the GPS series (described in Section 2.1).

2.3. Climate Models

The total load is estimated by the sum of atmospheric, oceanic, and hydrological models, which are monthly mass changes of the non-tidal atmosphere and ocean from the Atmosphere and Ocean Level-1B (AOD1B) De-Aliasing GAC products in degree 180 Stokes coefficients [32], and monthly terrestrial water storage (TWS) changes from the Global Land Data Assimilation System (GLDAS) Noah land surface model (V2.1) [33]. Consistent with the processing steps for GRACE data in Section 2.2, the mass distributions from climate models are converted to the load displacements using Equations (1)–(3). Noteworthily, in order to consider the global mass conservation, we set the degree 0 of GAC Stokes coefficients (representing the global total mass change of the atmosphere and ocean) to zero, and add to the ocean the negative mean value of the total GLDAS mass change over continent (assuming the total mass change of TWS is from the ocean).

3. Results

3.1. Uncertainty Assessment of GPS Height Solutions

The annual components (amplitudes and phases) and linear trends of the four GPS height time series products at the 31 selected sites are obtained using least squares fitting are listed in

Table 1. Annual amplitudes (ann. amp.), annual phases (ann. pha.) and trends of the four height time series products at the 31 GPS sites.

Sites	JPL			SOPAC			MEaSUREs				NGL
	ann. amp.1	ann. pha.	trend	ann. amp.	ann. pha.	trend	ann. amp.	ann. pha.	trend	ann. amp.		ann. pha.	trend
acor	0.55	134.0	−2.03	2.99	231.8	−2.15	7.94	222.3	−2.44	1.01		168.2	−2.31
alme	1.48	117.3	-0.36	3.47	216.4	−0.65	7.60	213.8	−0.70	1.67		151.2	−0.73
bucu	4.61	218.9	1.85	7.29	231.3	1.56	13.01	220.0	1.51	5.06		217.2	1.43
ebre	1.43	139.1	−0.26	2.91	204.8	−0.39	8.10	212.6	−0.57	1.43		125.2	−1.58
geno	0.94	211.6	−0.41	4.51	230.5	−0.53	9.51	219.5	−0.45	2.00		208.4	−0.86
glsv	5.06	217.0	0.55	7.42	236.1	0.37	12.49	222.6	−0.01	5.90		215.2	0.01
gope	2.76	175.9	0.50	5.34	208.8	0.35	10.73	210.0	0.33	3.13		182.9	0.19
hert	1.23	186.7	−0.31	4.11	228.6	−0.42	9.29	220.8	−0.41	1.99		197.8	−0.62
ieng	1.29	224.4	−0.21	5.55	224.9	−0.29	10.36	221.2	−0.07	2.34		213.8	−0.46
leij	1.88	196.2	−0.63	4.72	226.3	−1.24	9.86	219.0	−1.21	2.65		199.1	−1.15
mall	2.68	142.2	−0.77	4.26	202.8	−0.83	8.67	206.6	−0.89	2.88		156.8	−1.11
mikl	4.44	226.6	0.39	7.22	238.2	0.20	12.33	223.3	−0.15	4.74		221.3	0.54
mobn	7.85	212.1	0.72	8.59	225.3	0.32	16.52	216.5	0.19	8.06		214.0	0.37
nico	3.71	200.8	0.01	5.68	225.9	0.23	12.42	215.6	−0.11	4.51		208.4	−0.40
onsa	1.71	163.7	2.82	3.53	219.6	2.81	8.76	212.6	2.32	2.63		175.2	2.78
orid	2.23	248.7	−0.40	5.71	244.7	−0.39	9.88	228.0	−0.56	2.73		245.3	0.10
penc	3.73	199.6	−0.78	5.24	227.0	−0.99	11.73	216.2	−0.92	4.84		212.9	−0.46
ptbb	0.52	35.7	0.27	1.73	274.8	−0.37	7.85	222.3	0.15	0.84		157.6	0.08
riga	3.36	193.5	0.86	4.59	229.9	1.07	10.39	215.5	0.40	4.52		195.7	1.07
sass	3.08	197.5	0.50	5.54	218.4	0.66	10.74	215.2	0.60	4.12		198.0	0.19
sofi	4.19	216.6	−0.35	6.30	234.4	−0.57	12.79	220.1	−0.56	4.75		216.8	−1.30
spt0	1.09	165.0	3.95	3.80	237.9	3.85	9.06	219.0	3.41	1.77		187.8	4.32
tlse	1.03	190.7	0.34	3.66	237.8	−0.31	9.53	221.2	0.44	1.52		205.8	−1.10
tubi	3.24	223.8	−0.42	5.12	241.9	−1.37	11.39	222.2	−0.83	3.50		222.6	−1.18
vill	2.44	116.8	−1.44	2.37	189.9	−1.59	8.41	202.7	−1.87	2.81		130.1	−0.21
vis0	2.25	167.9	3.34	3.91	214.7	3.16	9.34	210.2	2.77	2.86		177.1	3.20
wab2	0.15	45.6	0.13	3.63	231.2	0.15	7.89	220.7	−0.42	0.84		190.5	−0.02
wroc	2.34	198.8	−0.53	5.02	226.8	−0.87	10.57	218.0	−0.90	3.54		204.2	−1.59
wsrt	2.04	170.5	−0.10	4.86	213.7	−0.49	9.94	211.1	−0.24	3.39		184.5	−0.58
yebe	1.01	199.1	−0.33	3.93	233.3	−0.39	9.45	222.0	−0.56	1.75		204.1	−0.57
zouf	2.32	222.0	0.75	5.02	241.0	0.74	10.31	222.6	0.35	3.25		220.7	0.53

¹ The units are mm, degree and mm/year for the annual amplitudes, annual phases, and linear trends, respectively.

. The linear trend signals at most sites are small (less than 1 mm/year), except for those in Scandinavia Peninsula with strong uplift rates (apparently due to the GIA effect over this region). To quantify the dispersion of the statistical values from the four products, we estimate the mean values and standard deviations (SD) of the annual amplitudes, annual phases, and linear trends at the GPS sites, and the results are shown in

Table 2. Mean values and standard deviations of the four statistical values in Table 1 at each site.

Sites	Mean Values			Standard Deviations (SD)
	ann. amp.1	ann. pha.	trend	ann. amp.	ann. pha.	trend
acor	3.12	189.1	−2.23	3.38	46.2	0.18
alme	3.55	174.7	−0.61	2.84	48.7	0.17
bucu	7.49	221.9	1.59	3.86	6.4	0.18
ebre	3.47	170.4	−0.70	3.17	44.6	0.60
geno	4.24	217.5	−0.56	3.82	9.8	0.20
glsv	7.72	222.7	0.23	3.33	9.5	0.27
gope	5.49	194.4	0.34	3.67	17.5	0.13
hert	4.16	208.5	−0.44	3.63	19.6	0.13
ieng	4.89	221.1	−0.26	4.07	5.1	0.16
leij	4.78	210.1	−1.06	3.59	14.8	0.29
mall	4.62	177.1	−0.90	2.79	32.5	0.15
mikl	7.18	227.4	0.24	3.65	7.6	0.30
mobn	10.26	217.0	0.40	4.19	5.8	0.23
nico	6.58	212.7	−0.07	3.98	10.7	0.26
onsa	4.16	192.8	2.68	3.16	27.5	0.24
orid	5.14	241.7	−0.31	3.52	9.3	0.28
penc	6.38	213.9	−0.79	3.62	11.3	0.23
ptbb	2.73	172.6	0.03	3.45	103.1	0.28
riga	5.72	208.6	0.85	3.17	17.3	0.31
sass	5.87	207.3	0.49	3.40	11.1	0.21
sofi	7.01	222.0	−0.69	3.96	8.4	0.41
spt0	3.93	202.4	3.88	3.61	32.4	0.38
tlse	3.93	213.9	−0.16	3.90	20.2	0.71
tubi	5.81	227.6	−0.95	3.81	9.5	0.42
vill	4.01	159.9	−1.28	2.94	42.8	0.73
vis0	4.59	192.5	3.12	3.24	23.4	0.24
wab2	3.13	172.0	−0.04	3.51	86.0	0.26
wroc	5.37	211.9	−0.97	3.64	12.8	0.44
wsrt	5.06	194.9	−0.35	3.45	21.0	0.22
yebe	4.03	214.6	−0.46	3.82	15.9	0.12
zouf	5.22	226.6	0.59	3.57	9.7	0.19
Average	5.15	204.5	0.05	3.54	23.9	0.29

¹ The units are mm, degree and mm/year for the annual amplitudes, annual phases and linear trends, respectively.

. Note that for sites with large differences among the four products, the mean values of annual amplitudes and phases can hardly reflect the real deformation signals, and are only calculated as a value for joint analysis combined with the SD.

The mean SD of the annual amplitude is 3.54 mm, which is at the same level as that of the load signal (with an average value of 5.15 mm), while the mean SD of the annual phase is 23.9 degree, equal to ~1 month in the annual cycle. Since the weak linear trend signal (with an average value of ~0.05 mm/year) may be under the precision of the GPS observations, it would be difficult to use the mean SD (0.29 mm/year) to quantify the dispersion among multi-institution GPS products. Apparently, the large differences of GPS height displacements are expected to hinder the correct interpretation of load deformation, especially for the annual signals.

Considering the much smaller magnitudes of the trends in the studied region, and that the differences are mostly at seasonal time scales, we detrend all the time series and apply the “Three-Cornered Hat” method [34] to further assess the accuracy for each GPS series. To be consistent with subsequent experiments with the GRACE and model data, the GPS series are monthly averaged. With the assumption that the noises of the GPS products are independent from each other, it is possible to compute the SD of noise residuals in each series using least squares estimation. For a common signal (e.g., surface deformation), each observation can be expressed as a sum of true signal plus noise in Equation (4) as follows.

$${\mathrm{GPS}}^{\mathrm{series}\mathrm{A}}={\mathrm{GPS}}^{\mathrm{True}}+\mathrm{Noise}\_{\mathrm{GPS}}^{\mathrm{series}\mathrm{A}}.$$

(4)

Computing the variance of the difference between any two of the time series by eliminating the common terms, we obtain the 6 equations with 4 variances to be determined, as shown in Equation (5).

$$\{\begin{array}{c}\mathrm{var}\left(\mathrm{Noise}\_{\mathrm{GPS}}^{\mathrm{JPL}}\right)+\mathrm{var}\left(\mathrm{Noise}\_{\mathrm{GPS}}^{\mathrm{SOPAC}}\right)=\mathrm{var}\left({\mathrm{GPS}}^{\mathrm{JPL}}-{\mathrm{GPS}}^{\mathrm{SOPAC}}\right) \hfill \\ \mathrm{var}\left(\mathrm{Noise}\_{\mathrm{GPS}}^{\mathrm{JPL}}\right)+\mathrm{var}\left(\mathrm{Noise}\_{\mathrm{GPS}}^{\mathrm{MEaSUREs}}\right)=\mathrm{var}\left({\mathrm{GPS}}^{\mathrm{JPL}}-{\mathrm{GPS}}^{\mathrm{MEaSUREs}}\right)\hfill \\ \mathrm{var}\left(\mathrm{Noise}\_{\mathrm{GPS}}^{\mathrm{JPL}}\right)+\mathrm{var}\left(\mathrm{Noise}\_{\mathrm{GPS}}^{\mathrm{NGL}}\right)=\mathrm{var}\left({\mathrm{GPS}}^{\mathrm{JPL}}-{\mathrm{GPS}}^{\mathrm{NGL}}\right)\hfill \\ \mathrm{var}\left(\mathrm{Noise}\_{\mathrm{GPS}}^{\mathrm{SOPAC}}\right)+\mathrm{var}\left(\mathrm{Noise}\_{\mathrm{GPS}}^{\mathrm{MEaSUREs}}\right)=\mathrm{var}\left({\mathrm{GPS}}^{\mathrm{SOPAC}}-{\mathrm{GPS}}^{\mathrm{MEaSUREs}}\right)\hfill \\ \mathrm{var}\left(\mathrm{Noise}\_{\mathrm{GPS}}^{\mathrm{SOPAC}}\right)+\mathrm{var}\left(\mathrm{Noise}\_{\mathrm{GPS}}^{\mathrm{NGL}}\right)=\mathrm{var}\left({\mathrm{GPS}}^{\mathrm{SOPAC}}-{\mathrm{GPS}}^{\mathrm{NGL}}\right)\hfill \\ \mathrm{var}\left(\mathrm{Noise}\_{\mathrm{GPS}}^{\mathrm{MEaSUREs}}\right)+\mathrm{var}\left(\mathrm{Noise}\_{\mathrm{GPS}}^{\mathrm{NGL}}\right)=\mathrm{var}\left({\mathrm{GPS}}^{\mathrm{MEaSUREs}}-{\mathrm{GPS}}^{\mathrm{NGL}}\right)\hfill \end{array}$$

(5)

The least squares solutions of Equation (5) are the noise variances, and the estimated SDs (square roots of variances) are shown in

Table 3. Standard deviation (SD) of the noise in each site’s height time series, from least squares fit to obtain the variance of difference between each time series pair with the four GPS products (JPL, SOPAC, MEaSUREs, NGL). The lowest SD of noise residuals for each site are highlighted in bold font. (Unit: mm).

Sites	JPL	SOPAC	MEaSUREs	NGL
acor	3.84	2.19	4.77	3.05
alme	3.71	2.57	4.63	3.00
bucu	2.97	1.82	5.57	2.54
ebre	3.49	2.70	5.05	5.51
geno	3.91	2.60	5.01	2.86
glsv	2.77	2.78	5.79	3.14
gope	3.63	2.12	5.10	2.82
hert	4.09	2.32	5.04	2.29
ieng	4.14	1.62	5.32	2.22
leij	3.51	2.51	4.97	2.21
mall	3.77	2.29	4.79	2.91
mikl	3.27	2.55	5.02	2.70
mobn	3.31	2.85	5.64	4.04
nico	4.05	2.74	6.11	3.35
onsa	3.75	2.14	4.94	2.18
orid	3.72	3.18	4.47	3.51
penc	3.75	2.93	5.10	3.70
ptbb	5.12	3.40	4.96	2.59
riga	3.68	3.00	5.18	2.85
sass	4.04	1.59	5.00	2.23
sofi	3.62	2.39	5.62	3.36
spt0	4.17	2.36	4.98	2.95
tlse	3.52	2.23	5.72	2.33
tubi	3.27	3.66	5.58	3.41
vill	3.84	2.92	6.52	3.16
vis0	3.25	2.39	4.92	1.84
wab2	3.55	1.79	5.12	2.64
wroc	3.90	2.10	5.87	2.79
wsrt	3.87	1.80	5.38	2.04
yebe	3.68	3.04	5.27	2.51
zouf	3.38	2.14	4.80	1.63
Average	3.70	2.47	5.23	2.85

. The SOPAC height time series show the lowest noise level at 22 of the 31 sites among the 4 GPS products, while the NGL series yields lowest noise level at 7 sites. The mean SD of noise residuals for the JPL, SOPAC, MEaSUREs, NGL solutions are ~3.70 mm, 2.47 mm, 5.23 mm, and 2.85 mm, respectively.

3.2. Uncertainty Assessment of Load Deformations from GPS, GRACE, and Models

We also estimate the SD of noise residuals using the TCH method by including load deformations at the 31 sites derived from GRACE and climate model data. Figure 3 shows the comparison among the 6 time series (4 GPS + GRACE + MODEL) at 4 selected sites (same as the sites in Figure 2). The comparison for the other sites is provided in Figures S3 and S4 for conciseness. The four GPS series are monthly averaged, and the GRACE series are resampled into monthly to be comparable with each other. The reasons why we consider the data from the observations by other technique observations are: (a) not all GPS time series products are completely independent from one another, which is the prerequisite of applying the TCH method, (b) GPS observations need independent data from other techniques to validate and interpret, and (c) we also aim to assess the noise level among different techniques.

When considering the 6 series, we generate 15 equations with 6 variances to be solved in the form of Equation (5).

Table 4. Standard deviation of the noise in each site’s height time series, from least squares fit to the variance of difference between each time series pair with the six estimates (JPL, SOPAC, MEaSUREs, NGL, GRACE, MODEL). The lowest SD of noise residuals for each site are highlighted in the bold font. (Unit: mm).

Sites	JPL	SOPAC	MEaSUREs	NGL	GRACE	MODEL
acor	3.50	3.03	5.12	2.70	2.39	2.41
alme	3.33	3.45	4.99	2.61	2.67	2.71
bucu	2.83	2.40	5.92	2.72	3.89	3.54
ebre	4.42	3.44	5.68	4.99	4.54	4.66
geno	4.06	2.83	5.39	3.11	3.44	3.71
glsv	3.23	3.14	5.59	3.97	3.96	3.69
gope	3.77	2.71	5.25	3.04	3.65	3.14
hert	3.98	2.97	5.16	2.76	3.30	3.21
ieng	3.75	2.60	5.77	2.19	3.09	3.29
leij	3.95	3.09	5.01	2.92	3.57	3.32
mall	3.44	2.88	5.32	2.83	2.70	3.30
mikl	3.35	2.79	4.89	3.08	2.74	2.32
mobn	3.32	3.50	6.71	5.20	6.76	6.65
nico	3.66	3.45	6.48	3.08	2.57	2.94
onsa	3.97	2.34	4.73	3.00	3.07	3.03
orid	3.68	3.78	4.36	3.81	3.03	3.15
penc	3.91	2.93	5.50	3.73	3.38	2.96
ptbb	5.76	3.88	4.34	3.35	4.13	3.77
riga	4.21	2.90	5.29	3.58	4.13	3.92
sass	4.03	2.19	5.60	2.72	4.16	4.30
sofi	3.80	3.48	6.10	2.82	4.40	4.02
spt0	4.25	2.75	4.85	3.80	3.75	3.48
tlse	3.57	2.74	5.72	2.57	2.89	2.68
tubi	3.86	3.62	5.56	3.45	3.05	2.80
vill	4.31	3.22	6.40	3.14	2.63	2.92
vis0	3.71	2.15	5.02	2.93	3.57	4.04
wab2	3.69	2.32	5.17	2.70	3.00	2.41
wroc	3.76	2.44	6.02	3.36	3.60	3.02
wsrt	3.58	2.45	5.60	2.64	3.29	3.07
yebe	3.66	3.36	5.48	2.44	2.68	2.74
zouf	3.49	2.53	4.93	2.25	3.37	3.33
Average	3.80	2.95	5.42	3.15	3.47	3.37

shows the estimated SD of the noise residuals for the time series at each site and the mean values (of the 31 sites) for each technique. The SOPAC height series have the lowest noise standard deviations for 14 of the 31 sites, with the smallest mean value of 2.95 mm. The MEaSUREs shows the largest mean value of 5.42 mm, which is consistent with the conclusion drawn in Table 3 (when using 4 GPS series only).

Besides assessing the accuracy with noise variance, we implement three additional indicators to assess the consistency/discrepancy among the six series, which are the zero-lag correlation coefficients in

Table 5. Mean correlation coefficients among the 6 series.

Correlation Pairs	JPL	SOP	MEa	NGL	GRC	Mod
JPL	-	0.67	0.63	0.76	0.38	0.34
SOP	-	-	0.81	0.73	0.54	0.58
MEa	-	-	-	0.70	0.67	0.75
NGL	-	-	-	-	0.49	0.45
GRC	-	-	-	-	-	0.87
Mod	-	-	-	-	-	-

, mean annual amplitude (AnnAmp) reduction in

Table 6. Mean annual amplitude reductions of the 6 series ^1.

Reduction Sources	JPL	SOP	MEa	NGL	GRC	Mod
JPL	-	−73.04	−313.65	30.70	−22.30	−18.93
SOP	28.41	-	−15.48	46.22	61.71	57.20
MEa	19.20	47.14	-	31.25	35.69	32.60
NGL	57.85	17.43	−125.17	-	48.10	38.45
GRC	33.68	44.97	−90.20	55.71	-	73.09
Mod	22.04	21.28	−144.04	33.70	66.00	-

¹ The mean annual amplitude reduction is defined in Equation (6). Taking the value in row of JPL and column of SOP as an example, the number “−73.04” denotes the percentage of “AnnAmp reduction_JPL,SOP”.

, and mean root mean squares (RMS) reduction in

Table 7. Mean RMS reductions of the 6 series ¹.

Reduction Sources	JPL	SOP	MEa	NGL	GRC	Mod
JPL	-	15.51	−19.43	31.44	3.72	5.77
SOP	21.56	-	15.08	29.28	15.82	18.17
MEa	22.33	40.58	-	28.91	22.62	22.35
NGL	30.61	22.81	−10.76	-	11.12	11.67
GRC	−54.12	−45.54	−90.70	−40.82	-	49.94
Mod	−111.67	−99.81	−170.24	−95.36	30.53	-

¹ The Mean RMS reduction is defined in Equation (7). Taking the value in row of JPL and column of SOP as an example, the number “15.51” denotes the percentage of “RMS reduction_JPL,SOP”.

. The correlation coefficient reflects the closeness between two variables, which describes the consistency of time variations (phases) between two time series in this study. The annual amplitude reduction is defined as Equation (6), and represents the consistency of annual amplitudes between the two series, while the RMS reduction is defined as Equation (7) and represents the consistency of the total series (including the annual signal as well as other signals/noises).

$$AnnAmp reductio{n}_{1, 2}=\frac{AnnAmp\left(series 1\right)-AnnAmp\left(series 1-series 2\right)}{AnnAmp\left(series 1\right)}\times 100\%,$$

(6)

$$RMS reductio{n}_{1,2}=\frac{RMS\left(series 1\right)-RMS\left(series 1-series 2\right)}{RMS\left(series 1\right)}\times 100\%.$$

(7)

The largest correlation coefficient is 0.87 (Table 5), which implies a good consistency of time variations between GRACE and models. Moreover, the corresponding values of mean AnnAmp reduction (73.09 and 66.00, in Table 6) and RMS reduction (49.94 and 30.53, in Table 7) is also large, representing their good consistencies in the studied time scales. In fact, this is in line with expectations since the GRACE series are derived from the mascon and GAC data, while the model series also consist of GAC data and the GAC effect accounts for a large proportion in the time series.

Among the four GPS series, the MEaSUREs series show apparent larger correlation coefficients with the GRACE and model (0.67 and 0.75) than the other three do (see Table 5). The MEaSUREs mean AnnAmp reduction values are all negative (see column 4 in Table 6), representing that the MEaSUREs series have specifically larger annual amplitudes, which can be further illustrated through the phasor diagram (as described subsequently) in the selected sites (see the green vectors in Figure 5). Similarly, the mean RMS reduction values of the MEaSUREs series (see column 4 in Table 7) are smaller than those of the other series, which implies that the MEaSUREs series include stronger fluctuations (or variations) than the others do. Besides, the relatively larger values of the three indicators between JPL and NGL series suggest that there is a good consistency between the two products.

The comparison of the annual signals among the GPS, GRACE and model series using phasor diagrams for all the 31 sites is illustrated in Figure 4. To examine the detailed information of the arrows, we show in Figure 5 the zoomed-in figures of the annual amplitudes and phases at the selected 9 sites, whose annual variations are significant and representative of the study region. The MEaSUREs series exhibit substantially large annual variations, which is consistent with that revealed by Figure 2. In addition, the JPL and NGL series show a consistent pattern of variation, with relatively smaller annual amplitudes and phases, while the SOPAC series show the largest annual phase among the four GPS series at most of the selected sites. Compared with the other three GPS series, the SOPAC annual signals (blue vectors in Figure 5) are closer to the GRACE and model (brown and pink vectors in Figure 5).

3.3. Uncertainty Assessment GPS Horizontal Time Series Solutions

In order to further evaluate the GPS load deformations, we analyze the horizontal displacements (east (E) and north (N) directions separately) in Europe using the same method. For the E-direction, Table S1 shows the annual and trend signals for each site using the four GPS time series products, and Table S2 shows the corresponding mean values and standard deviations of the four values. Similarly, Tables S3 and S4 show the same statistical values for the N-direction time series.

For the horizontal linear trend signals, which mainly reflect regional tectonic motions, the magnitudes reach tens of millimeters per year (much larger than that of the U-direction with less than 0.1 mm/year in Table 2), while the SD of the four products (E-direction mean: 0.14 mm/year, N-direction mean: 0.16 mm/y) are at the same level as the U-direction in Table 2 (0.29 mm/year). We assume that the differences in the linear trends estimated from multi-institutional GPS products are small for both vertical and horizontal directions.

Although GPS horizontal components have been proved to have higher precision than in the vertical direction [35], the load signal in the horizontal direction is only ~30% to 50% of that in the vertical direction [3], which is more difficult to isolate and interpret. Most of the annual amplitudes are less than 1 mm (see Tables S1 and S3), which can hardly reflect the true load signals under the current precision of GPS observation. The only five sites with annual amplitudes larger than 1 mm for all the four GPS series (ieng, sass, wroc for the E direction and glsv, ptbb for the N direction) are selected (marked with green dots in Figure 1), and the SD of their annual amplitudes from the four GPS products are ~0.3 mm, which is about 30% of the observed signal.

We plot the horizontal deformation time series of the five sites in Figure 6, and show residuals of the SD of noise estimated using the TCH method in

Table 8. Standard deviation of noise in the five selected sites’ east/north-direction time series, from least squares fit to variance of the difference between each time series pair with the four estimates (JPL, SOPAC, MEaSUREs, NGL). The lowest SD of noise residuals for each site is highlighted in bold font. (Unit: mm).

Sites	JPL	SOPAC	MEaSUREs	NGL
ieng (E)	1.16	1.25	0.84	0.98
sass (E)	1.08	1.31	0.69	0.90
wroc (E)	1.06	1.33	0.75	1.93
glsv (N)	1.07	1.32	0.71	1.51
ptbb (N)	1.10	1.59	0.37	1.16

. It is interesting to note that for the horizontal components, the MEaSUREs results have the lowest SD of noise for all the five sites, which is contrary to the conclusions in the vertical components.

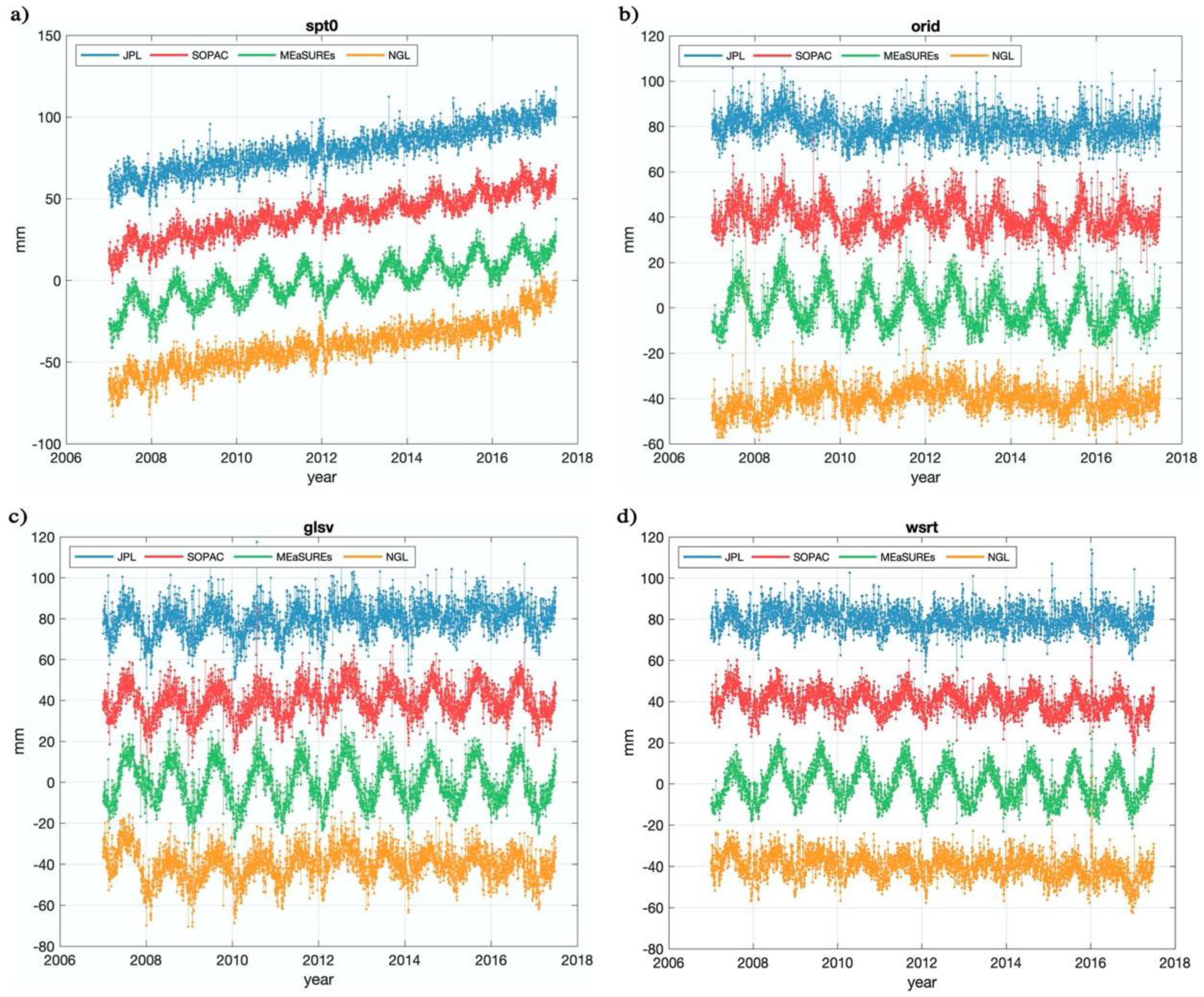
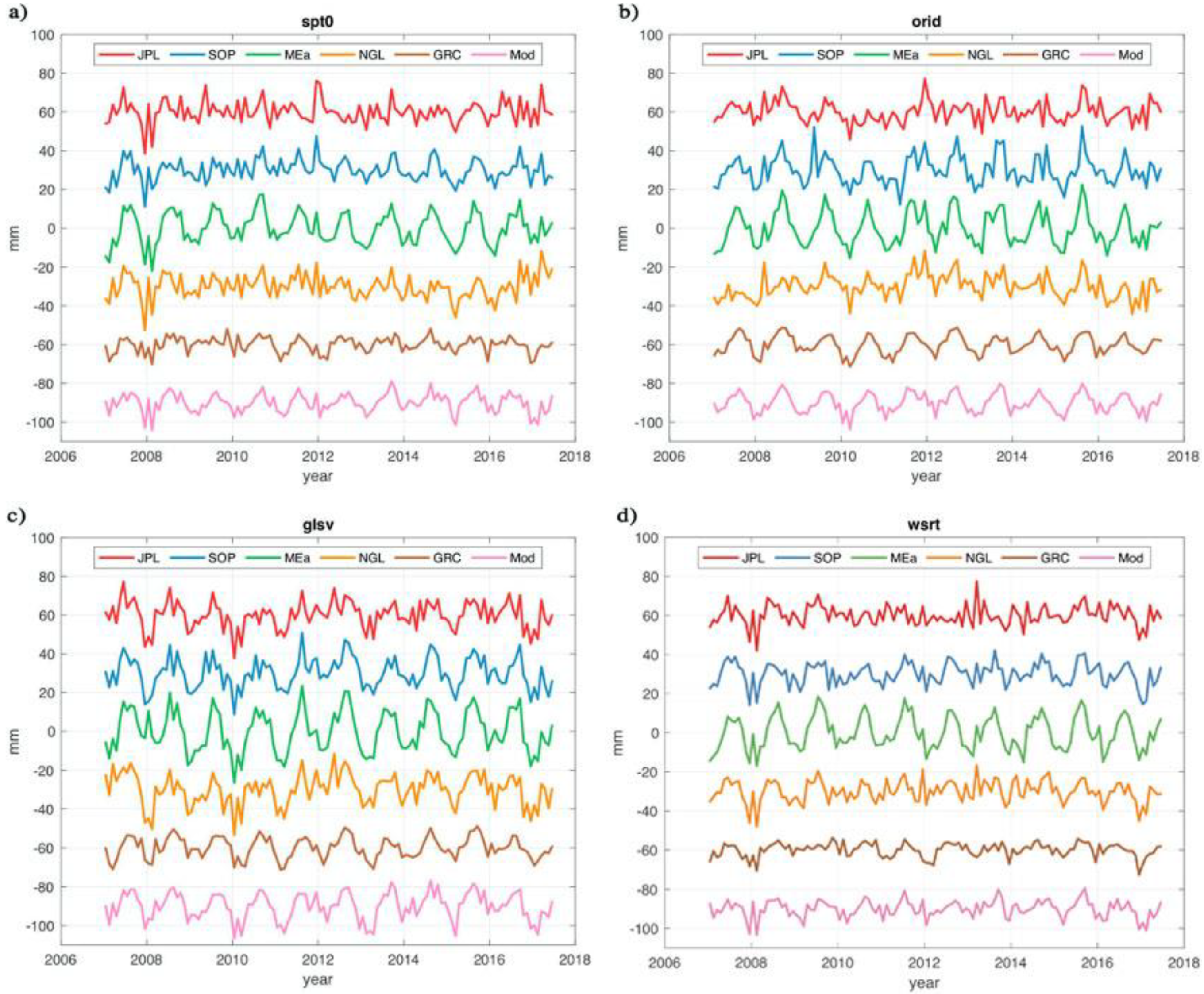
4. Discussion

The GPS data processing strategy affects the accuracy of generated time series, and may cause bias in load deformation analysis. The JPL and NGL products, which use the Precise Point Positioning (PPP) strategy, show comparable SD of noise residuals (see Table 3 and Table 4) and relatively large values of the three indicators (as shown in Table 5Table 6 and Table 7). The SOPAC series are generated using a distributed sub-network analysis strategy, which pays more attention to regional overall quality and may be more appropriate for regional-scale signal analysis (e.g., load deformation). As for the MEaSUREs, which is a post-processing product based on the JPL and SOPAC data, there may be some artificial variations associated with the processing procedure that is expected to be likely responsible for the large annual amplitudes in Figure 4 and Figure 5.

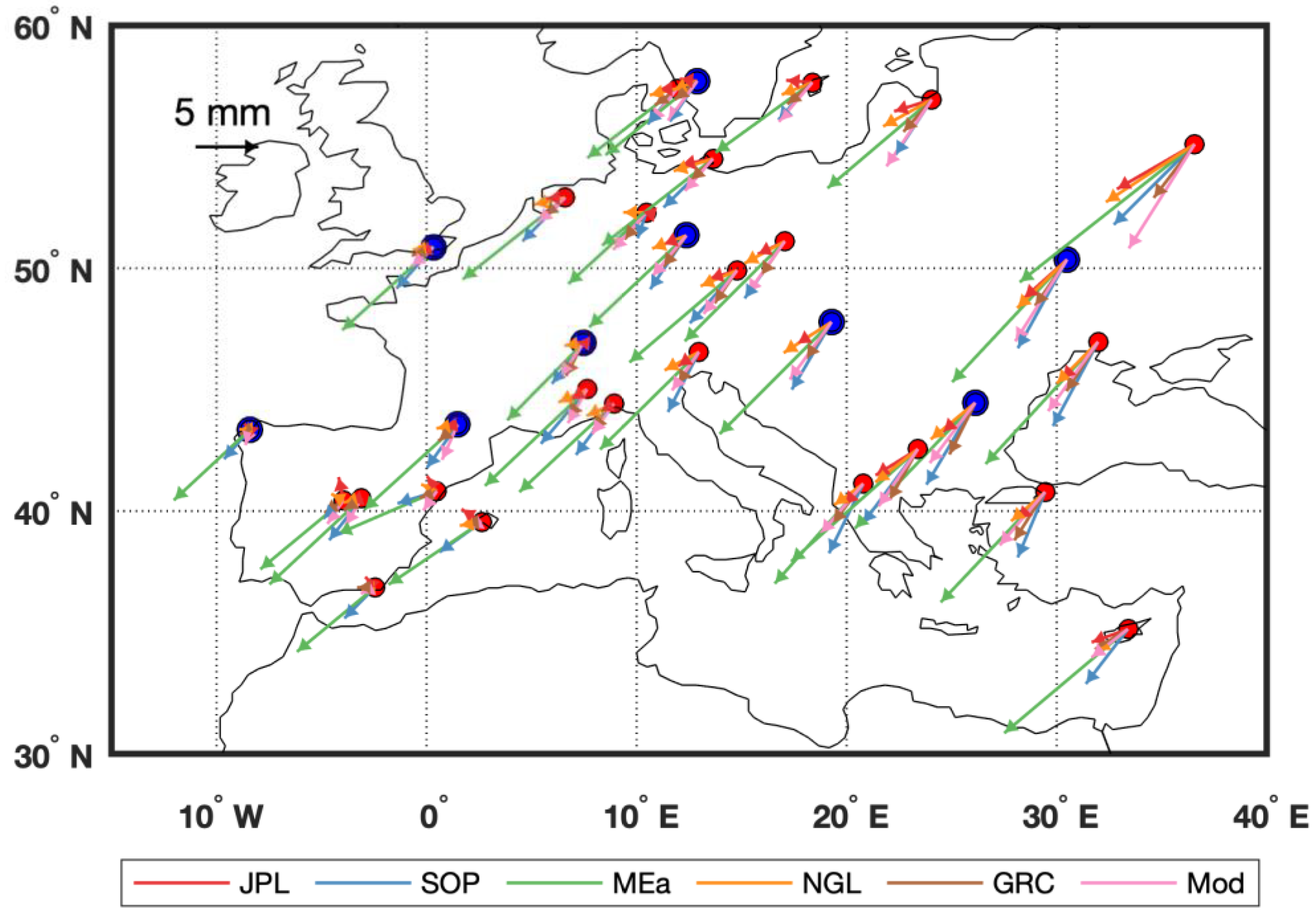
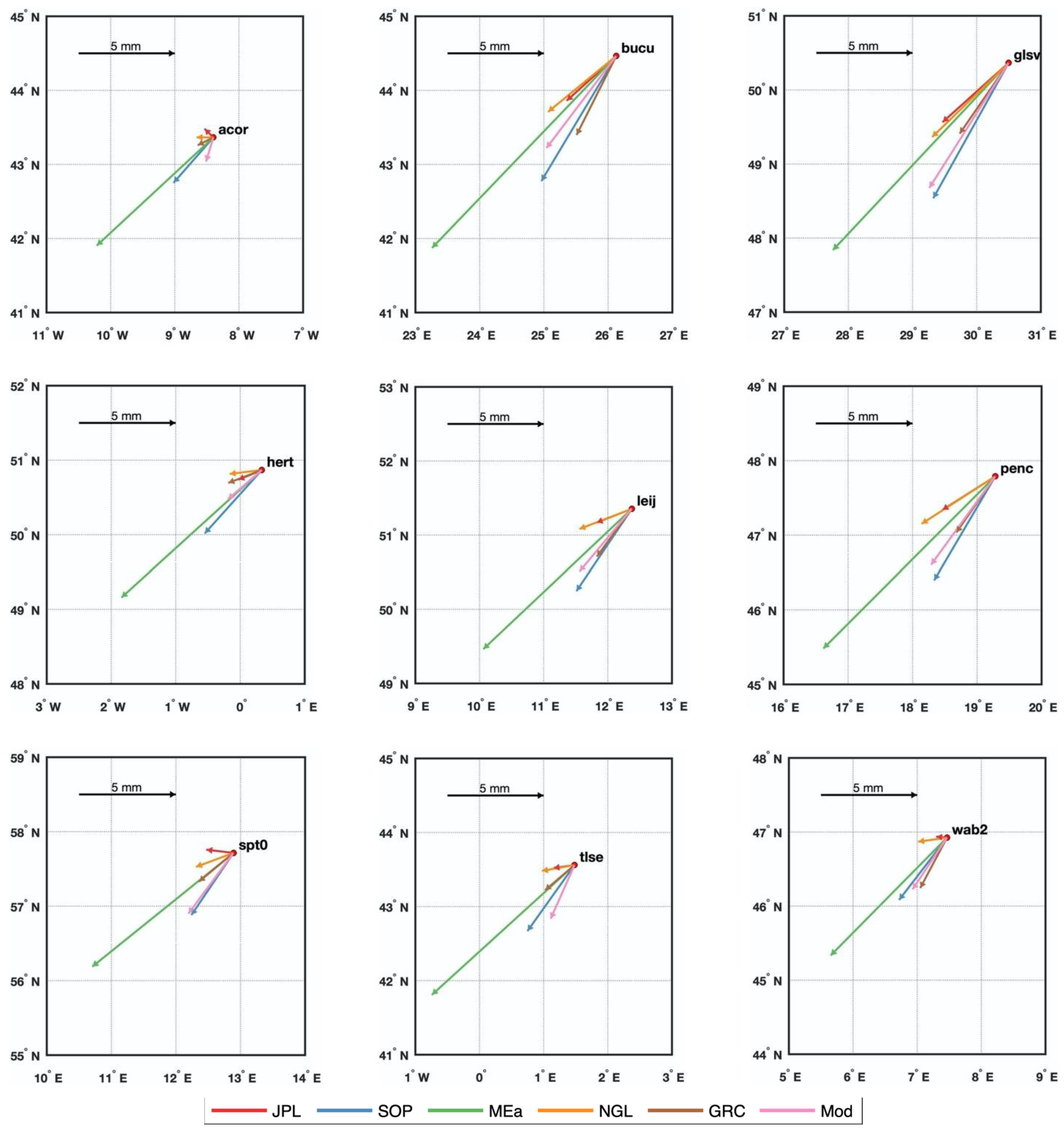
Compared to vertical deformation analysis, accurate quantification of horizontal deformation is also important for load studies, especially for the inversion of mass load using GPS observations. Our estimates of noise level for the horizontal components show that the MEaSUREs series have the lowest SD of noise. However, the annual amplitudes of horizontal load displacements over most part of Europe are less than 1 mm, which is most likely under the current precision of GPS positioning, and are difficult to be verified by GPS techniques. There could be some levels of dependence among the noises of the four GPS products, which affects the compliance with the assumption of noise independence in the TCH method. In regions with large load signals, more stations are needed so as to provide adequate spatial constraint to determine the location and magnitude of the mass load source. Further analysis is needed in future study.

5. Conclusions

We carried out a detailed comparison of GPS surface displacement time series products from four institutions (namely, JPL, SOPAC, MEaSUREs, and NGL) in Europe, and analyzed their influences on load deformation study. The extracted linear trends of GPS load deformation, whether in the east, north, or vertical direction, showed good agreement among the four products, while the annual variations showed large discrepancies. The mean value of the standard deviations of the four GPS series at 31 sites was ~3.54 mm in annual amplitude of the vertical component (~69% of the signal), and ~0.3 mm for the horizontal component (~30% of the signal). We used the TCH method to estimate the noise level of each series, and found that either four or six time series (four GPS + GRACE + model) were considered, and that the SOPAC vertical series had the lowest SD of noise, revealing its relatively better accuracy. The MEaSUREs time series are likely to include some artificial variations and deviate distinctly in vertical direction from the GRACE-derived and the climate-model-predicted load deformations in Europe. The horizontal displacements are smaller than 1 mm in annual amplitudes for most of the sites in Europe, which are still challenging to be used for load analysis. However, the potential spatial constraint introduced by horizontal displacements deserves continuous attention and further study.

With the development for more than 20 years, the GNSS positioning precision reaches cm or sub-cm level, which is similar to the magnitude of load deformation signals. Due to different data processing strategies (orbit/clock solving strategies, applied correction models, etc.), the discrepancies in the three-dimensional deformation time series are still significant and affect the practical accuracy of GNSS positioning, which cannot be ignored for reliable load deformation analysis, especially for mass load inversion studies using GNSS technique. How to improve the practical accuracy of GNSS load deformation remains a major challenge and is essential to reliable load deformation interpretation as well as quantitative mass variation study of the Earth system.