Ambiguity Suppression Based on Joint Optimization for Multichannel Hybrid and ±π/4 Quad-Pol SAR Systems
Abstract
1. Introduction
2. Materials and Methods
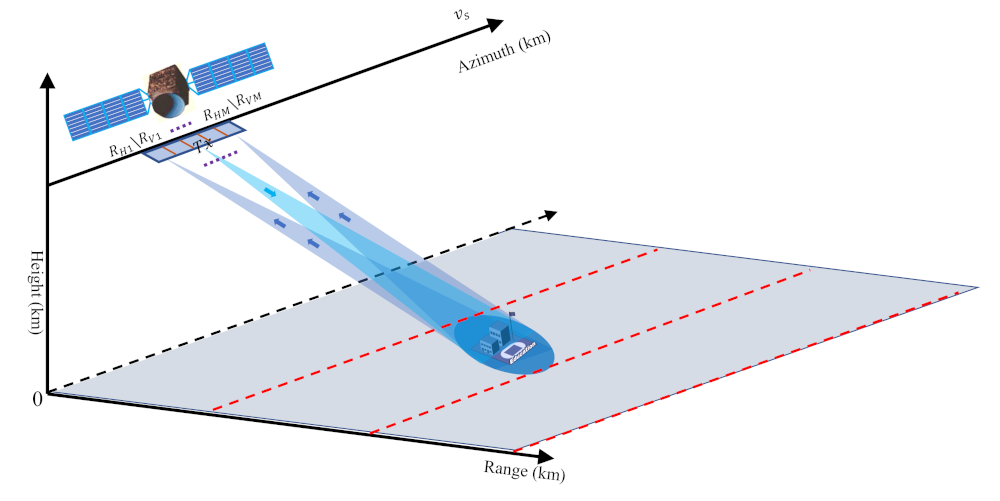
2.1. Multichannel Hybrid and Quad-Pol SAR Systems
2.1.1. Multichannel Quad-Pol SAR
2.1.2. Sampling Frequency (PRF)
2.2. Reconstruction Methods for Multichannel Quad-Pol SAR
2.2.1. Conventional Matrix Inversion (MI) Method
2.2.2. Joint Optimization to Suppress the Ambiguity (JOSA)
3. Results
- (1)
- AASR for different polarizations after reconstruction;
- (2)
- RASR for different polarizations after reconstruction;
- (3)
- Output signal-to-noise ratio (SNR) for different polarizations.
3.1. Characterization of the Reconstruction and Performance
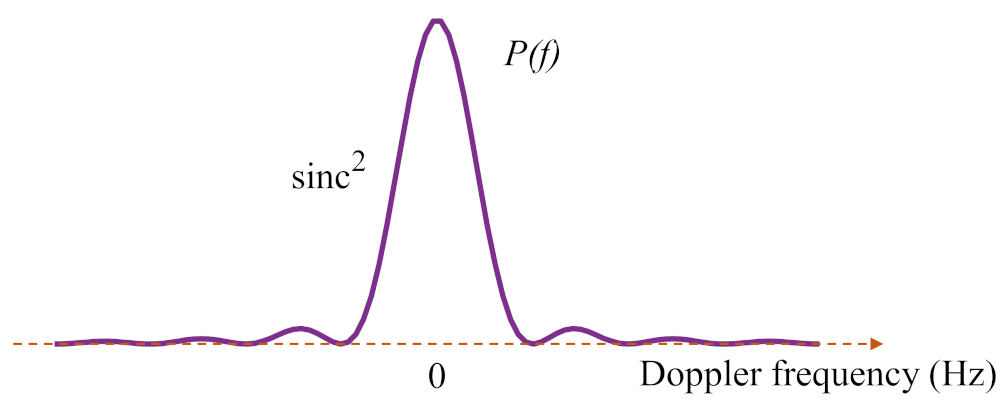
3.1.1. Effects of Reconstruction on Azimuth Ambiguity
3.1.2. Effects of Reconstruction on Range Ambiguity
3.2. Numerical Simulation Results of Reconstruction Methods
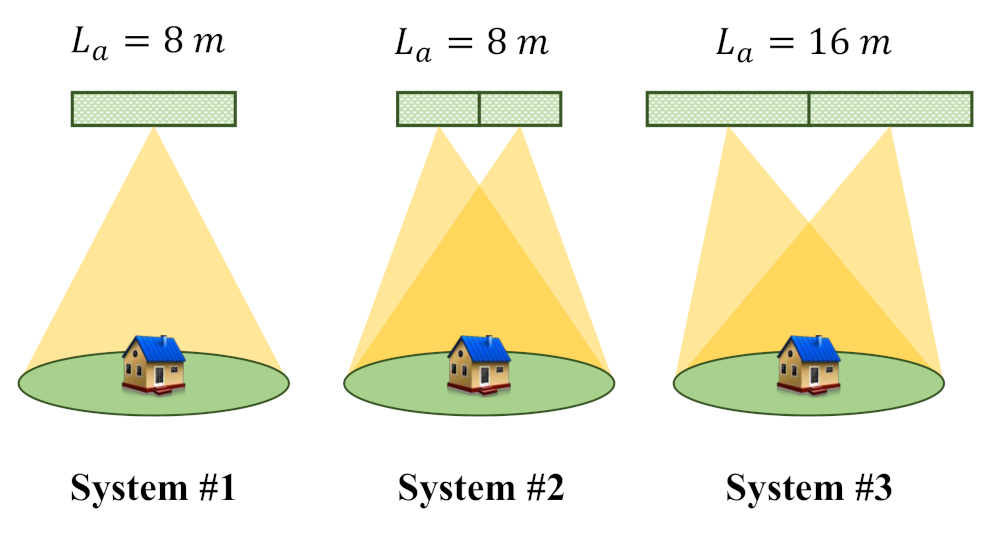
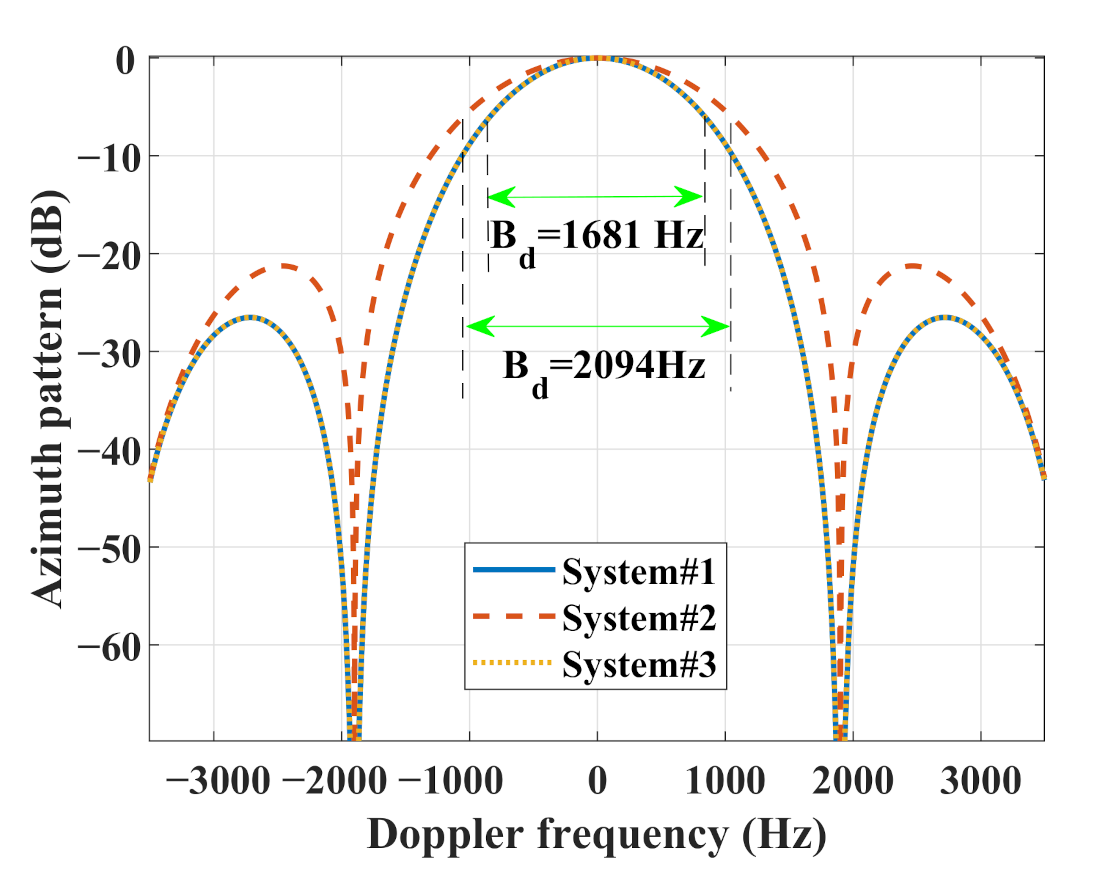
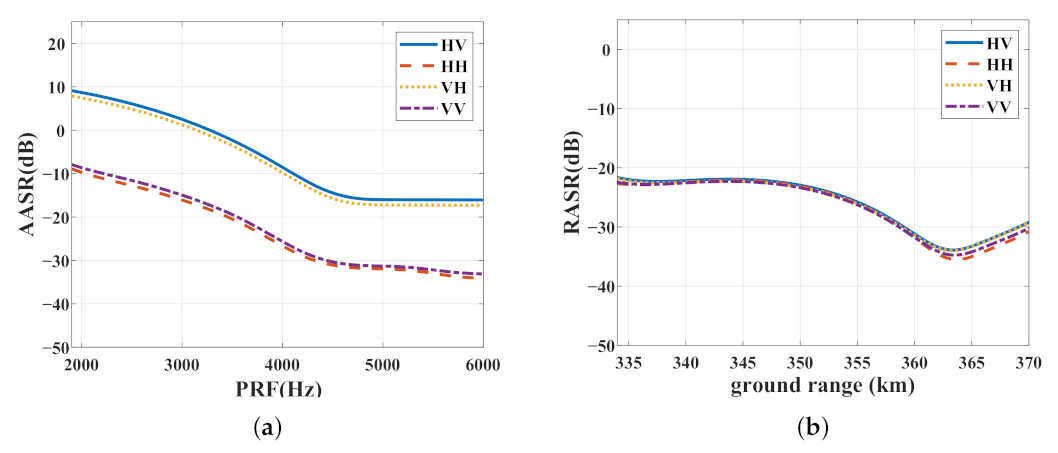
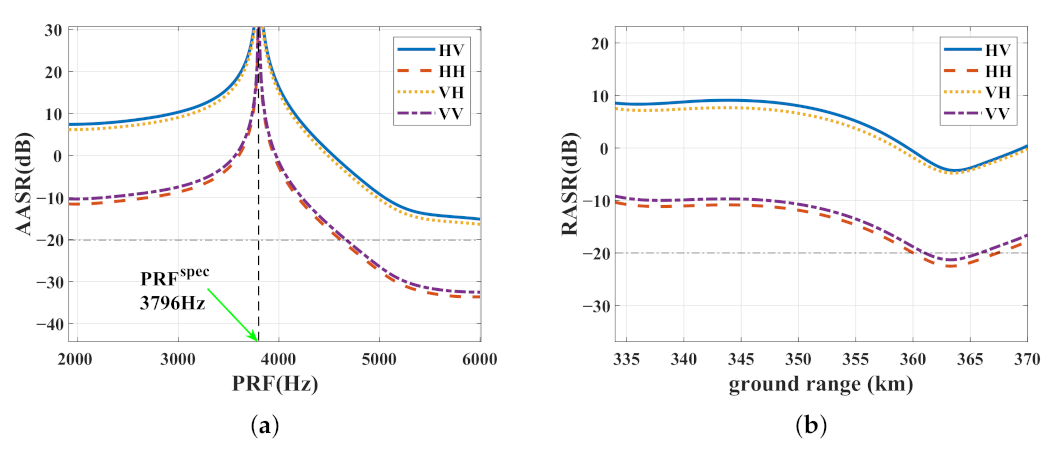
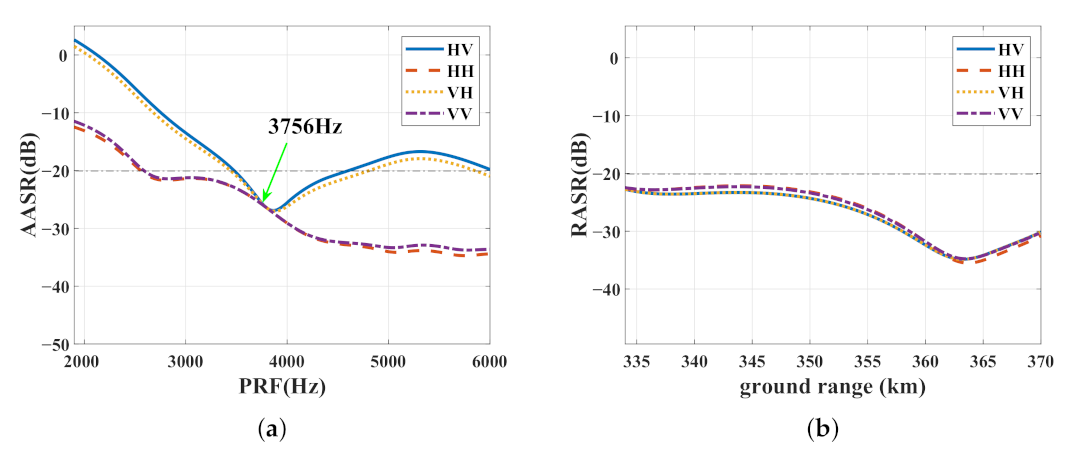
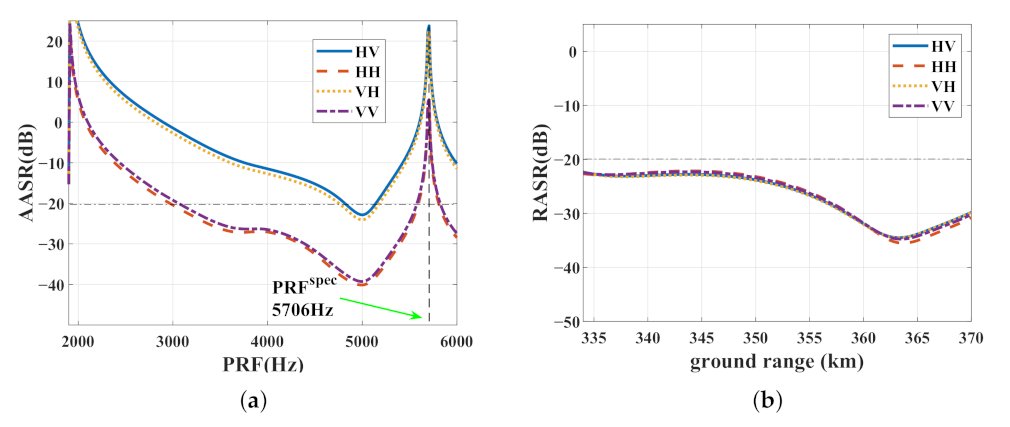
3.2.1. ASRs Performance of Single-Channel Quad-Pol SAR System (System #1)
3.2.2. ASRs Performance of Multichannel Quad-Pol SAR System (Systems #2 & #3)
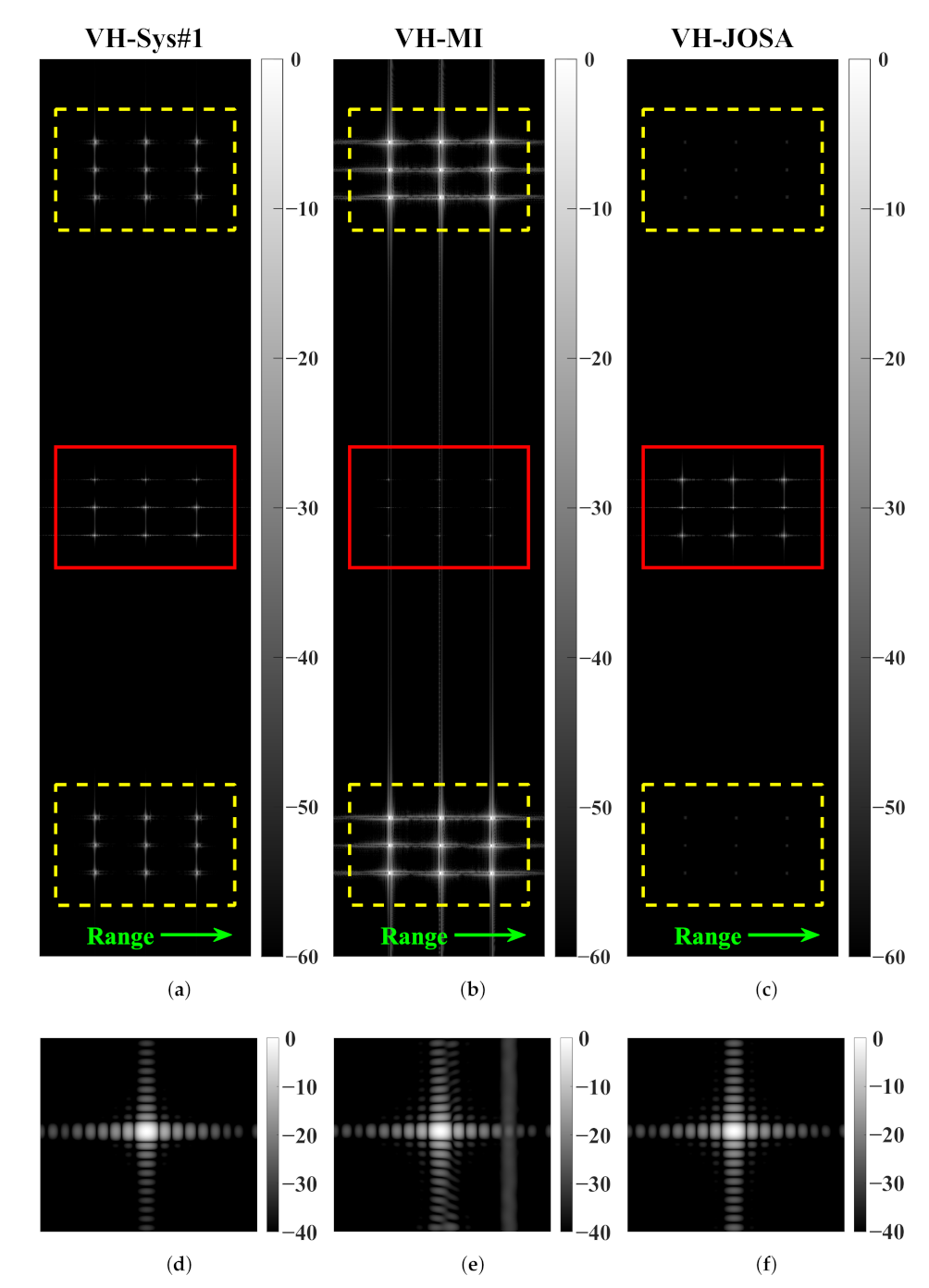
3.2.3. Imaging Simulation Results
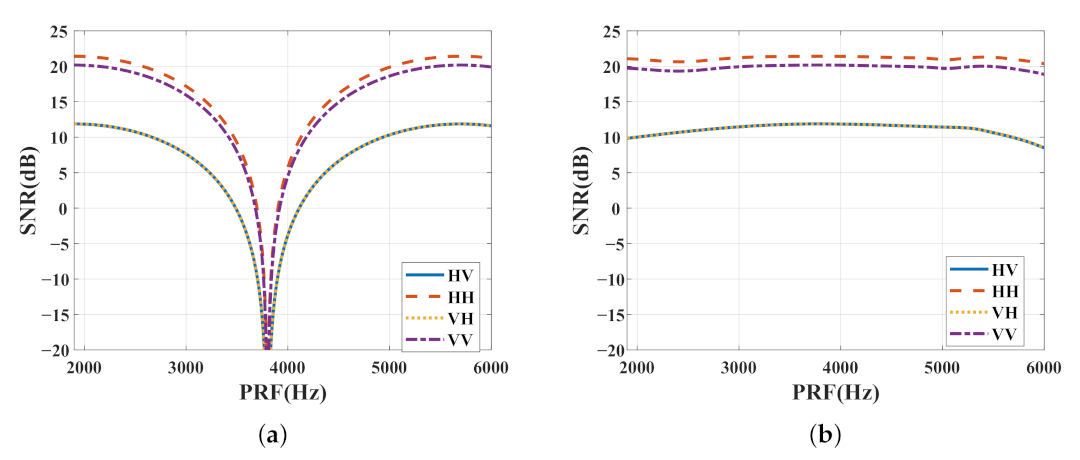
3.3. Effects of Reconstruction on Noise
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| AASR | Azimuth ambiguity-to-signal ratio |
| JOSA | Joint optimization to suppress the ambiguity |
| MI | Matrix inverse |
| PRF | Pulse repetition frequency |
| RASR | Range ambiguity-to-signal ratio |
| SNR | Signal-to-noise ratio |
| SAR | Synthetic aperture radar |
| UDR | Ratio of undesired azimuth signal power and desired azimuth signal power |
References
- Moreira, A.; Prats-Iraola, P.; Younis, M.; Krieger, G.; Hajnsek, I.; Papathanassiou, K.P. A tutorial on synthetic aperture radar. IEEE Geosci. Remote Sens. Mag. 2013, 1, 6–43. [Google Scholar] [CrossRef]
- Lee, J.S.; Pottier, E. Polarimetric Radar Imaging: From Basics to Applications; CRC Press: Boca Raton, FL, USA, 2009. [Google Scholar]
- Cloude, S. Polarisation: Applications in Remote Sensing; Oxford University Press: New York, NY, USA, 2009. [Google Scholar]
- Sinclair, G. The transmission and reception of elliptically polarized waves. Proc. IRE 1950, 38, 148–151. [Google Scholar] [CrossRef]
- Souyris, J.C.; Imbo, P.; Fjortoft, R.; Mingot, S.; Lee, J.S. Compact polarimetry based on symmetry properties of geophysical media: The pm pi-4 mode. IEEE Trans. Geosci. Remote Sens. 2005, 43, 634–646. [Google Scholar] [CrossRef]
- Raney, R.K. Hybrid-quad-pol SAR. In Proceedings of the IGARSS 2008-2008 IEEE International Geoscience and Remote Sensing Symposium IEEE, Boston, MA, USA, 8–11 July 2008; Volume 4, pp. IV–491. [Google Scholar]
- Raney, R.K.; Freeman, A.; Jordan, R.L. Improved range ambiguity performance in quad-pol SAR. IEEE Trans. Geosci. Remote Sens. 2011, 50, 349–356. [Google Scholar] [CrossRef]
- Villano, M.; Krieger, G.; Moreira, A. New insights into ambiguities in quad-Pol SAR. IEEE Trans. Geosci. Remote Sens. 2017, 55, 3287–3308. [Google Scholar] [CrossRef]
- Dall, J.; Kusk, A. Azimuth phase coding for range ambiguity suppression in SAR. In Proceedings of the IGARSS 2004. 2004 IEEE International Geoscience and Remote Sensing Symposium, Anchorage, AK, USA, 20–24 September 2004; Volume 3, pp. 1734–1737. [Google Scholar]
- Gebert, N.; Krieger, G.; Moreira, A. Digital beamforming on receive: Techniques and optimization strategies for high-resolution wide-swath SAR imaging. IEEE Trans. Aerosp. Electron. Syst. 2009, 45, 564–592. [Google Scholar] [CrossRef]
- Krieger, G.; Gebert, N.; Moreira, A. Unambiguous SAR signal reconstruction from nonuniform displaced phase center sampling. IEEE Geosci. Remote Sens. Lett. 2004, 1, 260–264. [Google Scholar] [CrossRef]
- Krieger, G.; Gebert, N.; Moreira, A. Multidimensional Waveform Encoding: A New Digital Beamforming Technique for Synthetic Aperture Radar Remote Sensing. IEEE Trans. Geosci. Remote Sens. 2008, 46, 31–46. [Google Scholar] [CrossRef]
- Cerutti-Maori, D.; Sikaneta, I.; Klare, J.; Gierull, C.H. MIMO SAR processing for multichannel high-resolution wide-swath radars. IEEE Trans. Geosci. Remote Sens. 2013, 52, 5034–5055. [Google Scholar] [CrossRef]
- Sikaneta, I.; Gierull, C.H.; Cerutti-Maori, D. Optimum signal processing for multichannel SAR: With application to high-resolution wide-swath imaging. IEEE Trans. Geosci. Remote Sens. 2014, 52, 6095–6109. [Google Scholar] [CrossRef]
- Wang, W.; Wang, R.; Deng, Y.; Xu, W.; Guo, L.; Hou, L. Azimuth ambiguity suppression with an improved reconstruction method based on antenna pattern for multichannel synthetic aperture radar systems. IET Radar Sonar Navig. 2015, 9, 492–500. [Google Scholar] [CrossRef]
- Li, Z.; Wang, H.; Su, T.; Bao, Z. Generation of wide-swath and high-resolution SAR images from multichannel small spaceborne SAR systems. IEEE Geosci. Remote Sens. Lett. 2005, 2, 82–86. [Google Scholar] [CrossRef]
- Liu, N.; Wang, R.; Deng, Y.; Zhao, S.; Wang, X. Modified multichannel reconstruction method of SAR with highly nonuniform spatial sampling. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2017, 10, 617–627. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, W.; Deng, Y.; Wang, R. Signal Reconstruction Algorithm for Azimuth Multichannel SAR System Based on a Multiobjective Optimization Model. IEEE Trans. Geosci. Remote Sens. 2020, 58, 3881–3893. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, W.; Deng, Y.; Zhang, Z.; Wang, N.; Lang, Y.; Zhao, P.; Wang, R. Ambiguity Suppression of Cross-Pol Signals by DPCA With DBF Reflector for Hybrid/ pm pi/4 Quad-Pol SAR. IEEE Trans. Geosci. Remote Sens. 2021, 1–13. [Google Scholar] [CrossRef]
- Raney, R. A’free’3-dB cross-polarized SAR data. IEEE Trans. Geosci. Remote Sens. 1988, 26, 700–702. [Google Scholar] [CrossRef]
- Boyd, S.; Boyd, S.P.; Vandenberghe, L. Convex Optimization; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Li, J.; Stoica, P.; Wang, Z. Doubly constrained robust Capon beamformer. IEEE Trans. Signal Process. 2004, 52, 2407–2423. [Google Scholar] [CrossRef]
- Cumming, I.G.; Wong, F.H. Digital Processing of Synthetic Aperture Radar Data: Algorithms and Implementation [with CDROM] (Artech House Remote Sensing Library); Artech Housel: Boston, MA, USA, 2005. [Google Scholar]
- Villano, M.; Krieger, G.; Moreira, A. Ambiguities and image quality in staggered SAR. In Proceedings of the 2015 IEEE 5th Asia-Pacific Conference on Synthetic Aperture Radar (APSAR), Singapore, 1–4 September 2015; pp. 204–209. [Google Scholar] [CrossRef]




| Parameters | System #1 | System #2 | System #3 |
|---|---|---|---|
| Radar frequency | 5.4 GHz (C-band) | ||
| Orbit height | 628 km | ||
| Radar velocity | 7600 m/s | ||
| Minimum ground range | 334 km | ||
| Maximum ground range | 370 km | ||
| Tilt angle | |||
| Antenna type | Planar | ||
| Antenna height | 2.1 m | ||
| Tx antenna length | 8 m | 8 m | 8 m |
| Rx antenna total length | 8 m | 8 m | 16 m |
| Number of Rx channels | 1 | 2 | 2 |
| Length of sub-antenna | – | 4 m | 8 m |
| Antenna spacing | – | 4 m | 8 m |
| Doppler bandwidth | 673 Hz | 838 Hz | 673 Hz |
| Backscatter model | Ulaby and Dobson, shrubs | ||
| Systems | System #1 | System #2 | System #3 | ||
|---|---|---|---|---|---|
| Methods | Combination | MI | JOSA | MI | JOSA |
| HV | dB | dB | dB | dB | dB |
| HH | dB | dB | dB | dB | dB |
| VH | dB | dB | dB | dB | dB |
| VV | dB | dB | dB | dB | dB |
| Systems | System #1 | System #2 | System #3 | ||
|---|---|---|---|---|---|
| Methods | Combination | MI | JOSA | MI | JOSA |
| HV | dB | dB | dB | dB | dB |
| HH | dB | dB | dB | dB | dB |
| VH | dB | dB | dB | dB | dB |
| VV | dB | dB | dB | dB | dB |
| Systems | System #1 | System #2 | |
|---|---|---|---|
| Methods | Combination | MI | JOSA |
| VH | dB | dB | dB |
| VV | dB | dB | dB |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, P.; Deng, Y.; Wang, W.; Zhang, Y.; Wang, R. Ambiguity Suppression Based on Joint Optimization for Multichannel Hybrid and ±π/4 Quad-Pol SAR Systems. Remote Sens. 2021, 13, 1907. https://doi.org/10.3390/rs13101907
Zhao P, Deng Y, Wang W, Zhang Y, Wang R. Ambiguity Suppression Based on Joint Optimization for Multichannel Hybrid and ±π/4 Quad-Pol SAR Systems. Remote Sensing. 2021; 13(10):1907. https://doi.org/10.3390/rs13101907
Chicago/Turabian StyleZhao, Pengfei, Yunkai Deng, Wei Wang, Yongwei Zhang, and Robert Wang. 2021. "Ambiguity Suppression Based on Joint Optimization for Multichannel Hybrid and ±π/4 Quad-Pol SAR Systems" Remote Sensing 13, no. 10: 1907. https://doi.org/10.3390/rs13101907
APA StyleZhao, P., Deng, Y., Wang, W., Zhang, Y., & Wang, R. (2021). Ambiguity Suppression Based on Joint Optimization for Multichannel Hybrid and ±π/4 Quad-Pol SAR Systems. Remote Sensing, 13(10), 1907. https://doi.org/10.3390/rs13101907








