2.1. Corner Cube Retro-Reflectors
CCR analysis started in the early 1970s with the expectation that they would prove useful for both lunar laser ranging and satellite laser ranging given their specific reflective capabilities [
13,
14,
15]. The CCR is a unique component that provides a reflection along the initial energy angle of incidence, somewhat mimicking a specular reflection as opposed to a diffuse return. Chang et al. [
16] studied the far-field diffraction pattern for corner cubes, using methods presented by Fraunhofer years earlier for circular apertures. This theoretical analysis was used to determine the appropriate size CCR for both ICESat and ICESat-2 mission use cases, with the implementation of putting CCR arrays on the Earth’s surface for independent space-based lidar geolocation validation [
12].
The CCR diameter selection is a function of the wavelength, distance from the aperture (e.g., altitude of the satellite), and energy level of the laser. The derived strength of the signal return is proportional to the radius of the CCR, which means larger corner cubes reflect larger signal levels back to the satellite receiver. However, as the CCR diameter increases, the central diffraction disk of returned energy becomes smaller (e.g., diameter of disk is inversely proportional to diameter of the CCR). The Fraunhofer diffraction disk diameter is important in satellite applications as it requires consideration in context of spacecraft velocity aberration. If the velocity aberration value does not converge with the reflected disk diameter, the signal will fall outside of the receiver telescope field of view. In this situation, there is a chance to receive signal from the outer lobes of the Fraunhofer diffraction pattern but it is much less than the signal in the central disk that may or may not be an adequate return given atmospheric scattering and other optical losses [
14]. The theoretical CCR size selection for ICESat-2 (wavelength and energy levels) was determined as a range of 6 to 8 mm diameters. This diameter range mitigates the issues with velocity aberration and yet provides an acceptable signal level given the estimated 60% atmospheric transmission each way, a 20% quantum efficiency and 50% detector efficiency [
9]. In contrast, the ICESat mission successfully used a 12 mm diameter CCRs to supply both a geolocation accuracy assessment [
11] as well as a signature tag for timing accuracy validation [
10].
In preparation for ICESat-2 CCR validation, studies using the ATLAS airborne engineering testbed, MABEL (Multiple Altimeter Beam Experimental Lidar) [
12] were conducted. The studies revealed that the CCR approach could provide an independent validation of geolocation despite the operational and technical differences between the ICESat’s full-waveform, high-energy lidar technology and the photon-counting technology of MABEL and ICESat-2. However, the analysis of the return signatures required a new approach to accommodate the transition from a single large footprint illumination of multiple corner cubes (ICESat) to the ICESat-2/MABEL illumination of one CCR with multiple laser shots. Understanding multiple laser illuminations of a single CCR for geolocation validation requires a geometric analysis of relative positioning of the CCR and footprint centerline (diameter) and knowledge of the ICESat-2 geolocation determination process.
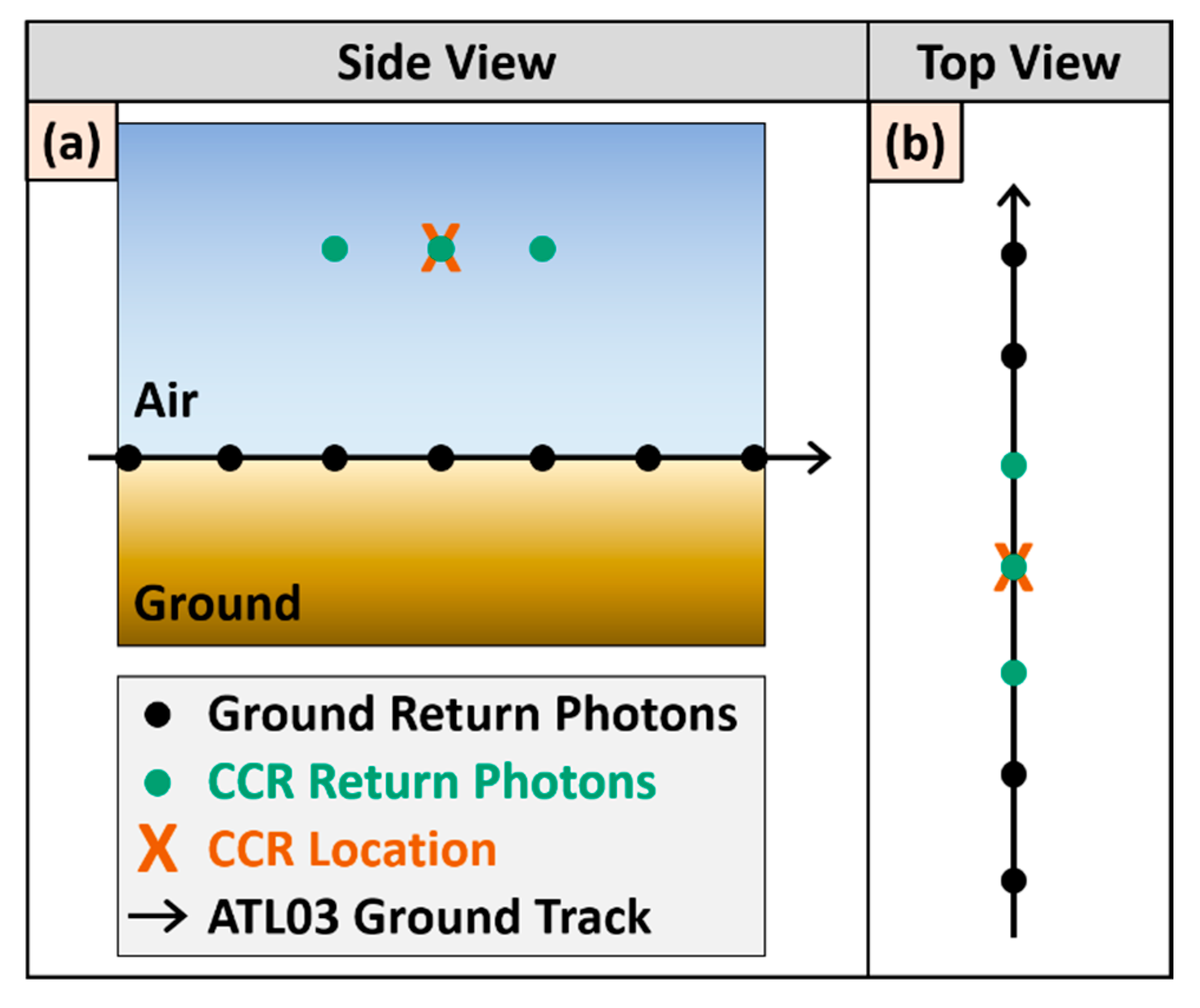
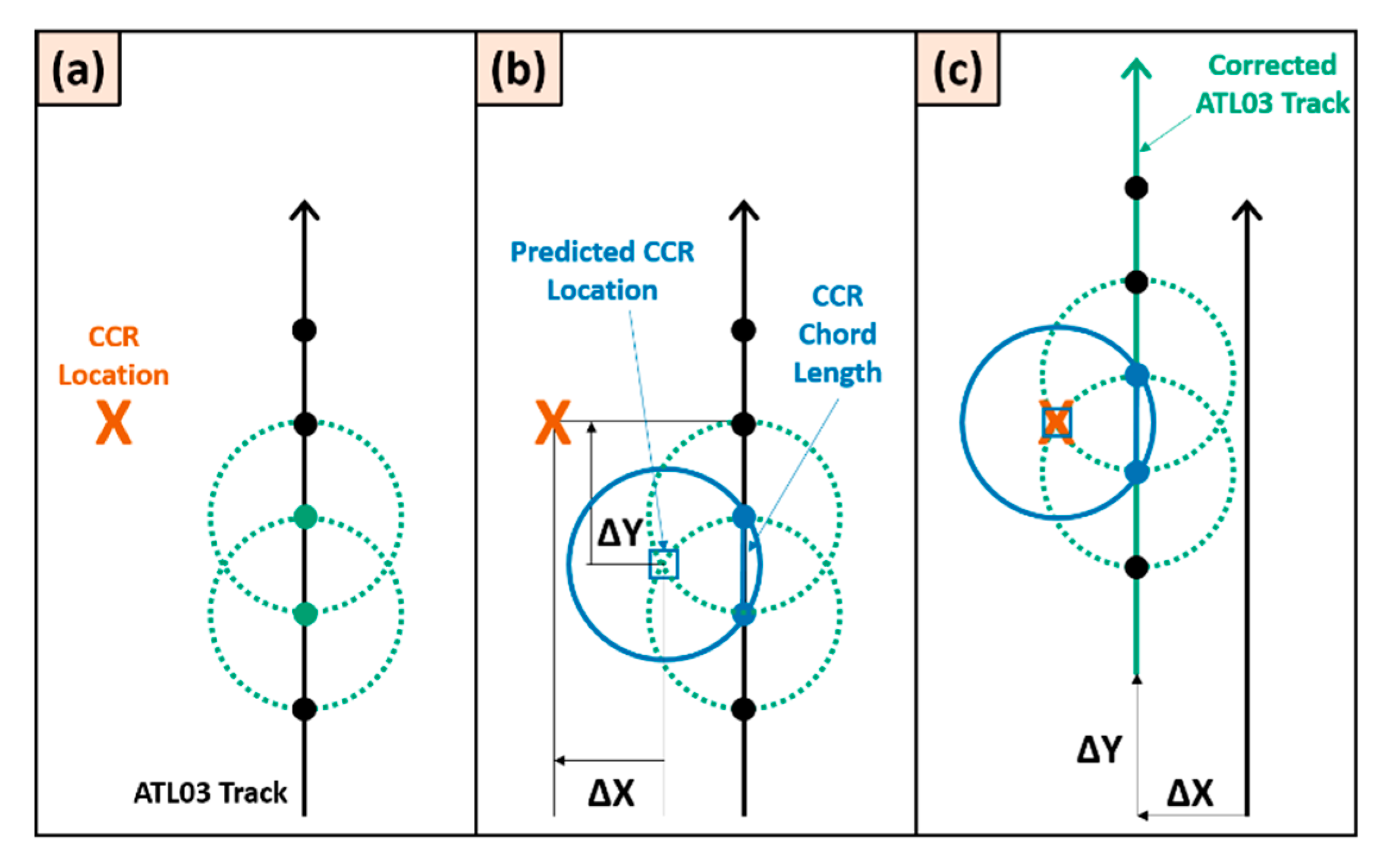
A theoretical scenario for a CCR position exactly aligned with the centerline of the laser footprint would nominally provide a signal every 0.7 m with a timestamp step of 0.1 msec (10 kHz) through the entire length of the ICESat-2 footprint diameter. If the diameter is equal to 17 m (pre-launch best estimate) then in an ideal case this would provide at least one CCR reflected photon from 24 individual laser shots over 2.4 msec. These points appear to be successive single, or multiple, photon detections along-track (at 0.1 msec delta timestamps) with an elevation representative of the height of the CCR above the surface. A conceptual view from a horizontal perspective of the CCR signal and associated ground return elevations is shown in
Figure 1a, each point representing a geolocated photon, black specific to terrain or ground photons and green from the CCR. The red X represents the actual position of the CCR. The top view of the CCR returns, in
Figure 1b, show the alignment, horizontally, of the CCR and ground returns despite the difference in elevation. This alignment brings an interesting challenge to the CCR signature analysis in that any detected photon associated with a specific laser shot will be geolocated at the geometric centroid or “bounce point” regardless of spatial separation between that reflection point source and the footprint centroid. As such, if the position of the CCR is coincident to the footprint centerline, then the CCR signature requires no additional interpretation with respect to comparing the ATL03 geolocation to the known position of the CCR.
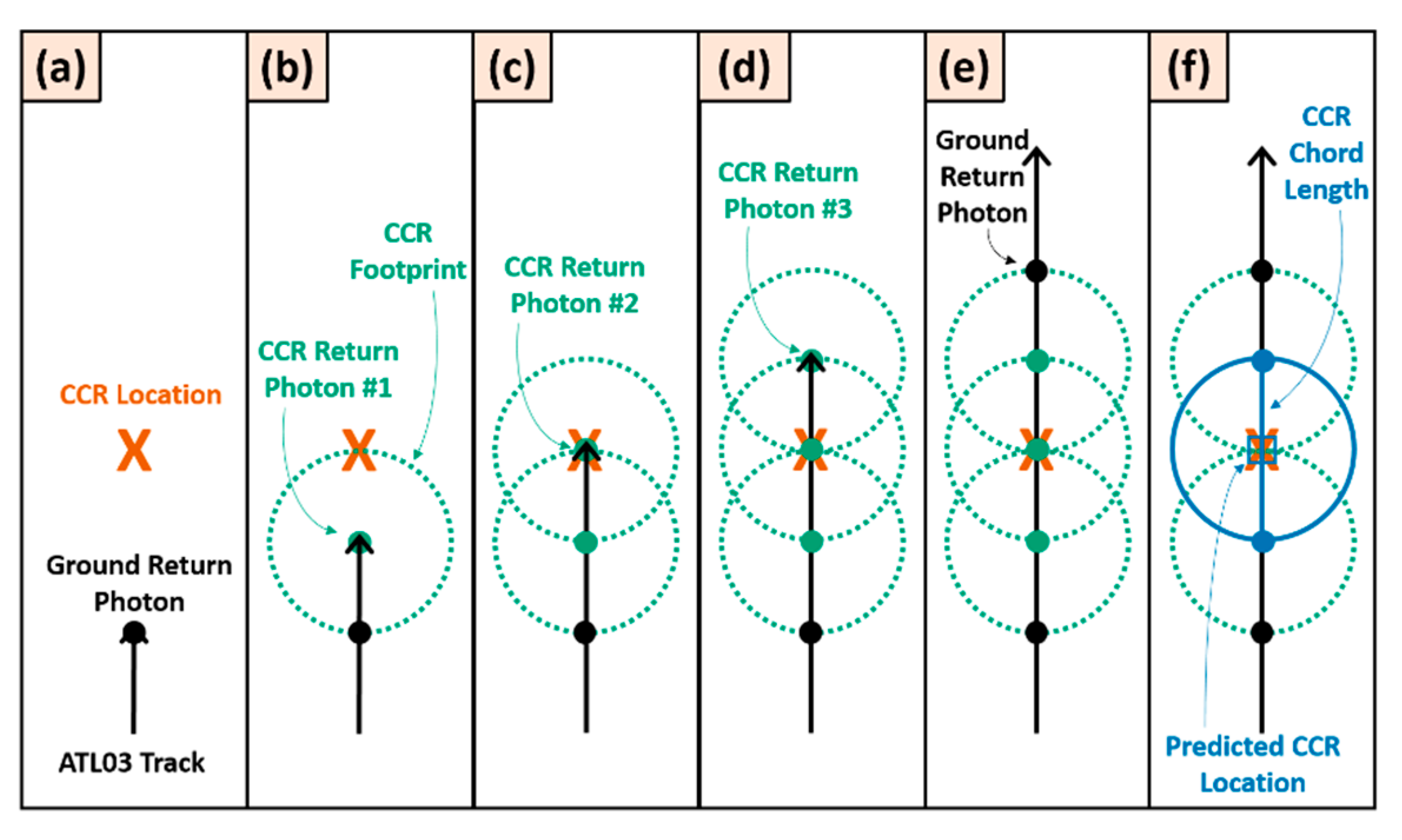
Keeping the focus on the scenario of the CCR and along-track ATL03 alignment in
Figure 1,
Figure 2 presents a time lapse representation of multiple footprint illuminations for one optical component and the expected detected photons. The dotted green circles are the individual laser footprints based on the ATL03 geolocated centroids and the blue circles are associated with statistical determination of the footprint location relative to the CCR position. Although not to scale, the diagram illustrates the collection of CCR detected signals as they initiate with the leading edge of the first relevant laser shot (
Figure 2b) and ends with the trailing edge of the final spot (
Figure 2f), in this case, the third footprint.
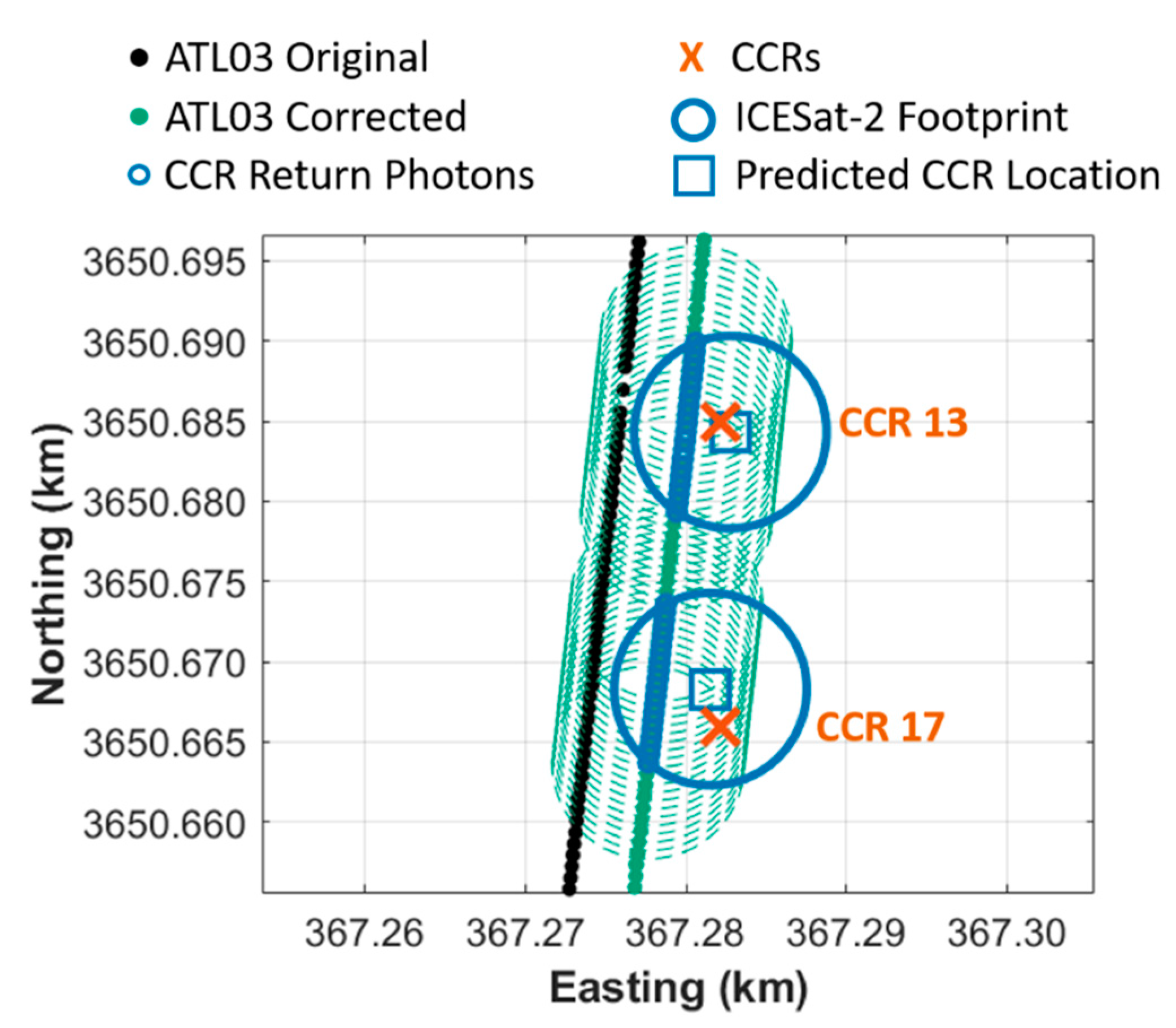
Figure 2f highlights the recovered chord length (along-track distance of the CCR photons) as equivalent to the diameter of the laser footprint. The mid-point of the CCR signature is directly compared to the known CCR position to assess the ATL03 geolocation accuracy in both along-track (ΔY) and across track (ΔX) directions. In the case of
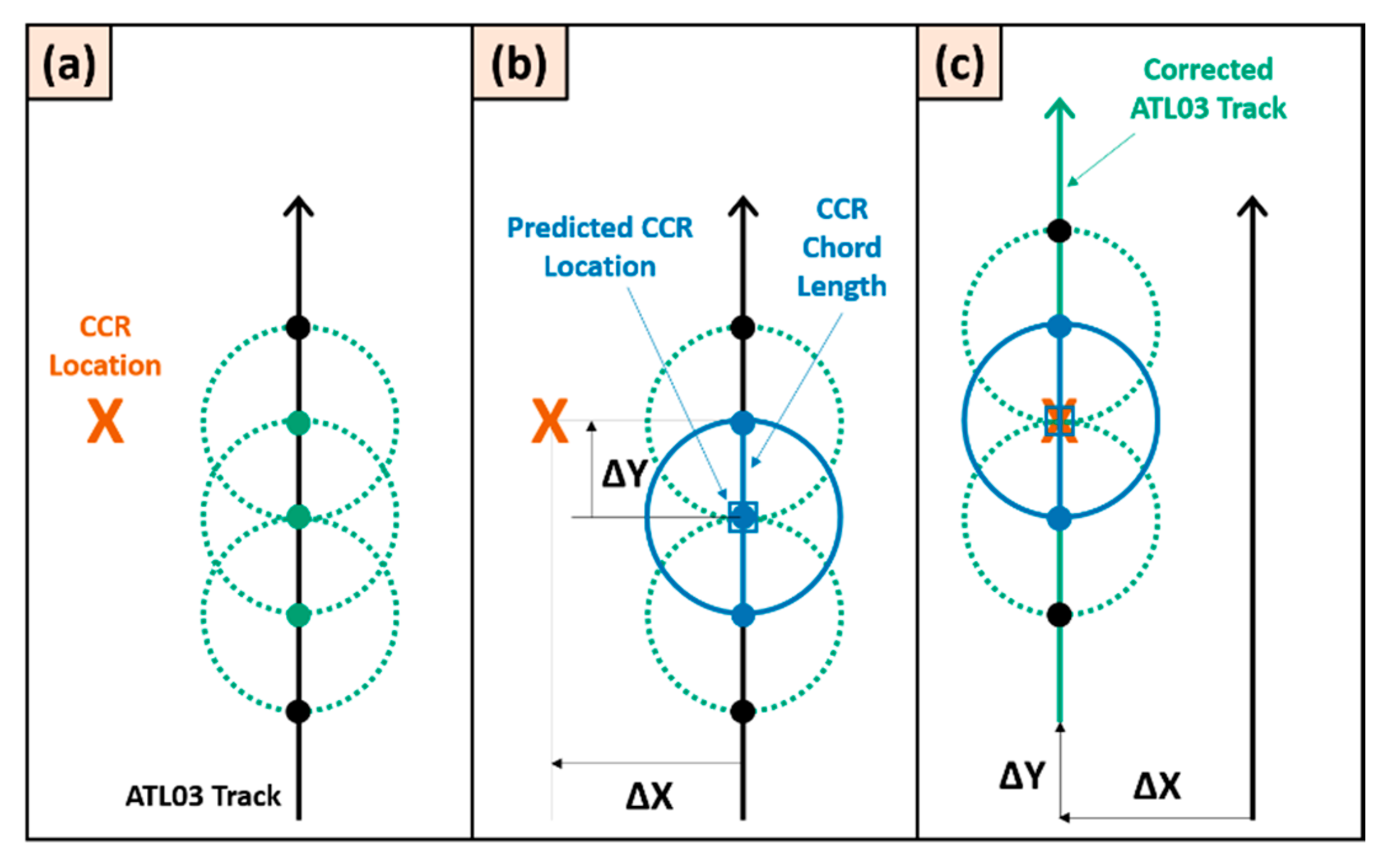
Figure 2, the geolocation has no error (ΔX = ΔY = 0) as the predicted mid-point of the CCR signature matches exactly with the known CCR coordinates.
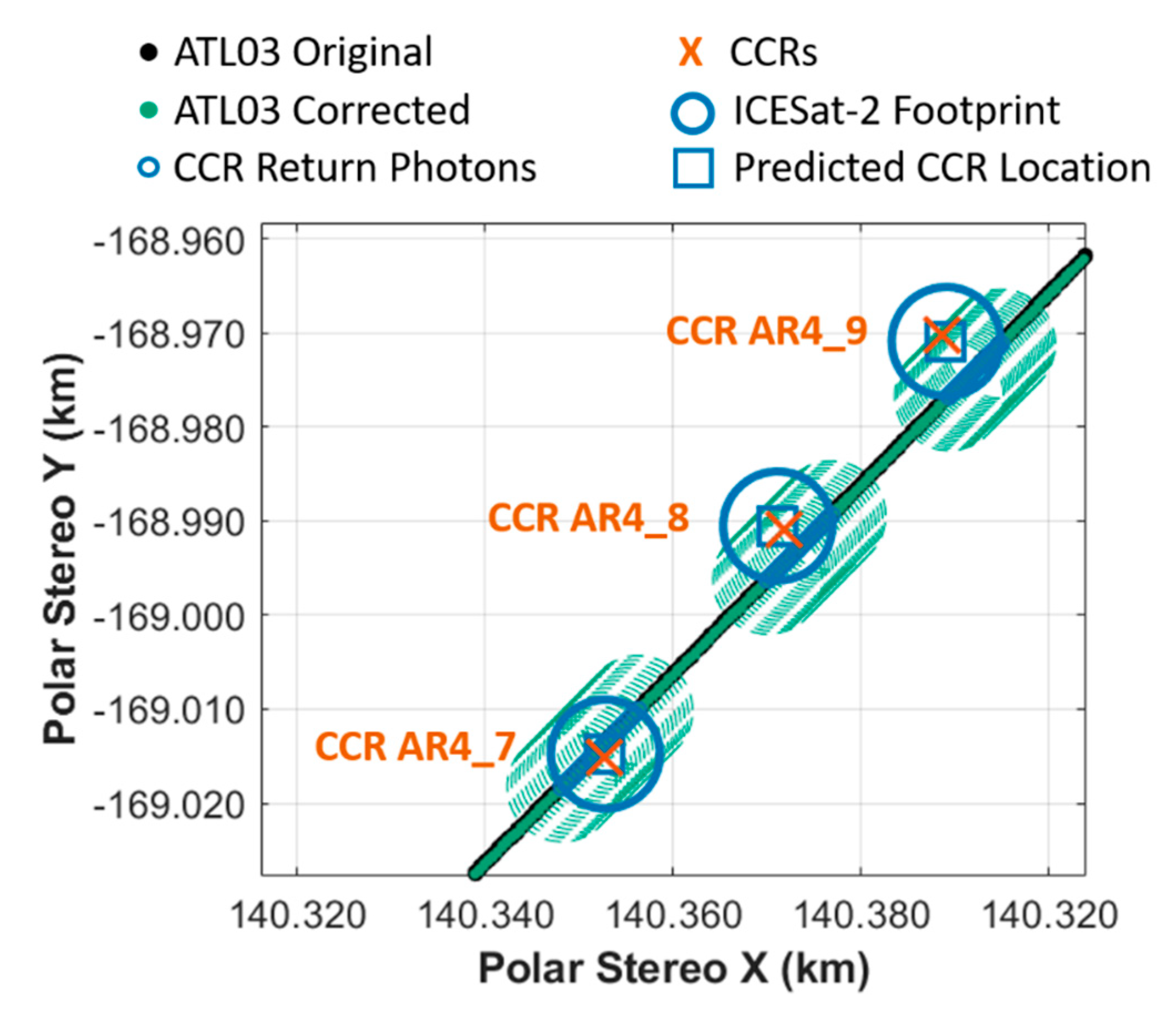
Figure 3 illustrates the case where the CCR is still aligned with the centerline but there is geolocation error in the data. In this example, the predicted CCR position (blue square) using the mid-point of the CCR signature is not equal to the actual CCR position (red X) such that ΔX and ΔY are the horizontal offsets and indicate the ATL03 geolocation accuracy.
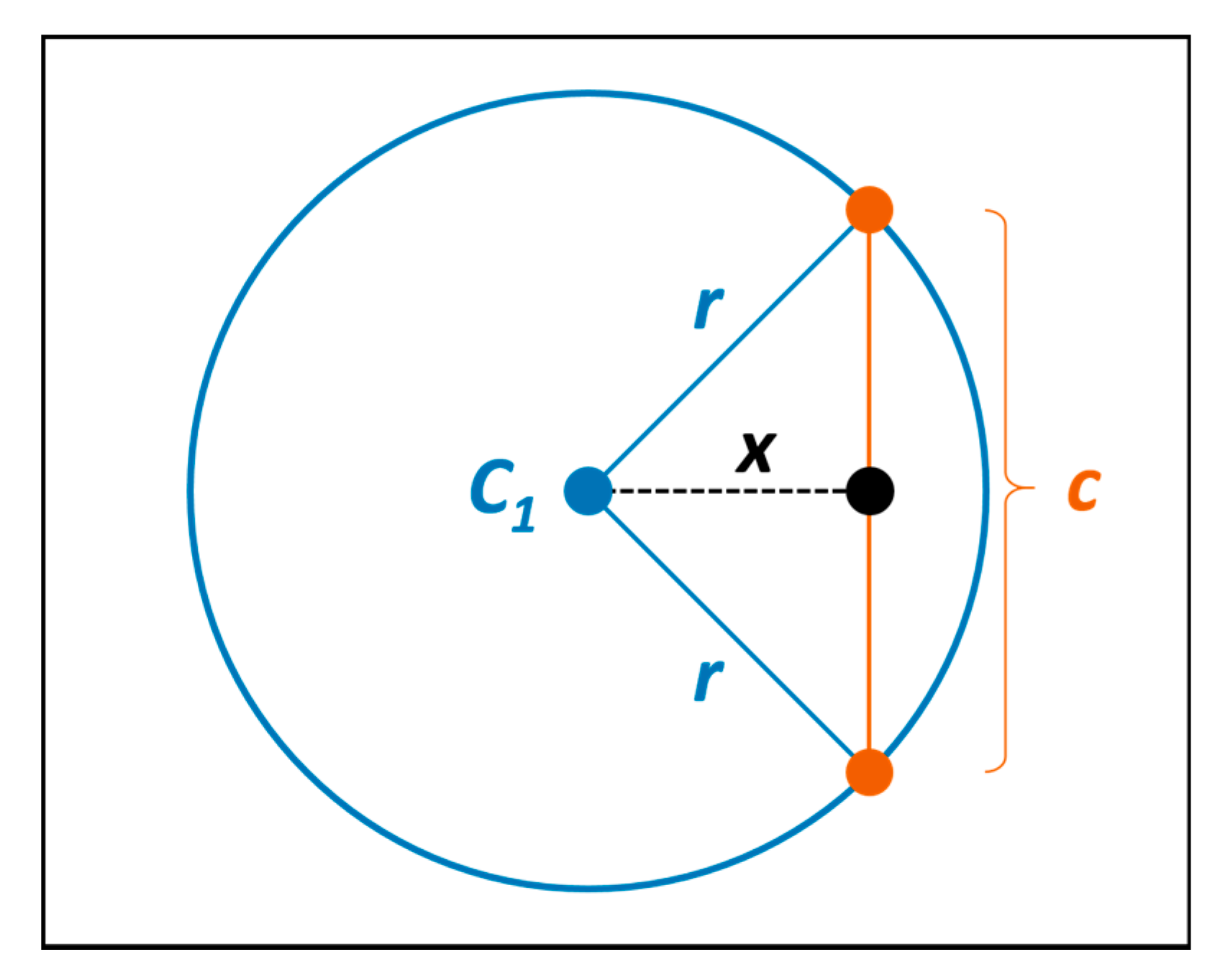
More complexity occurs when the CCR position and footprint centerline are not aligned. For those cases, the apparent along-track chord length of the CCR signature will decrease. This length will be inversely proportional to the across-track distance from the centroid of the footprint based on the geometry of a circle.
Figure 4 provides the relative chord length (c) of the CCR signature when its position (black dot) is a distance (x) from the footprint center (C
1). To determine the geolocation accuracy of the data, the horizontal offset between the CCR position and the footprint centroid must be calculated using simple geometry for a given footprint diameter and measured chord length.
Figure 5 depicts the scenario where the CCR and centerline are not aligned and there is a geolocation error. Similar to the other figures, the panel at the end of the time lapse of laser shots provides the results for the along-track (ΔY) and across-track offset (ΔX) between the predicted footprint centroid and the CCR position. This is the applicable approach used for the ICESat-2 CCR based geolocation validation moving forward.
An important detail in the geometric analysis for ATL03 geolocation validation using CCR arrays is the approach for systematic chord length extraction from ATL03 data to ensure statistical consistency for multiple overpasses and different ICESat-2 beams. Although the diagrams presented in
Figure 1,
Figure 2,
Figure 3,
Figure 4 and
Figure 5 clearly show a very stark beginning and end of the CCR signature length in theory, the reality is much different given the variability with radiometric (signal strength) influences such as atmospheric attenuation, surface reflectance, solar background noise and beam energy.
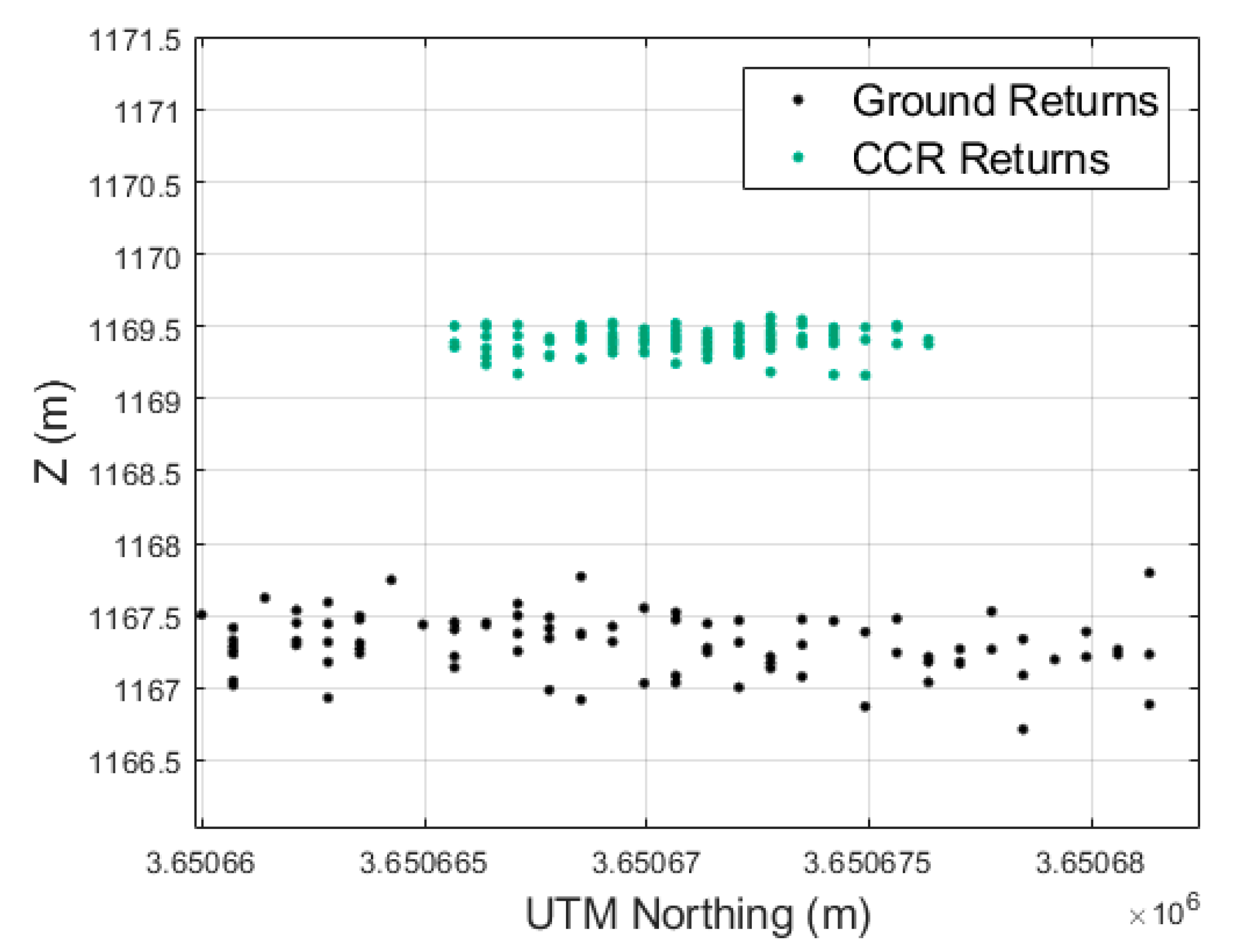
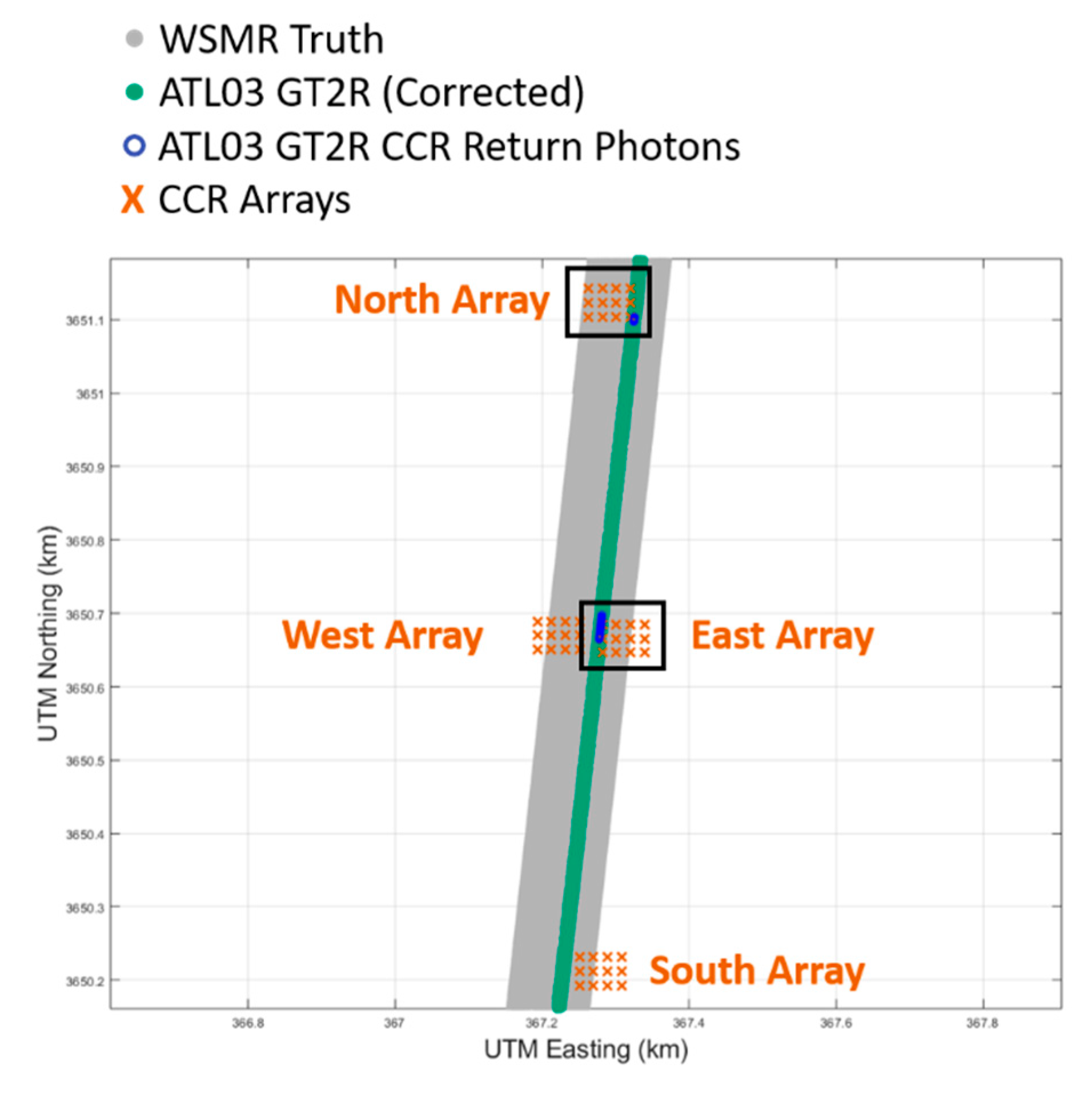
Figure 6 shows example CCR data from an ICESat-2 overpass collected at White Sands Missile Range (WSMR). The figure is a side view of the ATL03 geolocated point cloud, showing detected ground photons in black and CCR detected photons in green. Although not the case in this example, when heavy solar background noise is present, signal finding can be more difficult.
Typically, laser diameters are the equivalent to the 1/e
2 Gaussian beam diameter; representative of 86.6% of the total energy spatial profile. It is entirely possible to detect CCR returns from outside of the 1/e
2 boundary based on theoretical calculations, but those are more susceptible to radiometric losses. As such, the 1/e
2 assumption provides standardization with the fact that the 1/e
2 diameter is associated with a 2σ value of a Gaussian energy profile so the chord length extracted from the signature would mimic this estimation.
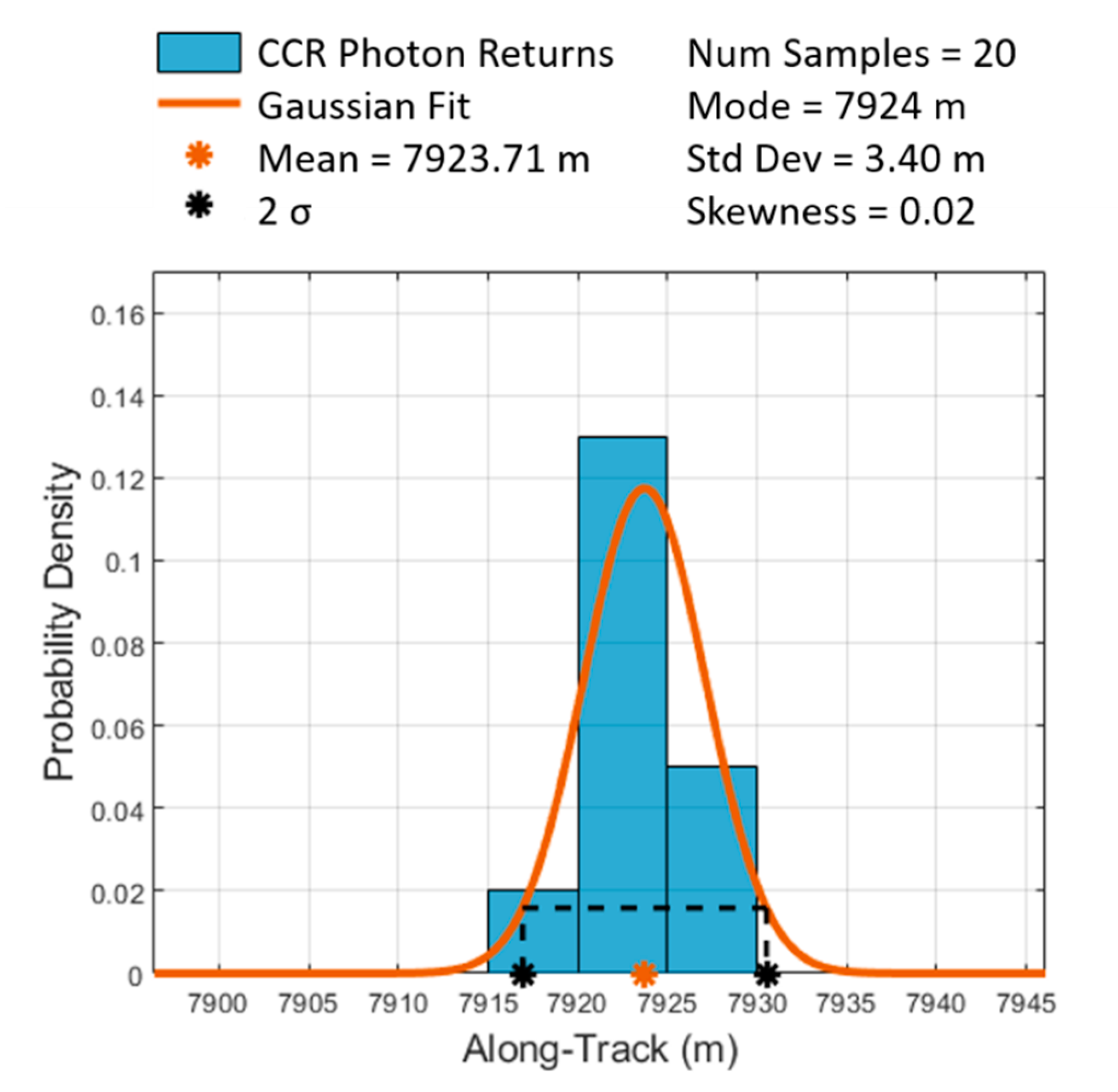
Figure 7 provides the statistical histogram representation of the CCR signature shown in
Figure 6 and the corresponding Gaussian profile. Although the along-track distance for those data in
Figure 6 is 10.1 m the 2σ value is 10.7 m. Pre-launch ATLAS laser measurements confirmed a Gaussian beam profile [
17] but certainly the CCR distribution profiles are not a perfect Gaussian representation, particularly when the sample size of photons is relatively small. More appropriate distribution statistics can be explored as more satellite overpasses are captured. However, as a preliminary effort the Gaussian fits provide the means for exploring the efficacy of the technique and have skew values that are still considered approximately symmetric.
The CCR return photon chord length geometrically constrains the geolocation solution but only if the diameter is known to determine the distance of the CCR position relative to the centerline. Although pre-launch estimates of diameter exist there is no on-orbit method capable of confirming the true operational value. Furthermore, even if the diameter is known if there is only one CCR illuminated, the symmetry of the spot creates ambiguity as to if the CCR position is east or west of the centroid. The mitigation for these uncertainties is the collection of multiple CCR returns within one overpass. The analysis of the signals in concert create a unique scenario purely based on the relative geometry of the CCR positions in the array and chord lengths of the signal signatures. This creates a deterministic method for finding the effective diameter of the laser footprint and the geolocation simultaneously.
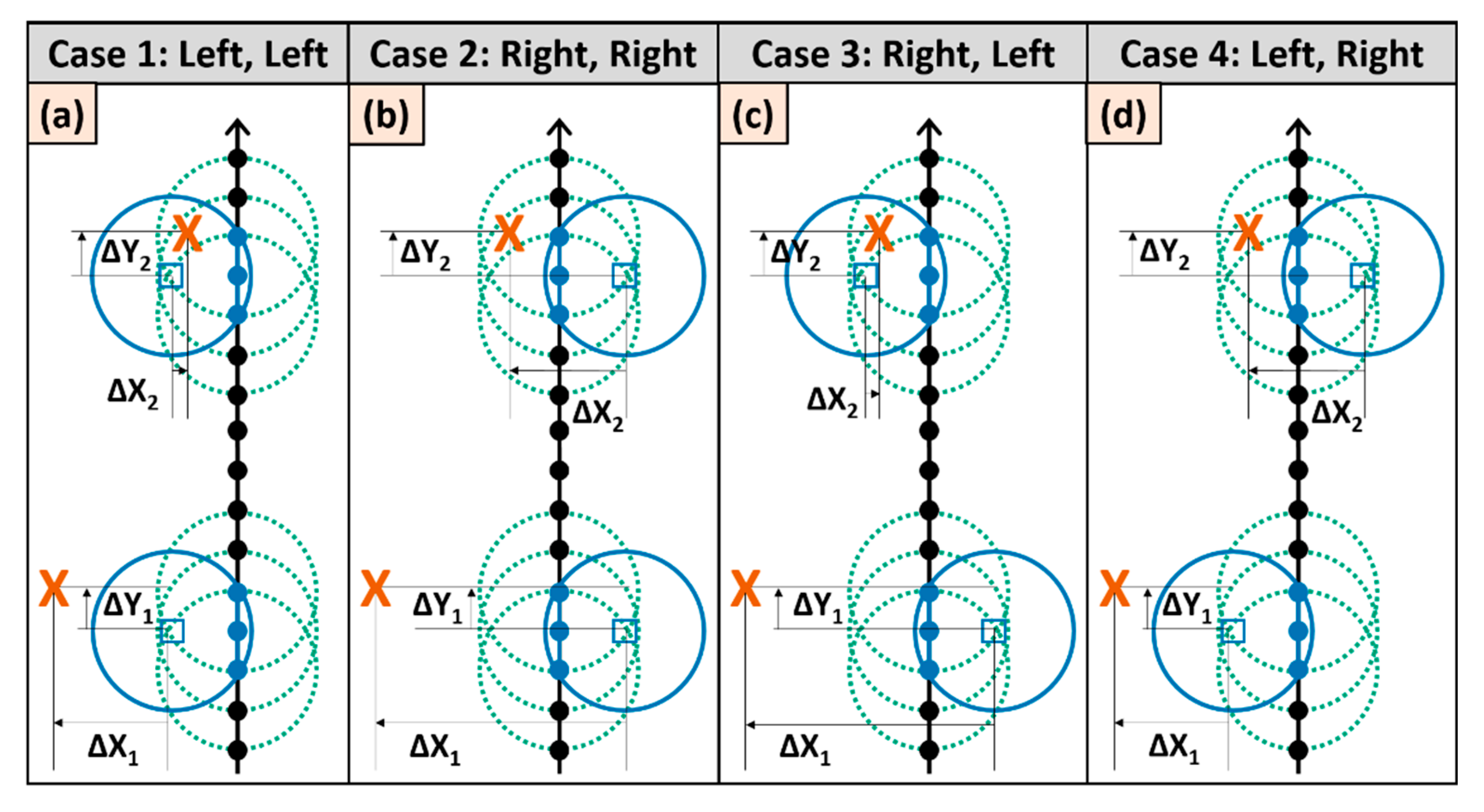
The comprehensive (diameter and geolocation accuracy) CCR analysis approach calculates both through an iterative process, varying the diameter and finding the best fit to the geometric constraints.
Figure 8 shows one iteration for diameter recovery for which the laser ground track passes to the east of two CCRs (panel 1). Starting with an initial estimate of footprint diameter, the algorithm determines the geolocation offsets (ΔX, ΔY) for each CCR signature. Since the goal is to determine one final geolocation offset for the track, the mean of the offset contributions from the individual CCRs is used, denoted as ΔX
μ and ΔY
μ in Panel 3 of
Figure 8. To assess the geolocation solution, the root mean squared error (RMSE) between all centroid predictions and CCR positions is calculated with the idea that the minimum RMSE is representative of the best fit to the geometry. This process is performed for all possible footprint diameters at 10 cm increments from 5 to 20 m and repeated for all footprint configurations, e.g., the relative track position to the CCR positions. The number of configuration combinations is 2
N, where N is the number of CCRs illuminated within one overpass. For the case presented in
Figure 8, there will be four possible geometries evaluated for mean geolocation offset and RMSE of the solution for a given diameter (
Figure 9). The lowest RMSE scenario will provide a final geolocation accuracy and estimate of the laser footprint diameter.
2.2. Ground-Based Validation Implementation: White Sands Missle Range (WSMR), New Mexico
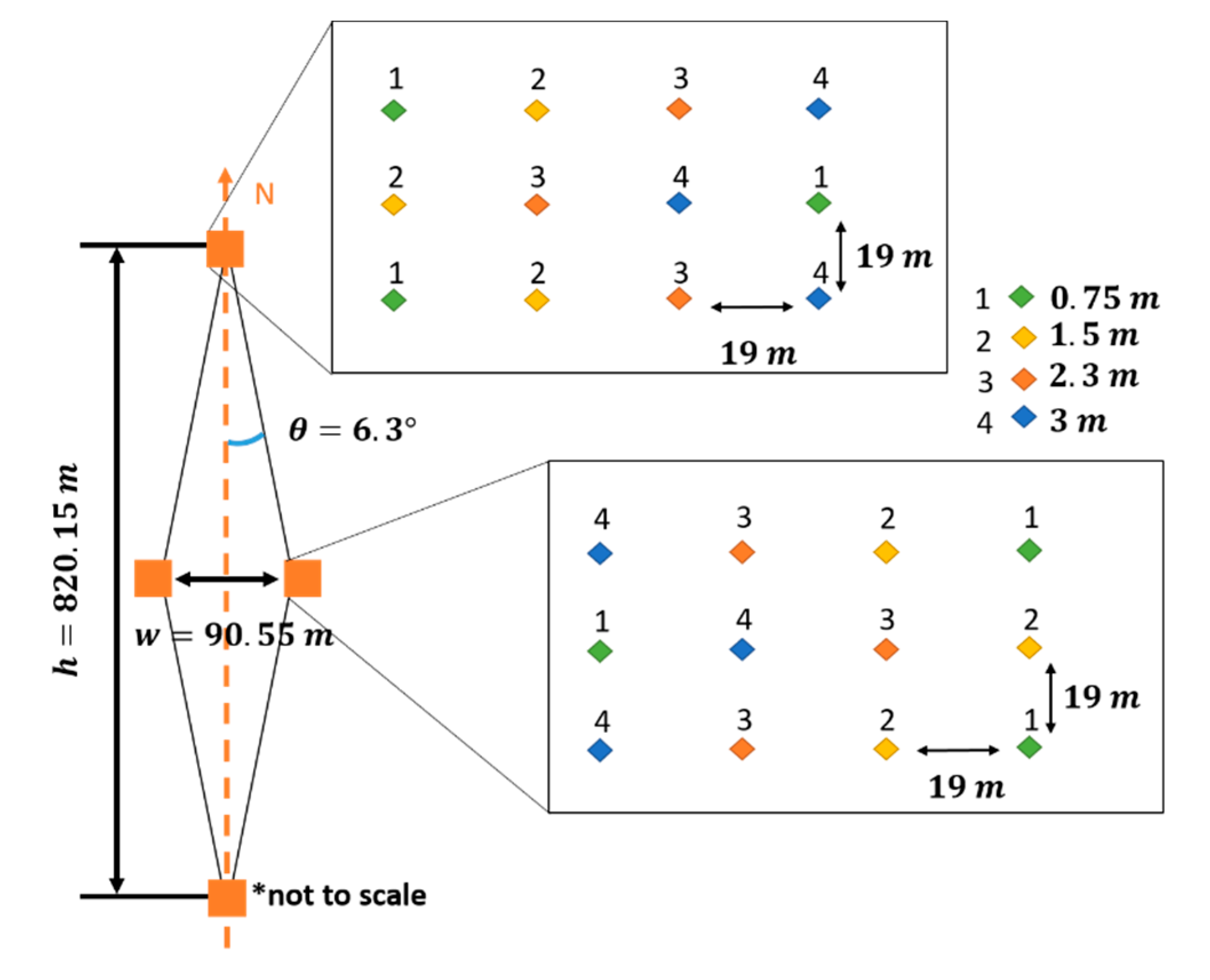
The analysis to determine the precise location, configuration and orientation of the CCR arrays was based on the pre-launch details for ATLAS beam spacing on the surface and the planned reference ground tracks. The spacecraft orbit is inclined at 92° relative to the celestial equator which means 2° from a true polar orbit [
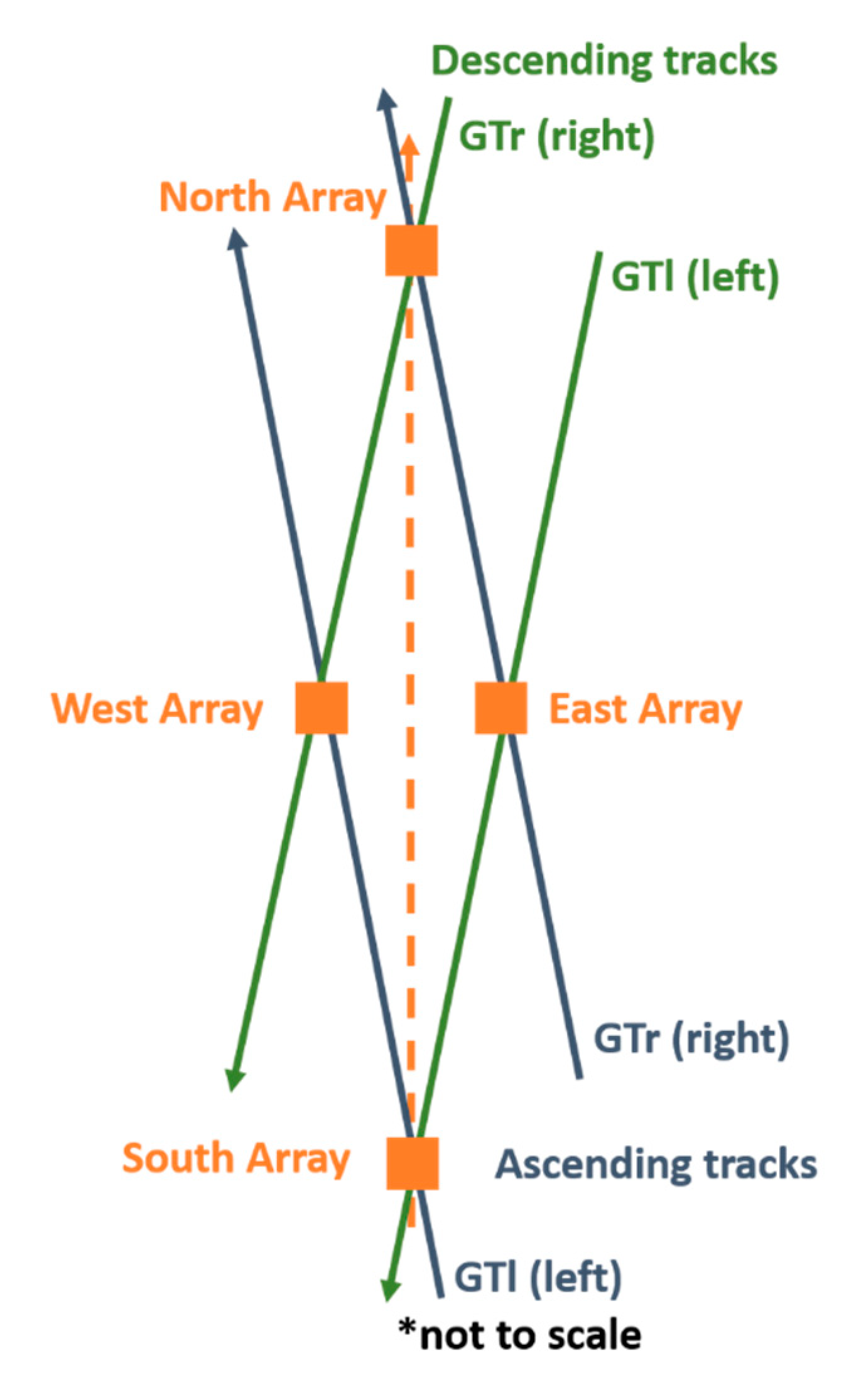
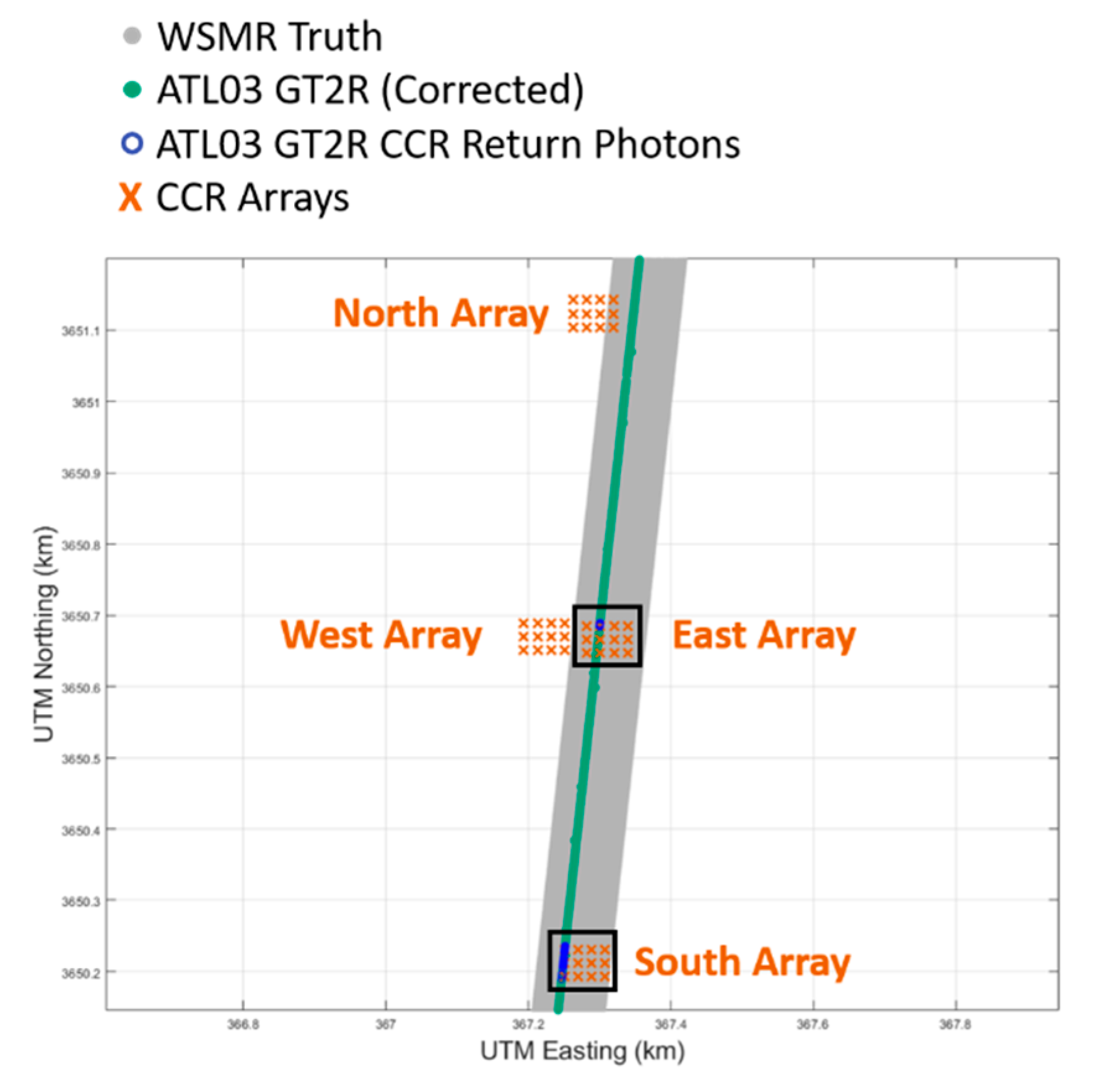
2]. The inclination creates a 6.3° angle relative to a north/south orientation of the satellite ground tracks at the WSMR latitude. The ground track orientation is an important geometry consideration in the physical design of the CCR arrays in the hope to capture multiple CCR signatures for a beam pair (weak and strong) during a single overpass. The global design of the arrays uses the diamond pattern (
Figure 10) created by the descending and ascending reference ground track intersections in nearest proximity of the target location and uses each of the four diamond vertices as a center point position for a local array. These four array locations coincide with the orange squares in
Figure 10. Since the array design goal is to capture one of the three beam pairs during a single overpass, it is important to understand how the pairs are identified. For the three pairs, pair 1 is on the left side of the satellite relative to the direction of motion. The individual components within the pair are deemed Ground Track 1 left (GT1l) and Ground track 1 right (GT1r). The nadir beam pair is pair 2 and the pair to the right of the satellite direction of motion is pair 3. Those individual beams are GT2l, GT2r, GT3l, and GT3r, respectively. The array positions, in
Figure 10, are designed to capture GTxl with the east and south array and GTxr with the north and west arrays for a descending pass. For an ascending pass the same configuration will capture GTxr with the east and north arrays and GTxl with the south and west respectively. The geographic distances of the arrays and the selected spacing of the CCRs within the arrays is provided in
Figure 11.
Although one ICESat-2 reference ground track (RGT) is planned to directly pass over the arrays, additional ground tracks in near proximity will require the observatory to point off-nadir to this target of opportunity (TOO). TOOs can be implemented via uploaded instrument command files if not in violation of the operational limit of 5°. These off-nadir instances are important for validation studies as they often magnify errors or biases in the position or pointing determination solutions not normally observed during typical near-nadir pointing [
18,
19,
20]. The success of a TOO is indicative of the observatory pointing control capability and confirms that the instrument command process, and on-board attitude control systems are working appropriately.
As previously discussed, the correlation of corner cube position and the resultant signal along-track chord length provides the opportunity to determine both the ground track’s positional offsets and the laser footprint diameter. However, there remains a need to appropriately identify which CCR belongs to a specific signature. If that information is unknown, it will be impossible to determine the appropriate geometric constraint. That said, if the CCR mount heights within an array are varied, any given linear (along-track) signal detection sequence is unique with respect to the vertical offsets within the array.
Figure 11 depicts the array content in terms of individual mount heights, sufficiently populated so as to increase the likelihood of ICESat-2 ground track intersections and to support unambiguous determination of CCR ownership to those signatures in the ATL03 product.
The deployment of the CCR arrays was completed just prior to the satellite launch date to ensure they were assembled in time for early on-orbit validation but were not overly-exposed to environmental conditions that might degrade the optics unnecessarily. The CCRs were embedded in 0.4 m PVC caps customized to hold the 8 mm diameter optical components. These caps were placed on PVC pipes of variable length to adhere to the pattern shown in
Figure 11: 0.75, 1.5, 2.3, and 3 m. These heights were chosen based on the vertical resolution within ATLAS, as they would provide distinct elevation separation from the surface as well from each other. Furthermore, none of these mount heights created significant logistical challenges associated with securing in place as taller poles are at risk of breaking or collapsing in the known environment experienced previously with the validation efforts for ICESat. The pipes were secured to the surface with partially submerged metal rebar and support wires. The position of each CCR was surveyed with differential Global Navigation Satellite System (GNSS) for a geolocation accuracy to within 1 m.
Another method for independent ICESat-2 geolocation validation can be accomplished by comparing the satellite data to known, high-resolution datasets. These “reference” surfaces provide accurate characterization of the region that can be directly compared to the ICESat-2 ATL03 elevations as well as the higher-level geophysical data products at variable length scales. The University of Texas at Austin Bureau of Economic Geology performed an airborne lidar survey over the region of WSMR within the broader range perimeter using a Leica Chiroptera lidar system and a high-resolution imager. The airborne collection acquired both near-infrared (NIR) and visible (green) data at ~10 pts/m2. Accuracy of the lidar dataset was enhanced using GNSS base-stations for data post processing. These receivers enabled differential solutions to produce the most accurate lidar measurement for surface characterization. Based on the effective post processing and the characteristics of the Leica system the airborne measurements provide, on average, better than 10 cm vertical accuracy and 1 m horizontal. The terrain is somewhat homogeneous at WSMR but there are distinct geophysical features within the scene that can be used as ground control, or reference points in the comparison to those relevant ICESat-2 transects across the region. This method provides an independent assessment of both the horizontal and vertical accuracy of the ATL03 product and was also planned to validate the results of the CCR proof of concept for geolocation validation.
2.3. Ground-Based Validation Implementation: 88S Traverse
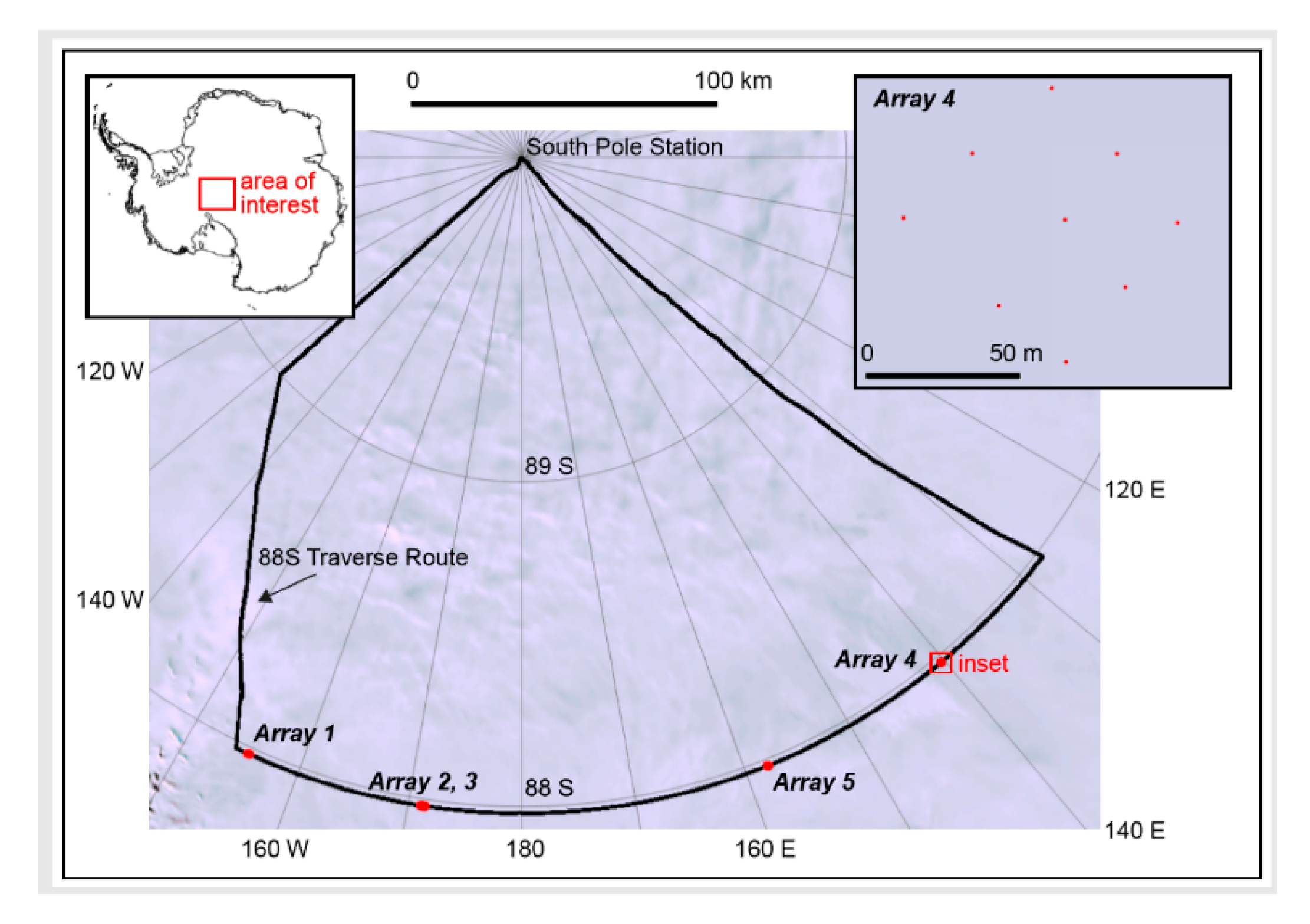
Since the validation efforts at WSMR provide assessment of the satellite measurement accuracy at a single northern-hemisphere point within the orbit, CCR arrays were also deployed along a portion of the 88° South (88S) line of latitude in Antarctica, where a large-scale ICESat-2 validation effort, referred to as the 88S Traverse, is underway [
21]. Given that ICESat-2 has an orbital inclination of 92°, the orbits converge along 88S, making it a dense region of ground tracks and cross-over points.
A total of 5 small arrays were deployed along the 88S Traverse during the two different traverse seasons (2017–2018 and 2018–2019). The geographical location of the traverse and the CCR arrays are provided in
Figure 12. The arrays contained anywhere from 4 to 9 CCRs and were mounted on bamboo poles using PVC caps. The bamboo poles varied in height from ~0.5 m to just under 2 m, in patterns such that a given CCR was distinguishable from the neighboring CCRs, similar to the concept of
Figure 3. The spacing between the bamboo poles was ~30 m, such that the spacing was larger than the ICESat-2 expected footprint diameter ~17 m, but much smaller than the across-track spacing of the ICESat-2 beams (~90 m) and the mission requirement of spacecraft pointing control (45 m).
The positions of the bamboo poles were measured using kinematic GNSS methods and Precise Point Positioning post-processing techniques in a commercial software package [
22]. Metal poles were also deployed in the center of each array to allow for easy resurveying of a single position of the array to assess horizontal movement of the arrays due to ice-sheet flow; the positions of the metal poles marking the centers of the arrays that were deployed during the first field season were resurveyed during the second season.
Revisiting and resurveying the arrays in the second season provided insight into the stability of this surface with respect to validation of both satellite ranging and the horizontal movement of the ice sheet (~0.3 m/year). The snow surface in this region is extremely flat on large length scales, varying by only 100 m over 300 km, but is relatively rough on short length scales. This roughness is associated with sastrugi, or fields of wind-driven ridges that are ~1 m high on ~10 m length scales. The position of sastrugi in the vicinity of our CCR arrays changed between seasons 1 and 2, as expected [
23]; thus, during the second survey of the arrays, the relative heights of the bamboo poles changed non-uniformly. Most poles were still within the height range of ~0.5 m to just under 2 m; one bamboo pole was 0.48 m while another bamboo pole was anomalously low at 0.06 m. The horizontal displacements due to ice flow of five of the metal poles marking the centers of the arrays were extremely small, with a mean value 0.35 m. Thus, CCR arrays along the 88S Traverse are an excellent means of validation of horizontal accuracy and to assess the size of the ICESat-2 footprint, but these arrays are not ideal for the validation of satellite ranging as the pole heights are relative to the changing surface.