The Applicability of an Inverse Schlumberger Array for Near-Surface Targets in Shallow Water Environments
Abstract
1. Introduction
- (i)
- Repeated measurements of parallel near-shore profiles in about 1 m water depth in different measurement setups to obtain a dataset that enables the statistical inquiry of measurement uncertainties and inversion reproducibility.
- (ii)
- Theoretical considerations based on forward modelling and inverse modelling to estimate the spatial resolution and depth penetration in 1D and 2D resistivity model data.
2. Materials and Methods
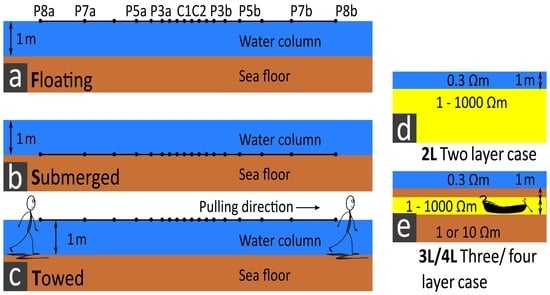
2.1. Measurement Configurations, Field Measurements and Investigated Materials
2.2. Repeated Field Measurements
2.2.1. Measurement Uncertainty
- (a)
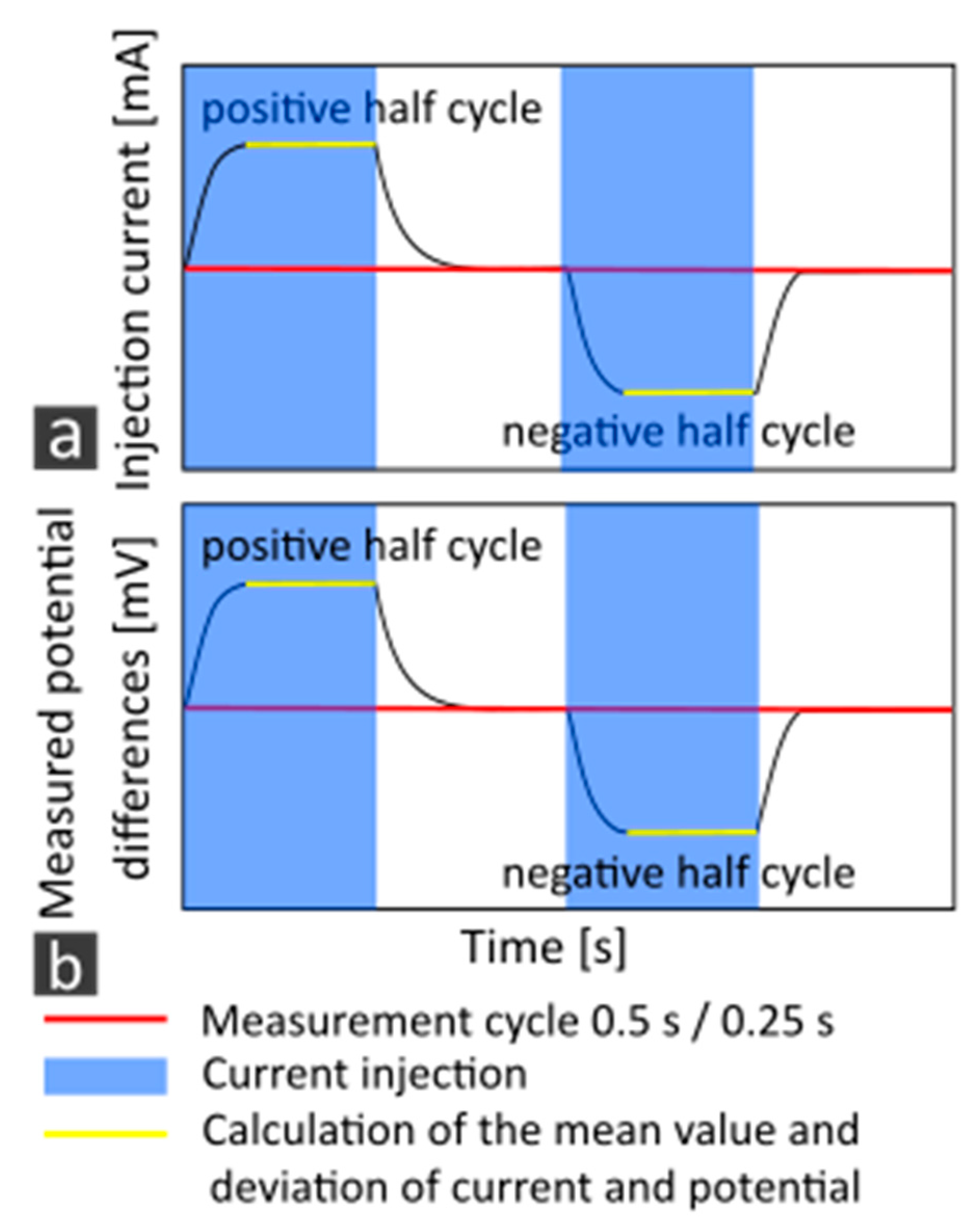
- Noise: As it is common for geoelectric instruments, the injected currents and related potential differences are reversed in polarity during one measurement cycle and follow typical rise and decay time functions (Figure 3). The recording unit evaluates the quality/noise level within the time intervals of the plateaus of the step functions and accepts or declines a single apparent resistivity value due to a selectable threshold. Single events, such as water waves, contribute to the noise level and are thus recorded by the acquisition unit. We quantify “noise” as the standard deviation of a single measurement within the time intervals of two half cycles (Figure 3, yellow line) during a measurement cycle of 0.25 or 0.5 s (Figure 3, red line) from their average value corresponding to the measured resistivity value [13].
- (b)
- Smearing effects: In setup T, the continuous movement of the electrode streamer causes a spatial “smearing” of data values along the profile (“inline”) during one cycle. This also affects the standard deviation of the single measurements within the intervals of two half cycles (Figure 3, yellow line) and can therefore not be distinguished from noise. Therefore, we do not consider this effect separately. It results in an increased standard deviation for setup T and is included in the term “noise”.
- (c)
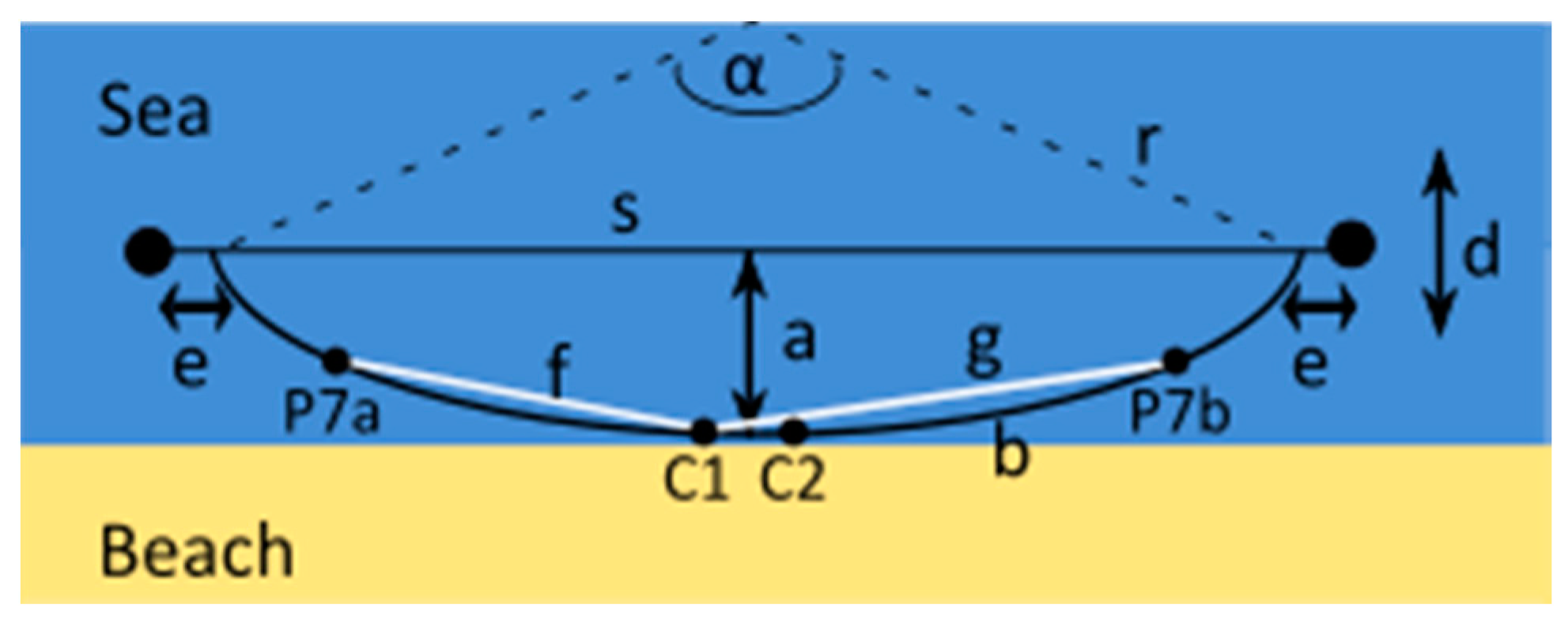
- Streamer deflections: Uncertainties in apparent resistivity are also caused by deflections of the electrode streamer that result from wind and swell, especially during towed prospections. Streamer deflections change the electrode spacing and, therefore, the geometry factor required for computing the apparent resistivity values. To estimate this error type, we assume a relative inline displacement e = 10 cm of each streamer end with respect to their midpoint (Figure 4). Since the length b of the streamer remains constant, this displacement causes a deflected streamer that we assume to be approximated by the shape of a circle segment, such that the streamer midpoint is displaced by a distance, a, orthogonally from the inline direction. In this geometry, the streamer is an arc segment of length b and its ends are connected inline by a secant of length s. The midpoint displacement a can be calculated using the center angle of the arc α and the circle radius r according to:From this, the geometry factor k can be calculated from the symmetrical distance of and (Figure 4) for floating electrodes:and for submerged electrodes using the mirror source technique, depending on the water depth tw:The uncertainty for the apparent resistivity of an electrode pair results from the difference of the geometry factor for the straight and deflected cable and the arithmetic mean value for the repeated measured current and voltage.
- (d)
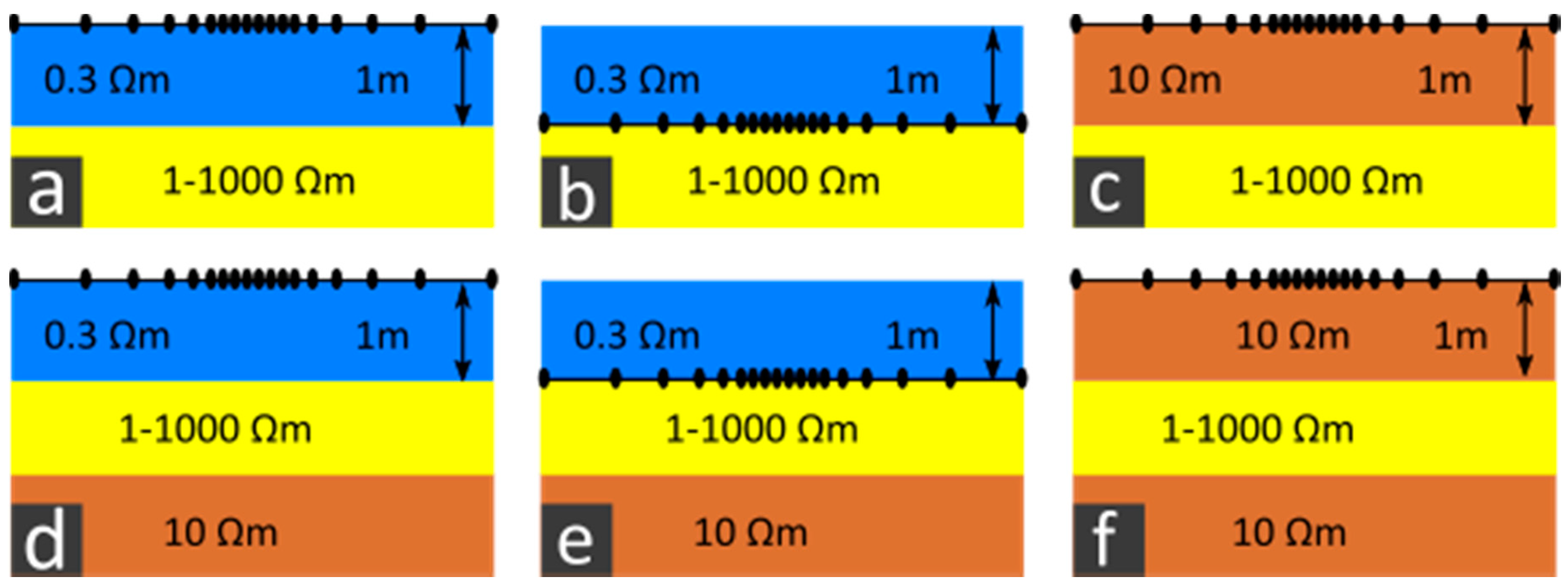
- Effective water-level variations: There are two effects, which we combine into an “effective water-level variation”. The first one is swell, which displaces the electrodes in the vertical direction, thus changing their distance to the sea bottom. It depends on the speed, lengths and heights of the water waves. The second effect is the possible variation of bathymetry in the cross-line direction, which affects the measurements through the varying cross-line deflections of the streamer. Quantifying these effects in an exact way would require, besides bathymetry, accurate knowledge of the 3D positions. As this is hardly achievable, we attempt to estimate the order of magnitude of the resulting uncertainties by assuming that they can be modelled by the temporal variation of an “effective water-level”. For this purpose, we compute the apparent resistivity values for a set of two-layer (2L) models. The thickness of the upper layer, identified with the water column of 0.3 Ωm resistivity, is varied between 0.8 and 1.2 m in intervals of 10 cm. At an average water depth of 1 m, the curves correspond to an effective water-level variation of ±10 and ±20 cm. For the bottom layer, resistivity values of 1 and 100 Ωm were chosen. A bottom resistivity of 1 Ωm corresponds to saturated sand or clay, whereas a bottom resistivity of 100 Ωm corresponds to saturated limestone [14]. In order to estimate the measurement uncertainty for the different setups, an effective water-level variation of 5 cm was assumed for setup S, of 10 cm for setup F and of 20 cm for setup T. The uncertainty is defined to be the difference between apparent resistivity of the average water depth and of the maximum water displacement.
- (e)
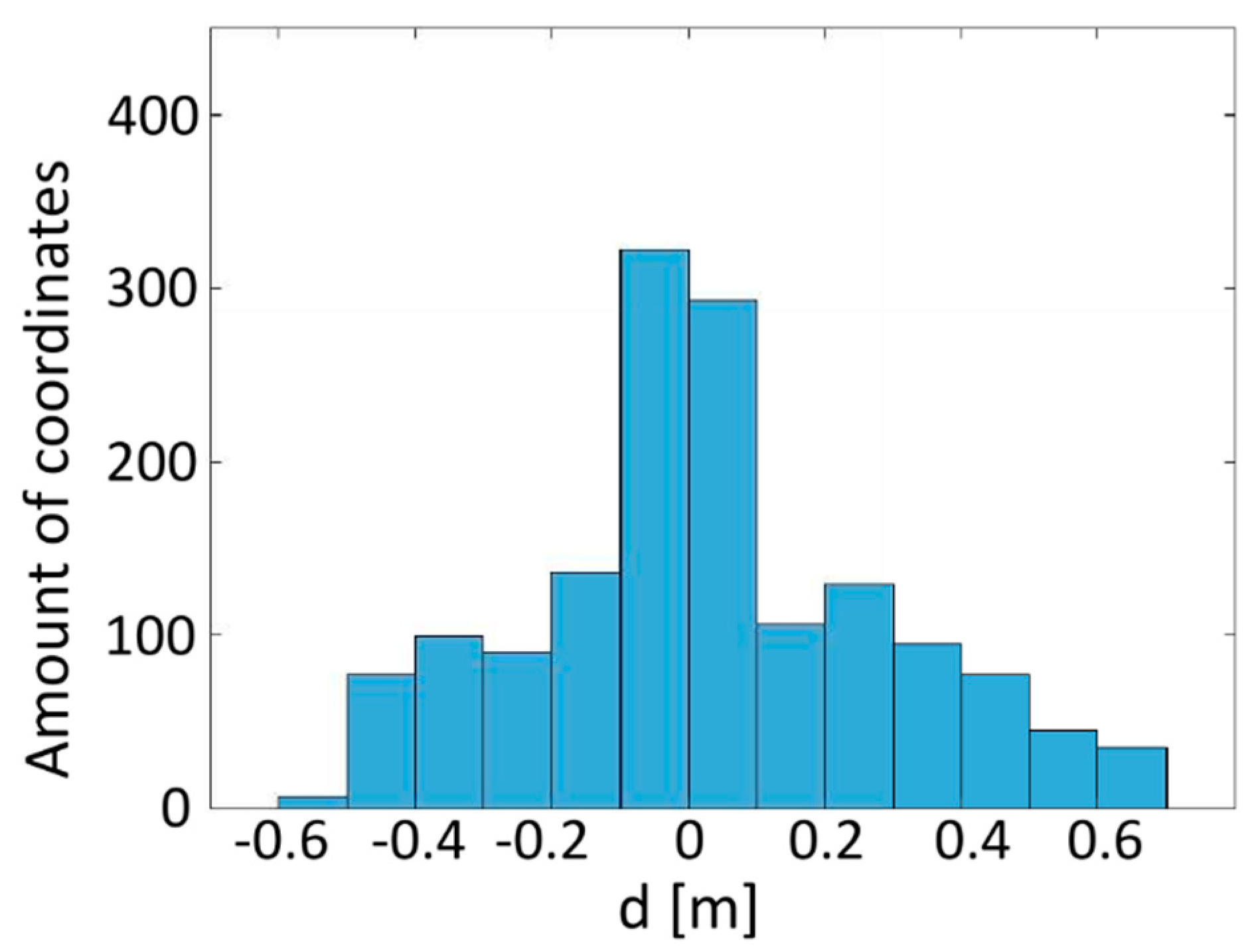
- Repeatability of profile locations: To complete the considerations on the errors of the three measurement setups (F, S, T), the general positioning uncertainty of the mid streamer point was evaluated in inline and cross-line directions using the data of setup T, which is assumed to have the largest uncertainties in positioning. This was estimated using the deviations of the GNSS (global navigation satellite systems) coordinates from the mean profile track, the possible streamer deflections and the inline “smearing interval length” of data due to the streamer movements during a measurement cycle for setup T. The positioning uncertainty provides effective water-level variations in a sloped seafloor, similar to cable deflections, and thus uncertainties in apparent resistivity. They have been summarized above in point (d). In addition, the positioning uncertainty restricts the prospection of laterally limited objects.
2.2.2. Inversion Reproducibility
2.3. Resolution of Layer Thickness and Resistivity in 1D Media
- Forward modelling of layered media, to investigate which apparent resistivity values and layer thicknesses can be differentiated due to field measurement inaccuracies in a number of scenarios for setups F, S and T.
- Stochastic inversion of the forward modelled data for setups F and S to investigate how the results of the forward modelling affect equivalent solutions of layer thickness and resistivity during the inversion.
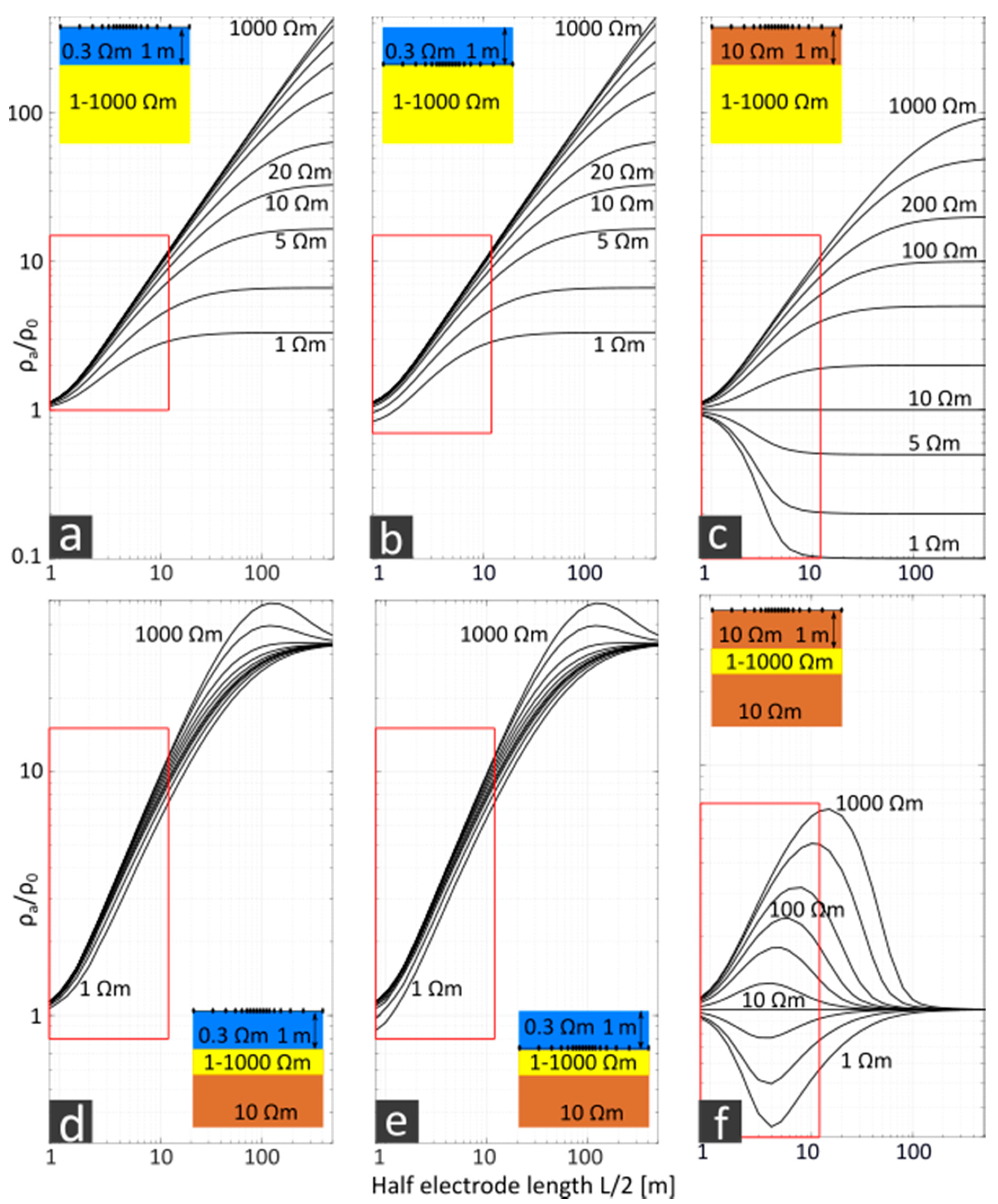
2.3.1. Forward Modelling of Layered Media
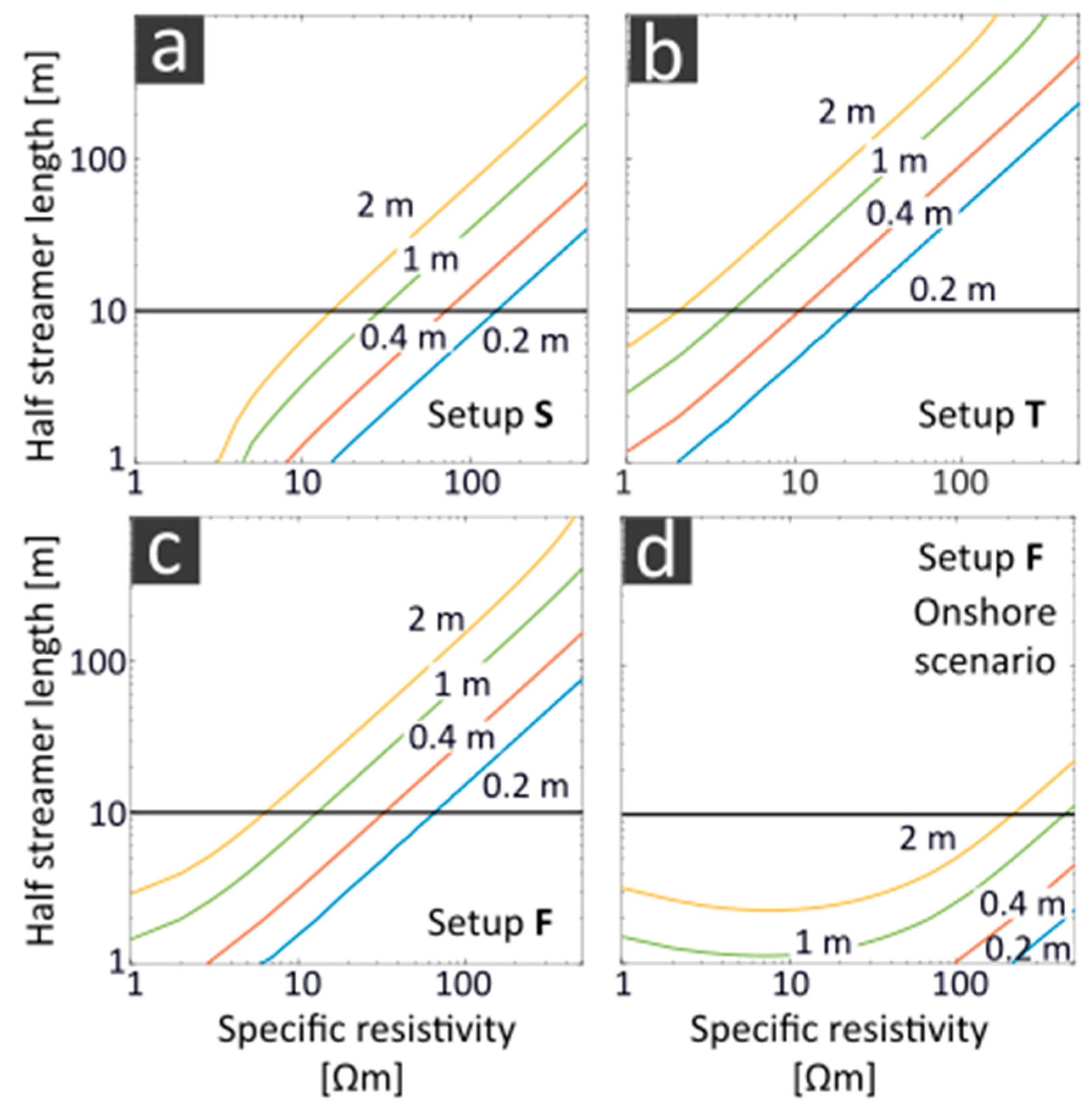
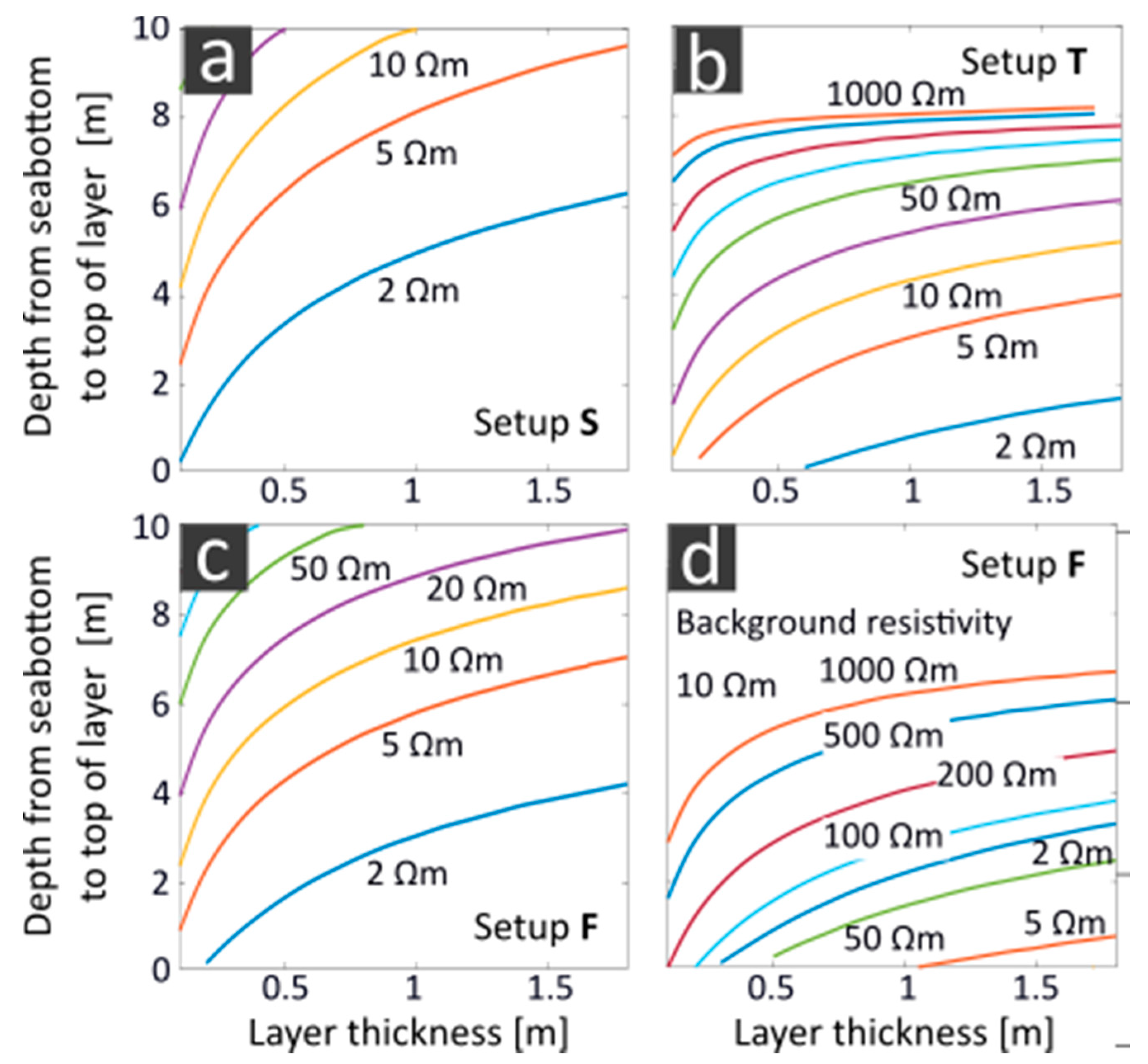
- We calculated the required streamer length for an inverse Schlumberger configuration to differentiate the specific resistivity of the subsurface from a 2L case. We distinguished between a shallow water and an onshore scenario, in which the depth of the first layer is varied.
- We defined a 2L shallow water scenario with a brackish water column of 1 m depth and a subsurface resistivity of 1, respectively 10 Ωm. The streamer used for the field measurements in Section 1 was chosen for the measurement configuration. We inserted a layer of variable thickness and resistivity into the homogeneous half-space of the 2L case at a variable depth, resulting in a 3L or 4L case. We investigated the conditions at which the layer can still be differentiated from the homogeneous half-space.
- 1.
- Required Streamer Length in the 2L Case
- 2.
- Resolution of a Layer in the Sea Bottom (3L/4L Case)
2.3.2. Calculation of Equivalent Model Solutions by Stochastic Inversion by Particle Swarm Optimization
2.4. Resolution of Layer Thickness and Resistivity in 1D Media
- Calculate sensitivity kernels for setup F, S under consideration of the water column.
- Perform checkerboard tests for setup F, S.
2.4.1. Sensitivity Kernels
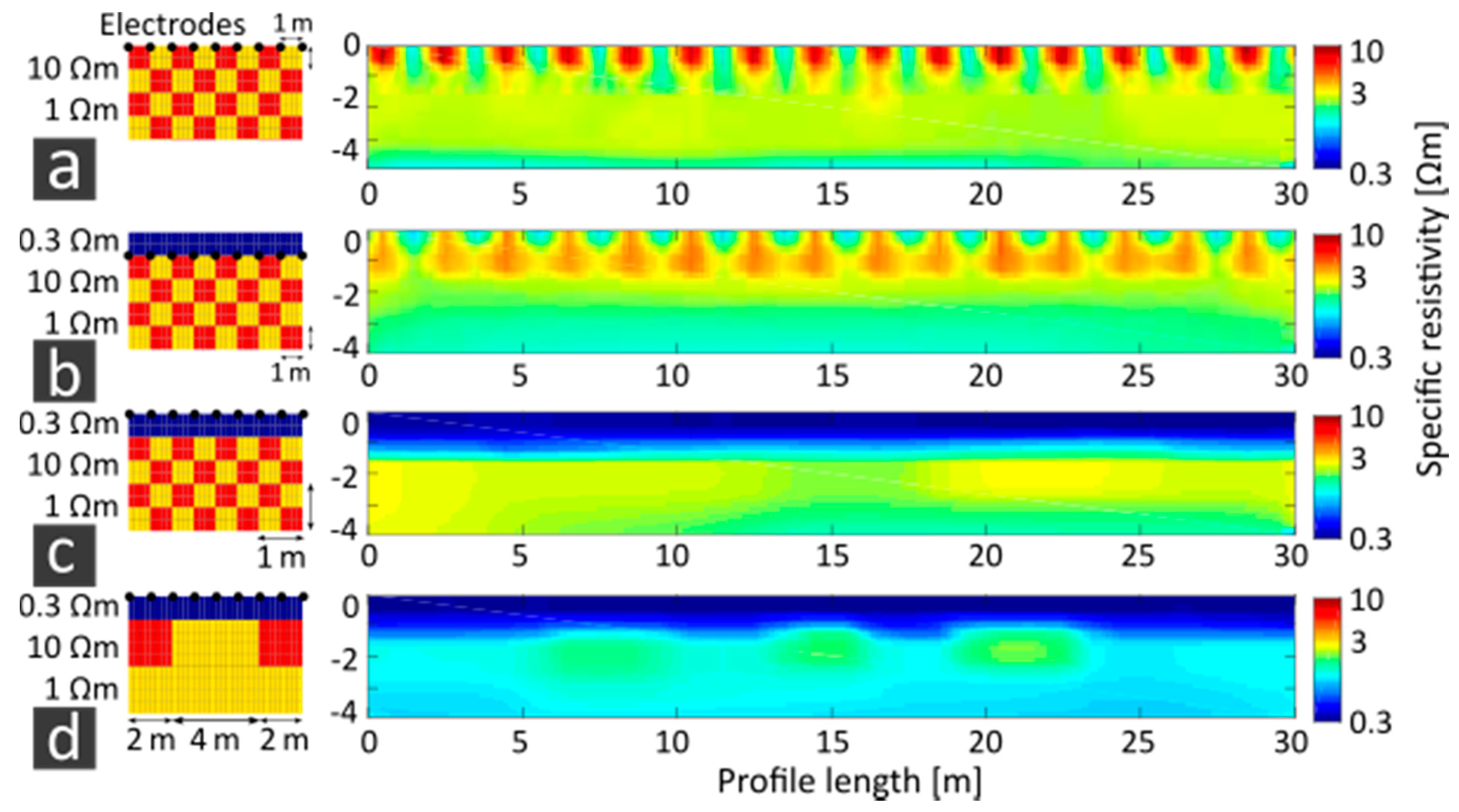
2.4.2. Checkerboard Tests
3. Results
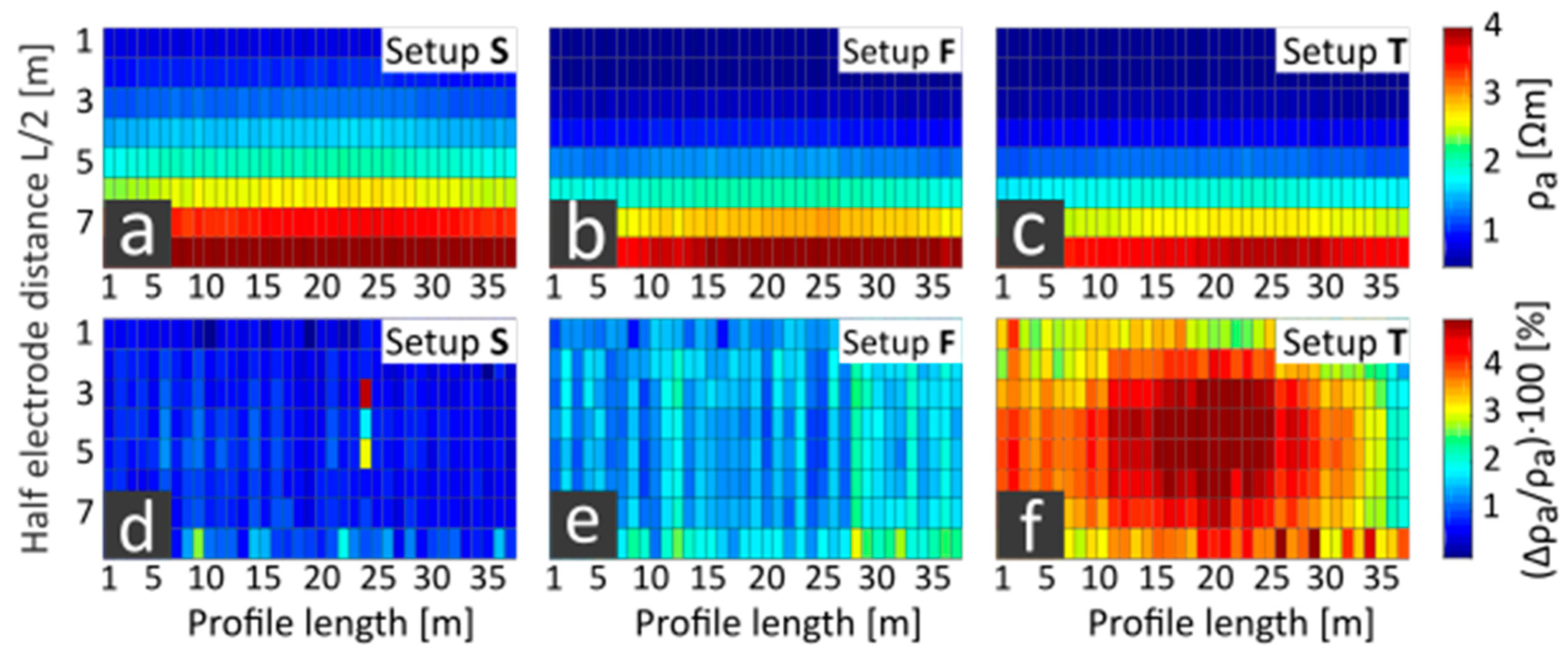
3.1. Repeated Field Measurements
3.1.1. Measurement Uncertainty
3.1.2. Inversion Reproducibility
3.2. Resolution of Layer Thickness and Resistivity in 1D Media
3.2.1. Forward Modelling of Layered Media
Sounding Curves for the 2L and 3L Case
Required Streamer Length in the 2L Case
Resolution of a Layer in the Sea Bottom (3L/4L Case)
3.2.2. Calculation of Equivalent Model Solutions by Stochastic Inversion Using Particle Swarm Optimization
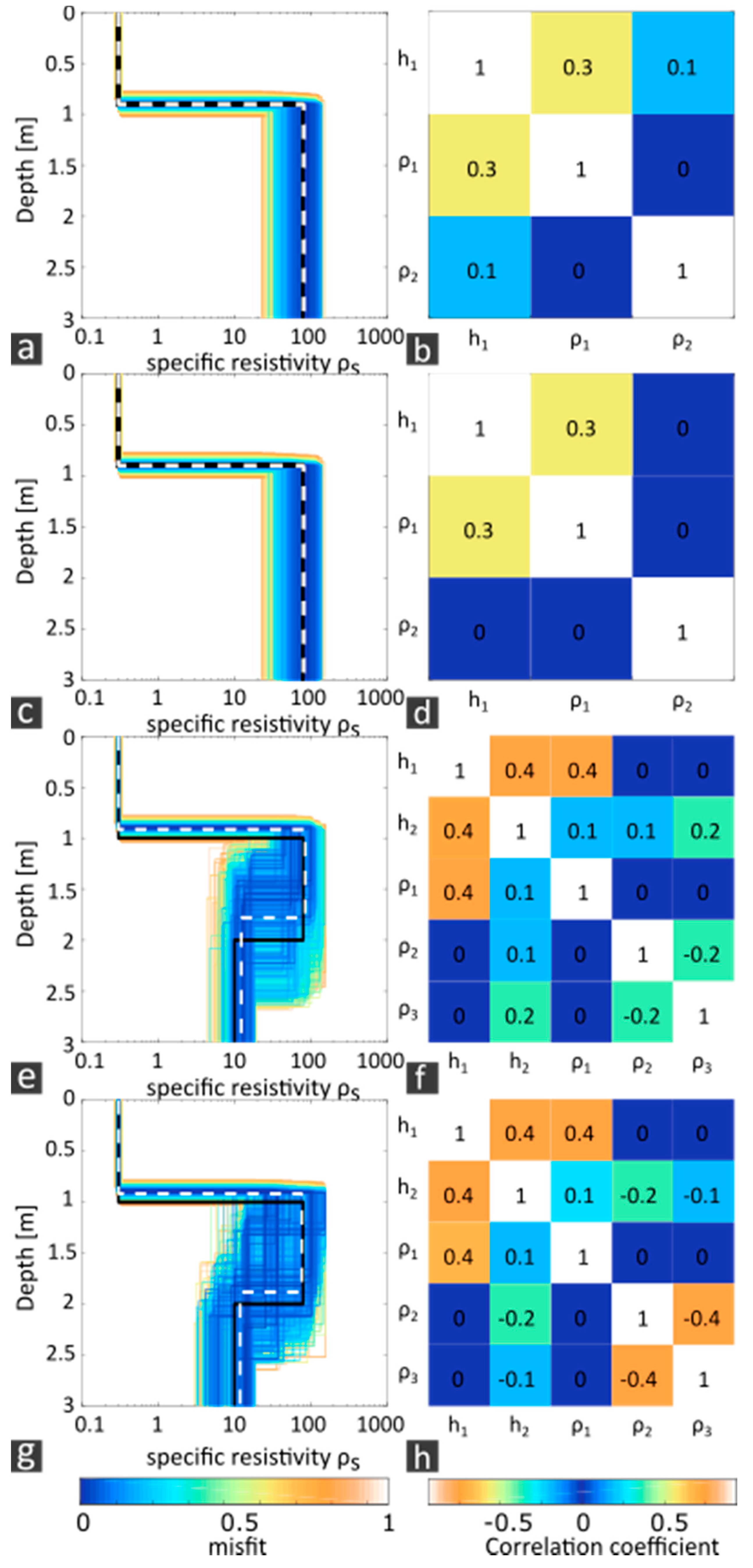
Resolution of Sediments Below the Water Column (2L Case)
Resolution of a Layer in the Sea Bottom (3L Case)
3.3. Spatial Resolution in 2D Media
3.3.1. Sensitivity Kernels
3.3.2. Checkerboard Tests
4. Discussion
4.1. Repeated Field Measurements
4.2. Spatial Resolution
4.3. Depth of Penetration
4.4. Inversion Ambiguity
4.5. The Preferable Measurement Setup for Prospecting a 2L and a 3L/4L Case
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
Resolution of Layer Thickness and Resistivity from Forward Modelling of Layered Media
Appendix B
Sensitivity Kernels
References
- Manheim, F.T.; Krantz, D.E.; Bratton, J.F. Studying ground water under Delmarva coastal bays using electrical resistivity. Groundwater 2004, 42, 1052–1068. [Google Scholar] [CrossRef]
- Okyar, M.; Yılmaz, S.; Tezcan, D.; Çavaş, H. Continuous resistivity profiling survey in Mersin Harbour, Northeastern Mediterranean Sea. Mar. Geophys. Res. 2013, 34, 127–136. [Google Scholar] [CrossRef]
- Snyder, D.D.; Wightman, W.E. Application of continuous resistivity profiling to aquifer characterization. In Proceedings of the 15th EEGS Symposium on the Application of Geophysics to Engineering and Environmental Problems, Las Vegas, NV, USA, 10–14 February 2002; European Association of Geoscientists & Engineers: Houten, The Netherlands, 2002. [Google Scholar]
- Papadopoulos, N.; Simyrdanis, K.; Theodoulou, T. Reconstructing the Cultural Dynamics of Shallow Marine Archeological Sites through Electrical Resistivity Tomography. In Proceedings of the Near Surface Geoscience 2016 22nd European Meeting of Environmental and Engineering Geophysics, Barcelona, Spain, 4–8 September 2016; European Association of Geoscientists & Engineers: Houten, The Netherlands, 2016. [Google Scholar]
- Simyrdanis, K.; Moffat, I.; Papadopoulos, N.; Kowlessar, J.; Bailey, M. 3D Mapping of the Submerged Crowie Barge Using Electrical Resistivity Tomography. Int. J. Geophys. 2018, 2018, 6480565. [Google Scholar] [CrossRef]
- Passaro, S. Marine electrical resistivity tomography for shipwreck detection in very shallow water: A case study from Agropoli (Salerno, southern Italy). J. Archaeol. Sci. 2010, 37, 1989–1998. [Google Scholar] [CrossRef]
- Lagabrielle, R. The effect of water on direct current resistivity measurement from the sea, river or lake floor. Geoexploration 1983, 21, 165–170. [Google Scholar] [CrossRef]
- Simyrdanis, K.; Papadopoulos, N.; Kim, J.H.; Tsourlos, P.; Moffat, I. Archaeological investigations in the shallow seawater environment with electrical resistivity tomography. Near Surf. Geophys. 2015, 13, 601–611. [Google Scholar] [CrossRef]
- Orlando, L. Some considerations on electrical resistivity imaging for characterization of waterbed sediments. J. Appl. Geophys. 2013, 95, 77–89. [Google Scholar] [CrossRef]
- Kwon, H.S.; Kim, J.H.; Ahn, H.Y.; Yoon, J.S.; Kim, K.S.; Jung, C.K.; Lee, S.B.; Uchida, T. Delineation of a fault zone beneath a riverbed by an electrical resistivity survey using a floating streamer cable. Explor. Geophys. 2005, 36, 50–58. [Google Scholar] [CrossRef]
- Baumgartner, F. A new method for geoelectrical investigations underwater. Geophys. Prospect. 1996, 44, 71–98. [Google Scholar] [CrossRef]
- Mansoor, N.; Slater, L. Aquatic electrical resistivity imaging of shallow-water wetlands. Geophysics 2007, 72, F211–F221. [Google Scholar] [CrossRef]
- GeoServe. Resecs 2015 Manual; GeoServe: Kiel, Germany, 2015. [Google Scholar]
- Schön, J. Petrophysik: Physikalische Eigenschaften von Gesteinen und Mineralen; Akademie-Verlag: Berlin, Germany, 1983. [Google Scholar]
- Archie, G.E. The electrical resistivity log as an aid in determining some reservoir characteristics. Trans. AIME 1942, 146, 54–62. [Google Scholar] [CrossRef]
- Fediuk, A.; Wilken, D.; Wunderlich, T.; Rabbel, W. Physical Parameters and Contrasts of Wooden Objects in Lacustrine Environment: Ground Penetrating Radar and Geoelectrics. Geosciences 2020, 10, 146. [Google Scholar] [CrossRef]
- Loke, M.H.; Barker, R.D. Rapid least-squares inversion of apparent resistivity pseudosections by a quasi-Newton method. Geophys Prospect. 1996, 44, 131–152. [Google Scholar] [CrossRef]
- Millonas, M. Swarms, Phase Transitions and Collective Intelligence. In Artificial Life III; Addison-Wesley: Boston, CA, USA, 1994; pp. 417–445. [Google Scholar]
- Wilken, D.; Rabbel, W. On the application of Particle Swarm Optimization strategies on Scholte-wave inversion. Geophys. J. Int. 2012, 190, 580–594. [Google Scholar] [CrossRef]
- Interpex. IX1D v3 Instruction Manual; Interpex: Golden, CO, USA, 2008; pp. 1–133. [Google Scholar]
- Loke, M.H. RES2DMOD ver. 3.01. Rapid 2D Resistivity Forward Modelling Using the Finite-Difference and Finite-Elements Method; Geotomo Software: Aarhus, Denmark, 2002. [Google Scholar]
- Parasnis, D.S. Principles of Applied Geophysics; Chapman and Hall: London, UK, 1997. [Google Scholar]
- Bhattacharya, P.K.; Patra, H.P. Direct Current Geoelectric, Sounding Methods in Geochemistry and Geophysics; Elsevier: Amsterdam, The Netherlands, 1968. [Google Scholar]





| Electrode Pair | Half Electrode Layout L/2 (m) |
|---|---|
| C1–C2 | ±0.25 m |
| P1a–P1b | ±0.75 m |
| P2a–P2b | ±1.25 m |
| P3a–P3b | ±1.75 m |
| P4a–P4b | ±2.50 m |
| P5a–P5b | ±3.50 m |
| P6a–P6b | ±5.00 m |
| P7a–P7b | ±7.00 m |
| P8a–P8b | ±10.00 m |
| Measurement Configuration | Rel. Stand. Deviation (1σ) |
|---|---|
| Setup F | 0.8% (L/2 = 10 m) |
| Setup S | 1.4% (L/2 = 10 m) |
| Setup T, 1 m bins With wind, measurement cycle 0.25 s | 3.7% (L/2 = 2.5 m) |
| Setup T, 30 cm bins With wind, measurement cycle 0.25 s | 4.3% (L/2 = 2.5 m) |
| Setup T, 30 cm bins With wind, measurement cycle 0.25 s | 4.3% (L/2 = 2.5 m) |
| Setup T, 1 m bins Against wind, measurement cycle 0.25 s | 4.2% (L/2 = 2.5 m) |
| Setup T, 1 m bins Both directions, measurement cycle 0.25 s | 5.4% (L/2 = 2.5 m) |
| Setup T, 1 m bins With wind, measurement cycle 0.5 s | 3.1% (L/2 = 2.5 m) |
| Setup T, 1 m bins Both directions, measurement cycle 0.5 s | 3.5% (L/2 = 2.5 m) |
| Measurement Cycle | Rel. Stand. Deviation (1σ) |
|---|---|
| 1 s | 2.2% |
| 0.5 s | 1.3% |
| 0.25 s | 1.4% |
| 0.2 s | 1.1% |
| Measurement Setup | Noise and Smearing | Streamer Deviations | Water-Level Variations | Total Error |
|---|---|---|---|---|
| Setup S | 0.03 Ωm (1%) | 0.04 Ωm (1%) | 0.15 Ωm (5%) | 0.22 Ωm (7%) |
| Setup F | 0.07 Ωm (2%) | 0.04 Ωm (1%) | 0.3 Ωm (11%) | 0.41 Ωm (14%) |
| Setup T | 0.12 Ωm (4%) | 0.04 Ωm (1%) | 0.45 Ωm (16%) | 1.61 Ωm (21%) |
| 2L Case, Setup F | 2L Case, Setup S | 3L Case, Setup F | 3L Case, Setup S | |
|---|---|---|---|---|
| h1 | 0.9 ± 0.09 m 10.4% | 0.9 ± 0.1 m 10.6% | 0.9 ± 0.14 m 14.8% | 0.92 ± 0.14 m 14.76% |
| h2 | - | - | 1.8 ± 0.41 m 22.8% | 1.88 ± 0.41 m 21.51% |
| ρ1 | 0.3 ± 0.04 Ωm 14.6% | 0.3 ± 0.04 Ωm 14.7% | 0.3 ± 0.05 Ωm 15% | 0.3 ± 0.05 Ωm 15.1% |
| ρ2 | 78.3 ± 25 Ωm 31.9% | 83 ± 31.3 Ωm 37.7% | 85.1 ± 28.4 Ωm 33.3% | 76.9 ± 37.54 Ωm 48.8% |
| ρ3 | - | - | 12.3 ± 3 Ωm 24.5% | 11.9 ± 3.9 Ωm 32.8% |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fediuk, A.; Wilken, D.; Thorwart, M.; Wunderlich, T.; Erkul, E.; Rabbel, W. The Applicability of an Inverse Schlumberger Array for Near-Surface Targets in Shallow Water Environments. Remote Sens. 2020, 12, 2132. https://doi.org/10.3390/rs12132132
Fediuk A, Wilken D, Thorwart M, Wunderlich T, Erkul E, Rabbel W. The Applicability of an Inverse Schlumberger Array for Near-Surface Targets in Shallow Water Environments. Remote Sensing. 2020; 12(13):2132. https://doi.org/10.3390/rs12132132
Chicago/Turabian StyleFediuk, Annika, Dennis Wilken, Martin Thorwart, Tina Wunderlich, Ercan Erkul, and Wolfgang Rabbel. 2020. "The Applicability of an Inverse Schlumberger Array for Near-Surface Targets in Shallow Water Environments" Remote Sensing 12, no. 13: 2132. https://doi.org/10.3390/rs12132132
APA StyleFediuk, A., Wilken, D., Thorwart, M., Wunderlich, T., Erkul, E., & Rabbel, W. (2020). The Applicability of an Inverse Schlumberger Array for Near-Surface Targets in Shallow Water Environments. Remote Sensing, 12(13), 2132. https://doi.org/10.3390/rs12132132