Polarimetric Calibration of the GaoFen-3 Mission Using Active Radar Calibrators and the Applicable Conditions of System Model for Radar Polarimeters
Abstract
1. Introduction
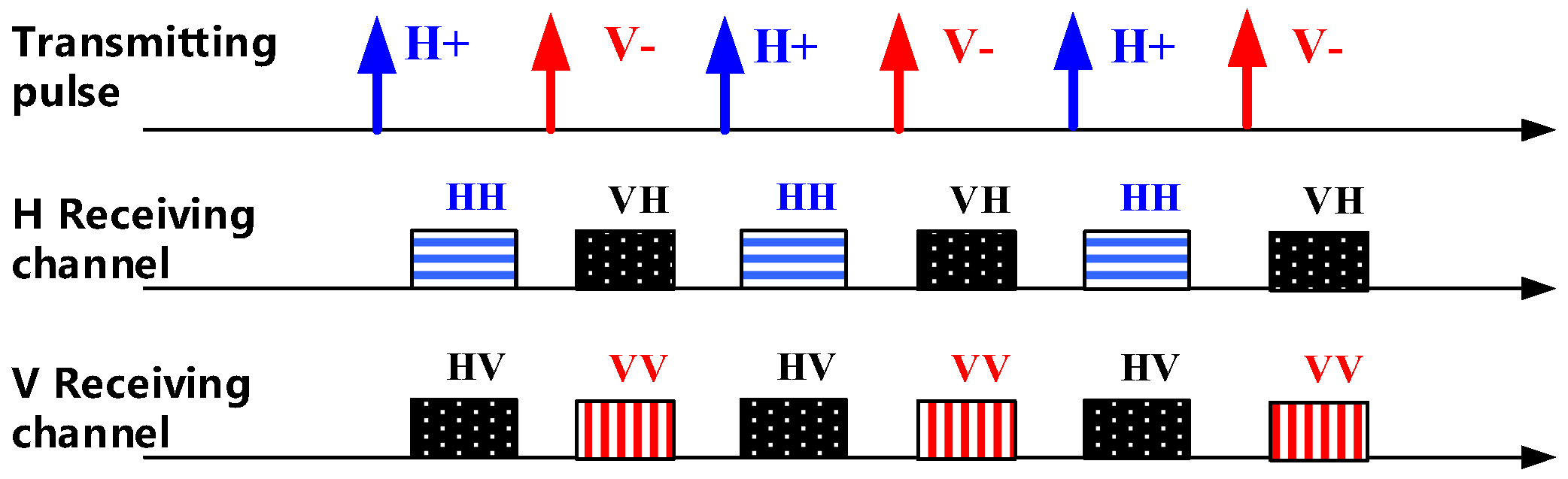
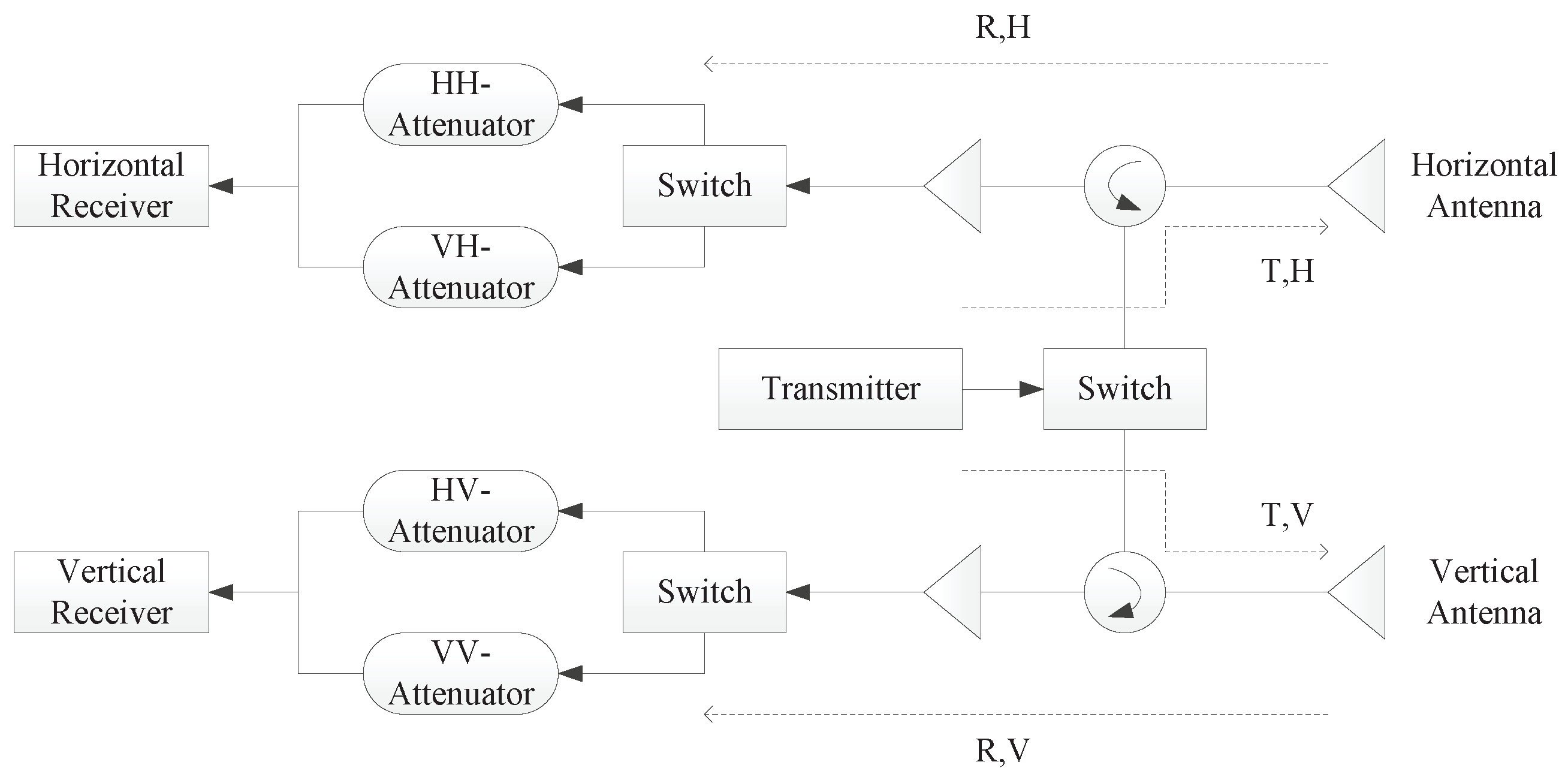
2. Calibration Method for GaoFen-3
2.1. The Classic System Model for Radar Polarimeters
2.2. Improved System Model of GaoFen-3
2.3. New Imbalance Factor Calibration Theory
2.4. Calibration Method Based on the Improved System Model
3. The GF-3 Calibration Experiment at Erdos Grassland
4. Data Processing, Results, and Discussion
4.1. The Data Processing Results of the Two Algorithms
4.2. GF-3 Radar Polarization Performance: Further Analysis
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Adaptation Conditions of Classic Model
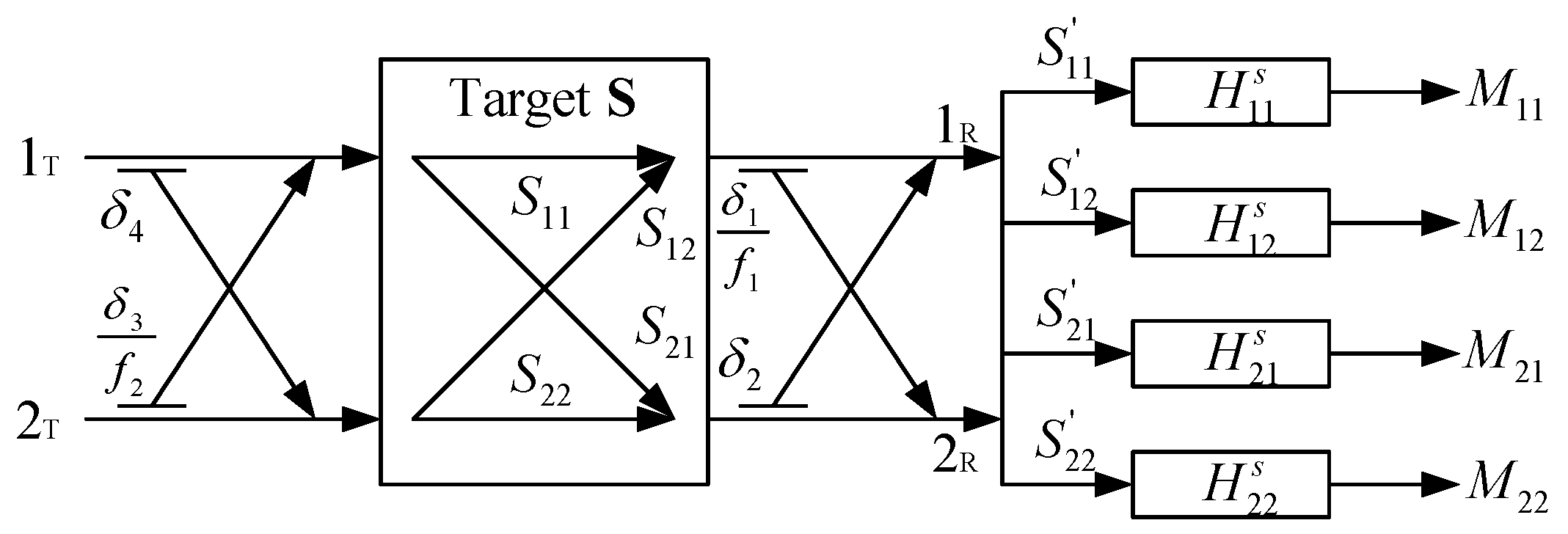
Appendix B. Another Derivation Process of the General Polarimetric System Model
Appendix C. Proof: (A1) Holds, Then (A8) Holds
Appendix D. Proof: (A8) Holds, Then (A1) Holds
References
- Sheen, D.R.; Freeman, A.J.; Kasischke, E.S. Phase calibration of polarimetric radar images. IEEE Trans. Geosci. Remote Sens. 1989, 27, 719–731. [Google Scholar] [CrossRef]
- Freeman, Y.S.A.; Werner, C. Polarimetric radar calibration experiment using active radar calibrators. IEEE Trans. Geosci. Remote Sens. 1990, 28, 224–240. [Google Scholar] [CrossRef]
- Freeman, A. A new system model for radar polarimeters. IEEE Trans. Geosci. Remote Sens. 1991, 29, 761–767. [Google Scholar] [CrossRef]
- Quegan, S. A unified algorithm for phase and cross-talk calibration of polarimetric data-theory and observations. IEEE Trans. Geosci. Remote Sens. 1994, 32, 89–99. [Google Scholar] [CrossRef]
- Zebker, H.A.; Zyl, J.J.; Durden, S.L.; Norikane, L. Calibrated imaging radar polarimetry: Technique, examples, and applications. IEEE Trans. Geosci. Remote Sens. 1991, 29, 942–961. [Google Scholar] [CrossRef]
- Zebker, H.A.; Zyl, J.J. Imaging radar polarimetry: A review. Proc. IEEE 1991, 79, 1583–1606. [Google Scholar] [CrossRef]
- Satake, M.; Kobayshi, T.; Manabe, T.; Masuko, H. Polarimetric calibration of x-band airborne synthetic aperture radar using corner reflectors and an active radar calibrator. In Proceedings of the 1998 IEEE International Geoscience and Remote Sensing, Symposium Proceedings, Seattle, WA, USA, 6–10 July 1998; Volume 2, pp. 660–662. [Google Scholar]
- Christensen, E.L.; Skou, N.; Dall, J.; Woelders, K.W.; Jorgensen, J.H.; Granholm, J.; Madsen, S.N. Emisar: An absolutely calibrated polarimetric l-and c-band sar. IEEE Trans. Geosci. Remote Sens. 1998, 36, 1852–1865. [Google Scholar] [CrossRef]
- Ainsworth, T.L.; Ferro-Famil, L.; Lee, J.S. Orientation angle preserving a posteriori polarimetric sar calibration. IEEE Trans. Geosci. Remote Sens. 2006, 44, 994–1003. [Google Scholar] [CrossRef]
- Shimada, M.; Kawano, N.; Watanabe, M.; Motooka, T.; Ohki, M. Calibration and validation of the pi-sar-l2. In Proceedings of the 2013 Asia-Pacific Conference on Synthetic Aperture Radar (APSAR), Tsukuba, Japan, 23–27 September 2014; Volume 478, pp. 194–197. [Google Scholar]
- Satake, M.; Kobayashi, T.; Uemoto, J.; Umehara, T.; Kojima, S. Polarimetric calibration of pi-sar2. In Proceedings of the 2013 Asia-Pacific Conference on Synthetic Aperture Radar (APSAR), Tsukuba, Japan, 23–27 September 2013; pp. 79–80. [Google Scholar]
- Ming, F.; Hong, J.; Zhang, L. Improved calibration method of the airborne polarimetric sar. In Proceedings of the 2011 3rd International Asia-Pacific Conference on Synthetic Aperture Radar (APSAR), Seoul, Korea, 26–30 September 2011; pp. 1–3. [Google Scholar]
- Williams, M.L.; Stacy, N.; Badger, D.; Preiss, M.; Preiss, A. Calibration and Performance Validation of the Ingara, High Resolution, Fully Polarimetric, x-Band Sar. In Proceedings of the CEOS Workshop on Polarimetric SAR, Ulm, Germany, 27–28 May 2004. [Google Scholar]
- Fujita, Y.F.M.; Masuda, T.; Satake, M. Polarimetric calibration of the sir-c c-band channel using active radar calibrators and polarization selective dihedrals. IEEE Trans. Geosci. Remote Sens. 1998, 36, 1872–1878. [Google Scholar] [CrossRef]
- Freeman, A. Sir-c calibration results. Math. Comput. Model. 2001, 33, 695–706. [Google Scholar]
- Freeman, A.; Alves, M.; Chapman, B.; Cruz, J. Sir-c data quality and calibration results. IEEE Trans. Geosci. Remote Sens. 1995, 33, 848–857. [Google Scholar] [CrossRef]
- Masaharu Fujita, Y.F.; Satake, M. Sir-c polarimetric calibration by using polarization selective dihedrals and a polarimetric active radar calibrator. Geosci. Remote Sens. 1997, 4, 1941–1943. [Google Scholar]
- Kimura, H. Calibration of polarimetric PALSAR imagery affected by faraday rotation using polarization orientation. IEEE Trans. Geosci. Remote Sens. 2009, 47, 3943–3950. [Google Scholar] [CrossRef]
- Sandberg, G.; Eriksson, L.E.B.; Ulander, L.M.H. Measurements of faraday rotation using polarimetric palsar images. IEEE Geosci. Remote Sens. Lett. 2009, 6, 142–146. [Google Scholar] [CrossRef]
- Touzi, R.; Shimada, M. Polarimetric PALSAR Calibration. IEEE Trans. Geosci. Remote Sens. 2009, 47, 3951–3959. [Google Scholar] [CrossRef]
- Shimada, M. Model-based polarimetric sar calibration method using forest and surface-scattering targets. IEEE Trans. Geosci. Remote Sens. 2011, 49, 1712–1733. [Google Scholar] [CrossRef]
- Meyer, F.J.; Nicoll, J.B. Prediction, detection, and correction of faraday rotation in full-polarimetric l-band sar data. IEEE Trans. Geosci. Remote Sens. 2008, 46, 3076–3086. [Google Scholar] [CrossRef]
- Touzi, R.; Hawkins, R.K.; Cote, S. High-Precision Assessment and Calibration of Polarimetric RADARSAT-2 SAR Using Transponder Measurements. IEEE Trans. Geosci. Remote Sens. 2013, 51, 487–503. [Google Scholar] [CrossRef]
- Iannini, G.L.; Tebaldini, S. Long term relative polarimetric calibration by natural targets. In Proceedings of the 2013 IEEE International Geoscience and Remote Sensing Symposium, Melbourne, VIC, Australia, 21–26 July 2014. [Google Scholar]
- Caves, R. Radarsat-2 polarimetric calibration performance over five years of operation. In Proceedings of the 10th European Conference on Synthetic Aperture Radar, Berlin, Germany, 3–5 June 2014; pp. 1–4. [Google Scholar]
- Moriyama, T. Polarimetric calibration of palsar2. In Proceedings of the 2015 IEEE International Geoscience and Remote Sensing Symposium, Milan, Italy, 26–31 July 2015; Volume 9, pp. 1284–1287. [Google Scholar]
- Touzi, R.; Shimada, M. Calibration and validation of polarimetric alos2. In Proceedings of the 2015 IEEE International Geoscience and Remote Sensing Symposium, Milan, Italy, 26–31 July 2015; pp. 4113–4116. [Google Scholar]
- Zhang, Q. System design and key technologies of the gf-3 satellite. Acta Geod. Cartogr. Sin. 2017. [Google Scholar] [CrossRef]
- Liu, J.; Qiu, X.; Hong, W. Automated ortho-rectified sar image of gf-3 satellite using reverse-range-doppler method. J. Geophys. Res. Solid Earth 2016, 12, 4445–4448. [Google Scholar]
- Jiang, S.; Qiu, X.; Han, B.; Hu, W. A quality assessment method based on common distributed targets for gf-3 polarimetric sar data. Sensors 2018, 18, 807. [Google Scholar] [CrossRef]
- Sarabandi, K.; Pierce, L.E.; Ulaby, F.T. Calibration of a polarimetric imaging SAR. IEEE Trans. Geosci. Remote Sens. 1992, 30, 540–549. [Google Scholar] [CrossRef]
- Sarabandi, K. Calibration of a polarimetric synthetic aperture radar using a known distributed target. IEEE Trans. Geosci. Remote Sens. 1994, 32, 575–582. [Google Scholar] [CrossRef]
- Sarabandi, K.; Pierce, L.E. Cross-calibration experiment of JPL AIRSAR and truck-mounted polarimetric scatterometer. IEEE Trans. Geosci. Remote Sens. 1994, 32, 975–985. [Google Scholar] [CrossRef]
- Klein, J.D. Calibration of complex polarimetric sar imagery using backscatter correlations. IEEE Trans. Aerosp. Electron. Syst. 1992, 28, 183–194. [Google Scholar] [CrossRef]
- Van Zyl, J.J. Calibration of polarimetric radar images using only image parameters and trihedral corner reflector responses. IEEE Trans. Geosci. Remote Sens. 1990, 28, 337–348. [Google Scholar] [CrossRef]
- Freeman, A.; Zyl, J.J.V.; Klein, J.D. Calibration of Stokes and scattering matrix format polarimetric SAR data. IEEE Trans. Geosci. Remote Sens. 1992, 30, 531–539. [Google Scholar] [CrossRef]
- Zebker, H.A.; Zyl, J.J.; Held, D.N. Imaging radar polarimetry from wave synthesis. J. Geophys. Res. Solid Earth 1987, 92, 683–701. [Google Scholar] [CrossRef]
- Freeman, A. Calibration of linearly polarized polarimetric sar data subject to faraday rotation. IEEE Trans. Geosci. Remote Sens. 2004, 42, 1617–1624. [Google Scholar] [CrossRef]
- Li, L.; Zhu, Y.; Hong, J. Design and Implementation of a Novel Polarimetric Active Radar Calibrator for Gaofen-3 SAR. Sensors 2018, 18, 2620. [Google Scholar] [CrossRef]
- Whitt, M.W.; Ulaby, F.T.; Polatin, P. A general polarimetric radar calibration technique. IEEE Trans. Anttenas Propag. 1991, 39, 62–67. [Google Scholar] [CrossRef]
- Sun, J.; Yu, W.; Deng, Y. The SAR Payload Design and Performance for the GF-3 Mission. Sensors 2017, 17, 2419. [Google Scholar] [CrossRef] [PubMed]
- Freeman, A. On the design of spaceborne polarimetric SARs. In Proceedings of the IEEE Radar Conference, Pasadena, CA, USA, 4–8 May 2009; pp. 1–4. [Google Scholar]
- Jiang, S.; Qiu, X.; Han, B.; Sun, J.; Ding, C. Error Source Analysis and Correction of GF-3 Polarimetric Data. Remote Sens. 2018, 10, 1685. [Google Scholar] [CrossRef]



| Parameters | 8 September 2016 | 19 September 2016 | 11 July 2017 | 16 July 2017 |
|---|---|---|---|---|
| CenterFrequency (GHz) | 5.400012 | 5.400012 | 5.400012 | 5.400012 |
| lookDirection | Right | Right | Right | Right |
| centerLookAngle() | 29.360000 | 41.180000 | 35.500000 | 26.900000 |
| prf(Hz) | 2792.176270 | 2632.106689 | 2461.089844 | 2524.653564 |
| sampleRate (MHz) | 66.666667 | 33.333333 | 66.666667 | 66.666667 |
| bandWidth (MHz) | 60.000000 | 30.000000 | 40.000000 | 60.000000 |
| pulseWidth (μs) | 24.990000 | 24.990000 | 24.990000 | 24.990000 |
| HH-MGCValue (dB) | 56 | 50 | 51 | 52 |
| VH-MGCValue (dB) | 47 | 41 | 42 | 42 |
| VV-MGCValue (dB) | 56 | 50 | 51 | 52 |
| HV-MGCValue (dB) | 47 | 41 | 42 | 42 |
| Date | 8 September 2016 | 19 September 2016 | 11 July 2017 | 16 July 2017 |
|---|---|---|---|---|
| 1.2842∠−6.0298 | 1.2308∠−10.4243 | 1.1970∠−8.6439 | 1.2164∠−8.4432 | |
| 0.8896∠0.5097 | 0.8974∠2.4225 | 0.9050∠−4.3705 | 0.8706∠−3.0841 | |
| 0.0056∠108.9447 | 0.0066∠116.5435 | 0.0087∠111.3989 | 0.0091∠120.1476 | |
| 0.0031∠−38.6639 | 0.0039∠5.0855 | 0.0057∠54.2000 | 0.0070∠28.2446 | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 0.0149∠−45.2715 | 0.0152∠−92.6368 | 0.0126∠−69.1254 | 0.0131∠−54.6146 | |
| 0.004∠168.4078 | 0.0026∠−49.6355 | 0.0042∠−177.2737 | 0.0032∠−178.2101 | |
| 0.9133∠19.3436 | 0.8752∠8.6810 | 0.9431∠10.4461 | 0.9382∠11.0117 |
| Date | 8 September 2016 | 19 September 2016 | 11 July 2017 | 16 July 2017 |
|---|---|---|---|---|
| 0.0008∠30.4265 | 0.0003∠−74.1516 | 0.0002∠18.4696 | 0.0004∠−54.8351 | |
| 0.0004∠109.2795 | 0.0002∠56.1568 | 0.0002∠−98.1809 | 0.0004∠−38.367 | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 0.0006∠46.2279 | 0.0001∠−179.165 | 0.0003∠94.45 | 0.0003∠155.9556 | |
| 0.001∠−11.8787 | 0.0006∠134.6603 | 0.0002∠120.7389 | 0.0003∠−0.7566 | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 0.0003∠35.8663 | 0.001∠−47.5768 | 0.0002∠156.6569 | 0.0001∠−56.3412 | |
| 0.0009∠142.3814 | 0.0006∠176.4359 | 0.0002∠139.859 | 0.0003∠−155.083 | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 1.0002∠−0.0119 | 1.0001∠0.0019 | 1.0001∠−0.0035 | 1.0003∠0.0063 | |
| 1.0001∠−179.9994 | 1∠−179.999 | 1.0∠−180.0 | 1.0001∠−179.9972 | |
| 1∠−179.9979 | 1∠179.996 | 1.0∠179.9961 | 1.0∠−179.9941 | |
| 1.0∠0.0 | − | − | − | |
| 0.0161∠106.446 | − | − | − | |
| 0.0082∠72.2699 | − | − | − | |
| 1.0367∠−4.1433 | − | − | − | |
| 1.0∠0.0 | − | − | − | |
| 0.0064∠127.5089 | − | − | − | |
| 0.0081∠−6.618 | − | − | − | |
| 1.0083∠10.9789 | − | − | − | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 0.019∠−160.1919 | 0.0032∠29.8867 | 0.0071∠117.4385 | 0.0069∠−87.1168 | |
| 0.0166∠−110.4146 | 0.0112∠−50.3038 | 0.0105∠−31.5742 | 0.0193∠−52.6077 | |
| 0.976∠0.6473 | 0.9791∠−0.8091 | 0.9723∠−4.6613 | 0.9267∠−4.6189 | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 0.0161∠94.6297 | 0.0142∠92.2183 | 0.0162∠−132.5888 | 0.0143∠−137.0717 | |
| 0.0091∠51.6909 | 0.0098∠19.7512 | 0.0432∠−43.8032 | 0.0218∠−82.9303 | |
| 0.9735∠−0.8264 | 0.9794∠−0.732 | 0.9923∠−3.2429 | 0.9233∠−1.51 | |
| 1.0∠0.0 | − | 1.0∠0.0 | 1.0∠0.0 | |
| 0.0255∠113.1313 | − | 0.0124∠126.2182 | 0.0119∠−129.9445 | |
| 0.016∠73.399 | − | 0.0043∠−5.5687 | 0.0223∠−76.7825 | |
| 0.9639∠0.4479 | − | 0.9679∠−6.1389 | 0.9373∠−5.2056 | |
| 0.1203∠−148.8865 | 0.0401∠107.2664 | − | − | |
| 1.0∠0.0 | 1.0∠0.0 | − | − | |
| 0.9788∠1.8672 | 1.0042∠−3.051 | − | − | |
| 0.0507∠−147.8115 | 0.0431∠177.0058 | − | − | |
| 0.0459∠7.1077 | − | − | − | |
| 1.0∠0.0 | − | − | − | |
| 0.9707∠1.8069 | − | − | − | |
| 0.0255∠4.8021 | − | − | − | |
| 0.0621∠−141.559 | − | − | − | |
| 1.0∠0.0 | − | − | − | |
| 0.9745∠1.9538 | − | − | − | |
| 0.007∠151.8828 | − | − | − | |
| − | 1.0∠0.0 | − | − | |
| − | 0.031∠126.3532 | − | − | |
| − | 0.0202∠−175.2802 | − | − | |
| − | 0.9289∠−179.2397 | − | − |
| Date | 8 September 2016 | 19 September 2016 | 11 July 2017 | 16 July 2017 |
|---|---|---|---|---|
| 1.1420∠−5.4918 | 1.1037∠−7.9557 | 1.0818∠−13.0290 | 1.0570∠−11.5047 | |
| 0.0072∠102.9431 | 0.0081∠106.1653 | 0.0105∠102.7403 | 0.0111∠112.1854 | |
| 0.004∠−44.6655 | 0.0048∠−5.2927 | 0.0068∠45.5414 | 0.0085∠19.8240 | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 0.0192∠−51.3402 | 0.0188∠−102.9260 | 0.0152∠−77.4302 | 0.0159∠−63.1131 | |
| 0.004∠168.4078 | 0.0026∠−49.6355 | 0.0042∠−177.2737 | 0.0032∠−176.4237 | |
| 0.9133∠19.6353 | 0.8793∠8.8452 | 0.9450∠10.6107 | 0.9392∠11.2238 |
| Date | 8 September 2016 | 19 September 2016 | 11 July 2017 | 16 July 2017 |
|---|---|---|---|---|
| 0.0008∠30.3894 | 0.0003∠−74.5779 | 0.0002∠18.5287 | 0.0004∠−30.5307 | |
| 0.0004∠113.2203 | 0.0002∠71.1966 | 0.0002∠−111.8448 | 0.0004∠−44.1218 | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 0.0008∠30.3894 | 0.0001∠−129.8421 | 0.0004∠85.2433 | 0.0003∠124.7732 | |
| 0.001∠−11.6002 | 0.0006∠134.8052 | 0.0002∠120.8926 | 0.0003∠−0.8321 | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 0.0003∠35.4797 | 0.001∠−47.2291 | 0.0002∠155.8671 | 0.0001∠−52.7921 | |
| 0.0011∠136.3854 | 0.0008∠166.045 | 0.0003∠131.171 | 0.0004∠−164.0078 | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 1.0005∠0.0945 | 1.001∠0.1181 | 1.0009∠0.1279 | 1.0008∠0.164 | |
| 1.001∠179.9557 | 1.0001∠−179.9534 | 1.0008∠179.9581 | 1.0008∠179.9699 | |
| 1.2835∠173.7351 | 1.2243∠169.4541 | 1.193∠171.1751 | 1.2129∠171.3833 | |
| 1.0∠0.0 | − | − | − | |
| 0.0193∠105.5052 | − | − | − | |
| 0.0082∠69.6111 | − | − | − | |
| 1.3307∠−10.4111 | − | − | − | |
| 1.0∠0.0 | − | − | − | |
| 0.0088∠112.1505 | − | − | − | |
| 0.0088∠−2.6916 | − | − | − | |
| 1.2942∠4.7115 | − | − | − | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 0.0184∠−170.1772 | 0.0068∠20.3131 | 0.007∠108.6478 | 0.0059∠−81.5293 | |
| 0.0163∠−108.7955 | 0.0095∠−47.7491 | 0.0103∠−39.0879 | 0.0186∠−55.8937 | |
| 1.2527∠−5.6204 | 1.1987∠−11.3527 | 1.1599∠−13.4834 | 1.124∠−13.2435 | |
| 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | 1.0∠0.0 | |
| 0.0192∠94.2743 | 0.0153∠78.0844 | 0.0157∠−132.4582 | 0.013∠−139.7729 | |
| 0.0096∠49.7707 | 0.0099∠28.3067 | 0.0439∠−44.6547 | 0.0217∠−84.8218 | |
| 1.2495∠−7.094 | 1.199∠−11.2756 | 1.1838∠−12.065 | 1.1199∠−10.1347 | |
| 1.0∠0.0 | − | 1.0∠0.0 | 1.0∠0.0 | |
| 0.0285∠110.6736 | − | 0.0128∠120.7902 | 0.0108∠−131.2024 | |
| 0.0163∠71.8355 | − | 0.0039∠−18.7665 | 0.0223∠−81.5054 | |
| 1.2373∠−5.8197 | − | 1.1547∠−14.961 | 1.1368∠−13.8302 | |
| 0.1203∠−148.5616 | 0.0403∠107.4153 | − | − | |
| 1.0∠0.0 | 1.0∠0.0 | − | − | |
| 0.9783∠2.2342 | 1.008∠−2.862 | − | − | |
| 0.0589∠1.1674 | 0.053∠166.6122 | − | − | |
| 0.0507∠−147.5066 | − | − | − | |
| 1.0∠0.0 | − | − | − | |
| 0.9703∠2.1571 | − | − | − | |
| 0.0327∠−1.1586 | − | − | − | |
| 0.0621∠−141.2556 | − | − | − | |
| 1.0∠0.0 | − | − | − | |
| 0.974∠2.3039 | − | − | − | |
| 0.009∠145.9104 | − | − | − | |
| − | 1.0∠0.0 | − | − | |
| − | 0.0324∠113.5859 | − | − | |
| − | 0.0211∠−175.5492 | − | − | |
| − | 1.1372∠170.2184 | − | − |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, W.; Jia, Z.; Qiu, X.; Hong, J.; Zhang, Q.; Lei, B.; Zhang, F.; Deng, Z.; Wang, A. Polarimetric Calibration of the GaoFen-3 Mission Using Active Radar Calibrators and the Applicable Conditions of System Model for Radar Polarimeters. Remote Sens. 2019, 11, 176. https://doi.org/10.3390/rs11020176
Liang W, Jia Z, Qiu X, Hong J, Zhang Q, Lei B, Zhang F, Deng Z, Wang A. Polarimetric Calibration of the GaoFen-3 Mission Using Active Radar Calibrators and the Applicable Conditions of System Model for Radar Polarimeters. Remote Sensing. 2019; 11(2):176. https://doi.org/10.3390/rs11020176
Chicago/Turabian StyleLiang, Weibin, Zengzeng Jia, Xiaolan Qiu, Jun Hong, Qingjun Zhang, Bin Lei, Fan Zhang, Zhaoguo Deng, and Aichun Wang. 2019. "Polarimetric Calibration of the GaoFen-3 Mission Using Active Radar Calibrators and the Applicable Conditions of System Model for Radar Polarimeters" Remote Sensing 11, no. 2: 176. https://doi.org/10.3390/rs11020176
APA StyleLiang, W., Jia, Z., Qiu, X., Hong, J., Zhang, Q., Lei, B., Zhang, F., Deng, Z., & Wang, A. (2019). Polarimetric Calibration of the GaoFen-3 Mission Using Active Radar Calibrators and the Applicable Conditions of System Model for Radar Polarimeters. Remote Sensing, 11(2), 176. https://doi.org/10.3390/rs11020176






