Galileo Single Point Positioning Assessment Including FOC Satellites in Eccentric Orbits
Abstract
:1. Introduction
2. Materials and Methods
2.1. Methodology
2.2. Experimental Setup
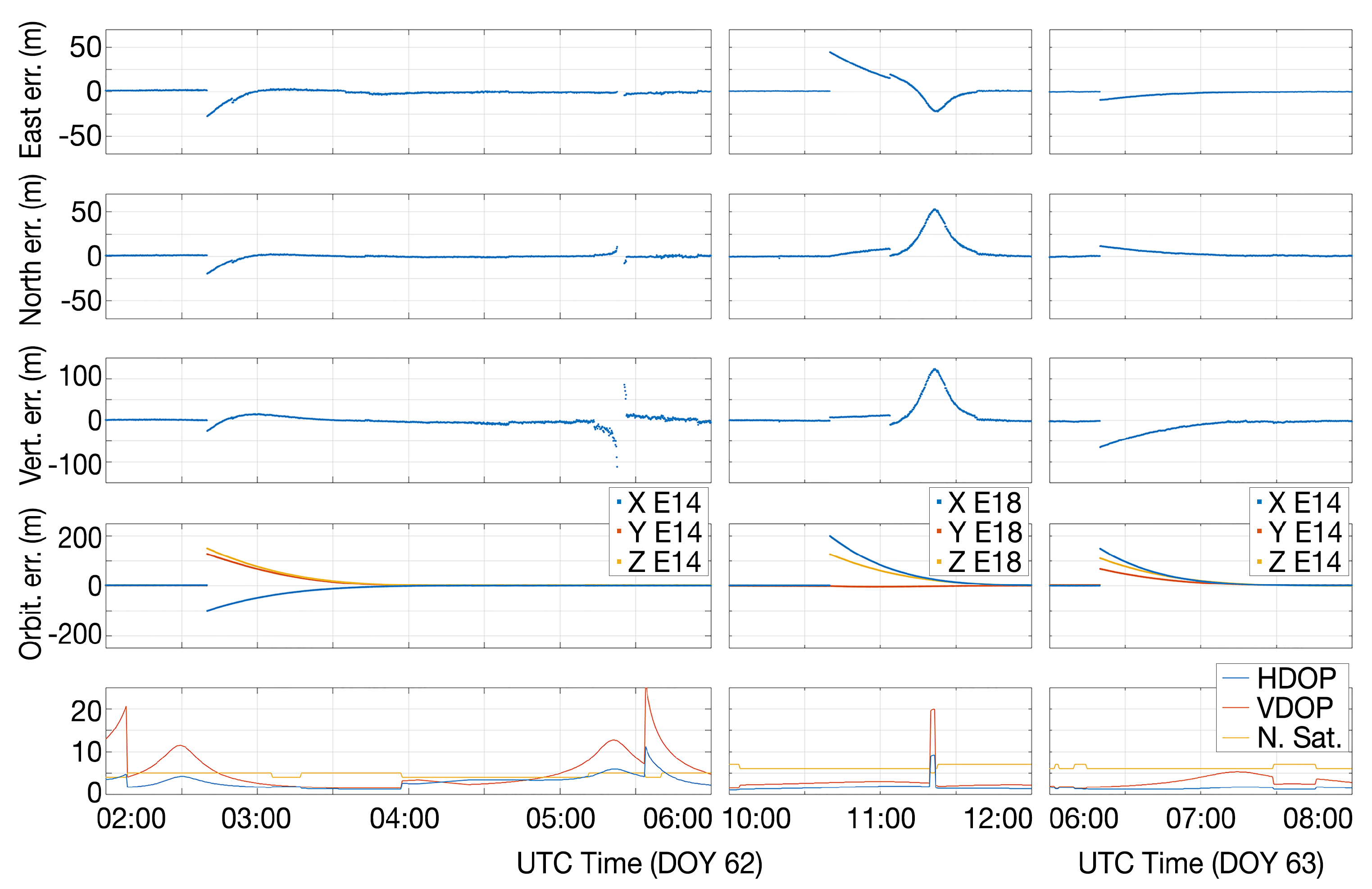
3. Results
4. Discussion
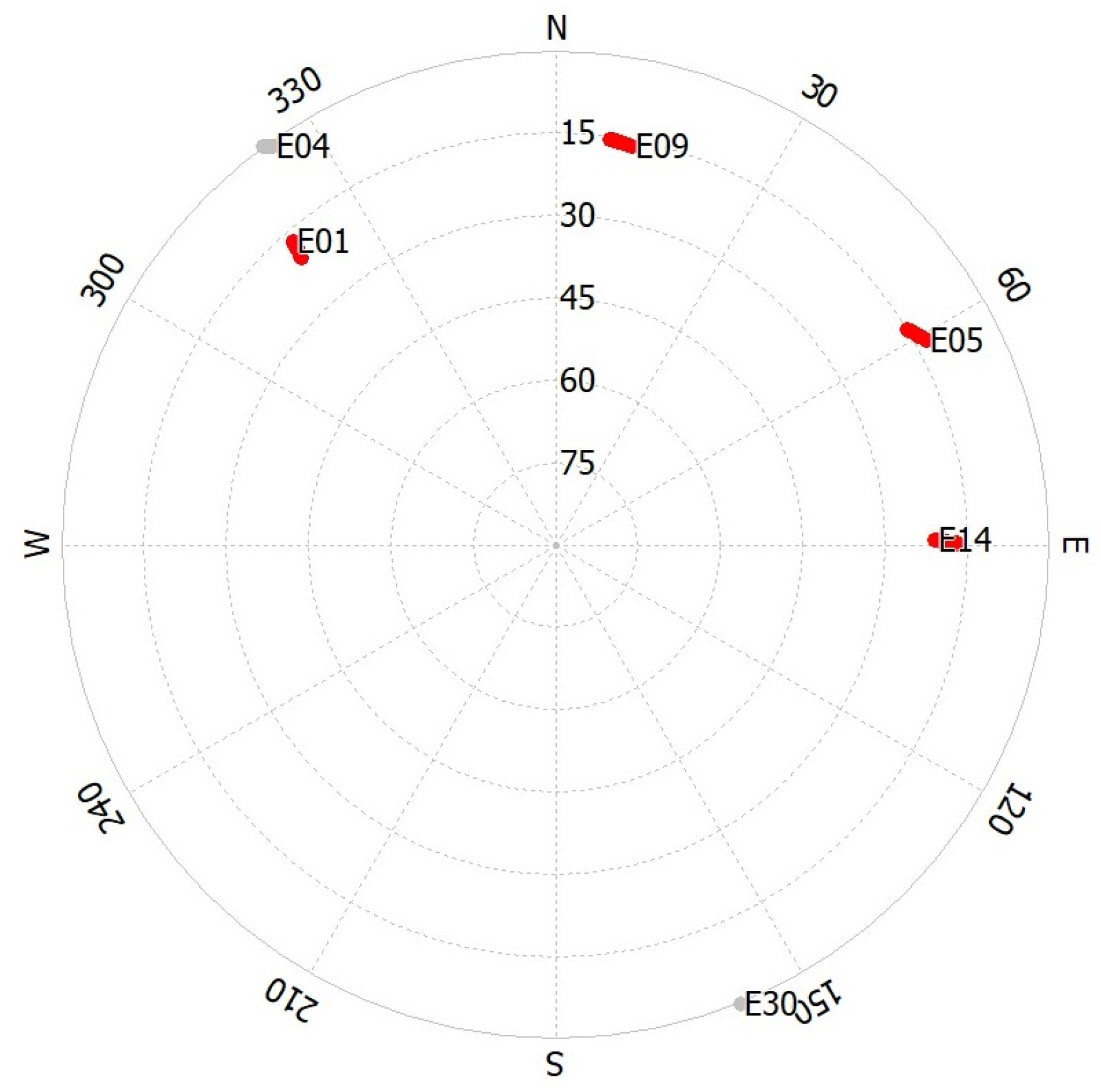
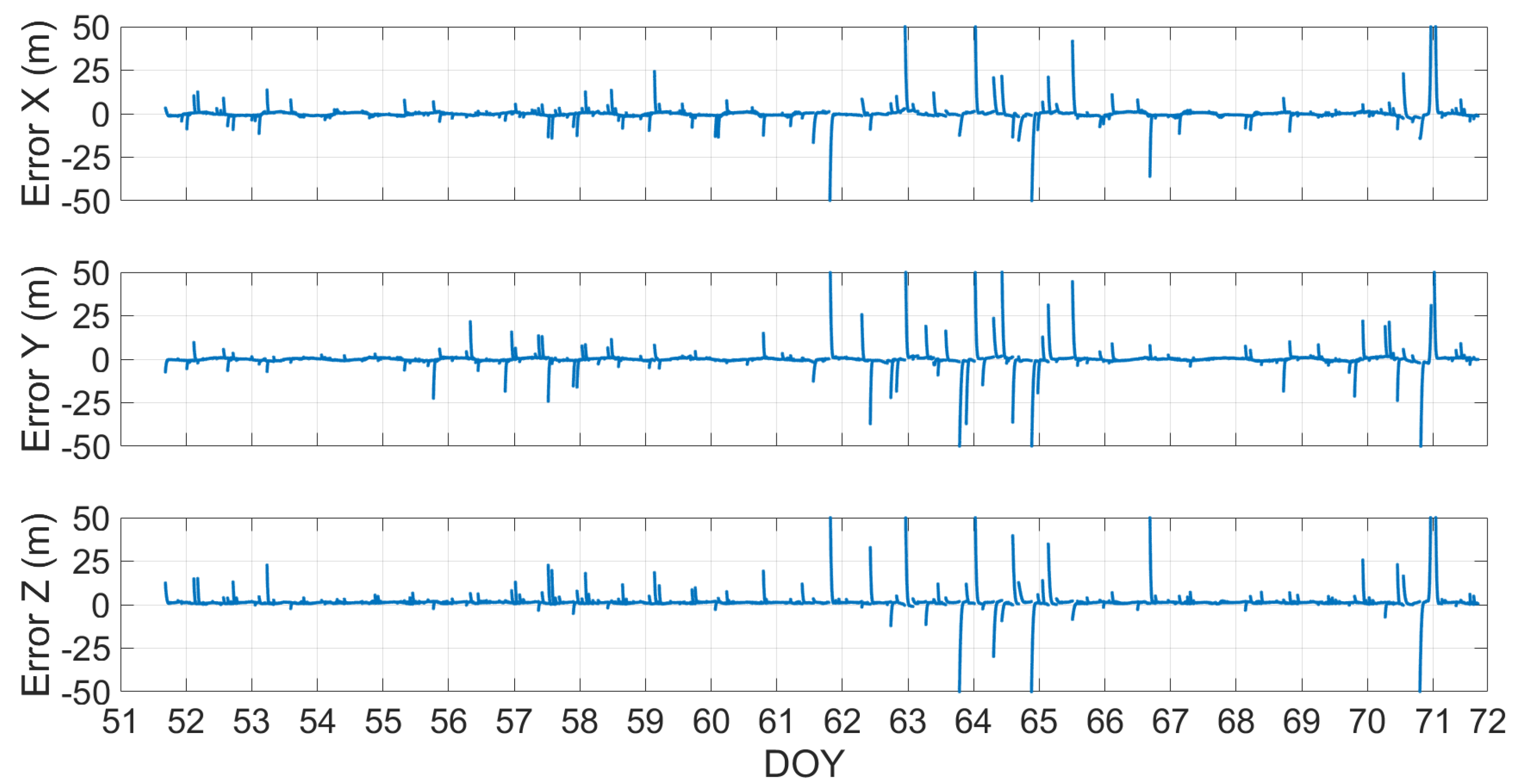
- The evolution of the C/N0 of the E14 and E18 satellites was similar to that of the other FOC satellites in the YEL2 site. As expected, the Milena (E14) and Doresa (E18) satellites showed a higher C/N0 with respect to the other FOC satellites, confirming results shown in previous studies. This behavior was due to the lower altitude of Milena (E14) and Doresa (E18) caused by their elliptical orbit. The comparison between the carrier-to-noise density ratio (C/N0) at the different frequencies of the Milena (E14) and Doresa (E18) satellites revealed that for both satellites, the E5 signal had a C/N0 higher than the others. Starting from 40 degrees, it can be seen that the E5b signal had a C/N0 higher than the E5a signal and this higher than E1. For elevation angles lower than 30 degrees, the E1 signal showed a carrier-to-noise density ratio higher than E5b.
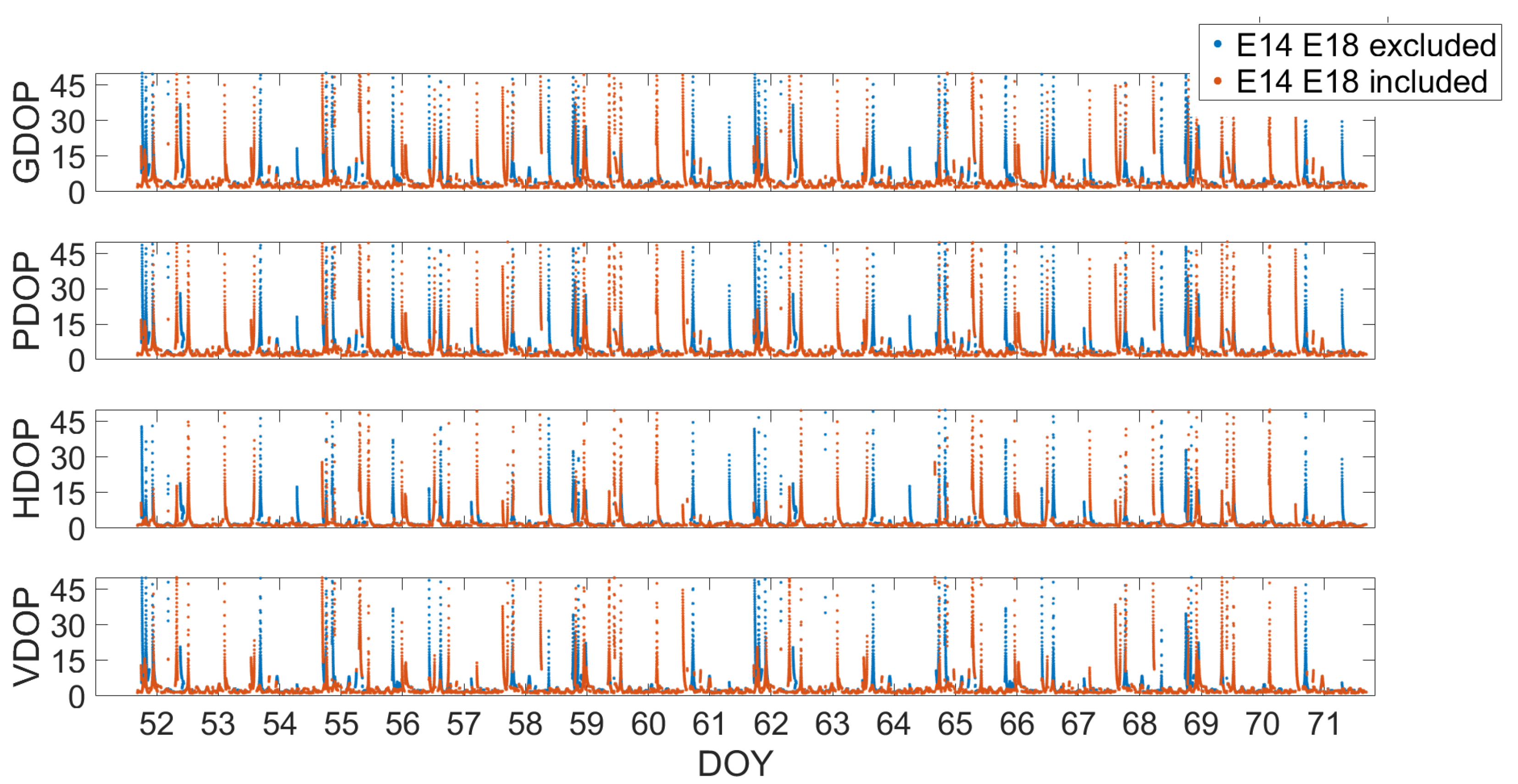
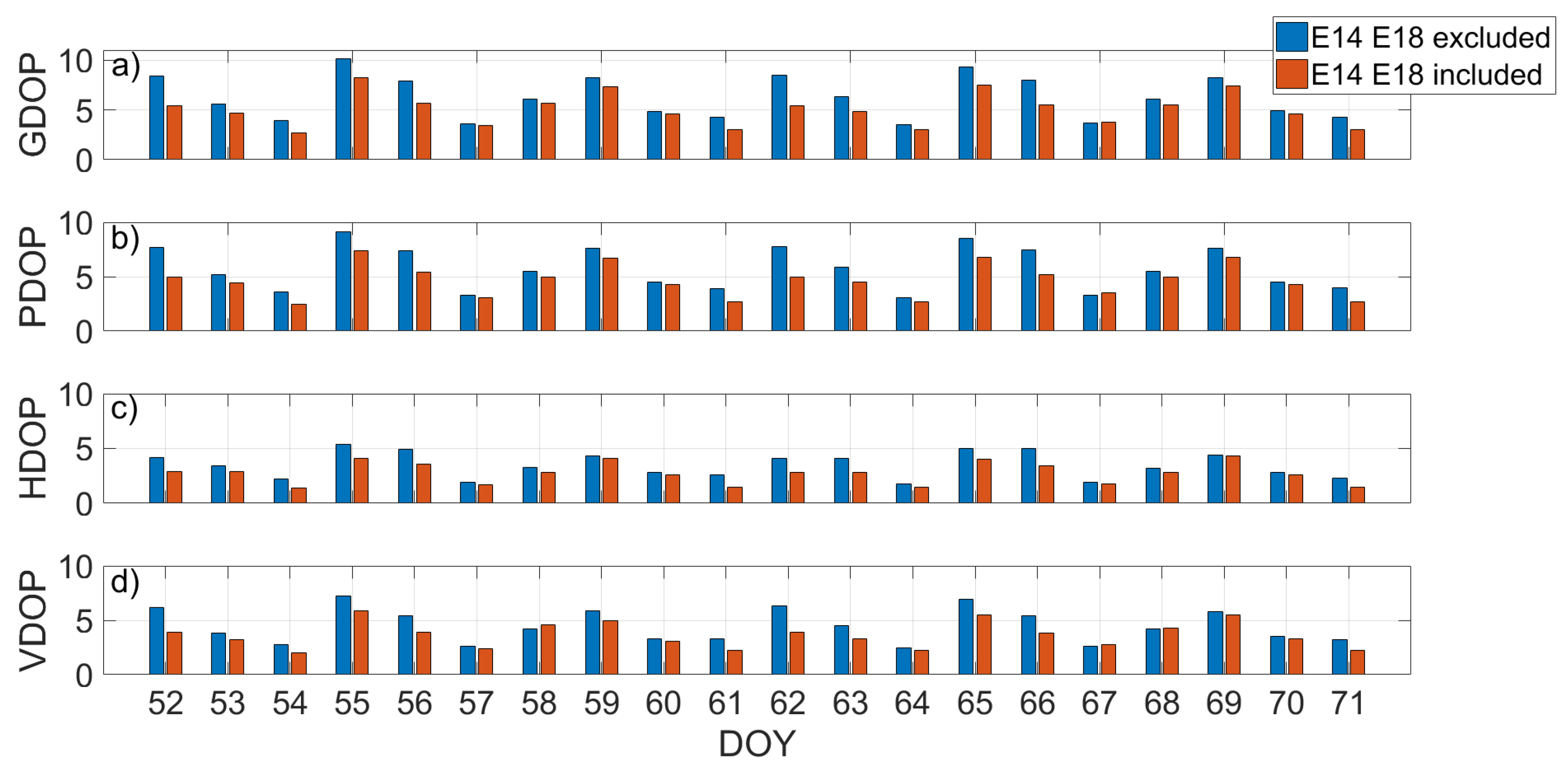
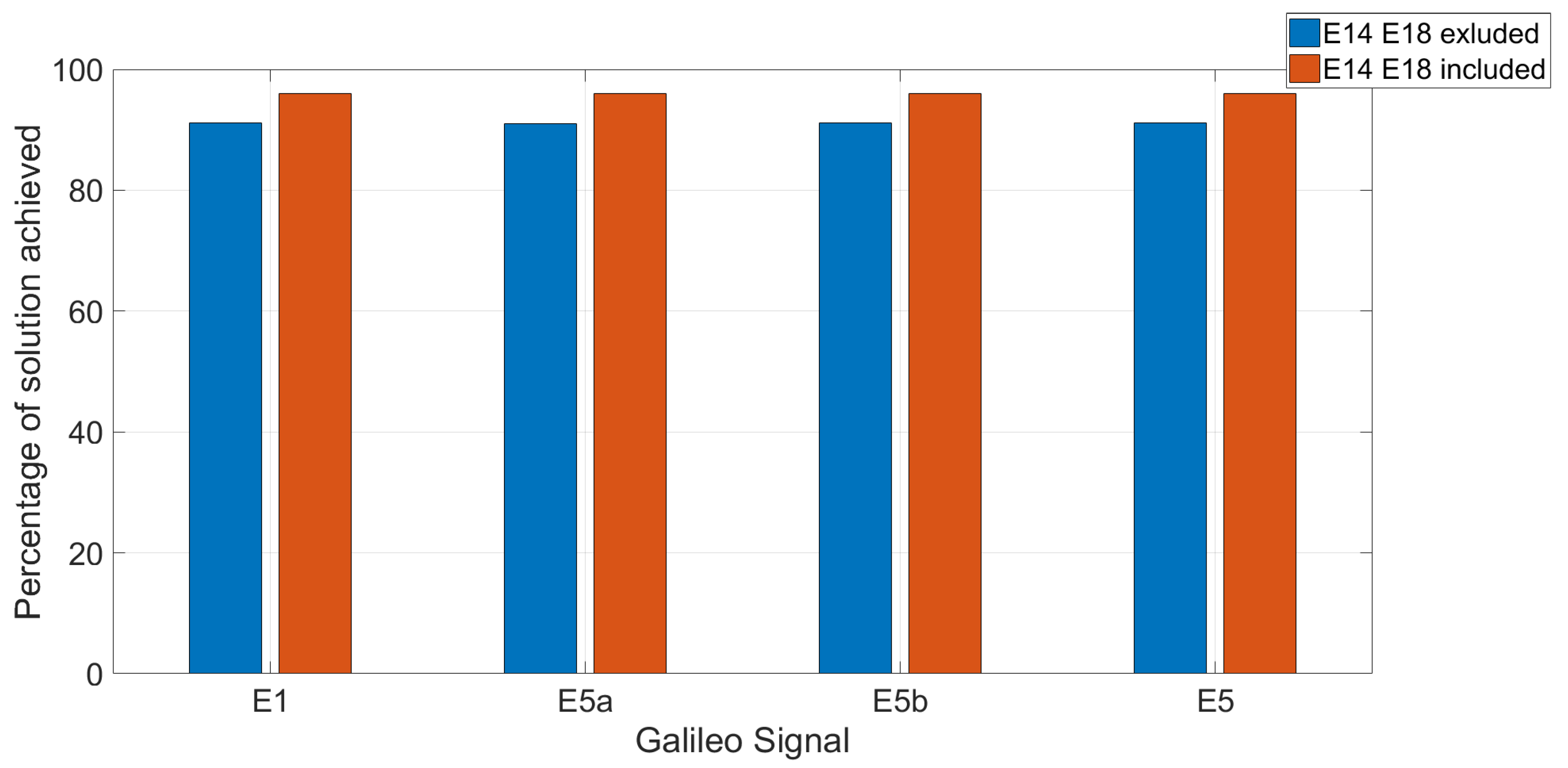
- As expected, the inclusion of the two satellites improved:
- -
- the system availability, varying it from 94.1–97.94%.
- -
- the GDOP, PDOP, HDOP, and VDOP parameters in each DOY analyzed;
- -
- the percentages of achieved positioning solutions by about 5% regardless of the frequency used.
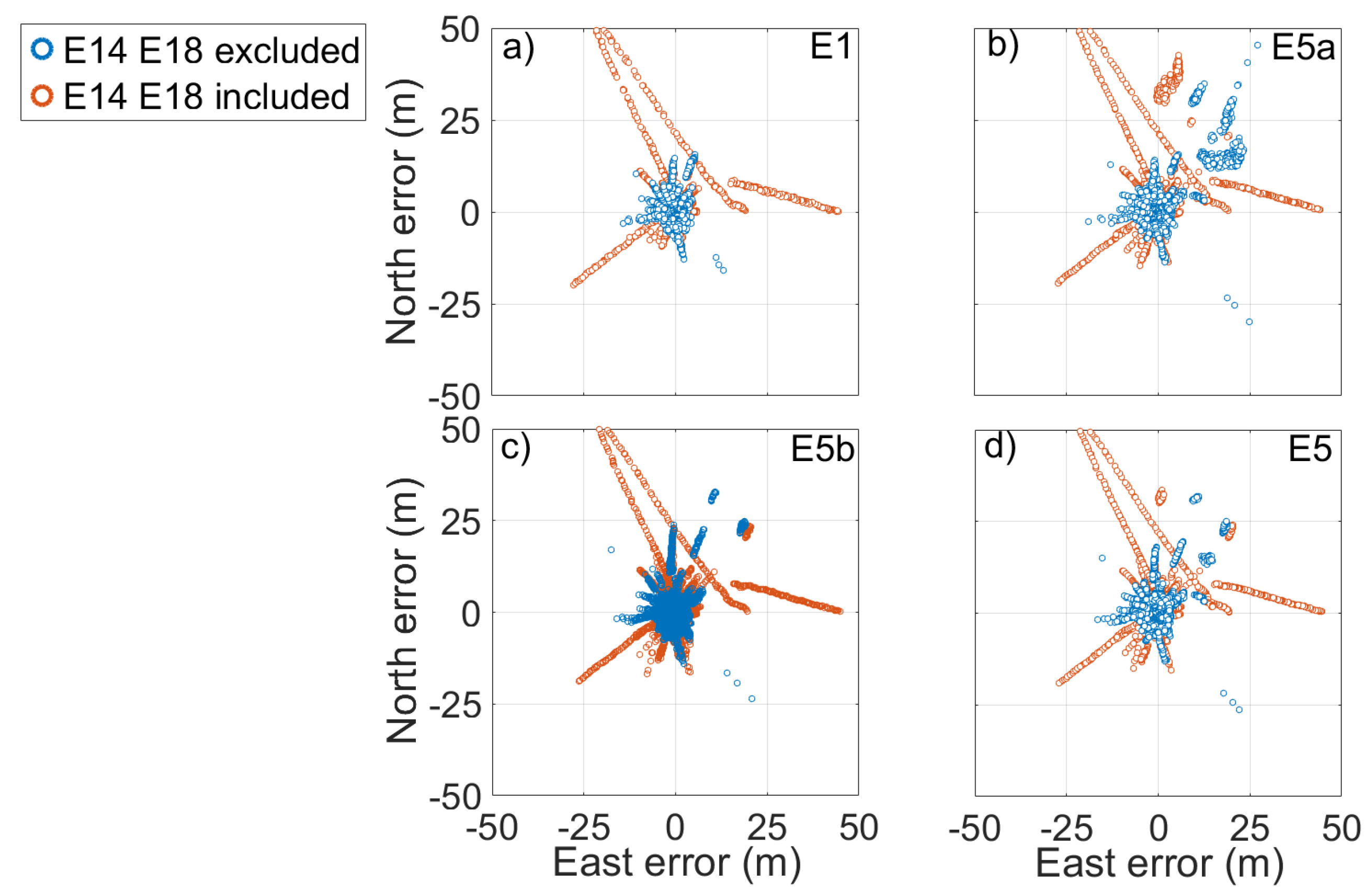
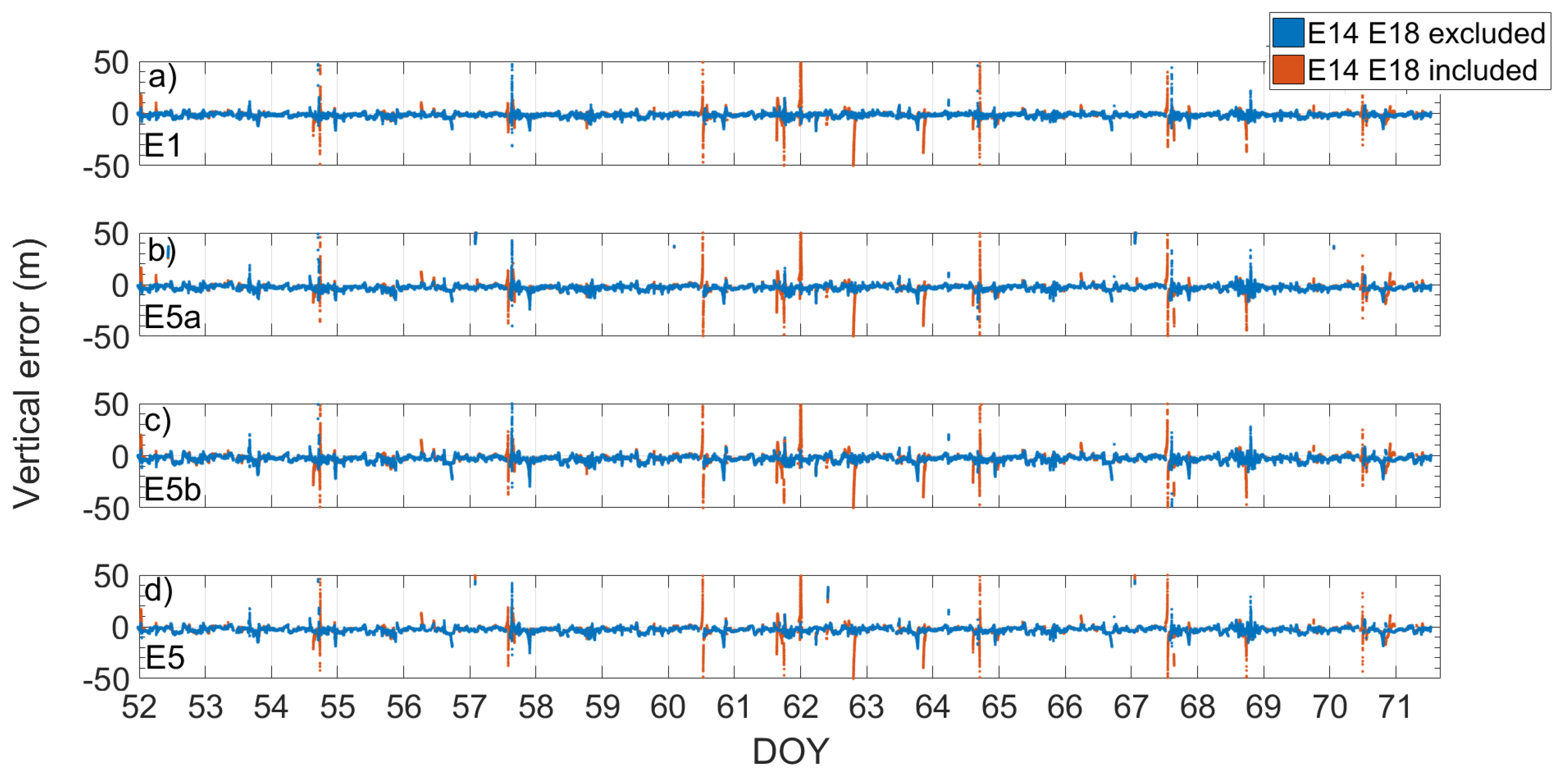
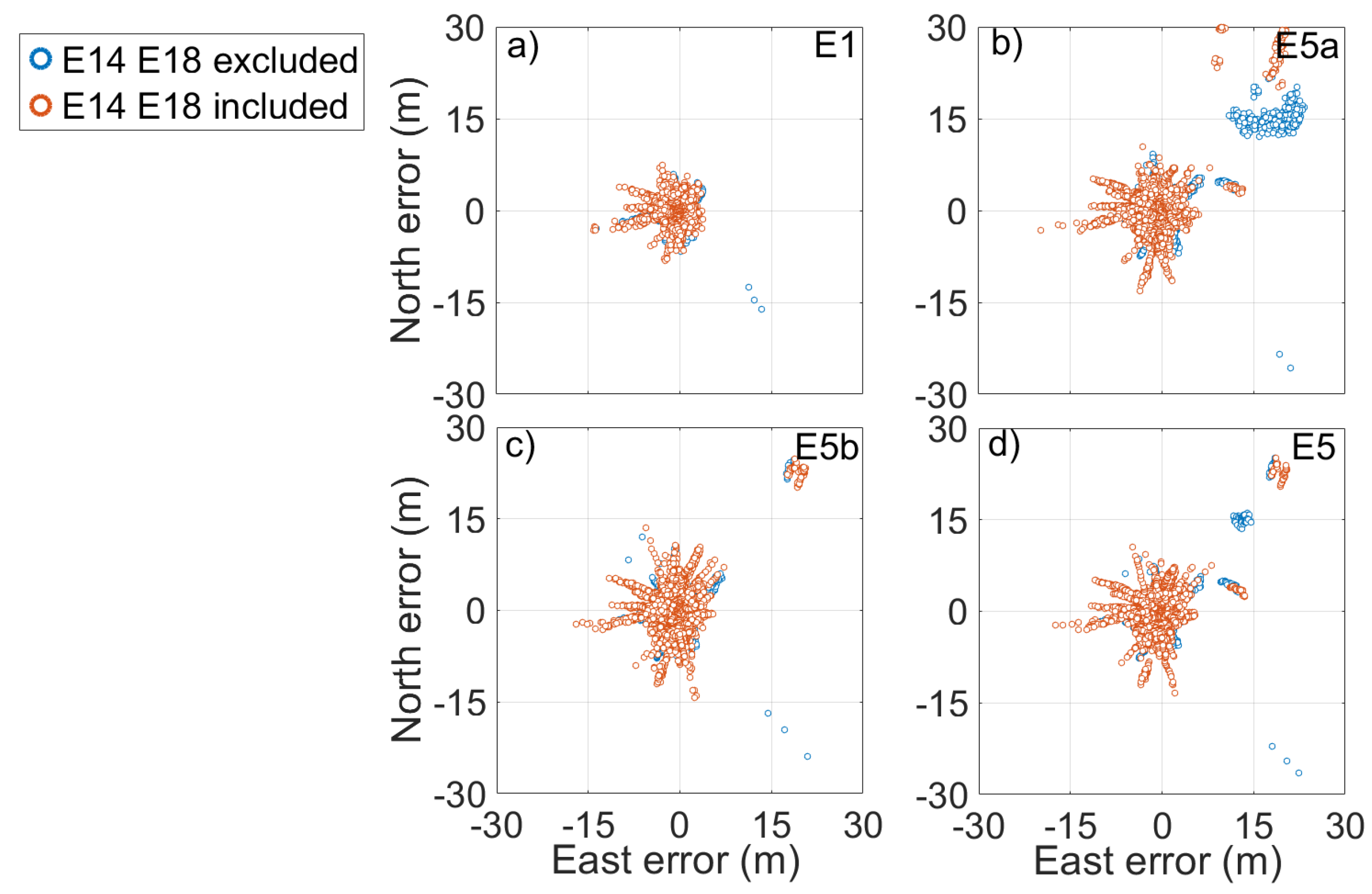
- When the broadcast ephemerides were used, nevertheless, the above results of the inclusion of the satellites worsened both the horizontal and vertical accuracy of the solution. The deterioration of the horizontal accuracy went from 0.17 m with the E5a frequency measurements to 0.74 m with the E1 measurements. The reduction of vertical accuracy went from 0.68 m for the E5a to 1.2 m for the E1 measurements. This was not completely expected as both the HDOP and VDOP parameters decreased when these satellites were included in the solution calculation. However, if precise ephemerides are used instead of broadcast ones, both the horizontal and the vertical accuracy remained stable; actually, for the E5b frequency, the DRMS improved by almost 0.5 m.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Available online: https://www.gsc-europa.eu/sites/default/files/NOTICE_ADVISORY_TO_Galileo_USERS_NAGU_2014014.txt (accessed on 23 November 2018).
- Navarro-Reyes, D.; Castro, R.; Bosch, P.R. Galileo first FOC launch: Recovery mission design. In Proceedings of the 25th International Symposium on Space Flight Dynamics ISSFD, Munich, Germany, 19–23 October 2015. [Google Scholar]
- Carlier, N.; Gülmüs, O. Spacecraft recovery operations conducted to the Galileo FOC-1 L3. In Proceedings of the 25th International Symposium on Space Flight Dynamics ISSFD, Munich, Germany, 19–23 October 2015. [Google Scholar]
- Pugliano, G.; Robustelli, U.; Rossi, F.; Santamaria, R. A new method for specular and diffuse pseudorange multipath error extraction using wavelet analysis. GPS Solut. 2016, 20, 499–508. [Google Scholar] [CrossRef]
- Robustelli, U.; Benassai, G.; Pugliano, G. Accuracy evaluation of Doresa and Milena Galileo satellites broadcast ephemerides. In Proceedings of the 2nd IEEE International Workshop on Metrology for the Sea, Bari, Italy, 8–10 October 2018. [Google Scholar] [CrossRef]
- Robustelli, U.; Benassai, G.; Pugliano, G. Signal in Space Error and Ephemeris Validity Time Evaluation of Milena and Doresa Galileo Satellites. Sensors 2019, 19, 1786. [Google Scholar] [CrossRef] [PubMed]
- Robustelli, U.; Pugliano, G. GNSS code multipath short time fourier transform analysis. Navi 2018, 65, 353–362. [Google Scholar] [CrossRef]
- Robustelli, U.; Pugliano, G. Code multipath analysis of Galileo FOC satellites by time-frequency representation. Appl. Geomat. 2018, 11, 69. [Google Scholar] [CrossRef]
- Robustelli, U.; Baiocchi, V.; Pugliano, G. Assessment of Dual Frequency GNSS Observations from a Xiaomi Mi 8 Android Smartphone and Positioning Performance Analysis. Electronics 2019, 8, 91. [Google Scholar] [CrossRef]
- Zaminpardaz, S.; Teunissen, P.J.G. Analysis of Galileo IOV + FOC signals and E5 RTK performance. GPS Solut. 2017, 21, 1855–1870. [Google Scholar] [CrossRef]
- Li, W.; Nadarajah, N.; Teunissen, P.J.; Khodabandeh, A.; Chai, Y. Array-aided single-frequency state-space RTK with combined GPS, Galileo, IRNSS, and QZSS L5/E5a observations. J. Surv. Eng. 2017, 143, 04017006. [Google Scholar] [CrossRef]
- Paziewski, J.; Sieradzki, R.; Wielgosz, P. On the applicability of Galileo FOC satellites with incorrect highly eccentric orbits: An evaluation of instantaneous medium-range positioning. Remote Sens. 2018, 10, 208. [Google Scholar] [CrossRef]
- Wang, K.; Khodabandeh, A.; Teunissen, P.J.G. Five-frequency Galileo long-baseline ambiguity resolution with multipath mitigation. GPS Solut. 2018, 22, 75. [Google Scholar] [CrossRef] [Green Version]
- Sosnica, K.; Prange, L.; Kazmierski, K.; Bury, G.; Drozdzewski, M.; Zajedel, R.; Hadas, T. Validation of Galileo orbits using SLR with a focus on satellites launched into incorrect orbital planes. J. Geod. 2018, 92, 131–148. [Google Scholar] [CrossRef]
- ESA. European Union. Galileo Open Service. Available online: http://www.esa.int/Our_Activities/Navigation/Galileo/What_is_Galileo (accessed on 23 April 2019).
- Kaplan, E.D.; Hegarty, C. Understanding GPS/GNSS: Principles and Applications, 3rd ed.; Artech House: Norwood, MA, USA, 2017. [Google Scholar]
- Strang, G.; Borre, K. Linear Algebra, Geodesy, and GPS; Wellesley-Cambridge Press: Wellesley, MA, USA, 1997. [Google Scholar]
- Langley, R. Diluition of precision. GPS World 1999, 10, 52–59. [Google Scholar]
- Dow, J.M.; Neilan, R.E.; Rizos, C. The International GNSS Service in a changing landscape of Global Navigation Satellite Systems. J. Geod. 2009, 83, 191–198. [Google Scholar] [CrossRef]
- IGS. Current IGS Definition. Available online: https://kb.igs.org/hc/en-us/articles/202011433-Current-IGS-Site-Guidelines (accessed on 23 April 2019).
- Nicolini, L.; Caporali, A. Investigation on Reference Frames and Time Systems in Multi-GNSS. Remote Sens. 2018, 10, 80. [Google Scholar] [CrossRef]
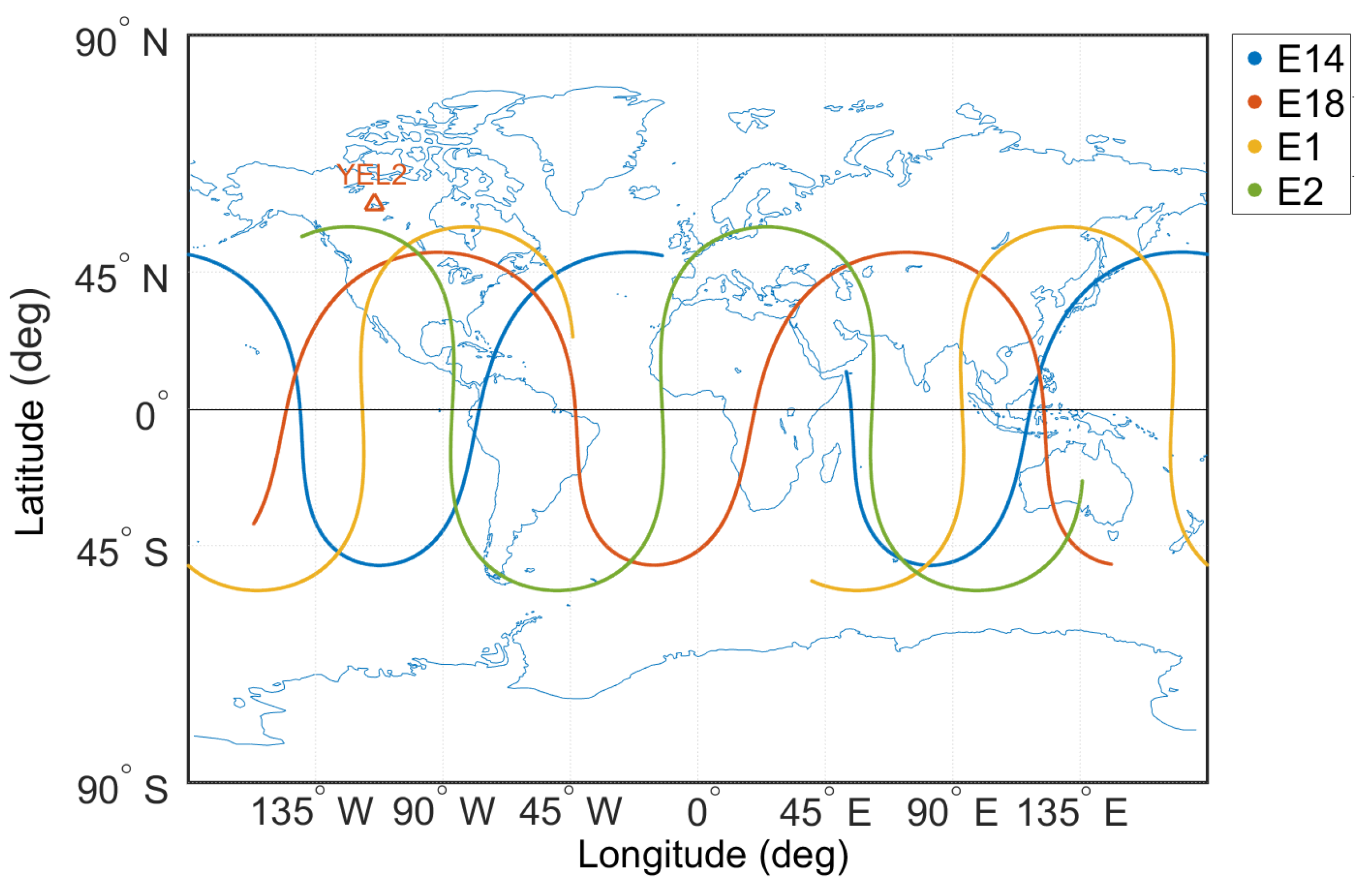
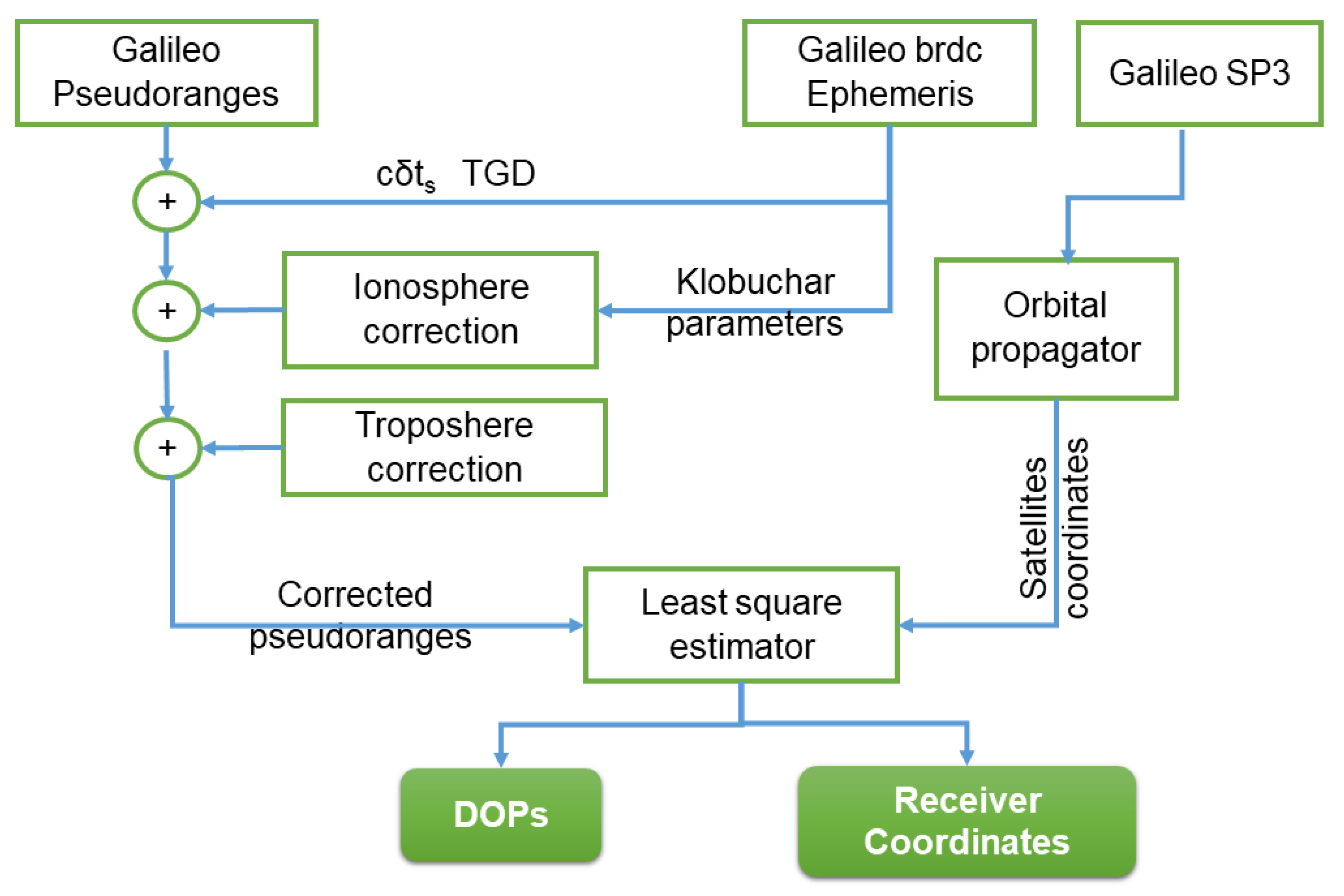

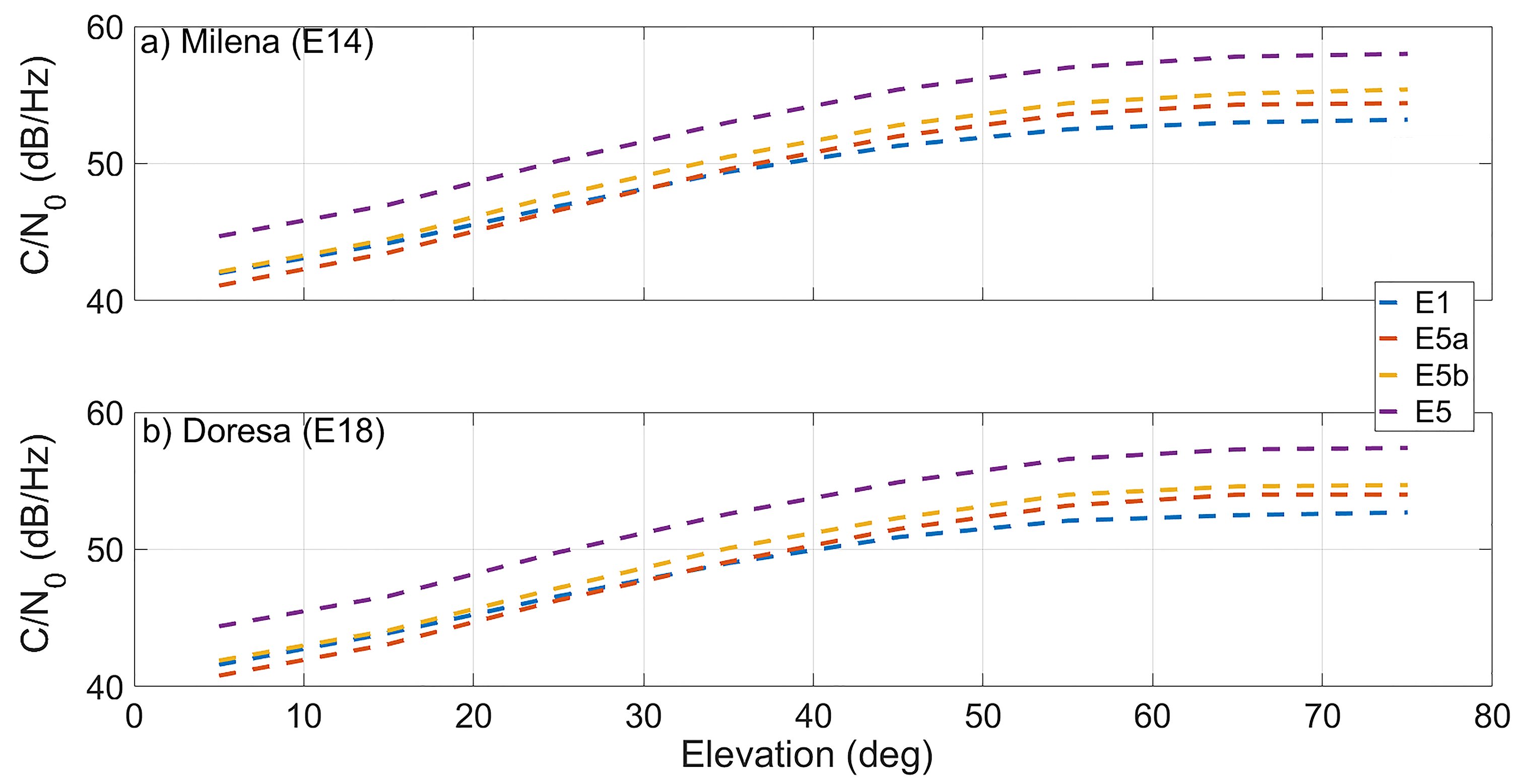
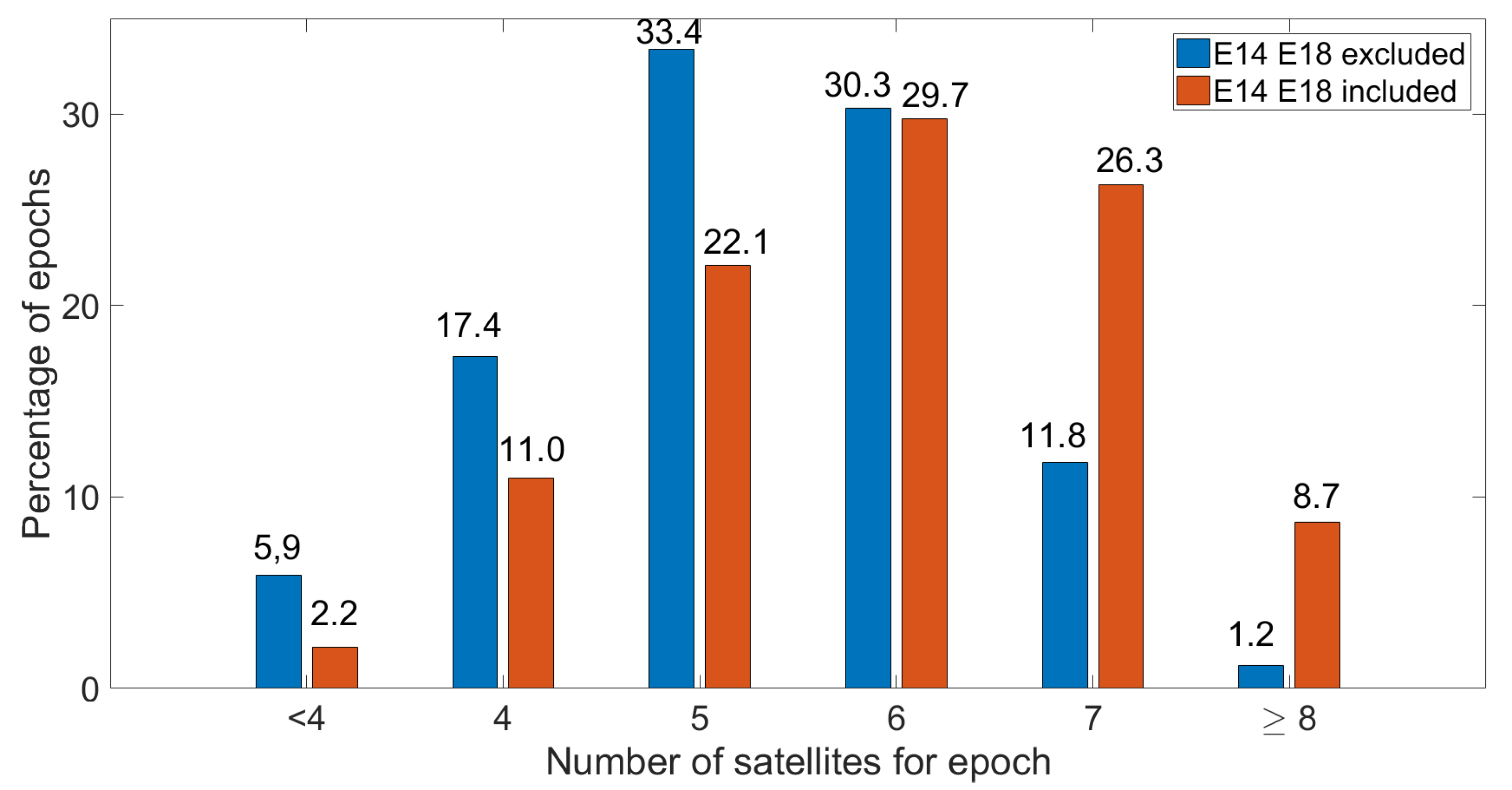




| Satellite | Semi-Major Axis (km) | Eccentricity | Inclination (deg) | Orbital Period (h) |
|---|---|---|---|---|
| IOV and FOC | 29,599.8 | 0.000 | 56.0 | 14.08 |
| E14 and E18 | 27,977.6 | 0.162 | 49.850 | 12.97 |
| Freq. | DRMS Excl. | Vert. RMS Excl. | DRMS Incl. | Vert. RMS Incl. |
|---|---|---|---|---|
| E1 | 1.21 | 2.74 | 1.95 | 3.97 |
| E5a | 2.21 | 5.80 | 2.38 | 6.48 |
| E5b | 1.91 | 4.66 | 2.45 | 5.77 |
| E5 | 1.85 | 4.82 | 2.38 | 5.71 |
| Freq. | DRMS Excl. | Vert. RMS Excl. | DRMS Incl. | Vert. RMS Incl. |
|---|---|---|---|---|
| E1 | 0.97 | 2.23 | 0.94 | 2.06 |
| E5a | 2.08 | 5.56 | 1.60 | 5.45 |
| E5b | 1.55 | 4.06 | 1.53 | 4.12 |
| E5 | 1.59 | 4.39 | 1.53 | 4.28 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Robustelli, U.; Pugliano, G. Galileo Single Point Positioning Assessment Including FOC Satellites in Eccentric Orbits. Remote Sens. 2019, 11, 1555. https://doi.org/10.3390/rs11131555
Robustelli U, Pugliano G. Galileo Single Point Positioning Assessment Including FOC Satellites in Eccentric Orbits. Remote Sensing. 2019; 11(13):1555. https://doi.org/10.3390/rs11131555
Chicago/Turabian StyleRobustelli, Umberto, and Giovanni Pugliano. 2019. "Galileo Single Point Positioning Assessment Including FOC Satellites in Eccentric Orbits" Remote Sensing 11, no. 13: 1555. https://doi.org/10.3390/rs11131555
APA StyleRobustelli, U., & Pugliano, G. (2019). Galileo Single Point Positioning Assessment Including FOC Satellites in Eccentric Orbits. Remote Sensing, 11(13), 1555. https://doi.org/10.3390/rs11131555






