Designing Sustainable Public Transportation: Integrated Optimization of Bus Speed and Holding Time in a Connected Vehicle Environment
Abstract
:1. Introduction
- Explicitly capture the dynamic interaction between bus acceleration, speed, and holding time;
- Optimize bus speed and holding time of buses dynamically in an united framework;
- Satisfy the objectives of both improving driving patterns of buses and minimizing the bus delay, fuel consumption, and pollution emissions, which is conducive for city sustainability.
2. Development of the Optimization Model
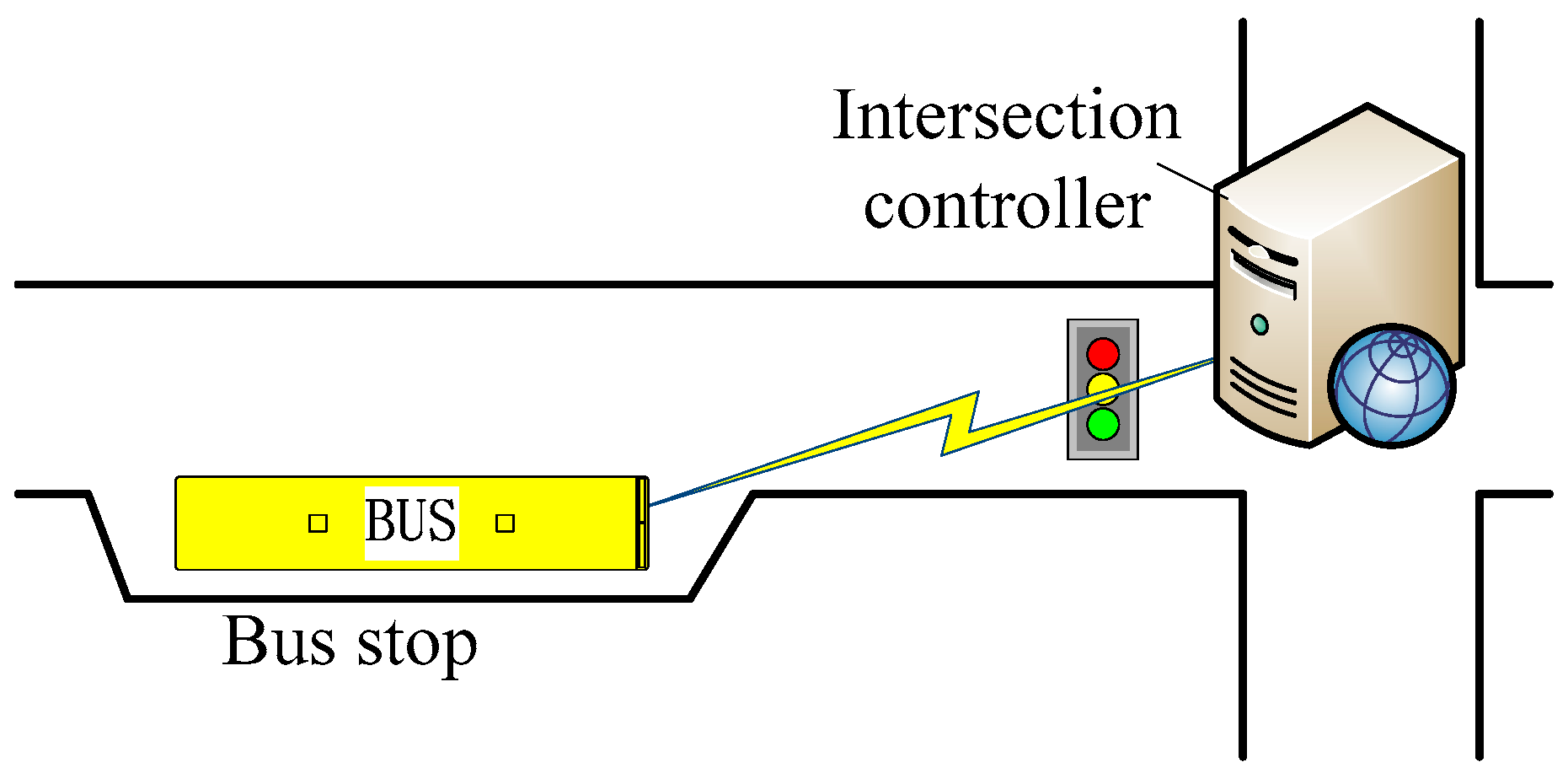
2.1. Problem Description
2.2. General Notations
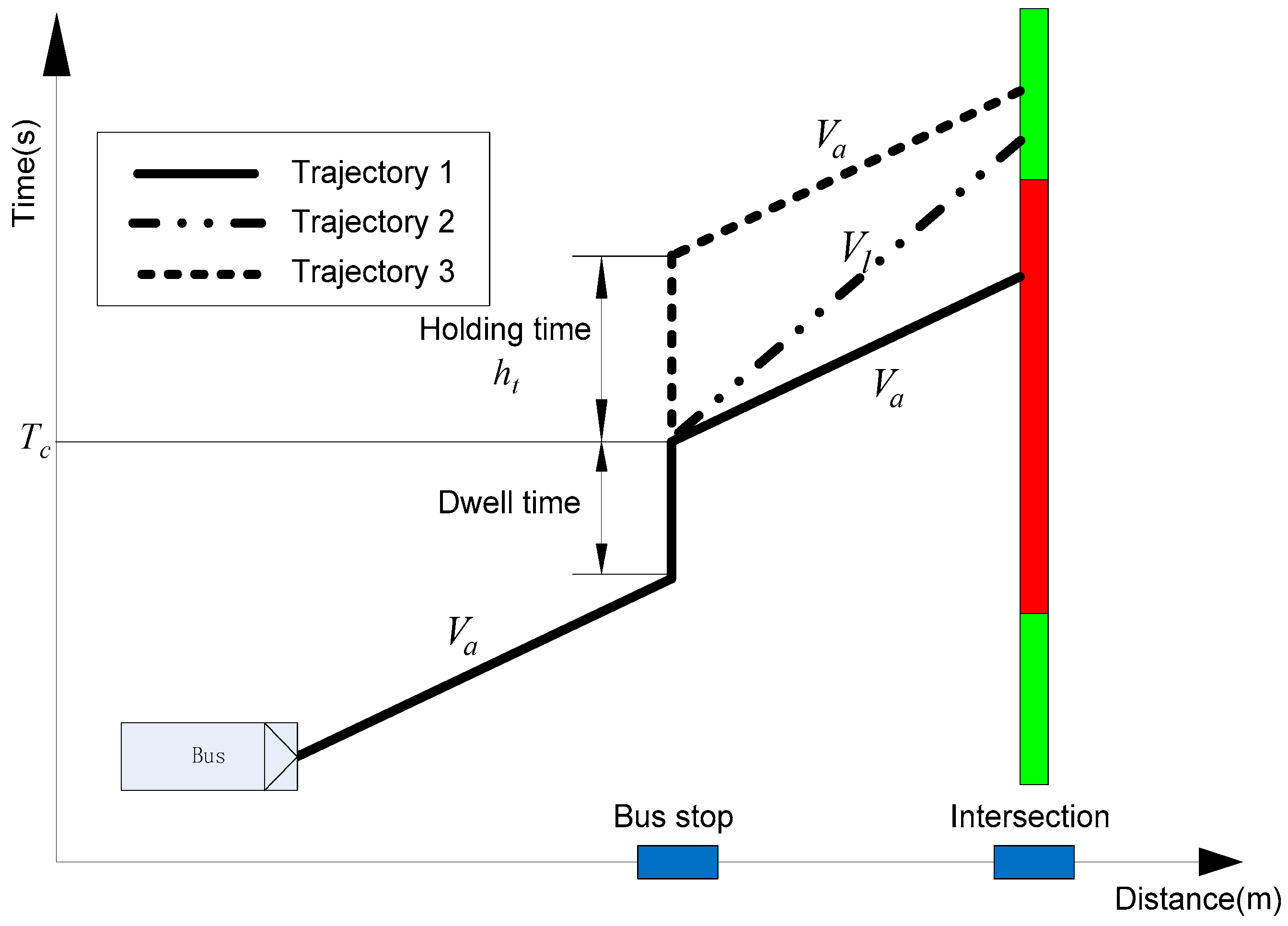
2.3. Objective Function
2.4. Decision Variables
- (1)
- Holding time at bus stop, .
- (2)
- Bus traveling speed, .
2.5. Constraints
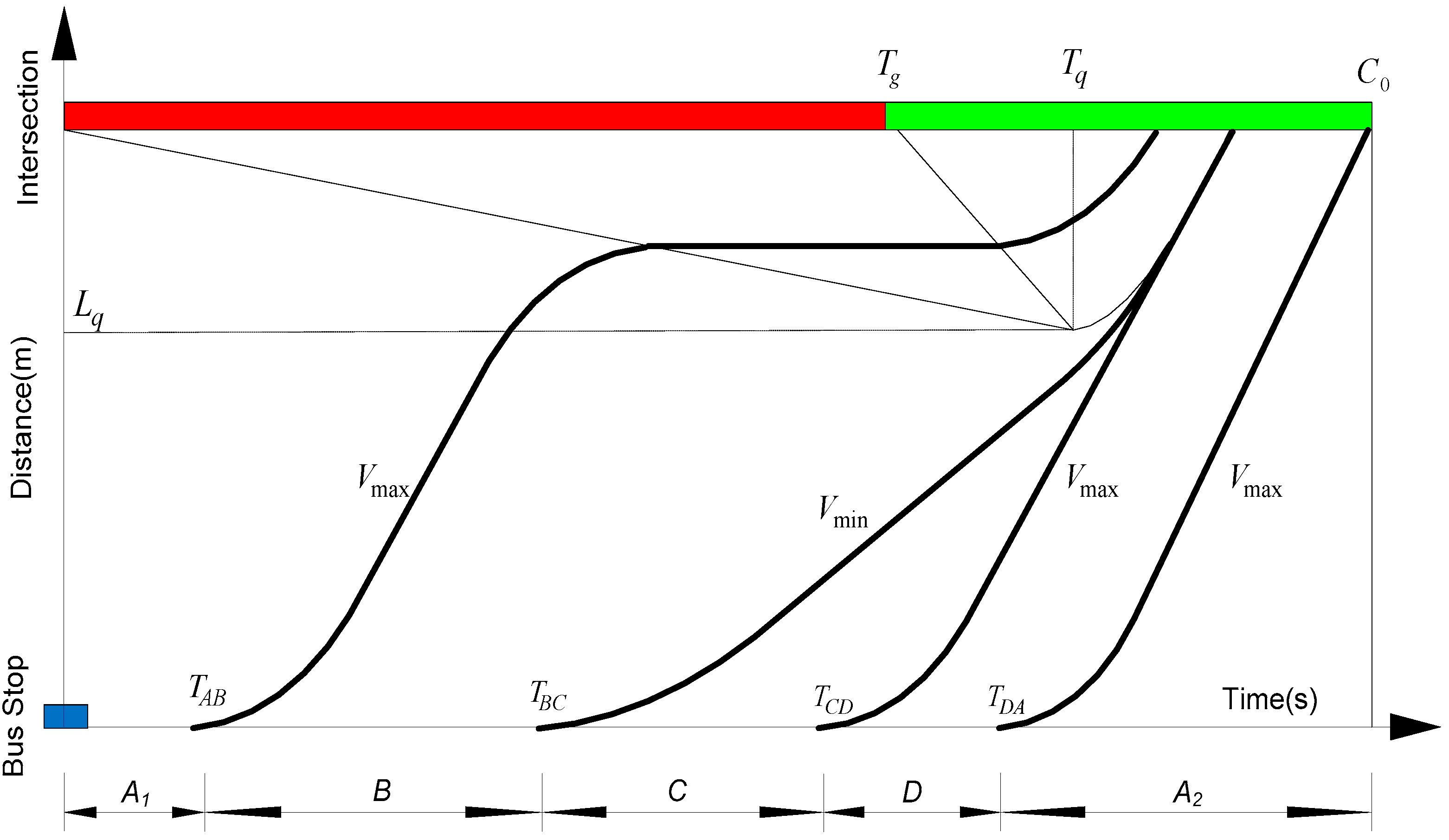
- Scenario A, when or (including A1 and A2);
- Scenario B, when ;
- Scenario C, when ;
- Scenario D, when .
2.5.1. Scenario A
2.5.2. Scenario B
2.5.3. Scenario C
2.5.4. Scenario D
2.5.5. Optimization of the Proposed Method
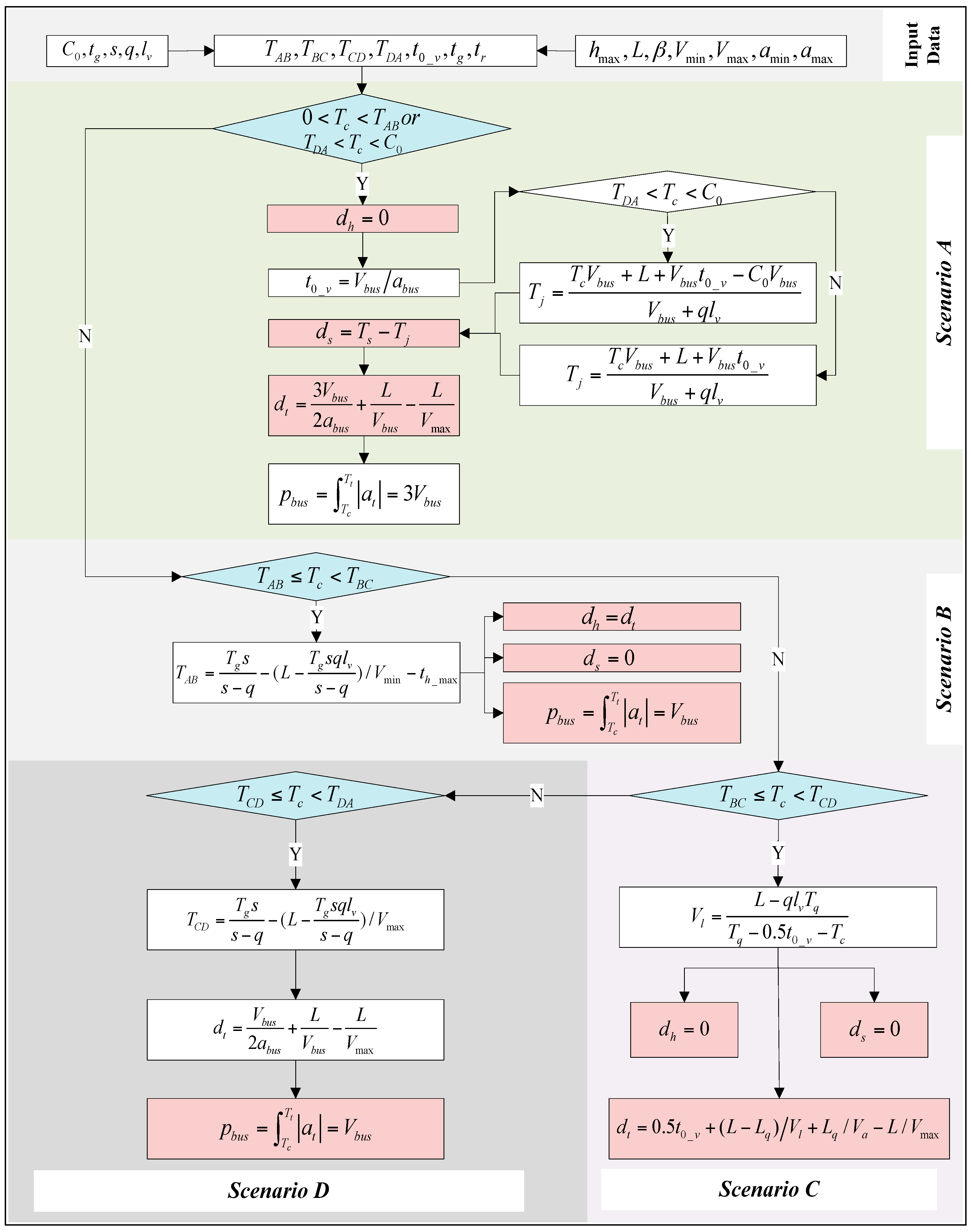
- Step 1: Given the cycle time, traffic demands, and the location of the bus stop, compute the green time duration, the maximum green extension time, and the time boundary points for each scenario.
- Step 2: If the bus arrival time is not located in scenario A, then turn to step 3. Otherwise, compute the time point at which the bus stopped by the red light and the time point at which the bus started to move by the green light. Then compute the bus signal delay caused by the red light, the queue, and the bus acceleration cost.
- Step 3: If the bus arrival time is not located in scenario B, then turn to step 4. Otherwise, compute the bus holding time and bus travel speed, then compute the bus holding delay, travel delay, and the bus acceleration cost.
- Step 4: If the bus arrival time is not located in scenario C, then turn to step 5. Otherwise, compute the optimal bus travel speed, and then compute the bus travel delay and the bus acceleration cost.
- Step 5: Compute the bus travelling delay and bus acceleration cost in scenario D.
3. Results and Discussion
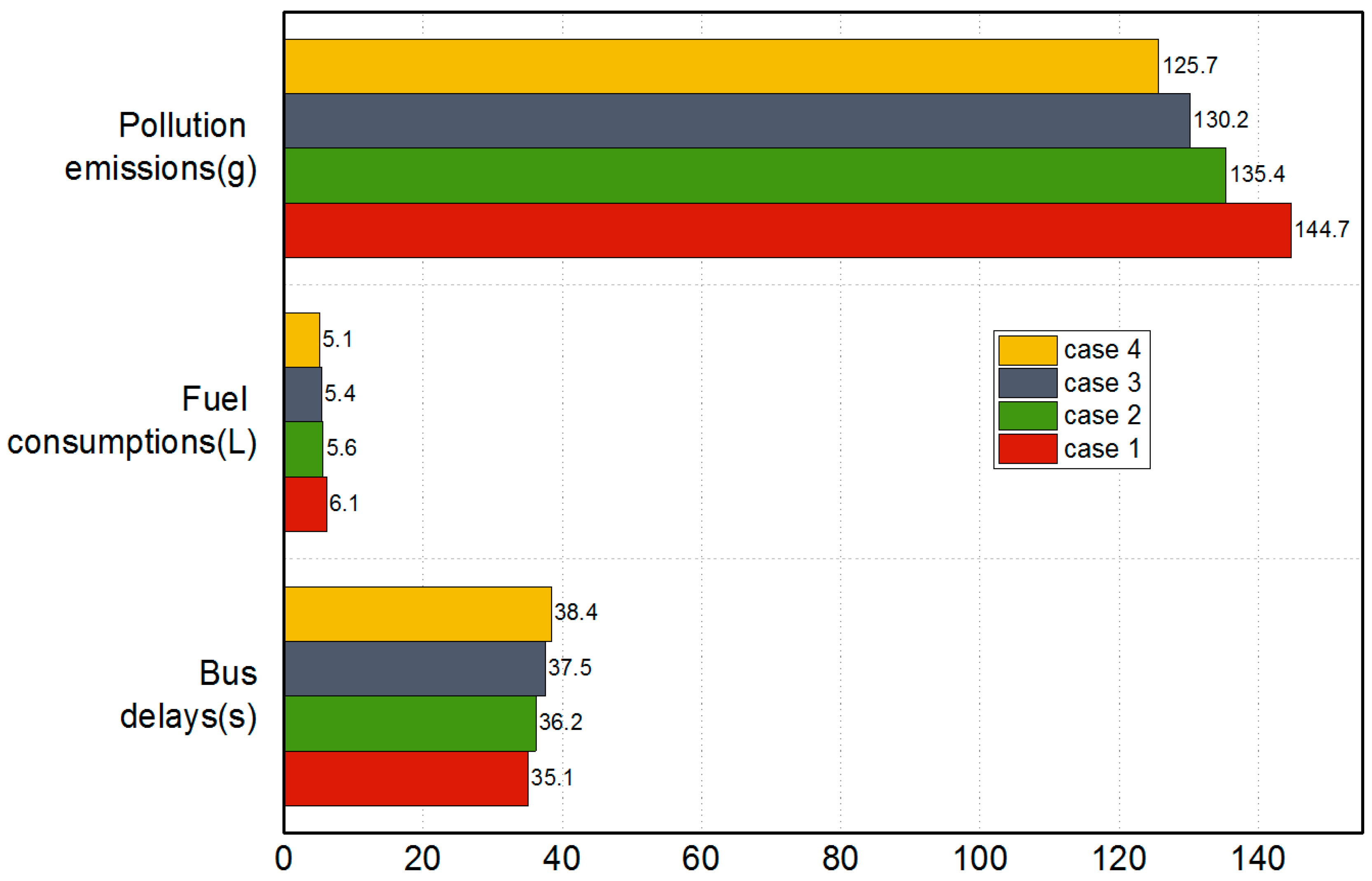
3.1. Performance Analysis
- Case 1: Traditional control method with neither holding nor speed control;
- Case 2: Speed control only;
- Case 3: Holding control only;
- Case 4: The proposed control method including both holding and speed control.
3.2. SimulationTest
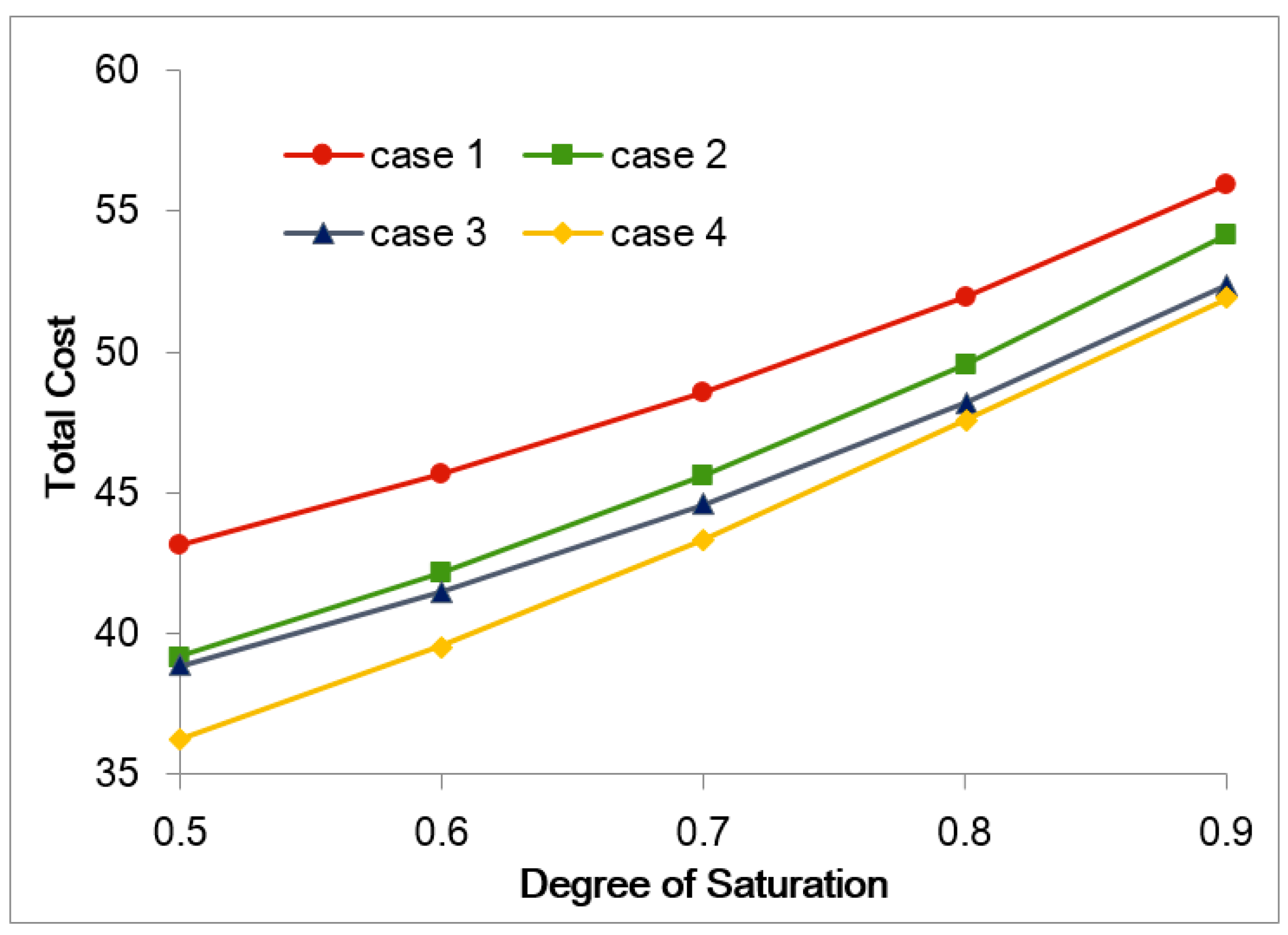
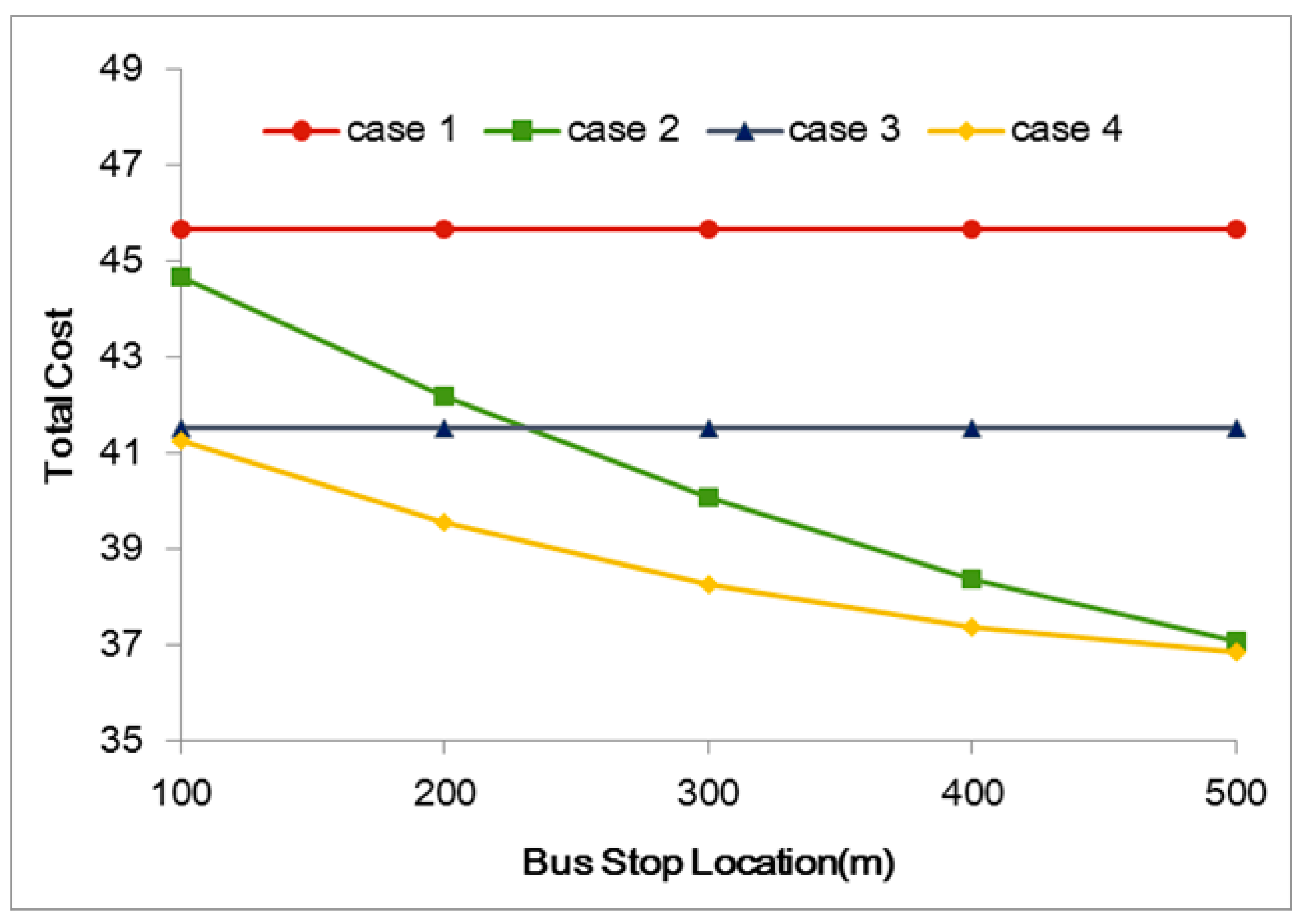
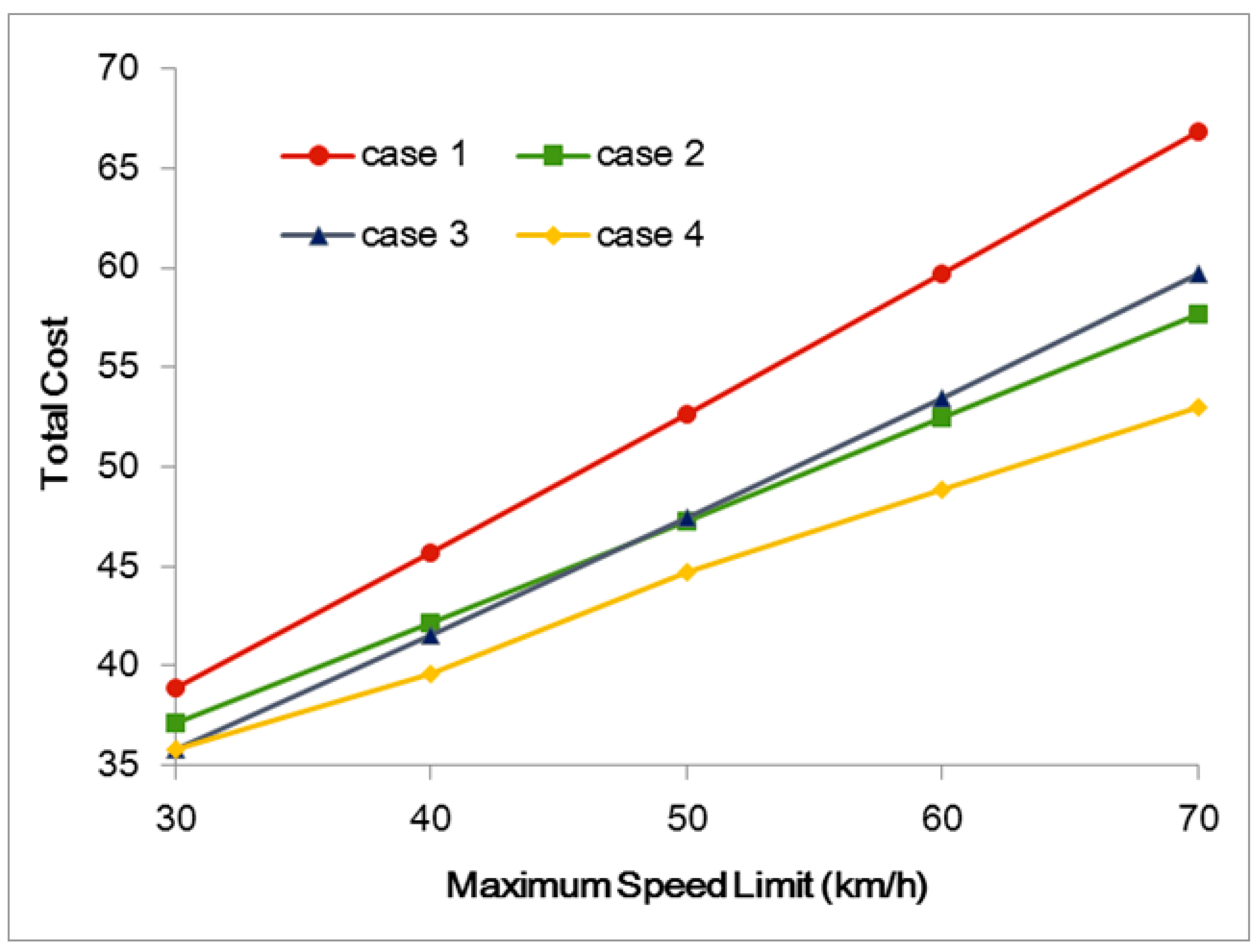
3.3. Sensitivity Analysis
3.3.1. Sensitivity Analysis with Degree of Saturation
3.3.2. Sensitivity Analysis with Bus Stop Location
3.3.3. Sensitivity Analysis with Maximum Speed Limits
4. Strengths and Limitations
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Schrank, D.; Eisele, B.; Lomax, T. 2014 Urban Mobility Report: Powered by INRIX Traffic Data; Southwest Region University Transportation Center: College Station, TX, USA, 2015. [Google Scholar]
- Zhang, W.; Lu, J.; Xu, P.; Zhang, Y. Moving towards Sustainability: Road Grades and On-Road Emissions of Heavy-Duty Vehicles—A Case Study. Sustainability 2015, 7, 12644–12671. [Google Scholar] [CrossRef]
- Verma, A.; Rahul, T.M.; Dixit, M. Sustainability impact assessment of transportation policies—A case study for Bangalore city. Case Stud. Trans. Policy 2015, 3, 321–330. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, Y.; Yang, X.; Liu, Q.; Li, C. Built Environment and Household Electric Bike Ownership: Insights from Zhongshan Metropolitan Area, China. Trans. Res. Rec. J. Trans. Res. Board 2013, 2387, 102–111. [Google Scholar] [CrossRef]
- Szeto, W.Y.; Jiang, Y.; Wang, D.Z.W.; Sumalee, A. A Sustainable Road Network Design Problem with Land Use Transportation Interaction over Time. Netw. Spat. Econ. 2013, 15, 1–32. [Google Scholar] [CrossRef]
- Hosseininasab, S.M.; Shetab-Boushehri, S.N. Integration of selecting and scheduling urban road construction projects as a time-dependent discrete network design problem. Eur. J. Oper. Res. 2015, 246, 762–771. [Google Scholar] [CrossRef]
- Zhao, J.; Ma, W.; Head, K.L.; Yang, X. Dynamic Turning Restriction Management for Multiple Signalized Intersections: A Lane-based Approach. In Proceedings of the Transportation Research Board 94th Annual Meeting, Washington, DC, USA, 11–15 January 2015.
- Han, K.; Liu, H.; Gayah, V.V.; Friesz, T.L.; Yao, T. A robust optimization approach for dynamic traffic signal control with emission considerations. Trans. Res. Part C Emerg. Technol. 2016, 7, 3–26. [Google Scholar] [CrossRef]
- Cui, C.Y.; Shin, J.S.; Miyazaki, M.; Lee, H.H. Real-time traffic signal control for optimization of traffic jam probability. IEEJ Trans. Electron. Inf. Syst. 2013, 96, 1–13. [Google Scholar] [CrossRef]
- Zhang, Y.; Wu, W.; Li, Y.; Liu, Q.; Li, C. Does the Built Environment Make a Difference? An Investigation of Household Vehicle Use in Zhongshan Metropolitan Area, China. Sustainability 2014, 6, 4910–4930. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, Y.; Liu, Q.; Li, C. The Built Environment and Walking Activity of the Elderly: An Empirical Analysis in the Zhongshan Metropolitan Area, China. Sustainability 2014, 6, 1076–1092. [Google Scholar] [CrossRef]
- Moorhead, J.; Nixon, T. Carbon Pricing on the Horizon; Thomson Reuters: New York, NY, USA, 2015. [Google Scholar]
- Almasi, M.H.; Sadollah, A.; Kang, S.; Karim, M.R. Optimization of an Improved Intermodal Transit Model Equipped with Feeder Bus and Railway Systems Using Metaheuristics Approaches. Sustainability 2016, 8, 537. [Google Scholar] [CrossRef]
- Nuzzolo, A.; Comi, A. Advanced Public Transport and ITS: New modelling challenges. Trans. Trans. Sci. 2016, 12, 674–699. [Google Scholar]
- Bigerna, S.; Polinori, P. Willingness to Pay and Public Acceptance for Hydrogen Buses: A Case Study of Perugia. Sustainability 2015, 7, 13270–13289. [Google Scholar] [CrossRef]
- He, H.; Guler, S.I.; Menendez, M. Adaptive control algorithm to provide bus priority with a pre-signal. Trans. Res. Part C Emerg. Technol. 2016, 64, 28–44. [Google Scholar] [CrossRef]
- Moreira-Matias, L.; Mendes-Moreira, J.; De Sousa, J.F.; Gama, J. Improving Mass Transit Operations by Using AVL-Based Systems: A Survey. IEEE Trans. Intell. Trans. Syst. 2015, 16, 1636–1653. [Google Scholar] [CrossRef]
- Hickman, M.D. An analytic stochastic model for the transit vehicle holding problem. Trans. Sci. 2001, 35, 215–237. [Google Scholar] [CrossRef]
- Eberlein, X.J.; Wilson, N.H.M.; Bernstein, D. The Holding Problem with Real-Time Information Available. Trans. Sci. 2001, 35, 1–18. [Google Scholar] [CrossRef]
- Zhao, J.; Bukkapatnam, S.; Dessouky, M.M. Distributed architecture for real-time coordination of bus holding in transit networks. IEEE Trans. Intell. Trans. Syst. 2003, 4, 43–51. [Google Scholar] [CrossRef]
- Xuan, Y.; Daganzo, C.F.; Cassidy, M.J. Increasing the capacity of signalized intersections with separate left turn phases. Trans. Res. Part B Methodol. 2011, 45, 769–791. [Google Scholar] [CrossRef]
- Zhao, J.; Dessouky, M.; Bukkapatnam, S. Optimal slack time for schedule-based transit operations. Trans. Sci. 2006, 40, 529–539. [Google Scholar] [CrossRef]
- Sun, A.; Mark, H. Scheduling considerations for a branching transit route. J. Adv. Trans. 2010, 38, 243–290. [Google Scholar] [CrossRef]
- Steinzen, I.; Gintner, V.; Suhl, L.; Kliewer, N. A Time-Space Network Approach for the Integrated Vehicle-and Crew-Scheduling Problem with Multiple Depots. Trans. Sci. 2010, 44, 367–382. [Google Scholar] [CrossRef]
- Daganzo, C.F. A headway-based approach to eliminate bus bunching: Systematic analysis and comparisons. Trans. Res. Part B Methodol. 2009, 43, 913–921. [Google Scholar] [CrossRef]
- Dell’Olio, L.; Ibeas, A.; Ruisánchez, F. Optimizing bus-size and headway in transit networks. Transportation 2012, 39, 449–464. [Google Scholar] [CrossRef]
- Zolfaghari, S.; Azizi, N.; Jaber, M.Y. A model for holding strategy in public transit systems with real-time information. Int. J. Trans. Manag. 2004, 2, 99–110. [Google Scholar] [CrossRef]
- Koehler, L.A.; Kraus, W. Simultaneous control of traffic lights and bus departure for priority operation. Trans. Res. Part C Emerg. Technol. 2010, 18, 288–298. [Google Scholar] [CrossRef]
- Wu, J.; Liu, M.; Sun, H.; Li, T.; Gao, Z.; Wang, D.Z. Equity-based timetable synchronization optimization in urban subway network. Trans. Res. Part C Emerg. Technol. 2015, 51, 1–18. [Google Scholar] [CrossRef]
- Ma, W.; Liu, Y.; Yang, X. A dynamic programming approach for optimal signal priority control upon multiple high-frequency bus requests. J. Intell. Trans. Syst. 2013, 17, 282–293. [Google Scholar] [CrossRef]
- Abdy, Z.R.; Hellinga, B.R. Analytical method for estimating the impact of transit signal priority on vehicle delay. J. Trans. Eng. 2011, 137, 589–600. [Google Scholar] [CrossRef]
- Xu, H.; Sun, J.; Zheng, M. Comparative analysis of unconditional and conditional priority for use at isolated signalized intersections. J. Trans. Eng. 2010, 136, 1092–1103. [Google Scholar] [CrossRef]
- Ma, W.; Yang, X.; Liu, Y. A dynamic programming model for optimal bus signal priority control with multiple requests. In Proceedings of the 90th Transportation Research Board Annual Meeting, Washington, DC, USA, 23–27 January 2011.
- Liu, Y.; Chang, G.L.; Yu, J. An integrated control model for freeway corridor under nonrecurrent congestion. IEEE Trans. Veh. Technol. 2011, 60, 1404–1418. [Google Scholar] [CrossRef]
- Smith, H.R.; Hemily, B.; Ivanovic, M. Transit Signal Priority (TSP): A Planning and Implementation Handbook. In Proceedings of the Ite Annual Meeting & Exhibit, Melbourne, Australia, 7–10 August 2015.
- Baker, R.J.; Collura, J.; Dale, J.J.; Head, L.; Hemily, B.; Ivanovic, M.; Jarzab, J.T.; Mccormick, D.; Obenberger, J.; Smith, L. An Overview of Transit Signal Priority. Available online: https://trid.trb.org/view.aspx?id=723986 (accessed on 27 October 2016).
- Dion, F.; Rakha, H. Evaluation of Potential Transit Signal Priority Benefits along a Fixed-Time Signalized Arterial. J. Trans. Eng. 2004, 130, 294–303. [Google Scholar] [CrossRef]
- Rakha, H.; Zhang, Y. Sensitivity Analysis of Transit Signal Priority Impacts on Operation of a Signalized Intersection. J. Trans. Eng. 2004, 130, 796–804. [Google Scholar] [CrossRef]
- Ghanim, M.; Dion, F.; Abu-Lebdeh, G. Integration of Signal Control and Transit Signal Priority Optimization in Coordinated Network Using Genetic Algorithms and Artificial Neural Networks. In Proceedings of the Transportation Research Board 88th Annual Meeting, Washington, DC, USA, 11–15 January 2009.
- Ma, W.; Liu, Y.; Han, B. A rule-based model for integrated operation of bus priority signal timings and traveling speed. J. Adv. Trans. 2013, 47, 369–383. [Google Scholar] [CrossRef]
- Abu-Lebdeh, G. Exploring the Potential Benefits of IntelliDrive-Enabled Dynamic Speed Control in Signalized Networks. In Proceedings of the 89th Annual Meeting of the Transportation Research Board, Washington, DC, USA, 10–14 January2010.
- Wu, W.; Li, P.K.; Zhang, Y. Modelling and simulation of vehicle speed guidance in connected vehicle environment. Int. J. Simul. Model. 2015, 14, 145–157. [Google Scholar] [CrossRef]
- Ding, J.; He, Q.; Head, L.; Saleem, F.; Wu, W. Development and Testing of Priority Control System in Connected Vehicle Environment. In Proceedings of the 92th Annual Meeting of the Transportation Research Board, Washington, DC, USA, 13–17 January2013.
| Key Variables | Definition |
|---|---|
| , | The maximum and minimum accelerations forbuses (m/s2) |
| Cycle length of the signal timing (s) | |
| Bus delay cost (s) | |
| Bus holding delay (s) | |
| Bus signal delay (s) | |
| Bus travel delay (s) | |
| The distance from bus stop to the intersection (m) | |
| The maximum queue length (m) | |
| The average vehicle length (m) | |
| Arrival flow rate (# of vehs/s) | |
| Saturation flow rate (# of vehs/s) | |
| Time for buses ready to depart from bus stop (s) | |
| Time for green light starts (s) | |
| Time for buses join in the queue (s) | |
| Time for queue dissipated (s) | |
| Time for buses clearing the intersection (s) | |
| , , , | The boundary point for scenario A, B, C and D (s) |
| Time duration for holding the bus at bus stop (s) | |
| Time duration for a bus accelerates from zero to bus traveling speed (s) | |
| Bus traveling speed (m/s) | |
| , | The maximum and minimum bus speed limits (m/s) |
| Scenario | Average Bus Delay Cost (Average Acceleration Cost) | |||
|---|---|---|---|---|
| Case 1 | Case 2 | Case 3 | Case 4 | |
| Scenario A (−24.9, 7.3) | 30.5 (33.3) | 30.5 (33.3) | 30.5 (33.3) | 30.5 (33.3) |
| Scenario B [7.3, 22.3) | 16.9 (33.3) | 18.7 (31.3) | 16.9 (33.3) | 26.1 (11.1) |
| Scenario C [22.3, 36.0) | 8.8 (33.3) | 10.2 (11.1) | 10.2 (11.1) | 10.2 (11.1) |
| Scenario D [36.0, 50.1] | 1.85 (11.1) | 1.85 (11.1) | 1.85 (11.1) | 1.85 (11.1) |
| Cases | |||
|---|---|---|---|
| Case 1 | [36.0, 50.1] | 14.1 | 20.1% |
| Case 2 | [21.0, 50.1] | 29.1 | 41.6% |
| Case 3 | [22.3, 50.1] | 27.8 | 39.7% |
| Case 4 | [7.3, 50.1] | 42.8 | 61.1% |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, W.; Ma, W.; Long, K.; Zhou, H.; Zhang, Y. Designing Sustainable Public Transportation: Integrated Optimization of Bus Speed and Holding Time in a Connected Vehicle Environment. Sustainability 2016, 8, 1170. https://doi.org/10.3390/su8111170
Wu W, Ma W, Long K, Zhou H, Zhang Y. Designing Sustainable Public Transportation: Integrated Optimization of Bus Speed and Holding Time in a Connected Vehicle Environment. Sustainability. 2016; 8(11):1170. https://doi.org/10.3390/su8111170
Chicago/Turabian StyleWu, Wei, Wanjing Ma, Kejun Long, Heping Zhou, and Yi Zhang. 2016. "Designing Sustainable Public Transportation: Integrated Optimization of Bus Speed and Holding Time in a Connected Vehicle Environment" Sustainability 8, no. 11: 1170. https://doi.org/10.3390/su8111170
APA StyleWu, W., Ma, W., Long, K., Zhou, H., & Zhang, Y. (2016). Designing Sustainable Public Transportation: Integrated Optimization of Bus Speed and Holding Time in a Connected Vehicle Environment. Sustainability, 8(11), 1170. https://doi.org/10.3390/su8111170





