Influence of Geometrical Features on the Cyclic Behavior of S-Shaped Steel Dampers Used in Sustainable Seismic Isolation: Experimental Insight with Numerical Validation
Abstract
1. Introduction
2. Experimental Investigation
2.1. Material Properties
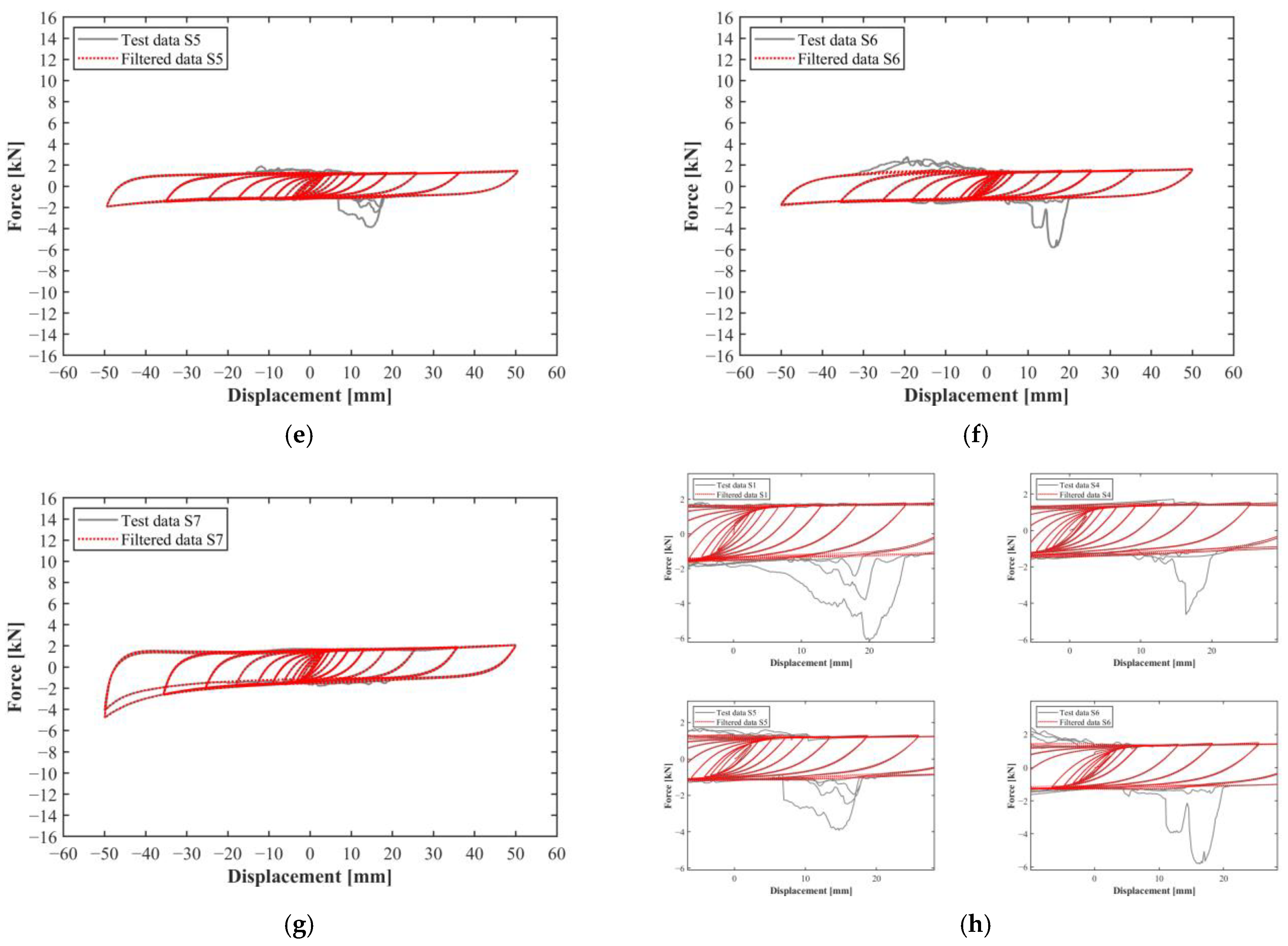
2.2. Quasi-Static Cyclic Shear Test
2.2.1. Specimen Design
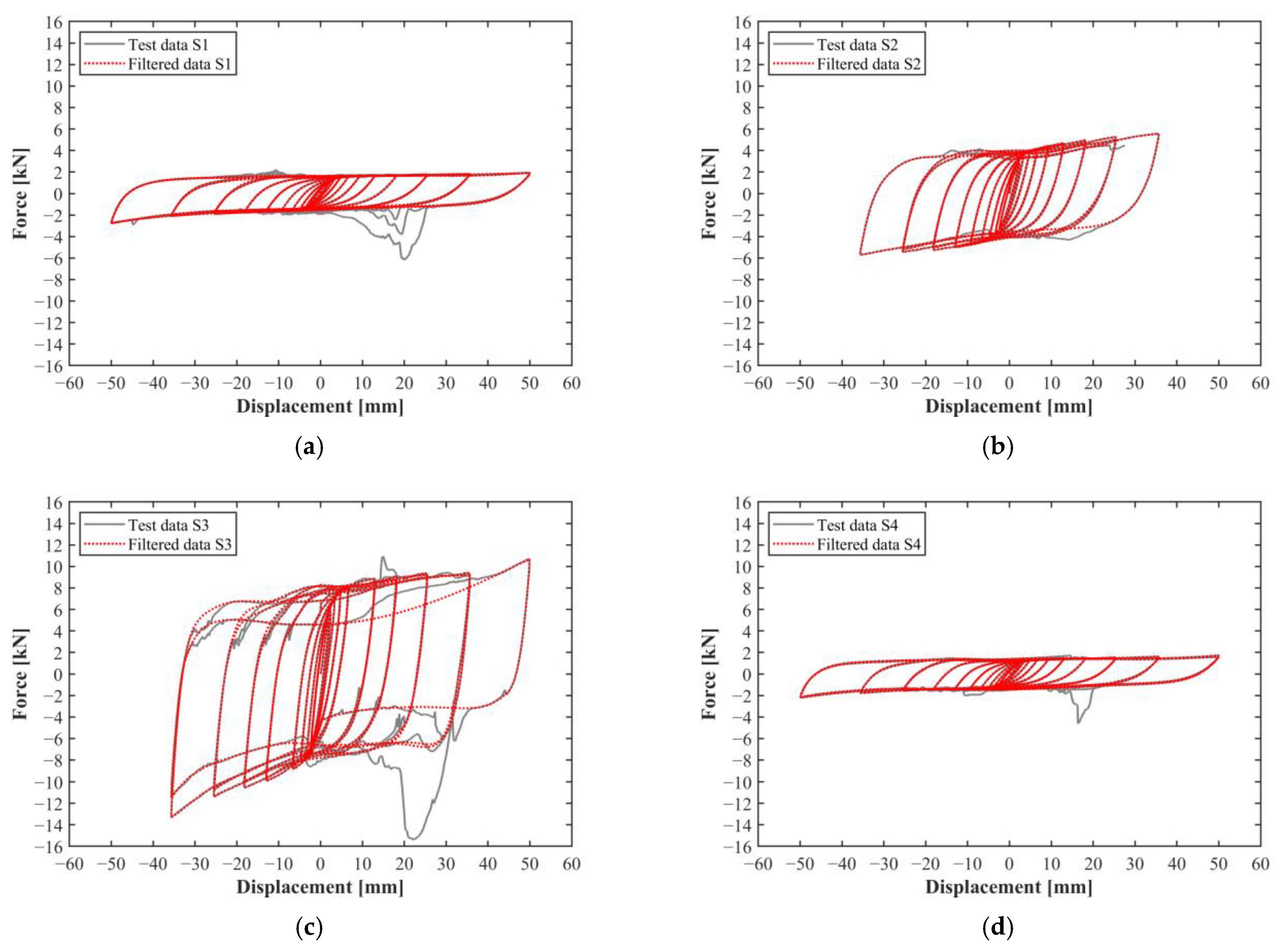
2.2.2. Experimental Tests
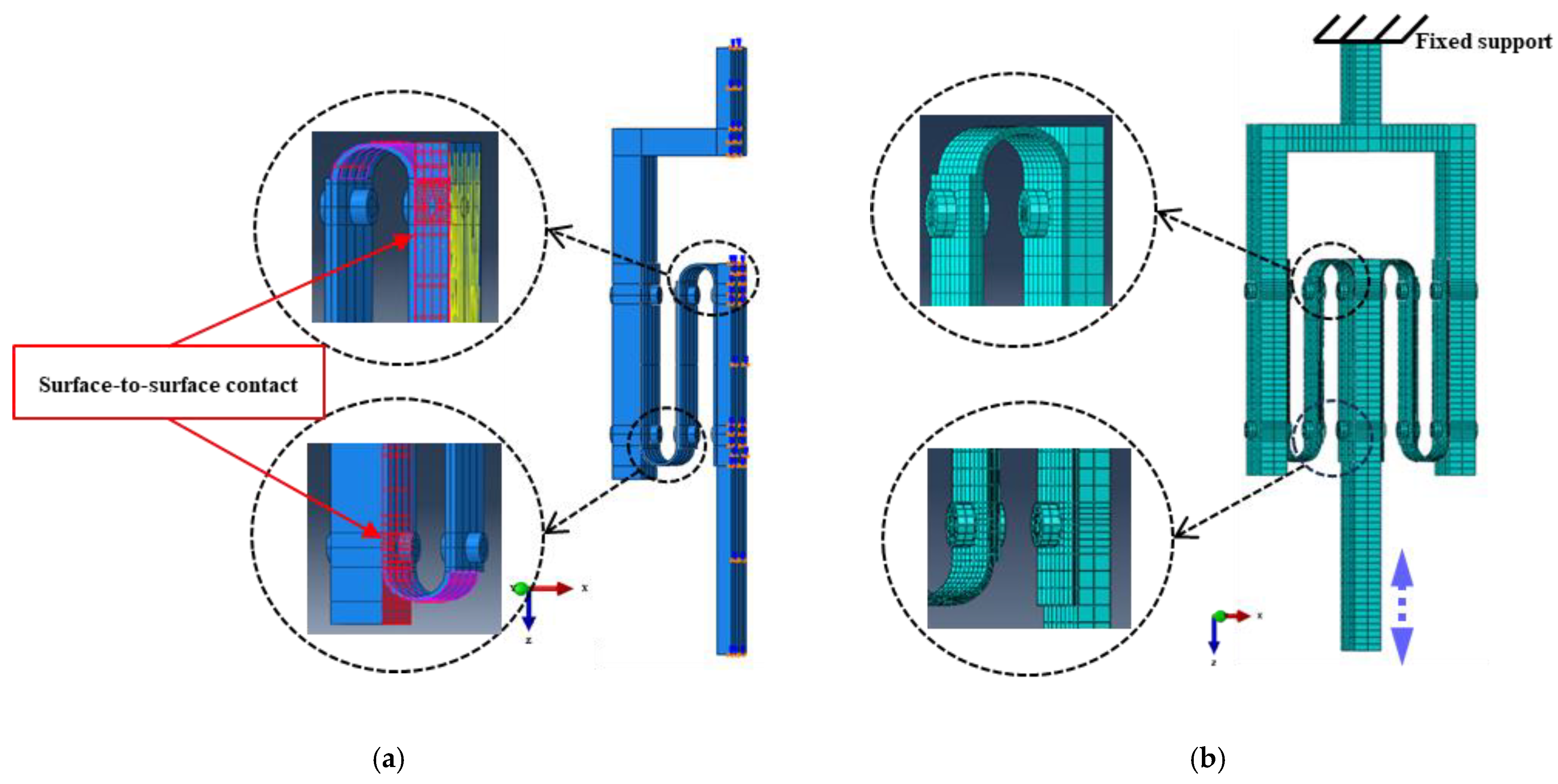
3. Numerical Investigation
3.1. Finite Element Model
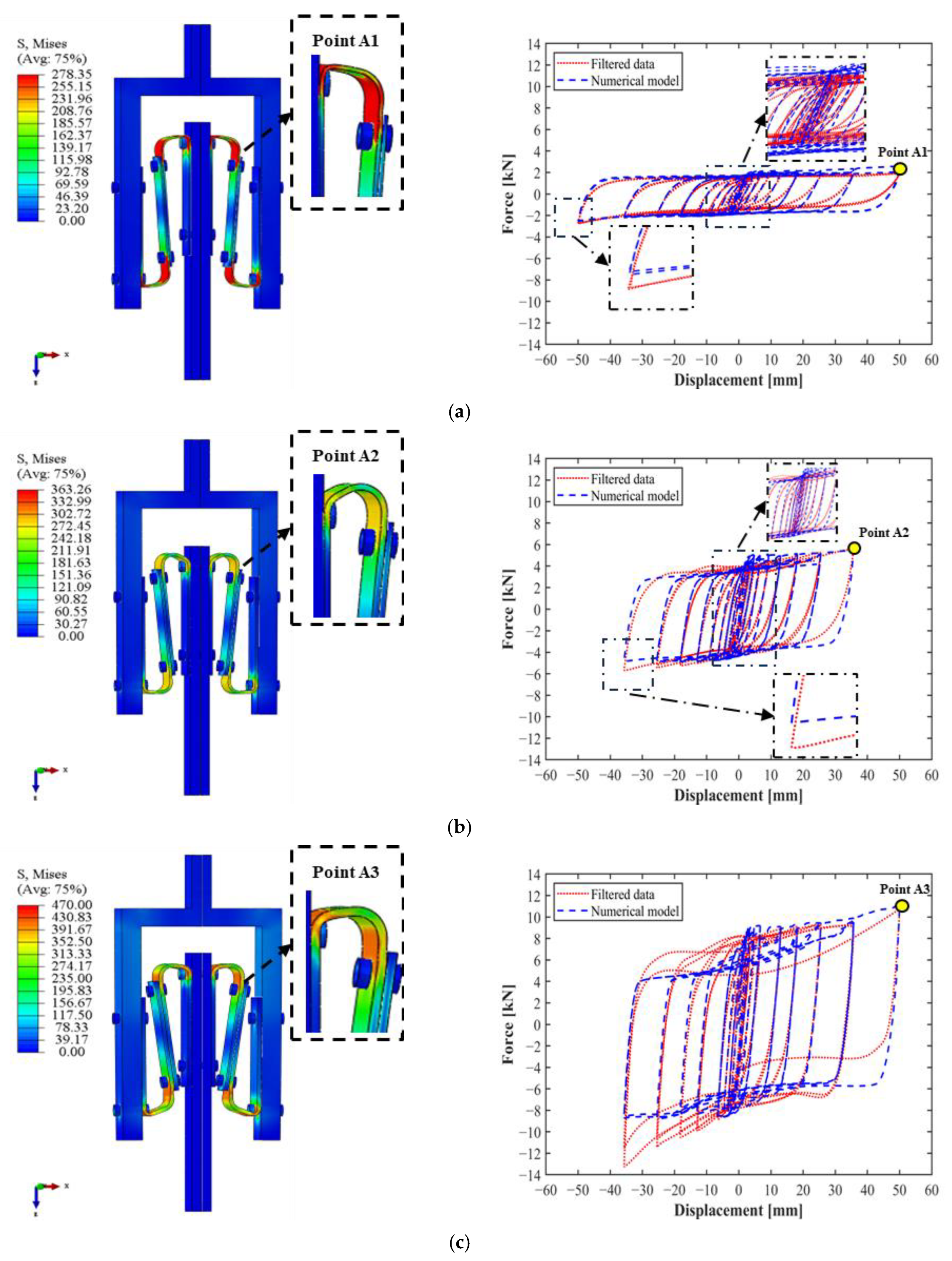
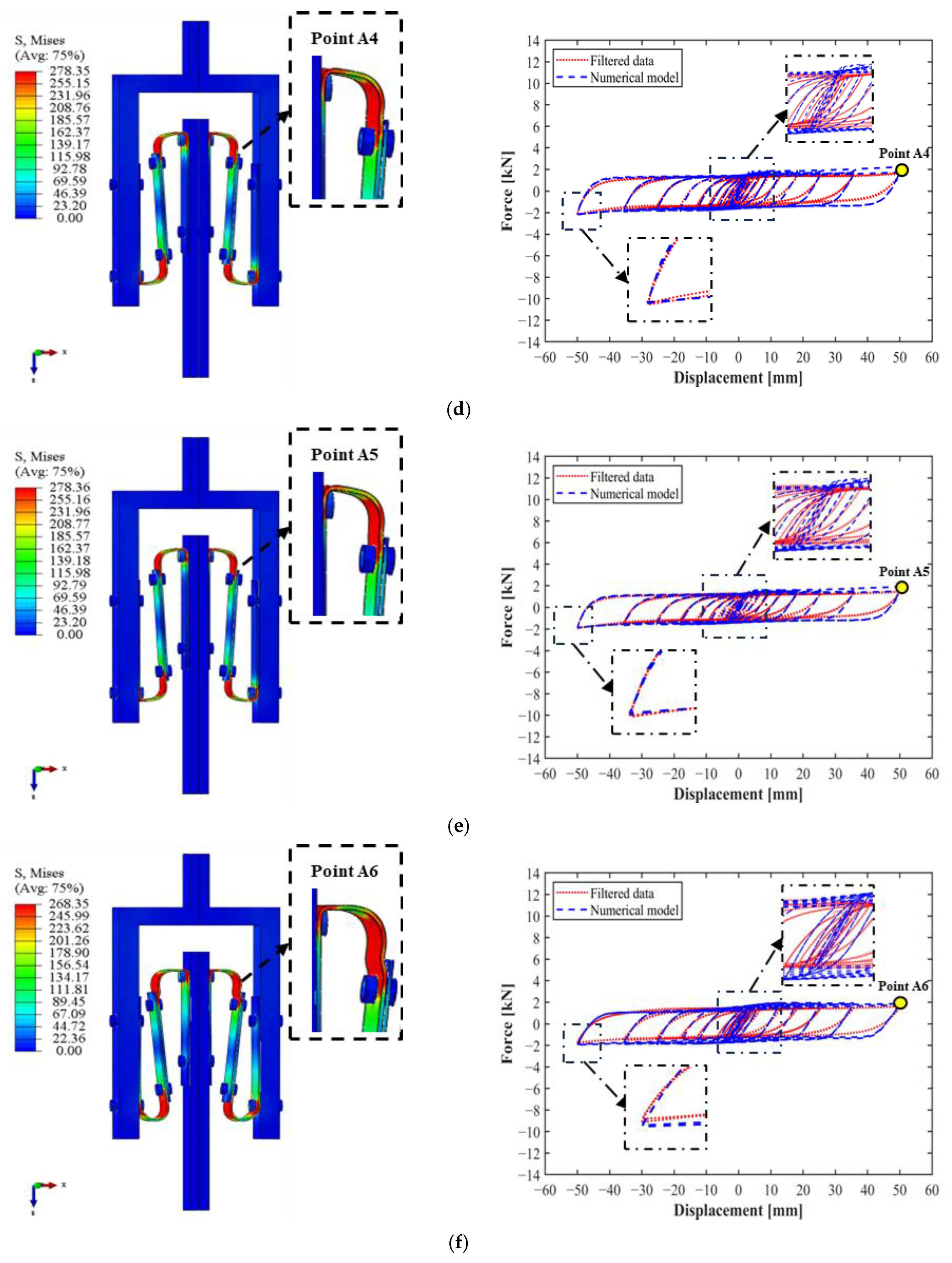
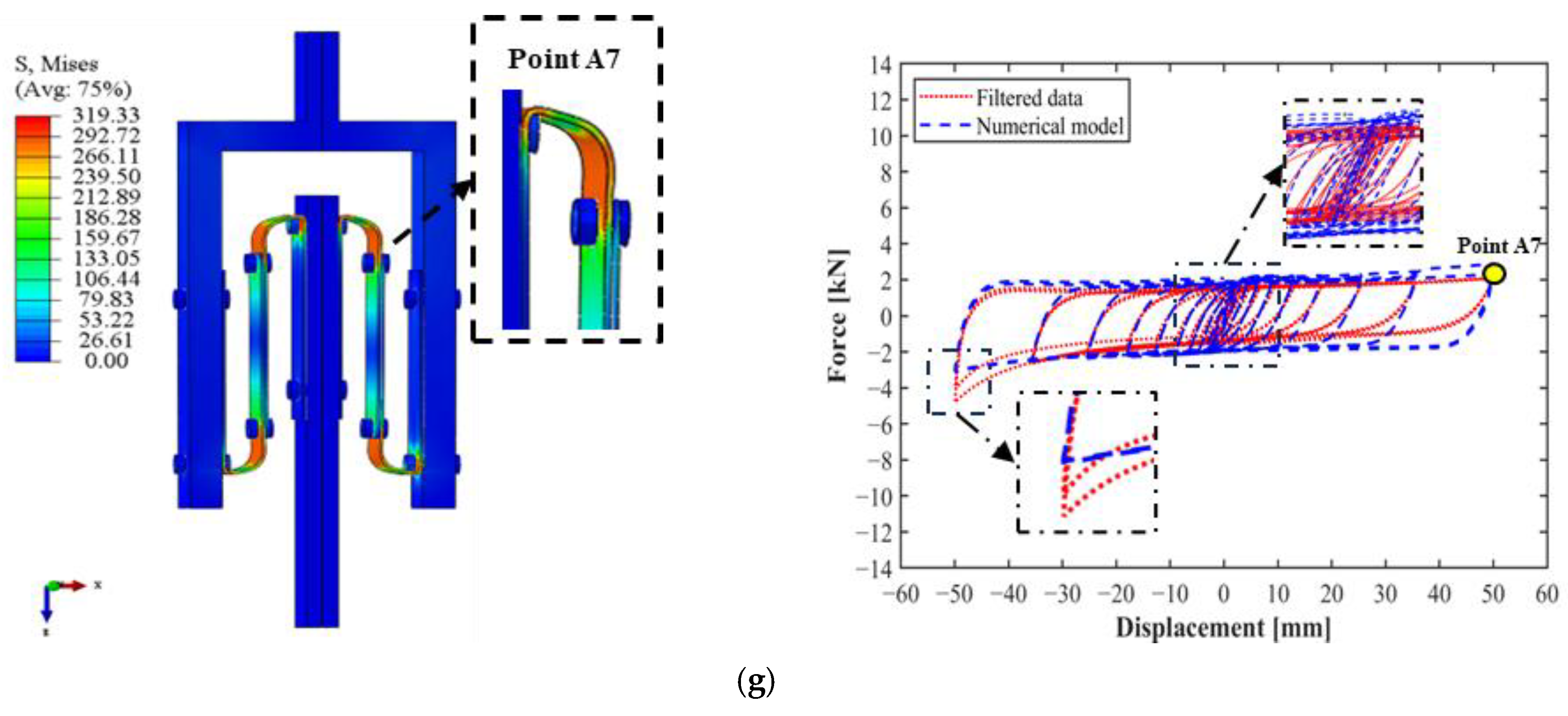
3.2. Results
4. Conclusions and Discussion
- The horizontal stiffness increases with the increase in the thickness and width of the steel dampers and the decrease in the distance between the bolt and the beginning of the arc of the S-shaped steel dampers.
- The damping ratio is not significantly affected by variations in the thickness and width parameters. However, the distance between the bolt and the arc has a notable impact on damping performance. A smaller distance increases the steel dampers’ sensitivity to stress, as the reduced spacing amplifies the stress distributions around the bolt holes and arc end regions. This enhanced sensitivity leads to a significant increase in the damping ratio.
- The FE model, with a mean average absolute error equal to 11.29% for the horizontal stiffness and 4.69% for the damping ratio, is able to capture the influence of the different geometrical properties on the final lateral behavior of the device. So, it can be used both for preliminary design and for FE structural analyses.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Soong, T.T.; Spencer, B.F. Supplemental Energy Dissipation: State-of-the-Art and State-of-the-Practice. Eng. Struct. 2002, 24, 243–259. [Google Scholar] [CrossRef]
- Esfahani, A.N.; Zareei, S.A.; Birzhandi, M.S.; Zafarani, M.M. Experimental and Numerical Study of the Centripetal Behavior of the Divergent Bracing Frame Equipped with Rotational Friction Damper and Shape Memory Bolts. Constr. Build. Mater. 2024, 438, 137245. [Google Scholar] [CrossRef]
- He, Z.; Shi, F.; Lin, Z.; Zhang, C.; Zhou, Y.; Zhao, F. Experimental Characterization on Cyclic Stability Behavior of a High-Damping Viscoelastic Damper. Constr. Build. Mater. 2023, 371, 130749. [Google Scholar] [CrossRef]
- Koutsoloukas, L.; Nikitas, N.; Aristidou, P. Passive, Semi-Active, Active and Hybrid Mass Dampers: A Literature Review with Associated Applications on Building-like Structures. Dev. Built Environ. 2022, 12, 100094. [Google Scholar] [CrossRef]
- Kelly, J.M.; Skinner, R.I.; Heine, A.J. Mechanisms of Energy Absorption in Special Devices for Use in Earthquake Resistant Structures. Bull. N. Z. Soc. Earthq. Eng. 1972, 5, 63–88. [Google Scholar] [CrossRef]
- Zeng, C.; Liu, L.; Hu, Y.; Zhao, W.; Xin, X.; Liu, Y.; Leng, J. Stair-Stepping Mechanical Metamaterials with Programmable Load Plateaus. Adv. Funct. Mater. 2024, 34, 2408887. [Google Scholar] [CrossRef]
- Chong, X.; Wu, Z.; Li, F. Vibration Isolation Properties of the Non-linear X-Combined Structure with a High-Static and Low-Dynamic Stiffness: Theory and Experiment. Mech. Syst. Signal Process. 2022, 179, 109352. [Google Scholar] [CrossRef]
- Nguyen, X.D.; Guizani, L. Analytical and Numerical Investigation of Natural Rubber Bearings Incorporating U-Shaped Dampers Behaviour for Seismic Isolation. Eng. Struct. 2021, 243, 112647. [Google Scholar] [CrossRef]
- Kishiki, S.; Zheng, H.; Ishida, T.; Tatsumi, N.; Watanabe, A. Inspection of U-Shaped Steel Dampers Based on Residual Plastic Deformation. Eng. Struct. 2021, 245, 112915. [Google Scholar] [CrossRef]
- Apostolidi, E.; Dritsos, S.; Giarlelis, C.; Jara, J.; Sutcu, F.; Takeuchi, T.; White, J. Seismic Isolation and Response Control; International Association for Bridge and Structural Engineering (IABSE): Zurich, Switzerland, 2021. [Google Scholar]
- Ene, D.; Yamada, S.; Jiao, Y.; Kishiki, S.; Konishi, Y. Reliability of U-Shaped Steel Dampers Used in Base-Isolated Structures Subjected to Biaxial Excitation. Earthq. Eng. Struct. Dyn. 2017, 46, 621–639. [Google Scholar] [CrossRef]
- Oh, S.H.; Song, S.H.; Lee, S.H.; Kim, H.J. Experimental Study of Seismic Performance of Base-Isolated Frames with U-Shaped Hysteretic Energy-Dissipating Devices. Eng. Struct. 2013, 56, 2014–2027. [Google Scholar] [CrossRef]
- Oh, S.H.; Song, S.H.; Lee, S.H.; Kim, H.J. Seismic Response of Base Isolating Systems with U-Shaped Hysteretic Dampers. Int. J. Steel Struct. 2012, 12, 285–298. [Google Scholar] [CrossRef]
- Song, G.; Ma, N.; Li, H.-N. Applications of Shape Memory Alloys in Civil Structures. Eng. Struct. 2006, 28, 1266–1274. [Google Scholar] [CrossRef]
- Deng, K.; Liang, H.; Yi, Y.; Zhao, C.; Dai, S.; Wu, D. Sliding U-Shaped Steel Damper for Multi-Directional Displacement. Int. J. Non-Linear Mech. 2023, 156, 104483. [Google Scholar] [CrossRef]
- Xie, X.; Chen, S.X.; Zhou, X. A Simplified Analytical Model for U-Shaped Steel Dampers Considering Horizontal Bidirectional Deformation. Bull. Earthq. Eng. 2018, 16, 6243–6268. [Google Scholar] [CrossRef]
- Qu, B.; Dai, C.; Qiu, J.; Hou, H.; Qiu, C. Testing of Seismic Dampers with Replaceable U-Shaped Steel Plates. Eng. Struct. 2019, 179, 625–639. [Google Scholar] [CrossRef]
- Hui, Y.X.; Li, L.S.; Cheng, H.; Zhang, Y.J.; Wang, D.S. Seismic Mitigation of Continuous Girder Bridges Equipped with U-Shaped Stainless Steel Dampers under near-Fault Earthquake Excitations. Structures 2023, 58, 105597. [Google Scholar] [CrossRef]
- Abebe, D.Y.; Kim, J.W.; Gwak, G.; Choi, J.H. Low-Cycled Hysteresis Characteristics of Circular Hollow Steel Damper Subjected to Inelastic Behavior. Int. J. Steel Struct. 2019, 19, 157–167. [Google Scholar] [CrossRef]
- Zhai, Z.; Guo, W.; Yu, Z.; He, C.; Zeng, Z. Experimental and Numerical Study of S-Shaped Steel Plate Damper for Seismic Resilient Application. Eng. Struct. 2020, 221, 111006. [Google Scholar] [CrossRef]
- Abebe, D.Y.; Jeong, S.J.; Getahune, B.M.; Segu, D.Z.; Choi, J.H. Hysteretic Characteristics of Shear Panel Damper Made of Low Yield Point Steel. Mater. Res. Innov. 2015, 19, S5-902–S5-910. [Google Scholar] [CrossRef]
- Choi, J.; Abebe, D.Y. Hysteresis Characteristics of Shear Panel Damper Using SLY120. APCBEE Procedia 2014, 9, 370–375. [Google Scholar] [CrossRef]
- Suzuki, T.; Motomura, S.; Kinoshita, T.; Inoue, Y.; Kushibe, A.; Iida, T. Shape Optimization of Hourglass-Shaped Damper Using Fe-Mn-Si-Based Alloy Considering Target Load-Displacement Relationship and Target Fatigue Characteristics. Constr. Build. Mater. 2023, 366, 130091. [Google Scholar] [CrossRef]
- Lee, C.H.; Ju, Y.K.; Min, J.K.; Lho, S.H.; Kim, S.D. Non-Uniform Steel Strip Dampers Subjected to Cyclic Loadings. Eng. Struct. 2015, 99, 192–204. [Google Scholar] [CrossRef]
- Guo, W.; Wang, X.; Yu, Y.; Chen, X.; Li, S.; Fang, W.; Zeng, C.; Wang, Y.; Bu, D. Experimental Study of a Steel Damper with X-Shaped Welded Pipe Halves. J. Constr. Steel Res. 2020, 170, 106087. [Google Scholar] [CrossRef]
- Guo, W.; Chen, X.; Yu, Y.; Bu, D.; Li, S.; Fang, W.; Wang, X.; Zeng, C.; Wang, Y. Development and Seismic Performance of Bolted Steel Dampers with X-Shaped Pipe Halves. Eng. Struct. 2021, 239, 112327. [Google Scholar] [CrossRef]
- Kato, S.; Kim, Y.B.; Nakazawa, S.; Ohya, T. Simulation of the Cyclic Behavior of J-Shaped Steel Hysteresis Devices and Study on the Efficiency for Reducing Earthquake Responses of Space Structures. J. Constr. Steel Res. 2005, 61, 1457–1473. [Google Scholar] [CrossRef]
- Kato, S.; Kim, Y.B. A Finite Element Parametric Study on the Mechanical Properties of J-Shaped Steel Hysteresis Devices. J. Constr. Steel Res. 2006, 62, 802–811. [Google Scholar] [CrossRef]
- Gao, J.; Xi, J.; Xu, Y.; Ding, J.; Zhu, J.; Chang, Y.; Chen, B. Analysis of the Mechanical Properties and Parameter Sensitivity of a U-Shaped Steel Damper. Front. Mater. 2021, 8, 713221. [Google Scholar] [CrossRef]
- Satria, E.; Son, L.; Bur, M.; Akbar, M.D. Finite Element Analysis to Determine Stiffness, Strength, and Energy Dissipation of U-Shaped Steel Damper under Quasi-Static Loading. Int. J. Automot. Mech. Eng. 2021, 18, 9042–9050. [Google Scholar] [CrossRef]
- Pianese, G.; van Engelen, N.; Toopchi-Nezhad, H.; Milani, G. High-Damping Fiber-Reinforced Elastomeric Seismic Isolator in Different Boundary Conditions: An Experimental Insight. Eng. Struct. 2024, 300, 117199. [Google Scholar] [CrossRef]
- Pianese, G.; van Engelen, N.; Tait, M.; Milani, G. Shake Table Tests on a Rigid Block Isolated with High-Damping Unbonded Fiber-Reinforced Elastomeric Isolators. Structures 2024, 64, 106595. [Google Scholar] [CrossRef]
- Sheikhi, J.; Fathi, M.; Rahnavard, R. Natural Rubber Bearing Incorporated with High Toughness Steel Ring Dampers. Structures 2020, 24, 107–123. [Google Scholar] [CrossRef]
- Sheikhi, J.; Fathi, M.; Rahnavard, R.; Napolitano, R. Numerical Analysis of Natural Rubber Bearing Equipped with Steel and Shape Memory Alloys Dampers. Structures 2021, 32, 1839–1855. [Google Scholar] [CrossRef]
- Deng, K.; Pan, P.; Su, Y.; Xue, Y. Shape Optimization of U-Shaped Damper for Improving Its Bi-Directional Performance under Cyclic Loading. Eng. Struct. 2015, 93, 27–35. [Google Scholar] [CrossRef]
- ISO 6892-1; Metallic Materials-Tensile Testing-Part 1: Method of Test at Room Temperature. International Organization for Standardization: Geneva, Switzerland, 2009.
- U.S. Department of Homeland Security; Federal Emergency Management Agency. Interim Testing Protocols for Determining the Seismic Performance Characteristics of Structural and Nonstructural Components: FEMA 461/June 2007; Createspace Independent Publishing Platform: Scotts Valley, CA, USA, 2013; ISBN 978-1-4840-1948-1.
- Applied Technology Council. NEHRP Guidelines for the Seismic Rehabilitation of Buildings: FEMA 273; Federal Emergency Management Agency: Washington, DC, USA, 1997.
- Smith, M. ABAQUS/Standard User’s Manual, Version 6.14; Dassault Systèmes: Waltham, MA, USA, 2014. [Google Scholar]
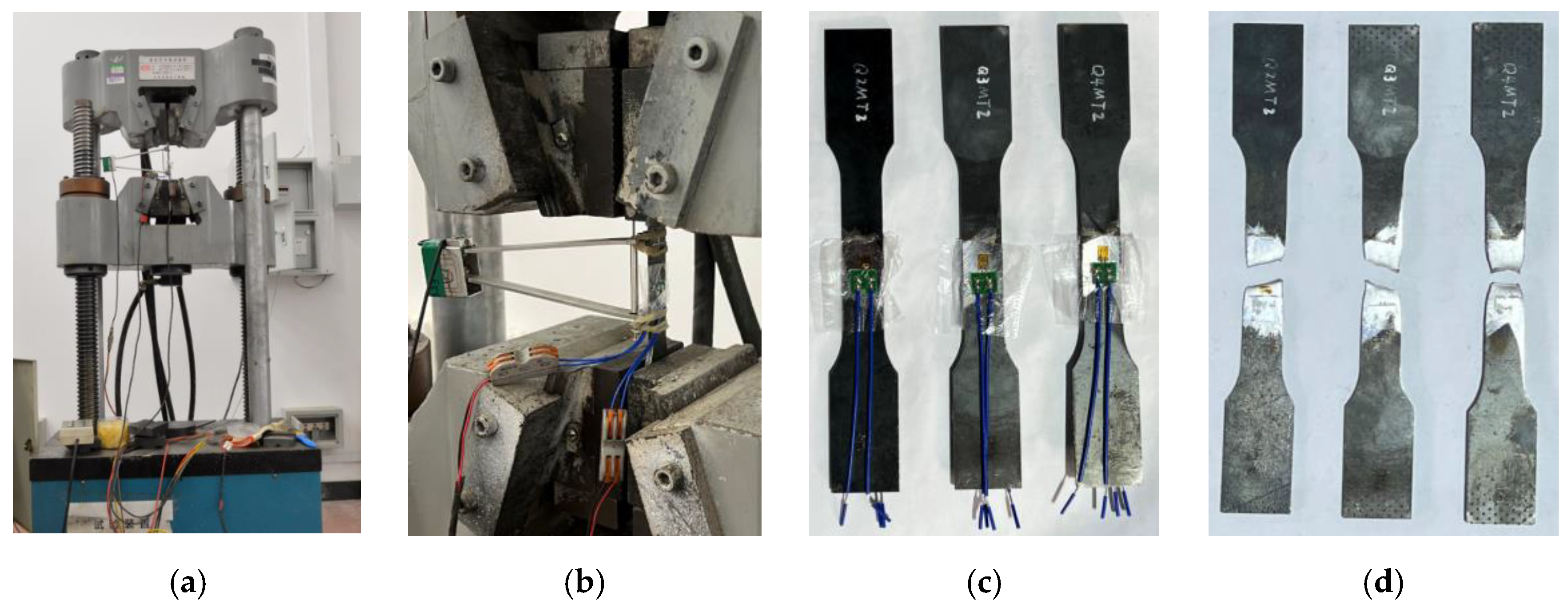

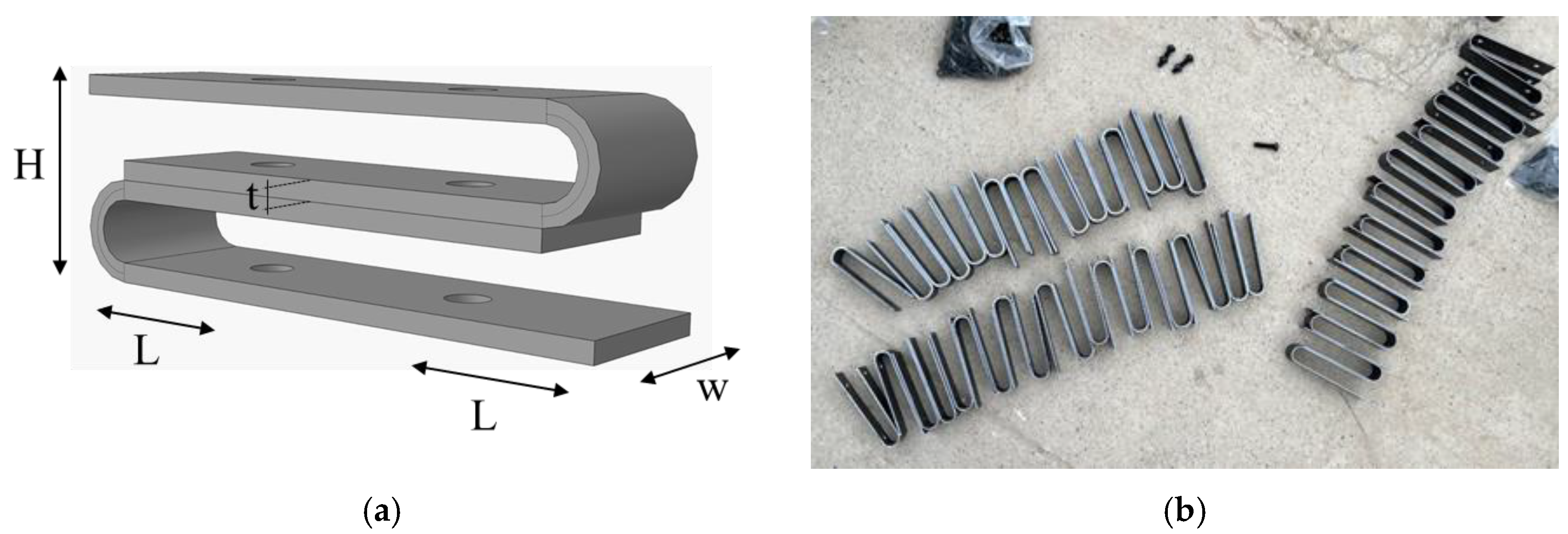
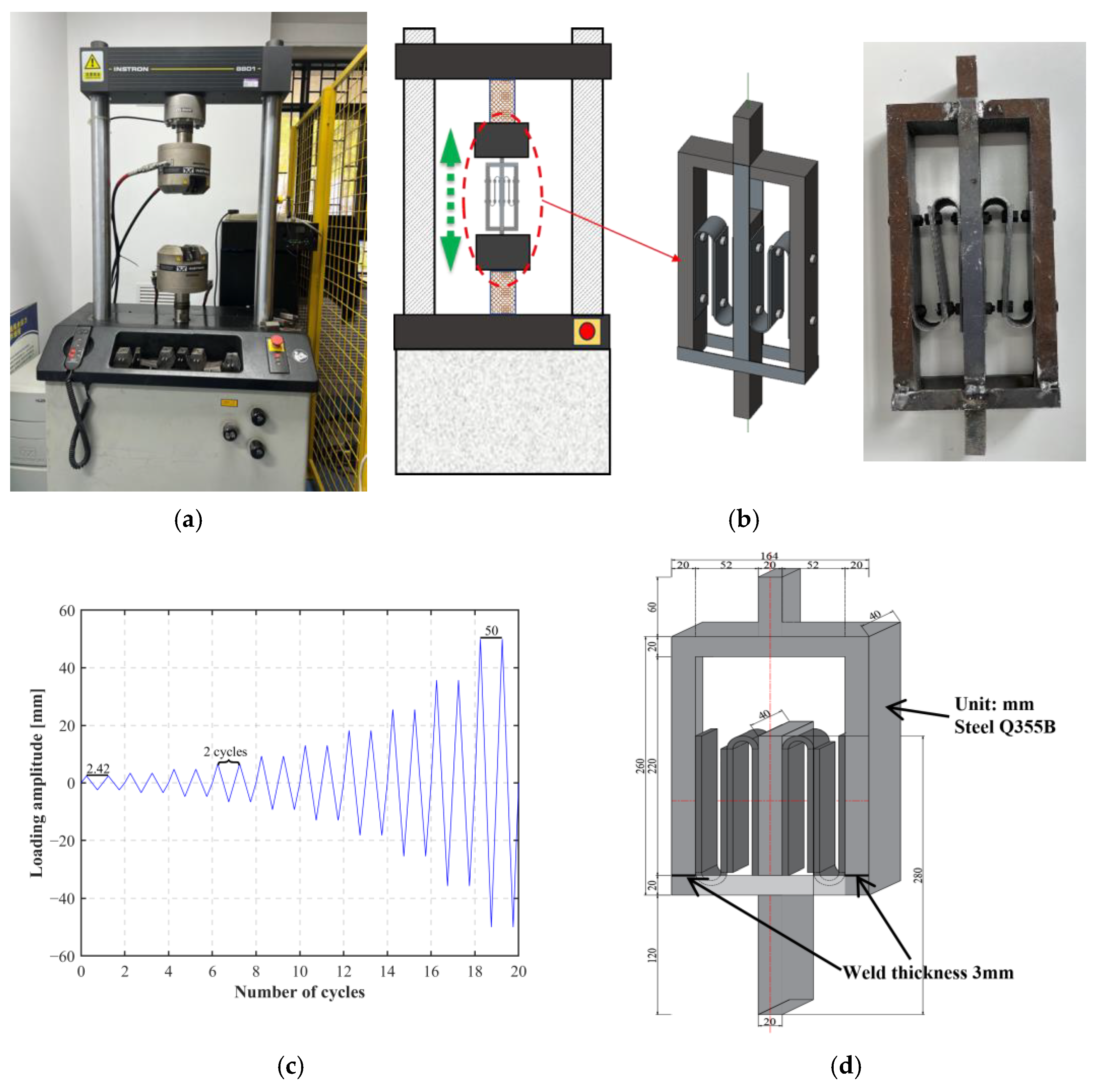

| Specimen | Young’s Modulus E (GPa) | (MPa) | (MPa) | (%) |
|---|---|---|---|---|
| 1 | 185.34 | 278.35 | 413.74 | 0.16 |
| 2 | 187.51 | 283.39 | 451.54 | 0.14 |
| 3 | 195.82 | 257.81 | 417.45 | 0.17 |
| Average | 189.56 | 273.18 | 427.58 | 0.16 |
| Specimen | (mm) | (mm) | (mm) | (mm) |
|---|---|---|---|---|
| S1 | 52 | 2 | 40 | 10.5 |
| S2 | 3 | 40 | 10.5 | |
| S3 | 4 | 40 | 10.5 | |
| S4 | 2 | 35 | 10.5 | |
| S5 | 2 | 30 | 10.5 | |
| S6 | 2 | 40 | 14.5 | |
| S7 | 2 | 40 | 6.5 |
| Specimen | Filtered Experimental Data | |
|---|---|---|
| (N/mm) | ||
| S1 | 47.06 | 0.39 |
| S2 | 157.24 | 0.38 |
| S3 | 289.88 | 0.40 |
| S4 | 39.31 | 0.40 |
| S5 | 33.81 | 0.41 |
| S6 | 33.43 | 0.41 |
| S7 | 69.03 | 0.44 |
| Specimen | Numerical Results | Error with Experimental Results | ||
|---|---|---|---|---|
| (N/mm) | (N/mm) | |||
| S1 | 50.74 | 0.42 | 7.82 | 7.44 |
| S2 | 145.12 | 0.43 | −7.71 | 13.69 |
| S3 | 253.19 | 0.42 | −12.66 | 3.55 |
| S4 | 43.61 | 0.42 | 10.94 | 3.99 |
| S5 | 36.47 | 0.42 | 11.27 | 2.33 |
| S6 | 38.09 | 0.41 | 13.94 | −0.87 |
| S7 | 58.87 | 0.44 | −14.72 | 0.98 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, K.; Pianese, G.; Pan, P.; Milani, G. Influence of Geometrical Features on the Cyclic Behavior of S-Shaped Steel Dampers Used in Sustainable Seismic Isolation: Experimental Insight with Numerical Validation. Sustainability 2025, 17, 660. https://doi.org/10.3390/su17020660
Guo K, Pianese G, Pan P, Milani G. Influence of Geometrical Features on the Cyclic Behavior of S-Shaped Steel Dampers Used in Sustainable Seismic Isolation: Experimental Insight with Numerical Validation. Sustainability. 2025; 17(2):660. https://doi.org/10.3390/su17020660
Chicago/Turabian StyleGuo, Kai, Gaetano Pianese, Peng Pan, and Gabriele Milani. 2025. "Influence of Geometrical Features on the Cyclic Behavior of S-Shaped Steel Dampers Used in Sustainable Seismic Isolation: Experimental Insight with Numerical Validation" Sustainability 17, no. 2: 660. https://doi.org/10.3390/su17020660
APA StyleGuo, K., Pianese, G., Pan, P., & Milani, G. (2025). Influence of Geometrical Features on the Cyclic Behavior of S-Shaped Steel Dampers Used in Sustainable Seismic Isolation: Experimental Insight with Numerical Validation. Sustainability, 17(2), 660. https://doi.org/10.3390/su17020660








