Experimental Investigation of Wind Effect on Roof Configurations with Photovoltaic Panel Systems for Sustainable Building Design
Abstract
1. Introduction
2. Theoretical Background and Basis for Calculations
2.1. Fundamental Equations for Wind Pressure Analysis
2.2. Aerodynamic Principles of Wind-Structure Interaction
2.3. Effect of Roof Geometry on Wind Pressure Distribution
2.4. Empirical Models and Experimental Data
2.5. Implications for Structural Design
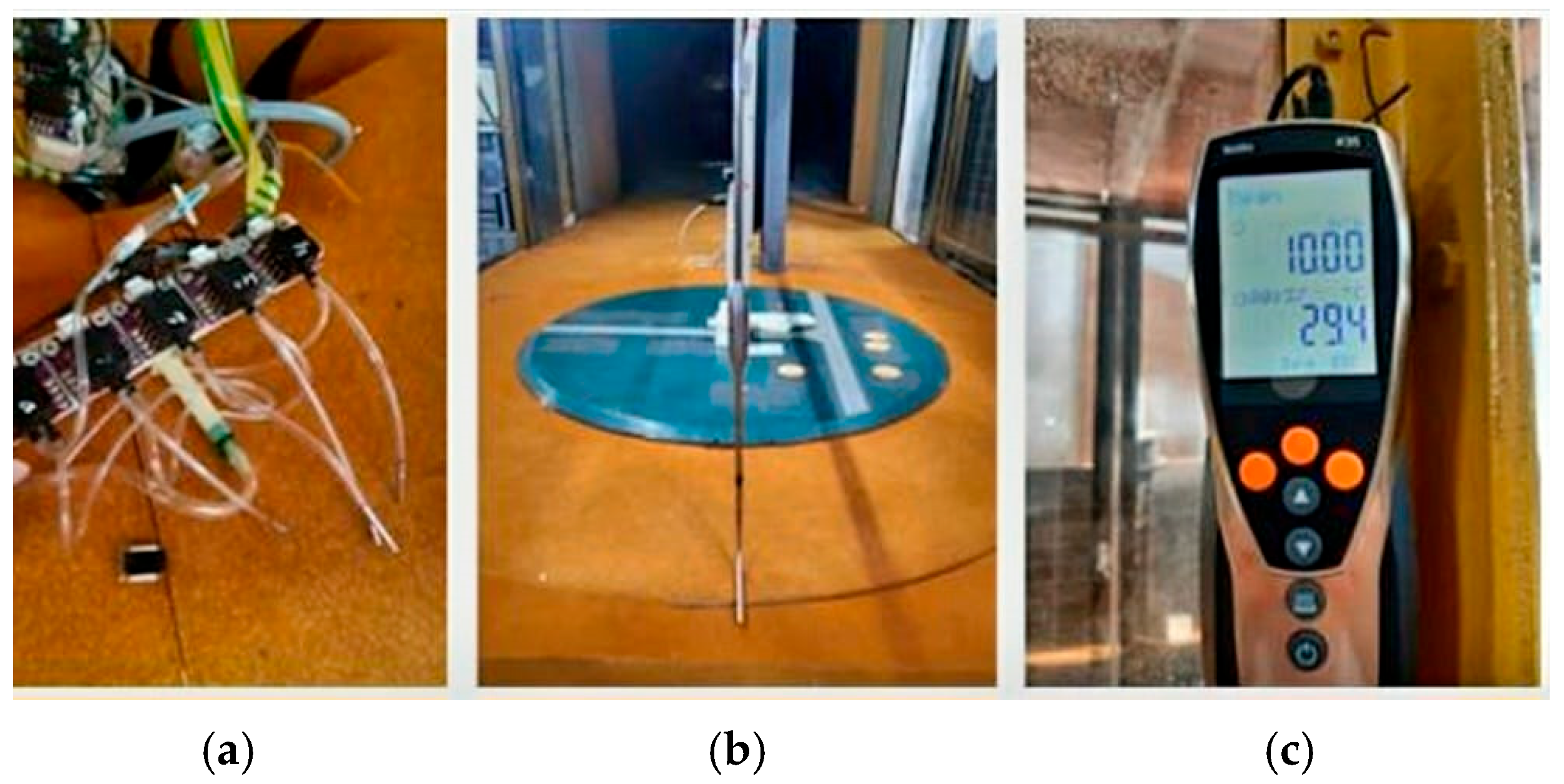
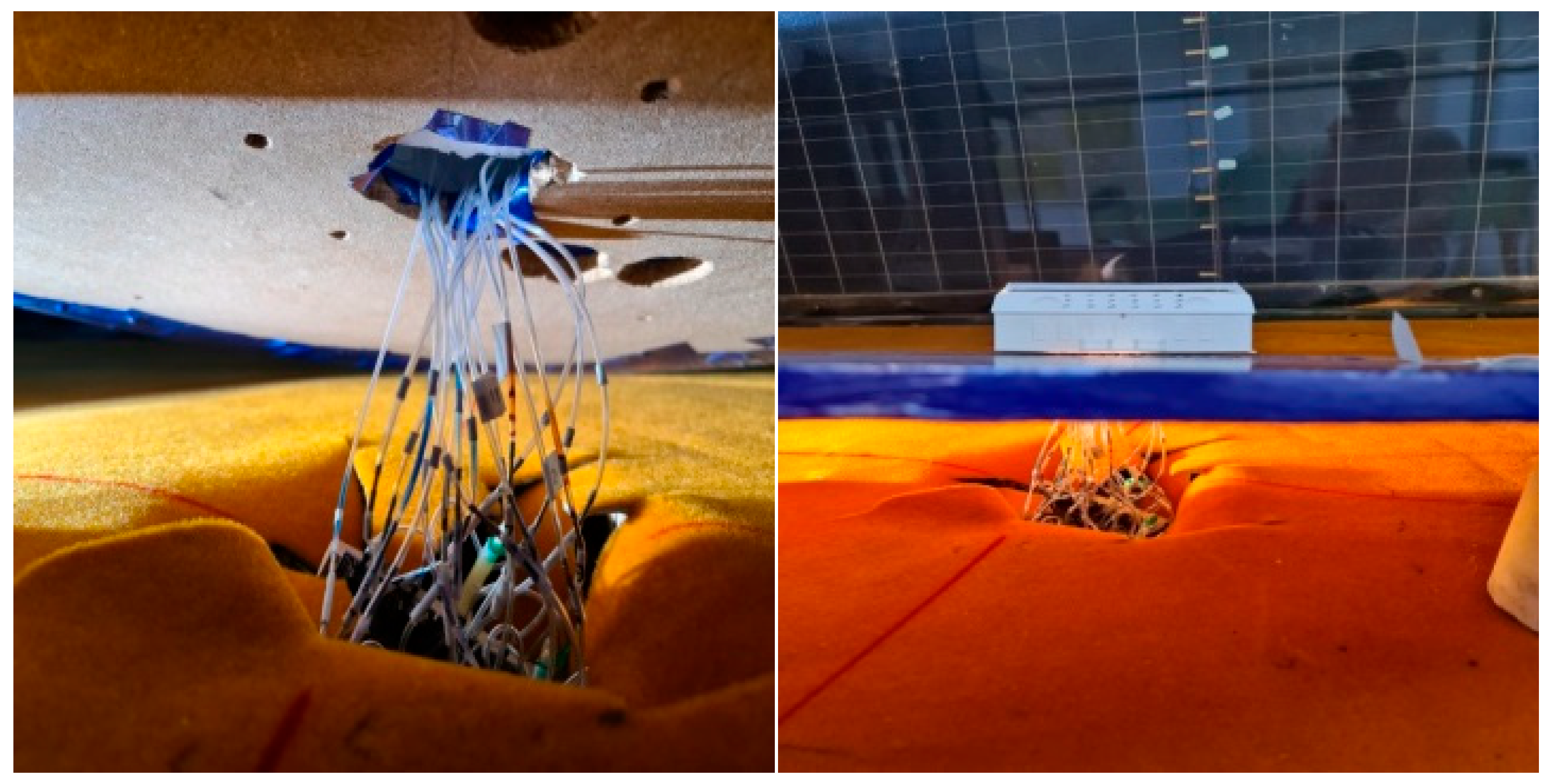
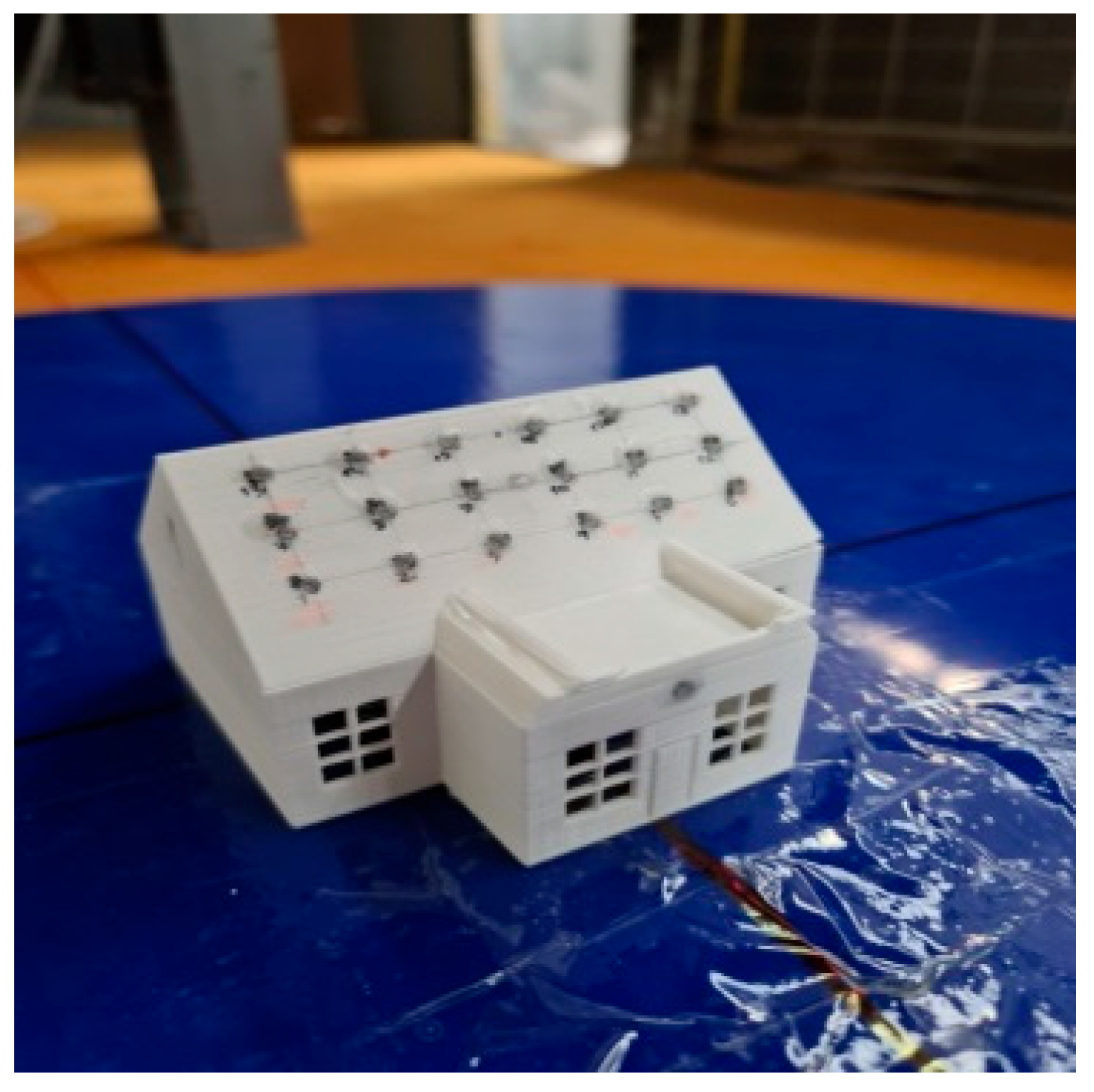
3. Experimental Setup and Measurement Methodology
3.1. Local Pressure Measurement Protocol
3.2. Data Acquisition and Processing Procedure
3.3. Scaling and Dimensional Analysis
4. Results and Discussion: Aerodynamic Behavior of PV-Equipped Roof Configurations
4.1. Research Objectives
4.2. Experimental Program Overview
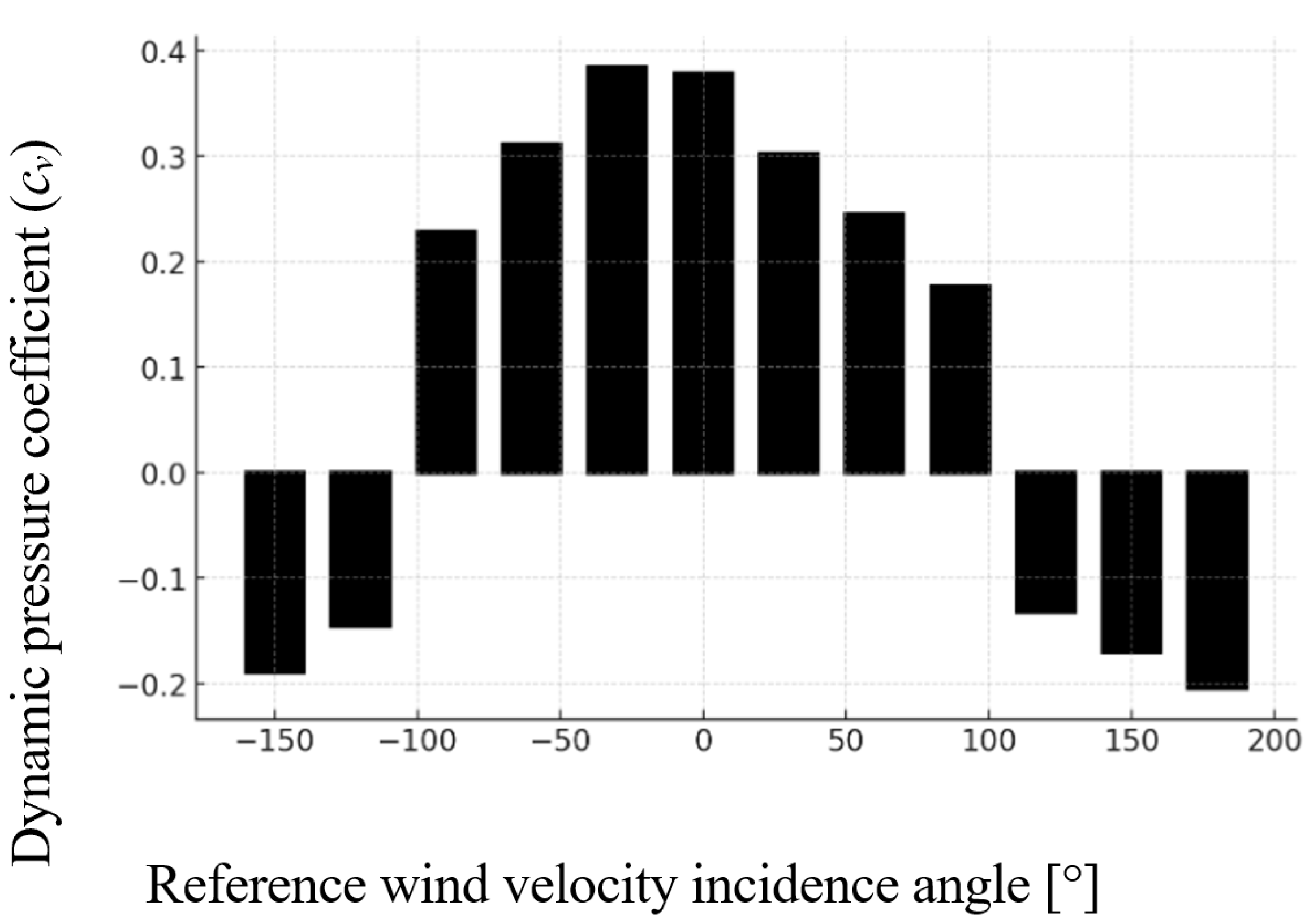
4.3. Results: Gable Roof (Model M1)
4.4. Results: Hip Roof (Model M2)
4.5. Results: Multi-Section Roof (Model M3)
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Polcovnicu, R.A.; Ţăranu, N.; Ungureanu, D.; Sbîrlea, C.; Cozmanciuc, R. Building Integrated Photovoltaics Systems: State-of-the-Art Review. Bull. Inst. Politeh. Iași. Archit. Sect. 2021, 67, 71. [Google Scholar] [CrossRef]
- Polcovnicu, R.A.; Ţăranu, N.; Ungureanu, D.; Zghibarcea, Ș.V.; Hudișteanu, V.S. Modern Manufacturing Technology for Modular Photovoltaic Panels: State-of-the-Art and Future Trends. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2020; p. 1182. [Google Scholar]
- Eslami Majd, A.; Adebayo, D.S.; Tchuenbou-Magaia, F.; Willetts, J.; Nwosu, D.; Matthews, Z.; Ekere, N.N. Wind Flow and Its Interaction with a Mobile Solar PV System Mounted on a Trailer. Sustainability 2024, 16, 2038. [Google Scholar] [CrossRef]
- Jackson, A.; Donaldson, G.; Gómez-Amo, J. Impact of Extreme Weather Conditions on the Performance of Utility-Scale PV Systems. Sustainability 2023, 15, 731. [Google Scholar]
- Tu, Z.; Zheng, G.; Yao, J.; Shen, G.; Lou, W. Computational Investigation of Wind Loads on Tilted Roof-Mounted Solar Array. Sustainability 2022, 14, 15653. [Google Scholar] [CrossRef]
- Kopp, G.A.; Morrison, M.J. Wind Effects on Rooftop-Mounted Solar Photovoltaic Systems. J. Struct. Eng. 2018, 144, 04018001. [Google Scholar]
- Khadimallah, M.A.; Abdelbaky, M.; Bouafia, Y. Numerical Modeling of Wind Loads on Solar PV Arrays in Built Environments. J. Wind Eng. Ind. Aerodyn. 2024, 200, 104985. [Google Scholar]
- Ke, Y.; Shen, G.; Yang, X.; Xie, J. Effects of Surface-Attached Vertical Ribs on Wind Loads and Wind-Induced Responses of High-Rise Buildings. Sustainability 2022, 14, 11394. [Google Scholar] [CrossRef]
- Aly, A.M.; Rone, E. Wind Loads on a Low-Rise Gable Roof with and without Solar Panels and Comparison to Design Standards. Sustain. Resil. Infrastruct. 2024, 9, 589–609. [Google Scholar] [CrossRef]
- Hudisteanu, S.V.; Popovici, C.G. Experimental investigation of the wind direction influence on the cooling of photovoltaic panels integrated in double skin facades. E3S Web Conf. 2019, 111, 03045. [Google Scholar] [CrossRef]
- Wang, L.; Shi, F.; Wang, Z.; Liang, S. Blockage Effects in Wind Tunnel Tests for Tall Buildings with Surrounding Buildings. Appl. Sci. 2022, 12, 7087. [Google Scholar] [CrossRef]
- Peng, H.Y.; Liang, H.; Dai, S.F.; Liu, H.J. Wind load analysis for rooftop solar photovoltaic panels in the presence of building interference: A wind tunnel study. J. Build. Eng. 2025, 100, 111702. [Google Scholar] [CrossRef]
- Hudișteanu, S.V.; Ţurcanu, F.E.; Cherecheș, N.-C.; Popovici, C.-G.; Verdeș, M.; Ancaș, D.-A.; Hudișteanu, I. Effect of Wind Direction and Velocity on PV Panels Cooling with Perforated Heat Sinks. Appl. Sci. 2022, 12, 9665. [Google Scholar] [CrossRef]
- EN 1991-1-4:2005+A1:2010; Eurocode 1: Actions on Structures—Part 1–4: General Actions—Wind Actions. CEN: Brussels, Belgium, 2010.
- Zhang, J.; Lou, Y. Study of Wind Load Influencing Factors of Flexibly Supported Photovoltaic Panels. Buildings 2024, 14, 1677. [Google Scholar] [CrossRef]
- Geurts, C.; van Bentum, C. Wind Loading on Buildings: Eurocode and Experimental Approach; CISM International Centre for Mechanical Sciences; Springer: Vienna, Austria, 2007; Volume 493. [Google Scholar] [CrossRef]
- Yao, J.; Tu, Z.; Wang, D.; Shen, G.; Lou, W. Experimental Investigation of Wind Loads on Roof-Mounted Solar Arrays. Sustainability 2022, 14, 8477. [Google Scholar] [CrossRef]
- Wang, W.; Zhu, Y.; Shu, Z.; Li, Y. A Review on Aerodynamic Characteristics and Wind-Induced Response of Flexible Support Photovoltaic System. Atmosphere 2023, 14, 731. [Google Scholar] [CrossRef]
- Nezamisavojbolaghi, M.; Davodian, E.; Bouich, A.; Tlemçani, M.; Mesbahi, O.; Janeiro, F.M. The Impact of Dust Deposition on PV Panels’ Efficiency and Mitigation Solutions: Review Article. Energies 2023, 16, 8022. [Google Scholar] [CrossRef]
- Wang, J.; Liu, M.; Yang, Q.; Hui, Y.; Nie, S. Extreme Wind Loading on Flat-Roof-Mounted Solar Arrays with Consideration of Wind Directionality. Buildings 2024, 14, 221. [Google Scholar] [CrossRef]
- Hu, J.; Wang, X.; Yang, H.; Huang, B. Experimental Study on Rooftop Flow Field of Building Based on the Operation of Vertical-Axis Wind Turbines. J. Renew. Sustain. Energy 2024, 16, 053301. [Google Scholar] [CrossRef]
- Shen, G.; Yu, S.; Lou, W. Experimental Investigation of the Parapet Effect on the Wind Load of Roof-Mounted Solar Arrays. Sustainability 2023, 15, 5052. [Google Scholar] [CrossRef]
- Kopp, G.A.; Banks, D. Use of Wind Tunnel Measurements in Wind Load Design of Solar Panels. J. Wind Eng. Ind. Aerodyn. 2013, 123, 232–240. [Google Scholar]
- Li, S.; Xiao, F.; Li, S.; Hui, Y.; Yang, Q.; Li, B.; Chen, Y.; Zhou, S.; Liu, M. Wind Loads on Solar Panels Mounted on Facade of High-Rise Residential Building. Int. J. Struct. Stab. Dyn. 2024, 24, 2240019. [Google Scholar] [CrossRef]
- ASME. Numerical Simulation of Wind Load on Roof-Mounted Solar Panels. In Proceedings of the ASME International Conference, Boston, MA, USA, 30 June–2 July 2014. [Google Scholar]
- ICRAES-2020. Wind Pressure Distribution on PV Panels Mounted on Saw-Type Roofs. In Proceedings of the International Conference on Recent Advances in Engineering & Science, New York, NY, USA, 17 November 2020. [Google Scholar]
- ASCE/SEI 7-22; Minimum Design Loads and Associated Criteria for Buildings and Other Structures. American Society of Civil Engineers: Reston, VA, USA, 2022.
- Bendat, J.S.; Piersol, A.G. Random Data: Analysis and Measurement Procedures, 4th ed.; Wiley: Hoboken, NJ, USA, 2010. [Google Scholar]
- Spanelis, A.; Georgiou, D. Assessment of Aerodynamic Wake Effects on PV-Mounted Rooftops. Resources 2023, 12, 90. [Google Scholar]



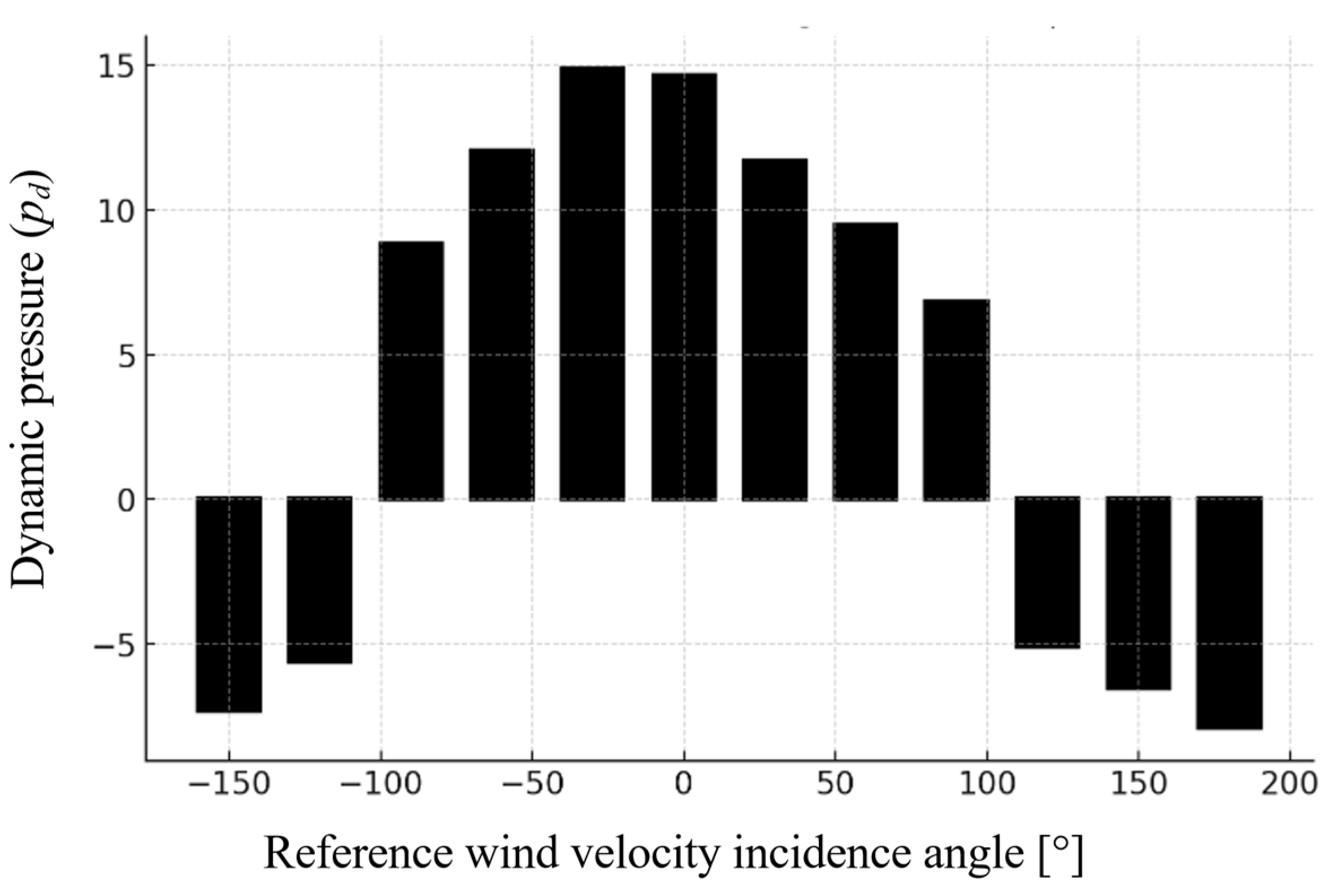


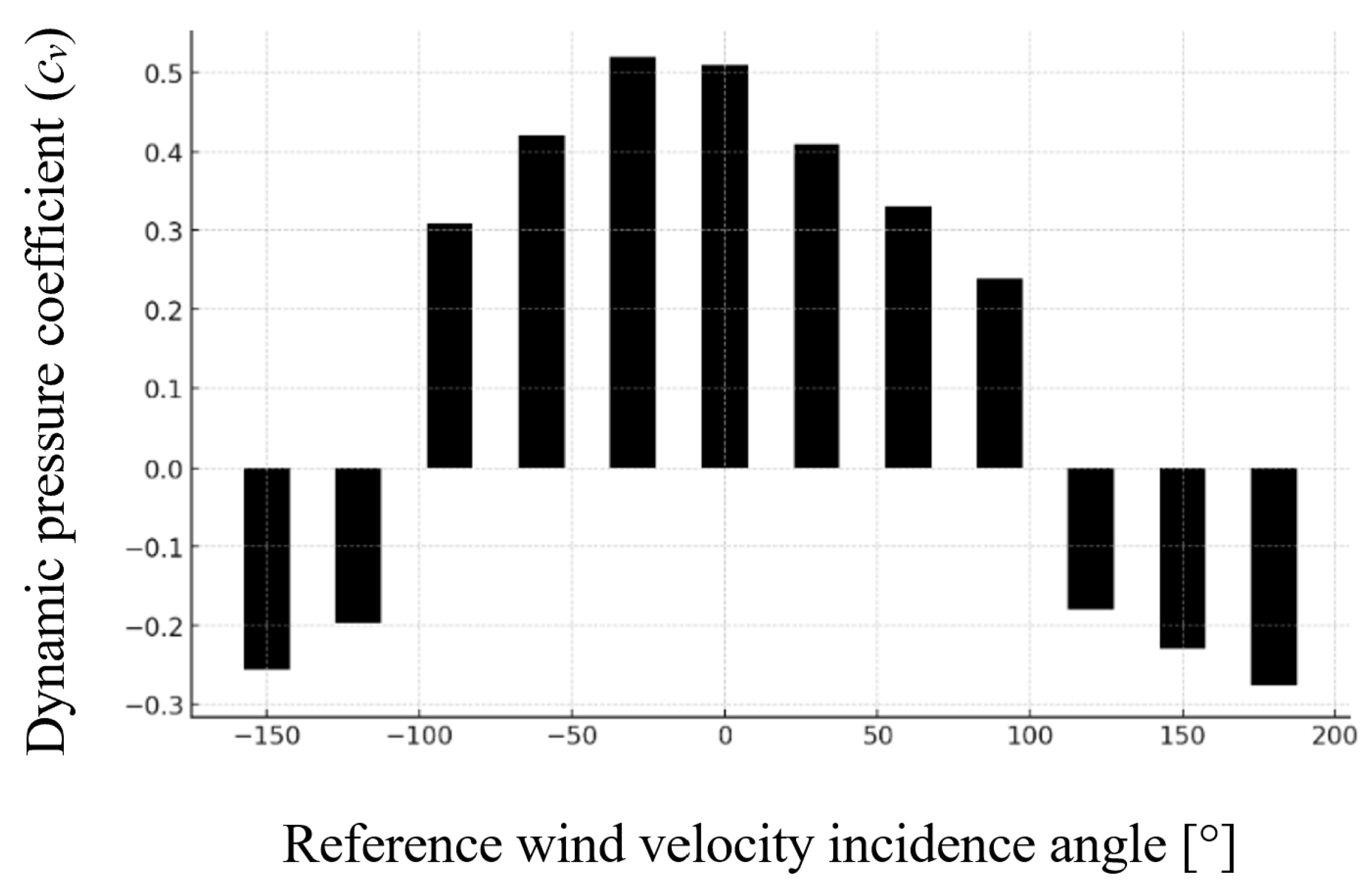

| Parameter | Description |
|---|---|
| Test facility | Wind tunnel with turbulent boundary layer, Technical University of Iași |
| Scale of physical models | 1:100 |
| Roof types tested | M1—Gable, M2—Hip, M3—Multi-section |
| Number of sensors per model | 18 pressure sensors + 1 reference sensor |
| Wind incidence angles | 0°, ±30°, ±60°, ±90°, ±120°, ±150°, 180° (12 angles total) |
| Reference wind speed | 7.95 m/s |
| Duration per test | 30 s |
| Number of samples per test | 30,000 |
| Total number of tests | 36 (12 per model) |
| Data acquisition frequency | 1000 Hz |
| Software used | LABVIEW and custom processing scripts |
| Reference Wind Speed (vref) [m/s] | Angle of Incidence of Reference Wind Speed [°] | Average Dynamic Pressure Coefficient (cv) | Dynamic Pressure (pd) [Pa] |
|---|---|---|---|
| 7.95 | 0° | 0.378 | 14.63 |
| 30° | 0.302 | 11.69 | |
| 60° | 0.245 | 9.48 | |
| 90° | 0.177 | 6.85 | |
| 120° | −0.132 | −5.11 | |
| 150° | −0.169 | −6.54 | |
| 180° | −0.204 | −7.89 | |
| −30° | 0.384 | 14.86 | |
| −60° | 0.311 | 12.04 | |
| −90° | 0.228 | 8.82 | |
| −120° | −0.145 | −5.61 | |
| −150° | −0.189 | −7.32 |
| Reference Wind Speed (vref) [m/s] | Angle of Incidence of Reference Wind Speed [°] | Average Dynamic Pressure Coefficient (cv) | Dynamic Pressure (pd) [Pa] |
|---|---|---|---|
| 7.95 | 0° | 0.555 | 21,536 |
| 30° | 0.444 | 17,190 | |
| 60° | 0.360 | 13,926 | |
| 90° | 0.260 | 10,055 | |
| 120° | −0.194 | −7511 | |
| 150° | −0.248 | −9617 | |
| 180° | −0.300 | −11,611 | |
| −30° | 0.564 | 21,827 | |
| −60° | 0.457 | 17,095 | |
| −90° | 0.335 | 12,964 | |
| −120° | −0.213 | −8252 | |
| −150° | −0.278 | −10,752 |
| Reference Wind Speed (vref) [m/s] | Angle of Incidence of Reference Wind Speed [°] | Average Dynamic Pressure Coefficient (cv) | Dynamic Pressure (pd) [Pa] |
|---|---|---|---|
| 7.95 | 0° | 0.510 | 19,809 |
| 30° | 0.409 | 15,830 | |
| 60° | 0.331 | 12,822 | |
| 90° | 0.239 | 9257 | |
| 120° | −0.179 | −6910 | |
| 150° | −0.229 | −8859 | |
| 180° | −0.276 | −10,682 | |
| −30° | 0.520 | 20,094 | |
| −60° | 0.421 | 15,730 | |
| −90° | 0.309 | 11,933 | |
| −120° | −0.196 | −7593 | |
| −150° | −0.256 | −9892 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Polcovnicu, R.-A.; Hudișteanu, S.-V.; Ţăranu, N.; Ungureanu, D.; Alexa, M.; Hudișteanu, I.; Onuțu, C.; Mustiață, A.-F. Experimental Investigation of Wind Effect on Roof Configurations with Photovoltaic Panel Systems for Sustainable Building Design. Sustainability 2025, 17, 4739. https://doi.org/10.3390/su17104739
Polcovnicu R-A, Hudișteanu S-V, Ţăranu N, Ungureanu D, Alexa M, Hudișteanu I, Onuțu C, Mustiață A-F. Experimental Investigation of Wind Effect on Roof Configurations with Photovoltaic Panel Systems for Sustainable Building Design. Sustainability. 2025; 17(10):4739. https://doi.org/10.3390/su17104739
Chicago/Turabian StylePolcovnicu, Răzvan-Andrei, Sebastian-Valeriu Hudișteanu, Nicolae Ţăranu, Dragoș Ungureanu, Marius Alexa, Iuliana Hudișteanu, Cătălin Onuțu, and Alexandru-Florin Mustiață. 2025. "Experimental Investigation of Wind Effect on Roof Configurations with Photovoltaic Panel Systems for Sustainable Building Design" Sustainability 17, no. 10: 4739. https://doi.org/10.3390/su17104739
APA StylePolcovnicu, R.-A., Hudișteanu, S.-V., Ţăranu, N., Ungureanu, D., Alexa, M., Hudișteanu, I., Onuțu, C., & Mustiață, A.-F. (2025). Experimental Investigation of Wind Effect on Roof Configurations with Photovoltaic Panel Systems for Sustainable Building Design. Sustainability, 17(10), 4739. https://doi.org/10.3390/su17104739








