Modelling Geothermal Energy Extraction from Low-Enthalpy Oil and Gas Fields Using Pump-Assisted Production: A Case Study of the Waihapa Oilfield
Abstract
1. Introduction
- How well can the flow from a petroleum system be predicted using a geothermal simulator?
- How important are the heat transfer effects during modelling energy extraction from oil and gas resources?
- How do you assess optimum extraction rates from a petroleum producer?
2. Methodology
- The goal is to create a history-matched model that provides reference values for key system parameters, i.e., wellbore deliverability curve, reservoir size, porosities and permeabilities of the reservoir, and distance between the wellbores. The history-matched model is essential for calibration and validation purposes. It begins with user input of wellhead pressure, mass flow rate, and dimensions of the wellbore obtained from well logs.
- Next, the wellbore model is used to estimate productivity index and construct a deliverability curve. In cases where some wellbore data are unavailable, an alternate strategy is adopted to address this issue.
- The wellbore model is then imported into Brynhild component of the Volsung package to integrate with the reservoir model. The reservoir model has stratigraphic information, which incorporates lithology information obtained from petroleum reports for the oilfield. This integration allows for a more realistic representation of the system.
- To complete the integrated model, an empirical heat-to-power conversion model is included, assuming a constant reinjection temperature.
2.1. Modelling an Oil and Gas System Using a Geothermal Tool
2.2. Heat-to-Power Conversion Model
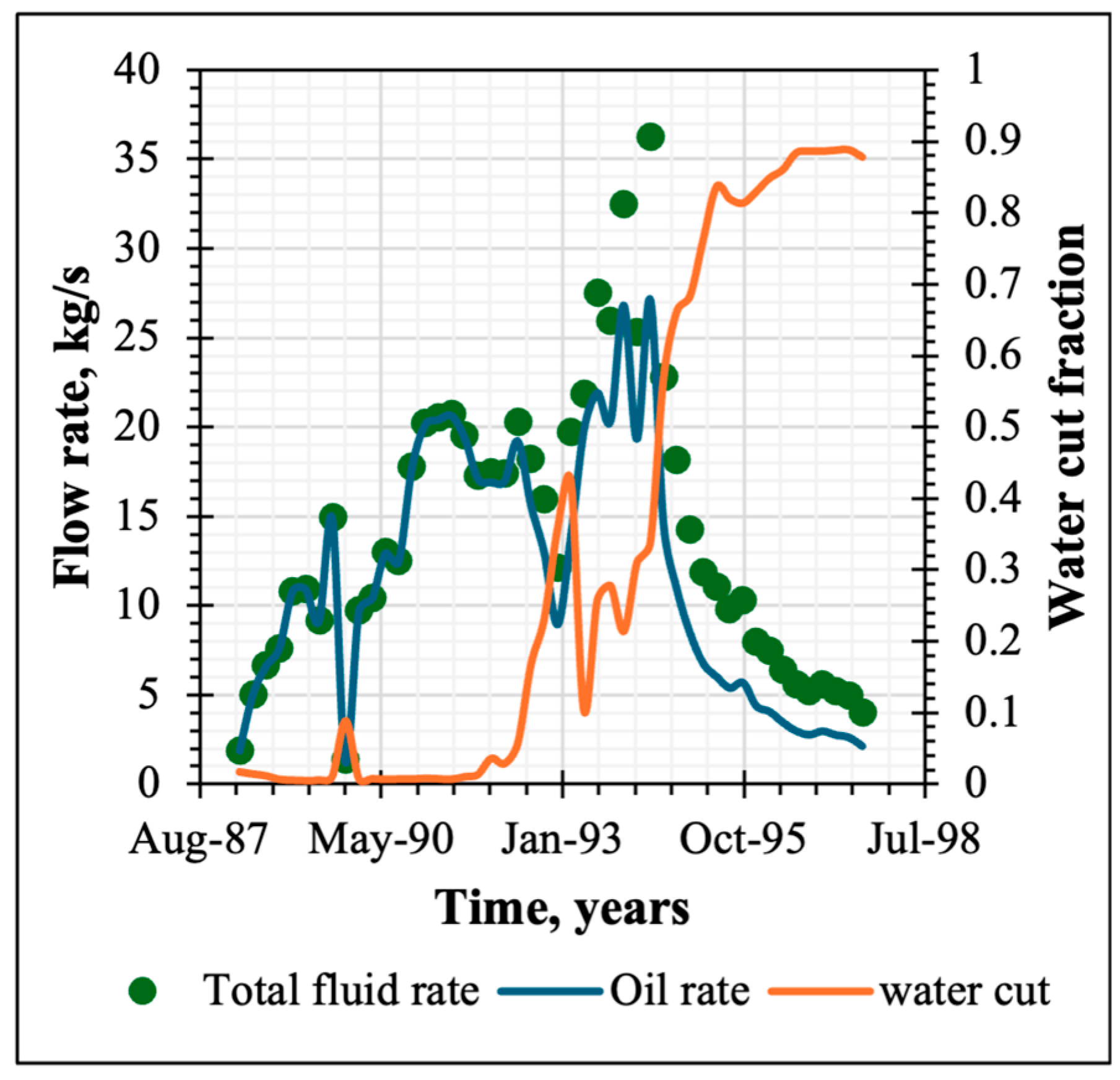
2.3. Use Case: Waihapa Oilfield
2.3.1. Waihapa Wellbore Model
2.3.2. Waihapa Reservoir Model
2.3.3. Natural State Model
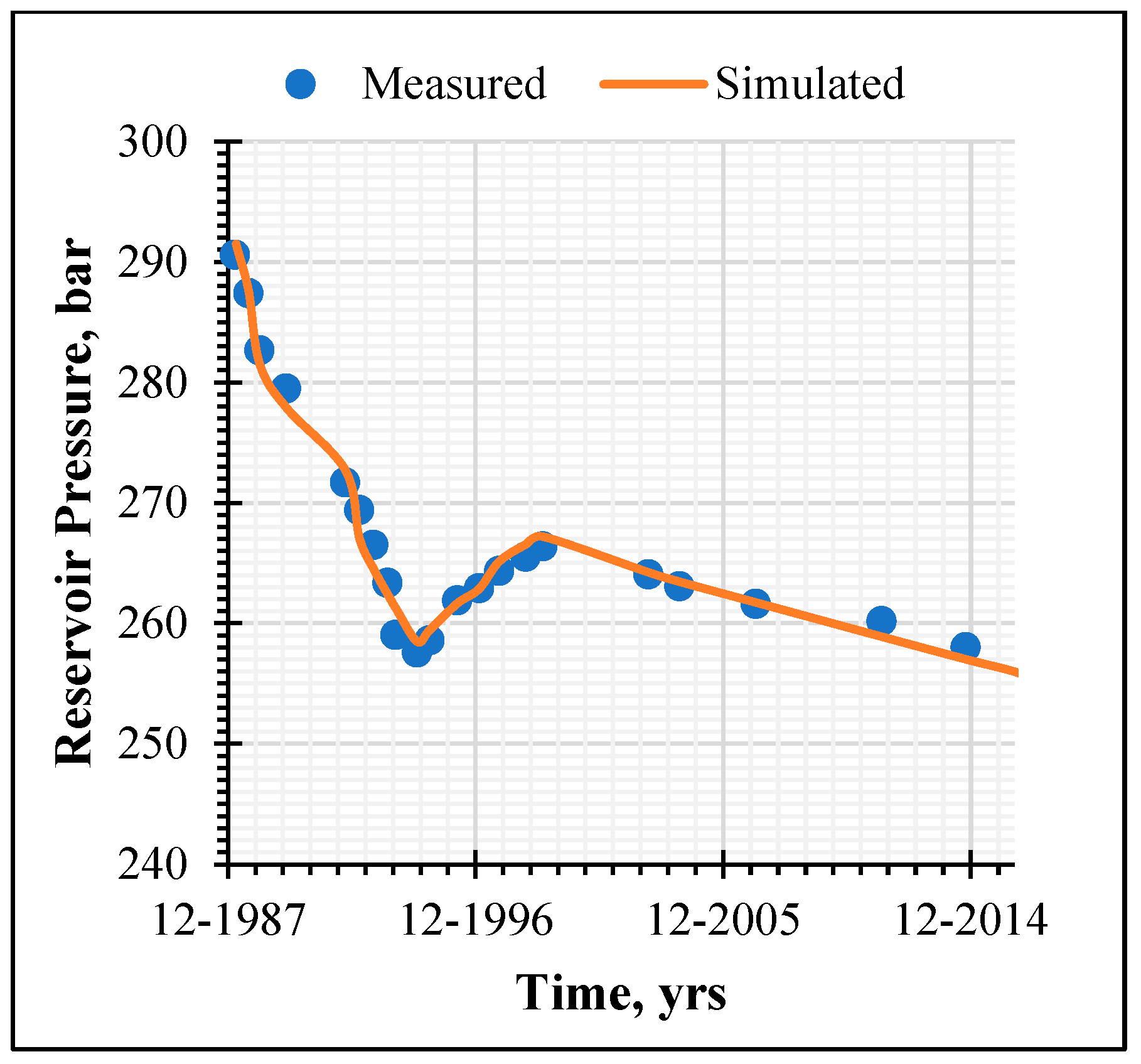
2.3.4. Pressure-Matched Model
2.4. Energy Extraction Simulations
2.4.1. Simulated Scenarios
2.4.2. Truncated Model
3. Results
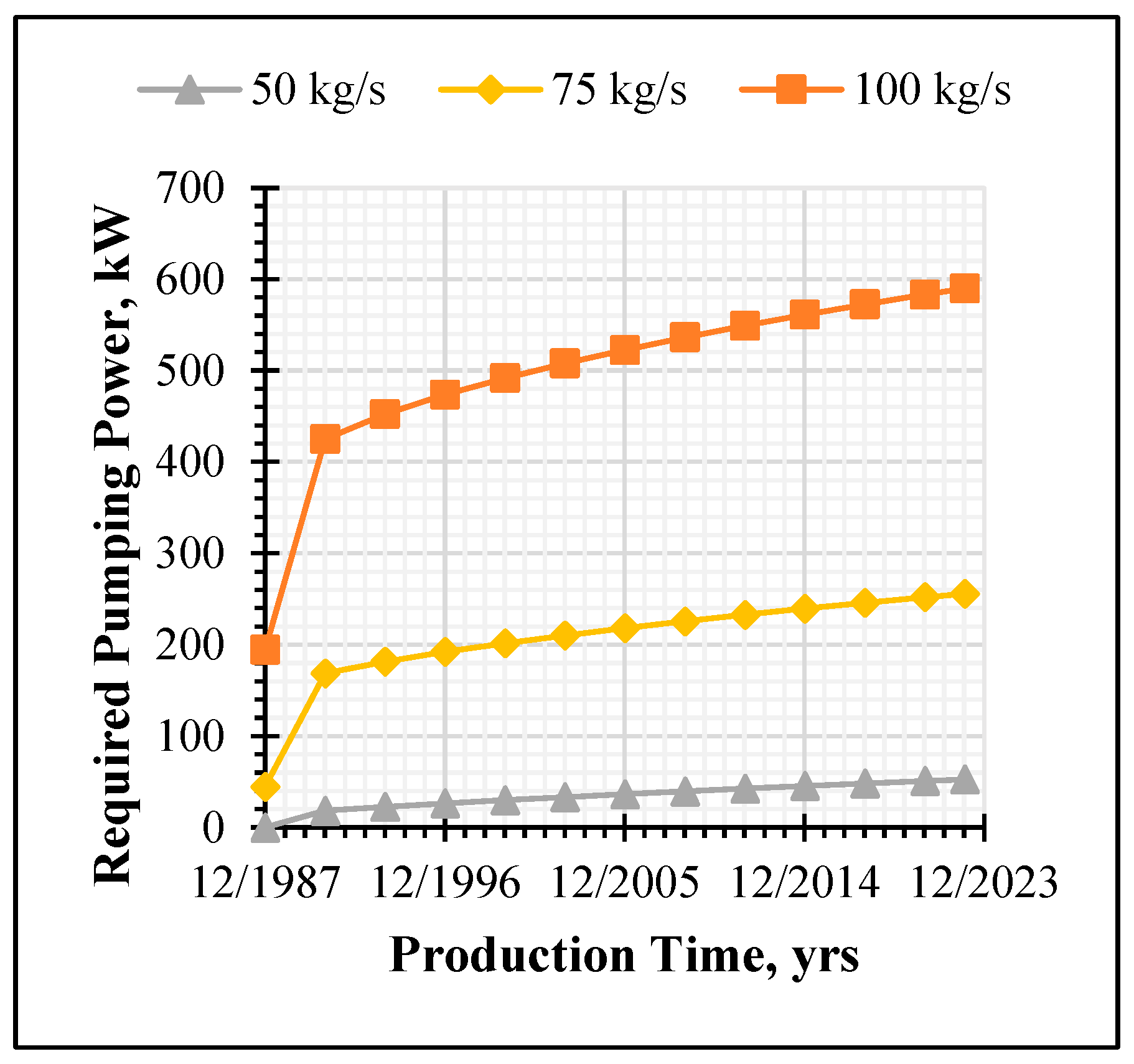
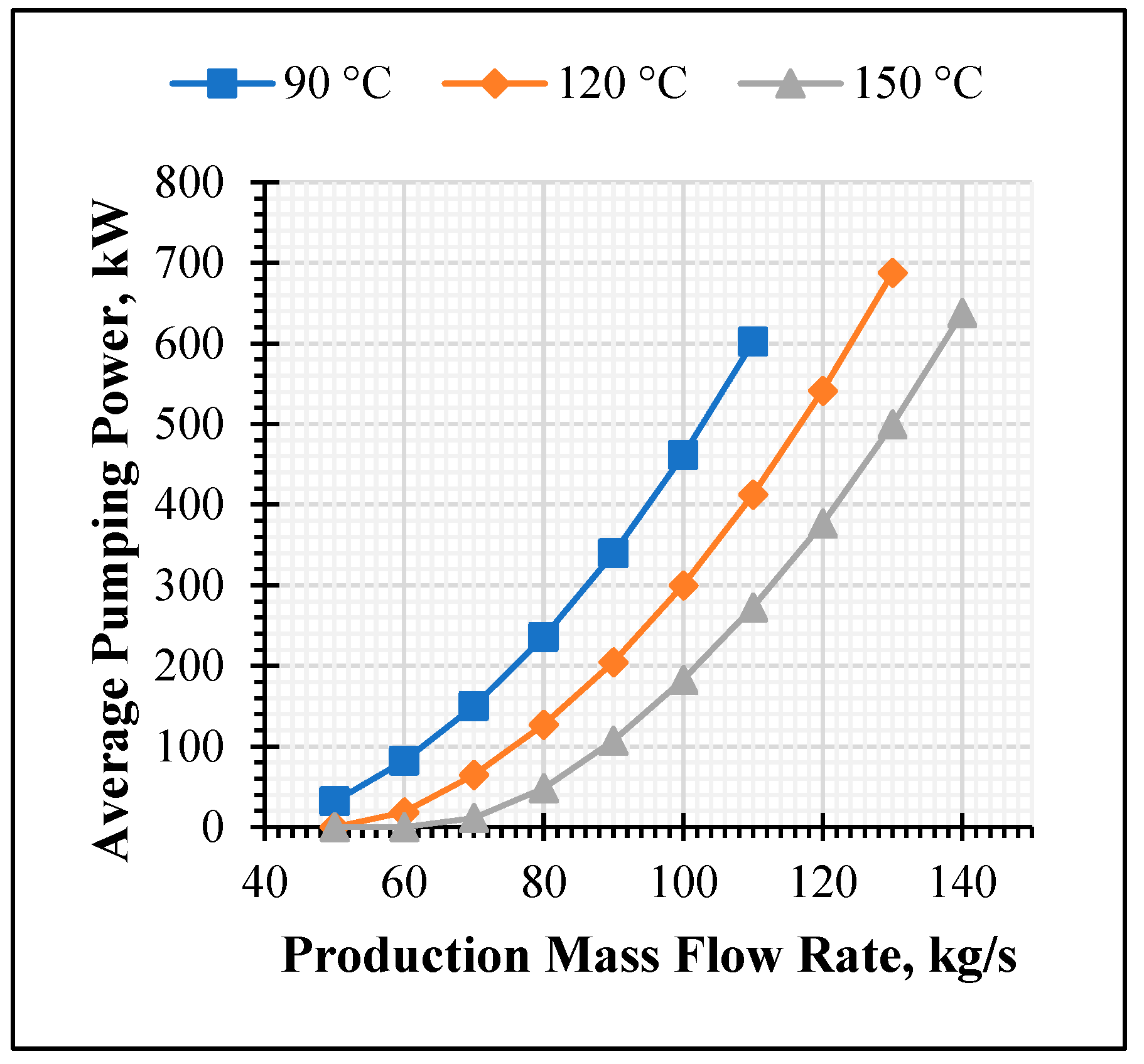
3.1. Energy Extraction
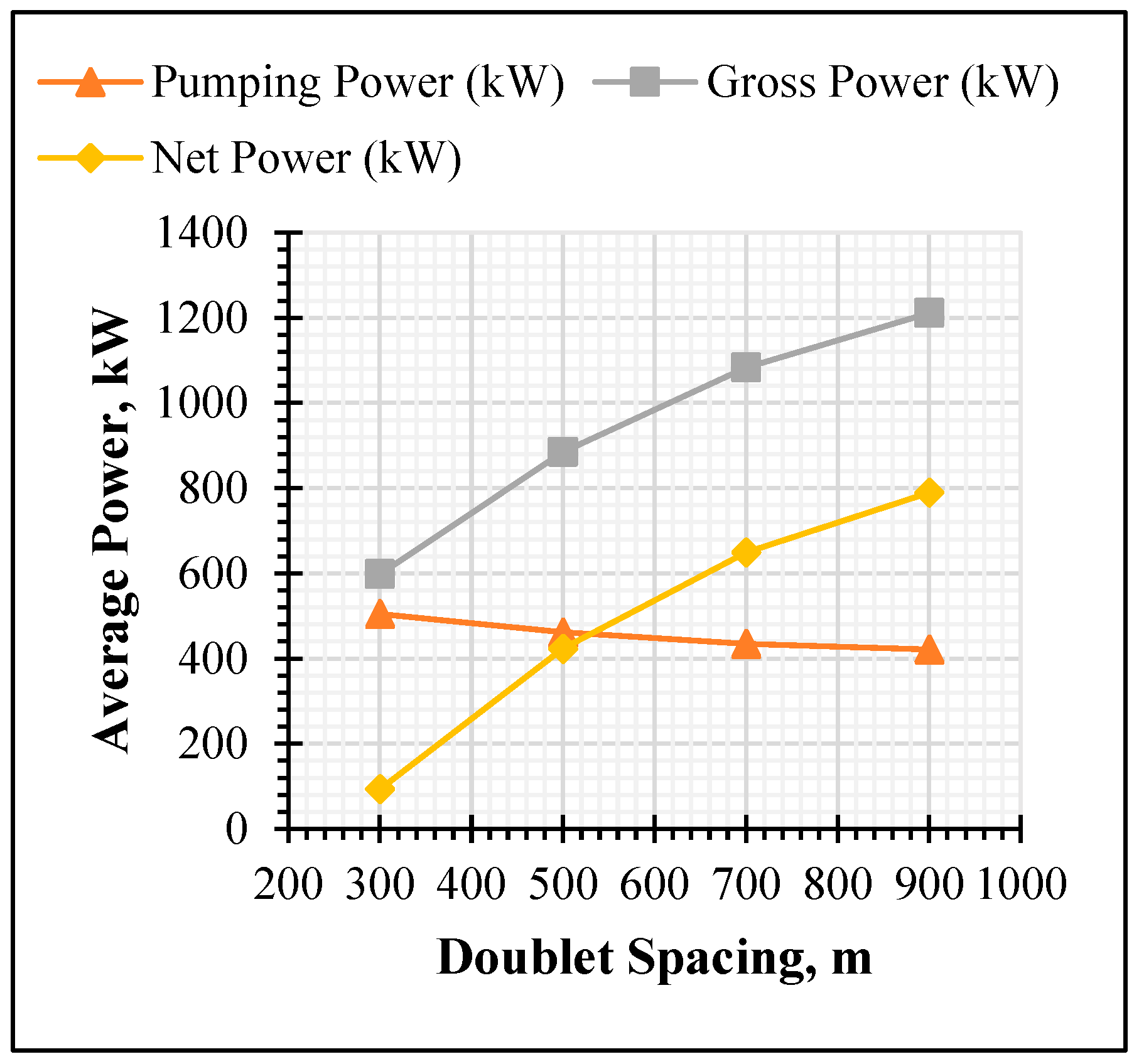
3.2. Doublet Spacing
3.3. Field Size
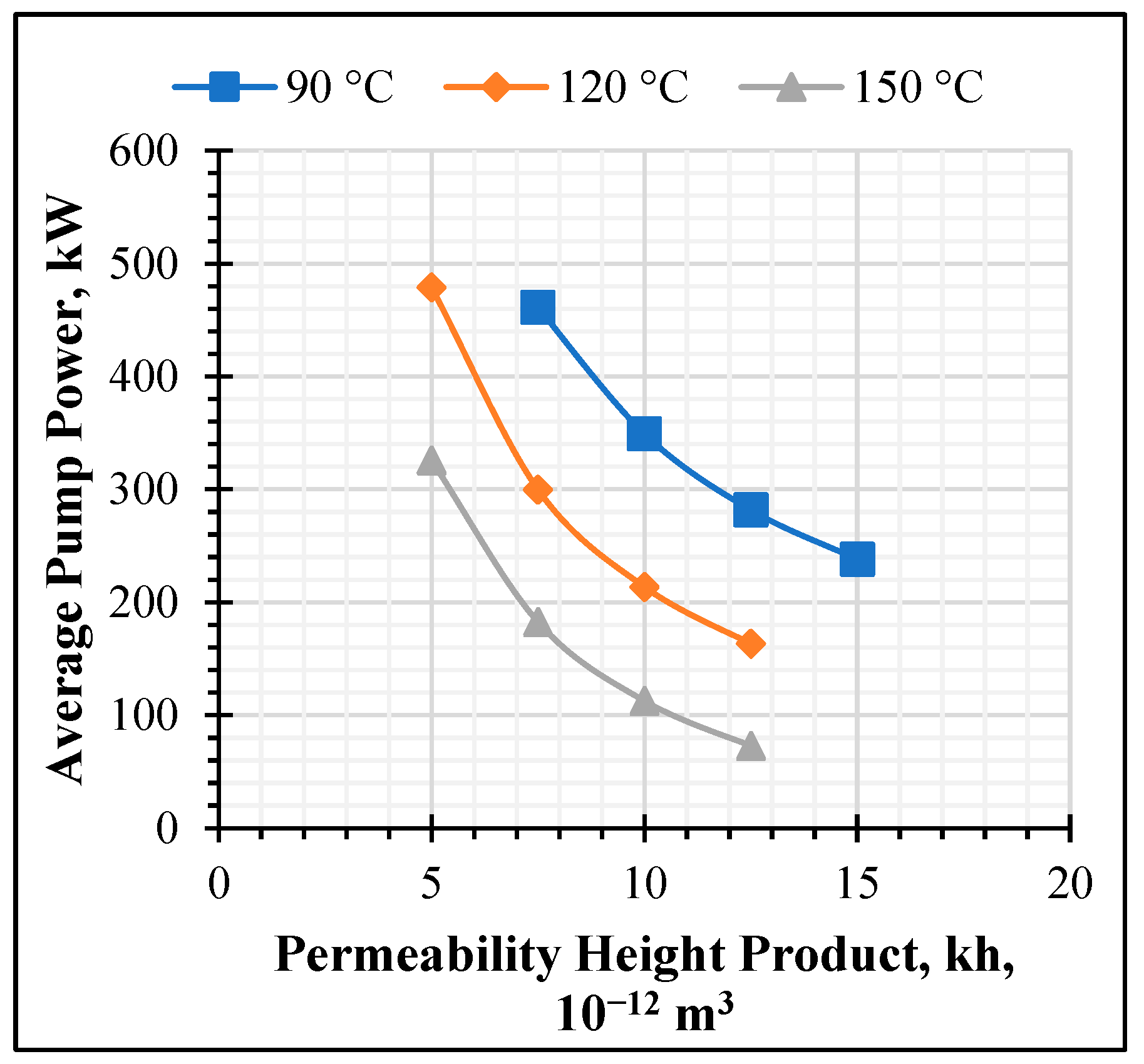
3.4. Pumping Power Correlation
3.5. Hotter Reservoirs
3.6. Reservoir Location
4. Summary
5. Conclusions
6. Future Work
- In future work, the focus could be shifted towards evaluating the geothermal energy extraction potential of gas fields, which are more abundant in New Zealand compared with oil fields. Gas fields have their own unique characteristics and challenges, and studying the feasibility and methodology for extracting geothermal energy from such fields would be valuable. This could involve investigating enhanced geothermal system (EGS) techniques that do not require pre-stimulation of the reservoir.
- Another avenue for future research is exploring the use of supercritical carbon dioxide (sCO2) as a heat transfer fluid in geothermal energy extraction from shallower reservoirs in oil and gas fields. While shallower reservoirs may have higher permeability, they typically have lower resource temperatures. sCO2 possesses properties that make it an attractive fluid for geothermal energy extraction, such as high heat-carrying capacity and a flowing potential like water. Additionally, sCO2 can exhibit a thermo-syphon effect, which means that no pumping power is required under certain conditions. However, the success of this approach may depend on ambient temperature conditions and the ability to cool the CO2 below its critical temperature.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Injectivity index, kg/(s-bar) | r | Reservoir | |
| P | Power, kW | reinj | Reinjection |
| Pressure, bars | w | Well | |
| Productivity index, kg/(s-bar) | wh | Wellhead | |
| Heat flow rate, W | |||
| T | Temperature, °C | ||
| Mass flow rate, kg/s | Symbols | ||
| Subscripts | ρ | Density, kg/m3 | |
| fr | Friction | d | Distance, m |
| hs | Hydrostatic | ||
| out | Output |
References
- Rybach, L.; Kohl, T. Geothermal Energy and a Future Earth. Glob. Change Future Earth 2018, 1, 364–376. [Google Scholar] [CrossRef]
- Stefansson, V. World Geothermal Assessment. In Proceedings of the World Geothermal Congress 2005, Antalya, Turkey, 24–29 April 2005; pp. 24–29. Available online: https://orkustofnun.is/gogn/Greinargerdir/Jardhitavettvangur/World-geothermal-assessment-VS.pdf (accessed on 12 March 2024).
- Franco, A.; Vaccaro, M. Numerical simulation of geothermal reservoirs for the sustainable design of energy plants: A review. Renew. Sustain. Energy Rev. 2014, 30, 987–1002. [Google Scholar] [CrossRef]
- Eyerer, S.; Schifflechner, C.; Hofbauer, S.; Bauer, W.; Wieland, C.; Spliethoff, H. Combined heat and power from hydrothermal geothermal resources in Germany: An assessment of the potential. Renew. Sustain. Energy Rev. 2020, 120, 109661. [Google Scholar] [CrossRef]
- Reyes, A.G. Low-temperature geothermal reserves in New Zealand. Geothermics 2015, 56, 138–161. [Google Scholar] [CrossRef]
- Beard, J.C.E.; Blasingame, T.A. The Future of Geothermal in Texas: Contemporary Prospects and Perspectives. In UT Faculty/Researcher Works; University of Texas at Austin: Austin, TX, USA, 2023. [Google Scholar] [CrossRef]
- Erdlac, R.J., Jr.; Armour, L.; Lee, R.; Snyder, S.; Sorensen, M.; Matteucci, M.; Horton, J. Ongoing Resource Assessment of Geothermal Energy from Sedimentary Basins in Texas. In Proceedings of the Thirty-Second Workshop on Geothermal Reservoir Engineering, Stanford, CA, USA, 22–24 January 2007; Available online: https://pangea.stanford.edu/ERE/pdf/IGAstandard/SGW/2007/erdlac.pdf (accessed on 12 March 2024).
- Nádor, A.; Sebess-Zilahi, L.; Rotár-Szalkai, Á.; Gulyás, Á.; Markovic, T. New methods of geothermal potential assessment in the Pannonian basin. Netherlands J. Geosci. 2019, 98, e10. [Google Scholar] [CrossRef]
- Procesi, M.; Ciotoli, G.; Mazzini, A.; Etiope, G. Sediment-hosted geothermal systems: Review and first global mapping. Earth-Sci. Rev. 2019, 192, 529–544. [Google Scholar] [CrossRef]
- Wang, S.; Hu, J.; Yan, J.; Li, F.; Chen, N.; Tang, Q.; Guo, B.; Zhan, L. Assessment of Geothermal Resources in Petroliferous Basins in China. Math. Geosci. 2019, 51, 271–293. [Google Scholar] [CrossRef]
- Popovsky, J. First Australian Geothermal Plant-Mulka Case Study. In Proceedings of the Australian Geothermal Energy Conferences, Brisbane, Australia, 14–15 November 2013; pp. 1–4. Available online: https://www.geothermal-energy.org/pdf/IGAstandard/AGEC/2013/Popovsky.pdf (accessed on 12 March 2024).
- Christ, A.; Rahimi, B.; Regenauer-Lieb, K.; Chua, H.T. Techno-economic analysis of geothermal desalination using Hot Sedimentary Aquifers: A pre-feasibility study for Western Australia. Desalination 2017, 404, 167–181. [Google Scholar] [CrossRef]
- Graham, I.; Quigley, E.; Janzwood, S.; Homer-Dixon, T. Deep Geothermal Superpower. 2022. Available online: https://cascadeinstitute.org/wp-content/uploads/2022/03/Deep-Geothermal-Superpower-version-1.0.pdf (accessed on 12 March 2024).
- Huang, K.; Gosnold, W.; Hickson, C.; Benoit, D. Using Oil and Gas Data to Assess Geothermal Resources Within the Western Canadian Sedimentary Basin in Alberta; BT—Geothermal Resources Council Virtual Annual Meeting and Expo: Clean, Renewable and Always On, GRC 2020, October 19, 2020–October 23, 2. in Transactions—Geothermal Resources Council, vol. 44. Alberta No. 1, CanadaUniversity of North DakotaSustainable Solutions; Geothermal Resources Council: Davis, CA, USA, 2020; pp. 729–742. Available online: https://publications.mygeoenergynow.org/grc/1034250.pdf (accessed on 12 March 2024).
- Ferguson, G.; Ufondu, L. Geothermal energy potential of the Western Canada Sedimentary Basin: Clues from coproduced and injected water. Environ. Geosci. 2017, 24, 113–121. [Google Scholar] [CrossRef]
- Climo, M.; Blair, A.; Carey, B.; Bendall, S.; Daysh, S. Driving the Uptake of Geothermal Direct Use in New Zealand: Successful Strategies, Empowered Champions, and Lessons Learnt Along the Way. In Proceedings of the World Geothermal Congress 2020+1, Reykjavik, Iceland, 24–27 October 2021; p. 11. Available online: https://pangea.stanford.edu/ERE/db/WGC/papers/WGC/2020/08001.pdf (accessed on 5 July 2022).
- Seward, A.; Carey, B. Geothermal Heat Pumps Role in Rebuilding Christchurch’s Commercial Business District. In Proceedings of the World Geothermal Congress 2020+1, Reykjavik, Iceland, 24–27 October 2021; p. 10. Available online: https://pangea.stanford.edu/ERE/db/WGC/papers/WGC/2020/29004.pdf (accessed on 5 July 2022).
- Reyes, A.G. Abandoned Oil and Gas Wells: A Reconnaissance Study of an Unconventional Geothermal Resource; GNS Science: Auckland, New Zealand, 2007; Available online: https://shop.gns.cri.nz/sr_2007-023-pdf/ (accessed on 12 March 2024).
- Reyes, A.G. Geothermal Energy From Abandoned Petroleum Wells in New Zealand: Benefits and Hurdles. In Proceedings of the 41st New Zealand Geothermal Workshop, Auckland, New Zealand, 25–27 November 2019; pp. 9–12. Available online: https://www.geothermal-energy.org/pdf/IGAstandard/NZGW/2019/011.pdf (accessed on 12 March 2024).
- Zarrouk, S.J.; McLean, K. Geothermal Well Test Analysis; Elsevier: Amsterdam, The Netherlands, 2019. [Google Scholar] [CrossRef]
- Duggal, R.; Rayudu, R.; Hinkley, J.; Burnell, J.; Wieland, C.; Keim, M. A comprehensive review of energy extraction from low-temperature geothermal resources in hydrocarbon fields. Renew. Sustain. Energy Rev. 2022, 154, 111865. [Google Scholar] [CrossRef]
- Kurnia, J.C.; Shatri, M.S.; Putra, Z.A.; Zaini, J.; Caesarendra, W.; Sasmito, A.P. Geothermal energy extraction using abandoned oil and gas wells: Techno-economic and policy review. Int. J. Energy Res. 2021, 46, 28–60. [Google Scholar] [CrossRef]
- Wang, K.; Yuan, B.; Ji, G.; Wu, X. A comprehensive review of geothermal energy extraction and utilization in oilfields. J. Pet. Sci. Eng. 2018, 168, 465–477. [Google Scholar] [CrossRef]
- Ashena, R. Analysis of some case studies and a recommended idea for geothermal energy production from retrofitted abandoned oil and gas wells. Geothermics 2023, 108, 102634. [Google Scholar] [CrossRef]
- Santos, L.; Taleghani, A.D.; Elsworth, D. Repurposing abandoned wells for geothermal energy: Current status and future prospects. Renew. Energy 2022, 194, 1288–1302. [Google Scholar] [CrossRef]
- Carpenter, C. Approach Evaluates Geothermal Potential in Existing Oil and Gas Wells. J. Pet. Technol. 2022, 74, 47–50. [Google Scholar] [CrossRef]
- Anderson, T.C. The geologic and hydrologic setting of NPR-3 (Teapot Dome) Wyoming and its EGS geothermal potential. In GRC Transactions; Wyoming Geological Association: Casper, WY, USA, 2010; Volume 34, pp. 285–290. Available online: https://publications.mygeoenergynow.org/grc/1028659.pdf (accessed on 12 March 2024).
- Sanyal, S.K.; Butler, S.J. Geothermal Power Capacity of Wells in Non-Convective Sedimentary Formations. In Proceedings of the World Geothermal Congress, Bali, Indonesia, 25–30 April 2010; Available online: https://www.geothermal-energy.org/pdf/IGAstandard/WGC/2010/3712.pdf (accessed on 12 March 2024).
- Xin, S.; Liang, H.; Hu, B.; Li, K. Electrical Power Generation from Low Temperature Co-Produced Geothermal Resources at Huabei Oilfield. In Proceedings of the Thirty-Seventh Workshop on Geothermal Reservoir Engineering Stanford University, Stanford, CA, USA, 30 January–1 February 2012; Available online: https://pangea.stanford.edu/ERE/pdf/IGAstandard/SGW/2012/Xin.pdf (accessed on 12 March 2024).
- Eagle-Bluestone, J.; Alamooti, M.; Namie, S.; Porlles, J. Mandaree, North Dakota: A Case Study on Oil and Gas Well Conversion to Geothermal District Heating Systems for Rural Communities; BT—2021 Geothermal Rising Conference: Using the Earth to Save the Earth, GRC 2021, October 3, 2021–October 6, 2021. in Transactions—Geothermal Resources Council, vol. 45. University of North DakotaIceland School of EnergyCalifornia Geologic Energy Management Division; Geothermal Resources Council: Davis, CA, USA, 2021; pp. 330–353. [Google Scholar]
- Gosnold, W.; LeFever, R.; Klenner, R.; Mann, M. Geothermal Power form Coproduced Fluids in the Williston Basin. In GRC Transactions; Wyoming Geological Association: Casper, WY, USA, 2010; Volume 34, Available online: https://publications.mygeoenergynow.org/grc/1028702.pdf (accessed on 12 March 2024).
- Bennett, K.P.; Li, K.; Horne, R.N.; Program, S.G. Power Generation Potential from Coproduced Fluids in the Los Angeles Basin; Stanford University: Stanford, CA, USA, 2012; Available online: https://pangea.stanford.edu/ERE/pdf/SGPreports/SGP-TR-196.pdf (accessed on 12 March 2024).
- Wang, S.; Yan, J.; Li, F.; Hu, J.; Li, K. Exploitation and Utilization of Oilfield Geothermal Resources in China. Energies 2016, 9, 798. [Google Scholar] [CrossRef]
- Reyes, A.G. Geothermal energy from abandoned petroleum wells in New Zealand. In Proceedings of the 12th Asian Geothermal Symposium; Asian Geothermal Symposium, Daejeon, Republic of Korea, 9–12 November 2018; pp. 1–9. Available online: https://www.geothermal-energy.org/pdf/IGAstandard/Asian/2018/021%7B%5C_%7Dreyes.pdf (accessed on 12 March 2024).
- Huang, Y.; Cheng, Y.; Ren, L.; Tian, F.; Pan, S.; Wang, K.; Wang, J.; Dong, Y.; Kong, Y. Assessing the Geothermal Resource Potential of an Active Oil Field by Integrating a 3D Geological Model With the Hydro-Thermal Coupled Simulation. Front. Earth Sci. 2022, 9, 1178. [Google Scholar] [CrossRef]
- Hickson, C.; Colombina, M. Launching Conventional Deep Geothermal Development Projects in Oil and Gas Dominated Regions of Canada; BT—2021 Geothermal Rising Conference: Using the Earth to Save the Earth, GRC 2021, October 3, 2021–October 6, 2021. in Transactions—Geothermal Resources Council, vol. 45. Alberta No. 1Terrapin Geothermics; Geothermal Resources Council: Davis, CA, USA, 2021; pp. 1669–1679. Available online: https://publications.mygeoenergynow.org/grc/1034482.pdf (accessed on 12 March 2024).
- Sidike, A. Geothermal Energy from Repurposed Oil and Gas Wells in Western North Dakota; University of North Dakota: Grand Forks, ND, USA, 2020; Available online: https://commons.und.edu/theses/3087/ (accessed on 12 March 2024).
- Wight, N.M.; Bennett, N.S. Geothermal energy from abandoned oil and gas wells using water in combination with a closed wellbore. Appl. Therm. Eng. 2015, 89, 908–915. [Google Scholar] [CrossRef]
- Liu, X.; Falcone, G.; Alimonti, C. A systematic study of harnessing low-temperature geothermal energy from oil and gas reservoirs. Energy 2018, 142, 346–355. [Google Scholar] [CrossRef]
- Wellmann, J.F.; Horowitz, F.G.; Ricard, L.P.; Regenauer-Lieb, K. Estimates of sustainable pumping in Hot Sedimentary Aquifers: Theoretical considerations, numerical simulations and their application to resource mapping. In Proceedings of the Australian Geothermal Conference, Adelaide, Australia, 16–19 November 2010; pp. 215–221. Available online: http://www.geothermal-energy.org/pdf/IGAstandard/AGEC/2010/Wellmann_et_al_2010.pdf (accessed on 12 March 2024).
- Crooijmans, R.A.; Willems, C.J.L.; Nick, H.M.; Bruhn, D.F. The influence of facies heterogeneity on the doublet performance in low-enthalpy geothermal sedimentary reservoirs. Geothermics 2016, 64, 209–219. [Google Scholar] [CrossRef]
- Sanyal, S.K.; Morrow, J.W.; Butler, S.J. Geothermal Well Productivity: Why Hotter is Not Always Better. In GRC Transactions; Wyoming Geological Association: Casper, WY, USA, 2007; Volume 31, pp. 573–579. Available online: https://publications.mygeoenergynow.org/grc/1025281.pdf (accessed on 12 March 2024).
- Sanyal, S.K.; Butler, S.J. Feasibility of Geothermal Power Generation from Petroleum Wells. In GRC Transactions; Geothermal Resources Council Transactions; Wyoming Geological Association: Casper, WY, USA, 2009; Volume 33, p. 679. Available online: https://publications.mygeoenergynow.org/grc/1028541.pdf (accessed on 12 March 2024).
- Clotworthy, A.W.; Quinlivan, P.F.; Coventry, R. Probabilistic Flow Modelling of Geothermal Wells in Sedimentary Aquifers. In Proceedings of the World Geothermal Congress, Bali, Indonesia, 25–30 April 2010; pp. 1–4. Available online: https://www.geothermal-energy.org/pdf/IGAstandard/WGC/2010/2240.pdf (accessed on 12 March 2024).
- Clotworthy, A.W.; Lawless, J.V.; Ussher, G. What is the end point for geothermal developments: Modeling depletion of geothermal fields. World Geotherm. Congree Pap. 2010, 22–39. Available online: https://www.geothermal-energy.org/pdf/IGAstandard/WGC/2010/2239.pdf (accessed on 12 March 2024).
- Kurevija, T.; Vulin, D. High Enthalpy Geothermal Potential of the Deep Gas Fields in Central Drava Basin, Croatia. Water Resour. Manag. 2011, 25, 3041–3052. [Google Scholar] [CrossRef]
- Gong, B.; Liang, H.; Xin, S.; Li, K. Effect of Water Injection on Reservoir Temperature During Power Generation in Oil Fields. In Proceedings of the Thirty-Sixth Workshop on Geothermal Reservoir Engineering; Standford Geothermal Workshop, Stanford University, Standford, CA, USA, 31 January–2 February 2011; Available online: https://pangea.stanford.edu/ERE/pdf/IGAstandard/SGW/2011/gong.pdf (accessed on 12 March 2024).
- Franco, A.; Vaccaro, M. Sustainable Sizing of Geothermal Power Plants: Appropriate Potential Assessment Methods. Sustainability 2020, 12, 3844. [Google Scholar] [CrossRef]
- Cui, G.; Ren, S.; Dou, B.; Ning, F. Geothermal energy exploitation from depleted high-temperature gas reservoirs by recycling CO2: The superiority and existing problems. Geosci. Front. 2020, 12, 101078. [Google Scholar] [CrossRef]
- Bujakowski, W.; Tomaszewska, B.; Miecznik, M. The Podhale geothermal reservoir simulation for long-term sustainable production. Renew. Energy 2016, 99, 420–430. [Google Scholar] [CrossRef]
- Franz, P.; Clearwater, J. Volsung User Manual Version 1.16.3. 2022. Available online: https://www.scribd.com/document/581026976/Manual (accessed on 12 March 2024).
- Duggal, R.; Rayudu, R.; Hinkley, J.; Burnell, J.; Ward, S. Performance of a Geothermal System in Petroleum Fields of the Taranaki Region, New Zealand. In Proceedings of the 43rd New Zealand Geothermal Workshop, Wellington, New Zealand, 23–25 November 2021; p. 25. Available online: http://www.geothermal-energy.org/pdf/IGAstandard/NZGW/2021/12.pdf (accessed on 12 March 2024).
- Fan, Y.; Zhang, S.; Huang, Y.; Pang, Z.; Li, H. Determining the Recoverable Geothermal Resources Using a Numerical Thermo-Hydraulic Coupled Modeling in Geothermal Reservoirs. Front. Earth Sci. 2022, 9, 787133. [Google Scholar] [CrossRef]
- Bahonar, M.; Azaiez, J.; Chen, Z. Two Issues in Wellbore Heat Flow Modelling Along With the Prediction of Casing Temperature in the Steam Injection Wells. J. Can. Pet. Technol. 2011, 50, 43–63. [Google Scholar] [CrossRef]
- Zhang, J.; Zhu, X. Productivity evaluation of geothermal energy production system based on abandoned oil and gas wells. In Utilization of Thermal Potential of Abandoned Wells; Elsevier: Amsterdam, The Netherlands, 2022; pp. 115–134. [Google Scholar] [CrossRef]
- O’Sullivan, M.J.; Pruess, K.; Lippmann, M.J. State of the art of geothermal reservoir simulation. Geothermics 2001, 30, 395–429. [Google Scholar] [CrossRef]
- O’Sullivan, J.; Popineau, J.; Gravatt, M.; Renaud, T.; Riffault, J.; Croucher, A.; Yeh, A.; O’Sullivan, M. An integrated, mesh-independent geothermal modelling framework. Environ. Model. Softw. 2023, 163, 105666. [Google Scholar] [CrossRef]
- Ezekiel, J.; Ebigbo, A.; Adams, B.M.; Saar, M.O. Combining natural gas recovery and CO2-based geothermal energy extraction for electric power generation. Appl. Energy 2020, 269, 115012. [Google Scholar] [CrossRef]
- Gringarten, A.C. Reservoir lifetime and heat recovery factor in geothermal aquifers used for urban heating. Pure Appl. Geophys. PAGEOPH 1978, 117, 297–308. [Google Scholar] [CrossRef]
- Tester, J.W.; Anderson, B.J.; Batchelor, A.S.; Blackwell, D.D.; DiPippo, R.; Drake, E.M.; Garnish, J.; Livesay, B.; Moore, M.C.; Nichols, K.; et al. The future of geothermal energy. Massachusetts Inst. Technol. 2006, 358, 243. Available online: https://energy.mit.edu/wp-content/uploads/2006/11/MITEI-The-Future-of-Geothermal-Energy.pdf (accessed on 12 March 2024).
- Duggal, R.; Rayudu, R.; Hinkley, J.; Burnell, J.; Ward, S. Identifying issues in geothermal energy production from petroleum fields. In Proceedings of the TENCON 2021–2021 IEEE Region 10 Conference (TENCON), Auckland, New Zealand, 7–10 December 2021; pp. 254–259. [Google Scholar] [CrossRef]
- Yearsley, E.N. Common Characteristics of Pumped Wells in Geothermal Power Projects. In Proceedings of the 41st New Zealand Geothermal Workshop, Auckland, New Zealand, 25–27 November 2019; Available online: https://www.geothermal-energy.org/pdf/IGAstandard/NZGW/2019/021.pdf (accessed on 12 March 2024).
- Hochwimmer, A.; Urzua, L.; Ussher, G.; Parker, C. Key Performance Indicators for Pumped Well Geothermal Power Generation. In Proceedings of the World Geothermal Congress, Melbourne, Australia, 19–25 April 2015; pp. 1–12. Available online: https://pangea.stanford.edu/ERE/db/WGC/papers/WGC/2015/26056.pdf (accessed on 12 March 2024).
- Adams, M. Waihapa—A New Look at an Old Oil Field. Petroleum Club Meeting. 2019. Available online: www.newzealandenergy.com (accessed on 12 March 2024).
- Hood, S.D.; Nelson, C.S.; Kamp, P.J.J. Petrogenesis of the Tikorangi Formation Fracture Reservoir, Waihapa-Ngaere Field, Taranaki Basin. 2002. Available online: https://researchcommons.waikato.ac.nz/bitstream/handle/10289/3618/Hood_Nelson_Kamp_02_Tikorangi.pdf?sequence=1 (accessed on 12 March 2024).
- Lock, R.; Carter, M.; Kelly, C.; Hillyer, M. Waihapa-1, 1A, 1B Well Completion Report, PPL 38034. 1986. Available online: https://data.gns.cri.nz/mapservice/Content/outputs/TRQ/Taranaki_Reservoirs/Pages_from_PRs/Waihapa_1/DST_Pages_from_PR1187.pdf (accessed on 12 March 2024).
- Hood, S.D. Subsurface Stratigraphy and Petrology of a Cool-Water Carbonate Fracture Reservoir-the Mid-Tertiary Tikorangi Formation, Taranaki Basin, New Zealand; The University of Waikato: Hamilton, New Zealand, 2000; Available online: https://hdl.handle.net/10289/14434 (accessed on 12 March 2024).
- Angalwa, A. Extraction of Geothermal Energy from Petroleum Fields. Master’s Thesis, Department of Engineering Science, The University of Auckland, Auckland, New Zealand, 2014. [Google Scholar]
- Clearwater, J.; Franz, P. Introducing the Volsung Geothermal Simulator: Features and Applications. In Proceedings of the 41st New Zealand Geothermal Workshop, Auckland, New Zealand, 25–27 November 2019; p. 27. Available online: https://files.seequent.com/PDFs/Clearwater_2019.pdf (accessed on 12 March 2024).
- Quinao, J.J.; Franz, P.; Clearwater, J. Well Performance Diagnostics and Forecasting Using the Gudrun Wellbore Simulator—Case Studies from Kawerau, New Zealand. In Proceedings of the 42nd New Zealand Geothermal Workshop, Bay of Islands, New Zealand, 24–26 November 2020; p. 26. Available online: http://www.geothermal-energy.org/pdf/IGAstandard/NZGW/2020/081.pdf (accessed on 12 March 2024).
- MBIE. Petroleum Reserves Data. Ministry of Business Innovation & Employment. Available online: https://www.mbie.govt.nz/building-and-energy/energy-and-natural-resources/energy-statistics-and-modelling/energy%02statistics/petroleum-reserves-data/ (accessed on 12 March 2024).
- Dumkwu, F.A.; Islam, A.W.; Carlson, E.S. Review of well models and assessment of their impacts on numerical reservoir simulation performance. J. Pet. Sci. Eng. 2012, 82–83, 174–186. [Google Scholar] [CrossRef]
- GNS PBE. New Zealand Petroleum Basin Explorer. Available online: https://data.gns.cri.nz/pbe/ (accessed on 12 March 2024).
- Kamila, Z.; Kaya, E.; Zarrouk, S.J. Reinjection in geothermal fields: An updated worldwide review 2020. Geothermics 2021, 89, 101970. [Google Scholar] [CrossRef]
- Franz, P.; Clearwater, J.; Burnell, J. Introducing the volsung geothermal simulator: Benchmarking and performance. In Proceedings of the 41st New Zealand Geothermal Workshop, Auckland, New Zealand, 25−27 November 2019; Available online: https://files.seequent.com/PDFs/Franz_2019.pdf (accessed on 12 March 2024).
- NZP&M. The New Zealand Petroleum & Minerals Online Exploration Database. Available online: https://data.nzpam.govt.nz/GOLD/system/mainframe.asp (accessed on 12 March 2024).
- Cho, J.; Augustine, C.; Zerpa, L.E. Validation of a Numerical Reservoir Model of Sedimentary Geothermal Systems Using Analytical Models. In Proceedings of the Fortieth Workshop on Geothermal Reservoir Engineering, Stanford, CA, USA, 26–28 January 2015; pp. 1–13. Available online: https://pangea.stanford.edu/ERE/pdf/IGAstandard/SGW/2015/Cho.pdf (accessed on 12 March 2024).
- Zerpa, L.E.; Cho, J.; Augustine, C. Assessing the Effect of Realistic Reservoir Features on the Performance of Sedimentary Geothermal Systems. In GRC Transactions; Wyoming Geological Association: Casper, WY, USA, 2015; Volume 39, pp. 959–966. Available online: https://publications.mygeoenergynow.org/grc/1032241.pdf (accessed on 12 March 2024).
- Pruess, K.; Narasimhan, T.N. A Practical Method for Modeling Fluid and Heat Flow in Fractured Porous Media. Soc. Pet. Eng. J. 1985, 25, 14–26. [Google Scholar] [CrossRef]
- Holmes, D. Petroleum Report Series PR2031—Review of Waihapa/Ngaere Field Production Capabilities and Artificial Lift Requirements. PML38140 PML38141; Petroleum and Minerals Database: Wellington, New Zealand, 1994. [Google Scholar]
- Sanyal, S.K. One Discipline, Two Arenas-Reservoir Engineering in Geothermal And Petroleum Industries. In Proceedings of the Twenty-Eighth Workshop on Geothermal Reservoir Engineering, Stanford, CA, USA, 27–29 January 2003; pp. 1–11. Available online: https://earthsciences.stanford.edu/ERE/pdf/IGAstandard/SGW/2003/Sanyal.pdf (accessed on 12 March 2024).
- Deo, M.; Roehner, R.; Rick, A.; Moore, J. Reservoir Modeling of Geothermal Energy Production from Stratigraphic Reservoirs in The Great Basin. In Proceedings of the Thirty-Eighth Workshop on Geothermal Reservoir Engineering, Stanford, CA, USA, 11–13 February 2013; Available online: https://pangea.stanford.edu/ERE/pdf/IGAstandard/SGW/2013/Deo.pdf (accessed on 12 March 2024).
- IAPWS. IAPWS R7-97(2012): IAPWS-IF97 Industrial Formulation for Thermodynamic Properties of Water and Steam. Available online: http://www.iapws.org/relguide/IF97-Rev.html (accessed on 12 March 2024).
- Stoltz, L.R.; Jones, M.S.; Wadsley, A.W. Probabilistic Reserves Assessment Using A Filtered Monte Carlo Method In A Fractured Limestone Reservoir. In Proceedings of the SPE Asia Pacific Conference on Integrated Modelling for Asset Management, Lumpur, Malaysia, 23–24 March 1998. [Google Scholar] [CrossRef]
- Green, S.; McLennan, J.; Panja, P.; Kitz, K.; Allis, R.; Moore, J. Geothermal battery energy storage. Renew. Energy 2021, 164, 777–790. [Google Scholar] [CrossRef]
- Febrianto, R.; Bydder, J.; Hochwimmer, A.; Ussher, G.; Libbey, R. Developing low temperature Geothermal projects in Indonesia using pumped well technology. IOP Conf. Ser. Earth Environ. Sci. 2019, 254, 012021. [Google Scholar] [CrossRef]
- Karytsas, C.; Kontoleontos, E.; Mendrinos, D. Electricity generation from low enthalpy geothermal fluids. Greece. 2009. Available online: https://www.researchgate.net/publication/258926276_Electricity_generation_from_low_enthalpy_geothermal_fluids (accessed on 12 March 2024).
- Karytsas, C.; Kontoleontos, E.; Mendrinos, D. Project LOW-BIN ‘Efficient Low Temperature Geothermal Binary Power. In Proceedings of the European Geothermal Congress, Unterhaching, Germany, 30 May–1 June 2007; Available online: https://pangea.stanford.edu/ERE/pdf/IGAstandard/EGC/2007/118.pdf (accessed on 12 March 2024).
- Ma, R.; Zheng, C. Effects of Density and Viscosity in Modeling Heat as a Groundwater Tracer. Ground Water 2009, 48, 380–389. [Google Scholar] [CrossRef]
- Hu, Z.; Xu, T.; Feng, B.; Yuan, Y.; Li, F.; Feng, G.; Jiang, Z. Thermal and fluid processes in a closed-loop geothermal system using CO2 as a working fluid. Renew. Energy 2020, 154, 351–367. [Google Scholar] [CrossRef]
- Liu, L.; Huang, C.Z.; Huang, G.; Baetz, B.; Pittendrigh, S.M. How a carbon tax will affect an emission-intensive economy: A case study of the Province of Saskatchewan, Canada. Energy 2018, 159, 817–826. [Google Scholar] [CrossRef]
- Climo, M.; Milicich, S.D.; Doorman, P.; Alcaraz, S.A.; Seward, A.; Carey, B. Geothermal Use Inventory Update—Data, Visualisation, and Information. In Proceedings of the World Geothermal Congress 2020+1, Reykjavik, Iceland, 24–27 October 2021; p. 11. Available online: http://www.geothermal-energy.org/pdf/IGAstandard/WGC/2020/28000.pdf (accessed on 5 July 2022).
- Climo, M.; Seward, A.; Blair, A.; Bendall, S.; Carey, B. Geoheat Strategy for Aotearoa New Zealand—2020 Progress Update. In Proceedings of the 42nd New Zealand Geothermal Workshop, Waitangi, New Zealand, 24–26 November 2020; p. 5. Available online: http://www.geothermal-energy.org/pdf/IGAstandard/NZGW/2020/016.pdf (accessed on 5 July 2022).
- MBIE. Process Heat in New Zealand: Opportunities and Barriers to Lowering Emissions.Technical Paper Released January. 2019. Available online: https://www.mbie.govt.nz/dmsdocument/4292-process-heat-in-new-zealand-opportunities-and-barriers-to-lowering-emissions (accessed on 12 March 2024).

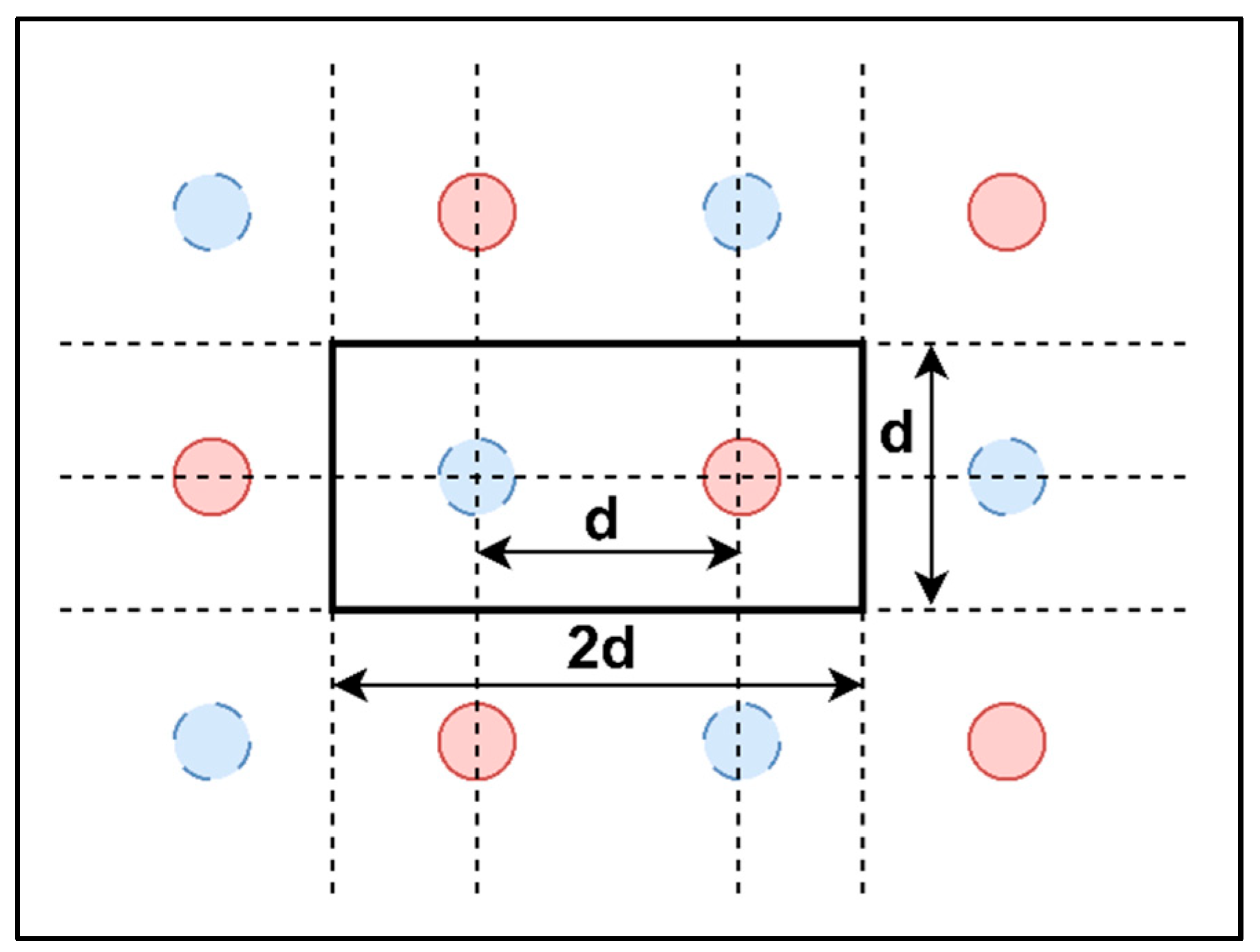


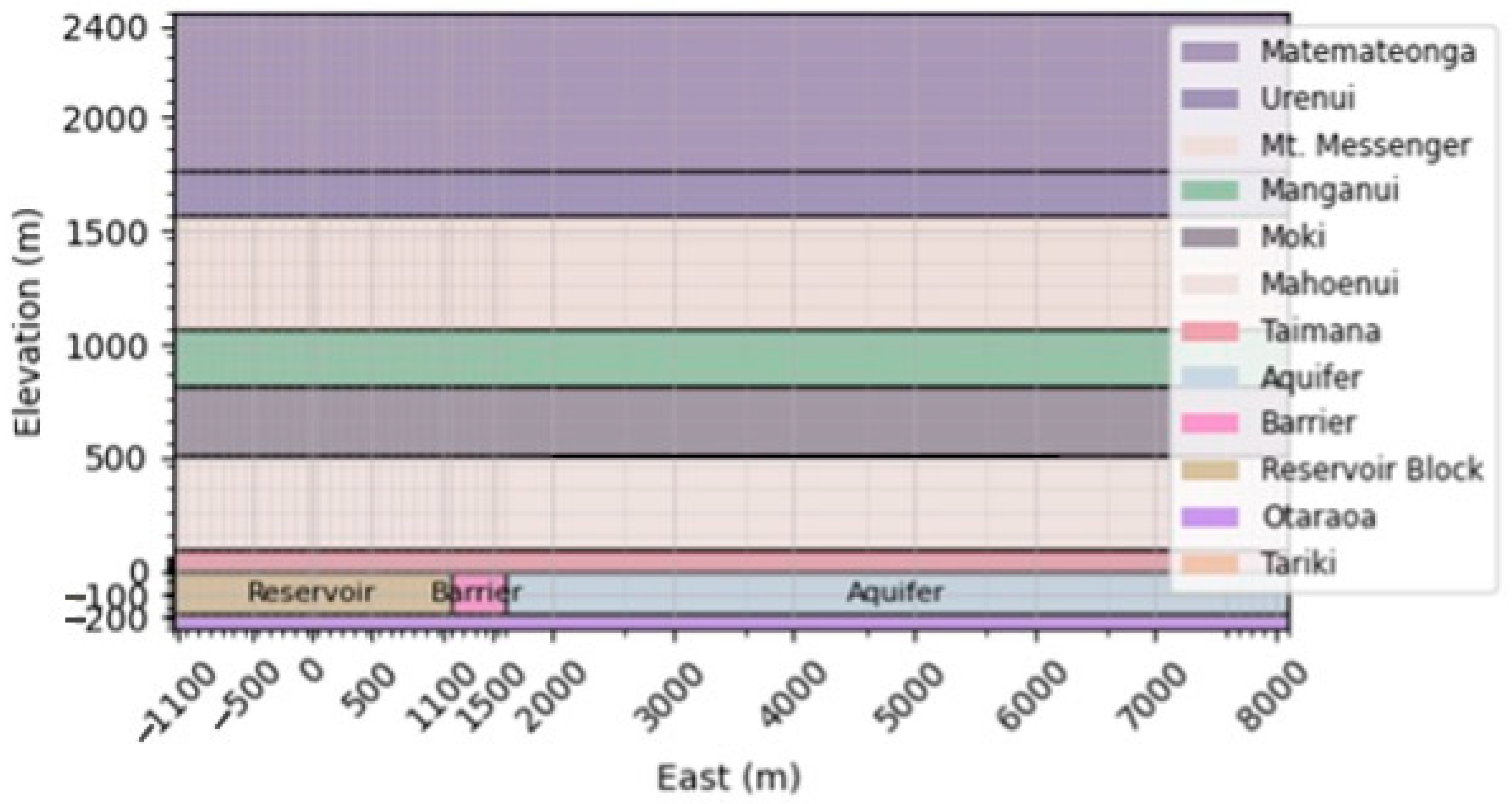
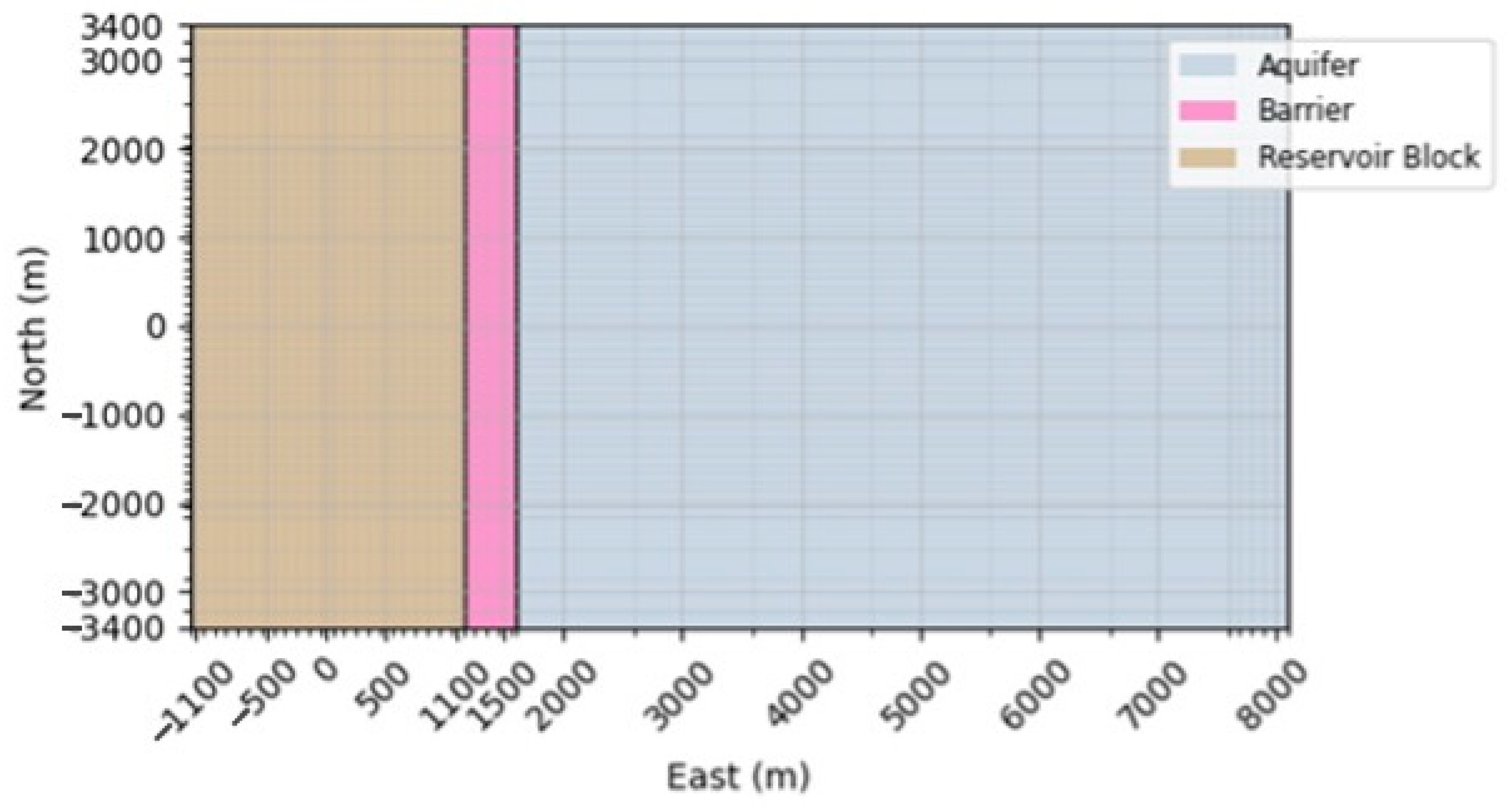
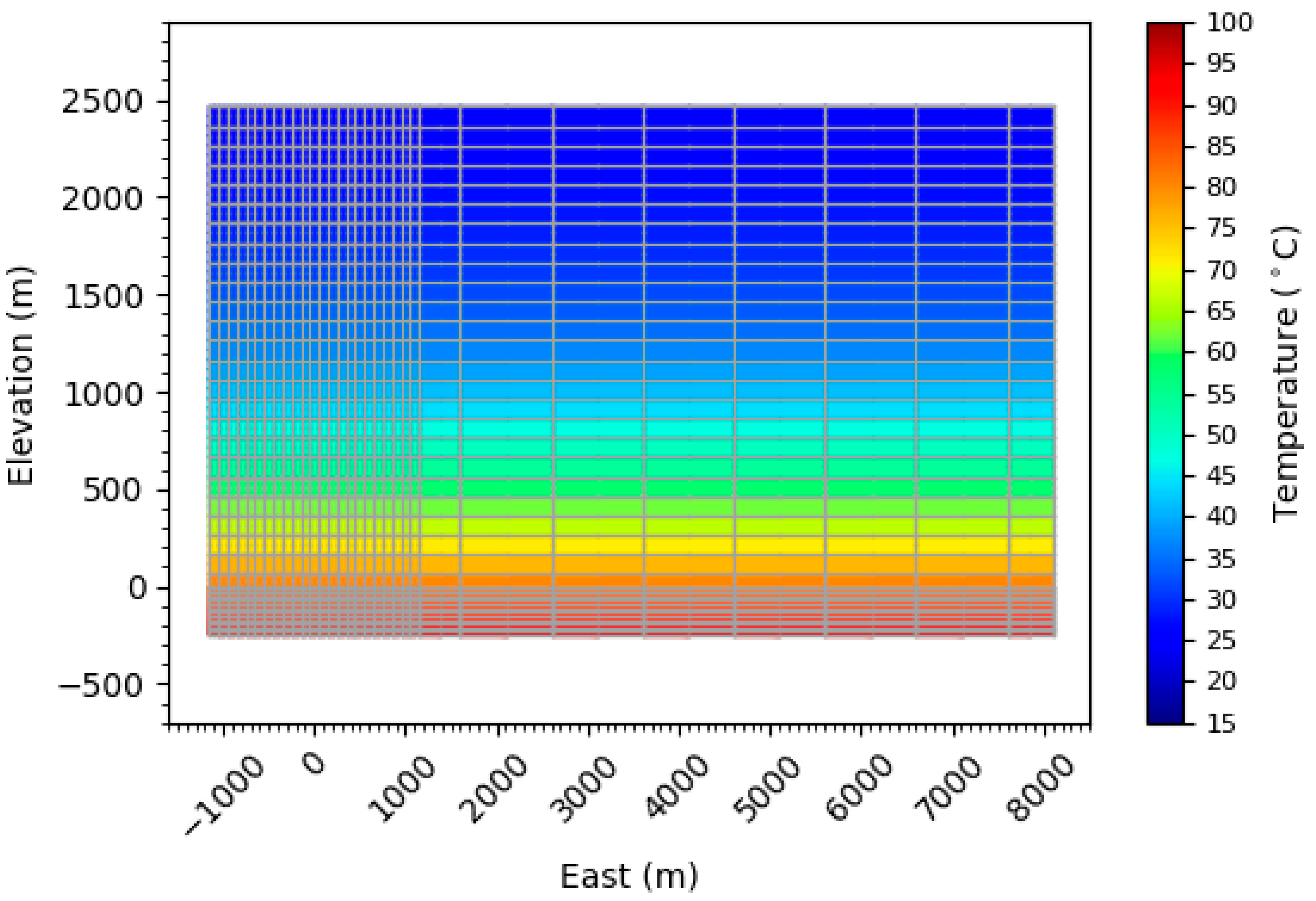






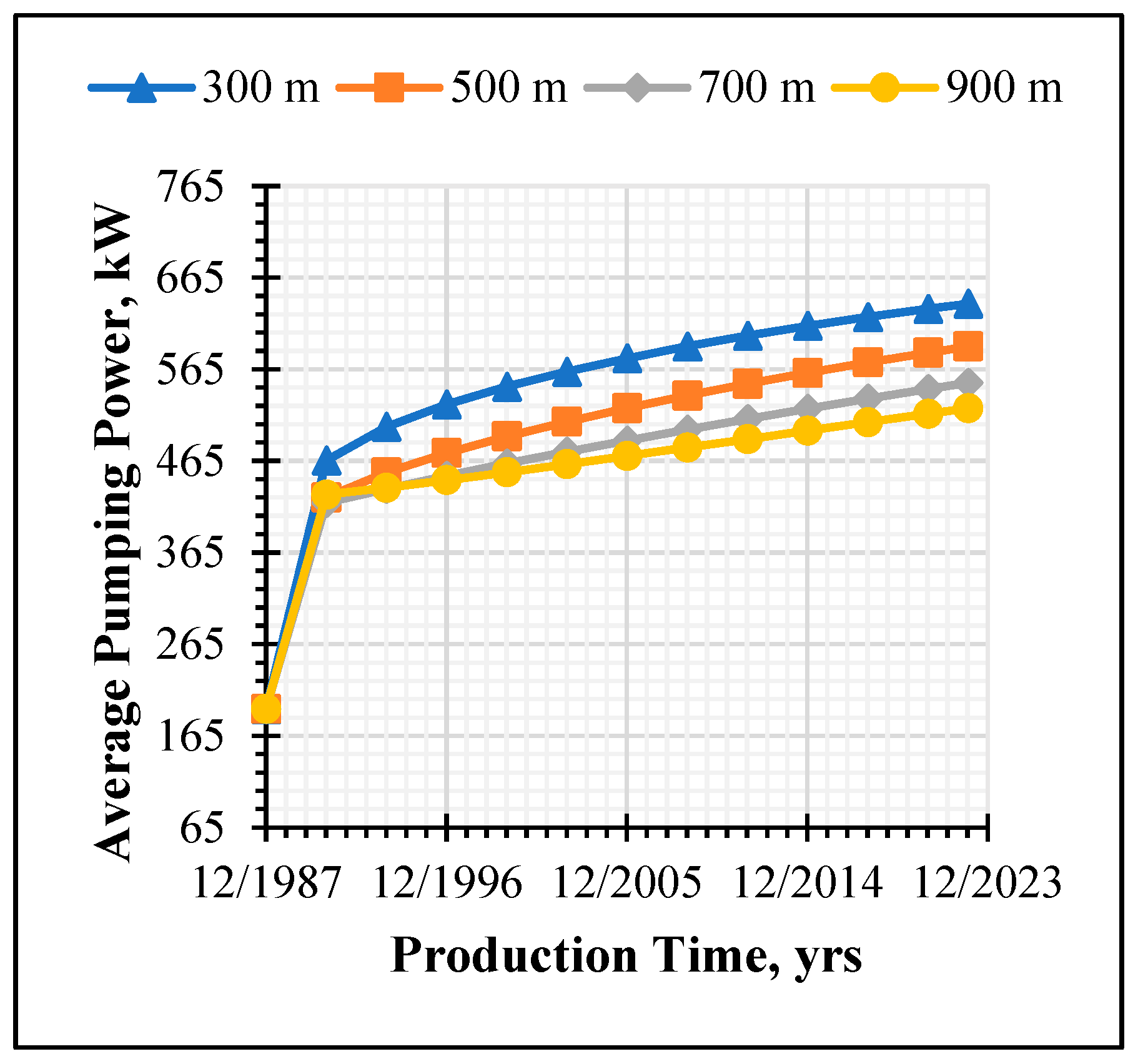
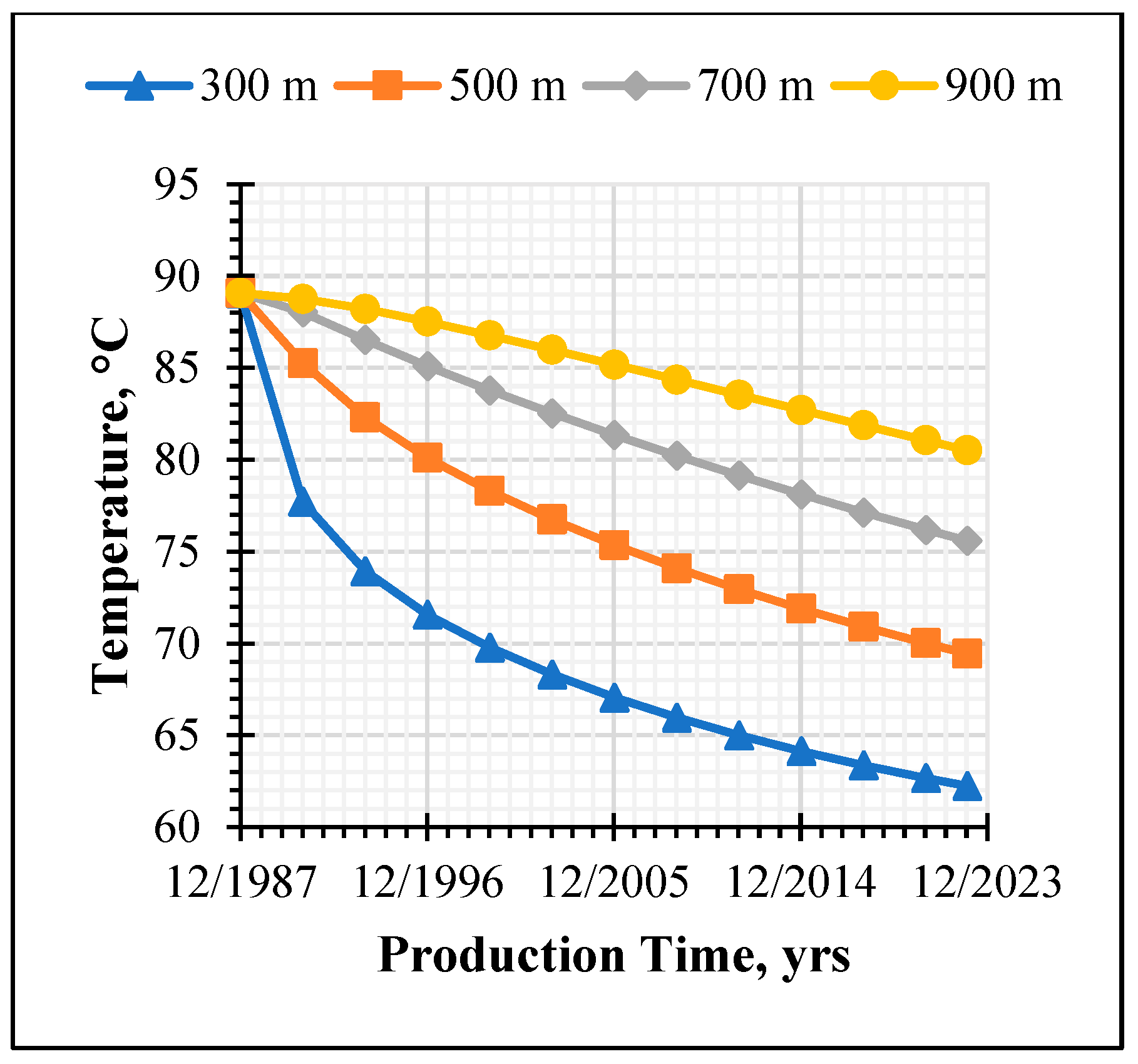

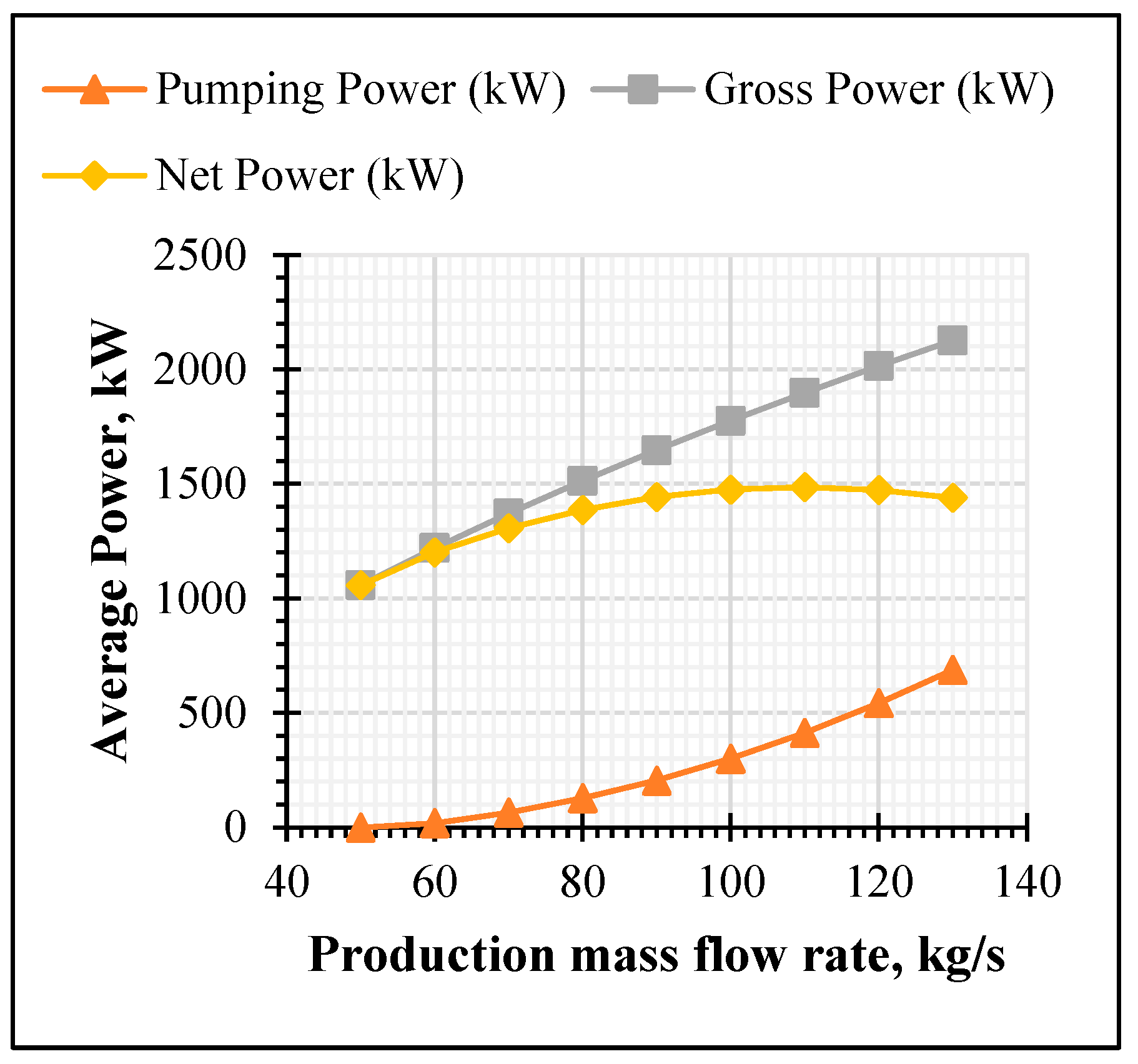
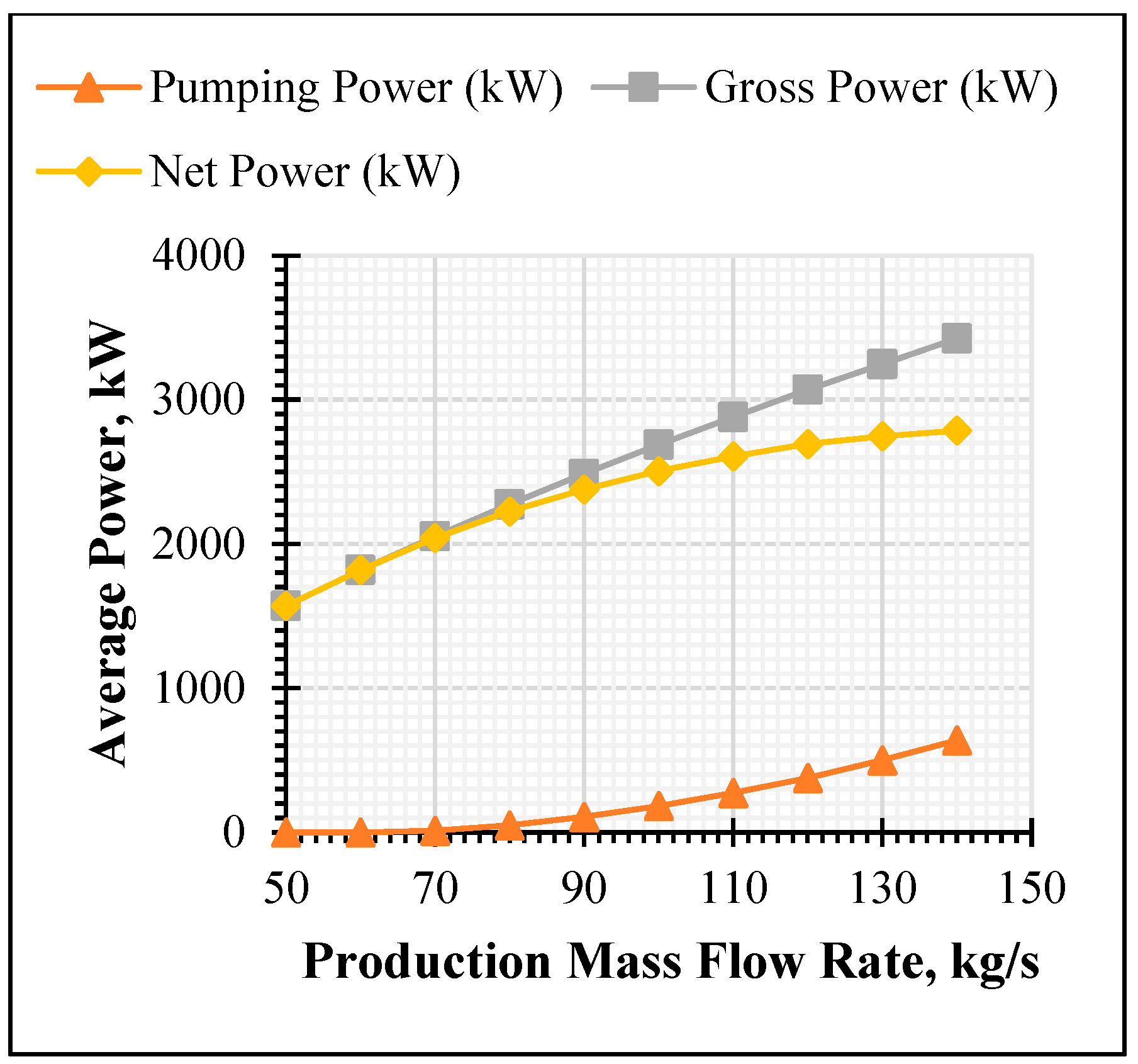

| Criteria | Waihapa Field | Ref |
|---|---|---|
| Maturity of the field | Long production history, infrastructure exists | [64,65,66] |
| High water cut | ~98% water content—ideal for coproduction | [64,65] |
| Reservoir temperature | 89 °C—viable for low-enthalpy geothermal extraction | [64] |
| Natural pressure support | Strong aquifer connectivity | [64] |
| Permeability and porosity | High permeability due to natural fractures (5 × 10−14 m2) | [64,67] |
| Available data | Detailed drilling, production, lithology data accessible | [64] |
| Reservoir depth | 2500 m—balanced for geothermal development | [64] |
| Infrastructure and proximity to grid | Near existing infrastructure and end-use locations | [5,68] |
| Unitised field | Easier to manage subsurface heat and fluid flow | [5,68] |
| Simple well geometry | Vertical wells (0.17 m dia), easy for simulating and modelling | [43,69,70] |
| Original-oil-in-place (OOIP) * | 5.08 × 106 m3 | [71] |
| Peak fluid production | 40 kg/s | [64] |
| * 1000 US barrels of oil = 158.9873 m3 |
| Element Size | No. of Elements | Production Temperature | Absolute Error |
|---|---|---|---|
| m | K | % | |
| 20 | 277,760 | 353.96 | 0.00 |
| 60 | 66,000 | 354.72 | 0.21 |
| 100 | 34,650 | 354.809 | 0.24 |
| Units | Reservoir Layer | Barrier Layer | Aquifer Layer | |
|---|---|---|---|---|
| Type | Minc3D | Porous | Porous | |
| Rock Properties | ||||
| Porosity | % | 0.2 | 0.2 | 0.25 |
| Density | kg/m3 | 2600 | 2600 | 2600 |
| Specific Heat | kJ/(kg · K) | 1 | 1 | 1 |
| Wet Heat Conductivity | W/(m · K) | 2 | 2 | 2 |
| Dry Heat Conductivity | W/(m · K) | 2 | 2 | 2 |
| Permeability | ||||
| kx | 1 × 10−14 m2 | 7 | 3 | 1 |
| ky | 1 × 10−14 m2 | 7 | 3 | 1 |
| kz | 1 × 10−14 m2 | 7 | 3 | 1 |
| Mass Flow Rate | Reservoir Depth | Resource Temperature | Well Productivity Index | Permeability-Thickness Product | Doublet Separation Distance | |
|---|---|---|---|---|---|---|
| kg/s | m | °C | (kg/s)/bar | mD-m | m | |
| Base Case | 100 | 2500 | 90 | 1.5 | 7.5 | 500 |
| Range | 50–150 | 2500 | 90–150 | 1.5 | 5.0–15.0 | 300–900 |
| S. No. | Oil and Gas Fields | |||
|---|---|---|---|---|
| Plant Name | Reservoir Temperature, °C | Flow Rate, kg/s | Net Installed Capacity, kW | |
| 1. | Teapot Dome Oilfield, Wyoming, US (Naval Petroleum Reserve No. 3) | ~110 | 90 | 250 |
| 2. | Huabei Oilfield, China (LB oil reservoir) | 120 | 33.3 | 400 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Duggal, R.; Burnell, J.; Hinkley, J.; Ward, S.; Wieland, C.; Massier, T.; Rayudu, R. Modelling Geothermal Energy Extraction from Low-Enthalpy Oil and Gas Fields Using Pump-Assisted Production: A Case Study of the Waihapa Oilfield. Sustainability 2025, 17, 4669. https://doi.org/10.3390/su17104669
Duggal R, Burnell J, Hinkley J, Ward S, Wieland C, Massier T, Rayudu R. Modelling Geothermal Energy Extraction from Low-Enthalpy Oil and Gas Fields Using Pump-Assisted Production: A Case Study of the Waihapa Oilfield. Sustainability. 2025; 17(10):4669. https://doi.org/10.3390/su17104669
Chicago/Turabian StyleDuggal, Rohit, John Burnell, Jim Hinkley, Simon Ward, Christoph Wieland, Tobias Massier, and Ramesh Rayudu. 2025. "Modelling Geothermal Energy Extraction from Low-Enthalpy Oil and Gas Fields Using Pump-Assisted Production: A Case Study of the Waihapa Oilfield" Sustainability 17, no. 10: 4669. https://doi.org/10.3390/su17104669
APA StyleDuggal, R., Burnell, J., Hinkley, J., Ward, S., Wieland, C., Massier, T., & Rayudu, R. (2025). Modelling Geothermal Energy Extraction from Low-Enthalpy Oil and Gas Fields Using Pump-Assisted Production: A Case Study of the Waihapa Oilfield. Sustainability, 17(10), 4669. https://doi.org/10.3390/su17104669









