Nonlinear Dynamic Analysis of High-Strength Concrete Bridges under Post-Fire Earthquakes Considering Hydrodynamic Effects
Abstract
1. Introduction
2. Engineering Conditions
3. Earthquake Selection
4. Theory of Hydrodynamic Temperature Effects
4.1. Theory of the Hydrodynamic Effects
4.2. Theory of the Temperature Effect
5. Results
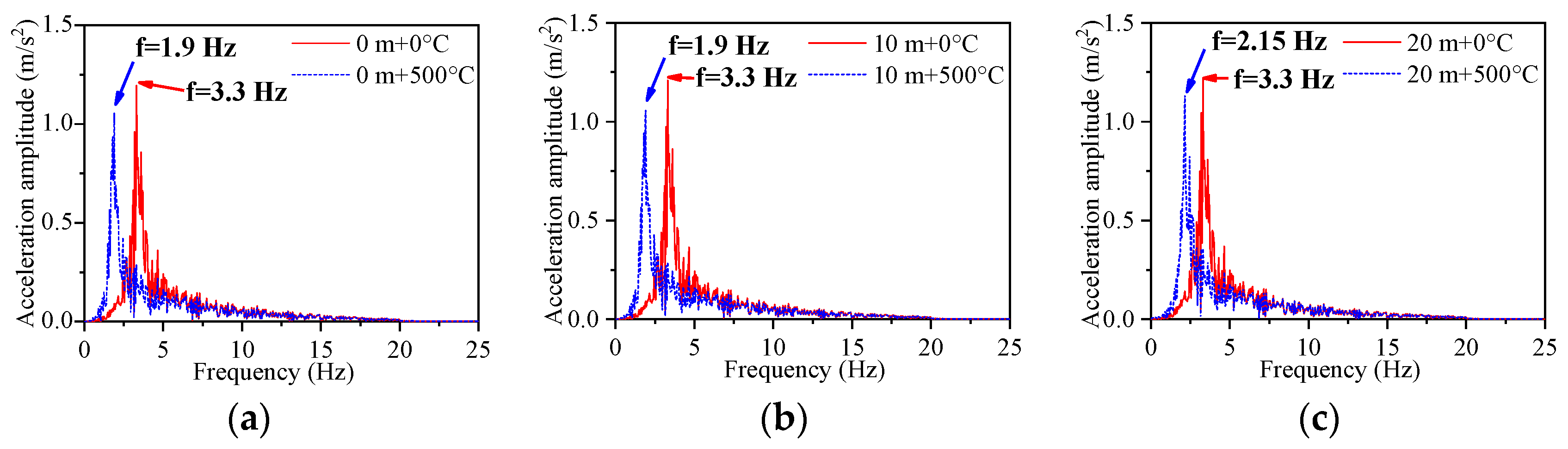
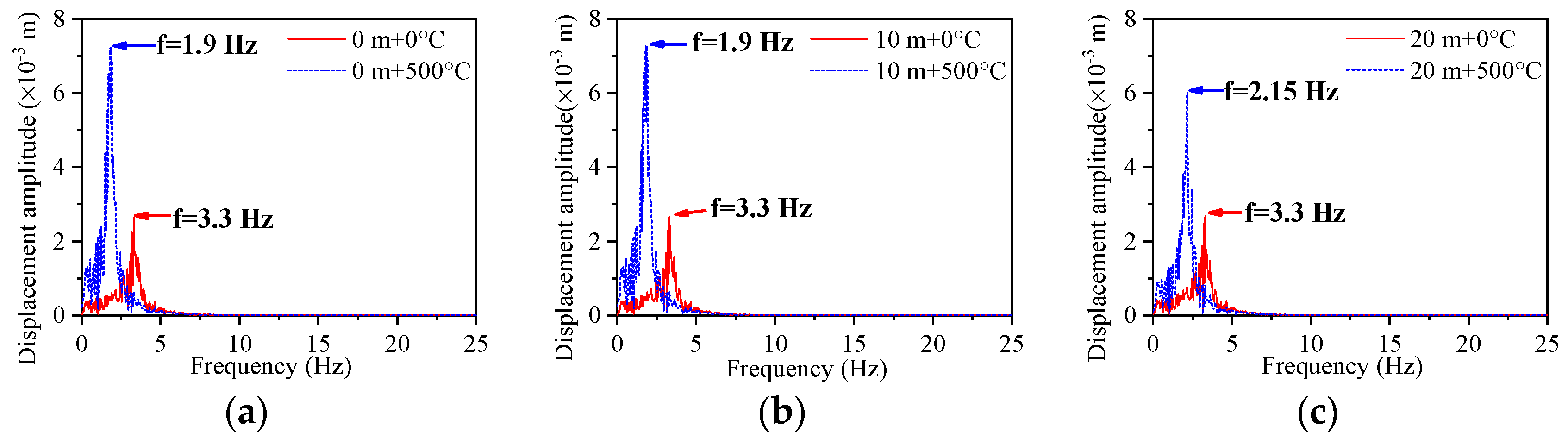
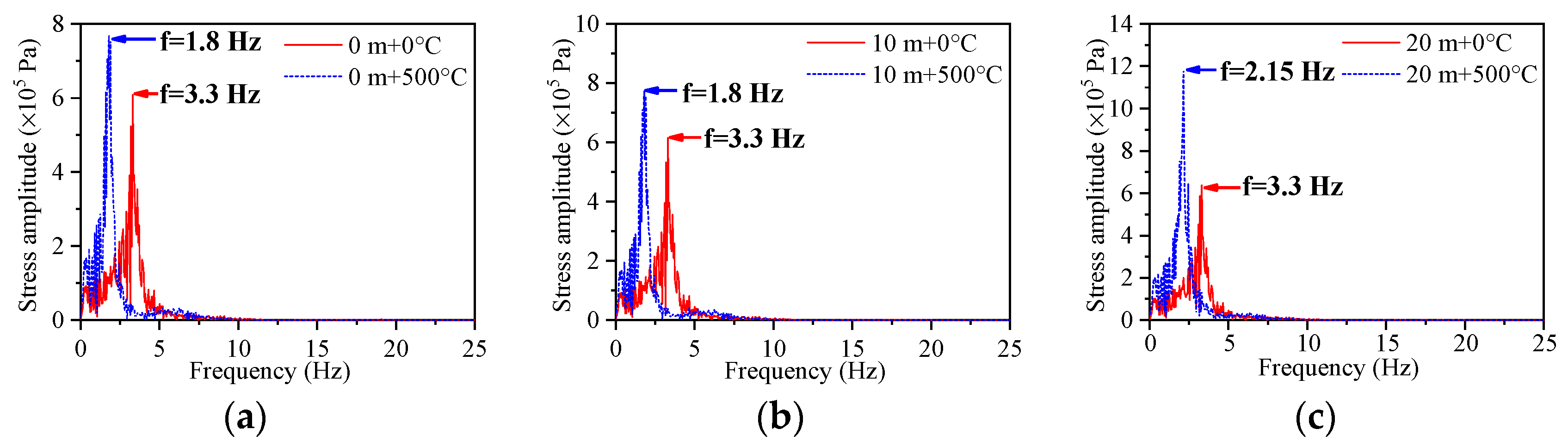
5.1. The Frequency Analysis
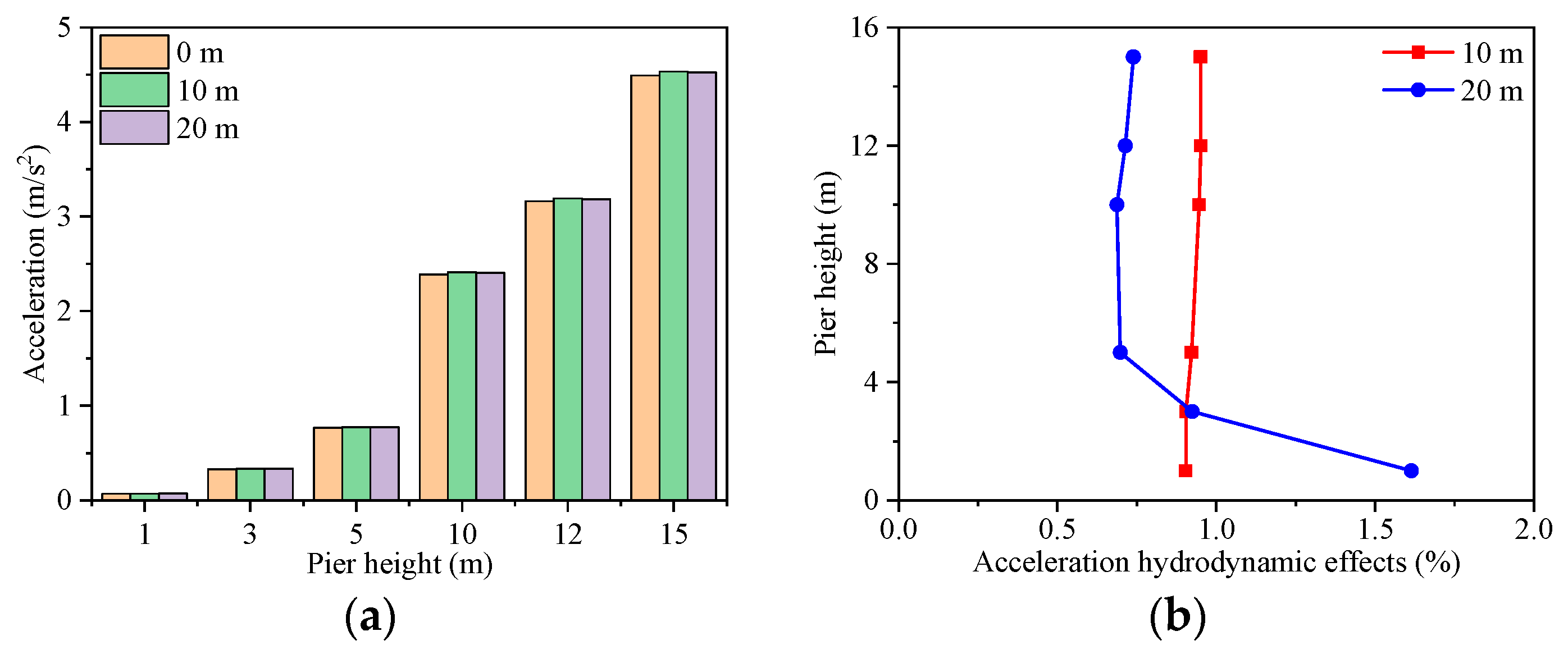
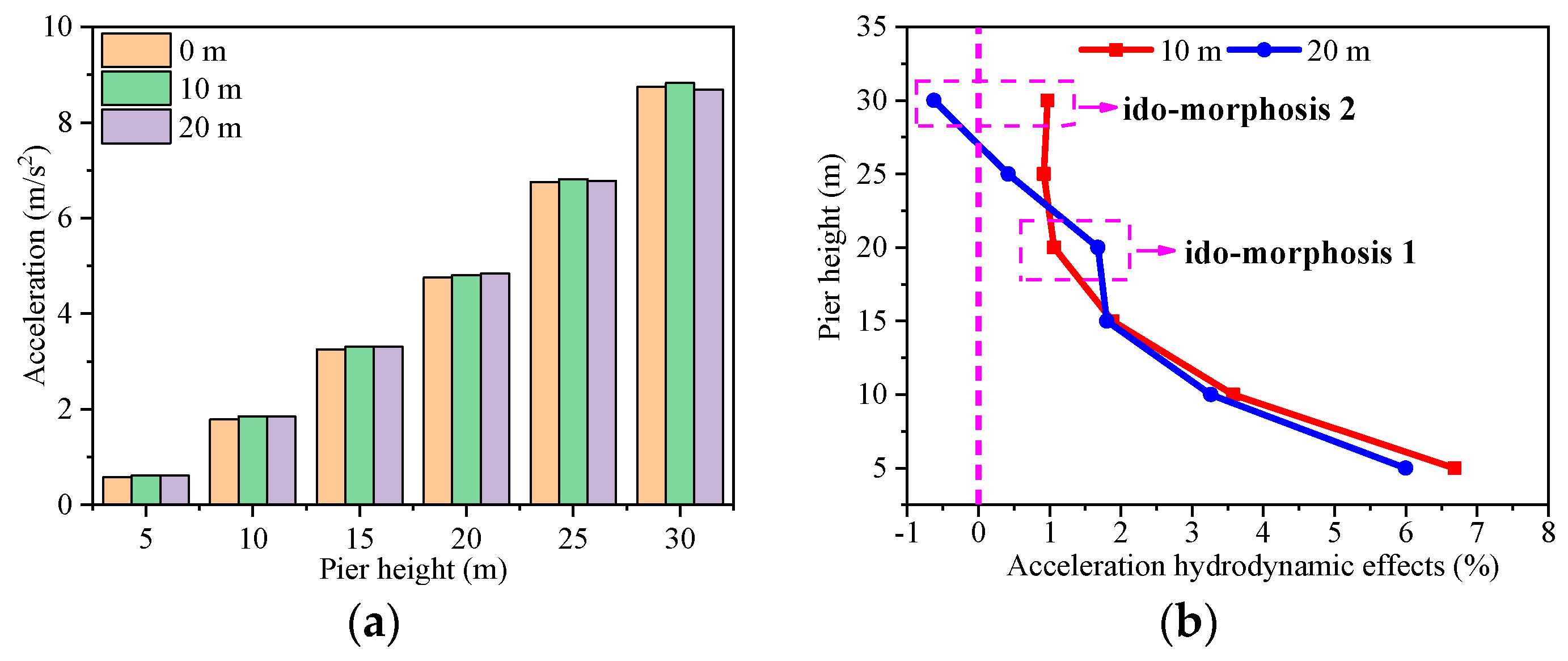
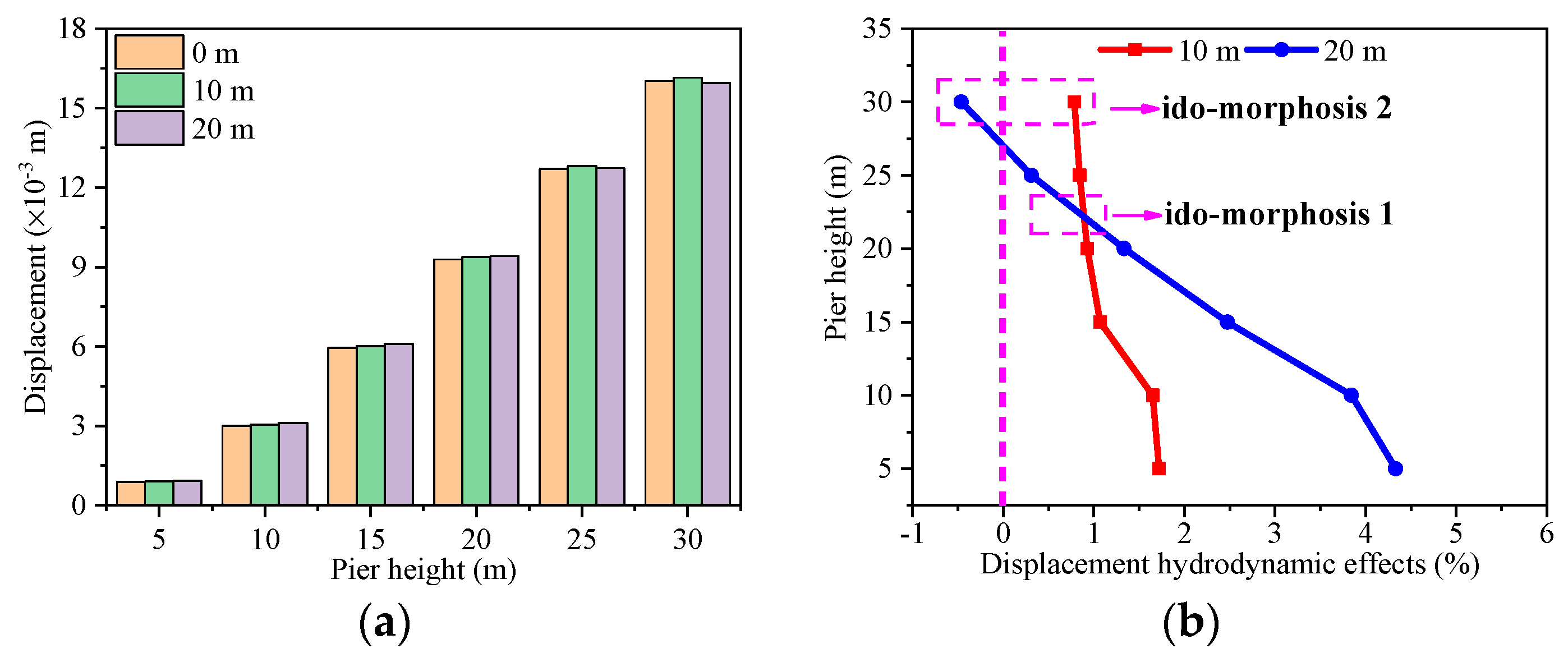
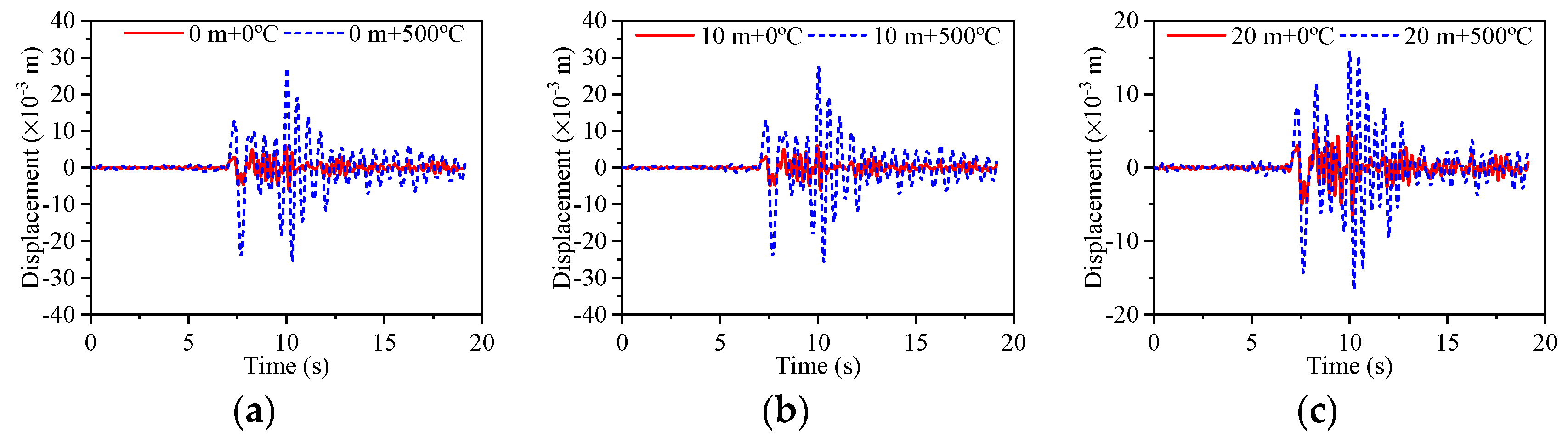
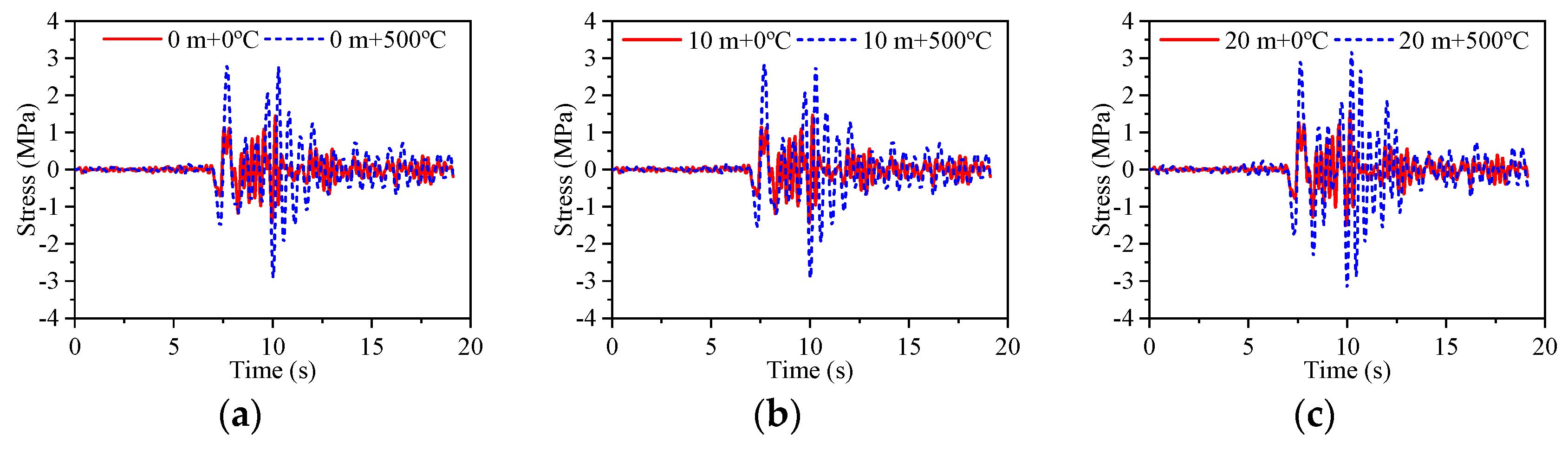
5.2. The Hydrodynamic Effects Analysis
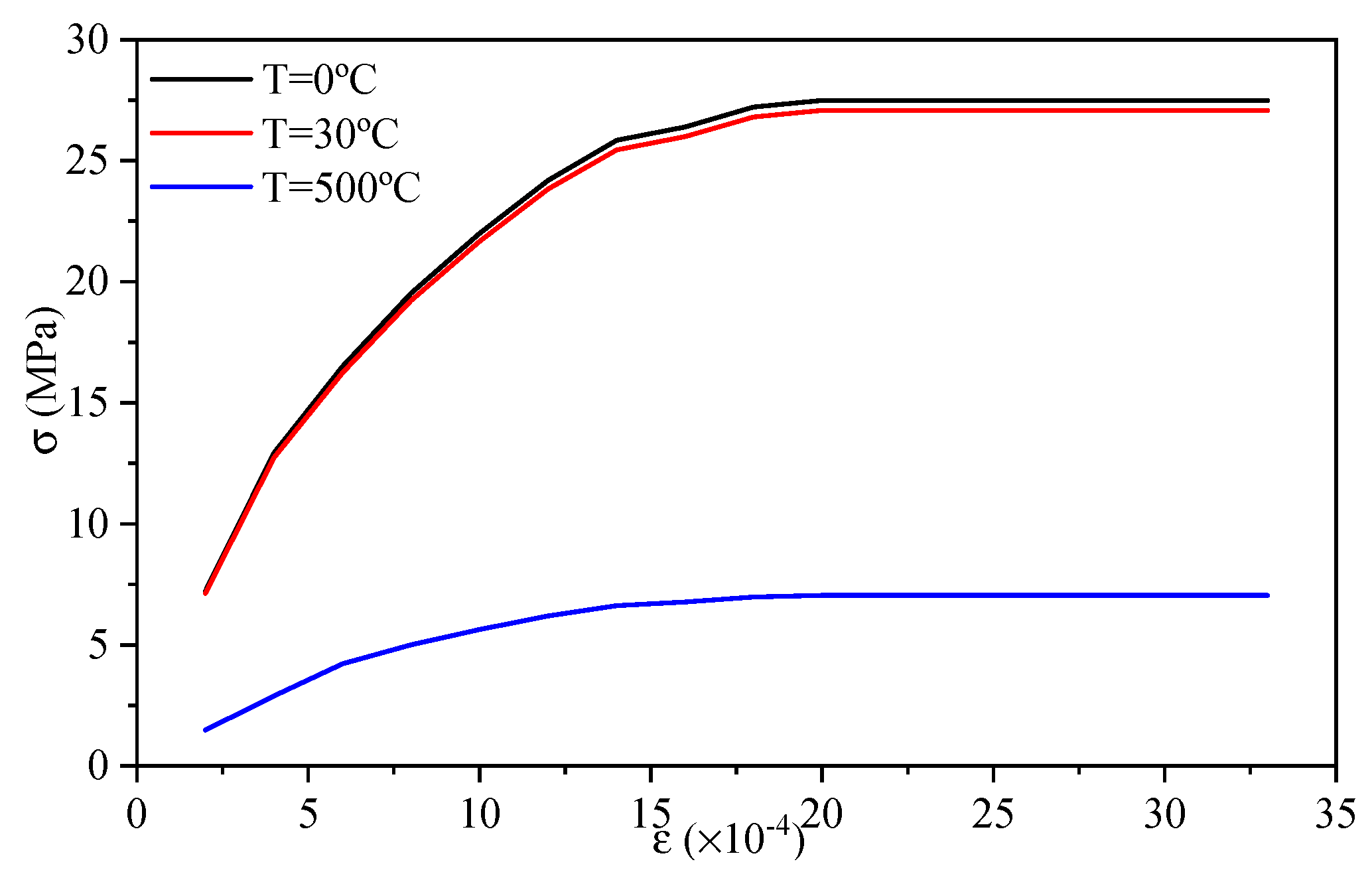
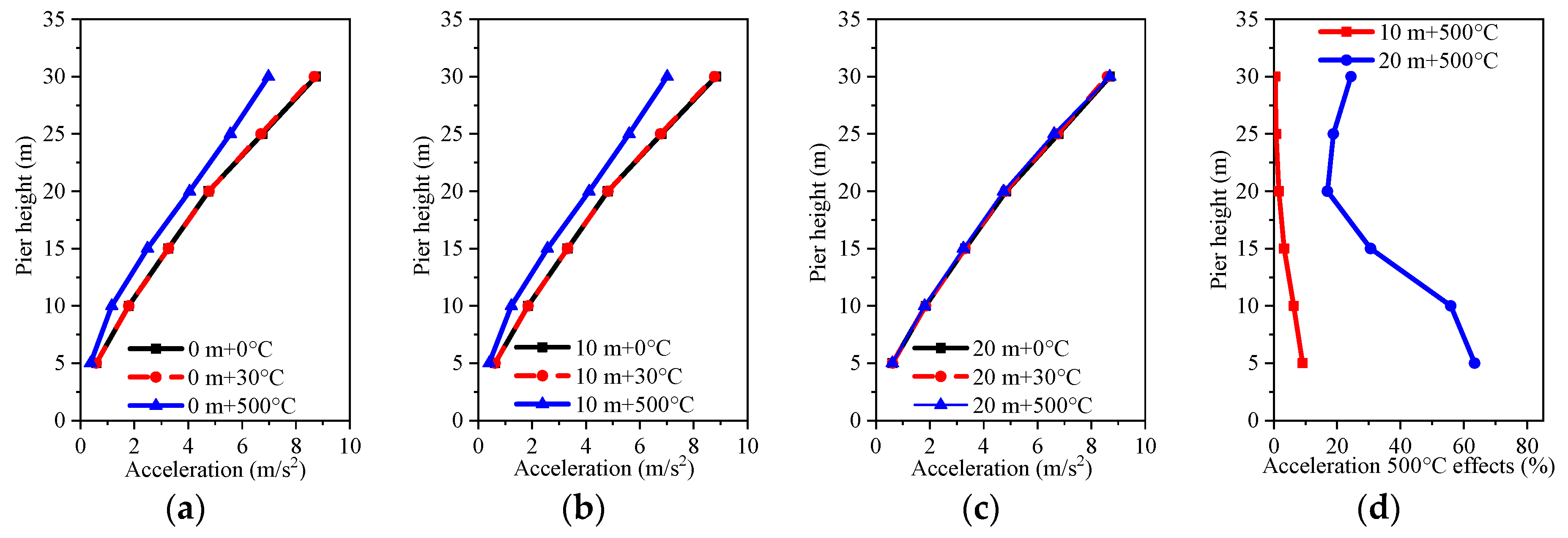
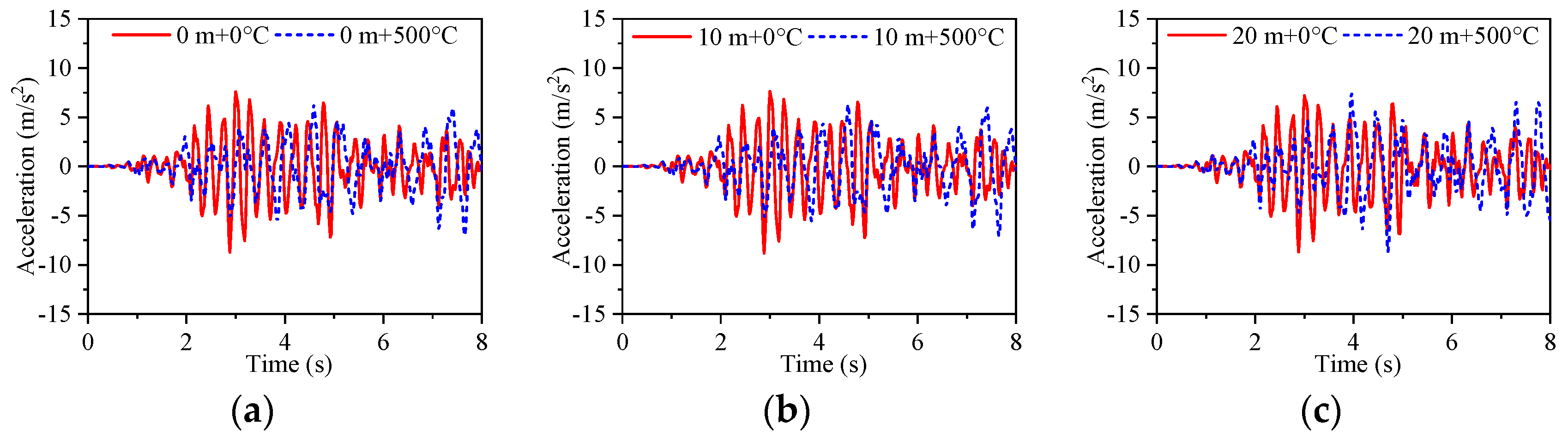
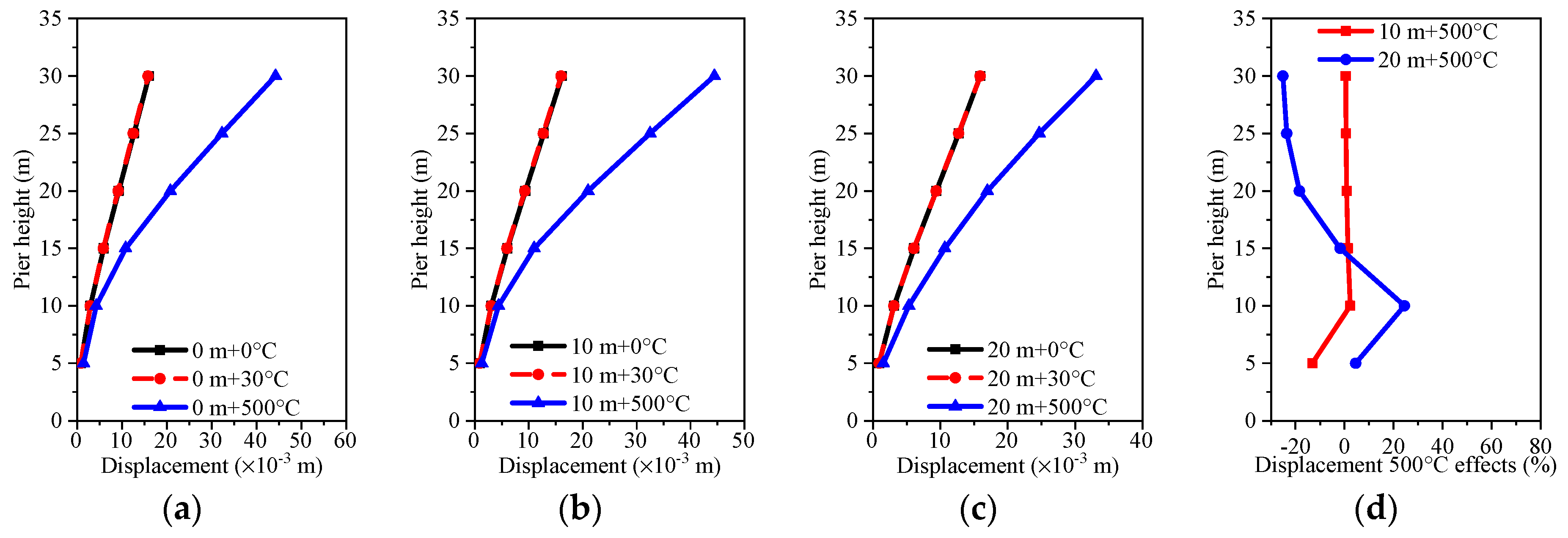
5.3. Analysis of the Temperature Effects
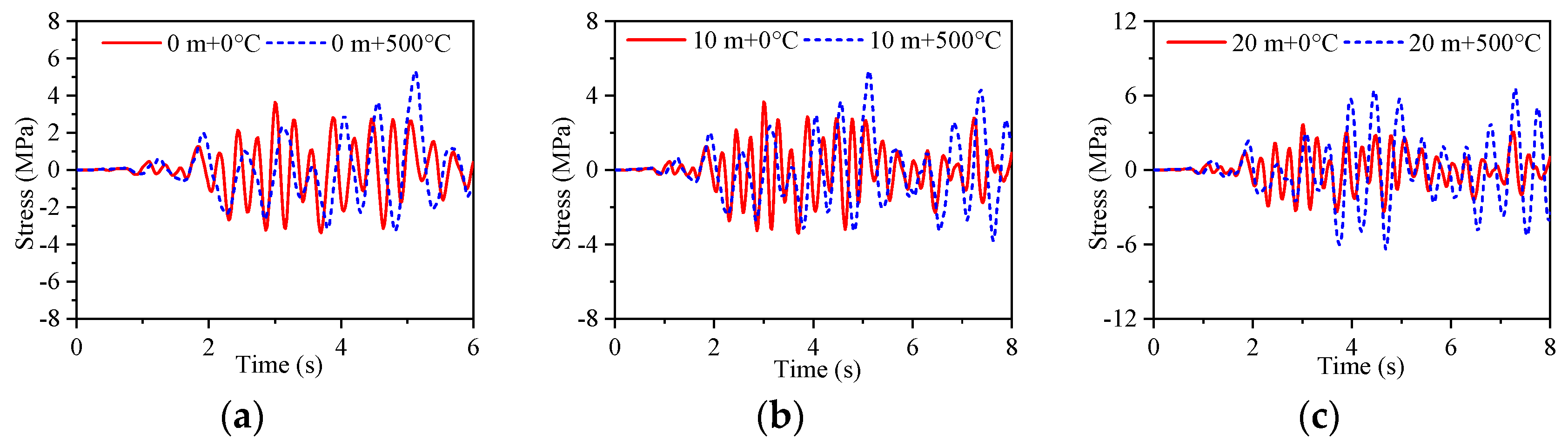
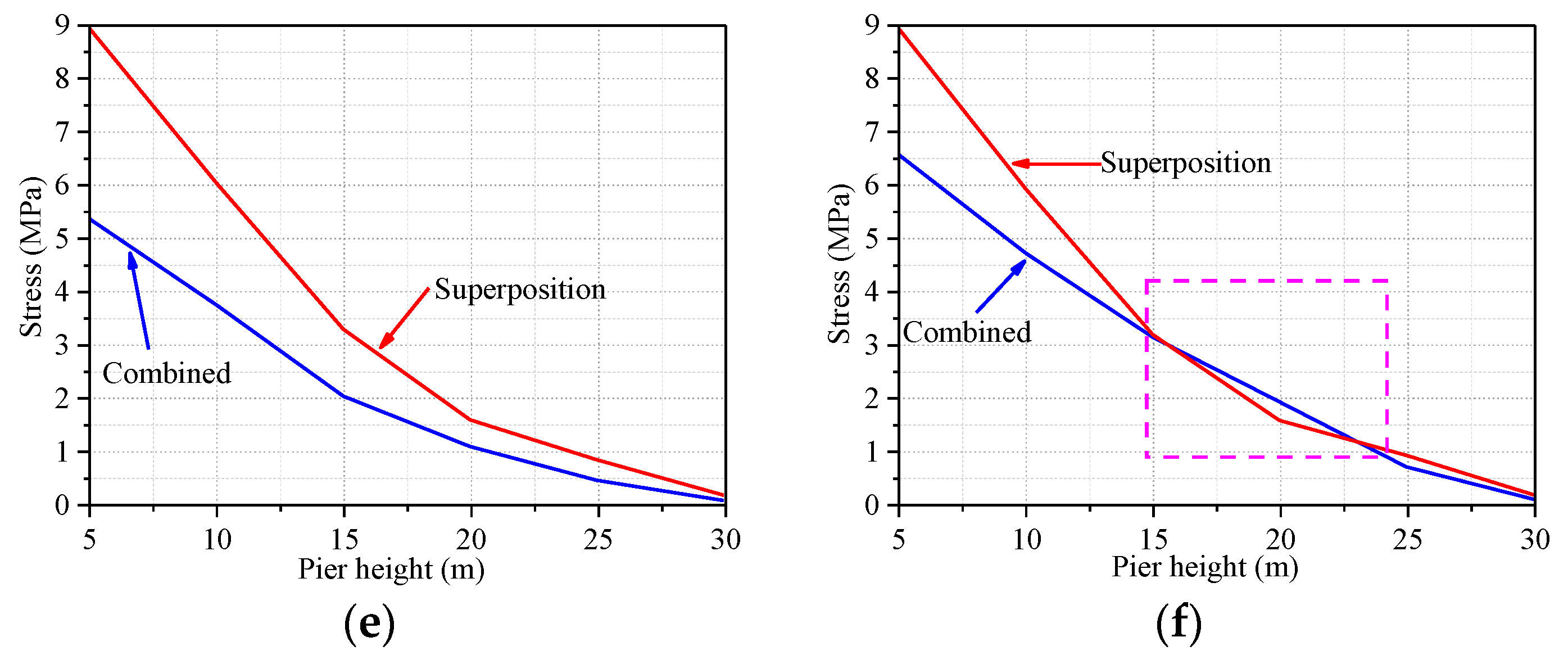
5.4. Superposition and Combined Action of Hydrodynamic Effects and Temperature Effects
6. Conclusions and Discussion
- (1)
- The mechanical properties of high-strength concrete and common concrete differ with respect to temperature. Before the critical temperature is reached, the rate of reduction in the strength and elastic modulus of common concrete with increasing temperature changes is greater than that of high-strength concrete. When the critical temperature is exceeded, the reduction rate of common concrete is significantly lower than that of high-strength concrete.
- (2)
- The various water levels and earthquake waves have different influences on the dynamic characteristics and dynamic response of the bridge structure.
- (3)
- When the temperature is between 0 °C and 30 °C, the temperature effect has less of an effect on the dynamic value analysis of the bridge structure. When the temperature is 500 °C, the acceleration response value decreases, but the displacement response value and stress response value of the pier structure increase. The temperature effect changes the influence law of the hydrodynamic effect on the structure at different water levels. At water levels between 0 m and 10 m, the temperature effect reduces the fundamental frequency of acceleration and displacement responses by 1.4 Hz, and decreases the fundamental frequency of stress responses by 1.5 Hz. At a water level of 20 m, the temperature effect lowers the fundamental frequency of acceleration, displacement, and stress responses by 1.15 Hz. The temperature effect on the structure is greater than the hydrodynamic effect.
- (4)
- When under the action of the Tianjin earthquake, taking the acceleration and displacement at the pier top and stress at the pier base as examples, at a 10 m water level, the superposition effects of temperature and hydrodynamic effects increase the acceleration, displacement, and stress by 2.3172 m/s2, 0.007 m, and 1.4443 MPa, respectively, compared to the combined effects. At a 20 m water level, compared to the combined effects of temperature and hydrodynamic effects, these values increase by 3.0577 m/s2, 0.017 m, and 4.0721 MPa, respectively. When the hydrodynamic effect and the temperature effect combine on the bridge structure, it cannot simply be considered a linear superposition when the hydrodynamic effect and temperature effect act separately. The interaction between the hydrodynamic effect and the temperature effect is extremely complicated.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yun, G.; Liu, C. Shaking table tests on a deep-water high-pier whole bridge under joint earthquake, wave and current action. Appl. Ocean Res. 2020, 103, 102329. [Google Scholar] [CrossRef]
- Yun, G.; Liu, C. A model for underwater shaking table tests on the basis of different similar criteria. Appl. Ocean Res. 2022, 118, 103010. [Google Scholar] [CrossRef]
- Kodur, V.; Naser, M.Z. Fire hazard in transportation infrastructure: Review, assessment, and mitigation strategies. Front. Struct. Civ. Eng. 2021, 15, 46–60. [Google Scholar] [CrossRef]
- Crespi, P.; Zucca, M.; Valente, M. On the collapse evaluation of existing RC bridges exposed to corrosion under horizontal loads. Eng. Fail. Anal. 2020, 116, 104727. [Google Scholar] [CrossRef]
- Crespi, P.; Zucca, M.; Valente, M.; Longarini, N. Influence of corrosion effects on the seismic capacity of existing RC bridges. Eng. Fail. Anal. 2022, 140, 106546. [Google Scholar] [CrossRef]
- Wang, Z.; Ge, J.; Wei, H. Seismic performance of precast hollow bridge piers with different construction details. Front. Struct. Civ. Eng. 2014, 8, 399–413. [Google Scholar] [CrossRef]
- Huang, M.; Guo, W.; Zhu, H.; Li, L. Dynamic test and finite element model updating of bridge structures based on ambient vibration. Front. Archit. Civ. Eng. China 2008, 2, 139–144. [Google Scholar] [CrossRef]
- Wu, K.; Ma, J.; Chen, Y.; Huang, X.; Qi, L.; Li, C. Study on the hydrodynamic added mass of rectangular pier under sine wave actions. Structures 2023, 54, 1371–1380. [Google Scholar] [CrossRef]
- Liaw, C.Y.; Chopra, A.K. Dynamics of towers surrounded by water. Earthq. Eng. Struct. Dyn. 1974, 3, 33–49. [Google Scholar] [CrossRef]
- Wang, P.; Zhao, M.; Li, H.; Du, X. An accurate and efficient time-domain model for simulating water-cylinder dynamic interaction during earthquakes. Eng. Struct. 2018, 166, 263–273. [Google Scholar] [CrossRef]
- Wang, P.; Zhao, M.; Du, X. Simplified Formula for Earthquake-Induced Hydrodynamic Pressure on Round-Ended and Rectangular Cylinders Surrounded by Water. J. Eng. Mech. 2019, 145, 04018137. [Google Scholar] [CrossRef]
- Wang, P.; Zhao, M.; Du, X.; Liu, J. Dynamic Response of Bridge Pier under Combined Earthquake and Wave–Current Action. J. Bridge Eng. 2019, 24, 04019095. [Google Scholar] [CrossRef]
- Liang, F.; Liang, X.; Wang, C. Simplified Added-Mass Model for Evaluating the Response of Rectangular Hollow Bridge Piers under Earthquakes. J. Bridge Eng. 2021, 26, 04021076. [Google Scholar] [CrossRef]
- Guo, J.; Zhao, M.; Wang, P.; Zhang, N. Comparative assessment of simplified methods for hydrodynamic force on cylinder under earthquakes. Ocean Eng. 2021, 234, 109219. [Google Scholar] [CrossRef]
- Liu, C.; Zhang, S.; Hao, E. Joint earthquake, wave and current action on the pile group cable-stayed bridge tower foundation: An experimental study. Appl. Ocean Res. 2017, 63, 157–169. [Google Scholar] [CrossRef]
- Ding, Y.; Ma, R.; Shi, Y.; Li, Z. Underwater shaking table tests on bridge pier under combined earthquake and wave-current action. Mar. Struct. 2018, 58, 301–320. [Google Scholar] [CrossRef]
- Qi, Q.; Shao, C.; Wei, W.; Xiao, Z.; He, J. Seismic performance of railway rounded rectangular hollow tall piers using the shaking table test. Eng. Struct. 2020, 220, 110968. [Google Scholar] [CrossRef]
- Nariman, N.A. Thermal fluid-structure interaction and coupled thermal-stress analysis in a cable stayed bridge exposed to fire. Front. Struct. Civ. Eng. 2018, 12, 609–628. [Google Scholar] [CrossRef]
- Zheng, X.; Jian, J.; Liu, L.; Sun, B.; Zhang, K.; Gao, H. Static and dynamic performance analysis of cable-stayed bridges with cables damaged fire. Buildings 2024, 14, 884. [Google Scholar] [CrossRef]
- Paya-Zaforteza, I.; Zmigrodzki, S.; Stochino, F. From flames to resilience: New perspectives for the design of structures against fire hazards. Struct. Eng. Int. 2023, 33, 547. [Google Scholar] [CrossRef]
- Sohn, H.; Dzwonczyk, M.; Straser, E.G.; Kiremidjian, A.S.; Law, K.H.; Meng, T. An experimental study of temperature effect on modal parameters of the Alamosa Canyon Bridge. Earthq. Eng. Struct. Dyn. 1999, 28, 879–897. [Google Scholar] [CrossRef]
- Xiao, J.-Z.; Li, J.; Jiang, F. Research on the seismic behavior of HPC shear walls after fire. Mater. Struct. 2004, 37, 506–512. [Google Scholar] [CrossRef]
- Mazza, F. Effects of near-fault vertical earthquakes on the nonlinear incremental response of r.c. base-isolated structures exposed to fire. Bull. Earthq. Eng. 2015, 14, 433–454. [Google Scholar] [CrossRef]
- Mazza, F. Wind and earthquake dynamic responses of fire-exposed steel framed structures. Soil Dyn. Earthq. Eng. 2015, 78, 218–229. [Google Scholar] [CrossRef]
- Ni, S.; Birely, A.C. Local and global response data from post-fire earthquake simulations of RC structural walls. Data Brief 2018, 19, 1650–1657. [Google Scholar] [CrossRef] [PubMed]
- Ni, S.; Birely, A.C. Simulation procedure for the post-fire seismic analysis of reinforced concrete structural walls. Fire Saf. J. 2018, 95, 101–112. [Google Scholar] [CrossRef]
- Ni, S.; Birely, A.C. A simplified model for the post-fire earthquake flexural response of reinforced concrete walls with boundary elements. Eng. Struct. 2018, 175, 721–730. [Google Scholar] [CrossRef]
- Li, L.; Liu, X.; Yu, J.-T.; Lu, Z.-D.; Su, M.-N.; Liao, J.-H.; Xia, M. Experimental study on seismic performance of post-fire reinforced concrete frames. Eng. Struct. 2019, 179, 161–173. [Google Scholar] [CrossRef]
- Li, W.; Wang, T.; Han, L.-H. Seismic performance of concrete-filled double-skin steel tubes after exposure to fire: Experiments. J. Constr. Steel Res. 2019, 154, 209–223. [Google Scholar] [CrossRef]
- Li, S.; Han, L.-H.; Wang, F.-C.; Hou, C.-C. Seismic behavior of fire-exposed concrete-filled steel tubular (CFST) columns. Eng. Struct. 2020, 224, 111085. [Google Scholar] [CrossRef]
- Wei, X.; Ngeljaratan, L.; Bruneau, M. Low-Cycle Fatigue of Buckling Restrained Braces in Bidirectional Ductile End Diaphragms Due to Temperature-Change Effect on Bridge Superstructure. J. Bridge Eng. 2019, 24, 04019014. [Google Scholar] [CrossRef]
- Bayane, I.; Mankar, A.; Brühwiler, E.; Sørensen, J.D. Quantification of traffic and temperature effects on the fatigue safety of a reinforced-concrete bridge deck based on monitoring data. Eng. Struct. 2019, 196, 109357. [Google Scholar] [CrossRef]
- Sernizon Costa, R.; Lavall, A.C.C.; da Silva, R.G.L.; dos Santos, A.P.; Viana, H.F. Cable structures: An exact geometric analysis using catenary curve and considering the material nonlinearity and temperature effect. Eng. Struct. 2022, 253, 113738. [Google Scholar] [CrossRef]
- Sun, L.; Zhou, Y.; Min, Z. Experimental Study on the Effect of Temperature on Modal Frequencies of Bridges. Int. J. Struct. Stab. Dyn. 2018, 18, 1850155. [Google Scholar] [CrossRef]
- Tran, H.; Robert, D.; Gunarathna, P.; Setunge, S. Estimating cost of bridge closure for bridge network rehabilitation priorities. J. Constr. Eng. Manag. 2024, 150, 04024071. [Google Scholar] [CrossRef]
- Wang, X. Ansys Numerical Analysis of Engineering Structures; China Communications Press: Beijing, China, 2007. (In Chinese) [Google Scholar]
- GB 18306-2015; National Standards of People’s Republic of China. Seismic Ground Motion Parameters Zonation Map of China. China Standard Press: Beijing, China, 2015. (In Chinese)
- Pan, C.; Zhang, R. A Useful Tool to Process and Generate Earthquake Signals [CP/OL]. Available online: www.eqsignal.com (accessed on 1 January 2022).
- Pan, C.; Zhang, R.; Luo, H.; Shen, H. Baseline correction of vibration acceleration signals with inconsistent initial velocity and displacement. Adv. Mech. Eng. 2016, 8, 1687814016675534. [Google Scholar] [CrossRef]
- Pan, C.; Zhang, R.; Luo, H.; Shen, H. Target-based algorithm for baseline correction of inconsistent vibration signals. J. Vib. Control 2017, 24, 2562–2575. [Google Scholar] [CrossRef]
- Former Ministry of Urban and Rural Building Environmental Protection of the People’s Republic of China. Code for Seismic Design of Building; China Construction Industry Press: Beijing, China, 2010. (In Chinese) [Google Scholar]
- Yun, G.; Liu, C. Nonlinear dynamic analysis of the deep-water bridge piers under combined earthquakes and wave actions. Ocean Eng. 2022, 261, 112076. [Google Scholar] [CrossRef]
- Morison, J.R.; Johnson, J.W.; Schaaf, S.A. The force exerted by surface waves on piles. J. Pet. Technol. 1950, 2, 149–154. [Google Scholar] [CrossRef]
- Li, Q.; Yang, W. An improved method of hydrodynamic pressure calculation for circular hollow piers in deep water under earthquake. Ocean Eng. 2013, 72, 241–256. [Google Scholar] [CrossRef]
- Yang, W.; Li, Q. The expanded Morison equation considering inner and outer water hydrodynamic pressure of hollow piers. Ocean Eng. 2013, 69, 79–87. [Google Scholar] [CrossRef]
- Yang, W.; Li, A.; Feng, X.; Deng, L.; Li, F. Calculation method of hydrodynamic force on one column of the twin columns under earthquake. Ocean Eng. 2020, 197, 106874. [Google Scholar] [CrossRef]
- Penzien, J.; Kaul, M.K.; Berge, B. Stochastic response of offshore towers to random sea waves and strong motion earthquakes. Comput. Struct. 1972, 2, 733–756. [Google Scholar] [CrossRef]
- Penzien, J.; Kaul, M.K. Response of offshore towers to strong motion earthquakes. Earthq. Eng. Struct. Dyn. 1972, 1, 55–68. [Google Scholar] [CrossRef]
- Song, B.; Zheng, F.; Li, Y. Study on a Simplified Calculation Method for Hydrodynamic Pressure to Slender Structures Under Earthquakes. J. Earthq. Eng. 2013, 17, 720–735. [Google Scholar] [CrossRef]
- Pang, Y.; Cai, L.; He, W.; Wu, L. Seismic assessment of deep water bridges in reservoir considering hydrodynamic effects using endurance time analysis. Ocean Eng. 2020, 198, 106846. [Google Scholar] [CrossRef]
- Huang, L. Great span concrete bridge temperature effect formula summary. China Water Transp. Theor. Ed. 2007, 5, 46–47. (In Chinese) [Google Scholar]
- Xiang, S.; Wang, D.; Yang, L.; Tan, B. Study on the life cycle simulation method of the temperature field and temperature effect of a steel–concrete composite bridge deck system. Meas. Control 2021, 54, 1068–1081. [Google Scholar] [CrossRef]
- Wu, B.; Yuan, J.; Wang, G. Experimental study on mechanical properties of high strngth concrete after high temperature. Chin. Civ. Eng. J. 2000, 33, 8–12+34. (In Chinese) [Google Scholar]
- Xiao, J.; König, G. Study on concrete at high temperature in China—An overview. Fire Saf. J. 2004, 39, 89–103. [Google Scholar] [CrossRef]
- Wu, B.; Ma, Z.; Ou, J. Experimental Research on Deformation and Constitutive Relationship of Concrete Under Axial Loading and High Temperature. J. Build. Struct. 1999, 20, 42–49. (In Chinese) [Google Scholar]
- Li, W.; Guo, Z. Experimental investigation of strength and deformation of Concrete at elevated temperature. J. Build. Struct. 1993, 14, 8–16. (In Chinese) [Google Scholar]
- Chang, Y.F.; Chen, Y.H.; Sheu, M.S.; Yao, G.C. Residual stress–strain relationship for concrete after exposure to high temperatures. Cem. Concr. Res. 2006, 36, 1999–2005. [Google Scholar] [CrossRef]
- Jing, R.; Kang, J.; Jiang, Y. Influence of temperature rising by 20 °C to 50 °C on concrete elastic modulus. Bull. Chin. Ceram. Soc. 2016, 35, 4207–4211. (In Chinese) [Google Scholar]


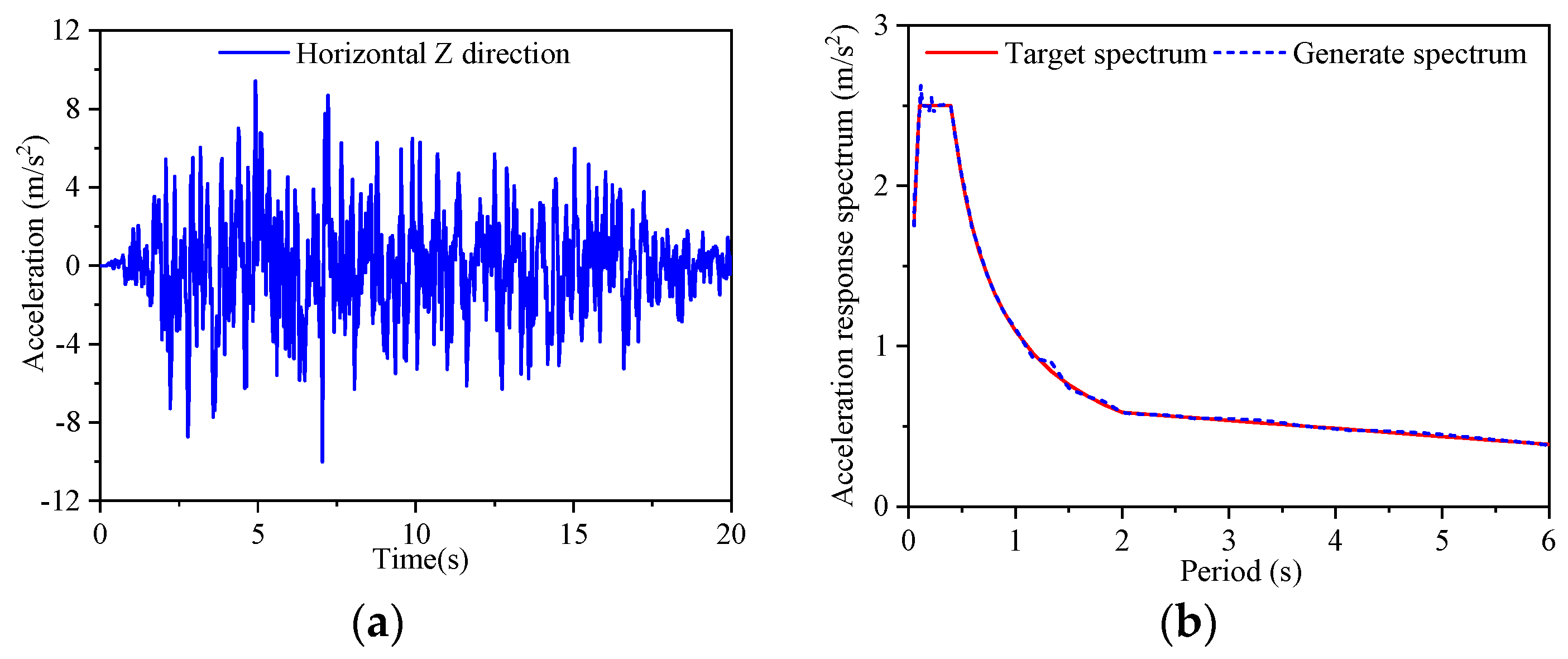
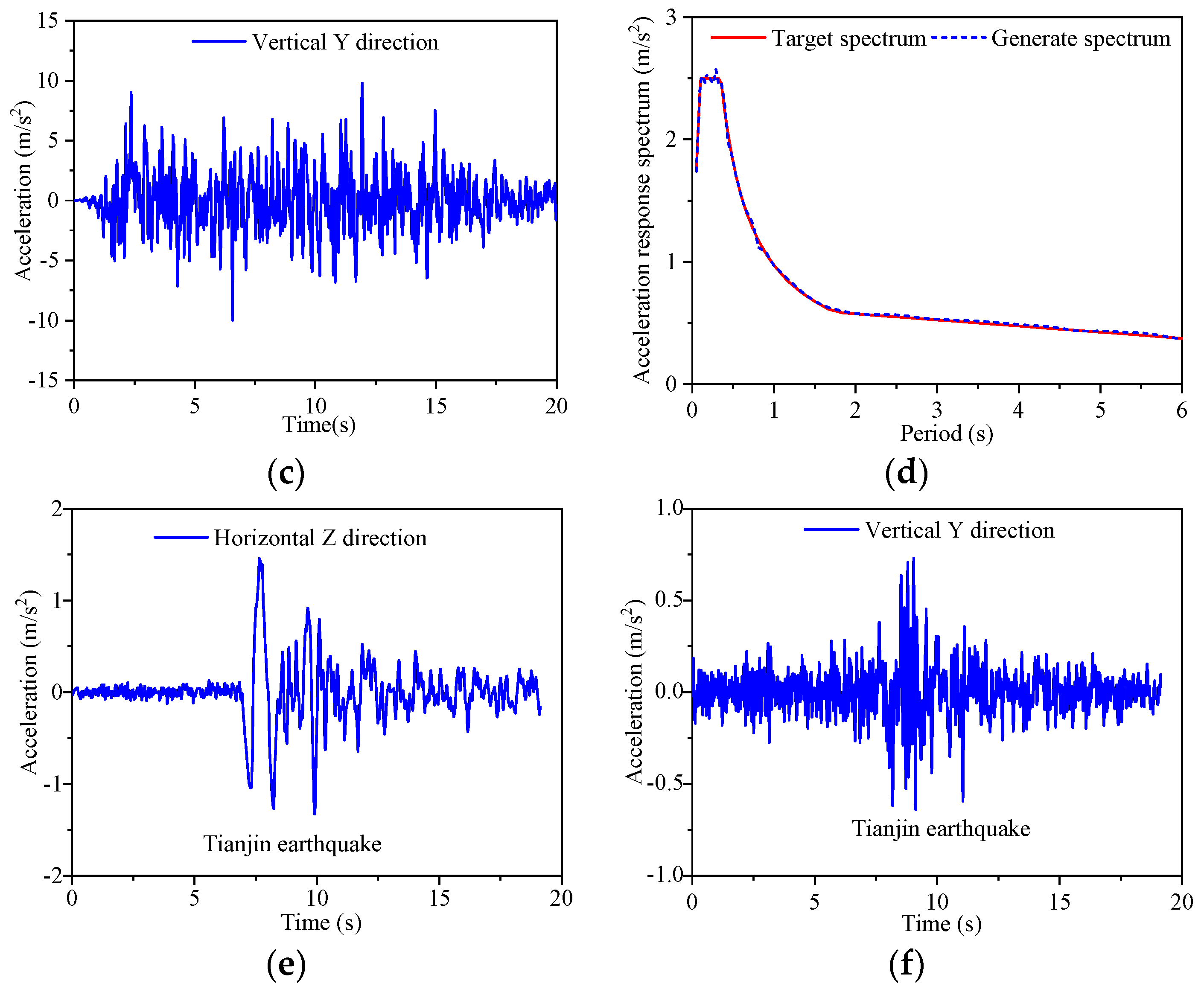









| Earthquakes | Characteristic Period | Amplification Factor | Damping Ratio | Fitting Tolerance |
|---|---|---|---|---|
| E1 | 0.40 | 2.50 | 0.05 | 0.02 |
| E2 | 0.35 | 2.50 | 0.05 | 0.02 |
| Dynamic Response | Conditions | Water Levels (m) | |
|---|---|---|---|
| 10 | 20 | ||
| Acceleration (m/s2) | Combined | 3.9231 | 3.2943 |
| Superposition | 6.2403 | 6.3520 | |
| Error | 59.06% | 92.82% | |
| Displacement (m) | Combined | 0.0270 | 0.0170 |
| Superposition | 0.0340 | 0.0340 | |
| Error | 25.93% | 100% | |
| Stress (MPa) | Combined | 2.9159 | 3.1851 |
| Superposition | 4.3602 | 7.2572 | |
| Error | 49.53% | 127.85% | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yun, G.; Liu, C. Nonlinear Dynamic Analysis of High-Strength Concrete Bridges under Post-Fire Earthquakes Considering Hydrodynamic Effects. Sustainability 2024, 16, 6486. https://doi.org/10.3390/su16156486
Yun G, Liu C. Nonlinear Dynamic Analysis of High-Strength Concrete Bridges under Post-Fire Earthquakes Considering Hydrodynamic Effects. Sustainability. 2024; 16(15):6486. https://doi.org/10.3390/su16156486
Chicago/Turabian StyleYun, Gaojie, and Chunguang Liu. 2024. "Nonlinear Dynamic Analysis of High-Strength Concrete Bridges under Post-Fire Earthquakes Considering Hydrodynamic Effects" Sustainability 16, no. 15: 6486. https://doi.org/10.3390/su16156486
APA StyleYun, G., & Liu, C. (2024). Nonlinear Dynamic Analysis of High-Strength Concrete Bridges under Post-Fire Earthquakes Considering Hydrodynamic Effects. Sustainability, 16(15), 6486. https://doi.org/10.3390/su16156486





