Multidisciplinary Research of Thermal Springs Area in Topusko (Croatia)
Abstract
1. Introduction
2. Materials and Methods
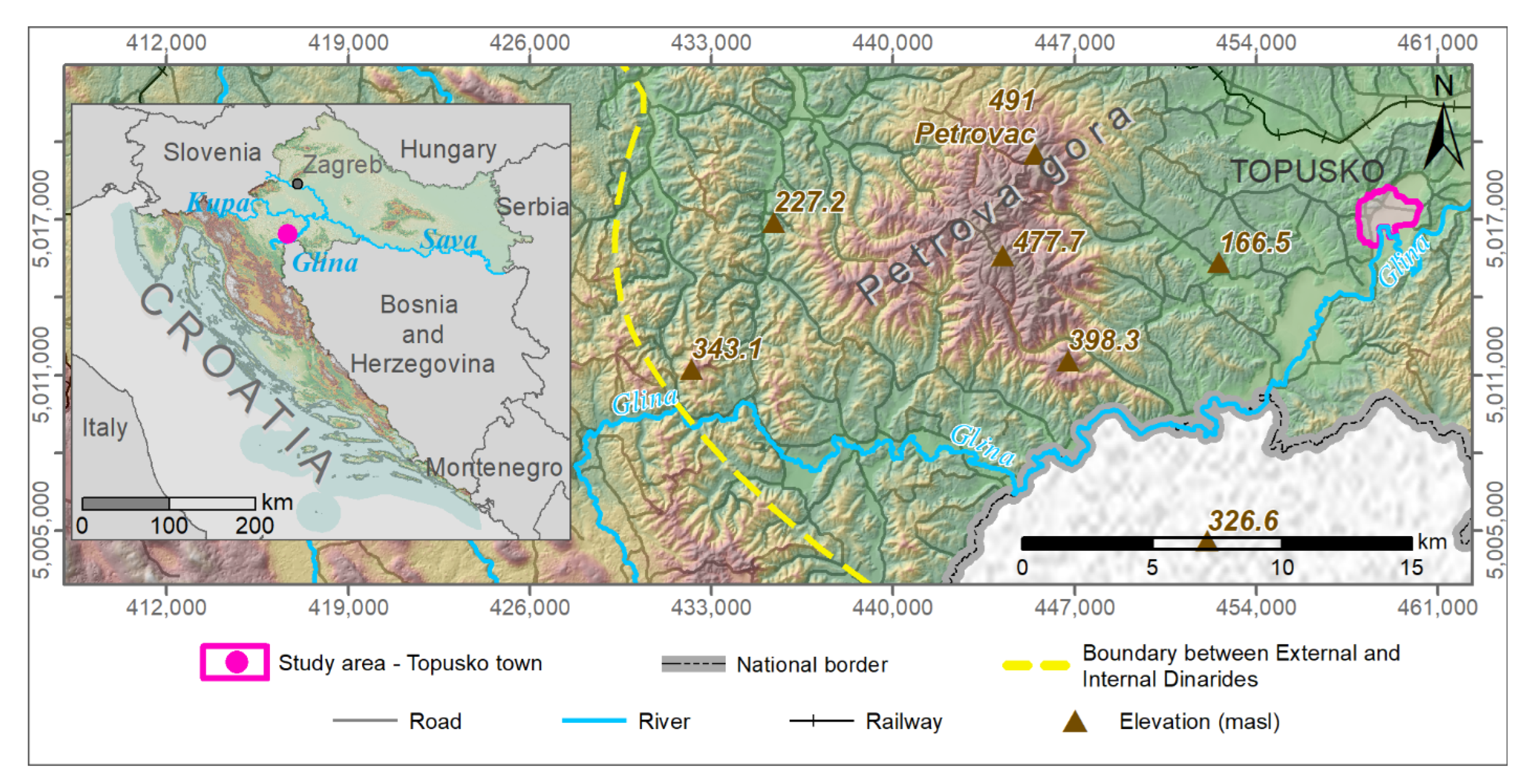
2.1. Study Area
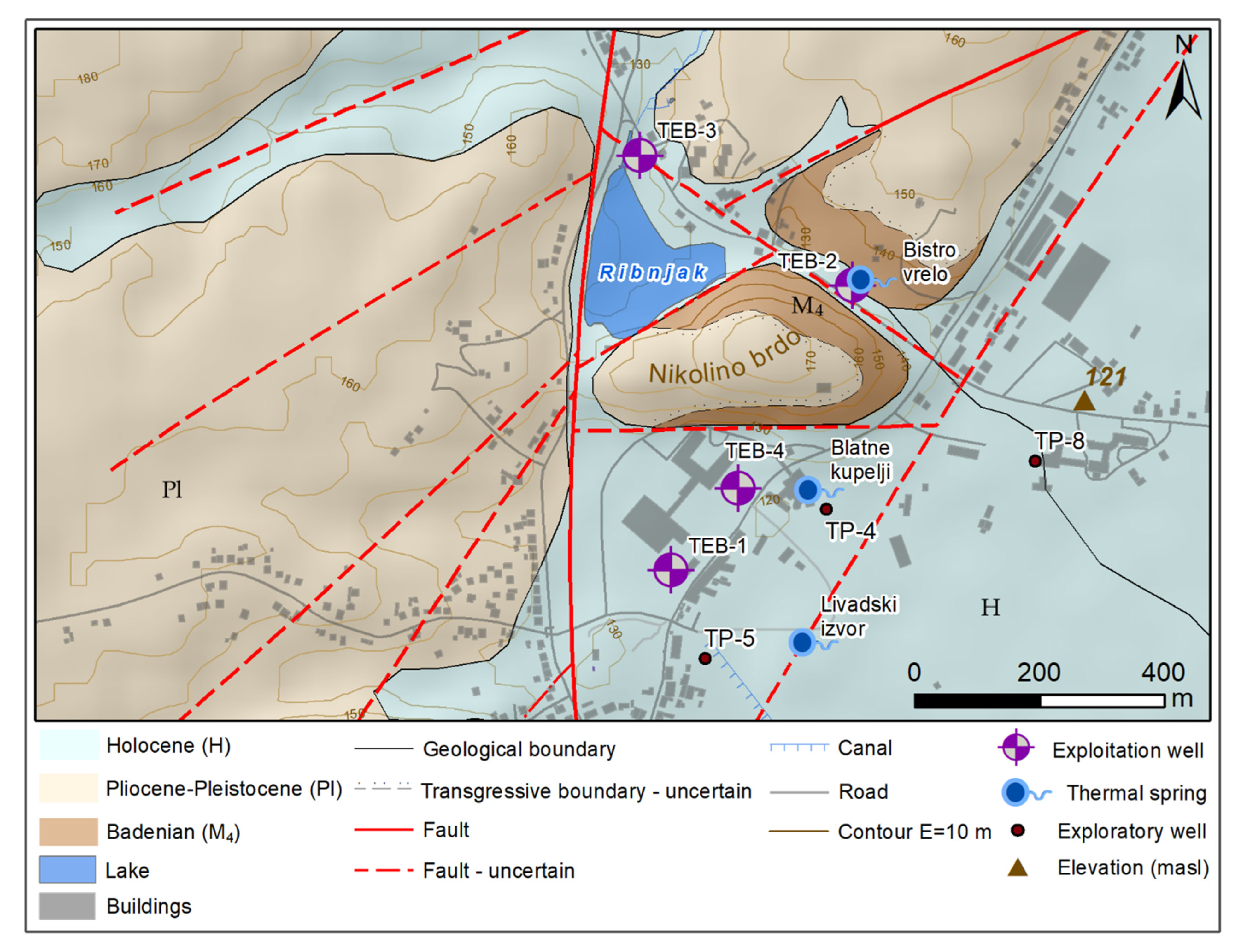
Geological and Hydrogeological Setting
2.2. Geochemical Investigations
2.2.1. Chemical Composition of Groundwater
2.2.2. Geothermometers
2.3. Hydrogeological Investigations
2.4. Geophysical Investigations
3. Results
3.1. Geochemical Characterisation of Thermal Groundwater
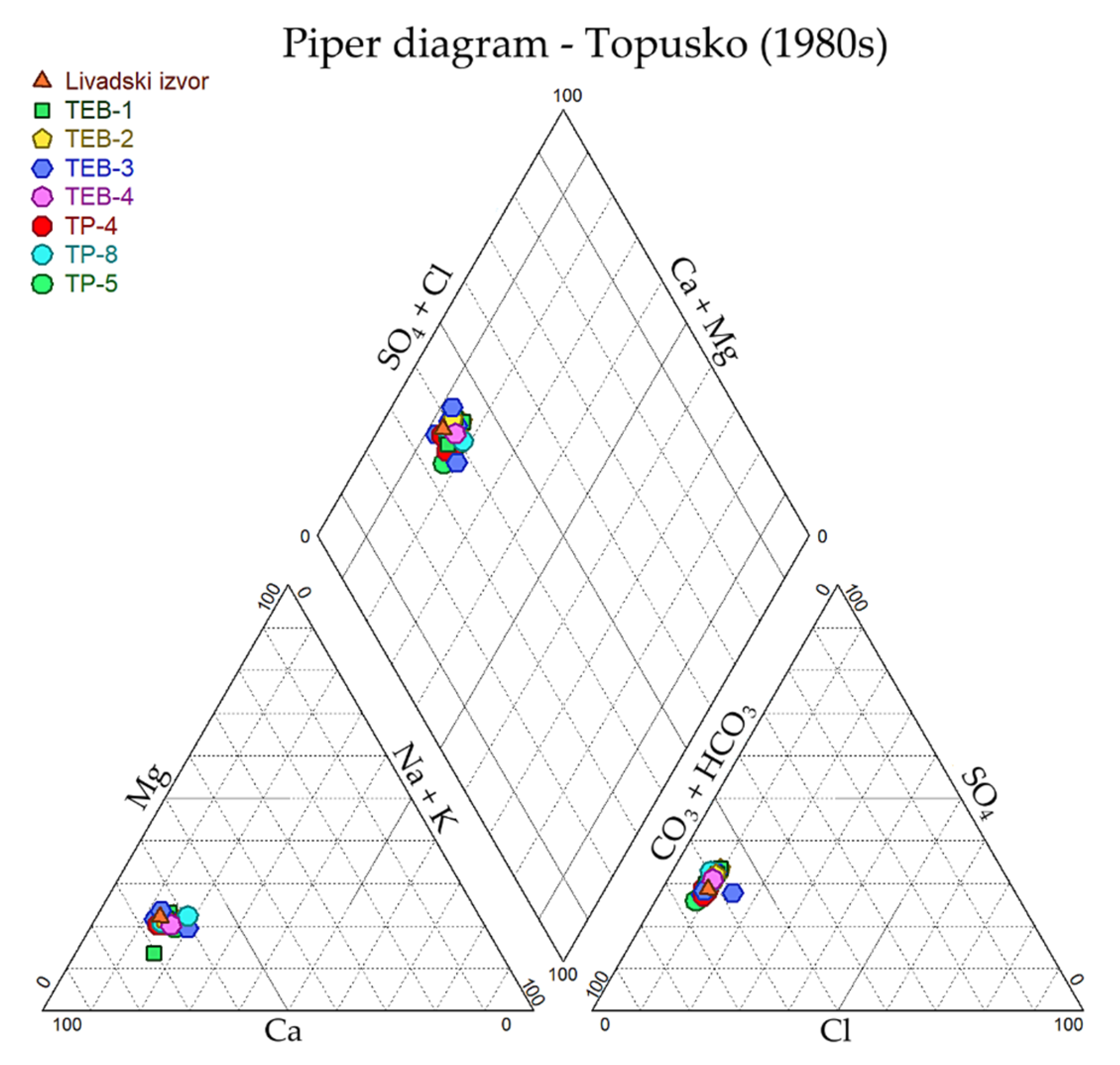
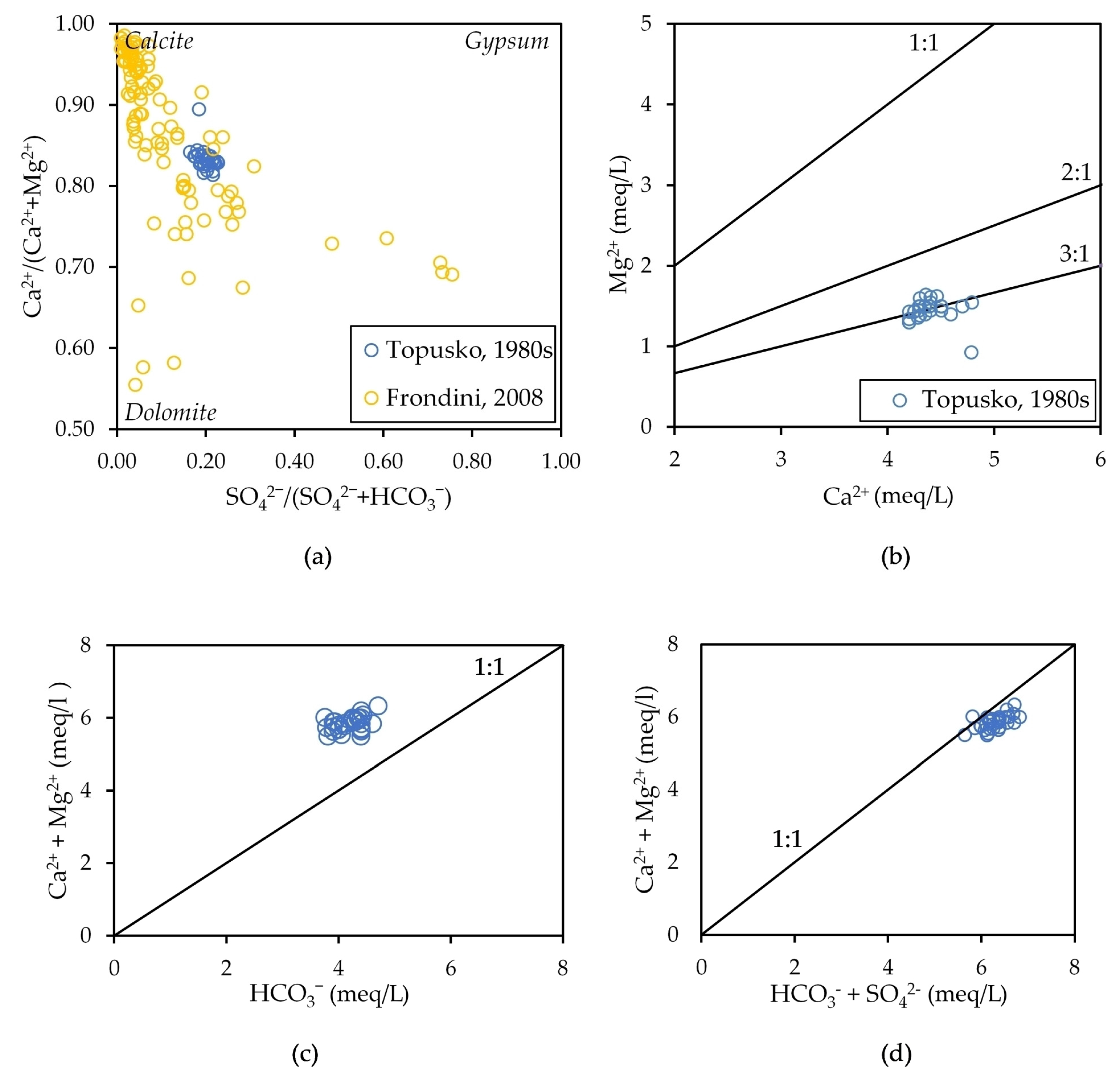
3.1.1. Major Ions Chemistry
3.1.2. Geothermometrical Results
3.2. Hydrogeological Parametrisation Results
3.2.1. TEB-1 Step-Drawdown Test
3.2.2. TEB-3 Step-Drawdown Test
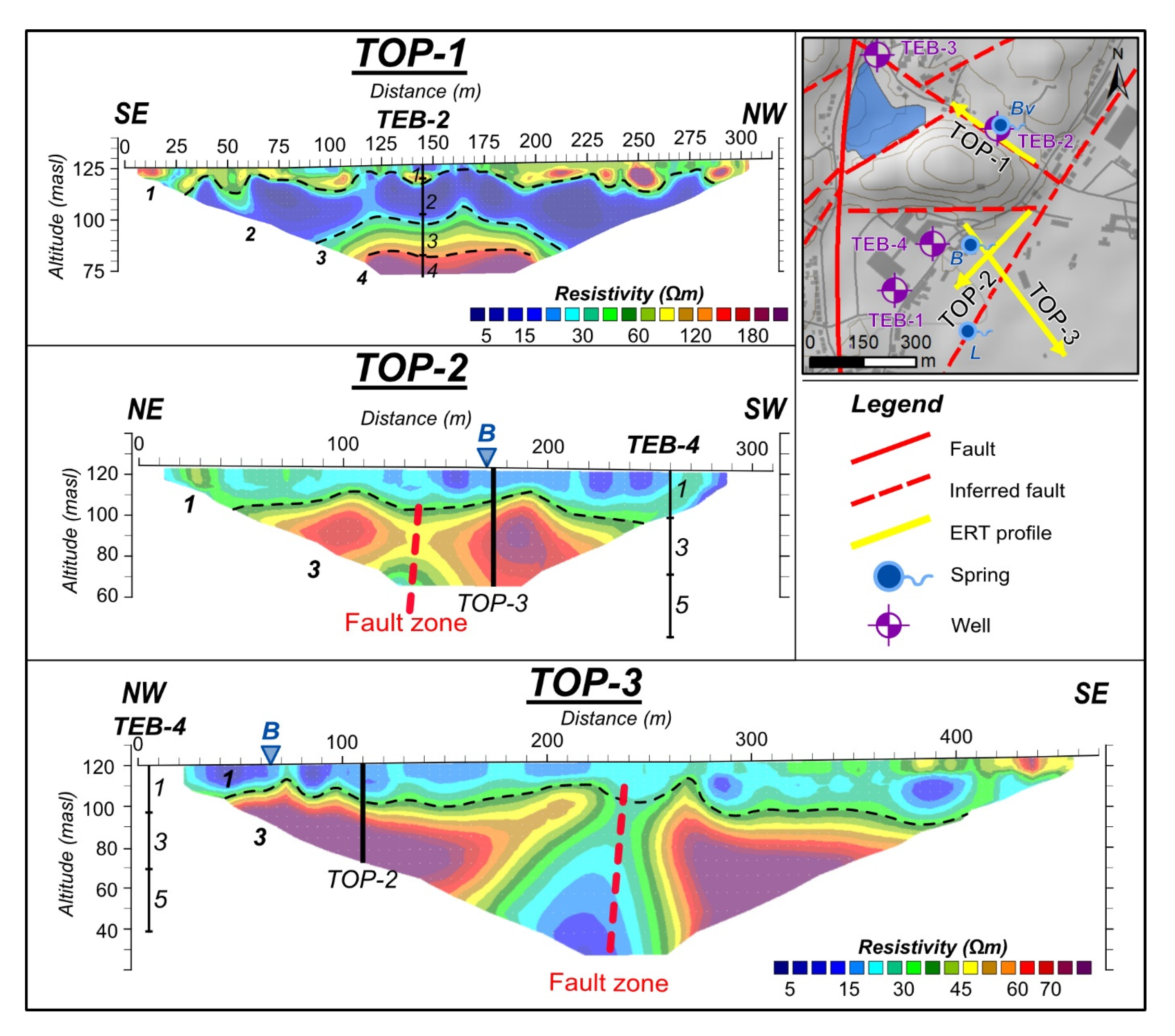
3.3. Interpretation of ERT Results
4. Discussion and Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- A European Green Deal—European Commission. Available online: europhttps://commission.europa.eu/strategy-and-policy/priorities-2019-2024/european-green-deal_ena.eu (accessed on 16 January 2022).
- CORDIS-EU Research Results—Supporting the Development of Europe’s Geothermal Energy Sector. Available online: https://cordis.europa.eu/article/id/442048-supporting-the-development-of-europe-s-geothermal-energy-sector (accessed on 16 January 2022).
- Aliyu, S.; Garba, M.M. Review on current global geothermal energy potentials and the future prospects. Int. J. Adv. Sci. Eng. 2019, 5, 10-31695. [Google Scholar] [CrossRef]
- Roscini, A.V.; Rapf, O.; Kockat, J.; Milne, C.; Jeffries, B.; D’angiolella, R. On the Way to a Climate-Neutral Europe Contributions from the Building Sector to A Strengthened 2030 Climate Target; Buildings Performance Institute Europe (BPIE): Brussels, Belgium, 2020; Available online: www.bpie.eu (accessed on 14 November 2022).
- Avci, A.C.; Kaygusuz, O.; Kaygusuz, K. Geothermal energy for sustainable development. J. Eng. Appl. Sci. 2020, 9, 1414–1426. [Google Scholar]
- Gupta, H.; Sukanta, R. Geothermal systems and resources. In Geothermal Energy; Gupta, H., Sukanta, R., Eds.; Elsevier: Amsterdam, The Netherlands, 2007; pp. 49–59. [Google Scholar] [CrossRef]
- Muffler, L.P.J.; Cataldi, R. Methods for regional assessment of geothermal resources. Geothermics 1978, 7, 53–89. [Google Scholar] [CrossRef]
- Moeck, I.S. Catalog of geothermal play types based on geologic controls. Renew. Sustain. Energy Rev. 2014, 37, 867–882. [Google Scholar] [CrossRef]
- Zarrouk, S.J.; McLean, K. Chapter 2—Geothermal systems. In Geothermal Well Test Analysis; Zarrouk, S.J., McLean, K., Eds.; Academic Press: Cambridge, MA, USA, 2019; pp. 13–38. [Google Scholar] [CrossRef]
- Blair, P.D. Geothermal Resources and Technology: Introduction. In Mechanical Engineers’ Handbook, 4th ed.; Kutz, M., Ed.; Energy and Power; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2014; Volume 4, pp. 1–17. [Google Scholar] [CrossRef]
- Hochstein, M.P. Classification and assessment of geothermal resources. In Small Geothermal Resources—A Guide to Development and Utilization; Dickson, M.H., Fanelli, M., Eds.; UNITAR/UNDP Centre for Small Energy Resources: Rome, Italy, 1990; pp. 31–59. [Google Scholar]
- Keegan-Treloar, R.; Irvine, D.J.; Solórzano-Rivas, S.C.; Werner, A.D.; Banks, E.W.; Currell, M.J. Fault-controlled springs: A review. Earth-Sci. Rev. 2022, 230, 104058. [Google Scholar] [CrossRef]
- Bowen, R. Geothermal Exploration. In Geothermal Resources, 2nd ed.; Springer: Dordrecht, The Netherlands, 1989; pp. 117–158. [Google Scholar] [CrossRef]
- Torresan, F.; Piccinini, L.; Cacace, M.; Pola, M.; Zampieri, D.; Fabbri, P. Numerical modeling as a tool for evaluating the renewability of geothermal resources: The case study of the Euganean Geothermal System (NE Italy). Env. Geochem. Health 2022, 44, 2135–2162. [Google Scholar] [CrossRef]
- Pola, M.; Cacace, M.; Fabbri, P.; Piccinini, L.; Zampieri, D.; Torresan, F. Fault control on a thermal anomaly: Conceptual and numerical modeling of a low-temperature geothermal system in the Southern Alps foreland basin (NE Italy). J. Geophys. Res. Solid Earth 2020, 125, e2019JB017394. [Google Scholar] [CrossRef]
- Pastorelli, S.; Marini, L.; Hunziker, J.C. Water chemistry and isotope composition of the Acquarossa thermal system, Ticino, Switzerland. Geothermics 1999, 28, 75–93. [Google Scholar] [CrossRef]
- Mazor, E. Chemical and Isotopic Groundwater Hydrology, 3rd ed.; Marcel Dekker: New York, NY, USA, 2004; pp. 13–179. [Google Scholar]
- D’Amore, F.; Arnórsson, S. Geothermometry. In Isotopic and Chemical Techniques in Geothermal Exploration, Development and Use; Arnórsson, S., Ed.; International Atomic Energy Agency: Vienna, Austria, 2000. [Google Scholar]
- Bruhn, D.; Manzella, A.; Vuataz, F.; Faulds, J.; Moeck, I.; Erbas, K. Exploration Methods. In Geothermal Energy Systems; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2010; pp. 37–112. ISBN 9783527408313. [Google Scholar]
- Hasan, M.; Shang, Y.; Meng, H.; Shao, P.; Yi, X. Application of electrical resistivity tomography (ERT) for rock mass quality evaluation. Sci. Rep. 2021, 11, 23683. [Google Scholar] [CrossRef]
- Nabi, A.; Liu, X.; Gong, Z.; Ali, A. Electrical resistivity imaging of active faults in palaeoseismology: Case studies from Karachi Arc, southern Kirthar Fold Belt, Pakistan. NRIAG J. Astron. Geophys. 2020, 9, 116–128. [Google Scholar] [CrossRef]
- Borović, S.; Marković, I. Utilization and tourism valorisation of geothermal waters in Croatia. Renew. Sustain. Energy Rev. 2015, 44, 52–63. [Google Scholar] [CrossRef]
- Horváth, F.; Musitz, B.; Balázs, A.; Végh, A.; Uhrin, A.; Nádor, A.; Koroknai, B.; Pap, N.; Tóth, T.; Wórum, G. Evolution of the Pannonian basin and its geothermal resources. Geothermics 2015, 53, 328–352. [Google Scholar] [CrossRef]
- Borović, S.; Marković, T.; Larva, O.; Brkić, Ž.; Mraz, V. Mineral and Thermal Waters in the Croatian Part of the Pannonian Basin. In Mineral and Thermal Waters of Southeastern Europe; Papić, P., Ed.; Springer: Cham, Switzerland, 2016; pp. 31–45. [Google Scholar] [CrossRef]
- Čučković, L.; Ožanić, M.; Abramović, M. Topusko: Monografija; Aura: Sisak, Croatia, 2009. (In Croatian) [Google Scholar]
- Državni Zavod za Statistiku—Popis ’21. Available online: https://popis2021.hr (accessed on 22 November 2022). (In Croatian).
- Zaninović, K.; Gajić-Čapka, M.; Perčec Tadić, M.; Vučetić, M.; Milković, J.; Bajić, A.; Cindrić, K.; Cvitan, L.; Katušin, Z.; Kaučić, D. Klimatski atlas Hrvatske/Climate atlas of Croatia 1961–1990, 1971–2000; Državni Hidrometeorološki Zavod: Zagreb, Croatia, 2008; (In Croatian and English). [Google Scholar]
- DHMZ—Državni Hidrometeorološki Zavod. Available online: https://meteo.hr/klima.php?section=klima_podaci¶m=k2_1 (accessed on 18 January 2021).
- Schmid, S.M.; Fügenschuh, B.; Kissling, E.; Schuster, R. Tectonic map and overall architecture of the Alpine orogen. Eclogae Geol. Helvetiae. 2004, 7, 93–117. [Google Scholar] [CrossRef]
- Schmid, S.M.; Bernoulli, D.; Fügenschuh, B.; Matenco, L.; Schefer, S.; Schuster, R.; Tischler, M.; Ustaszewski, K. The Alpine-Carpathian-Dinaridic orogenic system: Correlation and evolution of tectonic units. Swiss J. Geosci. 2008, 101, 139–183. [Google Scholar] [CrossRef]
- Handy, M.R.M.; Schmid, S.; Bousquet, R.; Kissling, E.; Bernoulli, D. Reconciling plate-tectonic reconstructions of Alpine Tethys with the geological–geophysical record of spreading and subduction in the Alps. Earth-Sci. Rev. 2010, 102, 121–158. [Google Scholar] [CrossRef]
- Vlahović, I.; Tišljar, J.; Velić, I.; Matičec, D. Evolution of the Adriatic Carbonate Platform: Palaeogeography, main events and depositional dynamics. Palaeogeogr. Palaeoclimatol. Palaeoecol. 2005, 220, 333–360. [Google Scholar] [CrossRef]
- Marković, T.; Borović, S.; Larva, O. Geochemical characteristics of thermal waters of Hrvatsko zagorje. Geol. Croat. 2015, 68, 67–77. [Google Scholar] [CrossRef]
- Šimunić, A. Topusko. In Mineral and Thermal Waters of the Republic of Croatia; Šimunić, A., Hećimović, I., Eds.; Croatian Geological Survey: Zagreb, Croatia, 2008; pp. 185–195. (In Croatian) [Google Scholar]
- Korolija, B.; Živaljević, T.; Šimunić, A. Osnovna geološka Karta SFRJ 1:100 000, List Slunj. L 33-104 [Basic Geological Map of SFRY 1:100000, Geology of the Slunj sheet L33-104]; Institut za geološka istraživanja: Zagreb, Croatia; Geološki zavod: Sarajevo, Bosnia and Herzegovina; Savezni Geološki Zavod: Beograd, Yugoslavia, 1980. (In Croatian) [Google Scholar]
- Bahun, S.; Raljević, B. Mineralna, Termalna i Ljekovita Vrela [Mineral and Thermal Springs]; unpublished report; Institute for Geological Research: Zagreb, Yugoslavia, 1969; p. 4769/5. (In Croatian) [Google Scholar]
- INA-Projekt Zagreb. Elaborat o Rezervama i Bilanci Termalnih Voda u Topuskom [Report on the Reserves and Mass Balance of Thermal Water in Topusko]; unpublished report; INA-Projekt, OOUR Kompleksna geološka istraživanja: Zagreb, Yugoslavia, 1984. (In Croatian) [Google Scholar]
- Čubranić, A. Osmatranje Termalnih Voda u Topuskom [Monitoring of Thermal Waters in Topusko]; unpublished report; INA-Projekt, OOUR Kompleksna Geološka Istraživanja: Zagreb, Croatia, 1984. (In Croatian) [Google Scholar]
- Šegotić, B.; Šmit, I. Studija Optimirane Energetske Učinkovitosti Korištenja Geotermalnih Voda [Study of Optimized Energy Efficiency of Geothermal Water Use]; unpublished report; Termoinženjering-projektiranje: Zagreb, Croatia, 2007. (In Croatian) [Google Scholar]
- Korolija, B.; Živaljević, T.; Šimunić, A. Osnovna geološka Karta SFRJ. Tumač za list Slunj [Basic Geological Map of SFRY 1:100000, Guide for the Slunj Sheet L33-104]; Institut za geološka istraživanja: Zagreb, Croatia; Geološki zavod: Sarajevo, Bosnia and Herzegovina; Savezni Geološki Zavod: Beograd, Yugoslavia, 1980. (In Croatian) [Google Scholar]
- Blake, S.; Henry, T.; Murray, J.; Flood, R.; Muller, M.R.; Jones, A.G.; Rath, V. Compositional multivariate statistical analysis of thermal groundwater provenance: A hydrogeochemical case study from Ireland. Appl. Geochem. 2016, 75, 171–188. [Google Scholar] [CrossRef]
- Verma, S.P.; Pandarinath, K.; Santoyo, E. SolGeo: A new computer program for solute geothermometers and its application to Mexican geothermal fields. Geothermics 2008, 37, 597–621. [Google Scholar] [CrossRef]
- Pola, M.; Fabbri, P.; Piccinini, L.; Zampieri, D. Conceptual and numerical models of a tectonically-controlled geothermal system: A case study of the Euganean Geothermal System, Northern Italy. Cent. Eur. Geol. 2015, 58, 129–151. [Google Scholar] [CrossRef]
- Powell, T.; Cumming, W. Spreadsheets for Geothermal Water and Gas Geochemistry. In Proceedings of the Thirty-Fifth Workshop on Geothermal Reservoir Engineering, Stanford University, California, CA, USA, 1–3 February 2010. [Google Scholar]
- Dumančić, E.; Čubranić, A. Prijedlog Projekta Sanacije Livadskih Izvora [Project Proposal—Revitalisation of Livadski Izvori Spring]; unpublished report; INA-Projekt Zagreb: Zagreb, Yugoslavia, 1989; p. 5517. (In Croatian) [Google Scholar]
- Blinja, T. Izvedba Eksploatacione Bušotine TEB-4 u Topuskom [Construction of the Exploitation Well TEB-4 in Topusko]; unpublished report; INA-Projekt: Zagreb, Yugoslavia, 1986. (In Croatian) [Google Scholar]
- Blinja, T. Sanacioni Radovi Eksploatacionih Bušotina TEB-1 i TEB-2 u Topuskom [Revitalisation of Exploitation Wells TEB-1 and 2 in Topusko]; unpublished report; INA-Projekt, OOUR Kompleksna geološka istraživanja: Zagreb, Croatia, 1986. (In Croatian) [Google Scholar]
- Nevada Division of Environmental Protection (NDEP). Evaluation of Inorganic Chemical Analysis. Available online: https://ndep.nv.gov/uploads/documents/sept-2009-cation-anion-balance-guide.pdf (accessed on 22 August 2022).
- Appelo, C.A.J.; Postma, D. Geochemistry, Groundwater and Pollution, 2nd ed.; A.A. Balkema Publishers: Leiden, The Netherlands, 2005; pp. 17–18. [Google Scholar]
- Simler, R. Software Diagrammes, V6.5, Laboratoire d’Hydrologie d’Avignon; Université d’Avignon et pays du Vaucluse: Avignon, France, 2012. [Google Scholar]
- Piper, A.M. A Graphic Procedure in the Geochemical Interpretation of Water-Analyses. Am. Geophys. Union Trans. 1944, 25, 914–923. [Google Scholar] [CrossRef]
- Fetter, C.W. Applied Hydrogeology, 4th ed.; Lynch, P., Ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2001. [Google Scholar]
- Romano, P.; Liotta, M. Using and abusing Giggenbach ternary Na-K-Mg diagram. Chem. Geol. 2020, 541, 119577. [Google Scholar] [CrossRef]
- Truesdell, A.H. Geochemical techniques in exploration, summary of section III. In Proceedings of the Second United Nations Symposium on the Development, Use of Geothermal Resources, San Francisco, CA, USA, 20 May 1975; pp. 3–29. [Google Scholar]
- Fournier, R.O. Chemical geothermometers and mixing models for geothermal systems. Geothermics 1977, 5, 41–50. [Google Scholar] [CrossRef]
- Michard, G. Gothermométres Chimiques. Bull. BRGM 1979, 2, 183–189. [Google Scholar]
- Arnòrsson, S.; Gunnlaugsson, E.; Svavarsson, H. The chemistry of geothermal waters in Iceland. III. Chemical geothermometry in geothermal investigations. Geochim.Cosmochim. Acta 1983, 47, 567–577. [Google Scholar] [CrossRef]
- Kruseman, G.P.; De Ridder, N.A.; Verweij, J.M. Analysis and Evaluation of Pumping Test Data, 2nd ed.; International institute for land reclamation and improvement: Wageningen, The Netherlands, 1994. [Google Scholar]
- Rorabaugh, M.J. Graphical and theoretical analysis of step-drawdown test of artesian well. Trans. Am. Soc. Civ. Eng. 1953, 79, 1–23. [Google Scholar]
- Jacob, C.E. Drawdown test to determine effective radius of artesian well. Trans. Am. Soc. Civ. Eng. 1947, 112, 1047–1064. [Google Scholar] [CrossRef]
- Bruin, J.; Hudson, H.E. Selected Methods for Pumping Test Analysis. In Illinois State Water Survey; State Ilinois University: Springfield, IL, USA, 1955. [Google Scholar]
- Fabbri, P. Transmissivity in the Geothermal Euganean Basin: A Geostatistical Analysis. Ground Water 1997, 35, 881–887. [Google Scholar] [CrossRef]
- Verbovšek, T. Estimation of transmissivity and hydraulic conductivity from specific capacity and specific capacity index in dolomite aquifers. J. Hydrol. Eng. 2008, 13, 817–823. [Google Scholar] [CrossRef]
- Diaferia, I.; Barchi, M.; Loddo, M.; Schiavone, D.; Siniscalchi, A. Detailed imaging of tectonic structures by multiscale earth resistivity tomographies: The Colfiorito normal faults (Central Italy). Geophys. Res. Lett. 2006, 33, L09305. [Google Scholar] [CrossRef]
- Pérez-Estay, N.; Molina-Piernas, E.; Roquer, T.; Aravena, D.; Araya Vargas, J.; Morata, D.; Arancibia, G.; Valdenegro, P.; García, K.; Elizalde, D. Shallow anatomy of hydrothermal systems controlled by the Liquiñe-Ofqui Fault System and the Andean Transverse Faults: Geophysical imaging of fluid pathways and practical implications for geothermal exploration. Geothermics 2022, 104, 102435. [Google Scholar] [CrossRef]
- Siniscalchi, A.; Tripaldi, S.; Neri, M.; Giammanco, S.; Piscitelli, S.; Balasco, M.; Behncke, B.; Magrì, C.; Naudet, V.; Rizzo, E. Insights into fluid circulation across the Pernicana Fault (Mt. Etna, Italy) and implications for flank instability. J. Volcanol. Geotherm. Res. 2010, 193, 137–142. [Google Scholar] [CrossRef]
- Briški, M.; Stroj, A.; Kosović, I.; Borović, S. Characterization of Aquifers in Metamorphic Rocks by Combined Use of Electrical Resistivity Tomography and Monitoring of Spring Hydrodynamics. Geosciences 2020, 10, 137. [Google Scholar] [CrossRef]
- Giustini, F.; Brilli, M.; Carlucci, G.; Ciotoli, G.; Gaudiosi, L.; Mancini, M.; Simionato, M. Geophysical and geochemical multi-method investigations for reconstructing subsurfaces, alluvial sedimentology, and structural geology (Tiber valley, Rome). Int. J. Earth. Sci. (Geol. Rundsch.) 2022, 112, 197–216. [Google Scholar] [CrossRef]
- Ward, S.H. Resistivity and induced polarization methods. In Geotechnical and Environmental Geophysics; Ward, S.H., Ed.; Society of Exploration Geophysicists: Tulsa, Ok, USA, 1990; Volume I, pp. 147–190. [Google Scholar]
- Sharma, P.V. Environmental and Engineering Geophysics; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Reynolds, J.M. An Introduction to Applied and Environmental Geophysics, 2nd ed.; Wiley: New York, NY, USA, 2011. [Google Scholar]
- Loke, M.H. Tutorial: 2-D and 3-D Electrical Imaging Surveys; Geotomo Software: Penang, Malaysia, 2020; Available online: https://www.geotomosoft.com/downloads.php (accessed on 27 February 2020).
- Loke, M.H.; Barker, R.D. Rapid least-squares inversion of apparent resistivity pseudosections using a quasi-Newton method. Geophys. Prospect. 1996, 44, 131–152. [Google Scholar] [CrossRef]
- DeGroot-Hedlin, C.; Constable, S. Occam’s inversion to generate smooth, two-dimensional models from magnetotelluric data. Geophysics 1990, 55, 1613–1624. [Google Scholar] [CrossRef]
- Sasaki, Y. Resolution of resistivity tomography inferred from numerical simulation. Geophys.Prospect. 1992, 40, 453–464. [Google Scholar] [CrossRef]
- Ellis, R.G.; Oldenburg, D.W. Applied geophysical inversion. Geophys. J. Int. 1994, 116, 5–11. [Google Scholar] [CrossRef]
- Ellis, R.G.; Farquharson, C.G.; Oldenburg, D.W. Approximate inverse mapping inversion of the COPROD2 data. J. Geomagn. Geoelectr. 1993, 45, 1001–1012. [Google Scholar] [CrossRef]
- Milenić, D.; Krunić, O.Ž.; Milankovic, D. Thermomineral waters of inner Dinarides Karst. Acta Carsologica 2012, 41, 235–252. [Google Scholar] [CrossRef]
- Freeze, R.A.; Cherry, J.A. Groundwater; Prentice Hall Inc.: Englewood Cliffs, NJ, USA, 1979; Volume 7632, p. 604. [Google Scholar]
- Li, Z.; Huang, T.; Ma, B.; Long, Y.; Zhang, F.; Tian, J.; Li, Y.; Pang, Z. Baseline groundwater quality before shale gas development in Xishui, Southwest China: Analyses of hydrochemistry and multiple environmental isotopes (2H, 18O, 13C, 87Sr/86Sr, 11B, and Noble Gas Isotopes). Water 2020, 12, 1741. [Google Scholar] [CrossRef]
- Wang, Z.; Torres, M.; Paudel, P.; Hu, L.; Yang, G.; Chu, X. Assessing the karst groundwater quality and hydrogeochemical characteristics of a prominent dolomite aquifer in Guizhou, China. Water 2020, 12, 2584. [Google Scholar] [CrossRef]
- Patekar, M.; Bašić, M.; Pola, M.; Kosović, I.; Terzić, J.; Lucca, A.; Mittempergher, S.; Berio, L.; Borović, S. Multidisciplinary investigations of a karst aquifer for managed aquifer recharge applications on the island of Vis (Croatia). Acque Sotter. Ital. J. Groundw. 2022, 11, 37–48. [Google Scholar] [CrossRef]
- Frondini, F. Geochemistry of regional aquifer systems hosted by carbonate-evaporite formations in Umbria and southern Tuscany (central Italy). Appl. Geochem. 2008, 23, 2091–2104. [Google Scholar] [CrossRef]
- Hilberg, S.; Schneider, J.F. The Aquifer Characteristics of the Dolomite Formation a New Approach for Providing Drinking Water in the Northern Calcareous Alps Region in Germany and Austria. Water Resour. Manag. 2011, 25, 2705–2729. [Google Scholar] [CrossRef]
- Fellehner, M. Der Hauptdolomit als Grundwasserspeicher in den Nördlichen Kalkalpen. Ph.D. Thesis, Philipps-Universität, Marburg, Deutschland, 30 June 2004. [Google Scholar]
- Li, X.; Wu, P.; Han, Z.; Zha, X.; Ye, H.; Qin, Y. Effects of mining activities on evolution of water quality of karst waters in Midwestern Guizhou, China: Evidences from hydrochemistry and isotopic composition. Environ. Sci. Pollut. Res. 2018, 25, 1220–1230. [Google Scholar] [CrossRef]
- Sun, C.; Wang, S.; Chen, W. Hydrochemical Characteristics and the Relationship between Surface and Groundwater in a Typical ‘Mountain–Oasis’ Ecosystem in Central Asia. Sustainability 2022, 14, 7453. [Google Scholar] [CrossRef]
- Blasco, M.; Auqué, L.F.; Gimeno, M.J. Geochemical evolution of thermal waters in Carbonate—Evaporitic systems: The triggering effect of halite dissolution in the dedolomitisation and albitisation processes. J. Hydrol. 2019, 570, 623–636. [Google Scholar] [CrossRef]
- Blasco, M.; Gimeno, M.J.; Auqué, L.F. Low temperature geothermal systems in carbonate-evaporitic rocks: Mineral equilibria assumptions and geothermometrical calculations. Insights from the Arnedillo thermal waters (Spain). Sci. Total Environ. 2018, 615, 526–539. [Google Scholar] [CrossRef]
- Hiscock, K.M.; Bense, V.F. Hydrogeology: Principles and Practice, 2nd ed.; John Wiley & Sons Ltd: Hoboken, NY, USA, 2014. [Google Scholar]
- Halle, R. Kemizam i Obradba Vode [Water Chemistry and Treatment]; Faculty of Mining, Geology and Petroleum Engineering, University of Zagreb: Zagreb, Croatia, 2004. (In Croatian) [Google Scholar]
- Macenić, M.; Kurevija, T.; Medved, I. Novel geothermal gradient map of the Croatian part of the Pannonian Basin System based on data interpretation from 154 deep exploration wells. Renew. Sustain. Energy Rev. 2020, 132, 110069. [Google Scholar] [CrossRef]
- Domenico, P.A.; Schwartz, F.W. Physical and Chemical Hydrogeology; John Wiley & Sons: New York, NY, USA, 1990. [Google Scholar]
- Heasler, H.; Jaworowski, C.; Foley, D. Geothermal systems and monitoring hydrothermal features. In Geological Monitoring; Young, R., Norby, L., Eds.; Geological Society of America: Boulder, CO, USA, 2009; pp. 105–140. [Google Scholar] [CrossRef]
| Name | Depth | Date | T | pH | Ca2+ | Mg2+ | Na+ | K+ | HCO3− | SO42− | Cl− | TDS* | SiO2 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| m | °C | - | mg/L | ||||||||||
| Livadski izvor | 0 | 1904 | 49.5 | - | 89.39 | 19.74 | 18.27 | 12.69 | 270.4 | 108.10 | 19.40 | 586 | 29.96 |
| 12/1988 | 50 | 7.0 | 85.20 | 17.50 | 15.20 | 7.20 | 244.4 | 89.20 | 21.30 | 521 | 25.6 | ||
| TEB-1 | 243 | 5/1983 | 66 | 7.2 | 87.10 | 18.20 | 23.30 | 9.30 | 268.5 | 103.30 | 22.00 | 581 | 31.0 |
| 7/1983 | 64.5 | 7.65 | 88.00 | 18.20 | 17.20 | 6.00 | 240.0 | 107.00 | 21.20 | 550 | 30.0 | ||
| 9/1983 | 65 | 7.6 | 88.00 | 18.20 | 24.00 | 4.00 | 238.0 | 110.00 | 22.70 | 554 | 30.5 | ||
| 11/1983 | 66 | 7.4 | 86.20 | 19.40 | 16.80 | 9.80 | 262.2 | 100.00 | 20.00 | 566 | 32.0 | ||
| 2/1984 | 65 | 7.3 | 95.90 | 11.28 | 18.10 | 11.30 | 268.4 | 95.63 | 21.94 | 564 | 25.8 | ||
| 10/1985 | 65 | 7.7 | 84.20 | 17.40 | 15.30 | 10.60 | 238.0 | 104.2 | 22.50 | 542 | 29.5 | ||
| TEB-2 | 150 | 5/1983 | 67 | 7.1 | 90.20 | 17.60 | 18.40 | 11.10 | 259.3 | 96.00 | 22.30 | 553 | 24.0 |
| 7/1983 | 66 | 7.1 | 90.20 | 18.20 | 22.00 | 10.10 | 265.4 | 104.00 | 21.50 | 579 | 30.0 | ||
| 9/1983 | 67 | 7.4 | 86.00 | 17.60 | 22.00 | 6.00 | 231.0 | 105.70 | 22.50 | 540 | 30.5 | ||
| 11/1983 | 67 | 7.2 | 86.00 | 18.20 | 18.70 | 11.10 | 250.2 | 102.80 | 23.00 | 560 | 31.0 | ||
| 10/1985 | 68 | 7.9 | 85.10 | 17.40 | 15.80 | 9.20 | 244.1 | 104.5 | 23.30 | 547 | 28.9 | ||
| TEB-3 | 163 | 5/1983 | 66 | 7 | 88.20 | 18.80 | 18.30 | 7.80 | 262.4 | 100.40 | 21.90 | 556 | 24.0 |
| 7/1983 | 62 | 7.65 | 86.20 | 18.20 | 17.00 | 6.00 | 244.0 | 100.80 | 20.90 | 539 | 29.0 | ||
| 9/1983 | 63 | 7.6 | 96.00 | 18.80 | 18.00 | 3.90 | 286.8 | 96.50 | 22.70 | 588 | 28.5 | ||
| 11/1983 | 66 | 7.3 | 88.20 | 17.60 | 18.40 | 10.00 | 250.2 | 108.60 | 22.00 | 566 | 32.0 | ||
| 2/1984 | 66 | 7.25 | 85.88 | 16.49 | 24.50 | 13.20 | 268.4 | 94.41 | 21.85 | 567 | 26.3 | ||
| 12/1988 | - | 7.2 | 88.20 | 19.60 | 12.80 | 11.40 | 228.8 | 98.76 | 39.00 | 563 | 30.80 | ||
| TEB-4 | 80.8 | 11/1985 | 64 | 7.1 | 84.20 | 16.30 | 15.42 | 15.60 | 247.10 | 100.40 | 22.10 | 544 | 23.5 |
| TP-5 | 50 | 1978 | 36 | 7.65 | 84.16 | 15.80 | 14.00 | 20.80 | 268.0 | 83.10 | 19.70 | 543 | 23.4 |
| TP-4 | 50 | 5/1983 | 52 | 7.2 | 87.10 | 18.20 | 23.10 | 8.26 | 280.6 | 101.00 | 21.60 | 578 | 23.6 |
| 7/1983 | 52 | 7.65 | 84.20 | 15.80 | 16.90 | 8.20 | 232.0 | 88.50 | 21.00 | 505 | 21.0 | ||
| 9/1983 | 54 | 7.1 | 92.00 | 17.00 | 19.00 | 4.00 | 259.3 | 90.00 | 22.90 | 549 | 28.0 | ||
| 11/1983 | 52 | 7.3 | 86.20 | 16.80 | 18.80 | 11.60 | 268.5 | 89.00 | 22.60 | 556 | 26.5 | ||
| TP-8 | 170 | 5/1983 | 58 | 7.15 | 90.20 | 18.20 | 16.10 | 11.40 | 265.0 | 107.80 | 22.30 | 576 | 28.0 |
| 7/1983 | 53 | 7.8 | 87.20 | 17.00 | 17.80 | 9.00 | 238.0 | 100.00 | 20.90 | 528 | 24.0 | ||
| 9/1983 | 53 | 7 | 90.20 | 18.20 | 18.00 | 10.70 | 259.0 | 102.70 | 22.10 | 566 | 28.0 | ||
| 11/1983 | 54 | 7.4 | 94.20 | 18.20 | 15.70 | 12.70 | 268.5 | 102.80 | 23.00 | 581 | 29.0 | ||
| 2/1984 | 48 | 7.3 | 87.31 | 19.96 | 24.60 | 11.30 | 268.4 | 116.40 | 20.71 | 592 | 26.9 | ||
| Name | Truesdell (1976) [54] | Fournier (1977) [55] | Michard (1979) [56] | Fournier (1977) [55] | Arnòrsson et al. (1983) [57] |
|---|---|---|---|---|---|
| SiO2-Quartz | SiO2-Chalcedony | ||||
| Livadski izvor | 76 | 76 | 78 | 45 | 48 |
| TEB-1 | 79 | 79 | 78 | 48 | 51 |
| TEB-2 | 78 | 78 | 80 | 46 | 49 |
| TEB-3 | 77 | 77 | 78 | 46 | 49 |
| TEB-4 | 70 | 70 | 71 | 38 | 41 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pavić, M.; Kosović, I.; Pola, M.; Urumović, K.; Briški, M.; Borović, S. Multidisciplinary Research of Thermal Springs Area in Topusko (Croatia). Sustainability 2023, 15, 5498. https://doi.org/10.3390/su15065498
Pavić M, Kosović I, Pola M, Urumović K, Briški M, Borović S. Multidisciplinary Research of Thermal Springs Area in Topusko (Croatia). Sustainability. 2023; 15(6):5498. https://doi.org/10.3390/su15065498
Chicago/Turabian StylePavić, Mirja, Ivan Kosović, Marco Pola, Kosta Urumović, Maja Briški, and Staša Borović. 2023. "Multidisciplinary Research of Thermal Springs Area in Topusko (Croatia)" Sustainability 15, no. 6: 5498. https://doi.org/10.3390/su15065498
APA StylePavić, M., Kosović, I., Pola, M., Urumović, K., Briški, M., & Borović, S. (2023). Multidisciplinary Research of Thermal Springs Area in Topusko (Croatia). Sustainability, 15(6), 5498. https://doi.org/10.3390/su15065498






