Analysis of Heat Dissipation Performance of Battery Liquid Cooling Plate Based on Bionic Structure
Abstract
:1. Introduction
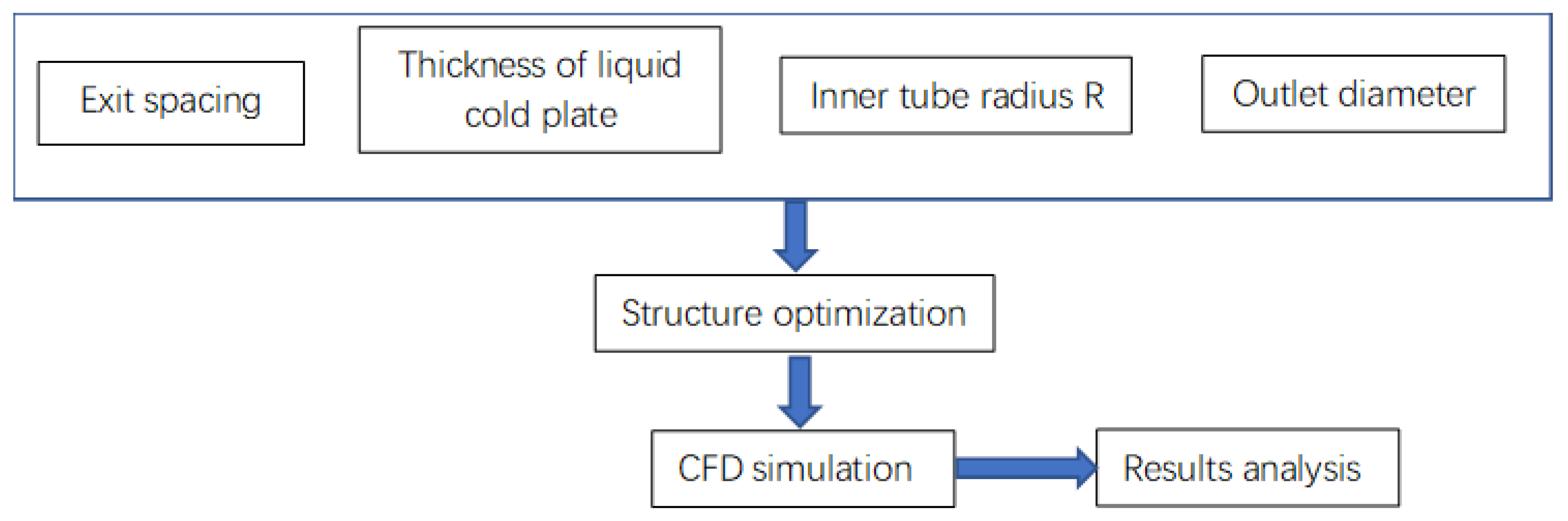
2. Structural Modeling and Calculation Settings
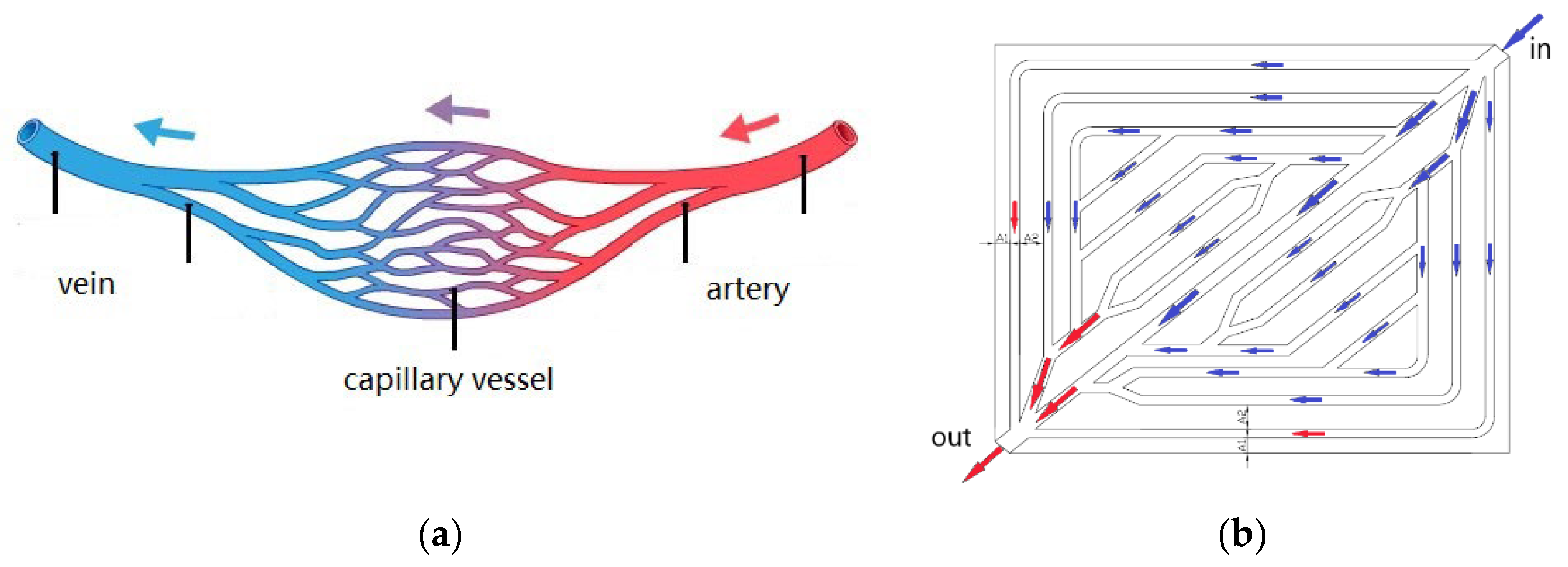
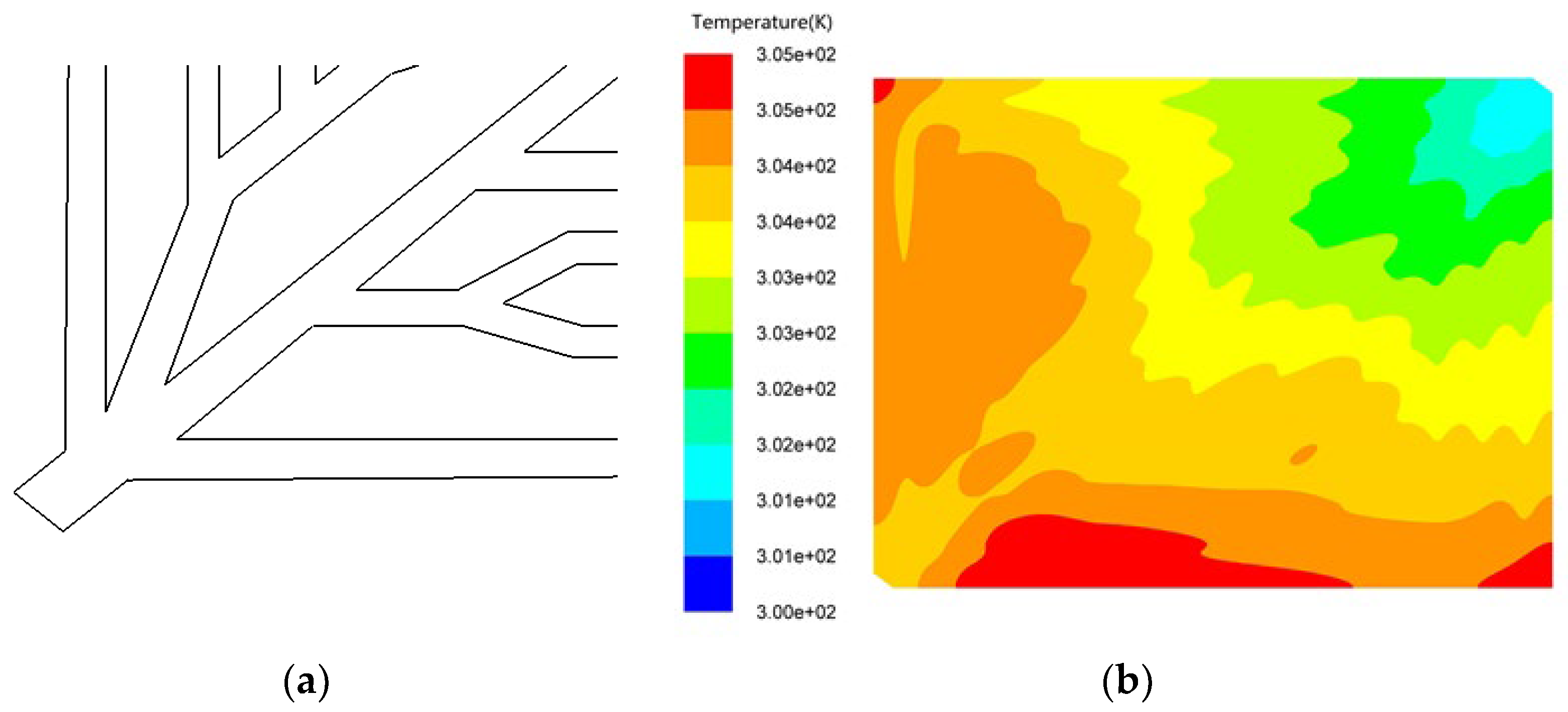
2.1. Modeling of Liquid Cooling Plate with Imitation Blood Vessel Structure
2.2. Verification of the Independence of Boundary Conditions and Grid
2.2.1. Boundary Conditions
2.2.2. Grid Independence Verification
3. Simulation Analysis of Liquid Cooling Plate Heat Dissipation
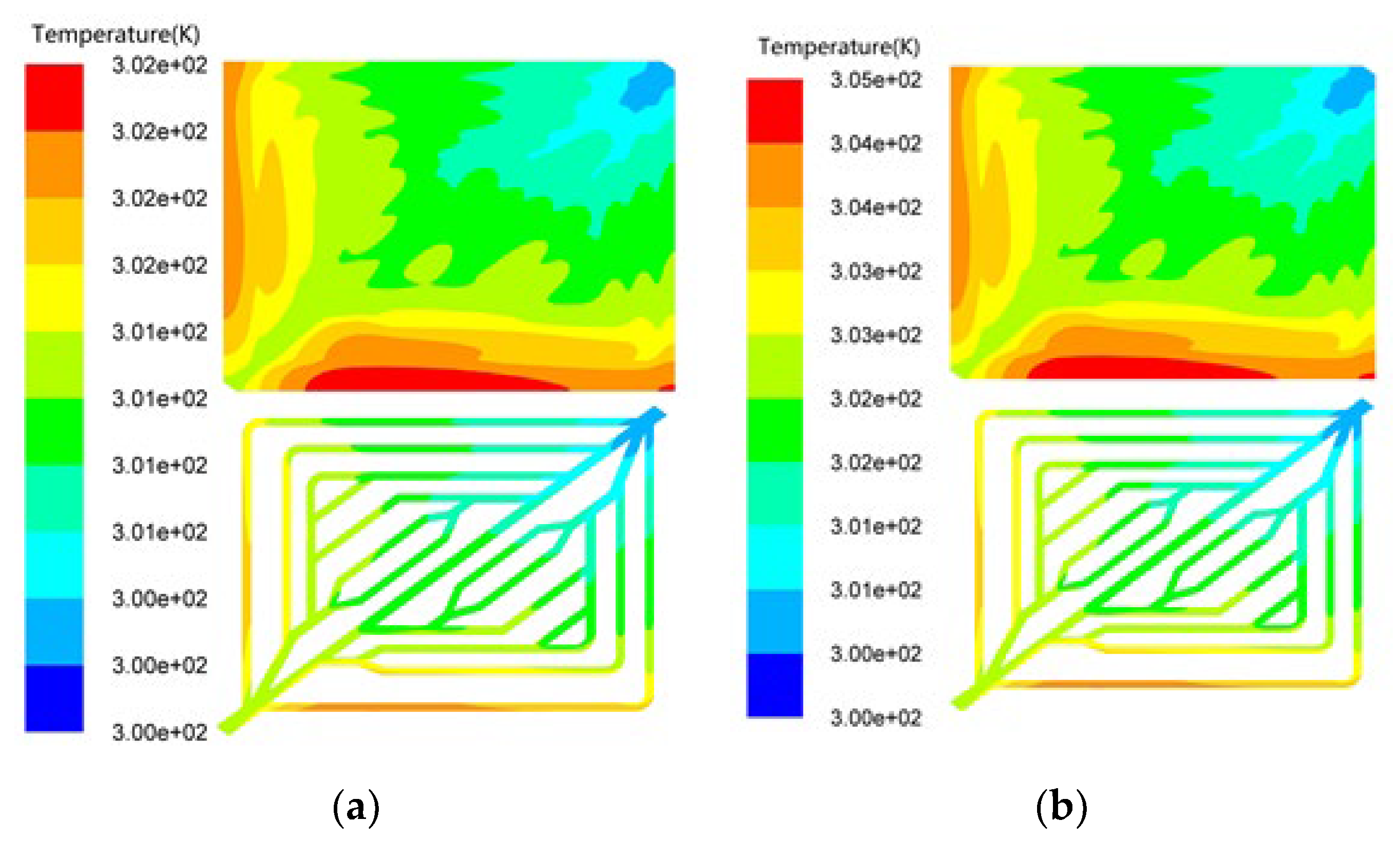
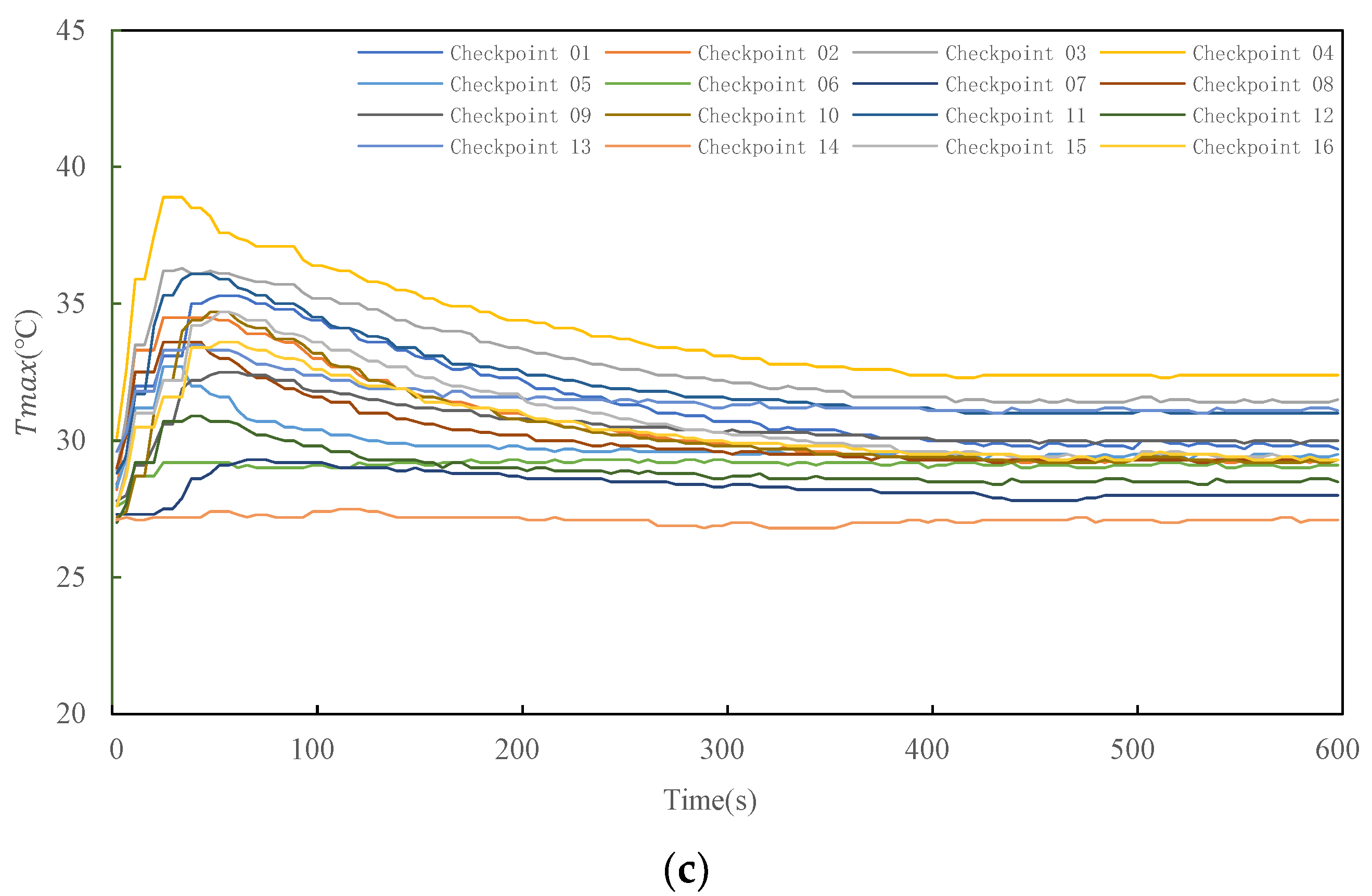
3.1. The Influence of the Pipe Distance at the Coolant Outlet on the Heat Dissipation Performance
3.2. The Influence of Liquid Cooling Plate Thickness on Heat Dissipation Performance
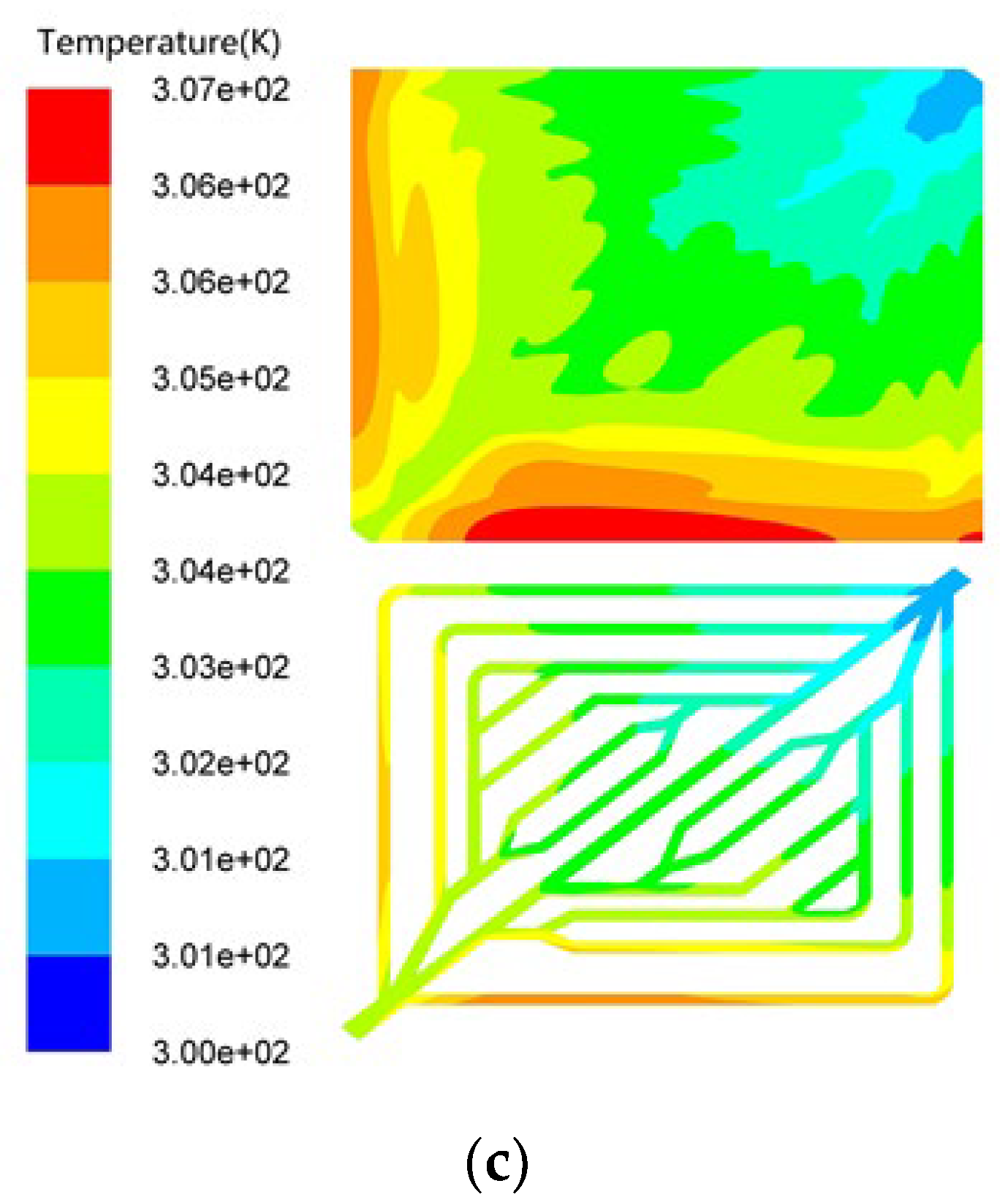
3.3. The Influence of Inner Tube Turning Radius on Heat Dissipation Performance
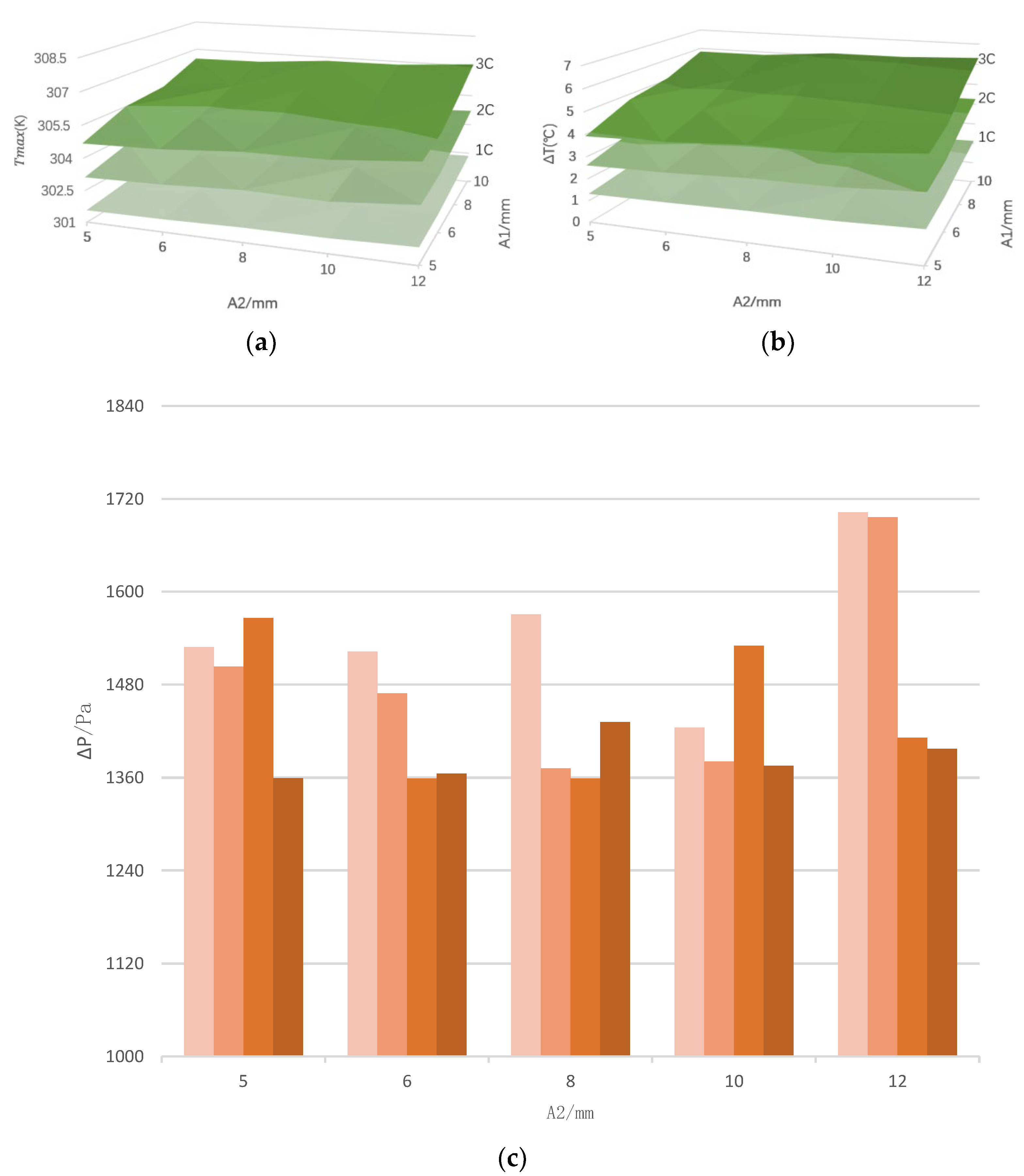
3.4. The Influence of the Flow Channel Area at the Coolant Outlet on the Heat Dissipation Performance of the Liquid Cooling Plate
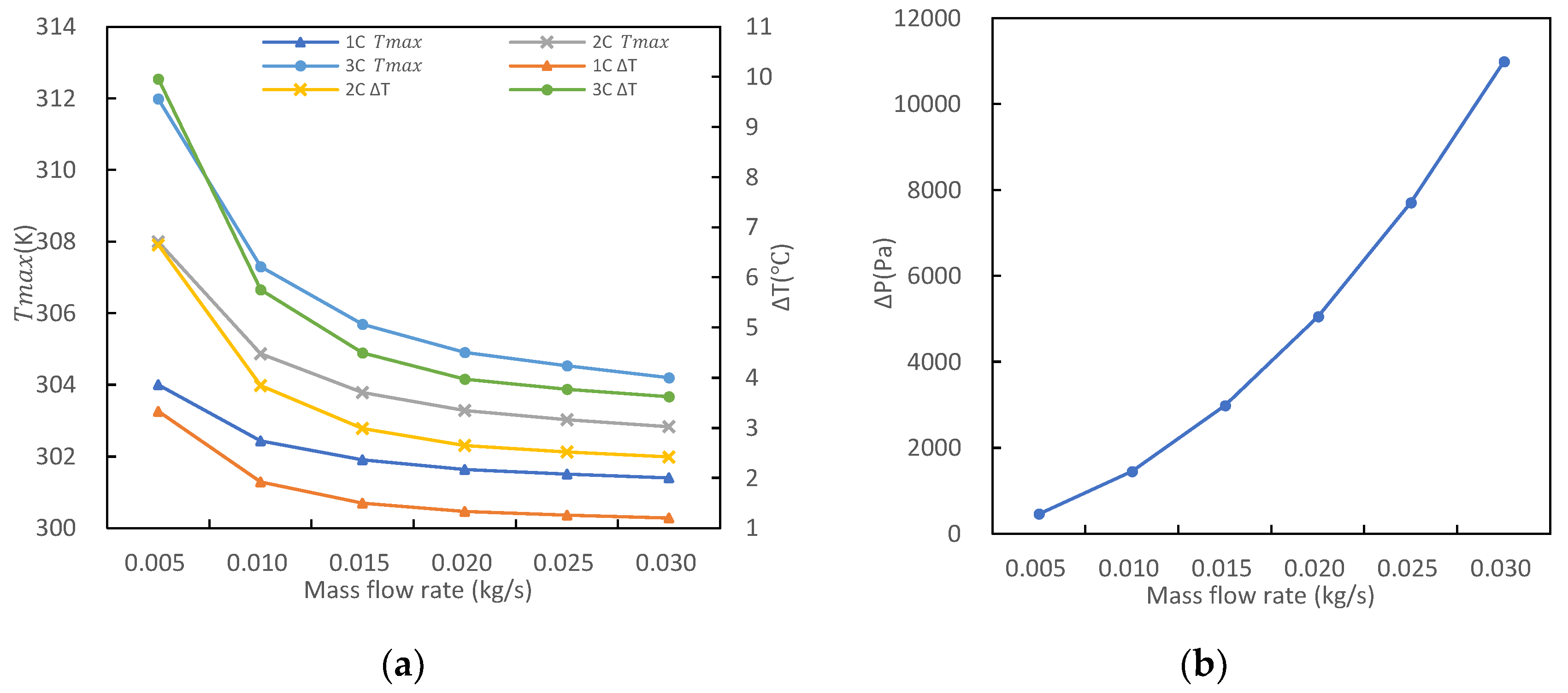
3.5. The Influence of the Coolant Mass Flow Rate on the Heat Dissipation Performance of the Liquid Cooling Plate
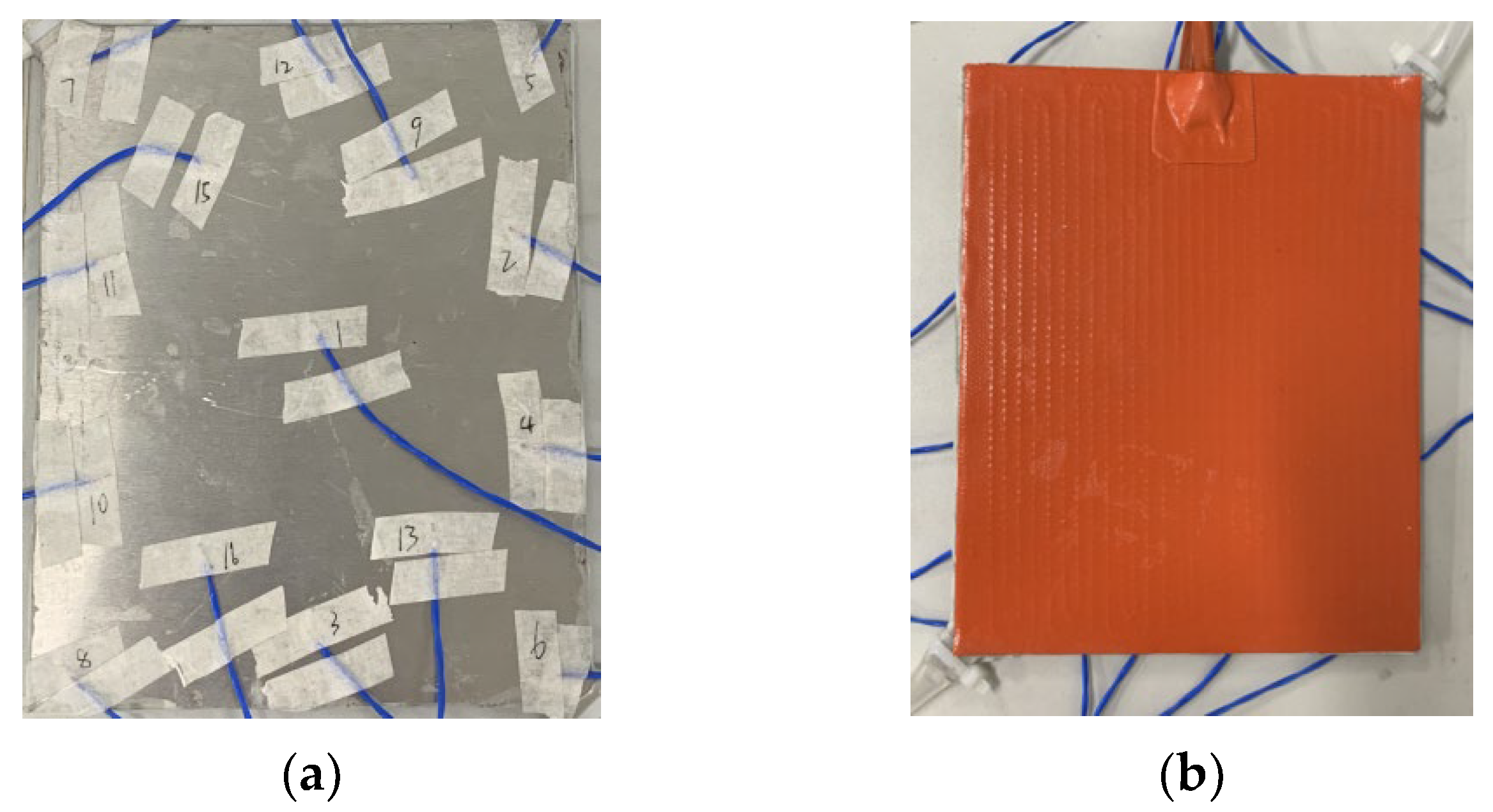
3.6. Experimental Verification
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, C.W.; Chen, S.R.; Gao, H.B.; Xu, K.J.; Xia, Z.; Li, S.T. Study of thermal management system using composite phase change materials and thermoelectric cooling sheet for power battery pack. Energies 2019, 12, 1937. [Google Scholar] [CrossRef] [Green Version]
- Hamut, H.S.; Javani, N.; Dinçer, I. Thermal Management of Electric Vehicle Battery Systems; John Wiley & Sons: Hoboken, NJ, USA, 2017. [Google Scholar]
- Li, L.; Li, W.; Cui, W. A Novel Liquid Cold Plate with Temperature Uniformity for Thermal Management of Power Battery Module. Chin. J. Automot. Eng. 2019, 9, 413–416. [Google Scholar]
- Xie, J.; Xie, N.; Li, B. Research of the thermal characteristics of EVs lithium-ion power battery. Chin. J. Power Sources 2019, 43, 1001–1004. [Google Scholar]
- Su, H.; Li, T.; Jiang, Y.; Guo, C.; Wang, T. Experimental study on visualization of U-shaped array thermosyphon. Appl. Therm. Eng. 2019, 152, 917–924. [Google Scholar] [CrossRef]
- Li, W.Q.; Qu, Z.G.; He, Y.L.; Tao, Y.B. Experimental study of a passive thermal management system for high-powered lithium ion batteries using porous metal foam saturated with phase change materials. J. Power Sources 2014, 255, 9–15. [Google Scholar] [CrossRef]
- Jarrett, A.; Kim, I.Y. Design optimization of electric vehicle battery cooling plates for thermal performance. J. Power Sources 2011, 196, 10359–10368. [Google Scholar] [CrossRef]
- Li, W.; Garg, A.; Xiao, M.; Gao, L. Optimization for liquid cooling cylindrical battery thermal management system based on Gaussian process model. J. Therm. Sci. Eng. Appl. 2020, 13, 021015. [Google Scholar] [CrossRef]
- Wei, Z.; Zhao, J.; Xiong, B. Dynamic electro-thermal modeling of all-vanadium redox flow battery with forced cooling strategies. Appl. Energy 2014, 135, 1–10. [Google Scholar] [CrossRef]
- Wang, Q.; Jiang, B.; Xue, Q.F.; Sun, H.L.; Li, B.; Zou, H.M.; Yan, Y.Y. Experimental investigation on EV battery cooling and heating by heat pipes. Appl. Therm. Eng. 2015, 88, 54–60. [Google Scholar] [CrossRef]
- Tian, Z.; Gan, W.; Zhang, X.; Gu, B.; Yang, L. Investigation on an integrated thermal management system with battery cooling and motor waste heat recovery for electric vehicle. Appl. Therm. Eng. 2018, 136, 16–27. [Google Scholar] [CrossRef]
- Li, W.; Peng, X.; Xiao, M.; Garg, A.; Gao, L. Multi-objective design optimization for mini channel cooling battery thermal management system in an electric vehicle. Int. J. Energy Res. 2019, 43, 3668–3680. [Google Scholar] [CrossRef]
- Du, X.; Qian, Z.; Chen, Z.; Rao, Z. Experimental investigation on mini-channel cooling-based thermal management for Li-ion battery module under different cooling schemes. Int. J. Energy Res. 2018, 42, 2781–2788. [Google Scholar] [CrossRef]
- Zhao, R.; Gu, J.; Liu, J. Optimization of a phase change material based internal cooling system for cylindrical Li-ion battery pack and a hybrid cooling design. Energy 2017, 135, 811–822. [Google Scholar] [CrossRef]
- Chung, Y.; Kim, M.S. Thermal analysis and pack level design of battery thermal management system with liquid cooling for electric vehicles. Energy Convers. Manag. 2019, 196, 105–116. [Google Scholar] [CrossRef]
- Liu, F.F.; Lan, F.C.; Chen, J.Q.; Li, Y.G. Experimental Investigation on Cooling/Heating Characteristics of Ultra-Thin Micro Heat Pipe for Electric Vehicle Battery Thermal Management. Chin. J. Mech. Eng. 2018, 31, 53. [Google Scholar] [CrossRef] [Green Version]
- Gu, Y.Q.; Fan, T.X.; Mou, J.G.; Liu, F.Q.; Jiang, L.F.; Wu, D.H. Advances in Researches on Bionics. J. Biomim. Biomater. Biomed. Eng. 2015, 25, 3–11. [Google Scholar]
- Canning, J.; Peng, G. Proceedings of SPIE-The International Society for Optical Engineering: Introduction. In Proceedings of the SPIE-The International Society for Optical Engineering, Sydney, Australia, 31 January–3 February 2012. [Google Scholar]
- Sun, J.; Dai, Z. Bionics today and tomorrow. Acta Biophys. Sin. 2007, 23, 109–115. [Google Scholar]
- Lininger, C.N.; Bruck, A.M.; Lutz, D.M.; Housel, L.M.; Takeuchi, K.J.; Takeuchi, E.S.; Huq, A.; Marschilok, A.C.; West, A.C. The systematic refinement for the phase change and conversion reactions arising from the lithiation of magnetite nanocrystals. Adv. Funct. Mater. 2020, 30, 1907337. [Google Scholar] [CrossRef]
- Guo, X. Tesla electric vehicle technology analysis. Sci. Technol. Rev. 2016, 34, 98–104. [Google Scholar]
- Brandner, J.J.; Anurjew, E.; Bohn, L.; Hansjosten, E.; Henning, T.; Schygulla, U.; Wenka, A.; Schubert, K. Concepts and realization of microstructure heat exchangers for enhanced heat transfer. Exp. Therm. Fluid Sci. 2006, 30, 801–809. [Google Scholar] [CrossRef]
- Hermann, M. Bionische Ansätze zur Entwicklung Energieeffizienter Fluidsysteme für den Wärmetrans-Port. Ph.D. Thesis, University of Karlsruhe, Karlsruhe, Germany, 2005. [Google Scholar]
- Xia, G.D.; Jiang, J.; Wang, J.; Zhai, Y.L.; Ma, D.D. Effects of different geometric structures on fluid flow and heat transfer performance in microchannel heat sinks. Int. J. Heat Mass Transfer 2015, 80, 439–447. [Google Scholar] [CrossRef]
- Donghai, H.; Yuteng, W.; Jianwei, L.; Qingqing, Y.; Jing, W. Investigation of optimal operating temperature for the PEMFC and its tracking control for energy saving in vehicle applications. Energy Convers. Manag. 2021, 249, 114842. [Google Scholar]
- Donghai, H.; Jie, L.; Fengyan, Y.; Qingqing, Y.; Jiaming, Z. Enhancing heat dissipation to improve efficiency of two-stage electric air compressor for fuel cell vehicle. Energy Convers. Manag. 2021, 251, 115007. [Google Scholar]
- Yi, F.; Lu, D.; Wang, X.; Pan, C.; Tao, Y.; Zhou, J.; Zhao, C. Energy Management Strategy for Hybrid Energy Storage Electric Vehicles Based on Pontryagin’s Minimum Principle Considering Battery Degradation. Sustainability 2022, 14, 1214. [Google Scholar] [CrossRef]
- Xu, S.; Wang, W.; Fang, K.; Wong, C.N. Heat transfer performance of a fractal silicon microchannel heat sin-k subjected to pulsation flow. Int. J. Heat Mass Transf. 2015, 81, 33–40. [Google Scholar] [CrossRef]
- Li, B.; Bei, S.; Zhang, L.; Dai, L.; Kong, C.; Ye, Y.; Zhang, W.; Shi, H. A Graphene Battery Thermal Management System. Chinese Patent ZL201610917145.7, 4 January 2017. [Google Scholar]
- Xie, J.; Ge, Z.; Zang, M.; Wang, S. Structural optimization of lithium-ion battery pack with forced air cooling system. Appl. Therm. Eng. 2017, 126, 583–593. [Google Scholar] [CrossRef]





| Parameter | ||||
|---|---|---|---|---|
| Aluminum | 871 | 202.4 | - | 2719 |
| Water | 4182 | 0.6 | 1.003 × 10−4 | 998.2 |
| Boundary Conditions | Parameter Value |
|---|---|
| Inlet mass flow (kg/s) | 0.02 |
| Inlet temperature T0 (K) | 300 |
| Outlet pressure (Pa) | 0 |
| Grid Size/mm | Total Number of Grids | Maximum Temperature (K) | Temperature Difference (°C) |
|---|---|---|---|
| 0.75 | 1,240,995 | 307.11 | 6.44 |
| 0.7 | 1,498,009 | 307.09 | 6.32 |
| 0.6 | 2,290,503 | 307.04 | 6.39 |
| 0.5 | 3,593,672 | 307.05 | 6.43 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, B.; Wang, W.; Bei, S.; Quan, Z. Analysis of Heat Dissipation Performance of Battery Liquid Cooling Plate Based on Bionic Structure. Sustainability 2022, 14, 5541. https://doi.org/10.3390/su14095541
Li B, Wang W, Bei S, Quan Z. Analysis of Heat Dissipation Performance of Battery Liquid Cooling Plate Based on Bionic Structure. Sustainability. 2022; 14(9):5541. https://doi.org/10.3390/su14095541
Chicago/Turabian StyleLi, Bo, Wenhao Wang, Shaoyi Bei, and Zhengqiang Quan. 2022. "Analysis of Heat Dissipation Performance of Battery Liquid Cooling Plate Based on Bionic Structure" Sustainability 14, no. 9: 5541. https://doi.org/10.3390/su14095541
APA StyleLi, B., Wang, W., Bei, S., & Quan, Z. (2022). Analysis of Heat Dissipation Performance of Battery Liquid Cooling Plate Based on Bionic Structure. Sustainability, 14(9), 5541. https://doi.org/10.3390/su14095541






