Experimental Study on the Mechanical Characteristics of Saturated Granite under Conventional Triaxial Loading and Unloading Tests
Abstract
:1. Introduction
| Rock Type | Stress Path | σ3 (MPa) | Unloading Rate vu (MPa/s) | Reference |
|---|---|---|---|---|
| Sandstone | 1. Increase σ1, unload σ3 | 4–19 | 0.02–0.14 | [47] |
| 4–10 | 0.05 | [48] | ||
| 5–30 | 0.005 | [49] | ||
| 15–30 | 0.05, 0.1, 0.2 | [50] | ||
| 15–45 | 2 | [51] | ||
| 2. Keep σ1, unload σ3 | 15–30 | 0.0003–0.1667 | [52] | |
| 1. Increase σ1, unload σ3 2. Keep σ1, unload σ3 | 10–30 | 0.1, 0.5 | [53] | |
| 3. Unload σ1, σ3 | 10–30 | 0.0008 | [33] | |
| Marble | 1. Increase σ1, unload σ3 | 40 | 0.05 | [37] |
| 2. Keep σ1, unload σ3 | 20 | 0.01–0.2 | [40,42] | |
| 10–60 | 0.01–1 | [54] | ||
| 3. Unload σ1, σ3 | 20–60 | 0.05 | [55] | |
| 20–40 | 0.1–10 | [11] | ||
| 1. Increase σ1, unload σ3 3. Unload σ1, σ3 | 20–40 | 0.26-1.28 | [26] | |
| Granite | 1. Increase σ1, unload σ3 | 5–30 | 0.0017–0.0333 | [56] |
| 30–60 | 0.05 | [57] | ||
| 2. Keep σ1, unload σ3 | 10 | 0.005–0.0115 | [19] | |
| 10–30 | 0.1 | [17,58] | ||
| 1. Increase σ1, unload σ3 3. Unload σ1, σ3 | 10–60 | 0.2 | [45] | |
| 1. Increase σ1, unload σ3 2. Keep σ1, unload σ3 3. Unload σ1, σ3 | 10–30 | - | [43] | |
| Mudstone | 1. Increase σ1, unload σ3 | 6-15 | 0.05, 0.1, 0.2 | [50] |
| 10–50 | 0.005–0.5 | [59] | ||
| Limestones | 2. Keep σ1, unload σ3 | 15 | 0.0217 | [39] |
| Shale | 1. Increase σ1, unload σ3 | 20–60 | 0.4–1.0 | [27] |
| 2. Keep σ1, unload σ3 | 20–60 | 0.4 | [27] | |
| Basalt | 1. Increase σ1, unload σ3 | 3–12 | 0.008 | [60] |
| 3. Unload σ1, σ3 | 3–12 | 0.008 | [60] | |
| Coal | 1. Increase σ1, unload σ3 | 30 | 0.001–0.005 | [34] |
| 4–8 | 0.012–0.024 | [35] | ||
| 4–10 | 0.02–0.14 | [47] | ||
| Dacite | 2. Keep σ1, unload σ3 | 5–15 | 0.0083 | [44] |
| Rock salt | 2. Keep σ1, unload σ3 | 15–25 | 0.005 | [10] |
| 23 | 0.001–0.5 | [31] | ||
| Mudstone | 2. Keep σ1, unload σ3 | 8–20 | 0.05 | [61] |
| 3. Unload σ1, σ3 | 8–20 | 0.05 | [61] | |
| Rock-Like | 2. Keep σ1, unload σ3 | 4–8 | 0.0083–0.0333 | [30] |
2. Materials and Methods
2.1. Specimen Preparation
2.2. Test Scheme
3. Results and Discussion
3.1. Mechanical Characteristics
3.1.1. Stress–Strain Curve
3.1.2. Strength Characteristics
3.1.3. Strain Characteristics
3.2. Energy Evolution Characteristics
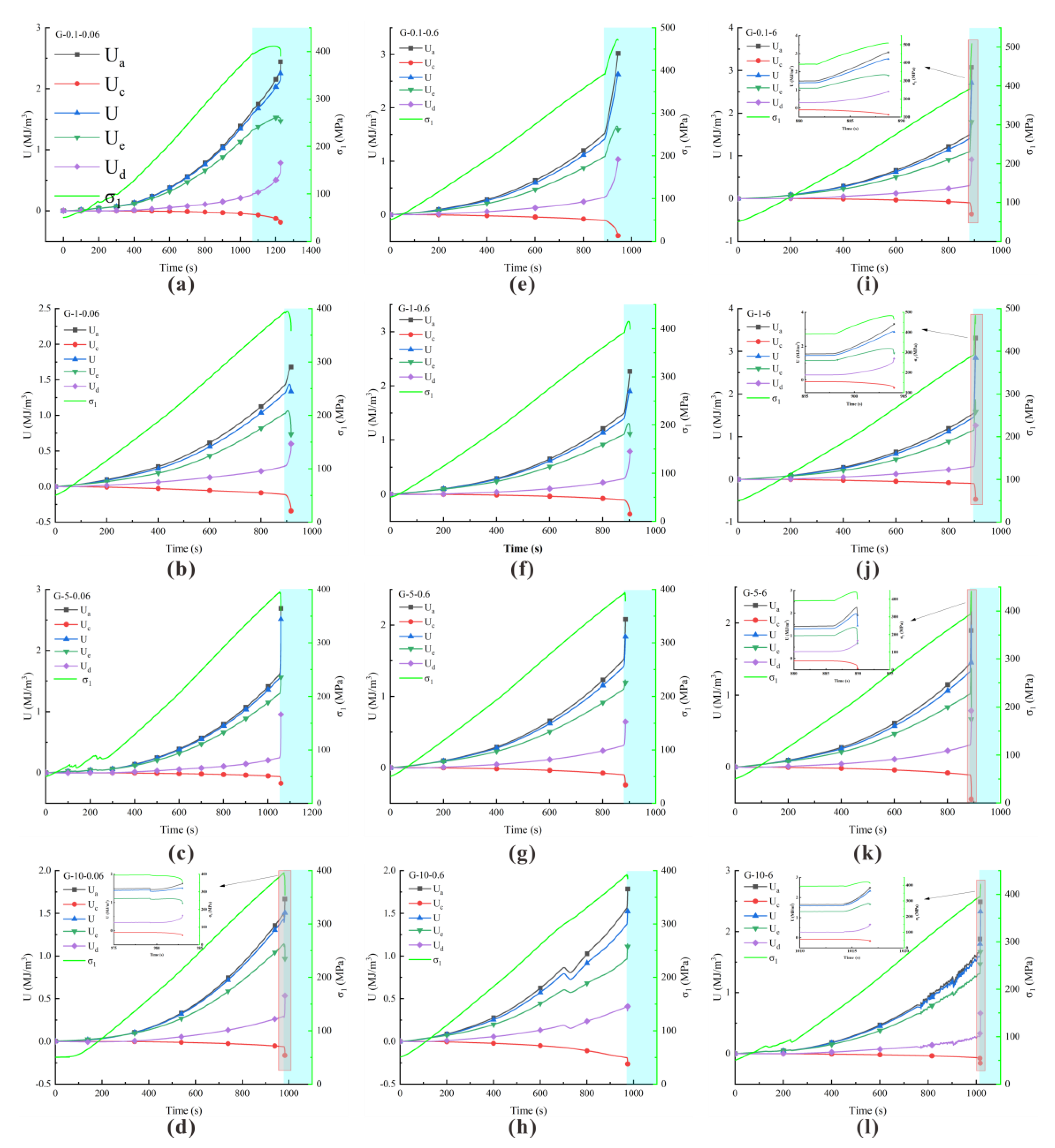
3.2.1. Evolution of Strain Energy in the Triaxial Compression Test
3.2.2. Evolution of Strain Energy in a Triaxial Compression Unloading Confining Pressure Test
3.3. Rock Failure Characteristics and Destruction Mechanism
4. Conclusions
- (1)
- In the conventional triaxial unloading test, the circumferential deformation is the leading cause of failure, and its strain is greater than that of conventional triaxial loading. Under the same axial loading rate, the faster the unloading rate and axial loading rate, the smaller the σ3f and σ1f at the time of failure, and the failure point is outside the envelope of conventional triaxial compression tests. While under the same unloading rate, a faster axial loading corresponds to a larger σ3f and σ1f.
- (2)
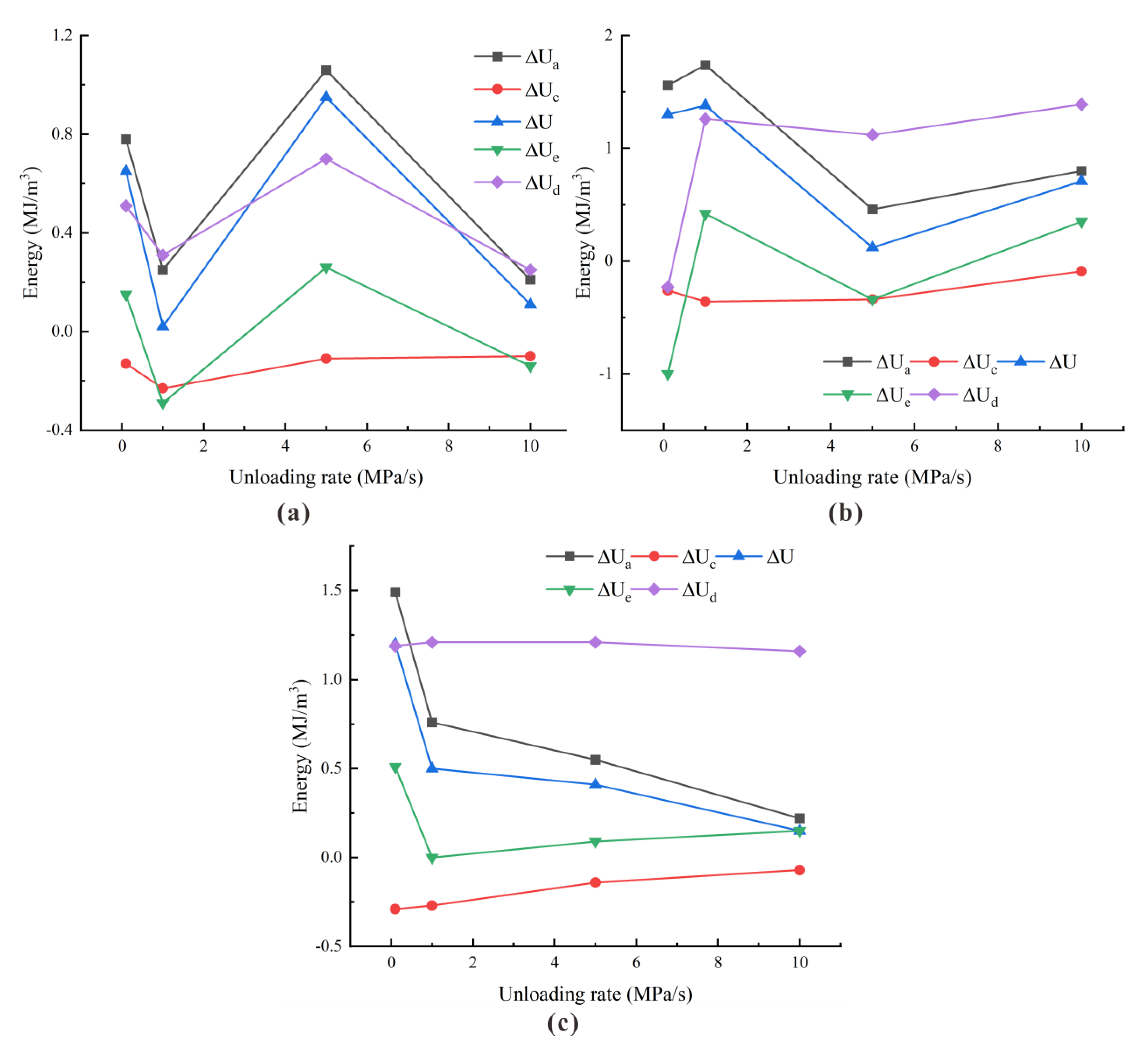
- The variation of circumferential energy ΔUc decreases with the unloading rate, and ΔUd, ΔUa, ΔU and ΔUe showed inverted V-shaped and V-shaped va = 0.06 and 0.6 mm/min, respectively. When va = 6 mm/min, except for a slight increase in ΔUa, the rest of the variation of energy decreases with the increase of the unloading rate. The variation of dissipation energy changes from being determined by the unloading rate and the axial loading rate together to being determined by the axial loading rate with the axial loading rate increasing.
- (3)
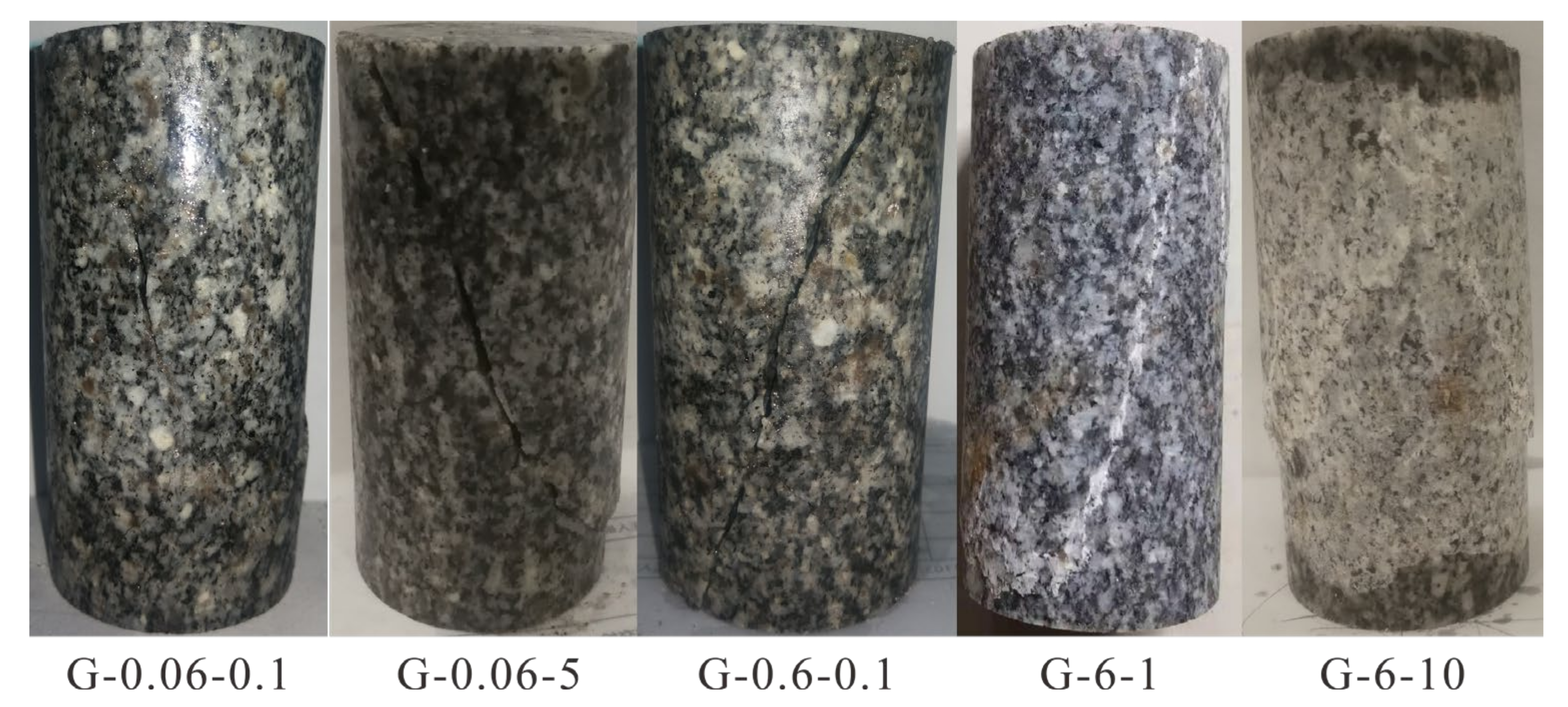
- The failure modes of the specimens were mainly controlled by the axial stress and showed a single shear crack before va = 0.6 mm/min, while it is influenced by both axial and circumferential stress after the axial loading rate of not less than 6 mm/min, and the failure surface is a conjugate shear failure and tensile strain failure.
- (4)
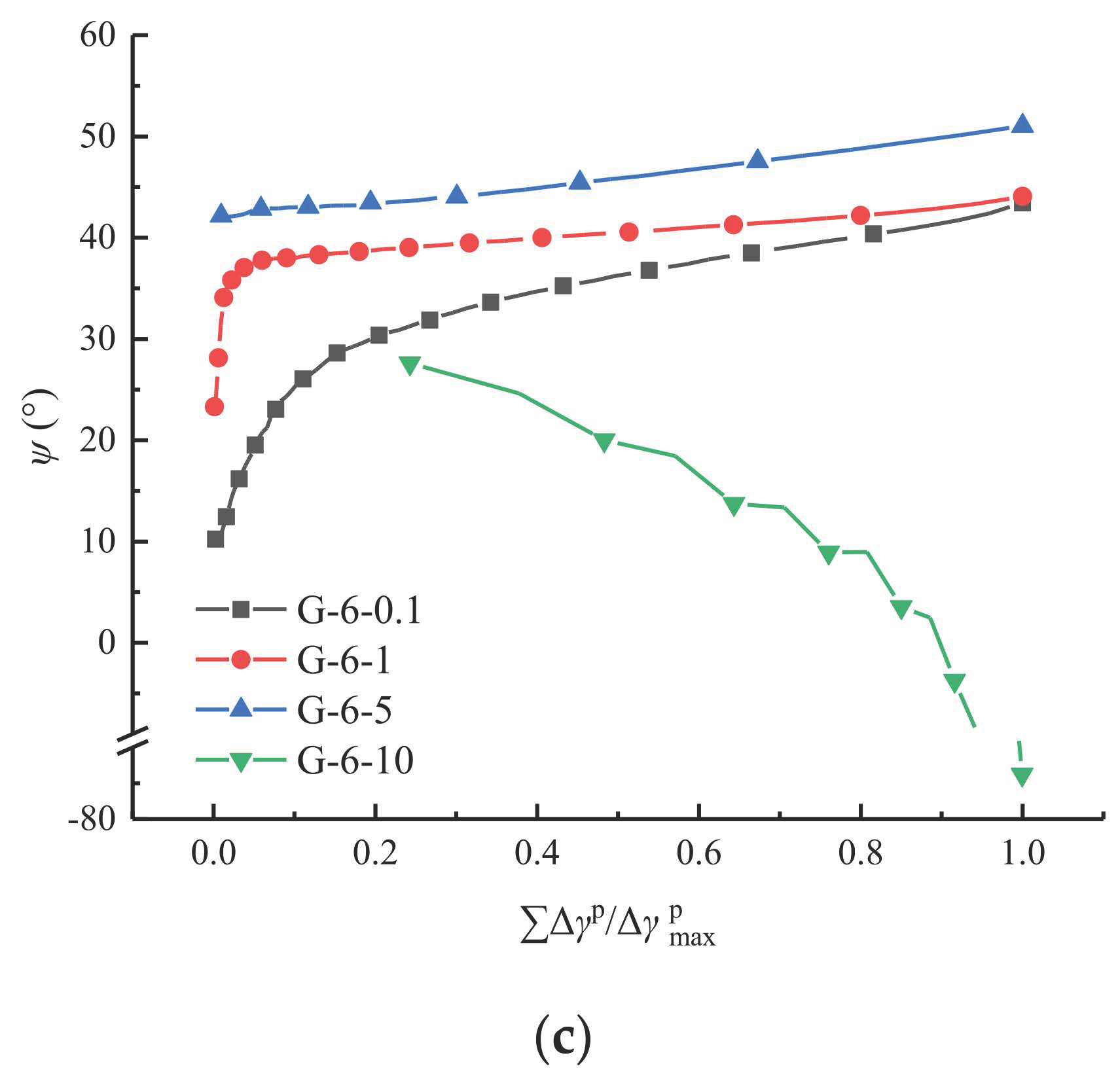
- In the triaxial unloading test, the specimens were in the high expansion process most of the time, and the dilatancy angle of the specimens showed an overall upward trend except for vu = 10 MPa/s, va = 0.6 mm/min and 6 mm/min.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, X.; Gong, F.; Tao, M.; Dong, L.; Du, K.; Ma, C.; Zhou, Z.; Yin, T. Failure mechanism and coupled static-dynamic loading theory in deep hard rock mining: A review. J. Rock Mech. Geotech. Eng. 2017, 9, 767–782. [Google Scholar] [CrossRef]
- Liu, Z.; Ma, C.; Wei, X.A.; Xie, W. Experimental Study of Rock Subjected to Triaxial Extension. Rock Mech. Rock Eng. 2022, 55, 1069–1077. [Google Scholar] [CrossRef]
- Yi, W.; Rao, Q.-H.; Sun, D.-L.; Shen, Q.-Q.; Zhang, J. A new integral equation method for calculating interacting stress-intensity factors of multiple holed-cracked anisotropic rock under both far-field and arbitrary surface stresses. Int. J. Rock Mech. Min. Sci. 2021, 148, 104926. [Google Scholar] [CrossRef]
- Fereidooni, D. Determination of the Geotechnical Characteristics of Hornfelsic Rocks with a Particular Emphasis on the Correlation Between Physical and Mechanical Properties. Rock Mech. Rock Eng. 2016, 49, 2595–2608. [Google Scholar] [CrossRef]
- Gautam, L.; Jain, J.K.; Jain, A.; Kalla, P. Valorization of bone-china ceramic powder waste along with granite waste in self-compacting concrete. Constr. Build. Mater. 2022, 315, 125730. [Google Scholar] [CrossRef]
- Gautam, P.K.; Dwivedi, R.; Kumar, A.; Kumar, A.; Verma, A.K.; Singh, K.H.; Singh, T.N. Damage Characteristics of Jalore Granitic Rocks After Thermal Cycling Effect for Nuclear Waste Repository. Rock Mech. Rock Eng. 2021, 54, 235–254. [Google Scholar] [CrossRef]
- Gautam, P.K.; Verma, A.K.; Sharma, P.; Singh, T.N. Evolution of Thermal Damage Threshold of Jalore Granite. Rock Mech. Rock Eng. 2018, 51, 2949–2956. [Google Scholar] [CrossRef]
- Gautam, P.K.; Jha, M.K.; Verma, A.K.; Singh, T.N. Evolution of absorption energy per unit thickness of damaged sandstone. J. Therm. Anal. Calorim. 2019, 136, 2305–2318. [Google Scholar] [CrossRef]
- Xie, H.Q.; He, C. Study of the unloading characteristics of a rock mass using the triaxial test and damage mechanics. Int. J. Rock Mech. Min. Sci. 2004, 41, 366. [Google Scholar] [CrossRef]
- Chen, J.; Jiang, D.Y.; Ren, S.; Yang, C.H. Comparison of the characteristics of rock salt exposed to loading and unloading of confining pressures. Acta Geotech. 2016, 11, 221–230. [Google Scholar] [CrossRef]
- Huang, D.; Li, Y. Conversion of strain energy in Triaxial Unloading Tests on Marble. Int. J. Rock Mech. Min. Sci. 2014, 66, 160–168. [Google Scholar] [CrossRef]
- Ma, C.; Xie, W.; Liu, Z.; Li, Q.; Xu, J.; Tan, G. A New Technology for Smooth Blasting without Detonating Cord for Rock Tunnel Excavation. Appl. Sci. 2020, 10, 6764. [Google Scholar] [CrossRef]
- Sato, T.; Kikuchi, T.; Sugihara, K. In-situ experiments on an excavation disturbed zone induced by mechanical excavation in Neogene sedimentary rock at Tone mine, central Japan. Eng. Geol. 2000, 56, 97–108. [Google Scholar] [CrossRef]
- Wei, X.A.; Li, Q.; Ma, C.; Dong, L.; Zheng, J.; Huang, X. Experimental investigations of direct measurement of borehole wall pressure under decoupling charge. Tunn. Undergr. Space Technol. 2022, 120, 104280. [Google Scholar] [CrossRef]
- Xiao, F.; Jiang, D.; Wu, F.; Zou, Q.; Chen, J.; Chen, B.; Sun, Z. Effects of prior cyclic loading damage on failure characteristics of sandstone under true-triaxial unloading conditions. Int. J. Rock Mech. Min. Sci. 2020, 132, 104379. [Google Scholar] [CrossRef]
- He, J.; Wang, Z.; Bai, J.; Zhang, L. Study on the Hoek-Brown parameters under loading and unloading triaxial laboratory tests. In Proceedings of the 1st International Conference on Civil Engineering, Architecture and Building Materials (CEABM 2011), Haikou, China, 18–20 June 2011; pp. 2074–2078. [Google Scholar]
- Duan, K.; Ji, Y.L.; Wu, W.; Kwok, C.Y. Unloading-induced failure of brittle rock and implications for excavation induced strain burst. Tunn. Undergr. Space Technol. 2019, 84, 495–506. [Google Scholar] [CrossRef]
- Ding, Q.-L.; Ju, F.; Mao, X.-B.; Ma, D.; Yu, B.-Y.; Song, S.-B. Experimental Investigation of the Mechanical Behavior in Unloading Conditions of Sandstone After High-Temperature Treatment. Rock Mech. Rock Eng. 2016, 49, 2641–2653. [Google Scholar] [CrossRef]
- Kang, P.; Jing, Z.; Zou, Q.L.; Xiao, S. Deformation characteristics of granites at different unloading rates after high-temperature treatment. Environ. Earth Sci. 2020, 79, 343. [Google Scholar] [CrossRef]
- Yi, W.; Rao, Q.-H.; Li, Z.; Sun, D.-L.; Shen, Q.-Q. Thermo-hydro-mechanical-chemical (THMC) coupling fracture criterion of brittle rock. Trans. Nonferrous Met. Soc. China 2021, 31, 2823–2835. [Google Scholar] [CrossRef]
- Ma, Y.; Rao, Q.; Huang, D.; Yi, W.; He, Y. A new theoretical model of local air-leakage seepage field for the compressed air energy storage lined cavern. J. Energy Storage 2022, 49, 104160. [Google Scholar] [CrossRef]
- Azarafza, M.; Ghazifard, A.; Akgun, H.; Asghari-Kaljahi, E. Geotechnical characteristics and empirical geo-engineering relations of the South Pars Zone marls, Iran. Geomech. Eng. 2019, 19, 393–405. [Google Scholar] [CrossRef]
- Faradonbeh, R.S.; Taheri, A.; Ribeiro e Sousa, L.; Karakus, M. Rockburst assessment in deep geotechnical conditions using true-triaxial tests and data-driven approaches. Int. J. Rock Mech. Min. Sci. 2020, 128, 104279. [Google Scholar] [CrossRef]
- Fern, E.J.; Di Murro, V.; Soga, K.; Li, Z.L.; Scibile, L.; Osborne, J.A. Geotechnical characterisation of a weak sedimentary rock mass at CERN, Geneva. Tunn. Undergr. Space Technol. 2018, 77, 249–260. [Google Scholar] [CrossRef] [Green Version]
- Yi, W.; Rao, Q.-H.; Luo, S.; Shen, Q.-Q.; Li, Z. A new integral equation method for calculating interacting stress intensity factor of multiple crack-hole problem. Theor. Appl. Fract. Mech. 2020, 107, 102535. [Google Scholar] [CrossRef]
- Huang, R.-Q.; Huang, D. Experimental research on affection laws of unloading rates on mechanical properties of Jinping marble under high geostress. Chin. J. Rock Mech. Eng. 2010, 29, 21–33. [Google Scholar]
- Guo, Y.; Wang, L.; Chang, X. Study on the damage characteristics of gas-bearing shale under different unloading stress paths. PLoS ONE 2019, 14, e0224654. [Google Scholar] [CrossRef]
- Liu, Z.; Ma, C.; Wei, X.A.; Xie, W. Experimental study on mechanical properties and failure modes of pre-existing cracks in sandstone during uniaxial tension/compression testing. Eng. Fract. Mech. 2021, 255, 107966. [Google Scholar] [CrossRef]
- Liu, Z.; Ma, C.; Wei, X.-A. Electron scanning characteristics of rock materials under different loading methods: A review. Geomech. Geophys. Geo 2022, 8, 80. [Google Scholar] [CrossRef]
- Xu, J.; Li, H.; Meng, Q.; Xu, W.; He, M.; Yang, J. A Study on Triaxial Unloading Test of Columnar-Jointed-Rock-Mass-Like Material with AW Velocity Analysis. Adv. Civ. Eng. 2020, 2020, 6693544. [Google Scholar] [CrossRef]
- Han, Y.; Ma, H.; Yang, C.; Li, H.; Yang, J. The mechanical behavior of rock salt under different confining pressure unloading rates during compressed air energy storage (CAES). J. Pet. Sci. Eng. 2021, 196, 107676. [Google Scholar] [CrossRef]
- Cong, Y.; Wang, Z.Q.; Zheng, Y.R.; Zhang, L.M. Effect of Unloading Stress Levels on Macro- and Microfracture Mechanisms in Brittle Rocks. Int. J. Geomech. 2020, 20, 04020066. [Google Scholar] [CrossRef]
- Chen, X.; Tang, C.A.; Yu, J.; Zhou, J.F.; Cai, Y.Y. Experimental investigation on deformation characteristics and permeability evolution of rock under confining pressure unloading conditions. J. Cent. South Univ. 2018, 25, 1987–2001. [Google Scholar] [CrossRef]
- Xue, Y.; Ranjith, P.G.; Gao, F.; Zhang, D.; Cheng, H.; Chong, Z.; Hou, P. Mechanical behaviour and permeability evolution of gas-containing coal from unloading confining pressure tests. J. Nat. Gas Sci. Eng. 2017, 40, 336–346. [Google Scholar] [CrossRef]
- Wang, K.; Zheng, J.-Y.; Zhu, K.-S. Analysis of deformation failure characteristics and energy of anthracite under two kinds of stress paths. Rock Soil Mech. 2015, 36, 259–266. [Google Scholar] [CrossRef]
- Yi, W.; Rao, Q.; Ma, W.; Sun, D.; Shen, Q. A new analytical-numerical method for calculating interacting stresses of a multi-hole problem under both remote and arbitrary surface stresses. Appl. Math. Mech. Engl. Ed. 2020, 41, 1539–1560. [Google Scholar] [CrossRef]
- Chen, J.; Zhou, H.; Zeng, Z.Q.; Lu, J.J. Macro- and Microstructural Characteristics of the Tension-Shear and Compression-Shear Fracture of Granite. Rock Mech. Rock Eng. 2020, 53, 201–209. [Google Scholar] [CrossRef]
- Yang, Y.J.; Zhou, Y.; Ma, D.P.; Ji, H.Y.; Zhang, Y.D. Acoustic Emission Characteristics of Coal under Different Triaxial Unloading Conditions. Acta Geodyn. Geomater. 2020, 17, 51–60. [Google Scholar] [CrossRef] [Green Version]
- Zhou, X.P.; Zhang, Y.X.; Ha, Q.L. Real-time computerized tomography (CT) experiments on limestone damage evolution during unloading. Theor. Appl. Fract. Mech. 2008, 50, 49–56. [Google Scholar] [CrossRef]
- Zhou, K.P.; Liu, T.Y.; Hu, Z.X. Exploration of damage evolution in marble due to lateral unloading using nuclear magnetic resonance. Eng. Geol. 2018, 244, 75–85. [Google Scholar] [CrossRef]
- Wu, H.; Zhu, H.-H.; Zhang, C.-C.; Zhou, G.-Y.; Zhu, B.; Zhang, W.; Azarafza, M. Strain integration-based soil shear displacement measurement using high-resolution strain sensing technology. Measurement 2020, 166, 108210. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, Q.H.; Xiao, Y.G.; Hou, Z.Q. Influence of time-lagged unloading paths on fracture behaviors of marble using energy analysis and post-test CT visualization. Environ. Earth Sci. 2020, 79, 217. [Google Scholar] [CrossRef]
- Zhao, G.Y.; Dai, B.; Dong, L.J.; Yang, C. Energy conversion of rocks in process of unloading confining pressure under different unloading paths. Trans. Nonferrous Met. Soc. China 2015, 25, 1626–1632. [Google Scholar] [CrossRef]
- Wang, S.; Wang, H.; Xu, W.; Qian, W. Investigation on mechanical behaviour of dacite under loading and unloading conditions. Geotech. Lett. 2019, 9, 130–135. [Google Scholar] [CrossRef]
- Li, D.Y.; Sun, Z.; Xie, T.; Li, X.B.; Ranjith, P.G. Energy evolution characteristics of hard rock during triaxial failure with different loading and unloading paths. Eng. Geol. 2017, 228, 270–281. [Google Scholar] [CrossRef]
- Yi, W.; Rao, Q.; Zhu, W.; Shen, Q.; Li, Z.; Ma, W. Interacting Stress Intensity Factors of Multiple Elliptical-Holes and Cracks Under Far-Field and Arbitrary Surface Stresses. Adv. Appl. Math. Mech. 2022, 14, 125–154. [Google Scholar] [CrossRef]
- Ma, T.R.; Ma, D.P.; Yang, Y.J. Fractal Characteristics of Coal and Sandstone Failure under Different Unloading Confining Pressure Tests. Adv. Mater. Sci. Eng. 2020, 2020, 2185492. [Google Scholar] [CrossRef] [Green Version]
- Yang, R.; Ma, D.P.; Yang, Y.J. Experimental Investigation of Energy Evolution in Sandstone Failure during Triaxial Unloading Confining Pressure Tests. Adv. Civ. Eng. 2019, 2019, 7419752. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.X.; Ma, W.J.; Huang, J.W.; Liao, Z.Y. Mechanical properties of sandstone under loading and unloading Conditions. In Proceedings of the 3rd International Conference on Materials Science and Engineering (ICMSE 2014), Jiujiang, China, 24–26 January 2014; pp. 441–446. [Google Scholar]
- Chen, X.J.; Li, L.Y.; Wang, L.; Qi, L.L. The current situation and prevention and control countermeasures for typical dynamic disasters in kilometer-deep mines in China. Saf. Sci. 2019, 115, 229–236. [Google Scholar] [CrossRef]
- Liu, X.-R.; Liu, J.; Li, D.-L.; He, C.-M.; Wang, Z.-J.; Xie, Y.-K. Experimental research on the effect of different initial unloading levels on mechanical properties of deep-buried sandstone. Rock Soil Mech. 2017, 38, 3081–3088. [Google Scholar] [CrossRef]
- Dong, X.H.; Yang, G.S.; Tian, J.F.; Rong, T.L.; Jia, H.L.; Liu, H. Characteristics of deformation properties of frozen sandstone under lateral unloading condition. Rock Soil Mech. 2018, 39, 2518–2526. [Google Scholar] [CrossRef]
- Xue, J.H.; Du, X.H.; Ma, Q.; Zhan, K.L. Experimental study on law of limit storage energy of rock under different confining pressures. Arab. J. Geosci 2021, 14, 62. [Google Scholar] [CrossRef]
- Qiu, S.; Feng, X.; Zhang, C.Q.; Zhou, H.; Sun, F. Experimental research on mechanical properties of deep-buried marble under different unloading rates of confining pressures. Chin. J. Rock Mech. Eng. 2010, 29, 1807–1817. [Google Scholar]
- Li, X.; Zhao, H.; Wang, B.; Xiao, T. Mechanical properties of deep-buried marble material under loading and unloading tests. J. Wuhan Univ. Technol. Mater. Sci. Ed. 2013, 28, 514–520. [Google Scholar] [CrossRef]
- Wang, C.S.; Zhou, H.W.; He, S.S.; Wang, Z.H.; Liu, J.F. Effect of unloading rates on strength of Beishan granite. Rock Soil Mech. 2017, 38, 151–157. [Google Scholar] [CrossRef]
- Wang, C.; Liu, J.; Chen, L.; Liu, J.; Wang, L. Mechanical behaviour and damage evolution of Beishan granite considering the transient and time-dependent effects of excavation unloading. Eur. J. Environ. Civ. Eng. 2020, 1–17. [Google Scholar] [CrossRef]
- Peng, C.; Wang, J.; Liu, H.; Li, G.; Zhao, W. Investigating the Macro-Micromechanical Properties and Failure Law of Granite under Loading and Unloading Conditions. Adv. Civ. Eng. 2021, 2021, 9983427. [Google Scholar] [CrossRef]
- Huang, X.; Liu, Q.S.; Liu, B.; Liu, X.W.; Pan, Y.C.; Liu, J.P. Experimental Study on the Dilatancy and Fracturing Behavior of Soft Rock Under Unloading Conditions. Int. J. Civ. Eng. 2017, 15, 921–948. [Google Scholar] [CrossRef]
- Xu, X.; Huang, R.; Li, H.; Huang, Q. Determination of Poisson’s Ratio of Rock Material by Changing Axial Stress and Unloading Lateral Stress Test. Rock Mech. Rock Eng. 2015, 48, 853–857. [Google Scholar] [CrossRef]
- Ren, Q.Y.; Zhang, H.M.; Liu, J.S. Rheological properties of mudstone under two unloading paths in experiments. Rock Soil Mech. 2019, 40, 127–134. [Google Scholar] [CrossRef]
- Ulusay, R. The ISRM Suggested Methods for Rock Characterization, Testing and Monitoring: 2007–2014; Springer International Publishing: Berlin, Germany, 2014. [Google Scholar]
- Vermeer, P.A.; De Borst, R. Non-associated plasticity for soils, concrete and rock. HERON 1984, 29, 3–64. [Google Scholar]
- Liu, Z.; Ma, C.; Wei, X.A.; Xie, W. Experimental Study on the Mechanical Characteristics of Single-Fissure Sandstone Under Triaxial Extension. Rock Mech. Rock Eng. 2022, 2020, 9374352. [Google Scholar] [CrossRef]
- Srinivasan, V.; Gupta, T.; Ansari, T.A.; Singh, T.N. An experimental study on rock damage and its influence in rock stress memory in a metamorphic rock. Bull. Eng. Geol. Environ. 2020, 79, 4335–4348. [Google Scholar] [CrossRef]

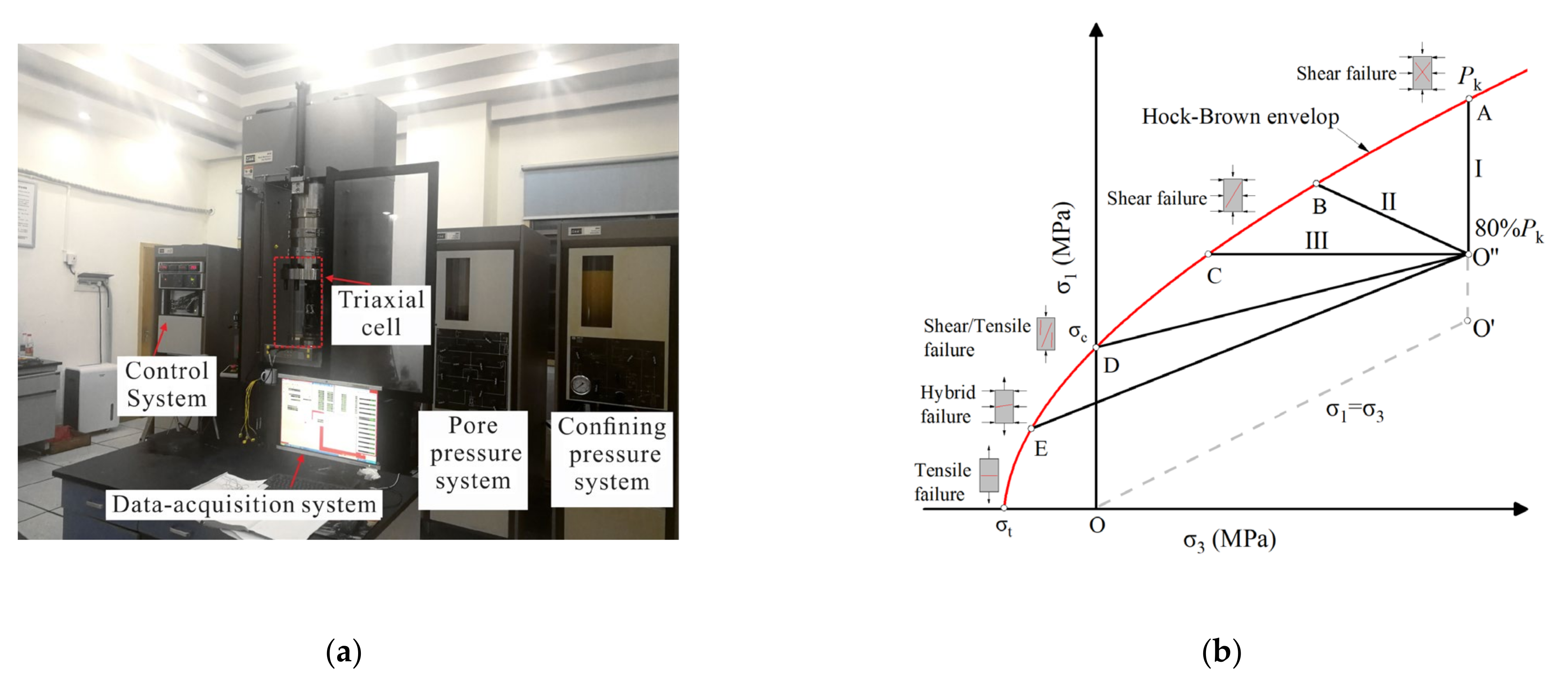

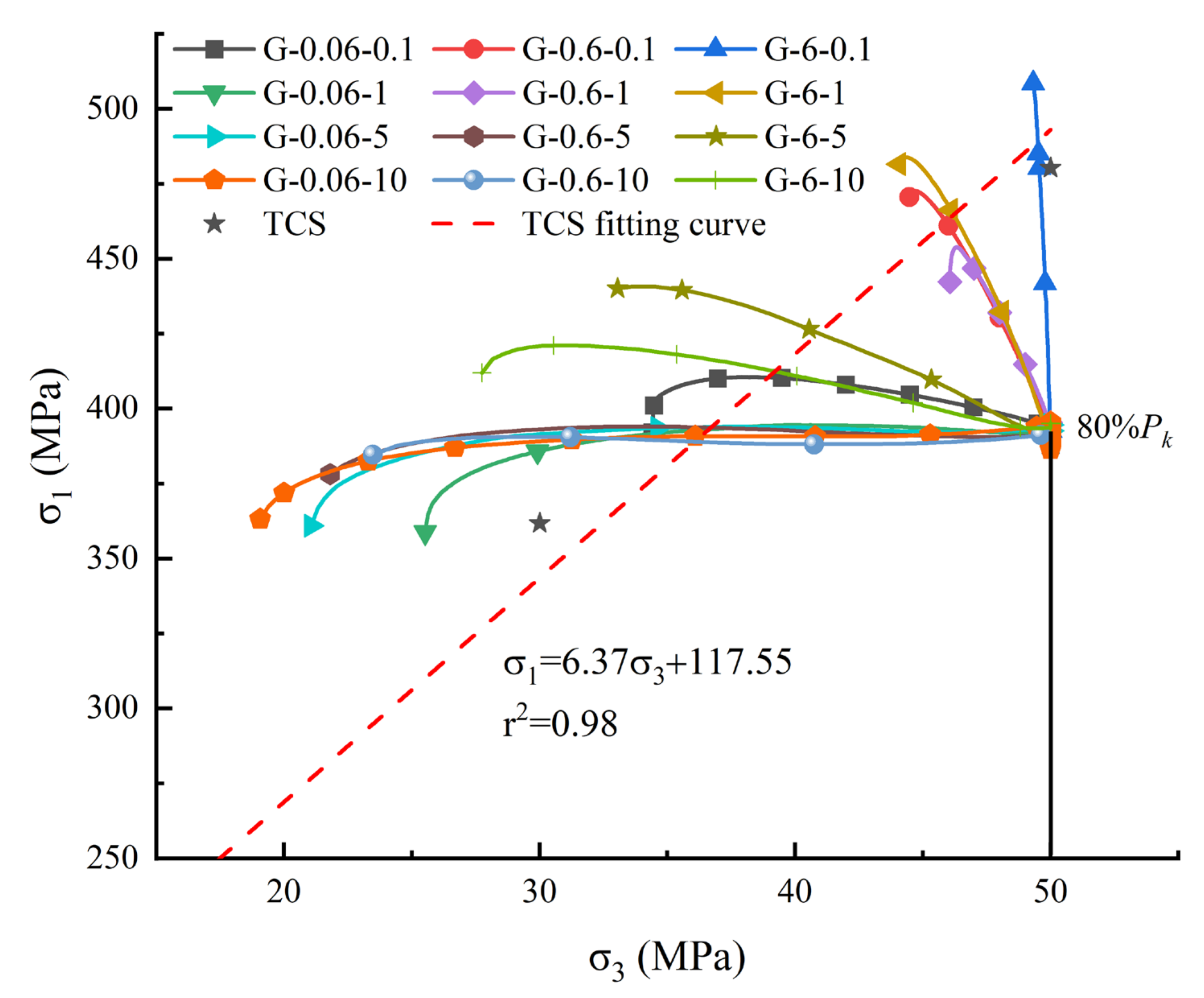
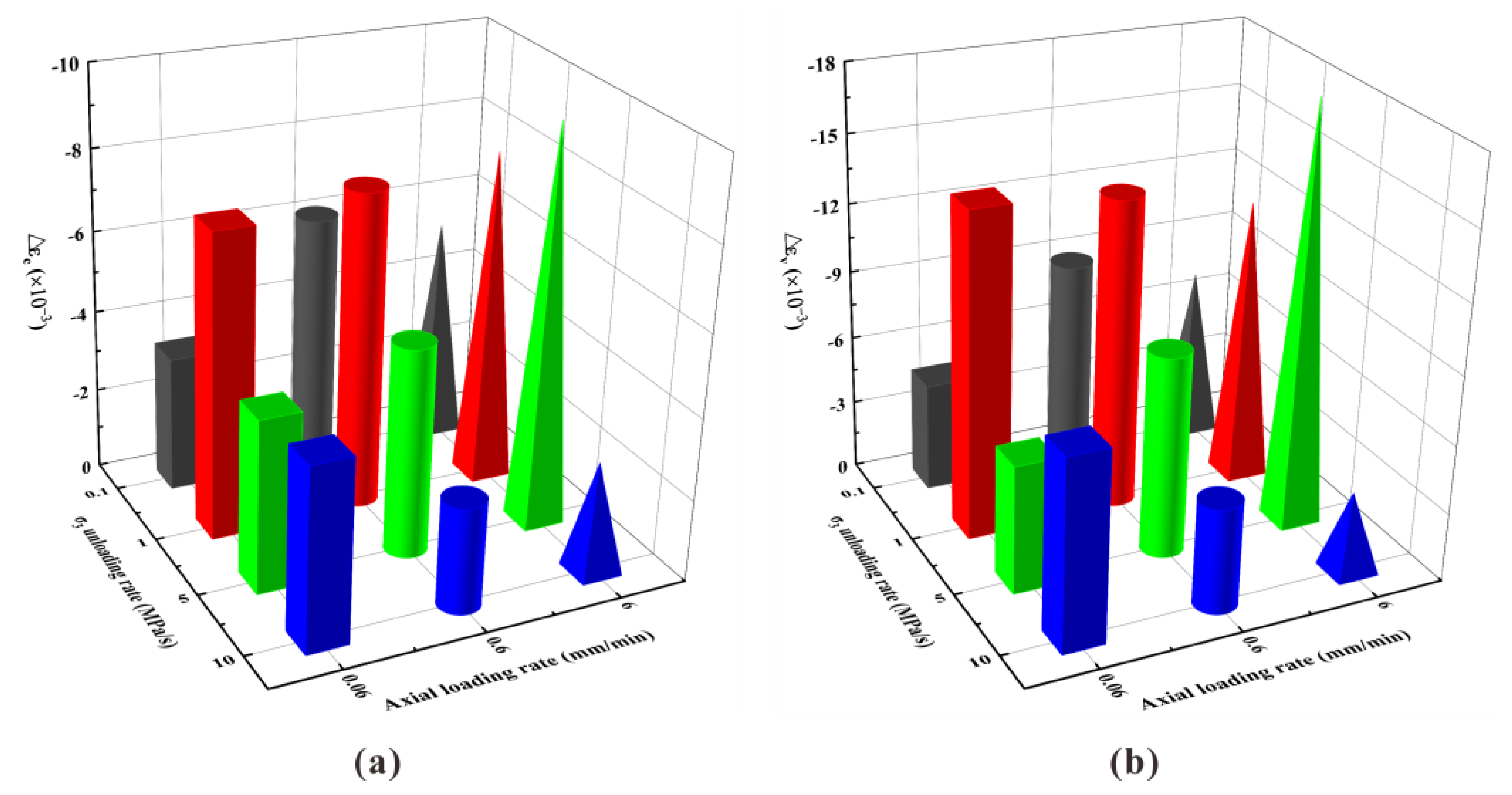
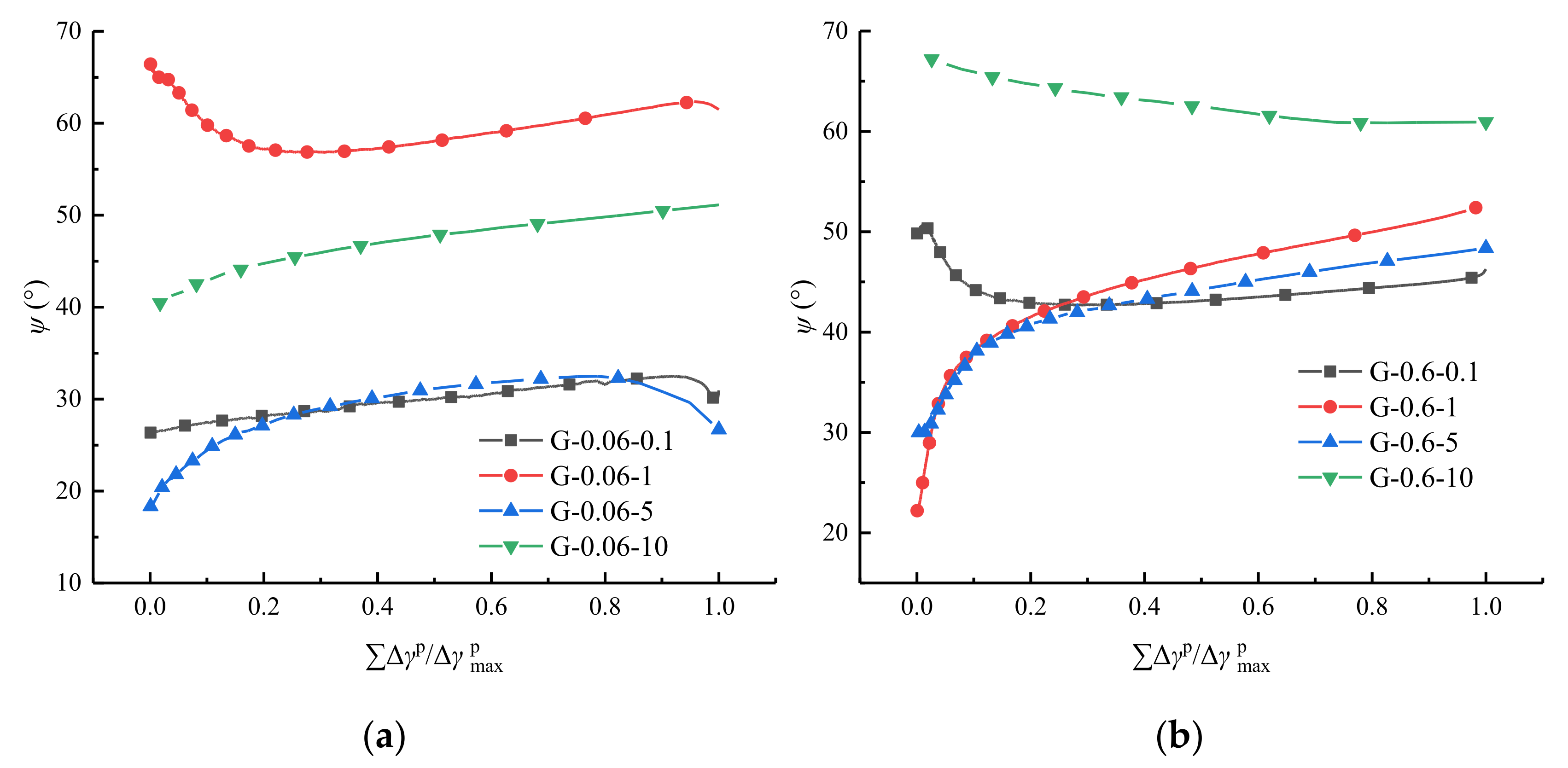

| Test Scheme | Specimen Number | Initial Confining Pressure (MPa) | Axial Loading Rate | Unloading Rate of σ3 |
|---|---|---|---|---|
| Group-I | TC-0 | 0 | 0.12 mm/min | - |
| TC-10 | 10 | - | ||
| TC-30 | 30 | - | ||
| TC-50 | 50 | - | ||
| Group-II | G-0.06-X * | 50 | 0.06 mm/min | 0.1 MPa/s, 1 MPa/s, 5 MPa/s, 10 MPa/s |
| G-0.6-X | 50 | 0.6 mm/min | ||
| G-6-X | 50 | 6 mm/min |
| Test Scheme | Specimen No. | Dry Density (kg/m3) | Saturated Density (kg/m3) | σ30 (MPa) | σ3f (MPa) | σ1f (MPa) | E (GPa) | ν |
|---|---|---|---|---|---|---|---|---|
| Group I | TC-0 | 2637.49 | 2644.55 | 0 | 0 | 104.93 | 43.04 | 0.10 |
| TC-10 | 2631.32 | 2637.24 | 10 | 10 | 202.99 | 42.67 | 0.10 | |
| TC-30 | 2635.72 | 2641.16 | 30 | 30 | 361.84 | 53.96 | 0.11 | |
| TC-50 | 2635.67 | 2639.43 | 50 | 50 | 480.32 | 50.57 | 0.12 | |
| Group II | G-0.06-0.1 | 2635.13 | 2638.36 | 50 | 34.42 | 390.08 | 51.4 | 0.10 |
| G-0.06-1 | 2637.42 | 2641.45 | 50 | 25.53 | 358.82 | 58.04 | 0.28 | |
| G-0.06-5 | 2635.50 | 2641.38 | 50 | 21.06 | 361.00 | 51.71 | 0.11 | |
| G-0.06-10 | 2638.97 | 2643.01 | 50 | 18.77 | 354.14 | 62.64 | 0.18 | |
| G-0.6-0.1 | 2632.97 | 2637.00 | 50 | 44.48 | 470.63 | 58.39 | 0.17 | |
| G-0.6-1 | 2651.75 | 2655.78 | 50 | 28.83 | 398.89 | 59.08 | 0.23 | |
| G-0.6-5 | 2638.89 | 2642.93 | 50 | 21.81 | 378.21 | 59.03 | 0.21 | |
| G-0.6-10 | 2636.85 | 2640.88 | 50 | 23.47 | 384.67 | 56.05 | 0.20 | |
| G-6-0.1 | 2632.28 | 2636.32 | 50 | 49.31 | 508.61 | 60.45 | 0.24 | |
| G-6-1 | 2602.96 | 2606.98 | 50 | 43.97 | 465.05 | 56.32 | 0.18 | |
| G-6-5 | 2620.01 | 2624.07 | 50 | 32.07 | 400.16 | 62.90 | 0.18 | |
| G-6-10 | 2592.90 | 2596.95 | 50 | 27.74 | 412.31 | 53.09 | 0.26 |
| Specimen No. | σ30 (MPa) | Unloading Point | Failure Point | Δεvf (×10−3) | ||||
|---|---|---|---|---|---|---|---|---|
| εa0 (×10−3) | εc0 (×10−3) | εv0 (×10−3) | εaf (×10−3) | εcf (×10−3) | εvf (×10−3) | |||
| TC-0 | 0 | 2.19 | −0.14 | 1.91 | 2.92 | −2.43 | −1.94 | −3.85 |
| TC-10 | 10 | 3.55 | −0.49 | 2.57 | 4.53 | −2.81 | −1.09 | −3.66 |
| TC-30 | 30 | 5.17 | −0.97 | 3.23 | 7.38 | −3.70 | −0.02 | −3.25 |
| TC-50 | 50 | 8.16 | −1.84 | 4.48 | 10.24 | −3.91 | 2.42 | −2.06 |
| G-0.06-0.1 | 50 | 7.14 | −1.22 | 4.70 | 9.06 | −4.55 | −0.05 | −4.75 |
| G-0.06-1 | 6.26 | −2.30 | 1.67 | 6.89 | −9.75 | −12.60 | −14.27 | |
| G-0.06-5 | 7.07 | −1.28 | 4.51 | 9.88 | −5.49 | −1.09 | −5.60 | |
| G-0.06-10 | 6.12 | −1.24 | 3.64 | 6.67 | −5.66 | −4.65 | −8.29 | |
| G-0.6-0.1 | 50 | 6.55 | −2.24 | 2.07 | 9.90 | −8.39 | −6.88 | −8.95 |
| G-0.6-1 | 6.56 | −2.07 | 2.42 | 8.43 | −9.84 | −11.25 | −13.67 | |
| G-0.6-5 | 6.53 | −2.04 | 2.46 | 7.94 | −7.16 | −6.37 | −8.83 | |
| G-0.6-10 | 6.78 | −3.86 | −0.94 | 7.34 | −6.44 | −5.53 | −4.59 | |
| G-6-0.1 | 50 | 6.46 | −1.99 | 2.49 | 9.85 | −7.35 | −4.84 | −7.33 |
| G-6-1 | 6.74 | −1.95 | 2.85 | 10.59 | −10.05 | −9.50 | −12.35 | |
| G-6-5 | 6.19 | −2.25 | 1.68 | 7.20 | −11.92 | −16.63 | −18.31 | |
| G-6-10 | 7.23 | −1.43 | 4.38 | 9.15 | −4.12 | 0.91 | −3.47 | |
| Specimen No. | σ30 (MPa) | Unloading Point | Failure Point | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Ua0 | Uc0 | U0 | Ue0 | Ud0 | Uaf | Ucf | Uf | Uef | Udf | ||
| TC-0 | 0 | - | - | - | - | - | 0.16 | - | - | 0.13 | 0.03 |
| TC-10 | 10 | - | - | - | - | - | 0.91 | 0.03 | 0.94 | 0.55 | 0.39 |
| TC-30 | 30 | - | - | - | - | - | 2.55 | 0.14 | 2.70 | 1.74 | 0.95 |
| TC-50 | 50 | - | - | - | - | - | 4.88 | 0.24 | 5.11 | 3.35 | 1.77 |
| G-0.06-0.1 | 50 | 1.66 | −0.06 | 1.60 | 1.32 | 0.28 | 2.44 | −0.19 | 2.25 | 1.47 | 0.79 |
| G-0.06-1 | 1.43 | −0.11 | 1.32 | 1.02 | 0.29 | 1.68 | −0.34 | 1.34 | 0.73 | 0.60 | |
| G-0.06-5 | 1.63 | −0.06 | 1.56 | 1.30 | 0.26 | 2.69 | −0.17 | 2.51 | 1.56 | 0.96 | |
| G-0.06-10 | 1.46 | −0.06 | 1.39 | 1.11 | 0.28 | 1.67 | −0.16 | 1.50 | 0.97 | 0.53 | |
| G-0.6-0.1 | 50 | 1.53 | −0.11 | 1.42 | 1.08 | 0.34 | 3.02 | −0.40 | 2.62 | 1.59 | 1.53 |
| G-0.6-1 | 1.51 | −0.10 | 1.40 | 1.11 | 0.30 | 2.27 | −0.37 | 1.90 | 1.11 | 1.51 | |
| G-0.6-5 | 1.53 | −0.10 | 1.43 | 1.10 | 0.32 | 2.08 | −0.24 | 1.84 | 1.19 | 1.53 | |
| G-0.6-10 | 1.57 | −0.19 | 1.37 | 0.96 | 0.41 | 1.79 | −0.26 | 1.52 | 1.11 | 1.57 | |
| G-6-0.1 | 50 | 1.51 | −0.10 | 1.41 | −0.34 | 1.74 | 3.07 | −0.36 | 2.71 | −1.34 | 1.51 |
| G-6-1 | 1.57 | −0.10 | 1.47 | 1.16 | 0.31 | 3.31 | −0.46 | 2.85 | 1.58 | 1.57 | |
| G-6-5 | 1.44 | −0.11 | 1.33 | 1.01 | 0.32 | 1.90 | −0.45 | 1.45 | 0.67 | 1.44 | |
| G-6-10 | 1.69 | −0.07 | 1.62 | 1.32 | 0.30 | 2.49 | −0.16 | 2.33 | 1.67 | 1.69 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Z.; Yi, W. Experimental Study on the Mechanical Characteristics of Saturated Granite under Conventional Triaxial Loading and Unloading Tests. Sustainability 2022, 14, 5445. https://doi.org/10.3390/su14095445
Liu Z, Yi W. Experimental Study on the Mechanical Characteristics of Saturated Granite under Conventional Triaxial Loading and Unloading Tests. Sustainability. 2022; 14(9):5445. https://doi.org/10.3390/su14095445
Chicago/Turabian StyleLiu, Zelin, and Wei Yi. 2022. "Experimental Study on the Mechanical Characteristics of Saturated Granite under Conventional Triaxial Loading and Unloading Tests" Sustainability 14, no. 9: 5445. https://doi.org/10.3390/su14095445
APA StyleLiu, Z., & Yi, W. (2022). Experimental Study on the Mechanical Characteristics of Saturated Granite under Conventional Triaxial Loading and Unloading Tests. Sustainability, 14(9), 5445. https://doi.org/10.3390/su14095445






