Application of Sustainable Concrete in the Seismic Evaluation of an Innovative Type of Buckling Restrained Brace
Abstract
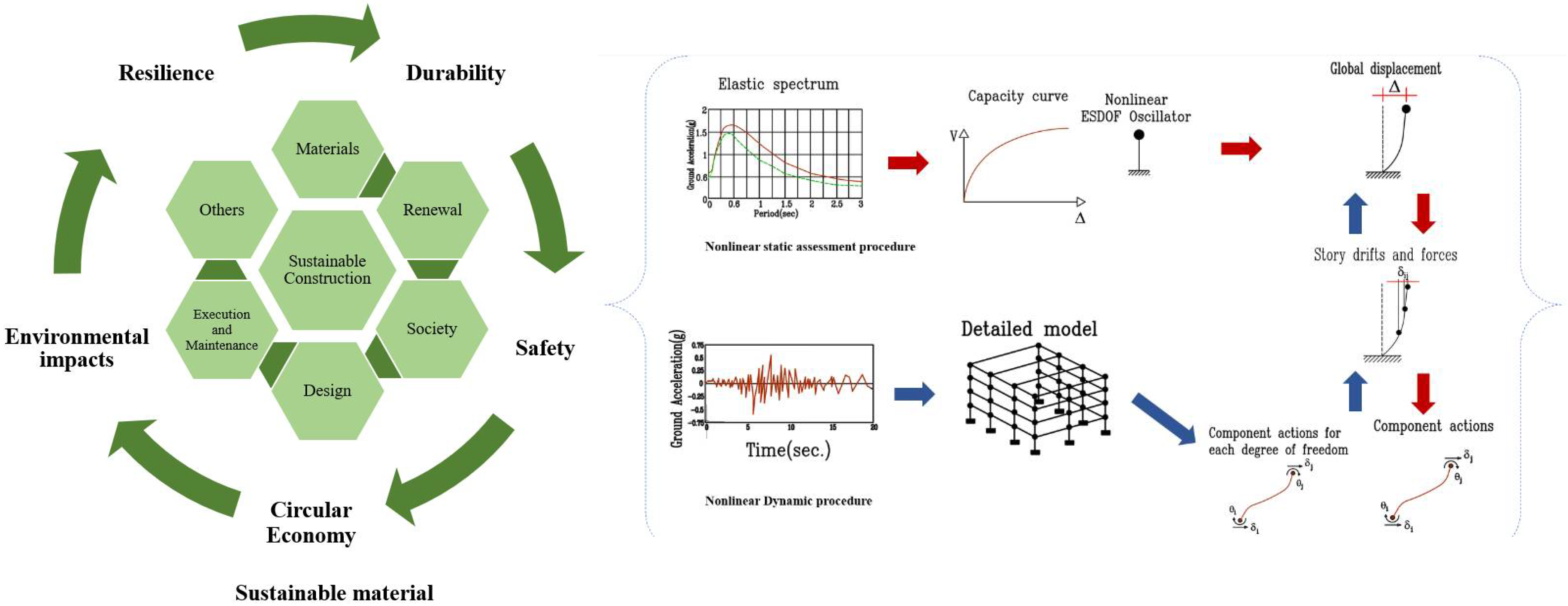
1. Introduction
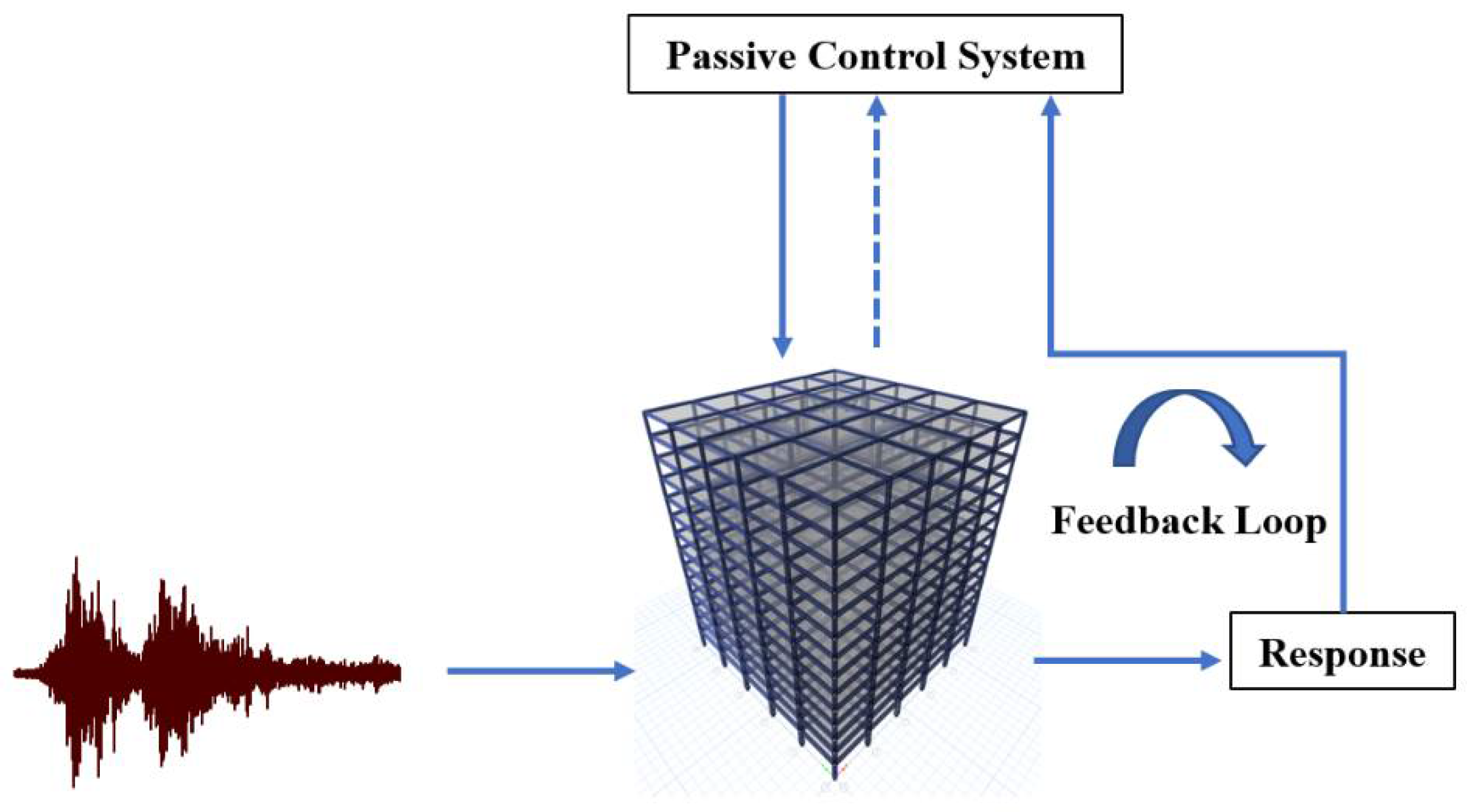
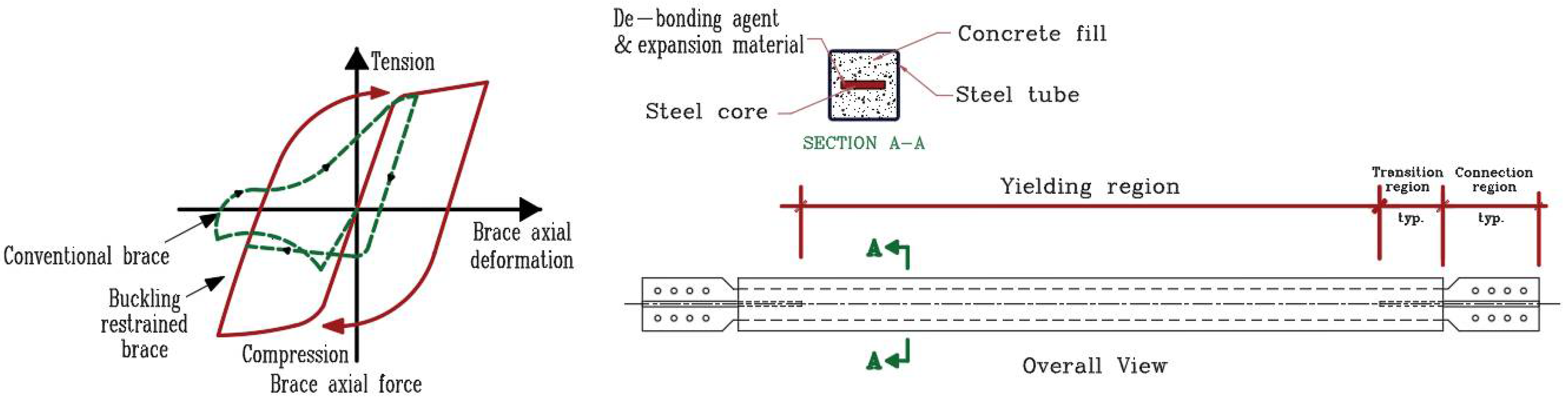
2. Design of Buckling Restrained Braced Frame
3. Response Modification Factor
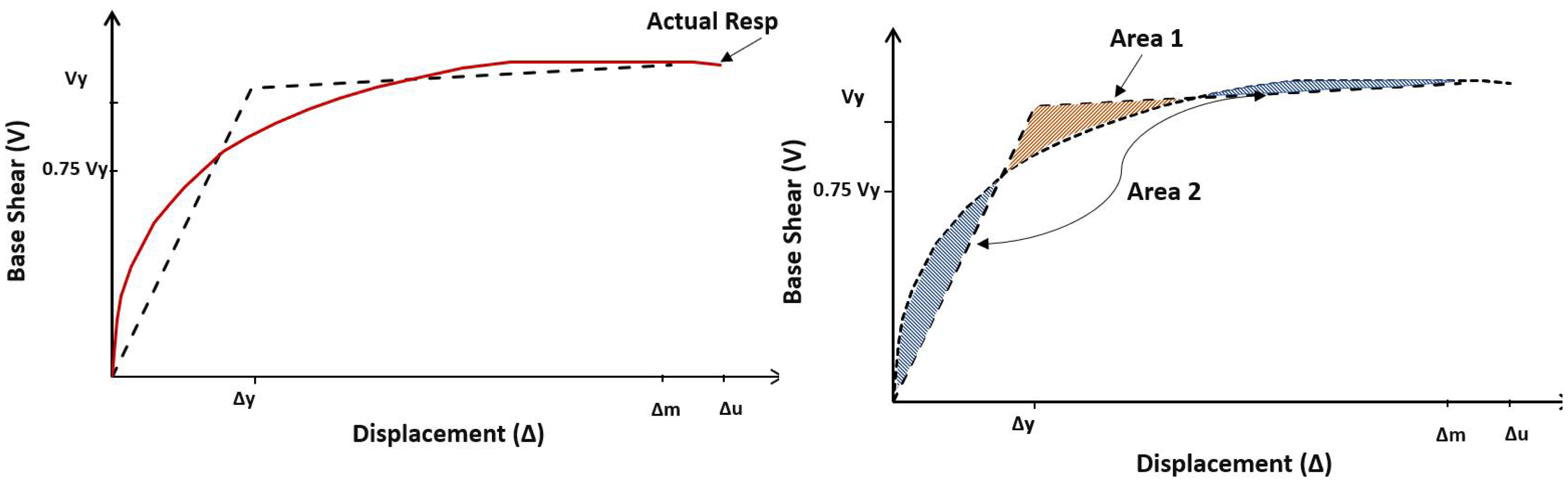
4. Overstrength Factors
5. Ductility Factors
6. Design of Structural Models
7. Modal Analysis
8. Nonlinear Static Analysis
9. Inelastic Response History Analysis
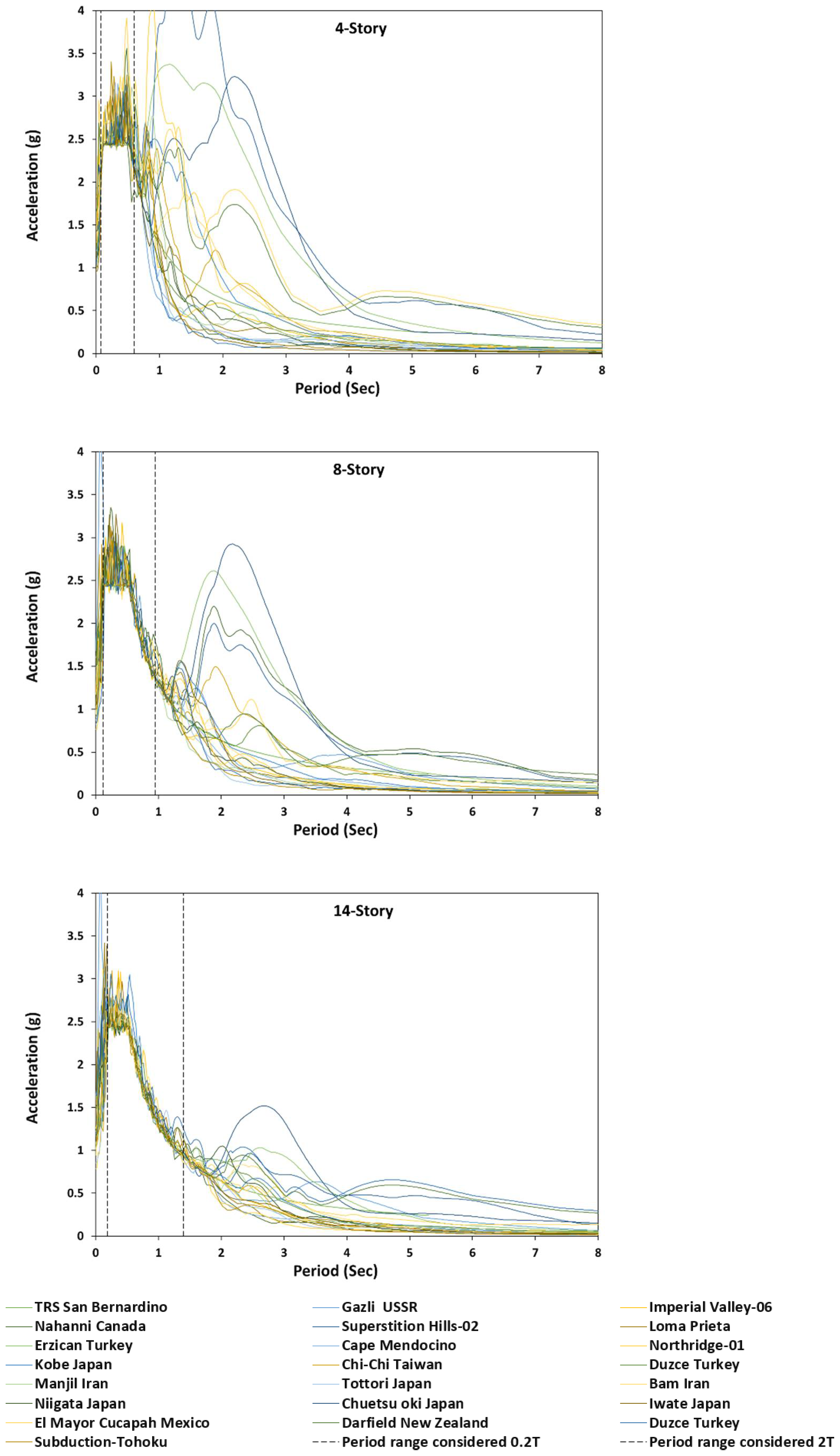
10. Ground Motion Selection
11. Ground Motion Scaling
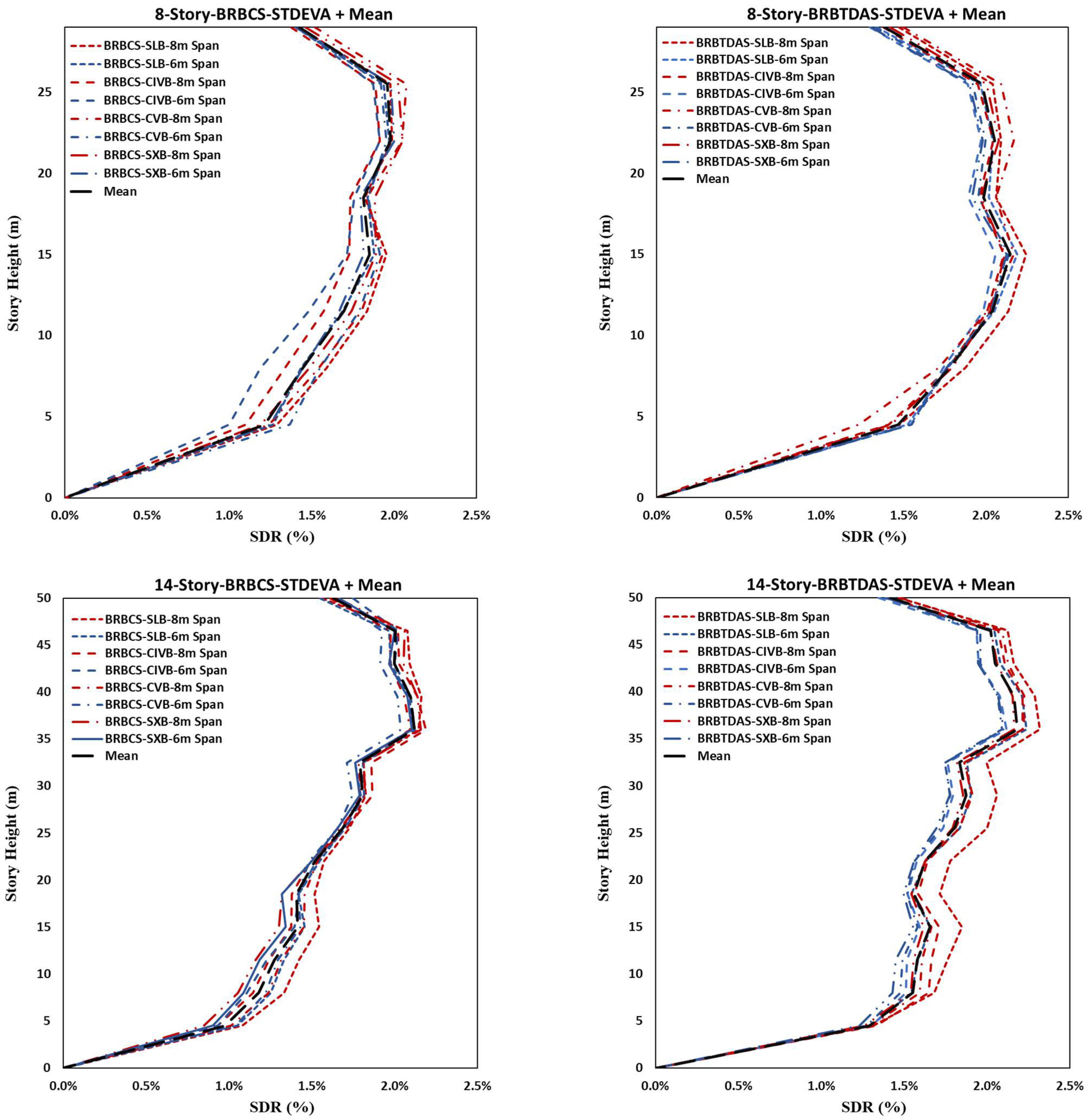
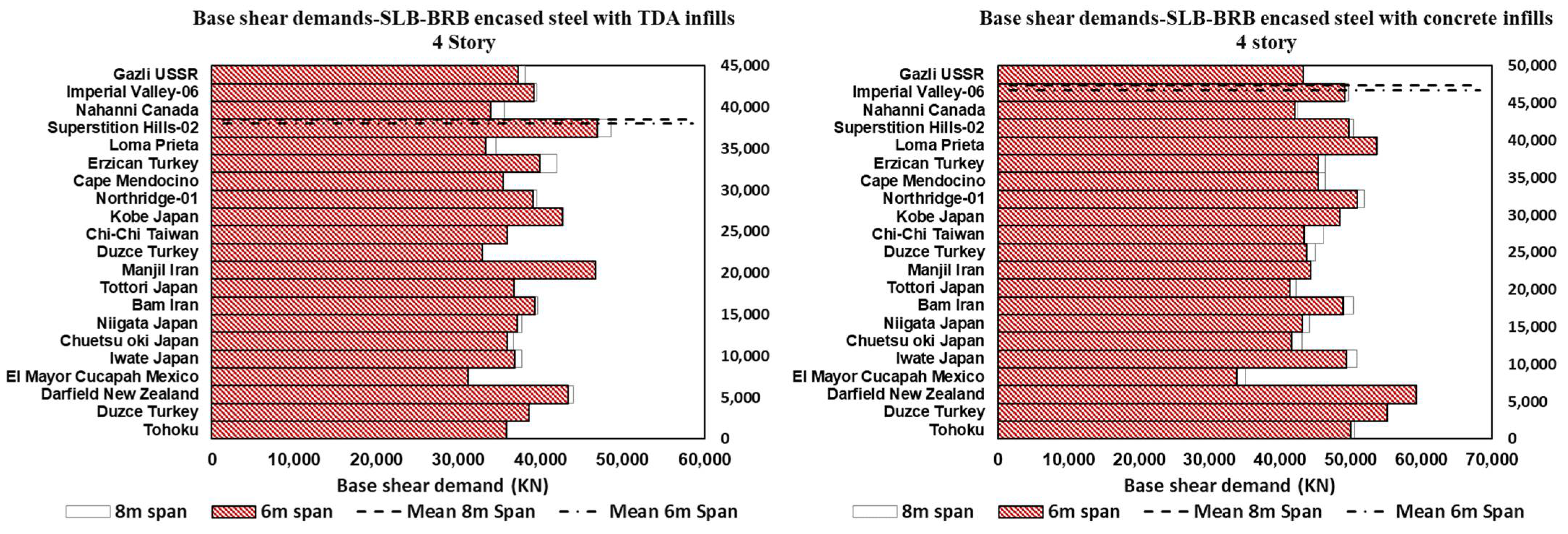
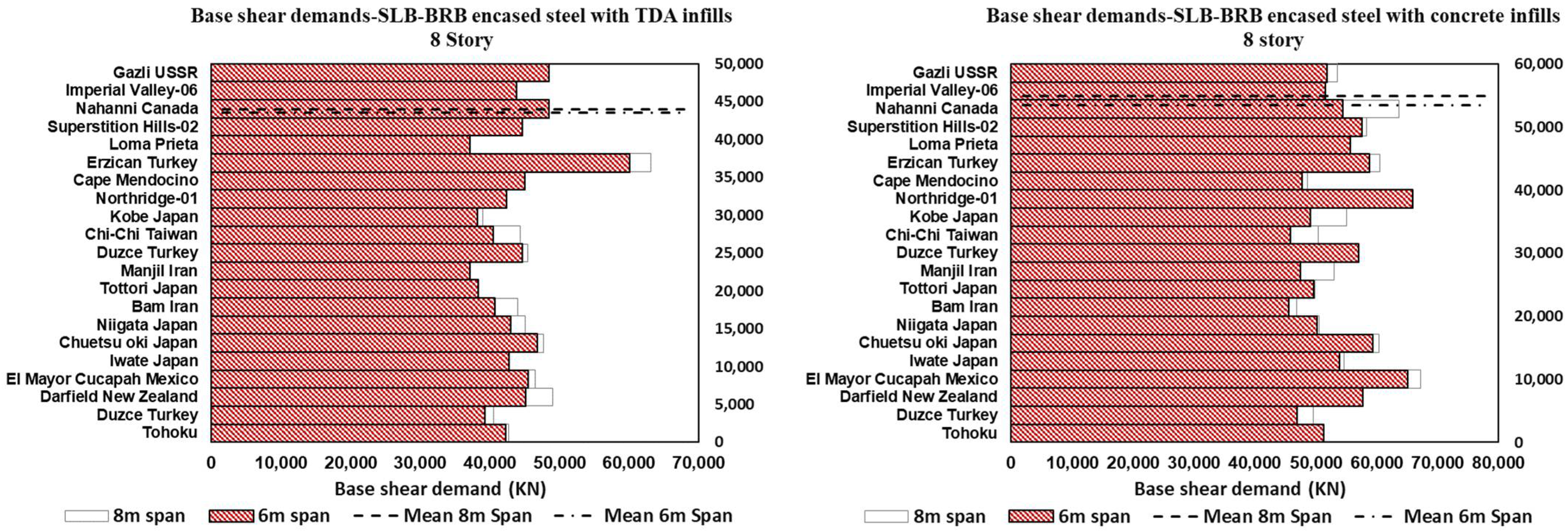
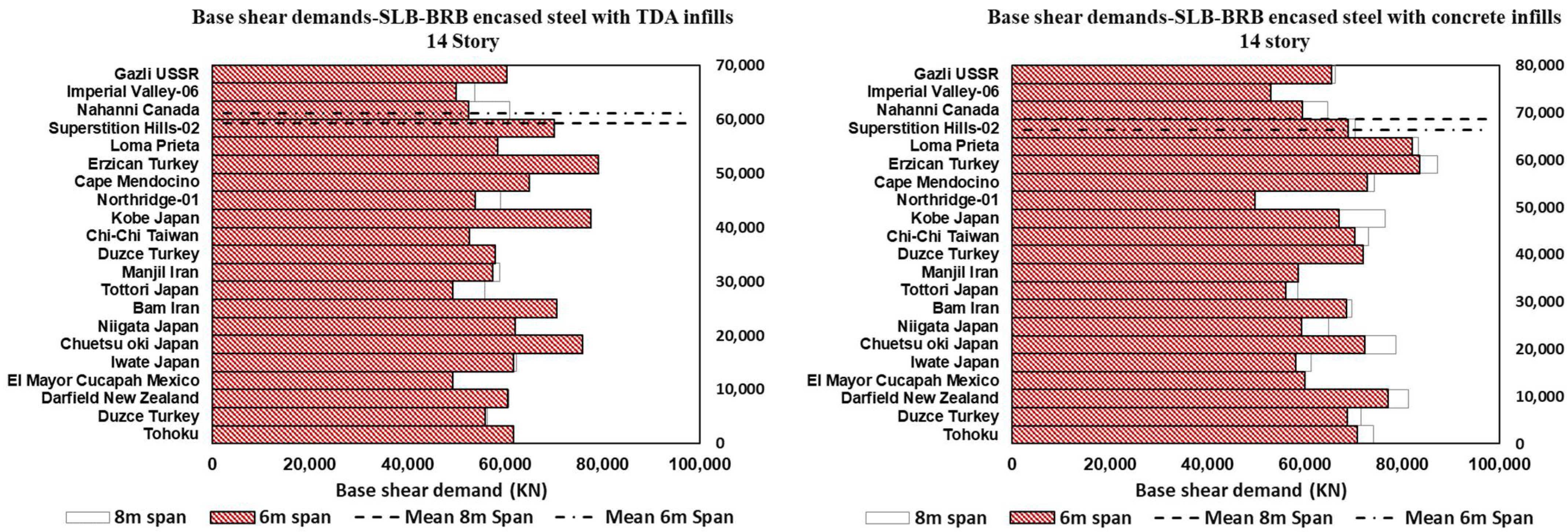
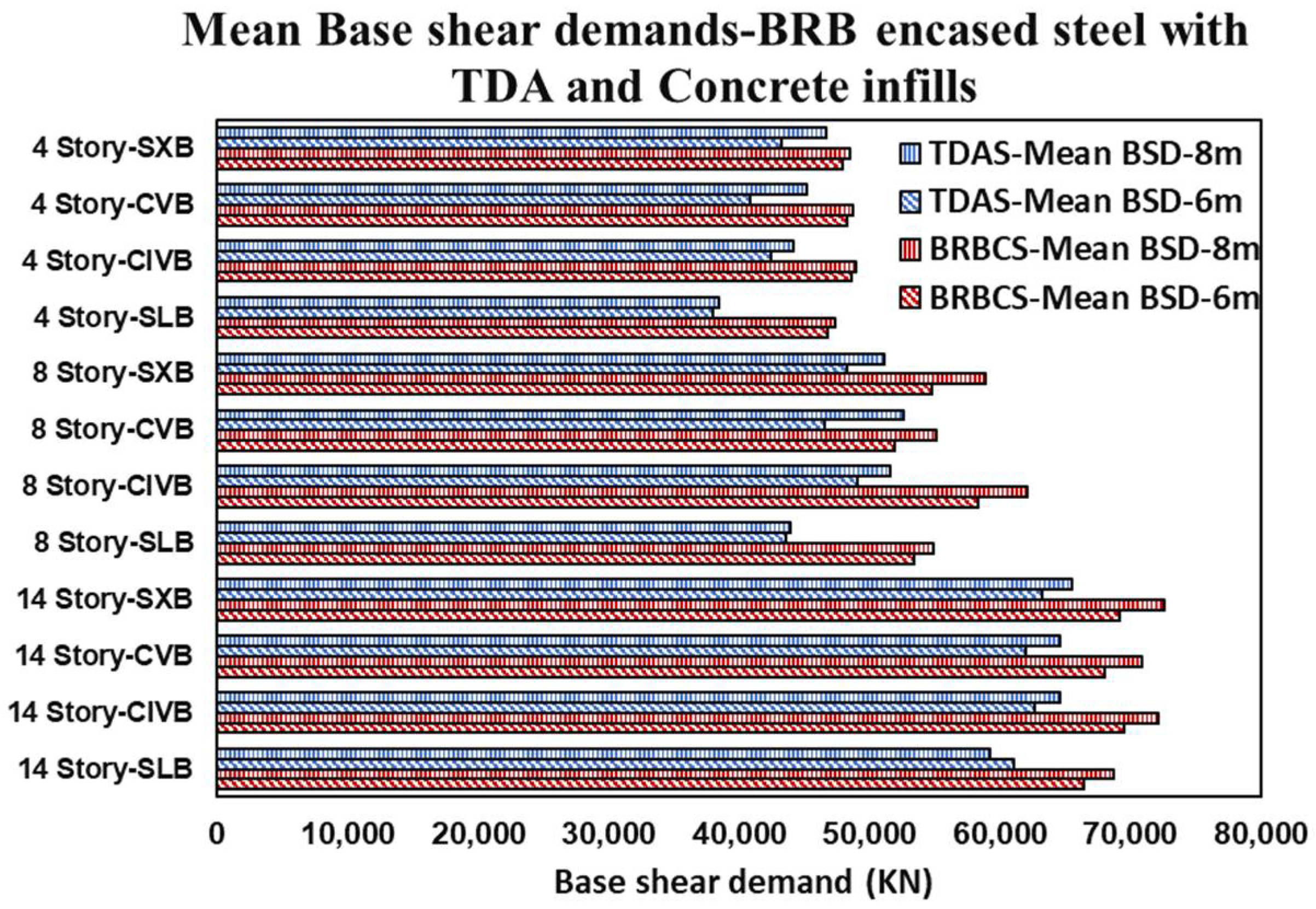
12. Roof, Inter-Story, and Base Shear Demand Results
13. Results and Discussions
14. Conclusions
- The average fundamental periods of BRB-encased steel with concrete with different span lengths and bracing configurations are 0.47(s), 0.86(s), and 1.32(s) for four-, eight-, and fourteen-story SCMFs. These values are increased to 0.55(s), 1.01(s), and 1.47(s) for four-, eight-, and fourteen-story SCMFs of BRB-encased steel with TDA. In general, the fundamental period of the structures, when calculated by an empirical equation, is more conservative than the result when using eigenvalue analysis of the structures for BRB-encased steel with concrete infill. It underestimates the period of the structure in higher buildings equipped with BRB encased steel with TDA. The fundamental period of BRB-encased steel with TDA infill is about 15% higher than BRB-encased steel with concrete infill. Furthermore, while computing the natural period of the structures, the effect of bracing configuration can be ignored;
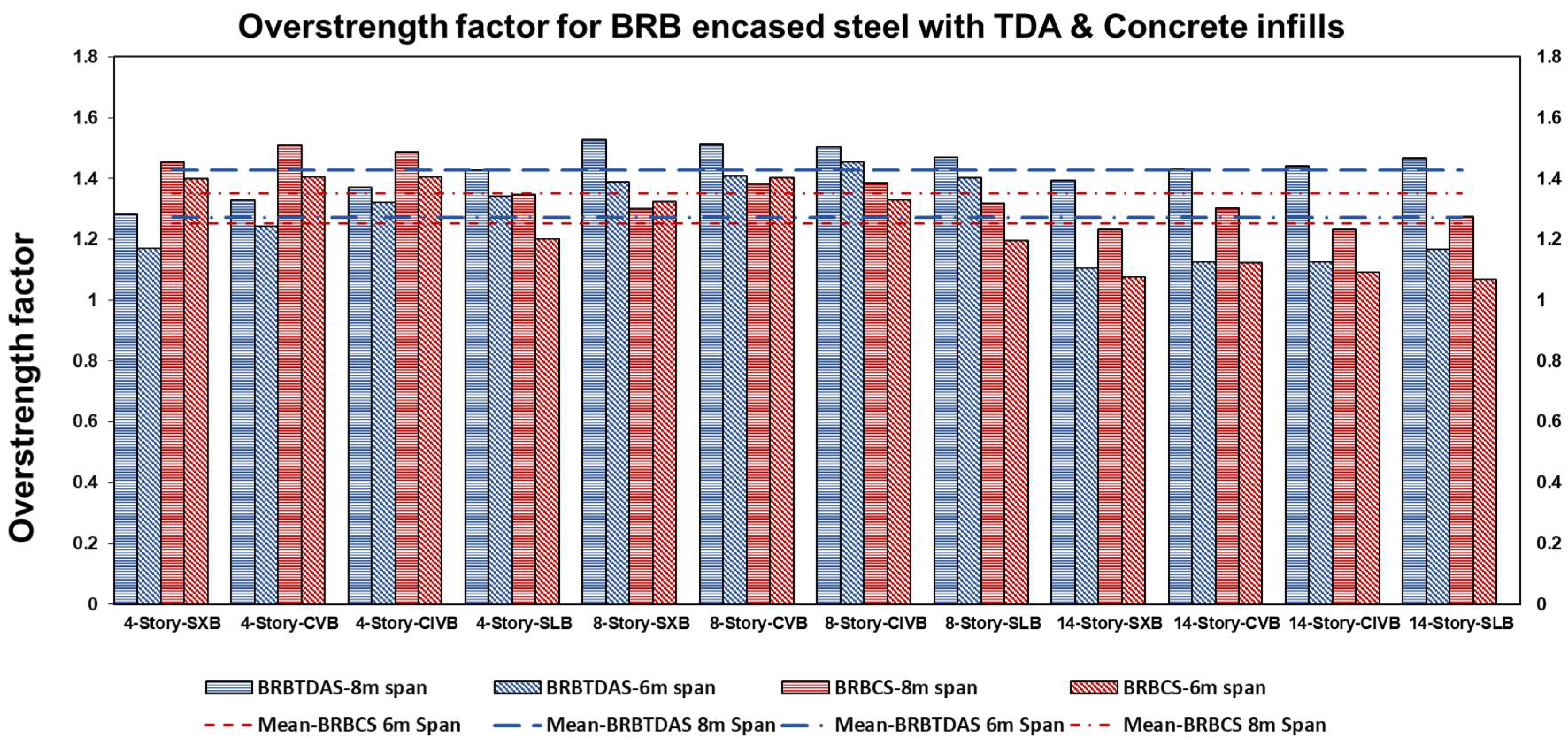
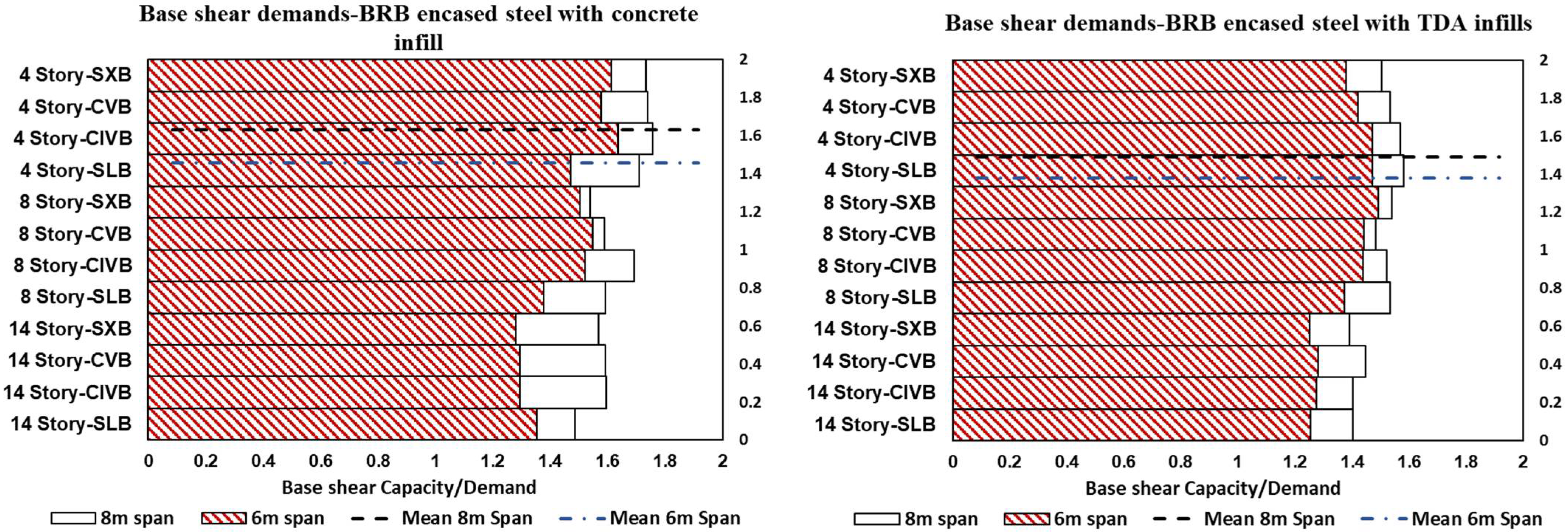
- The overstrength factor ranges from 1.10 to 1.52 and 1.06 to 1.51 for the BRB-encased steel with TDA and concrete infills. The prescribed overstrength factors in ASCE 7-22 and NBCC 2015 [13] are 2.5 and 1.2. ASCE 7-22 [12] gives a conservative value that accounts for several factors, including member size, effects of structural redundancy, and infill walls [44]. Moreover, span length directly affects the overstrength factor, and longer span lengths result in a greater overstrength factor;
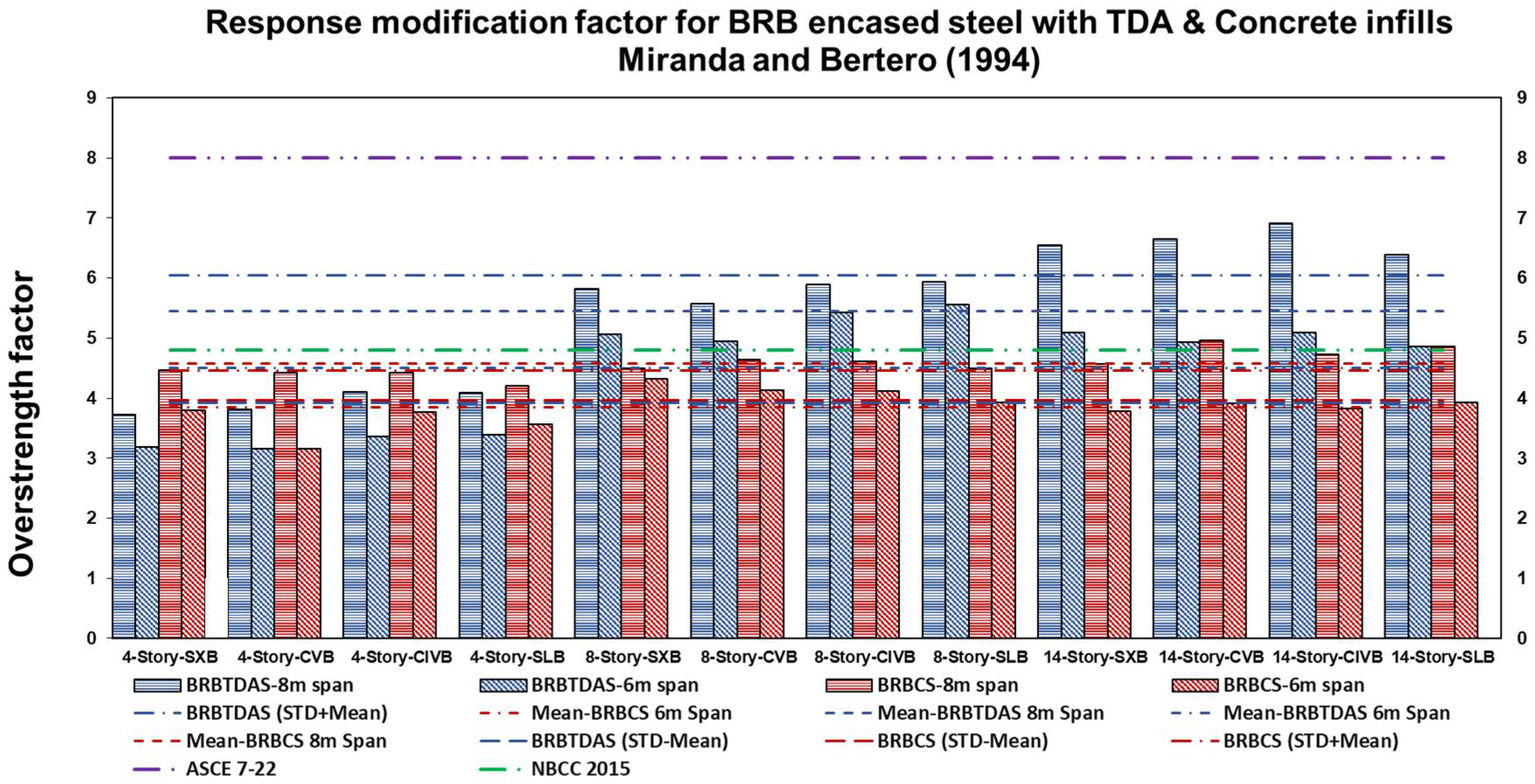
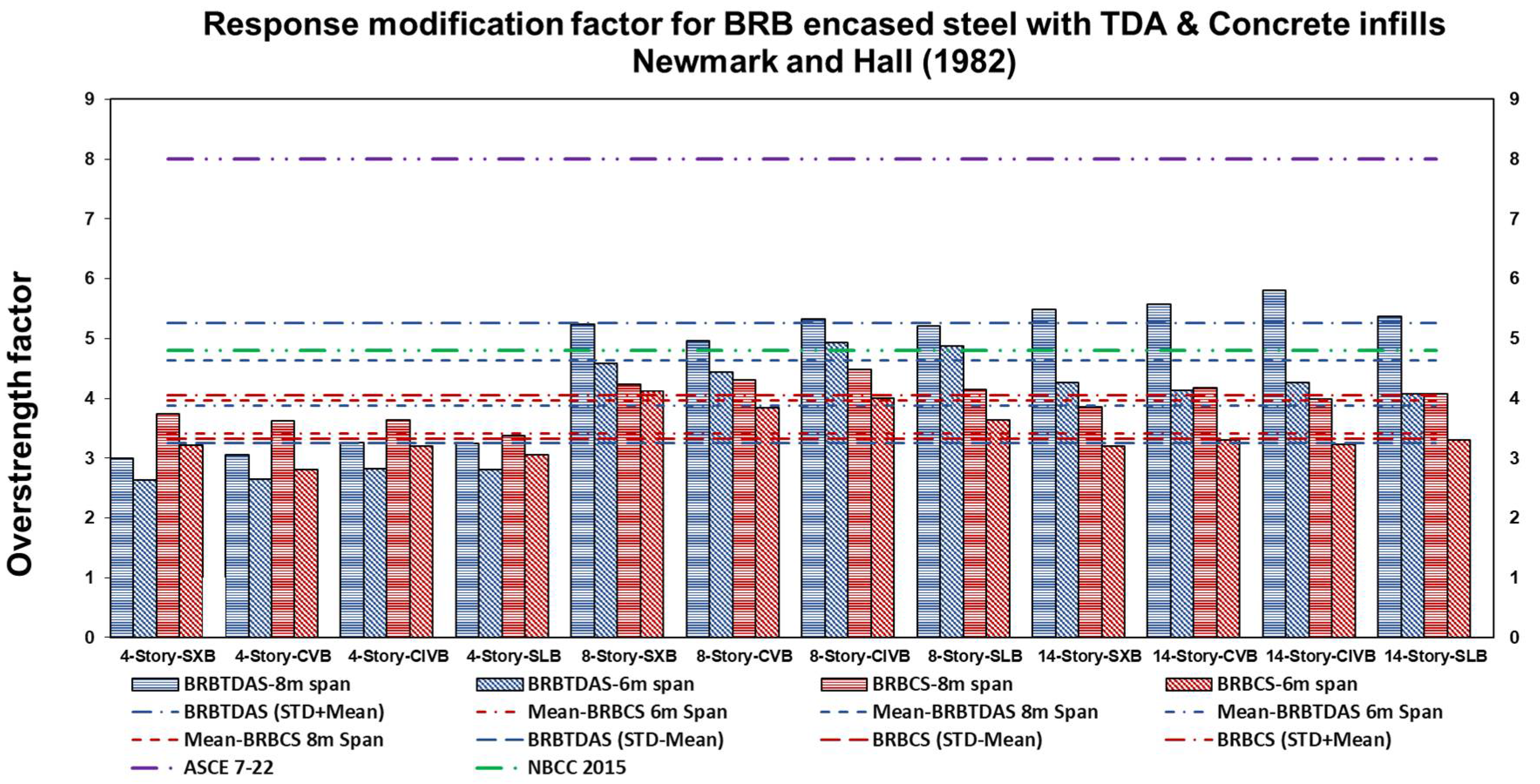
- The ductility factors have been calculated and compared using the Miranda and Bertero (1994) [35] and Newmark and Hall (1982) [36] methods. We found that a longer span length and a higher building height increase the ductility factor. The BRB-encased steel with TDA infill increased the average ductility factors by 5% compared to the BRB-encased steel with concrete infill. The average ductility factor calculated using the Newmark and Hall (1982) [36] method is approximately 15% lower than that derived using the Miranda and Bertero (1994) [35] method. Additionally, because the variance in bracing configuration impact is less than 4%, their effect can be ignored;
- The calculated mean value of RMF based on the Miranda and Bertero (1994) [35] method for BRB encased with TDA infill is 4.97, and it is 4.21 for concrete infill, while the computed mean value of RMF based on the Newmark and Hall (1982) [36] approach is 4.25, and it is 3.68 for BRB encased with TDA and concrete infills. The Miranda and Bertero (1994) [35] method achieves a better result than the Newmark and Hall (1982) [36] method because it considers more parameters, such as soil condition, ductility, and the natural period of the structure. The response modification factor increases as the building height and span length increase. The response modification factor for BRB encased with TDA and concrete infills was in the range of 3.13 to 3.61, with a mean value minus standard deviation of about 4 in both cases. Accordingly, ASCE 7-22 [12] and NBCC 2015 [13] specify response modification values of 8 and 4.8 for BRBFs, which are conservative. As a result, for structures with a height equal to or less than 50 m, a response modification factor of 4 is recommended, which covers about 95% of the cases. It is evident that different bracing configurations range from 5% to 12%, hence their effect should be addressed;
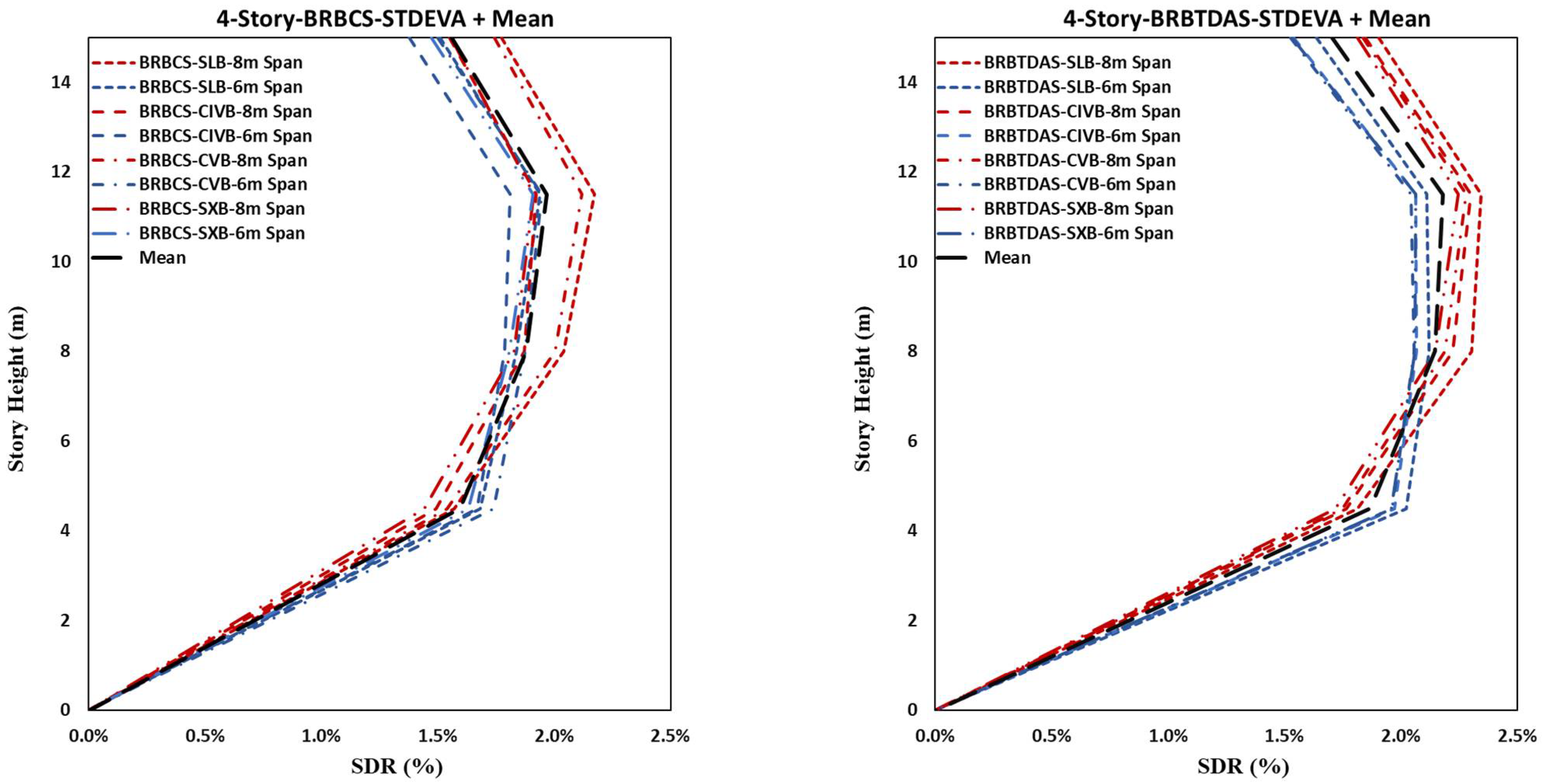
- The maximum drift demand of nonlinear response history analysis increases with height. For four-story BRBFs, the maximum mean value of interstory demand was found at the third level, while it shifted to the fourth and tenth levels for eight- and fourteen-story structures. We found that with greater heights of the structure, the maximum interstory drifts move to higher levels, and longer span lengths increase the story drift ratios. The interstory demands are higher for BRB-encased steel with TDA infill than BRB-encased steel with concrete infill;
- Base shear demands increase with greater span lengths and heights and vary with different framing configurations. Furthermore, compared to concrete infill, the base shear demands for BRB-encased steel with TDA infill are reduced in shorter span lengths, and are greater in longer span lengths.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ko, E.; Field, C. The Unbonded Brace™: From Research to Californian Practice 2003, SEAOC convention. In Proceedings of the 13th World Conference on Earthquake Engineering, Vancouver, BC, Canada, 1–6 August 2004. NO.1321. [Google Scholar]
- Tehrani, F.M.; Nazari, M.; Naghshineh, A. Role of Seismic Isolation and Protection Devices in Enhancing Structural Resilience, EMI ORC MOP Part III Chapter 21. In Objective Resilience: Technology; American Society of Civil Engineers: Reston, VA, USA, 2022. [Google Scholar]
- Soong, T.T.; Dargush, G.F. Passive Energy Dissipation and Active Control 1999. In Boca Raton: Structural Engineering Handbook; CRC Press LLC: Boca Raton, FL, USA; pp. 1–28.
- Symans, M.D.; Constantinou, M.C. Semi-active control systems for seismic protection of structures: A state-of-the-art review. Eng. Struct. 1997, 21, 469–487. [Google Scholar] [CrossRef]
- UNESCO. Sustainable Development. 2022, UNESCO. Available online: https://en.unesco.org/themes/education-sustainable-development/what-is-esd/sd (accessed on 3 December 2022).
- ACI (American Concrete Institute). Report on the Role of Materials in Sustainable Concrete Construction; Reported by ACI Committee 130; ACI: Michigan, MI, USA, 2019. [Google Scholar]
- Tehrani, F.M.; Miller, N.M. Tire-Derived Aggregate Cementitious Materials: A Review of Mechanical Properties. In Cement-Based Materials; Saleh, H.E.-D.M., Rahman, R.O.A., Eds.; IntechOpen: London, UK, 2018. [Google Scholar] [CrossRef]
- ASTM D6270 (American Society for Testing and Materials). Standard Practice for Use of Scrap Tires in Civil Engineering Applications. 2008. Available online: https://www.astm.org/d6270-08.html (accessed on 3 December 2022).
- Miller, N.M.; Tehrani, F.M. Mechanical properties of rubberized lightweight aggregate concrete. Constr. Build. Mater. 2017, 147, 264–271. [Google Scholar] [CrossRef]
- ASTM C469 (American Society for Testing and Materials). Standard Test Method for Static Modulus of Elasticity and Poisson’s Ratio of Concrete in Compression. 2021. Available online: https://www.astm.org/c0469_c0469m-14e01.html (accessed on 3 December 2022).
- Asgarian, B.; Shokrgozar, H.R. BRBF response modification factor 2009. J. Constr. Steel Res. 2009, 65, 290–298. [Google Scholar] [CrossRef]
- ASCE (American Society of Civil Engineers). Minimum Design Loads and Associated Criteria for Buildings and Other Structures. 2022. Available online: https://ascelibrary.org/doi/abs/10.1061/9780784414248 (accessed on 3 December 2022).
- NBCC (National Research Council of Canada). National Building Code of Canada; NBCC: Ottawa, ON, Canada, 2015. [Google Scholar]
- Moni, M.; Moradi, S.; Alam, M.S. Response modification factors for steel buckling restrained braced frames designed as per the 2010 National Building Code of Canada. Can. J. Civ. Eng. 2016, 43, 702–715. [Google Scholar] [CrossRef]
- Mahmoudi, M.; Zaree, M. Determination the Response Modification Factors of Buckling Restrained Braced Frames. Procedia Eng. 2013, 54, 222–231. [Google Scholar] [CrossRef]
- Hosseinzadeh, S.H.; Mohebi, B. Seismic evaluation of all-steel buckling restrained braces using finite element analysis. J. Constr. Steel Res. 2016, 119, 76–84. [Google Scholar] [CrossRef]
- Bai, J.; Ou, J. Earthquake-resistant design of buckling-restrained braced RC moment frames using performance-based plastic design method. Eng. Struct. 2016, 107, 66–79. [Google Scholar] [CrossRef]
- Della Corte, G.; D’Aniello, M.; Landolfo, R. Field Testing of All-Steel Buckling-Restrained Braces Ap-plied to a Damaged Reinforced Concrete Building. J. Struct. Eng. 2015, 141, D4014004. [Google Scholar] [CrossRef]
- Nguyen, A.H.; Chintanapakdee, C.; Hayashikawa, T. Assessment of current nonlinear static procedures for seismic evaluation of BRBF buildings. J. Constr. Steel Res. 2010, 66, 1118–1127. [Google Scholar] [CrossRef]
- Balling, R.J.; Balling, L.J.; Richards, P.W. Design of Buckling-Restrained Braced Frames Using Nonlinear Time History Analysis and Optimization. J. Struct. Eng. 2009, 135, 461–468. [Google Scholar] [CrossRef]
- Kumar, R.G.; Kumar, S.R.; Kalyanaraman, V. Behaviour of frames with non-Buckling bracings under earthquake loading. J. Constr. Steel Res. 2005, 63, 254–262. [Google Scholar] [CrossRef]
- Kim, T.W.; Foutch, D.A.; LaFave, J.M.; Wilcoski, J. Performance Assessment of Reinforced Concrete Structural Walls for Seismic Loads; University of Illinois Engineering Experiment Station 2004, College of Engineering; University of Illinois at Urbana-Champaign: Urbana, IL, USA, 2004. [Google Scholar]
- Sabelli, R.; Mahin, S.; Chang, C. Seismic demands on steel braced frame buildings with buckling restrained braces. Eng. Struct. 2003, 25, 655–666. [Google Scholar] [CrossRef]
- Tehrani, F.M.; Masswadi, N.A.; Miller, N.M.; Sadrinezhad, A. An Experimental Investigation of Dynamic Properties of Fiber-Reinforced Tire-Derived Lightweight-Aggregate Concrete. Eur. J. Eng. Technol. Res. 2020, 5, 702–707. [Google Scholar] [CrossRef]
- RILEM. GLOBE—Global Consensus on Sustainability in the Built Environment. 2022. Available online: https://www.rilem.net/globe (accessed on 3 December 2022).
- FEMA 356; Prestandard and Commentary for the Seismic Rehabilitation of Buildings. Federal Emergency Management Agency: Washington, DC, USA, 2000.
- FEMA-440; Improvement of Nonlinear Static Seismic Analysis Procedures. Federal Emergency Management Agency: Washington, DC, USA, 2005.
- Alam, M.S.; Moni, M.; Tesfamariam, S. Seismic overstrength and ductility of concrete buildings reinforced with superelastic shape memory alloy rebar. Eng. Struct. 2012, 34, 8–20. [Google Scholar] [CrossRef]
- Kersting, R.A.; Fahnestock, L.A.; Lopez, W.A. Seismic Design of Steel Buckling-Restrained Braced Frames: A Guide for Practicing Engineers; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2015; NIST GCR 15-917-34.
- AISC (American Institute of Steel Construction). Seismic Provisions for Structural Steel Buildings; American Institute of Steel Construction: Chicago, IL, USA, 2016. [Google Scholar]
- ATC (Applied Technology Council). Tentative Provisions for the Development of Seismic Regulations for Buildings; National Bureau of Standards: Gaithersburg, MD, USA, 1982. [Google Scholar]
- ATC (Applied Technology Council). Structural Response Modification Factors; ATC: Redwood City, CA, USA, 1995. [Google Scholar]
- Paulay, T.; Priestley MJ, N. Seismic Design of Reinforced Concrete and Masonry Buildings; John Wiley and Sons, Inc.: Hoboken, NJ, USA, 1992. [Google Scholar]
- Krawinkler, H.; Seneviratna, G.D.P.K. Pros and cons of a pushover analysis of seismic performance evaluation. Eng. Struct. 1998, 20, 452–464. [Google Scholar] [CrossRef]
- Miranda, E.; Bertero, V.V. Evaluation of Strength Reduction Factors for Earthquake Resistant Design. Earthq. Spectra 1994, 10, 357–379. [Google Scholar] [CrossRef]
- Newmark, N.M.; Hall, W.J. Earthquake Spectra and Design; EERI Monograph, Earthquake Engineering Research Institute: Berkeley, CA, USA, 1982. [Google Scholar]
- ACI (American Concrete Institute). Building Code Requirements for Structural Concrete and Commentary; ACI Committee 318; ACI: Michigan, MI, USA, 2019. [Google Scholar]
- Lawson, R.S.; Vance, V.; Krawinkler, H. Nonlinear static pushover analysis—why, when and how? In Proceedings of the Fifth US National Conference on Earthquake Engineering, Chicago, IL, USA, 10–14 July 1994; Volume 1, pp. 283–292. [Google Scholar]
- Fajfar, P.; Fischinger, M. N2 a method for non-linear seismic analysis of regular structures. In Proceedings of the Ninth World Conference in Earthquake Engineering, Tokyo-Kyoto, Japan, 2–9 August 1988; Volume 5, pp. 111–116. [Google Scholar]
- Sajidi, M.; Sozen, M.A. Simple nonlinear seismic analysis of R/C structures. J. Struct. Div. ASCE 1981, 107, 937–951. [Google Scholar]
- Miranda, E. Evaluation of Seismic Performance of a Ten Story RC Building during the Whittier Narrows Earthquake. Ph.D. Dissertation, Department of Civil Engineering, University of California, Berkeley, CA, USA, 1991. [Google Scholar]
- SEAOC (Structural Engineers Association of California). Vision 2000, A Framework for Performance-Based Engineering; Structural Engineering Association of California: Sacramento, CA, USA, 1995. [Google Scholar]
- Mwafy, A.M. Seismic Performance of Code-Designed RC Buildings. Ph.D. Thesis, Department of Civil and Environmental Engineering, Imperial College of Science, Technology and Medicine, London, UK, 2001. [Google Scholar]
- Elnashai, A.S.; Di Sarno, L. Fundamentals of Earthquake Engineering: From Source to Fragility; John Wiley & Sons: Hoboken, NJ, USA, 2015. [Google Scholar]
- ASCE (American Society of Civil Engineers). Seismic Evaluation and Retrofit of Existing Buildings; American Society of Civil Engineers: Reston, VA, USA, 2017. [Google Scholar]
- Chopra, A.K. Dynamics of Structures: Theory and Applications to Earthquake Engineering, 4th ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2012. [Google Scholar]
- Kramer, S.L. Geotechnical earthquake engineering. In Prentice-Hall International Series in Civil Engineering and Engineering Mechanics; Prentice Hall: Upper Saddle River, NJ, USA, 1996. [Google Scholar]
- Gavin, H.P.; Dickinson, B.W. Generation of Uniform-Hazard Earthquake Ground Motions. J. Struct. Eng. 2011, 137, 423–432. [Google Scholar] [CrossRef]
- Cordova, P.P.; Deierlein, G.G.; Mehanny, S.S.F.; Cornell, C.A. Development of a two-parameter seismic intensity measure and probabilistic assessment procedure. In Proceedings of the 2nd U.S.-Japan Workshop on Performance-Based Seismic Design Methodology for Reinforced Concrete Building Structures, Sapporo, Japan, 11–13 September 2000; Pacific Earthquake Engineering Research Center, University of California: Berkeley, CA, USA, 2000; pp. 187–206. [Google Scholar]
- Baker, J.W. Correlation of ground motion intensity parameters used for predicting structural and geotechnical response. In Tenth International Conference on Application of Statistics and Probability in Civil Engineering; Taylor & Francis Group: London, UK, 2007; ISBN 978-0-415-45134-5. [Google Scholar]
- Stewart, J.P.; Douglas, J.; Javanbarg, M.; Bozorgnia, Y.; Abrahamson, N.A.; Boore, D.M.; Campbell, K.W.; Delavaud, E.; Erdik, M.; Stafford, P.J. Selection of ground motion prediction equations for the global earthquake model. Earthq. Spectra 2015, 31, 19–45. [Google Scholar] [CrossRef]
- TBI (Tall Buildings Initiative). Guidelines for Performance-Based Seismic Design of Tall Buildings; Pacific Earthquake Engineering Research Center, College of Engineering, University of California: Berkeley, CA, USA, 2017. [Google Scholar]
- PEER Ground Motion Database, PEER Center. Available online: https://ngawest2.berkeley.edu (accessed on 3 December 2022).
- SeismoSoft, Earthquake Software for Response Spectrum Matching; SeismoSoft: Pavia, Italy, 2018.
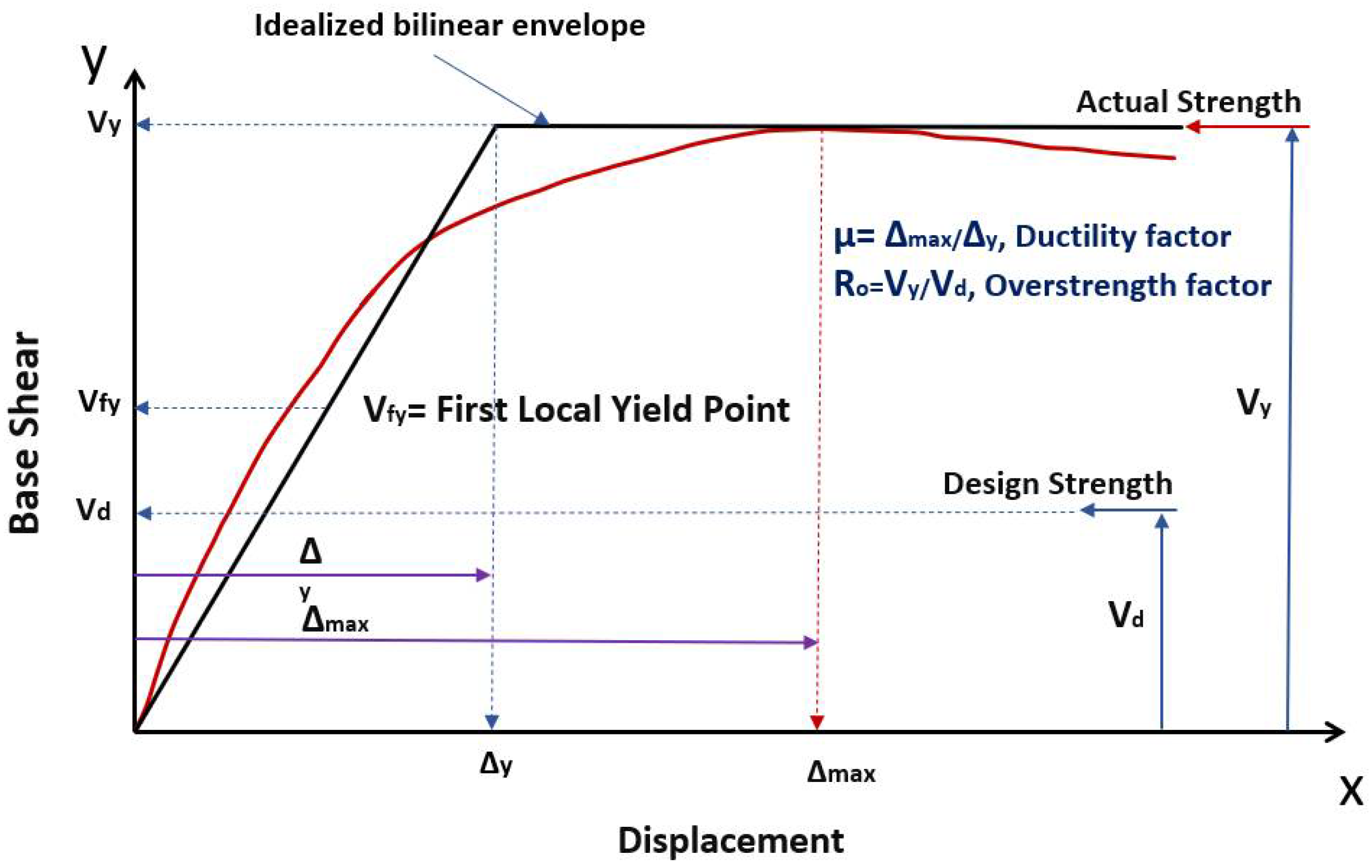
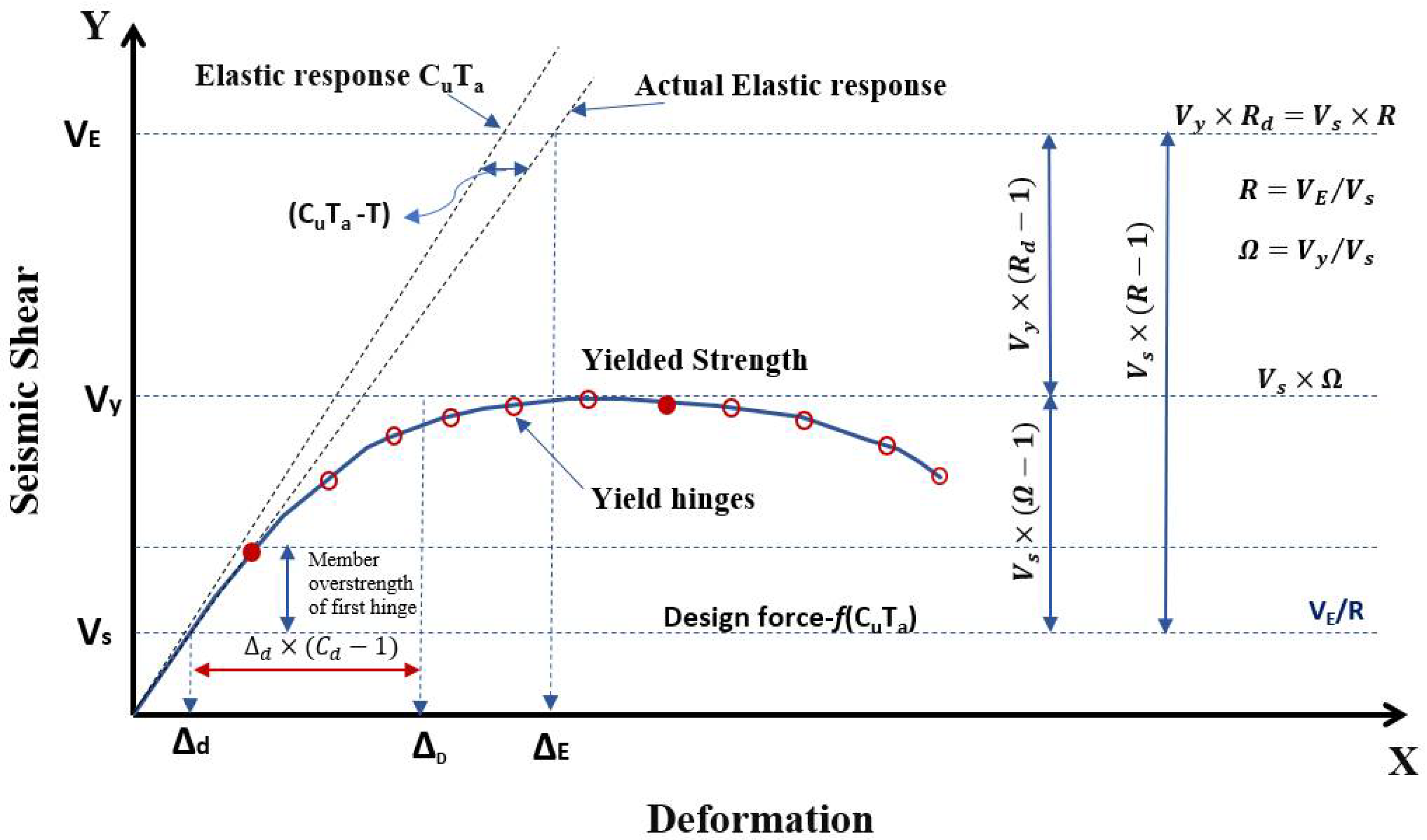
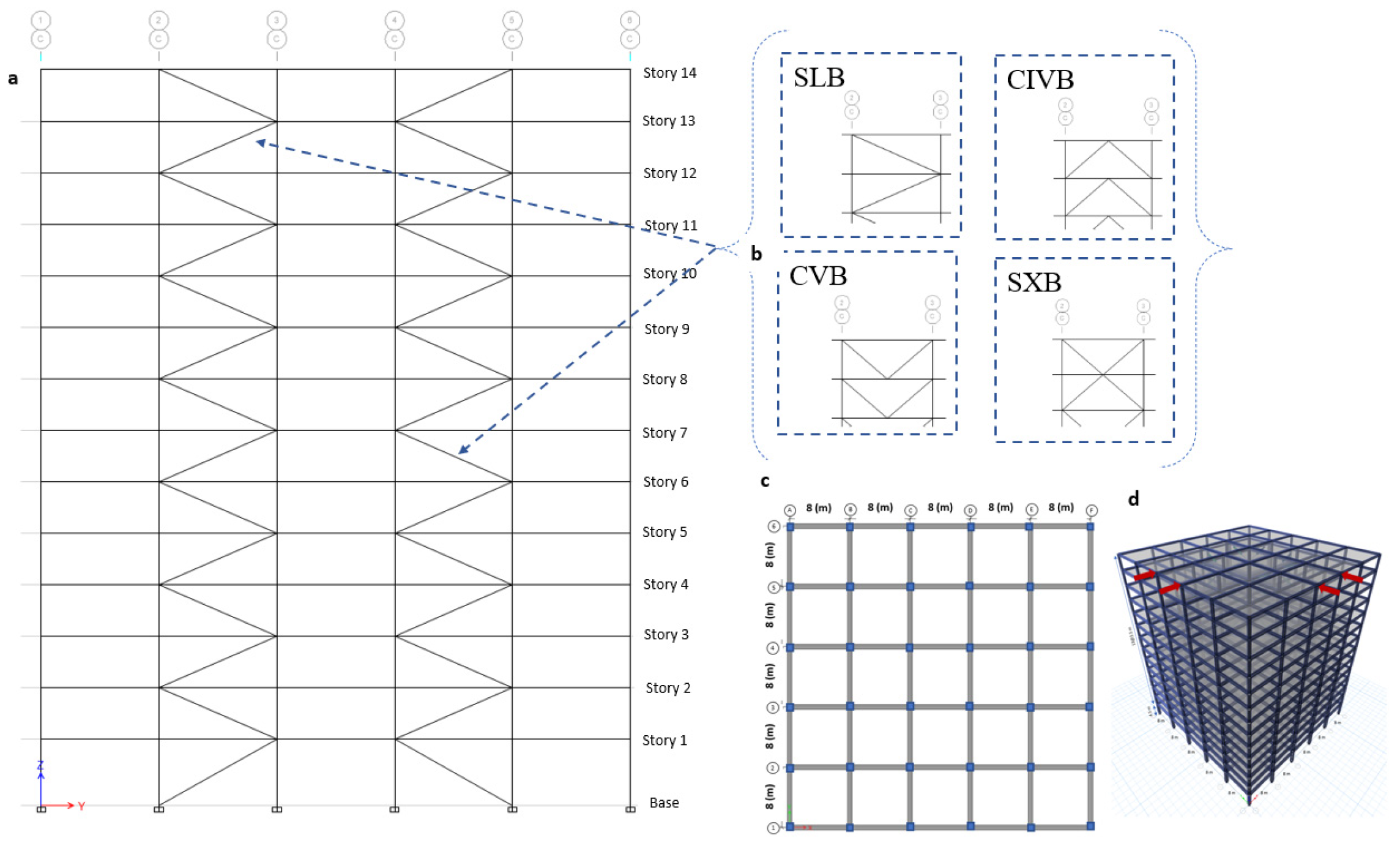





| Period of Structure Ta (Sec) | Ductility Reduction Factor Rµ |
|---|---|
| Ta < 0.1 | |
| 0.12 < Ta < 0.5 | |
| Ta > 1 |
| Span Length | 6 | 8 | Ta | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Bracing | SLB | CIVB | CVB | SXB | SLB | CIVB | CVB | SXB | (S) |
| Story | |||||||||
| 4 | 0.48 | 0.46 | 0.48 | 0.46 | 0.49 | 0.46 | 0.49 | 0.47 | 0.55 |
| 8 | 0.91 | 0.79 | 0.89 | 0.84 | 0.92 | 0.80 | 0.90 | 0.87 | 0.92 |
| 14 | 1.36 | 1.30 | 1.32 | 1.29 | 1.37 | 1.31 | 1.33 | 1.30 | 1.37 |
| Span Length | 6 | 8 | Ta | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Bracing | SLB | CIVB | CVB | SXB | SLB | CIVB | CVB | SXB | (S) |
| Story | |||||||||
| 4 | 0.59 | 0.54 | 0.54 | 0.52 | 0.59 | 0.55 | 0.56 | 0.56 | 0.55 |
| 8 | 1.08 | 0.98 | 1.02 | 0.99 | 1.08 | 0.98 | 1.02 | 0.99 | 0.92 |
| 14 | 1.54 | 1.46 | 1.46 | 1.43 | 1.55 | 1.47 | 1.47 | 1.44 | 1.37 |
| Story | Bracing | Span Length | Yield Strength Vy (kN) | Design Strength Vd (kN) | Overstrength Factor Ro | Maximum Displacement Δmax (mm) | Yield Displacement Δy (mm) | Ductility µ |
|---|---|---|---|---|---|---|---|---|
| 4 | SLB | 6 | 7113 | 5918 | 1.20 | 72 | 21 | 3.44 |
| 8 | 8278 | 6149 | 1.34 | 80 | 22 | 3.63 | ||
| CIVB | 6 | 8314 | 5911 | 1.41 | 65 | 21 | 3.09 | |
| 8 | 9130 | 6139 | 1.48 | 6 | 20 | 3.48 | ||
| CVB | 6 | 8315 | 5914 | 1.41 | 60 | 24 | 2.50 | |
| 8 | 9262 | 6139 | 1.51 | 74 | 22 | 3.37 | ||
| SXB | 6 | 8267 | 5909 | 1.39 | 69 | 22 | 3.15 | |
| 8 | 8926 | 6136 | 1.45 | 72 | 20 | 3.61 | ||
| 8 | SLB | 6 | 8464 | 7085 | 1.9 | 149 | 49 | 3.04 |
| 8 | 9551 | 7246 | 1.32 | 176 | 56 | 3.14 | ||
| CIVB | 6 | 10,468 | 7877 | 1.33 | 138 | 46 | 3.01 | |
| 8 | 11,403 | 8240 | 1.38 | 149 | 46 | 3.24 | ||
| CVB | 6 | 11,403 | 8240 | 1.38 | 156 | 57 | 2.73 | |
| 8 | 10,468 | 7877 | 1.33 | 165 | 53 | 3.11 | ||
| SXB | 6 | 9498 | 7179 | 1.33 | 157 | 50 | 3.11 | |
| 8 | 10,033 | 7710 | 1.31 | 155 | 47 | 3.25 | ||
| 14 | SLB | 6 | 8983 | 8420 | 1.06 | 220 | 71 | 3.49 |
| 8 | 10,935 | 8590 | 1.27 | 250 | 78 | 3.65 | ||
| CIVB | 6 | 9208 | 8447 | 1.09 | 222 | 74 | 3.78 | |
| 8 | 11,209 | 9084 | 1.23 | 260 | 80 | 4.03 | ||
| CVB | 6 | 9531 | 8493 | 1.12 | 230 | 78 | 3.67 | |
| 8 | 11,417 | 8768 | 1.31 | 270 | 84 | 3.88 | ||
| SXB | 6 | 9459 | 8781 | 1.07 | 215 | 72 | 3.85 | |
| 8 | 11,332 | 9180 | 1.24 | 257 | 82 | 3.93 |
| Story | Bracing | Span Length | Yield Strength Vy (kN) | Design Strength Vd (kN) | Overstrength Factor Ro | Maximum Displacement Δmax (mm) | Yield Displacement Δy (mm) | Ductility µ |
|---|---|---|---|---|---|---|---|---|
| 4 | SLB | 6 | 7008 | 5222 | 1.34 | 85 | 31 | 2.68 |
| 8 | 7723 | 5409 | 1.42 | 101 | 32 | 3.07 | ||
| CIVB | 6 | 7402 | 5599 | 1.32 | 75 | 27 | 2.77 | |
| 8 | 8088 | 5904 | 1.36 | 90 | 27 | 3.33 | ||
| CVB | 6 | 6785 | 5460 | 1.24 | 75 | 27 | 2.77 | |
| 8 | 7973 | 5990 | 1.33 | 88 | 28 | 3.14 | ||
| SXB | 6 | 6618 | 5654 | 1.17 | 73 | 24 | 3.04 | |
| 8 | 7855 | 6122 | 1.28 | 84 | 26 | 3.23 | ||
| 8 | SLB | 6 | 8383 | 5976 | 1.41 | 205 | 62 | 3.47 |
| 8 | 8806 | 5997 | 1.46 | 220 | 62 | 3.54 | ||
| CIVB | 6 | 9315 | 6405 | 1.45 | 190 | 56 | 3.39 | |
| 8 | 10,167 | 6753 | 1.51 | 205 | 58 | 3.53 | ||
| CVB | 6 | 8697 | 6171 | 1.41 | 186 | 59 | 3.28 | |
| 8 | 8806 | 5997 | 1.47 | 197 | 60 | 3.15 | ||
| SXB | 6 | 8757 | 6312 | 1.38 | 185 | 56 | 3.30 | |
| 8 | 10,190 | 6673 | 1.52 | 199 | 58 | 3.43 | ||
| 14 | SLB | 6 | 8995 | 7708 | 1.16 | 295 | 84 | 3.49 |
| 8 | 11,470 | 7819 | 1.46 | 370 | 101 | 3.65 | ||
| CIVB | 6 | 8900 | 7913 | 1.12 | 299 | 78 | 3.78 | |
| 8 | 11,489 | 7981 | 1.43 | 360 | 89 | 4.03 | ||
| CVB | 6 | 8887 | 7897 | 1.12 | 298 | 81 | 3.67 | |
| 8 | 11,422 | 7971 | 1.43 | 360 | 92 | 3.88 | ||
| SXB | 6 | 8862 | 8011 | 1.11 | 299 | 77 | 3.85 | |
| 8 | 11,332 | 8125 | 1.39 | 350 | 89 | 3.93 |
| ID | Scale Factor | Earthquake | Year | Magnitude | Arias Intensity (cm/s, OA) | Arias Intensity (cm/s, MA) | PGA (g) |
|---|---|---|---|---|---|---|---|
| 1 | 1.0797 | Gazli USSR | 1976 | 6.8 | 5.28 | 13.19 | 0.702 |
| 2 | 1.3647 | Imperial Valley-06 | 1979 | 6.53 | 3.98 | 14.91 | 0.598 |
| 3 | 1.3146 | Nahanni Canada | 1985 | 6.76 | 3.88 | 8.64 | 1.108 |
| 4 | 1.6071 | Superstition Hills | 1987 | 6.54 | 3.74 | 17.34 | 0.432 |
| 5 | 1.7282 | Loma Prieta | 1989 | 6.93 | 5.36 | 20.59 | 0.456 |
| 6 | 1.6220 | Erzican Turkey | 1992 | 6.69 | 1.53 | 10.62 | 0.386 |
| 7 | 0.9724 | Cape Mendocino | 1992 | 7.01 | 5.96 | 8.49 | 1.493 |
| 8 | 1.6892 | Northridge-01 | 1994 | 6.69 | 3.08 | 17.91 | 0.443 |
| 9 | 1.2280 | Kobe Japan | 1995 | 6.90 | 8.39 | 16.09 | 0.834 |
| 10 | 1.1306 | Chi-Chi Taiwan | 1999 | 7.62 | 5.34 | 17.22 | 0.636 |
| 11 | 1.3235 | Duzce Turkey | 1999 | 7.14 | 3.72 | 12.12 | 0.739 |
| 12 | 1.4563 | Manjil Iran | 1990 | 7.37 | 4.64 | 31.79 | 0.514 |
| 13 | 1.7718 | Tottori Japan | 2000 | 6.61 | 5.29 | 18.07 | 0.733 |
| 14 | 1.1297 | Bam Iran | 2003 | 6.60 | 8.02 | 18.22 | 0.807 |
| 15 | 0.7919 | Niigata Japan | 2004 | 6.63 | 14.51 | 17.35 | 1.166 |
| 16 | 1.3327 | Chuetsu-oki Japan | 2007 | 6.80 | 2.81 | 16.73 | 0.482 |
| 17 | 1.0688 | Iwate Japan | 2008 | 6.90 | 11.82 | 19.25 | 1.343 |
| 18 | 1.5870 | El Mayor Mexico | 2010 | 7.20 | 6.01 | 29.53 | 0.537 |
| 19 | 1.0332 | Darfield NZ | 2010 | 7.00 | 4.49 | 10.71 | 0.764 |
| 20 | 1.6745 | Duzce Turkey | 1999 | 7.14 | 13.36 | 20.66 | 1.031 |
| 21 | 1.3840 | Tohoku | 1923 | 7.90 | 11.59 | 78.15 | 0.427 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Naghshineh, A.; Tehrani, F.M.; Fischer, O. Application of Sustainable Concrete in the Seismic Evaluation of an Innovative Type of Buckling Restrained Brace. Sustainability 2022, 14, 16344. https://doi.org/10.3390/su142416344
Naghshineh A, Tehrani FM, Fischer O. Application of Sustainable Concrete in the Seismic Evaluation of an Innovative Type of Buckling Restrained Brace. Sustainability. 2022; 14(24):16344. https://doi.org/10.3390/su142416344
Chicago/Turabian StyleNaghshineh, Ali, Fariborz M. Tehrani, and Oliver Fischer. 2022. "Application of Sustainable Concrete in the Seismic Evaluation of an Innovative Type of Buckling Restrained Brace" Sustainability 14, no. 24: 16344. https://doi.org/10.3390/su142416344
APA StyleNaghshineh, A., Tehrani, F. M., & Fischer, O. (2022). Application of Sustainable Concrete in the Seismic Evaluation of an Innovative Type of Buckling Restrained Brace. Sustainability, 14(24), 16344. https://doi.org/10.3390/su142416344