Development and Application of an Intelligent Assessment System for Mathematics Learning Strategy among High School Students—Take Jianzha County as an Example
Abstract
1. Introduction
2. Literature Review
2.1. The Relationship between Learning Strategies and Academic Performance
2.2. Assessment Tool for Mathematics Learning Strategies
2.3. Intervention Studies to Improve Mathematics Learning Strategies
2.4. Research Questions
- 1.
- How to develop an intelligent assessment system of mathematics learning strategy for high school students?
- 2.
- How to apply the intelligent assessment system in Jianza County, Huangnan Tibetan Autonomous Prefecture, Qinghai Province, China?
- 3.
- How to test the effectiveness of its application?
3. Methodology
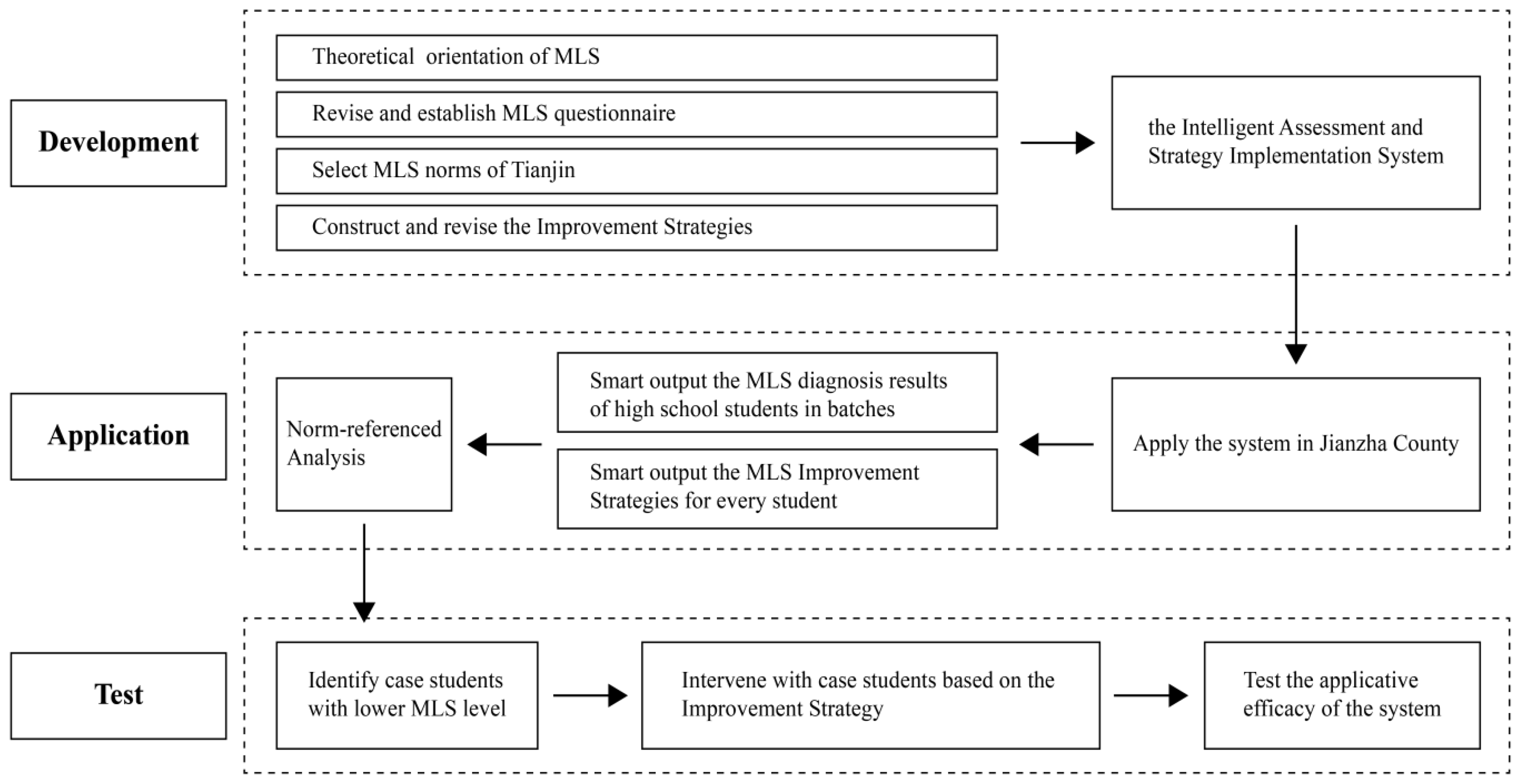
3.1. Research Design
3.2. Instruments
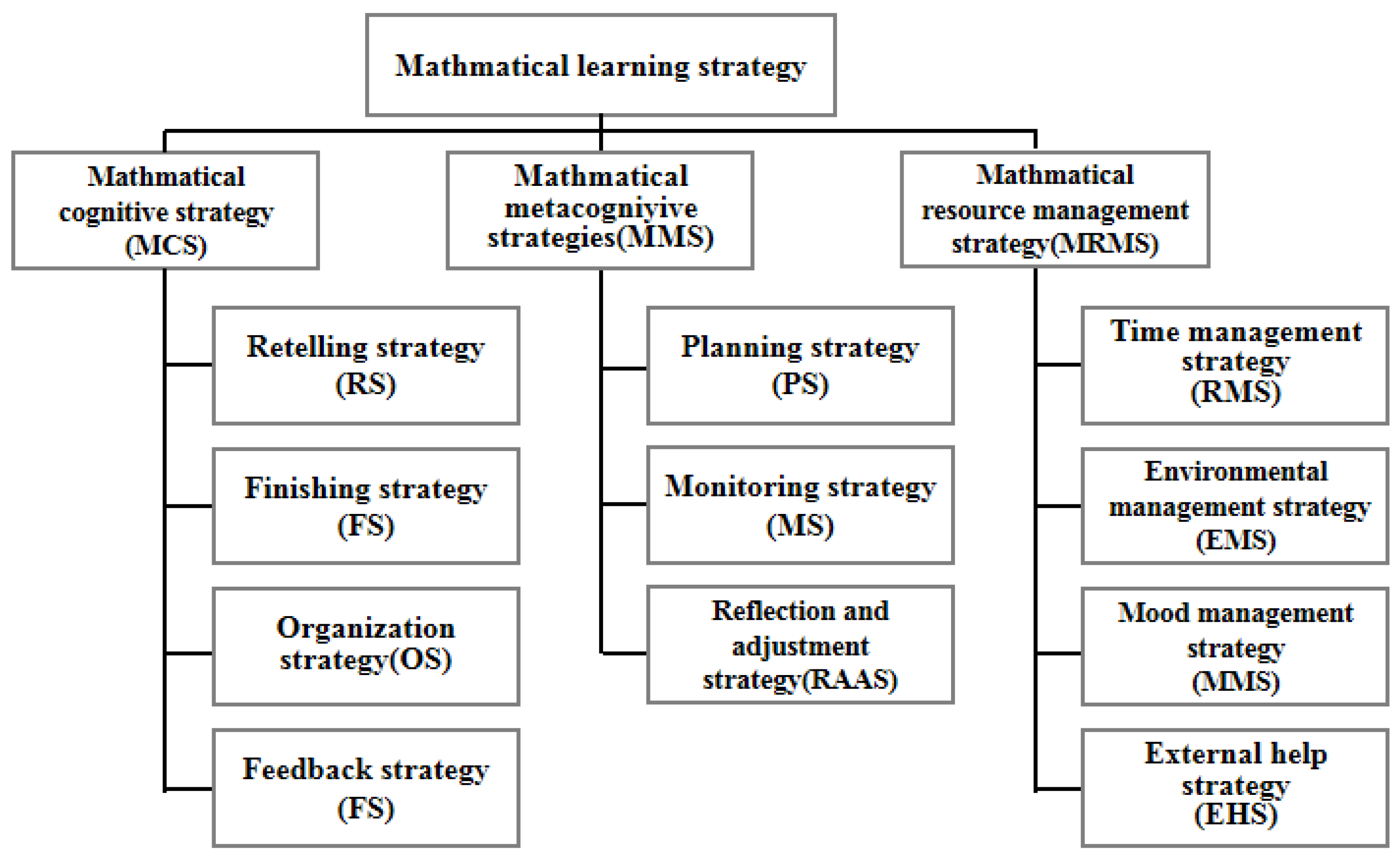
3.2.1. Mathematics Learning Strategies Questionnaire
3.2.2. Test of Mathematics Academic Achievement
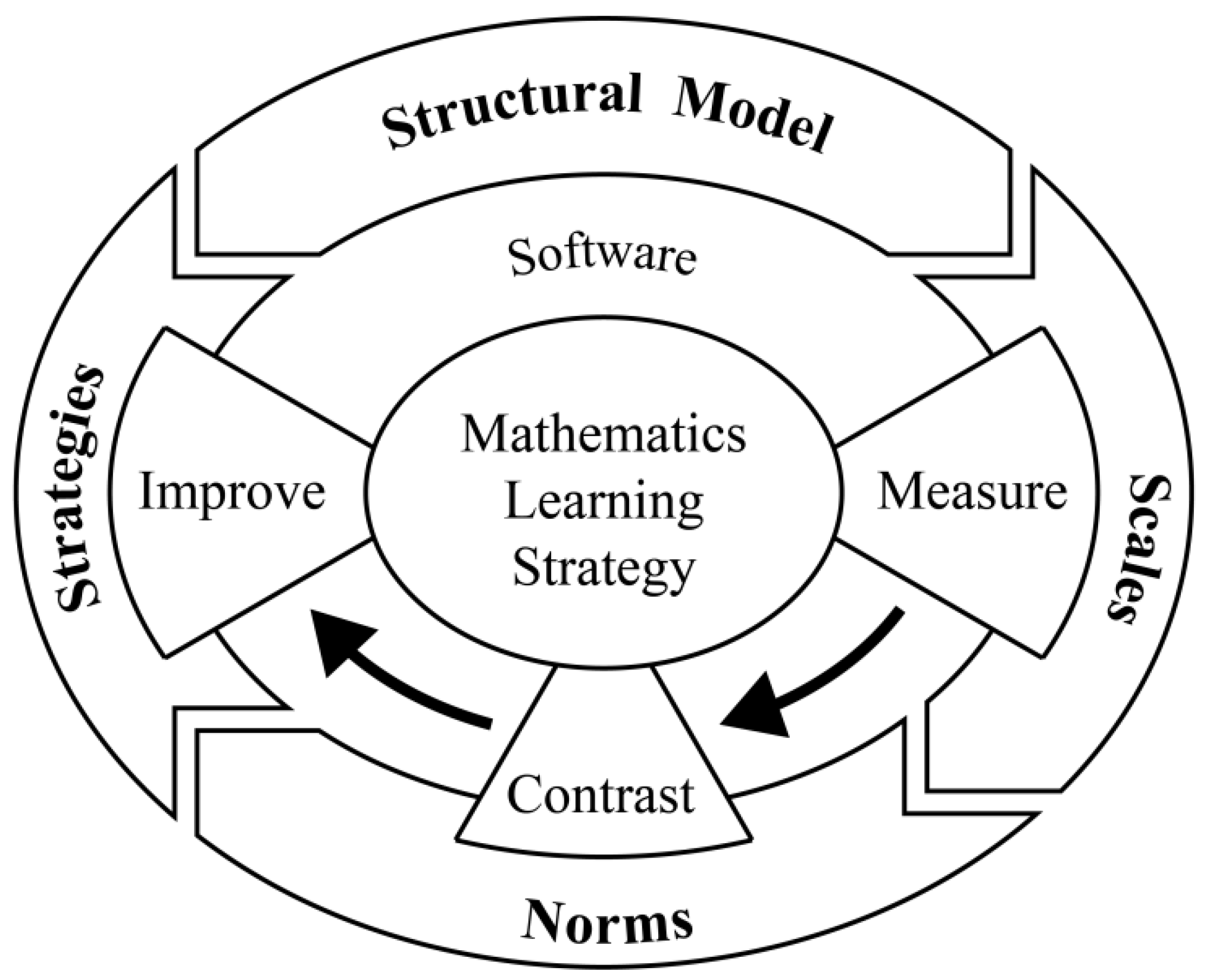
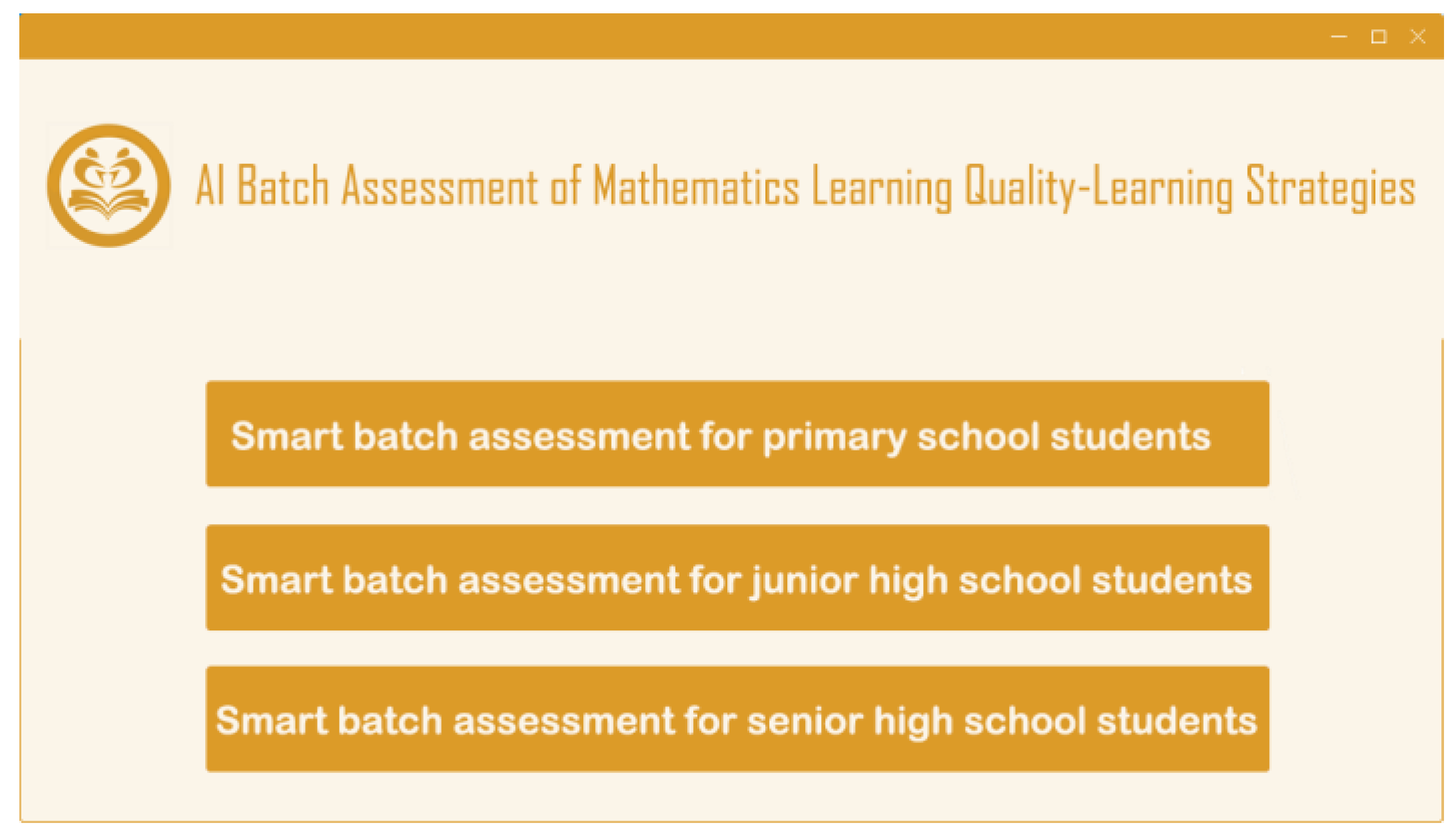
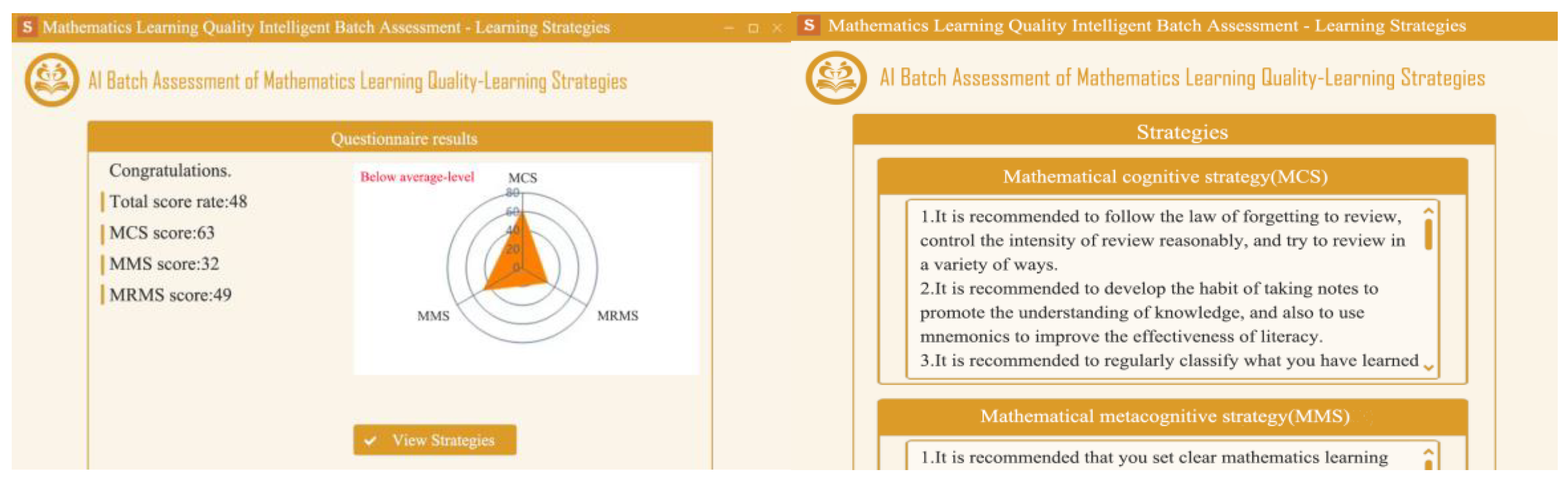
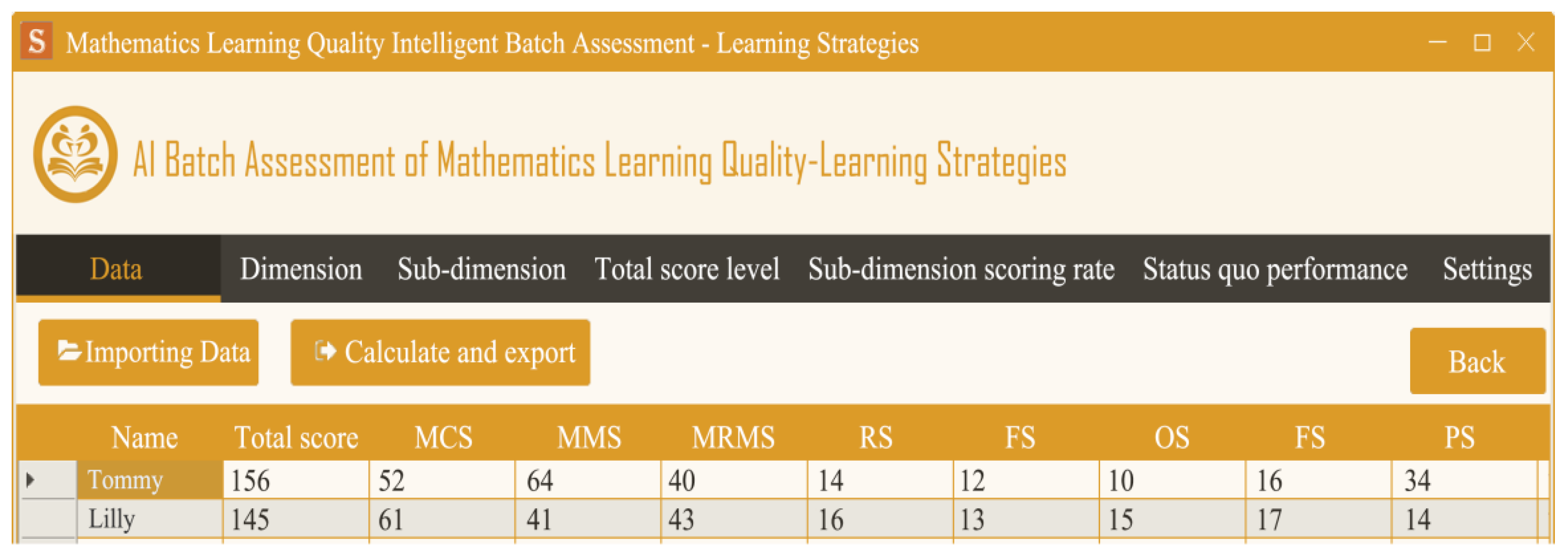
3.2.3. Intelligent Assessment System
3.3. Sample and Data Collection
3.4. Intelligent Intervention Process
4. Results
4.1. Development of Intelligent Assessment System
4.1.1. Theoretical Framework and Assessment Scale
4.1.2. Regional Norms
4.1.3. Development of Improvement Strategy
4.1.4. Intelligent Assessment Software
4.2. Intelligence Assessment Diagnosis and Norm-Referenced Analysis
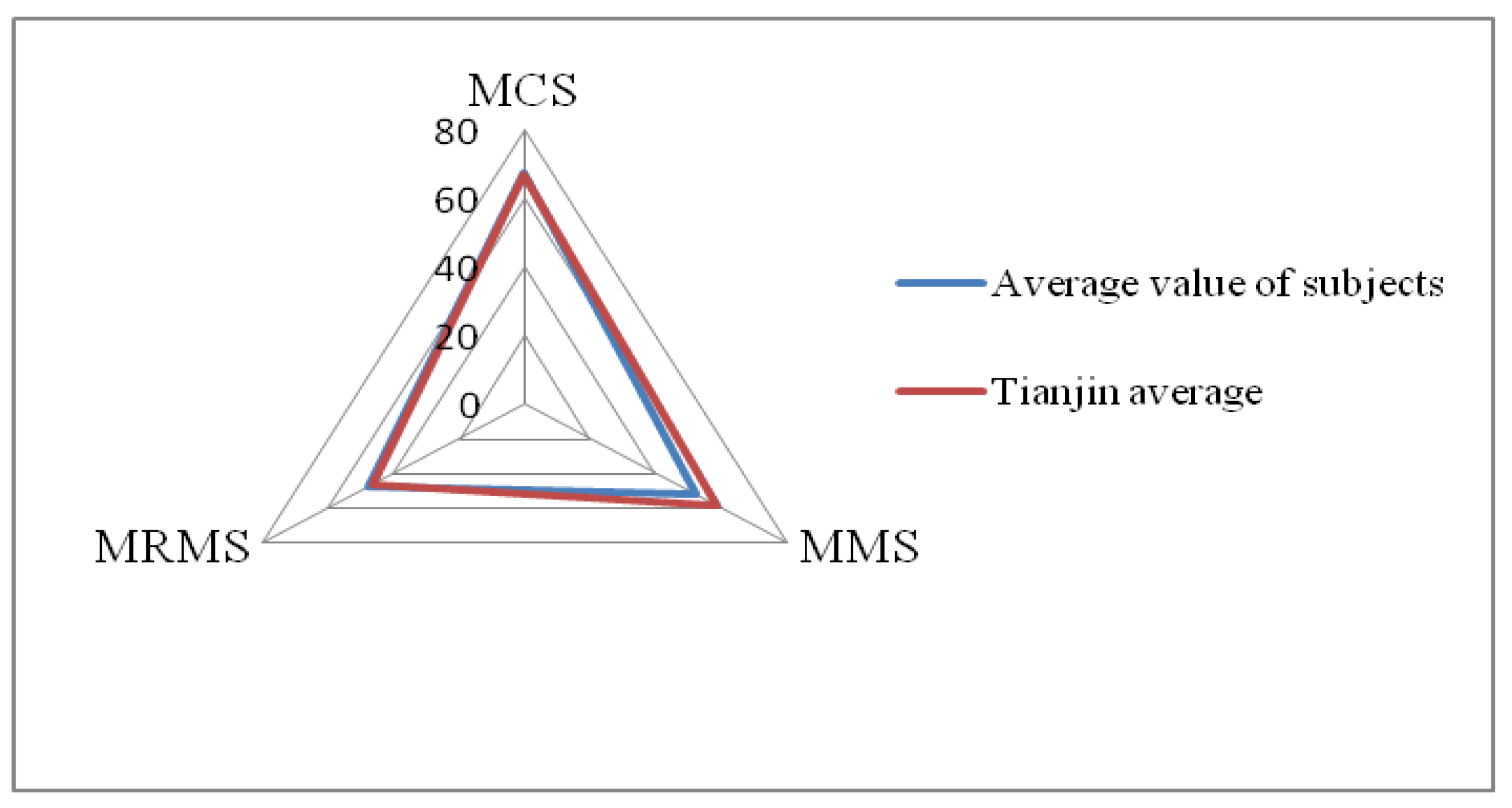
4.2.1. Diagnostic Results of Mathematics Learning Strategies and the Norm Reference Analysis of High School Students in Jianzha County
4.2.2. Diagnostic Results and Norm-Referenced Analysis of the Case Students’ Mathematics Learning Strategies
4.3. Test of Applicative Efficacy of the Intelligence Assessment and Strategy Implementation System
4.3.1. Analysis of Applicative Efficacy on Student A with Weak Cognitive Strategies
4.3.2. Analysis of Applicative Efficacy on Student B with Weak Metacognitive Strategies
4.3.3. Analysis of Applicative Efficacy on Student C with Weak Resource Management Strategies
5. Discussion and Conclusions
5.1. About the Results of Intelligent Diagnosis Using an Intelligent Assessment System
5.2. About the Effect of Case Intervention Using an Intelligent Strategy Implementation System
5.3. Conclusions
6. Recommendations
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Mathematical Learning Strategies | Score | |||||
|---|---|---|---|---|---|---|
| 1 | When I find similar mathematical concepts and formulas, I will compare their similarities and differences. For example, point-slope, oblique-intercept, intercept, and general expressions and their applicability. | 1 | 2 | 3 | 4 | 5 |
| 2 | I will compare myself to classmates with differences in learning behavior to find ways to improve math performance. | 1 | 2 | 3 | 4 | 5 |
| 3 | When learning mathematical content with more knowledge points such as functions and conic sections, I will organize its images, properties, and expressions in a table, with pictures and texts, to assist learning. | 1 | 2 | 3 | 4 | 5 |
| 4 | I will summarize and summarize the mathematical knowledge, problem-solving methods, and typical examples involved in the same type of questions. | 1 | 2 | 3 | 4 | 5 |
| 5 | When I take a math test, I always have to look at the test paper first to understand the number and type of questions, so that I know what to do. | 1 | 2 | 3 | 4 | 5 |
| 6 | Teachers ____ teach us mathematics learning methods, such as effective organization of review, setting learning goals, efficient use of time, etc. | 1 | 2 | 3 | 4 | 5 |
| 7 | I attach importance to analogy learning and variant training. For example, after learning the proof of the cosine theorem of acute triangles, I try to use this method to prove the cosine theorem of right triangles and obtuse triangles. | 1 | 2 | 3 | 4 | 5 |
| 8 | When I am learning new mathematical concepts and formulas, I am often disturbed by similar concepts and formulas. For example, due to the influence of the prism volume formula, I forget to multiply by 1/3 when calculating the pyramid volume. | 1 | 2 | 3 | 4 | 5 |
| 9 | In math class, I notice and manage my distractions. | 1 | 2 | 3 | 4 | 5 |
| 10 | I am aware of my mathematics learning status, and I am aware of the changes in my learning status. | 1 | 2 | 3 | 4 | 5 |
| 11 | When solving the problem, I will pay attention to what conditions are given in the question, what problems are required to be solved, and analyze which conditions are helpful for the problems I solve. | 1 | 2 | 3 | 4 | 5 |
| 12 | Parents tell us about math learning methods, such as: making study plans, using time efficiently, etc. | 1 | 2 | 3 | 4 | 5 |
| 13 | When I encounter a complex mathematical problem, I will break it down into several small problems and break them down. | 1 | 2 | 3 | 4 | 5 |
| 14 | I make predictions about the time it takes for math learning tasks, and guarantee them. | 1 | 2 | 3 | 4 | 5 |
| 15 | I organize the mathematics study case, homework, test papers, and other materials in high school in an orderly manner, which is convenient for review and reference. | 1 | 2 | 3 | 4 | 5 |
| 16 | When encountering math teachers with different teaching styles, I pay attention to whether my learning style is compatible with them. | 1 | 2 | 3 | 4 | 5 |
| 17 | I often find out what I have mastered by reciting and dictating mathematical concepts, formulas, and theorems. | 1 | 2 | 3 | 4 | 5 |
| 18 | I learned about mathematics learning methods by browsing the Internet, reading books, etc. | 1 | 2 | 3 | 4 | 5 |
| 19 | Before the math class, I will preview and figure out key points and difficulties, so that I can focus on listening in class. | 1 | 2 | 3 | 4 | 5 |
| 20 | When I can’t solve a problem with one method, I use other methods. | 1 | 2 | 3 | 4 | 5 |
| 21 | I don’t know how to improve my math learning efficiency. | 1 | 2 | 3 | 4 | 5 |
| 22 | In high school mathematics study, I can effectively use the scattered time (such as breaks between classes, waiting time, etc.) and the whole time (such as evening self-study, weekends, etc.). | 1 | 2 | 3 | 4 | 5 |
| 23 | I resisted the evaluation of my math study by teachers and parents and rarely listened to their opinions. | 1 | 2 | 3 | 4 | 5 |
| 24 | In the math test, I will pay attention to my problem-solving speed and answering time. | 1 | 2 | 3 | 4 | 5 |
| 25 | When I study mathematics, I often focus on two uses, such as listening to music while studying. | 1 | 2 | 3 | 4 | 5 |
| 26 | I attach great importance to the results of the mathematics test and rank and use this as the basis for my evaluation of the adjustment of my mathematics learning situation. | 1 | 2 | 3 | 4 | 5 |
| 27 | When I am frustrated in the process of mathematics learning, I will review my experience of mathematics progress to improve my self-confidence in mathematics learning. | 1 | 2 | 3 | 4 | 5 |
| 28 | For the math homework, the things I did wrong and won’t do in the test paper, I would put them together and revisit them after a while. | 1 | 2 | 3 | 4 | 5 |
| 29 | In math class, I control myself not to get distracted. | 1 | 2 | 3 | 4 | 5 |
| 30 | I will create a good environment for myself to study mathematics, such as maintaining the environment’s quietness, neatness of the desktop, etc., to improve the efficiency of mathematics learning. | 1 | 2 | 3 | 4 | 5 |
| 31 | I have a clear math study plan. | 1 | 2 | 3 | 4 | 5 |
| 32 | After entering high school, no matter how busy I am, I can always arrange time reasonably and complete my math homework on time. | 1 | 2 | 3 | 4 | 5 |
| 33 | In the process of solving mathematical problems, I will choose the problem-solving method according to the problem type and degree of difficulty. For example, when answering multiple-choice questions, I use the substitution method and the special value method; when solving complex solid geometric problems, I use the vector method. | 1 | 2 | 3 | 4 | 5 |
| 34 | When solving math problems, I often encourage myself to think independently, not to be too unreasonable. Rely on teacher explanations or answer prompts. | 1 | 2 | 3 | 4 | 5 |
| 35 | I enrich my mathematics knowledge and cultivate my interest in mathematics by participating in high school mathematics competitions, high school mathematics summer camps, and other activities. | 1 | 2 | 3 | 4 | 5 |
| 36 | When learning solid geometry, I will use objects such as walls, rulers, books, etc. to build models. help to understand. | 1 | 2 | 3 | 4 | 5 |
| 37 | I know goal A and direct action of my mathematics learning and encourage myself to work hard for it. | 1 | 2 | 3 | 4 | 5 |
| 38 | I try to use some online resources (such as micro-courses) and mathematical software (such as Geometric Sketchpad, etc.) to assist in learning. | 1 | 2 | 3 | 4 | 5 |
| 39 | After completing my math homework, I check my answer against the correct answer Is it correct and standard? | 1 | 2 | 3 | 4 | 5 |
| 40 | When I can’t remember some mathematical concepts, formulas, theorems, properties, etc., I will review the materials in time for review. | 1 | 2 | 3 | 4 | 5 |
| 41 | I often review the content of each chapter of high school mathematics that I have learned, and sort out the heavy weight of each chapter. Points, difficulties, and common question types and test sites. | 1 | 2 | 3 | 4 | 5 |
| 42 | I often summarize my approach to learning mathematics and reflect on whether it works. | 1 | 2 | 3 | 4 | 5 |
| 43 | After entering high school, I often asked my teachers and classmates for some math problems. | 1 | 2 | 3 | 4 | 5 |
| 44 | In the study of various modules of high school mathematics, I will consciously practice more for the modules that I am not good at. | 1 | 2 | 3 | 4 | 5 |
| 45 | After finishing the math knowledge of each module, I will sort out the knowledge and form a knowledge network. | 1 | 2 | 3 | 4 | 5 |
| 46 | When I study mathematics, I will mark important content and memorize it repeatedly. | 1 | 2 | 3 | 4 | 5 |
| 47 | I will describe related concepts and theorems in various ways such as text language, graphic language, and symbolic language. | 1 | 2 | 3 | 4 | 5 |
| 48 | I always feel that the efficiency of studying mathematics is the highest only before the exam. | 1 | 2 | 3 | 4 | 5 |
| 49 | When encountering an unfamiliar math problem, I often try to relate it to a problem I am familiar with. | 1 | 2 | 3 | 4 | 5 |
| 50 | During my high school mathematics study, I was able to adjust my mentality so that I was neither too slack nor too nervous. | 1 | 2 | 3 | 4 | 5 |
| 51 | When learning new mathematics content, I will preview or read related materials in advance (such as completing a study guide or reading-related mathematics history, etc.). | 1 | 2 | 3 | 4 | 5 |
| 52 | During my high school mathematics study, I will arrange rest and study reasonably according to the activity of my brain. | 1 | 2 | 3 | 4 | 5 |
| 53 | Since high school, I have not systematically developed a math study plan. | 1 | 2 | 3 | 4 | 5 |
| 54 | I often pass a simple test after a certain stage of study to test the effectiveness of my mathematics learning. | 1 | 2 | 3 | 4 | 5 |
Appendix B
| Number | Questions |
|---|---|
| 1 | Are parents able to provide guidance and assistance in the study and use of your mathematics learning methods? |
| 2 | Are teachers able to provide guidance and assistance in the study and use of your mathematics learning methods? |
| 3 | Please evaluate your learning methods in mathematics learning |
| 4 | Do you regularly categorize the math problems you have done? For example: Integrate mathematical problem-solving ideas and refine mathematical problem-solving methods. |
| 5 | Will you use your usual math homework and test paper feedback to conduct self-analysis and adjust your attitude and method of math learning? |
| 6 | After learning new mathematical knowledge, can you review it in time and often? |
| 7 | When you listen to the math class, will you grasp the key points and make comments on the key points? |
| 8 | Based on understanding mathematical concepts and other knowledge, will you try to explain them in your language to deepen your understanding and memory of knowledge? |
| 9 | Will you actively use math problems to test your mastery of math knowledge? For example, when dealing with the wrong questions in the exercises, we should correct them in time, and think about “why did I make mistakes”, and “have I mastered all the knowledge points of the wrong question inspection”. |
| 10 | Do you try to summarize the main points and framework of the mathematical knowledge of a module or unit regularly in the form of outlines, tables, graphs, etc.? |
| 11 | Do you review math in a variety of practice formats? For example, reviewing knowledge points by asking and answering questions and doing math exercises with the same desk. |
| 12 | Do you use memory skills to help you memorize some mathematical formulas and theorems? For example: memorizing formulas. |
Appendix C
| Number | Questions |
|---|---|
| 1 | Are parents able to provide guidance and assistance in the study and use of your mathematics learning methods? |
| 2 | Are teachers able to provide guidance and assistance in the study and use of your mathematics learning methods? |
| 3 | Please evaluate your learning methods in mathematics learning |
| 4 | Will you formulate reasonable and specific short-term and long-term goals for mathematics learning according to your current mathematics learning situation? For example: set a time to do homework. |
| 5 | Do you plan your math test time properly? |
| 6 | Do you have the habit of checking the results after completing your math homework and exam papers? For example: bring the answer to the question for verification; recalculate the question again, etc. |
| 7 | When solving math problems, do you compare your results with your life and learning experiences? For example, when learning plane geometry, the positional relationship between two straight lines, line-surface, and surface-surface relationship can be considered about the corners in life. |
| 8 | Are you able to reflect on your learning attitude and learning methods from time to time? For example: often reflect on “whether I can listen carefully in the math class” and “whether I can take the math homework assigned by the teacher seriously”. |
| 9 | In general, what do you think your progress in mathematics depends on? For example effort, luck, ability? |
| 10 | Please evaluate the students around you who have good grades in mathematics. What is the reason for them to achieve good grades in mathematics? |
| 11 | Do you deepen your thinking and understanding of mathematics learning through self-questioning? For example: in mathematics learning, I often ask myself “Have I mastered all the mathematics knowledge today” and “What is the reason for not mastering all of them”. |
Appendix D
| Number | Questions |
|---|---|
| 1 | Are parents able to provide guidance and assistance in the study and use of your mathematics learning methods? |
| 2 | Are teachers able to provide guidance and assistance in the study and use of your mathematics learning methods? |
| 3 | Please evaluate your learning methods in mathematics learning |
| 4 | Do you have some thoughts about math problems before seeking help from others? |
| 5 | Will you choose a suitable math learning environment? For example, choose to study math in a quiet environment with suitable light, temperature, and quiet. |
| 6 | Are you able to correctly face the negative emotions generated in mathematics learning? For example: when you have bad emotions in the process of mathematics learning, take a short rest, take a deep breath, or do some other healthy activities that you are interested in to divert your attention or experience the beauty and value of mathematics, cultivate and stimulate good mathematics learning motivation, and actively adjust, Get rid of a bad mood? |
| 7 | Have you raised your hand to ask the teacher a question when you have questions during math class? Do you choose to ask the teacher when you have questions after class? |
| 8 | Would you create an ideal math learning environment for yourself? For example, pay attention to the orderly arrangement of desks, chairs, and books; after the mathematics study of a certain module or a certain unit, regularly organize mathematics study materials such as mathematics papers, mathematics study cases, and mathematics homework books. |
| 9 | Do you make a to-do list daily and prioritize the things that need to be done? |
| 10 | When you encounter difficulties in the process of mathematics learning, will you be afraid of difficulties? If so, would you use self-motivation to increase your motivation to learn mathematics? |
| 11 | Do you have a strict time limit for completing math homework and avoid procrastination? For example: make a detailed homework schedule, the contents of the schedule include what homework to write in a certain period, how much to write, and then complete the math homework on time within the period specified in the homework plan. |
References
- Weinstein, C.E.; Acee, T.W.; Jung, J. Self-regulation and learning strategies. New Dir. Teach. Learn. 2011, 126, 45–53. [Google Scholar] [CrossRef]
- Neroni, J.; Meijs, C.; Gijselaers, H.J.; Kirschner, P.A.; de Groot, R.H. Learning strategies and academic performance in distance education. Learn. Individ. Differ. 2019, 73, 1–7. [Google Scholar] [CrossRef]
- Cho, M.-H.; Heron, M.L. Self-regulated learning: The role of motivation, emotion, and use of learning strategies in students’ learning experiences in a self-paced online mathematics course. Distance Educ. 2015, 36, 80–99. [Google Scholar] [CrossRef]
- Areepattamannil, S.; Caleon, I.S. Relationships of cognitive and metacognitive learning strategies to mathematics achievement in four high-performing East Asian education systems. J. Genet. Psychol. 2013, 174, 696–702. [Google Scholar] [CrossRef]
- In’am, A.; Sutrisno E, S. Strengthening Students’ Self-Efficacy and Motivation in Learning Mathematics through the Cooperative Learning Model. Int. J. Instr. 2021, 14, 395–410. [Google Scholar] [CrossRef]
- Khanal, B.; Panthi, R.K.; Kshetree, M.P.; Acharya, B.R.; Belbase, S. Mathematics learning strategies of high school students in Nepal. SN Soc. Sci. 2021, 1, 1–28. [Google Scholar] [CrossRef]
- Lee, C.-Y.; Kung, H.-Y. Math self-concept and mathematics achievement: Examining gender variation and reciprocal relations among junior high school students in Taiwan. Eurasia J. Math. Sci. Technol. Educ. 2018, 14, 1239–1252. [Google Scholar]
- Masitoh, L.F.; Fitriyani, H. Improving students’ mathematics self-efficacy through problem-based learning. Malikussaleh J. Math. Learn. MJML 2018, 1, 26–30. [Google Scholar] [CrossRef]
- Dignath, C.; Büttner, G. Teachers’ direct and indirect promotion of self-regulated learning in primary and secondary school mathematics classes—Insights from video-based classroom observations and teacher interviews. Metacognition Learn. 2018, 13, 127–157. [Google Scholar] [CrossRef]
- Etherton, J.L.; Oberle, C.D.; Rhoton, J.; Ney, A. Effects of Cogmed working memory training on cognitive performance. Psychol. Res. 2019, 83, 1506–1518. [Google Scholar] [CrossRef]
- Giofrè, D.; Donolato, E.; Mammarella, I.C. The differential role of verbal and visuospatial working memory in mathematics and reading. Trends Neurosci. Educ. 2018, 12, 1–6. [Google Scholar] [CrossRef]
- Obergriesser, S.; Stoeger, H. Students’ emotions of enjoyment and boredom and their use of cognitive learning strategies—How do they affect one another? Learn. Instr. 2020, 66, 101–285. [Google Scholar] [CrossRef]
- Guo, W.; Lau, K.L.; Wei, J. Teacher feedback and students’ self-regulated learning in mathematics: A comparison between a high-achieving and a low-achieving secondary schools. Stud. Educ. Eval. 2019, 63, 48–58. [Google Scholar] [CrossRef]
- Wang, Y.-L.; Liang, J.-C.; Lin, C.-Y.; Tsai, C.-C. Identifying Taiwanese junior-high school students’ mathematics learning profiles and their roles in mathematics learning self-efficacy and academic performance. Learn. Individ. Differ. 2017, 54, 92–101. [Google Scholar] [CrossRef]
- Liu, Q.; Du, X.; Zhao, S.; Liu, J.; Cai, J. The role of memorization in students’ self-reported mathematics learning: A large-scale study of Chinese eighth-grade students. Asia Pac. Educ. Rev. 2019, 20, 361–374. [Google Scholar] [CrossRef]
- Bai, Y.-L.; Zhang, L.-X.; Sun, M.-X.; Xu, X.-B. Status and path of intergenerational transmission of poverty in rural China: A human capital investment perspective. J. Integr. Agric. 2021, 20, 1080–1091. [Google Scholar] [CrossRef]
- OECD. PISA 2012 Assessment and Analytical Framework: Mathematics, Reading, Science, Problem Solving and Financial Literacy; OECD Publishing: Washington, DC, USA, 2013; pp. 139–165. [Google Scholar]
- Wolters, C.A. The relation between high school students’ motivational regulation and their use of learning strategies, effort, and classroom performance. Learn. Individ. Differ. 1999, 11, 281–299. [Google Scholar] [CrossRef]
- Wu, H.-M. Cognition strategies, metacognition strategies and academic performance in Taiwan. In Cognition, Metacognition and Academic Performance; Routledge: London, UK, 2017; pp. 70–86. [Google Scholar]
- Zhou, Y.; Wang, J. Goal orientation, learning strategies, and academic performance in adult distance learning. Soc. Behav. Personal. Int. J. 2019, 47, 1–20. [Google Scholar] [CrossRef]
- Lugosi, E.; Uribe, G. Active learning strategies with positive effects on student’s achievements in undergraduate mathematics education. Int. J. Math. Educ. Sci. Technol. 2022, 53, 403–424. [Google Scholar] [CrossRef]
- Ramirez, G.; Shaw, S.T.; Maloney, E.A. Math anxiety: Past research, promising interventions, and a new interpretation framework. Educ. Psychol. 2018, 53, 145–164. [Google Scholar] [CrossRef]
- Delima, N.; Cahyawati, D. Students’ Mathematics Self-Concept, Mathematics Anxiety and Mathematics Self-Regulated Learning during the Covid-19 Pandemic. J. Pendidik. Mat. 2021, 15, 103–114. [Google Scholar] [CrossRef]
- Khanal, B. Approaches for Enhancing Mathematics Learning of Students with Learning Difficulties. Educ. J. 2022, 10, 88–92. [Google Scholar] [CrossRef]
- Jose, M.; Cordero, M.G.-I. The effect of teaching strategies on student achievement: An analysis using TALIS-PISA-link. J. Policy Modeling 2018, 40, 1313–1331. [Google Scholar] [CrossRef]
- Magen-Nagar, N. The Effects of Learning Strategies on Mathematical Literacy: A Comparison between Lower and Higher Achieving Countries. Int. J. Res. Educ. Sci. 2016, 2, 306–321. [Google Scholar] [CrossRef]
- Zimmerman, B.J.; Pons, M.M. Development of a structured interview for assessing student use of self-regulated learning strategies. Am. Educ. Res. J. 1986, 23, 614–628. [Google Scholar] [CrossRef]
- Weinstein, C.E.; Mayer, R.E. The teaching of learning strategies. In Handbook of Research on Teaching; Wittrock, M., Ed.; Macmillan: New York, NY, USA, 1986; pp. 315–327. [Google Scholar]
- Weinstein, C.E.; Palmer, D.R. Learning and Study Strategies Inventory—High School Version; H&H Publishing: Clearwater, FL, USA, 1990. [Google Scholar]
- Zhou, Y.; Zhang, Q.; Han, Y. A study of learning strategies for students with learning difficulties. Spec. Educ. China 2003, 3, 58–59. (In Chinese) [Google Scholar]
- An, Q.; Xi, J.; Zuo, Z. A Chinese revision of the Learning Strategy Scale LASSI-HS for high school students. J. Cap. Norm. Univ. Soc. Sci. Ed. 2019, 3, 180–188. (In Chinese) [Google Scholar]
- Li, J.; Sun, Y.; Wang, G. Research on the norm of high school students’ mathematics learning strategies and their level standards—Taking Tianjin as an example. J. Math. Educ. 2017, 4, 8–14. (In Chinese) [Google Scholar]
- Wang, G.; Liao, J.; Huang, Q.; Wang, Z.; McDougall, D. Development of a questionnaire on mathematics learning strategies for high school students. J. Math. Educ. 2015, 5, 29–36. (In Chinese) [Google Scholar]
- Oxford, R.; Nyikos, M. Variables affecting the choice of language learning strategies by university students. Mod. Lang. J. 1989, 73, 291–300. [Google Scholar] [CrossRef]
- Gasco, J.; Villarroel, J.D.; Goñi, A. Differences in the use of learning strategies in mathematics in 8th and 9th grade. Procedia-Soc. Behav. Sci. 2014, 116, 1040–1043. [Google Scholar] [CrossRef]
- Cao, Y.; Chen, P. A study on the use of mathematics learning strategies and their effects on secondary school students of different ability levels. Educ. Res. Exp. 2018, 4, 50–56. (In Chinese) [Google Scholar]
- Hacker, D.J.; Kiuhara, S.A.; Levin, J.R. A metacognitive intervention for teaching fractions to students with or at-risk for learning disabilities in mathematics. ZDM 2019, 51, 601–612. [Google Scholar] [CrossRef]
- Wang, G.; Li, J.; Kang, Y. The Intelligent Batch Assessment of Mathematics Learning Quality for Primary and Secondary School Students—Learning Strategies. Intelligent Assessment and Strategy Implementation System; National Copyright Administration of the People’s Republic of China: Beijing, China, 2021SR1219917.2021-08-17.
- Li, Z. A case study on the diagnosis and guidance of learning strategies for students with low mathematics learning efficiency in high school. Master Thesis, Tianjin Normal University, Tianjin, China, 2017; pp. 26–27. [Google Scholar]
- Chen, C. Intervention study of mathematics learning strategies for junior high school students. Master’s Thesis, Guangxi University, Nanning, China, 2018; pp. 23–29. [Google Scholar]
| Main Dimension | Sub-Dimension | Question Number | Amount |
|---|---|---|---|
| Mathematical cognitive strategy (MLS) | Retelling strategy (RS) | 8 *, 28, 40, 46, 47 | 5 |
| Finishing strategy (FS) | 1, 3, 7, 11, 13 | 5 | |
| Organizing strategy (OS) | 4, 41, 45, 49, 51 | 5 | |
| Feedback strategy (FS) | 17, 23 *, 26, 39, 54 | 5 | |
| Mathematical metacognitive strategy (MMS) | Planning strategy (PS) | 5, 14, 19, 33, 53 * | 5 |
| Monitoring strategy (MS) | 2, 9, 10, 16, 24 | 5 | |
| Reflection and adjustment strategy (RAAS) | 20, 21 *, 42, 44, 52 | 5 | |
| Mathematical resource-management strategy (MRMS) | Time-management strategy (TMS) | 22, 32, 48 | 3 |
| Environmental-management strategy (EMS) | 15, 25 *, 30, 35 | 4 | |
| Mood-management strategy (MMS) | 27, 34, 37, 50 | 4 | |
| External help strategy (EHS) | 36, 38, 43 | 3 | |
| Polygraph Questions | 31–53, 9–29 | 4 | |
| Background questions | 6, 12, 18 | 3 | |
| Dimension | Cronbach’s a Coefficient | Split-Half Reliability |
|---|---|---|
| Mathematics Learning Strategies | 0.946 | 0.930 |
| Mathematical cognitive strategy | 0.894 | 0.883 |
| Mathematical metacognitive strategy | 0.881 | 0.871 |
| Mathematical resource-management strategy | 0.768 | 0.809 |
| Dimension | MLS | MCS | MMS | MRMS |
|---|---|---|---|---|
| MLS | 1 | |||
| MCS | 0.964 ** | 1 | ||
| MMS | 0.946 ** | 0.882 ** | 1 | |
| MRMS | 0.916 ** | 0.821 ** | 0.793 ** | 1 |
| Level | T-Score | Raw Score (X) | Percentage Rating (PR) | |
|---|---|---|---|---|
| MLS | excellent | T ≥ 68 | X ≥ 213 | PR ≥ 96.49 |
| upper–middle | 68 > T ≥ 56 | 213 > X ≥ 176 | 96.49 > PR ≥ 73.78 | |
| middle | 56 > T ≥ 44 | 176 > X ≥ 148 | 73.78 > PR ≥ 27.39 | |
| lower–middle | 44 > T ≥ 32 | 148 > X ≥ 125 | 27.39 > PR ≥ 3.61 | |
| poor | 32 > T | 125 > X | 3.61 > PR | |
| MCS | excellent | T ≥ 68 | X ≥ 88 | PR ≥ 96.49 |
| upper–middle | 68 > T ≥ 56 | 88 > X ≥ 73 | 96.49 > PR ≥ 74.46 | |
| middle | 56 > T ≥ 44 | 73 > X ≥ 61 | 74.46 > PR ≥ 29.43 | |
| lower–middle | 44 > T ≥ 32 | 61 > X ≥ 50 | 29.43 > PR ≥ 3.61 | |
| poor | 32 > T | 50 > X | 3.61 > PR | |
| MMS | excellent | T ≥ 68 | X ≥ 67 | PR ≥ 96.49 |
| upper–middle | 68 > T ≥ 56 | 67 > X ≥ 61 | 96.49 > PR ≥ 75.05 | |
| middle | 56 > T ≥ 44 | 61 > X ≥ 56 | 75.05 > PR ≥ 33.53 | |
| lower–middle | 44 > T ≥ 32 | 56 > X ≥ 39 | 33.53 > PR ≥ 4.68 | |
| poor | 32 > T | 39 > X | 4.68 > PR | |
| MRMS | excellent | T ≥ 68 | X ≥ 59 | PR ≥ 96.59 |
| upper–middle | 68 > T ≥ 56 | 59 > X ≥ 50 | 96.59 > PR ≥ 76.51 | |
| middle | 56 > T ≥ 44 | 50 > X ≥ 43 | 76.51 > PR ≥ 37.33 | |
| lower–middle | 44 > T ≥ 32 | 43 > X ≥ 35 | 37.33 > PR ≥ 4.00 | |
| poor | 32 > T | 35 > X | 4.00 > PR |
| Performance | Improvement Strategy |
|---|---|
| Dimension: Mathematical Cognitive Strategy (MCS) | |
| Sub-dimension: Retelling strategy (RS) | |
| Middle-level student : There is still room for improvement in learning through review, practice, etc. Low-level student : There are deficiencies in learning mathematics through review and practice. | 1. Review at the right time, review in time, and review often Ebbinghaus’ law of forgetting points outlines that forgetting begins immediately after learning, and the forgetting speed is fast at first, and then gradually decreases over time. To achieve the best memory effect, the review should be carried out as soon as possible after the study, and the review content and time should be reasonably arranged to improve review efficiency. The content of daily review should be appropriate, so as not to be too tense or too fatiguing and so as not to affect the review effect. 2. Participate in memory with multiple senses The simultaneous participation of eyes, ears, hands, and mouth in the process of memorization can effectively improve the efficiency of memory. For example, listening while watching, talking while writing, listening while doing, and thinking while doing after-school examples and exercises. 3. Diversify the forms of practice to prevent negative emotions such as boredom due to monotonous practice forms. Diversified forms of practice are conducive to the better consolidation of knowledge, enhancing learning effects, and do not easily lead to boredom. For example, when reviewing mathematical concepts, formulas, theorems and algorithms, you can read them aloud, copy them, write them down, use them to solve examples in books and exercises after class, and ask and answer each other among classmates in various ways. |
| Sub-dimension: Finishing strategy (FS) | |
| Middle-level students : 1. There is room for improvement in analyzing and processing learning materials in depth and detail and in understanding the deeper meaning inherent in them. 2. There is still room for improvement in deepening mathematical understanding by establishing connections between old and new knowledge. Low-level students : 1. There are deficiencies in analyzing and processing learning materials in depth and detail and in understanding the deeper meanings inherent in them. 2. There are deficiencies in deepening mathematical understanding by making connections between old and new knowledge. | 1. Use mnemonics to improve the effectiveness of memorization In the process of learning mathematics, use the mnemonics taught by the teacher to assist in the memorization of knowledge. For example, in trigonometric functions, you can use the phrase “odd change and even change unchanged, symbols look at the quadrant” to aid memory. 2. Develop the habit of taking mathematical notes to promote understanding of knowledge By taking notes, you can establish connections between old and new knowledge and promote understanding of knowledge. When taking notes, keep the following in mind: (1) Grasp the key points and make comments during the lecture; (2) Use mind maps, circle annotations, etc., to enrich the content of notes; (3) Add examples and classic question types under the properties of the corresponding theorems; (4) Read the notes at any time, and constantly revise and improve the notes. 3. Try to explain the mathematical concepts and other knowledge in your language to deepen the understanding and memory of knowledge. After a series of studies such as previews and lectures, you will have a certain understanding of the mathematical knowledge you have learned. You can further try to express the results of your understanding of knowledge and the process of thinking in your language. This is better than mere memorization. For example, after learning about “sets”, use your language to describe the definition and related properties of sets. |
| Sub-dimension: Organizing strategy (OS) | |
| Middle-level students : 1. There is still room for improvement in integrating the internal connection between old and new knowledge; 2. There is still room for improvement in refining the knowledge learned and constructing it to form a new knowledge structure. Low-level students : 1. There are deficiencies in integrating the internal connection between old and new knowledge; 2. There are deficienciesin refining the knowledge learned and constructing it to form a new knowledge structure. | 1. Regularly summarize the knowledge points and framework of a module or unit by using outlines, tables, graphics, etc. Present the learned mathematical concepts, principles, formulas and other knowledge in the form of diagrams or outlines, which not only helps to understand the connection between various knowledge points, but also facilitates memory and application. It can be presented as follows: (1) Outlines Write out the main points of knowledge. For example, since the vector is something that students have not been exposed to, and is highly abstract, the knowledge points are relatively fragmented. Therefore, you need to list the key knowledge in the textbook in the form of an outline to promote understanding and memory. (2) Tables In the general review, the form of tables can be used more often to compare the knowledge. For example, when learning the equation of a line, because their equation forms are easily confused, you can organize them with the help of tables. (3) Graphs Explain the connection between knowledge with the help of graphs. For example, a flow chart can be used to summarize the steps of solving a problem, which helps to improve the overall grasp of knowledge and the ability to solve problems. For example, when performing three-dimensional geometry, first build the system, then write the coordinates and vectors, and finally bring the formula. 2. Categorize and organize the questions you have formulated, integrate the solutions and refine them You can prepare a notebook to organize the questions you have asked, and annotate the knowledge points involved with eye-catching colors or marks next to the questions. Sort out and integrate the examination points and solutions of the same type of questions, and refine the methods of solving the questions so that you can apply them flexibly and learn from them. 3. Regularly classify and organize what you have learned Regularly classify the knowledge you have learned according to the logical relationship, and organize it according to your thinking habits, so that you can comprehensively understand all the contents of the knowledge you have learned, and also facilitate the extraction of knowledge. For example, after learning “point, line and surface”, you can organize the three parts by combining them in pairs. |
| Sub-dimension: Feedbacking strategy (FS) | |
| Middle-level students : It is easier for them to adjust their attitude and methods of mathematics learning through the external assessment and diagnosis of their mathematics learning situation; Low-level students : It is difficult for them to adjust their mathematics learning attitude and methods through the external assessment and diagnosis of their mathematics learning situation. | 1. Make timely use of the teacher’s feedback in the classroom Pay attention to the teacher’s feedback, such as the teacher’s comments and follow-up questions given to your own or other students’ answers; an affirmative smile and gesture from the teacher. In this way, you can obtain encouragement or find out the shortcomings of your own or your classmates’ thinking, and then gain experience in mathematical learning activities, so that your mathematical ability can be further developed at the original level and enhance your self-confidence in mathematics learning. 2. Use timely feedback from homework and test papers to analyze and adjust study attitudes and methods independently By analyzing the feedback of the homework and test papers, you can effectively understand your learning situation, find deficiencies and adjust your learning attitude and methods in time, to improve the current situation of learning and improve learning efficiency. For example, the teacher’s judgment on the correctness of your homework, the marking at a certain place, or the comments. Think about and correct your mistakes promptly, and then reflect on how you master the mathematical knowledge content covered by the homework, and whether your attitude towards mathematics learning in the recent period is correct and whether the method of mathematics learning is appropriate. 3. Actively test your mastery of knowledge through exercises By reflecting on the exercises, you can effectively understand your mastery of knowledge. For example, if you make a mistake in an exercise, you should revise it in time and think about what type of question is being examined. Which aspect of knowledge is being examined? Why did I make a mistake? At the same time, reflect on the process of solving the problem and the method of solving it, and summarize your experience to improve your knowledge and learning ability. |
| Dimension: Mathematical metacognitive strategy (MMS) | |
| Sub-dimension: Planning strategy (PS) | |
| Middle-level students : 1. There is room for improvement in planning the various activities involved in completing the task, predicting the results, selecting strategies, envisioning ways to solve problems, and estimating their effectiveness before the learning activity begins; 2. There is room for improvement in clarifying activity goals before learning activities begin. Low-level students : 1. There are deficiencies in planning the various activities involved in completing the task, predicting the results, selecting strategies, envisioning ways to solve the problem, and predicting its effectiveness before the learning activity begins; 2. There are deficiencies in clarifying the objectives of the learning activities before they begin. | 1. Set reasonable and specific short-term and long-term goals for mathematics learning based on your current mathematics learning status (situations) Mathematics learning goals play a role in guiding, motivating and regulating the mathematics learning process. By breaking down long-term goals into short-term goals, you can overcome the difficulties and challenges you encounter in mathematics learning by accomplishing one short-term goal after another, and eventually reaching your long-term goals. For example, if you set a goal for yourself at the beginning of the semester “to achieve a good grade in mathematics at the end of the semester”, you can break this long-term goal down into several short-term goals, such as paying attention and listening carefully in math class every day, completing homework assignments in a timely and quality manner, and reading the textbook or looking up your notes in time when it comes to mathematical knowledge you have forgotten or not mastered. 2. Formulate a clear mathematics learning plan, according to the mathematics learning objectives A mathematics learning plan can promote and regulate mathematics learning, make mathematics learning more organized, and thus improve the efficiency of mathematics learning. Make a clear plan for each stage of mathematics learning according to your specific situation. For example, make a semester plan for mathematics learning at the beginning of the semester; make a daily plan for mathematics learning after school. In general, you can review first and then answer the questions to consolidate the mathematical knowledge learned that day, master the basic questions covered by the content, and then preview the mathematical knowledge to be learned in the next class, and clarify the key points, difficulties, doubts, and confusion points. Finally, summarize and evaluate the completion of the plan promptly is completed well, you can give yourself a small reward to satisfy the sense of achievement; if the plan is poorly completed, you can give yourself a certain small punishment. 3. Arrange the test time reasonably during the math test When you receive the papers for the exam, you should have an overview of the papers, including the type of questions and the number of questions, and arrange the order and time of the questions reasonably. When encountering a problem that cannot be solved, learn to temporarily give up or use other methods to help solve it. After answering all the questions that can be answered in the test paper, think about the questions that cannot be answered during the inspection process that are guaranteed to obtain all the points. Make sure that you receive all the marks you can. |
| Sub-dimension: Monitoring strategy (MS) | |
| Middle-level students : 1. There is room for improvement in testing the learning process promptly and the learning objectives. 2. There is room for improvement in finding the gaps between learning objectives or plans and current learning activities to successfully achieve effective mathematical learning. Low-level students : 1. There are deficiencies in testing the learning process according to the learning objectives; 2. There are deficiencies in finding the gaps between learning objectives or plans and current learning activities to successfully achieve effective mathematical learning. | 1. When solving mathematical problems, compare the results obtained with life experience and learning experience Abstracting mathematical knowledge in real life can arouse students’ enthusiasm for learning and deepen students’ understanding of knowledge. For example, when learning plane geometry, you can think about the positional relationship between two straight lines, the positional relationship between lines and surfaces, and the positional relationship between surfaces and surfaces by looking for examples in life, such as corners, buildings, etc. 2. Develop the habit of checking results After completing the homework or test paper, you need to check whether the solution result is correct. You can solve the problem with different ideas to see if you can obtain the same answer, bring the answer into the question for verification, or recalculate the question. When recalculating, pay attention to overcoming the influence of inertial thinking, which means rereading the question, trying not to be disturbed by the thinking of the first time you did the problem. 3. Narrate problem-solving ideas by “thinking aloud” “Thinking aloud” is to express the whole process of thinking in your language and make it “externalized”. For example, in the process of solving the problem, identify the theorems and conditions on which your problem-solving is based; state the goal of the problem and the plan to achieve the goal; evaluate the advantages and disadvantages of your problem-solving thinking process; discover and describe the different ideas that may appear in the problem-solving process. These are all conducive to actively mobilizing the role of self-monitoring in problem-solving by improving the accuracy of problem-solving and improving the level of mathematical thinking. |
| Sub-dimension: Reflection and adjustment strategy (RAAS) | |
| Middle-level students : 1. There is still room for improvement in identifying deviations in the mathematics learning process based on the results of monitoring learning activities, and adjust learning strategies or revise learning goals in a timely manner. 2. There is still room for improvement in evaluating the learning results at the end of the mathematics learning activities, correct your mathematics learning behaviors, and remedy deficiencies. Low-level students : 1. There are deficiencies in identifying deviations in the mathematics learning process based on the results of monitoring learning activities, and adjust learning strategies or revise learning goals. 2. There are deficiencies in evaluating the learning results at the end of the mathematics learning activities, correct your mathematics learning behaviors, and remedy deficiencies. | 1. Always reflect on your learning attitude and state You should always reflect on whether you can pay attention in class, whether you can control your behavior effectively when studying, whether you have the confidence to overcome learning difficulties, and what methods should be used to solve them in the future if there are deficiencies. Try to propose corresponding goals, summarize promptly and reflect on your progress and deficiencies. 2. Correct attribution of learning results Mathematics performance is affected by many factors, so you need to understand it correctly and rationally. From a subjective point of view, your efforts are strongly associated with learning results. In general, attributing learning results to subjective factors will increase your motivation to learn and, when learning results are poor, you will not be less inclined to study harder because of it. From an objective point of view, academic performance is also associated with the difficulty of test questions, luck, physical and mental state, and external environment, so attributing learning results to objective factors can help you to relieve anxiety to some extent. 3. Deepen thinking and understanding of learning through self-questioning You will have deep thinking and understanding of mathematical problems by constantly asking yourself questions, thus improving your mathematical learning ability and learning effectiveness. You should often ask yourself “did I solve this question this way?” “Could it be easier to do it differently?” “Why did I do it wrong?” |
| Dimension: Mathematical resource-management strategy (MRMS) | |
| Sub-dimension: Time-management strategy (RMS) | |
| Middle-level students : 1. There is still room for improvement in the overall arrangement of time; 2. There is still room for improvement in the efficient use of optimal mathematics learning time; 3. There is still room for improvement in the flexible use of fragmented time to learn mathematics. Low-level students : 1. There are deficiencies in the overall arrangement of time; 2. There are deficiencies in the efficient use of optimal mathematics learning time; 3. There are deficiencies in the flexible use of fragmented time to learn mathematics. | 1. List the things that need to be performed and classify them List the things that need to be performed on a list of activities, sort and classify them according to priority: the important and urgent things, the important and not urgent things, and the unimportant and not urgent things. Prioritize the important and urgent tasks, and then perform the important and non-urgent tasks, and ask your family and classmates to help you with the unimportant and urgent tasks. The unimportant and non-urgent tasks will not take much time. 2. Make the most of your prime study time and study the most important content when you are at your best The same hour of work can make a significant difference in learning efficiency depending on the mental state of the individual. The key to effective learning is to use the most efficient study time wisely and to make good use of the golden hours of the day, such as in the morning and before going to bed. In addition to receiving enough rest, it is also important to enjoy a good amount of physical exercise to maintain the best study condition. In addition, whenever you feel tired, you should stop for a short break, drink a glass of water or take a walk, and then concentrate on your studies. 3. Strictly set the time to finish homework to avoid procrastination Before doing homework each time, set the time required to complete the homework according to the amount of homework and your actual situation, and then complete the homework on time within the specified time, which will help to cultivate your time concept. For example, you can make a detailed homework schedule for yourself. The content of the schedule includes what homework to write in a certain period and how much to write, and then completing the homework on time within the time period specified in the homework plan. |
| Sub-dimension: Environmental-management strategy (EMS) | |
| Middle-level students : 1. There is room for improvement in attributing mathematics learning outcomes correctly. 2. There is still room for improvement in adjusting the bad mood of mathematics learning. 3. There is still room for improvement in promoting mathematics learning through perseverance and self-reinforcement. Low-level students : 1. There are deficiencies in attributing mathematics learning outcomes correctly. 2. There are deficiencies in adjusting the bad mood of mathematics learning; 3. There are deficiencies in promoting mathematics learning by perseverance and self-reinforcing. | 1. Cultivate a positive mindset in learning mathematics through self-encouragement Encouraging yourself frequently can help to improve self-confidence, reverse the bad mindset of mathematics learning, give full play to your inner potential, and thus improve the self-awareness of learning. In the process of learning mathematics, you should always encourage yourself with affirmative and motivational words and phrases, including positive psychological suggestions such as telling yourself “I am capable of learning mathematics well”. 2. Correctly face the bad emotions in mathematics learning When bad emotions arise in the process of mathematics learning, you can divert your attention by taking a short break, taking a deep breath, or doing some other healthy activities that you are interested in. In addition, you should also appreciate the mathematical principles in your daily life by heart, appreciate the beauty and value of mathematics, cultivate and stimulate good motivation to learn mathematics, and actively regulate and rid yourself of bad emotions. 3. Develop good willpower such as self-control and persistence Learning mathematics is a long-term, complex mental task. Perseverance and independence are essential qualities for successful mathematics learning activities. We can sharpen our will in the process of solving difficulties, for example, by doing some difficult problems to cultivate our perseverance and not giving up easily in the face of difficulties; we can also use our spare time to read mathematics extracurricular books and learn the rigorous scientific attitude and hard work of mathematical scientists. |
| Sub-dimension: Mood-management strategy (MMS) | |
| Middle-level students : 1. There is still room for improvement in finding favorable conditions to assist mathematics learning; 2. There is still room for improvement in storing orderly mathematical materials, planning the learning space, and creating a mathematical learning atmosphere. Low-level students : 1. There are deficiencies in finding favorable conditions to assist mathematics learning; 2. There are deficiencies in the orderly storage of mathematical materials, the planning of learning space, and the creation of a mathematical learning atmosphere. | 1. Create an ideal learning environment An ideal learning environment can facilitate your efficient learning, on the one hand, and cultivate your good study habits on the other hand. For example, pay attention to the orderly arrangement of desks, chairs and books; regularly organize mathematics study materials such as mathematics papers, mathematics study cases, and mathematics homework books after finishing the mathematics study of a certain module or a certain unit. 2. Establish a good teacher–student relationship and create a harmonious and pleasant atmosphere in the mathematics classroom A good teacher–student relationship helps to create a harmonious and pleasant atmosphere in mathematics classrooms, and can encourage you to learn mathematics happily in mathematics classrooms. Therefore, you must learn to respect and appreciate teachers, form a harmonious teacher–student relationship with teachers, and actively interact with them. 3. Choose an appropriate math learning environment A quiet and comfortable learning environment is conducive to your mathematics learning and thinking. On the contrary, a noisy environment will make you restless and inattentive, which is not conducive to normal study and life. Therefore, you should choose to study mathematics in a quiet environment with suitable light and temperature. |
| Sub-dimension: External help strategy (EHS) | |
| Middle-level students : 1. There is still room for improvement in seeking help from others to overcome difficulties or solve problems when encountering difficulties in mathematics learning, to improve learning efficiency and achieve expected learning goals. 2. There is still room for improvement in choosing an appropriate time for help and seeking targeted help, when encountering difficulties or problems in mathematics learning. Low-level students : 1. There are deficiencies in seeking help from others to overcome difficulties or solve problems when encountering difficulties in mathematics learning. 2. There are deficiencies in choosing an appropriate time for help and seeking targeted help. | 1. Correct your attitude towards helping A good attitude will make your inquiry the best. Before asking for help, be clear about the questions you want to ask and find out what you do not understand. For example, read the topic first, understand the conditions in the topic and the questions asked, try to find ideas, and then seek help from others after no results. 2. Choose the right person for help according to different problems Anyone who helps to solve the problem can become the object of academic help. Therefore, you should choose different help objects according to your own needs, such as classmates who you get along with well, teachers who know how to solve problems and can give you advice and guidance promptly, parents, books, network resources, etc. 3. Identify the problem and choose the right time to ask for help Before seeking help from others, it is important to think about the problem, rather than asking for help immediately without thinking. Additionally, consider the time and emotional state of others, and do not ask for help when they are not available. 4. Choose the appropriate way to communicate effectively with the object of assistance, so as to seek targeted help Effective communication with the person you are seeking help from is the key to attaining help effectively in the process of seeking help to solve the problem. You can choose the appropriate communication channel according to your actual situation, such as face-to-face, by telephone, online and so on. In the process of asking for help, you should express your problems in an orderly manner, so that the person you are asking for help can understand your problems and provide accurate help. |
| N | Min | Max | Mean (M) | Standard Deviation | Dimension Full Score | Score Rating (S) | |
|---|---|---|---|---|---|---|---|
| Mathematics learning strategy | 195 | 113.0 | 241.00 | 166.78 | 25.38 | 245 | 68.07% |
| MCS | 195 | 42.00 | 97.00 | 67.66 | 10.61 | 100 | 67.66% |
| MMS | 195 | 32.00 | 74.00 | 51.90 | 8.41 | 75 | 69.20% |
| MRMS | 195 | 27.00 | 73.00 | 47.23 | 7.87 | 70 | 67.47% |
| Dimensions | N | Min | Max | Mean (M) | Standard Deviation | Dimension Full Score | Score Rating (S) |
|---|---|---|---|---|---|---|---|
| Finishing Strategy | 195 | 8 | 25 | 17.21 | 3.23 | 25 | 68.84% |
| RS | 195 | 10 | 25 | 17.06 | 3.12 | 25 | 68.26% |
| OS | 195 | 9 | 25 | 16.67 | 3.29 | 25 | 66.69% |
| Feedback Strategy | 195 | 10 | 25 | 16.71 | 2.78 | 25 | 66.85% |
| MS | 195 | 9 | 25 | 17.78 | 3.17 | 25 | 71.14% |
| PS | 195 | 10 | 25 | 16.89 | 3.09 | 25 | 67.57% |
| RAAS | 195 | 9 | 25 | 17.22 | 3.07 | 25 | 68.88% |
| TMS | 195 | 4 | 15 | 10.05 | 3.01 | 15 | 66.97% |
| EHS | 195 | 5 | 15 | 10.24 | 2.12 | 15 | 68.24% |
| MMS | 195 | 7 | 20 | 13.80 | 2.62 | 20 | 69.00% |
| EMS | 195 | 7 | 20 | 13.14 | 2.51 | 20 | 65.72% |
| Level | T-score | Raw Score (X) | Percentage Rating (PR) | |
|---|---|---|---|---|
| MLS | Top | T ≥ 68 | X ≥ 213 | PR ≥ 96.49% |
| Above Average | 56 ≤ T < 68 | 176 ≤ X < 213 | 73.78% ≤ PR < 96.49% | |
| Average | 44 ≤ T < 56 | 148 ≤ X < 176 | 27.39% ≤ PR < 73.78% | |
| Below Average | 32 ≤ T < 44 | 125 ≤ X < 148 | 3.61% ≤ PR < 27.39% | |
| Low | T < 32 | X < 125 | PR < 3.61% | |
| MCS | Top | T ≥ 68 | X ≥ 88 | PR ≥ 96.49% |
| Above Average | 56 ≤ T < 68 | 73 ≤ X < 88 | 74.46% ≤ PR < 96.49% | |
| Average | 44 ≤ T < 56 | 61 ≤ X < 73 | 29.43% ≤ PR < 74.46% | |
| Below Average | 32 ≤ T < 44 | 50 ≤ X < 61 | 3.61% ≤ PR < 29.43% | |
| Low | T < 32 | X < 50 | PR < 3.61% | |
| MMS | Top | T ≥ 68 | X ≥ 67 | PR ≥ 96.49% |
| Above Average | 56 ≤ T < 68 | 61 ≤ X < 67 | 75.05% ≤ PR < 96.49% | |
| Average | 44 ≤ T < 56 | 56 ≤ X < 61 | 33.53% ≤ PR < 75.05% | |
| Below Average | 32 ≤ T < 44 | 39 ≤ X < 56 | 4.68% ≤ PR < 33.53% | |
| Low | T < 32 | X < 39 | PR < 4.68% | |
| MRMS | Top | T ≥ 68 | X ≥ 59 | PR ≥ 96.59% |
| Above Average | 56 ≤ T < 68 | 50 ≤ X < 59 | 76.51% ≤ PR < 96.59% | |
| Average | 44 ≤ T < 56 | 43 ≤ X < 50 | 37.33% ≤ PR < 76.51% | |
| Below Average | 32 ≤ T < 44 | 35 ≤ X < 43 | 4.00% ≤ PR < 37.33% | |
| Low | T < 32 | X < 35 | PR < 4.00% |
| Student | Raw Score | Norm Level | Scoring Rate (S) |
|---|---|---|---|
| A | 116 | Low | 47.35% |
| B | 119 | Low | 48.57% |
| C | 131 | below average | 53.47% |
| Sub-Dimensions | Student | Raw Score | Norm Level | Scoring Rate (S) |
|---|---|---|---|---|
| MCS | A | 41 | Low (X < 50) | 41.00% |
| B | 49 | Low (X < 50) | 49.00% | |
| C | 57 | Below Average (50 ≤ X < 61) | 57.00% | |
| MMS | A | 39 | Below Average (39 ≤ X < 56) | 52.00% |
| B | 30 | Low ) | 40.00% | |
| C | 47 | Below Average (39 ≤ X < 56) | 62.67% | |
| MRMS | A | 36 | Below Average (35 ≤ X < 43) | 51.43% |
| B | 40 | Below Average (35 ≤ X < 43) | 57.14% | |
| C | 27 | Low (X < 35) | 38.57% |
| Student | Score Before Intervention | Score After Intervention | |
|---|---|---|---|
| MLS | A | 116 | 156 |
| MCS | 41 | 63 |
| A’s Math Score | A’s Class Rank | |||
|---|---|---|---|---|
| Before Intervention | After Intervention | Before Intervention | After Intervention | |
| monthly test scores | 80 | 92 | 40 | 32 |
| midterm exam results | 72 | 90 | 40 | 25 |
| final exam results | 69 | 91 | 40 | 27 |
| Student | Score Before Intervention | Score After Intervention | |
|---|---|---|---|
| MLS | B | 119 | 154 |
| MMS | 30 | 48 |
| B’s Math Score | B’s Class Rank | |||
|---|---|---|---|---|
| Before Intervention | After Intervention | Before Intervention | After Intervention | |
| monthly test scores | 85 | 95 | 35 | 29 |
| midterm exam results | 81 | 92 | 35 | 24 |
| final exam results | 79 | 93 | 36 | 24 |
| Student | Score Before Intervention | Score After Intervention | |
|---|---|---|---|
| MLS | C | 131 | 152 |
| MRMS | 27 | 44 |
| C’s Math Score | C’s Class Rank | |||
|---|---|---|---|---|
| Before Intervention | After Intervention | Before Intervention | After Intervention | |
| monthly test scores | 91 | 98 | 33 | 26 |
| midterm exam results | 87 | 93 | 32 | 23 |
| final exam results | 85 | 97 | 34 | 21 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, G.; Chen, X.; Zhang, D.; Kang, Y.; Wang, F.; Su, M. Development and Application of an Intelligent Assessment System for Mathematics Learning Strategy among High School Students—Take Jianzha County as an Example. Sustainability 2022, 14, 12265. https://doi.org/10.3390/su141912265
Wang G, Chen X, Zhang D, Kang Y, Wang F, Su M. Development and Application of an Intelligent Assessment System for Mathematics Learning Strategy among High School Students—Take Jianzha County as an Example. Sustainability. 2022; 14(19):12265. https://doi.org/10.3390/su141912265
Chicago/Turabian StyleWang, Guangming, Xia Chen, Dongli Zhang, Yueyuan Kang, Fang Wang, and Mingyu Su. 2022. "Development and Application of an Intelligent Assessment System for Mathematics Learning Strategy among High School Students—Take Jianzha County as an Example" Sustainability 14, no. 19: 12265. https://doi.org/10.3390/su141912265
APA StyleWang, G., Chen, X., Zhang, D., Kang, Y., Wang, F., & Su, M. (2022). Development and Application of an Intelligent Assessment System for Mathematics Learning Strategy among High School Students—Take Jianzha County as an Example. Sustainability, 14(19), 12265. https://doi.org/10.3390/su141912265




