Adsorption of Metformin on Activated Carbon Produced from the Water Hyacinth Biowaste Using H3PO4 as a Chemical Activator
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
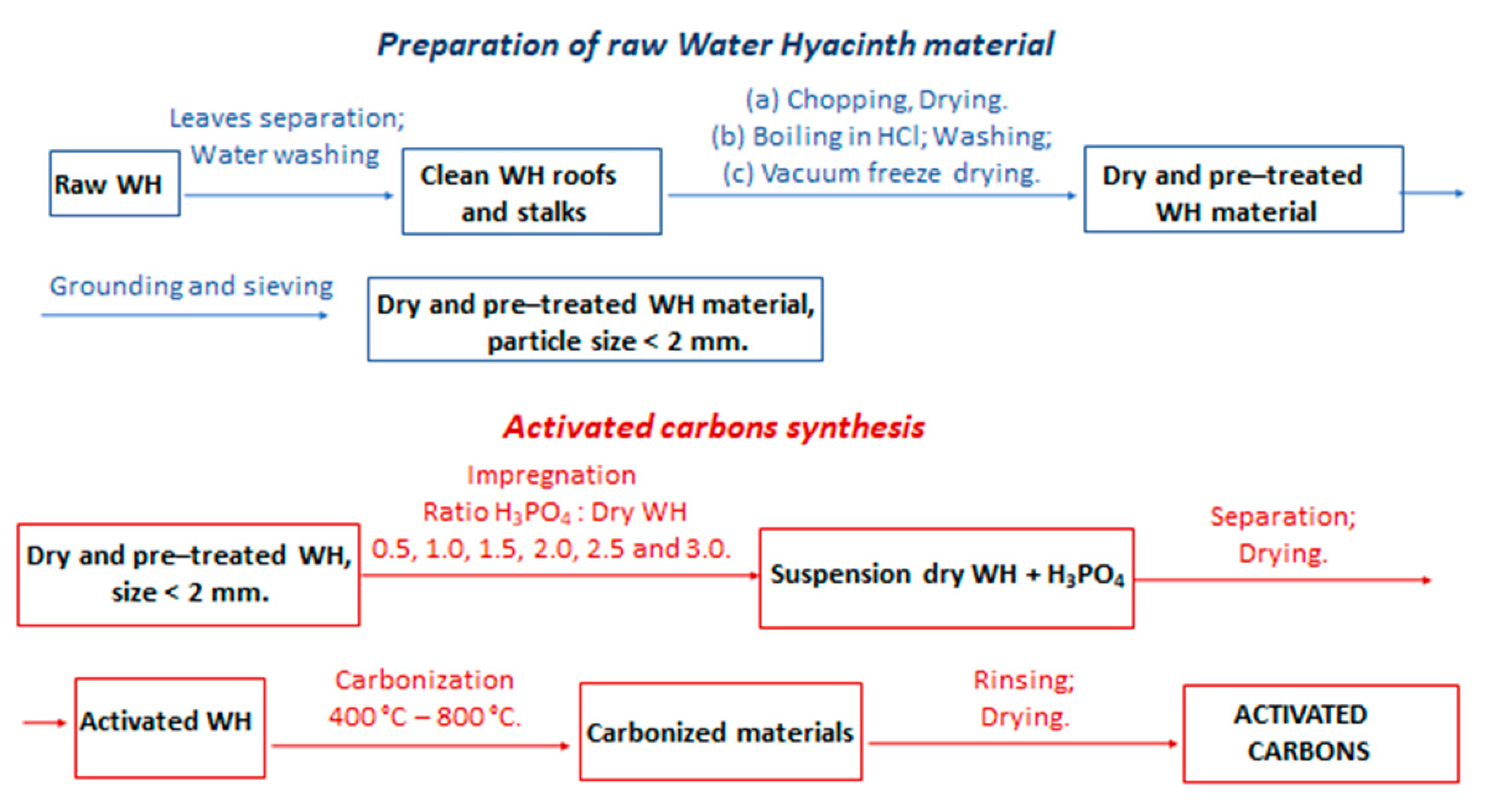
2.2. Preparation of the Activated Carbons
2.3. Methods of Characterization
2.4. Adsorption—Desorption Experiments
3. Results and Discussions
3.1. Results of the Characterization
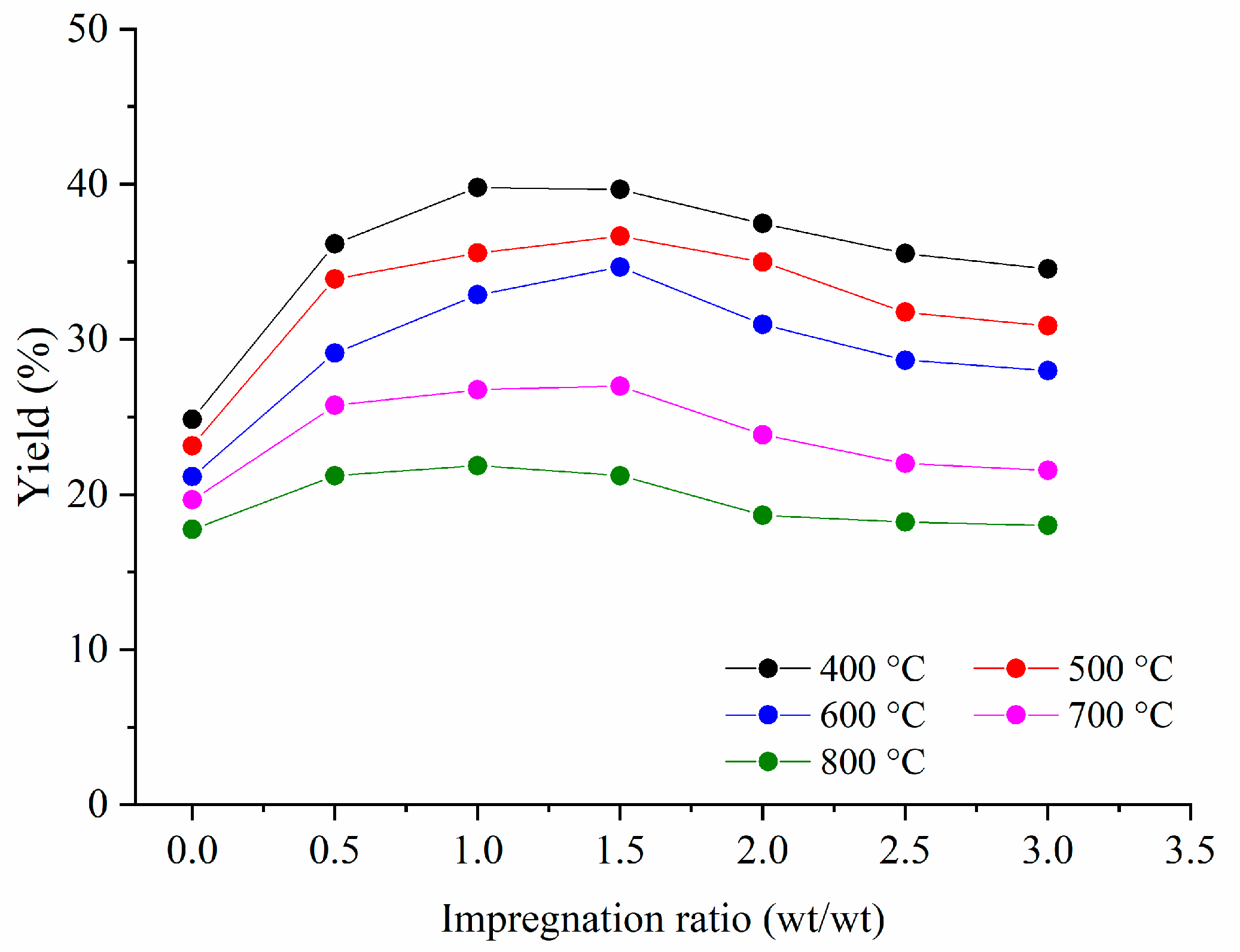
3.1.1. Yield of the Activated Carbons
3.1.2. Effect of the Activation Agent and Carbonization Temperature on Surface Characteristics
3.1.3. Textural Properties
3.1.4. Elemental Analysis
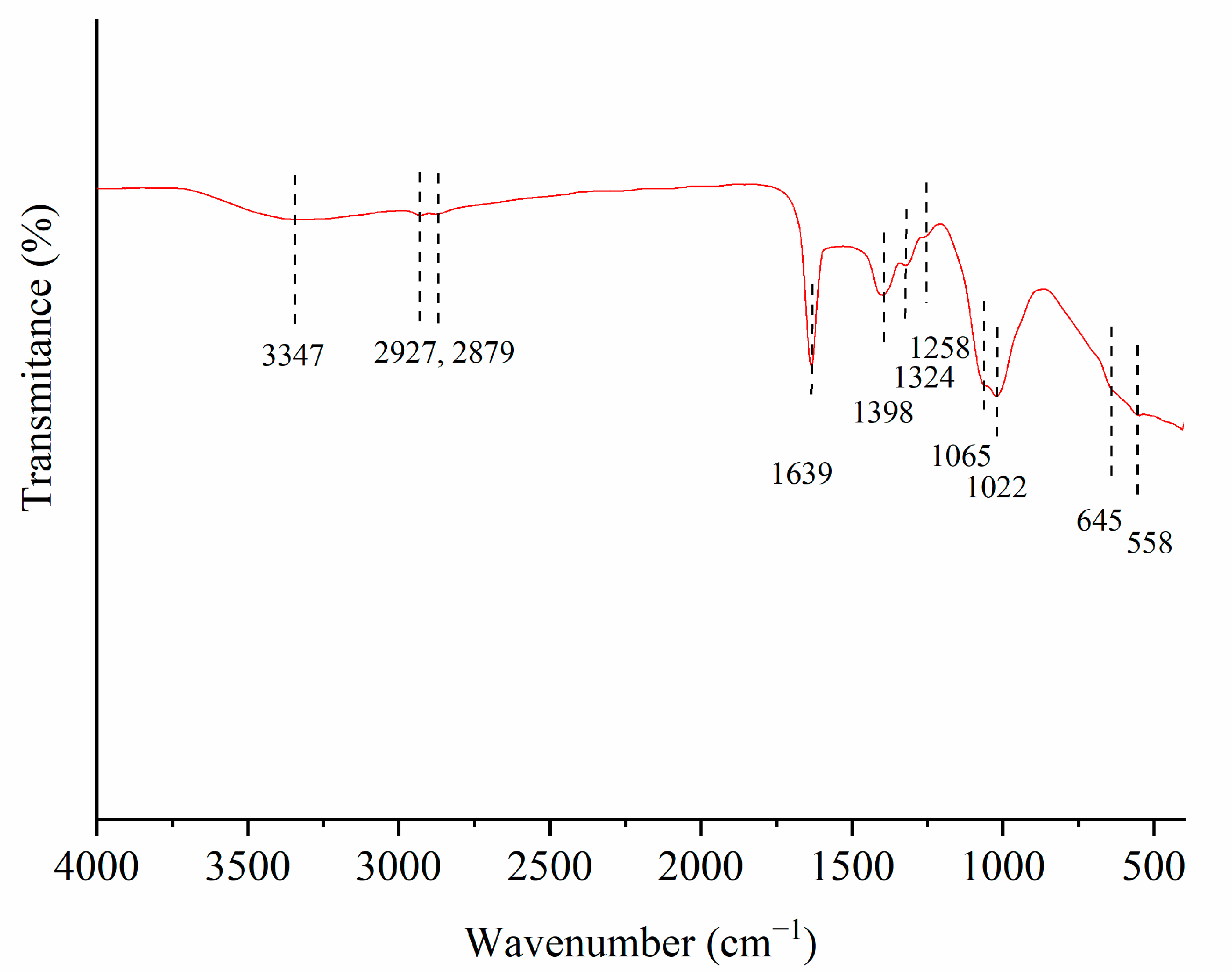
3.1.5. FTIR Analysis
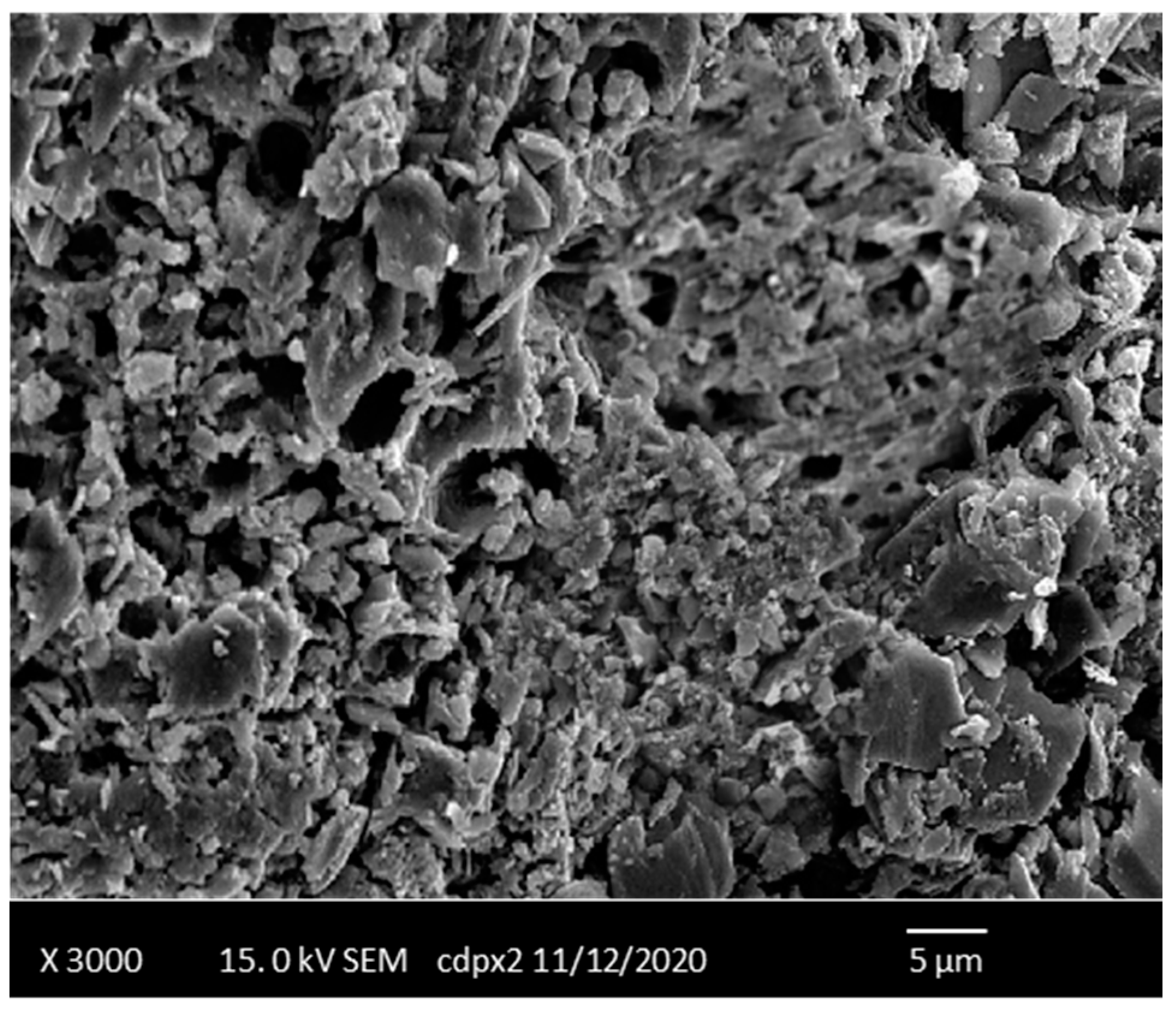
3.1.6. Morphological Analysis
3.2. Adsorption Study
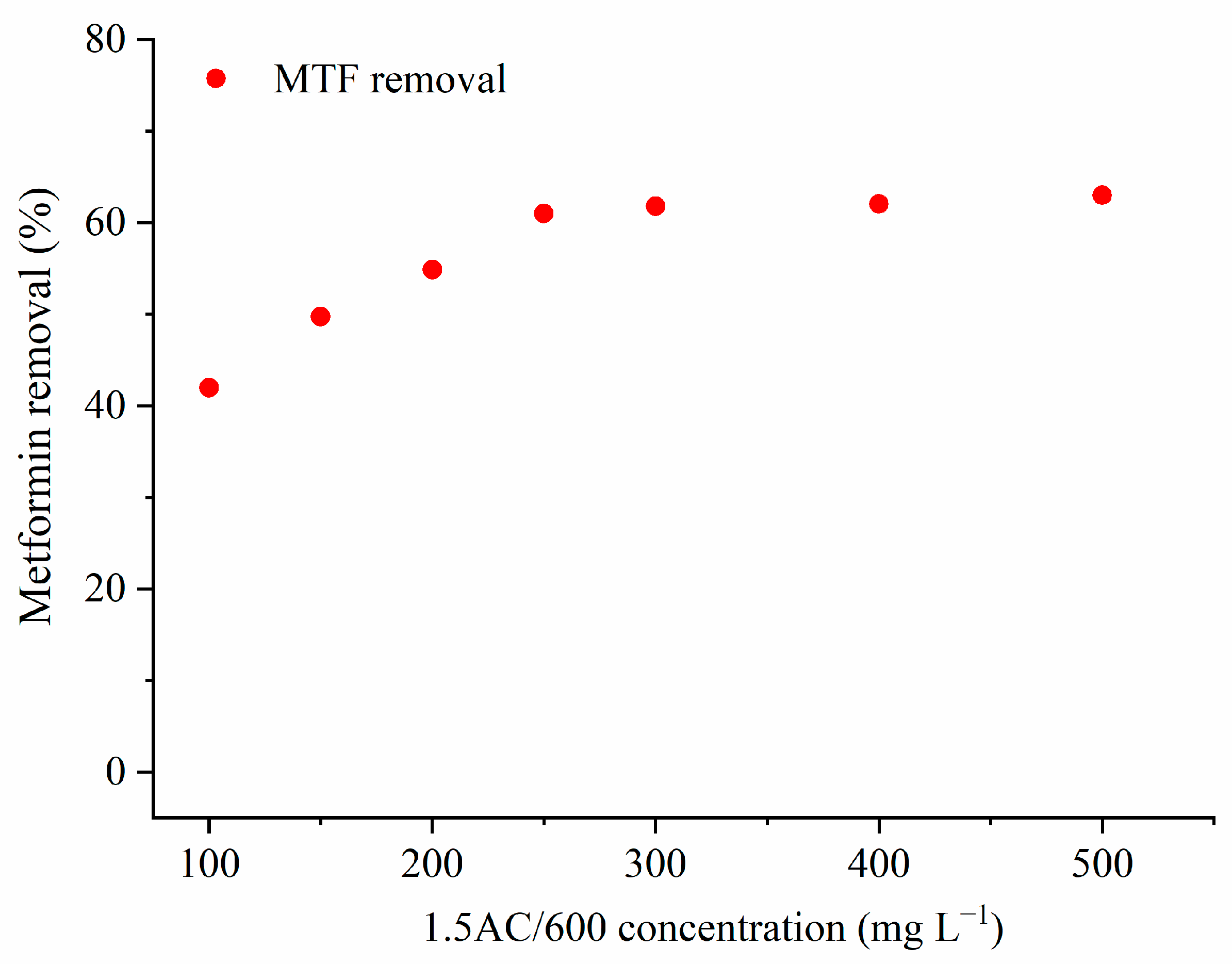
3.2.1. Effect of the Adsorbent Concentration
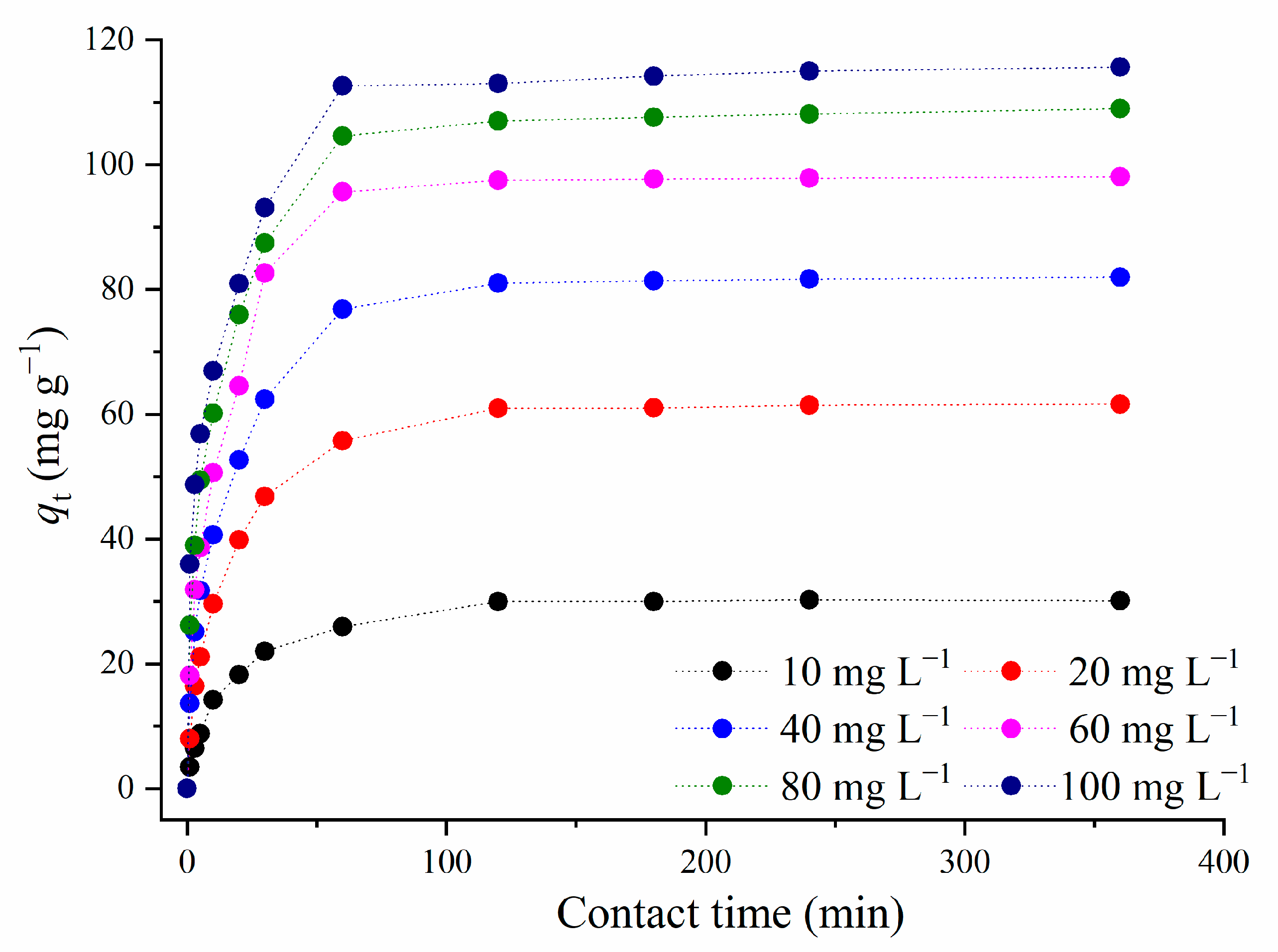
3.2.2. Influence of the Metformin Concentration
3.2.3. Effect of the pH of Metformin Solution
3.3. Modeling of the Experimental Adsorption Data
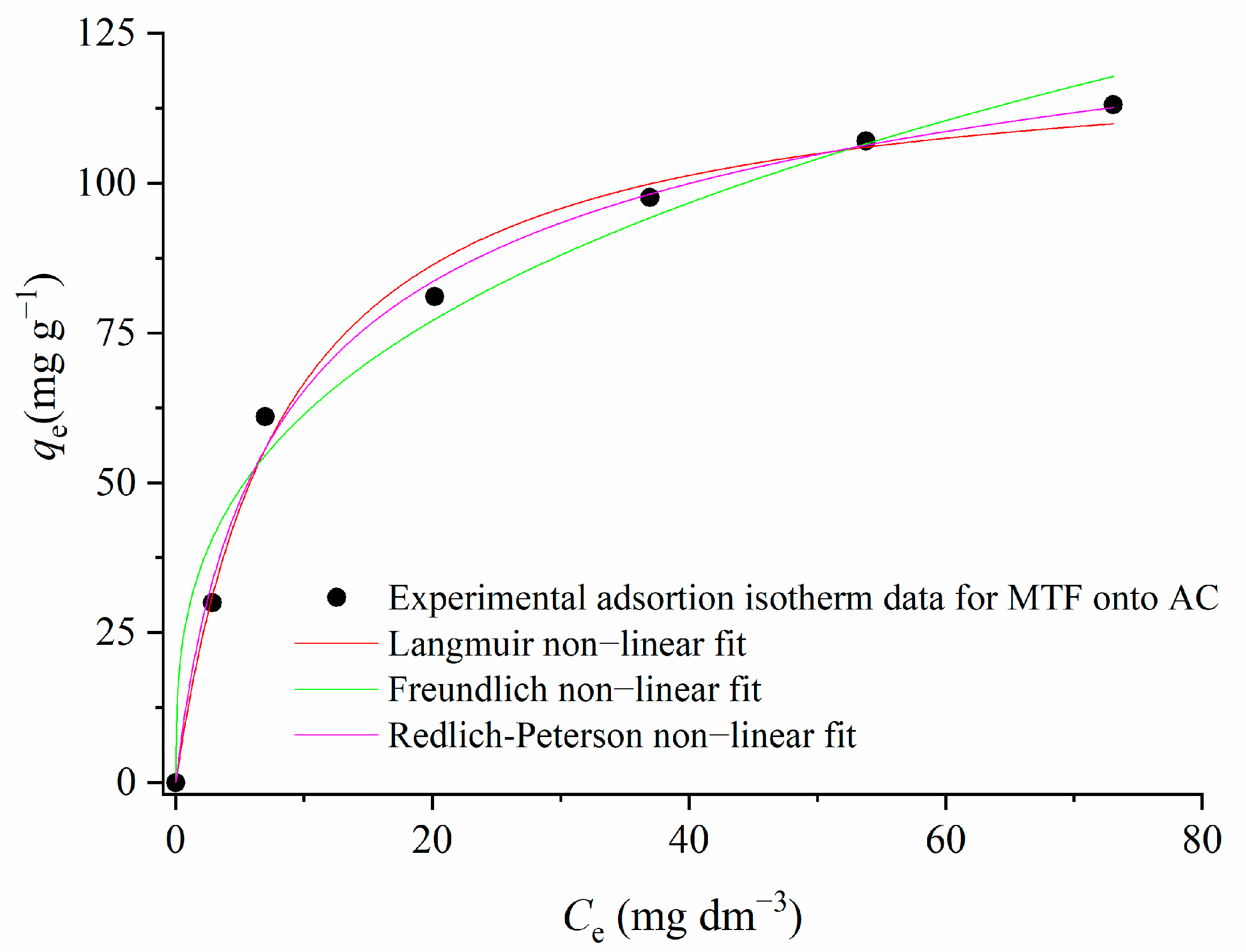
3.3.1. Adsorption Isotherms
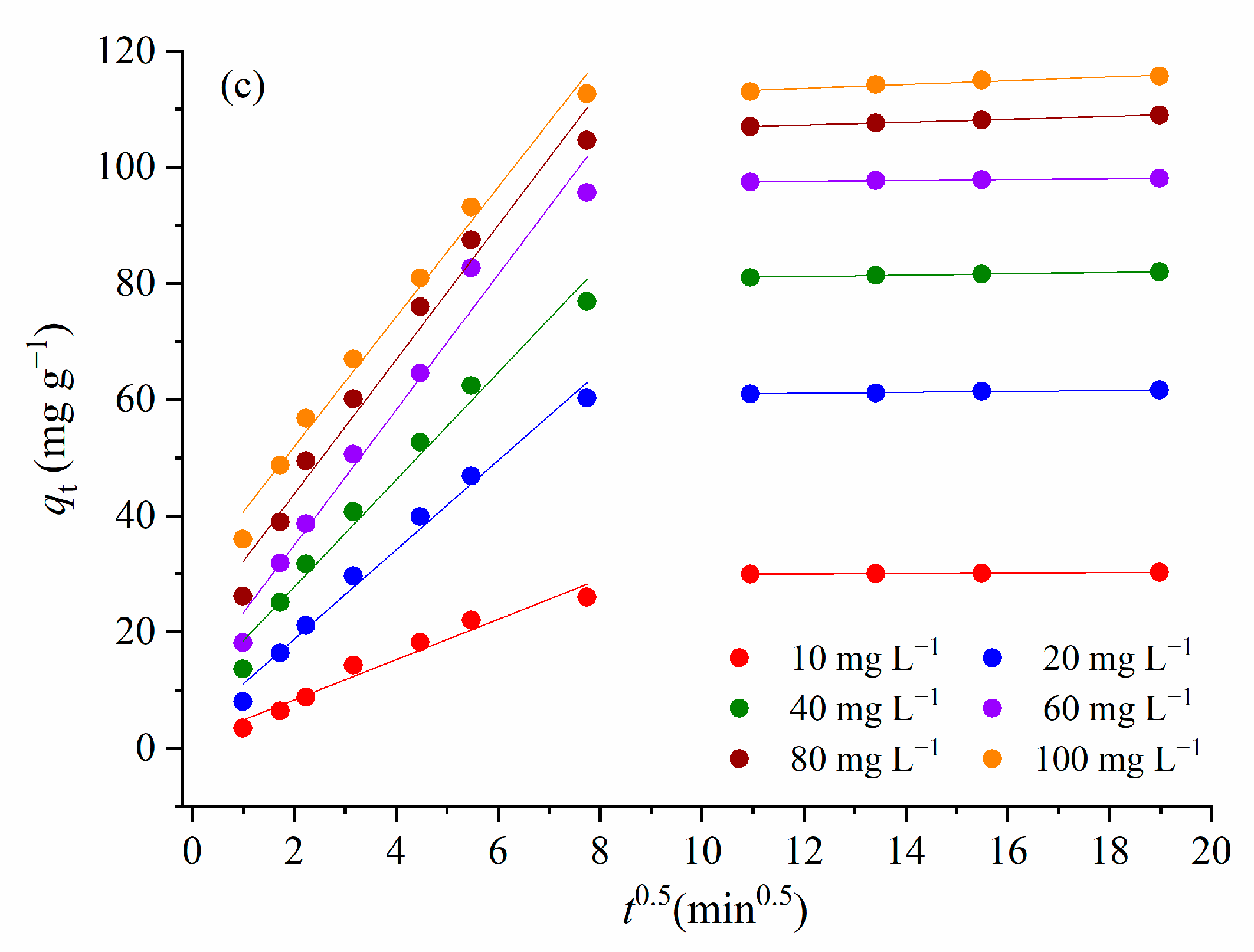
3.3.2. Kinetics of the Adsorption
3.4. Thermodynamics of the Adsorption
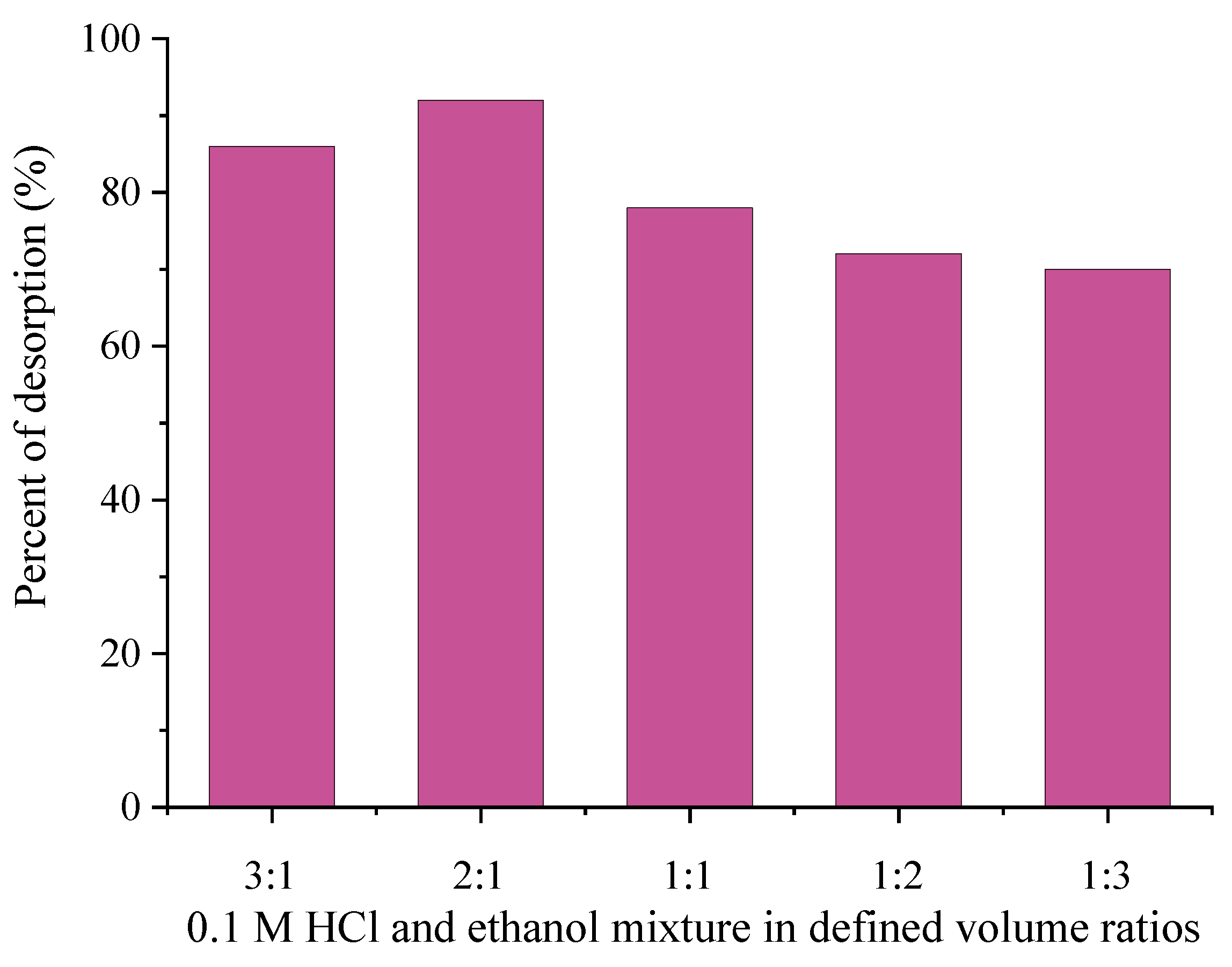
3.5. Desorption and Reusability Study
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhu, S.; Liu, Y.G.; Liu, S.B.; Zeng, G.M.; Jiang, L.H.; Tan, X.F.; Zhou, L.; Zeng, W.; Li, T.T.; Yang, C.P. Adsorption of emerging contaminant metformin using graphene oxide. Chemosphere 2017, 179, 20–28. [Google Scholar] [CrossRef] [PubMed]
- Macías-García, A.; García-Sanz-Calcedo, J.; Carrasco-Amador, J.P.; Segura-Cruz, R. Adsorption of paracetamol in hospital wastewater through activated carbon filters. Sustainability 2019, 11, 2672. [Google Scholar] [CrossRef]
- Piccirillo, G.; Aroso, R.T.; Rodrigues, F.M.S.; Carrilho, R.M.B.; Pinto, S.M.A.; Calvete, M.J.F.; Pereira, M.M. Oxidative degradation of pharmaceuticals: The role of tetrapyrrole-based catalysts. Catalysts 2021, 11, 1335. [Google Scholar] [CrossRef]
- Kovalova, L.; Siegrist, H.; Singer, H.; Wittmer, A.; McArdell, C. Hospital wastewater treatment by membrane bioreactor: Performance and efficiency for organic micropollutant elimination. Environ. Sci. Technol. 2012, 46, 1536–1545. [Google Scholar] [CrossRef] [PubMed]
- Clasen, T.; Menon, S. Microbiological performance of common water treatment devices for household use in India. Int. J. Environ. Health Res. 2007, 17, 83–93. [Google Scholar] [CrossRef] [PubMed]
- Kalderis, D.; Koutoulakis, D.; Paraskeva, P.; Diamadopoulos, E.; Otal, E.; del Valle, J.O.; Fernán-dez-Pereira, C. Adsorption of polluting substances on activated carbons prepared from rice husk and sugarcane bagasse. Chem. Eng. J. 2008, 144, 42–50. [Google Scholar] [CrossRef]
- Ioannidou, O.; Zabaniotou, A. Agricultural residues as precursors for activated carbon production—A Review. Renew. Sust. Energ. Rev. 2007, 11, 1966–2005. [Google Scholar] [CrossRef]
- Mohsen Nourouzi, M.; Chuah, T.G.; Choong, T.S.Y. Adsorption of glyphosate onto activated carbon derivedfrom waste newspaper. Desalination Water Treat. 2010, 24, 321–326. [Google Scholar] [CrossRef]
- Maulina, W.; Kusumaningtyas, R.; Rachmawati, Z.; Supriyadi; Arkundato, A.; Rohman, L.; Purwandari, E. Carbonization process of water hyacinth as an alternative renewable energy material for biomass cook stoves applications. IOP Conf. Ser. Earth Environ. Sci. 2019, 239, 012035. [Google Scholar] [CrossRef]
- Bote, M.A.; Naik, V.R.; Jagadeeshgouda, K.B. Review on water hyacinth weed as a potential bio fuel crop to meetcollective energy needs. Mater. Sci. Energy Technol. 2020, 3, 397–406. [Google Scholar]
- Abdel-Fattah, A.F.; Abdel-Naby, M.A. Pretreatment and enzymic saccharification of water hyacinth cellulose. Carbohydr. Polym. 2012, 87, 2109–2113. [Google Scholar] [CrossRef]
- Njogu, P.; Kinyua, R.; Muthoni, P.; Nemoto, Y. Biogas production using water hyacinth (Eicchornia Crassipes) for electricity generation in Kenya. Energy Power Eng. 2015, 7, 209–216. [Google Scholar] [CrossRef]
- Kumar, A.; Jena, H.M. High surface area microporous activated carbons prepared from Fox nut (Euryale ferox) shell by zinc chloride activation. Appl. Surf. Sci. 2015, 356, 753–761. [Google Scholar] [CrossRef]
- Kumar, A.; Jena, H.M. Preparation and characterization of high surface area activated carbon from Fox nut (Euryale ferox) shell by chemical activation with H3PO4. Results Phys. 2016, 6, 651–658. [Google Scholar] [CrossRef]
- Prahas, D.; Kartika, Y.; Indraswati, N.; Ismadji, S. Activated carbon from jackfruit peel waste by H3PO4 chemical activation: Pore structure and surface chemistry characterization. Chem. Eng. J. 2008, 140, 32–42. [Google Scholar] [CrossRef]
- Hui, T.S.; Zaini, M.A.A. Potassium hydroxide activation of activated carbon: A commentary. Carb. Lett. 2015, 16, 275–280. [Google Scholar] [CrossRef]
- Yorgun, S.; Yıldız, D. Preparation and characterization of activated carbons from Paulownia wood by chemical activation with H3PO4. J. Taiwan Inst. Chem. 2015, 53, 122–131. [Google Scholar] [CrossRef]
- Mohammad, A.H.; Kijevcanin, M. Synthesis of activated carbons from water hyacinth biomass and its application as adsorbents in water pollution control. J. Serb. Chem. Soc. 2022. [Google Scholar] [CrossRef]
- Rouquerol, J.; Llewellyn, P.; Rouquerol, F. Is the BET Equation Applicable to Microporous Adsorbents? Studies in Stud. Surf. Sci. Cat. 2007, 160, 49–56. [Google Scholar]
- Dubinin, M.M. Adsorption in micropores. J. Coll. Interface Sci. 1967, 23, 487–499. [Google Scholar] [CrossRef]
- Barrett, E.P.; Joyner, L.G.; Halenda, P.P. The determination of pore volume and area distributions in porous substances. I. Computations from nitrogen isotherms. J. Am. Chem. Soc. 1951, 73, 373–380. [Google Scholar] [CrossRef]
- Liu, Q.X.; Zhou, Y.R.; Wang, M.; Zhang, Q.; Ji, T. Adsorption of methylene blue from aqueous solution onto viscose-based activated carbon fiber felts: Kinetics and equilibrium studies. Ads. Sci. Technol. 2019, 37, 312–332. [Google Scholar] [CrossRef] [Green Version]
- Rodriguez-Reinoso, F.; Molina-Sabio, M. Role of chemical activation in the development of carbon porosity. Coll. Surf. A Physicochem. Eng. Asp. 2004, 241, 15–25. [Google Scholar]
- Qian, Q.; Machida, M.; Tatsumoto, H. Preparation of activated carbons from cattle-manure compost by zinc chloride activation. Bioresour. Technol. 2007, 98, 353–360. [Google Scholar] [CrossRef]
- Castro, J.B.; Bonelli, P.R.; Cerrella, E.G.; Cukierman, A.L. Phosphoric acid activation of agricultural residues and bagasse from sugar cane: Influence of the experimental conditions on adsorption. Characteristics of Activated Carbons. Ind. Eng. Chem. Res. 2000, 39, 4166–4172. [Google Scholar] [CrossRef]
- Corcho-Corral, B.; Olivares-Marin, M.; Fernandez-Gonzalez, C.; Gomez-Serrano, V.; Marcias-Garcia, A. Preparation and textural characterization of activated carbon from vine shoots (Vitis vinifera) by H3PO4-chemical activation. Appl. Surf. Sci. 2006, 252, 5961–5966. [Google Scholar] [CrossRef]
- Deng, H.; Zhang, G.; Xu, X.; Tao, G.; Dai, J. Optimization of preparation of activated carbon from cotton stalk by microwave assisted phosphoric acid-chemical activation. J. Hazard. Mater. 2010, 182, 217–224. [Google Scholar] [CrossRef]
- Groen, J.C.; Peffer, L.A.A.; Pérez-Ramírez, J. Pore size determination in modified mi-cro- and mesoporous materials. Pitfalls and limitations in gas adsorption data analysis. Microp. Mesopor. Mater. 2003, 60, 1–3. [Google Scholar] [CrossRef]
- Liou, T.H. Development of mesoporous structure and high adsorption capacity of biomass-based activated carbon by phosphoric acid and zinc chloride activation. Chem. Eng. J. 2010, 158, 129–142. [Google Scholar] [CrossRef]
- Infrared Spectroscopy Absorption Table. Available online: https://chem.libretexts.org/Ancillary_Materials/Reference/Reference_Tables/Spectroscopic_Reference_Tables/Infrared_Spectroscopy_Absorption_Table (accessed on 16 June 2022).
- Huang, Y.; Li, S.; Chen, J.; Zhang, X.; Chen, Y. Adsorption of Pb(II) on mesoporous activated carbons fabricated from water hyacinth using H3PO4 activation: Adsorption capacity, kinetic and isotherm studies. Appl. Surf. Sci. 2014, 293, 160–168. [Google Scholar] [CrossRef]
- Kilic, M.; Apaydin-Varol, E.; Putun, A.E. Preparation and surface characterization of activated carbons from Euphorbia rigida by chemical activation with ZnCl2, K2CO3, NaOH and H3PO4. Appl. Surf. Sci. 2010, 261, 247–254. [Google Scholar] [CrossRef]
- Semerjian, L. Equilibrium and kinetics of cadmium adsorption from aqueous solutions using untreated Pinus halepensis sawdust. J. Hazard. Mater. 2010, 173, 236–242. [Google Scholar] [CrossRef]
- Yu, F.; Sun, S.; Han, S.; Zheng, J.; Ma, J. Adsorption removal of ciprofloxacin by multi-walled carbon nanotubes with different oxygen contents from aqueous solutions. Chem. Eng. J. 2016, 285, 588–595. [Google Scholar] [CrossRef]
- Spessato, L.; Duarte, V.A.; Viero, P.; Zanella, H.; Fonseca, J.M.; Arroyo, P.A.; Almeida, V.C. Optimization of Sibipiruna activated carbon preparation by simplex-centroid mixture design for simultaneous adsorption of rhodamine B and metformin. J. Hazard. Mater. 2021, 411, 125166. [Google Scholar] [CrossRef]
- Langmuir, I. The adsorption of gases on plane surfaces of glass, mica and platinum. J. Am. Chem. Soc. 1918, 40, 1361–1403. [Google Scholar] [CrossRef]
- Freundlich, H.M.F. Über die Adsorption in Lösungen. Z. Phys. Chem. 1906, 57A, 385–470. [Google Scholar] [CrossRef]
- Quintelas, C.; Rocha, Z.; Silva, B.; Fonseca, B.; Figueiredo, H.; Tavares, T. Adsorption of Cr(III) from an Aqueous Solution by Chitosan Beads Modified with Sodium Dodecyl Sulfate (SDS). Chem. Eng. J. 2009, 149, 319–324. [Google Scholar] [CrossRef]
- Redlich, O.; Peterson, D.L. A useful adsorption isotherm. J. Phys. Chem. 1959, 63, 1024–1026. [Google Scholar] [CrossRef]
- Lagergren, S. Zur theorie der sogenannten adsorption gelösterstoffe. K. Sven. Vetensk. Handl. 1989, 24, 1–39. [Google Scholar]
- Ho, Y.S.; Ng, J.C.Y.; McKay, G. Kinetics of pollutant sorption by biosorbents: Review. Sep. Purif. Meth. 2000, 29, 189–232. [Google Scholar] [CrossRef]
- Weber, W.J.; Morris, J.C. Kinetics of adsorption on carbon from solution. ASCE Sanit. Eng. Div. J. 1963, 1, 1–2. [Google Scholar] [CrossRef]
- Wu, F.C.; Liu, B.L.; Wu, K.T.; Tseng, R.L. A new linear form analysis of Redlich–Peterson isotherm equation for the adsorptions of dyes. Chem. Eng. J. 2010, 162, 21–27. [Google Scholar] [CrossRef]
- Lotfi, H.; Khoshanabad, J.R.; Vadi, M. Adsorption of metformin as anti-diabetic drug on carbon nanotube and activated carbon. RJPBCS 2015, 6, 1442. [Google Scholar]
- Kalumpha, M.; Guyo, U.; Zinyama, N.P.; Vakira, F.M.; Nyamunda, B.C. Adsorptive potential of Zea mays tassel activated carbon towards the removal of metformin hydrochloride from pharmaceutical effluent. Int. J. Phytoremed. 2020, 22, 148–156. [Google Scholar] [CrossRef] [PubMed]
- Elamin, M.R.; Abdulkhair, B.Y.; Algethami, F.K.; Khezami, L. Linear and nonlinear investigations for the adsorption of paracetamol and metformin from water on acid-treated clay. Sci. Rep. 2021, 11, 13606. [Google Scholar] [CrossRef]
- Niaei, H.A.; Rostamizadeh, M. Adsorption of metformin from an aqueous solution by Fe-ZSM-5 nano-adsorbent: Isotherm, kinetic and thermodynamic studies. J. Chem. Thermodyn. 2020, 142, 106003. [Google Scholar] [CrossRef]
- Oluwatimileyin, S.J.; Asiata Omotayo, I.; Olugbenga, S.B. Metformin adsorption onto activated carbon prepared by acid activation and carbonization of orange peel. Inter. J. Phytorem. 2022, 20, 1–12. [Google Scholar]
- Huang, X.; Liu, Y.; Liu, S.; Li, Z.; Tan, X.; Ding, Y.; Zeng, G.; Xu, Y.; Zeng, W.; Zheng, B. Removal of metformin hydrochloride by Alternanthera philoxeroides biomass derived porous carbon materials treated with hydrogen peroxide. RSC Adv. 2016, 6, 79275. [Google Scholar] [CrossRef]
- Plazinski, W.; Rudzinski, W.; Plazinska, A. Theoretical models of sorption kinetics including a surface reaction mechanism: A review. Adv. Coll. Interf. Sci. 2009, 152, 2–13. [Google Scholar] [CrossRef]
- Kavitha, D.; Namasivayam, C. Experimental and kinetic studies on methylene blue adsorption by coir pith Carbon. Biores. Technol. 2007, 98, 14–21. [Google Scholar] [CrossRef]
- Rudzinski, W.; Plazinski, W. Kinetics of solute adsorption at solid/solution interfaces: On the special features of the initial adsorption kinetics. Langmuir 2008, 24, 6738–6744. [Google Scholar] [CrossRef] [PubMed]
- Tan, K.L.; Hameed, B.H. Insight into the adsorption kinetics models for the removal of contaminants from aqueous solutions. J. Taiwan Inst. Chem. Eng. 2017, 74, 25–48. [Google Scholar] [CrossRef]
- Raji, F.; Pakizeh, M. Kinetic and thermodynamic studies of Hg(II) adsorption onto MCM-41 modified by ZnCl2 . Appl. Surf. Sci. 2014, 301, 568–575. [Google Scholar] [CrossRef]
- Herath, I.; Kumarathilaka, P.; Al-Wabel, M.; Abduljabbar, A.; Ahmad, M.; Usman, A.R.A.; Vithanage, M. Mechanistic modeling of glyphosate interaction with rice husk derived engineered biochar. Micropor. Mesopor. Mater. 2016, 225, 280–288. [Google Scholar] [CrossRef]
- Bayuo, J.; Pelig-Ba, K.B.; Abukari, M.A. Optimization of adsorption parameters for effective removal of lead (II) from aqueous solution. Phys. Chem. Indian J. 2019, 14, 1–25. [Google Scholar]
- Valizadeh, S.; Younesi, H.; Bahramifar, N. Highly mesoporous K2CO3 and KOH/activated carbon for SDBS removal from water samples: Batch and fixed-bed column adsorption process. Environ. Nanotechnol. Monit. Manag. 2016, 6, 1–13. [Google Scholar] [CrossRef]
- Zhang, B.; Zhang, T.; Zhang, Z.; Xie, M. Hydrothermal synthesis of a graphene/magnetite/montmorillonite nanocomposite and its ultrasonically assisted methylene blue adsorption. J. Mater. Sci. 2019, 54, 11037–11055. [Google Scholar] [CrossRef]







| Sample | SBET (m2 g−1) | Vtot (cm3 g−1) | Vmic (cm3 g−1) | Vmeso (cm3 g−1) | DP (nm) |
|---|---|---|---|---|---|
| WH | 2.4 | 0.00872 | - | - | - |
| 1.5/600C | 1421.0 | 0.741 | 0.294 | 0.446 | 2.8 |
| Isotherm Model | Adsorption Models Parameters | |||
|---|---|---|---|---|
| Langmuir | KL (dm3 mg−1) | RL | qmax (mg g−1) | R2 |
| 0.1194 | 0.457 | 122.47 | 0.997 | |
| Freundlich | KF (mg g−1) (dm3 mg−1)1/n | nF | R2 | |
| 28.871 | 3.05 | 0.977 | ||
| Redlich–Peterson | KR (L g−1) | A (L mg−1)n | nR (g mg−1) | R2 |
| 19.163 | 0.246 | 0.894 | 0.995 | |
| Adsorbent | Adsorption Parameters | qmax (mg g−1) | References |
|---|---|---|---|
| 1.5AC/600 | SBET = 1421 m2 g−1; pH = 8.20; T = 25 °C, CMTF = 10–100 mg dm−3 | 122.47 | Current study |
| Multi-walled carbon nanotubes, commercial | SBET = 250–280 m2 g−1; T = 295 K, CMTF = 10–88 mg dm−3 | 79.94 | [44] |
| Granular activated carbon | SBET = 1500 m2 g−1; T = 295 K; CMTF = 10–88 mg dm−3 | 72.56 | [44] |
| Graphene oxide | pH = 6; CMTF = 8–40 mg L−1, contact time 160 min; T = 288, 303 and 318 K. | 96.748 89.099 88.517 | [1] |
| Activated carbon from agricultural waste | T = 20 °C, CMTF = 10–200 mg L−1 pH = 7, contact time 125 min. | 44.84 | [45] |
| Sibipiruna activated carbon | CMTF = 500 mg L−1, T = 30 °C, pH = 13, contact time 360 min. | 248.48 | [35] |
| Water-treated clay Acid-treated clay | SBET = 9.5 –11.5 m2 g−1; pH = 6, CMTF = 1–20 mg L−1 T = 298 K; contact time 30 min. | 25.268 33.788 | [46] |
| Fe-ZSM-5 nano-adsorbent | T = 25 °C, CMTF = 5–20 mg L−1, contact time 20 min. | 14.992 | [47] |
| Activated carbon from orange peel | T = 323 K, pH = 7; contact time 240 min. | 50.99 | [48] |
| Hydrogen peroxide modified biochar | T = 308.15 K, CMTF = 0.05–3.6 mmol L−1, contact time 24 h. | 107.33 | [49] |
| Model Parameters | C0 (mg g−1) | |||||
| 10 | 20 | 40 | 60 | 80 | 100 | |
| qeexp (mg g−1) | 30.01 | 60.99 | 81.02 | 97.52 | 107.00 | 113.01 |
| Pseudo-first order kinetics model | ||||||
| qe (mg g−1) | 24.11 | 48.76 | 59.34 | 69.77 | 86.22 | 109.19 |
| k1 10−3 (min−1) | 5.99 | 7.25 | 8.42 | 9.03 | 10.9 | 16.4 |
| R2 | 0.972 | 0.991 | 0.899 | 0.983 | 0.987 | 0.929 |
| Pseudo-second order kinetics model | ||||||
| qe (mg g−1) | 32.79 | 65.79 | 86.96 | 104.17 | 111.11 | 119.05 |
| k2·10−3 (g mg−1 min) | 2.34 | 1.44 | 1.24 | 1.18 | 1.35 | 1.45 |
| R2 | 0.996 | 0.998 | 0.996 | 0.996 | 0.997 | 0.996 |
| Weber–Morris intra-particle diffusion model | ||||||
| Cid1 | 1.40 | 3.37 | 9.26 | 11.172 | 11.57 | 11.68 |
| kWM1 (mg g−1 min−1/2) | 3.46 | 7.69 | 9.23 | 11.64 | 20.56 | 29.536 |
| R2 | 0.962 | 0.987 | 0.980 | 0.974 | 0.976 | 0.988 |
| kWM2 (mg g−1 min−1/2) | 0.031 | 0.086 | 0.119 | 0.069 | 0.247 | 0.327 |
| R2 | 0.985 | 0.969 | 0.980 | 0.994 | 0.999 | 0.946 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mohammad, A.H.; Radovic, I.; Ivanović, M.; Kijevčanin, M. Adsorption of Metformin on Activated Carbon Produced from the Water Hyacinth Biowaste Using H3PO4 as a Chemical Activator. Sustainability 2022, 14, 11144. https://doi.org/10.3390/su141811144
Mohammad AH, Radovic I, Ivanović M, Kijevčanin M. Adsorption of Metformin on Activated Carbon Produced from the Water Hyacinth Biowaste Using H3PO4 as a Chemical Activator. Sustainability. 2022; 14(18):11144. https://doi.org/10.3390/su141811144
Chicago/Turabian StyleMohammad, Ahmad Hakky, Ivona Radovic, Marija Ivanović, and Mirjana Kijevčanin. 2022. "Adsorption of Metformin on Activated Carbon Produced from the Water Hyacinth Biowaste Using H3PO4 as a Chemical Activator" Sustainability 14, no. 18: 11144. https://doi.org/10.3390/su141811144
APA StyleMohammad, A. H., Radovic, I., Ivanović, M., & Kijevčanin, M. (2022). Adsorption of Metformin on Activated Carbon Produced from the Water Hyacinth Biowaste Using H3PO4 as a Chemical Activator. Sustainability, 14(18), 11144. https://doi.org/10.3390/su141811144







