Study on Overburden Movement Deformation and Roof Breakage Law of Under-Protective Steeply Inclined Coal Seam Mining
Abstract
:1. Introduction
2. Roof Breaking Regular of Under-Protective Steeply Inclined Coal Seam Mining
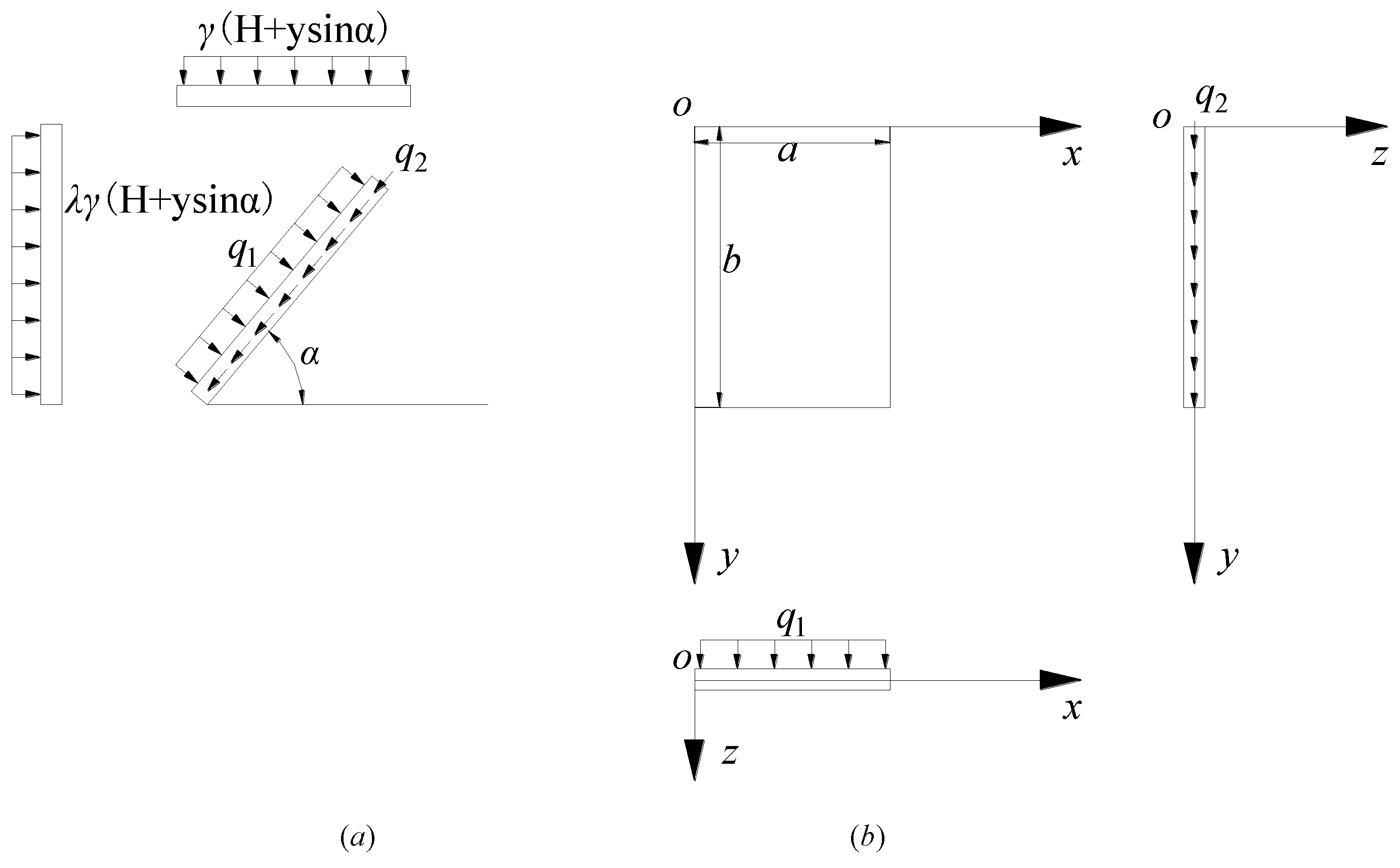
2.1. Stress Analysis of Steep Roof
2.2. Analysis of the First Roof Collapse with the Thin-Plate Theory
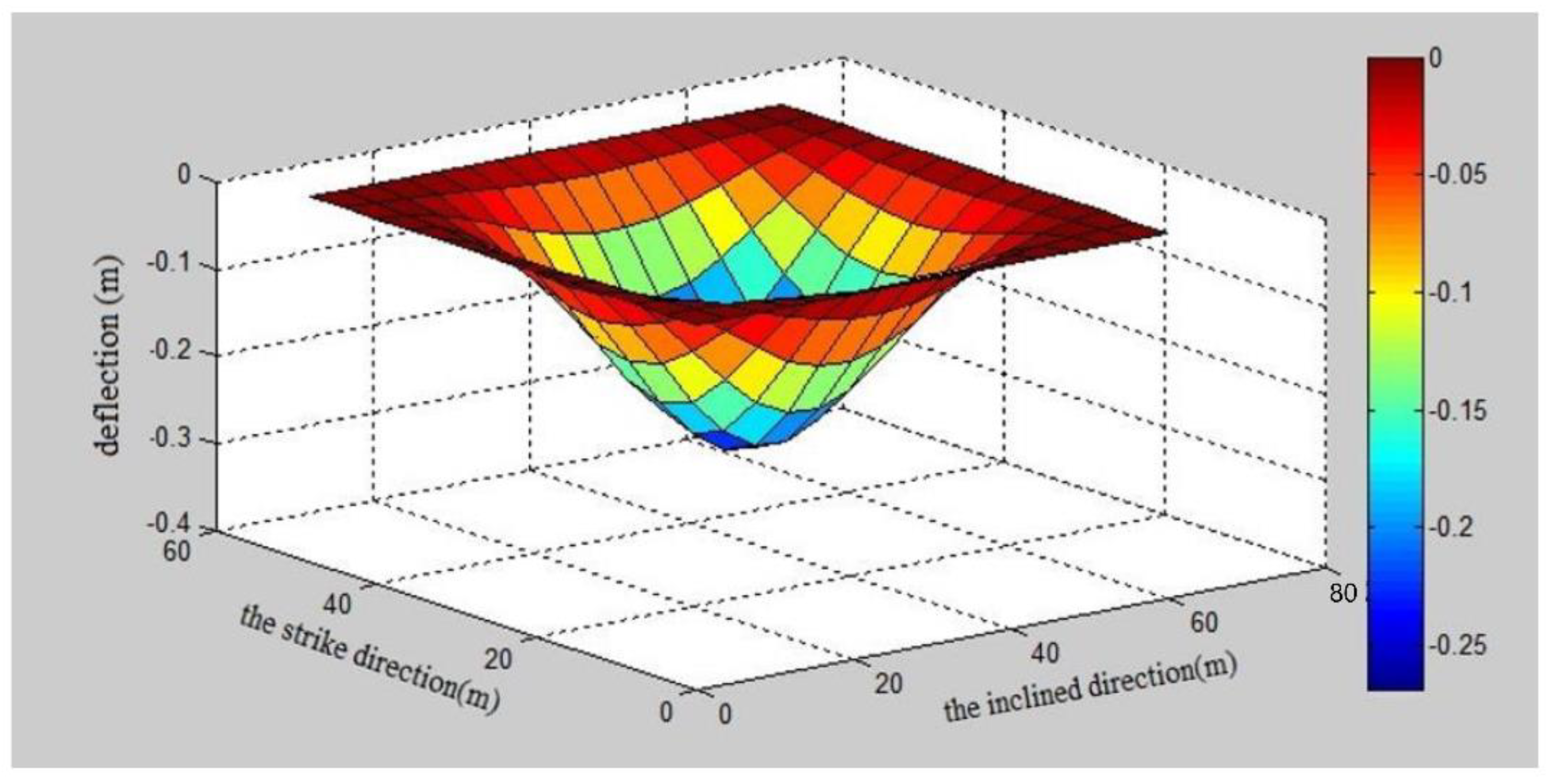
2.3. Analysis in Normal Mining Stage with the Thin-Plate Theory
3. Similar Simulation Experiment of Overburden Movement Deformation of Under-Protective Steeply Inclined Coal Seam Mining
3.1. Experimental Model Design
3.2. Experimental Procedure
- (1)
- Lay the model
- (2)
- Layout of rock stratum mobile measuring points
- (3)
- Compensate stress
- (4)
- Excavation and data collection
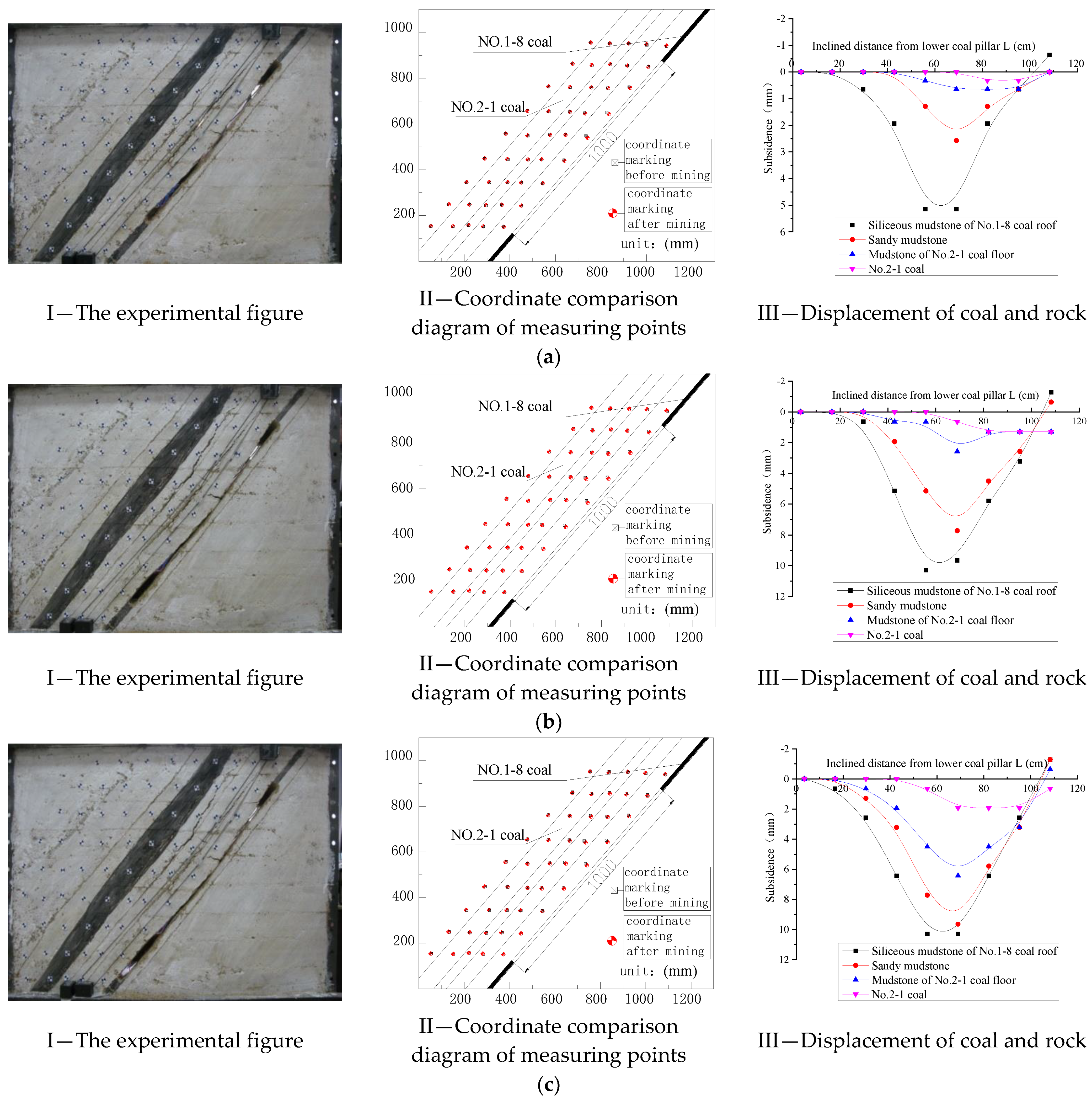
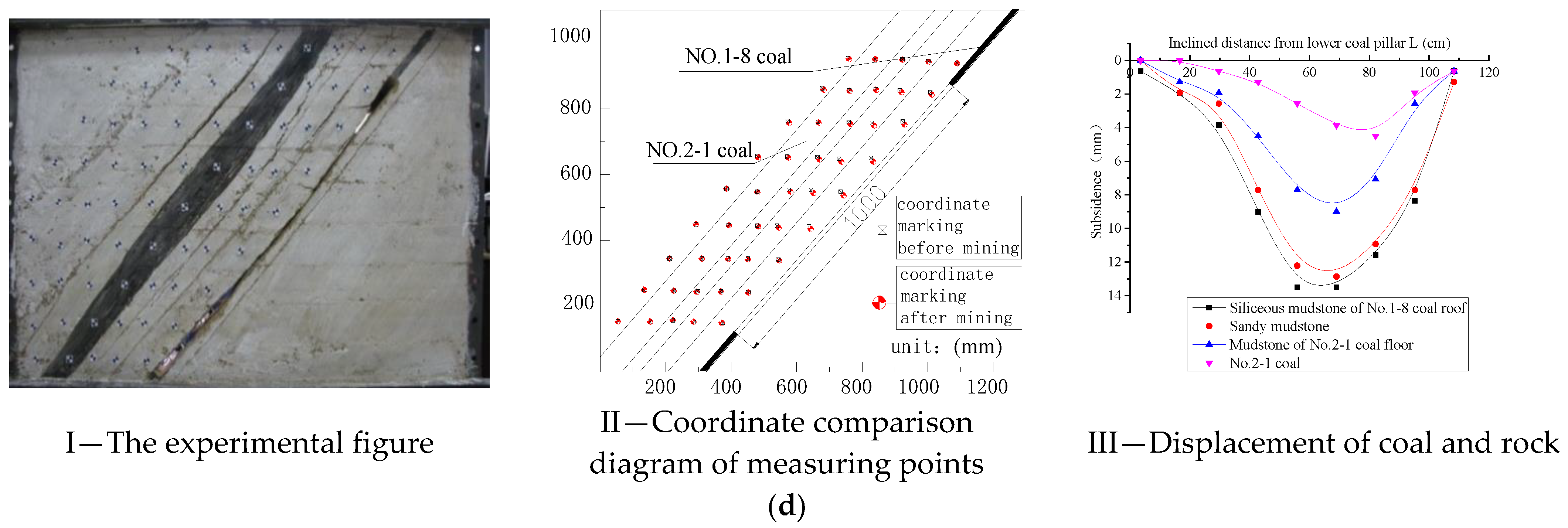
3.3. Experimental Results
4. Analysis and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tu, H.; Tu, S.; Yuan, Y.; Wang, F.; Bai, Q. Present Situation of Fully Mechanized Mining Technology for Steeply Inclined Coal Seams in China. Arab. J. Geosci. 2015, 8, 4485–4494. [Google Scholar] [CrossRef]
- Liu, H.; Liu, H.; Cheng, Y. The Elimination of Coal and Gas Outburst Disasters by Ultrathin Protective Seam Drilling Combined with Stress-Relief Gas Drainage in Xinggong Coalfield. J. Nat. Gas Sci. Eng. 2014, 21, 837–844. [Google Scholar] [CrossRef]
- Peng, X.; Wang, Z.; Qi, L. Numerical Simulation of Gas-Solid Coupling in Pressure-Relief Gas Drainage of Short-Distance and Underprotective Steeply Inclined Coal Seam Mining. Geofluids 2022, 2022, 1979775. [Google Scholar] [CrossRef]
- Cheng, X.; Zhao, G.; Li, Y.; Meng, X.; Tu, Q.; Huang, S.; Qin, Z. Mining-Induced Pressure-Relief Mechanism of Coal-Rock Mass for Different Protective Layer Mining Modes. Adv. Mater. Sci. Eng. 2021, 2021, 3598541. [Google Scholar] [CrossRef]
- Niu, Y.; Wang, E.; Li, Z.; Gao, F.; Zhang, Z.; Li, B.; Zhang, X. Identification of Coal and Gas Outburst-Hazardous Zones by Electric Potential Inversion During Mining Process in Deep Coal Seam. Rock Mech. Rock Eng. 2022, 55, 3439–3450. [Google Scholar] [CrossRef]
- Yang, W.; Lin, B.Q.; Qu, Y.A.; Zhao, S.; Zhai, C.; Jia, L.L.; Zhao, W.Q. Mechanism of Strata Deformation under Protective Seam and Its Application for Relieved Methane Control. Int. J. Coal Geol. 2011, 85, 300–306. [Google Scholar] [CrossRef]
- Yin, G.; Li, M.; Wang, J.G.; Xu, J.; Li, W. Mechanical Behavior and Permeability Evolution of Gas Infiltrated Coals during Protective Layer Mining. Int. J. Rock Mech. Min. Sci. 2015, 80, 292–301. [Google Scholar] [CrossRef]
- Chen, H.; Cheng, Y.; Ren, T.; Zhou, H.; Liu, Q. Permeability Distribution Characteristics of Protected Coal Seams during Unloading of the Coal Body. Int. J. Rock Mech. Min. Sci. 2014, 71, 105–116. [Google Scholar] [CrossRef]
- Wang, H.; Cheng, Y.; Yuan, L. Gas Outburst Disasters and the Mining Technology of Key Protective Seam in Coal Seam Group in the Huainan Coalfield. Nat. Hazards 2013, 67, 763–782. [Google Scholar] [CrossRef]
- Wang, L.; Lu, Z.; Chen, D.P.; Liu, Q.Q.; Chu, P.; Shu, L.Y.; Ullah, B.; Wen, Z.J. Safe Strategy for Coal and Gas Outburst Prevention in Deep-and-Thick Coal Seams Using a Soft Rock Protective Layer Mining. Saf. Sci. 2020, 129, 104800. [Google Scholar] [CrossRef]
- Liu, H.; Cheng, Y. The Elimination of Coal and Gas Outburst Disasters by Long Distance Lower Protective Seam Mining Combined with Stress-Relief Gas Extraction in the Huaibei Coal Mine Area. J. Nat. Gas Sci. Eng. 2015, 27, 346–353. [Google Scholar] [CrossRef]
- Liao, Z.; Feng, T.; Yu, W.; Wu, G.; Li, K. Experimental and Theoretical Investigation of Overburden Failure Law of Fully Mechanized Work Face in Steep Coal Seam. Adv. Civ. Eng. 2020, 2020, 8843172. [Google Scholar] [CrossRef]
- Li, X.; Wang, Z.; Zhang, J. Stability of Roof Structure and Its Control in Steeply Inclined Coal Seams. Int. J. Min. Sci. Technol. 2017, 27, 359–364. [Google Scholar] [CrossRef]
- Xie, P.; Wu, Y.; Wang, H.; Gao, X.; Zhang, Y.; Zeng, Y. Mechanism Analysis on Deformation and Failure of Gob-Side Entry in Soft Rock and Steep Coal Seam Group with Repeated Mining Operation. Liaoning Gongcheng Jishu Daxue Xuebao (Ziran Kexue Ban)/J. Liaoning Tech. Univ. (Nat. Sci. Ed.) 2013, 32, 44–49. [Google Scholar]
- Zhang, J.W.; Wang, J.C.; Wei, W.J.; Chen, Y.; Song, Z.Y. Experimental and Numerical Investigation on Coal Drawing from Thick Steep Seam with Longwall Top Coal Caving Mining. Arab. J. Geosci. 2018, 11, 96. [Google Scholar] [CrossRef]
- Wang, S.C.; Dou, L.M.; Mu, Z.L.; Cao, J.R.; Li, X.W. Study on Roof Breakage-Induced Roadway Coal Burst in an Extrathick Steeply Inclined Coal Seam. Shock Vib. 2019, 2019, 2969483. [Google Scholar] [CrossRef]
- Hu, B.; Wu, Y.; Wang, H.; Tang, Y.; Wang, C. Risk Mitigation for Rockfall Hazards in Steeply Dipping Coal Seam: A Case Study in Xinjiang, Northwestern China. Geomat. Nat. Hazards Risk 2021, 12, 988–1014. [Google Scholar] [CrossRef]
- Yuan, Z.; Shao, Y.; Zhu, Z. Similar Material Simulation Study on Protection Effect of Steeply Inclined Upper Protective Layer Mining with Varying Interlayer Distances. Adv. Civ. Eng. 2019, 2019, 9849635. [Google Scholar] [CrossRef]
- Xie, P.; Wu, Y. Deformation and Failure Mechanisms and Support Structure Technologies for Goaf-Side Entries in Steep Multiple Seam Mining Disturbances. Arch. Min. Sci. 2019, 64, 561–574. [Google Scholar] [CrossRef]
- Wu, Y.P.; Xie, P.S.; Wang, H.W.; Ren, S.G. Incline Masonry Structure around the Coal Face of Steeply Dipping Seam Mining. Meitan Xuebao/J. China Coal Soc. 2010, 35, 1252–1256. [Google Scholar]
- Zeng, C.; Zhou, Y.; Zhang, L.; Mao, D.; Bai, K. Study on Overburden Failure Law and Surrounding Rock Deformation Control Technology of Mining through Fault. PLoS ONE 2022, 17, e0262243. [Google Scholar] [CrossRef] [PubMed]
- Ning, J.; Wang, J.; Bu, T.; Hu, S.; Liu, X. An Innovative Support Structure for Gob-Side Entry Retention in Steep Coal Seam Mining. Minerals 2017, 7, 75. [Google Scholar] [CrossRef]
- Liu, S.; Yang, K.; Tang, C.; Chi, X. Rupture and Migration Law of Disturbed Overburden during Slicing Mining of Steeply Dipping Thick Coal Seam. Adv. Civ. Eng. 2020, 2020, 8863547. [Google Scholar] [CrossRef]
- Ventsel, E.; Krauthammer, T.; Carrera, E. Thin Plates and Shells: Theory, Analysis, and Applications. Appl. Mech. Rev. 2002, 55, B72–B73. [Google Scholar] [CrossRef]
- Tu, H.; Tu, S.; Chen, F.; Wang, C.; Feng, Y. Study on the Deformation and Fracture Feature of Steep Inclined Coal Seam Roof Based on the Theory of Thin Plates. J. Min. Saf. Eng. 2014, 31, 49–54. [Google Scholar]
- Li, X.; Chen, S.; Zhang, Q.; Gao, X.; Feng, F. Research on Theory, Simulation and Measurement of Stress Behavior under Regenerated Roof Condition. Geomech. Eng. 2021, 26, 49–61. [Google Scholar] [CrossRef]
- Liu, H.; Zhang, B.; Li, X.; Liu, C.; Wang, C.; Wang, F.; Chen, D. Research on Roof Damage Mechanism and Control Technology of Gob-Side Entry Retaining under Close Distance Gob. Eng. Fail. Anal. 2022, 138, 106331. [Google Scholar] [CrossRef]
- Zhang, B.; Cao, S. Study on First Caving Fracture Mechanism of Overlying Roof Rock in Steep Thick Coal Seam. Int. J. Min. Sci. Technol. 2015, 25, 133–138. [Google Scholar] [CrossRef]
- Fan, X.G.; Wang, H.T.; Hu, G.Z.; Li, X.H.; Yuan, Z.G. Pressure-Relief Scope for the Exploiting of Steep-Inclined Oblique under-Protecting Strata. Zhongguo Kuangye Daxue Xuebao/J. China Univ. Min. Technol. 2010, 39, 380–385. [Google Scholar]
- Wu, Y.; Yun, D.; Xie, P.; Wang, H.; Lang, D.; Hu, B. Progress, Practice and Scientific Issues in Steeply Dipping Coal Seams Fully-Mechanized Mining. Meitan Xuebao/J. China Coal Soc. 2020, 45, 24–34. [Google Scholar] [CrossRef]
- Hongwei, W.; Yongping, W.; Jianqiang, J.; Peipei, C. Stability Mechanism and Control Technology for Fully Mechanized Caving Mining of Steeply Inclined Extra-Thick Seams with Variable Angles. Min. Metall. Explor. 2021, 38, 1047–1057. [Google Scholar] [CrossRef]
- Chen, D.; Sun, C.; Wang, L. Collapse Behavior and Control of Hard Roofs in Steeply Inclined Coal Seams. Bull. Eng. Geol. Environ. 2021, 80, 1489–1505. [Google Scholar] [CrossRef]
- Lu, P.; Zhang, P.Q.; Lee, H.P.; Wang, C.M.; Reddy, J.N. Non-Local Elastic Plate Theories. Proc. R. Soc. A Math. Phys. Eng. Sci. 2007, 463, 3225–3240. [Google Scholar] [CrossRef]
- Wei, Z.; Yang, K.; Chi, X.; Liu, W.; Zhao, X. Dip Angle Effect on the Main Roof First Fracture and Instability in a Fully-Mechanized Workface of Steeply Dipping Coal Seams. Shock Vib. 2021, 2021, 5557107. [Google Scholar] [CrossRef]
- Hong-Sheng, T.; Shi-Hao, T.; Cun, Z.; Lei, Z.; Xiao-Gang, Z. Characteristics of the Roof Behaviors and Mine Pressure Manifestations During the Mining of Steep Coal Seam. Arch. Min. Sci. 2017, 62, 871–891. [Google Scholar] [CrossRef]
- Yin, G.; Dai, G.; Pi, W.; Zhang, D. Study on the Uneven Ground Pressure in Pitching Oblique Underhand Mining. Yanshilixue Yu Gongcheng Xuebao/Chin. J. Rock Mech. Eng. 2003, 22, 1483–1488. [Google Scholar]
- Wu, Y.P.; Xie, P.S.; Ren, S.G. Analysis of Asymmetric Structure around Coal Face of Steeply Dipping Seam Mining. Meitan Xuebao/J. China Coal Soc. 2010, 35, 182–184. [Google Scholar]
- Zhang, J.F.; Shi, P.W.; Zhang, H.M. Stability Analysis of Basic Roof after First Destruction in the Steep Seam. Meitan Xuebao/J. China Coal Soc. 2009, 34, 1160–1164. [Google Scholar]
- Shi, F.; Wang, H.T.; Fan, X.G.; Yuan, Z.G.; Hu, G.Z. Mechanical Analysis of Main Roof Breakage with Dip Oblique Coal Mining Method. Meitan Xuebao/J. China Coal Soc. 2013, 38, 1001–1005. [Google Scholar]
- Wu, Y.; Lang, D.; Xie, P.; Wang, H. Regional Fracture of Top-Coal along the Inclined Direction of Fully-Mechanized Caving Face in Soft Steep Dipping Seam. Caikuang Yu Anquan Gongcheng Xuebao/J. Min. Saf. Eng. 2018, 35, 553–560. [Google Scholar] [CrossRef]
- Guo, Z.P.; Huang, W.P. Parameter Optimization and Stability Analysis of Inclined Gangue Strip-Fillings. Meitan Xuebao/J. China Coal Soc. 2011, 36, 234–238. [Google Scholar]
- Tu, H.S.; Tu, S.H.; Zhu, D.F.; Hao, D.Y.; Miao, K.J. Force-Fracture Characteristics of the Roof above Goaf in a Steep Coal Seam: A Case Study of Xintie Coal Mine. Geofluids 2019, 2019, 7639159. [Google Scholar] [CrossRef]
- Xie, P.S.; Wu, Y.P.; Wang, H.W.; Gao, X.C.; Ren, S.G.; Zeng, Y.F. Stability Analysis of Incline Masonry Structure and Support around Longwall Mining Face Area in Steeply Dipping Seam. Meitan Xuebao/J. China Coal Soc. 2012, 37, 1275–1280. [Google Scholar]
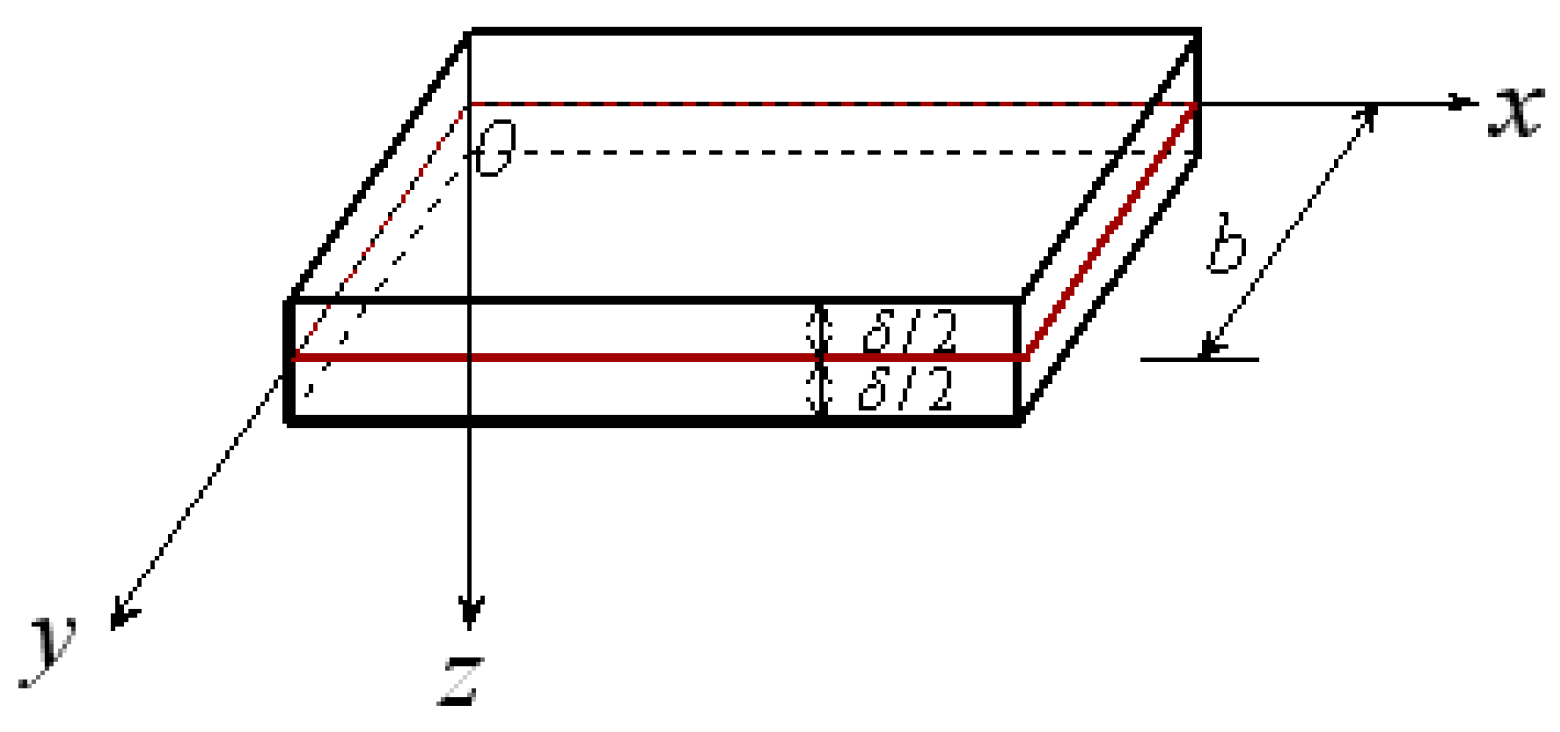





| The Type of Plate | The Thin Films | The Thin Plate | The Thick Plate |
|---|---|---|---|
| The ratio of thickness (δ) to minimum side length (b) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, X.; Qi, L.; Wang, Z.; Zhou, X.; Hua, C. Study on Overburden Movement Deformation and Roof Breakage Law of Under-Protective Steeply Inclined Coal Seam Mining. Sustainability 2022, 14, 10068. https://doi.org/10.3390/su141610068
Peng X, Qi L, Wang Z, Zhou X, Hua C. Study on Overburden Movement Deformation and Roof Breakage Law of Under-Protective Steeply Inclined Coal Seam Mining. Sustainability. 2022; 14(16):10068. https://doi.org/10.3390/su141610068
Chicago/Turabian StylePeng, Xinshan, Lingling Qi, Zhaofeng Wang, Xiaoqing Zhou, and Chunlei Hua. 2022. "Study on Overburden Movement Deformation and Roof Breakage Law of Under-Protective Steeply Inclined Coal Seam Mining" Sustainability 14, no. 16: 10068. https://doi.org/10.3390/su141610068
APA StylePeng, X., Qi, L., Wang, Z., Zhou, X., & Hua, C. (2022). Study on Overburden Movement Deformation and Roof Breakage Law of Under-Protective Steeply Inclined Coal Seam Mining. Sustainability, 14(16), 10068. https://doi.org/10.3390/su141610068







