Experimental Study on the Shear Mechanical Properties of Anchor Cable with C-Shaped Tube

Abstract

Faced with serious tensile–shear fracturing of anchor bolts and cables in deep roadways, it is of great significance to investigate Anchor Cable with C-shaped tube (ACC), a combined structure of a C-shaped tube and an anchor cable with high strength and shear resistance. The shear mechanical properties of the anchor cable and ACC are systematically investigated using the technical means of theoretical analysis and double-shear tests. The improved equipment for a double-shear test not only considers the initial normal stress but also ensures the continuity of the anchor cable’s axial force transmission while considering the influence of the inclination angle on the shear performance of supporting components. The research indicates that the C-shaped tube inhibits the occurrence of stress concentration near the joint surface and improves the stress state of the anchor cable, transforming its failure mode from tensile–shear failure to tensile failure. Compared with the anchor cable, ACC has a higher shear capacity, greater shear stiffness and better ductility in the shear direction. The shear capacity of ACC is higher when it is perpendicular to the joint plane than when inclined to 80°, and the shear capacity of the joint plane is linear with the initial normal stress. Two methods were proposed to contrast the shear capacity between the anchor cable and ACC, including the shear capacity of supporting components and the shear capacity of the joint surface. The former is to evaluate the shear capability of the anchor cable and ACC, while the latter is to evaluate the ability of the anchor cable and ACC to control the shear deformation of the joint surface. The tests results show that the average shear capacity of ACC is 279 kN higher than the anchor cable, with an average increase of 34.9%. The average shear capacity of the joint surface anchored by ACC is 306 kN higher than the anchor cable, with an average increase of 25.2%. ACC can effectively improve the shear capacity of the anchor cable in the free section and has wide application prospects.

1. Introduction

As a low-cost, efficient and convenient support component, bolt and anchor cables are widely used in roadway support. With increasing mining depths, complex geological conditions such as high ground stress, soft and broken surrounding rock, and mining impact pose new challenges to the bolt and cable support technology [1,2,3]. Many scholars have found that under high stress [4] and rockburst conditions [5], the bolt is prone to shear failure. Yangrenshu et al. [6] found that many anchor cables were sheared and broken within 2.5 m of the roadway roof in coal-measured strata. Therefore, the mechanical properties that are the focus of bolt and cable research have developed from simple tensile behavior to the composite properties of tension, bending and shear. Shan Renliang et al. [7] also developed a tube cable with a combined support structure, i.e., an Anchor Cable with a C-shaped tube (ACC), which enhances the shear resistance of anchor cables and has achieved promising application results on site [8,9].

Many scholars have studied the tensile and shear properties of the bolt and cable. G. Grasselli [10] analyzed the deformation and failure mode of the fully grouted bolt and the Sweelex bolt on the bolt-supporting joint surface and the mechanical response to the shear load through mechanical tests and numerical simulation. L. Li et al. [5] analyzed the influence of various parameters on the shear strength of a bolt through model tests and numerical simulations and concluded that rock strength, inclination and bolt diameter are the main factors affecting the shear strength of the bolt. Xuwei Li et al. [11] compared and analyzed the shear mechanical behavior and failure mode of FRP anchors, rock anchors and anchor cables through a double shear test. Naj Aziz et al. [12] compared the shear load of grouting anchor cables under the conditions of a single shear test and double shear test. According to the results, the shear value of a double shear test is 13–18% higher than that of a single shear test, and it is considered that the double shear test is more suitable for evaluating the shear behavior of rock bolts than the single shear test. Mirzaghorbanali A. et al. [13] studied the influence of the surface profile type and preload on the shear strength of five kinds of anchor cables using a double shear test. SR and FL [14] used large-size concrete blocks to study the shear resistance of bolts with different diameters under different anchor angles and found that the shear resistance of bolts with an anchor angle of 45° or 60° was higher than that of bolts with an anchor angle of 90°. Li et al. [15] studied the influence of anchor diameter, installation angle and other factors on the shear performance of anchors under dynamic loading through a double shear test. They found significant differences in the effectiveness of the shear stress of anchors under static loading and dynamic loading, and the differences were reflected in the shear deformation level and energy absorption. Egger PH et al. [16] analyzed the displacement–stress relationship of the bolt in the shear process and proposed an analysis model for analyzing the failure mode of the bolt based on the differential equation of elastic foundation beam deflection and the principle of minimum potential energy. Li C [17] analyzed the influence of parameters such as rod type, preload, surrounding rock strength and borehole diameter on the shear performance of anchor bolts using a double shear test and numerical simulation. Yang G [18] studied the shear mechanical response of different types of anchor cables using a single shear test and double shear test and analyzed the influence of different factors on the shear resistance of anchor cables. In addition, many scholars have used theoretical derivation, model tests and numerical simulation methods to study the shear resistance of anchor cables [19,20,21,22].

Many scholars have studied the tensile and shear properties of anchor cables, but there are still some deficiencies:

(1)

After the roadway is excavated, under the action of in situ stress, there is normal stress in the joint surface itself (hereinafter referred to as the initial normal stress for the convenience of differentiation). However, the influence of the initial normal stress has not been considered in the reported papers.

(2)

When using the double shear test method to study the influence of the angle between the bolt and the joint surface on the shear resistance, two bolts are used to penetrate the joint surface on both sides, respectively. On the one hand, this test method does not consider the reinforcement effect of the axial force of the bolt on the joint surface. On the other hand, the two bolts are independent of each other, and the force transmission is not continuous.

(3)

Most of the papers mainly focus on the tensile and shear properties of the anchor rod. Compared with the anchor rod, the anchor cable is composed of multiple steel strands, and its stress situation is more complex, more discrete, and more tensile than shear fracture phenomena.

(4)

Currently, the research on the tensile and shear properties of bolts and cables mainly focuses on the anchorage section of bolts and cables. However, in China’s coal mine roadway, many bolt and cable shears break in the free section. A tube cable composite structure—Anchor Cable with a C-shaped tube (hereinafter referred to as ACC) developed by Shan Renliang et al. [7]—could effectively optimize the shear resistance of free sections and restrain the occurrence of shear fractures, which make contributions to roadway stability and the sustainable development of the coal industry.

To further study the tensile and shear properties of anchor cables and ACC, this paper improves the existing double shear test equipment, not only constructively bringing the initial normal stress of the joint plane into the research scope, but also ensuring the continuity of axial force transmission when studying the influence of the included angle on the shear properties of the anchor cable and ACC (only one anchor cable or ACC is used). For the convenience of description, the anchor cable and ACC are collectively referred to as supporting components in this paper. The differences in shear resistance between the anchor cable and ACC are studied and compared using a double shear test with improved test equipment.

2. Shear Mechanical Behavior of Anchored Rock Mass

The shear strength of the joint plane in the surrounding rock is the ability of the rock mass on both sides of the joint plane to resist the relative shear displacement. It is an important rock mechanics index. Numerous studies show that the shear strength of the joint surface meets the Mohr–Coulomb criterion [23]:

$$\tau ={\sigma }_{\mathrm{n}}\tan \phi +c$$

(1)

where $\tau$ is the shear strength of the joint surface, ${\sigma }_{\mathrm{n}}$ is the normal stress on the joint surface, $\phi$ is the friction angle in the joint surface and c is the cohesion of the joint surface.

When the anchored rock mass has shear behavior, the supporting components will deform with the increase in shear displacement. The reinforcement effect of the support components on the joint surface can be divided into two parts: the first part is the shear reaction force parallel to the joint surface provided by the support components, namely the “dowel effect”. The second part is the additional normal stress applied by the axial force of the support member to the joint surface during the shear process. The additional normal stress provides the additional shear-bearing capacity to the joint surface through friction. Therefore, FL and PH [16] simplified the reinforcement effect of support components on the joint surface as an additional normal stress and the additional cohesion of the joint surface:

$$\tau =\left({\sigma }_{\mathrm{n}}+\mathsf{\Delta }{\sigma }_{\mathrm{n}}\right)\tan \phi +\left(c+\mathsf{\Delta }c\right)$$

(2)

where $\Delta {\sigma }_{\mathrm{n}}$ and $\Delta c$ are the additional normal stress and additional internal friction angle of the joint surface, respectively.

It can be seen that the shear strength of jointed rock mass mainly comes from two parts: the shear strength of the joint surface itself and the shear strength of the supporting components.

According to Formulas (1) and (2), combined with previous research results, it can be deduced that the shear strength of jointed rock mass anchored by support members is:

$$\tau ={\tau }_0+{\tau }_{\mathrm{n}}+{\tau }_{\mathrm{s}}$$

(3)

$${\tau }_0={\sigma }_0\tan \phi +c$$

(4)

$${\tau }_{\mathrm{n}}=\frac{N}{S}\tan \phi$$

(5)

$${\tau }_{\mathrm{s}}=\frac{T}{S}$$

(6)

where ${\tau }_0$ is the shear strength of the joint plane itself as affected by the in situ stress, ${\tau }_{\mathrm{n}}$ is the shear strength converted by the axial force of the support member, ${\tau }_{\mathrm{s}}$ is the shear strength converted by the shear force of the support member, ${\sigma }_0$ is the initial normal stress of the joint plane itself as affected by the in situ stress, N is the normal force applied by the support member to the joint plane perpendicular to the joint plane, T is the component of the resultant force of the axial force and shear force of the support member in the direction parallel to the joint plane, and S is the area of the joint plane.

There are two methods to improve the shear strength of the joint surface. One is to apply a greater normal force N to the joint surface, and the other is to increase the component T of the resultant force of the axial force and shear force of the supporting component in the direction parallel to the joint surface. The tube cable composite structure (ACC) developed by Shan Renliang et al. [7] makes up for the shortcoming of the low shear strength of the anchor cable when providing high support resistance. It not only ensures that the anchor cable can give full play to the axial bearing capacity but also that it has a high shear-bearing capacity, which has a broad application prospect.

3. Test Preparation

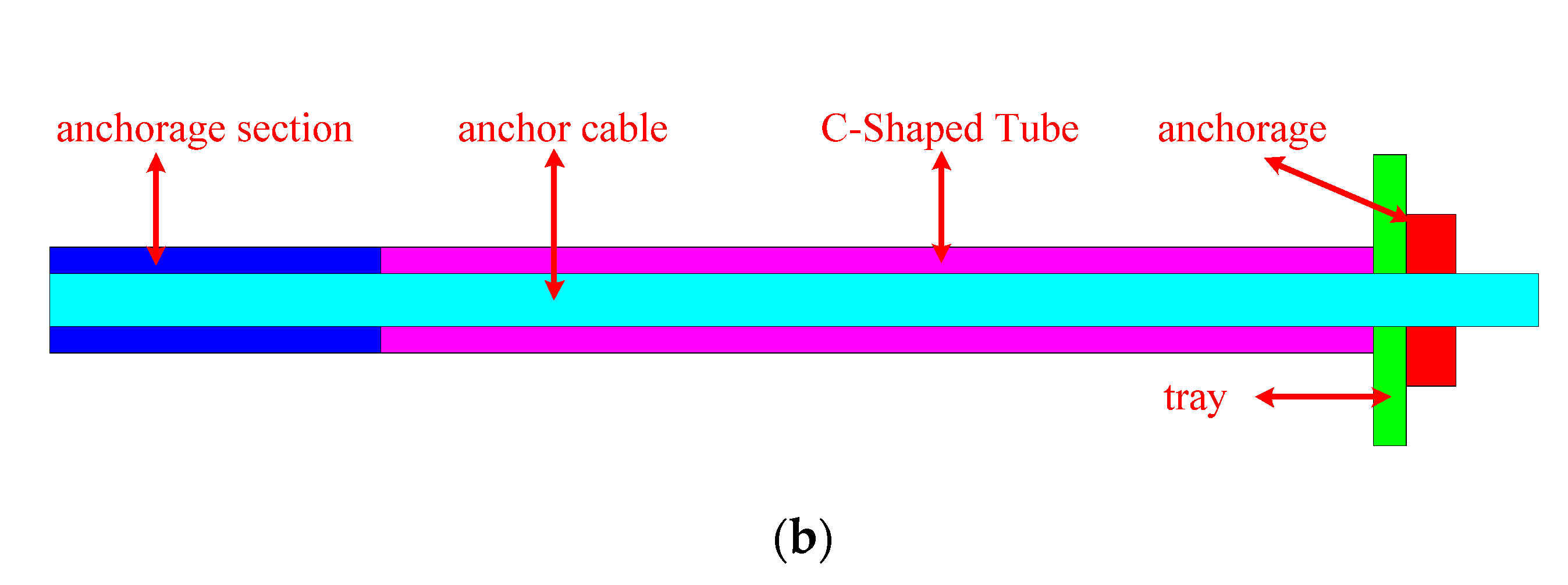
3.1. Brief Introduction of Tube Cable Composite Structurse

The tube cable composite structure comprises an anchor cable and a C-shaped slotted steel tube (hereinafter called a C-shaped tube). Figure 1 shows the deformation and support schematic diagram of the tube cable composite structure. At the free section of the anchor cable, a C-shaped tube with an appropriate length is arranged. The C-shaped tube has an outer diameter of 28 mm, an inner diameter of 24 mm, a wall thickness of 2 mm and joint width of 12 mm. It is rolled from Q345B steel. Under the action of in situ stress, the roadway’s surrounding rock has radial displacement and shear deformation along the joint surface. A C-shaped tube cannot only protect the anchor cable in the free section from shear failure caused by random lateral loads such as hole collapse, but also limit the shear slip and dilatancy of the surrounding rock through its high shear strength. Under the action of the surrounding rock, the C-shaped tube is gradually closed under stress. Finally, the anchor cable is wrapped, forming a whole with the anchor cable, bearing force together and deforming cooperatively.

3.2. Test Equipment

3.2.1. Cutting Box

It is well established that the shear box can effectively restrain the cracking and expansion of concrete blocks, achieve the purpose of transferring the load from the shear box to the concrete, and then from the concrete to the supporting components, and avoid a loss in the bearing capacity of concrete before the supporting components are damaged. It is worth noting that when predecessors studied the tensile and shear mechanical properties of supporting components such as anchor bolts and cables through double shear tests, the impact of the size of the shear box on the test results was not considered. Herein, it is considered that if the internal size of the shear box is equal to the size of the concrete block, there are the following disadvantages:

• During the deformation of the anchor cable, a large axial force will be generated. The axial force is applied to the concrete block through the anchor and the large diaphragm so that the concrete block will deform along the length of the anchor cable, resulting in the size of the concrete block being slightly smaller than the size of the shear box, as shown in Figure 2a.
• During the installation process, it is difficult to ensure that the concrete block and the shear box are closely connected, and the joint surface being located inside the shear box could barely be avoided; that is, the joint surface is not coplanar with the shear line, as shown in Figure 2b.

The above two disadvantages will cause the shear box to shear the concrete block during the test, thus affecting the test results, with the impact depending on the relative position of the joint surface and the shear box. Therefore, this paper redesigns the size of the shear box. Since the size of the concrete block is 300 mm × 300 mm × 300 mm, the internal size of the designed shear box is 295 mm × 300 mm × 300 mm, as shown in Figure 2c.

3.2.2. Included Angle

In practical engineering, the support components are usually arranged so that the support components are perpendicular to the joint surface. However, in the field, due to the problems of rock stratum inclination or construction technology, the support components are usually not perpendicular to the joint surface, but have a certain included angle. Therefore, it is significant to study the influence of the included angle between the support components and the joint surface on the shear mechanical behavior. Many scholars have studied the influence of the angle between the support member and the joint surface on the shear resistance of the support member through double shear tests. Li et al. [24] used the double shear test to study the shear performance when the bolt and the joint plane were at 90° and 45°. However, in this test, the two bolts were not connected, the axial force transmission was discontinuous and the axial force of the bolt could not act on the joint plane, which could not truly reflect the interaction between the bolt and the joint plane in the actual project. To simulate the interaction between the support member and the joint surface in practical engineering, this paper studies the shear mechanical behavior when the installation angle of the support member is 80° (that is, the included angle with the normal direction of the joint surface is 10°), ensuring the continuity of the axial force transmission of the support member.

3.2.3. Initial Normal Stress

To study the mechanical properties of the tube cable’s composite structure, Shan Renliang, Bao Yongsheng and others [21] used the test equipment shown in Figure 3a to study the mechanical properties of ACC in a pure shear state (i.e., the joint surface has no friction). However, this equipment has some shortcomings: (1) the joint surface has no friction, so it cannot simulate the interaction between the anchor cable and the joint surface in the actual project. (2) The effect of the initial normal stress was not considered in the test. (3) The influence of the included angle between the support member and the joint surface is not considered.

Based on Section 3.2.1, Section 3.2.2, Section 3.2.3, we improved the test equipment, so that the initial normal stress is applied to the joint surface through two horizontal sleeves. Specific procedures for applying the initial normal stress are as follows.

1. Before the test, the initial force shall be applied through the horizontal sleeve according to the shear surface area and the initial normal stress

$$F_0={\sigma }_{0}S$$

(7)

2. During the test, the area of the shear plane gradually decreases with the increase in the shear displacement. In order to ensure that the initial normal stress does not change, the reduction in the area of the shear plane in unit time $\Delta S$ is calculated according to the shear speed, and when combined with the size of the initial normal stress, the reduction of the initial force in the unit time can be obtained

$$\Delta F={\sigma }_0\Delta S={\sigma }_{0}vl$$

(8)

where F₀ is the initial force (kN); ${\sigma }_0$ is the initial normal stress (MPa); S is the cross-sectional area of the block (mm²); v is the shear speed (mm/min); l is the width of the block (mm).

By setting the initial force and the change value of the initial force per unit time in the computer software port, the purpose of maintaining the initial normal stress during the test can be achieved.

The modified test equipment is shown in Figure 3b,c.

3.3. Test Plan

A 21.8 mm anchor cable composed of 19 steel strands (abbreviated as 21.8 mm anchor cable) is widely used in coal mine roadway supports due to its high bearing capacity and elongation advantages. Therefore, the anchor cable with a diameter of 21.8 mm is selected for the test. The C-shaped tube with an outer diameter of 28 mm and an inner diameter of 24 mm is selected for the C-shaped tube, and the ACC support component composed of a 21.8 mm anchor cable with a C-shaped tube is abbreviated as 21.8 mm ACC. According to the tensile test results, the average uniaxial tensile capacity of a 21.8 mm anchor cable is 596 kN. Taking the inclination and initial normal stress as research variables, the tensile and shear properties of the 21.8 mm anchor cable and 21.8 mm ACC are studied. The specific test scheme is shown in Table 1.

3.4. Test Procedure

(1)

Mix the materials evenly according to the ratio of water:cement:stone:sand = 1:2:4:4, and make concrete blocks with a size of 300 mm × 300 mm × 300 mm in the mold. Place a steel tube with an outer diameter of 32 mm horizontally in the center of the mold in advance to form precast holes with a diameter of 32 mm. Meanwhile, make small blocks of 100 mm × 100 mm × 100 mm to characterize the uniaxial compressive strength of large blocks.

(2)

After formwork removal and curing for more than 28 days, a uniaxial compression test shall be conducted to test the strength of concrete blocks. The uniaxial compression rate is 2 mm/min. According to the test results, the uniaxial compressive strength of concrete blocks is determined to be 40 MPa.

(3)

Coat the inside of the shear box with grease to reduce the friction between the block and the shear box, then install each test device component and carry out the double shear test with a shear rate of 2 mm/min according to Table 1 of the test scheme.

Table 1. Test scheme.

Test Number	Support Component	Angle °	Initial Normal Stress MPa	Designed Preload kN	Actual Preload kN	Maximum of Shear Force kN	Average of Shear Stiffness kN/mm
NO1	ϕ21.8 mm Anchor cable	90	1.5	200	236.4	1159.5	8.6
NO2	ϕ21.8 mm ACC	90	1.5	200	201.9	1719.8	12.3
NO3	ϕ21.8 mm Anchor cable	80	1.5	200	184.2	1341.7	8.2
NO4	ϕ21.8 mm ACC	80	1.5	200	195.7	1557.7	11.2
NO5	ϕ21.8 mm Anchor cable	90	0	200	212.2	1352.8	10.0
NO6	ϕ21.8 mm ACC	90	0	200	223.3	1406.3	10.0
NO7	ϕ21.8 mm Anchor cable	90	3	200	216.1	1300.0	7.9
NO8	ϕ21.8 mm ACC	90	3	200	201.8	1604.0	9.0
NO9	ϕ21.8 mm ACC	90	9	200	219.5	2531.8	8.2

Table 1. Test scheme.

Test Number	Support Component	Angle °	Initial Normal Stress MPa	Designed Preload kN	Actual Preload kN	Maximum of Shear Force kN	Average of Shear Stiffness kN/mm
NO1	ϕ21.8 mm Anchor cable	90	1.5	200	236.4	1159.5	8.6
NO2	ϕ21.8 mm ACC	90	1.5	200	201.9	1719.8	12.3
NO3	ϕ21.8 mm Anchor cable	80	1.5	200	184.2	1341.7	8.2
NO4	ϕ21.8 mm ACC	80	1.5	200	195.7	1557.7	11.2
NO5	ϕ21.8 mm Anchor cable	90	0	200	212.2	1352.8	10.0
NO6	ϕ21.8 mm ACC	90	0	200	223.3	1406.3	10.0
NO7	ϕ21.8 mm Anchor cable	90	3	200	216.1	1300.0	7.9
NO8	ϕ21.8 mm ACC	90	3	200	201.8	1604.0	9.0
NO9	ϕ21.8 mm ACC	90	9	200	219.5	2531.8	8.2

4. Analysis of Test Results

4.1. Failure Characteristics of Supporting Components

Taking the test results of test 7 and test 8 as examples, the failure characteristics of the 21.8 mm anchor cable and 21.8 mm ACC are analyzed, respectively. The failure photos are shown in Figure 4.

Figure 4 shows that the failure fracture of the 21.8 mm anchor cable is mainly the oblique shear fracture, while the failure form of the anchor cable fracture in the 21.8 mm ACC under the same test conditions is not unique, including the oblique shear fracture mainly bearing the shear force and the conical fracture with an obvious necking phenomenon mainly bearing the tensile force. However, the overall fracture is mainly a tensile fracture, while the C-shaped tube faces the tensile fracture.

4.2. Failure Characteristics of the Joint Surface

Figure 5 shows the failure photos of the joint surface of test 8 after the test. It can be seen that the block presents horn-shaped damage at the orifice due to the extrusion of the supporting components. There is a “+”-shaped crack on both sides of the joint surface of the middle block starting from the orifice, and the cracks on the joint surface of the blocks on both sides expand toward the direction of gravity. Figure 5c shows the photos of the intermediate blocks left in the shear box, and Figure 5b shows the photos of the intermediate blocks taken out of the shear box. It can be seen that the cracks are small and not completely continuous before the blocks are taken out of the shear box. The shear box effectively inhibits the damage to the concrete blocks and avoids the exhaustion of the bearing capacity of the blocks before the supporting components are damaged. In addition, observing the four joint planes of the block, we found that except for obvious deformation and damage near the hole, other positions of the joint plane show good flatness, with the top of the block especially showing no trace of being sheared, which proves that the stiffness and strength of the shear box are sufficient, and the transverse size design is reasonable.

4.3. Internal Friction Angle and Cohesion

According to the shear mechanical behavior of the anchored rock mass in Section 2, the shear strength of the joint surface meets the Mohr–Coulomb criterion. It is known that the joint surface in the double shear test is the contact surface of two concrete blocks. It should be considered that the joint surface at this time has no cohesion, i.e., c = 0. According to the shear force that just overcomes the friction resistance of the joint surface during the double shear test (i.e., the maximum shear force when the shear surface is in the sliding process, but the concrete block does not produce a shear effect on the supporting component), combined with the axial force at this time, the normal stress and shear stress acting on the shear surface at this time can be calculated. Finally, the normal stress and shear stress that just overcome the friction resistance of the joint surface in each group of tests are linearly fitted according to Formula (1), as shown in Figure 6.

It can be concluded that the constitutive relation of the joint surface approximately meets:

$$\tau =0.67814{\sigma }_{\mathrm{n}}{\mathrm{R}}^2=0.99$$

(9)

Moreover, according to the inverse trigonometric function, the friction angle in the joint plane is 34°.

4.4. Shear-Bearing Capacity of the Joint Surface

Figure 7 shows the shear displacement shear force and shear displacement axial force curves of test 1 (an anchor cable) and test 2 (ACC).

Figure 7 shows that the shear-bearing capacity of the joint plane anchored by ACC is significantly higher than that of the anchor cable, because:

(1)

During the shear process of the joint plane, while the anchor cable has its bearing capacity, the C-shaped tube, as a part of the tube cable’s composite structure, not only gives full play to its shear performance but also effectively reduces the occurrence of stress concentration near the joint plane and plays a protective role for the anchor cable.

(2)

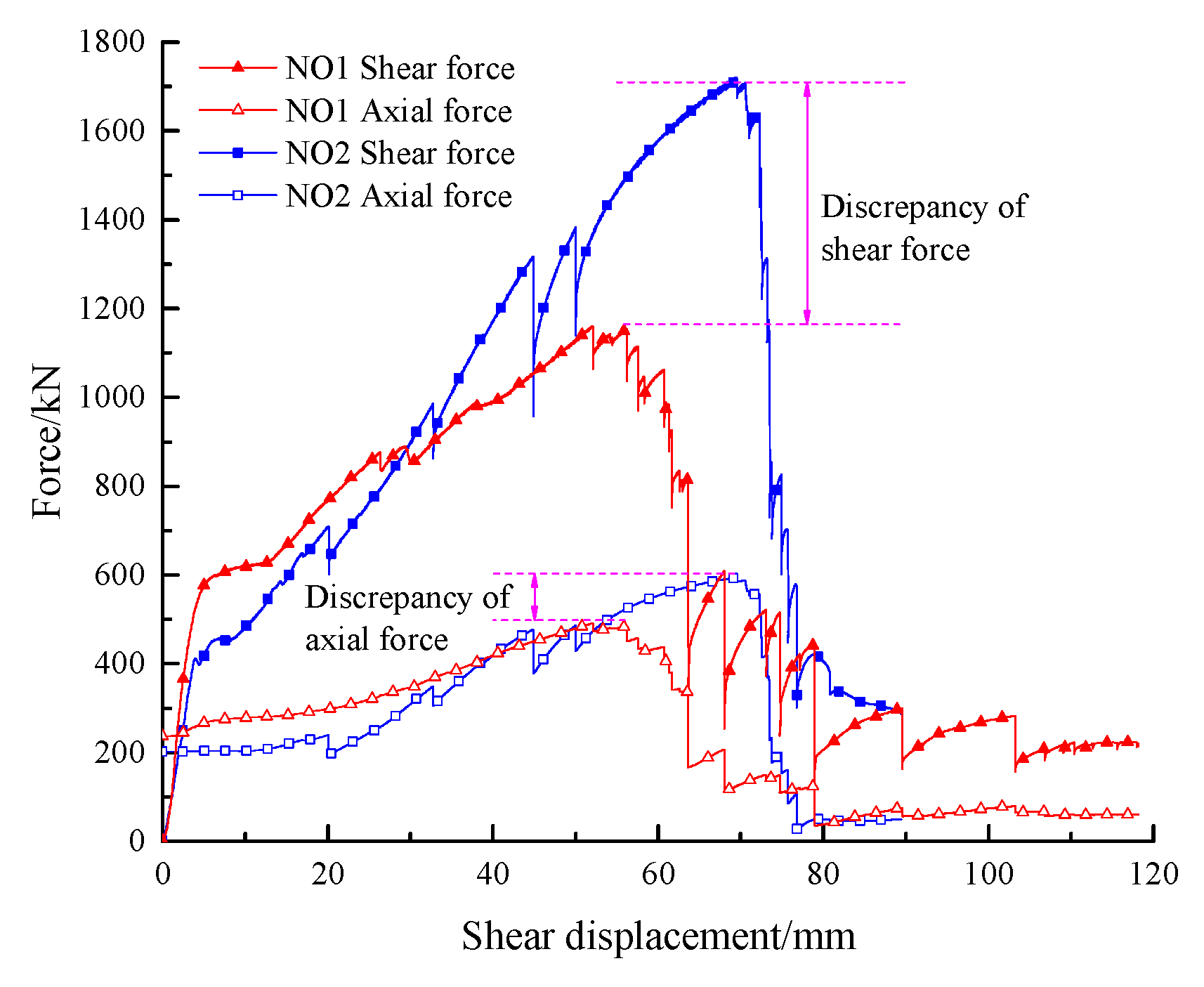
After the C-shaped tube holds and wraps the anchor cable through the friction between the C-shaped tube and the anchor cable, the mechanical properties of the anchor cable in the original state are improved, making the anchor cable gradually transition from tensile shear failure to tensile failure. This is also proved by the shear displacement axial force curve, as shown in Figure 7. It is also consistent with the fracture failure characteristics of the anchor cable and ACC in Figure 4 in Section 3.1.

Figure 8 shows the shear displacement shear force curves of the anchor cable and ACC at angles $\alpha$ of 90° and 80° to the joint surface, respectively.

Figure 8 shows that whether the included angle $\alpha$ between the support member and the joint surface is 90° or 80°, the shear-bearing capacity of the joint surface anchored by ACC is higher than that of the anchor cable. In addition, comparing test 2 and test 4, we found that the shear-bearing capacity of the joint surface at an angle of 90° between ACC and the joint surface is higher than that at an angle of 80°.

Figure 9 shows the shear capacity of the joint surface of ACC under different initial normal stresses. It can be seen that the shear capacity FS of the joint surface anchored by ACC is approximately linear with the initial normal stress, and the shear capacity of the joint surface increases with the increase in the initial normal stress:

$$F_{\mathrm{s}}=121.42{\sigma }_{\mathrm{n}}+1405.6{\mathrm{R}}^2=0.91$$

(10)

Among them, the shear-bearing capacity of the joint surface when the initial normal stress is 1.5 MPa is greater than that when the initial normal stress is 3 MPa. We believe that the reason is that although the 21.8 mm anchor cable is produced by the same manufacturer, due to production technology problems, there are still differences in the performance of the same batch of anchor cables from the same model. The anchor cable in ACC that just carried out the double shear test when the initial normal stress is 1.5 MPa has excellent performance. The performance of the anchor cable in ACC is poor when the initial normal stress is 3 MPa, but it still does not affect the trend that the shear-bearing capacity of the joint surface changes with the change in the initial normal stress.

4.5. Shear Displacement

Compared with the anchor cable in the anchorage section, there is a gap between the anchor cable in the free section and the hole. In addition, the local expansion of the crack will also cause the fluctuation in the shear force, which first decreases and then increases during the test. This paper believes that the influence of these factors should be eliminated when analyzing the shear displacement, and the concept of effective shear displacement should be used for analysis. Only the shear displacement generated when the joint has an effective mechanical effect on the supporting component should be studied. Figure 10 shows the effective shear displacement of the anchor cable and ACC at different initial normal stresses.

It can be seen from Figure 10 that ACC can improve the ductility of the anchor cable in the shear direction to a certain extent. When the initial normal stress is 1.5 MPa and 3 MPa, the effective shear displacement of the anchor cable is 32.8 mm and 44.5 mm, respectively, and the effective shear displacement of ACC is 53.3 mm and 56.2 mm, respectively. The effective shear displacement of ACC is 20.5 mm and 11.7 mm higher than that of the anchor cable under the same test conditions, with growth rates of 62.5% and 26.3%, respectively. The effective shear displacement increased by 16.1 mm, with an average growth rate of 44.4%. We concluded that the C-shaped tube not only plays the role of shear resistance, but also protects the anchor cable, reduces the occurrence of stress concentration near the joint surface, improves the stress state of the anchor cable through the friction with the anchor cable and fully mobilizes the ductility of the anchor cable in the shear direction.

5. Discussion

5.1. Shear Stiffness

Stiffness is a parameter used to measure the ability of a structure or material to resist elastic deformation under stress. It is usually used to characterize the difficulty of a structure or material in elastic deformation. In this paper, the shear force required by the joint surface to produce the unit of effective shear displacement is defined as the shear stiffness of the joint surface. Because the shear stiffness of the joint surface changes continuously during the shear process, to facilitate comparative analysis, this paper calculates the average shear stiffness from the time when the joint surface overcomes the friction resistance to the time when the initial fracture occurs, and the calculation results are listed in Table 1.

It can be seen from Table 1 that with the increase in the initial normal stress, the average shear stiffness of both the anchor cable and ACC fluctuates randomly within a certain range without obvious rules. Therefore, it can be considered that when the initial normal stress is within the range of 0–9 MPa, the anchoring effect of the support member on the joint surface is the dominant factor affecting the shear stiffness. The anchor cable and ACC under the same test conditions are divided into the control group. When the angle between the support member and the joint surface is 90°, the average shear stiffness of ACC is 43.4%, 0.1%, 14.8% and 19.4% greater than the anchor cable under the initial normal stress of 0 MPA, 1.5 MPa and 3 MPa, respectively; when the angle between the support member and the joint surface is 80° and the initial normal stress is 1.5 MPa, the average shear stiffness of ACC is 36.9% greater than that of the anchor cable. Therefore, it can be concluded that with the support of a C-shaped tube, the shear stiffness of ACC is greater than that of the anchor cable, and the ability to resist shear deformation is significantly improved.

5.2. Increase in Shear Capacity

Compared with the anchor cable, ACC is a load-bearing structure, so it is of great significance to study the contribution of ACC to the shear-bearing capacity of the joint surface. To better understand the shear performance of ACC, the author compares the test data of Tong et al. [25] with the test data in the current paper. When the preload is 100 kN and the joint surface has no friction, the shear capacity of the joint surface of the 21.8 mm ACC is 955.5 kN, while under the condition of having the friction and initial normal stress of the joint surface, when the preload is 200 kN, the shear capacity of the joint surface anchored by the 21.8 mm ACC is 1719.8 kN. We found that the presence or absence of friction on the joint surface greatly influences the test results. The shear-bearing capacity of the joint surface without friction is much smaller than that with friction. Therefore, this paper compares the contribution of the anchor cable and ACC to the shear-bearing capacity of a joint surface from two different angles: one is to compare the “pin effect” of support components, and the other is to compare the shear-bearing capacity of the joint surface. L. Li, Shan Renliang, et al. [21,24,25] respectively used various methods to restrain the axial force of the support member from transmitting to the joint surface to study the contribution of the mechanical properties of the support member to the shear-bearing capacity of the joint surface. Therefore, this paper divides the shear-bearing capacity of the joint surface into two parts: (1) the initial normal stress and the normal stress exerted by the axial force of the support member on the joint surface provide the shear-bearing capacity in the form of friction reaction. (2) The force parallel to the joint surface the support member provides for resisting the shear deformation, i.e., the “dowel effect”. For the convenience of distinguishing between them, the shear-bearing capacity considering only the “dowel effect” of the supporting component is named “shear-bearing capacity of the supporting component”. Group the tests and calculate the bearing capacity of the supporting components caused by the “dowel effect” according to the internal friction angle fitted in Section 4.3.

Table 2. Calculation of shear capacity.

Test Number	Support Component	Test Group	SBC-JS kN	Increase in SBC-JS kN	Percentage of the Increase in SBC-JS %	SBC-SC kN	Increase in SBC-SC kN	Percentage of the Increase in SBC-SC %
NO1	ϕ21.8 mm Anchor cable	A	1159.5	560.3	48.3	745.6	501.0	67.2
NO2	ϕ21.8 mmACC		1719.8			1246.5
NO3	ϕ21.8 mm Anchor cable	B	1341.7	216.1	16.1	949.4	191.2	20.1
NO4	ϕ21.8 mm ACC		1557.7			1140.6
NO5	ϕ21.8 mm Anchor cable	C	1352.8	53.5	4.0	955.4	122.5	12.8
NO6	ϕ21.8 mm ACC		1406.3			1077.9
NO7	ϕ21.8 mm Anchor cable	D	1299.9	304.1	23.4	866.0	213.3	24.6
NO8	ϕ21.8 mm ACC		1604.0			1079.4

SBC-JS: Shear bearing capacity of the joint surface. SBC-SC: Shear bearing capacity of supporting components.

shows the calculation results and relevant important data.

5.2.1. Interaction between Supporting Components and Joint Surface

The anchor cable and ACC will produce tensile deformation in the shear process. When the anchor cable is stretched, its axial force will increase, which will exert greater normal stress on the joint surface and inhibit the slip of the joint surface. This stress condition is also consistent with the anchor cable and ACC in the actual project. Therefore, when comparing the control effect of the ACC ratio to the anchor cable on the shear deformation of the joint surface, one of the simplest methods is to directly make a difference and calculate the difference in the shear-bearing capacity of the joint surface.

Table 2 shows that when the included angle between the support member and the joint surface is 90°, under different initial normal stress conditions compared with the anchor cable under the same test conditions, the shear-bearing capacity of the joint surface of group A, C and D is increased by 560.3 kN, 53.5 kN and 304.1 kN, respectively, with an average increase of 306 kN. The lifting rates are 48.3%, 4.0% and 23.4%, respectively, and the average increase range is 25.2%.

5.2.2. Dowel Effect

It can be seen from Table 2 that when the included angle between the supporting component and the joint surface is 90°, under different initial normal stress conditions compared with the anchor cable under the same test conditions, the bearing capacity of the supporting component of ACC in group A, C and D is increased by 501.0 kN, 122.5 kN and 213.3 kN, respectively, with an average increase of 279 kN. The lifting rates are 67.2%, 12.8% and 24.6%, respectively, with an average increase of 34.9%.

Compared with the results in Section 5.2.1, it can be seen that the average increase in the shear capacity of the supporting components only considering the “dowel effect” is less than the average increase in the shear capacity of the joint surface. However, the increase in the bearing capacity of the supporting components is higher than the maximum shear force. Herein, we considered that when comparing the contribution of the properties of the anchor cable and ACC to the shear capacity of the joint surface, it is recommended to analyze the bearing capacity of the supporting component only by considering the “pin effect”, and when comparing the control effects of the anchor cable and ACC on the shear deformation of the joint surface, it is recommended to analyze the shear capacity of the joint surface.

6. Conclusions

In this paper, the tensile and shear properties of the prestressed anchor cable and ACC in a free section are studied using theoretical analysis and a double shear test, and the test equipment is improved according to the shortcomings of the existing double shear test. On the one hand, the initial normal stress is included in the study. The initial normal stress is applied to the joint surface through the horizontal loading sleeve, and the initial normal stress is kept unchanged during the test. On the other hand, the size of the shear box is modified to avoid the shear box cutting the block in the test process, resulting in the test error. Several groups of comparative tests were carried out with the improved double shear test equipment. After the test, the deformation and failure characteristics of the support members were observed and recorded. The test results analyzed the effects of the initial normal stress and included angle on the shear resistance. The main research conclusions are as follows:

(1)

The fracture failure mode of the anchor cable is mainly an oblique shear fracture, and the fracture failure mode of the anchor cable in ACC is mainly a conical fracture with a necking phenomenon under tension. The joint surfaces on both sides of the middle block have “+”-shaped cracks starting from the orifice, respectively, and the cracks on the joint surfaces of the blocks on both sides expand in the direction of gravity, respectively.

(2)

The C-shaped tube not only bears the load itself but also protects the anchor cable, effectively reduces the occurrence of stress concentration near the joint surface, improves the stress state of the anchor cable through the friction between the C-shaped tube and the anchor cable, maximizes the tensile performance of the anchor cable and makes the failure mode of the anchor cable transition from tensile shear failure to tensile failure.

(3)

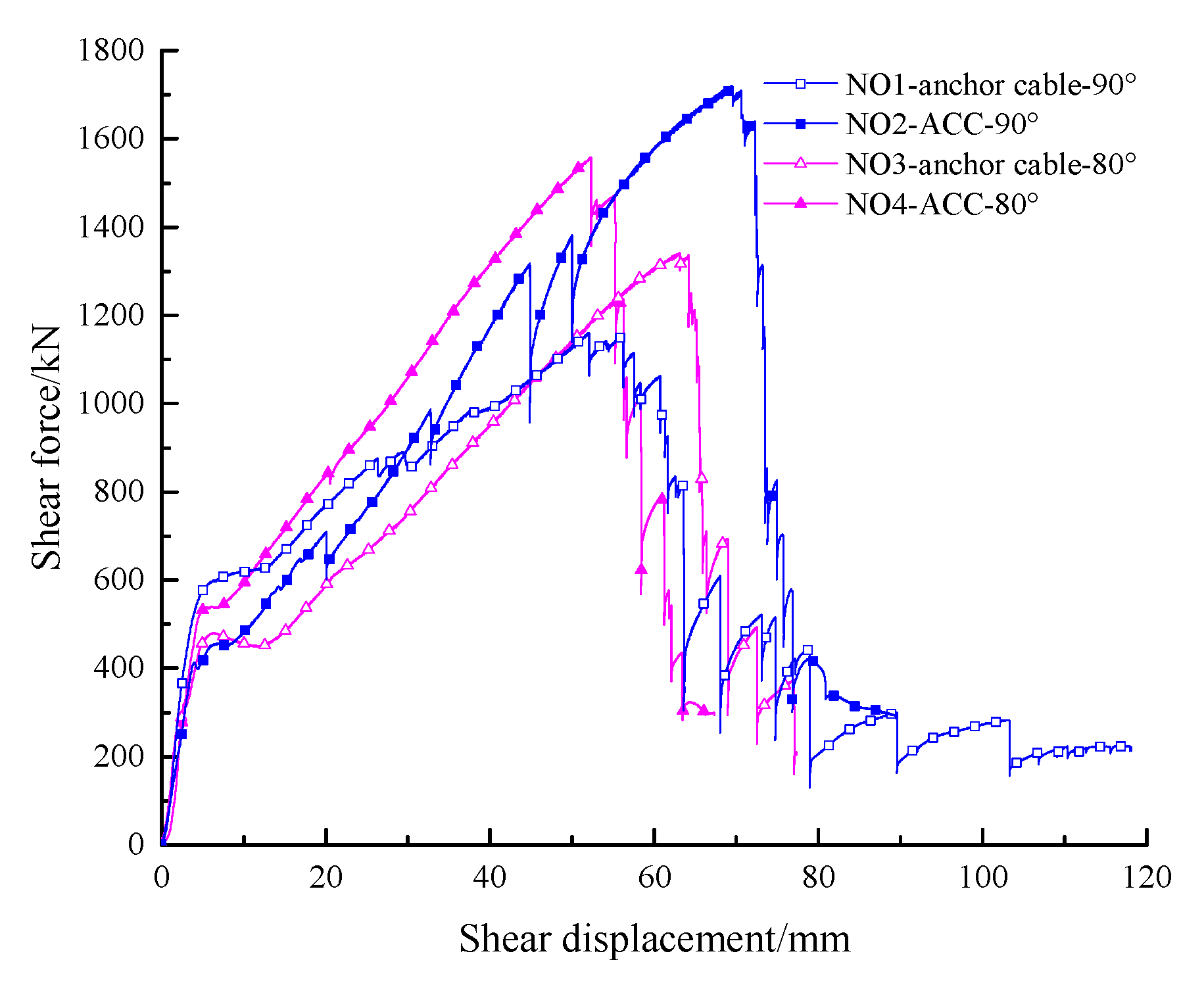
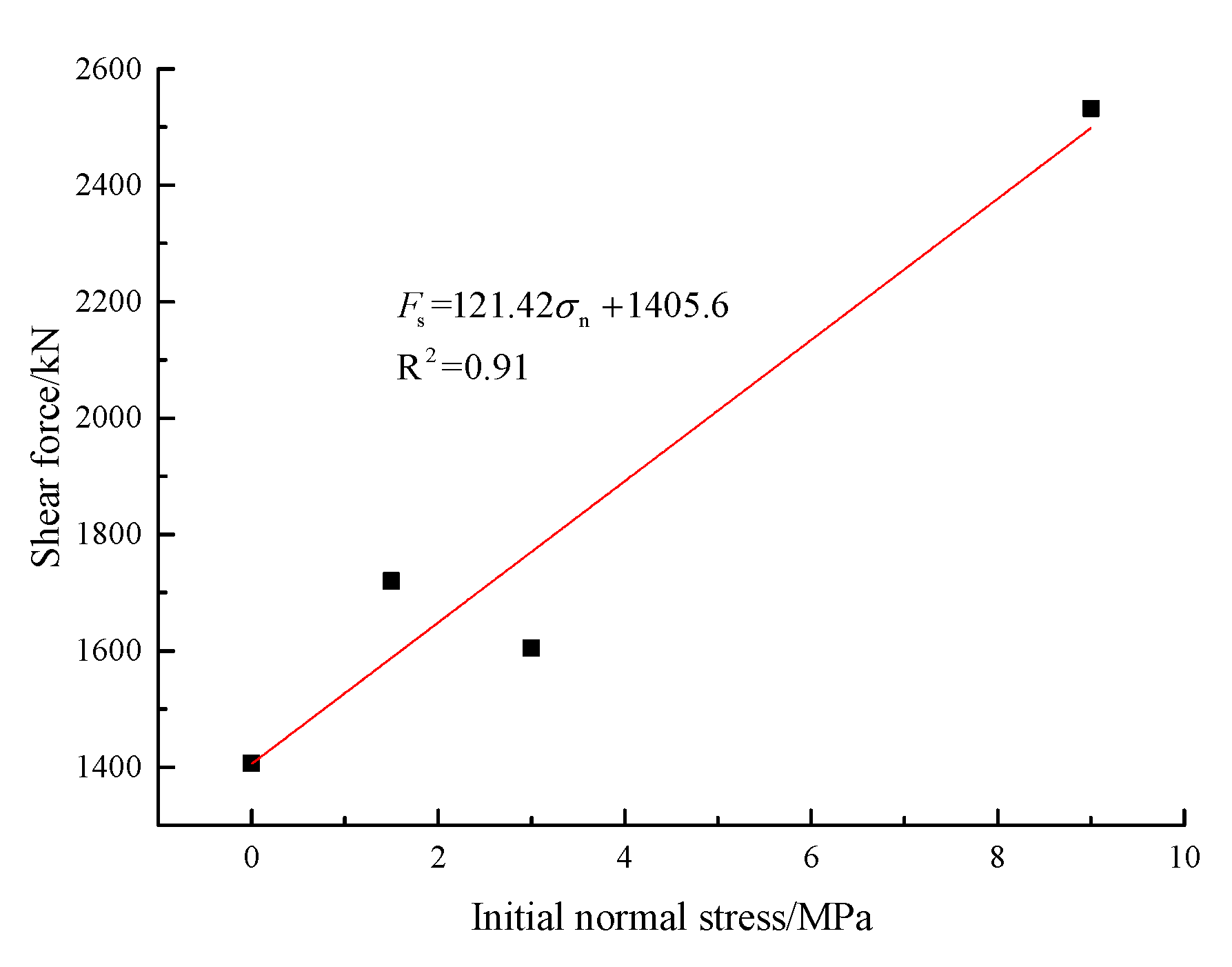
Compared with the anchor cable, ACC has a higher shear capacity, greater shear stiffness and higher ductility in the shear direction. When ACC is perpendicular to the joint plane, the shear capacity of the joint surface is higher than when it is inclined and linear with the initial normal stress.

(4)

Two methods are proposed to evaluate the increased range of the ACC shear capacity. One is the joint plane shear capacity method considering the interaction between the support member and the joint plane. The other is the support member bearing capacity method considering only the “dowel effect” of the support member. Compared with the anchor cable, the average increase in the shear capacity of the joint surface of ACC is 306 kN, which is greater than the average increase in the shear capacity of the supporting components by 279 kN. However, the average increase in the shear capacity of the joint surface is only 25.2%, which is less than the average increase in the shear capacity of the supporting components by 34.9%. When comparing the shear-bearing capacity of the anchor cable and ACC, it is suggested that the shear-bearing capacity of support components should be used to evaluate the ability of support components to resist shear deformation. The shear-bearing capacity of the joint surface should be used to evaluate the control effect of support components on the joint surface’s shear deformation.