Strength Variation of Rocks Surrounding Road Tunnel Entrance/Exit in High–Altitude Mountain Areas under Freeze–Thaw Cycles
Abstract
:1. Introduction
1.1. Studies of High-Altitude Mountain Areas
1.2. Studies of Freezing–Thawing Cycles and Rocks
2. Materials and Methods
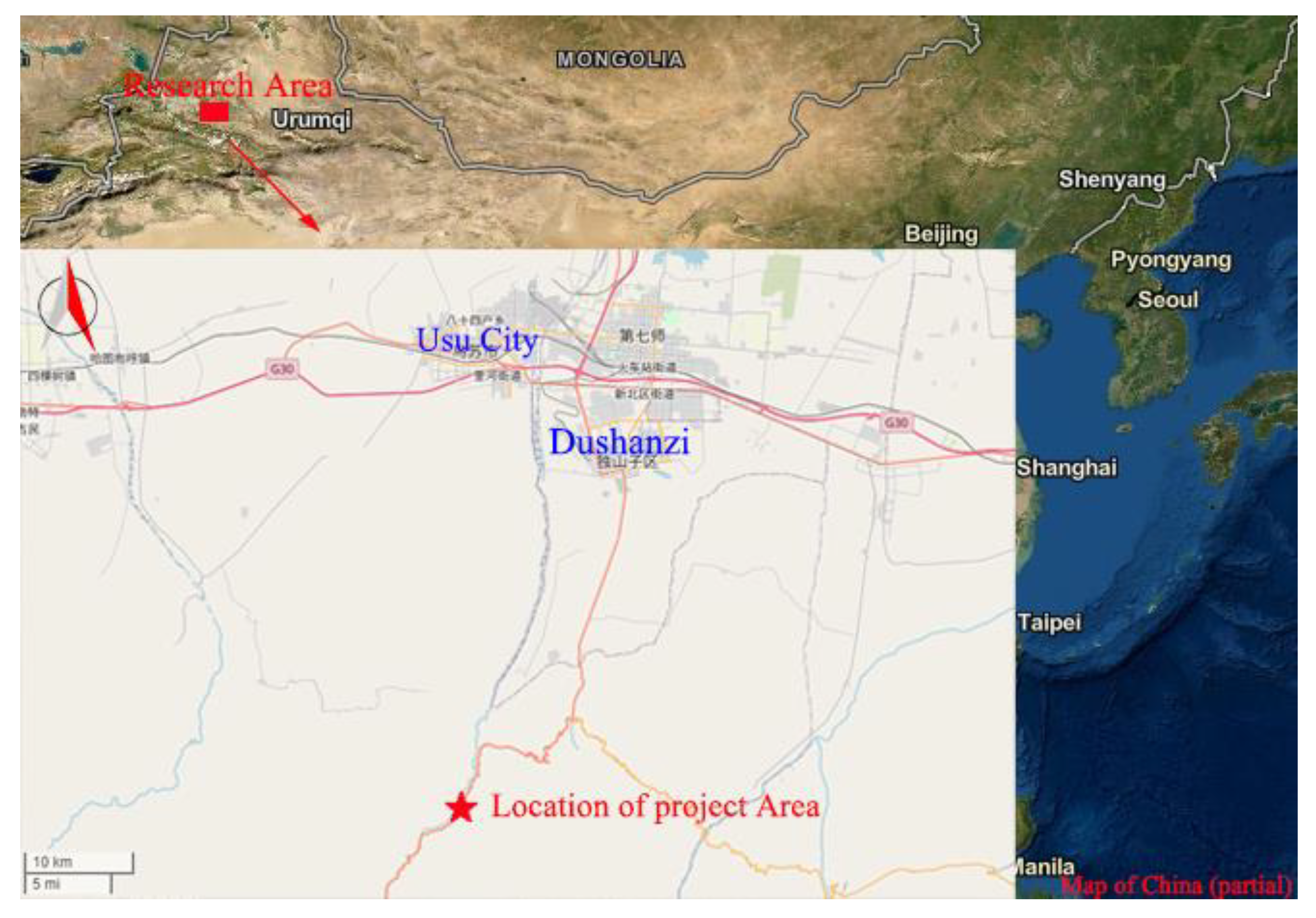
2.1. Selection of Study Area
2.2. Sample Selection and Preparation
2.3. Specimen Characteristics
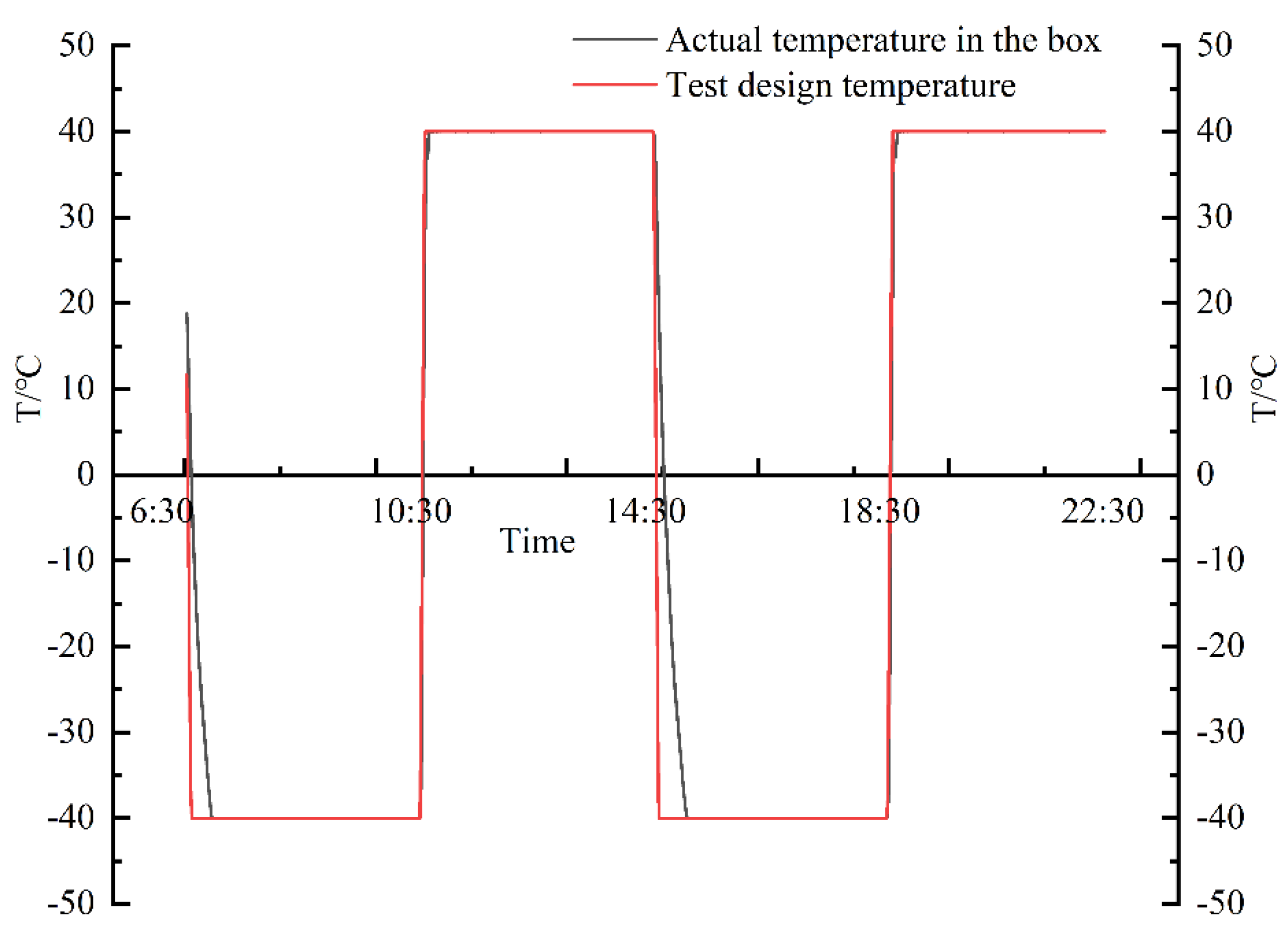
2.4. Experimental Design
2.5. Procedures
2.5.1. Sample Grouping
2.5.2. Freeze–Thaw Test
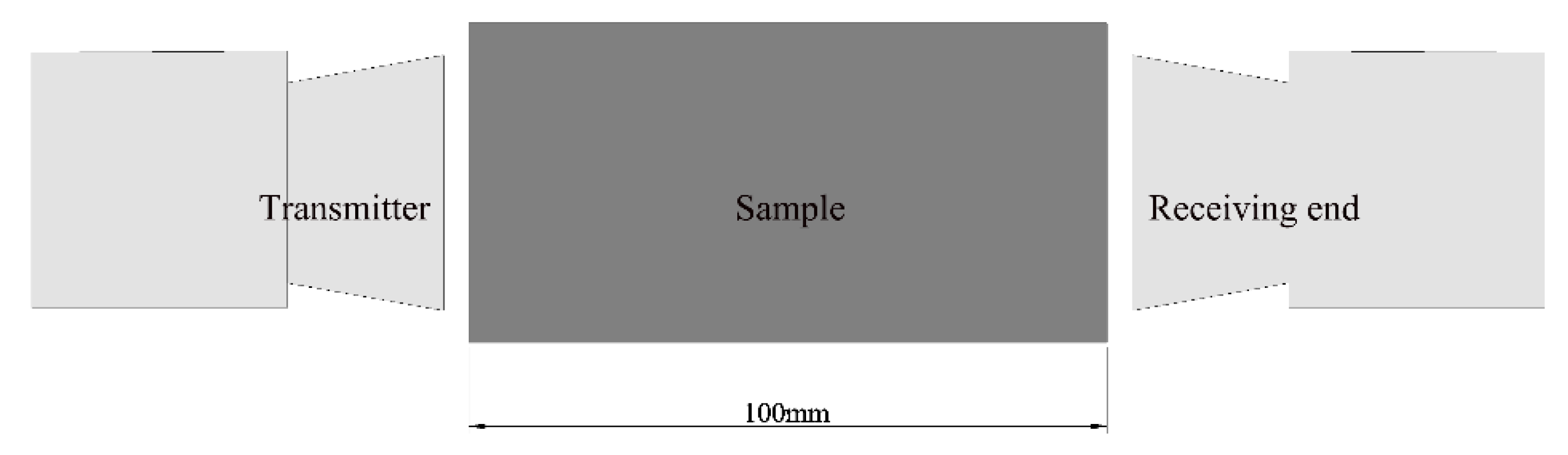
2.5.3. Shear Wave Velocity Test
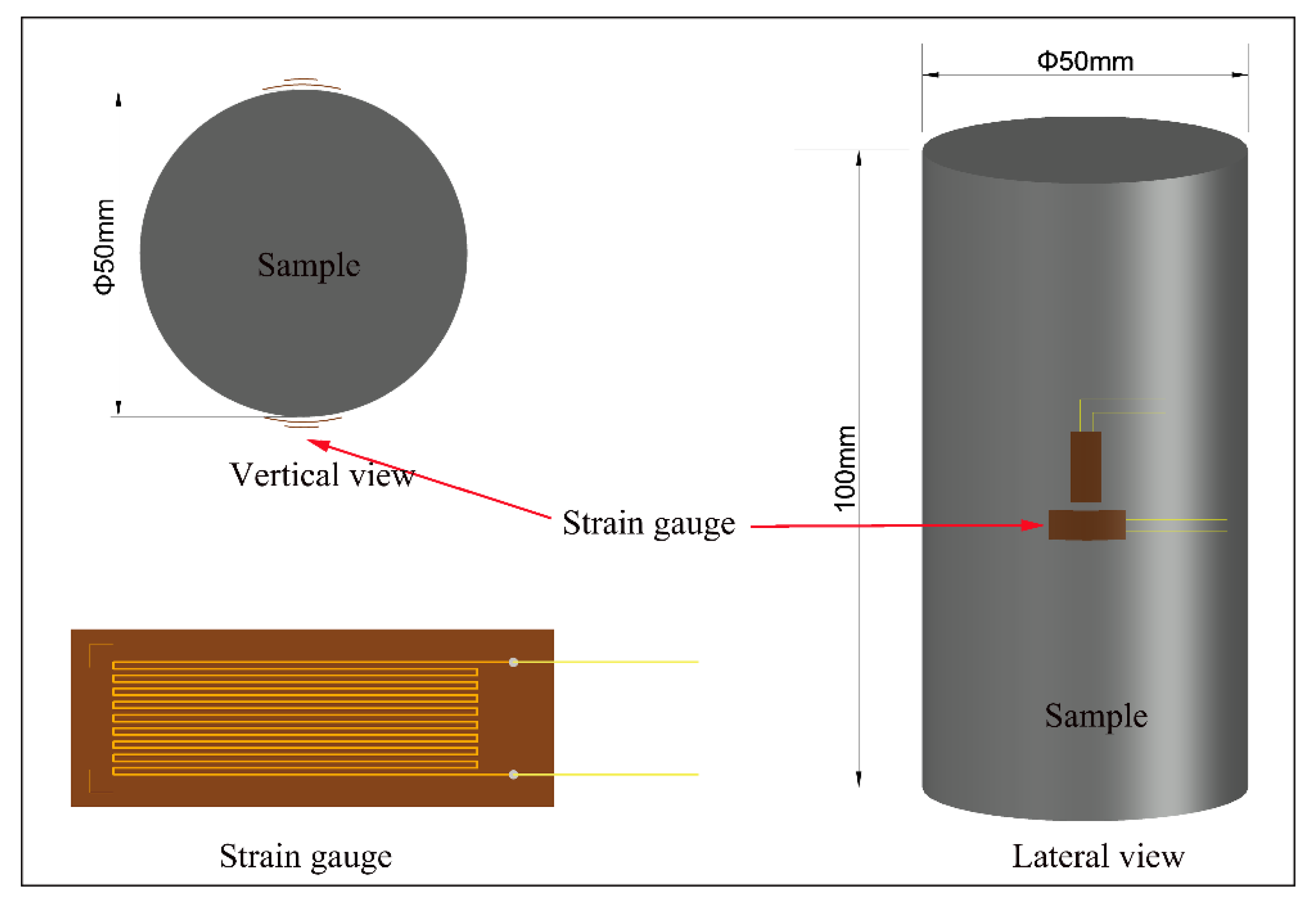
2.5.4. Uniaxial Compressive Strength Test
3. Results and Analysis
3.1. Analysis of Apparent Phenomena of Freeze–Thaw Testing
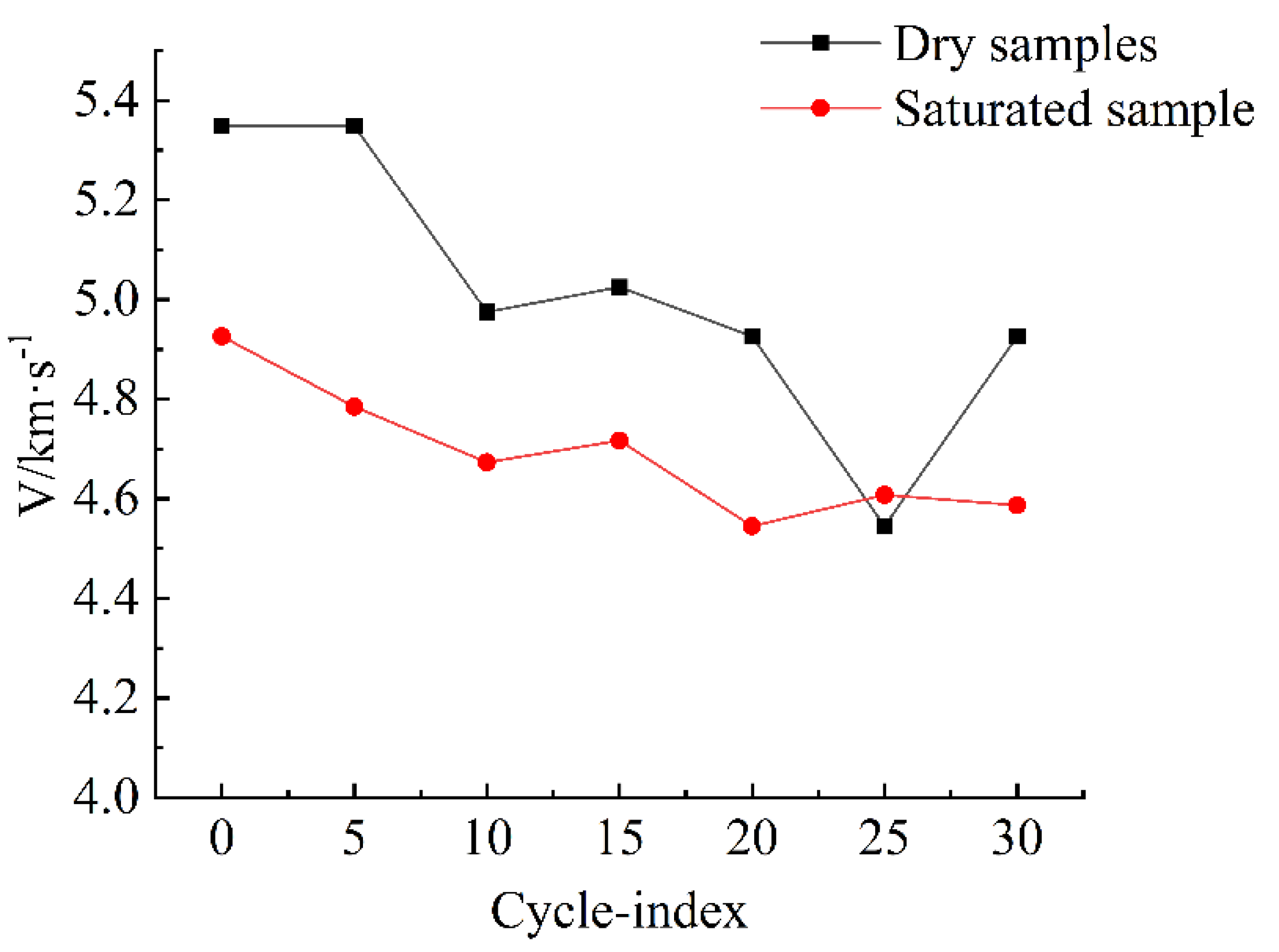
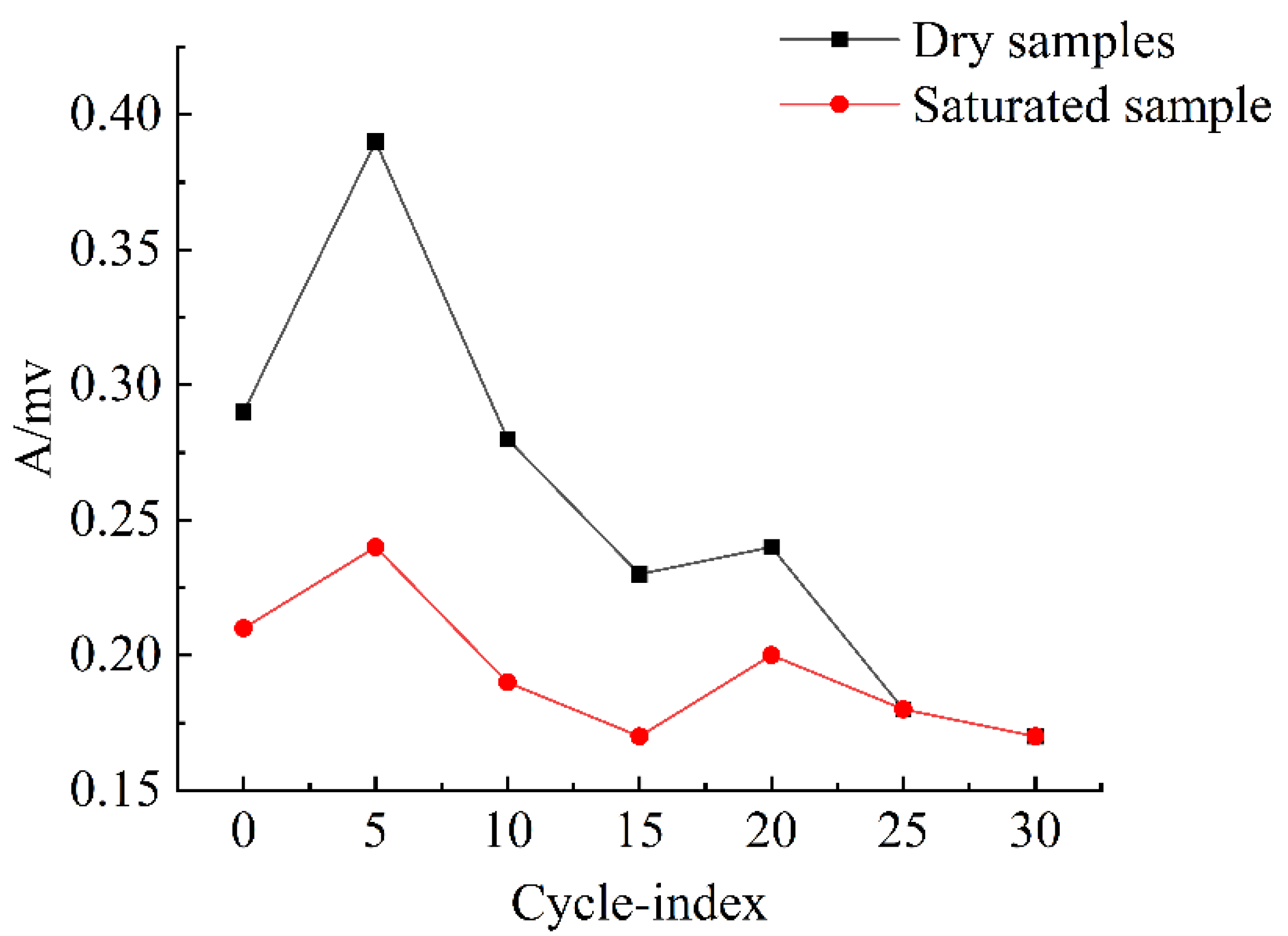
3.2. Shear Wave Velocity Test
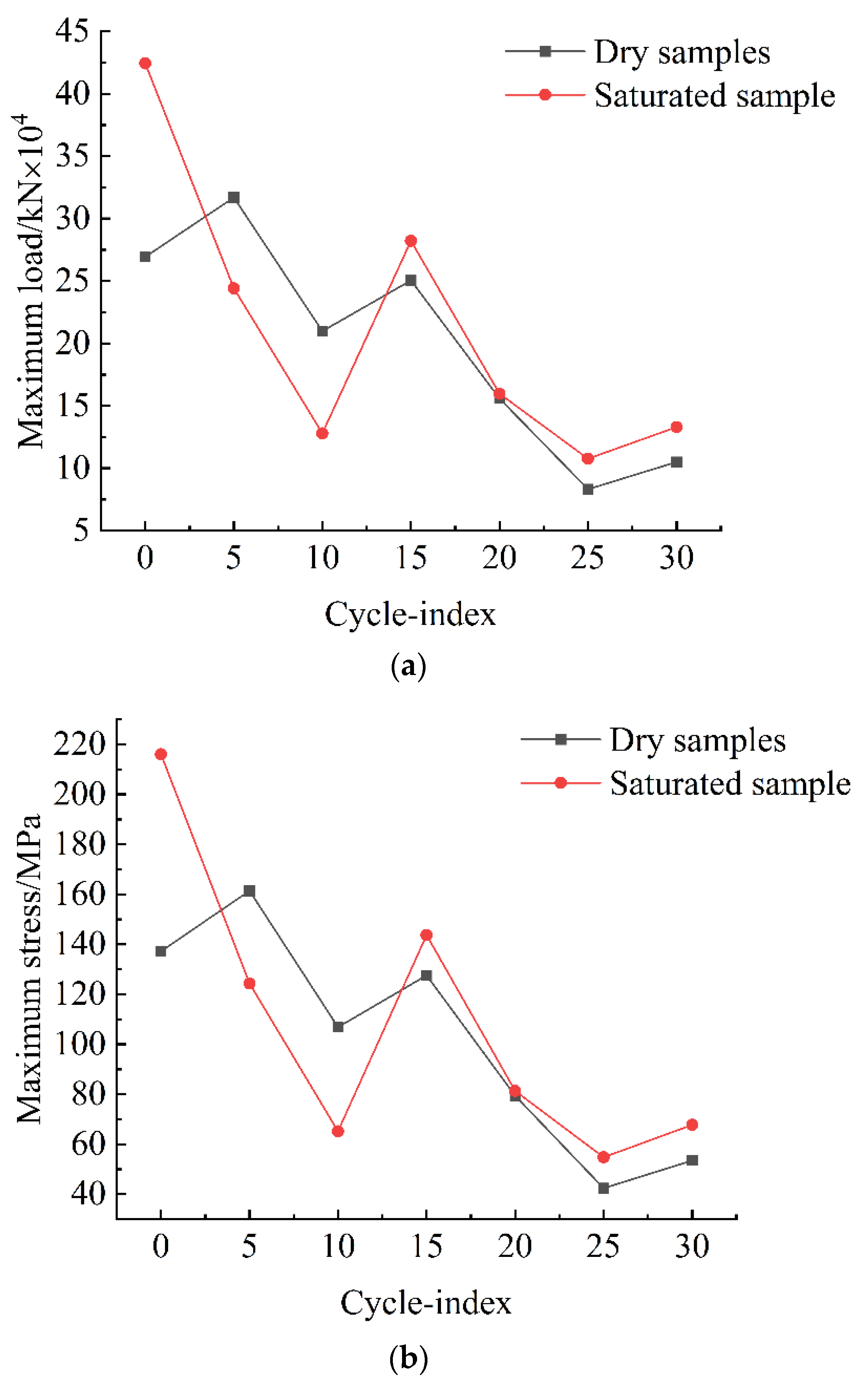
3.3. Uniaxial Compressive Strength Test
3.4. Summary and Analysis of Test Data
4. Conclusions and Discussion for Further Research
4.1. Conclusions
- (1)
- When the temperature was between −40 °C and 40 °C, the rock temperature had a rate of increase 2.57 times greater than that of decrease.
- (2)
- The shear wave velocity of two rock groups decreased as the number of freeze–thaw cycles grew. Meanwhile, the samples’ uniaxial compressive strength, elastic modulus, Poisson’s ratio, secant modulus, and secant Poisson’s ratio showed a reduction.
- (3)
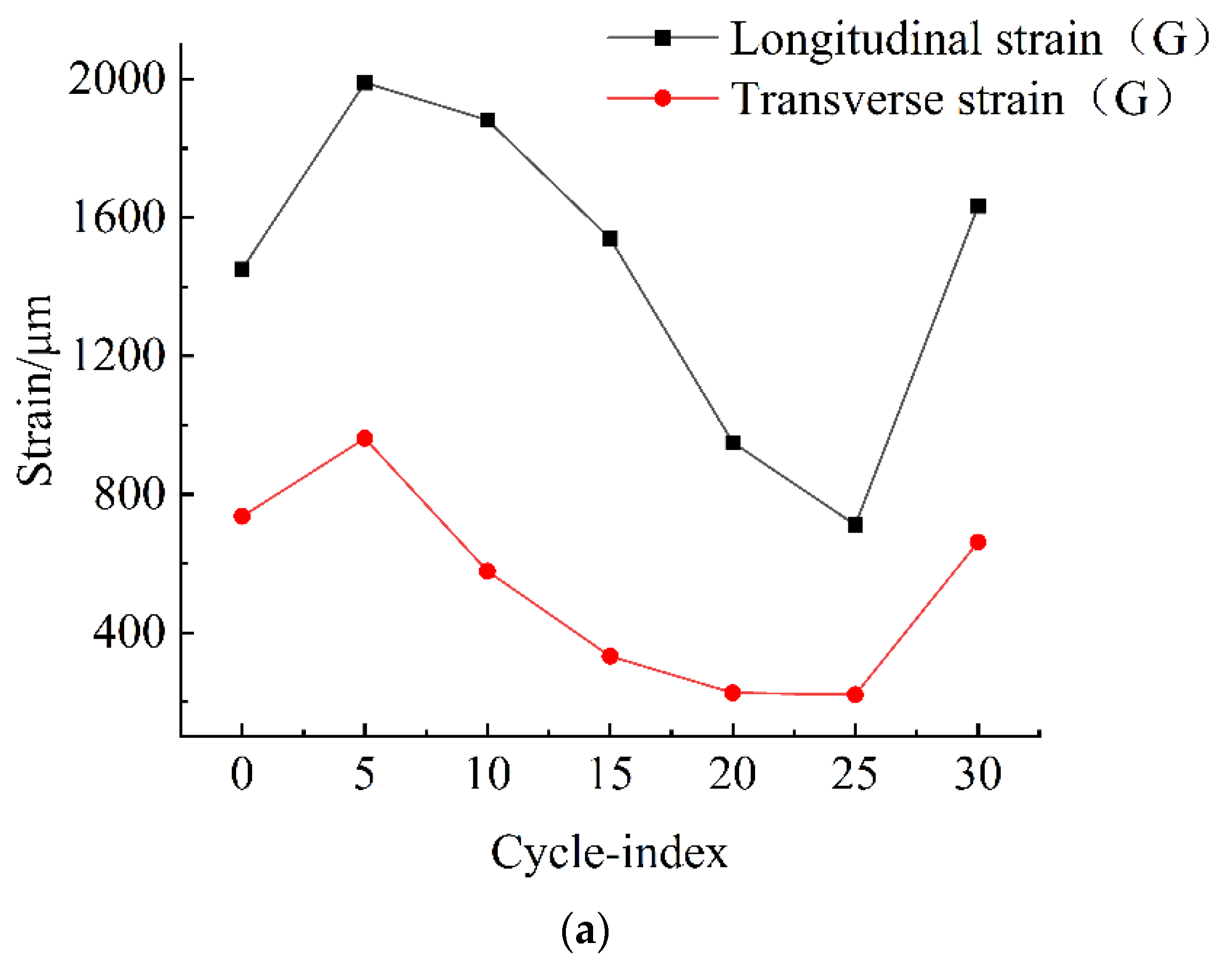
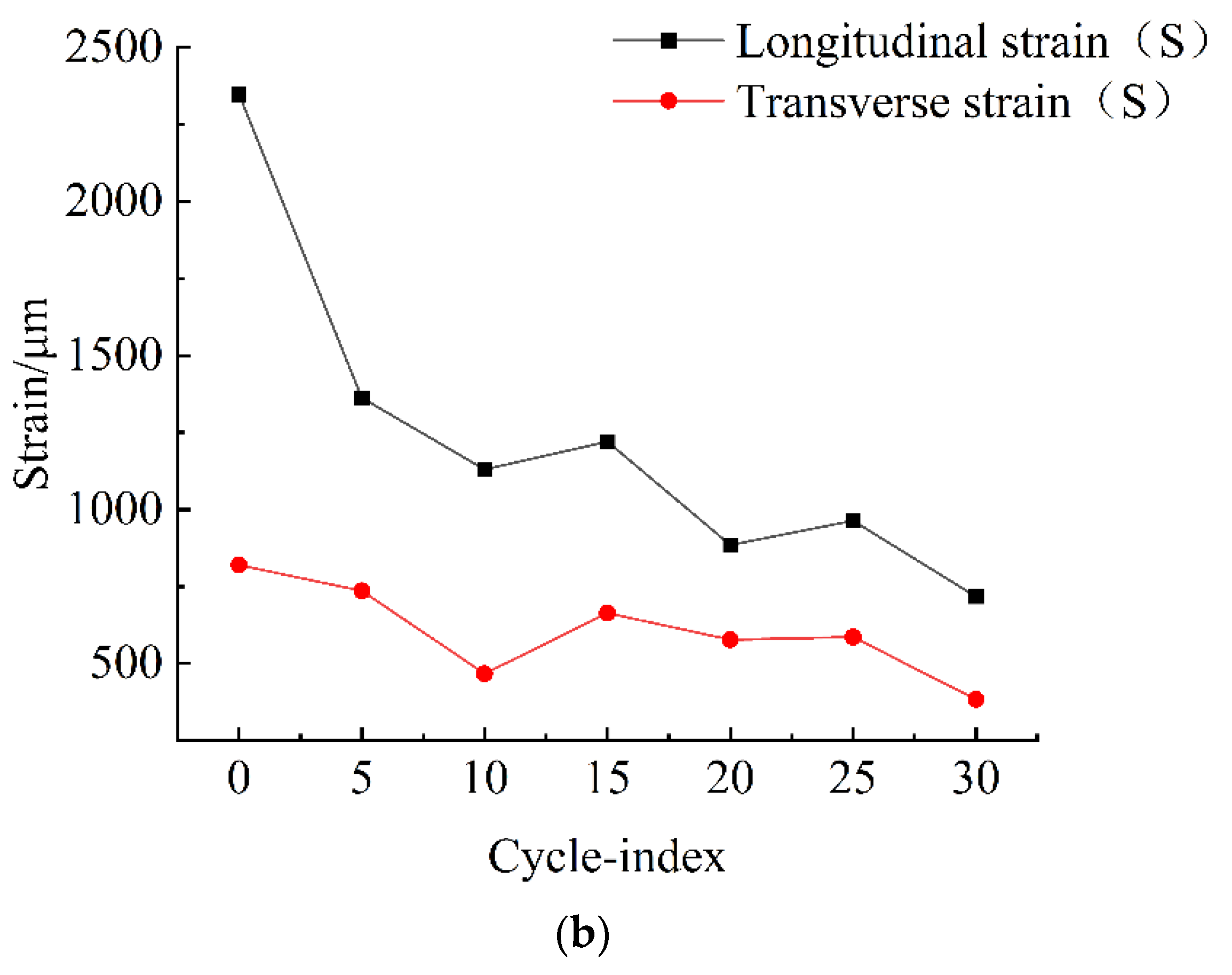
- Transverse strain of the two groups was more petite than the longitudinal strain, while the longitudinal strain of the dry sample was more significant than the immersion-saturated sample. Furthermore, it showed a trend of first increasing, then decreasing, and then increasing. On the other hand, longitudinal and transverse strains of the immersion-saturated sample showed a decreasing trend when it failed.
- (4)
- As the number of freeze–thaw cycles increased, the wave velocity of rock gradually decreased, and all strength parameters of rock also decayed. However, the influence of freeze–thaw cycling on the strength of the surrounding rock also had a specific limit. When the influence limit of freeze–thaw cycling was exceeded, the strength parameters of the surrounding rock would no longer change with the increase in the number of freeze–thaw cycles.
- (5)
- We believe that freeze–thaw cycles lead to deterioration of tuff strength. The reason for this phenomenon is that we believe that the freeze–thaw cycling action changes the rock internally, affecting its density, which, in turn, causes a decay in strength, etc.
4.2. Discussion for Future Research
- (1)
- After how many freeze–thaw cycles will the strength parameters of rock no longer have large fluctuations?
- (2)
- Strength parameters of the surrounding rock will be stable after reaching the influence limit of freeze–thaw cycling. What is the relationship between the value and the initial index of surrounding rock strength parameters of surrounding rocks?
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tian, J.; Yang, G.; Liu, H. Study on the freezing damage mechanism and its prevention in cold region rock tunnel. Chin. J. Undergr. Space Eng. 2007, 3 (Suppl. S2), 1484–1489. [Google Scholar]
- Lai, Y.; Wu, H.; Wu, Z. Analytical viscoelastic solution for frost force in cold-region tunnels. Cold Reg. Sci. Technol. 2000, 31, 227–234. [Google Scholar] [CrossRef]
- Li, G.; Huang, Y. Study on viscous debris flow rate of high altitude alpine highway in Xinjiang. Sci. Technol. Inf. 2008, 27, 50–76. [Google Scholar]
- Huang, Y.; Yang, S.; Zhou, Y.; Chen, H. Research on prevention and control of slope debris flow on alpine high altitude mountain roads. Resour. Environ. Eng. 2009, 23 (Suppl. S1), 107–110. [Google Scholar]
- Geng, W. Prevention and control measures of salivary ice disease on high altitude cold mountain roads. Build. Mater. Decor. 2018, 17, 222–223. [Google Scholar]
- Hu, Y. Evaluation of Geological Hazards of Mountain Roads. Master’s Thesis, Xi’an University of Science and Technology, Xi’an, China, 2017. [Google Scholar]
- Zhang, Z.; Lin, L. Key points of roadbed design in alpine and high-altitude snow-affected areas. Eng. Constr. 2016, 30, 473–475. [Google Scholar]
- He, E.; Li, S.; He, Y. Safety evaluation and guarantee measures for alpine high altitude mountain roads. Highw. Transp. Technol. (Appl. Technol. Ed.) 2016, 12, 273–276. [Google Scholar]
- Yao, X. Research on Crack Resistance of Semi-Rigid Subgrade for High Altitude Mountain Roads. Master’s Thesis, Chongqing Jiaotong University, Chongqing, China, 2017. [Google Scholar]
- Yavuz, H.; Altindag, R.; Sarac, S. Estimating the index properties of deteriorated carbonate rocks due to freeze-thaw and thermal shock weathering. Int. J. Rock Mech. Min. Sci. 2006, 43, 767–775. [Google Scholar] [CrossRef]
- Momeni, A.; Abdilor, Y.; Khanlari, G.R. The effect of freeze-thaw cycles on physical and mechanical properties of granitoid hard rocks. Bull. Eng. Geol. Environ. 2015, 75, 1649–1656. [Google Scholar] [CrossRef]
- Ghobadi, M.H.; Babazadeh, R. Experimental Studies on the Effects of Cyclic Freezing-Thawing, Salt Crystallization, and Thermal Shock on the Physical and Mechanical Characteristics of Selected Sandstones. Rock Mech. Rock Eng. 2014, 48, 1001–1016. [Google Scholar] [CrossRef]
- Yamabe, T.; Neaupane, K.M. Determination of some thermo-mechanical properties of Sirahama sandstone under subzero temperature condition. Int. J. Rock Mech. Min. Sci. 2001, 38, 1029–1034. [Google Scholar] [CrossRef]
- Fahey, B.D. Frost action and hydration as rock weathering mechanisms on schist: Alaboratory study. Catena 1995, 25, 7–20. [Google Scholar] [CrossRef]
- Matsuoka, N. Mechanisms of rock breakdown by frost action: An experimental approach. Cold Reg. Sci. Technol. 1990, 17, 253–270. [Google Scholar] [CrossRef]
- Liu, H.; Niu, F.; Xu, Z. Experimental study on the physical and mechanical properties of andesite and granite under cyclic freeze-thaw conditions. Glacial Permafr. 2011, 33, 231–237. [Google Scholar]
- Wan, Y. Study on Freeze-Thaw Damage Characteristics and Tunnel Stability of Quantai Tunnel. Master’s Thesis, Jilin University, Jilin, China, 2020. [Google Scholar]
- Xu, G.; Liu, Q.; Peng, W. Experimental study of basic mechanical properties of rocks under low temperature. J. Rock Mech. Eng. 2006, 25, 2502–2508. [Google Scholar]
- Xu, G.; Liu, Q. Analysis of freeze-thaw damage mechanism and freeze-thaw mechanics of rocks. J. Rock Mech. Eng. 2005, 24, 3076–3082. [Google Scholar]
- Rong, Z. Analysis and Evaluation of Stability of Rocky and Steep Slopes in Alpine Mines. Master’s Thesis, China University of Geosciences, Wuhan, China, 2010. [Google Scholar]
- Zhang, H.; Yang, Q. Experimental freeze-thaw mechanics and damage extension characteristics of rocks. J. China Univ. Min. Technol. 2011, 40, 123–126. [Google Scholar]
- Liu, C. Study on the Mechanism of Freeze-Thaw Damage in the Surrounding Rock of Houwaizi Tunnel in the Seasonal Freezing Zone. Master’s Thesis, Jilin University, Jilin, China, 2020. [Google Scholar]
- Inada, Y.; Yokota, K. Some Studies of Low Temperature Rock Strength. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1984, 21, 145–153. [Google Scholar] [CrossRef]
- Tang, M.; Xu, Q.; Deng, W. Deterioration law of typical rock mechanical properties of Sichuan-Tibet Railway under freeze-thaw and loading-unloading conditions. Earth Sci. 2022, 47, 1917–1931. [Google Scholar]
- Hou, Z. Study on Freeze-Thaw Mechanical Properties of Fractured Rock and Its Stability of Mine Slope in High Altitude Cold Area. Master’s Thesis, University of Science and Technology Beijing, Beijing, China, 2022. [Google Scholar]
- Shen, X. Freeze-Thaw Cycle Characteristics and Deterioration Damage Mechanism of Slope Rock. Master’s Thesis, Changjiang Academy of Sciences, Wuhan, China, 2020. [Google Scholar]
- Wu, Y. Study on Strength, Damage and Creep Properties of Rock-Like Materials under Freeze-Thaw Cycles. Ph.D. Thesis, Qingdao University of Science and Technology, Qingdao, China, 2018. [Google Scholar]
- Jiang, S. Research on Physical and Mechanical Properties and Damage Characteristics of Freeze-Thaw Rocks in Alpine Mountains. Master’s Thesis, Chengdu University of Technology, Chengdu, China, 2016. [Google Scholar]
- Liu, H.; Zhao, Y. Theoretical calculation of freeze-swelling force in tunnel surrounding rock under freeze-thaw cycle. J. Cent. South Univ. (Nat. Sci. Ed.) 2020, 51, 1049–1058. [Google Scholar]
- Zhang, H.; Yang, Q. Study of rock damage model under coupled freeze-thaw and load. J. Rock Mech. Eng. 2010, 29, 471–476. [Google Scholar]
- Sayevand, K.; Arab, H. A fresh view on particle swarm optimization to develop a precise model for predicting rock fragmentation. Eng. Comput. 2019, 36, 533–550. [Google Scholar] [CrossRef]
- Bost, M.; Pouya, A. Stress generated by the freeze-thaw process in open cracks of rockwalls: Empirical model for tight limestone. Bull. Eng. Geol. Environ. 2017, 74, 1491–1505. [Google Scholar] [CrossRef] [Green Version]
- Ince, I.; Fener, M. A prediction model for uniaxial compressive strength of deteriorated pyroclastic rocks due to freeze-thaw cycle. J. Afr. Earth Sci. 2016, 120, 134–140. [Google Scholar] [CrossRef]
- Al-Omari, A.; Brunetaud, X.; Beck, K. Effect of thermal stress, condensation and freezing-thawing action on the degradation of stones on the Castle of Chambord, France. Environ. Earth Sci. 2013, 71, 3977–3989. [Google Scholar] [CrossRef]
- Wang, M.; Zhu, Z.; Dong, Y. Study of mixed-mode I/II fractures using single cleavage semicircle compression specimens under impacting loads. Eng. Fract. Mech. 2017, 177, 33–44. [Google Scholar] [CrossRef]
- Wang, M.; Wang, F.; Zhu, Z. Modelling of crack propagation in rocks under SHPB impacts using a damage method. Fatigue Fract. Eng. Mater. Struct. 2019, 38, 1139–1148. [Google Scholar] [CrossRef]
- Wang, F.; Wang, M.; Nezhad, M. Rock Dynamic Crack Propagation under Different Loading Rates Using Improved Single Cleavage Semi-Circle Specimen. Appl. Sci. 2019, 9, 4944. [Google Scholar] [CrossRef] [Green Version]
- Niu, C.; Zhu, Z.; Zhou, L. Study on the microscopic damage evolution and dynamic fracture properties of sandstone under freeze-thaw cycles. Cold Reg. Sci. Technol. 2021, 191, 103328. [Google Scholar] [CrossRef]
- Zhu, G.; Gui, C.; Xiong, X. Density versus longitudinal and transverse wave velocity relationships. J. Geophys. 1995, 38 (Suppl. S1), 260–264. [Google Scholar]
- Wang, R.; Ren, M.; Liu, J. Correlation study of rock wave velocity and strength parameters. Min. Res. Dev. 2021, 41, 87–91. [Google Scholar]








| Cycle-Index | 0 | 5 | 10 | 15 | 20 | 25 | 30 |
| Dry samples groups | G1 | G2 | G3 | G4 | G5 | G6 | G7 |
| Saturated sample groups | S1 | S2 | S3 | S4 | S5 | S6 | S7 |
| Cycle-Index | Group No. | T/μs | A/mv | V/km·s−1 |
|---|---|---|---|---|
| 0 | G1 | 18.7 | 0.29 | 5.348 |
| 5 | G2 | 18.7 | 0.39 | 5.348 |
| 10 | G3 | 20.1 | 0.28 | 4.975 |
| 15 | G4 | 19.9 | 0.23 | 5.025 |
| 20 | G5 | 20.3 | 0.24 | 4.926 |
| 25 | G6 | 22.0 | 0.18 | 4.545 |
| 30 | G7 | 20.3 | 0.17 | 4.926 |
| 0 | S1 | 20.3 | 0.21 | 4.926 |
| 5 | S2 | 20.9 | 0.24 | 4.785 |
| 10 | S3 | 21.4 | 0.19 | 4.673 |
| 15 | S4 | 21.2 | 0.17 | 4.717 |
| 20 | S5 | 22.0 | 0.20 | 4.545 |
| 25 | S6 | 21.7 | 0.18 | 4.608 |
| 30 | S7 | 21.8 | 0.17 | 4.587 |
| Cycle-Index | Group No. | Maximum Load/kN | Maximum Stress/MPa |
|---|---|---|---|
| 0 | G1 | 269,362.2500 | 137.1851 |
| 5 | G2 | 316,925.4000 | 161.4088 |
| 10 | G3 | 209,809.1000 | 106.8549 |
| 15 | G4 | 250,503.1000 | 127.5802 |
| 20 | G5 | 155,875.8000 | 79.3869 |
| 25 | G6 | 83,218.7656 | 42.3830 |
| 30 | G7 | 105,022.6484 | 53.4876 |
| 0 | S1 | 424,275.7813 | 216.0819 |
| 5 | S2 | 244,133.1651 | 124.3360 |
| 10 | S3 | 128,023.9531 | 65.2021 |
| 15 | S4 | 282,091.4583 | 143.6680 |
| 20 | S5 | 159,700.8990 | 81.3350 |
| 25 | S6 | 107,604.4000 | 54.8025 |
| 30 | S7 | 133,001.5000 | 67.7371 |
| Cycle-Index | Group No. | Longitudinal Strain/μm | Transverse Strain/μm |
|---|---|---|---|
| 0 | G1 | 1451.00 | 736.00 |
| 5 | G2 | 1990.00 | 962.00 |
| 10 | G3 | 1882.00 | 578.00 |
| 15 | G4 | 1540.00 | 332.00 |
| 20 | G5 | 950.00 | 226.00 |
| 25 | G6 | 712.00 | 221.00 |
| 30 | G7 | 1633.00 | 662.00 |
| 0 | S1 | 2348.00 | 820.00 |
| 5 | S2 | 1362.00 | 736.00 |
| 10 | S3 | 1130.00 | 467.00 |
| 15 | S4 | 1220.00 | 664.00 |
| 20 | S5 | 885.00 | 577.00 |
| 25 | S6 | 964.00 | 586.00 |
| 30 | S7 | 717.00 | 384.00 |
| Cycle-Index | Group No. | Elastic Modulus | Elastic Poisson’s Ratio |
|---|---|---|---|
| 0 | G1 | 77.34 | 0.67 |
| 5 | G2 | 58.11 | 0.48 |
| 10 | G3 | 47.37 | 0.26 |
| 15 | G4 | 63.04 | 0.26 |
| 20 | G5 | 55.75 | 0.24 |
| 25 | G6 | 44.69 | 0.29 |
| 30 | G7 | 27.33 | 0.33 |
| 0 | S1 | 88.47 | 0.37 |
| 5 | S2 | 81.20 | 0.56 |
| 10 | S3 | 40.93 | 0.34 |
| 15 | S4 | 76.81 | 0.47 |
| 20 | S5 | 64.30 | 0.45 |
| 25 | S6 | 43.84 | 0.48 |
| 30 | S7 | 67.18 | 0.41 |
| Cycle-Index | Group No. | Secant Modulus | Poisson’s Ratio |
|---|---|---|---|
| 0 | G1 | 85.74 | 0.38 |
| 5 | G2 | 63.80 | 0.48 |
| 10 | G3 | 41.40 | 0.30 |
| 15 | G4 | 55.83 | 0.20 |
| 20 | G5 | 60.28 | 0.25 |
| 25 | G6 | 47.99 | 0.34 |
| 30 | G7 | 22.92 | 0.31 |
| 0 | S1 | 60.66 | 0.36 |
| 5 | S2 | 62.31 | 0.53 |
| 10 | S3 | 53.47 | 0.52 |
| 15 | S4 | 71.76 | 0.50 |
| 20 | S5 | 63.36 | 0.48 |
| 25 | S6 | 47.98 | 0.41 |
| 30 | S7 | 61.32 | 0.45 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, Z.; Zhang, Z.; Zhang, Y.; Shi, G. Strength Variation of Rocks Surrounding Road Tunnel Entrance/Exit in High–Altitude Mountain Areas under Freeze–Thaw Cycles. Sustainability 2022, 14, 9305. https://doi.org/10.3390/su14159305
Guo Z, Zhang Z, Zhang Y, Shi G. Strength Variation of Rocks Surrounding Road Tunnel Entrance/Exit in High–Altitude Mountain Areas under Freeze–Thaw Cycles. Sustainability. 2022; 14(15):9305. https://doi.org/10.3390/su14159305
Chicago/Turabian StyleGuo, Zezhou, Zizhao Zhang, Yanyang Zhang, and Guangming Shi. 2022. "Strength Variation of Rocks Surrounding Road Tunnel Entrance/Exit in High–Altitude Mountain Areas under Freeze–Thaw Cycles" Sustainability 14, no. 15: 9305. https://doi.org/10.3390/su14159305
APA StyleGuo, Z., Zhang, Z., Zhang, Y., & Shi, G. (2022). Strength Variation of Rocks Surrounding Road Tunnel Entrance/Exit in High–Altitude Mountain Areas under Freeze–Thaw Cycles. Sustainability, 14(15), 9305. https://doi.org/10.3390/su14159305






