Determination of Partial Depth Repair Size for Spalling of Jointed Concrete Pavements Using the Impact Echo Method
Abstract
:1. Introduction
2. Laboratory Tests for Applicability of IE Method
2.1. Test Method
2.1.1. Dynamic Modulus Test Conducted Using IE Method
- : acceleration (m/s2),
- : maximum acceleration (4900 m/s2),
- : minimum acceleration (−4900 m/s2),
- : maximum voltage (12 V),
- : minimum voltage (7 V), and
- : measured voltage (V).
- : velocity of P-wave (m/s),
- : first-order resonant frequency (Hz),
- : correction factor (0.96), and
- : thickness (height of the specimen in this study, m).
- : dynamic modulus (MPa),
- : Poisson’s ratio, and
- : density (kg/m3).
- : RDM obtained by laboratory test (%),
- : dynamic modulus at n freeze–thaw cycles (MPa), and
- : dynamic modulus at 0 freeze–thaw cycles (MPa).
2.1.2. Dynamic Modulus Test Conducted Using FR Method
- : length of specimen (mm),
- : area of top or bottom of specimen (m2), and
- : weight of specimen (kg).
2.1.3. Compressive Strength Test
2.1.4. Absorption Test
- : absorption coefficient at t (kg/m2/h0.5),
- : mass of absorbed water per sectional area of specimen at t (kg/m2), and
- : elapsed time since specimen soaking (h).
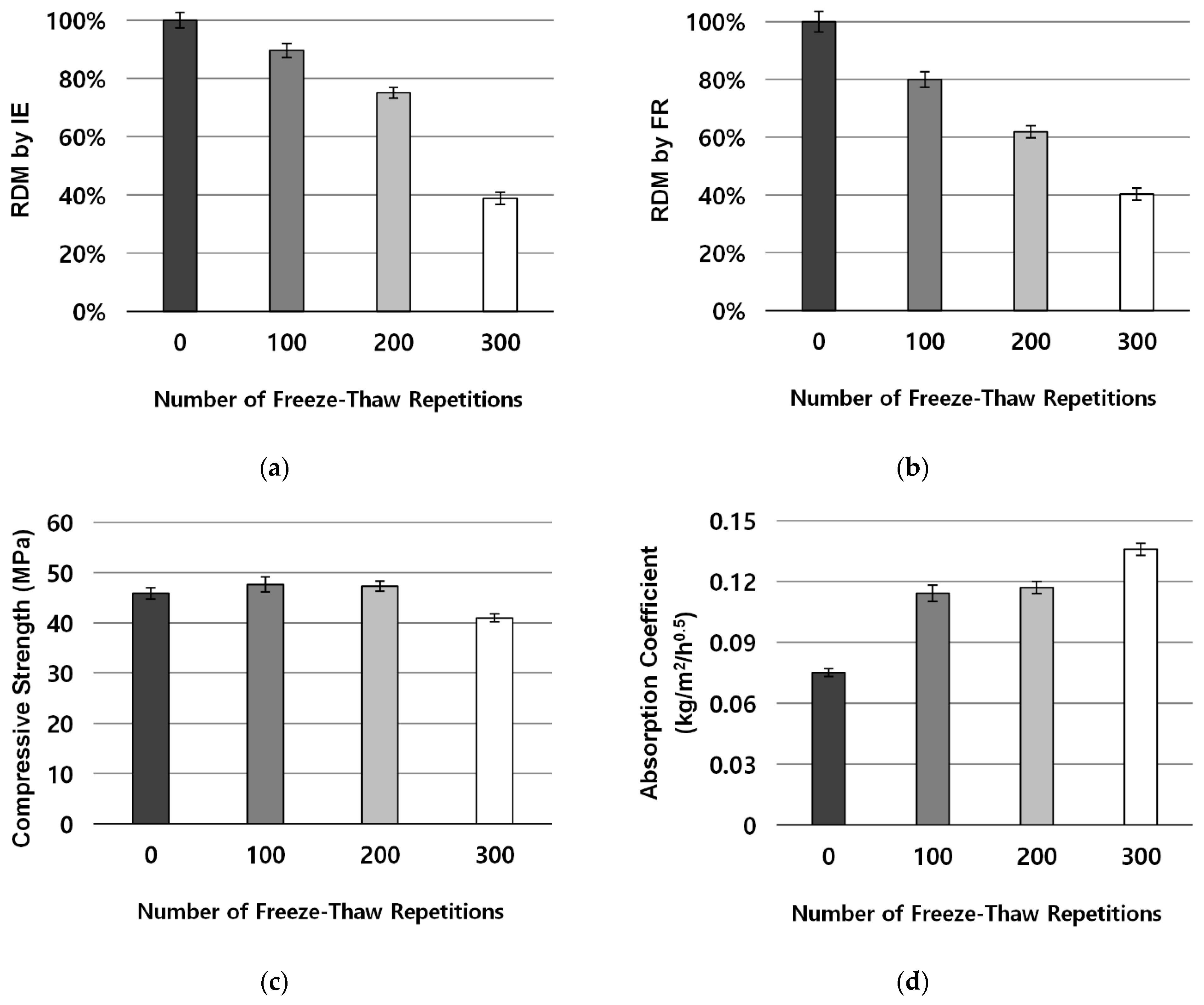
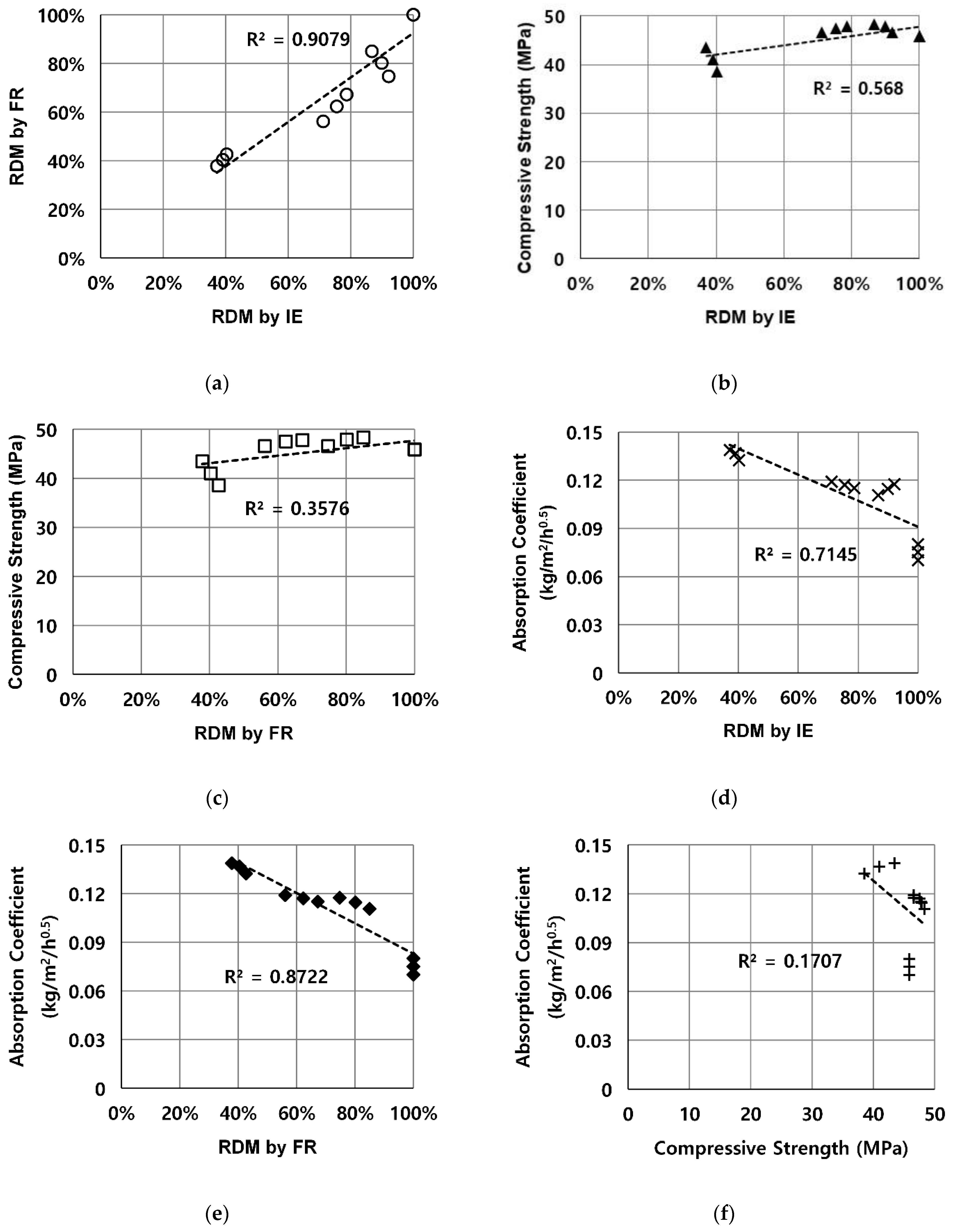
2.2. Test Results
3. Field Test for Determination of Damaged Range
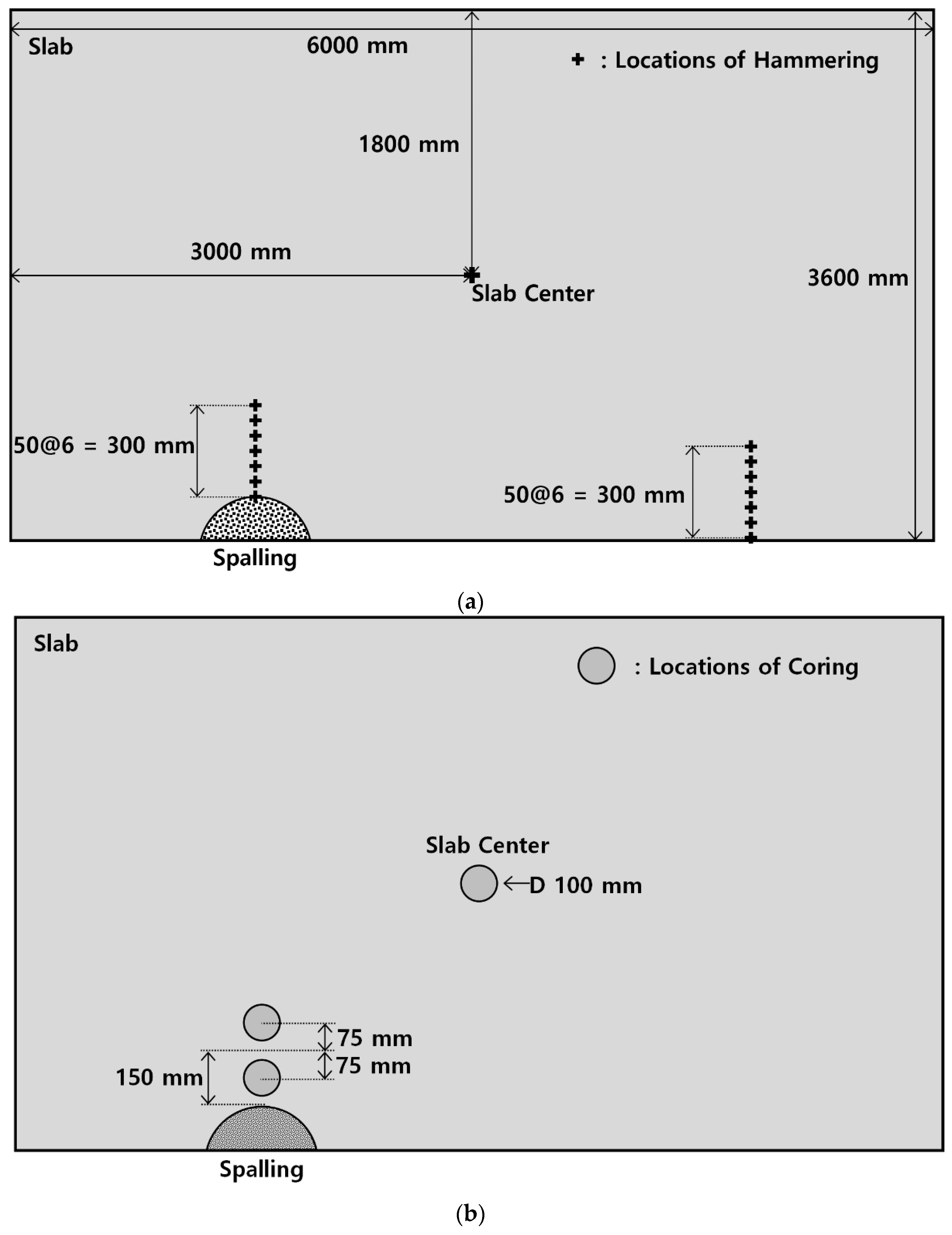
3.1. Test Method
3.2. Test Results
4. Laboratory Tests for Suggestion of PDR Size
4.1. Test Method
4.2. Test Results
5. Conclusions
- (1)
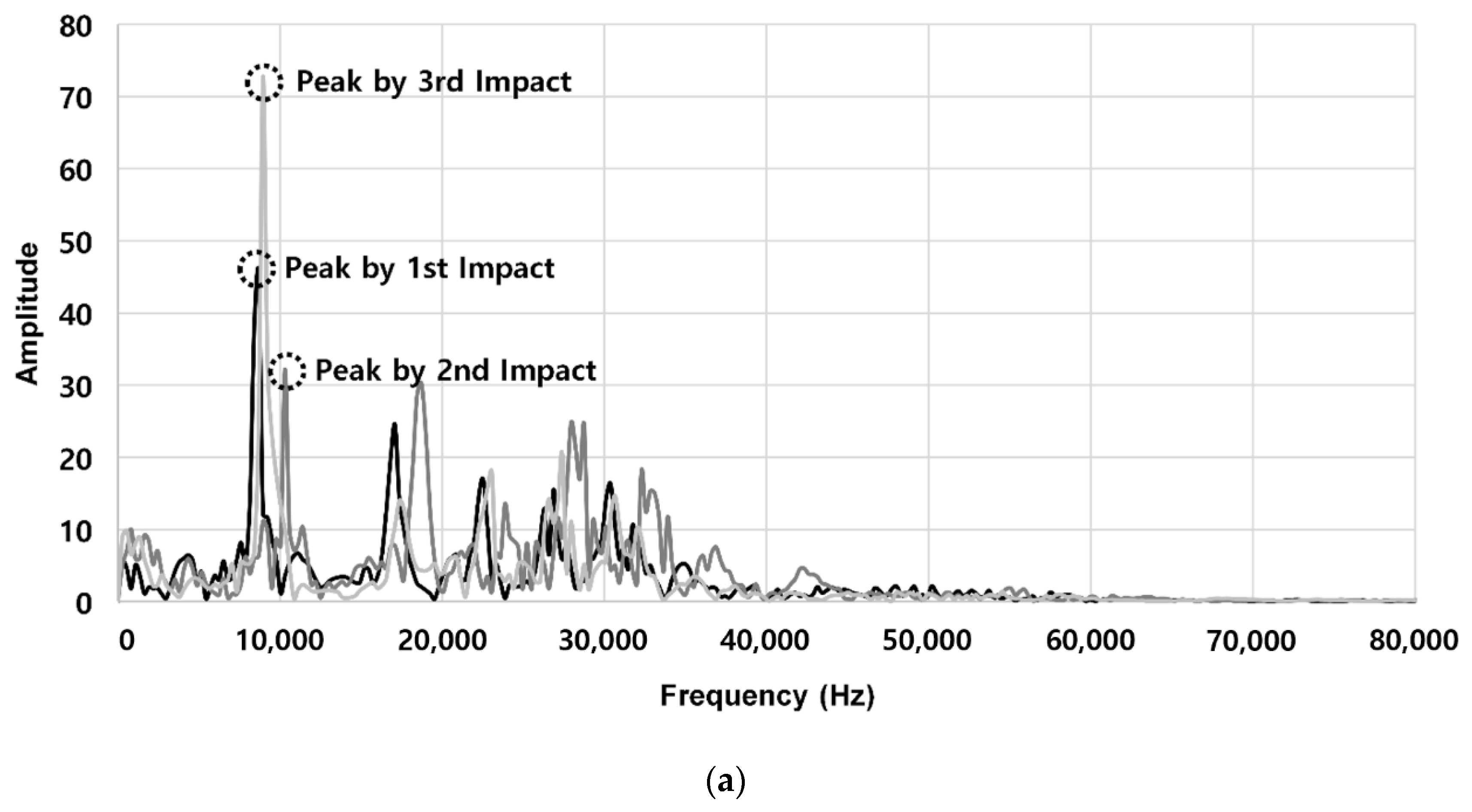
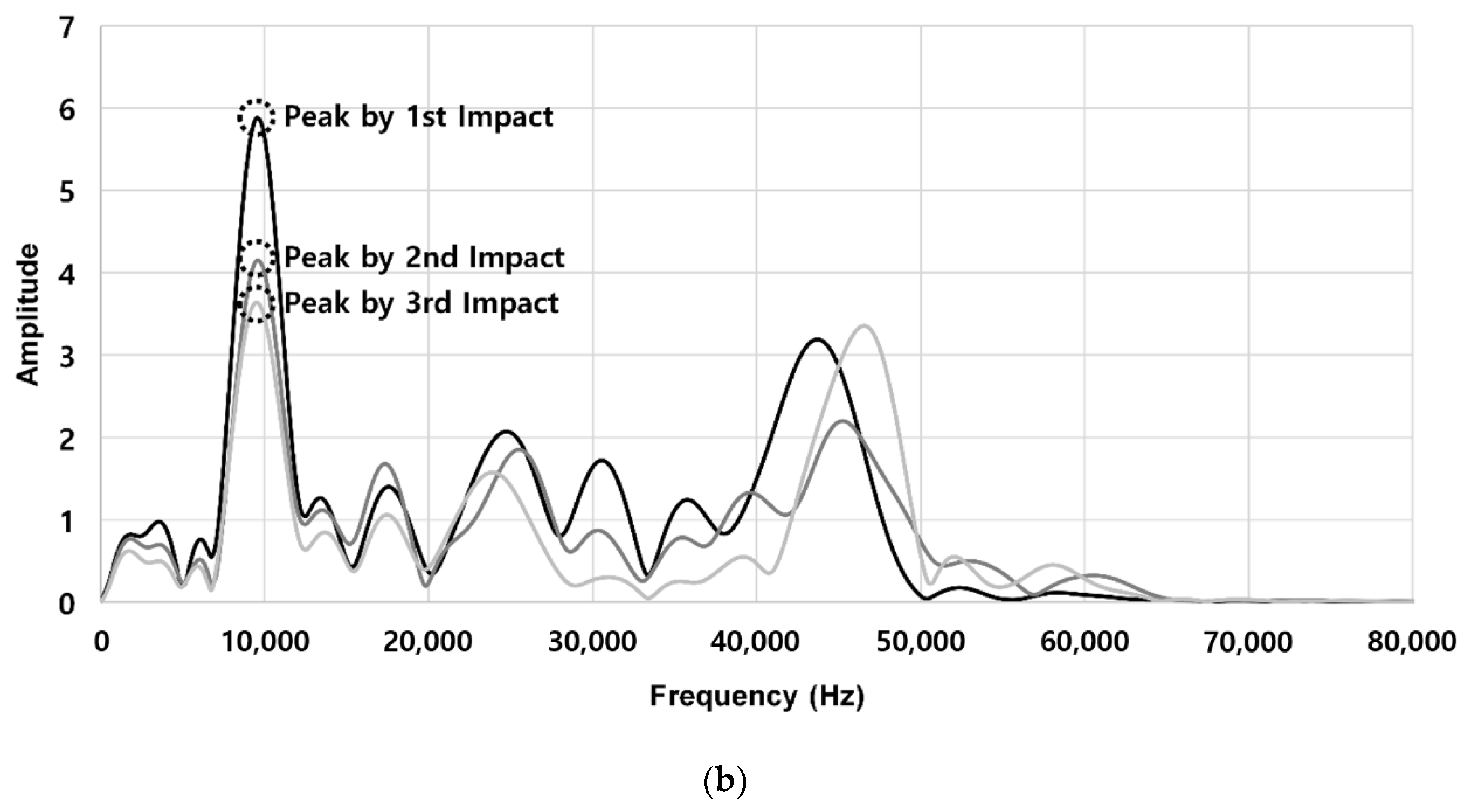
- The IE method, which allows vibrations of impaction to be estimated at the same specimen location, was used in this study given the test condition of the concrete pavement, rather than the FR method, which requires the forced vibrations to be estimated on the opposite side. In addition, the noise that occurs under field conditions can be minimized, and as a result, a relatively more accurate dynamic modulus was estimated using the STFT technique instead of the FFT technique.
- (2)
- An RDM of 80% was determined as the criterion for dividing the damaged and undamaged parts of the slab based on the existing criteria. Measuring the RDM of the concrete pavement where spalling occurred using the IE method indicated that the locations between 100 and 150 mm from the end of spalling were the boundary between the damaged and undamaged parts of the slab in the case of high severity. Similarly, the locations between 50 and 100 mm from the end of spalling were the boundary between the damaged and undamaged parts of the slab in the case of medium severity. In the case of low severity, it was judged that the entire slab, including the end of spalling, was not damaged when compared to the sound slab, thus a boundary was not required.
- (3)
- A laboratory test was conducted on specimens cored from the concrete pavement where spalling with high and medium severity occurred. Consequently, in both cases of high and medium severities, the RDM of the specimens cored from the damaged part of the slab, 75 mm from the end of spalling, did not satisfy the 80% criterion for soundness, whereas that from the undamaged part of the slab, 225 mm from the end of spalling, satisfied the criterion.
- (4)
- Additional laboratory tests were conducted on the upper and lower parts of the core specimens. The RDM of the upper part of the specimens cored from the undamaged part of the slab satisfied the criterion for soundness, whereas that of the damaged part of the slab did not satisfy the criterion regardless of the spalling severity. On the other hand, the RDM of the lower part of the specimens satisfied the criterion regardless of the location of the coring and severity of spalling.
- (5)
- According to the field and laboratory test results, a PDR of up to 150 mm from the end of spalling in both the parallel and perpendicular directions was suggested for high severity. In the case of medium severity, a PDR up to 100 mm from the end of spalling in both the parallel and perpendicular directions was suggested. The minimum depth of the PDR was conservatively suggested to be 100 mm for both high-and medium spalling severity. In addition, it was suggested that the depth of the PDR can be determined by engineers, considering factors such as the depth of spalling, slab thickness, depth of dowel bars, and tie bars.
- (6)
- The soundness of the concrete slab directly below spalling could not be investigated in this study. In addition, the soundness at a 50 mm depth of the slab, which is the minimum PDR depth designated in most criteria, could not be investigated. Therefore, a more accurate and reasonable depth of the PDR needs to be suggested by performing additional studies.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Darter, M. Concrete Repair Best Practices: A Series of Case Studies; Missouri Department of Transportation: Jefferson City, MO, USA, 2017. [Google Scholar]
- FHWA. Concrete Pavement Preservation Guide; Federal Highway Administration: Washington, DC, USA, 2014. [Google Scholar]
- KEC. Expressway Pavement Management and Maintenance Manual; Korea Expressway Corporation: Seongnam, Korea, 2010. [Google Scholar]
- MnDOT. Standard Specifications for Construction; Minnesota Department of Transportation: St. Paul, MN, USA, 2018. [Google Scholar]
- MoLIT. Road Pavement Maintenance Practice Manual; Ministry of Land, Infrastructure, and Transport: Seoul, Korea, 2013. [Google Scholar]
- MoLIT. National Highway Construction Special Specification; Ministry of Land, Infrastructure, and Transport: Seoul, Korea, 2015. [Google Scholar]
- Lee, Y.H.; Kim, H.S.; Jung, W.K.; Oh, H.J.; Kim, H.B. Re-repair method for deterioration of partial depth repair section in Portland cement concrete pavement. Int. J. Highw. Eng. 2017, 19, 33–42. [Google Scholar] [CrossRef]
- Helal, J.; Sofi, M.; Mendis, P. Non-destructive testing of concrete: A review of methods. Electron. J. Struct. Eng. 2015, 14, 97–105. [Google Scholar]
- FHWA. Ensuring Durability of Concrete Paving Mixtures; Tech Brief; FHWA-HIF-16-033; Federal Highway Administration: Washington, DC, USA, 2016. [Google Scholar]
- MoLIT. Road Pavement Integration Guideline; Ministry of Land, Infrastructure, and Transport: Seoul, Korea, 2011. [Google Scholar]
- ASTM C 39; Standard Test Method for Compressive Strength of Cylindrical Concrete Specimens. ASTM International: West Conshohocken, PA, USA, 2020. [CrossRef]
- ASTM C 1548; Standard Test Method for Dynamic Young’s Modulus, Shear Modulus, and Poisson’s Ratio of Refractory Materials by Impulse Excitation of Vibration. ASTM International: West Conshohocken, PA, USA, 2015. [CrossRef]
- ASTM C 1585; Standard Test Method for Measurement of Rate of Absorption of Water by Hydraulic-Cement Concretes. ASTM International: West Conshohocken, PA, USA, 2004. [CrossRef]
- ASTM C 192; Standard Practice for Making and Curing Concrete Test Specimens in the Laboratory. ASTM International: West Conshohocken, PA, USA, 2019. [CrossRef]
- KEC. Standard for Quality of Expressway Construction Materials; Korea Expressway Corporation: Seongnam, Korea, 2018. [Google Scholar]
- ASTM C 666; Standard Test Method for Resistance of Concrete to Rapid Freezing and Thawing. ASTM International: West Conshohocken, PA, USA, 2015. [CrossRef]
- Model 352C22 ICP® Accelerometer Installation and Operating Manual; PCB Piezotronics, Inc.: Enchede, The Netherlands, 2013.
- Cooley, J.W.; Tukey, J.W. An algorithm for the machine calculation of complex Fourier series. Math. Comput. 1965, 19, 297–301. [Google Scholar] [CrossRef]
- Sethares, W.A. Rhythm and Transforms, 1st ed.; Springer: London, UK, 2007. [Google Scholar]
- Gabor, D. Theory of communication. J. Inst. Electr. Eng. 1946, 93, 429–457. [Google Scholar] [CrossRef]
- Schubert, F.; Lausch, R.; Wiggenhauser, H. Geometrical effects on impact-echo testing of finite concrete specimens. In Proceedings of the International Symposium Non-Destructive Testing in Civil Engineering, Berlin, Germany, 16–19 September 2003. [Google Scholar]
- Ye, J.; Iwata, M.; Takumi, K.; Murakawa, M.; Tetsuya, H.; Kubota, Y.; Yui, T.; Mori, K. Statistical impact-echo analysis based on Grassmann Manifold Learning: Its preliminary results for concrete condition assessment. In Proceedings of the EWSHM—7th European Workshop on Structural Health Monitoring, Nantes, France, 8–11 July 2014. [Google Scholar]
- Qu, H.; Li, T.; Chen, G. Synchro-squeezed adaptive wavelet transform and its application to impact echo signals for pavement defect detection. In Proceedings of the INSPIRE-UTC 2019 Annual Meeting, St. Louis, MO, USA, 7 August 2019. [Google Scholar]
- Yao, F.; Chen, G.; Abula, A. Research on signal processing of segment-grout defect in tunnel based on impact-echo method. Constr. Build. Mater. 2018, 187, 280–289. [Google Scholar] [CrossRef]
- ASTM C 1383; Standard Test Method for Measuring the P-Wave Speed and the Thickness of Concrete Plates Using the Impact-Echo Method. ASTM International: West Conshohocken, PA, USA. [CrossRef]
- Jang, J.H.; Jeong, J.H.; Kwon, S.M.; Park, H.G. Long-term monitoring operation of the test-road in Korea Highway Corporation (KHC). In Sensing Issues in Civil Structural Health Monitoring; Springer: Dordrecht, The Netherlands, 2005; pp. 505–514. [Google Scholar] [CrossRef]
- ACPA. Concrete Pavement Field Reference-Preservation and Repair; Report EB239P; American Concrete Pavement Association: Skokie, IL, USA, 2006. [Google Scholar]
- FHWA. PCC Pavement Evaluation and Rehabilitation; NHI Course 131062; Federal Highway Administration: Washington, DC, USA, 2001. [Google Scholar]








| Repair Size | MnDOT * [4] | FHWA ** [2] | KEC *** [3] | MoLIT **** [5] | MoLIT **** [6] |
|---|---|---|---|---|---|
| Distance from End of Spalling (mm) | 75 | 75 | 75 | 75 | 75 |
| Min. Depth (mm) | 50 | 50 | 50 | 40–50 | 50 |
| Max. Coarse Aggregate Size (mm) | Slump (mm) | Air Content (%) | Water Cement Ratio | Fine Aggregate Percentage | Unit Weight (kg/m3) | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| Water | Cement | Fine Aggregate | Coarse Aggregate | Admixture | ||||||
| Air Entraining Agent | Water Reducer | |||||||||
| 25 | Less than 40 | 5–7 | 40 | 40 | 155 | 388 | 649 | 1052 | 0.14 (132 mL) | 2.71 (2557 mL) |
| Severity | Definition [2] | Figure |
|---|---|---|
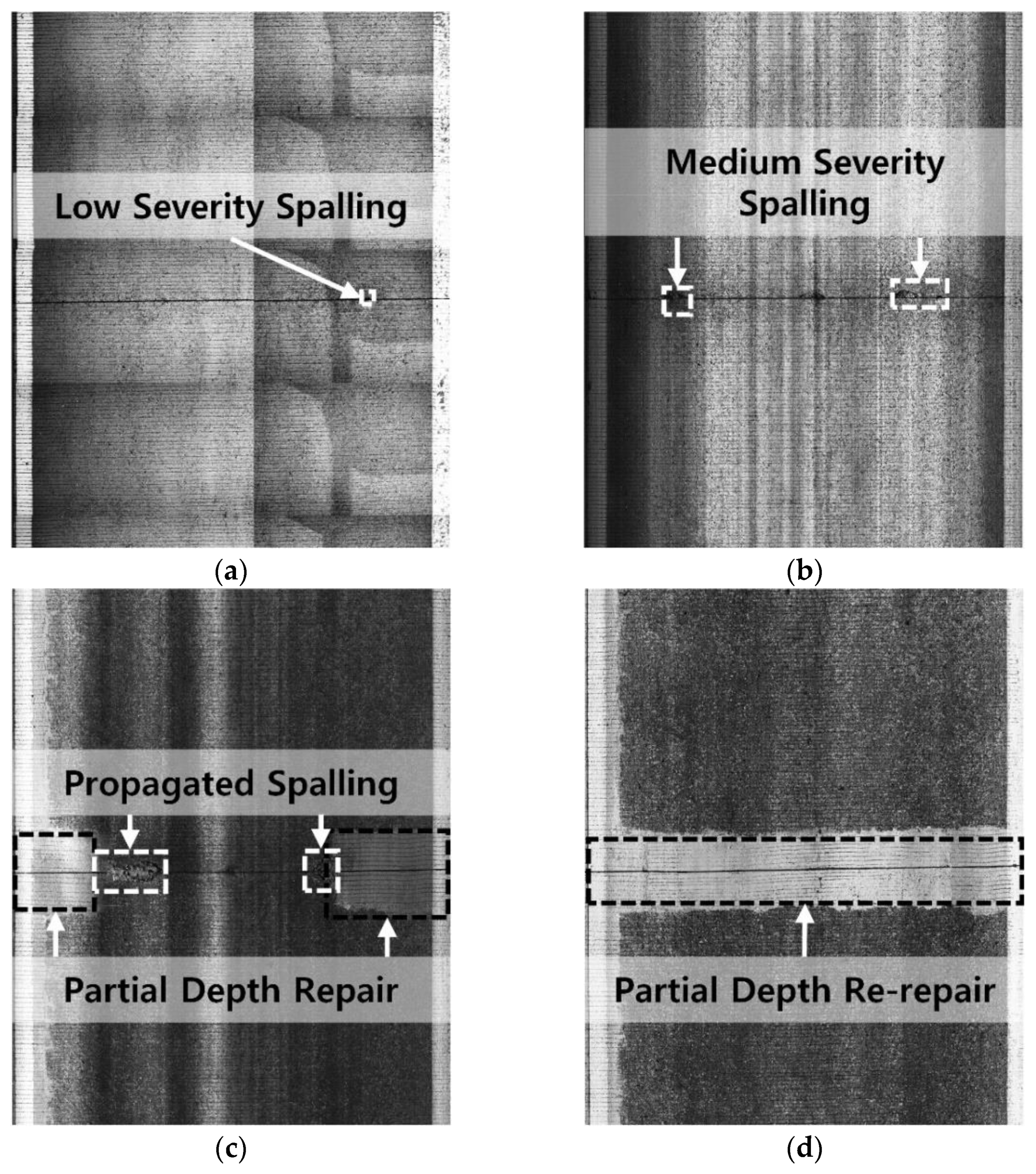
| Low | <75 mm width |  |
| Medium | 75 to 150 mm width |  |
| High | >150 mm width |  |
| Agency | FHWA [9] | MoLIT [10] | |
|---|---|---|---|
| Regulation | Relative dynamic modulus (RDM) using resonance frequency method | RDM when crushed fine aggregate is included in concrete pavement | RDM when chemical admixture is included in concrete pavement |
| Criteria | 60% or 80% | 80% | 80% |
| Length of Specimen | Moistness of Specimen | Test Method |
|---|---|---|
| Full Length (Initial length over 200 mm) | Dried | Absorption |
| 200 mm (Grind top and eliminate below 200 mm) | Dried and Saturated | Dynamic Modulus by Impact Echo (IE) Dynamic Modulus by Forced Resonance (FR) |
| 100 mm (Divide into two pieces) | Dried and Saturated | Dynamic Modulus by IE Dynamic Modulus by FR Compressive Strength |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, D.-H.; Choi, M.-K.; Han, S.-H.; Jeong, J.-H. Determination of Partial Depth Repair Size for Spalling of Jointed Concrete Pavements Using the Impact Echo Method. Sustainability 2022, 14, 8143. https://doi.org/10.3390/su14138143
Kim D-H, Choi M-K, Han S-H, Jeong J-H. Determination of Partial Depth Repair Size for Spalling of Jointed Concrete Pavements Using the Impact Echo Method. Sustainability. 2022; 14(13):8143. https://doi.org/10.3390/su14138143
Chicago/Turabian StyleKim, Dong-Hyuk, Min-Kyu Choi, Seung-Hwan Han, and Jin-Hoon Jeong. 2022. "Determination of Partial Depth Repair Size for Spalling of Jointed Concrete Pavements Using the Impact Echo Method" Sustainability 14, no. 13: 8143. https://doi.org/10.3390/su14138143
APA StyleKim, D.-H., Choi, M.-K., Han, S.-H., & Jeong, J.-H. (2022). Determination of Partial Depth Repair Size for Spalling of Jointed Concrete Pavements Using the Impact Echo Method. Sustainability, 14(13), 8143. https://doi.org/10.3390/su14138143






