Study on Interparticle Interaction Force Model to Correct Saturation Density of Real Cryogenic Fluid for LBM Simulation
Abstract
:1. Introduction
2. Method
3. Simulation of Real Cryogenic Fluid
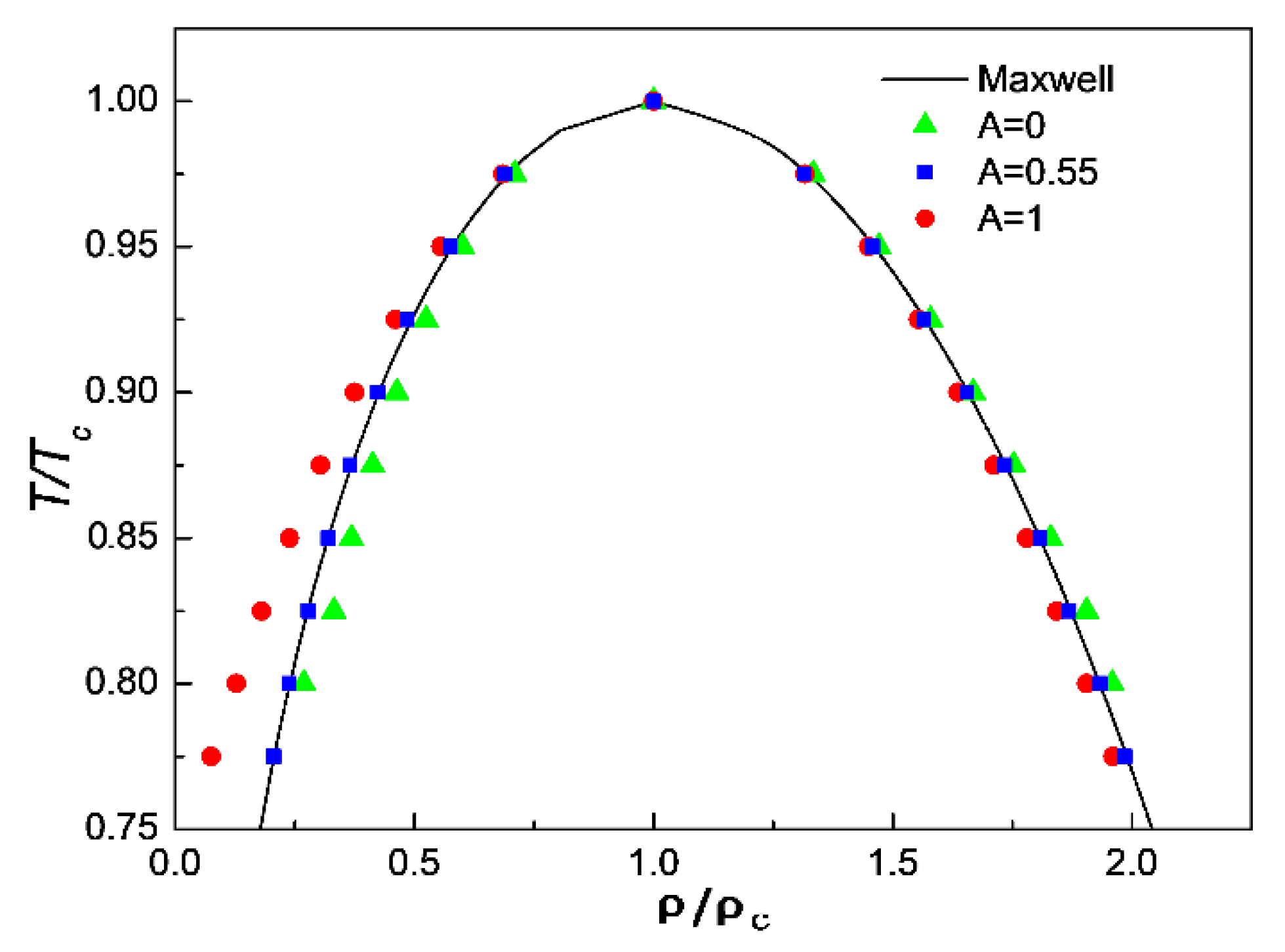
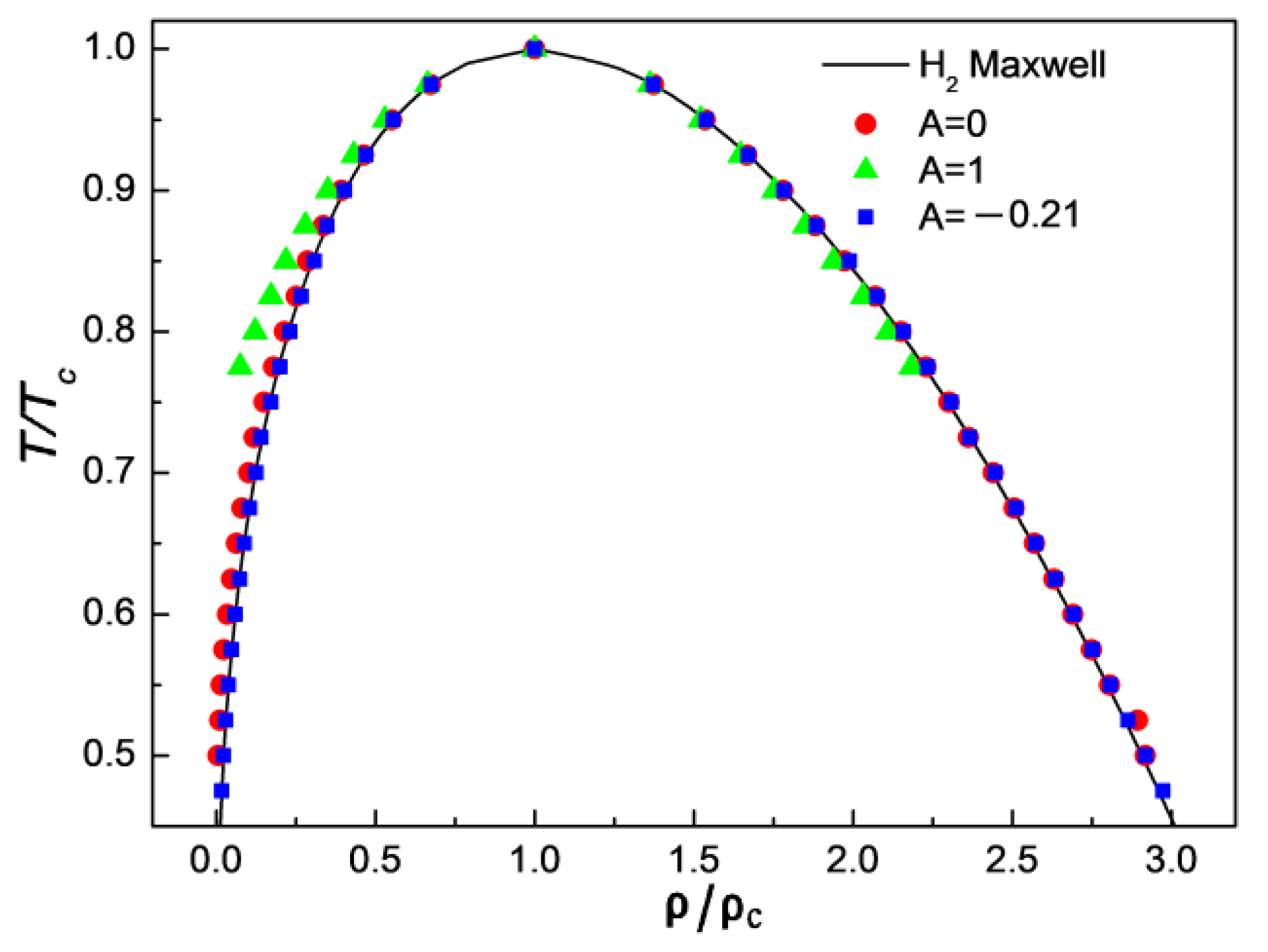
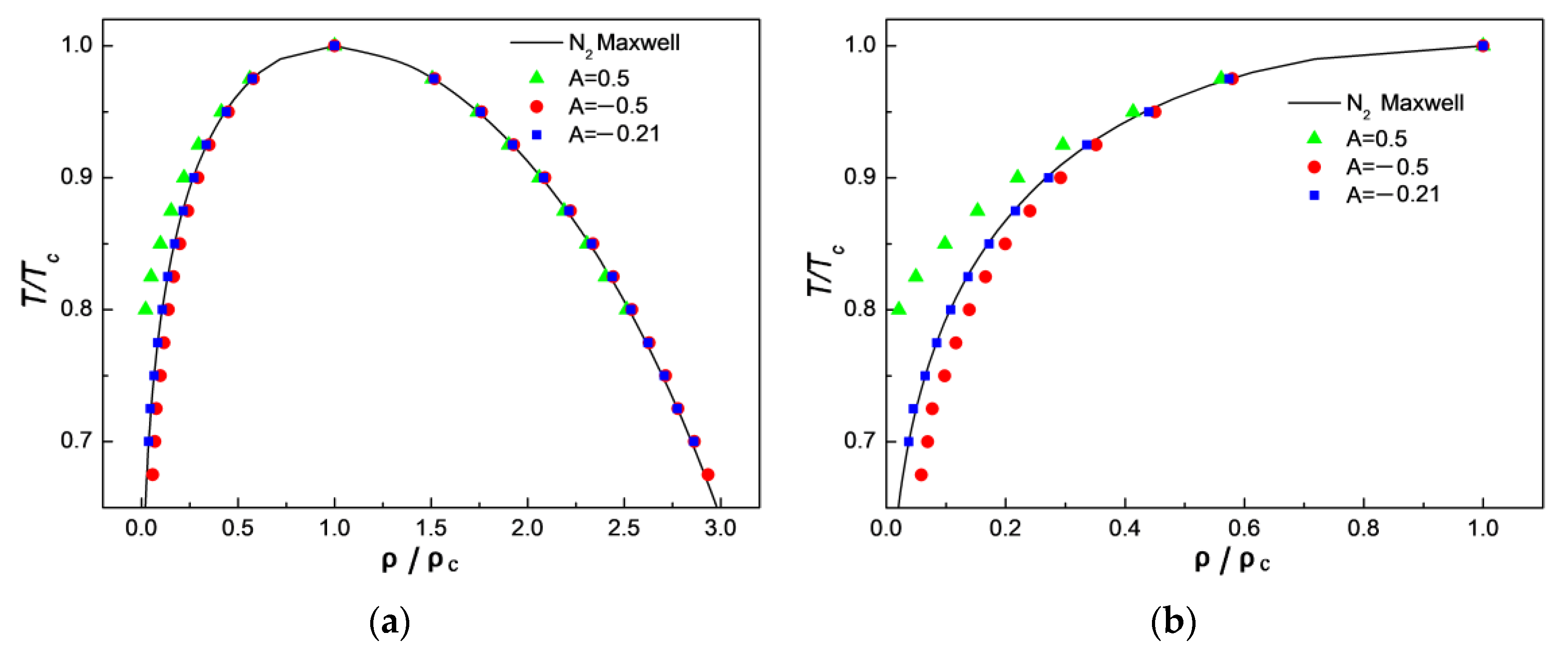
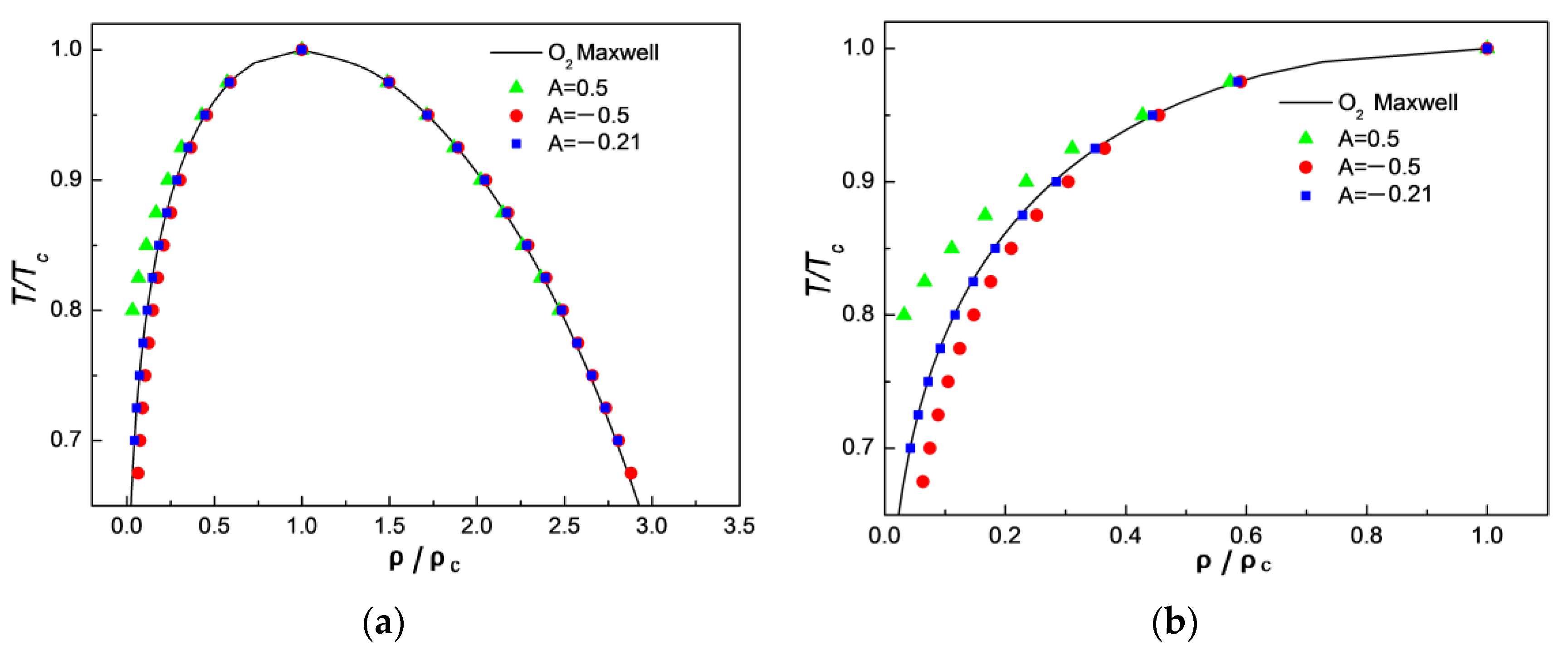
3.1. Comparison of Simulation Results with Maxwell’s Theoretical Solution
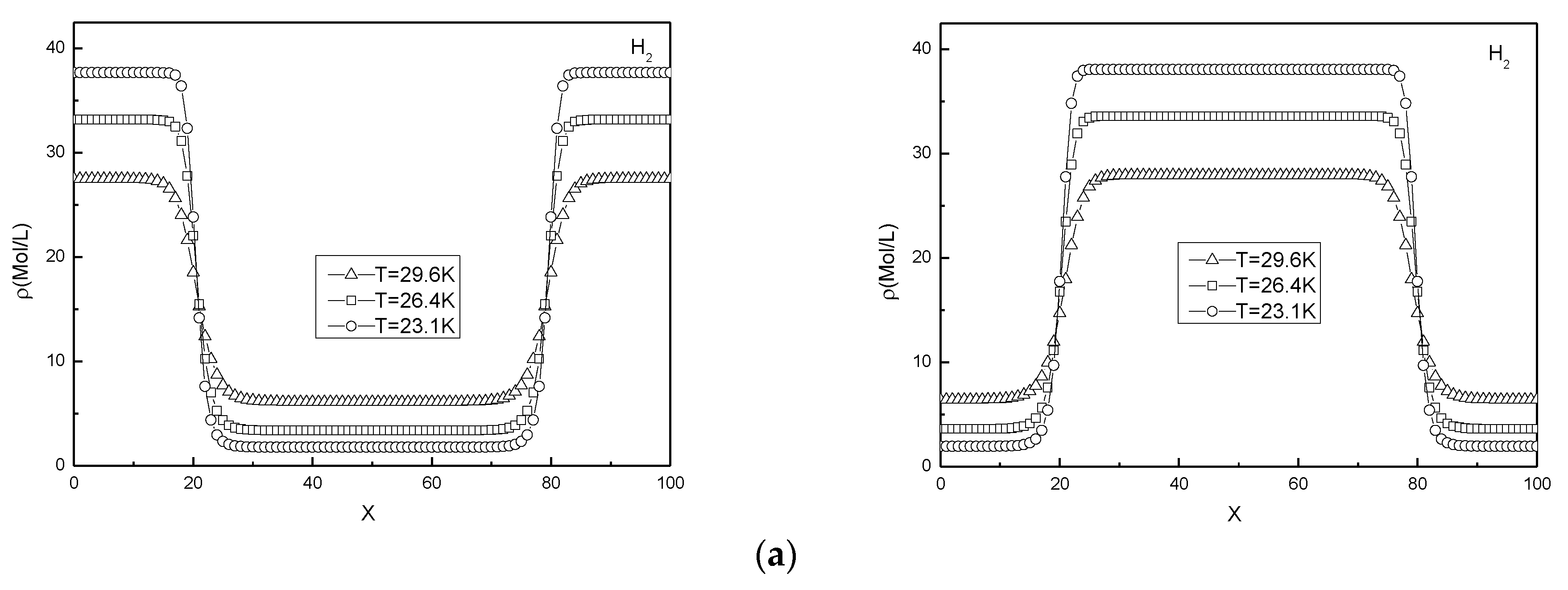
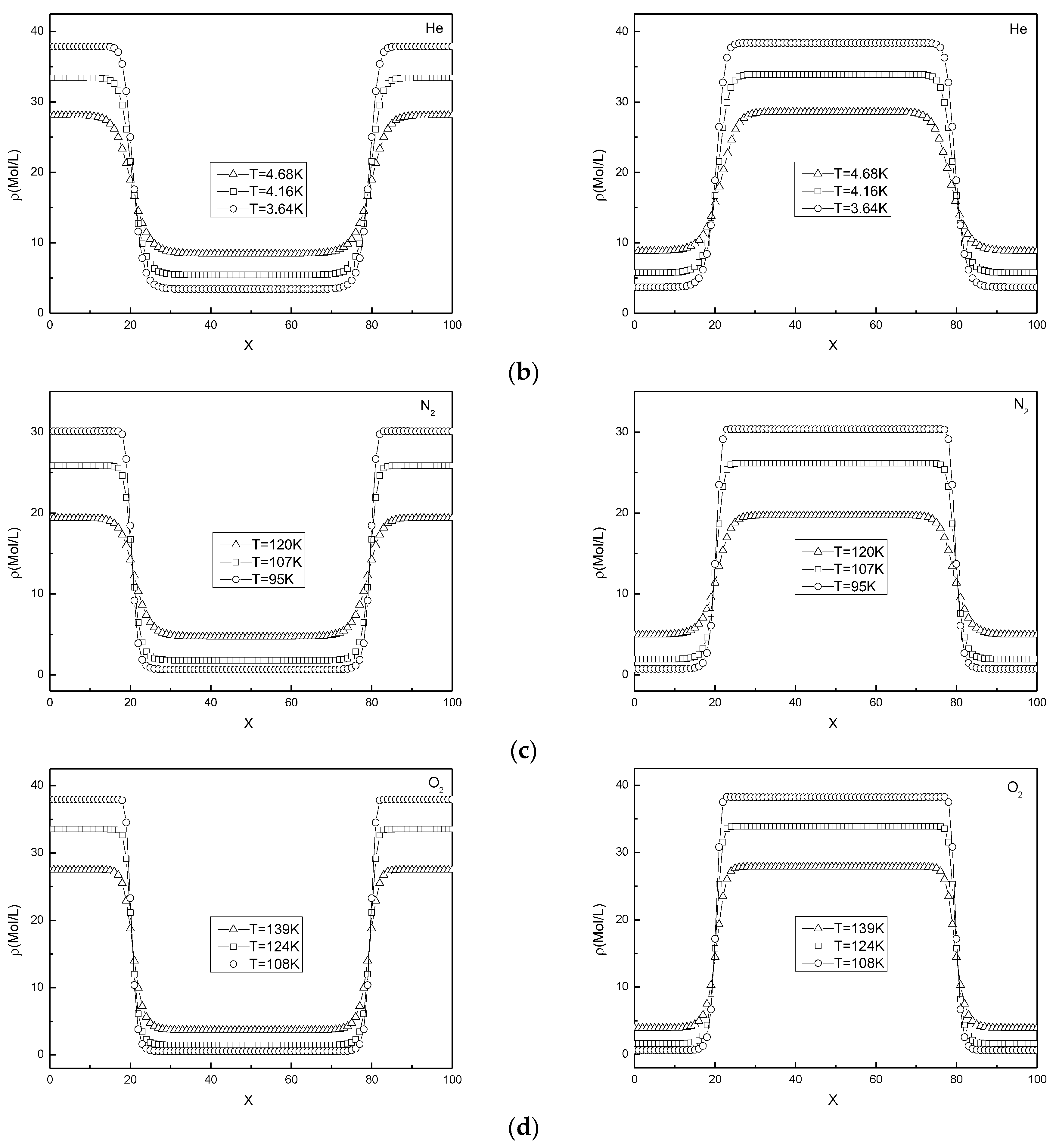
3.2. Interface Density Gradient Simulation
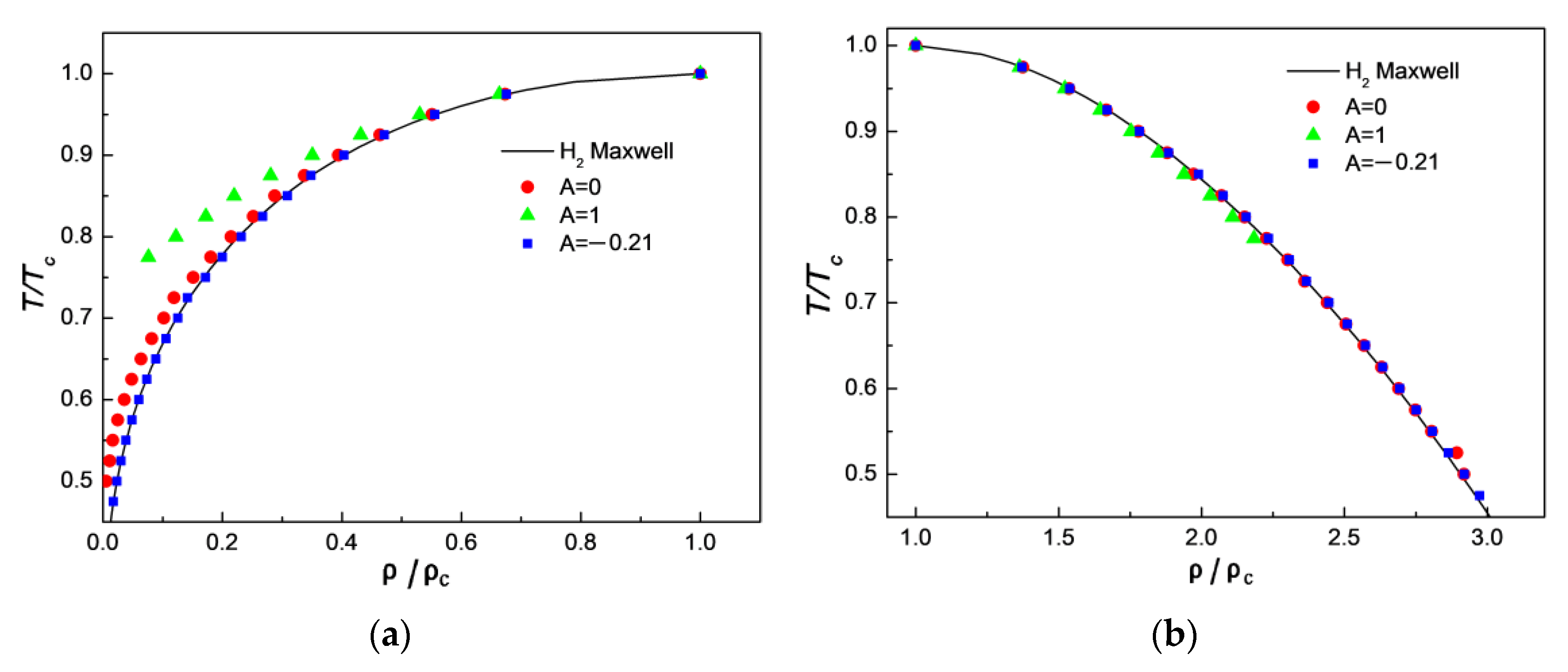
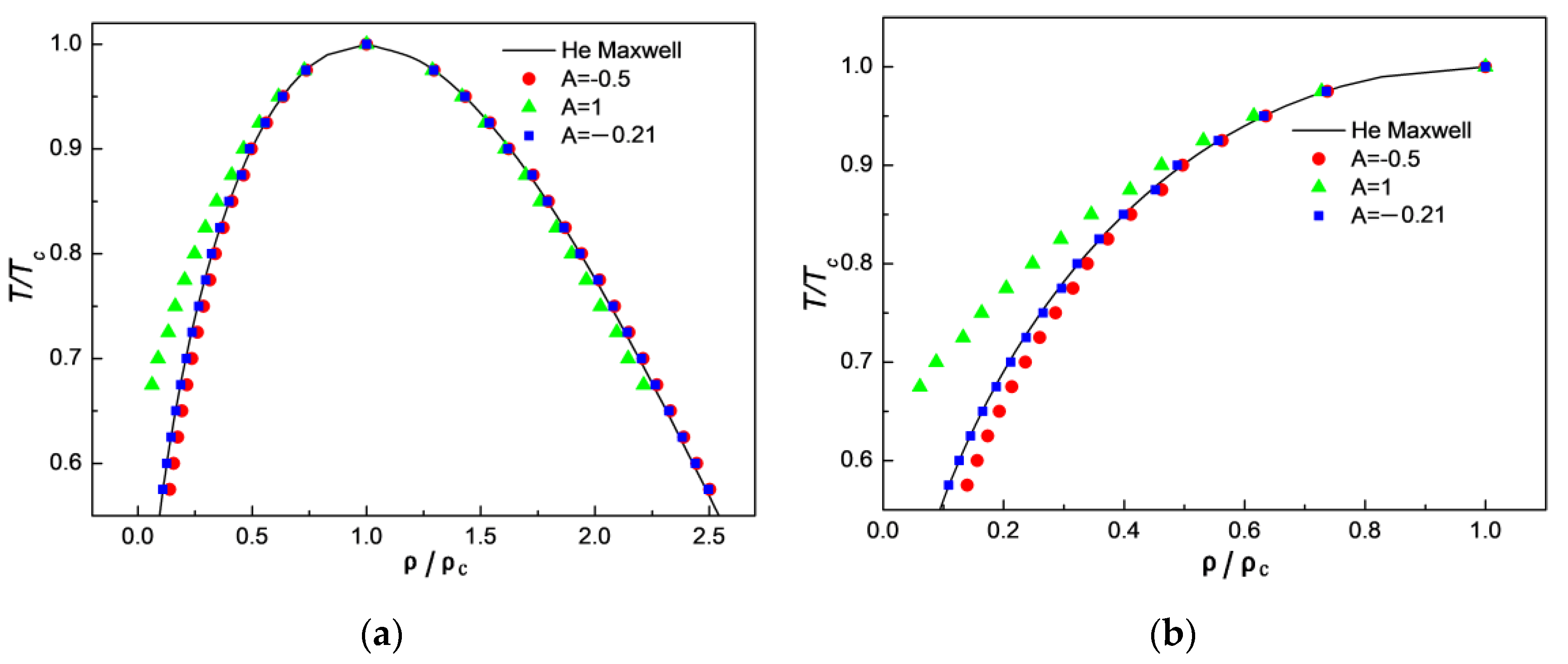
3.3. Comparison of Simulation Results with Experimental Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Krastev, V.; Falcucci, G. Simulating Engineering Flows through Complex Porous Media via the Lattice Boltzmann Method. Energies 2018, 11, 715. [Google Scholar] [CrossRef] [Green Version]
- Honari, S.; Hosseininia, E.S. Particulate Modeling of Sand Production Using Coupled DEM-LBM. Energies 2021, 14, 906. [Google Scholar] [CrossRef]
- Liang, W.; Xun, Q.; Shu, Z.; Lu, F.; Qian, H. Numerical Simulation of Erosion Characteristics for Solid-Air Particles in Liquid Hydrogen Elbow Pipe. Sustainability 2021, 13, 13303. [Google Scholar] [CrossRef]
- Che Zain, M.S.; Yeoh, J.X.; Lee, S.Y.; Shaari, K. Physicochemical Properties of Choline Chloride-Based Natural Deep Eutectic Solvents (NaDES) and Their Applicability for Extracting Oil Palm Flavonoids. Sustainability 2021, 13, 12981. [Google Scholar] [CrossRef]
- Zhu, Q.; Yan, J. A moving-domain CFD solver in FEniCS with applications to tidal turbine simulations in turbulent flows. Comput. Math. Appl. 2019, 81, 532–546. [Google Scholar] [CrossRef]
- Nguyen, G.T.; Chan, E.L.; Tsuji, T.; Tanaka, T.; Washino, K. Interface control for resolved CFD-DEM with capillary interactions. Adv. Powder Technol. 2021, 32, 1410–1425. [Google Scholar] [CrossRef]
- Kaya, U.; Gopireddy, S.; Urbanetz, N.; Nopens, I.; Verwaeren, J. Predicting the hydrodynamic properties of a bioreactor: Conditional density estimation as a surrogate model for CFD simulations. Chem. Eng. Res. Des. 2022, 182, 342–359. [Google Scholar] [CrossRef]
- Kabdenova, B.; Rojas-Solórzano, L.R.; Monaco, E. Lattice boltzmann simulation of near/supercritical CO2 flow featuring a crossover formulation of the equation of state. Comput. Fluids 2020, 216, 104820. [Google Scholar] [CrossRef]
- Grunau, D.; Chen, S.; Eggert, K. A lattice Boltzmann model for multiphase fluid flows. Phys. Fluids A Fluid Dyn. 1993, 5, 2557–2562. [Google Scholar] [CrossRef] [Green Version]
- Shan, X.; Chen, H. Lattice Boltzmann model for simulating flows with multiple phases and components. Phys. Rev. E 1993, 47, 1815. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Swift, M.; Osborn, W.; Yeomans, J. Lattice Boltzmann simulation of nonideal fluids. Phys. Rev. Lett. 1995, 75, 830–833. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Inamuro, T.; Ogata, T.; Tajima, S.; Konishi, N. A lattice Boltzmann method for incompressible two-phase flows with large density differences. J. Comput. Phys. 2004, 198, 628–644. [Google Scholar] [CrossRef]
- Lee, T.; Lin, C. A stable discretization of the lattice Boltzmann equation for simulation of in compressible two-phase flows at high density ratio. J. Comput. Phys. 2005, 206, 16–47. [Google Scholar] [CrossRef]
- Kupershtokh, A.L.; Medvedev, D.A.; Karpov, D.I. On equations of state in a lattice Boltzmann method. Comput. Math. Appl. 2009, 58, 965–974. [Google Scholar] [CrossRef] [Green Version]
- Mehrizi, A.A.; Sedighi, K.; Afrouzi, H.H.; Aghili, A.L. Lattice boltzmann simulation of forced convection in vented cavity filled by porous medium with obstruction. World Appl. Sci. J. 2012, 16, 31–36. [Google Scholar]
- Li, J.W.; Li, Z.; Yuan, W.J.; Lei, X.M.; Torres, S.A.G.; Cherubini, C.; Scheuermann, A.; Li, L. Numerical investigation of liquid and supercritical CO2 flow behaviors through 3d self-affine rough fractures. Fuel 2019, 251, 669–682. [Google Scholar] [CrossRef]
- Huang, J.; Feng, X.; Yin, X. Lattice boltzmann simulation of pressure-driven two-phase flows in capillary tube and porous medium. Comput. Fluids 2017, 155, 134–145. [Google Scholar] [CrossRef]
- Zhu, W.B.; Wang, M.; Chen, H. Study on multicomponent pseudo-potential model with large density ratio and heat transfer. Int. Commun. Heat Mass Transf. 2017, 87, 183–191. [Google Scholar] [CrossRef]
- Tian, Z.W.; Xing, H.L.; Tan, Y.L.; Gu, S.; Golding, S.D. Reactive transport LBM model for CO2 injection in fractured reservoirs. Comput. Geosci. 2016, 86, 15–22. [Google Scholar] [CrossRef] [Green Version]
- Zhang, D.; Li, S.; Ren, W.; Li, Y.; Mei, N. Co-existing boiling and condensation phase changes of a multicomponent fluid in a confined micro-space analysed by a modified LBM. Int. J. Therm. Sci. 2021, 170, 107147. [Google Scholar] [CrossRef]
- Jourabiana, M.; Darzi, A.A.; Akbari, O.A.; Toghrai, D. The enthalpy-based lattice Boltzmann method (LBM) for simulation of NePCM melting in inclined elliptical annulus. Phys. A Stat. Mech. Its Appl. 2020, 548, 123887. [Google Scholar] [CrossRef]
- Feng, S.J.; Chen, H.; Zheng, Q.T.; Chen, H.X. Fractal analytical solution of gas diffusivity in heterogeneous porous media over the entire saturation range. Int. J. Heat Mass Transf. 2022, 188, 122652. [Google Scholar] [CrossRef]
- Liu, C.; Qian, R.S.; Liu, Z.Y.; Liu, G.J.; Zhang, Y.S. Multi-scale modelling of thermal conductivity of phase change material/recycled cement paste incorporated cement-based composite material. Mater. Des. 2020, 191, 108646. [Google Scholar] [CrossRef]
- Jiang, F.; Yang, J.H.; Boek, E.; Tsuji, T. Investigation of viscous coupling effects in three-phase flow by lattice Boltzmann direct simulation and machine learning technique. Adv. Water Resour. 2021, 147, 103797. [Google Scholar] [CrossRef]
- Xu, R.; Prodanović, M.; Landry, C.J. Study of subcritical and supercritical gas adsorption behavior in different nanopore systems in shale using lattice Boltzmann method. Int. J. Coal Geol. 2019, 212, 103263. [Google Scholar] [CrossRef]
- Delavar, M.A.; Wang, J. Lattice Boltzmann Method in Modeling Biofilm Formation, Growth and Detachment. Sustainability 2021, 13, 7968. [Google Scholar] [CrossRef]
- Qian, Y.; Humieres, D.; Lallemand, P. Lattice BGK models for Navier-Stokes equation. Europhys. Lett. 1992, 17, 479–484. [Google Scholar] [CrossRef]
- Zhang, R.; Chen, H. Lattice Boltzmann method for simulations of liquid-vapor thermal flows. Phys. Rev. E 2003, 67, 066711. [Google Scholar] [CrossRef] [Green Version]
- Yuan, P.; Schaefer, L. Equations of state in a lattice Boltzmann model. Phys. Fluids 2006, 18, 042101. [Google Scholar] [CrossRef]
- Chen, G.; Yang, Y. Study on Equition of State for Cryogenic Fluids. Chin. J. Low Temp. Phys. 1996, 18, 70–75. [Google Scholar]


| Crogenic Fluid Working Mass | Critical Parameters | Lattice Units | Real Units |
|---|---|---|---|
| H2 | 0.086 | ||
| 0.079 | |||
| 2.728 | |||
| O2 | 0.084 | ||
| 0.075 | |||
| 2.718 | |||
| N2 | 0.081 | ||
| 0.070 | |||
| 2.699 | |||
| He | 0.086 | ||
| 2.726 | |||
| 0.078 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, W.; Shu, Z.; Lu, F.; Wang, Y.; Zheng, X.; Qian, H. Study on Interparticle Interaction Force Model to Correct Saturation Density of Real Cryogenic Fluid for LBM Simulation. Sustainability 2022, 14, 7414. https://doi.org/10.3390/su14127414
Liang W, Shu Z, Lu F, Wang Y, Zheng X, Qian H. Study on Interparticle Interaction Force Model to Correct Saturation Density of Real Cryogenic Fluid for LBM Simulation. Sustainability. 2022; 14(12):7414. https://doi.org/10.3390/su14127414
Chicago/Turabian StyleLiang, Wenqing, Zhiyong Shu, Fuming Lu, Yong Wang, Xiaohong Zheng, and Hua Qian. 2022. "Study on Interparticle Interaction Force Model to Correct Saturation Density of Real Cryogenic Fluid for LBM Simulation" Sustainability 14, no. 12: 7414. https://doi.org/10.3390/su14127414
APA StyleLiang, W., Shu, Z., Lu, F., Wang, Y., Zheng, X., & Qian, H. (2022). Study on Interparticle Interaction Force Model to Correct Saturation Density of Real Cryogenic Fluid for LBM Simulation. Sustainability, 14(12), 7414. https://doi.org/10.3390/su14127414







